Saccadic Suppression of Displacement Does Not Reflect a Saccade-Specific Bias to Assume Stability
Abstract
1. Introduction
1.1. Saccadic Suppression of Displacement and Visual Stability across Eye Movements
1.2. The Blanking Effect
1.3. Saccadic Suppression of Displacement as the Result of a Bias for Stability
1.4. Is the Stability Bias Saccade-Specific?
1.5. Saccadic Suppression of Displacement as the Result of Saccadic Suppression of Vision
1.6. Do Saccades and Masks Affect Displacement Perception in Similar Ways?
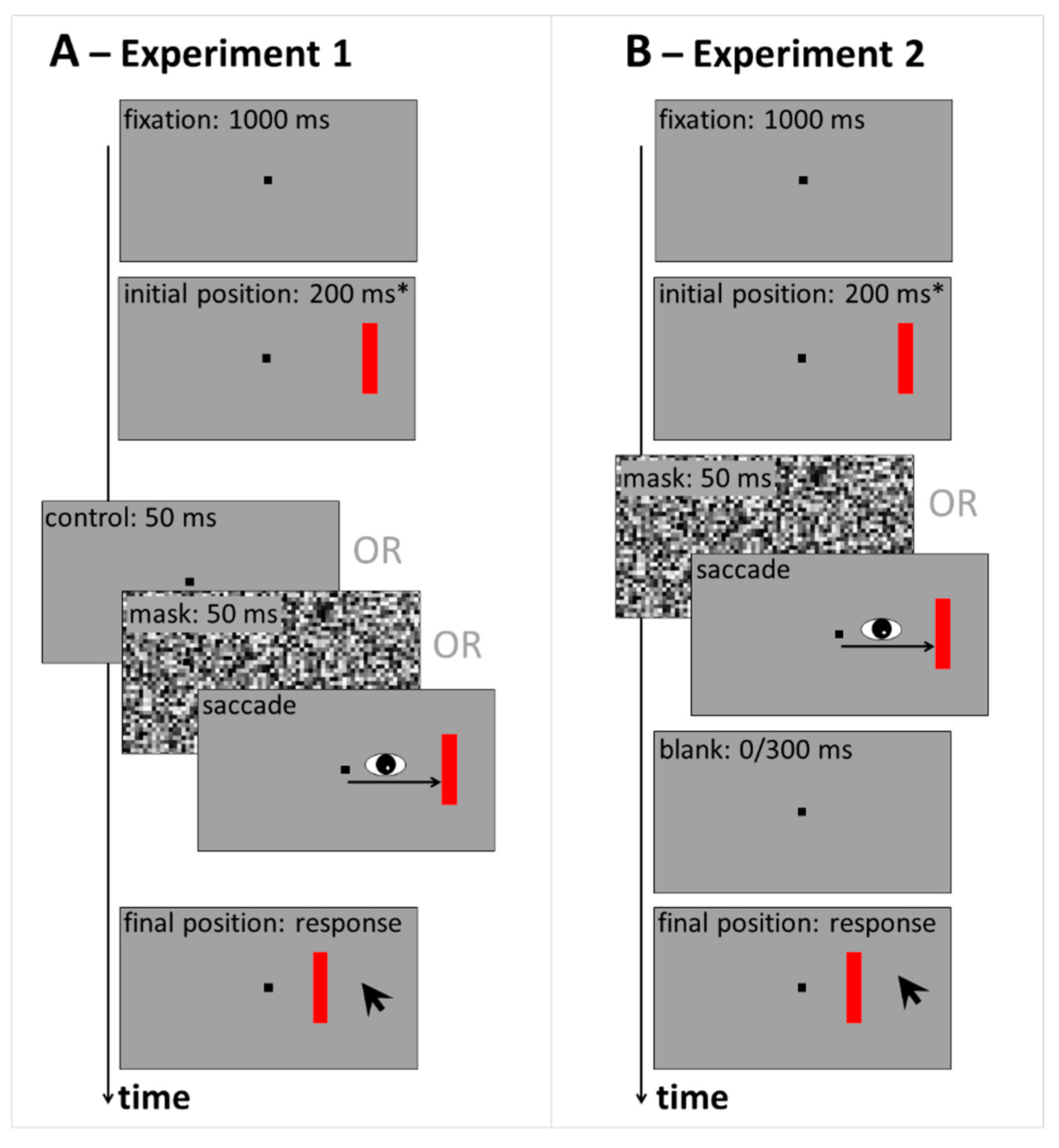
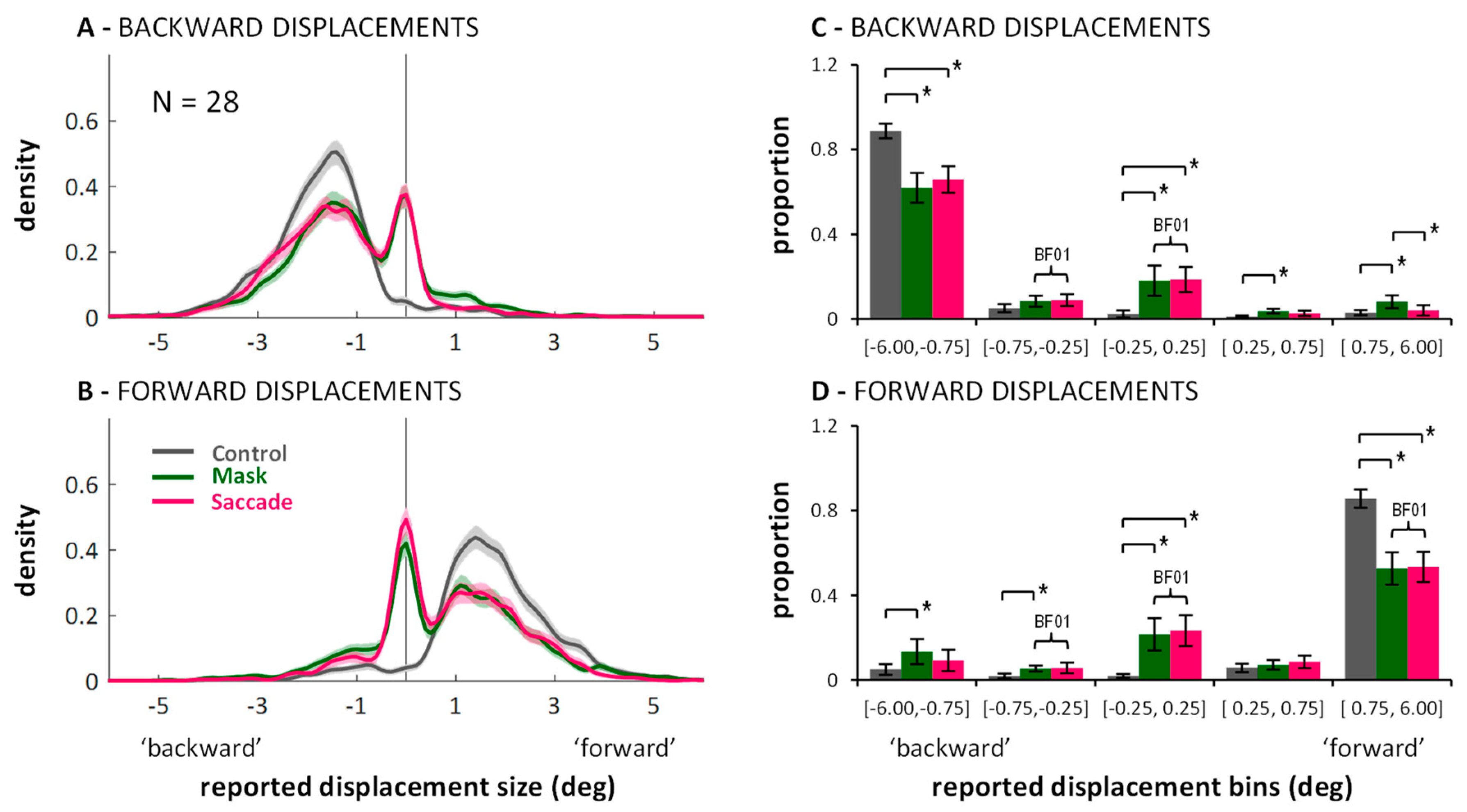
2. Experiment 1: Displacement Estimates in Control, Saccade and Masking Conditions
2.1. Direction Discrimination: Suppression of Displacement (Recoded Data)
2.2. Distributions of Displacement Estimates
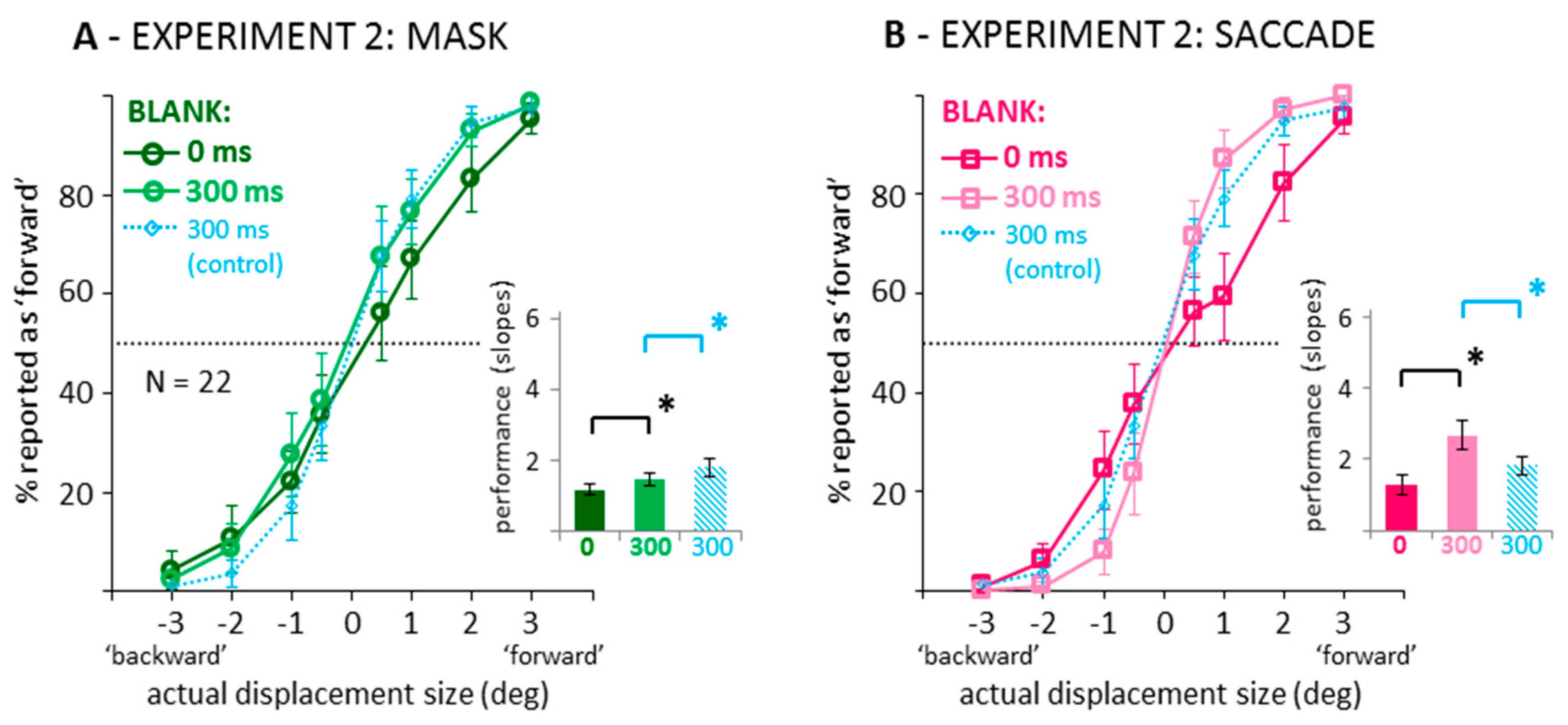
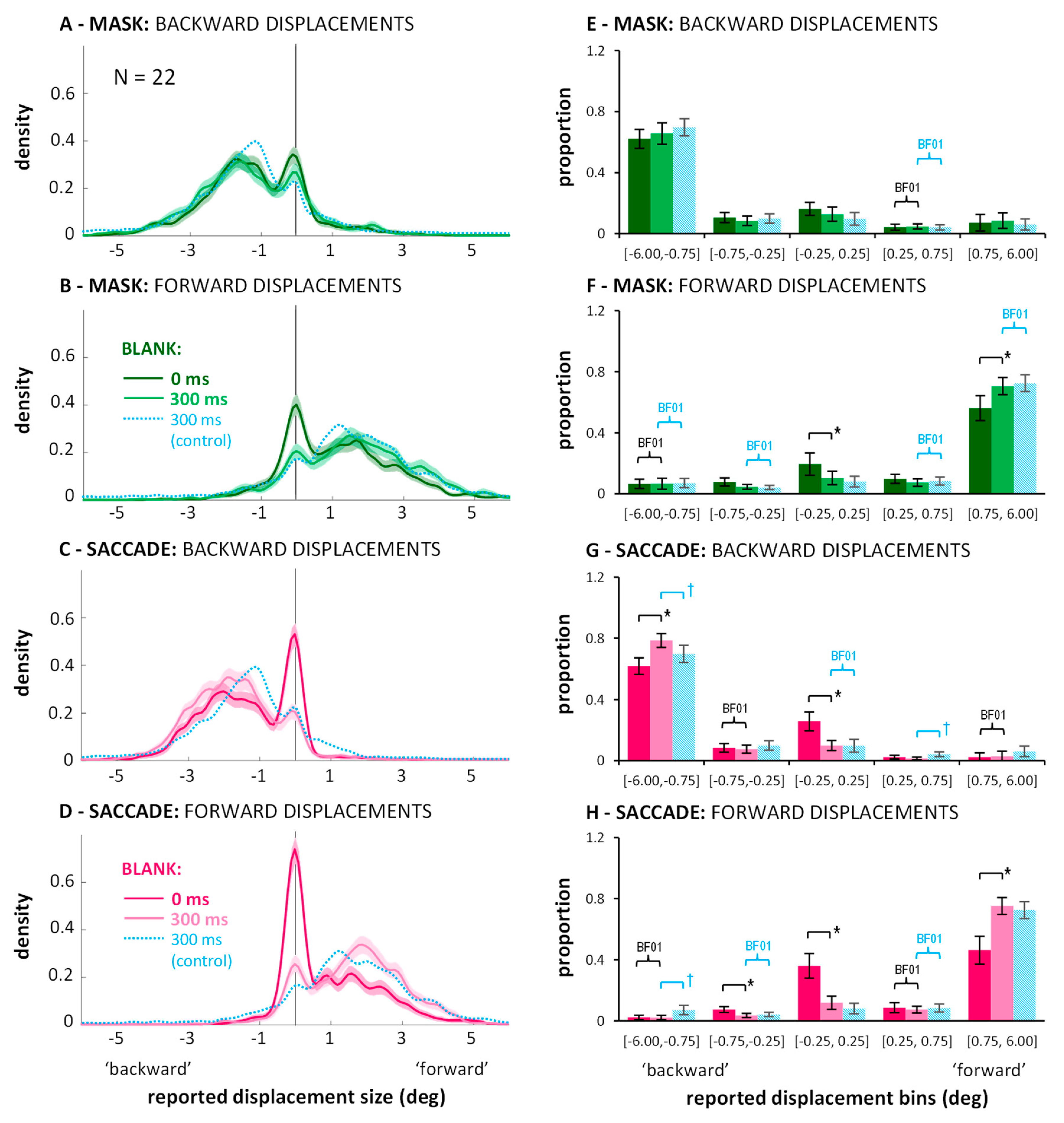
3. Experiment 2: Estimates of Displacement Size in Blanking Conditions with Saccades or Mask
3.1. Direction Discrimination: Suppression of Displacement (Recoded Data)
3.2. Distributions of Displacement Estimates
4. General Discussion
4.1. A General Bias Revealed under Conditions of Impoverished Vision
4.2. Improvement with Blanking: Relaxed Bias or Better Evidence?
4.3. Saccade-Specific Effects?
5. Materials and Methods
5.1. Experiment 1
5.1.1. Participants
5.1.2. Apparatus
5.1.3. Stimuli, Design and Procedure
5.2. Experiment 2
5.3. Saccade Detection and Trial Exclusion Criteria for Both Experiments
Supplementary Materials
Funding
Acknowledgments
Conflicts of Interest
Appendix A. Distributions across Different Jump Sizes

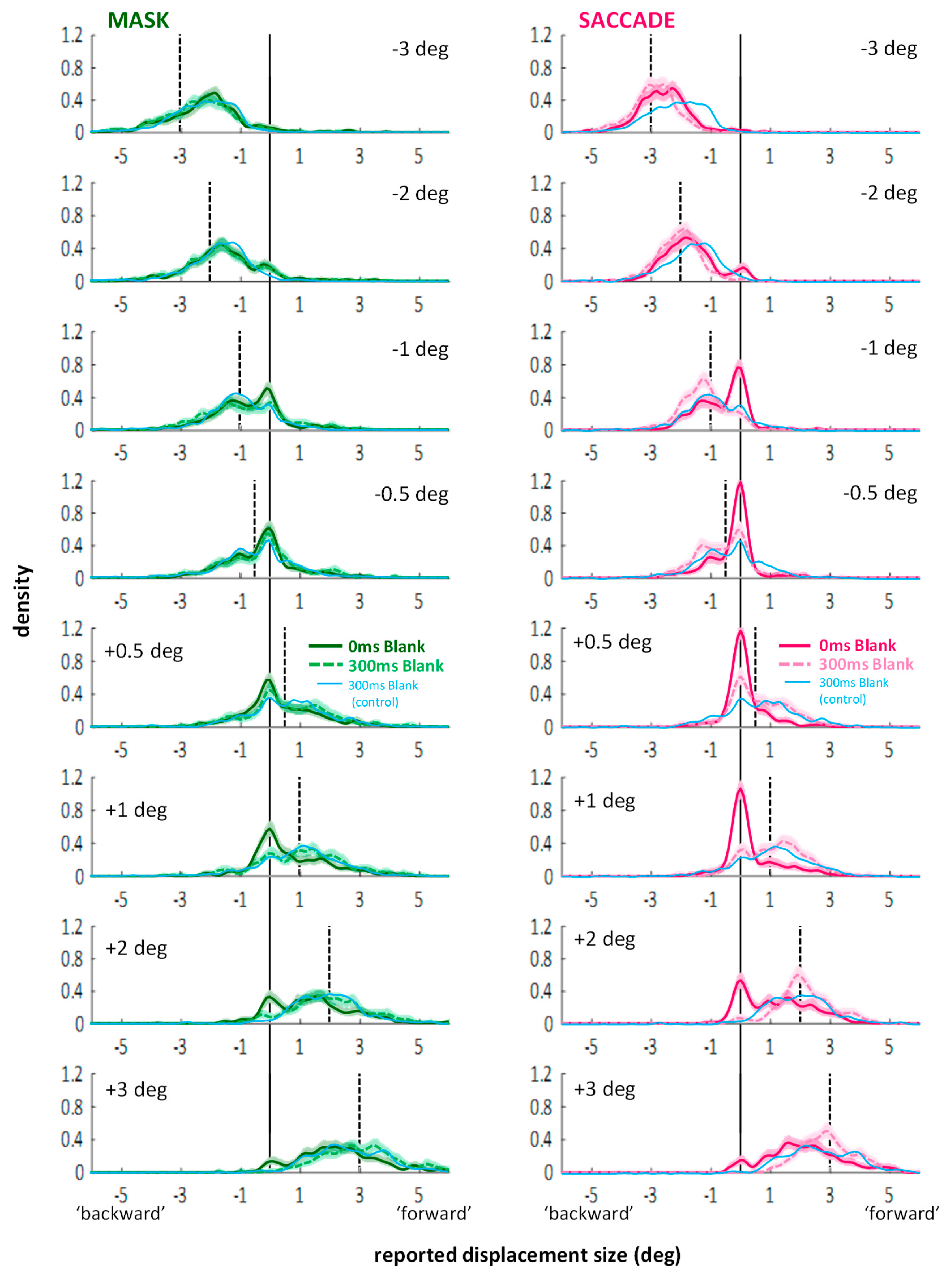
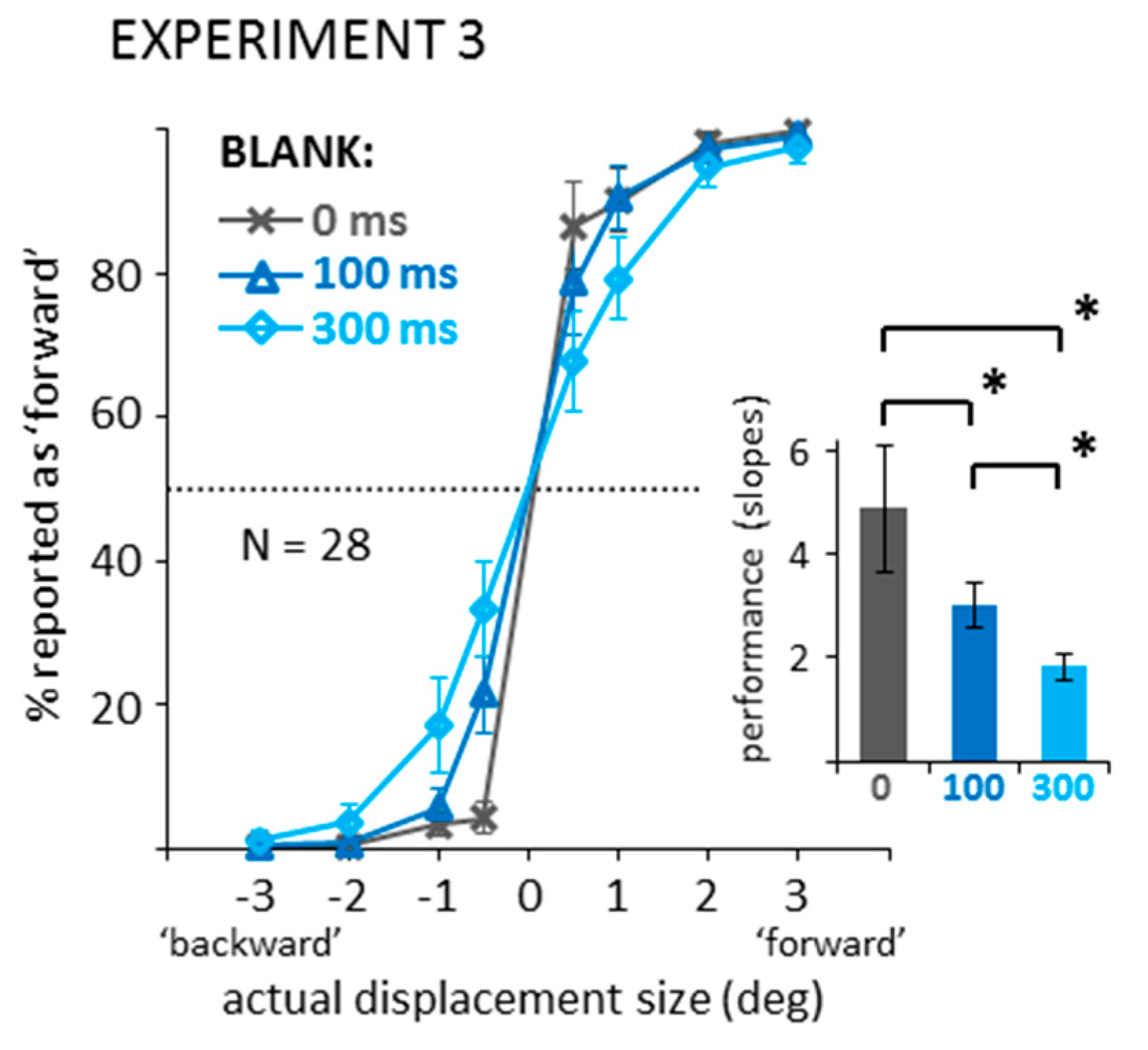
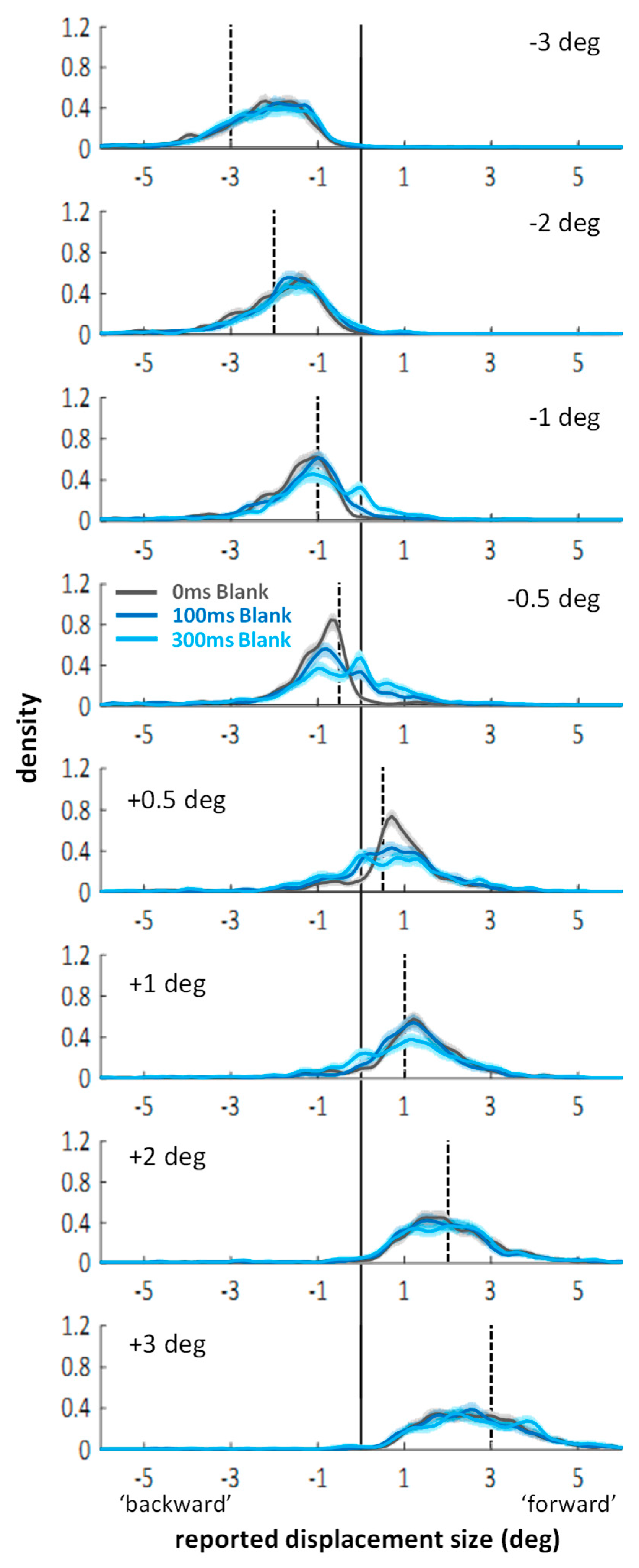
Appendix B. Experiment 3—Displacement Estimates in Blanking Conditions without Saccades or Mask (Control for Experiment 2)
Appendix B.1. Methods
Appendix B.2. Results and Discussion
Appendix B.2.1. Direction Discrimination: Suppression of Displacement (Recoded Data)
Appendix B.2.2. Distributions of Displacement Estimates
References
- Simons, D.J.; Rensink, R.A. Change blindness: Past, present, and future. Trends Cogn. Sci. 2005, 9, 16–20. [Google Scholar] [CrossRef]
- Wallach, H.; Lewis, C. The effect of abnormal displacement of the retinal image during eye movements. Percept. Psychophys. 1966, 1, 25–29. [Google Scholar] [CrossRef]
- Mack, A. An investigation of the relationship between eye and retinal image movement in the perception of movement. Percept. Psychophys. 1970, 8, 291–298. [Google Scholar] [CrossRef]
- Bridgeman, B.; Hendry, D.; Stark, L. Failure to detect displacement of the visual world during saccadic eye movements. Vis. Res. 1975, 15, 719–722. [Google Scholar] [CrossRef]
- Li, W.X.; Matin, L. The influence of saccade length on the saccadic suppression of displacement detection. Percept. Psychophys. 1990, 48, 453–458. [Google Scholar] [CrossRef] [PubMed]
- Deubel, H.; Schneider, W.X.; Bridgeman, B. Postsaccadic target blanking prevents saccadic suppression of image displacement. Vis. Res. 1996, 36, 985–996. [Google Scholar] [CrossRef]
- McConkie, G.W.; Currie, C.B. Visual stability across saccades while viewing complex pictures. J. Exp. Psychol. Hum. Percept. Perform. 1996, 22, 563–581. [Google Scholar] [CrossRef] [PubMed]
- Bridgeman, B.; Van der Heijden, A.H.C.; Velichkovsky, B.M. A theory of visual stability across saccadic eye movements. Behav. Brain Sci. 1994, 17, 247–292. [Google Scholar] [CrossRef]
- Grüsser, O.J. Early concepts on efference copy and reafference. Behav. Brain Sci. 1994, 17, 262–265. [Google Scholar] [CrossRef]
- Li, W.X.; Matin, L. Saccadic suppression of displacement: Influence of postsaccadic exposure duration and of saccadic stimulus elimination. Vis. Res. 1990, 30, 945–955. [Google Scholar] [CrossRef]
- Tas, A.C.; Moore, C.M.; Hollingworth, A. An object-mediated updating account of insensitivity to transsaccadic change. J. Vis. 2012, 12, 18. [Google Scholar] [CrossRef] [PubMed]
- Wexler, M.; Collins, T. Orthogonal steps relieve saccadic suppression. J. Vis. 2014, 14, 13. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Demeyer, M.; De Graef, P.; Wagemans, J.; Verfaillie, K. Object form discontinuity facilitates displacement discrimination across saccades. J. Vis. 2010, 10, 17. [Google Scholar] [CrossRef] [PubMed]
- Poth, C.H.; Herwig, A.; Schneider, W.X. Breaking object correspondence across saccadic eye movements deteriorates object recognition. Front. Syst. Neurosci. 2015, 9, 176. [Google Scholar] [CrossRef] [PubMed]
- Irwin, D.E.; Robinson, M.M. How post-saccadic target blanking affects the detection of stimulus displacements across saccades. Vis. Res. 2018, 142, 11–19. [Google Scholar] [CrossRef] [PubMed]
- Niemeier, M.; Crawford, J.D.; Tweed, D.B. Optimal transsaccadic integration explains distorted spatial perception. Nature 2003, 422, 76–80. [Google Scholar] [CrossRef]
- Collins, T.; Rolfs, M.; Deubel, H.; Cavanagh, P. Post-saccadic location judgments reveal remapping of saccade targets to non-foveal locations. J. Vis. 2009, 9, 29. [Google Scholar] [CrossRef] [PubMed]
- Ostendorf, F.; Liebermann, D.; Ploner, C.J. Human thalamus contributes to perceptual stability across eye movements. Proc. Natl. Acad. Sci. USA 2010, 107, 1229–1234. [Google Scholar] [CrossRef]
- Currie, C.B.; McConkie, G.W.; Carlson-Radvansky, L.A.; Irwin, D.E. The role of the saccade target object in the perception of a visually stable world. Percept. Psychophys. 2000, 62, 673–683. [Google Scholar] [CrossRef]
- Niemeier, M.; Crawford, J.D.; Tweed, D.B. Optimal inference explains dimension-specific contractions of spatial perception. Exp. Brain Res. 2007, 179, 313–323. [Google Scholar] [CrossRef]
- MacKay, D.M. Visual stability and voluntary eye movements. In Central Processing of Visual Information A: Integrative Functions and Comparative Data; Jung, R., Ed.; Springer: Berlin/Heidelberg, Germany, 1973; pp. 307–331. [Google Scholar] [CrossRef]
- Gysen, V.; De Graef, P.; Verfaillie, K. Detection of intrasaccadic displacements and depth rotations of moving objects. Vis. Res. 2002, 42, 379–391. [Google Scholar] [CrossRef]
- Gysen, V.; Verfaillie, K.; De Graef, P. The effect of stimulus blanking on the detection of intrasaccadic displacements of translating objects. Vis. Res. 2002, 42, 2021–2030. [Google Scholar] [CrossRef]
- Irwin, D.E.; Robinson, M.M. Detection of stimulus displacements across saccades is capacity-limited and biased in favor of the saccade target. Front. Syst. Neurosci. 2015, 9, 161. [Google Scholar] [CrossRef] [PubMed]
- Matsumiya, K.; Sato, M.; Shioiri, S. Contrast dependence of saccadic blanking and landmark effects. Vis. Res. 2016, 129, 1–12. [Google Scholar] [CrossRef] [PubMed]
- Atsma, J.; Maij, F.; Koppen, M.; Irwin, D.E.; Medendorp, W.P. Causal inference for spatial constancy across saccades. PLoS Comput. Biol. 2016, 12, e1004766. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Breitmeyer, B.G.; Ganz, L. Implications of sustained and transient channels for theories of visual pattern masking, saccadic suppression, and information processing. Psychol. Rev. 1976, 83, 1–36. [Google Scholar] [CrossRef]
- Burr, D.C.; Morrone, M.C.; Ross, J. Selective suppression of the magnocellular visual pathway during saccadic eye movements. Nature 1994, 371, 511–513. [Google Scholar] [CrossRef]
- Matin, E. Saccadic suppression: A review and an analysis. Psychol. Bull. 1974, 81, 899–917. [Google Scholar] [CrossRef]
- Zimmermann, E.; Born, S.; Fink, G.R.; Cavanagh, P. Masking produces compression of space and time in the absence of eye movements. J. Neurophysiol. 2014, 112, 3066–3076. [Google Scholar] [CrossRef]
- Zimmermann, E.; Morrone, M.C.; Burr, D.C. Spatial position information accumulates steadily over time. J. Neurosci. 2013, 33, 18396–18401. [Google Scholar] [CrossRef]
- Breitmeyer, B.G.; Öğmen, H. Visual Masking: Time Slices through Conscious and Unconscious Vision, 2nd ed.; Oxford University Press: Oxford, UK, 2006; ISBN 9780198530671. [Google Scholar]
- Shioiri, S.; Cavanagh, P. Saccadic suppression of low-level motion. Vis. Res. 1989, 29, 915–928. [Google Scholar] [CrossRef]
- Bays, P.M.; Catalao, R.F.; Husain, M. The precision of visual working memory is set by allocation of a shared resource. J. Vis. 2009, 9, 7–11. [Google Scholar] [CrossRef] [PubMed]
- Zhang, W.; Luck, S.J. Discrete fixed-resolution representations in visual working memory. Nature 2008, 453, 233–235. [Google Scholar] [CrossRef] [PubMed]
- Rouder, J.N.; Speckman, P.L.; Sun, D.; Morey, R.D.; Iverson, G. Bayesian t tests for accepting and rejecting the null hypothesis. Psychon. Bull. Rev. 2009, 16, 225–237. [Google Scholar] [CrossRef] [PubMed]
- JASP (Version 0.8.2). Computer Software. Available online: https://jasp-stats.org/ (accessed on 11 September 2019).
- Weiss, K.; Schneider, W.X.; Herwig, A. A “blanking effect” for surface features: Transsaccadic spatial-frequency discrimination is improved by postsaccadic blanking. Atten. Percept. Psychophys. 2015, 77, 1500–1506. [Google Scholar] [CrossRef] [PubMed]
- Whitney, D. The influence of visual motion on perceived position. Trends Cogn. Sci. 2002, 6, 211–216. [Google Scholar] [CrossRef]
- Li, H.H.; Shim, W.M.; Cavanagh, P. Backward position shift in apparent motion. J. Vis. 2014, 14, 16. [Google Scholar] [CrossRef] [PubMed]
- Kerzel, D.; Gegenfurtner, K.R. Spatial distortions and processing latencies in the onset repulsion and frohlich effects. Vis. Res. 2004, 44, 577–590. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Bergelt, J.; Hamker, F.H. Suppression of displacement detection in the presence and absence of eye movements: A neuro-computational perspective. Biol. Cybern. 2016, 110, 81–89. [Google Scholar] [CrossRef]
- Cicchini, G.M.; Binda, P.; Burr, D.C.; Morrone, M.C. Transient spatiotopic integration across saccadic eye movements mediates visual stability. J. Neurophysiol. 2013, 109, 1117–1125. [Google Scholar] [CrossRef]
- Ziesche, A.; Bergelt, J.; Deubel, H.; Hamker, F.H. Pre- and post-saccadic stimulus timing in saccadic suppression of displacement—A computational model. Vis. Res. 2017, 138, 1–11. [Google Scholar] [CrossRef] [PubMed]
- Szinte, M.; Cavanagh, P. Spatiotopic apparent motion reveals local variations in space constancy. J. Vis. 2011, 11, 4. [Google Scholar] [CrossRef] [PubMed]
- Fracasso, A.; Caramazza, A.; Melcher, D. Continuous perception of motion and shape across saccadic eye movements. J. Vis. 2010, 10, 14. [Google Scholar] [CrossRef] [PubMed]
- Rock, I.; Ebenholtz, S. Stroboscopic movement based on change of phenomenal rather than retinal location. Am. J. Psychol. 1962, 75, 193–207. [Google Scholar] [CrossRef] [PubMed]
- Anstis, S. The perception of apparent movement. Philos. Trans. R. Soc. Lond. B Biol. Sci. 1980, 290, 153–168. [Google Scholar] [CrossRef] [PubMed]
- Enns, J.T.; Lleras, A.; Moore, C.M. Object updating: A force for perceptual continuity and scene stability in human vision. In Space and Time in Perception and Action; Nijhawan, R., Khurana, B., Eds.; Cambridge University Press: Cambridge, UK, 2010; pp. 503–520. [Google Scholar] [CrossRef]
- Klingenhoefer, S.; Bremmer, F. Saccadic suppression of displacement in face of saccade adaptation. Vis. Res. 2011, 51, 881–889. [Google Scholar] [CrossRef]
- Ostendorf, F.; Kilias, J.; Ploner, C.J. Theta-burst stimulation over human frontal cortex distorts perceptual stability across eye movements. Cereb. Cortex 2012, 22, 800–810. [Google Scholar] [CrossRef][Green Version]
- Cavanaugh, J.; Berman, R.A.; Joiner, W.M.; Wurtz, R.H. Saccadic corollary discharge underlies stable visual perception. J. Neurosci. 2016, 36, 31–42. [Google Scholar] [CrossRef]
- Kleiner, M.; Brainard, D.; Pelli, D. What’s new in psychtoolbox-3? Perception 2007, 36, 14. [Google Scholar]
- Cornelissen, F.W.; Peters, E.M.; Palmer, J. The eyelink toolbox: Eye tracking with matlab and the psychophysics toolbox. Behav. Res. Methods Instrum. Comput. 2002, 34, 613–617. [Google Scholar] [CrossRef]

© 2019 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Born, S. Saccadic Suppression of Displacement Does Not Reflect a Saccade-Specific Bias to Assume Stability. Vision 2019, 3, 49. https://doi.org/10.3390/vision3040049
Born S. Saccadic Suppression of Displacement Does Not Reflect a Saccade-Specific Bias to Assume Stability. Vision. 2019; 3(4):49. https://doi.org/10.3390/vision3040049
Chicago/Turabian StyleBorn, Sabine. 2019. "Saccadic Suppression of Displacement Does Not Reflect a Saccade-Specific Bias to Assume Stability" Vision 3, no. 4: 49. https://doi.org/10.3390/vision3040049
APA StyleBorn, S. (2019). Saccadic Suppression of Displacement Does Not Reflect a Saccade-Specific Bias to Assume Stability. Vision, 3(4), 49. https://doi.org/10.3390/vision3040049




