The Effect of Training with Weightlifting Catching or Pulling Derivatives on Squat Jump and Countermovement Jump Force–Time Adaptations
Abstract
1. Introduction
2. Materials and Methods
2.1. Participants
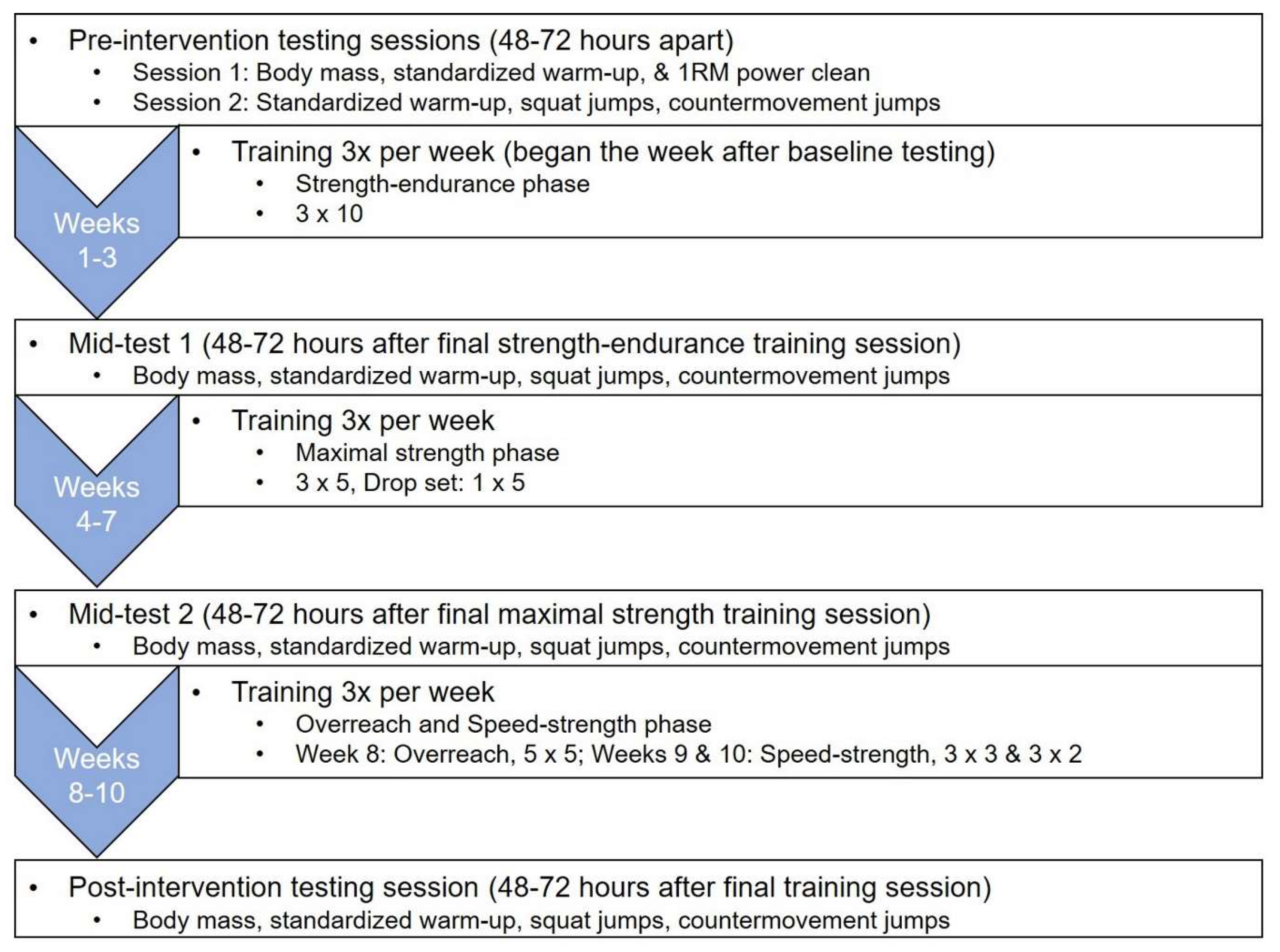
2.2. Procedures and Design
2.3. 1RM Power Clean Assessment
2.4. Vertical Jump Assessment
2.5. Training Intervention
2.6. Data Analysis
2.7. Statistical Analyses
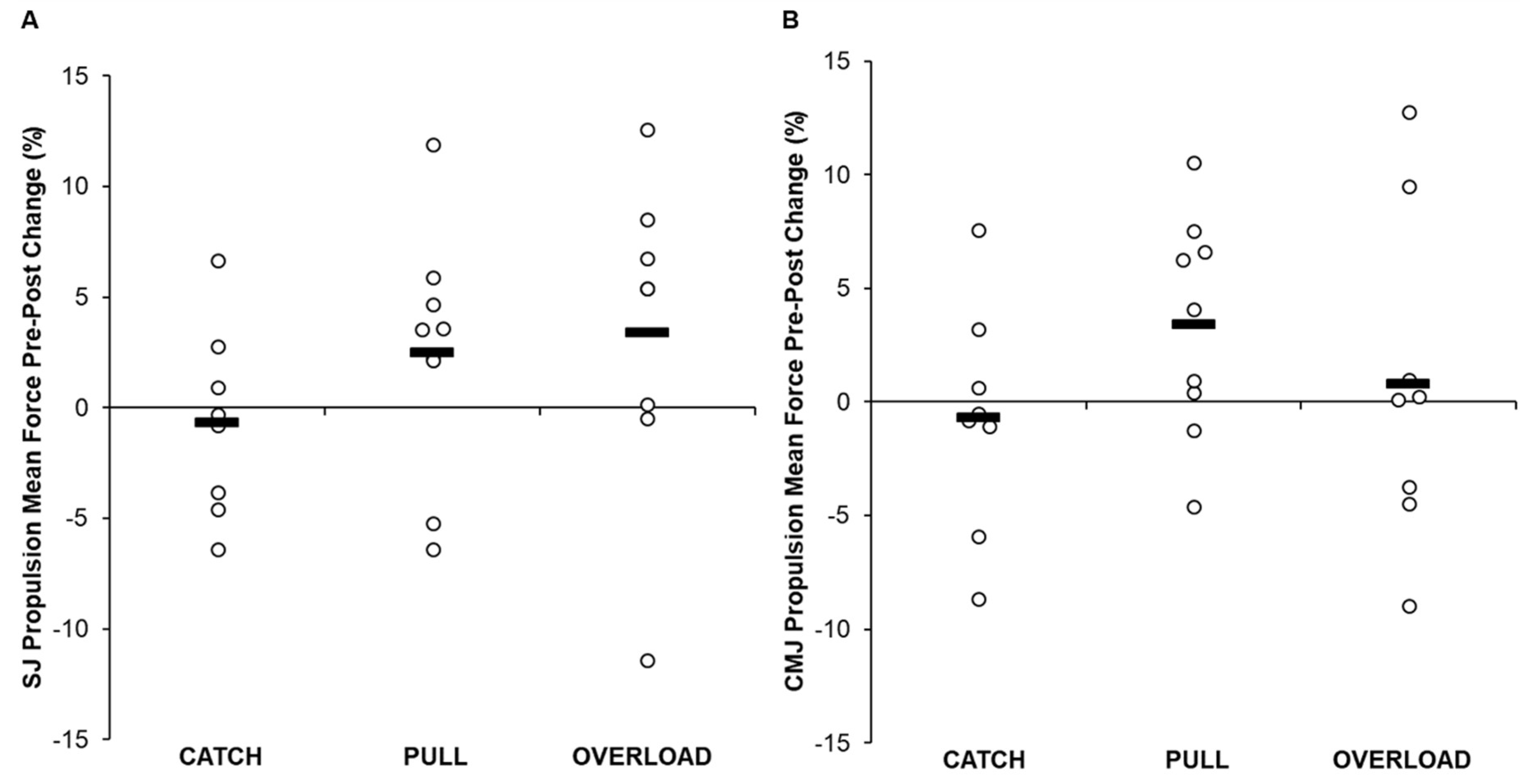
3. Results
3.1. Between- and Within-Group Comparisons
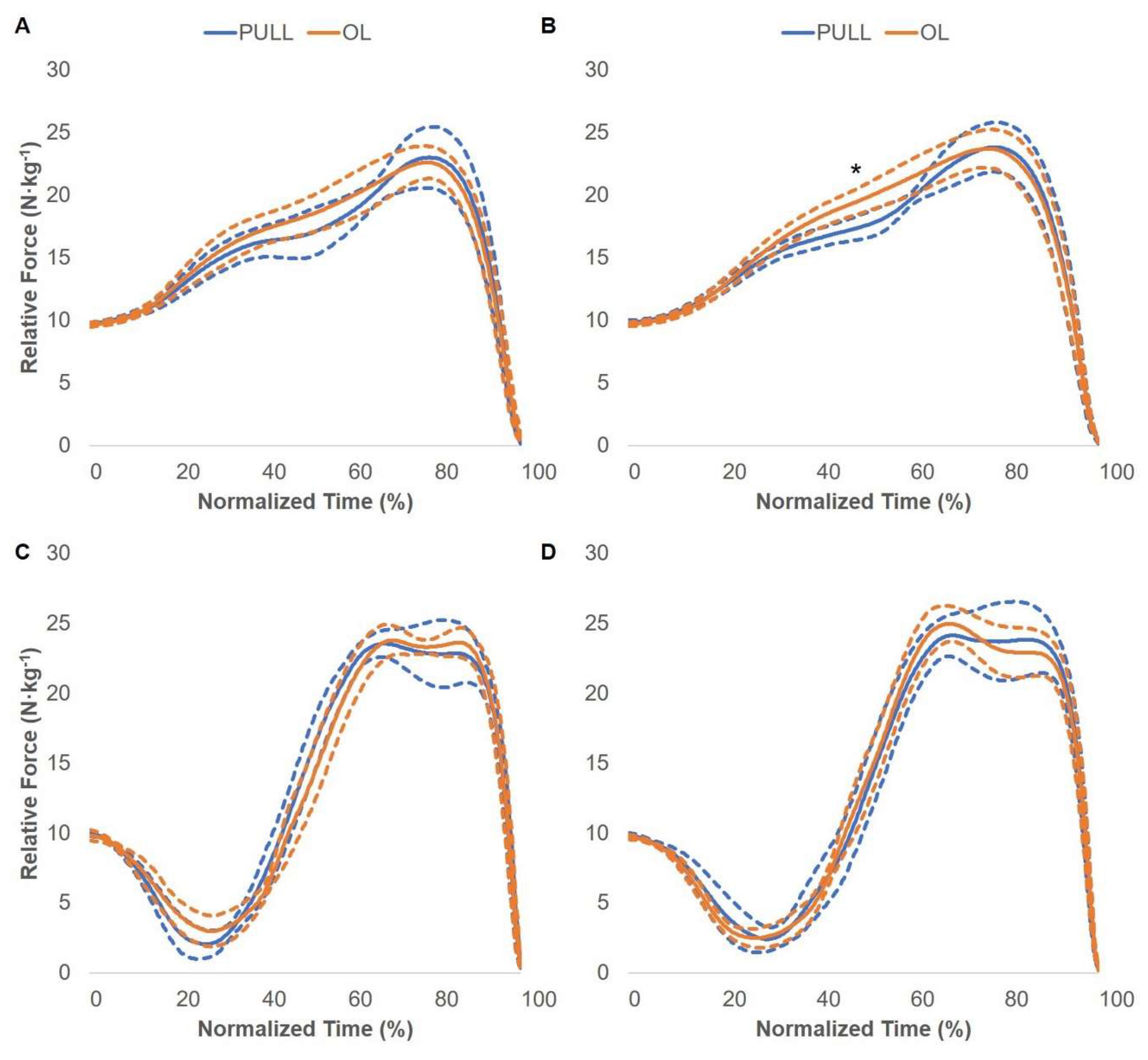
3.2. Force–Time Curve Comparisons
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Sole, C.J.; Mizuguchi, S.; Sato, K.; Moir, G.L.; Stone, M.H. Phase characteristics of the countermovement jump force-time curve: A comparison of athletes by jumping ability. J. Strength Cond. Res. 2018, 32, 1155–1165. [Google Scholar] [CrossRef] [PubMed]
- Gathercole, R.; Sporer, B.; Stellingwerff, T.; Sleivert, G. Alternative countermovement-jump analysis to quantify acute neuromuscular fatigue. Int. J. Sports Physiol. Perform. 2015, 10, 84–92. [Google Scholar] [CrossRef] [PubMed]
- McMahon, J.J.; Lake, J.P.; Suchomel, T.J. Vertical jump testing. In Performance Assessment in Strength and Conditioning; Comfort, P., Jones, P.A., McMahon, J.J., Eds.; Routledge: New York, NY, USA, 2019; pp. 96–116. [Google Scholar]
- Kirby, T.J.; McBride, J.M.; Haines, T.L.; Dayne, A.M. Relative net vertical impulse determines jumping performance. J. Appl. Biomech. 2011, 27, 207–214. [Google Scholar] [CrossRef] [PubMed]
- Garhammer, J.; Gregor, R. Propulsion forces as a function of intensity for weightlifting and vertical jumping. J. Strength Cond. Res. 1992, 6, 129–134. [Google Scholar]
- Burkhardt, E.; Barton, B.; Garhammer, J. Maximal impact and propulsive forces during jumping and explosive lifting exercises. J. Appl. Sports Sci. Res. 1990, 4, 107. [Google Scholar]
- Hackett, D.; Davies, T.; Soomro, N.; Halaki, M. Olympic weightlifting training improves vertical jump height in sportspeople: A systematic review with meta-analysis. Br. J. Sports Med. 2016, 50, 865–872. [Google Scholar] [CrossRef]
- Teo, S.Y.; Newton, M.J.; Newton, R.U.; Dempsey, A.R.; Fairchild, T.J. Comparing the effectiveness of a short-term vertical jump versus weightlifting program on athletic power development. J. Strength Cond. Res. 2016, 30, 2741–2748. [Google Scholar] [CrossRef]
- Hoffman, J.R.; Cooper, J.; Wendell, M.; Kang, J. Comparison of olympic vs. Traditional power lifting training programs in football players. J. Strength Cond. Res. 2004, 18, 129–135. [Google Scholar]
- Tricoli, V.; Lamas, L.; Carnevale, R.; Ugrinowitsch, C. Short-term effects on lower-body functional power development: Weightlifting vs. Vertical jump training programs. J. Strength Cond. Res. 2005, 19, 433–437. [Google Scholar] [CrossRef]
- Hori, N.; Newton, R.U.; Andrews, W.A.; Kawamori, N.; McGuigan, M.R.; Nosaka, K. Does performance of hang power clean differentiate performance of jumping, sprinting, and changing of direction? J. Strength Cond. Res. 2008, 22, 412–418. [Google Scholar] [CrossRef]
- Kipp, K.; Suchomel, T.J.; Comfort, P. Correlational analysis between joint-level kinetics of countermovement jumps and weightlifting derivatives. J. Sports Sci. Med. 2019, 18, 663–668. [Google Scholar] [PubMed]
- Otto III, W.H.; Coburn, J.W.; Brown, L.E.; Spiering, B.A. Effects of weightlifting vs. Kettlebell training on vertical jump, strength, and body composition. J. Strength Cond. Res. 2012, 26, 1199–1202. [Google Scholar] [CrossRef] [PubMed]
- Suchomel, T.J.; Comfort, P.; Stone, M.H. Weightlifting pulling derivatives: Rationale for implementation and application. Sports Med. 2015, 45, 823–839. [Google Scholar] [CrossRef] [PubMed]
- Simenz, C.J.; Dugan, C.A.; Ebben, W.P. Strength and conditioning practices of national basketball association strength and conditioning coaches. J. Strength Cond. Res. 2005, 19, 495–504. [Google Scholar]
- Ebben, W.P.; Carroll, R.M.; Simenz, C.J. Strength and conditioning practices of national hockey league strength and conditioning coaches. J. Strength Cond. Res. 2004, 18, 889–897. [Google Scholar]
- Comfort, P.; Allen, M.; Graham-Smith, P. Kinetic comparisons during variations of the power clean. J. Strength Cond. Res. 2011, 25, 3269–3273. [Google Scholar] [CrossRef]
- Comfort, P.; Allen, M.; Graham-Smith, P. Comparisons of peak ground reaction force and rate of force development during variations of the power clean. J. Strength Cond. Res. 2011, 25, 1235–1239. [Google Scholar] [CrossRef]
- Takei, S.; Hirayama, K.; Okada, J. Comparison of the power output between the hang power clean and hang high pull across a wide range of loads in weightlifters. J. Strength Cond. Res. 2020. [Google Scholar] [CrossRef]
- Suchomel, T.J.; Sole, C.J. Force-time curve comparison between weightlifting derivatives. Int. J. Sports Physiol. Perform. 2017, 12, 431–439. [Google Scholar] [CrossRef]
- Suchomel, T.J.; Wright, G.A.; Kernozek, T.W.; Kline, D.E. Kinetic comparison of the power development between power clean variations. J. Strength Cond. Res. 2014, 28, 350–360. [Google Scholar] [CrossRef]
- Kipp, K.; Malloy, P.J.; Smith, J.; Giordanelli, M.D.; Kiely, M.T.; Geiser, C.F.; Suchomel, T.J. Mechanical demands of the hang power clean and jump shrug: A joint-level perspective. J. Strength Cond. Res. 2018, 32, 466–474. [Google Scholar] [CrossRef] [PubMed]
- Suchomel, T.J.; Sole, C.J. Power-time curve comparison between weightlifting derivatives. J. Sports Sci. Med. 2017, 16, 407–413. [Google Scholar] [PubMed]
- Kipp, K.; Comfort, P.; Suchomel, T.J. Comparing biomechanical time series data during the hang-power clean and jump shrug. J. Strength Cond. Res. 2019. [Google Scholar] [CrossRef] [PubMed]
- Suchomel, T.J.; Wright, G.A.; Lottig, J. Lower Extremity Joint Velocity Comparisons during the Hang Power Clean and Jump Shrug at Various Loads, Proceedings of the XXXIInd International Conference of Biomechanics in Sports, Johnson City, TN, USA, 12–16 July 2014; Sato, K., Sands, W.A., Mizuguchi, S., Eds.; International Society of Biomechanics in Sports: Johnson City, TN, USA, 2014; pp. 749–752. [Google Scholar]
- Comfort, P.; Dos‘Santos, T.; Thomas, C.; McMahon, J.J.; Suchomel, T.J. An investigation into the effects of excluding the catch phase of the power clean on force-time characteristics during isometric and dynamic tasks: An intervention study. J. Strength Cond. Res. 2018, 32, 2116–2129. [Google Scholar] [CrossRef]
- Suchomel, T.J.; Comfort, P.; Lake, J.P. Enhancing the force-velocity profile of athletes using weightlifting derivatives. Strength Cond. J. 2017, 39, 10–20. [Google Scholar] [CrossRef]
- Comfort, P.; Jones, P.A.; Udall, R. The effect of load and sex on kinematic and kinetic variables during the mid-thigh clean pull. Sports Biomech. 2015, 14, 139–156. [Google Scholar] [CrossRef]
- Comfort, P.; Udall, R.; Jones, P.A. The effect of loading on kinematic and kinetic variables during the midthigh clean pull. J. Strength Cond. Res. 2012, 26, 1208–1214. [Google Scholar] [CrossRef]
- Meechan, D.; Suchomel, T.J.; McMahon, J.J.; Comfort, P. A comparison of kinetic and kinematic variables during the mid-thigh pull and countermovement shrug, across loads. J. Strength Cond. Res. 2019; in press. [Google Scholar]
- Meechan, D.; McMahon, J.J.; Suchomel, T.J.; Comfort, P. A comparison of kinetic and kinematic variables during the pull from the knee and hang pull, across loads. J. Strength Cond. Res. 2020. [Google Scholar] [CrossRef]
- Haff, G.G.; Whitley, A.; McCoy, L.B.; O’Bryant, H.S.; Kilgore, J.L.; Haff, E.E.; Pierce, K.; Stone, M.H. Effects of different set configurations on barbell velocity and displacement during a clean pull. J. Strength Cond. Res. 2003, 17, 95–103. [Google Scholar]
- Suchomel, T.J.; McKeever, S.M.; Comfort, P. Training with weightlifting derivatives: The effects of force and velocity overload stimuli. J. Strength Cond. Res. 2020, in press. [Google Scholar]
- Moulton, L.H. Covariate-based constrained randomization of group-randomized trials. Clin. Trials 2004, 1, 297–305. [Google Scholar] [CrossRef] [PubMed]
- Cormie, P.; McGuigan, M.R.; Newton, R.U. Adaptations in athletic performance after ballistic power versus strength training. Med. Sci. Sports Exerc. 2010, 42, 1582–1598. [Google Scholar] [CrossRef] [PubMed]
- Suchomel, T.J.; McKeever, S.M.; Sijuwade, O.; Carpenter, L.; McMahon, J.J.; Loturco, I.; Comfort, P. The effect of load placement on the power production characteristics of three lower extremity jumping exercises. J. Hum. Kinet. 2019, 68, 109–122. [Google Scholar] [CrossRef] [PubMed]
- Suchomel, T.J.; Beckham, G.K.; Wright, G.A. The impact of load on lower body performance variables during the hang power clean. Sports Biomech. 2014, 13, 87–95. [Google Scholar] [CrossRef] [PubMed]
- Suchomel, T.J.; Beckham, G.K.; Wright, G.A. Effect of various loads on the force-time characteristics of the hang high pull. J. Strength Cond. Res. 2015, 29, 1295–1301. [Google Scholar] [CrossRef] [PubMed]
- Suchomel, T.J.; Beckham, G.K.; Wright, G.A. Lower body kinetics during the jump shrug: Impact of load. J. Trainol. 2013, 2, 19–22. [Google Scholar] [CrossRef][Green Version]
- Suchomel, T.J.; DeWeese, B.H.; Beckham, G.K.; Serrano, A.J.; Sole, C.J. The jump shrug: A progressive exercise into weightlifting derivatives. Strength Cond. J. 2014, 36, 43–47. [Google Scholar] [CrossRef][Green Version]
- Suchomel, T.J.; DeWeese, B.H.; Beckham, G.K.; Serrano, A.J.; French, S.M. The hang high pull: A progressive exercise into weightlifting derivatives. Strength Cond. J. 2014, 36, 79–83. [Google Scholar] [CrossRef]
- DeWeese, B.H.; Serrano, A.J.; Scruggs, S.K.; Burton, J.D. The midthigh pull: Proper application and progressions of a weightlifting movement derivative. Strength Cond. J. 2013, 35, 54–58. [Google Scholar] [CrossRef]
- DeWeese, B.H.; Serrano, A.J.; Scruggs, S.K.; Sams, M.L. The clean pull and snatch pull: Proper technique for weightlifting movement derivatives. Strength Cond. J. 2012, 34, 82–86. [Google Scholar] [CrossRef]
- DeWeese, B.H.; Scruggs, S.K. The countermovement shrug. Strength Cond. J. 2012, 34, 20–23. [Google Scholar] [CrossRef]
- DeWeese, B.H.; Hornsby, G.; Stone, M.; Stone, M.H. The training process: Planning for strength–power training in track and field. Part 2: Practical and applied aspects. J. Sport Health Sci. 2015, 4, 318–324. [Google Scholar] [CrossRef]
- DeWeese, B.H.; Sams, M.L.; Serrano, A.J. Sliding toward sochi—Part 1: A review of programming tactics used during the 2010-2014 quadrennial. Natl. Strength Cond. Assoc. Coach 2014, 1, 30–42. [Google Scholar]
- Stone, M.H.; O’Bryant, H.S. Weight Training: A Scientific Approach; Burgess International: Minneapolis, MN, USA, 1987. [Google Scholar]
- Hardee, J.P.; Lawrence, M.M.; Zwetsloot, K.A.; Triplett, N.T.; Utter, A.C.; McBride, J.M. Effect of cluster set configurations on power clean technique. J. Sports Sci. 2013, 31, 488–496. [Google Scholar] [CrossRef]
- Owen, N.J.; Watkins, J.; Kilduff, L.P.; Bevan, H.R.; Bennett, M.A. Development of a criterion method to determine peak mechanical power output in a countermovement jump. J. Strength Cond. Res. 2014, 28, 1552–1558. [Google Scholar] [CrossRef]
- Moir, G.L. Three different methods of calculating vertical jump height from force platform data in men and women. Meas. Phys. Educ. Exerc. Sci. 2008, 12, 207–218. [Google Scholar] [CrossRef]
- Koo, T.K.; Li, M.Y. A guideline of selecting and reporting intraclass correlation coefficients for reliability research. J. Chiropr. Med. 2016, 15, 155–163. [Google Scholar] [CrossRef]
- Cormack, S.J.; Newton, R.U.; McGuigan, M.R.; Doyle, T.L.A. Reliability of measures obtained during single and repeated countermovement jumps. Int. J. Sports Physiol. Perform. 2008, 3, 131–144. [Google Scholar] [CrossRef]
- Hedges, L.V.; Olkin, I. Estimation of a single effect size: Parametric and nonparametric methods. In Statistical Methods for Meta-Analysis; Academic Press: San Diego, CA, USA, 1985; pp. 75–106. [Google Scholar]
- Rhea, M.R. Determining the magnitude of treatment effects in strength training research through the use of the effect size. J. Strength Cond. Res. 2004, 18, 918–920. [Google Scholar]
- Enoka, R.M. The pull in olympic weightlifting. Med. Sci. Sports 1979, 11, 131–137. [Google Scholar]
- McMahon, J.J.; Suchomel, T.J.; Lake, J.P.; Comfort, P. Understanding the key phases of the countermovement jump force-time curve. Strength Cond. J. 2018, 40, 96–106. [Google Scholar] [CrossRef]
- Moolyk, A.N.; Carey, J.P.; Chiu, L.Z.F. Characteristics of lower extremity work during the impact phase of jumping and weightlifting. J. Strength Cond. Res. 2013, 27, 3225–3232. [Google Scholar] [CrossRef] [PubMed]
- Comfort, P.; Williams, R.; Suchomel, T.J.; Lake, J.P. A comparison of catch phase force-time characteristics during clean derivatives from the knee. J. Strength Cond. Res. 2017, 31, 1911–1918. [Google Scholar] [CrossRef] [PubMed]
- Suchomel, T.J.; Giordanelli, M.D.; Geiser, C.F.; Kipp, K. Comparison of joint work during load absorption between weightlifting derivatives. J. Strength Cond. Res. 2018. [Google Scholar] [CrossRef]
- Suchomel, T.J.; Lake, J.P.; Comfort, P. Load absorption force-time characteristics following the second pull of weightlifting derivatives. J. Strength Cond. Res. 2017, 31, 1644–1652. [Google Scholar] [CrossRef] [PubMed]
- Suchomel, T.J.; Nimphius, S.; Stone, M.H. The importance of muscular strength in athletic performance. Sports Med. 2016, 46, 1419–1449. [Google Scholar] [CrossRef] [PubMed]
- James, L.P.; Comfort, P.; Suchomel, T.J.; Kelly, V.G.; Beckman, E.M.; Haff, G.G. The impact of power clean ability and training age on adaptations to weightlifting-style training. J. Strength Cond. Res. 2019, 33, 2936–2944. [Google Scholar] [CrossRef]




| CATCH (n = 8) | PULL (n = 9) | OL (n = 8) | |
|---|---|---|---|
| Age (years) | 22.1 ± 3.3 | 22.2 ± 2.3 | 22.5 ± 1.2 |
| Body mass (kg) | 85.7 ± 14.3 | 84.3 ± 17.3 | 84.9 ± 13.2 |
| Height (cm) | 180.7 ± 6.1 | 179.6 ± 3.7 | 173.8 ± 9.9 |
| Power clean experience (years) | 6.4 ± 3.1 | 6.4 ± 2.4 | 6.6 ± 1.8 |
| Relative 1RM power clean (kg · kg−1) | 1.16 ± 0.10 | 1.19 ± 0.18 | 1.25 ± 0.16 |
| Relative 1RM back squat (kg · kg−1) | 1.64 ± 0.22 | 1.73 ± 0.17 | 1.76 ± 0.34 |
| Training Block | Day 1 | Day 2 | Day 3 |
|---|---|---|---|
| Strength–endurance, Weeks 1–3, 3 × 10 | Back squat, Military press, Split squat, Bench press | Power clean from floor/Clean pull from floor Stiff-legged deadlift Bent-over row Pull-up | Power clean from floor/Clean pull from floor Back squat Incline bench press Bent-over row |
| Max-strength, Weeks 4–7, 3 × 5 + Overreach, Week 8, 5 × 5 | Push press, Back squat, Bench press, Lunge | Mid-thigh power clean/Mid-thigh pull Power clean from floor/Clean pull from floor Stiff-legged deadlift Pull-up | Mid-thigh power clean/Mid-thigh pull Back squat Incline bench press Dumbbell row |
| Speed–strength, Weeks 9–10, 3 × 3, 3 × 2 | Jerk ¼ squat + Squat jump, Bench press | CM power clean/CM shrug Hang power clean/Hang high pull | Jerk Hang power clean/Jump shrug |
| Wk | S × R | Day 1 | Day 2 | Day 3 | CATCH | PULL | OL |
|---|---|---|---|---|---|---|---|
| Traditional Exercises | PC from Floor | CP from Floor | CP from Floor | ||||
| 1 | 3 × 10 | 80% | 80% | 70% | 55–57.5% | 55–57.5% | 75–77.5% |
| 2 | 3 × 10 | 85% | 85% | 75% | 57.5–60% | 57.5–60% | 77.5–80% |
| 3 | 3 × 10 | 90% | 90% | 80% | 60–62.5% | 60–62.5% | 80–82.5% |
| Mid-Thigh PC, PC from Floor | Mid-Thigh Pull, CP from Floor | Mid-Thigh Pull, CP from Floor | |||||
| 4 | 3 × 5 | 85% | 85% | 70% | 55–60%, 70–75% | 55–60%, 70–75% | 110–120%, 90–95% |
| 5 | 3 × 5 | 90% | 90% | 75% | 60–65%, 75–80% | 60–65%, 75–80% | 120–127.5%, 95–100% |
| 6 | 3 × 5 | 95% | 95% | 77.5% | 65–70%, 80–82.5% | 65–70%, 80–82.5% | 127.5–135%, 100–102.5% |
| 7 | 3 × 5 | 80% | 80% | 65% | 50–55%, 65–70% | 50–55%, 65–70% | 112.5–120%, 85–87.5% |
| 8 | 5 × 5 | 85% | 85% | 75% | 53–58%, 63–68% | 53–58%, 63–68% | 107–112%, 80–85% |
| CM PC, Hang PC | CM Shrug, Hang High Pull, Jump Shrug | CM Shrug, Hang High Pull, Jump Shrug | |||||
| 9 | 3 × 3 | 90% | 90% | 77.5% | 60–65%, 70–75% (Day 2) and 55–60% (Day 3) | 60–65%, 70–75%, 55–60% | 105–110%, 40–45%, 35–40% |
| 10 | 3 × 2 | 85% | 85% | 75% | 55–60%, 65–70% (Day 2) and 50–55% (Day 3) | 55–60%, 65–70%, 50–55% | 100–105%, 35–44%, 30–35% |
| Variable | Pre | MT1 | MT2 | Post | ||||
|---|---|---|---|---|---|---|---|---|
| ICC (CI) | CV% | ICC (CI) | CV% | ICC (CI) | CV% | ICC (CI) | CV% | |
| SJ Height | 0.96 (0.91–0.98) | 5.2 | 0.94 (0.85–0.97) | 5.8 | 0.95 (0.89–0.98) | 5.2 | 0.92 (0.83–0.97) | 7.1 |
| SJ MF | 0.98 (0.96–0.99) | 3.2 | 0.98 (0.97–0.99) | 2.9 | 0.98 (0.95–0.99) | 3.3 | 0.97 (0.93–0.99) | 3.6 |
| SJ Time | 0.83 (0.62–0.93) | 5.9 | 0.89 (0.75–0.95) | 4.2 | 0.90 0.77–0.96) | 3.7 | 0.77 (0.47–0.90) | 5.8 |
| CMJ Height | 0.98 (0.95–0.99) | 4.6 | 0.96 (0.92–0.98) | 4.6 | 0.96 (0.90–0.98) | 4.3 | 0.95 (0.88–0.98) | 4.8 |
| CMJ MF | 0.99 (0.98–0.99) | 2.4 | 0.99 (0.98–0.99) | 1.8 | 0.99 (0.98–0.99) | 1.9 | 0.99 (0.98–0.99) | 2.0 |
| CMJ Time | 0.94 (0.86–0.97) | 4.3 | 0.96 (0.91–0.98) | 3.7 | 0.97 (0.92–0.98) | 3.3 | 0.98 (0.94–0.99) | 3.4 |
| Variable | CATCH | PULL | OL | ||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Pre | MT1 | MT2 | Post | Pre | MT1 | MT2 | Post | Pre | MT1 | MT2 | Post | ||||||||||
| SJ Height (cm) | M | 33.5 | 32.7 | 32.9 | 33.2 | 35.0 | 36.2 | 36.3 | 35.8 | 34.2 | 37.1 | 35.7 | 36.8 | ||||||||
| SD | 4.8 | 3.8 | 3.1 | 4.5 | 6.8 | 4.7 | 6.5 | 6.2 | 6.9 | 7.3 | 7.0 | 6.9 | |||||||||
| g | −0.18 | 0.06 | 0.08 | 0.19 | 0.03 | −0.08 | 0.40 | −0.20 | 0.15 | ||||||||||||
| −0.06 | 0.11 | 0.35 | |||||||||||||||||||
| SJ MF (N·kg−1) | M | 16.7 | 16.5 | 16.8 | 16.5 | 16.3 | 17.3 | 17.1 | 16.7 | 16.8 | 16.8 | 17.8 | 17.2 | ||||||||
| SD | 1.0 | 1.0 | 1.3 | 0.9 | 0.8 | 0.7 | 0.8 | 0.8 | 2.0 | 1.5 | 1.7 | 1.2 | |||||||||
| g | −0.19 | 0.28 | −0.23 | 1.29 | −0.30 | −0.48 | 0.02 | 0.57 | −0.35 | ||||||||||||
| −0.13 | 0.45 | 0.26 | |||||||||||||||||||
| SJ Time (ms) | M | 401 | 418 * | 384 | 398 | 423 | 393 | 387 | 400 * | 400 | 391 | 364 | 366 | ||||||||
| SD | 37 | 46 | 37 | 34 | 40 | 24 | 27 | 25 | 66 | 33 | 25 | 27 | |||||||||
| g | 0.38 | −0.77 | 0.37 | −0.85 | −0.25 | 0.48 | −0.18 | −0.87 | 0.09 | ||||||||||||
| −0.08 | −0.65 | −0.64 | |||||||||||||||||||
| CMJ Height (cm) | M | 34.4 | 33.7 | 34.0 | 35.6 | 35.1 | 36.3 | 36.4 | 38.4 | 35.5 | 37.7 | 37.1 | 38.1 | ||||||||
| SD | 7.3 | 5.5 | 4.8 | 3.8 | 5.6 | 5.2 | 5.1 | 5.3 | 7.5 | 6.2 | 5.0 | 6.0 | |||||||||
| g | −0.10 | 0.04 | 0.36 | 0.22 | 0.00 | 0.37 | 0.30 | −0.11 | 0.16 | ||||||||||||
| 0.19 | 0.57 | 0.35 | |||||||||||||||||||
| CMJ MF (N·kg−1) | M | 19.8 | 19.6 | 19.4 | 19.6 | 20.3 | 20.7 | 20.6 | 21.1 | 20.6 | 20.4 | 20.7 | 20.8 | ||||||||
| SD | 1.5 | 1.1 | 1.3 | 1.0 | 1.8 | 2.1 | 2.0 | 2.5 | 1.0 | 2.2 | 1.2 | 1.5 | |||||||||
| g | −0.17 | −0.13 | 0.18 | 0.17 | 0.03 | 0.18 | −0.13 | 0.13 | 0.10 | ||||||||||||
| −0.14 | 0.31 | 0.11 | |||||||||||||||||||
| CMJ Time (ms) | M | 258 | 263 | 264 | 260 | 249 | 247 | 247 | 244 | 235 | 249 | 243 | 243 | ||||||||
| SD | 27 | 22 | 29 | 23 | 37 | 37 | 39 | 42 | 18 | 36 | 22 | 37 | |||||||||
| g | 0.18 | 0.06 | −0.15 | −0.04 | 0.00 | −0.08 | 0.47 | −0.19 | −0.01 | ||||||||||||
| 0.07 | −0.12 | 0.25 | |||||||||||||||||||
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Suchomel, T.J.; McKeever, S.M.; McMahon, J.J.; Comfort, P. The Effect of Training with Weightlifting Catching or Pulling Derivatives on Squat Jump and Countermovement Jump Force–Time Adaptations. J. Funct. Morphol. Kinesiol. 2020, 5, 28. https://doi.org/10.3390/jfmk5020028
Suchomel TJ, McKeever SM, McMahon JJ, Comfort P. The Effect of Training with Weightlifting Catching or Pulling Derivatives on Squat Jump and Countermovement Jump Force–Time Adaptations. Journal of Functional Morphology and Kinesiology. 2020; 5(2):28. https://doi.org/10.3390/jfmk5020028
Chicago/Turabian StyleSuchomel, Timothy J., Shana M. McKeever, John J. McMahon, and Paul Comfort. 2020. "The Effect of Training with Weightlifting Catching or Pulling Derivatives on Squat Jump and Countermovement Jump Force–Time Adaptations" Journal of Functional Morphology and Kinesiology 5, no. 2: 28. https://doi.org/10.3390/jfmk5020028
APA StyleSuchomel, T. J., McKeever, S. M., McMahon, J. J., & Comfort, P. (2020). The Effect of Training with Weightlifting Catching or Pulling Derivatives on Squat Jump and Countermovement Jump Force–Time Adaptations. Journal of Functional Morphology and Kinesiology, 5(2), 28. https://doi.org/10.3390/jfmk5020028







