Numerical Study of a Model and Full-Scale Container Ship Sailing in Regular Head Waves
Abstract
1. Introduction
2. Numerical Approach
3. Computational Strategy
3.1. Ship Geometry
3.2. Test Cases
3.3. Computational Domain
3.4. Computational Grid and Grid Convergence Test
3.5. Simulation Setup
4. Results and Discussions
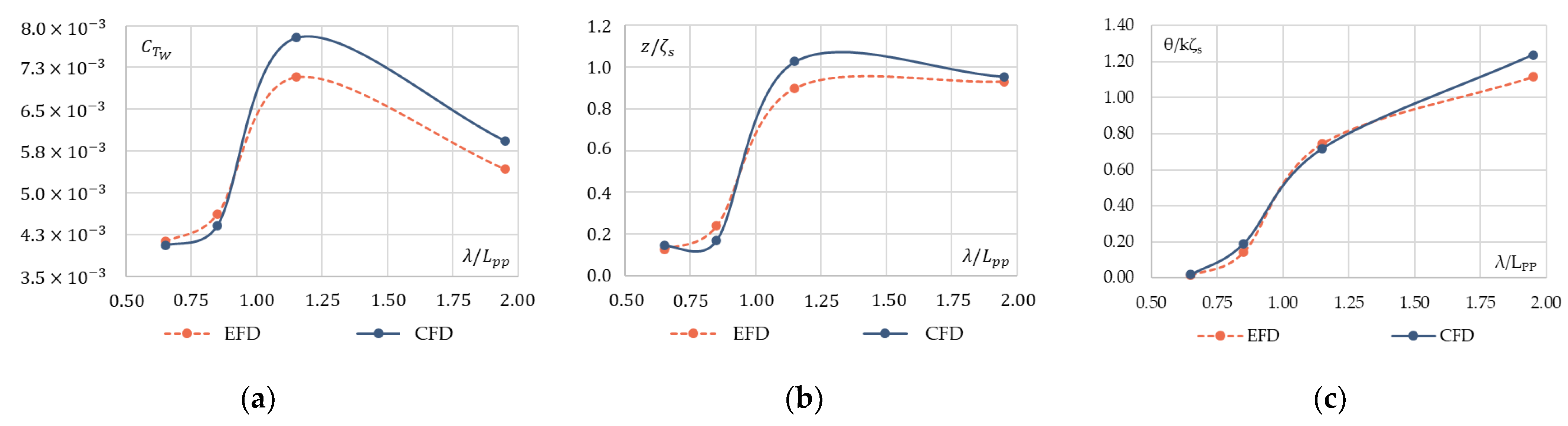
4.1. Verification and Validation
4.1.1. Calm Water Conditions
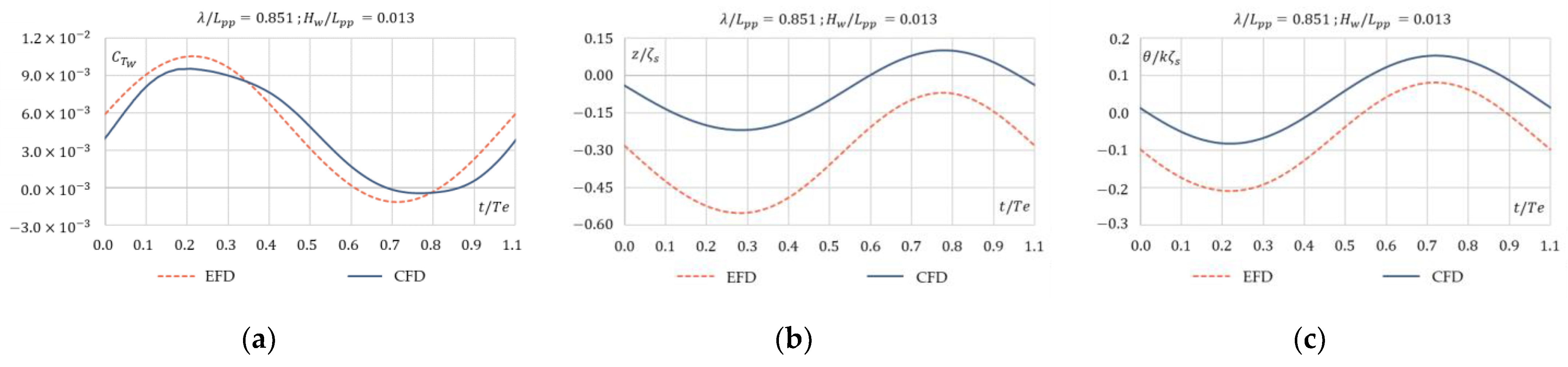
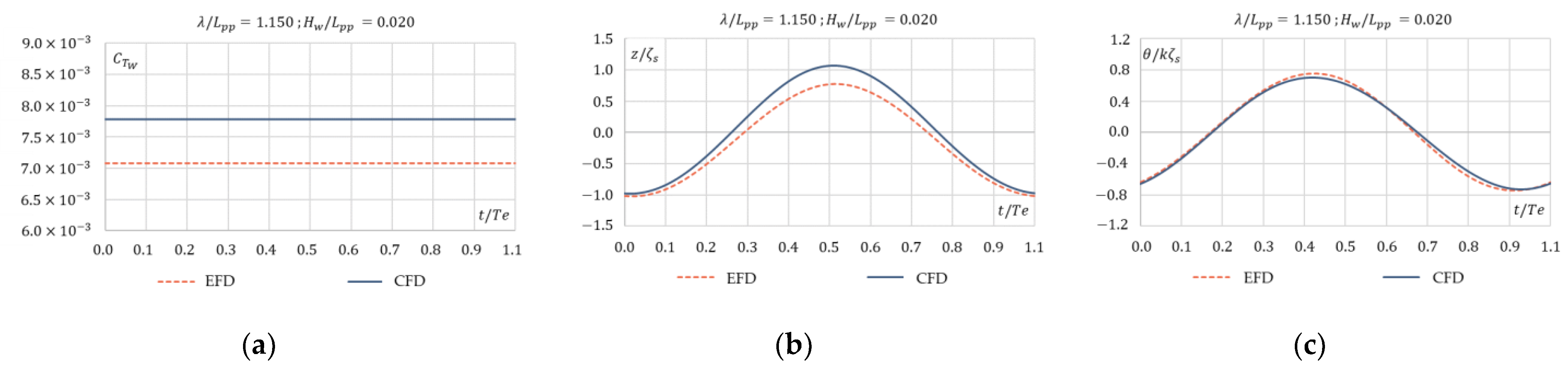
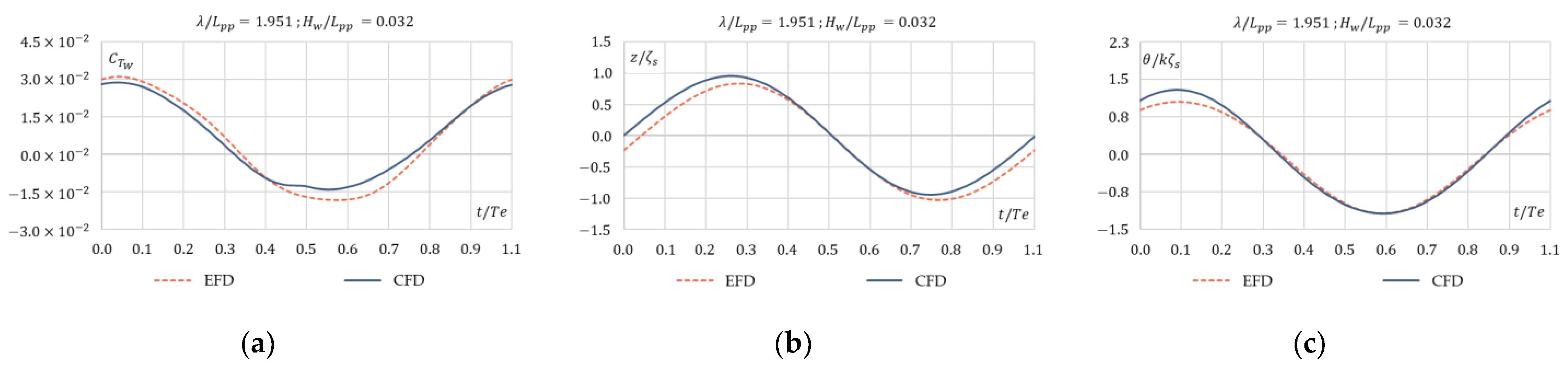
4.1.2. Head Wave Conditions
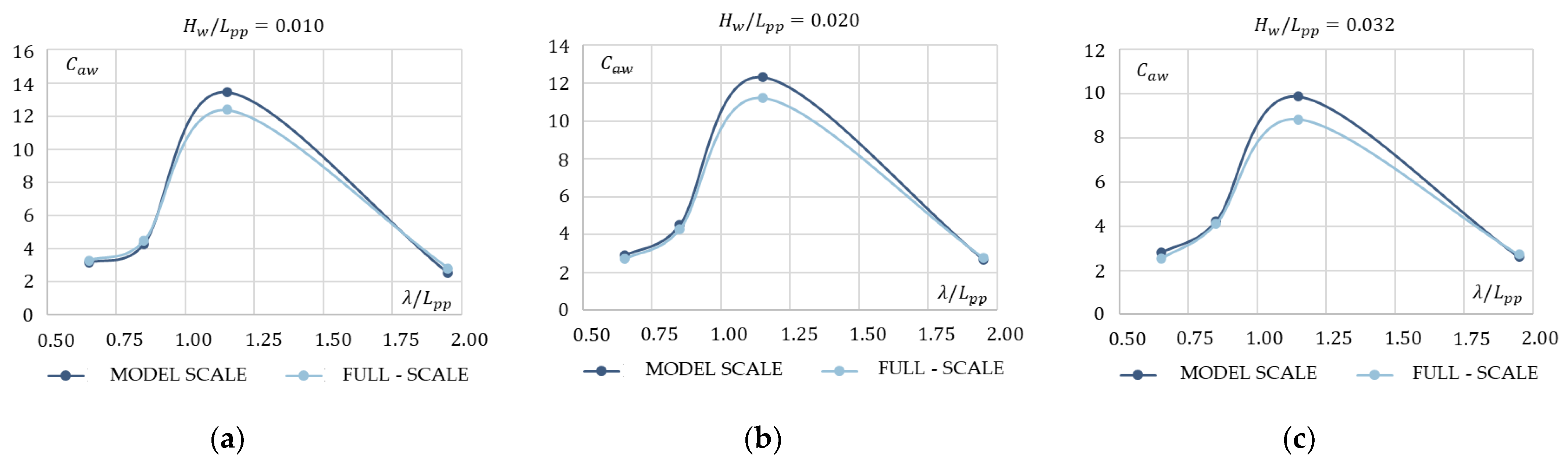
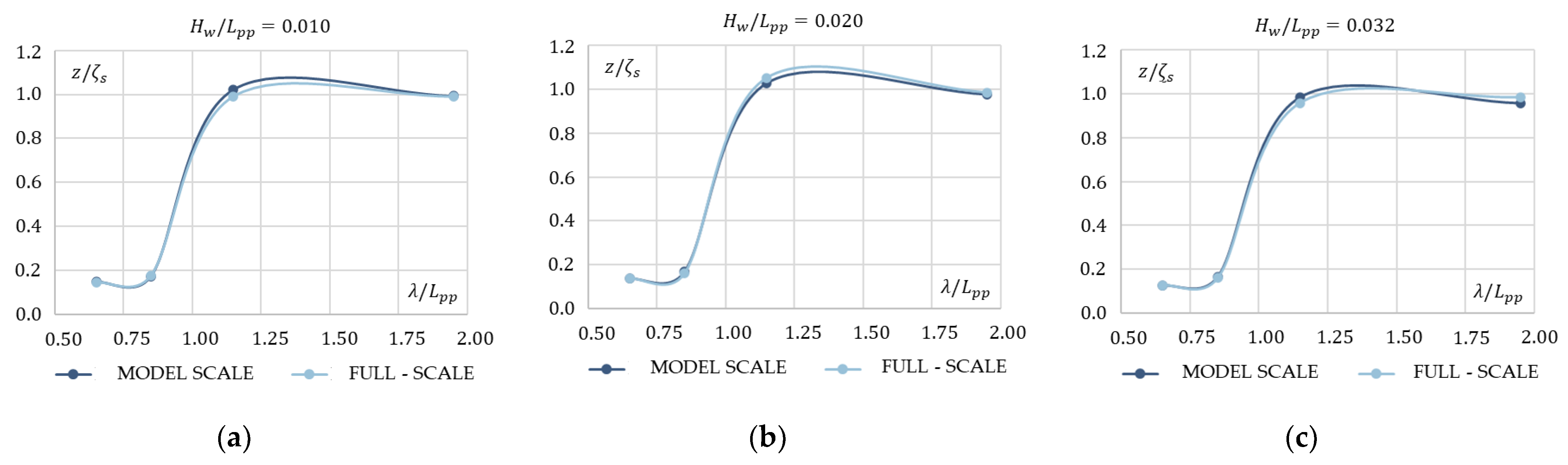
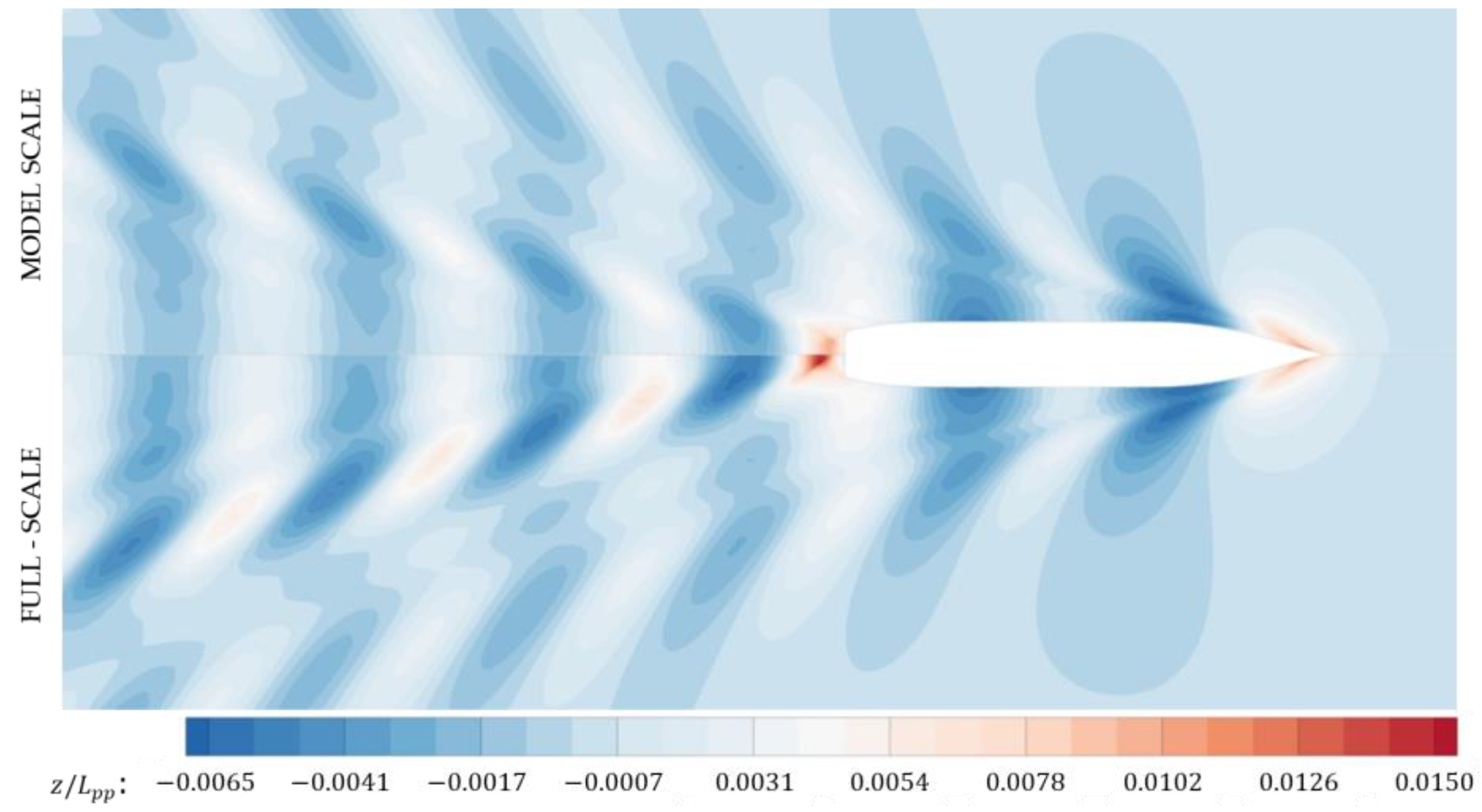
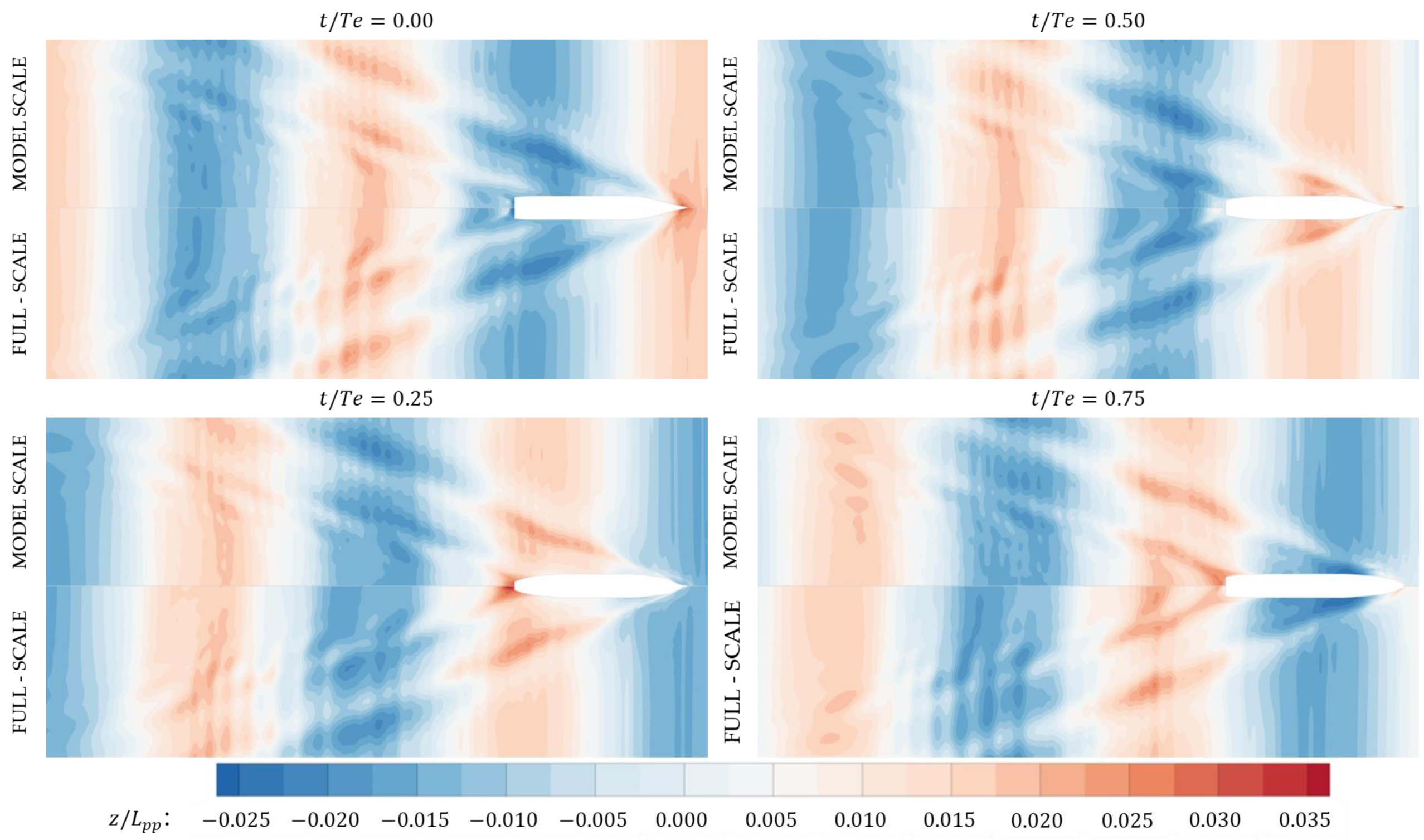
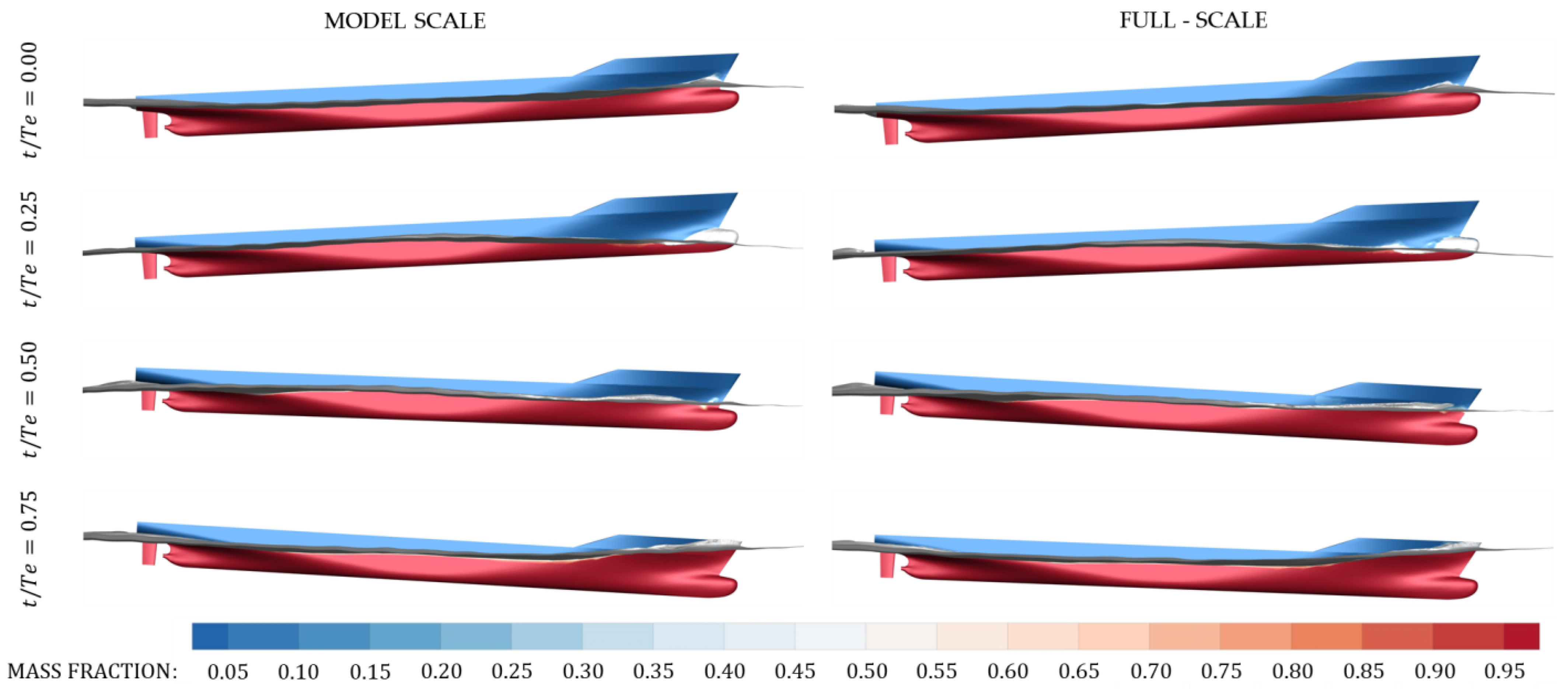
4.2. Scale Effect
5. Conclusions
- On the validation side, the CFD results were in good agreement with EFD data, especially in terms of total resistance coefficients, with a relative difference 1.22%; however, for the vertical motions, the values were slightly significant, at 6.88% for sinkage and 3.96% for trim. This indicates that the simulation is accurate, and the result deviation is acceptable in calm water condition.
- As far as the verification study is concerned, the simulations showed a monotonic convergence for all the test cases performed in this study. The numerical errors are more affected by the grid compared to the time step. The time step convergence showed that 600 iterations per wave period is sufficient for effective prediction of the simulation parameters. Further refinement of the time step had no impact on the results. On the other hand, the grid refinement was shown to have a significant effect on the results; the finer the grid, the better the accuracy. This complies with the theory and common practice in CFD simulations.
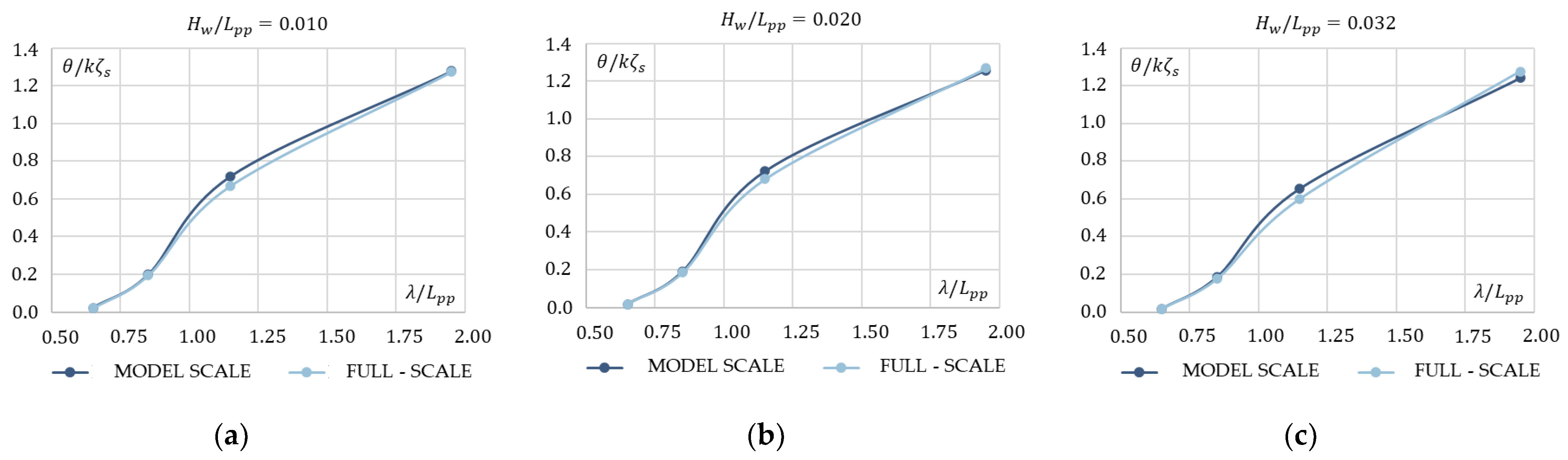
- Result validation in waves had a significant relative difference compared to that in calm water. Total resistance coefficient difference had an average value of 6.4%, while the average values for heave and pitch nondimensionalized parameters were 15.2% and 20.7%, respectively. The maximum difference for resistance was less than 10%, for heave it was within 29%, and it was within 34% for pitch motion. Though the difference between EFD and CFD was significant for ship sailing in waves, taking into consideration the fact that the study proposes a comparison between the same ship at different scales, the results were sufficient for comparative purposes, especially for the main target of the study, which is the added resistance in waves.
- Free-surface predictions showed that there was a slight difference observed between the model and full scale, especially at the stern zone and downstream; this was apparently related to the difference in form between model and full scale at the stern shoulders. Further investigation in that scope will be carried in separate research.
- The flow in the propeller plane was also investigated, revealing an obvious difference between model and full scale comparing the velocity contours and vortex formation in the propeller plane. The vortical formations are more significant at the model scale, while the contours are finely distributed, with fewer vortices at the full scale.
- A boundary layer fluctuation was observed as the ship sails in waves for both model and full scale. The effect was more accentuated at the model scale. Results showed that the change is significant, and may have an impact on the propulsion performance in waves. This is a subject of concern for the authors and shall be investigated in the future.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Spinelli, F.; Mancini, S.; Vitiello, L.; Bilandi, R.N.; De Carlini, M. Shipping Decarbonization: An Overview of the Different Stern Hydrodynamic Energy Saving Devices. J. Mar. Sci. Eng. 2022, 10, 574. [Google Scholar] [CrossRef]
- Terziev, M.; Tezdogan, T.; Incecik, A. Scale effects and full-scale ship hydrodynamics: A review. Ocean Eng. 2022, 245, 110496. [Google Scholar] [CrossRef]
- Larsson, L.; Raven, H.C. Principles of Naval Architecture: Ship Resistance and Flow; Publisher Society of Naval Architects and Marine Engineers: New Jersey, NJ, USA, 2010. [Google Scholar]
- Raven, H.; Van der Ploeg, A.; Starke, A.R.; Eça, L. Towards a CFD-based prediction of ship performance—Progress in predicting full-scale resistance and scale effects. Int. J. Marit. Eng. 2008, 150, 31–42. [Google Scholar]
- Terziev, M.; Tezdogan, T.; Incecik, A. A geosim analysis of ship resistance decomposition and scale effects with the aid of CFD. Appl. Ocean Res. 2019, 92, 101930. [Google Scholar] [CrossRef]
- Pena, B.; Muk-Pavic, E.; Fitzsimmons, P. Detailed analysis of the flow within the boundary layer and wake of a full-scale ship. Ocean Eng. 2020, 218, 108022. [Google Scholar] [CrossRef]
- Niklas, K.; Pruszko, H. Full-scale CFD simulations for the determination of ship resistance as a rational, alternative method to towing tank experiments. Ocean Eng. 2019, 190, 106435. [Google Scholar] [CrossRef]
- Bhushan, S.; Xing, T.; Carrica, P.; Stern, F. Model and Full-Scale URANS Simulations of Athena Resistance, Powering, Seakeeping, and 5415 Maneuvering. J. Ship Res. 2009, 53, 179–198. [Google Scholar] [CrossRef]
- Tezdogan, T.; Demirel, Y.K.; Kellett, P.; Khorasanchi, M.; Incecik, A.; Turan, O. Full-scale unsteady RANS CFD simulations of ship behaviour and performance in head seas due to slow steaming. Ocean Eng. 2015, 97, 186–206. [Google Scholar] [CrossRef]
- Mikkelsen, H.; Shao, Y.; Walther, J.H. Numerical study of nominal wake fields of a container ship in oblique regular waves. Appl. Ocean Res. 2022, 119, 102968. [Google Scholar] [CrossRef]
- Schuiling, B.; Lafeber, F.H.; van der Ploeg, A.; van Wijngaarden, E. The influence of the wake scale effect on the prediction of hull pressures due to cavitating propellers. In Proceedings of the Second International Symposium on Marine Propulsors, Hamburg, Germany, 15–17 June 2011. [Google Scholar]
- Zhang, H.; Zhang, D.; Guo, C.; Wang, L.; Liu, T. Numerical analysis of the scale effect of the nominal wake field of KCS. Chin. J. Ship Res. 2017, 12, 1–7. [Google Scholar] [CrossRef]
- Delen, C.; Bal, S. Telfer’s GEOSIM method revisited by CFD. Int. J. Marit. Eng. 2019, 161, A467–A478. [Google Scholar] [CrossRef]
- Can, U.; Delen, C.; Bal, S. Effective wake estimation of KCS hull at full-scale by GEOSIM method based on CFD. Ocean Eng. 2020, 218, 108052. [Google Scholar] [CrossRef]
- Guilmineau, E.; Deng, G.B.; Leroyer, A.; Queutey, P.; Visonneau, M.; Wackers, J. Influence of the turbulence closures for the wake prediction of a marine propeller. In Proceedings of the 4th International Symposium on Marine Propulsors, Austin, TX, USA, 31 May 2015. [Google Scholar]
- Menter, F.R. Two-equation eddy-viscosity turbulence models for engineering applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- Rhie, C.; Chow, W.L. A numerical study of the turbulent flow past an isolated airfoil with trailing edge separation. AIAA J. 1983, 17, 1525–1532. [Google Scholar] [CrossRef]
- Queutey, P.; Visnonneau, M. An interface capturing method for free-surface hydrodynamic flows. Comput. Fluids 2007, 36, 1481–1510. [Google Scholar] [CrossRef]
- Queutey, P.; Visonneau, M.; Leroyer, A.; Deng, G.; Guilmineau, E. RANSE simulations of a naval combatant in head waves. In Proceedings of the 11th Numerical Towing Tank Symposium, Brest, France, 7–9 September 2008. [Google Scholar]
- Deng, G.B.; Queutey, M.; Visonneau, M. RANS prediction of the KVLCC2 tanker in head waves. J. Hydrodyn. 2010, 22, 476–481. [Google Scholar] [CrossRef]
- Van, S.H.; Kim, W.J.; Yim, G.T.; Kim, D.H.; Lee, C.J. Experimental Investigation of the Flow Characteristics Around Practical Hull Forms. In Proceedings of the 3rd Osaka Colloquium on Advanced CFD Applications to Ship Flow and Hull Form Design, Osaka, Japan, 25–27 May 1998. [Google Scholar]
- Kim, W.J.; Van, S.H.; Kim, D.H. Measurement of flows around modern commercial ship models. Exp. Fluids 2001, 31, 567–578. [Google Scholar] [CrossRef]
- Zou, L.; Larsson, L. Additional data for resistance, sinkage and trim. In Numerical Ship Hydrodynamics: An Assessment of the Gothenburg 2010 Workshop; Springer Business Media: Dordrecht, The Netherlands, 2013; pp. 255–264. [Google Scholar]
- Simonsen, C.; Otzen, J.; Stern, F. EFD and CFD for KCS heaving and pitching in regular head waves. J. Mar. Sci. Technol. 2013, 18, 435–459. [Google Scholar] [CrossRef]
- Hino, T.; Ster, F.; Larsson, L.; Visonneau, M.; Hirata, N.; Kim, J. (Eds.) Numerical Ship Hydrodynamics. In An Assessment of the Tokyo 2015 Workshop; Lecture Notes in Applied and Computational Mechanics; Springer Nature: Cham, Switzerland, 2021; Volume 94, pp. 61–99. [Google Scholar]
- T2015 Workshop. Available online: http://www.t2015.nmri.go.jp (accessed on 30 December 2023).
- ITTC. Recommended Procedures and Guidelines: Practical Guidelines for Ship CFD Applications; 7.5-03 02-03, 2011, Rev. 01; ITTC: Rio de Janeiro, Brazil, 2011; pp. 1–18.
- Bekhit, A.; Lungu, A. URANSE simulation for the Seakeeping of the KVLCC2 Ship Model in Short and Long Regular Head Waves. In Proceedings of the IOP Conference Series: Materials Science and Engineering; IOP Publishing: Bristol, UK, 2019; Volume 591, p. 12102. [Google Scholar] [CrossRef]
- ITTC. Recommended Procedures and Guidelines, Uncertainty Analysis in CFD Verification and Validation Methodology and Procedures; Rev. 03; ITTC: Wuxi, China, 2017; pp. 1–13.
- Sun, W.; Hu, Q.; Hu, S.; Su, J.; Xu, J.; Wei, J.; Huang, G. Numerical Analysis of Full-Scale Ship Self-Propulsion Performance with Direct Comparison to Statistical Sea Trail Results. J. Mar. Sci. Eng. 2020, 8, 24. [Google Scholar] [CrossRef]
- Dogrul, A.; Song, S.; Demirel, Y.K. Scale effect on ship resistance components and form factor. Ocean Eng. 2020, 209, 107428. [Google Scholar] [CrossRef]
- Carrica, P.M.; Wilson, R.V.; Stern, F. Single-Phase Level Set Method for Unsteady Viscous Free Surface Flows. Int. J. Numer. Methods Fluids 2007, 53, 229–256. [Google Scholar] [CrossRef]
- Nakamura, S.; Naito, S. Propulsive performance of a container ship in waves. J. Soc. Nav. Archit. Jpn. 1977, 15, 24–48. [Google Scholar]
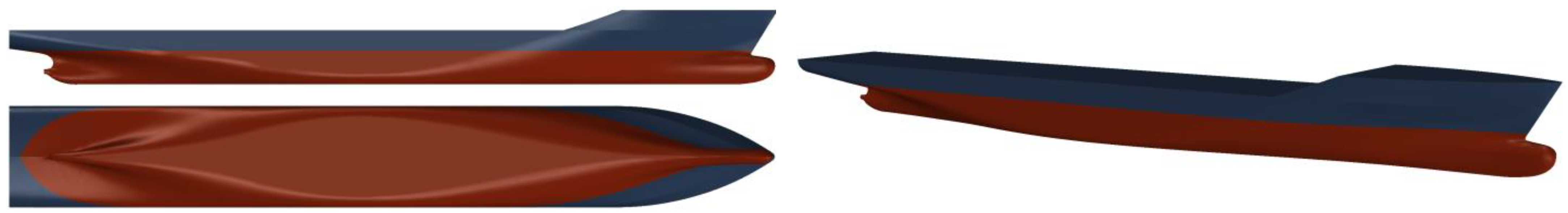

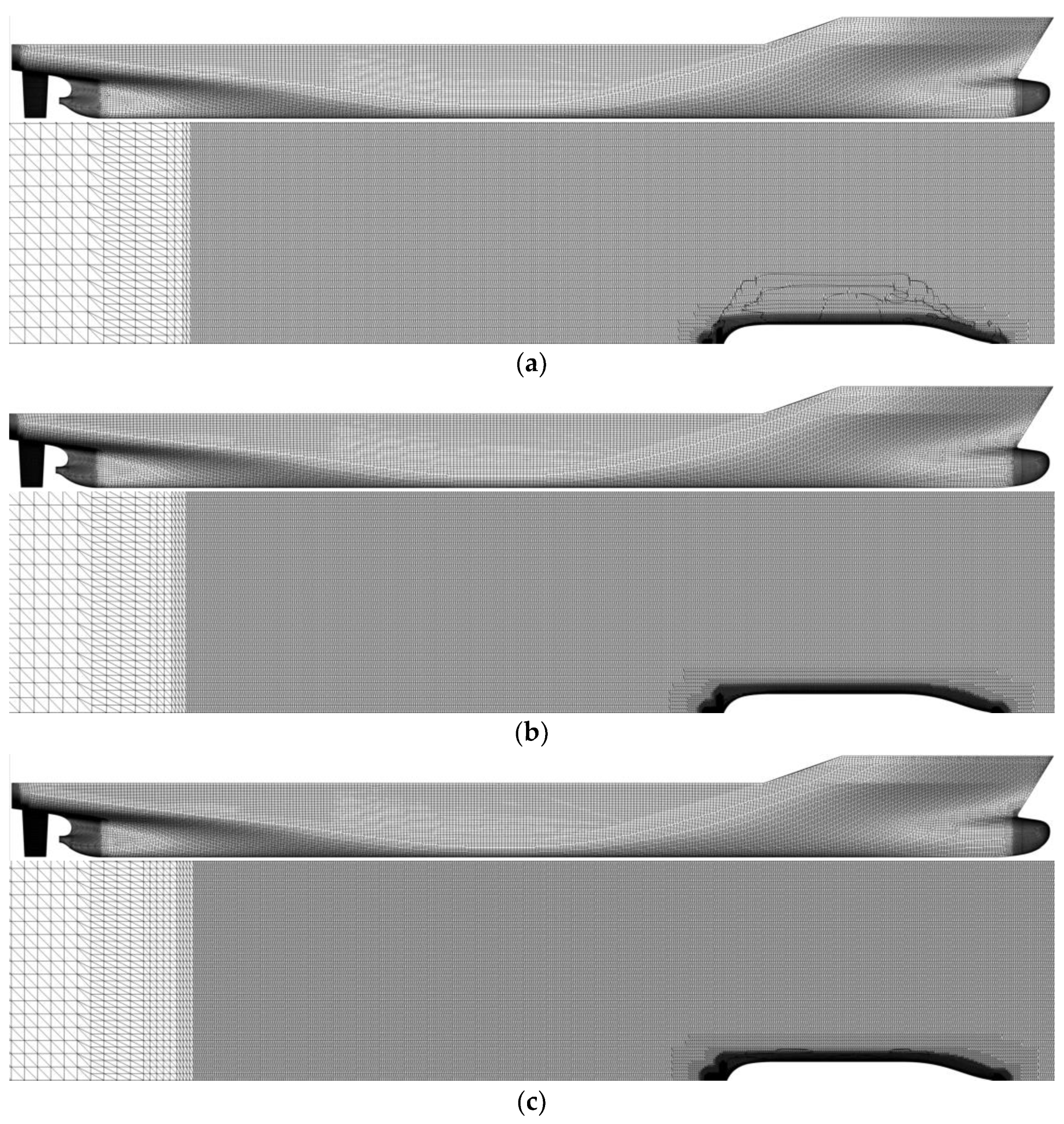


| Main Particulars | Symbol | Model Scale | Full Scale |
|---|---|---|---|
| Length between perpendiculars | 6.0702 | 230.0 | |
| Length of waterline | 6.1357 | 232.5 | |
| Maximum beam of waterline | 0.8498 | 32.3 | |
| Draft | 0.2850 | 10.8 | |
| Displacement volume | 0.9571 | 53,030 | |
| Block coefficient | 0.6505 | 0.6505 | |
| Wetted surface area | 6.6978 | 9539 |
| 0.261 | 0.000 | 0.000 | 0.000 | 0.000 | 0.000 | 0.000 |
| 0.651 | 3.949 | 149.628 | 0.010 | 0.063 | 2.349 | |
| 0.020 | 0.123 | 4.660 | ||||
| 0.032 | 0.196 | 7.426 | ||||
| 0.851 | 5.164 | 195.664 | 0.010 | 0.063 | 2.349 | |
| 0.013 | 0.078 | 2.955 | ||||
| 0.020 | 0.123 | 4.660 | ||||
| 0.032 | 0.196 | 7.426 | ||||
| 1.150 | 6.979 | 264.434 | 0.010 | 0.063 | 2.349 | |
| 0.020 | 0.123 | 4.660 | ||||
| 0.032 | 0.196 | 7.426 | ||||
| 1.951 | 11.840 | 448.618 | 0.010 | 0.063 | 2.349 | |
| 0.020 | 0.123 | 4.660 | ||||
| 0.032 | 0.196 | 7.426 |
| Scale | Parameter | |||||
|---|---|---|---|---|---|---|
| MODEL-SCALE | 6.394 | 11.023 | 0.580 | 8.832 | 8.832 | |
| 6.118 | 9.966 | 0.614 | 9.727 | 9.727 | ||
| −0.008 | −0.012 | 0.689 | −0.018 | 0.018 | ||
| −0.002 | −0.003 | 0.593 | −0.003 | 0.003 | ||
| FULL-SCALE | 4.529 | 7.113 | 0.637 | 7.935 | 7.935 | |
| 7.779 | 17.827 | 0.525 | 8.584 | 8.584 | ||
| −0.008 | −0.017 | 0.458 | −0.007 | 0.009 | ||
| −0.002 | −0.008 | 0.227 | −0.001 | 0.003 |
| 0.261 | 2.017 | −1.22% | −6.88% | 3.96% |
| 0.651 | 0.010 | −1.87% | 14.42% | 34.51% |
| 0.851 | 0.013 | −4.56% | −29.11% | 33.81% |
| 1.150 | 0.020 | 9.95% | 14.37% | −3.61% |
| 1.951 | 0.032 | 9.32% | 2.74% | 10.88% |
| 0.651 | 0.010 | 3.37% | −1.09% | −10.59% |
| 0.020 | −6.70% | 1.24% | 5.57% | |
| 0.032 | −9.29% | 1.34% | −2.10% | |
| 0.851 | 0.010 | 4.17% | 1.89% | −1.73% |
| 0.020 | −5.38% | −4.14% | −3.24% | |
| 0.032 | −2.66% | −3.11% | −5.92% | |
| 1.150 | 0.010 | −7.81% | −3.08% | −6.83% |
| 0.020 | −9.07% | 2.32% | −5.69% | |
| 0.032 | −10.61% | −2.66% | −8.42% | |
| 1.951 | 0.010 | 11.20% | −0.23% | −0.20% |
| 0.020 | 2.78% | 0.84% | 0.98% | |
| 0.032 | 4.36% | 2.74% | 2.79% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mandru, A.; Rusu, L.; Bekhit, A.; Pacuraru, F. Numerical Study of a Model and Full-Scale Container Ship Sailing in Regular Head Waves. Inventions 2024, 9, 22. https://doi.org/10.3390/inventions9010022
Mandru A, Rusu L, Bekhit A, Pacuraru F. Numerical Study of a Model and Full-Scale Container Ship Sailing in Regular Head Waves. Inventions. 2024; 9(1):22. https://doi.org/10.3390/inventions9010022
Chicago/Turabian StyleMandru, Andreea, Liliana Rusu, Adham Bekhit, and Florin Pacuraru. 2024. "Numerical Study of a Model and Full-Scale Container Ship Sailing in Regular Head Waves" Inventions 9, no. 1: 22. https://doi.org/10.3390/inventions9010022
APA StyleMandru, A., Rusu, L., Bekhit, A., & Pacuraru, F. (2024). Numerical Study of a Model and Full-Scale Container Ship Sailing in Regular Head Waves. Inventions, 9(1), 22. https://doi.org/10.3390/inventions9010022







