A Wide Operating Range Ejector with Part Nested Nozzles for PEMFC Hydrogen Recirculation System
Abstract
:1. Introduction
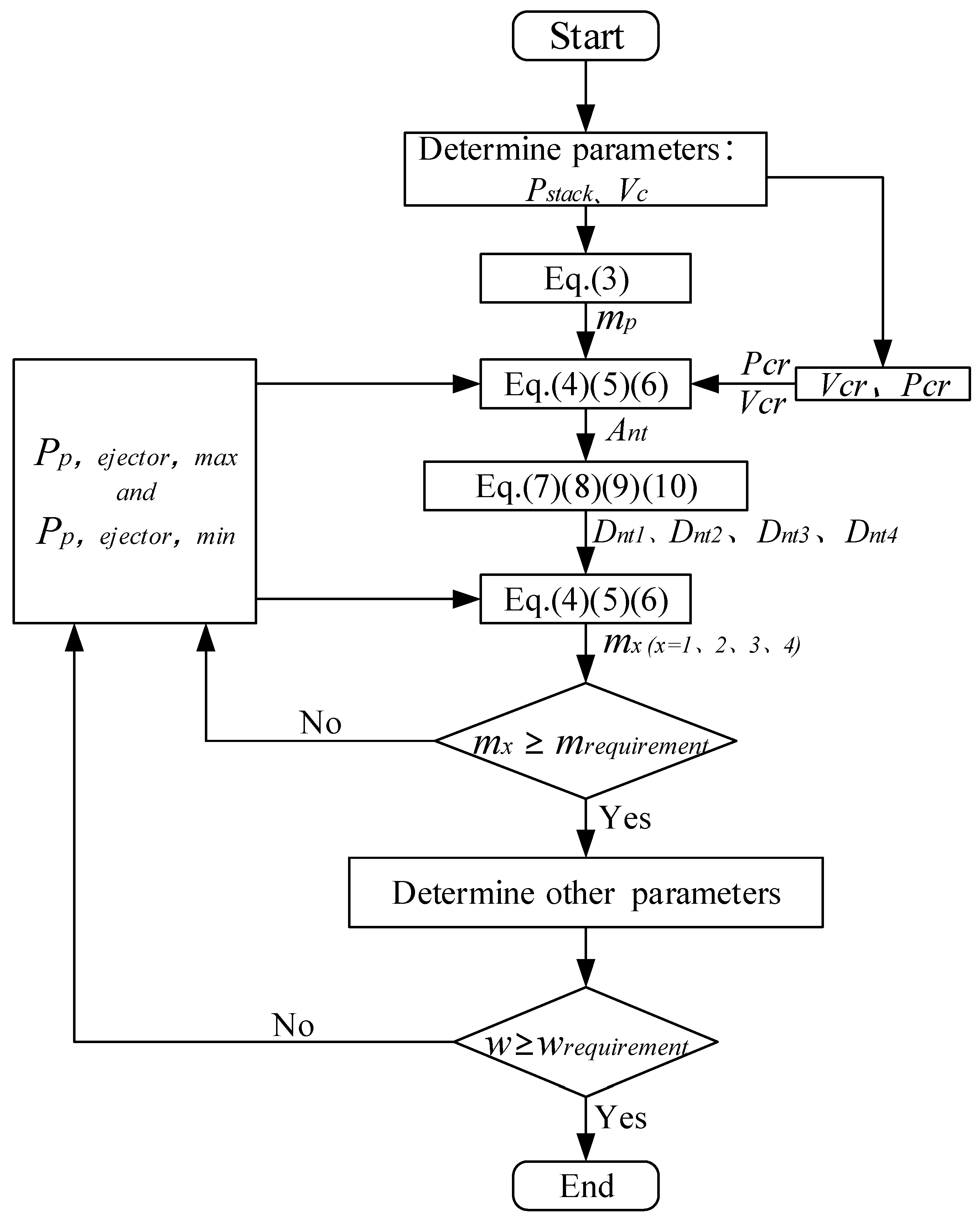
2. PNFN Ejector Design Method
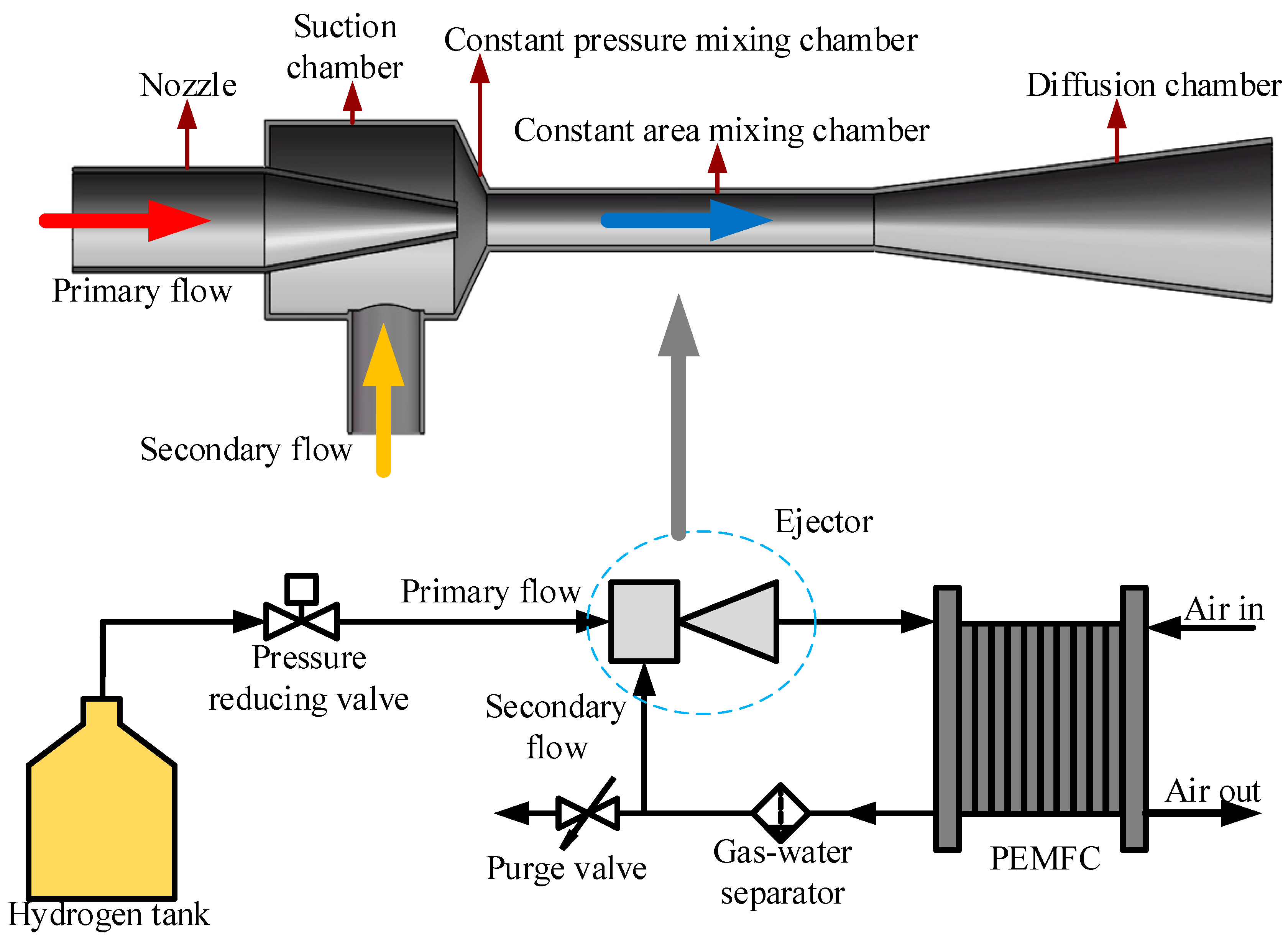
2.1. Ejector in the PEMFC Operating Theory
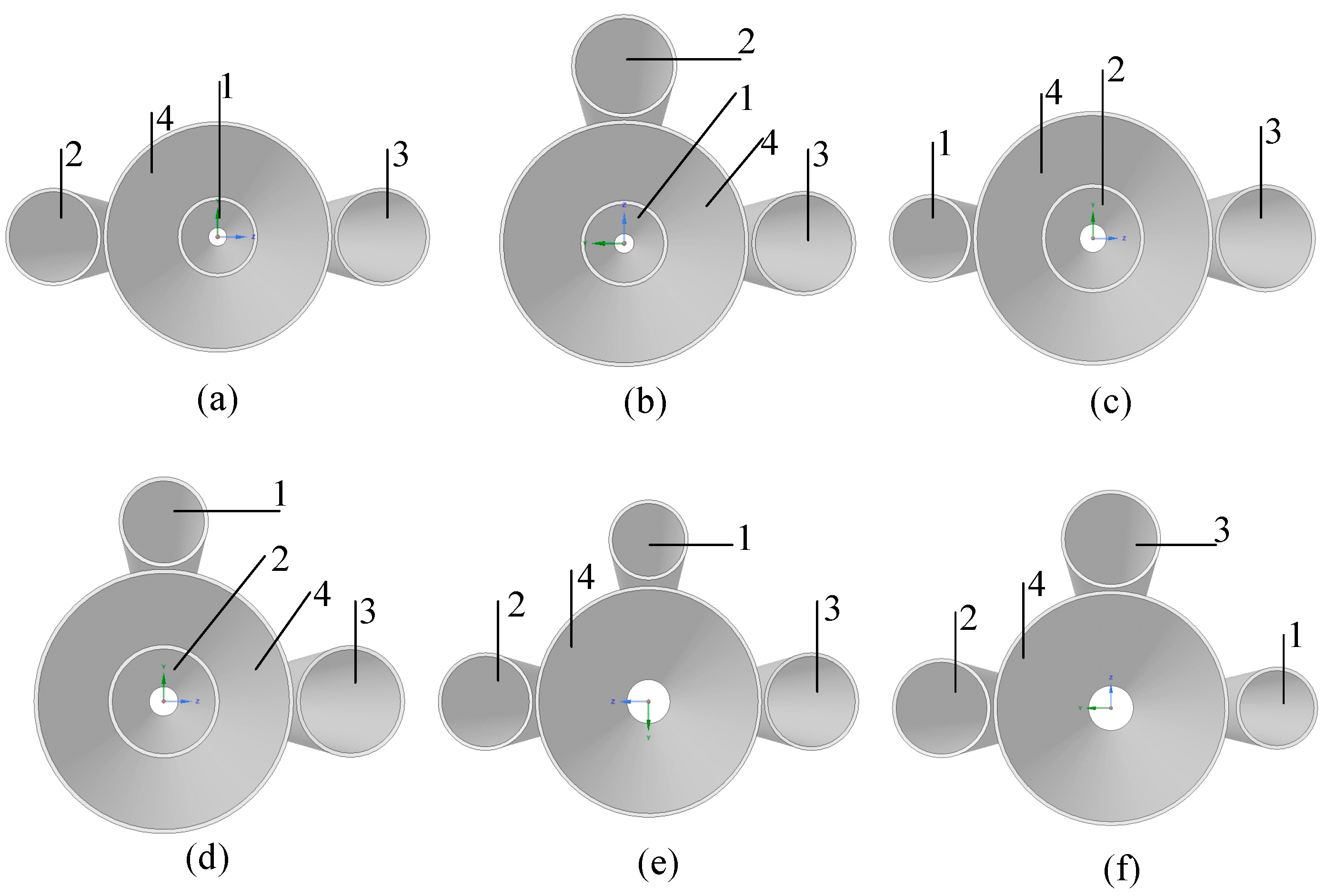
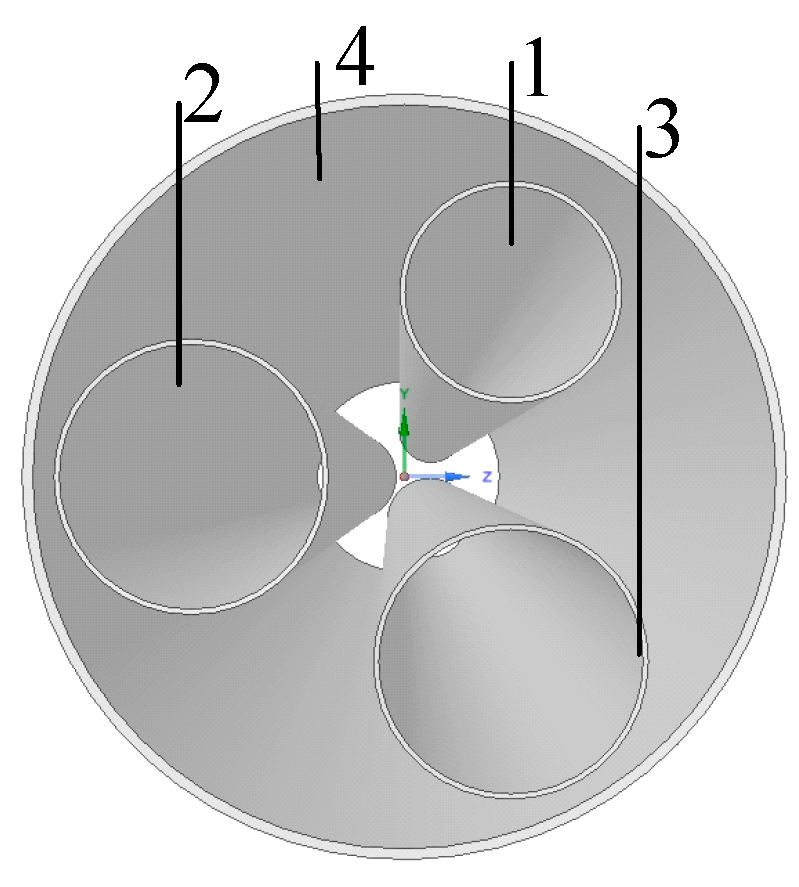
2.2. Structural Design of the PNFN Ejector
3. Numerical Model and Simulation
3.1. Governing Equation
- (1)
- The walls are considered adiabatic.
- (2)
- The internal gas is considered an ideal gas.
- (3)
- The inner fluid of the ejector is fully mixed.
- (4)
- The inner fluid is regarded as compressible fluid.
3.2. Grid Generation
3.3. Boundary Conditions
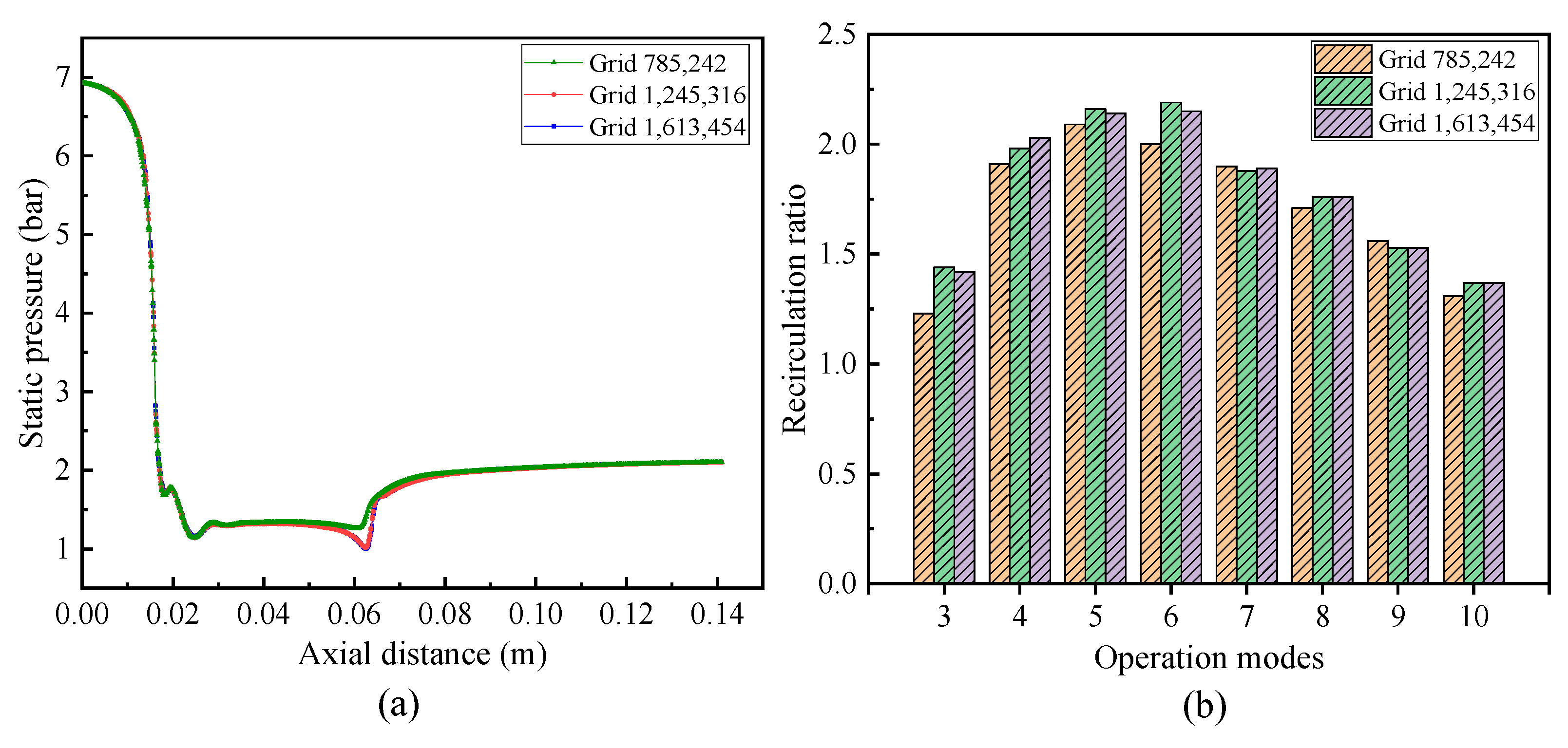
3.4. Grid Independence Verification
4. Experimental Facility and Model Verification
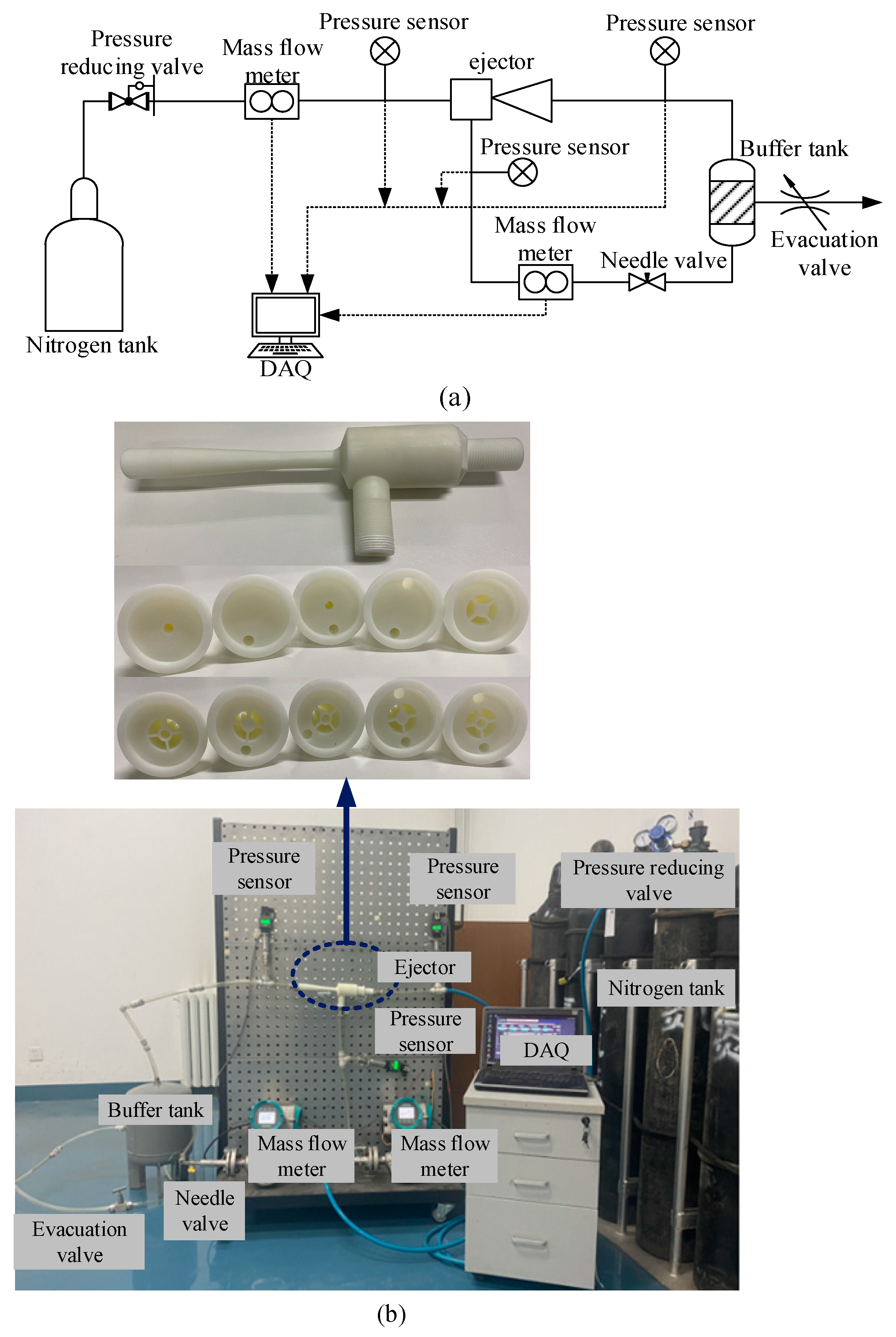
4.1. Experimental Facility
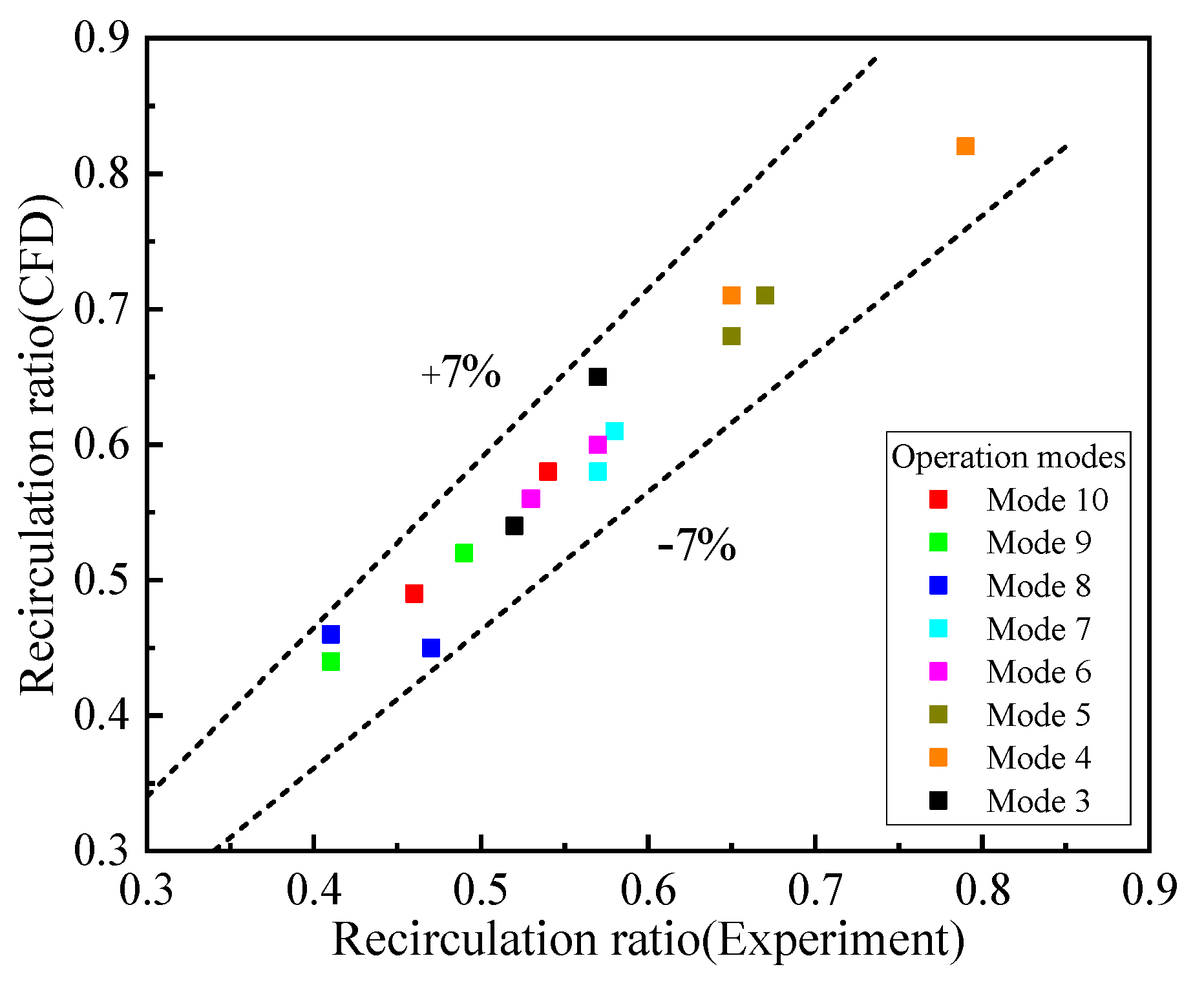
4.2. Model Verification
5. Results and Analysis
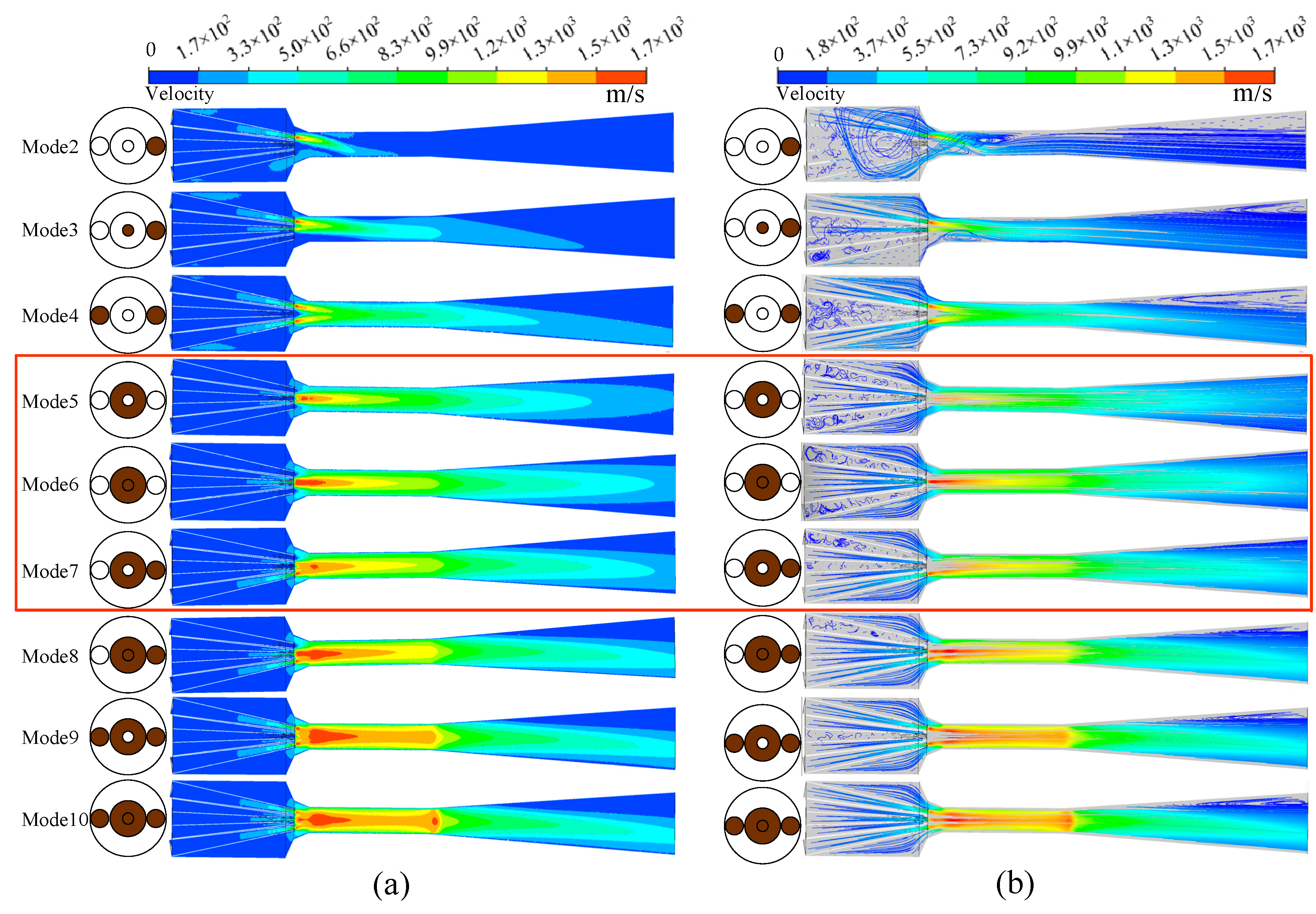
5.1. Characteristics of the PNFN Ejector’s Flow
5.2. Performance of the PNFN Ejector
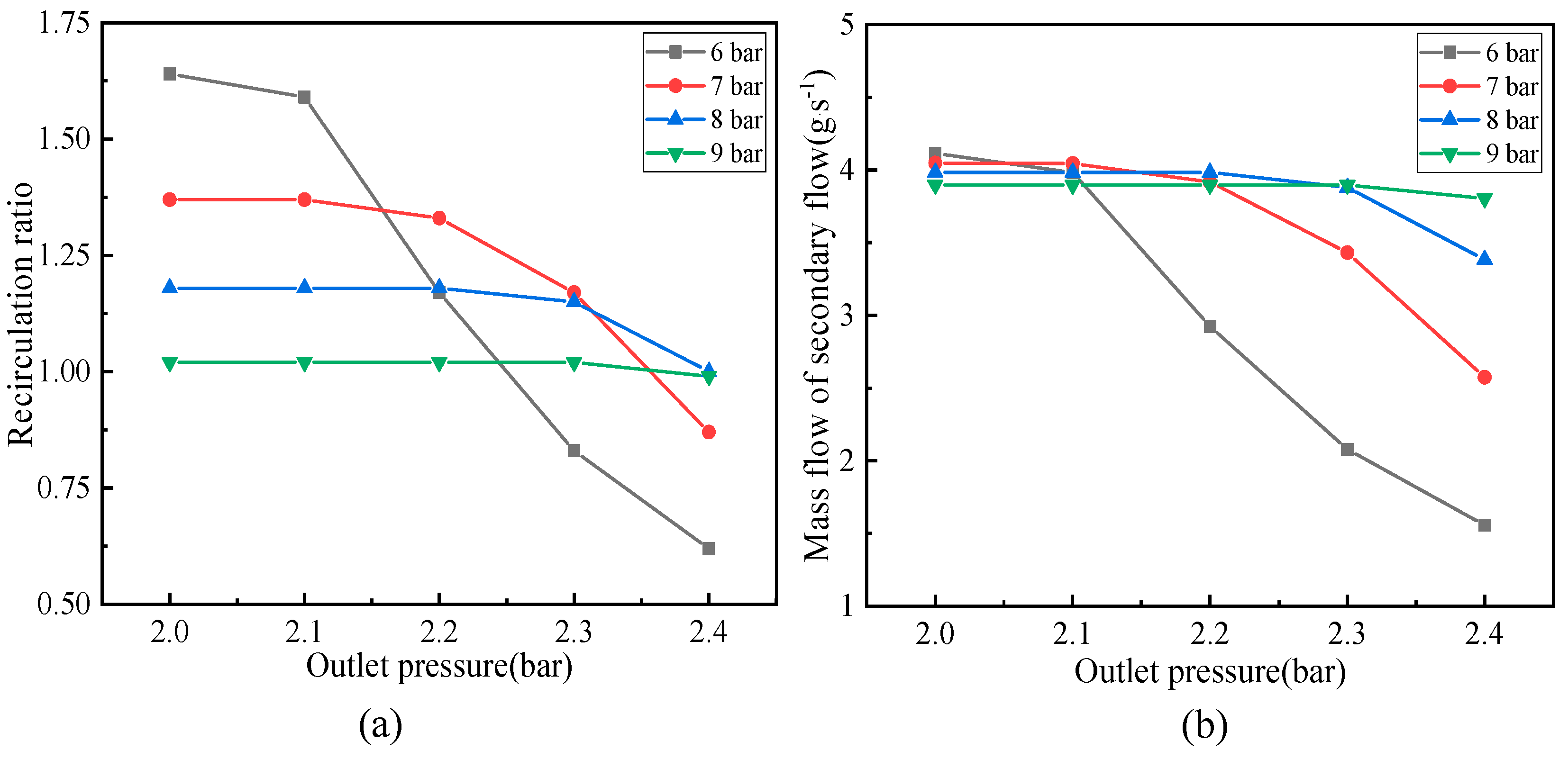
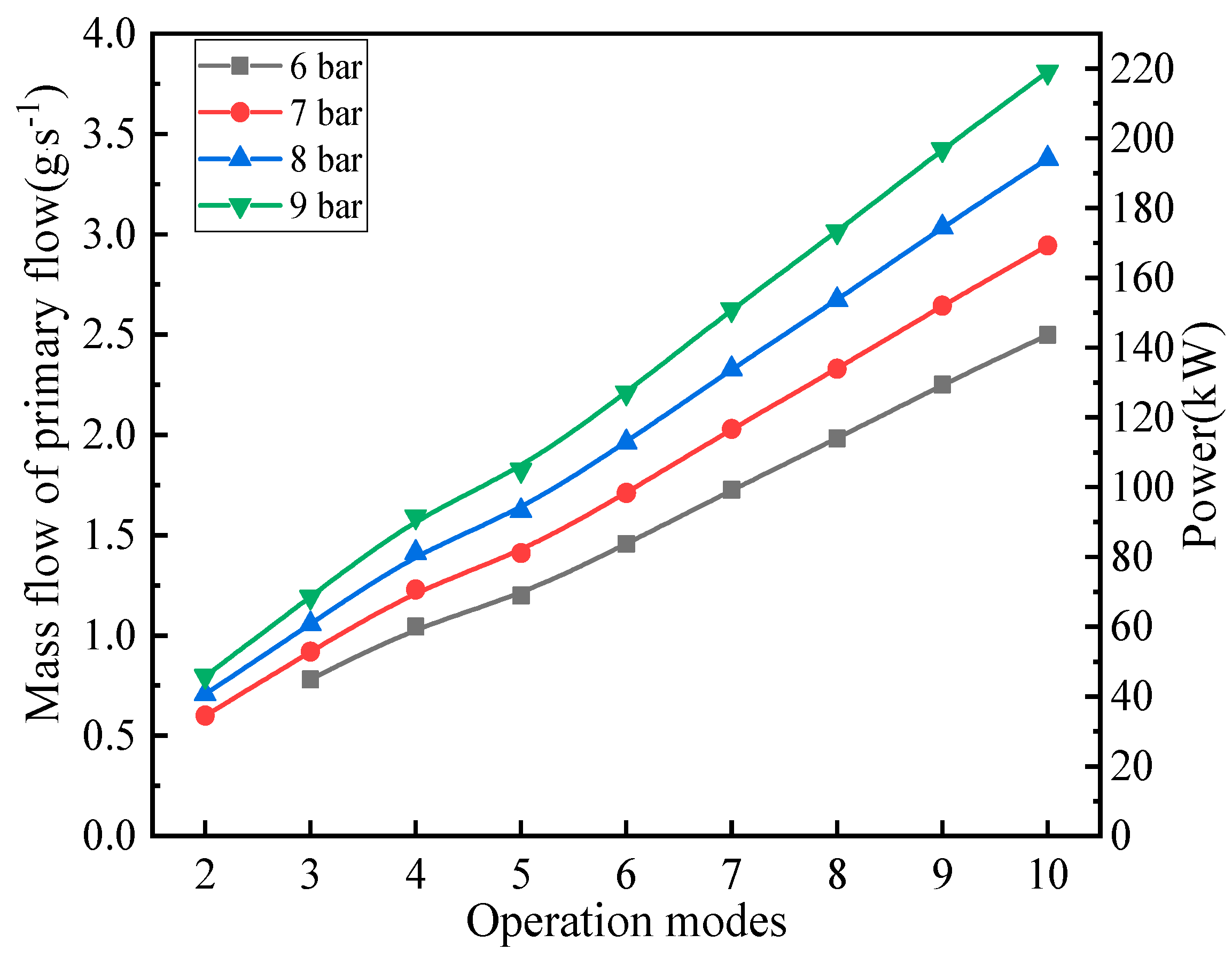
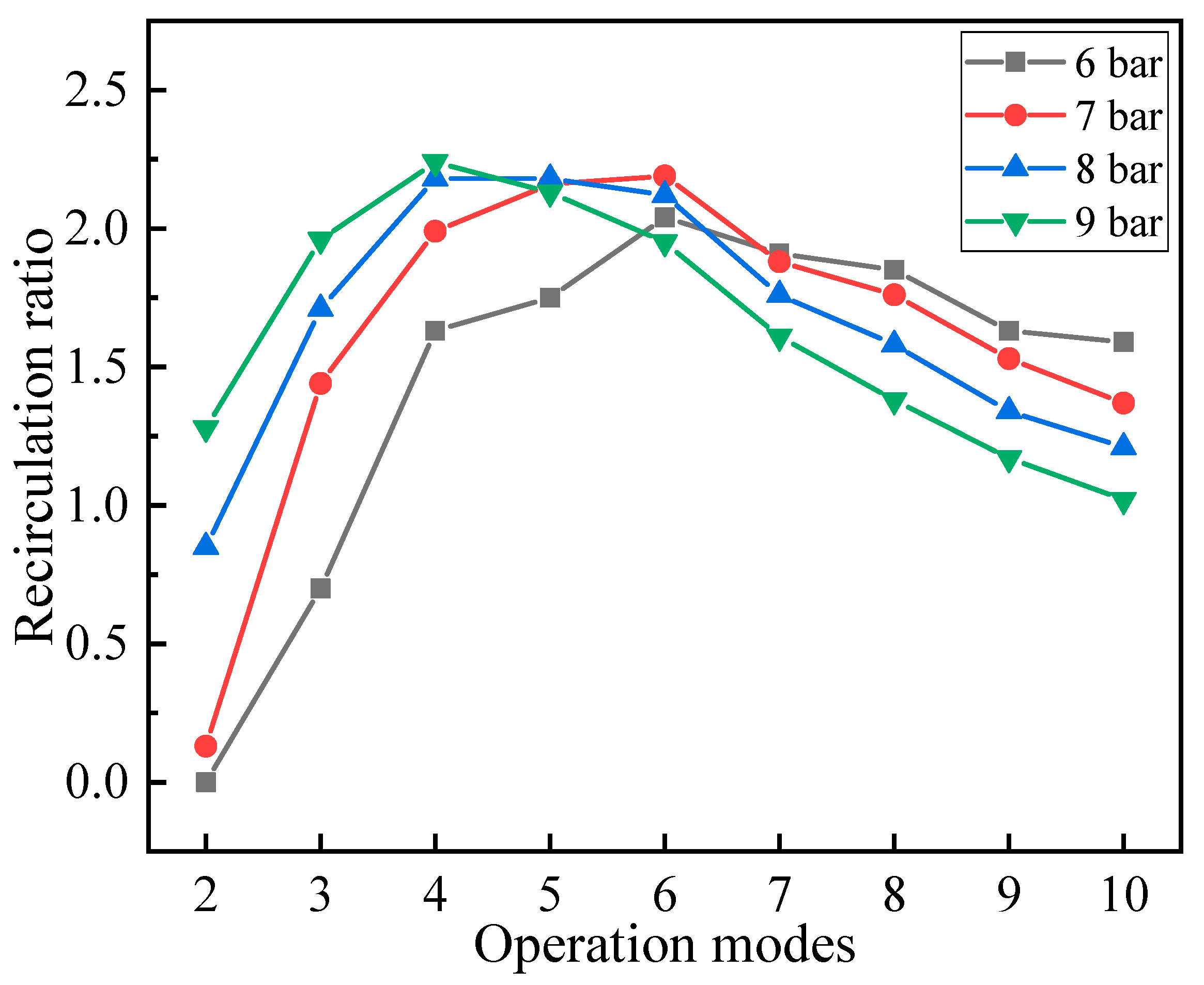
5.3. Performance of the PEMFC System’s PNFN Ejector in Terms of Recirculation
5.4. Performance Comparison of the PNFN and Traditional Ejectors
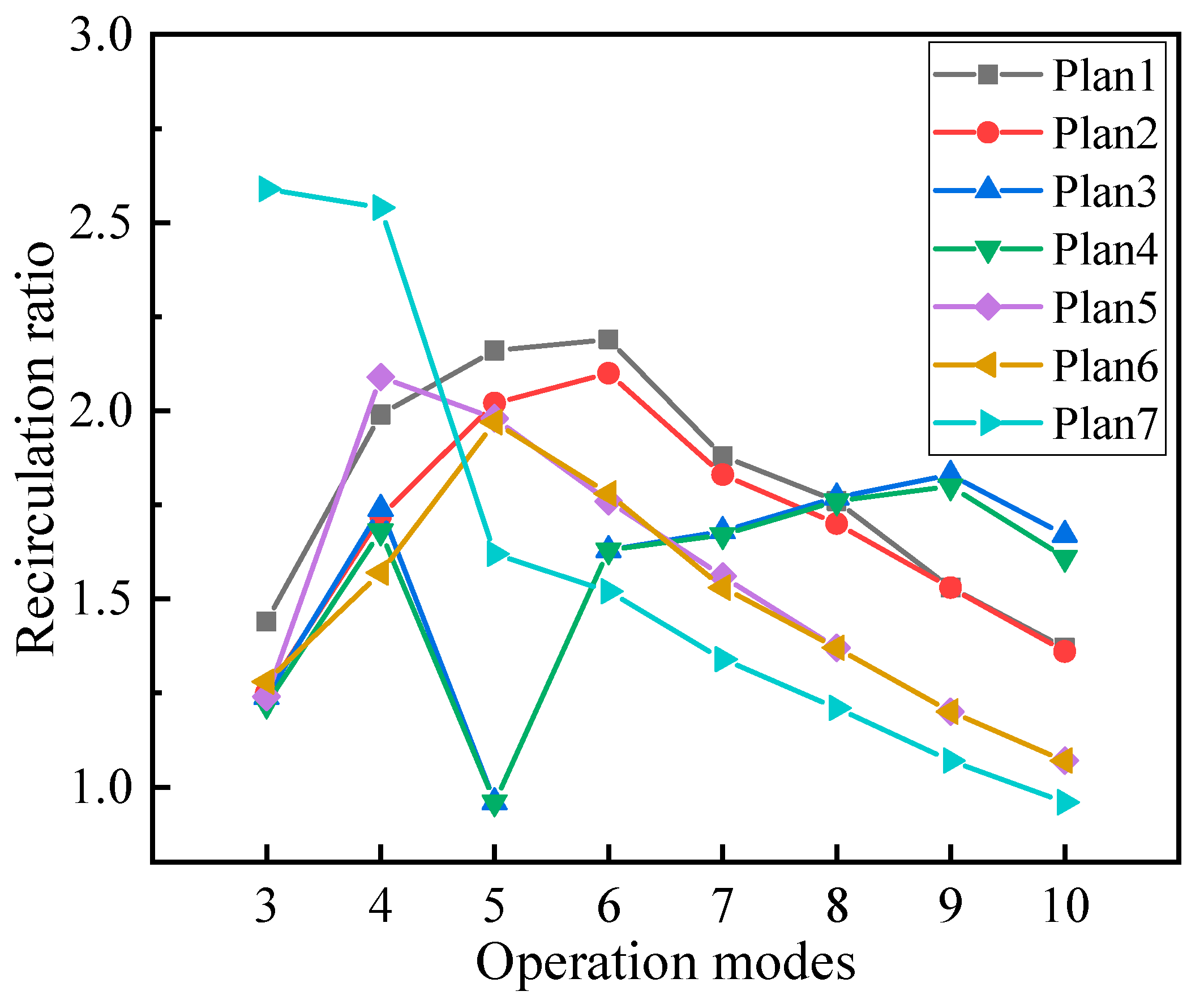
5.5. Performance Comparison of Different Configuration Four-Nozzle Ejector
6. Conclusions
- The ejector inner flow field is at the most stable state and ejector performance reach the best value point when the first nozzle and the fourth nozzle (mode 6) work together. By observing the velocity field image and velocity streamline map, the ejector inner flow mechanism becomes more complex with the increase of working nozzles.
- The PNFN ejector with different operation modes can operate in the 34–220 kW power range with the recirculation ratio above 1.0 when the PF pressure range is between 7–9 bar.
- The PNFN ejector performs better than the traditional single nozzle ejector in the whole power range. It’s worth noting that the PNFN ejector has better performance compared to traditional ejectors in the low power zone.
- The performance of the PNFN ejector (Plan 1) is better than other combined plans by comparing the typical combined plans of the four nozzles.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| A | area (m2) | λ | stoichiometric ratio/thermal conductivity (W m−1 K−1) |
| D | diameter (m) | μ | dynamic viscosity (N s m−2) |
| Dq,m | mass diffusion coefficient (m2 s−1) | ρ | density (kg m−3) |
| DT,q | Thermal diffusion coefficient (m2 s−1) | ω | recirculation ratio |
| E | total energy (J kg−1) | μ | dynamic viscosity (N s m−2) |
| F | Faraday’s constant (C mol−1) | ρ | density (kg m−3) |
| gravitational acceleration (m s−2) | Subscript | ||
| GbGk | turbulence kinetic energy generation (J m−3 s−1) | r | recirculated hydrogen |
| h | enthalpy (J kg−1) | c | hydrogen consumption |
| I | unit tensor diffusion flux (kg m−2 s−1) | cam | constant-area mixing chamber |
| k | turbulent kinetic energy (m2 s−2) | cpm | constant-pressure mixing chamber |
| L | length (m) | d | diffusion |
| m | mass flow rate (kg s−1) | H2 | hydrogen |
| M | molar weight (kg mol−1) | i, j | direction |
| P | pressure (Pa) | nt | nozzle throat |
| Pstack | power (W) | N1 | first nozzle |
| R | gas constant (J mol−1 K−1) | N2 | second nozzle |
| Sct | Schmidt number | N3 | |
| T | temperature (K) | N4 | fourth nozzle |
| vcr | critical velocity (m s−1) | Abbreviations | |
| velocity vector (m s−1) | CFD | computational fluid dynamics | |
| Vc | single cell voltage (V) | NXP | nozzle exit position |
| Y | mass fraction | PEMFC | polymer electrolyte membrane fuel cell |
| YM | the fluctuating expansion to total dissipation ratio in compressible turbulent | PNFN | part nested four-nozzle |
| Greek letters | PF | primary flow | |
| ε | turbulence kinetic energy dissipation rate (m2 s−3) | SF | secondary flow |
| θ | angle (°) | ||
| inverse effective Prandtl numbers | |||
| κ | specific heat ratio | ||
References
- Liu, Z.; Chen, J.; Liu, H.; Yan, C.; Hou, Y.; He, Q.; Zhang, J.; Hissel, D. Anode purge management for hydrogen utilization and stack durability improvement of PEM fuel cell systems. Appl. Energy 2020, 275, 115110. [Google Scholar] [CrossRef]
- Rodosik, S.; Poirot-Crouvezier, J.-P.; Bultel, Y. Simplified anode architecture for PEMFC systems based on alternative fuel feeding: Experimental characterization and optimization for automotive applications. Int. J. Hydrogen Energy 2020, 45, 19720–19732. [Google Scholar] [CrossRef]
- Wang, X.; Zhong, W.; Xiao, B.; Liu, Q.; Yang, L.; Covaci, A.; Zhu, L. Bioavailability and biomagnification of organophosphate esters in the food web of Taihu Lake, China: Impacts of chemical properties and metabolism. Environ. Int. 2019, 125, 25–32. [Google Scholar] [CrossRef] [PubMed]
- Zhu, Y.; Yousefi, N. Optimal parameter identification of PEMFC stacks using Adaptive Sparrow Search Algorithm. Int. J. Hydrogen Energy 2021, 46, 9541–9552. [Google Scholar] [CrossRef]
- Chen, H.; Song, Z.; Zhao, X.; Zhang, T.; Pei, P.; Liang, C. A review of durability test protocols of the proton exchange membrane fuel cells for vehicle. Appl. Energy 2018, 224, 289–299. [Google Scholar] [CrossRef]
- Kazim, A. Introduction of PEM fuel-cell vehicles in the transportation sector of the United Arab Emirates. Appl. Energy 2003, 74, 125–133. [Google Scholar] [CrossRef]
- Pei, P.; Chen, H. Main factors affecting the lifetime of Proton Exchange Membrane fuel cells in vehicle applications: A review. Appl. Energy 2014, 125, 60–75. [Google Scholar] [CrossRef]
- Li, W.; Wang, X.; Wang, L.; Jia, L.; Song, R.; Fu, Z.; Xu, W. An LSTM and ANN Fusion Dynamic Model of a Proton Exchange Membrane Fuel Cell. IEEE Trans. Ind. Inform. 2023, 19, 5743–5751. [Google Scholar] [CrossRef]
- Hwang, J.-J. Passive hydrogen recovery schemes using a vacuum ejector in a proton exchange membrane fuel cell system. J. Power Sources 2014, 247, 256–263. [Google Scholar] [CrossRef]
- Li, F.; Du, J.; Zhang, L.; Li, J.; Li, G.; Zhu, G.; Ouyang, M.; Chai, J.; Li, H. Experimental determination of the water vapor effect on subsonic ejector for proton exchange membrane fuel cell (PEMFC). Int. J. Hydrogen Energy 2017, 42, 29966–29970. [Google Scholar] [CrossRef]
- Toghyani, S.; Afshari, E.; Baniasadi, E. A parametric comparison of three fuel recirculation system in the closed loop fuel supply system of PEM fuel cell. Int. J. Hydrogen Energy 2019, 44, 7518–7530. [Google Scholar] [CrossRef]
- Liu, Y.; Luo, X.; Tu, Z.; Chan, S.H. Droplet Dynamics in a Proton Exchange Membrane Fuel Cell with Ejector-Based Recirculation. Energy Fuels 2021, 35, 11533–11544. [Google Scholar] [CrossRef]
- Liu, Y.; Tu, Z.; Chan, S.H. Applications of ejectors in proton exchange membrane fuel cells: A review. Fuel Process. Technol. 2021, 214, 106683. [Google Scholar] [CrossRef]
- Kim, M.; Sohn, Y.-J.; Cho, C.-W.; Lee, W.-Y.; Kim, C.-S. Customized design for the ejector to recirculate a humidified hydrogen fuel in a submarine PEMFC. J. Power Sources 2008, 176, 529–533. [Google Scholar] [CrossRef]
- Dadvar, M.; Afshari, E. Analysis of design parameters in anodic recirculation system based on ejector technology for PEM fuel cells: A new approach in designing. Int. J. Hydrogen Energy 2014, 39, 12061–12073. [Google Scholar] [CrossRef]
- Yin, Y.; Fan, M.; Jiao, K.; Du, Q.; Qin, Y. Numerical investigation of an ejector for anode recirculation in proton exchange membrane fuel cell system. Energy Convers. Manag. 2016, 126, 1106–1117. [Google Scholar] [CrossRef]
- Maghsoodi, A.; Afshari, E.; Ahmadikia, H. Optimization of geometric parameters for design a high-performance ejector in the proton exchange membrane fuel cell system using artificial neural network and genetic algorithm. Appl. Therm. Eng. 2014, 71, 410–418. [Google Scholar] [CrossRef]
- Yang, Y.; Du, W.; Ma, T.; Lin, W.; Cong, M.; Yang, H.; Yu, Z. Numerical studies on ejector structure optimization and performance prediction based on a novel pressure drop model for proton exchange membrane fuel cell anode. Int. J. Hydrogen Energy 2020, 45, 23343–23352. [Google Scholar] [CrossRef]
- Pei, P.; Ren, P.; Li, Y.; Wu, Z.; Chen, D.; Huang, S.; Jia, X. Numerical studies on wide-operating-range ejector based on anodic pressure drop characteristics in proton exchange membrane fuel cell system. Appl. Energy 2019, 235, 729–738. [Google Scholar] [CrossRef]
- Wang, L.; Yan, J.; Wang, C.; Li, X. Numerical study on optimization of ejector primary nozzle geometries. Int. J. Refrig. 2017, 76, 219–229. [Google Scholar] [CrossRef]
- Amin, B.; Tavousi, E.; Noghrehabadi, A.; Behbahani-Nejad, M. Study of a novel inlet geometry for ejectors. Int. J. Refrig. 2022, 139, 113–127. [Google Scholar] [CrossRef]
- Brunner, D.A.; Marcks, S.; Bajpai, M.; Prasad, A.K.; Advani, S.G. Design and characterization of an electronically controlled variable flow rate ejector for fuel cell applications. Int. J. Hydrogen Energy 2012, 37, 4457–4466. [Google Scholar] [CrossRef]
- Jenssen, D.; Berger, O.; Krewer, U. Improved PEM fuel cell system operation with cascaded stack and ejector-based recirculation. Appl. Energy 2017, 195, 324–333. [Google Scholar] [CrossRef]
- He, J.; Choe, S.-Y.; Hong, C.-O. Analysis and control of a hybrid fuel delivery system for a polymer electrolyte membrane fuel cell. J. Power Sources 2008, 185, 973–984. [Google Scholar] [CrossRef]
- Nikiforow, K.; Koski, P.; Ihonen, J. Discrete ejector control solution design, characterization, and verification in a 5 kW PEMFC system. Int. J. Hydrogen Energy 2017, 42, 16760–16772. [Google Scholar] [CrossRef]
- Xue, H.; Wang, L.; Zhang, H.; Jia, L.; Ren, J. Design and investigation of multi-nozzle ejector for PEMFC hydrogen recirculation. Int. J. Hydrogen Energy 2020, 45, 14500–14516. [Google Scholar] [CrossRef]
- Wang, X.; Xu, S.; Xing, C. Numerical and experimental investigation on an ejector designed for an 80 kW polymer electrolyte membrane fuel cell stack. J. Power Sources 2019, 415, 25–32. [Google Scholar] [CrossRef]



| Power | Operation Nozzle | Operation Mode |
|---|---|---|
| 10% Pe | N1 | 1 |
| 20% Pe | N2 (or N3) | 2 |
| 30% Pe | N1 and N2 (or N3) | 3 |
| 40% Pe | N2 and N3 | 4 |
| 50% Pe | N4 | 5 |
| 60% Pe | N4 and N1 | 6 |
| 70% Pe | N4 and N2 (or N3) | 7 |
| 80% Pe | N4, N2 (or N3) and N1 | 8 |
| 90% Pe | N4, N2 and N3 | 9 |
| 100% Pe | N1, N2, N3 and N4 | 10 |
| Nozzles | Values |
|---|---|
| First nozzle diameter | 1 mm |
| Second nozzle diameter | 1.2 mm |
| Third nozzle diameter | 1.2 mm |
| Fourth nozzle diameter | 2.4 mm |
| Parameters | Values |
|---|---|
| Suction chamber length | 37 mm |
| Nozzle length | 40 mm |
| Constant-pressure mixing chamber convergence angle | 21° |
| Constant-pressure mixing chamber length | 5 mm |
| Constant-area mixing chamber diameter | 8 mm |
| Constant-area mixing chamber length | 40 mm |
| Diffusion chamber length | 80 mm |
| Diffusion chamber divergence angle | 4° |
| Diffusion chamber outlet diameter | 19.2 mm |
| Power (kW) | Current (A) | Mass Flow Rate of the Hydrogen (g s−1) | Fuel Cell Entry Pressure (kPa) |
|---|---|---|---|
| 170 | 495 | 2.84 | 270 |
| 153 | 445 | 2.44 | 270 |
| 136 | 396 | 2.16 | 270 |
| 102 | 297 | 1.63 | 260 |
| 85 | 247 | 1.36 | 247 |
| 68 | 198 | 1.08 | 228 |
| 51 | 148 | 0.81 | 205 |
| 34 | 99 | 0.54 | 177 |
| Grid | ) | ) | ||||
|---|---|---|---|---|---|---|
| 785,242 | 1.195 | - | 2.312 | - | 1.935 | - |
| 1,245,316 | 1.229 | 2.81 | 2.441 | 5.54 | 1.986 | 2.64 |
| 1,613,454 | 1.221 | 0.65 | 2.482 | 1.64 | 2.033 | 2.36 |
| Grid | ) | ) | ||||
|---|---|---|---|---|---|---|
| 785,242 | 1.738 | - | 3.497 | - | 2.012 | - |
| 1,245,316 | 1.711 | 1.55 | 3.751 | 7.26 | 2.192 | 8.95 |
| 1,613,454 | 1.705 | 0.35 | 3.678 | 1.95 | 2.157 | 1.61 |
| Fuel Cell Output Power (kW) | Four-Nozzle Ejector Operation Modes | ) | Traditional Ejector Throat Diameter (mm) |
|---|---|---|---|
| 20% Pe | 2 | 0.56 | 1.4 |
| 30% Pe | 3 | 0.81 | 1.7 |
| 40% Pe | 4 | 1.10 | 1.9 |
| 50% Pe | 5 | 1.38 | 2.0 |
| 60% Pe | 6 | 1.66 | 2.2 |
| 70% Pe | 7 | 1.93 | 2.4 |
| 80% Pe | 8 | 2.25 | 2.5 |
| 90% Pe | 9 | 2.49 | 2.7 |
| 100% Pe | 10 | 2.78 | 2.8 |
| Plans | Plan 1 | Plan 2 | Plan 3 | Plan 4 | Plan 5 | Plan 6 | Plan 7 | |
|---|---|---|---|---|---|---|---|---|
| Modes | ||||||||
| 3 | 1.44 | 1.25 | 1.24 | 1.22 | 1.24 | 1.28 | 2.59 | |
| 4 | 1.99 | 1.72 | 1.74 | 1.68 | 2.09 | 1.57 | 2.54 | |
| 5 | 2.16 | 2.02 | 0.96 | 0.96 | 1.98 | 1.97 | 1.62 | |
| 6 | 2.19 | 2.1 | 1.63 | 1.63 | 1.76 | 1.78 | 1.52 | |
| 7 | 1.88 | 1.83 | 1.68 | 1.67 | 1.56 | 1.53 | 1.34 | |
| 8 | 1.76 | 1.7 | 1.76 | 1.76 | 1.37 | 1.37 | 1.21 | |
| 9 | 1.53 | 1.53 | 1.83 | 1.8 | 1.2 | 1.2 | 1.07 | |
| 10 | 1.37 | 1.36 | 1.67 | 1.61 | 1.07 | 1.07 | 0.96 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yi, A.; Wang, C.; Wang, L.; Wang, X. A Wide Operating Range Ejector with Part Nested Nozzles for PEMFC Hydrogen Recirculation System. Inventions 2023, 8, 133. https://doi.org/10.3390/inventions8060133
Yi A, Wang C, Wang L, Wang X. A Wide Operating Range Ejector with Part Nested Nozzles for PEMFC Hydrogen Recirculation System. Inventions. 2023; 8(6):133. https://doi.org/10.3390/inventions8060133
Chicago/Turabian StyleYi, Anning, Chen Wang, Lei Wang, and Xinli Wang. 2023. "A Wide Operating Range Ejector with Part Nested Nozzles for PEMFC Hydrogen Recirculation System" Inventions 8, no. 6: 133. https://doi.org/10.3390/inventions8060133
APA StyleYi, A., Wang, C., Wang, L., & Wang, X. (2023). A Wide Operating Range Ejector with Part Nested Nozzles for PEMFC Hydrogen Recirculation System. Inventions, 8(6), 133. https://doi.org/10.3390/inventions8060133









