Robust Control and Active Vibration Suppression in Dynamics of Smart Systems
Abstract
:1. Introduction
2. Materials and Methods
2.1. Frequency Domain
2.2. Design Objectives
- Small control effort.
- Attenuation of disturbances with acceptable transient characteristics (overshoot, settling time).
- Strength of closed loop system (plant + controller).
- 4.
- The above criteria (1)–(3) should be satisfied even when noise exists in the modeling procedure.
2.3. System Specifications
- I.
- If M is internally stable, the system is presumably stable;
- II.
- If the system performs about average;
- III.
- If and only if, the system (M, Δ) is robustly stable,
- IV.
- The system (N, Δ) exhibits robust performance if and only if,
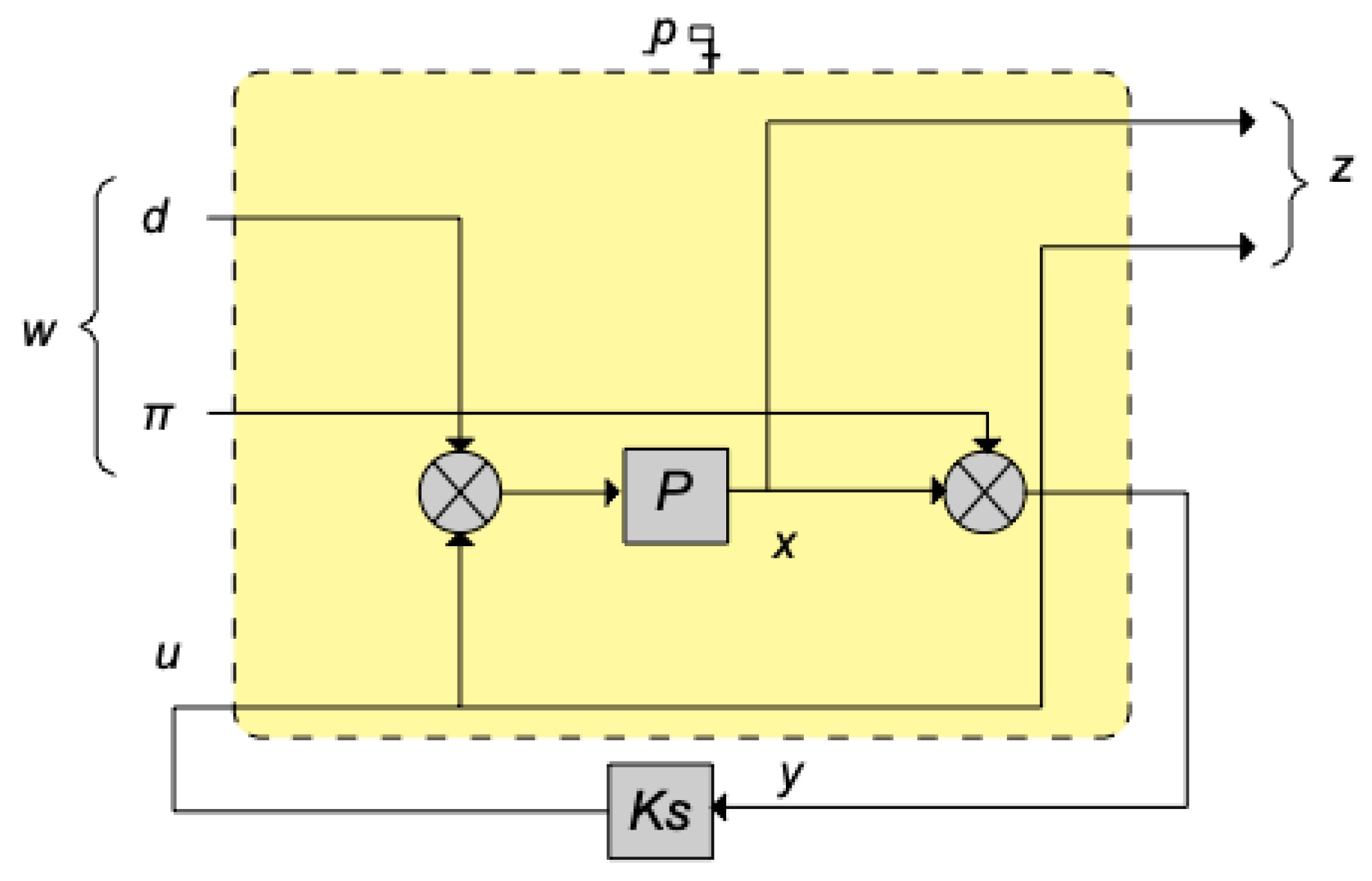
2.4. Controller Synthesis
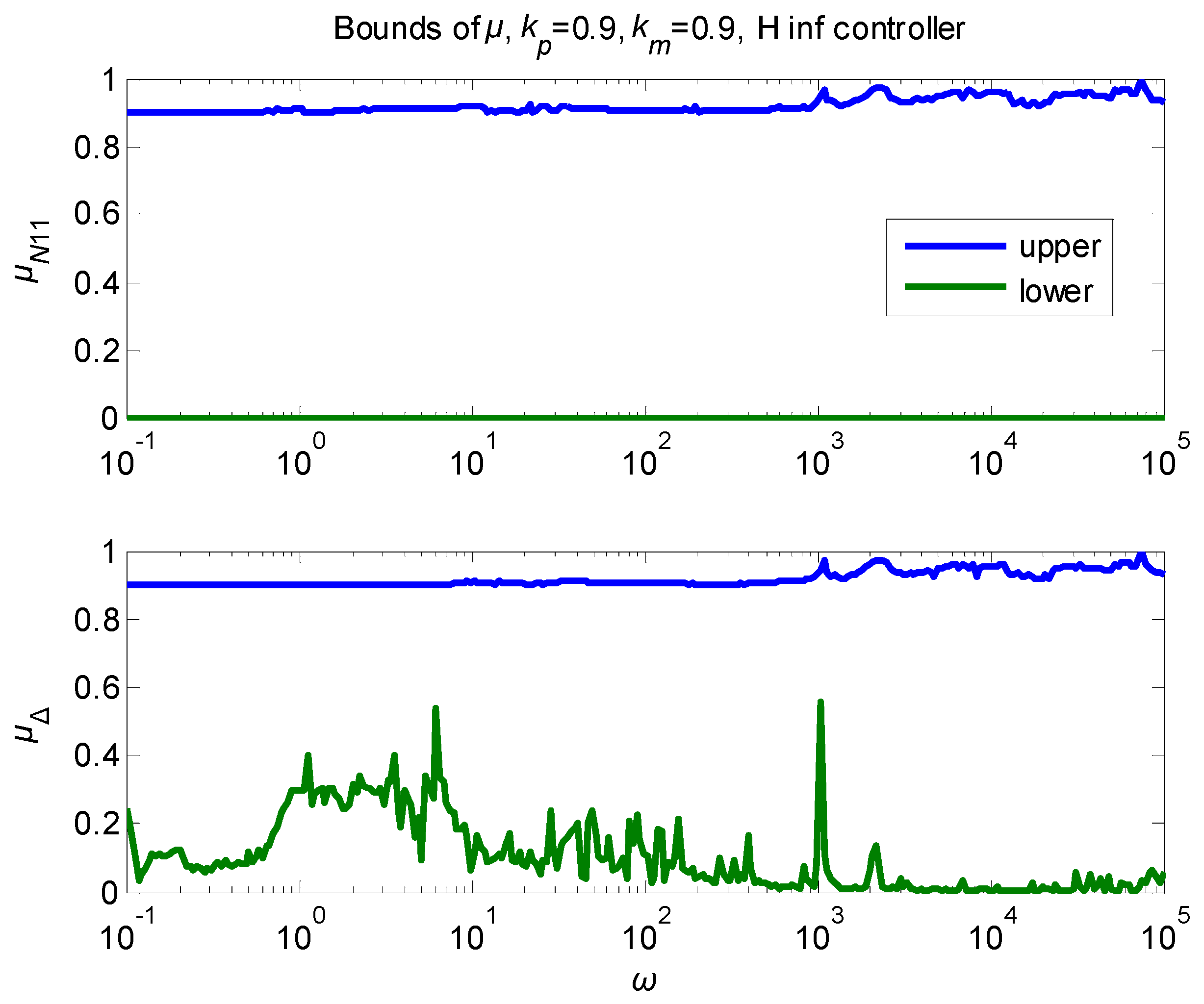
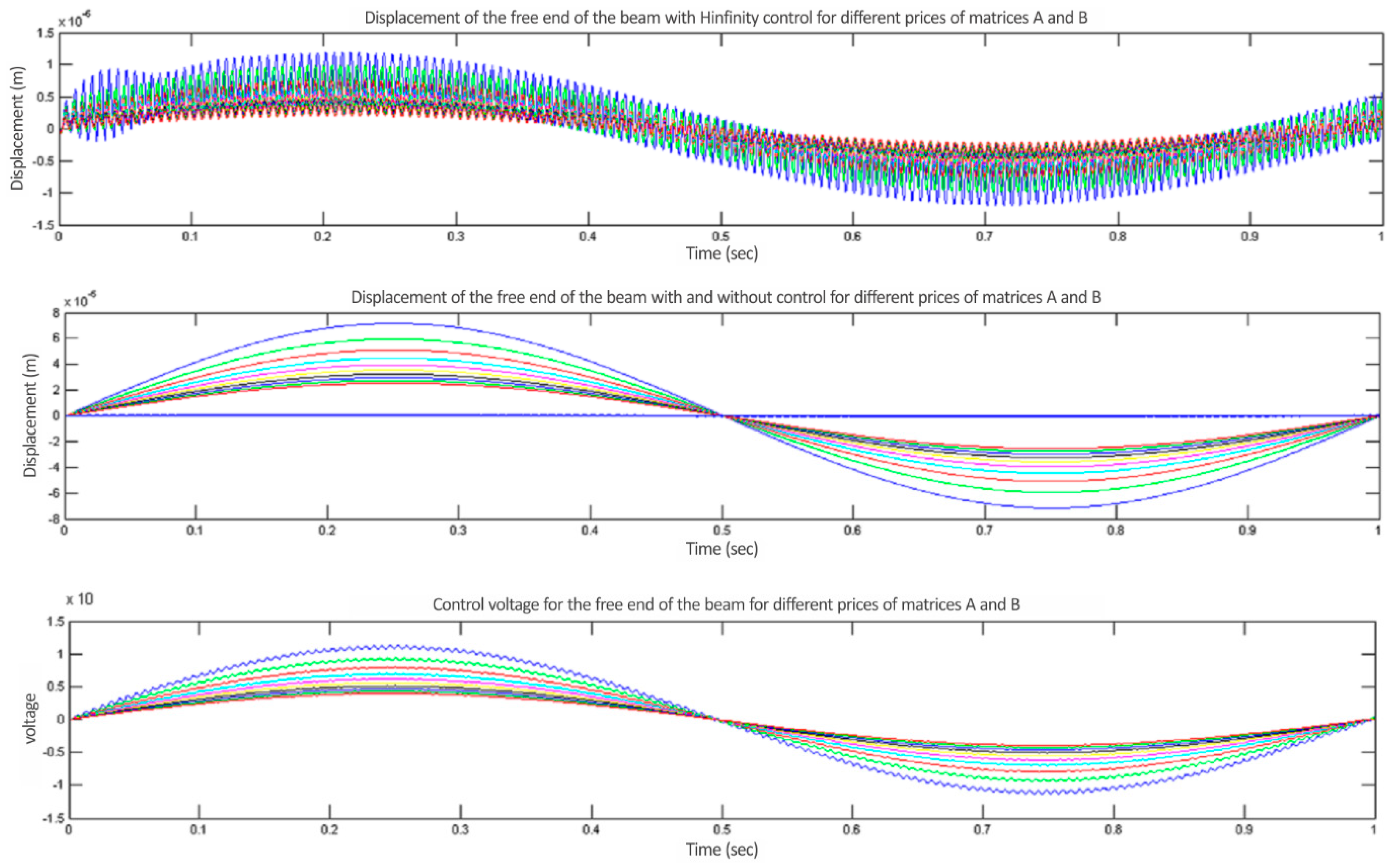
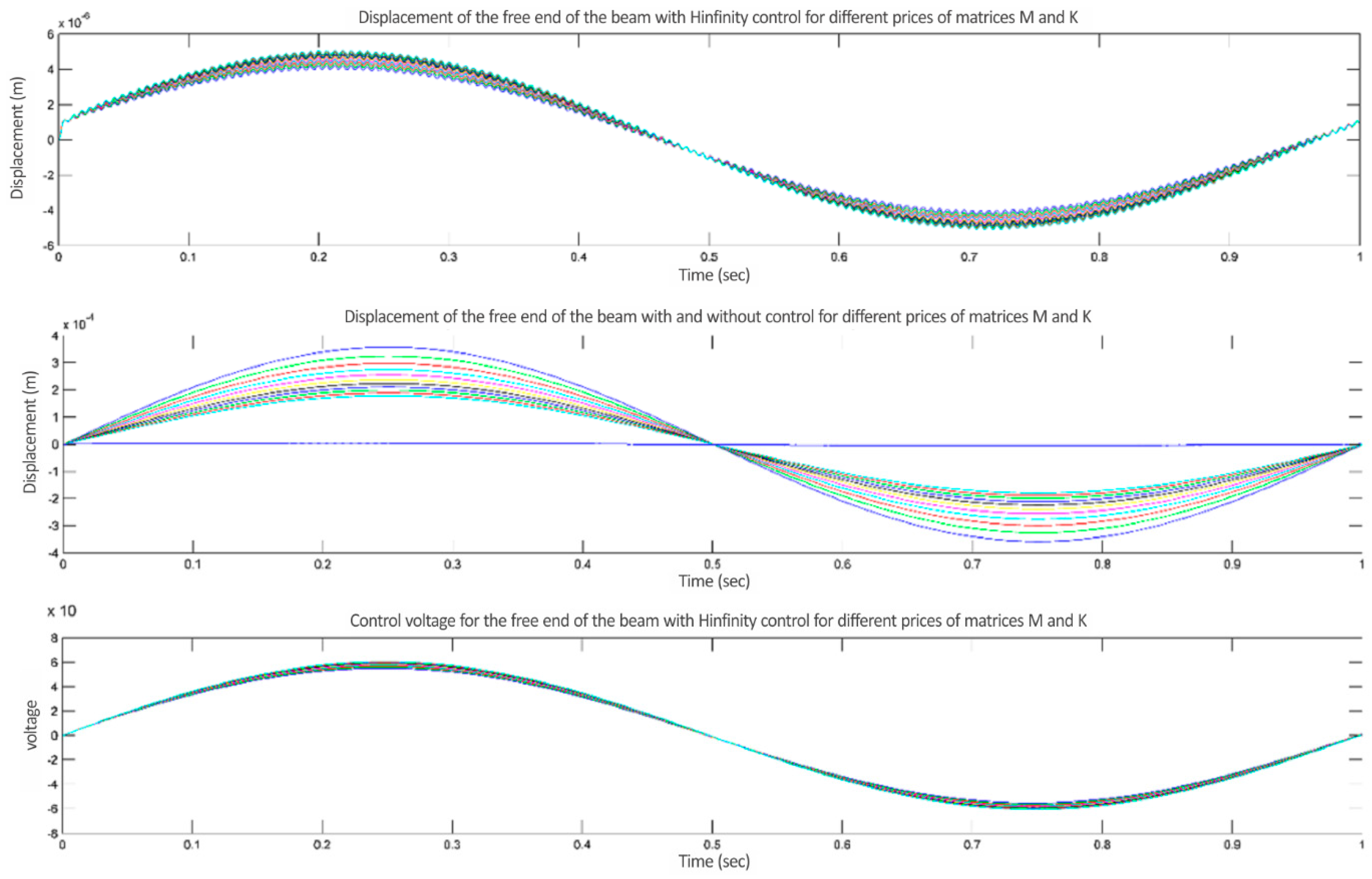
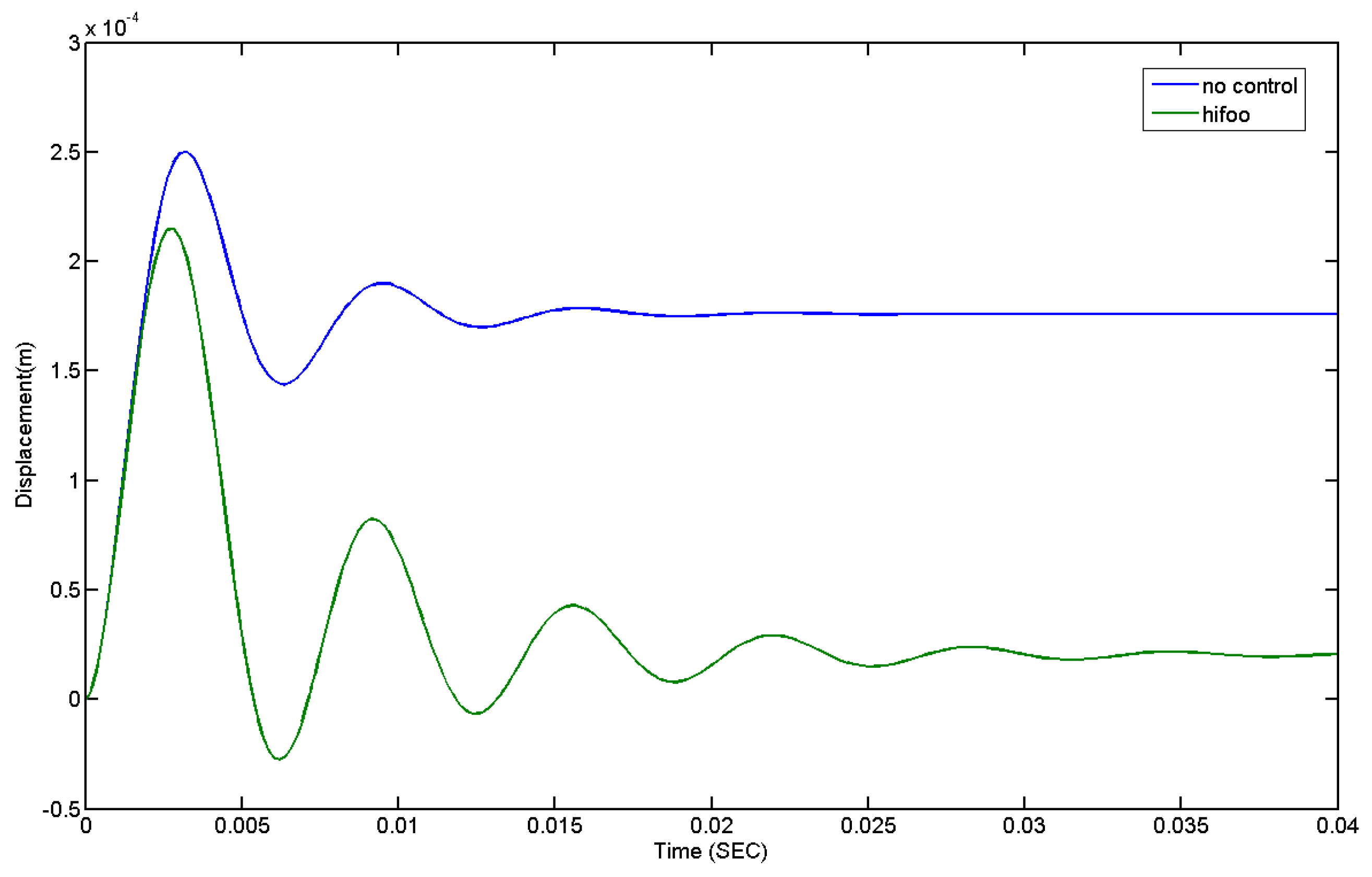
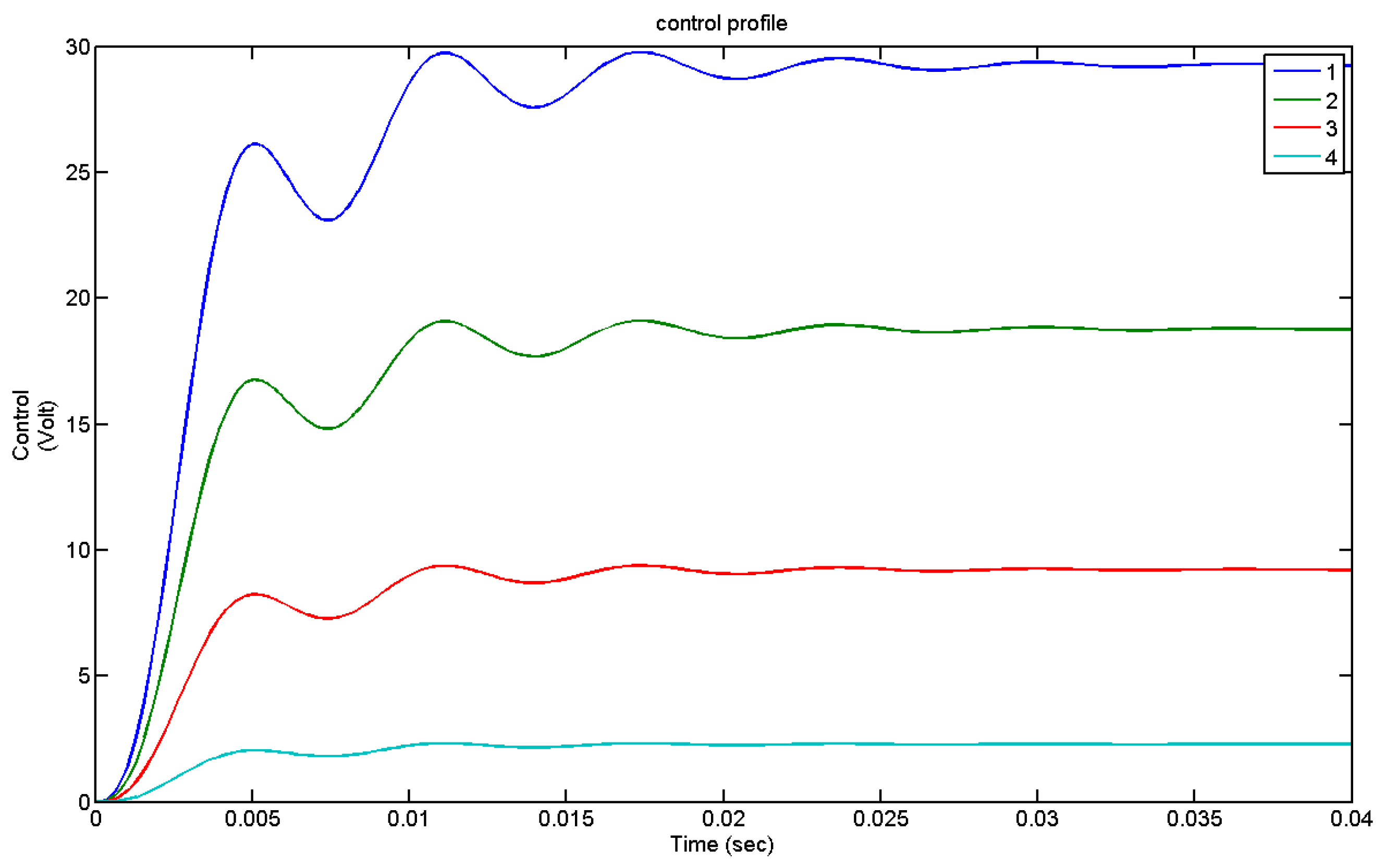
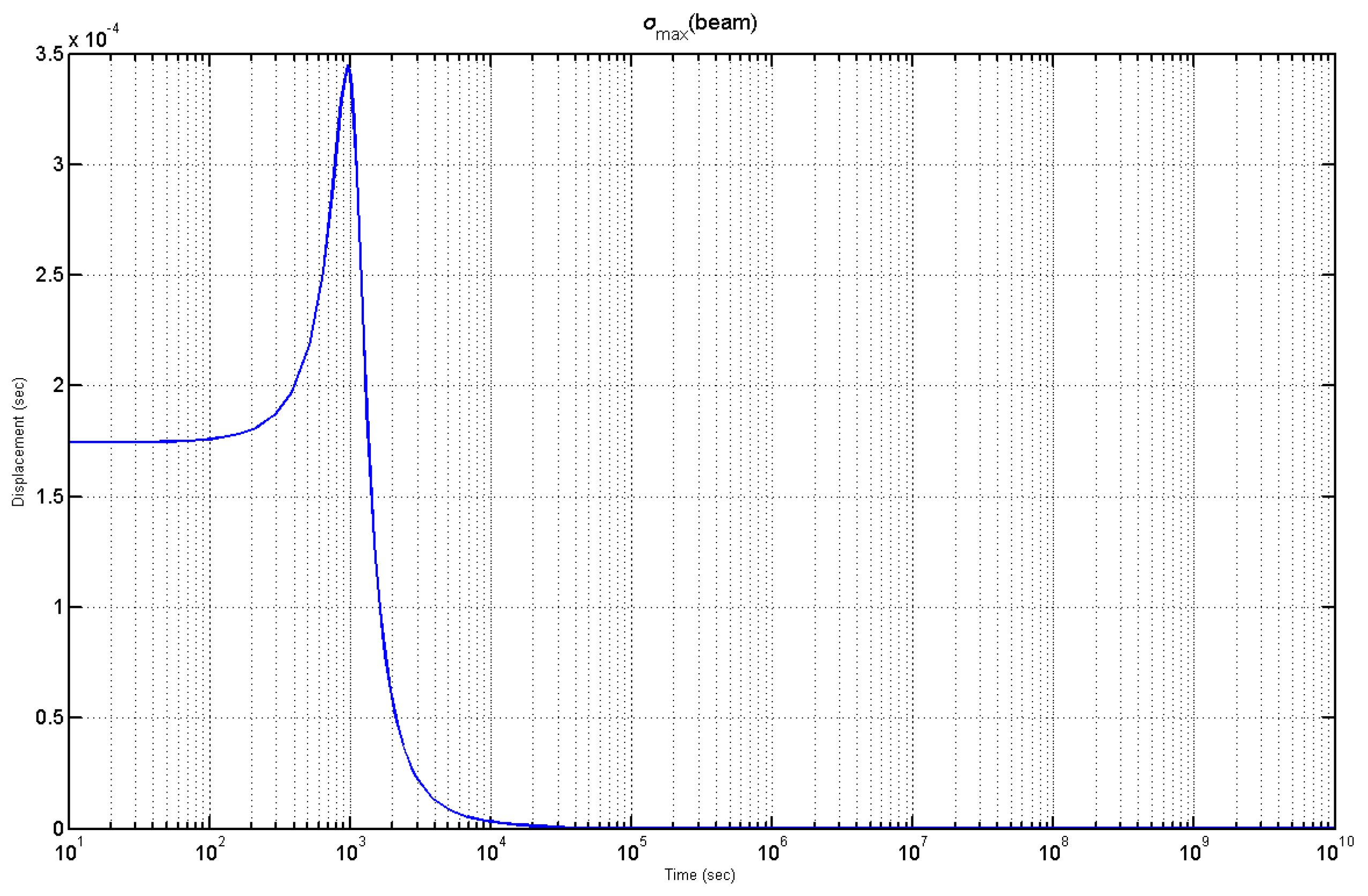
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bandyopadhyay, B.; Manjunath, T.C.; Unapathy, M. Modeling, Control, and Implementation of Smart Structures; Springer: Berlin, Heidelberg, Germany, 2007; ISBN 10 3-540-48393-4. [Google Scholar]
- Burke, J.V.; Henron, D.; Kewis, A.S.; Overton, M.L. Stabilization via Nonsmooth, Non-convex Optimization. IEEE Trans. Autom. Control 2006, 5, 1760–1769. [Google Scholar]
- Doyle, J.C.; Glover, K.; Khargoneker, P.; Francis, B. State space solutions to standard H2 and H∞ control problems. In Proceedings of the 1988 American Control Conference, Atlanta, GA, USA, 15–17 June 1988; Volume 34, pp. 831–847. [Google Scholar]
- Francis, B.A. A Course on H∞ Control Theory; Springer: Berlin, Heidelberg, Germany, 1987. [Google Scholar]
- Friedman, J.; Kosmatka, K. An improved two node Timoshenko beam finite element. J. Comput. Struct. 1993, 47, 473–481. [Google Scholar]
- Kimura, H. Robust stability for a class of transfer functions. IEEE Trans. Autom. Control 1984, 29, 788–793. [Google Scholar]
- Miara, B.; Stavroulakis, G.; Valente, V. Topics on Mathematics for Smart Systems, Proceedings of the European Conference, Rome, Italy, 26–28 October 2006; World Scientific Publishers: Singapore, 2007. [Google Scholar]
- Moutsopoulou, A.; Pouliezos, A.; Stavroulakis, G.E. Modelling with Uncertainty and Robust Control of Smart Beams. Paper 35, Proceedings of the Ninth International Conference on Computational Structures Technology, Athens, Greece, 2–5 September 2008; Topping, B.H.V., Papa-drakakis, M., Eds.; Civil Comp Press: Edinburgh, UK, 2008. [Google Scholar]
- Yang, S.M.; Lee, Y.J. Optimization of non collocated sensor, actuator location and feedback gain and control systems. Smart Mater. Struct. J. 1993, 8, 96–102. [Google Scholar]
- Chandrashekara, K.; Varadarajan, S. Adaptive shape control of composite beams with piezoelectric actuators. Intell. Mater. Syst. Struct. 1997, 8, 112–124. [Google Scholar]
- Lim, Y.H.; Gopinathan, V.S.; Varadhan, V.V.; Varadan, K.V. Finite element simulation of smart structures using an optimal output feedback controller for vibration and noise control. Int. J. Smart Mater. Struct. 1999, 8, 324–337. [Google Scholar]
- Zhang, N.; Kirpitchenko, I. Modelling dynamics of a continuous structure with a piezoelectric sensor/actuator for passive structural control. J. Sound Vib. 2002, 249, 251–261. [Google Scholar]
- Zhang, X.; Shao, C.; Li, S.; Xu, D. Robust H∞ vibration control for flexible linkage mechanism systems with piezoelectric sensors and actuators. J. Sound Vib. 2001, 243, 145–155. [Google Scholar] [CrossRef]
- Kwakernaak, H. Robust control and H∞ optimization. Tutor. Hper JFAC Autom. 1993, 29, 255–273. [Google Scholar]
- Stavroulakis, G.E.; Foutsitzi, G.; Hadjigeorgiou, E.; Marinova, D.; Baniotopoulos, C.C. Design and robust optimal control of smart beams with application on vibrations suppression. Adv. Eng. Softw. 2005, 36, 806–813. [Google Scholar] [CrossRef]
- Packard, A.; Doyle, J.; Balas, G. Linear, multivariable robust control with a μ perspective. ASME J. Dyn. Syst. Meas. Control 50th Anniv. Issue 1993, 115, 310–319. [Google Scholar] [CrossRef]
- Tiersten, H.F. Linear Piezoelectric Plate Vibrations; Plenum Press: New York, NY, USA, 1969. [Google Scholar]
- Zames, G. Feedback minimax sensitivity and optimal robustness. IEEE Trans. Autom. Control 1993, 28, 585–601. [Google Scholar] [CrossRef]
- Chen, B.M. Robust and Hinf Control; Springer: London, UK, 2000. [Google Scholar]
- Iorga, L.; Baruh, H.; Ursu, I. A Review of H∞ Robust Control of Piezoelectric Smart Structures. ASME. Appl. Mech. Rev. 2008, 61, 040802. [Google Scholar] [CrossRef]
- Millston, M. HIFOO 1.5: Structured Control of Linear Systems with a Non-Trivial Feedthrough. Master’s Thesis, Courant Institute of Mathematical Sciences, New York University, New York, NY, USA, 2006. [Google Scholar]
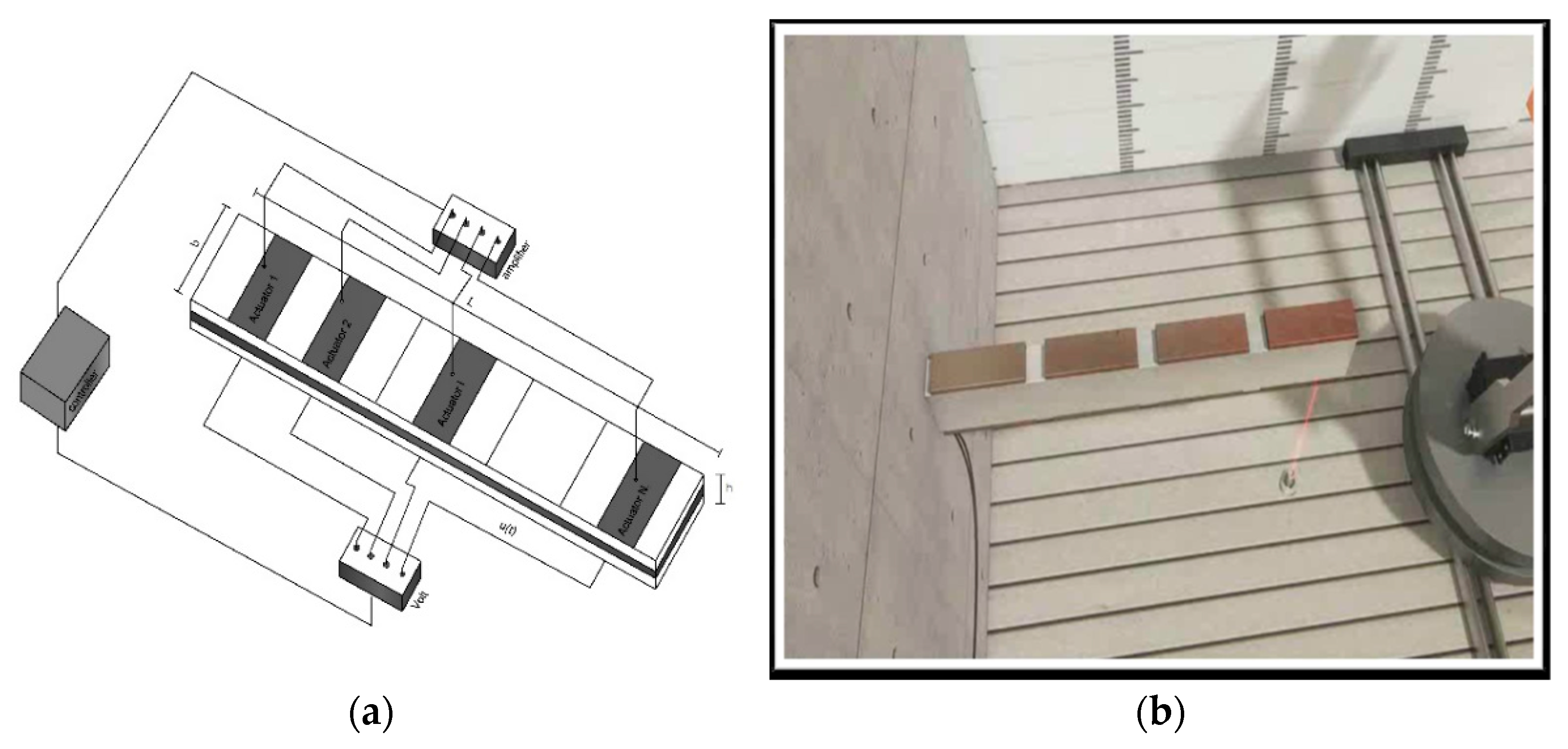



| Parameters | Values |
|---|---|
| Beam length, L | 0.8 m |
| Beam width, W | 0.07 m |
| Beam thickness, h | 0.0095 m |
| Beam density, ρ | 1600 kg/m3 |
| Young’s modulus of the beam, E | 1.5 × 1011 N/m2 |
| Piezoelectric constant, d31 | 254 × 10−12 m/V |
| Nominal stability (NS) ⇔ | N internally stable |
| Nominal performance (NP) ⇔ | ║N22(jω)║∞ < 1, ∀ω and NS |
| Robust stability (RS) ⇔ | F = Φu(N, Δ) stable ∀Δ, ║Δ║∞ < 1 and NS |
| Robust performance (RP) ⇔ | ║F║∞ < 1, ∀Δ, ║Δ║∞ < 1 and NS |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Moutsopoulou, A.; Stavroulakis, G.E.; Pouliezos, A.; Petousis, M.; Vidakis, N. Robust Control and Active Vibration Suppression in Dynamics of Smart Systems. Inventions 2023, 8, 47. https://doi.org/10.3390/inventions8010047
Moutsopoulou A, Stavroulakis GE, Pouliezos A, Petousis M, Vidakis N. Robust Control and Active Vibration Suppression in Dynamics of Smart Systems. Inventions. 2023; 8(1):47. https://doi.org/10.3390/inventions8010047
Chicago/Turabian StyleMoutsopoulou, Amalia, Georgios E. Stavroulakis, Anastasios Pouliezos, Markos Petousis, and Nectarios Vidakis. 2023. "Robust Control and Active Vibration Suppression in Dynamics of Smart Systems" Inventions 8, no. 1: 47. https://doi.org/10.3390/inventions8010047
APA StyleMoutsopoulou, A., Stavroulakis, G. E., Pouliezos, A., Petousis, M., & Vidakis, N. (2023). Robust Control and Active Vibration Suppression in Dynamics of Smart Systems. Inventions, 8(1), 47. https://doi.org/10.3390/inventions8010047







