Hidden Dynamics Investigation, Fast Adaptive Synchronization, and Chaos-Based Secure Communication Scheme of a New 3D Fractional-Order Chaotic System
Abstract
:1. Introduction
2. Fractional Calculus Fundamentals
3. New 3D Fractional-Order Chaotic System
3.1. Case1: Hidden Attractors
3.2. Case2: Self-Excited Attractor
4. Dynamical Behavior of New System
4.1. Lyapunov Exponents
4.2. Bifurcation Diagrams
5. Synchronization Strategy
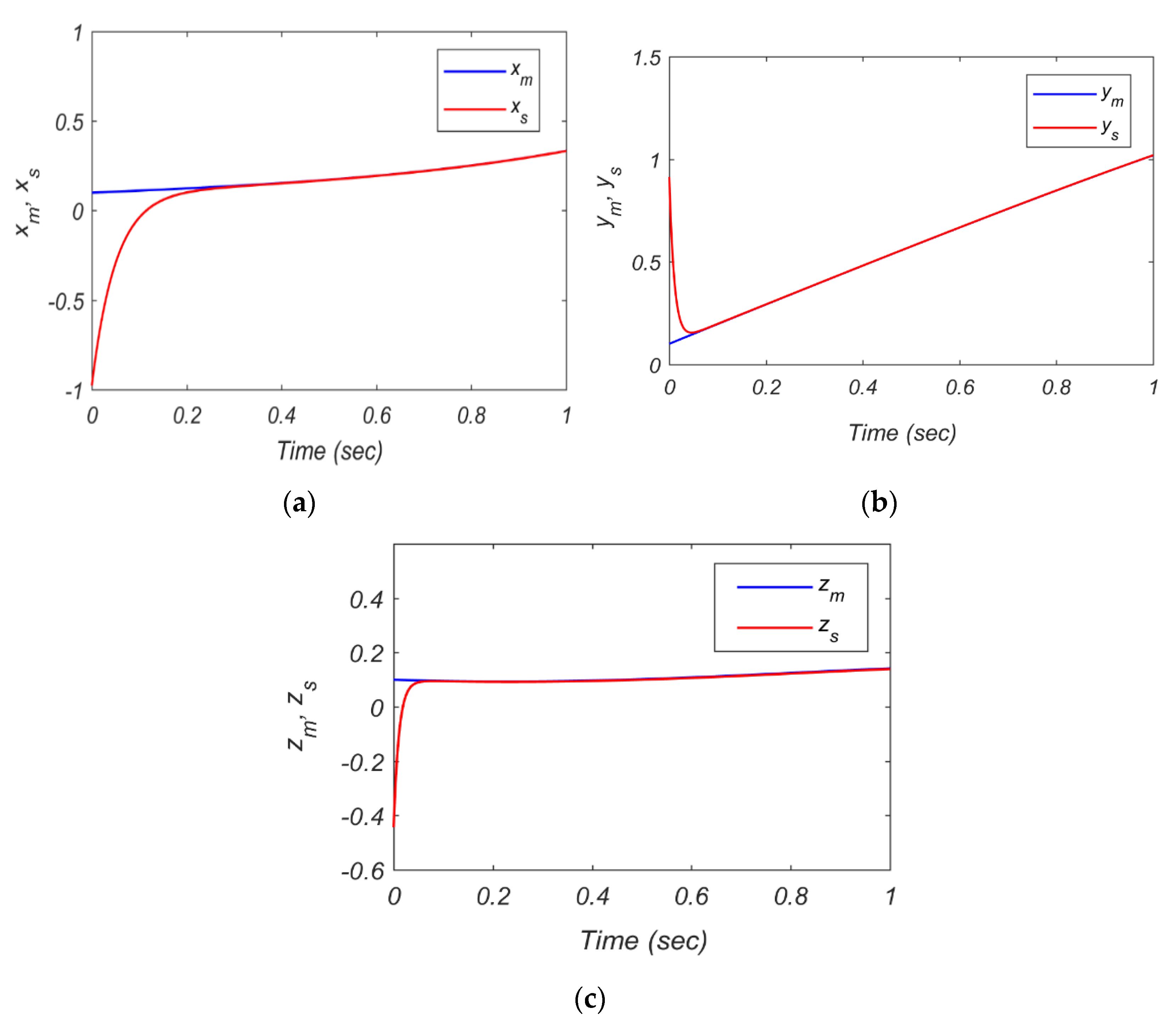
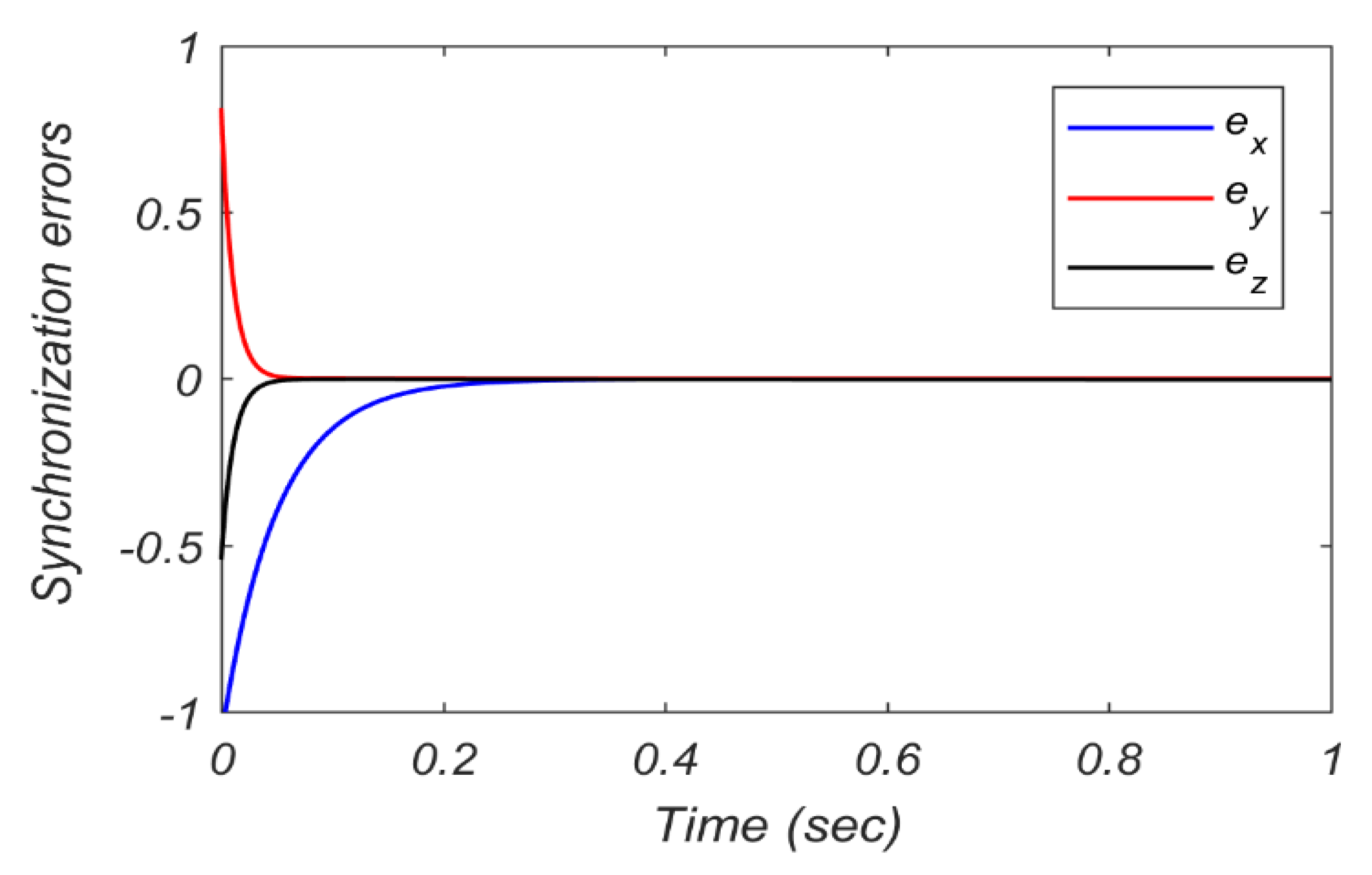
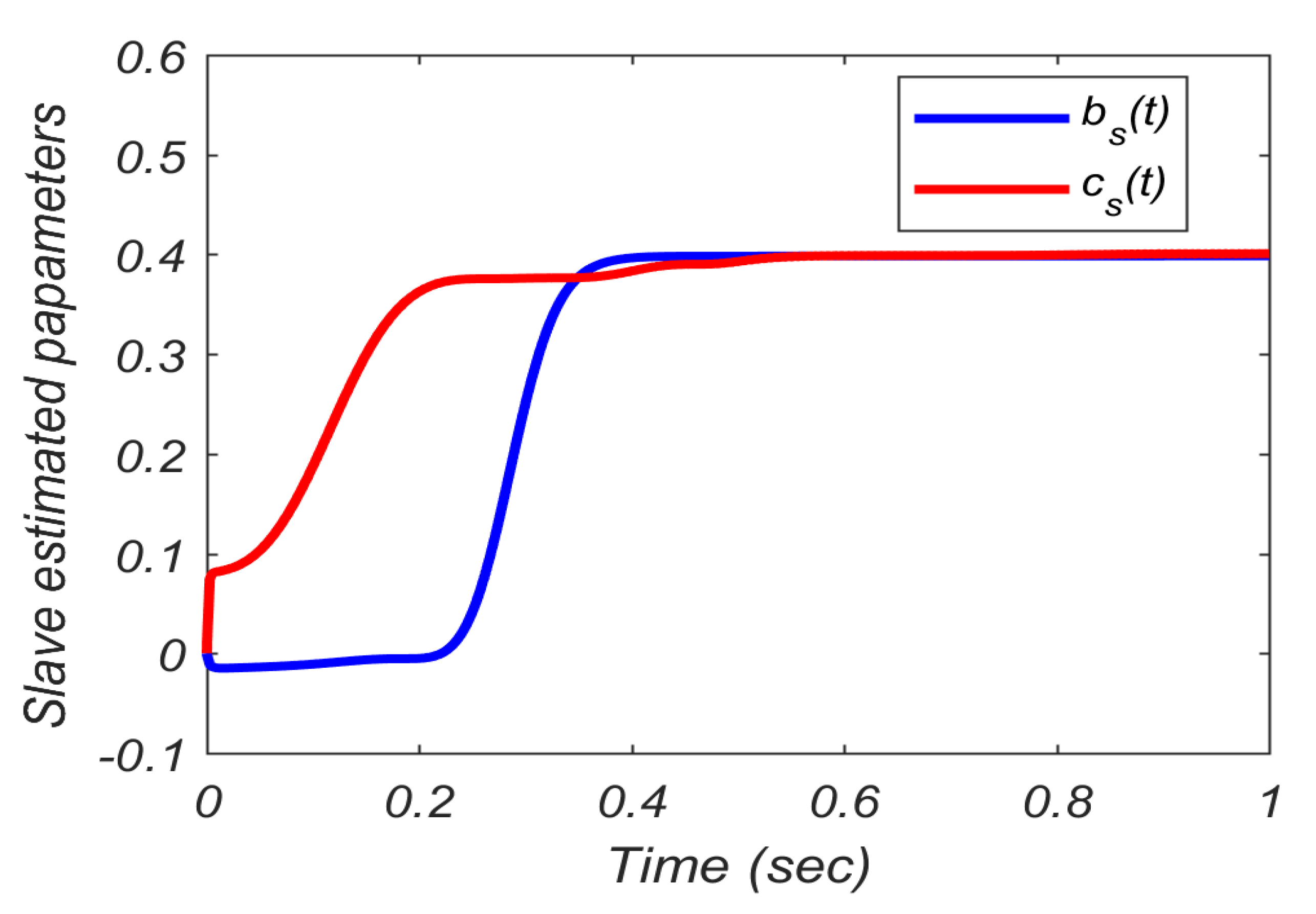
5.1. Fast Adaptive Controller Design
5.2. Numerical Simulation Results
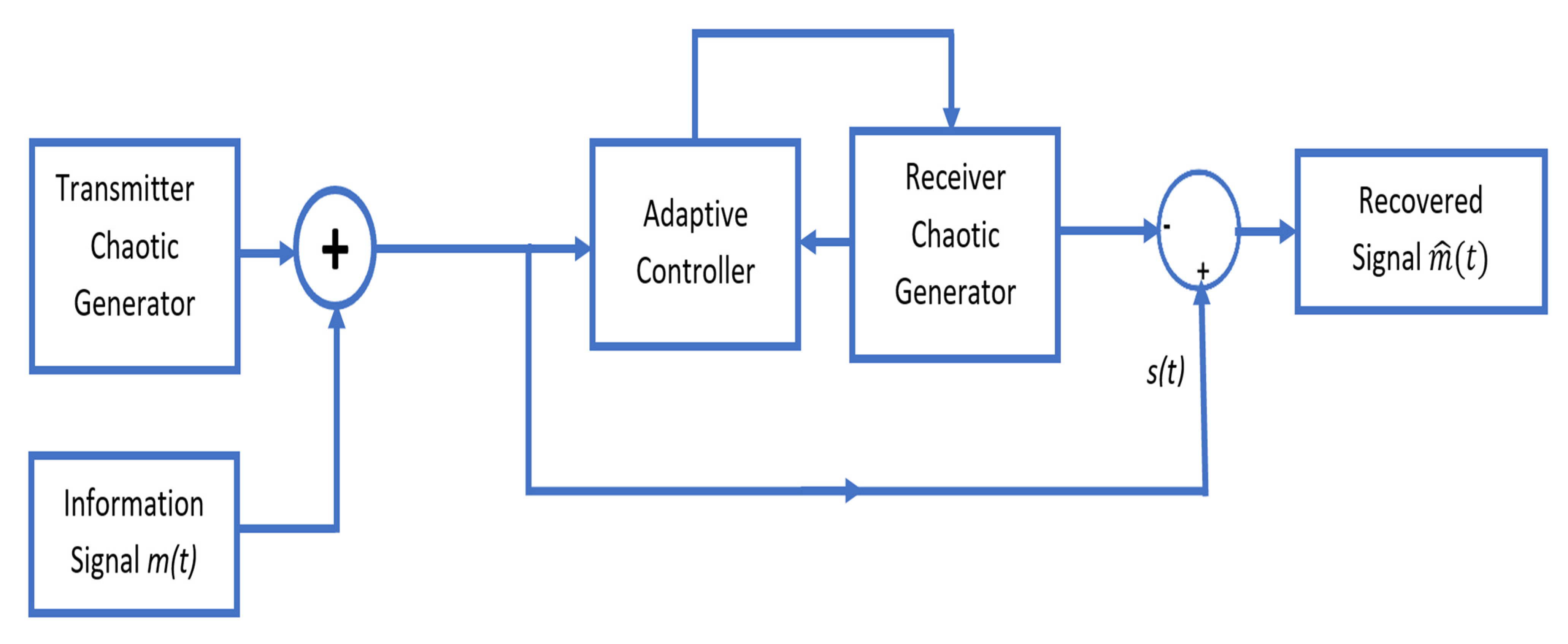
6. Chaos-Based Secure Communication System
7. Communication System’s Security Analysis
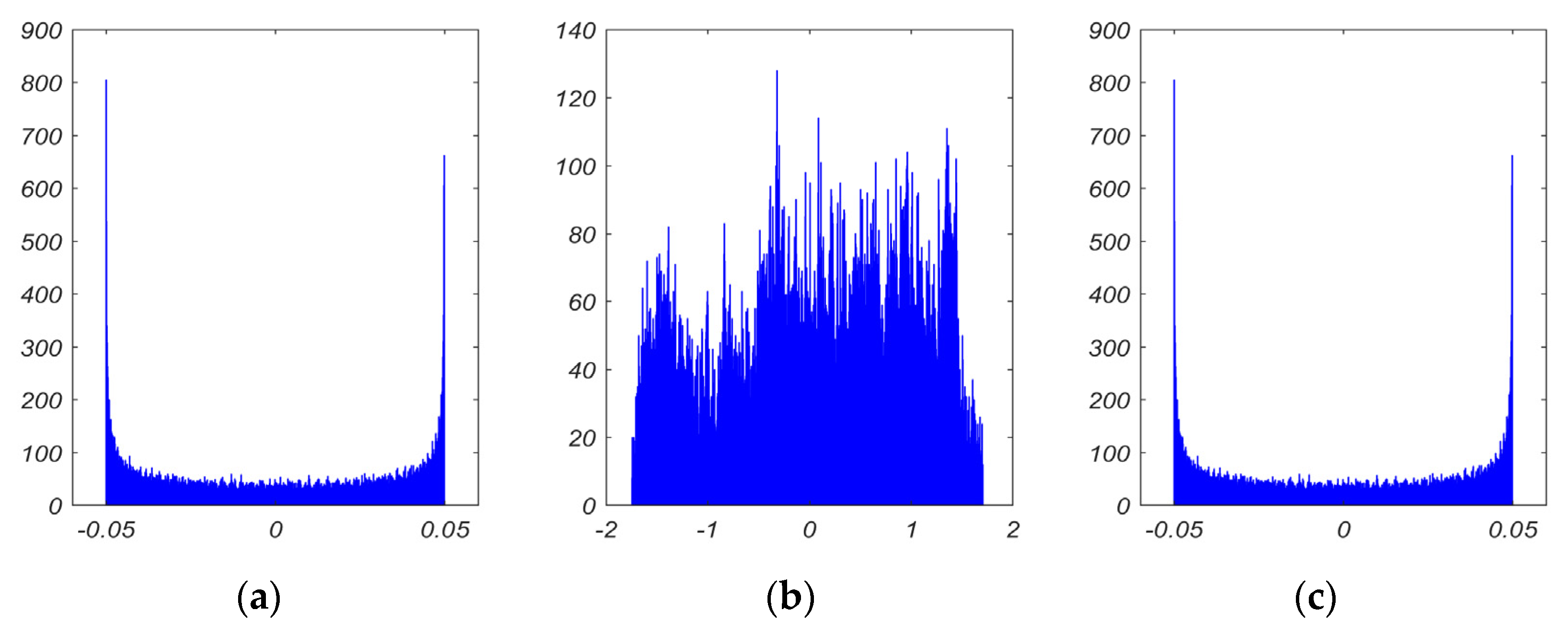
7.1. Histogram Analysis
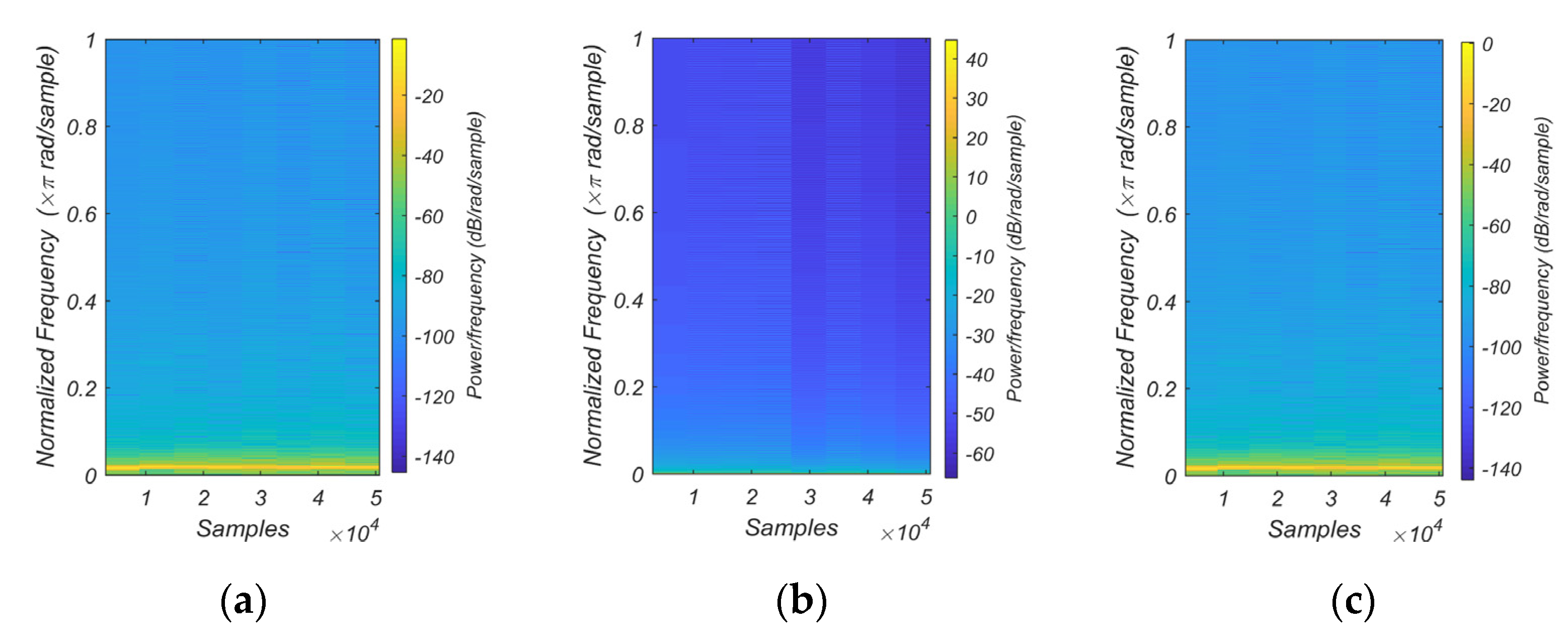
7.2. Spectrogram Analysis
8. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Brady, P. Chaos Theory, Control Theory, and Literary Theory or: A Story of Three Butterflies. Mod. Lang. Stud. 1990, 20, 65. [Google Scholar] [CrossRef]
- Rahman, Z.-A.S.A.; Jasim, B.H.; Al-Yasir, Y.I.A.; Abd-Alhameed, R.A. Efficient Colour Image Encryption Algorithm Using a New Fractional-Order Memcapacitive Hyperchaotic System. Electronics 2022, 11, 1505. [Google Scholar] [CrossRef]
- Solano, J.; Balibrea, F.; Moreno, J. Applications of the Network Simulation Method to Differential Equations with Singularities and Chaotic Behaviour. Mathematics 2021, 9, 1442. [Google Scholar] [CrossRef]
- Leonov, G.A.; Kuznetsov, N.V. Hidden attractors in dynamical systems. From hidden oscillations in Hilbert–Kolmogorov, Aizerman, and Kalman problems to hidden chaotic attractor in Chua circuits. Int. J. Bifurc. Chaos 2013, 23, 1330002. [Google Scholar] [CrossRef] [Green Version]
- Jasim, B.H.; Hassan, K.H.; Omran, K.M. A new 4-D hyperchaotic hidden attractor system: Its dynamics, coexisting attractors, synchronization and microcontroller implementation. Int. J. Electr. Comput. Eng. (IJECE) 2021, 11, 2068–2078. [Google Scholar] [CrossRef]
- Jafari, S.; Sprott, J.C.; Nazarimehr, F. Recent new examples of hidden attractors. Eur. Phys. J. Spéc. Top. 2015, 224, 1469–1476. [Google Scholar] [CrossRef]
- Kiseleva, M.; Kuznetsov, N.; Leonov, G.; Neittaanmäki, P. Hidden oscillations in drilling system actuated by induction motor. IFAC Proc Vol 2013, 46, 86–89. [Google Scholar] [CrossRef]
- Zhang, S.; Zeng, Y.C.; Li, Z.J. A Novel Four-Dimensional No-Equilibrium Hyper-Chaotic System With Grid Multiwing Hyper-Chaotic Hidden Attractors. J. Comput. Nonlinear Dyn. 2018, 13, 090908. [Google Scholar] [CrossRef]
- Vaidyanathan, S. Global chaos synchronization of chemical chaotic reactors via novel sliding mode control method. Parameters 2015, 1, 4. [Google Scholar]
- Bi, H.; Qi, G.; Hu, J.; Faradja, P.; Chen, G. Hidden and transient chaotic attractors in the attitude system of quadrotor unmanned aerial vehicle. Chaos Solitons Fractals 2020, 138, 109815. [Google Scholar] [CrossRef]
- Yu, J.; Li, C.; Song, X.; Guo, S.; Wang, E. Parallel Mixed Image Encryption and Extraction Algorithm Based on Compressed Sensing. Entropy 2021, 23, 278. [Google Scholar] [CrossRef] [PubMed]
- Kuznetsov, N.V.; Leonov, G.A.; Yuldashev, M.V.; Yuldashev, R.V. Hidden attractors in dynamical models of phase-locked loop circuits: Limitations of simulation in MATLAB and SPICE. Commun. Nonlinear. Sci. Numer. Simul. 2017, 51, 39–49. [Google Scholar]
- Lorenz, E.N.; Haman, K. The essence of chaos. Pure Appl. Geophys. 1996, 147, 598–599. [Google Scholar]
- Pham, V.-T.; Vaidyanathan, S.; Volos, C.; Tlelo-Cuautle, E.; Tahir, F.R. A memristive system with hidden attractors and its engineering application. In Advances in Memristors, Memristive Devices and Systems; Springer: New York, NY, USA, 2017; pp. 81–99. [Google Scholar]
- Pisarchik, A.; Jaimes-Reátegui, R.; Rodríguez-Flores, C.; García-López, J.; Huerta-Cuellar, G.; Martín-Pasquín, F. Secure chaotic communication based on extreme multistability. J. Frankl. Inst. 2021, 358, 2561–2575. [Google Scholar] [CrossRef]
- Vanchinathan, K.; Valluvan, K.R.; Gnanavel, C.; Gokul, C. Design methodology and experimental verification of intelligent speed controllers for sensorless permanent magnet Brushless DC motor: Intelligent speed controllers for electric motor. Electr. Energy Syst. 2021, 31, e12991. [Google Scholar] [CrossRef]
- Kamal, F.M.; Elsonbaty, A.; Elsaid, A. A novel fractional nonautonomous chaotic circuit model and its application to image encryption. Chaos Solitons Fractals 2021, 144, 110686. [Google Scholar] [CrossRef]
- Rahman, Z.-A.S.A.; Jasim, B.H.; Al-Yasir, Y.I.A.; Abd-Alhameed, R.A. High-Security Image Encryption Based on a Novel Simple Fractional-Order Memristive Chaotic System with a Single Unstable Equilibrium Point. Electronics 2021, 10, 3130. [Google Scholar] [CrossRef]
- Lin, L.; Wang, Q.; He, B.; Chen, Y.; Peng, X.; Mei, R. Adaptive Predefined-Time Synchronization of Two Different Fractional-Order Chaotic Systems With Time-Delay. IEEE Access 2021, 9, 31908–31920. [Google Scholar] [CrossRef]
- Razminia, A.; Majd, V.J.; Baleanu, D. Chaotic incommensurate fractional order Rössler system: Active control and synchronization. Adv. Differ. Equ. 2011, 2011, 15. [Google Scholar] [CrossRef] [Green Version]
- Kayalvizhi, S.; Malarvizhi, S. A novel encrypted compressive sensing of images based on fractional order hyper chaotic Chen system and DNA operations. Multimed. Tools Appl. 2020, 79, 3957–3974. [Google Scholar] [CrossRef]
- Ma, C.; Mou, J.; Liu, J.; Yang, F.; Yan, H.; Zhao, X. Coexistence of multiple attractors for an incommensurate fractional-order chaotic system. Eur. Phys. J. Plus 2020, 135, 1–21. [Google Scholar] [CrossRef]
- Gupta, S.; Varshney, P.; Srivastava, S. Whale optimization based synchronization and control of two identical fractional order financial chaotic systems. J. Intell. Fuzzy Syst. 2022, 42, 929–942. [Google Scholar] [CrossRef]
- Khan, H.; Li, Y.; Khan, A.; Khan, A. Existence of solution for a fractional-order Lotka-Volterra reaction-diffusion model with Mittag-Leffler kernel. Math. Methods Appl. Sci. 2019, 42, 3377–3387. [Google Scholar] [CrossRef]
- Tepljakov, A. Fractional-Order Modeling and Control of Dynamic Systems; Springer: New York, NY, USA, 2017. [Google Scholar]
- Rahman, Z.; AL-Kashoash, H.; Ramadhan, S.; Al-Yasir, Y. Adaptive control synchronization of a novel Menristive chaotic system for secure communication application. Inventions 2019, 10, 1–11. [Google Scholar]
- Vaseghi, B.; Pourmina, M.A.; Mobayen, S. Secure communication in wireless sensor networks based on chaos synchronization using adaptive sliding mode control. Nonlinear Dyn. 2017, 89, 1689–1704. [Google Scholar] [CrossRef]
- Jasim, B.H.; Mjily, A.H.; Al-Aaragee, A.M.J. A novel 4 dimensional hyperchaotic system with its control, Synchronization and Implementation. Int. J. Electr. Comput. Eng. (IJECE) 2021, 11, 2974–2985. [Google Scholar] [CrossRef]
- Yuan, L.-G.; Yang, Q.-G. Parameter identification and synchronization of fractional-order chaotic systems. Commun. Nonlinear Sci. Numer. Simul. 2012, 17, 305–316. [Google Scholar] [CrossRef]
- Keyong, S.; Ruixuan, B.; Wang, G.; Qiutong, W.; Yi, Z. Passive Synchronization Control for Integer-order Chaotic Systems and Fractional-order Chaotic Systems. In Proceedings of the 2019 Chinese Control Conference (CCC), Guangzhou, China, 27–30 July 2019; pp. 1115–1119. [Google Scholar]
- Liu, B.; Sun, Z.; Luo, Y.; Zhong, Y. Uniform synchronization for chaotic dynamical systems via event-triggered impulsive control. Phys. A: Stat. Mech. its Appl. 2019, 531, 121725. [Google Scholar] [CrossRef]
- Azar, A.T.; Vaidyanathan, S.; Ouannas, A. Fractional-Order Control and Synchronization of Chaotic Systems; Springer: New York, NY, USA, 2017; Volume 688. [Google Scholar]
- Rahman, Z.A.S.A.; Jassim, B.H.; Al-Yasir, Y.I.A. New Fractional Order Chaotic System: Analysis, Synchronization, and it’s Application. Iraqi J. Electr. Electron. Eng. 2021, 17, 116–123. [Google Scholar] [CrossRef]
- Tlelo-Cuautle, E.; Pano-Azucena, A.D.; Guillén-Fernández, O.; Silva-Juárez, A. Analog/Digital Implementation of Fractional-Order Chaotic Circuits and Applications; Springer: New York, NY, USA, 2020. [Google Scholar]
- Petrzela, J. Chaos in Analog Electronic Circuits: Comprehensive Review, Solved Problems, Open Topics and Small Example. Mathematics 2022, 10, 4108. [Google Scholar] [CrossRef]
- Hashemi, S.; Pourmina, M.A.; Mobayen, S.; Alagheband, M.R. Design of a secure communication system between base transmitter station and mobile equipment based on finite-time chaos synchronisation. Int. J. Syst. Sci. 2020, 51, 1969–1986. [Google Scholar] [CrossRef]
- Vanchinathan, K.; Selvaganesan, N. Adaptive fractional order PID controller tuning for brushless DC motor using Artificial Bee Colony algorithm. Results Control Optim. 2021, 4, 100032. [Google Scholar] [CrossRef]
- Petráš, I. Fractional-Order Nonlinear Systems: Modeling, Analysis and Simulation; Springer Science & Business Media: Berlin, Germany, 2011. [Google Scholar]
- Vanchinathan, K.; Valluvan, K.R. A Metaheuristic Optimization Approach for Tuning of Fractional-Order PID Controller for Speed Control of Sensorless BLDC Motor. J. Circuits Syst. Comput. 2018, 27, 1850123. [Google Scholar] [CrossRef]
- Das, S. Functional Fractional Calculus; Springer Science & Business Media: Berlin, Germany, 2011. [Google Scholar]
- Wen, C.; Yang, J. Complexity evolution of chaotic financial systems based on fractional calculus. Chaos Solitons Fractals 2019, 128, 242–251. [Google Scholar] [CrossRef]
- Ashpazzadeh, E.; Chu, Y.M.; Hashemi, M.S.; Moharrami, M.; Inc, M. Hermite multiwavelets representation for the sparse solution of nonlinear Abel’s integral equation. Appl. Math. Comput. 2022, 427, 127171. [Google Scholar] [CrossRef]
- Cao, Y. Chaotic synchronization based on fractional order calculus financial system. Chaos Solitons Fractals 2020, 130, 109410. [Google Scholar] [CrossRef]
- Rahman, Z.A.S.A.; Jasim, B.H.; Al-Yasir, Y.I.A.; Abd-Alhameed, R.A.; Alhasnawi, B.N. A New No Equilibrium Fractional Order Chaotic System, Dynamical Investigation, Synchronization, and Its Digital Implementation. Inventions 2021, 6, 49. [Google Scholar] [CrossRef]
- Liu, T.; Yan, H.; Banerjee, S.; Mou, J. A fractional-order chaotic system with hidden attractor and self-excited attractor and its DSP implementation. Chaos Solitons Fractals 2021, 145, 110791. [Google Scholar] [CrossRef]
- Gong, L.; Wu, R.; Zhou, N. A New 4D Chaotic System with Coexisting Hidden Chaotic Attractors. Int. J. Bifurc. Chaos 2020, 30, 2050142. [Google Scholar] [CrossRef]
- Zhou, C.; Yang, C.; Xu, D.; Chen, C. Dynamic analysis and synchronisation control of a novel chaotic system with coexisting attractors. Pramana 2020, 94, 19. [Google Scholar] [CrossRef]
- Lai, Q.; Wan, Z.; Kuate, P.D.K.; Fotsin, H. Coexisting attractors, circuit implementation and synchronization control of a new chaotic system evolved from the simplest memristor chaotic circuit. Commun. Nonlinear Sci. Numer. Simul. 2020, 89, 105341. [Google Scholar] [CrossRef]
- Dabiri, A.; Moghaddam, B.; Machado, J.T. Optimal variable-order fractional PID controllers for dynamical systems. J. Comput. Appl. Math. 2018, 339, 40–48. [Google Scholar] [CrossRef]
- Ma, L.; Liu, X.; Moradi, Z. On the chaotic behavior of graphene-reinforced annular systems under harmonic excitation. Eng. Comput. 2022, 38, 2583–2607. [Google Scholar] [CrossRef]
- Parsa, M.B.; Dabiri, A.; Machado, J.A.T. Application of variable-order fractional calculus in solid mechanics. Appl. Eng. Life Soc. Sci. Part A 2019, 7, 207–224. [Google Scholar]
- Garrappa, R. Numerical Solution of Fractional Differential Equations: A Survey and a Software Tutorial. Mathematics 2018, 6, 16. [Google Scholar] [CrossRef]
- Wang, C.; Fan, C.; Ding, Q. Constructing Discrete Chaotic Systems with Positive Lyapunov Exponents. Int. J. Bifurc. Chaos 2018, 28, 1850084. [Google Scholar] [CrossRef]
- Danca, M.-F.; Kuznetsov, N. Matlab code for Lyapunov exponents of fractional-order systems. Int. J. Bifurc. Chaos 2018, 28, 1850067. [Google Scholar] [CrossRef] [Green Version]
- Vaidyanathan, S.; Volos, C. Advances and Applications in Chaotic Systems; Springer: Berlin, Germany, 2016; Volume 636. [Google Scholar]
- Haddad, W.M.; Bernstein, D.S. Explicit construction of quadratic Lyapunov functions for the small gain, positivity, circle, and Popov theorems and their application to robust stability. In Control of Uncertain Dynamic Systems; CRC Press: Boca Raton, FL, USA, 2020; pp. 149–173. [Google Scholar]
- Cai, G.; Tan, Z. Chaos synchronization of a new chaotic system via nonlinear control. J. Uncertain Syst. 2007, 1, 235–240. [Google Scholar]
- Dutta, M.; Roy, B.K. A new fractional-order system displaying coexisting multiwing attractors; its synchronisation and circuit simulation. Chaos Solitons Fractals 2020, 130, 109414. [Google Scholar] [CrossRef]
- Rashidnejad, Z.; Karimaghaee, P. Synchronization of a class of uncertain chaotic systems utilizing a new finite-time fractional adaptive sliding mode control. Chaos Solitons Fractals X 2020, 5, 100042. [Google Scholar] [CrossRef]
- Martínez-Guerra, R.; Gómez-Cortés, G.C.; Pérez-Pinacho, C.A. Synchronization of Integral and Fractional Order Chaotic Systems; Springer: Berlin, Germany, 2015. [Google Scholar]
- Martínez-Guerra, R.; Pérez-Pinacho, C.A. Advances in Synchronization of Coupled Fractional Order Systems: Fundamentals and Methods; Springer: Berlin, Germany, 2018. [Google Scholar]
- Kharel, R. Design and Implementation of Secure Chaotic Communication Systems. Doctoral Dissertation, Northumbria University, Newcastle-upon-Tyne, UK, 2011. [Google Scholar]
- Cuomo, K.M.; Oppenheim, A.V. Circuit implementation of synchronized chaos with applications to communications. Phys. Rev. Lett. 1993, 71, 65. [Google Scholar] [CrossRef] [PubMed]
- Alanazi, A.S.; Munir, N.; Khan, M.; Asif, M.; Hussain, I. Cryptanalysis of Novel Image Encryption Scheme Based on Multiple Chaotic Substitution Boxes. IEEE Access 2021, 9, 93795–93802. [Google Scholar] [CrossRef]
- Kacha, A.; Grenez, F.; Orozco-Arroyave, J.R.; Schoentgen, J. Principal component analysis of the spectrogram of the speech signal: Interpretation and application to dysarthric speech. Comput. Speech Lang. 2020, 59, 114–122. [Google Scholar] [CrossRef]





Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rahman, Z.-A.S.A.; Jasim, B.H. Hidden Dynamics Investigation, Fast Adaptive Synchronization, and Chaos-Based Secure Communication Scheme of a New 3D Fractional-Order Chaotic System. Inventions 2022, 7, 108. https://doi.org/10.3390/inventions7040108
Rahman Z-ASA, Jasim BH. Hidden Dynamics Investigation, Fast Adaptive Synchronization, and Chaos-Based Secure Communication Scheme of a New 3D Fractional-Order Chaotic System. Inventions. 2022; 7(4):108. https://doi.org/10.3390/inventions7040108
Chicago/Turabian StyleRahman, Zain-Aldeen S. A., and Basil H. Jasim. 2022. "Hidden Dynamics Investigation, Fast Adaptive Synchronization, and Chaos-Based Secure Communication Scheme of a New 3D Fractional-Order Chaotic System" Inventions 7, no. 4: 108. https://doi.org/10.3390/inventions7040108
APA StyleRahman, Z.-A. S. A., & Jasim, B. H. (2022). Hidden Dynamics Investigation, Fast Adaptive Synchronization, and Chaos-Based Secure Communication Scheme of a New 3D Fractional-Order Chaotic System. Inventions, 7(4), 108. https://doi.org/10.3390/inventions7040108






