Current Advances in Ejector Modeling, Experimentation and Applications for Refrigeration and Heat Pumps. Part 2: Two-Phase Ejectors
Abstract
1. Introduction
2. Two-Phase Ejector Characteristics
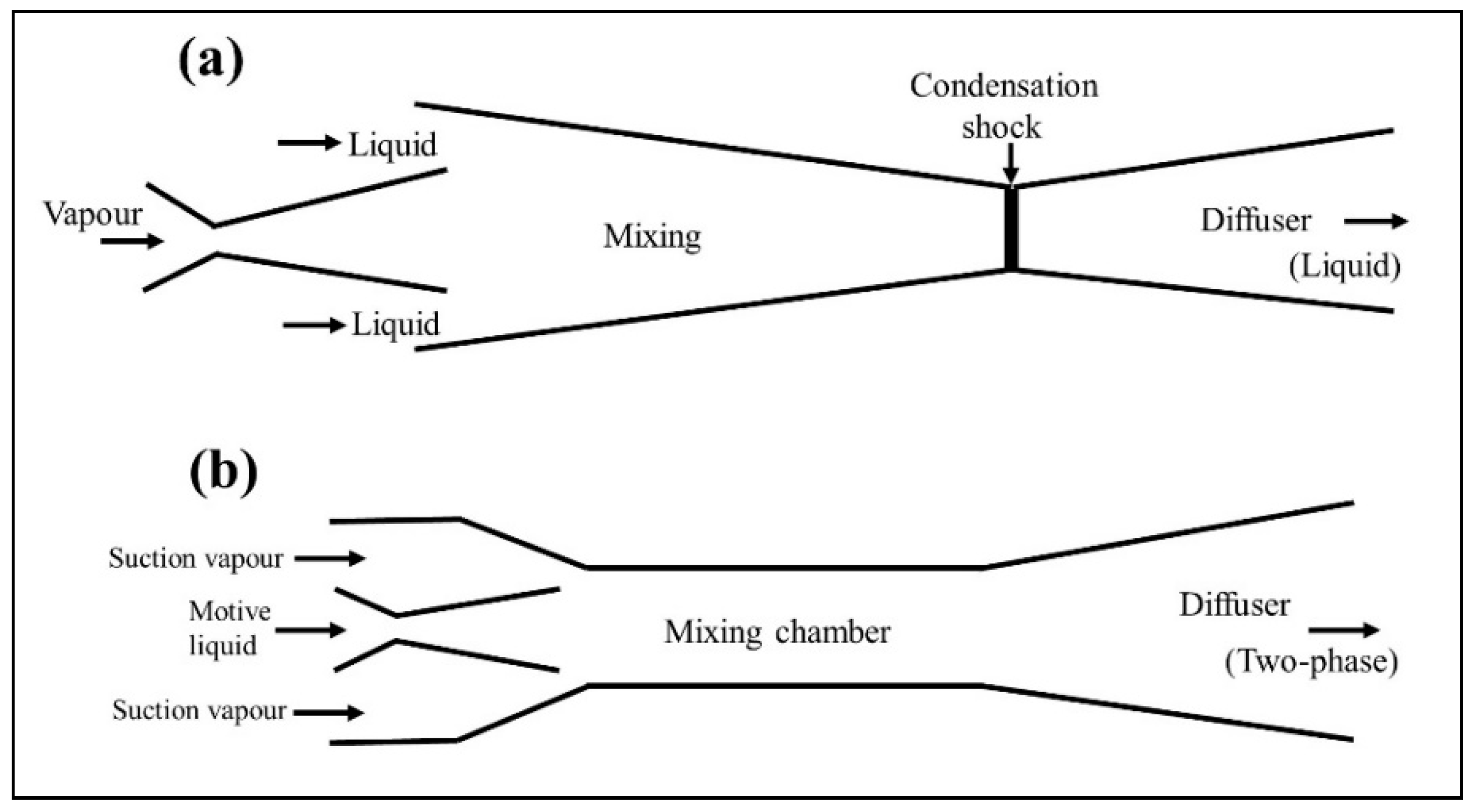
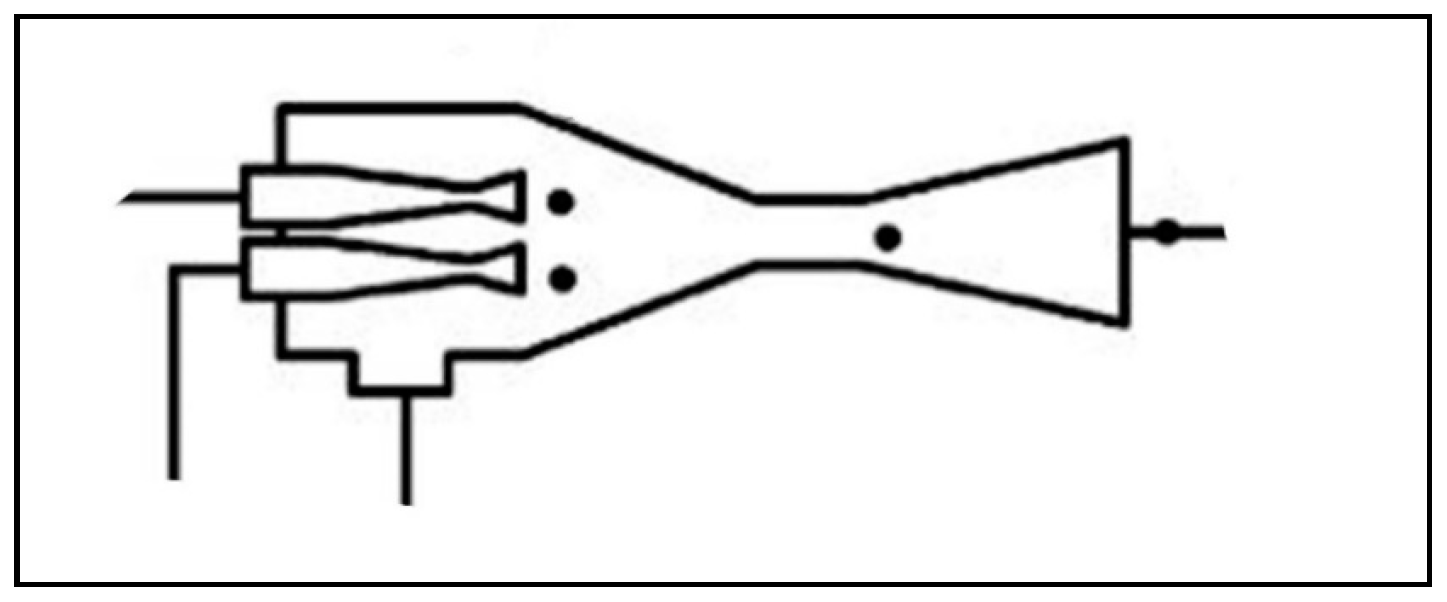
2.1. Ejector Types
2.1.1. Gas–Liquid Injectors
2.1.2. Liquid–Gas Ejectors
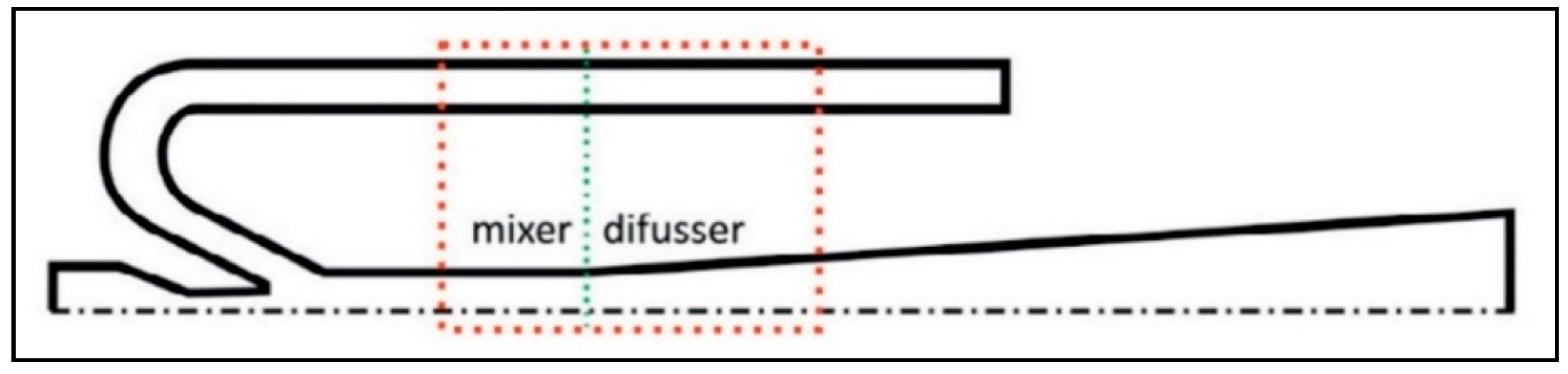
2.2. Ejector Geometry
2.3. Ejector Performance
2.3.1. Ejector Efficiency
2.3.2. Second Law Analysis of Ejectors
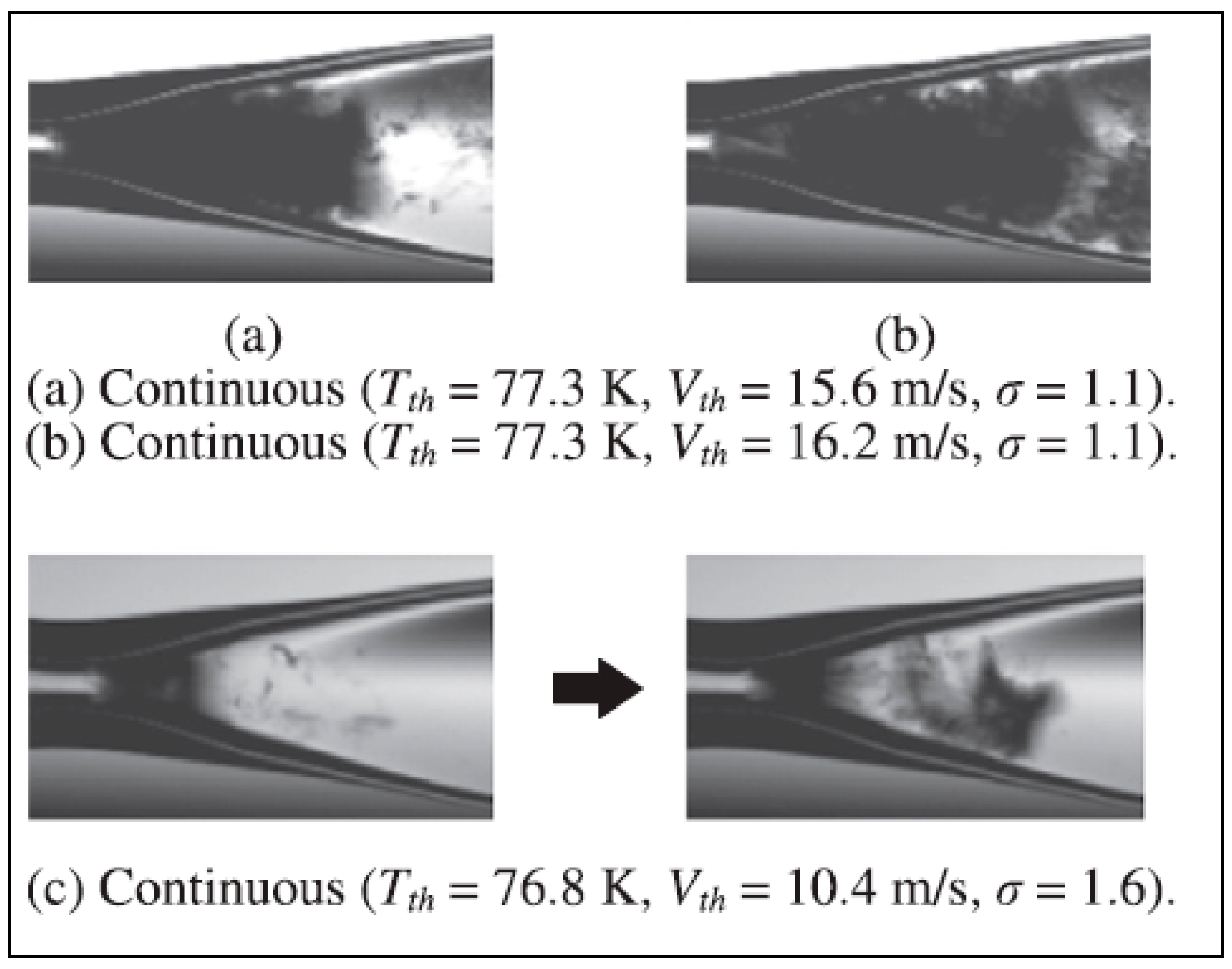
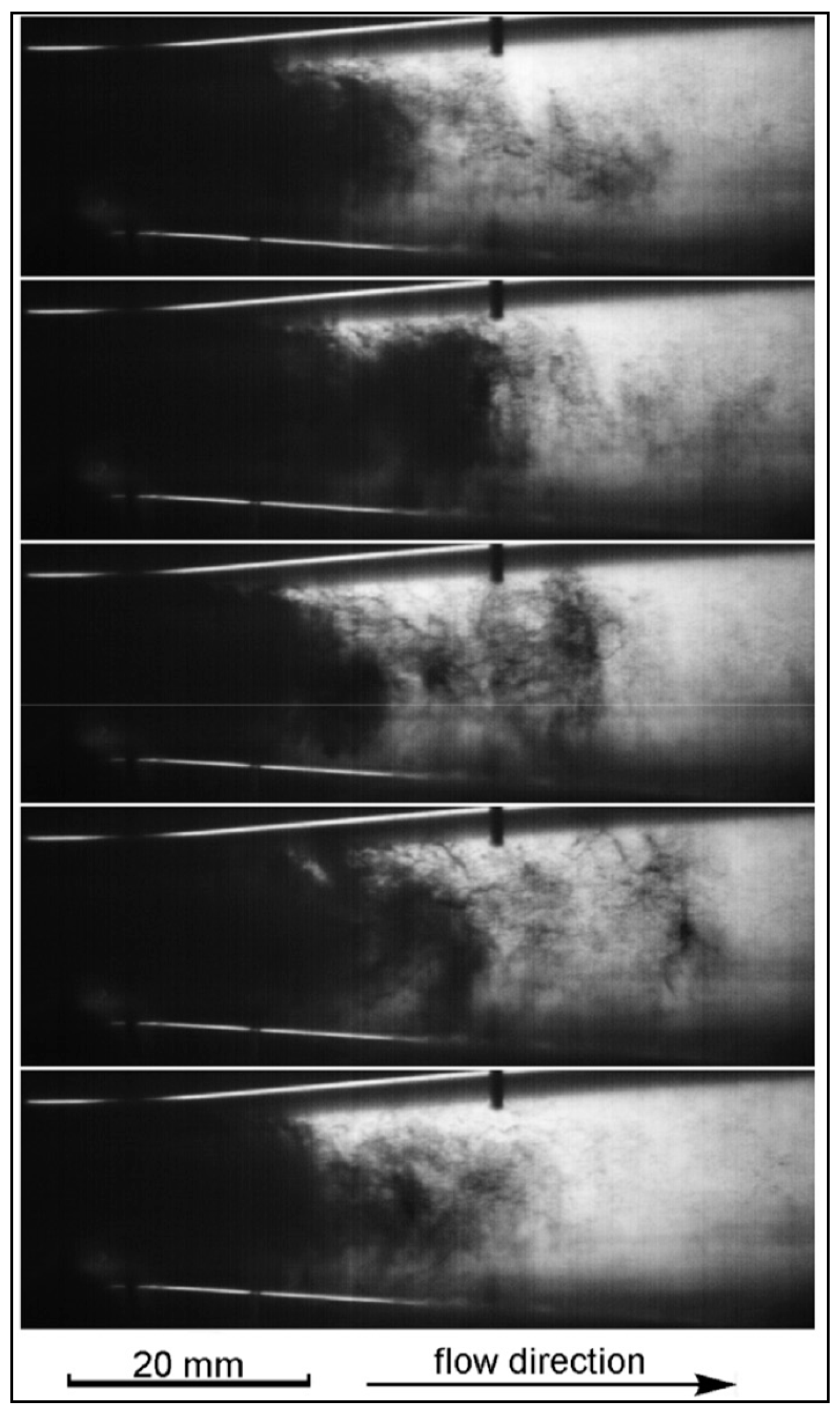
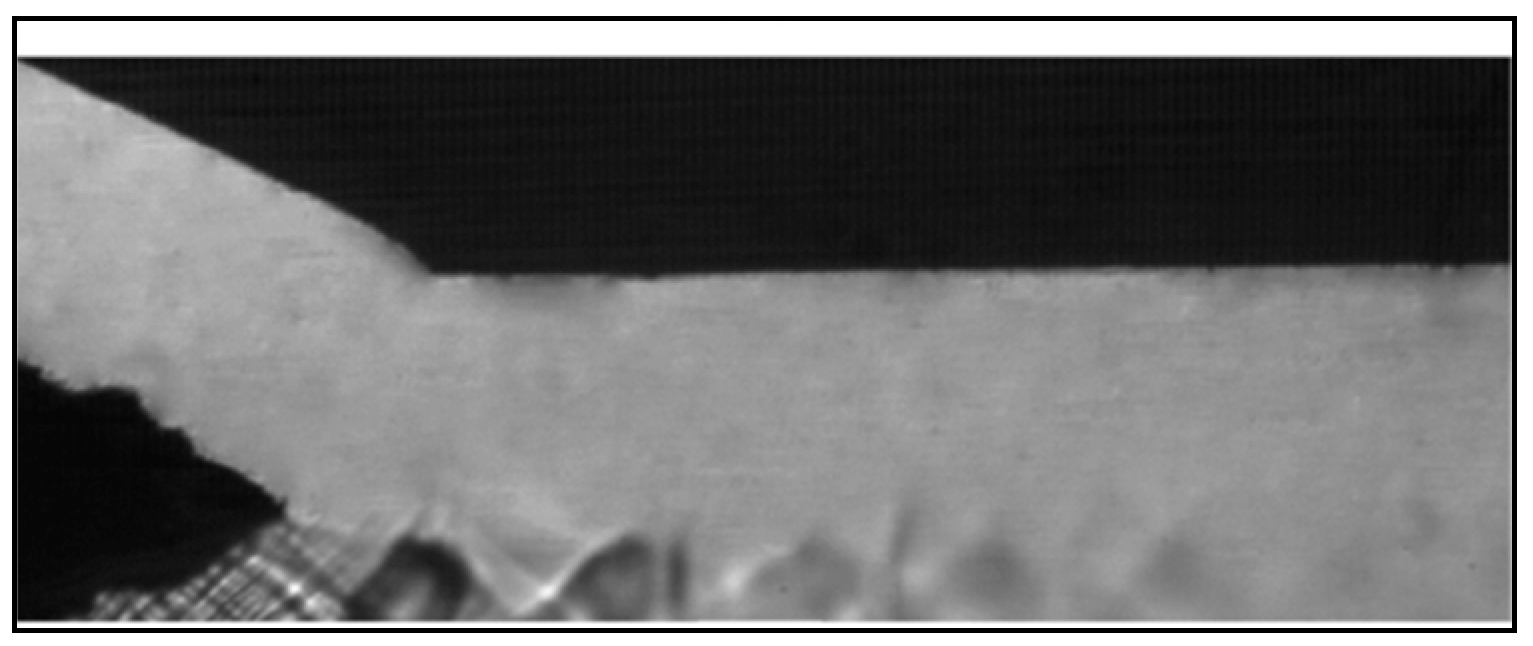
2.4. Internal Flow Structure
2.5. Applications Potential of the Two-Phase Ejector
3. Two-Phase Ejector Modeling
3.1. Thermodynamic and Analytical Modeling
3.2. CFD Modeling of Two-Phase Ejectors
3.2.1. Treatment of Two-Phase CO2 Ejectors
3.2.2. Phase Change in the Motive Nozzle
4. Experiments on Ejectors
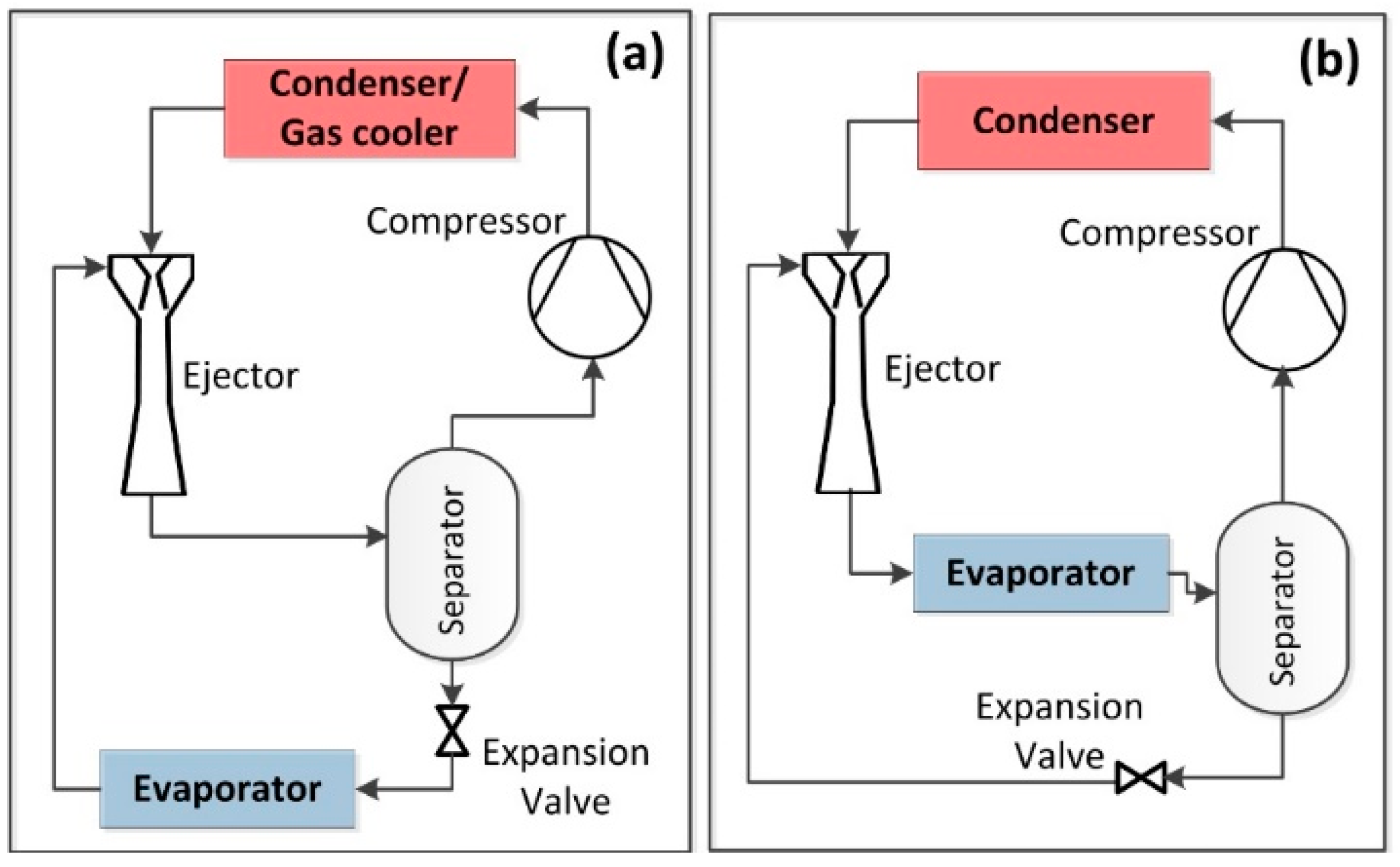
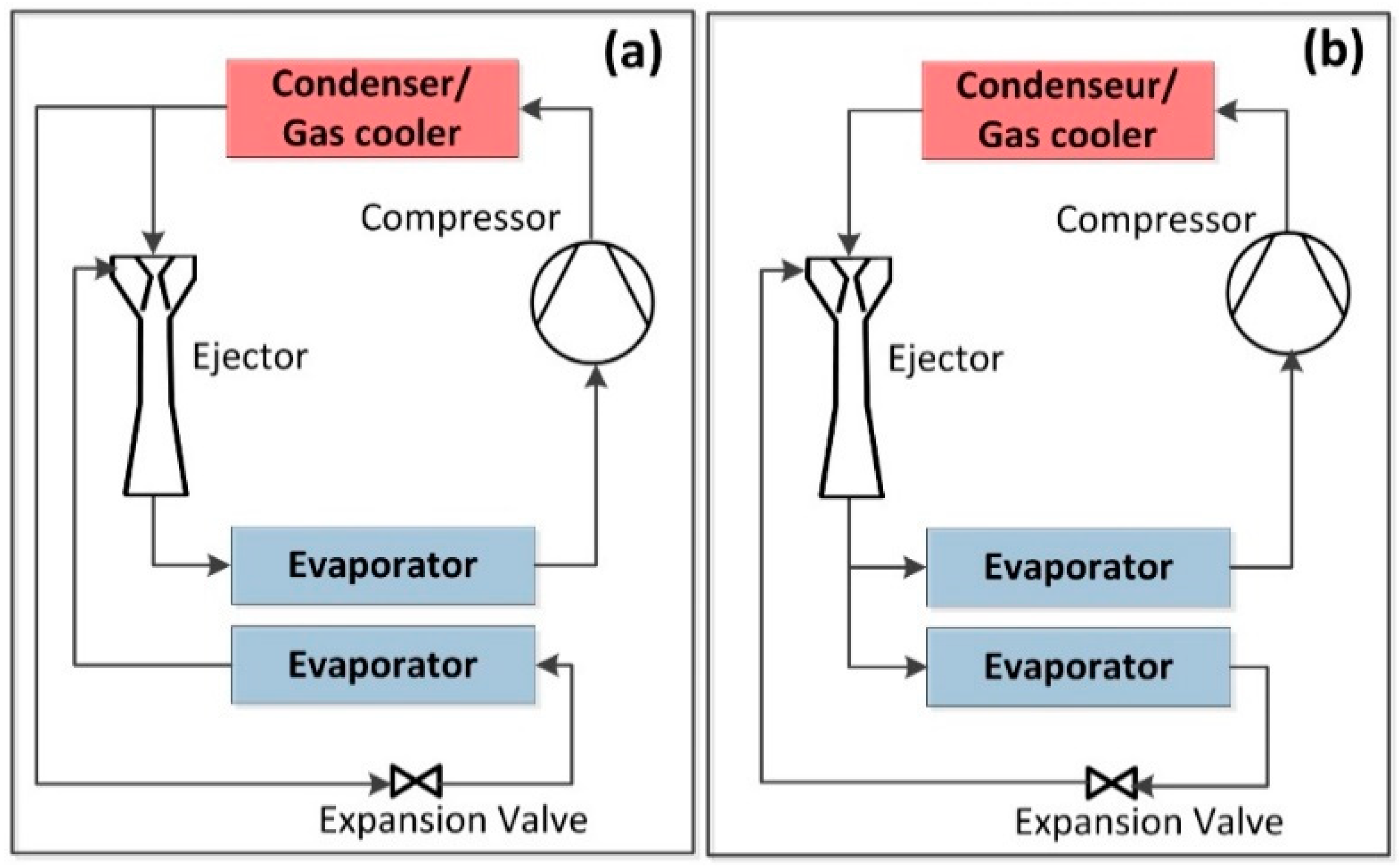
5. Two-Phase Ejector Cycles and Systems
5.1. The Conventional Ejector Expansion Refrigeration Cycle
5.1.1. EERC Theoretical Studies
5.1.2. EERC Experimental Studies
5.2. Miscellaneous Two-Phase Ejector Cycles
5.2.1. Theoretical Studies
5.2.2. Experimental Studies
6. General Remarks and Challenges
7. Conclusions
Funding
Conflicts of Interest
Abbreviation
| A | area |
| CAM | Constant Area Mixing |
| CFD | Computational Fluid Dynamics |
| COP | Coefficient of performance |
| CPM | Constant Pressure Mixing |
| D | diameter |
| EERC | Ejector Expansion Refrigeration Cycle |
| ERC | Ejector recirculation cycle |
| ERS | Ejector Refrigeration System |
| G | mass flow rate |
| h | enthalpy |
| HEM | Homogeneous Equilibrium Model |
| HRM | Homogenous Relaxation Model |
| IHE | Isentropic Homogeneous Equilibrium |
| IHX | Internal Heat Exchanger |
| L | length |
| mass flow rate | |
| NXP | nozzle exit position |
| P | pressure |
| PIV | Particle Image Velocimetry |
| Q | capacity |
| T | temperature |
| W | energy consumption |
| Greek | |
| x | vapor quality |
| α | nozzle convergent angle |
| β | nozzle divergent angle |
| Δ | difference; improvement |
| η | diffuser angle; efficiency |
| θ | nozzle area ratio |
| ξ | exergy efficiency |
| ρ | density |
| ς | entropy increase avoided |
| τ | compression ratio |
| ϕ | area ratio |
| φ | mixing convergent angle |
| ω | entrainment ratio |
| exergy flow rate | |
| Subscripts | |
| 0 | stagnation |
| amb | ambient |
| b | back |
| c | condenser |
| com | compressor |
| dif | diffuser |
| e | evaporator |
| ej | ejector |
| gc | gas cooler |
| m | mixing |
| n | nozzle |
| p | primary |
| ref | reference |
| s | secondary |
| sub | sub-cooling |
| sup | superheating |
| t | throat |
| w | water |
| x | nozzle outlet |
References
- Elbel, S.; Hrnjak, P. Ejector Refrigeration: An Overview of Historical and Present Developments with an Emphasis on Air-Conditioning Applications. In Proceedings of the International Refrigeration and Air Conditioning Conference, West Lafayette, IN, USA, 14–17 July 2008; p. 884. [Google Scholar]
- Kumar, R.S.; Kumaraswamy, S.; Mani, A. Experimental investigations on a two-phase jet pump used in desalination systems. Desalination 2007, 204, 437–447. [Google Scholar] [CrossRef]
- Sumeru, K.; Nasution, H.; Ani, F.N. A review on two-phase ejector as an expansion device in vapor compression refrigeration cycle. Renew. Sustain. Energy Rev. 2012, 16, 4927–4937. [Google Scholar] [CrossRef]
- Li, Y.; Tan, L.; Zhang, X.; Du, K. Experimental evaluation of an ejector as liquid re-circulator in a falling-film water chiller. Int. J. Refrig. 2014, 40, 309–316. [Google Scholar] [CrossRef]
- Besagni, G.; Mereu, R.; Inzoli, F. Ejector refrigeration: A comprehensive review. Renew. Sustain. Energy Rev. 2016, 53, 373–407. [Google Scholar] [CrossRef]
- Sarkar, J. Review on Cycle Modifications of Transcritical CO2 Refrigeration and Heat Pump Systems. J. Adv. Res. Mech. Eng. 2010, 1, 22–29. [Google Scholar]
- Elbel, S. Historical and present developments of ejector refrigeration systems with emphasis on transcritical carbon dioxide air-conditioning applications. Int. J. Refrig. 2011, 34, 1545–1561. [Google Scholar] [CrossRef]
- Sarkar, J. Ejector enhanced vapor compression refrigeration and heat pump systems—A review. Renew. Sustain. Energy Rev. 2012, 16, 6647–6659. [Google Scholar] [CrossRef]
- Banasiak, K.; Hafner, A.; Palacz, M. State of the Art in Identification of Two-Phase Transonic Flow Phenomena in Transcritical CO2 Ejectors. In Proceedings of the ICR 2015, Yokohama, Japan, 16–22 August 2015. [Google Scholar]
- Elbel, S.; Lawrence, N. Review of recent developments in advanced ejector technology. Int. J. Refrig. 2016, 62, 1–18. [Google Scholar] [CrossRef]
- Besagni, G. Ejectors on the cutting edge: The past, the present and the perspective. Energy 2019, 170, 998–1003. [Google Scholar] [CrossRef]
- Yazdani, M.; Alahyari, A.A.; Radcliff, T.D. Numerical modeling of two-phase supersonic ejectors for work-recovery applications. Int. J. Heat Mass Transf. 2012, 55, 5744–5753. [Google Scholar] [CrossRef]
- Colarossi, M.; Trask, N.; Schmidt, D.P.; Bergander, M.J. Multidimensional modeling of condensing two-phase ejector flow. Int. J. Refrig. 2012, 35, 290–299. [Google Scholar] [CrossRef]
- Miwa, S.; Endo, H.; Moribe, T.; Sakashita, H.; Mori, M.; Hibiki, T. Investigation of the thermal-hydraulic characteristics of supersonic steam injector. Appl. Therm. Eng. 2016, 109, 261–271. [Google Scholar] [CrossRef]
- Butrymowicz, D.; Matysko, R.; Angielczyk, W.; Trela, M.; Bergander, M. Model of steam-water injector. In Proceedings of the International Seminar on Ejector/Jet-Pump Technology and Application, Louvain-la-Neuve, Belgium, 7–9 September 2009; p. 27. [Google Scholar]
- Lawrence, N.; Elbel, S. Mathematical modeling and thermodynamic investigation of the use of two-phase ejectors for work recovery and liquid recirculation in refrigeration cycles. Int. J. Refrig. 2015, 58, 41–52. [Google Scholar] [CrossRef]
- Sarevski, M.N.; Sarevski, V.N. Characteristics of R718 refrigeration/heat pump systems with two-phase ejectors. Int. J. Refrig. 2016, 70, 13–32. [Google Scholar] [CrossRef]
- Ameur, K.; Aidoun, Z.; Ouzzane, M. Analysis of the Critical Conditions and the Effect of Slip in Two-Phase Ejectors. JAFM 2016, 9, 213–222. [Google Scholar]
- Narabayashi, T.; Mizumachi, W.; Mori, M. Study on two-phase flow dynamics in steam injectors. Nucl. Eng. Des. 1997, 175, 147–156. [Google Scholar] [CrossRef]
- Trela, M.; Kwidzinski, R.; Butrymowicz, D.; Karwacki, J. Exergy analysis of two-phase steam-water injector. Appl. Therm. Eng. 2010, 30, 340–346. [Google Scholar] [CrossRef]
- Deberne, N.; Leone, J.F.; Duque, A.; Lallemand, A. A model for calculation of steam injector performance. Int. J. Multiph. Flow 1999, 25, 841–855. [Google Scholar] [CrossRef]
- Takeya, Y.; Miwa, S.; Hibiki, T.; Mori, M. Application of steam injector to improved safety of light water reactors. Prog. Nucl. Energy 2015, 78, 80–100. [Google Scholar] [CrossRef]
- Miwa, S.; Endo, H.; Moribe, T.; Mori, M. Investigation of the supersonic steam injector operation mode. Nucl. Eng. Des. 2018, 334, 57–65. [Google Scholar] [CrossRef]
- Bergander, M. Refrigeration cycle with two-phase condensing ejector. In Proceedings of the International Refrigeration and Air Conditioning Conference, West Lafayette, IN, USA, 17–20 July 2006; p. 748. [Google Scholar]
- Ameur, K.; Aidoun, Z.; Ouzzane, M. Modeling and numerical approach for the design and operation of two-phase ejectors. Appl. Therm. Eng. 2016, 109, 809–818. [Google Scholar] [CrossRef]
- Keenan, J.H.; Neumann, E.P.; Lustwerk, F. An investigation of ejector design by analysis and experiment. J. Appl. Mech. 1950, 72, 299–309. [Google Scholar]
- Atmaca, A.U.; Erek, A.; Ekren, O. Impact of the mixing theories on the performance of ejector expansion refrigeration cycles for environmentally-friendly refrigerants. Int. J. Refrig. 2019, 97, 211–225. [Google Scholar] [CrossRef]
- Liu, F.; Groll, E.A.; Li, D. Investigation on performance of variable geometry ejectors for CO2 refrigeration cycles. Energy 2012, 45, 829–839. [Google Scholar] [CrossRef]
- Hu, J.; Shi, J.; Liang, Y.; Yang, Z.; Chen, J. Numerical and experimental investigation on nozzle parameters for R410A ejector air conditioning system. Int. J. Refrig. 2014, 40, 338–346. [Google Scholar] [CrossRef]
- Wang, X.; Yu, J. Experimental investigation on two-phase driven ejector performance in a novel ejector enhanced refrigeration system. Energy Convers. Manag. 2016, 111, 391–400. [Google Scholar] [CrossRef]
- Ameur, K.; Aidoun, Z. Nozzle displacement effects on two-phase ejector performance: An experimental study. JAFM 2018, 11, 1–8. [Google Scholar] [CrossRef]
- Nehdi, E.; Kairouani, L.; Bouzaina, M. Performance analysis of the vapour compression cycle using ejector as an expander. Int. J. Energy Res. 2007, 31, 364–375. [Google Scholar] [CrossRef]
- Sarkar, J. Geometric parameter optimization of ejector-expansion refrigeration cycle with natural refrigerants. Int. J. Energy Res. 2010, 34, 84–94. [Google Scholar] [CrossRef]
- Nakagawa, M.; Marasigan, A.R.; Matsukawa, T. Experimental analysis of two-phase ejector system with varying mixing cross-sectional area using natural refrigerant CO2. Int. J. Air-Cond. Refrig. 2010, 18, 297–307. [Google Scholar] [CrossRef]
- Nakagawa, M.; Matumi, T.; Takeuchi, H.; Kokubo, N. Mixing of the Confined Jet of Mist Flow. JSME Int. J. 1996, 39, 381–386. [Google Scholar] [CrossRef]
- Nakagawa, M.; Marasigan, A.R.; Matsukawa, T.; Kurashina, A. Experimental investigation on the effect of mixing length on the performance of two-phase ejector for CO2 refrigeration cycle with and without heat exchanger. Int. J. Refrig. 2011, 34, 1604–1613. [Google Scholar] [CrossRef]
- Banasiak, K.; Hafner, A.; Andresen, T. Experimental and numerical investigation of the influence of the two-phase ejector geometry on the performance of the R744 heat pump. Int. J. Refrig. 2012, 35, 1617–1625. [Google Scholar] [CrossRef]
- Jeon, Y.; Jung, J.; Kim, D.; Kim, S.; Kim, Y. Effects of ejector geometries on performance of ejector-expansion R410A air conditioner considering cooling seasonal performance factor. Appl. Energy 2017, 205, 761–768. [Google Scholar] [CrossRef]
- Randheer, Y.L.; Patwardhan, A.W. Design aspects of ejectors: Effects of suction chamber geometry. Chem. Eng. Sci. 2008, 63, 3886–3897. [Google Scholar]
- Li, C.; Li, Y.; Wang, L. Configuration dependence and optimization of the entrainment performance for gas-gas and gas-liquid ejectors. Appl. Therm. Eng. 2012, 48, 237–248. [Google Scholar] [CrossRef]
- Nakagawa, M.; Berana, M.S.; Kishine, A. Supersonic two-phase flow of CO2 through converging–diverging nozzles for the ejector refrigeration cycle. Int. J. Refrig. 2009, 32, 1195–1202. [Google Scholar] [CrossRef]
- He, Y.; Deng, J.; Zheng, L.; Zhang, Z. Performance optimization of a transcritical CO2 refrigeration system using a controlled ejector. Int. J. Refrig. 2017, 75, 250–261. [Google Scholar] [CrossRef]
- Zhou, M.; Wang, X.; Yu, J. Theoretical study on a novel dual-nozzle ejector enhanced refrigeration cycle for household refrigerator-freezers. Energy Convers. Manag. 2013, 73, 278–284. [Google Scholar] [CrossRef]
- Bodys, J.; Smolka, J.; Banasiak, K.; Palacz, M.; Haida, M.; Nowak, A.J. Performance improvement of the R744 two-phase ejector with an implemented suction nozzle bypass. Int. J. Refrig. 2018, 90, 216–228. [Google Scholar] [CrossRef]
- Disawas, S.; Wongwises, S. Experimental investigation on the performance of the refrigeration cycle using a two-phase ejector as an expansion device. Int. J. Refrig. 2004, 27, 587–594. [Google Scholar] [CrossRef]
- Ameur, K.; Aidoun, Z.; Ouzzane, M. Experimental performances of a two-phase R134a ejector. Exp. Therm. Fluid Sci. 2018, 97, 12–20. [Google Scholar] [CrossRef]
- Lawrence, N.; Elbel, S. Analysis of two-phase ejector performance metrics and comparison of R134a and CO2 ejector performance. Sci. Technol. Built Environ. 2015, 21, 515–525. [Google Scholar] [CrossRef]
- Elbel, S.; Hrnjak, P. Experimental validation of a prototype ejector designed to reduce throttling losses encountered in transcritical R744 system operation. Int. J. Refrig. 2008, 31, 411–422. [Google Scholar] [CrossRef]
- Koehler, J.; Richter, C.; Tegethoff, W.; Tischendorf, C. Experimental and theoretical study of a CO2 ejector refrigeration cycle. In Proceedings of the VDA Alternative Refrigerant Winter Meeting, Saalfelden, Austria, 13–14 February 2007. [Google Scholar]
- Ersoy, H.K.; Bilir, N. The influence of ejector component efficiencies on performance of ejector expander refrigeration cycle and exergy analysis. Int. J. Exergy 2010, 7, 425–438. [Google Scholar] [CrossRef]
- Liu, F.; Groll, E.A.; Li, D. Modeling study of an ejector expansion residential CO2 air conditioning system. Energy Build. 2012, 53, 127–136. [Google Scholar] [CrossRef]
- Liu, F.; Groll, E.A. Study of ejector efficiencies in refrigeration cycles. Appl. Therm. Eng. 2013, 52, 360–370. [Google Scholar] [CrossRef]
- Wang, X.; Yu, J. An investigation on the component efficiencies of a small two-phase ejector. Int. J. Refrig. 2016, 71, 26–38. [Google Scholar] [CrossRef]
- Banasiak, K.; Palacz, M.; Hafner, A.; Bulinski, Z.; Smołka, J.; Nowak, A.J.; Fic, A. A CFD-based investigation of the energy performance of two-phase R744 ejectors to recover the expansion work in refrigeration systems: An irreversibility analysis. Int. J. Refrig. 2014, 40, 328–337. [Google Scholar] [CrossRef]
- Bilir Sag, N.; Ersoy, H.K.; Hepbasli, A.; Halkaci, H.S. Energetic and exergetic comparison of basic and ejector expander refrigeration systems operating under the same external conditions and cooling capacities. Energy Convers. Manag. 2015, 90, 184–194. [Google Scholar] [CrossRef]
- Bullard, C.W. Internal heat exchange, expanders and intercooling in trans-critical CO2 cycles. In Proceedings of the 6 th IIR Gustav Lorentzen Conference on Natural Refrigerants, Glasgow, UK, 2004. [Google Scholar]
- Takashima, Y. Studies on liquid-jet gas pumps. J. Sci. Res. Inst. 1952, 46, 230–246. [Google Scholar]
- Simoes-Moreira, J.R.; Vieira, M.M.; Angelo, E. Highly Expanded Flashing Liquid Jets. J. Thermophys. Heat Transf. 2002, 16, 415–424. [Google Scholar]
- Berana, M.S.; Nakagawa, M.; Harada, A. Shock waves in supersonic two-phase flow of CO2 in Converging-Diverging Nozzles. HVAC R Res. 2009, 15, 1081–1098. [Google Scholar] [CrossRef]
- Ohira, K.; Nakayama, T.; Nagai, T. Cavitation flow instability of subcooled liquid nitrogen in converging–diverging nozzles. Cryogenics 2012, 52, 35–44. [Google Scholar] [CrossRef]
- Kim, Y.K.; Lee, D.Y.; Kim, H.D.; Ahn, J.H.; Kim, K.C. An experimental and numerical study on hydrodynamic characteristics of horizontal annular type water-air ejector. J. Mech. Sci. Technol. 2012, 26, 2773–2781. [Google Scholar] [CrossRef]
- Kwidzinski, R. Experimental investigation of condensation wave structure in steam-water injector. Int. J. Heat Mass Transf. 2015, 91, 594–601. [Google Scholar] [CrossRef]
- Choi, S.H.; Ji, H.S.; Kim, K.C. Comparative study of hydrodynamic characteristics with respect to direction of installation of gas-liquid ejector system. J. Mech. Sci. Technol. 2015, 29, 3267–3276. [Google Scholar] [CrossRef]
- Little, A.B.; Garimella, S. Shadowgraph visualization of condensing R134a flow through ejectors. Int. J. Refrig. 2016, 68, 118–129. [Google Scholar] [CrossRef]
- Elbel, S.; Hrnjak, P. Experimental validation of a CO2 prototype ejector with integrated high-side pressure control. In Proceedings of the VDA Alternative Refrigerant Winter Meeting, Saalfelden, Austria, 13–14 February 2007. [Google Scholar]
- Deng, J.; Zhang, Y.; He, Y.; Zheng, L. Visual investigation on effect of structural parameters and operation condition of two-phase ejector. In Proceedings of the International Compressor Engineering, Refrigeration and Air Conditioning, and High Performance Buildings Conferences, West Lafayette, IN, USA, 11–14 July 2016; p. 1632. [Google Scholar]
- Zhu, Y.; Wang, Z.; Yang, Y.; Jiang, P. Flow visualization of supersonic two-phase transcritical flow of CO2 in an ejector of a refrigeration system. Int. J. Refrig. 2017, 74, 354–361. [Google Scholar] [CrossRef]
- Barrault, É. Histoire d’une grande invention, l’éjecteur Giffard. In La Nature; Masson, G., Ed.; La Nature: Paris, France, 1882; pp. 65–70. [Google Scholar]
- Kim, M.; Sohn, Y.; Cho, C.; Lee, W.; Kim, C. Customized design for the ejector to recirculate a humidified hydrogen fuel in a submarine PEMFC. J. Power Sources 2008, 176, 529–533. [Google Scholar] [CrossRef]
- Chen, J.; Li, J.; Zhou, D.; Zhang, H.; Weng, S. Control strategy design for a SOFC-GT hybrid system equipped with anode and cathode recirculation ejectors. Appl. Therm. Eng. 2018, 132, 67–79. [Google Scholar] [CrossRef]
- Yuan, G.; Zhang, L.; Zhang, H.; Wang, Z. Numerical and experimental investigation of performance of the liquid-gas and liquid jet pumps in desalination systems. Desalination 2011, 276, 89–95. [Google Scholar] [CrossRef]
- Song, X.; Cao, M.; Shin, W.; Cao, W.; Kang, S.; Park, Y. Numerical investigation of a liquid-gas ejector used for shipping ballast water treatment. Math. Probl. Eng. 2014, 2014, 259593. [Google Scholar] [CrossRef]
- Borovykh, A.E. Calculation of the parameters of evacuation systems for tanks with a liquid-gas ejector. Chem. Pet. Eng. 1993, 29, 269–270. [Google Scholar] [CrossRef]
- Opletal, M.; Novotný, P.; Linek, V.; Moucha, T.; Kordač, M. Gas suction and mass transfer in gas-liquid up-flow ejector loop reactors. Effect of nozzle and ejector geometry. Chem. Eng. J. 2018, 353, 436–452. [Google Scholar] [CrossRef]
- Kim, M.I.; Sin Kim, O.; Lee, D.H.; Done Kim, S. Numerical and experimental investigations of gas-liquid dispersion in an ejector. Chem. Eng. Sci. 2007, 62, 7133–7139. [Google Scholar] [CrossRef]
- Kandakure, M.T.; Gaikar, V.G.; Patwardhan, A.W. Hydrodynamic aspects of ejectors. Chem. Eng. Sci. 2005, 60, 6391–6402. [Google Scholar] [CrossRef]
- Balamurugan, S.; Gaikar, V.G.; Patwardhan, A.W. Effect of ejector configuration on hydrodynamic characteristics of gas-liquid ejectors. Chem. Eng. Sci. 2008, 63, 721–731. [Google Scholar] [CrossRef]
- Abe, Y.; Shibayama, S. Study on the characteristics of the supersonic steam injector. Nucl. Eng. Des. 2014, 268, 191–202. [Google Scholar] [CrossRef]
- Kemper, C.A.; Harper, G.F.; Brown, G.A. Multiple-Phase Ejectors Refrigerating System. U.S. Patent 3,277,660, 11 October 1966. [Google Scholar]
- Menegay, P.; Kornhauser, A.A. Improvements to the ejector expansion refrigeration cycle. In Proceedings of the 31st Intersociety Energy Conversion Engineering Conference, IECEC 96, Washington, DC, USA, 11–16 August 1996. [Google Scholar]
- Boccardi, G.; Botticella, F.; Lillo, G.; Mastrullo, R.; Mauro, A.W.; Trinchieri, R. Experimental investigation on the performance of a transcritical CO2 heat pump with multi-ejector expansion system. Int. J. Refrig. 2017, 82, 389–400. [Google Scholar] [CrossRef]
- Dopazo, J.A.; Fernández-Seara, J. Experimental evaluation of an ejector as liquid re-circulator in an overfeed NH3 system with a plate evaporator. Int. J. Refrig. 2011, 34, 1676–1683. [Google Scholar] [CrossRef]
- Li, Y.L.; Wang, K.; Wu, W.; Xia, X.Y.; Niu, B.L.; Zhang, Z. Bin Investigation on the effect of ejector liquid recirculation system on the performance of falling-film water chiller with R134a. Int. J. Refrig. 2017, 74, 333–344. [Google Scholar] [CrossRef]
- Oshitani, H.; Oshitani, H.; Yamanaka, Y.; Takeuchi, H.; Kusano, K.; Ikegami, M.; Aikawa, Y. Ejector Cycle Having Multiple Evaporators. U.S. Patent 20050178150A1, 18 August 2005. [Google Scholar]
- Burk, R.; Dürr, G.; Feuerecker, G.; Feuerecker, G.; Kohl, M.; Manski, R.; Strauss, T. Device for Automotive Air Conditioning. European Patent EP1719650A1, 8 November 2006. [Google Scholar]
- Hassanain, M.; Elgendy, E.; Fatouh, M. Ejector expansion refrigeration system: Ejector design and performance evaluation. Int. J. Refrig. 2015, 58, 1–13. [Google Scholar] [CrossRef]
- Zhu, Y.; Jiang, P. Theoretical model of transcritical CO2 ejector with non-equilibrium phase change correlation. Int. J. Refrig. 2018, 86, 218–227. [Google Scholar] [CrossRef]
- Baek, S.; Ko, S.; Song, S.; Ryu, S. Numerical study of high-speed two-phase ejector performance with R134a refrigerant. Int. J. Heat Mass Transf. 2018, 126, 1071–1082. [Google Scholar] [CrossRef]
- Zheng, P.; Li, B.; Qin, J. CFD simulation of two-phase ejector performance influenced by different operation conditions. Energy 2018, 155, 1129–1145. [Google Scholar] [CrossRef]
- Starkman, E.S.; Schrock, V.E.; Neusen, K.F.; Maneely, D.J. Expansion of a Very Low Quality Two-Phase Fluid Through a Convergent-Divergent Nozzle. J. Basic Eng. 1964, 86, 247–254. [Google Scholar] [CrossRef]
- Abuaf, N.; Jones, O.C.; Wu, B.J.C. Critical Flashing Flows in Nozzles with Subcooled Inlet Conditions. J. Heat Transf. 1983, 105, 379–383. [Google Scholar] [CrossRef]
- Kim, Y.S. Overview of geometrical effects on the critical flow rate of sub-cooled and saturated water. Ann. Nucl. Energy 2015, 76, 12–18. [Google Scholar] [CrossRef]
- Ameur, K.; Aidoun, Z.; Ouzzane, M. Expansion of subcooled refrigerant in two-phase ejectors with no flux induction. Exp. Therm. Fluid Sci. 2017, 82, 424–432. [Google Scholar] [CrossRef]
- Liu, J.; Chen, J.; Chen, Z. Critical flashing flow in convergent-divergent nozzles with initially subcooled liquid. Int. J. Therm. Sci. 2008, 47, 1069–1076. [Google Scholar] [CrossRef]
- Banasiak, K.; Hafner, A. Mathematical modelling of supersonic two-phase R744 fl ows through converging e diverging nozzles: The effects of phase transition models. Appl. Therm. Eng. 2013, 51, 635–643. [Google Scholar] [CrossRef]
- Kong, N.; Qi, Z. Influence of speed of sound in two-phase region on 1-D ejector performance modelling. Appl. Therm. Eng. 2018, 139, 352–355. [Google Scholar] [CrossRef]
- Kornhauser, A.A. The Use of an Ejector as a Refrigerant Expander. In Proceedings of the International Refrigeration and Air Conditioning Conference, West Lafayette, IN, USA, 17–20 July 1990; p. 82. [Google Scholar]
- Al-Ansary, H.; Jeter, S. Development of a simple homogeneous flow model of a two-phase ejector. In Proceedings of the 34th Intersociety Energy Conversion Engineering Conference, Vancouver, BC, Canada, 2–5 August 1999; p. 2698. [Google Scholar]
- Elbel, S.; Hrnjak, P.S. Effect of Internal Heat Exchanger on Performance of Transcritical CO2 Systems with Ejector. In Proceedings of the International Refrigeration and Air Conditioning Conference, West Lafayette, IN, USA, 12–15 July 2004; p. 708. [Google Scholar]
- Li, D.; Groll, E.A. Transcritical CO2 Refrigeration Cycle with Ejector-Expansion Device. In Proceedings of the International Refrigeration and Air Conditioning Conference, West Lafayette, IN, USA, 12–15 July 2004; p. 707. [Google Scholar]
- Lawrence, N.; Elbel, S. Experimental and Analytical Investigation of Automotive Ejector Air-Conditioning Cycles Using Low-Pressure Refrigerants. In Proceedings of the International Refrigeration and Air Conditioning Conference, West Lafayette, IN, USA, 16–19 July 2012; p. 116. [Google Scholar]
- Menegay, P.; Kornhauser, A. Ejector expansion refrigeration cycle with underexpanded motive nozzle. In Proceedings of the Intersociety Energy Conversion Engineering Conference, Monterey, CA, USA, 7–12 August 1994; pp. 915–920. [Google Scholar]
- Liu, F.; Groll, E.A. Analysis of a Two Phase Flow Ejector For Transcritical CO2 Cycle. In Proceedings of the International Refrigeration and Air Conditioning Conference, West Lafayette, IN, USA, 14–17 July 2008; p. 924. [Google Scholar]
- Attou, A.; Seynhaeve, J.M. Steady-state critical two-phase flashing flow with possible multiple choking phenomenon Part 1: Physical modelling and numerical procedure. J. Loss Prev. Process Ind. 1999, 12, 335–345. [Google Scholar] [CrossRef]
- Owen, I.; Abdul-Ghani, A.; Amini, A.M. Diffusing a Homogenized Two-Phase. Int. J. Multiph. Flow 1992, 18, 531–540. [Google Scholar] [CrossRef]
- Kwidziński, R. Control-volume-based model of the steam-water injector flow. Arch. Thermodyn. 2010, 31, 45–59. [Google Scholar] [CrossRef]
- Banasiak, K.; Hafner, A. 1D Computational model of a two-phase R744 ejector for expansion work recovery. Int. J. Therm. Sci. 2011, 50, 2235–2247. [Google Scholar] [CrossRef]
- Liao, Y.; Lucas, D. Possibilities and limitations of CFD simulation for flashing flow scenarios in nuclear applications. Energies 2017, 10, 139. [Google Scholar] [CrossRef]
- Mazzelli, F.; Giacomelli, F.; Milazzo, A. CFD modelling of the condensation inside a Supersonic Nozzle: Implementing customized wet-steam model in commercial codes. Energy Procedia 2017, 126, 34–41. [Google Scholar]
- Bulinski, Z.; Smolka, J.; Fic, A.; Banasiak, K.; Nowak, A.J. A comparison of heterogenous and homogenous models of two-phase transonic compressible CO2 flow through a heat pump ejector. IOP Conf. Ser. Mater. Sci. Eng. 2010, 10, 012019. [Google Scholar] [CrossRef]
- Yazdani, M.; Alahyari, A.A.; Radcliff, T.D. Numerical Modeling and Validation of Supersonic Two-Phase Flow of CO2 in Converging-Diverging Nozzles. J. Fluids Eng. 2014, 136, 14503. [Google Scholar] [CrossRef]
- Smolka, J.; Bulinski, Z.; Fic, A.; Nowak, A.J.; Banasiak, K.; Hafner, A. A computational model of a transcritical R744 ejector based on a homogeneous real fluid approach. Appl. Math. Model. 2013, 37, 1208–1224. [Google Scholar] [CrossRef]
- Lucas, C.; Rusche, H.; Schroeder, A.; Koehler, J. Numerical investigation of a two-phase CO2 ejector. Int. J. Refrig. 2014, 43, 154–166. [Google Scholar] [CrossRef]
- Palacz, M.; Smolka, J.; Fic, A.; Bulinski, Z.; Nowak, A.J.; Banasiak, K.; Hafner, A. Application range of the HEM approach for CO2 expansion inside two-phase ejectors for supermarket refrigeration systems. Int. J. Refrig. 2015, 59, 251–258. [Google Scholar] [CrossRef]
- Haida, M.; Smolka, J.; Hafner, A.; Palacz, M.; Banasiak, K.; Nowak, A.J. Modified homogeneous relaxation model for the R744 trans-critical flow in a two-phase ejector. Int. J. Refrig. 2018, 85, 314–333. [Google Scholar] [CrossRef]
- Palacz, M.; Smolka, J.; Nowak, A.J.; Banasiak, K.; Hafner, A. Shape optimisation of a two-phase ejector for CO2 refrigeration systems. Int. J. Refrig. 2017, 74, 212–223. [Google Scholar] [CrossRef]
- Haida, M.; Smolka, J.; Hafner, A.; Mastrowski, M.; Palacz, M.; Madsen, K.B.; Nowak, A.J.; Banasiak, K. Numerical investigation of heat transfer in a CO2 two-phase ejector. Energy 2018, 163, 682–698. [Google Scholar] [CrossRef]
- Mikasser, S. Transfert de Masse et de Chaleur dans les Injecteurs-Condenseurs. Ph.D. Thesis, Institut National des Sciences Appliquées de Lyon, Villeurbanne, France, 2004. [Google Scholar]
- Teymourtash, A.R.; Esfahani, J.A.; Mousavi Shaegh, S.A. The effects of rate of expansion and injection of water droplets on the entropy generation of nucleating steam flow in a Laval nozzle. Heat Mass Transf. 2009, 45, 1185–1198. [Google Scholar] [CrossRef]
- Yang, Y.; Shen, S.; Kong, T.; Zhang, K. Numerical investigation of homogeneous nucleation and shock effect in high-speed transonic steam flow. Heat Transf. Eng. 2010, 31, 1007–1014. [Google Scholar] [CrossRef]
- Grazzini, G.; Milazzo, A.; Piazzini, S. Prediction of condensation in steam ejector for a refrigeration system. Int. J. Refrig. 2011, 34, 1641–1648. [Google Scholar] [CrossRef]
- Wang, X.D.; Dong, J.L.; Wang, T.; Tu, J.Y. Numerical analysis of spontaneously condensing phenomena in nozzle of steam-jet vacuum pump. Vacuum 2012, 86, 861–866. [Google Scholar] [CrossRef]
- Ariafar, K.; Buttsworth, D.; Sharifi, N.; Malpress, R. Ejector primary nozzle steam condensation: Area ratio effects and mixing layer development. Appl. Therm. Eng. 2014, 71, 519–527. [Google Scholar] [CrossRef]
- Little, A.B.; Garimella, S. A critical review linking ejector flow phenomena with component- and system-level performance. Int. J. Refrig. 2016, 70, 243–268. [Google Scholar] [CrossRef]
- Al-Ansary, H.A.M.; Jeter, S.M. Numerical and experimental analysis of single-phase and two-phase flow in ejectors. HVAC&R Res. 2004, 10, 521–538. [Google Scholar]
- Croquer, S. Combined CFD and Thermodynamic Analysis of a Supersonic Ejector with Liquid Droplets. Ph.D. Thesis, Université de Sherbrooke, Sherbrooke, QC, Canada, 2018. [Google Scholar]
- Hemidi, A.; Henry, F.; Leclaire, S.; Seynhaeve, J.-M.; Bartosiewicz, Y. CFD analysis of a supersonic air ejector. Part I: Experimental validation of single-phase and two-phase operation. Appl. Therm. Eng. 2009, 29, 1523–1531. [Google Scholar] [CrossRef]
- Nakagawa, M.; Berana, M.S.; Harada, A. Shock Waves in Supersonic Two-Phase Flow of CO2 in Converging-Diverging Nozzles. In Proceedings of the International Refrigeration and Air Conditioning Conference, West Lafayette, IN, USA, 14–17 July 2008; p. 926. [Google Scholar]
- Kim, K.C.; Kim, H.D.; Kim, Y.K.; Ahn, J.H. Numerical simulation and experimental study on a water-air ejector system for VOC recovery. In Proceedings of the 12th International Conference on Multiphase Flow in Industrial Plants, Napoli, Italy, 21–23 September 2011. [Google Scholar]
- Butrymowicz, D.; Karwacki, J.; Miaskowska, D.; Trela, M. Performance of two-phase ejector of various geometries. In Proceedings of the International Congress of Refrigeration, Beijing, China, 21–26 August 2007. [Google Scholar]
- Smolka, J.; Palacz, M.; Bodys, J.; Banasiak, K.; Fic, A.; Bulinski, Z.; Nowak, A.J.; Hafner, A. Performance comparison of fixed- and controllable-geometry ejectors in a CO2 refrigeration system. Int. J. Refrig. 2016, 65, 172–182. [Google Scholar] [CrossRef]
- Zhu, J.; Elbel, S. Experimental investigation of a novel expansion device control mechanism: Vortex control of initially subcooled flashing R134a flow expanded through convergent-divergent nozzles. Int. J. Refrig. 2018, 85, 167–183. [Google Scholar] [CrossRef]
- Zhu, Y.; Huang, Y.; Li, C.; Zhang, F.; Jiang, P.X. Experimental investigation on the performance of transcritical CO2 ejector-expansion heat pump water heater system. Energy Convers. Manag. 2018, 167, 147–155. [Google Scholar] [CrossRef]
- Pottker, G.; Hrnjak, P. Ejector in R410A vapor compression systems with experimental quantification of two major mechanisms of performance improvement: Work recovery and liquid feeding. Int. J. Refrig. 2015, 50, 184–192. [Google Scholar] [CrossRef]
- Lawrence, N.; Elbel, S. Theoretical and practical comparison of two-phase ejector refrigeration cycles including First and Second Law analysis. Int. J. Refrig. 2013, 36, 1220–1232. [Google Scholar] [CrossRef]
- Khosravi, A.; Koury, R.N.N.; Machado, L. Thermo-economic analysis and sizing of the components of an ejector expansion refrigeration system. Int. J. Refrig. 2018, 86, 463–479. [Google Scholar] [CrossRef]
- Sarkar, J. Performance characteristics of natural-refrigerants-based ejector expansion refrigeration cycles. Proc. Inst. Mech. Eng. Part A J. Power Energy 2009, 223, 543–550. [Google Scholar] [CrossRef]
- Domanski, P.A. Minimizing Throttling Losses in the Refrigeration Cycle. In Proceedings of the 19th International Congress of Refrigeration, The Hague, The Netherlands, 20–25 August 1995. [Google Scholar]
- Bilir, N.; Ersoy, H.K. Performance improvement of the vapour compression refrigeration cycle by a two-phase constant area ejector. Int. J. Energy Res. 2009, 33, 469–480. [Google Scholar] [CrossRef]
- Li, H.; Cao, F.; Bu, X.; Wang, L.; Wang, X. Performance characteristics of R1234yf ejector-expansion refrigeration cycle. Appl. Energy 2014, 121, 96–103. [Google Scholar] [CrossRef]
- Zhao, L.; Yang, X.; Deng, S.; Li, H.; Yu, Z. Performance analysis of the ejector-expansion refrigeration cycle using zeotropic mixtures. Int. J. Refrig. 2015, 57, 197–207. [Google Scholar] [CrossRef]
- Luo, B. Theoretical assessment of an ejector enhanced oil flooded compression cycle. Int. J. Refrig. 2017, 73, 154–162. [Google Scholar] [CrossRef]
- Rodríguez-Muñoz, J.L.; Pérez-García, V.; Belman-Flores, J.M.; Ituna-Yudonago, J.F.; Gallegos-Muñoz, A. Energy and exergy performance of the IHX position in ejector expansion refrigeration systems. Int. J. Refrig. 2018, 93, 122–131. [Google Scholar] [CrossRef]
- Li, D.; Groll, E.A. Transcritical CO2 refrigeration cycle with ejector-expansion device. Int. J. Refrig. 2005, 28, 766–773. [Google Scholar] [CrossRef]
- Ksayer, E.B.; Clodic, D. Enhancement of CO2 Refrigeration Cycle Using an Ejector: 1D Analysis. In Proceedings of the International Refrigeration and Air Conditioning Conference, West Lafayette, IN, USA, 17–20 July 2006; p. 790. [Google Scholar]
- Deng, J.; Jiang, P.; Lu, T.; Lu, W. Particular characteristics of transcritical CO2 refrigeration cycle with an ejector. Appl. Therm. Eng. 2007, 27, 381–388. [Google Scholar] [CrossRef]
- Sarkar, J. Optimization of ejector-expansion transcritical CO2 heat pump cycle. Energy 2008, 33, 1399–1406. [Google Scholar] [CrossRef]
- Pérez-García, V.; Rodríguez-Muñoz, J.L.; Ramírez-Minguela, J.J.; Belman-Flores, J.M.; Méndez-Díaz, S. Comparative analysis of energy improvements in single transcritical cycle in refrigeration mode. Appl. Therm. Eng. 2016, 99, 866–872. [Google Scholar] [CrossRef]
- Fangtian, S.; Yitai, M. Thermodynamic analysis of transcritical CO2 refrigeration cycle with an ejector. Appl. Therm. Eng. 2011, 31, 1184–1189. [Google Scholar] [CrossRef]
- Zhang, Z.; Ma, Y.; Wang, H.; Li, M. Theoretical evaluation on effect of internal heat exchanger in ejector expansion transcritical CO2 refrigeration cycle. Appl. Therm. Eng. 2013, 50, 932–938. [Google Scholar] [CrossRef]
- Zhang, Z.; Tian, L. Effect of suction nozzle pressure drop on the performance of an ejector-expansion transcritical CO2 refrigeration cycle. Entropy 2014, 16, 4309–4321. [Google Scholar] [CrossRef]
- Zheng, L.; Deng, J.; He, Y.; Jiang, P. Dynamic model of a transcritical CO2 ejector expansion refrigeration system. Int. J. Refrig. 2015, 60, 247–260. [Google Scholar] [CrossRef]
- Liu, F.; Deng, J.; Pan, W. Model-based Dynamic Optimal Control of an Ejector Expansion CO2 Heat Pump Coupled with Thermal Storages. Energy Procedia 2018, 152, 156–161. [Google Scholar] [CrossRef]
- Sarkar, J.; Bhattacharyya, S. Operating characteristics of transcritical CO2 heat pump for simultaneous water cooling and heating. Arch. Thermodyn. 2012, 33, 23–40. [Google Scholar] [CrossRef]
- Henry, R.E.; Fauske, H.K. The Two-Phase Critical flow of One-Component Mixtures in Nozzles, Orifice, and Short Tubes. J. Heat Transf. 1971, 93, 179–187. [Google Scholar] [CrossRef]
- Harrell, G.S.; Kornhauser, A.A. Performance tests of a two phase ejector. In Proceedings of the 30th Intersociety Energy Conversion Engineering Conference, Orlando, FL, USA, 30 July–4 August 1995. [Google Scholar]
- Wongwises, S.; Disawas, S. Performance of the two-phase ejector expansion refrigeration cycle. Int. J. Heat Mass Transf. 2005, 48, 4282–4286. [Google Scholar] [CrossRef]
- Chaiwongsa, P.; Wongwises, S. Effect of throat diameters of the ejector on the performance of the refrigeration cycle using a two-phase ejector as an expansion device. Int. J. Refrig. 2007, 30, 601–608. [Google Scholar] [CrossRef]
- Chaiwongsa, P.; Wongwises, S. Experimental study on R-134a refrigeration system using a two-phase ejector as an expansion device. Appl. Therm. Eng. 2008, 28, 467–477. [Google Scholar] [CrossRef]
- Reddick, C.; Mercadier, Y.; Ouzzane, M. Experimental study of an ejector refrigeration system. In Proceedings of the International Refrigeration and Air Conditioning Conference, West Lafayette, IN, USA, 16–19 July 2012; p. 2136. [Google Scholar]
- Lawrence, N.; Elbel, S. Experimental investigation of a two-phase ejector cycle suitable for use with low-pressure refrigerants R134a and R1234yf. Int. J. Refrig. 2014, 38, 310–322. [Google Scholar] [CrossRef]
- Ersoy, H.K.; Bilir Sag, N. Preliminary experimental results on the R134a refrigeration system using a two-phase ejector as an expander. Int. J. Refrig. 2014, 43, 97–110. [Google Scholar] [CrossRef]
- Pottker, G.; Guo, B.; Hrnjak, P.S. Experimental Investigation of an R410A Vapor Compression System Working With an Ejector. In Proceedings of the International Refrigeration and Air Conditioning Conference, West Lafayette, IN, USA, 12–15 July 2010; p. 1135. [Google Scholar]
- Popovac, M.; Lauermann, M.; Baumhakel, A.; Drexler-, G. Performance analysis of a high-temperature heat pump with ejector based on butane as the refrigerant. In Proceedings of the 12th IEA Heat Pump Conference 2017, Rotterdam, The Netherlands, 15–18 May 2017; pp. 1–8. [Google Scholar]
- Nakagawa, M.; Marasigan, A.R.; Matsukawa, T. Experimental analysis on the effect of internal heat exchanger in transcritical CO2 refrigeration cycle with two-phase ejector. Int. J. Refrig. 2011, 34, 1577–1586. [Google Scholar] [CrossRef]
- Lee, J.S.; Kim, M.S.; Kim, M.S. Experimental study on the improvement of CO2 air conditioning system performance using an ejector. Int. J. Refrig. 2011, 34, 1614–1625. [Google Scholar] [CrossRef]
- Lee, J.S.; Kim, M.S.; Kim, M.S. Studies on the performance of a CO2 air conditioning system using an ejector as an expansion device. Int. J. Refrig. 2014, 38, 140–152. [Google Scholar] [CrossRef]
- Liu, F.; Li, Y.; Groll, E.A. Performance enhancement of CO2 air conditioner with a controllable ejector. Int. J. Refrig. 2012, 35, 1604–1616. [Google Scholar] [CrossRef]
- Lucas, C.; Koehler, J. Experimental investigation of the COP improvement of a refrigeration cycle by use of an ejector. Int. J. Refrig. 2012, 35, 1595–1603. [Google Scholar] [CrossRef]
- Lucas, C.; Koehler, J.; Schroeder, A.; Tischendorf, C. Experimentally validated CO2 ejector operation characteristic used in a numerical investigation of ejector cycle. Int. J. Refrig. 2013, 36, 881–891. [Google Scholar] [CrossRef]
- Guangming, C.; Xiaoxiao, X.; Shuang, L.; Lixia, L.; Liming, T. An experimental and theoretical study of a CO2 ejector. Int. J. Refrig. 2010, 33, 915–921. [Google Scholar] [CrossRef]
- Minetto, S.; Brignoli, R.; Banasiak, K.; Hafner, A.; Zilio, C. Performance assessment of an off-the-shelf R744 heat pump equipped with an ejector. Appl. Therm. Eng. 2013, 59, 568–575. [Google Scholar] [CrossRef]
- Hafner, A.; Forsterling, S.; Banasiak, K. Multi-ejector concept for R-744 supermarket refrigeration. Int. J. Refrig. 2014, 43, 1–13. [Google Scholar] [CrossRef]
- Zhu, J.; Botticella, F.; Elbel, S. Experimental investigation and theoretical analysis of oil circulation rates in ejector cooling cycles. Energy 2018, 157, 718–733. [Google Scholar] [CrossRef]
- Haida, M.; Banasiak, K.; Smolka, J.; Hafner, A.; Eikevik, T.M. Experimental analysis of the R744 vapour compression rack equipped with the multi-ejector expansion work recovery module. Int. J. Refrig. 2016, 64, 93–107. [Google Scholar] [CrossRef]
- Boumaraf, L.; Haberschill, P.; Lallemand, A. Investigation of a novel ejector expansion refrigeration system using the working fluid R134a and its potential substitute R1234yf. Int. J. Refrig. 2014, 45, 148–159. [Google Scholar] [CrossRef]
- Unal, Ş.; Yilmaz, T. Thermodynamic analysis of the two-phase ejector air-conditioning system for buses. Appl. Therm. Eng. 2015, 79, 108–116. [Google Scholar] [CrossRef]
- Liu, X.; Yu, J.; Yan, G. Theoretical investigation on an ejector-expansion refrigeration cycle using zeotropic mixture R290/R600a for applications in domestic refrigerator/freezers. Appl. Therm. Eng. 2015, 90, 703–710. [Google Scholar] [CrossRef]
- Zhu, L.; Yu, J.; Zhou, M.; Wang, X. Performance analysis of a novel dual-nozzle ejector enhanced cycle for solar assisted air-source heat pump systems. Renew. Energy 2014, 63, 735–740. [Google Scholar] [CrossRef]
- Wang, X.; Yu, J.; Zhou, M.; Lv, X. Comparative studies of ejector-expansion vapor compression refrigeration cycles for applications in domestic refrigerator-freezers. Energy 2014, 70, 635–642. [Google Scholar] [CrossRef]
- Sarkar, J. Performance analyses of novel two-phase ejector enhanced multi-evaporator refrigeration systems. Appl. Therm. Eng. 2017, 110, 1635–1642. [Google Scholar] [CrossRef]
- Xing, M.; Yan, G.; Yu, J. Performance evaluation of an ejector subcooled vapor-compression refrigeration cycle. Energy Convers. Manag. 2015, 92, 431–436. [Google Scholar] [CrossRef]
- Sarevski, M.N.; Sarevski, V.N. Preliminary study of a novel R718 refrigeration cycle with single stage centrifugal compressor and two-phase ejector. Int. J. Refrig. 2014, 40, 435–449. [Google Scholar] [CrossRef]
- Shen, S.; Qu, X.; Zhang, B.; Riffat, S.; Gillott, M. Study of a gas-liquid ejector and its application to a solar-powered bi-ejector refrigeration system. Appl. Therm. Eng. 2005, 25, 2891–2902. [Google Scholar] [CrossRef]
- Vereda, C.; Ventas, R.; Lecuona, A.; Venegas, M. Study of an ejector-absorption refrigeration cycle with an adaptable ejector nozzle for different working conditions. Appl. Energy 2012, 97, 305–312. [Google Scholar] [CrossRef]
- Vereda, C.; Ventas, R.; Lecuona, A.; López, R. Single-effect absorption refrigeration cycle boosted with an ejector-adiabatic absorber using a single solution pump. Int. J. Refrig. 2014, 38, 22–29. [Google Scholar] [CrossRef]
- Garousi Farshi, L.; Mosaffa, A.H.; Infante Ferreira, C.A.; Rosen, M.A. Thermodynamic analysis and comparison of combined ejector-absorption and single effect absorption refrigeration systems. Appl. Energy 2014, 133, 335–346. [Google Scholar] [CrossRef]
- Yari, M.; Sirousazar, M. Cycle improvements to ejector-expansion transcritical CO2 two-stage refrigeration cycle. Int. J. Energy Res. 2008, 32, 677–687. [Google Scholar] [CrossRef]
- Goodarzi, M.; Gheibi, A.; Motamedian, M. Comparative analysis of an improved two-stage multi-inter-cooling ejector-expansion trans-critical CO2 refrigeration cycle. Appl. Therm. Eng. 2015, 81, 58–65. [Google Scholar] [CrossRef]
- Manjili, F.E.; Yavari, M.A. Performance of a new two-stage multi-intercooling transcritical CO2 ejector refrigeration cycle. Appl. Therm. Eng. 2012, 40, 202–209. [Google Scholar] [CrossRef]
- Yari, M.; Mahmoudi, S.M.S. Thermodynamic analysis and optimization of novel ejector-expansion TRCC (transcritical CO2) cascade refrigeration cycles (Novel transcritical CO2 cycle). Energy 2011, 36, 6839–6850. [Google Scholar] [CrossRef]
- Dokandari, D.A.; Hagh, A.; Mahmoudi, S.M.S. Thermodynamic investigation and optimization of novel ejector-expansion CO2/NH3 cascade refrigeration cycles (novel CO2/NH3 cycle). Int. J. Refrig. 2014, 46, 26–36. [Google Scholar] [CrossRef]
- Bai, T.; Yan, G.; Yu, J. Thermodynamic analyses on an ejector enhanced CO2 transcritical heat pump cycle with vapor-injection. Int. J. Refrig. 2015, 58, 22–34. [Google Scholar] [CrossRef]
- Bai, T.; Yan, G.; Yu, J. Thermodynamics analysis of a modified dual-evaporator CO2 transcritical refrigeration cycle with two-stage ejector. Energy 2015, 84, 325–335. [Google Scholar] [CrossRef]
- Liu, Y.; Yu, J.; Yan, G. Theoretical analysis of a double ejector-expansion autocascade refrigeration cycle using hydrocarbon mixture R290/R170. Int. J. Refrig. 2018, 94, 33–39. [Google Scholar] [CrossRef]
- Lawrence, N.; Elbel, S. Numerical investigation of the effect of microchannel evaporator design and operation on the improvement potential of ejector refrigeration cycles. Energy 2018, 164, 21–34. [Google Scholar] [CrossRef]
- Haida, M.; Smolka, J.; Hafner, A.; Ostrowski, Z.; Palacz, M.; Madsen, K.B.; Försterling, S.; Nowak, A.J.; Banasiak, K. Performance mapping of the R744 ejectors for refrigeration and air conditioning supermarket application: A hybrid reduced-order model. Energy 2018, 153, 933–948. [Google Scholar] [CrossRef]
- Huang, Z.; Zhao, H.; Yu, Z.; Han, J. Simulation and optimization of a R744 two-temperature supermarket refrigeration system with an ejector. Int. J. Refrig. 2018, 90, 73–82. [Google Scholar] [CrossRef]
- Bodys, J.; Hafner, A.; Banasiak, K.; Smolka, J.; Ladam, Y. Design and simulations of refrigerated sea water chillers with CO2 ejector pumps for marine applications in hot climates. Energy 2018, 161, 90–103. [Google Scholar] [CrossRef]
- Smierciew, K.; Butrymowicz, D.; Kwidziński, R.; Przybyliński, T. Analysis of application of two-phase injector in ejector refrigeration systems for isobutane. Appl. Therm. Eng. 2015, 78, 630–639. [Google Scholar] [CrossRef]
- Man-O, T.; Tanino, M.; Okazaki, T.; Koyama, S. The enhanced heat transfer in the plate-type evaporator by using an ejector for recirculation. In Proceedings of the International Congress of Refrigeration, Beijing, China, 21–26 August 2007. [Google Scholar]
- Jeon, Y.; Kim, D.; Jung, J.; Jang, D.S.; Kim, Y. Comparative performance evaluation of conventional and condenser outlet split ejector-based domestic refrigerator-freezers using R600a. Energy 2018, 161, 1085–1095. [Google Scholar] [CrossRef]
- Lawrence, N.; Elbel, S. Experimental Investigation of Two-Phase Ejector Liquid Recirculation Cycles With R410a. In Proceedings of the ICR 2015, Yokohama, Japan, 16–22 August 2015; p. 194. [Google Scholar]
- Lawrence, N.; Elbel, S. Experimental investigation on the effect of evaporator design and application of work recovery on the performance of two-phase ejector liquid recirculation cycles with R410A. Appl. Therm. Eng. 2016, 100, 398–411. [Google Scholar] [CrossRef]
- Bai, T.; Yan, G.; Yu, J. Experimental investigation of an ejector-enhanced auto-cascade refrigeration system. Appl. Therm. Eng. 2018, 129, 792–801. [Google Scholar] [CrossRef]
- Smierciew, K.; Butrymowicz, D. Investigations of Two-Phase Injector Operating With Isobutane. In Proceedings of the ICR 2015, Yokohama, Japan, 16–22 August 2015; p. 506. [Google Scholar]
- Smierciew, K.; Butrymowicz, D.; Przybylinski, T. Investigations of Heat and Momentum Transfer in Vapor-Liquid Isobutane Injector. In Proceedings of the International Refrigeration and Air Conditioning Conference, West Lafayette, IN, USA, 11–14 July 2016; p. 1823. [Google Scholar]
- Banasiak, K.; Hafner, A.; Kriezi, E.E.; Madsen, K.B.; Birkelund, M.; Fredslund, K.; Olsson, R. Development and performance mapping of a multi-ejector expansion work recovery pack for R744 vapour compression units. Int. J. Refrig. 2015, 57, 265–276. [Google Scholar] [CrossRef]
- Minetto, S.; Brignoli, R.; Zilio, C.; Marinetti, S. Experimental analysis of a new method for overfeeding multiple evaporators in refrigeration systems. Int. J. Refrig. 2014, 38, 1–9. [Google Scholar] [CrossRef]
- Jeon, Y.; Kim, S.; Kim, D.; Chung, H.J.; Kim, Y. Performance characteristics of an R600a household refrigeration cycle with a modified two-phase ejector for various ejector geometries and operating conditions. Appl. Energy 2017, 205, 1059–1067. [Google Scholar] [CrossRef]
- Kim, S.; Jeon, Y.; Joon Chung, H.; Kim, Y. Performance optimization of an R410A air-conditioner with a dual evaporator ejector cycle based on cooling seasonal performance factor. Appl. Therm. Eng. Accept. Manuscr. 2018, 131, 988–997. [Google Scholar] [CrossRef]
- Bartosiewicz, Y.; Aidoun, Z.; Mercadier, Y. Numerical assessment of ejector operation for refrigeration applications based on CFD. Appl. Therm. Eng. 2006, 26, 604–612. [Google Scholar] [CrossRef]
- Croquer, S.; Poncet, S.; Aidoun, Z. Turbulence modeling of a single-phase R134a supersonic ejector. Part 2: Local flow structure and exergy analysis. Int. J. Refrig. 2016, 61, 153–165. [Google Scholar] [CrossRef]
- Zegenhagen, M.T.; Ziegler, F. Experimental investigation of the characteristics of a jet-ejector and a jet-ejector cooling system operating with R134a as a refrigerant. Int. J. Refrig. 2015, 56, 173–185. [Google Scholar] [CrossRef]
- Hamzaoui, M.; Nesreddine, H.; Aidoun, Z.; Balistrou, M. Experimental study of a low grade heat driven ejector cooling system using the working fluid R245fa. Int. J. Refrig. 2018, 86, 388–400. [Google Scholar] [CrossRef]

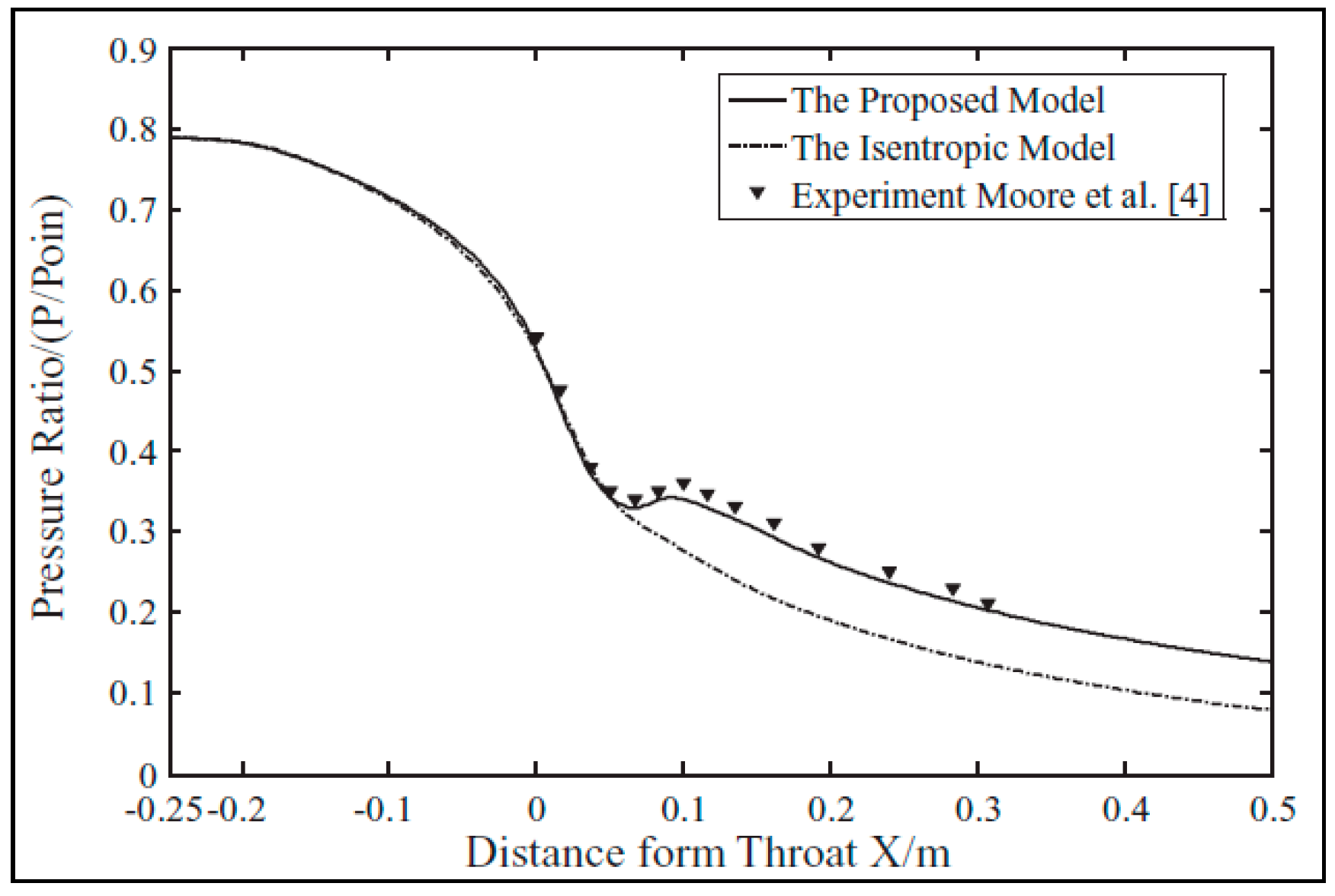
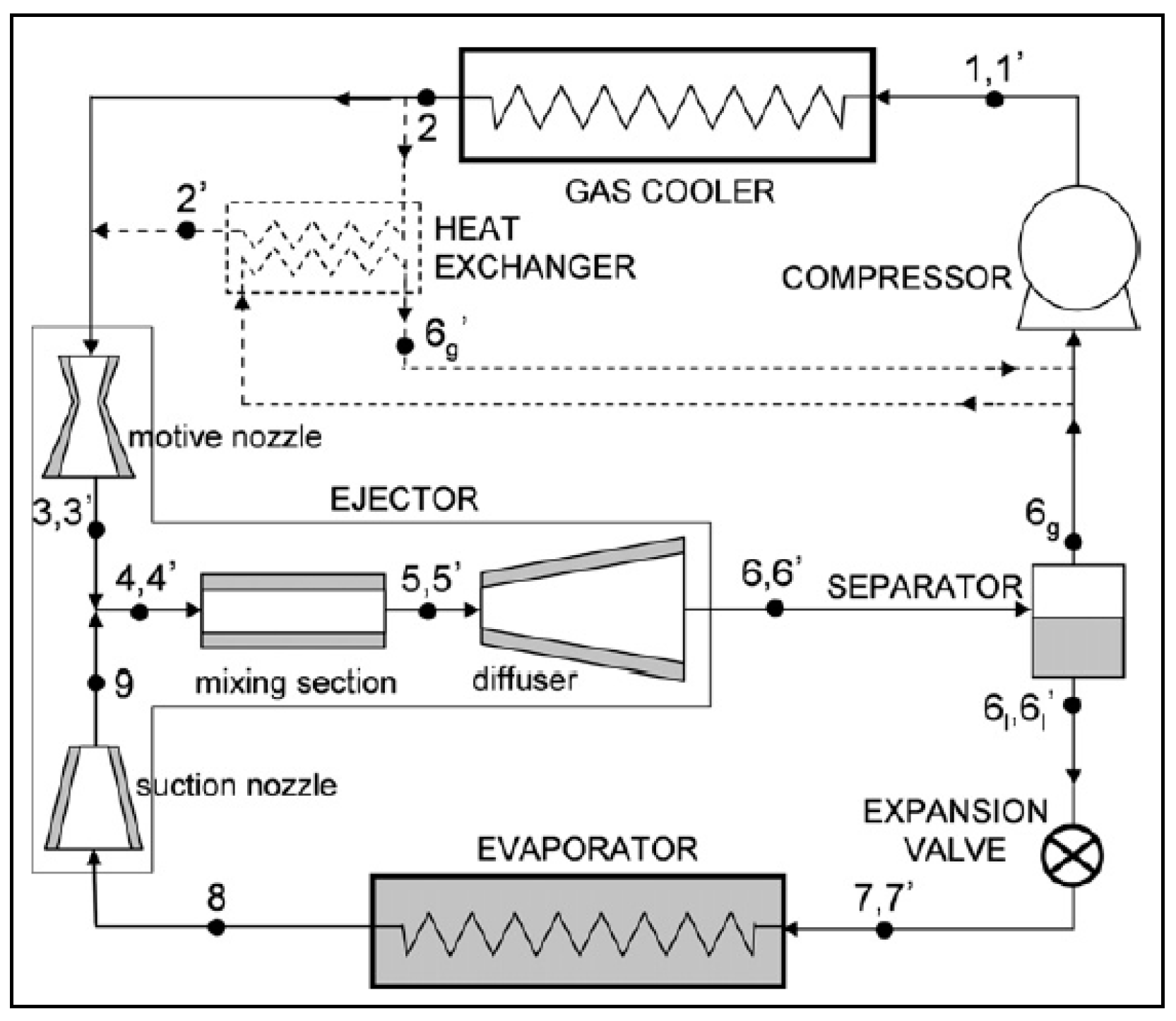

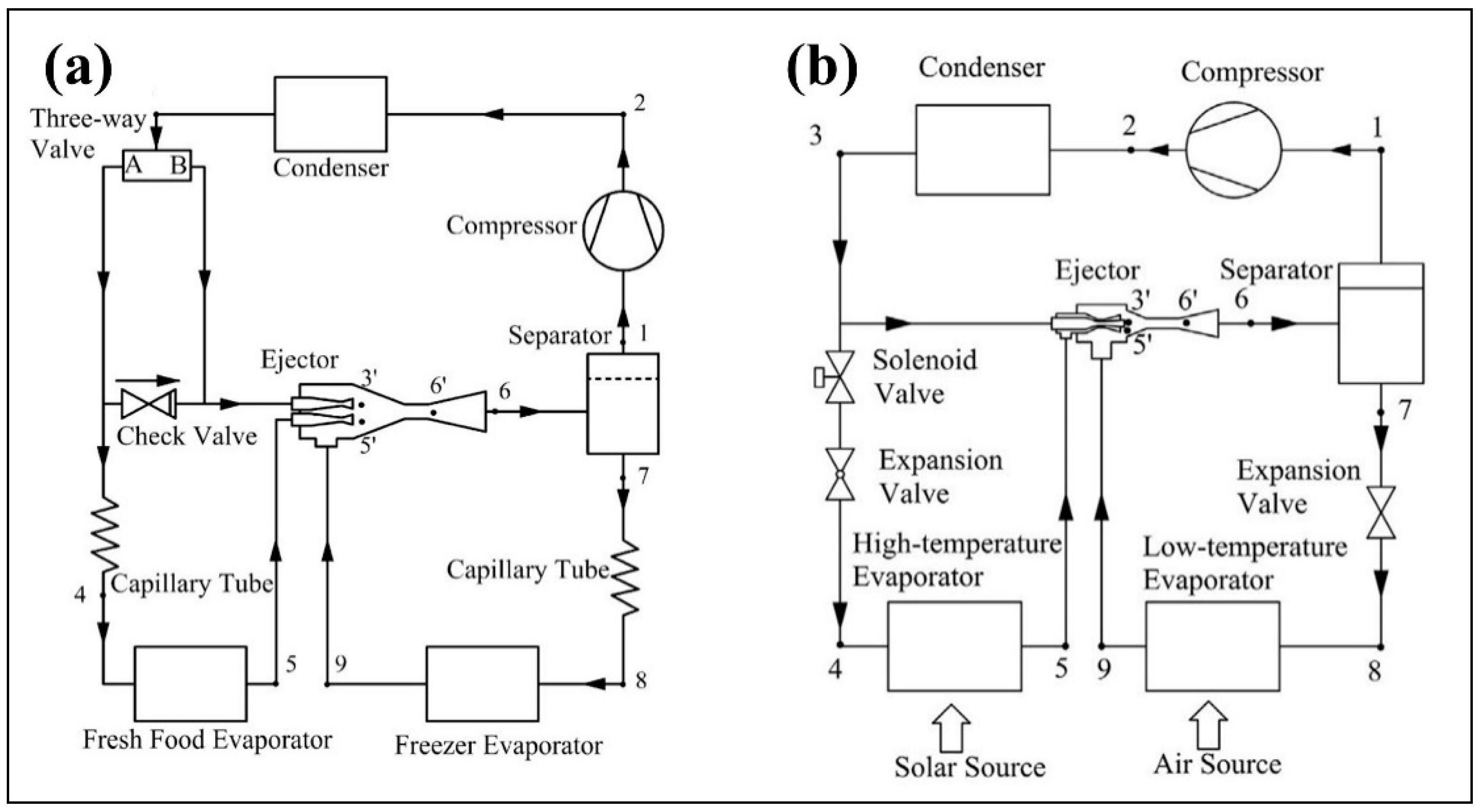

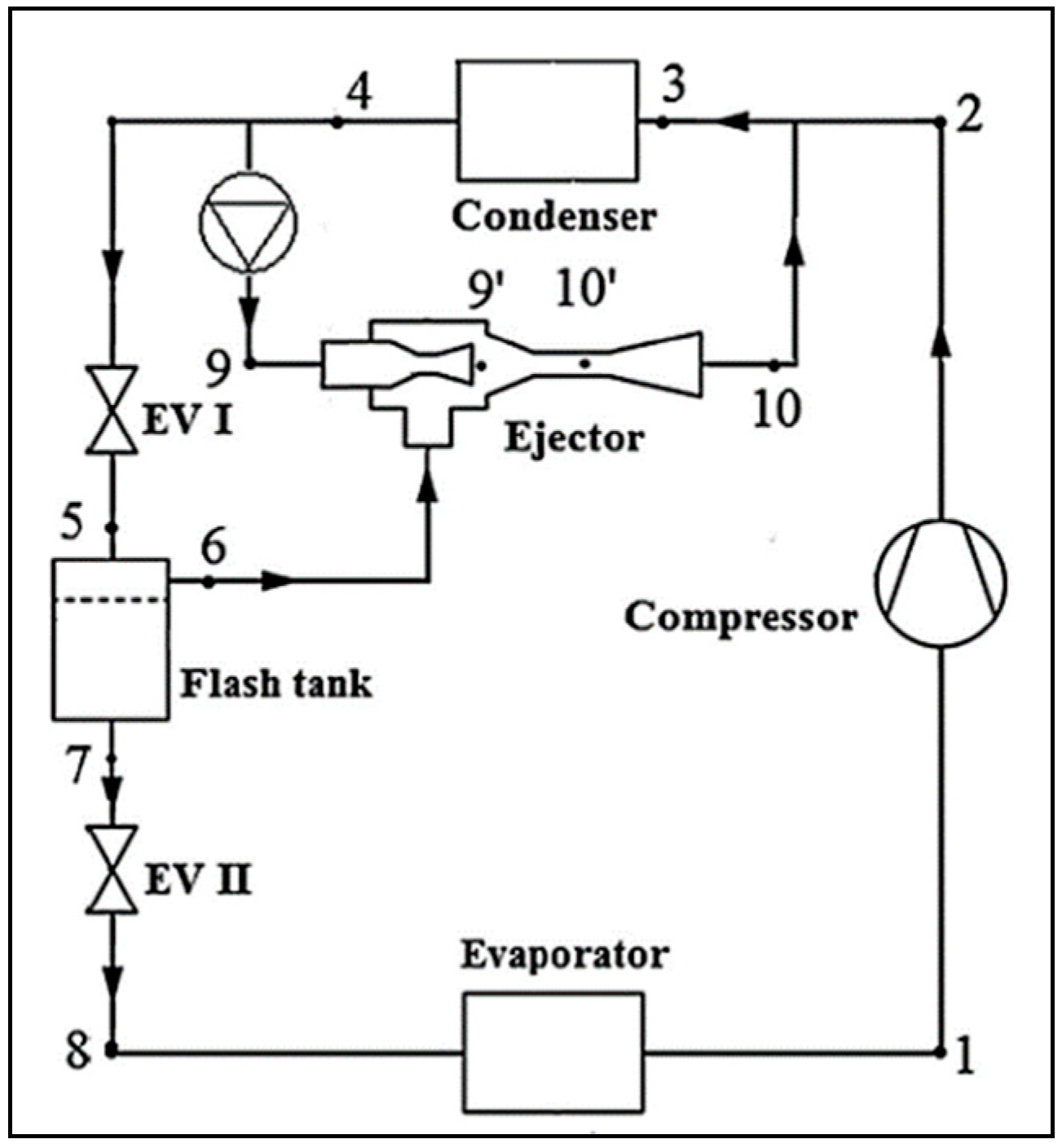
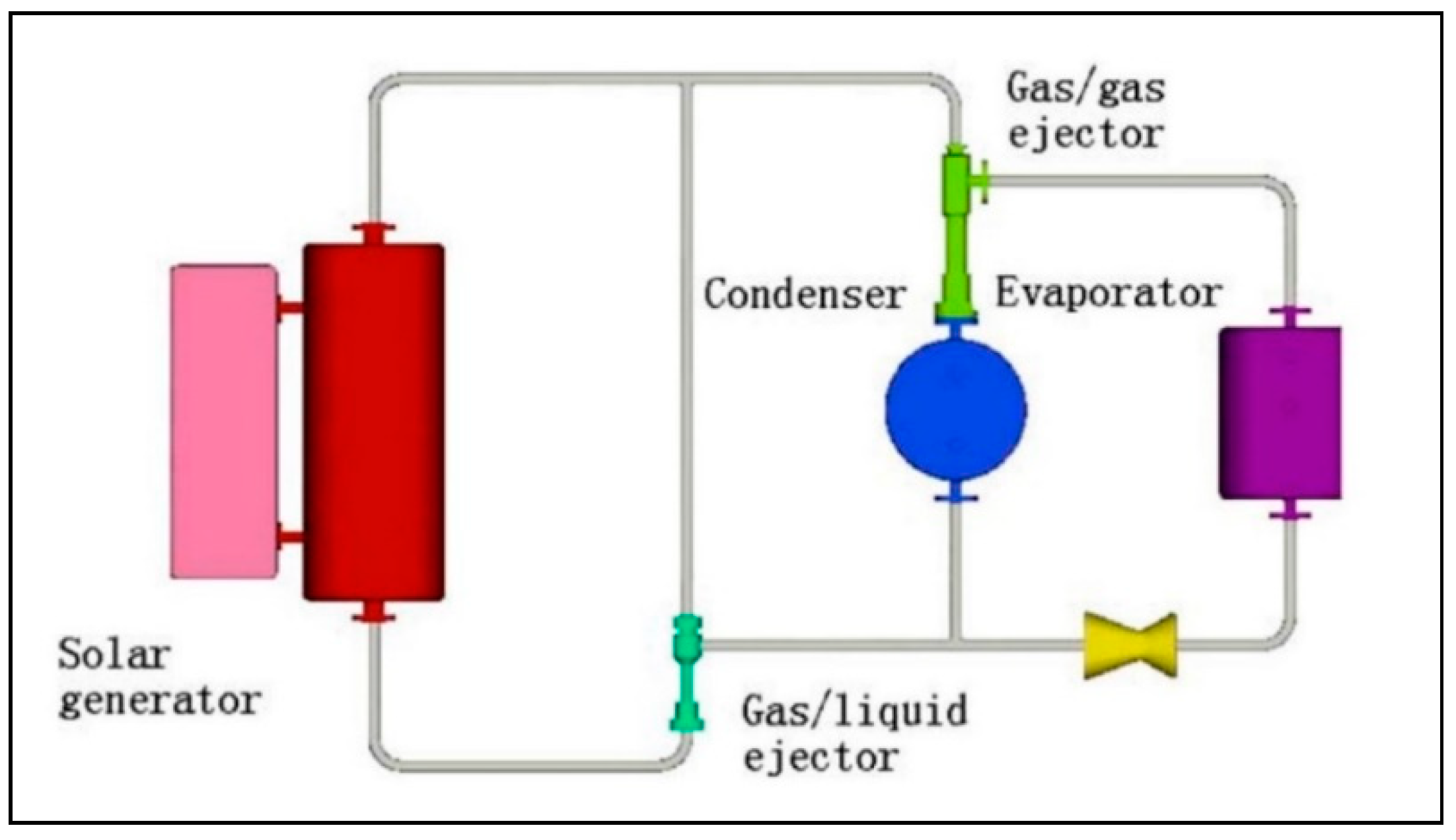
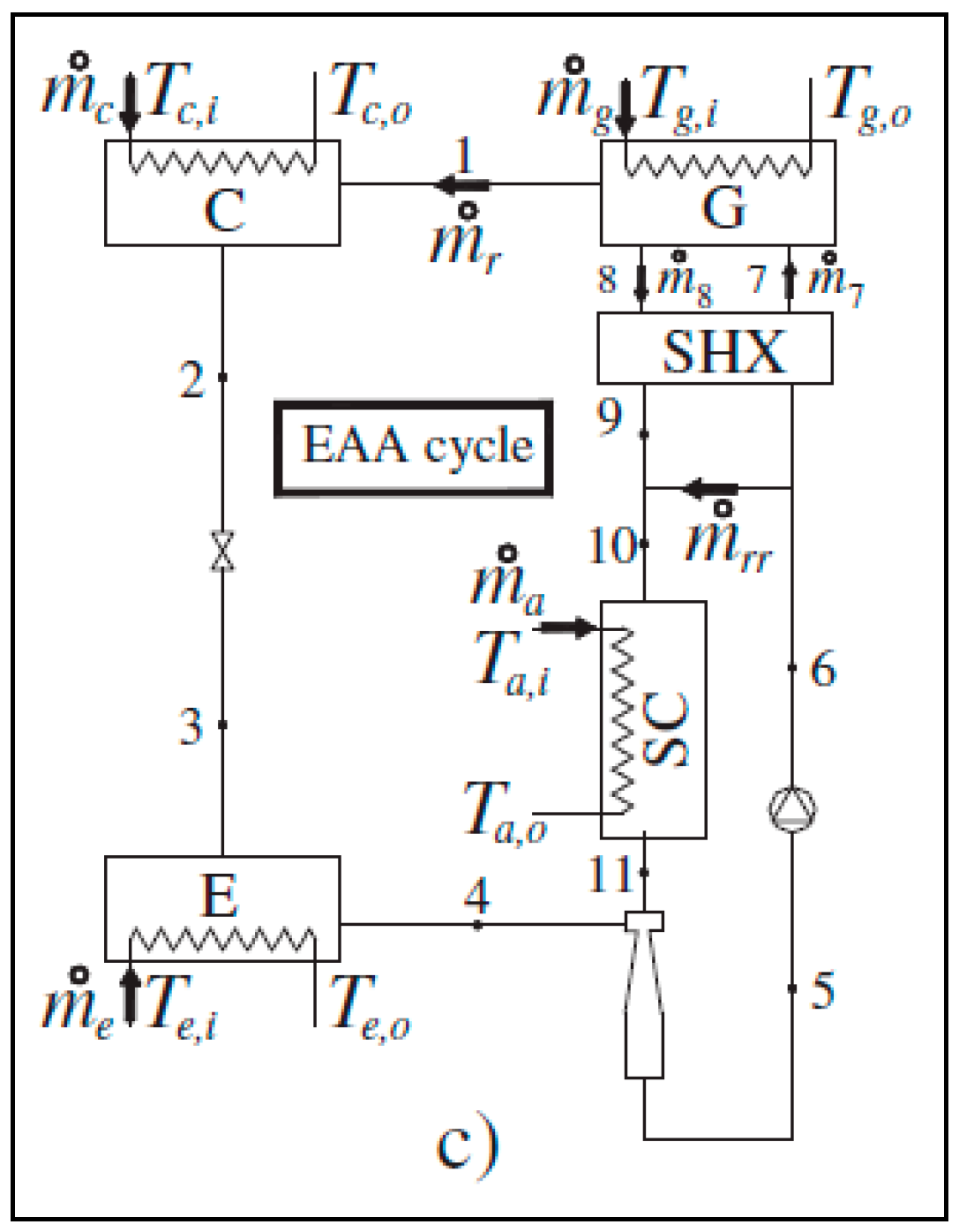
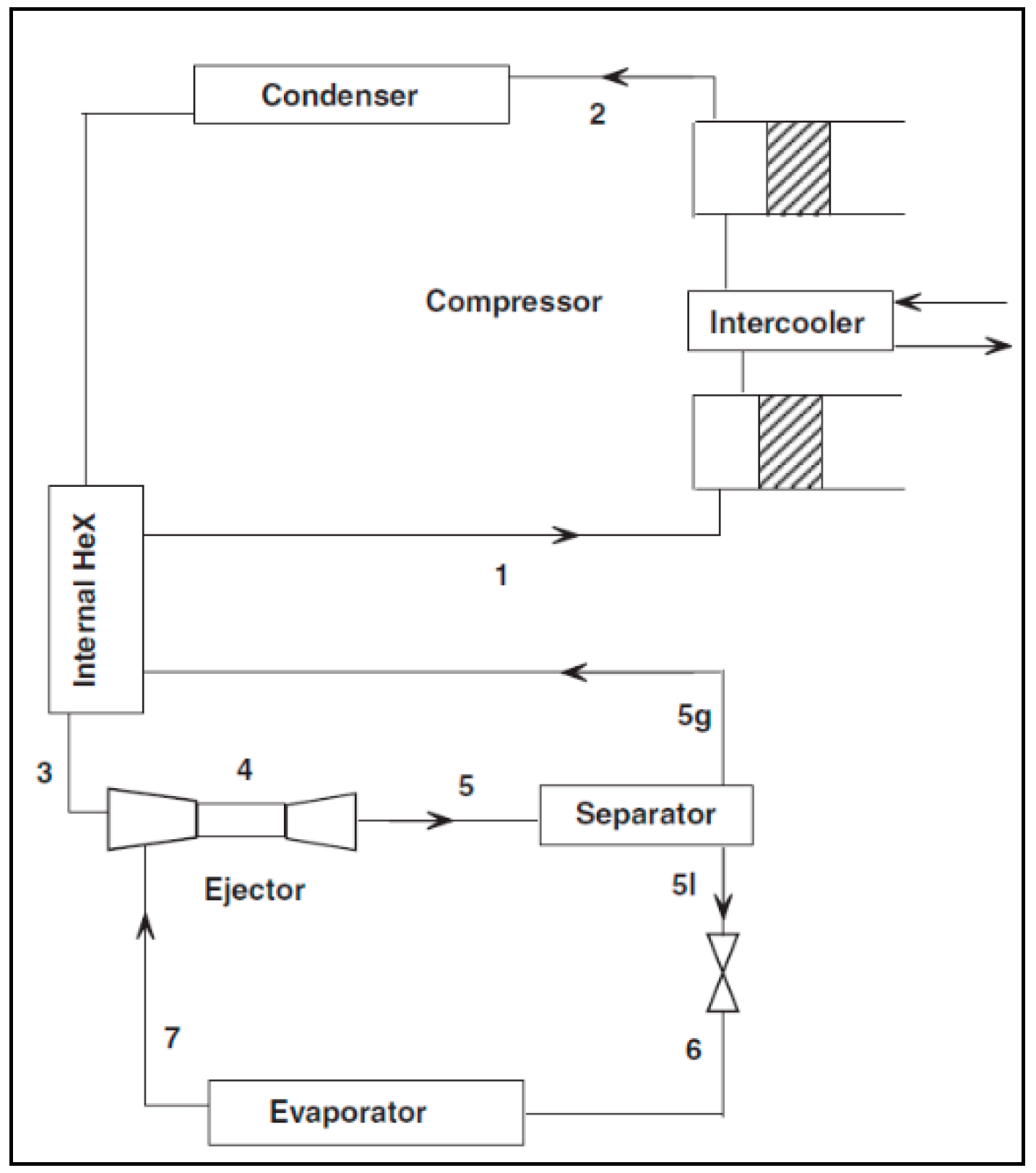
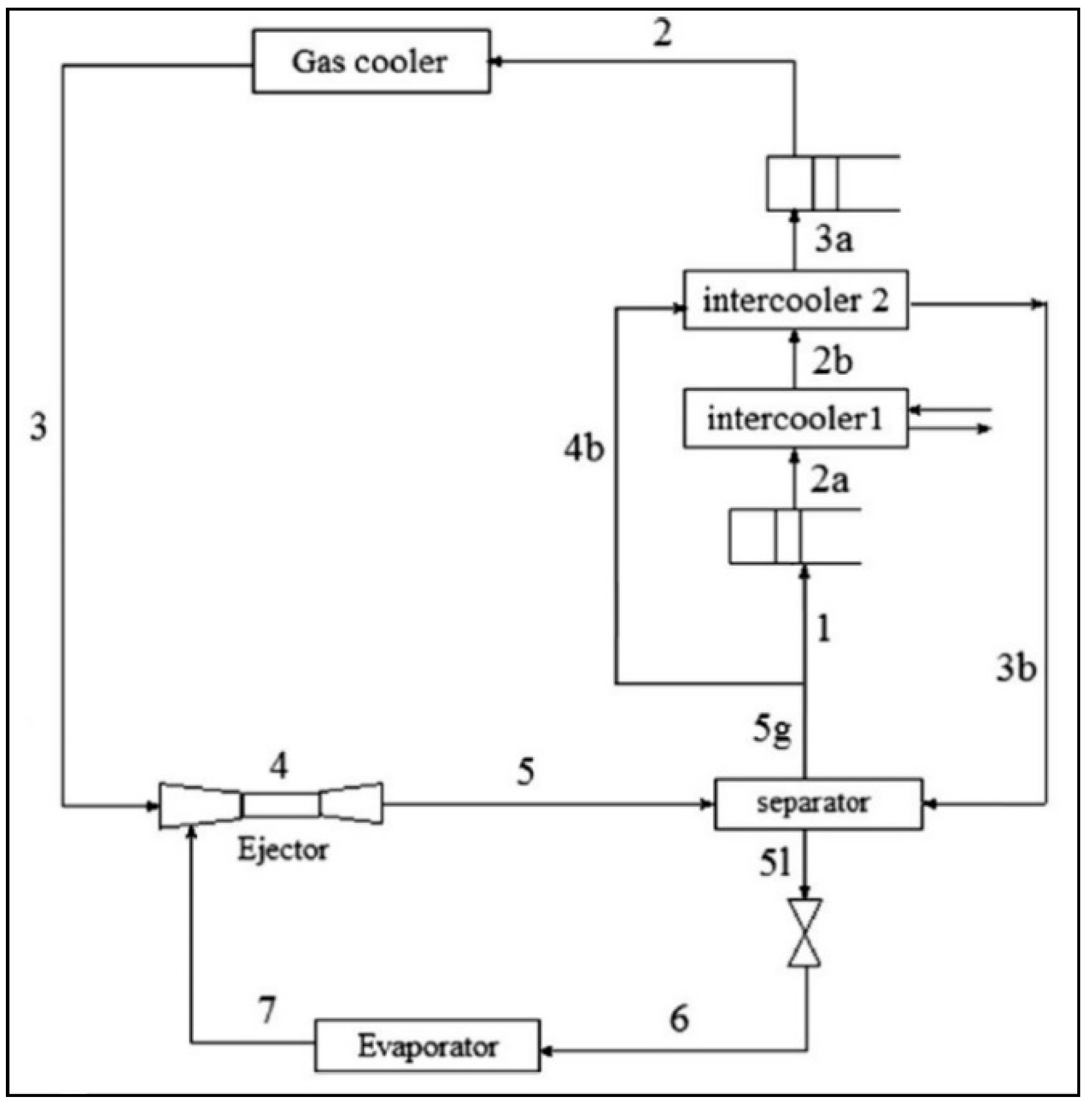
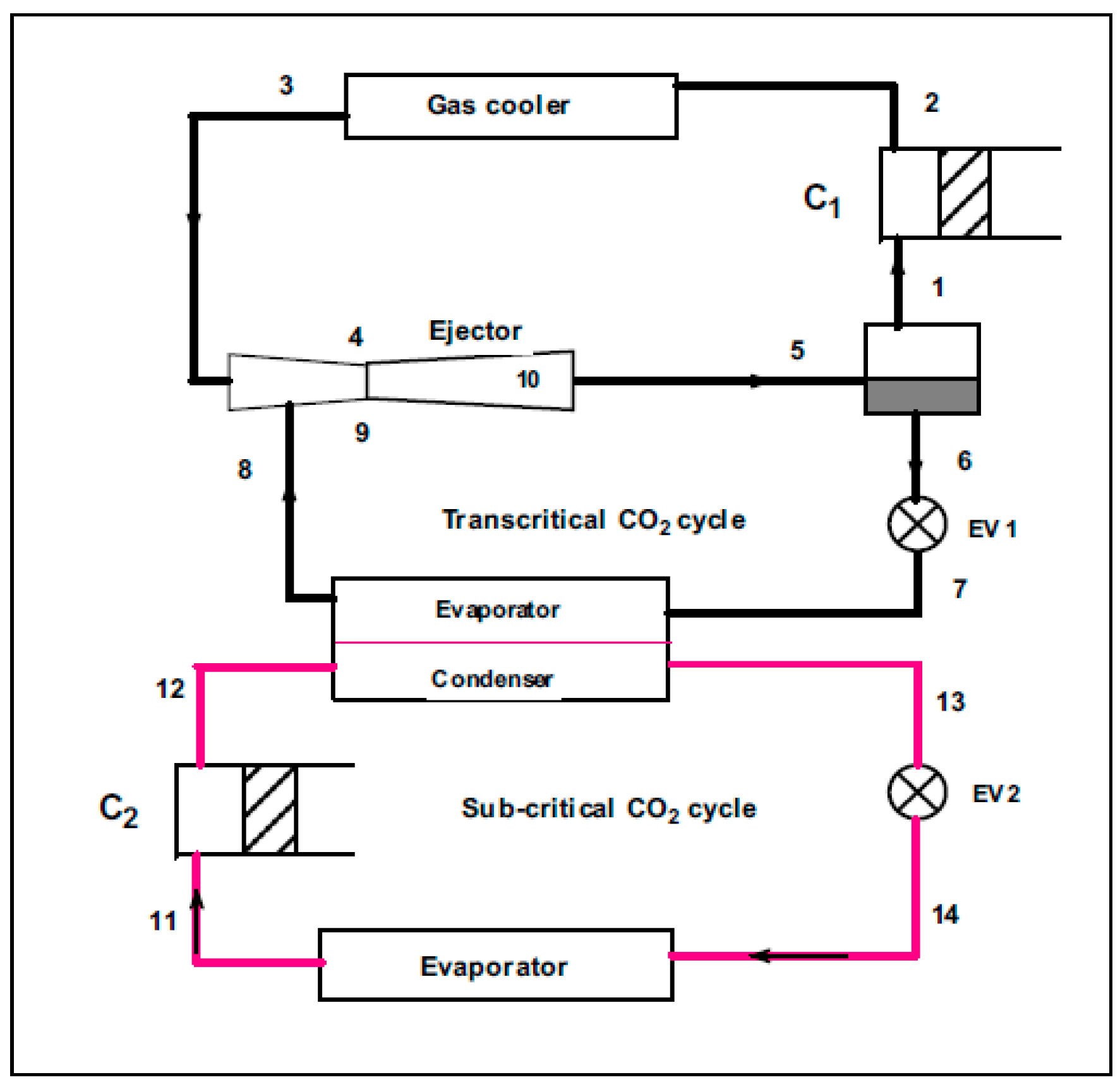
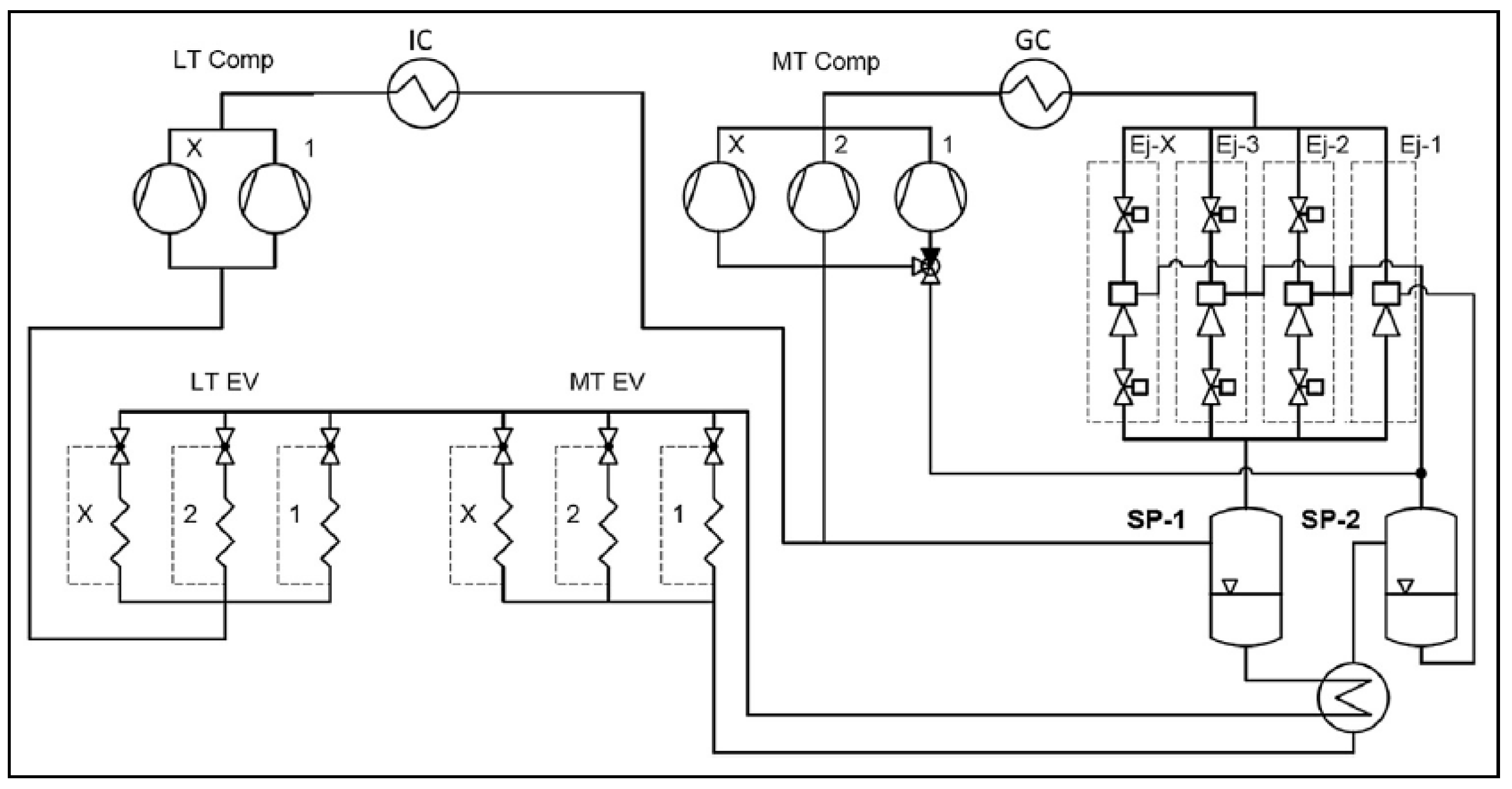

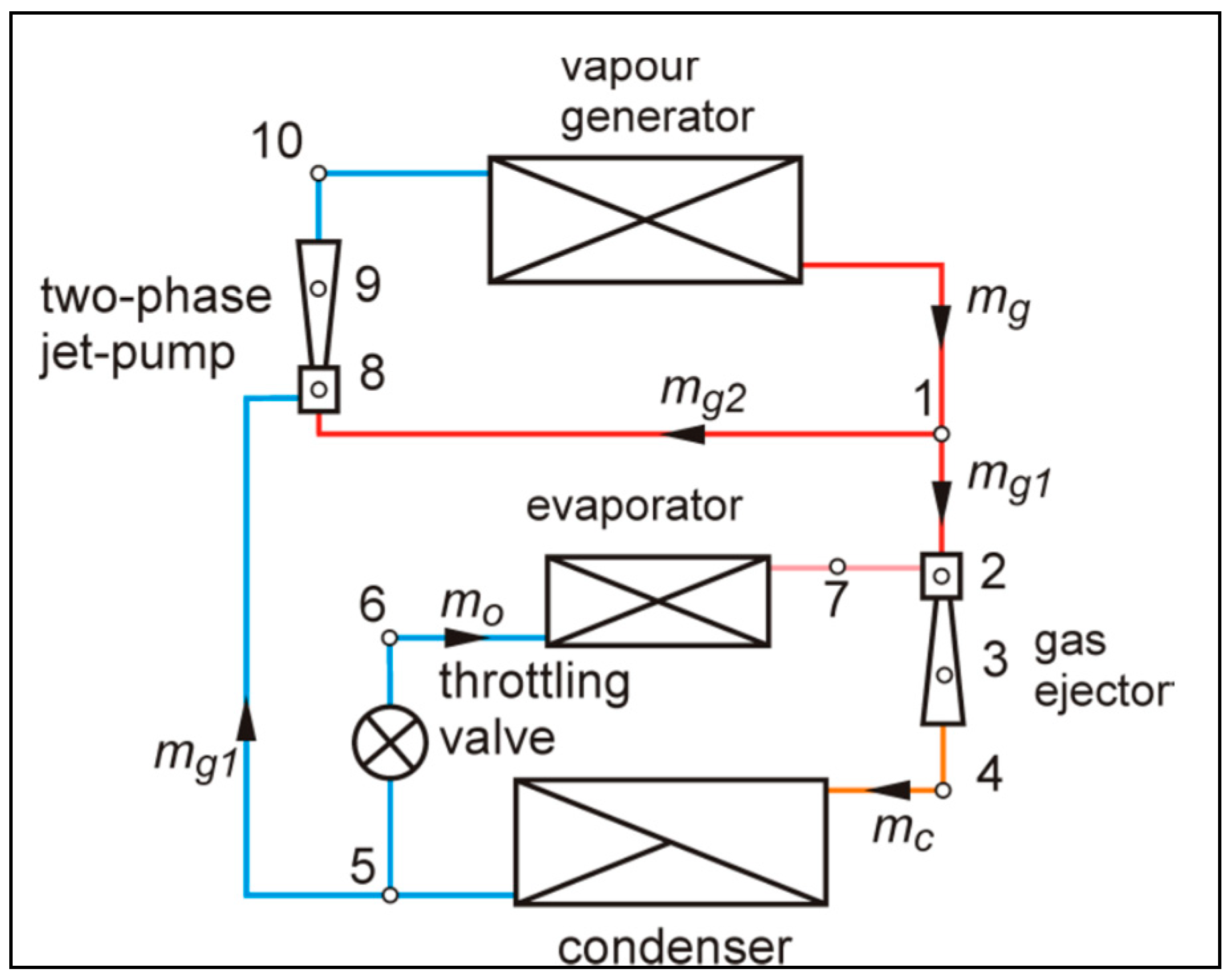
| Author(s) | Fluid(s) | Boundary | Ejector Component Efficiencies | Validation | Remarks |
|---|---|---|---|---|---|
| Kornhauser, 1990 [97] | R11, R12, R22, R113, R114, R500, R502, R717 | Te: −15 °C Tc: 30 °C | -ηp: 0–1 -ηs: 0–1 -ηdif: 0–1 | - | Neglecting losses in mixing process. |
| Menegay and Korhauser, 1994 [102] | R134A | Te: −15 °C Tc: 30 °C ΔTsup: 5 °C ΔTsub: 5 °C | -ηp: 0.75–1 -ηs: 0.9–1 | - | Extension of Kornhauser model, accounting for flow under-expansion and efficiencies. |
| Liu and Groll, 2008 [103] | CO2 | Pgc: 9.5 MPa Tgc: 30 °C Ps: 3.7 MPa | -ηp: 0.986 -ηs: 0.972 -ηdif: 0.882 | -Efficiencies adjusted to reflect the experiments. -Uncertainty <5.9%. | -Correlation of Attou [103], for the speed of sound. -Correlation of Owen [104] for the diffuser. |
| Sarkar, 2010 [33] | Isobutane, Propane, Ammonia | Tc: 35–30 °C Te: −5 to 15 °C | -ηp: 0.8 -ηs: 0.8 -ηdif: 0.8 | - | Correlations of optimal ϕ for each refrigerant in terms of Te and Tc. |
| Kwidinski, 2010 [106] | Water–steam | Steam: 85–130 kg/h and 3.9 bar (ΔTsup: 0–40 °C) Water: 1–6 m3/h | -Nozzle (velocity coefficient): 0.9 -Diffuser (resistance coeff.): 0.1–0.2 | Pb within 15% and Tb 1 K of experiments. | Steam injector model for pressure discharge prediction. |
| Banasiak et al., 2011 [107] | CO2 | Pgc: 9.94–11.1 MPa Pe: 3.68–4.6 Mpa | -Friction factor considered. | Discrepancies less than 5% on ΔPs and mp. | -Hybrid method (0D + 1D). -Delayed Equilibrium Model. |
| Liu & Groll, 2012 [51] | CO2 | Pgc: 4.5 MPa Pe: 3.8 MPa : 0.07 kg/s | -ηp: 0.7–0.9 -ηs: 0.8–0.9 -ηmix: 1 | Predictions within 7.6% on COP and 11.23% on Qe. | Efficiency coefficients correlations provided. |
| Hassanian et al., 2015 [86] | R134a | Pc: 1.4–1.65 MPa Pe: 0.37–0.43 MPa ΔTsup: 14.1–0.6 ΔTsub: 1.38–2.25 | -ηp: 0.5–1 -ηs: 0.5–1 -ηdif: 0.5–1 | Errors on COP less than 3%. | Design procedure using Henry-Fauske to evaluate the critical mass flux. |
| Ameur et al., 2016 [25] | R134a, R410A, CO2 | CO2: Pp: 6.1–9.1 MPa Tp: 21.8–35.8 °C R410A: Pp: 2.9–3.0 MPa Ps: 0.97–1.19 MPa R134a: Pp: 15.3 MPa Ps: 0.35 MPa | -ηp: 0.85 -ηs: 0.85 -ηmix: 0.97 -ηdif: 0.7 | -Error on Pth: 0.21–14.2% -Error on ΔP: 0.63–2.26% (R410a) 0.09–6.14%(R134a) | Design nozzle performed by maximizing the mass flow at the throat. |
| Zhu & Jiang, 2018 [87] | CO2 | Pgc: 8–10.3 MPa Tgc: 32–43 °C Pe: 2.6–4.3 MPa Te: 22 °C. | -ηp: 0.95 -ηmix: -ηdif: 0.9 | Error on mass flow rate: : ±3.5% : ±15% | Primary flow: use of correlation accounts for non-equilibrium when x > 0.65 |
| Author(s) | Fluid(s) | Solver | Turbulence Model(s) | Boundary Conditions | Validation | Remarks |
|---|---|---|---|---|---|---|
| Burlinski et al., 2010 [110] | CO2 | ANSYS Fluent | RNG k-ε | - | High discrepancies in the entrainment ratio prediction. | Homogeneous/heterogeneous model for non-equilibrium effects. |
| Colarossi et al., 2012 [13] | CO2 | Open-FOAM | k-ε | Pgc: 9.5–10.5 MPa Tgc: 42°C, Te: 2 °C | Average error on pressure recovery: 18.6%. | -Assumed non-equilibrium state. -Nucleation delay treatment by HRM. |
| Yazdani et al., 2012 [12] | CO2 | ANSYS Fluent V12.0 | k-ω SST | Pp: 12.33 MPa Tp: 313.1 K Pb: 3.71 MPa Ts: 268.2 K | Within 10% of own data of ω and τ. | Used a non-homogeneous mixture model and NIST Refprop. |
| Yazdani et al., 2014 [111] | CO2 | ANSYS Fluent V12 | k-ω SST | Used data from Nakagawa et al., 2009 [40] | Fairly good concordance simulations-experiments | Phase change on walls and throat. Non-homogenous model + drift flux model for slip of phases. |
| Smolka et al., 2013 [112] | R141B, CO2 | ANSYS Fluent V12.0 | k-ε RNG | Pp:8.4–9.9 MPa Tp:30–36 °C Ps: 3.5–5.1 MPa Ts:6–20 °C Pb: 3.8–5.5 MPa | -Average discrepancies for and : 5.6% and 10.1%. | HEM assumption. Phases in thermal and mechanical equilibrium. |
| Lucas et al., 2014 [113] | CO2 | Open-FOAM | k-ω SST | Tgc: 36.3–29.9 °C Te: 20.7–6.6 °C | Error max on and : 8% and 6.4%. | Assumption of homogeneous equilibrium. |
| Banasiak et al., 2014 [54] | CO2 | ANSYS Fluent | k-ε RNG | Pp: 8–8.5 MPa Tgc: 303 K Ps: 3.5 MPa | Prediction error on and : 7.4% and 13%. | Performance and entropy generation in the flow was proposed for analysis. |
| Palacz et al., 2015 [114] | CO2 | ANSYS Fluent | realizable k-ε | Pp: 4–9.5 MPa Tp: 6–36 °C Ps: 2.7–3.2 MPa Ts: 3–21 °C | Accuracy of mass flow rate predictions are highly variable. | -HEM approach. -Model generally accurate close to saturation line but deteriorates with the temperature decrease. |
| Palacz et al., 2017 [116] | CO2 | ANSYS Fluent | realizable k-ε | Pp: 7.2–9.8 MPa Tp: 26.8–38.7 °C Ps: 2.5–2.9 MPa Ts: −2 + 3 °C | - | -HEM approach. -Genetic algorithm used for optimization (efficiency increase of up to 6%). |
| Haida et al., 2018 [115] | CO2 | ANSYS Fluent | k-ω SST | Pgc: 50–95 bar Pe: −10 to −6 °C. | Error on < 15% (for Pp > 59 bar) | -Improvement of HRM. -Influence of relaxation time on the flow by using a correlation. |
| Baek et al., 2018 [88] | R134a | ANSYS Fluent V16.1.0 | Realizable k-ε | Pp: 10.3–10.7 bar Tp: 40–41 °C Ps: 2.75–3.87 bar Tp: 18–20 °C | Error on ms ≤ 8.47%. | Evaporation-condensation model of the phase transition calibrated with experimental data of Lawrence, 2012 [100]. |
| Author(s) | Fluid(s) | Capacity | Operating Conditions | Performance | Remarks |
|---|---|---|---|---|---|
| Starkman et al., 1964 [90] | Steam–water | Flow rates up to 2.5 lbs/s | Pp < 1000 psia Tp < 580 °F xp < 20% | Weak shocks at overexpansion. | -Convergent–divergent nozzles. -Satisfactory for HEM except near saturation where other models apply. |
| Menegay and Kornhauser, 1996 [80] | R12 | 3.5 kW | -EERC standard operation. | Experiments not conclusive | -Oversized nozzle design, mainly due to non-equilibrium effects. -Bubble formation in size and quantity controlled. |
| Nakagawa, Berana et al., 2008–2009 [41,59,128] | CO2 | 1.3–2 kW | Pp: 9–10 MPa Tp: 37–50 °C | Thick shock in divergent. Increase in amplitude with temperature. | -Experiments on ejector nozzles. -Shock-wave behavior assessment in accordance with geometry and temperature. |
| Wang and Yu, 2016 [53] | R600A | - | Pp: 100–200 kPa Ps: 50–70 kPa xp: 0.313–0.531 | - | Correlations for ejector component efficiency established. |
| Zhu et al., 2017 [67] | CO2 | 5 kW | Pp: 7–9 MPa Tp: 30–35 °C Ps: 3–4.5 MPa. | The expansion angle and ω were measured for varying conditions. | -Visualization study highlighting internal flow structure of CO2. |
| Ameur et al., 2016, 2017 [18,93] | R134A | 5 kW | Pp: 7.7–16.8 bar Tp: 30–56°C ΔTsub: 0.7–55 °C Pb: 3–7.5 bar | The critical mass flow rate significantly depends on the level of the degree of sub-cooling. | -Ejector operated with no induced flow. -Experimental critical flow rate compared with different models. |
| Ameur et al., 2018 [46] | R134A | 5 kW | Pp: 8.8–14.9 bar Tp: 30–56°C ΔTsub: 0.2–45 °C Ps: 3–4.44 bar ΔTsup: 6–13 °C Pb: 3.1–4.8 bar | Performance curves established for Pp: 14.9 bar and Ps: 3–4.4 bar. | -Ejector operated with induced flow. -Pressure variation inside the ejector monitored. |
| Author(s) | Fluid | Operating Conditions | Performance | Remarks |
|---|---|---|---|---|
| Li et al., 2014 [140] | R1234yf | Tc: 30–55 °C Te: −10 to +10 °C | -ΔCOP, up to 13%, ΔQe up to 12% (at Tc = 50 °C, Te = 5 °C, and same ΔP in suction nozzle.) -An optimum ΔP in suction nozzle exist for maximized performance. | R1234yf EERC has a better performance than R134a. |
| Zhao et al., 2015 [141] | Mixture R134a/R143a | Tc: 30–50 °C Te: −15 to −10 °C | With mixture 0.9/0.1, ΔCOP = 10.47% (compared to system with pure R143a). | EERC using zeotropic mixtures, fluid composition and working conditions effects investigated. |
| Luo, 2017 [142] | R32 | Tc: 45 °C Te: −25 to +5 °C | -ΔCOP ≈ 4.3%. -Expected improvement in COP of 8.5% with addition of IHX. | EERC with injecting oil into the compressor to approach a more isothermal compression process (oil-flooded compression cycle). |
| Rodríguez-Muñoz et al., 2018 [143] | R134a, R1234ze(E), R290 | Air-conditioning conditions | IHX presence promotes a decrease in COP. | Effects of heat recovery by IHX in EERC |
| Khosravi et al., 2018 [136] | R134A R407C R410A | Tc: 50–63 °C Te: 20 °C Q: 1631 kW | Fuel consumption reduced by 22% and total cost of EERC system 15.2% less than conventional refrigeration with IHX. | -Application of a large scale industrial EERC for process water-cooling in an oil refinery. -Energy, exergy and economic analyses showed EERC as the best choice. |
| Author(s) | Operating Conditions | Performance | Remarks |
|---|---|---|---|
| Deng et al., 2007 [146] | Pgc: 7.5–12.5 MPa Te: 0–10 °C | -ΔCOP = 18.6%. ΔQe = 8.2% (with IHX). -ΔCOP = 22%, ΔQe = 11.5% (without IHX). | Exergy analysis showed that EERC greatly reduces the throttling losses. |
| Fangian and Yitai, 2011 [149] | Pgc: 8–9.2 MPa Tgc: 312–318 K Te: 267–290 K ΔTsub: 5 K | Ejector instead of throttling valve can reduce 25% of exergy losses and increase COP by 30%. | Effects of working conditions on COP and exergy loss. |
| Sarkar and Bhattacharyya, 2012 [154] | Tgc,w,in: 30–40 °C Te,w,in: 25–35 °C : 0.7–2 kg/min Qev: 1.5–2.3 kW Qgc: 2.8–4 kW | -The effect of on system performances is more pronounced compared to . -The effect of Tgc,w,in is more significant compared to Te,w,in. | Theoretical and experimental investigations on the water-side operating conditions of heat pump for water cooling and heating. |
| Zhang et al., 2013 [150] | Pgc: 8.5–13 MPa Tgc: 40–50 °C Te: 0–10 °C | IHX inclusion in EERC: -increases ω and ejector efficiency. -Pressure recovery decreases under the same gas cooler pressures. | IHX is only applicable with low ejector isentropic efficiencies or high gas cooler exit/evaporator temperatures for the EERC system from the view of energy efficiency. |
| Zhang and Tian, 2014 [151] | Pgc: 8.5–11 MPa Tgc: 40–50 °C Te: 0–10 °C | -ΔCOP up to 45%. -Exergy loss reduction up to 43%. | ΔP suction nozzle impact on ω is small but exist an optimum value for which COP and recovered pressure are maximized. |
| Zheng et al., 2015 [152] | Pgc; 8–9 MPa Pe: 3.2–3.6 MPa ω: 0.48–0.57 | Pressure predictions in gas cooler, evaporator and separator within 1.8%, 4.2% and 6.7%, respectively. | The dynamic behaviors of the EERC system undergoing the change of expansion valve opening and ejector area ratio are predicted by the developed model. |
| Author(s) | Fluid | Operating Conditions | Performance | Remarks |
|---|---|---|---|---|
| Pottker et al., 2010 [163] | R410A | Tc: 40–60 °C Te: 0–15 °C Qe: 1.5–2.5 kW | -ΔCOP up to 14.8% over conventional system. -ΔCOP up to 8.4% over flash gas bypass system. -ω: 0.62–0.71, τ: 1.04–1.11. | The two benefits effects of EERC system (flash gas separation and work recovery) were investigated and quantified. |
| Ersoy and Bilir, 2014 [162] | R134a | Tc: 52–60 °C Te: 10 °C Qe: 4.47 kW | -ΔCOP: 6.2–14.5% over conventional, depending on operating conditions. -ω: 0.63–0.65, τ: 1.063. | Under same external conditions, overall ΔP (in the evaporator particularly) is higher in conventional cycle. |
| Hu et al., 2014 [29] | R410A | Pc: 1.9–2.4 MPa Pe: 1.05–1.28 MPa Qe: 4.2 kW | -ΔCOP: up to 9.1% over conventional system. -ω: 0.58–0.78 | Adjustable ejector investigated under different conditions. |
| Bilir-Sag et al., 2015 [55] | R134a | Tc: 40 °C Te: 5 °C Qe: 4.5 kW | Over a conventional system: -ΔCOP up by 7.34–12.24%. -Exergy efficiency up by 6.6%–11.24%. -ω: 0.73–0.83 | The irreversibility and efficiency of each cycle component determined and compared with those of a vapor compression refrigeration system. |
| Pottker and Hrnjak, 2015 [134] | R410A | Tc: 40–60 °C Tsink: 38–52 °C Te: 0–15 °C Tsource: 10–27 °C | -ΔCOP: 12.2–19.2%. -ω: 0.62–0.71, τ: 1.04–1.11. | Work recovery and liquid-fed evaporator in EERC separately quantified. |
| Wang and Yu, 2016 [30] | R600a | Pp: 1–2.6 bar xp: 0.3–0.6 Ps: 0.4–0.7 bar | ω: 0.18–0.33, τ: 1.01–1.30. | τ increases and ω decreases with increasing the quality of the primary fluid. |
| Jeon et al., 2018 [38] | R410A | Pc: 24–31 bar Pe: 10–14 bar Qe: 7.5 kW | -ΔCOP: 7.5% over the base line cycle. -ω: 0.6–0.95, τ: 1.02–1.07. | Effects of ejector geometries on the performance of an ejector expansion air conditioner. |
| Author(s) | Operating Conditions | Performance | Remarks |
|---|---|---|---|
| Nakagawa et al., 2011, [36,165] | Pgc: 9–10.5 MPa Tgc: 41–47 °C Te: 0–8 °C Qe: 0.4–2.7 kW | - ΔCOP: up to 27% over base case when IHX is properly sized. - ω: 0.1–0.7, τ: 1.04–1.13 | -Effect of IHX size on EERC performance. -Effect of the mixing length on the performance investigated. |
| Banasiak et al., 2012 [37] | Tgc: 30–70 °C Te: 20 °C Qgc: 5–13 kW | -ΔCOP: 8%. -ω: 0.41–0.7. | Effects of different ejector geometries on performance were examined. |
| Lucas and Koehler, 2012 [169] | Pgc: 71–103 bar Tgc: 30–40 °C Pe: 26–34 bar Te: −10 to −1 °C | -ΔCOP: 17%. -ω: 0.38–0.65, τ: 1.05–1.14. | Investigation of the working conditions on the performance. |
| Minetto et al., 2013 [172] | Pgc: 100 bar Tgc: 35 °C, Te: 0 °C Qgc: 5 kW | -ΔCOP: 7.5–23.3%. -ω: 0.8–1.6, τ: 1–1.143. | Technological issues related to lubricant recovery were faced. |
| Lee et al., 2014 [167] | Tgc: 30–40 °C Te: 27 °C Qe: 3–5.7 kW | Depending on converter frequency adjustment, -ΔCOP: 6–9%. -ΔQ: 5%. | ω and the temperature of external fluid in the gas cooler were among the main controlling factors. |
| Haida et al., 2016 [175] | Tgc: 26–36 °C Pe: 28 bar Te: −8 °C Qe: 46 kW | -COP improved up to 7% over parallel compression system. -ω: 0.15–0.4, τ: 1–1.4 | -Performance of multi-ejector expansion work recovery module compared to parallel compression system. |
| Boccardi et al., 2017 [81] | Pgc: 80–100 bar Tgc: 40–60 °C Pe: 20–30 bar Te: −5 to 12 °C Qgc: 29–36 kW | -ΔCOP: 13.8%. -ΔQgc: 20%. when proper configuration of multi-ejector is used. -ω: 0.35–0.52, τ: 1.06–1.14. | -Heat pump system with multi-ejector pack and IHX for space heating. -There is a threshold value of the ambient temperature to switch from an ejector to another one in order to maximize the performance. |
| He et al., 2017 [42] | Pgc: 90–114 bar Nozzle throat area: 0.638–1.217 m2 | A controller based on a dynamic model tracking the optimal gas cooler pressure in real time to increase the system performance. | Improving the operating performance of the transcritical CO2 EERC by controlling the nozzle throat area. |
| Zhu et al., 2018 [133] | Pgc: 81–121 bar Tgc: 35–55 °C Pe: 50 bar (Tair: 22 °C), Qgc: 5 kW Tw,in: 20 °C, Tw,out: 50–90 °C | -COP improvement of 10.3% over the basic cycle. -ω: 0.5–0.9, τ: 1.1. | Effects of working conditions on the performance of transcritical CO2 ejector-expansion heat pump water heater system. |
| Author(s) | Application | Fluid | Operating Conditions | Performance | Remarks |
|---|---|---|---|---|---|
| Balamurugan et al., 2008 [77] | Liquid–gas contactor | Air-water | -Fixed water- and airflows. -Ejector outlet open to the atmosphere. | Optimum area ratio for highest liquid rate of entrainment was determined numerically. | -Theory and experiments of gas—liquid ejectors for use as contactors in industrial and process applications. - Validated CFD model. |
| Dokandari et al., 2014 [192] | Ejector expansion cascade absorption cycle with two-phase ejector in each loop. | CO2/NH3 | Tc: 30–40 °C, Te: −55 to −45°C, Qe: 175 kW | With respect to conventional cycle: -ΔCOP up by 7%. - Exergy destruction reduced by 8%. | Experiments required for validation and economic analysis for cost effectiveness, considering additional hardware and controls. |
| Zhu et al., 2014 [179] | Two-phase ejector with 2 nozzles in a vapor-compression cycle for solar assisted air-source heat pump systems | R410A | Tc: 40 °C, Te1: −5 °C, Te2: −18 °C, ΔTsup: 0°C | Improvement over conventional EERC: -ΔCOP: 4.6–34%. -ΔQ: 7.8–51.9%. | -Good potential of using simultaneously two energy sources for heat pumps. -Need to be experimentally validated |
| Boumaraf et al., 2014 [176] | EERC with 2 evaporators | R134a, R1234yf | Tc: 40 °C, Te1: −5 °C, Te2: 0 °C | ΔCOP: more than 17% over conventional cycle for both refrigerants. | R134a performance somewhat higher than for R1234yf but improvement is comparable especially at high TC. |
| Wang et al., 2014 [180] | Modified EERC | R600A | Tc: 40 °C, ΔTsub: 10 °C, Te1: −5 °C, Te2: −25 °C | -ΔCOP: 11.4%. -ΔQ: 22%. | Application of EERC concept to refrigerator-freezers. |
| Unal and Yilmaz, 2015 [177] | EERC with two evaporators (air-conditioner for buses) | R134a | Tc: 48–48.8 °C, Te1: 1.3–6.3 °C Te2: −1.6–7.2 °C, Q: 2–2.52 kW | -ΔCOP: less than 15%. -ω: 0.06–0.59. | The heat transfer surface areas of the condenser and evaporator can be reduced 5% and 51%, respectively. |
| Liu et al., 2015 [178] | A modified vapor refrigeration cycle with a two-phase ejector for applications in domestic refrigerator freezers | R290/ R600A | Tc: 35–55 °C, ΔTsub: 5–30 °C Te: −35 to −25 °C, ΔTsup: 10 °C mcomp: 1 g/s | -ΔCOP: 16.7%. -ΔQe: 34.9%. -Exergy efficiency: 6.71%. -Exergy destruction reduced by 24.4% | -Using zeotropic mixture was investigated in terms of performances. -An optimal mixture composition can further be found for maximizing system performance. |
| Xing et al., 2015 [182] | Two-phase ejector specifically assigned to provide mechanical sub-cooling to vapor-compression refrigeration cycle. | R410A, R290 | Tc: 45 °C, Te: −40 °C to −10 °C | R410a: ΔQ: 11.7%. ΔCOP: 9.5%. R290: ΔQ: 7.2%. ΔCOP: 7%. | Need for experiments to confirm theoretical predictions for the real potential of the system and under which conditions. |
| Goodarzi et al., 2015 [189] | Transcritical two-stage mechanical-EERC system with multi-cooling and IHX. | CO2 | Pgc: 80–120 bars Tgc: 36–44 °C, Te: −30 to −5 °C | Potential increase of COP, in particular for low gas cooler pressures | The model used was validated by data from similar setup, without IHX. |
| Bai et al., 2015 [193] | Vapor-injection in transcritical ejector heat pump cycle for cold climates. | CO2 | Pgc: 8.55 MPa Tgc: 35–50 °C, Te: −25 to −5 °C | ΔCOP up to 7.7%, ΔQgc up to 9.5% ω: 0.75–1.13, τ: 1.06–1.12 | -Vapor injection with sub-cooler for lower discharge temperature and higher capacity. -Exergy destruction showed gas cooler and evaporator as main contributors. |
| Bai et al., 2015 [194] | CO2 transcritical refrigeration cycle with bi-evaporator and with two-stage ejector. | CO2 | Tgc: 35–50 °C, Te1: −5 to 5 °C Te2: −35 to −15 °C | Improvement over conventional dual-evaporator cycle: -ΔCOP: 37.61% -Exergy efficiency: 31.9%. | Need for experiments to confirm theoretical predictions for the real potential of the system and under which conditions |
| Smirciew et al., 2015 [200] | Two-phase injector as a feeding pump of the vapor generator in ejector refrigeration cycle. | Isobutane | Pp: 1.08–1.64 MPa; Tp: 70–90 °C Ps: 0.404 MPa; Ts: 15 °C (liquid) ΔTsub: 15 °C | ω: 18–28, τ: 2.7–4.2 (condensation shock wave captured by calculations) | The replacement of the mechanical pump by a two-phase injector inside a conventional supersonic ejector cycle system leads to decrease the COP of the system. |
| Sarkar, 2017 [181] | Multi-evaporator EERC systems. | R32, Propane | Tc: 40 °C Te: 5, −20, −40 °C (multi-level evaporators) | ΔCOP: 20% over basic valve expansion two-stage mechanical cycle, 117% over single-stage and 67% over EERC. | More studies (theory and experiments) for data on the potential of these concepts are needed. |
| Lawrence and Elbel, 2018 [196] | The ejector recirculation cycle and the conventional EERC. | R410A, CO2 | Qe: 1 kW R410A, Tc: 45 °C, ΔTsub: 1 K CO2 Pgc: 100 bar Tgc: 44 °C | Ejector recirculation cycle expected to perform more favorably at lower ambient temperature or with an ejector with low-pressure lift. | -Effect of microchannel heat exchangers design and operation on ejector cycles. -CO2 ejector cycle performance is much less sensitive to evaporator design. |
| Author(s) | Application | Fluid | Operating Conditions | Performance | Remarks |
|---|---|---|---|---|---|
| Man et al., 2007 [201] | Refrigeration cycle with two-phase ejector for recirculation (ERC) | R404A | Tc: 50 °C Te: −10 to 0 °C | -ΔCOP: 10% compared to conventional cycle. -ω: 0–0.98. | Vapor quality and refrigerant mass flow rate increase at the evaporator inlet. |
| Lawrence and Elbel, 2012, 2014 [101,161] | Refrigeration cycle with two-phase ejector without separator and with two evaporators (COS). | R134a, R1234yf | Tp: 45 °C Ts: 6–12.5 °C | -ΔCOP: 10% for R134a 12% for R1234yf -ω: 0.05–0.7. | COS cycle had a slight performance advantage over typical EERC. |
| Minetto et al., 2014 [209] | Three Evaporators overfeeding by means of ejector recirculator. | CO2 | Tamb: 16 °C Te: −6 °C ΔTsup: 6 K Qe1,2: 3.1 kW Qe3: 5.5 kW | The compressor energy saving was about 13% of the case of thermostatic control. | Method for feeding flooded evaporators arranged in parallel in CO2 (subcritical) plants. |
| Banasiak et al., 2015 [208] | Multi-ejector compressors system, typical supermarket application. | CO2 | Tgc: 35 °C, Te1: −3 °C Te2: −30 °C Capacity: 70 kW (at MT) 23 kW (at LT) | At specific subcritical condition, ΔCOP: 9.8% Δξ: 13.1% | Obtained low efficiency due to system design for performance mapping, nor representative of a complete supermarket installation. |
| Lawrence and Elbel, 2016 [204] | ERC system. Refrigeration cycle with two-phase ejector for recirculation. | R410A | Tc: 35 °C Te: 4–9 °C Qe: 1 kW | -ΔCOP up to 16% for the ERC system and 9% with the standard EERC. -ω: 0.7–1.1, τ: 1.05. | The COP of each tested cycle is very dependent on evaporator design. |
| Li et al., 2017 [83] | A falling-film water chiller with ejector for recirculation. | R134a | Tamb: 35 °C Te: 4.8 °C Qe: 55 kW | Evaporating capacity increases 9.5% with appropriate liquid recirculating ratio (1.21). | Using liquid recirculating ratio larger than 1.2 is not significant for enhancing the performance of falling-film heat transfer. |
| Jeon et al., 2017–2018 [202,210] | COS cycle. | R600a | Pc: 500 kPa Pe: 70 kPa Qe: 0.3 kW | -ΔCOP: 6.8–11.4% over the baseline cycle. -ω: 0–0.6, τ: 1–1.09. | Effects of operating conditions and ejector geometries on the performance of a small-sized household refrigeration cycle. |
| Kim et al., 2017 [211] | COS cycle. | R410A | Pc: 25–31 bar Tc: 41–51 °C Pe: 10.2–14.6 bar Te: 8–20 °C Qe1,2: 12 kW | -ΔCOP: 14% over the baseline cycle (at ω = 0.1). -ω: 0–0.6, τ: 1–1.2. | No improvement of the performance was noted for an entrainment ratio larger than 0.3 |
| Bai et al., 2018 [205] | Two-phase ejector auto-cascade refrigeration system. | R134a/ R23 | Tamb: 15–27 °C Te: −50 to −40 °C Qe: 100 W | -ΔCOP: 9.6% and Δξ: 25.1% over the conventional cycle. -ω: 0.5–1.3, τ: 1.19–1.22. | The refrigerant R134a/R23 with the optimal mass fraction ratio of 0.70/0.30 was proposed to get the maximum system exergy efficiency |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Aidoun, Z.; Ameur, K.; Falsafioon, M.; Badache, M. Current Advances in Ejector Modeling, Experimentation and Applications for Refrigeration and Heat Pumps. Part 2: Two-Phase Ejectors. Inventions 2019, 4, 16. https://doi.org/10.3390/inventions4010016
Aidoun Z, Ameur K, Falsafioon M, Badache M. Current Advances in Ejector Modeling, Experimentation and Applications for Refrigeration and Heat Pumps. Part 2: Two-Phase Ejectors. Inventions. 2019; 4(1):16. https://doi.org/10.3390/inventions4010016
Chicago/Turabian StyleAidoun, Zine, Khaled Ameur, Mehdi Falsafioon, and Messaoud Badache. 2019. "Current Advances in Ejector Modeling, Experimentation and Applications for Refrigeration and Heat Pumps. Part 2: Two-Phase Ejectors" Inventions 4, no. 1: 16. https://doi.org/10.3390/inventions4010016
APA StyleAidoun, Z., Ameur, K., Falsafioon, M., & Badache, M. (2019). Current Advances in Ejector Modeling, Experimentation and Applications for Refrigeration and Heat Pumps. Part 2: Two-Phase Ejectors. Inventions, 4(1), 16. https://doi.org/10.3390/inventions4010016




