Atmospheric Muon Flux Measurement near Earth’s Equatorial Line
Abstract
1. Introduction
2. Muography Applications
3. Physical Principles of Muography
3.1. Transmission Muography
3.2. Muon Flux Zenith Angle Dependence
4. Materials and Methods
4.1. Scintillation Detectors
4.2. Triggering System and Event Discrimination
4.3. Detector Performances
5. Results
6. Discussion and Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| BCD | Binary Coded Decimal |
| BSM | Beyond Standard Model |
| CV | Cutoff Value |
| CR | Cosmic Rays |
| DAQ | Data Acquisition |
| HV | High-Voltage |
| LFV | Lepton Flavor Violation |
| LG | Light Guide |
| LLT | Low-Level Threshold |
| PMT | Photomultiplier Tube |
| PW | Pulse Width |
| QDC | Charge to Digital Converter |
| SL | Sea Level |
| SM | Standard Model |
| TDC | Time to Digital Converter |
| ToF | Time of Flight |
References
- Uretsky, J.L. Penetration of cosmic ray muons into the Earth. Nucl. Instrum. Methods Phys. Res. A 1997, 399, 285–300. [Google Scholar] [CrossRef][Green Version]
- Neddermeyer, S.H.; Anderson, C.D. Note on the nature of cosmic-ray particles. Phys. Rev. 1937, 51, 884–886. [Google Scholar] [CrossRef]
- Tanabashi, M.; Hagiwara, K.; Hikasa, K.; Nakamura, K.; Sumino, Y.; Takahashi, F.; Tanaka, J.; Agashe, K.; Aielli, G.; Amsler, C.; et al. Review of Particle Physics. Phys. Rev. D 2018, 98, 030001. [Google Scholar] [CrossRef]
- Lattes, C.M.G.; Muirhead, H.; Occhialini, G.P.S.; Powell, C.F. Processes involving charged mesons. Nature 1947, 159, 694–697. [Google Scholar] [CrossRef]
- Bernauer, J.; Pohl, R. The Proton Radius Problem. Sci. Am. 2014, 310, 32–39. [Google Scholar] [CrossRef]
- Pacetti, S.; Tomasi-Gustafsson, E. The origin of the proton radius puzzle. Eur. Phys. J. A 2021, 57. [Google Scholar] [CrossRef]
- Mihara, S.; Miller, J.; Paradisi, P.; Piredda, G. Charged Lepton Flavor–Violation Experiments. Annu. Rev. Nucl. Part. Sci. 2013, 63, 531–552. [Google Scholar] [CrossRef]
- Measurement of Higgs Boson Decay to a Pair of Muons in Proton-Proton Collisions at =3 TeV; Technical Report; CERN: Geneva, Switzerland, 2020.
- Aaij, R. Measurement of the →μ+μ− decay properties and search for the B0→μ+μ− and →μ+μ−γ decays. Phys. Rev. D 2022, 105, 012010. [Google Scholar] [CrossRef]
- A moment for muons. Nat. Phys. 2021, 17, 541. [CrossRef]
- Abi, B.; Albahri, T.; Al-Kilani, S.; Allspach, D.; Alonzi, L.P.; Anastasi, A.; Anisenkov, A.; Azfar, F.; Badgley, K.; Baeßler, S.; et al. Measurement of the Positive Muon Anomalous Magnetic Moment to 0.46 ppm. Phys. Rev. Lett. 2021, 126, 141801. [Google Scholar] [CrossRef]
- Logashenko, I.B.; Eidelman, S.I. Anomalous magnetic moment of the muon. Phys. Usp. 2018, 61, 480–510. [Google Scholar] [CrossRef]
- Aoyama, T.; Asmussen, N.; Benayoun, M.; Bijnens, J.; Blum, T.; Bruno, M.; Caprini, I.; Carloni Calame, C.; Cè, M.; Colangelo, G.; et al. The anomalous magnetic moment of the muon in the Standard Model. Phys. Rep. 2020, 887, 1–166. [Google Scholar] [CrossRef]
- Sirunyan, A.M.; Tumasyan, A.; Adam, W.; Bergauer, T.; Dragicevic, M.; Erö, J.; Escalante Del Valle, A.; Fruehwirth, R.; Jeitler, M.; Krammer, N.; et al. Evidence for Higgs boson decay to a pair of muons. J. High Energy Phys. 2021, 1, 148. [Google Scholar] [CrossRef]
- The ATLAS Collaboration. A search for the dimuon decay of the Standard Model Higgs boson with the ATLAS detector. Phys. Lett. B 2021, 812, 135980. [Google Scholar] [CrossRef]
- Motoki, M.; Sanuki, T.; Orito, S.; Abe, K.; Anraku, K.; Asaoka, Y.; Fujikawa, M.; Fuke, H.; Haino, S.; Imori, M.; et al. Precise measurements of atmospheric muon fluxes with the BESS spectrometer. Astropart. Phys. 2003, 19, 113–126. [Google Scholar] [CrossRef]
- Dragić, A.; Joković, D.; Banjanac, R.; Udovičić, V.; Panić, B.; Puzović, J.; Aničin, I. Measurement of cosmic ray muon flux in the Belgrade ground level and underground laboratories. Nucl. Instrum. Methods Phys. Res. A 2008, 591, 470–475. [Google Scholar] [CrossRef]
- Mauri, N. Annual modulation of the atmospheric muon flux measured by the OPERA experiment. J. Phys. Conf. 2020, 1342, 012013. [Google Scholar] [CrossRef]
- Aguilar, J.; Albert, A.; Anton, G.; Anvar, S.; Ardid, M.; Assis Jesus, A.; Astraatmadja, T.; Aubert, J.J.; Auer, R.; Baret, B.; et al. Zenith distribution and flux of atmospheric muons measured with the 5-line ANTARES detector. Astropart. Phys. 2010, 34, 179–184. [Google Scholar] [CrossRef]
- Abe, K.; Hayato, Y.; Iida, T.; Iyogi, K.; Kameda, J.; Kishimoto, Y.; Koshio, Y.; Marti, L.; Miura, M.; Moriyama, S.; et al. Calibration of the Super-Kamiokande detector. Nucl. Instrum. Methods Phys. Res. A 2014, 737, 253–272. [Google Scholar] [CrossRef]
- Briki, I.; Mazouz, M.; Ghedira, L. Angular distribution of low momentum atmospheric muons at ground level. arXiv 2022, arXiv:2206.13061. [Google Scholar]
- Heck, D.; Knapp, J.; Capdevielle, J.N.; Schatz, G.; Thouw, T. CORSIKA: A Monte Carlo Code to Simulate Extensive Air Showers. 1998. Available online: https://digbib.ubka.uni-karlsruhe.de/volltexte/fzk/6019/6019.pdf (accessed on 30 September 2022).
- Ferrari, A.; Sala, P.; Fasso, A.; Ranft, J. FLUKA: A Multi-Particle Transport Code; Number SLAC-R-773; OSTI Identifier: 877507; SLAC National Accelerator Lab.: Menlo Park, CA, USA, 2005. [Google Scholar] [CrossRef]
- George, E.P. Cosmic Rays Measure Overburden of Tunnel. Commonw. Eng. 1955, 43, 455–457. [Google Scholar]
- Alvarez, L.W.; Anderson, J.A.; Bedwei, F.E.; Burkhard, J.; Fakhry, A.; Girgis, A.; Goneid, A.; Hassan, F.; Iverson, D.; Lynch, G.; et al. Search for Hidden Chambers in the Pyramids: The structure of the Second Pyramid of Giza is determined by cosmic-ray absorption. Science 1970, 167, 832–839. [Google Scholar] [CrossRef] [PubMed]
- Morishima, K.; Kuno, M.; Nishio, A.; Kitagawa, N.; Manabe, Y.; Moto, M.; Takasaki, F.; Fujii, H.; Satoh, K.; Kodama, H.; et al. Discovery of a big void in Khufu’s Pyramid by observation of cosmic-ray muons. Nature 2017, 552, 386–390. [Google Scholar] [CrossRef] [PubMed]
- Bross, A.D.; Dukes, E.C.; Ehrlich, R.; Fernandez, E.; Dukes, S.; Gobashy, M.; Jamieson, I.; La Riviere, P.J.; Liu, M.; Marouard, G.; et al. Tomographic Muon Imaging of the Great Pyramid of Giza. J. Adv. Instrum. Sci. 2022. [Google Scholar] [CrossRef]
- Saracino, G.; Amato, L.; Ambrosino, F.; Antonucci, G.; Bonechi, L.; Cimmino, L.; Consiglio, L.; Alessandro, R.D.; Luzio, E.D.; Minin, G.; et al. Imaging of underground cavities with cosmic-ray muons from observations at Mt. Echia (Naples). Sci. Rep. 2017, 7, 1181. [Google Scholar] [CrossRef]
- Gómez, H.; Carloganu, C.; Gibert, D.; Jacquemier, J.; Karyotakis, Y.; Marteau, J.; Niess, V.; Katsanevas, S.; Tonazzo, A. Studies on muon tomography for archaeological internal structures scanning. J. Phys. Conf. Ser. 2016, 718, 052016. [Google Scholar] [CrossRef]
- Tanaka, H.K.M.; Sumiya, K.; Oláh, L. Muography as a new tool to study the historic earthquakes recorded in ancient burial mounds. Geosci. Instrum. Methods Data Syst. 2020, 9, 357–364. [Google Scholar] [CrossRef]
- Feder, T. Muons May Unlock Secrets of Teotihuacan. Phys. Today 2004, 57, 31–32. [Google Scholar] [CrossRef]
- Menchaca-Rocha, A. Using cosmic muons to search for cavities in the Pyramid of the Sun, Teotihuacan: Preliminary results. In Proceedings of the 10th Latin American Symposium on Nuclear Physics and Applications—PoS(X LASNPA), Montevideo, Uruguay, 1–6 December 2013; Sissa Medialab: Montevideo, Uruguay, 2014; p. 12. [Google Scholar] [CrossRef][Green Version]
- Nagamine, K.; Iwasaki, M.; Shimomura, K.; Ishida, K. Method of probing inner-structure of geophysical substance with the horizontal cosmic-ray muons and possible application to volcanic eruption prediction. Nucl. Instrum. Methods Phys. Res. A 1995, 356, 585–595. [Google Scholar] [CrossRef]
- Tanaka, H.; Nakano, T.; Takahashi, S.; Yoshida, J.; Takeo, M.; Oikawa, J.; Ohminato, T.; Aoki, Y.; Koyama, E.; Tsuji, H. High resolution imaging in the inhomogeneous crust with cosmic-ray muon radiography: The density structure below the volcanic crater floor of Mt. Asama, Japan. Earth Planet. Sci. Lett. 2007, 263, 104–113. [Google Scholar] [CrossRef]
- Tanaka, H.K.M.; Uchida, T.; Tanaka, M.; Takeo, M.; Oikawa, J.; Ohminato, T.; Aoki, Y.; Koyama, E.; Tsuji, H. Detecting a mass change inside a volcano by cosmic-ray muon radiography (muography): First results from measurements at Asama volcano, Japan. Geophys. Res. Lett. 2009, 36, L17302. [Google Scholar] [CrossRef]
- Tanaka, H.K.M.; Kusagaya, T.; Shinohara, H. Radiographic visualization of magma dynamics in an erupting volcano. Nat. Commun. 2014, 5, 3381. [Google Scholar] [CrossRef] [PubMed]
- Okubo, S.; Tanaka, H.K.M. Imaging the density profile of a volcano interior with cosmic-ray muon radiography combined with classical gravimetry. Meas. Sci. Technol. 2012, 23, 042001. [Google Scholar] [CrossRef]
- D’Alessandro, R.; Ambrosino, F.; Baccani, G.; Bonechi, L.; Bongi, M.; Caputo, A.; Ciaranfi, R.; Cimmino, L.; Ciulli, V.; D’Errico, M.; et al. Volcanoes in Italy and the role of muon radiography. Philos. Trans. R. Soc. A 2019, 377, 20180050. [Google Scholar] [CrossRef]
- Nishiyama, R.; Ariga, A.; Ariga, T.; Käser, S.; Lechmann, A.; Mair, D.; Scampoli, P.; Vladymyrov, M.; Ereditato, A.; Schlunegger, F. First measurement of ice-bedrock interface of alpine glaciers by cosmic muon radiography. Geophys. Res. Lett. 2017, 44, 6244–6251. [Google Scholar] [CrossRef]
- Marteau, J.; d’Ars, J.d.B.; Gibert, D.; Jourde, K.; Ianigro, J.C.; Carlus, B. DIAPHANE: Muon tomography applied to volcanoes, civil engineering, archaelogy. J. Instrum. 2017, 12, C02008. [Google Scholar] [CrossRef]
- Lesparre, N.; Gibert, D.; Marteau, J.; Komorowski, J.C.; Nicollin, F.; Coutant, O. Density muon radiography of La Soufrière of Guadeloupe volcano: Comparison with geological, electrical resistivity and gravity data. Geophys. J. Int. 2012, 190, 1008–1019. [Google Scholar] [CrossRef]
- Jourde, K.; Gibert, D.; Marteau, J.; de Bremond d’Ars, J.; Komorowski, J.C. Muon dynamic radiography of density changes induced by hydrothermal activity at the La Soufrière of Guadeloupe volcano. Sci. Rep. 2016, 6, 33406. [Google Scholar] [CrossRef]
- Rosas-Carbajal, M.; Jourde, K.; Marteau, J.; Deroussi, S.; Komorowski, J.C.; Gibert, D. Three-dimensional density structure of La Soufrière de Guadeloupe lava dome from simultaneous muon radiographies and gravity data. Geophys. Res. Lett. 2017, 44, 6743–6751. [Google Scholar] [CrossRef]
- Le Gonidec, Y.; Rosas-Carbajal, M.; Bremond d’Ars, J.d.; Carlus, B.; Ianigro, J.C.; Kergosien, B.; Marteau, J.; Gibert, D. Abrupt changes of hydrothermal activity in a lava dome detected by combined seismic and muon monitoring. Sci. Rep. 2019, 9, 3079. [Google Scholar] [CrossRef]
- Varga, D.; Nyitrai, G.; Hamar, G.; Oláh, L. High Efficiency Gaseous Tracking Detector for Cosmic Muon Radiography. Adv. High Energy Phys. 2016, 2016, 1962317. [Google Scholar] [CrossRef]
- Oláh, L.; Tanaka, H.K.M.; Ohminato, T.; Varga, D. High-definition and low-noise muography of the Sakurajima volcano with gaseous tracking detectors. Sci. Rep. 2018, 8, 3207. [Google Scholar] [CrossRef] [PubMed]
- Oláh, L.; Tanaka, H.K.; Ohminato, T.; Hamar, G.; Varga, D. Plug Formation Imaged Beneath the Active Craters of Sakurajima Volcano With Muography. Geophys. Res. Lett. 2019, 46, 10417–10424. [Google Scholar] [CrossRef]
- Oláh, L.; Tanaka, H.K.M.; Hamar, G. Muographic monitoring of hydrogeomorphic changes induced by post-eruptive lahars and erosion of Sakurajima volcano. Sci. Rep. 2021, 11, 17729. [Google Scholar] [CrossRef] [PubMed]
- Carbone, D.; Gibert, D.; Marteau, J.; Diament, M.; Zuccarello, L.; Galichet, E. An experiment of muon radiography at Mt Etna (Italy). Geophys. J. Int. 2014, 196, 633–643. [Google Scholar] [CrossRef]
- Anastasio, A.; Ambrosino, F.; Basta, D.; Bonechi, L.; Brianzi, M.; Bross, A.; Callier, S.; Caputo, A.; Ciaranfi, R.; Cimmino, L.; et al. The MU-RAY detector for muon radiography of volcanoes. Nucl. Instrum. Methods Phys. Res. A 2013, 732, 423–426. [Google Scholar] [CrossRef]
- Ambrosino, F.; Anastasio, A.; Basta, D.; Bonechi, L.; Brianzi, M.; Bross, A.; Callier, S.; Caputo, A.; Ciaranfi, R.; Cimmino, L.; et al. The MU-RAY project: Detector technology and first data from Mt. Vesuvius. J. Instrum. 2014, 9, C02029. [Google Scholar] [CrossRef]
- Ambrosino, F.; Anastasio, A.; Bross, A.; Béné, S.; Boivin, P.; Bonechi, L.; Cârloganu, C.; Ciaranfi, R.; Cimmino, L.; Combaret, C.; et al. Joint measurement of the atmospheric muon flux through the Puy de Dôme volcano with plastic scintillators and Resistive Plate Chambers detectors. J. Geophys. Res. Solid Earth 2015, 120, 7290–7307. [Google Scholar] [CrossRef]
- Tioukov, V.; Alexandrov, A.; Bozza, C.; Consiglio, L.; D’Ambrosio, N.; De Lellis, G.; De Sio, C.; Giudicepietro, F.; Macedonio, G.; Miyamoto, S.; et al. First muography of Stromboli volcano. Sci. Rep. 2019, 9, 6695. [Google Scholar] [CrossRef]
- Lo Presti, D.; Gallo, G.; Bonanno, D.; Bonanno, G.; Bongiovanni, D.; Carbone, D.; Ferlito, C.; Immè, J.; La Rocca, P.; Longhitano, F.; et al. The MEV project: Design and testing of a new high-resolution telescope for muography of Etna Volcano. Nucl. Instrum. Methods Phys. Res. A 2018, 904, 195–201. [Google Scholar] [CrossRef]
- Peña-Rodríguez, J.; Pisco-Guabave, J.; Sierra-Porta, D.; Suárez-Durán, M.; Arenas-Flórez, M.; Pérez-Archila, L.; Sanabria-Gómez, J.; Asorey, H.; Núñez, L. Design and construction of MuTe: A hybrid Muon Telescope to study Colombian volcanoes. J. Instrum. 2020, 15, P09006. [Google Scholar] [CrossRef]
- Useche, J.; Ávila, C. Estimation of cosmic-muon flux attenuation by Monserrate Hill in Bogota. J. Instrum. 2019, 14, P02015. [Google Scholar] [CrossRef]
- Lobo-Guerrero, A. La falla de Bogotá en Cundinamarca. X Congr. Colomb. Geol. Available online: https://drive.google.com/file/d/1SJAfBh5j-5gcpCo-h9mw0V2wWo2-_14U/view?usp=share_link (accessed on 30 September 2022).
- Bonechi, L.; D’Alessandro, R.; Giammanco, A. Atmospheric muons as an imaging tool. Rev. Phys. 2020, 5, 100038. [Google Scholar] [CrossRef]
- Rhodes, C.J. Muon Tomography: Looking inside Dangerous Places. Sci. Prog. 2015, 98, 291–299. [Google Scholar] [CrossRef]
- Procureur, S. Muon imaging: Principles, technologies and applications. Nucl. Instrum. Methods Phys. Res. A 2018, 878, 169–179. [Google Scholar] [CrossRef]
- Bonomi, G.; Checchia, P.; D’Errico, M.; Pagano, D.; Saracino, G. Applications of cosmic-ray muons. Prog. Part. Nucl. Phys. 2020, 112, 103768. [Google Scholar] [CrossRef]
- Tanaka, H.K.M.; Oláh, L. Overview of muographers. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2019, 377, 20180143. [Google Scholar] [CrossRef]
- Leone, G.; Tanaka, H.K.M.; Holma, M.; Kuusiniemi, P.; Varga, D.; Oláh, L.; Presti, D.L.; Gallo, G.; Monaco, C.; Ferlito, C.; et al. Muography as a new complementary tool in monitoring volcanic hazard: Implications for early warning systems. Proc. R. Soc. A Math. Phys. Eng. Sci. 2021, 477, 20210320. [Google Scholar] [CrossRef]
- Kaiser, R. Muography: Overview and future directions. Philos. Trans. R. Soc. A 2019, 377, 20180049. [Google Scholar] [CrossRef]
- Oláh, L.; Tanaka, H.; Varga, D. Muography: Exploring Earth’s Subsurface with Elementary Particles; Geophysical Monograph Series; Wiley: Hoboken, NJ, USA, 2022. [Google Scholar]
- Giannini, C.; Holy, V.; De Caro, L.; Mino, L.; Lamberti, C. Watching nanomaterials with X-ray eyes: Probing different length scales by combining scattering with spectroscopy. Prog. Mater. Sci. 2020, 112, 100667. [Google Scholar] [CrossRef]
- Hussein, E.M. Imaging with naturally occurring radiation. Appl. Radiat. Isot. 2019, 145, 223–239. [Google Scholar] [CrossRef] [PubMed]
- Kempa, J.; Brancus, I. Zenith angle distributions of cosmic ray muons. Nucl. Phys. B Proc. Suppl. 2003, 122, 279–281. [Google Scholar] [CrossRef]
- Ngoc Diep, P.; Ngoc Dinh, P.; Hai Duong, N.; Thi Tuyet Nhung, P.; Darriulat, P.; Thi Thao, N.; Quang Thieu, D.; Van Thuan, V. Measurement of the east–west asymmetry of the cosmic muon flux in Hanoi. Nucl. Phys. B 2004, 678, 3–15. [Google Scholar] [CrossRef]
- Kudela, K.; Storini, M. Cosmic ray variability and geomagnetic activity: A statistical study. J. Atmos. Sol. Terr. Phys. 2005, 67, 907–912. [Google Scholar] [CrossRef]
- Xue, B.; Ma, B.Q. Muon charge information from geomagnetic deviation in inclined extensive air showers. Astropart. Phys. 2007, 27, 286–295. [Google Scholar] [CrossRef][Green Version]
- Bahmanabadi, M. A method for determining the angular distribution of atmospheric muons using a cosmic ray telescope. Nucl. Instrum. Methods Phys. Res. A 2019, 916, 1–7. [Google Scholar] [CrossRef]
- Dmitrieva, A.; Kokoulin, R.; Petrukhin, A.; Timashkov, D. Corrections for temperature effect for ground-based muon hodoscopes. Astropart. Phys. 2011, 34, 401–411. [Google Scholar] [CrossRef]
- Maghrabi, A.; Almutairi, M. The influence of several atmospheric variables on cosmic ray muons observed by KACST detector. Adv. Space Res. 2018, 62, 3267–3277. [Google Scholar] [CrossRef]
- Maghrabi, A.; Aldosari, A.; Almutairi, M.; Altilasi, M. Atmospheric effects on secondary cosmic ray muons observed by multi-wire muon detector at a high cutoff rigidity station. Adv. Space Res. 2019, 64, 1629–1637. [Google Scholar] [CrossRef]
- Judge, R.J.R.; Nash, W.F. Measurements on the muon flux at various zenith angles. Il Nuovo Cimento 1965, 35, 999–1024. [Google Scholar] [CrossRef]
- Pal, S.; Acharya, B.; Majumder, G.; Mondal, N.; Samuel, D.; Satyanarayana, B. Measurement of integrated flux of cosmic ray muons at sea level using the INO-ICAL prototype detector. J. Cosmol. Astropart. Phys. 2012, 2012, 33. [Google Scholar] [CrossRef]
- Shukla, P.; Sankrith, S. Energy and angular distributions of atmospheric muons at the Earth. Int. J. Mod. Phys. A 2018, 33, 1850175. [Google Scholar] [CrossRef]
- Cohu, A.; Tramontini, M.; Chevalier, A.; Ianigro, J.C.; Marteau, J. Atmospheric and Geodesic Controls of Muon Rates: A Numerical Study for Muography Applications. Instruments 2022, 6, 24. [Google Scholar] [CrossRef]
- Greisen, K. The Intensities of the Hard and Soft Components of Cosmic Rays as Functions of Altitude and Zenith Angle. Phys. Rev. 1942, 61, 212–221. [Google Scholar] [CrossRef]
- Fukui, S.; Kitamura, T.; Murata, Y. On the Range Spectrum of µ-Mesons at Sea-Level at Geomagnetic Latitude 24°N. J. Phys. Soc. Jpn. 1957, 12, 854–863. [Google Scholar] [CrossRef]
- Allkofer, O.C.; Andresen, R.D.; Dau, W.D. The muon spectra near the geomagnetic equator. Can. J. Phys. 1968, 46, S301–S305. [Google Scholar] [CrossRef]
- Pethuraj, S.; Datar, V.; Majumder, G.; Mondal, N.; Ravindran, K.; Satyanarayana, B. Measurement of cosmic muon angular distribution and vertical integrated flux by 2 m × 2 m RPC stack at IICHEP-Madurai. J. Cosmol. Astropart. Phys. 2017, 2017, 021. [Google Scholar] [CrossRef][Green Version]
- Sioli, M. Underground muon physics with the MACRO experiment. Nucl. Phys. B Proc. Suppl. 2000, 85, 349–354. [Google Scholar] [CrossRef]
- Ambrosio, M. Moon and Sun shadowing effect in the MACRO detector. Astropart. Phys. 2003, 20, 145–156. [Google Scholar] [CrossRef]
- Serna, J.; IDEAM. Boletín Climatológico, Julio 2022. Available online: http://www.ideam.gov.co/documents/21021/122201016/07_Boletín_Climatológico_Julio_2022.pdf/645823b8-ec75-4d6a-af4b-b62a2ef5c663?version=1.0 (accessed on 30 September 2022).
- IDEAM. Boletín Climatológico, Julio 2022. Seguimiento Diario de las Temperaturas Mínima, Media y Máxima. IDEAM. Available online: http://www.ideam.gov.co/documents/21021/122827570/07+-+BC+-+Temperaturas+-+2022.pdf/52dd41c1-758d-4766-8eec-a8bd0c7b2887?version=1.0 (accessed on 30 September 2022).
- Groom, D.E.; Mokhov, N.V.; Striganov, S.I. Muon stopping power and range Tables 10-MeV to 100-TeV. Atom. Data Nucl. Data Tabl. 2001, 78, 183–356. [Google Scholar] [CrossRef]
- Berger, M. Stopping-Power and Range Tables for Electrons, Protons, and Helium Ions; National Institute of Standards and Technology: Gaithersburg, MD, USA, 1993. [Google Scholar]
- Rossi, B. Interpretation of Cosmic-Ray Phenomena. Rev. Mod. Phys. 1948, 20, 537–583. [Google Scholar] [CrossRef]
- Crookes, J.; Rastin, B. An investigation of the absolute intensity of muons at sea-level. Nucl. Phys. B 1972, 39, 493–508. [Google Scholar] [CrossRef]
- Sogarwal, H.; Shukla, P. Measurement of atmospheric muon angular distribution using a portable setup of liquid scintillator bars. J. Cosmol. Astropart. Phys. 2022, 2022, 11. [Google Scholar] [CrossRef]
- Arneodo, F.; Benabderrahmane, M.; Bruno, G.; Di Giovanni, A.; Fawwaz, O.; Messina, M.; Mussolini, C. Measurement of cosmic muons angular distribution in Abu Dhabi at sea level. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2019, 936, 242–243. [Google Scholar] [CrossRef]
- Karmakar, N.L.; Paul, A.; Chaudhuri, N. Measurements of absolute intensities of cosmic-ray muons in the vertical and greatly inclined directions at geomagnetic latitudes 16 degrees n. Nuovo Cim. B 1973, 17, 173–186. [Google Scholar] [CrossRef]
- Bhattacharyya, D. Absolute sea-level integral muon spectra at zenith angles 45 °W and 60 °W near the geomagnetic equator in the momentum range (0.4÷3) GeV/c. Il Nuovo Cimento B 1974, 24, 78–84. [Google Scholar] [CrossRef]
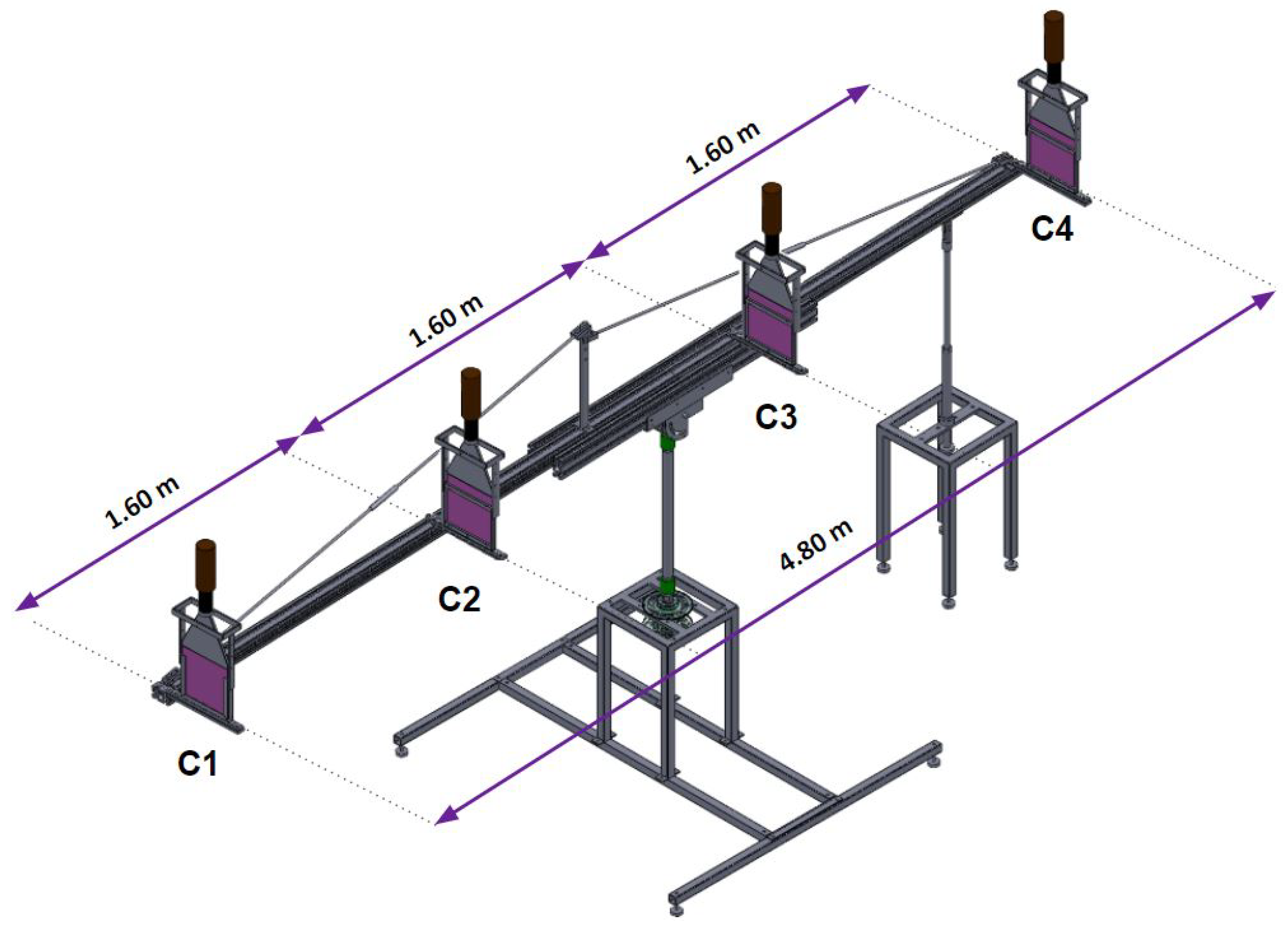



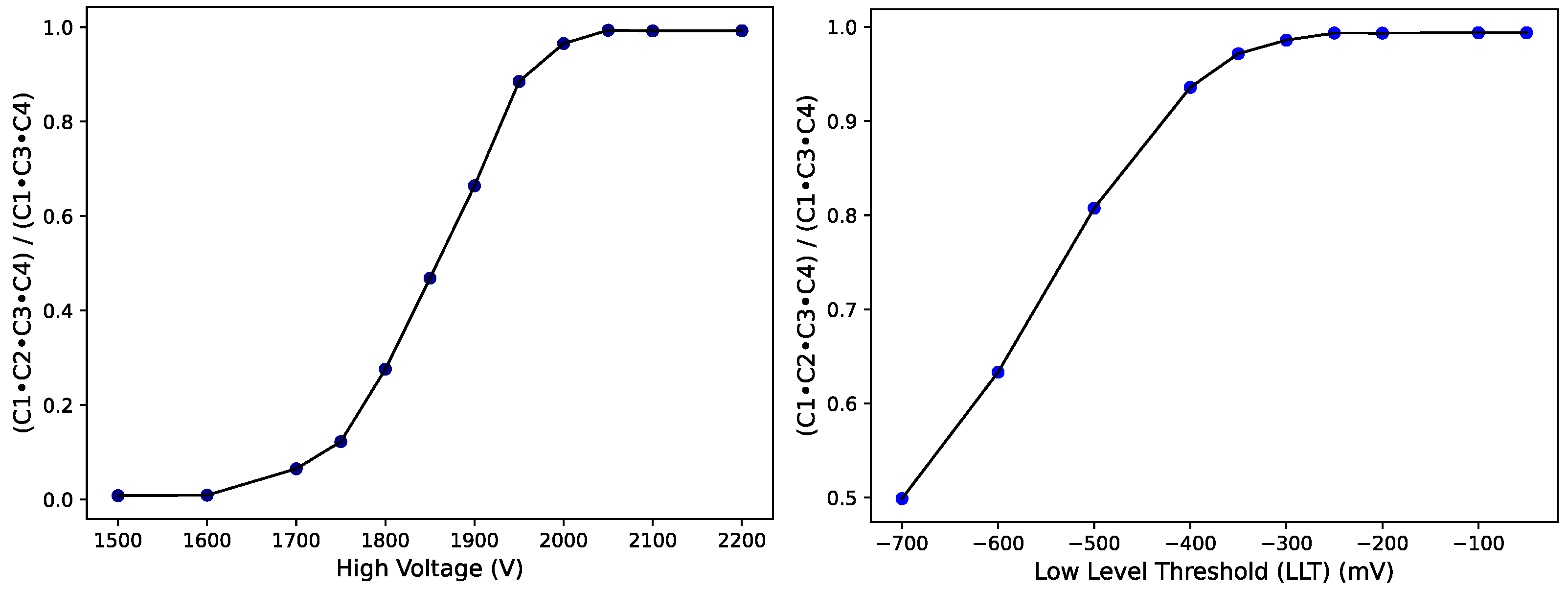
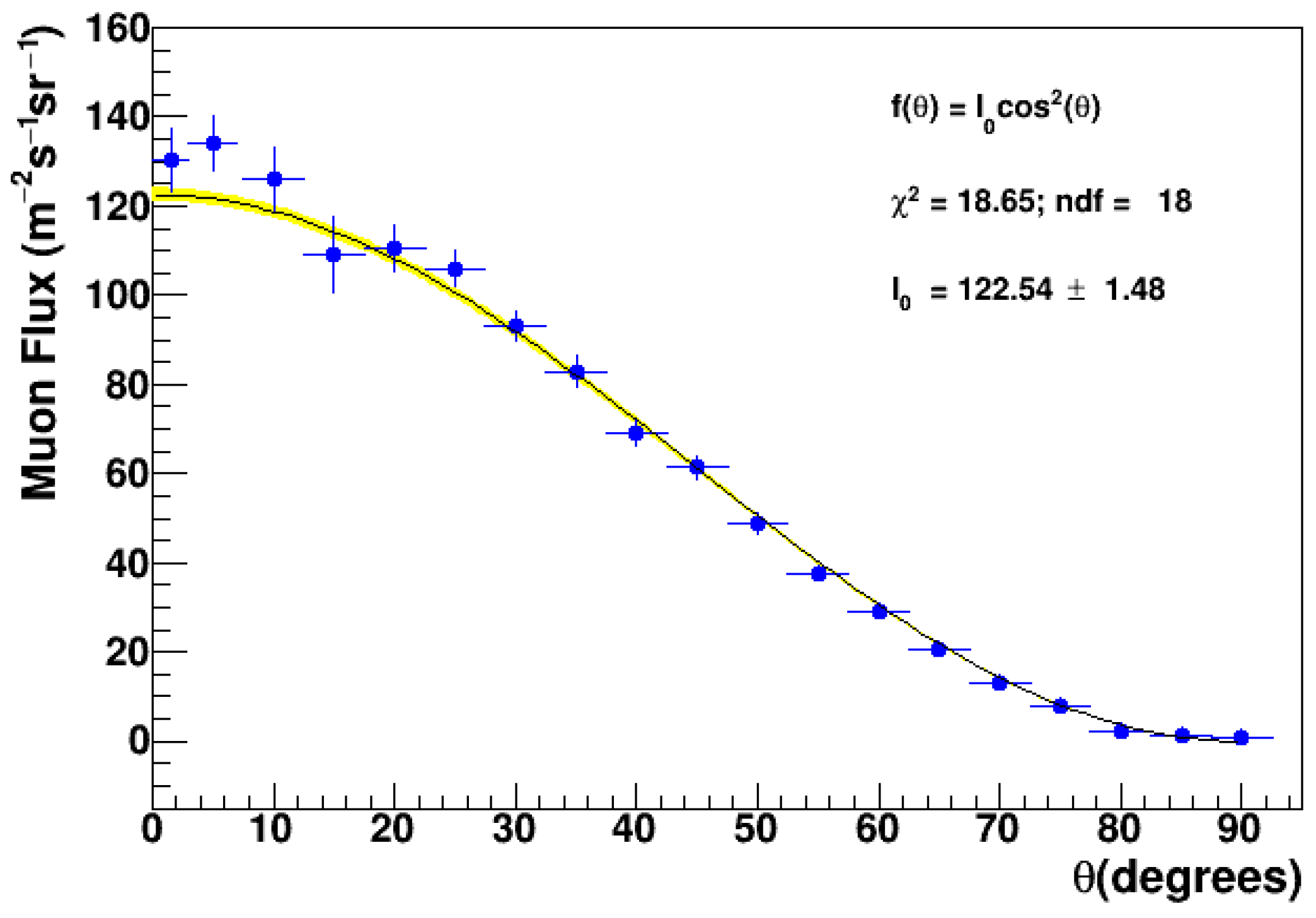

| Detector | HV (V) | PW (ns) | LLT (mV) | QDC CV (DAQ Units) | Efficiency |
|---|---|---|---|---|---|
| C1 | 2100 | 20 | −200 | 200 | 0.996 |
| C2 | 2100 | 20 | −200 | 200 | 0.983 |
| C3 | 2300 | 20 | −200 | 200 | 0.979 |
| C4 | 2100 | 20 | −200 | 200 | 0.992 |
| θ (Degrees) | dN/dΩ (m−2s−1sr−1) | Δ(dN/dΩ) (m−2s−1sr−1) |
|---|---|---|
| 1.5 | 130.25 | 6.89 |
| 5 | 134.07 | 6.12 |
| 10 | 125.97 | 7.26 |
| 15 | 109.11 | 8.56 |
| 20 | 110.53 | 5.09 |
| 25 | 106.05 | 3.84 |
| 30 | 92.89 | 3.29 |
| 35 | 82.97 | 3.31 |
| 40 | 68.98 | 2.75 |
| 45 | 61.33 | 2.46 |
| 50 | 48.85 | 2.22 |
| 55 | 37.67 | 1.84 |
| 60 | 29.28 | 1.61 |
| 65 | 20.60 | 1.36 |
| 70 | 12.97 | 1.16 |
| 75 | 7.94 | 1.06 |
| 80 | 2.37 | 1.04 |
| 85 | 1.45 | 0.90 |
| 90 | 0.72 | 0.85 |
| Authors | Latitude (°N) | Altitude (m) | n Value | Vertical Integrated Flux, I0 (m−2s−1sr−1) |
|---|---|---|---|---|
| Greisen [80,90] | 54 | 259 | 2.1 | 82.0 ± 10.0 |
| Crookes and Rastin [91] | 53 | 40 | 2.16 ± 0.01 | 91.3 ± 1.2 |
| Judge and Nash [76] | 53 | SL | 1.96 ± 0.22 | - |
| Dragić et al. [17] | 44.85 | 78 | - | 84 ± 4 |
| Briki et al. [21] | 35.76 | 38 | 1.82 ± 0.11 | 68.77 ± 1.94 |
| Arneodo et al. [93] | 24.54 | SL | 1.91 ± 0.10 (stat) ± 0.15 (syst) | 75.4 ± 1.3 (stat) ± 1.5 (syst) |
| Fukui et al. [81] | 24 | SL | - | 73.5 ± 2.0 |
| Sogarwal et al. [92] | 19 | SL | 2.10 ± 0.25 | 66.70 ± 1.54 |
| Karmakar et al. [94] | 16 | 122 | 2.2 | 89.9 ± 0.5 |
| Bhattacharyya [95] | 12 | 24 | 1.85 ± 0.10 | - |
| S. Pal [77] | 10.61 | SL | 2.15 ± 0.01 | 62.17 ± 0.05 |
| Pethuraj et al. [83] | 1.44 | 160 | 2.00 ± 0.04 (stat) ± 0.16 (syst) | 70.07 ± 0.02 (stat) ± 5.26 (syst) |
| Present data | 4.60 | 2657 | 2.145 ± 0.046 | 127.7 ± 2.2 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Borja, C.; Ávila, C.; Roque, G.; Sánchez, M. Atmospheric Muon Flux Measurement near Earth’s Equatorial Line. Instruments 2022, 6, 78. https://doi.org/10.3390/instruments6040078
Borja C, Ávila C, Roque G, Sánchez M. Atmospheric Muon Flux Measurement near Earth’s Equatorial Line. Instruments. 2022; 6(4):78. https://doi.org/10.3390/instruments6040078
Chicago/Turabian StyleBorja, Cristian, Carlos Ávila, Gerardo Roque, and Manuel Sánchez. 2022. "Atmospheric Muon Flux Measurement near Earth’s Equatorial Line" Instruments 6, no. 4: 78. https://doi.org/10.3390/instruments6040078
APA StyleBorja, C., Ávila, C., Roque, G., & Sánchez, M. (2022). Atmospheric Muon Flux Measurement near Earth’s Equatorial Line. Instruments, 6(4), 78. https://doi.org/10.3390/instruments6040078





