Two Prism Critical Angle Refractometry with Attenuating Media

Abstract

We present a concept that enables the determination of the complex refractive index of attenuating media from two critical angles, measured sequentially at two interfaces between a single sample and two different prisms. The proposed method is general in that it applies with s and p polarisation states, thus it is suited for the characterisation of isotropic as well as anisotropic media. Uncertainty analysis indicates that relative error in the determination of the real (imaginary) index can be less than ${10}^{-4}$ (in the order of $10\%$), respectively.

1. Introduction

The refractive index of a medium derives from the reflectance profile at an interface between the sample and a front reference medium, which is typically a transparent prism [1,2]. When the sample is transparent, the refractive index is a real number that can be determined from just one out of several features of the reflectance profile, such as the critical angle of total internal reflection (${\theta }_c$) [3,4,5] or the Brewster angle (${\theta }_B$) [6,7,8,9]. When the sample attenuates light, the refractive index becomes a complex quantity, the imaginary part of which incorporates light extinction properties (absorption and/or scattering). Then, a pair of input values is needed to recover the real and imaginary component. This pair may comprise, for example, the critical angle of attenuated total reflection along with the reflectance at that point (${\theta }_c,R_c$) [10,11], the pseudo-Brewster angle together with the reflectance at that point (${\theta }_B,R_B$) [12], or the the pseudo-Brewster angle and the reflectance at normal incidence (${\theta }_B,R_{\perp }$) [13].

Typical prism-coupling refractometers [14,15,16,17] are equipped with high resolution rotary tables (see Figure 1a). Hence, the angular features of the reflectance profile (e.g., ${\theta }_c$) can be resolved with high accuracy [18]. On the contrary, accuracy in the measurement of reflectance (e.g., $R_c$) is seriously compromised by unavoidable laser power fluctuations [18,19]. In that respect, it is advantageous to devise methods whereby the complex refractive index results from an input pair that contains two angles.

Here, we demonstrate how to retrieve the complex optical constant $n_r+i·n_i$ of an attenuating medium from the critical angle pair (${\theta }_c,{\theta }_c^{\prime }$) measured at two interfaces between the same sample and two different prisms. The real indices of the prisms are $n_p$ and $n_p^{\prime }$, such that $n_p/n_p^{\prime }=\mathrm{\Lambda }$. To obtain a first insight, Figure 1b depicts corresponding reflectance profiles. Calculations are based on the Fresnel equation for the exemplary case of s polarised light, while parameters

$$n=\frac{n_r}{n_p},k=\frac{n_i}{n_p},n^{\prime }=\frac{n_r}{n_p^{\prime }}=n\mathrm{\Lambda },k^{\prime }=\frac{n_i}{n_p^{\prime }}=k\mathrm{\Lambda }$$

(1)

assume the values that are indicated in the figure’s caption. Located at the maximum of the respective reflectance derivative $dR/d\theta$, which is also plotted in Figure 1b, critical angles ${\theta }_c$ and ${\theta }_c^{\prime }$ are separated by $\mathrm{\Delta }={\theta }_c-{\theta }_c^{\prime }$. Reflectances $R_c$ and $R_c^{\prime }$ are also tracked in Figure 1b.

The sample’s complex refractive index derives from the input pair (${\theta }_c,{\theta }_c^{\prime }$) as the unique numerical solution of a well-posed equation system that is fully described next. The proposed method is general in the sense that it applies with s and p polarised light, hence it is suited for isotropic as well as anisotropic media. Uncertainty analysis indicates that within an extinction range, say, $0.001<k<0.01$, which includes several material families of interest, the real (or the imaginary) index can be determined with relative error less than ∼${10}^{-4}$ (or ∼$10\%$), respectively.

The proposed method resembles the two prism, two angle approach introduced by Azzam several decades ago [20], utilizing the pseudo-Brewster angle pair (${\theta }_B,{\theta }_B^{\prime }$) as input. Despite the conceptual similarity, Azzam’s routine was found to be inaccurate (or even unusable) when $n_i/n_r\ll 1$, a condition that automatically excludes most non-metal samples (The review of literature within the space limits of this letter is not exhaustive. For example, the interested reader should be referred to Hirschfeld’s two prism method, which is based on the measurement of two reflectances ($R,R^{\prime }$) at preset angles [21]. Another report on single prism two angle method [22] was also published recently, lacking however generality, since it applies only to isotropic media. Finally, we acknowledge a conceptually different route to determine the complex optical constants of attenuating samples, which depends on fitting experimental data $R\left(\theta \right)$ to Fresnel equations [23,24,25,26,27,28]. This approach is not a “point” method and has been proven sensitive to the choice of fit range [29,30]. An indepth comparison of the proposed method to Fresnel fitting is beyond our current scopes).

2. Background Theory

We start by recalling the mathematical framework that is derived from Fresnel equations in Ref. [10]. The involved formulas are compactly expressed in terms of the real dielectric constant of the prism ${ϵ}_p=n_p^2$ and the complex dielectric constant of the sample ${ϵ}_r+i·{ϵ}_i={(n_r+i·n_i)}^2$. Shorthands t and $\rho$, which relate to ${\theta }_c$ and $R_c$ via

$$t=tan{\theta }_c\mathrm{and}\rho =\frac{1+R_c}{1-R_c}$$

(2)

are also convenient. Then, it is

$$\frac{{ϵ}_r}{{ϵ}_p}=\frac{\alpha +t^2}{1+t^2}\mathrm{and}\frac{{ϵ}_i}{{ϵ}_p}=\frac{\sqrt{{\gamma }^2-{\alpha }^2}}{1+t^2}.$$

(3)

Parameters $\alpha$ and $\gamma$ depend on polarisation. Analytic solutions exist for s polarisation. These are

$$\gamma =\frac{2t}{(3{\rho }^2-2\rho -2)t+\rho \sqrt{(9{\rho }^2-12\rho -8)t^2-4}},$$

(4)

$$\alpha =\frac{{(1+\gamma )}^2}{2{\rho }^2}-\gamma .$$

(5)

The situation is slightly more complicated for p polarization, in which case $\alpha$ and $\gamma$ can be numerically obtained as solutions of two algebraic equations. Originally reported in [10] and reduced to an equivalent but more appealing notation in [22], these equations are:

$$\begin{array}{c}\hfill 2\sqrt{2}{t}^2\sqrt{\alpha +\gamma }=\rho (1+t^2)(t^2+\gamma )-\sqrt{{\rho }^2{(1+t^2)}^2{(t^2+\gamma )}^2-4t^2(1+\gamma )(t^4+\gamma )},\end{array}$$

(6)

$$\begin{array}{c}(1+t^2)(1+\rho )(t^2+\gamma )\sqrt{\alpha +\gamma }=\sqrt{2}{t}^2\gamma \times (1-2\alpha +{\gamma }^2){\left[t^2(\gamma -2\alpha )-{\gamma }^2+t^4(t^2+\gamma )\right]}^2\times \hfill \\ [t^6\left(2\alpha \gamma (2\gamma -1)+{\gamma }^2(2{\gamma }^2+9\gamma +5)-4{\alpha }^2\right)+t^4\gamma \left(6\alpha \gamma +{\gamma }^2(5\gamma +3)-12{\alpha }^2\right)+\\ \hfill t^2{\gamma }^3\left(5\gamma -8\alpha \right)+t^{10}\left(2\alpha +2{\gamma }^2+\gamma \right)+t^8\gamma \left(6\alpha +\gamma (4\gamma +5)\right)-{\gamma }^5{]}^{-1}.\end{array}$$

(7)

Equations (4) and (5) for s polarisation and Equations (6) and (7) for p polarisation enable the calculation of ${ϵ}_r$ and ${ϵ}_i$ (equivalently, $n_r$ and $n_i$) at either polarisation state, from experimental input values (${\theta }_c,R_c)$. This procedure is fully described in Ref. [10]. In what follows, we show how to eliminate reflectance $R_c$ from the input, via its replacement by an additional critical angle ${\theta }_c^{\prime }$, which may be accurately measured at an interface with a second prism.

3. Method’s Description

Collecting two reflectance profiles by use of two different prisms introduces additional conditions to the problem. These conditions are already stated in Equation (1) ($n^{\prime }=n\mathrm{\Lambda }$ and $k^{\prime }=k\mathrm{\Lambda }$). By use of Equation (3), they can be written in the alternative form

$$F_1=\frac{{\alpha }^{\prime }+{\left(t^{\prime }\right)}^2}{1+{\left(t^{\prime }\right)}^2}-\frac{\alpha +t^2}{1+t^2}·{\mathrm{\Lambda }}^2=0,$$

(8)

$$F_2=\frac{\sqrt{{\left({\gamma }^{\prime }\right)}^2-{\left({\alpha }^{\prime }\right)}^2}}{1+{\left(t^{\prime }\right)}^2}-\frac{\sqrt{{\gamma }^2-{\alpha }^2}}{1+t^2}·{\mathrm{\Lambda }}^2=0.$$

(9)

Throughout, accent marks denote parameters that relate to the second prism.

Parameters $\alpha ,{\alpha }^{\prime },\gamma$ and ${\gamma }^{\prime }$ in Equations (8) and (9) are essentially functions of $\rho ,{\rho }^{\prime },t$ and $t^{\prime }$ (equivalently, ${\theta }_c,{\theta }_c^{\prime },R_c$ and $R_c^{\prime }$). These functions are given explicitly by Equations (4) and (5) for s polarisation, or implicitly by Equations (6) and (7) for p polarisation. In that sense, Equations (8) and (9) define a system of two equations on four independent variables. Two of them are the measured input quantities (${\theta }_c,{\theta }^{\prime }$). The other two are the unknowns to be determined ($R_c,R_c^{\prime }$). Solving numerically this system is the method’s first step. To that end, we performed extensive numerical studies using Wolfram Mathematica’s noniterative global solver NSolve, to conclude that the real roots are always unique, at least within the physically meaningful range $0<R_c,R_c^{\prime }<1$.

Then, in the method’s second step, the directly measured ${\theta }_c$ along with the numerically retrieved $R_c$ enable the calculation of the complex optical constants via Equation (3). Exactly the same result is obtained by use of ${\theta }_c^{\prime }$ and $R_c^{\prime }$, instead.

Figure 2a depicts graphically the method’s operating principle. Therein, the iso-${\theta }_c$ curve is plotted in the ($n,k$) plane, representing the hypothetical measurement of a critical angle ${\theta }_c=48.5^{\circ }$ with the first prism; the critical angle measured with the second prism ${\theta }_c^{\prime }$ (or equivalently, the angular shift $\mathrm{\Delta }$) varies in steps of $0.{001}^{\circ }$, an amount that simulates the resolution of state-of-the-art rotary tables. Any given value of $\mathrm{\Delta }$ defines a single point in the plot; its coordinates yield the sample’s optical constants.

A complementary picture is drawn in Figure 2b, where families of iso-n and iso-k curves are plotted in the (${\theta }_c,\mathrm{\Delta }$) plane. Examining Figure 2 leads to several conclusions, the most important of which are the following. (1) For constant ${\theta }_c$, k increases monotonically with increasing $\mathrm{\Delta }$; n does the same for low-k values, eventually reaching a characteristic turning point past which, n decreases with increasing $\mathrm{\Delta }$. (2) At the transparency limit ($k=0$), curves for s and p polarisation converge into a single point (Figure 2a) or a single line (Figure 2b); there, the polarisation independent condition of total internal reflection holds true, that is $n=sin{\theta }_c$ and $n^{\prime }=sin{\theta }_c^{\prime }$. (3) The density of points in Figure 2a is lower for p polarisation than it is for s polarisation. This observation is reflected in Figure 2b, where the grid pattern for p polarisation is more squeezed than it is for s polarisation. These facts are first indications that the method’s sensitivity is generally higher with s polarised light, as we shall further discuss next.

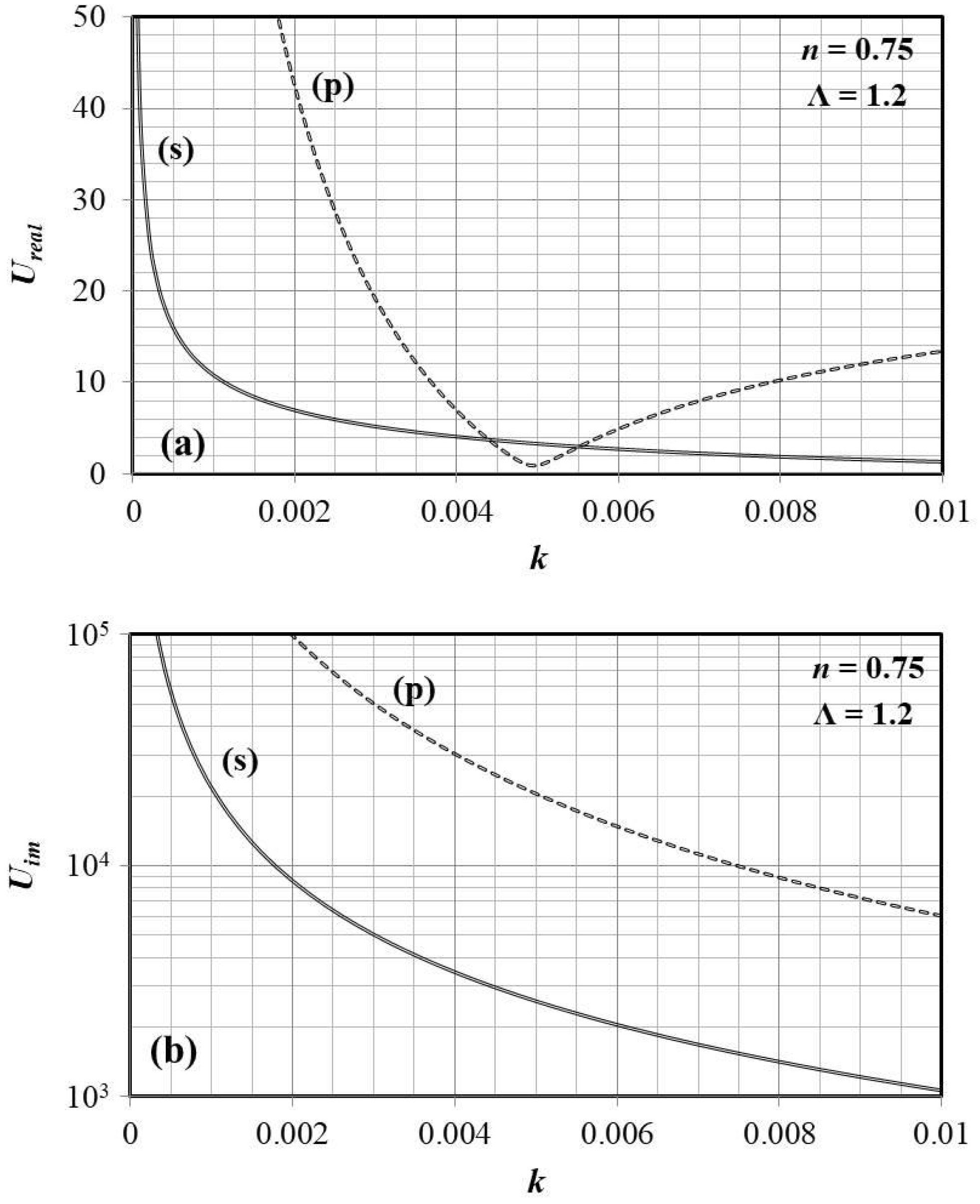
4. Uncertainty Assessment

The distinctive advantage of the proposed method is that in its first step, reflectance $R_c$ can be numerically computed from experimental input data (${\theta }_c,{\theta }_c^{\prime }$) with higher accuracy than it can be directly measured. Uncertainty $u_{R_c}$ in this computation can be expressed in terms of the input (P) and output (S) sensitivity matrices [31]

$$P=\left[\begin{array}{cc}{\displaystyle \frac{\partial F_1}{\partial {\theta }_c}}& {\displaystyle \frac{\partial F_1}{\partial {\theta }_c^{\prime }}}\\ {\displaystyle \frac{\partial F_2}{\partial {\theta }_c}}& {\displaystyle \frac{\partial F_2}{\partial {\theta }_c^{\prime }}}\end{array}\right],S=\left[\begin{array}{cc}{\displaystyle \frac{\partial F_1}{\partial R_c}}& {\displaystyle \frac{\partial F_1}{\partial R_c^{\prime }}}\\ {\displaystyle \frac{\partial F_2}{\partial R_c}}& {\displaystyle \frac{\partial F_2}{\partial R_c^{\prime }}}\end{array}\right],$$

(10)

where functions $F_1$ and $F_2$ are defined in Equations (8) and (9), respectively. The former definitions assume independence between the input variables ${\theta }_c$ and ${\theta }_c^{\prime }$, which is reasonable, since these measurands are obtained via separate experimental runs. Then, it is

$$u_{R_c}=\sqrt{K_{11}^2·u_{{\theta }_c}^2+K_{12}^2·u_{{\theta }_c^{\prime }}^2}$$

(11)

where $u_{{\theta }_c}$, $u_{{\theta }_c^{\prime }}$ are the experimental uncertainties in the measurement of the respective critical angles, while $K_{11}$, $K_{12}$ are the first row elements in the overall sensitivity matrix [31]

$$K=-S^{-1}P$$

(12)

Since the same experimental setup is to be used with both prisms, it is reasonable to assume that $u_{{\theta }_c^{\prime }}\approx u_{{\theta }_c}$. We may thus define the normalised (with respect to $u_{{\theta }_c}$) uncertainty

$$U_{R_c}=\frac{u_{R_c}}{u_{{\theta }_c}}=\sqrt{K_{11}^2+K_{12}^2}$$

(13)

The preceding expressions can be used to evaluate under what circumstances errors in the numerical determination of $R_c$ are (as we claim) smaller than corresponding errors in its direct measurement (the latter should be typically $>1\%$). We shall skip this exercise to proceed right away with the investigation of a more interesting problem: the calculation of uncertainties in the determination of the complex optical constants from the directly measured ${\theta }_c$ and the indirectly retrieved $R_c$, during the method’s second step. Based on standard error propagation rules and assuming zero covariance between ${\theta }_c$ and $R_c$, we may write for the relative uncertainties of $n_r$ (or n) and $n_i$ (or k)

$$\frac{u_{n_r}}{n_r}=\frac{u_n}{n}=\frac{1}{n}·\sqrt{{\left(\frac{\partial n}{\partial {\theta }_c}{u}_{\theta }\right)}^2+{\left(\frac{\partial n}{\partial R_c}{u}_{R_c}\right)}^2},$$

(14a)

$$\frac{u_{n_i}}{n_i}=\frac{u_k}{k}=\frac{1}{k}·\sqrt{{\left(\frac{\partial k}{\partial {\theta }_c}{u}_{\theta }\right)}^2+{\left(\frac{\partial k}{\partial R_c}{u}_{R_c}\right)}^2}.$$

(14b)

It is now more convenient to define the normalised (with respect to $u_{{\theta }_c}$) relative uncertainties of the real ($U_{real}$) and the imaginary ($U_{im}$) index which, by use of Equation (13), read:

$$U_{real}=\frac{u_{n_r}/n_r}{u_{{\theta }_c}}=\frac{1}{n}·\sqrt{{\left(\frac{\partial n}{\partial {\theta }_c}\right)}^2+{\left(\frac{\partial n}{\partial R_c}·U_{R_c}\right)}^2},$$

(15a)

$$U_{im}=\frac{u_{n_i}/n_i}{u_{{\theta }_c}}=\frac{1}{k}·\sqrt{{\left(\frac{\partial k}{\partial {\theta }_c}\right)}^2+{\left(\frac{\partial k}{\partial R_c}·U_{R_c}\right)}^2}.$$

(15b)

For any given sample and prism pair, that is for fixed $n,k$ and $\mathrm{\Lambda }$, normalised relative uncertainties $U_{real}$ and $U_{im}$ can be calculated via Equation (Section 4). Figure 3 depicts such calculations for indicative values $n=0.75,\mathrm{\Lambda }=1.2$ and variable k. In agreement with the interpretation of Figure 2, we observe that the proposed method is generally more accurate for s, than it is for p polarisation. Another striking observation is that, within the k range we account for, the method’s accuracy generally improves with increasing attenuation (that is, with increasing k). This seems to be a unique feature of our technique, since other approaches are typically expected to behave in the opposite way. Exceptionally, uncertainty $U_{real}$ for p polarisation decreases with increasing k only up to a characteristic local minimum, thereafter increasing as attenuation grows. The local minimum may be interpreted as the equivalent of the turning point observed in Figure 2a. S polarisation exhibits the same behaviour at k values beyond the scale of the horizontal axis in Figure 3.

Modern refractometers equipped with high resolution rotary tables measure critical angles with an uncertainty $u_{{\theta }_c}$ between $0.{001}^{\circ }$ and $0.{005}^{\circ }$ (that is, between 20 µrad and 100 µrad). This uncertainty range is commonly assumed to be reasonable in the bibliography [22,32], suggesting that $u_{{\theta }_c}$ is primarily limited by the minimal incremental motion of the rotary table and remains practically insensitive to other factors, such as the divergence of the input laser beam or random noise (e.g., due to laser power fluctuations and the electronic detection system). It is also assumed that systematic errors in the measurement of critical angles, such as those resulting from the off-axis placement of the prism on the rotary table, have been properly removed (to that end, we remark that it is possible to calibrate the experimental setup with the use of a refractive index certified reference material).

Therefore, to estimate the magnitude of relative errors $u_{n_r}/n_r$ and $u_{n_i}/n_i$ we need to multiply $U_{real}$ and $U_{im}$ by a factor ranging from $2·{10}^{-5}$ to ${10}^{-4}$, respectively. As k approaches $0.01$ for s polarisation (or the local minimum at $k\approx 0.005$ for p polarisation), it is $U_{real}\approx 2$, indicating that $u_{n_r}/n_r$ can be as low as $4·{10}^{-5}$, or at least remain below $2·{10}^{-4}$. Similarly, as k approaches $0.01$, it is $U_{im}\approx {10}^3$, suggesting that $u_{n_i}/n_i$ should be in the range of $2\%$ to $10\%$ for s polarisation (increased five-fold for p polarisation).

Up to now, our assessment assumed fixed n and $\mathrm{\Lambda }$. There exists some space for further error optimisation by proper choice of first and second prism, so as to tune n and $\mathrm{\Lambda }$, respectively. In general, higher n and higher $\mathrm{\Lambda }$ values reduce the real and imaginary index error for s polarised light. For p polarisation, higher $\mathrm{\Lambda }$ values have the same effect; yet, a smaller n shifts the observed local minimum of the real index error to a lower k, an effect that might be desirable in several situations.

We shall not elaborate further on these dependencies, which however attest that the proposed method can determine the complex optical constants with relative uncertainties $u_{n_r}/n_r\sim {10}^{-4}$ and $u_{n_i}/n_i\sim 10\%$, within a spectral range that extends from $k<0.001$ to $k>0.01$. These specifications compare well to standard refractometry with transparent samples (in terms of the real index), as well as ellipsometry (in terms of the imaginary index).

5. Limitations and Further Insights

The evident limitation in the practical use of our method relates to the confined attenuation span for which it remains highly accurate. However, even the narrow window, say, $0.001<k<0.01$, contains several material families of great scientific interest, ranging from food products and fuel oils to semiconductors, water and aqueous solutions (towards infrared wavelengths), as well as nearly the entirety of biological matter.

A second limitation that should be accounted for relates to the validity of Fresnel equations from which, the proposed mathematical framework originates. It is commonly accepted that, if light attenuation comes from absorption, Fresnel equations apply unconditionally. On the contrary, as is further elaborated in the bibliography [33,34], if light attenuation rises from scattering effects (that is, with inhomogeneus samples) Fresnel equations are valid only when the size of the scatterers remains small compared to the wavelength of light. Moreover, the applicability of the proposed method with inhomogeneous samples is further complicated by the fact that the same spot on the sample surface should be irradiated with both prisms.

A final aspect worth commenting on is the time duration of measurement; at first instance, one might assume that performing separate measurements on two different prisms may cause unwelcome delays. In reality, however, the proposed method spares the need for reflectance callibration, which is commonly required in prism coupling refractometry of attenuating media and involves a follow-up measurement at the prism–air interface. This simplification is due to the fact that critical angles ${\theta }_c$ and ${\theta }_c^{\prime }$ can be determined by differentiating directly the uncalibrated reflectance profile, as is measured (in arbitrary units) by the photodiode.

6. Conclusions

This letter introduces two prism critical angle refractometry as a novel method for determining the complex optical constants of attenuating media, by means of a well-posed nonlinear equation system that can be solved numerically. The proposed method exhibits several appealing features, the most important of which are summarised below.

1.

A pair of angles (${\theta }_c,{\theta }_c^{\prime }$) is all that the method needs as input. To the benefit of precision, reflectance values are not required in the computational process.

2.

The routine is general, since it applies with s and p polarised light, facilitating the characterisation of optically isotropic and anisotropic samples.

3.

Uncertainty in the determination of the output quantities ($n_r,n_i$) decreases as attenuation grows from zero, which is ideally suited for analysing media that attenuate light as much as, for example, most forms of biological matter.

Uncertainty assessment indicated that the real (or the imaginary) index can be determined with a relative error ∼${10}^{-4}$ (or ∼$10\%$), respectively. The method’s specifications meet the needs of several application in various fields, such as biomedical optics, analytical chemistry and quality control.