The Impact of Short-Range (Gaussian) Disorder Correlations on Superconducting Characteristics
Abstract
1. Introduction
2. Model
Disorder Model
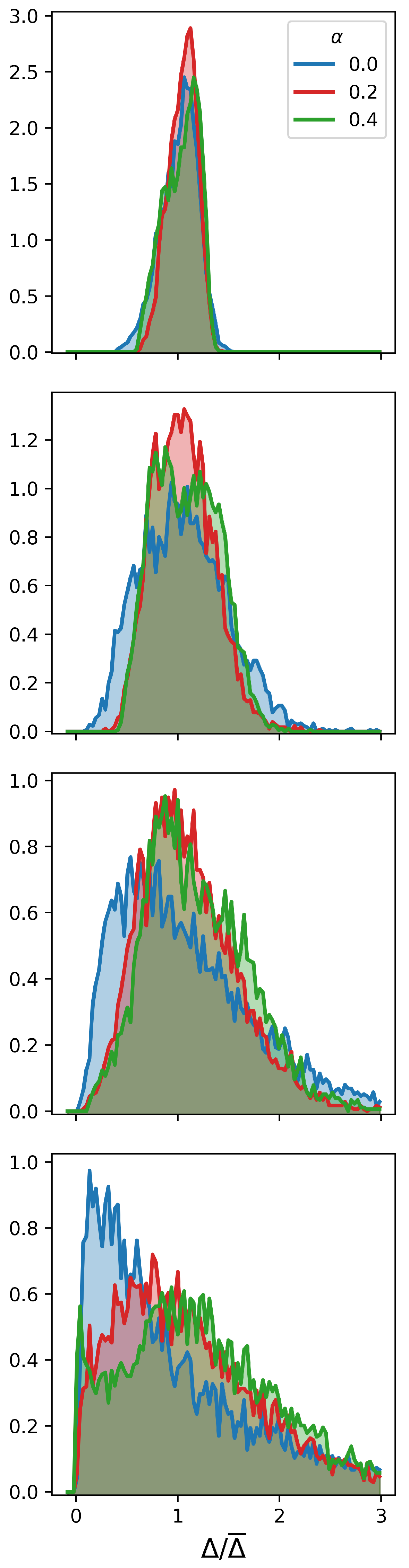
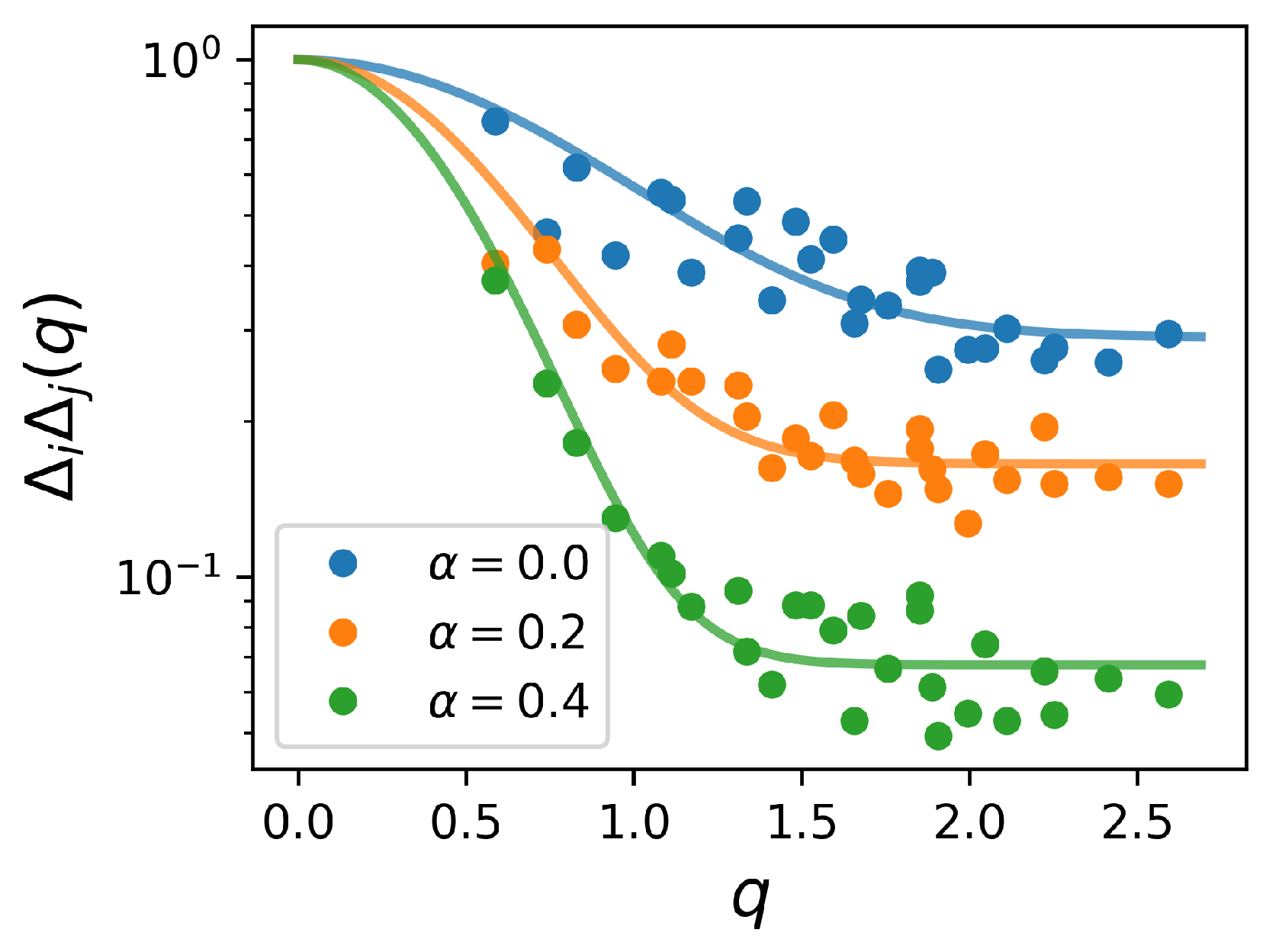
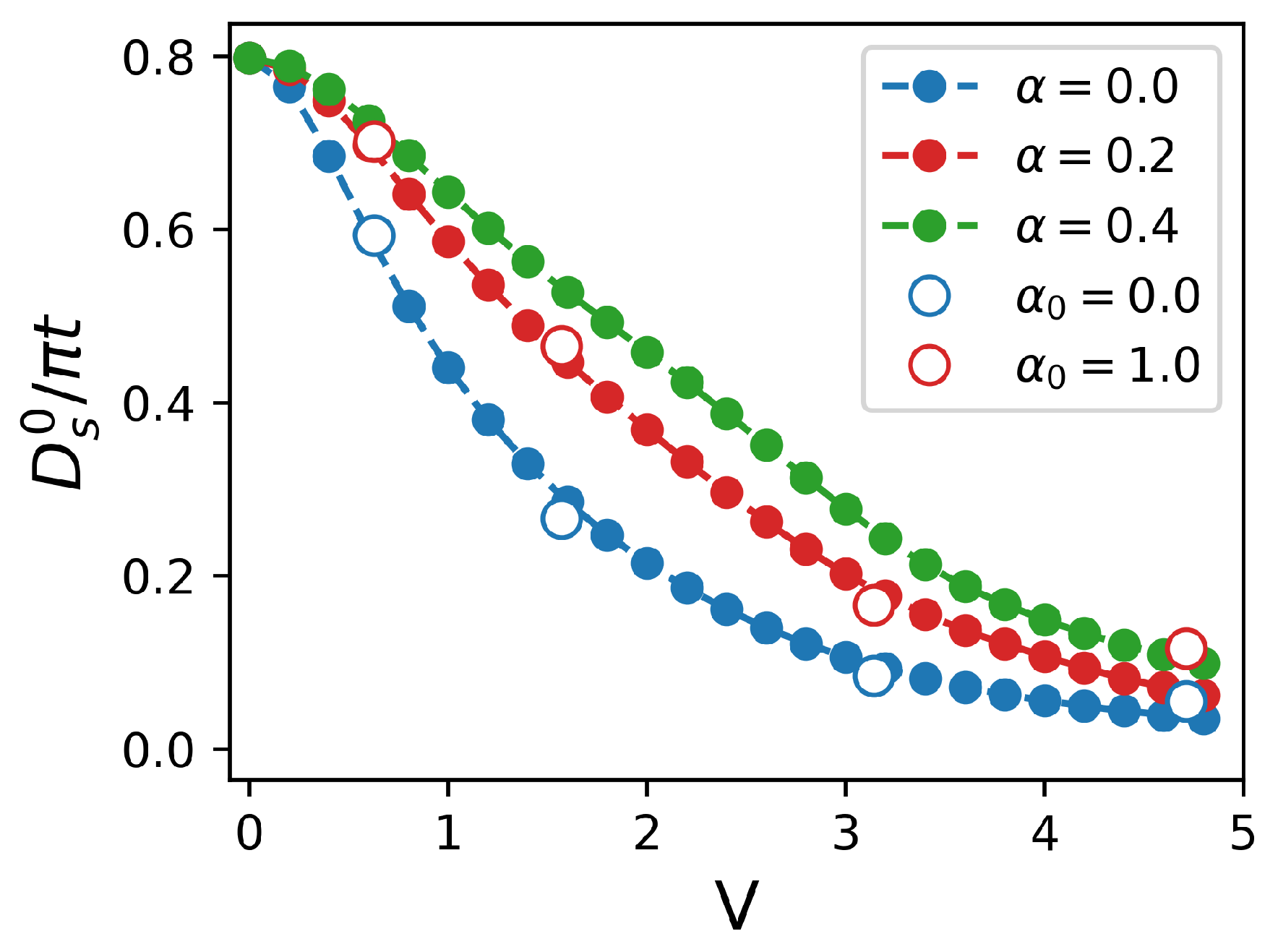
3. Results
4. Discussion
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Feigel’man, M.V.; Ioffe, L.B.; Kravtsov, V.E.; Yuzbashyan, E.A. Eigenfunction Fractality and Pseudogap State near the Superconductor-Insulator Transition. Phys. Rev. Lett. 2007, 98, 27001. [Google Scholar] [CrossRef]
- Burmistrov, I.S.; Gornyi, I.V.; Mirlin, A.D. Enhancement of the Critical Temperature of Superconductors by Anderson Localization. Phys. Rev. Lett. 2012, 108, 17002. [Google Scholar] [CrossRef] [PubMed]
- Stosiek, M.; Lang, B.; Evers, F. Self-consistent-field ensembles of disordered Hamiltonians: Efficient solver and application to superconducting films. Phys. Rev. B 2020, 101, 144503. [Google Scholar] [CrossRef]
- Goldman, A.M.; Marković, N. Superconductor-Insulator Transitions in the Two-Dimensional Limit. Phys. Today 1998, 51, 39–44. [Google Scholar] [CrossRef]
- Ma, M.; Lee, P.A. Localized superconductors. Phys. Rev. B 1985, 32, 5658–5667. [Google Scholar] [CrossRef] [PubMed]
- Gantmakher, V.F.; Dolgopolov, V.T. Superconductor-insulator quantum phase transition. Uspekhi Fizicheskih Nauk 2010, 180, 3. [Google Scholar] [CrossRef]
- Sadovskii, M.V. Superconductivity and localization. Phys. Rep. 1997, 282, 225–348. [Google Scholar] [CrossRef]
- Trivedi, N.; Scalettar, R.T.; Randeria, M. Superconductor-insulator transition in a disordered electronic system. Phys. Rev. B 1996, 54, R3756–R3759. [Google Scholar] [CrossRef]
- Trivedi, N.; Loh, Y.L.; Bouadim, K.; Randeria, M. Emergent granularity and pseudogap near the superconductor-insulator transition. J. Phys. Conf. Ser. 2012, 376, 12001. [Google Scholar] [CrossRef]
- Gastiasoro, M.N.; Andersen, B.M. Enhancing superconductivity by disorder. Phys. Rev. B 2018, 98, 184510. [Google Scholar] [CrossRef]
- Neverov, V.D.; Lukyanov, A.E.; Krasavin, A.V.; Vagov, A.; Croitoru, M.D. Correlated disorder as a way towards robust superconductivity. Commun. Phys. 2022, 5, 177. [Google Scholar] [CrossRef]
- Sacépé, B.; Feigel’man, M.; Klapwijk, T.M. Quantum breakdown of superconductivity in low-dimensional materials. Nat. Phys. 2020, 16, 734–746. [Google Scholar] [CrossRef]
- Croitoru, M.D.; Shanenko, A.A.; Peeters, F.M.; Axt, V.M. Parity-fluctuation induced enlargement of the ratio ΔE/kBTc in metallic grains. Phys. Rev. B 2011, 84, 214518. [Google Scholar] [CrossRef]
- Saini, N.L.; Rossetti, T.; Lanzara, A.; Missori, M.; Perali, A.; Oyanagi, H.; Bianconi, A. Tuning of the Fermi level at the second subband of a superlattice of quantum wires in the CuO2 plane: A possible mechanism to raise the critical temperature. J. Supercond. 1996, 9, 343–348. [Google Scholar] [CrossRef]
- Castro, D.D.; Agrestini, S.; Campi, G.; Cassetta, A.; Colapietro, M.; Congeduti, A.; Continenza, A.; Negri, S.D.; Giovannini, M.; Massidda, S.; et al. European Physical Society Italian Physical Society EDP Sciences The Institute of Physics The amplification of the superconducting Tc by combined effect of tuning of the Fermi level and the tensile micro-strain in Al1-xMgxB2. Europhys. Lett. (EPL) 2002, 58, 278–284. [Google Scholar] [CrossRef]
- Silveira, R.d.L.; Croitoru, M.D.; Pugach, N.G.; Romaguera, A.R.d.C.; Albino Aguiar, J. Engineering low-temperature proximity effect in clean metals by spectral singularities. New J. Phys. 2023, 25, 93009. [Google Scholar] [CrossRef]
- Fang, L.; Jia, Y.; Mishra, V.; Chaparro, C.; Vlasko-Vlasov, V.K.; Koshelev, A.E.; Welp, U.; Crabtree, G.W.; Zhu, S.; Zhigadlo, N.D.; et al. Huge critical current density and tailored superconducting anisotropy in SmFeAsO0.8F0.15 by low-density columnar-defect incorporation. Nat. Commun. 2013, 4, 3655. [Google Scholar] [CrossRef]
- Ghigo, G.; Torsello, D.; Ummarino, G.; Gozzelino, L.; Tanatar, M.; Prozorov, R.; Canfield, P. Disorder-Driven Transition Superconducting Order Parameter in Proton Irradiated Single Crystals. Phys. Rev. Lett. 2018, 121, 107001. [Google Scholar] [CrossRef]
- Zhao, K.; Lin, H.; Xiao, X.; Huang, W.; Yao, W.; Yan, M.; Xing, Y.; Zhang, Q.; Li, Z.X.; Hoshino, S.; et al. Disorder-induced multifractal superconductivity in monolayer niobium dichalcogenides. Nat. Phys. 2019, 15, 904–910. [Google Scholar] [CrossRef]
- Tsai, W.F.; Yao, H.; Läuchli, A.; Kivelson, S.A. Optimal inhomogeneity for superconductivity: Finite-size studies. Phys. Rev. B 2008, 77, 214502. [Google Scholar] [CrossRef]
- Ghosal, A.; Randeria, M.; Trivedi, N. Role of Spatial Amplitude Fluctuations in Highly Disordereds-Wave Superconductors. Phys. Rev. Lett. 1998, 81, 3940–3943. [Google Scholar] [CrossRef]
- Ghosal, A.; Randeria, M.; Trivedi, N. Inhomogeneous pairing in highly disordereds-wave superconductors. Phys. Rev. B 2001, 65, 14501. [Google Scholar] [CrossRef]
- Dubi, Y.; Meir, Y.; Avishai, Y. Nature of the superconductor–insulator transition in disordered superconductors. Nature 2007, 449, 876–880. [Google Scholar] [CrossRef]
- Brun, C.; Cren, T.; Cherkez, V.; Debontridder, F.; Pons, S.; Fokin, D.; Tringides, M.C.; Bozhko, S.; Ioffe, L.B.; Altshuler, B.L.; et al. Remarkable effects of disorder on superconductivity of single atomic layers of lead on silicon. Nat. Phys. 2014, 10, 444–450. [Google Scholar] [CrossRef]
- Lemarié, G.; Kamlapure, A.; Bucheli, D.; Benfatto, L.; Lorenzana, J.; Seibold, G.; Ganguli, S.C.; Raychaudhuri, P.; Castellani, C. Universal scaling of the order-parameter distribution in strongly disordered superconductors. Phys. Rev. B 2013, 87, 184509. [Google Scholar] [CrossRef]
- Noat, Y.; Cherkez, V.; Brun, C.; Cren, T.; Carbillet, C.; Debontridder, F.; Ilin, K.; Siegel, M.; Semenov, A.; Hubers, H.W.; et al. Unconventional superconductivity in ultrathin superconducting NbN films studied by scanning tunneling spectroscopy. Phys. Rev. B 2013, 88, 14503. [Google Scholar] [CrossRef]
- Mondal, M.; Kamlapure, A.; Chand, M.; Saraswat, G.; Kumar, S.; Jesudasan, J.; Benfatto, L.; Tripathi, V.; Raychaudhuri, P. Phase Fluctuations in a Strongly Disordereds-Wave NbN Superconductor Close to the Metal-Insulator Transition. Phys. Rev. Lett. 2011, 106, 47001. [Google Scholar] [CrossRef]
- Mondal, M.; Kamlapure, A.; Ganguli, S.C.; Jesudasan, J.; Bagwe, V.; Benfatto, L.; Raychaudhuri, P. Enhancement of the finite-frequency superfluid response in the pseudogap regime of strongly disordered superconducting films. Sci. Rep. 2013, 3, 1357. [Google Scholar] [CrossRef]
- Saito, Y.; Nojima, T.; Iwasa, Y. Highly crystalline 2D superconductors. Nat. Rev. Mater. 2016, 2, 16094. [Google Scholar] [CrossRef]
- Petrović, A.P.; Ansermet, D.; Chernyshov, D.; Hoesch, M.; Salloum, D.; Gougeon, P.; Potel, M.; Boeri, L.; Panagopoulos, C. A disorder-enhanced quasi-one-dimensional superconductor. Nat. Commun. 2016, 7, 12262. [Google Scholar] [CrossRef]
- Peng, J.; Yu, Z.; Wu, J.; Zhou, Y.; Guo, Y.; Li, Z.; Zhao, J.; Wu, C.; Xie, Y. Disorder Enhanced Superconductivity toward TaS2 Monolayer. ACS Nano 2018, 12, 9461–9466. [Google Scholar] [CrossRef]
- Feigel’man, M.; Ioffe, L.; Kravtsov, V.; Cuevas, E. Fractal superconductivity near localization threshold. Ann. Phys. 2010, 325, 1390–1478. [Google Scholar] [CrossRef]
- Weinrib, A.; Halperin, B.I. Critical phenomena in systems with long-range-correlated quenched disorder. Phys. Rev. B 1983, 27, 413–427. [Google Scholar] [CrossRef]
- de Moura, F.A.B.F.; Lyra, M.L. Delocalization in the 1D Anderson Model with Long-Range Correlated Disorder. Phys. Rev. Lett. 1998, 81, 3735. [Google Scholar] [CrossRef]
- Carpena, P.; Bernaola-Galván, P.; Ivanov, P.C.; Stanley, H.E. Metal–insulator transition in chains with correlated disorder. Nature 2002, 418, 955–959. [Google Scholar] [CrossRef] [PubMed]
- Kawarabayashi, T.; Ono, Y.; Ohtsuki, T.; Kettemann, S.; Struck, A.; Kramer, B. Unconventional conductance plateau transitions in quantum Hall wires with spatially correlated disorder. Phys. Rev. B 2007, 75, 235317. [Google Scholar] [CrossRef]
- Pilati, S.; Giorgini, S.; Prokof’ev, N. Superfluid Transition in a Bose Gas with Correlated Disorder. Phys. Rev. Lett. 2009, 102, 150402. [Google Scholar] [CrossRef] [PubMed]
- Liew, S.F.; Cao, H. Optical properties of 1D photonic crystals with correlated and uncorrelated disorder. J. Opt. 2010, 12, 24011. [Google Scholar] [CrossRef]
- Keen, D.A.; Goodwin, A.L. The crystallography of correlated disorder. Nature 2015, 521, 303–309. [Google Scholar] [CrossRef]
- Simonov, A.; Goodwin, A.L. Designing disorder into crystalline materials. Nat. Rev. Chem. 2020, 4, 657–673. [Google Scholar] [CrossRef]
- Damasceno, P.F.; Engel, M.; Glotzer, S.C. Predictive Self-Assembly of Polyhedra into Complex Structures. Science 2012, 337, 453–457. [Google Scholar] [CrossRef] [PubMed]
- Dzero, M.; Huang, X. Correlated disorder in a Kondo lattice. J. Phys. Condens. Matter 2012, 24, 75603. [Google Scholar] [CrossRef] [PubMed]
- Bonzom, V.; Gurau, R.; Smerlak, M. Universality in p-spin glasses with correlated disorder. J. Stat. Mech. Theory Exp. 2013, 2013, L02003. [Google Scholar] [CrossRef]
- Girschik, A.; Libisch, F.; Rotter, S. Topological insulator in the presence of spatially correlated disorder. Phys. Rev. B 2013, 88, 14201. [Google Scholar] [CrossRef]
- Alamir, A.; Capuzzi, P.; Kashanian, S.V.; Vignolo, P. Probing quantum transport by engineering correlations in a speckle potential. Phys. Rev. A 2014, 89, 23613. [Google Scholar] [CrossRef]
- Pilati, S.; Fratini, E. Ferromagnetism in a repulsive atomic Fermi gas with correlated disorder. Phys. Rev. A 2016, 93, 51604. [Google Scholar] [CrossRef]
- Zierenberg, J.; Fricke, N.; Marenz, M.; Spitzner, F.P.; Blavatska, V.; Janke, W. Percolation thresholds and fractal dimensions for square and cubic lattices with long-range correlated defects. Phys. Rev. E 2017, 96, 62125. [Google Scholar] [CrossRef]
- Campi, G.; Gioacchino, M.D.; Poccia, N.; Ricci, A.; Burghammer, M.; Ciasca, G.; Bianconi, A. Nanoscale Correlated Disorder in Out-of-Equilibrium Myelin Ultrastructure. ACS Nano 2017, 12, 729–739. [Google Scholar] [CrossRef] [PubMed]
- Dikopoltsev, A.; Herzig Sheinfux, H.; Segev, M. Localization by virtual transitions in correlated disorder. Phys. Rev. B 2019, 100, 140202. [Google Scholar] [CrossRef]
- Mangelis, P.; Koch, R.J.; Lei, H.; Neder, R.B.; McDonnell, M.T.; Feygenson, M.; Petrovic, C.; Lappas, A.; Bozin, E.S. Correlated disorder-to-order crossover in the local structure of KxFe2-ySe2-zSz. Phys. Rev. B 2019, 100, 94108. [Google Scholar] [CrossRef]
- Derlet, P.M. Correlated disorder in a model binary glass through a local SU(2) bonding topology. Phys. Rev. Mater. 2020, 4, 125601. [Google Scholar] [CrossRef]
- Gavrichkov, V.A.; Shan’ko, Y.; Zamkova, N.G.; Bianconi, A. Is There Any Hidden Symmetry in the Stripe Structure of Perovskite High-Temperature Superconductors? J. Phys. Chem. Lett. 2019, 10, 1840–1844. [Google Scholar] [CrossRef]
- Fomin, I.A. Effect of correlated disorder on the temperature of unconventional cooper pairing: 3He in aerogel. JETP Lett. 2008, 88, 59–63. [Google Scholar] [CrossRef][Green Version]
- Fomin, I.A. Anomalous temperature dependence of the order parameter of a superconductor with weakly correlated impurities. JETP Lett. 2011, 93, 144–146. [Google Scholar] [CrossRef]
- Shi, R.; Fang, W.H.; Vasenko, A.S.; Long, R.; Prezhdo, O.V. Efficient passivation of DY center in CH3NH3PbBr3 by chlorine: Quantum molecular dynamics. Nano Res. 2021, 15, 2112–2122. [Google Scholar] [CrossRef]
- Zhao, X.; Vasenko, A.S.; Prezhdo, O.V.; Long, R. Anion Doping Delays Nonradiative Electron–Hole Recombination in Cs-Based All-Inorganic Perovskites: Time Domain ab Initio Analysis. J. Phys. Chem. Lett. 2022, 13, 11375–11382. [Google Scholar] [CrossRef]
- Wu, Y.; Liu, D.; Chu, W.; Wang, B.; Vasenko, A.S.; Prezhdo, O.V. Fluctuations at Metal Halide Perovskite Grain Boundaries Create Transient Trap States: Machine Learning Assisted Ab Initio Analysis. ACS Appl. Mater. Interfaces 2022, 14, 55753–55761. [Google Scholar] [CrossRef] [PubMed]
- Wu, Y.; Chu, W.; Vasenko, A.S.; Prezhdo, O.V. Common Defects Accelerate Charge Carrier Recombination in CsSnI3 without Creating Mid-Gap States. J. Phys. Chem. Lett. 2021, 12, 1948–7185. [Google Scholar] [CrossRef]
- Liu, D.; Wu, Y.; Vasenko, A.S.; Prezhdo, O.V. Grain boundary sliding and distortion on a nanosecond timescale induce trap states in CsPbBr3: ab initio investigation with machine learning force field. Nanoscale 2023, 15, 285–293. [Google Scholar] [CrossRef]
- Clément, D.; Varón, A.F.; Retter, J.A.; Sanchez-Palencia, L.; Aspect, A.; Bouyer, P. Experimental study of the transport of coherent interacting matter-waves in a 1D random potential induced by laser speckle. New J. Phys. 2006, 8, 165. [Google Scholar] [CrossRef]
- Billy, J.; Josse, V.; Zuo, Z.; Bernard, A.; Hambrecht, B.; Lugan, P.; Clément, D.; Sanchez-Palencia, L.; Bouyer, P.; Aspect, A. Direct observation of Anderson localization of matter waves in a controlled disorder. Nature 2008, 453, 891–894. [Google Scholar] [CrossRef] [PubMed]
- Aspect, A.; Inguscio, M. Anderson localization of ultracold atoms. Phys. Today 2009, 62, 30–35. [Google Scholar] [CrossRef]
- Delande, D.; Orso, G. Mobility Edge for Cold Atoms in Laser Speckle Potentials. Phys. Rev. Lett. 2014, 113, 60601. [Google Scholar] [CrossRef] [PubMed]
- Astrakharchik, G.E.; Krutitsky, K.V.; Navez, P. Phase diagram of quasi-two-dimensional bosons in a laser-speckle potential. Phys. Rev. A 2013, 87, 61601. [Google Scholar] [CrossRef]
- Fratini, M.; Poccia, N.; Ricci, A.; Campi, G.; Burghammer, M.; Aeppli, G.; Bianconi, A. Scale-free structural organization of oxygen interstitials in La2CuO4+y. Nature 2010, 466, 841–844. [Google Scholar] [CrossRef]
- Gurevich, E.; Iomin, A. Generalized Lyapunov exponent and transmission statistics in one-dimensional Gaussian correlated potentials. Phys. Rev. E 2011, 83, 11128. [Google Scholar] [CrossRef]
- Jing-Hui, L. System Driven by Correlated Gaussian Noises Related with Disorder. Chin. Phys. Lett. 2007, 24, 2505–2508. [Google Scholar] [CrossRef]
- Wang, Y.; Nandkishore, R.M. Interplay between short-range correlated disorder and Coulomb interaction in nodal-line semimetals. Phys. Rev. B 2017, 96, 115130. [Google Scholar] [CrossRef]
- Horner, H. Drift, creep and pinning of a particle in a correlated random potential. Zeitschrift für Phys. B Condens. Matter 1996, 100, 243–257. [Google Scholar] [CrossRef][Green Version]
- Izrailev, F.M.; Krokhin, A.A. Localization and the Mobility Edge in One-Dimensional Potentials with Correlated Disorder. Phys. Rev. Lett. 1999, 82, 4062–4065. [Google Scholar] [CrossRef]
- Lugan, P.; Aspect, A.; Sanchez-Palencia, L.; Delande, D.; Grémaud, B.; Müller, C.A.; Miniatura, C. One-dimensional Anderson localization in certain correlated random potentials. Phys. Rev. A 2009, 80, 23605. [Google Scholar] [CrossRef]
- Das, S.K.; Singh, V.N.; Majumdar, P. Magnon spectrum in the domain ferromagnetic state of antisite-disordered double perovskites. Phys. Rev. B 2013, 88, 214428. [Google Scholar] [CrossRef]
- Marcuzzi, M.; Minář, J.; Barredo, D.; de Léséleuc, S.; Labuhn, H.; Lahaye, T.; Browaeys, A.; Levi, E.; Lesanovsky, I. Facilitation Dynamics and Localization Phenomena in Rydberg Lattice Gases with Position Disorder. Phys. Rev. Lett. 2017, 118, 63606. [Google Scholar] [CrossRef] [PubMed]
- Ro, S.; Kafri, Y.; Kardar, M.; Tailleur, J. Disorder-Induced Long-Ranged Correlations in Scalar Active Matter. Phys. Rev. Lett. 2021, 126, 48003. [Google Scholar] [CrossRef] [PubMed]
- Micnas, R.; Ranninger, J.; Robaszkiewicz, S. Superconductivity in narrow-band systems with local nonretarded attractive interactions. Rev. Mod. Phys. 1990, 62, 113–171. [Google Scholar] [CrossRef]
- Zhu, J.X. Bogoliubov-de Gennes Method and Its Applications; Springer: Berlin/Heidelberg, Germany, 2016; Volume 924. [Google Scholar]
- de Braganca, R.H.; Croitoru, M.D.; Shanenko, A.A.; Aguiar, J.A. Effect of Material-Dependent Boundaries on the Interference Induced Enhancement of the Surface Superconductivity Temperature. J. Phys. Chem. Lett. 2023, 14, 5657–5664. [Google Scholar] [CrossRef]
- Hannibal, S.; Kettmann, P.; Croitoru, M.D.; Axt, V.M.; Kuhn, T. Persistent oscillations of the order parameter and interaction quench phase diagram for a confined Bardeen-Cooper-Schrieffer Fermi gas. Phys. Rev. A 2018, 98, 53605. [Google Scholar] [CrossRef]
- Hannibal, S.; Kettmann, P.; Croitoru, M.D.; Axt, V.M.; Kuhn, T. Dynamical vanishing of the order parameter in a confined Bardeen-Cooper-Schrieffer Fermi gas after an interaction quench. Phys. Rev. A 2018, 97, 13619. [Google Scholar] [CrossRef]
- Tarat, S.; Majumdar, P. Charge dynamics across the disorder-driven superconductor-insulator transition. EPL (Europhys. Lett.) 2014, 105, 67002. [Google Scholar] [CrossRef][Green Version]
- Croitoru, M.D.; Shanenko, A.A.; Vagov, A.; Milošević, M.V.; Axt, V.M.; Peeters, F.M. Phonon limited superconducting correlations in metallic nanograins. Sci. Rep. 2015, 5, 16515. [Google Scholar] [CrossRef]
- Croitoru, M.D.; Shanenko, A.A.; Vagov, A.; Vasenko, A.S.; Milošević, M.V.; Axt, V.M.; Peeters, F.M. Influence of Disorder on Superconducting Correlations in Nanoparticles. J. Supercond. Nov. Magn. 2016, 29, 605–609. [Google Scholar] [CrossRef]
- Makse, H.A.; Havlin, S.; Schwartz, M.; Stanley, H.E. Method for generating long-range correlations for large systems. Phys. Rev. E 1996, 53, 5445–5449. [Google Scholar] [CrossRef]
- Hu, K.; Ivanov, P.C.; Chen, Z.; Carpena, P.; Eugene Stanley, H. Effect of trends on detrended fluctuation analysis. Phys. Rev. E 2001, 64, 11114. [Google Scholar] [CrossRef] [PubMed]
- Chen, Z.; Hu, K.; Carpena, P.; Bernaola-Galvan, P.; Stanley, H.E.; Ivanov, P.C. Effect of nonlinear filters on detrended fluctuation analysis. Phys. Rev. E 2005, 71, 11104. [Google Scholar] [CrossRef]
- Coronado, A.V.; Carpena, P. Size Effects on Correlation Measures. J. Biol. Phys. 2005, 31, 121–133. [Google Scholar] [CrossRef] [PubMed]
- Croitoru, M.D.; Gladilin, V.N.; Fomin, V.M.; Devreese, J.T.; Kemerink, M.; Koenraad, P.M.; Sauthoff, K.; Wolter, J.H. Electroluminescence spectra of an STM-tip-induced quantum dot. Phys. Rev. B 2003, 68, 195307. [Google Scholar] [CrossRef]
- Croitoru, M.D.; Gladilin, V.N.; Fomin, V.M.; Devreese, J.T.; Magnus, W.; Schoenmaker, W.; Sorée, B. Quantum transport in a nanosize double-gate metal-oxide-semiconductor field-effect transistor. J. of Appl. Phys. 2003, 96, 2305–2310. [Google Scholar] [CrossRef]
- Kettmann, P.; Hannibal, S.; Croitoru, M.D.; Axt, V.M.; Kuhn, T. Pure Goldstone mode in the quench dynamics of a confined ultracold Fermi gas in the BCS-BEC crossover regime. Phys. Rev. A 2017, 96, 33618. [Google Scholar] [CrossRef]
- Yang, C.N. Concept of Off-Diagonal Long-Range Order and the Quantum Phases of Liquid He and of Superconductors. Rev. Mod. Phys. 1962, 34, 694–704. [Google Scholar] [CrossRef]
- Scalapino, D.J.; White, S.R.; Zhang, S.C. Superfluid density and the Drude weight of the Hubbard model. Phys. Rev. Lett. 1992, 68, 2830–2833. [Google Scholar] [CrossRef]
- Scalapino, D.J.; White, S.R.; Zhang, S. Insulator, metal, or superconductor: The criteria. Phys. Rev. B 1993, 47, 7995–8007. [Google Scholar] [CrossRef] [PubMed]
- Croitoru, M.D.; Buzdin, A.I. The Fulde–Ferrell–Larkin–Ovchinnikov state in layered d-wave superconductors: In-plane anisotropy and resonance effects in the angular dependence of the upper critical field. J. Phys. Condens. Matter 2013, 25, 125702. [Google Scholar] [CrossRef] [PubMed]
- Croitoru, M.; Buzdin, A. In Search of Unambiguous Evidence of the Fulde–Ferrell–Larkin–Ovchinnikov State in Quasi-Low Dimensional Superconductors. Condens. Matter 2017, 2, 30. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Neverov, V.D.; Lukyanov, A.E.; Krasavin, A.V.; Vagov, A.; Croitoru, M.D. The Impact of Short-Range (Gaussian) Disorder Correlations on Superconducting Characteristics. Condens. Matter 2024, 9, 6. https://doi.org/10.3390/condmat9010006
Neverov VD, Lukyanov AE, Krasavin AV, Vagov A, Croitoru MD. The Impact of Short-Range (Gaussian) Disorder Correlations on Superconducting Characteristics. Condensed Matter. 2024; 9(1):6. https://doi.org/10.3390/condmat9010006
Chicago/Turabian StyleNeverov, Vyacheslav D., Alexander E. Lukyanov, Andrey V. Krasavin, Alexei Vagov, and Mihail D. Croitoru. 2024. "The Impact of Short-Range (Gaussian) Disorder Correlations on Superconducting Characteristics" Condensed Matter 9, no. 1: 6. https://doi.org/10.3390/condmat9010006
APA StyleNeverov, V. D., Lukyanov, A. E., Krasavin, A. V., Vagov, A., & Croitoru, M. D. (2024). The Impact of Short-Range (Gaussian) Disorder Correlations on Superconducting Characteristics. Condensed Matter, 9(1), 6. https://doi.org/10.3390/condmat9010006










