Two-Dimensional Discommensurations: An Extension to McMillan’s Ginzburg–Landau Theory
Abstract
:1. Introduction
2. Results
Single-Q Free Energy
3. Incommensurate Charge Density Wave
4. Commensurate Charge Density Wave
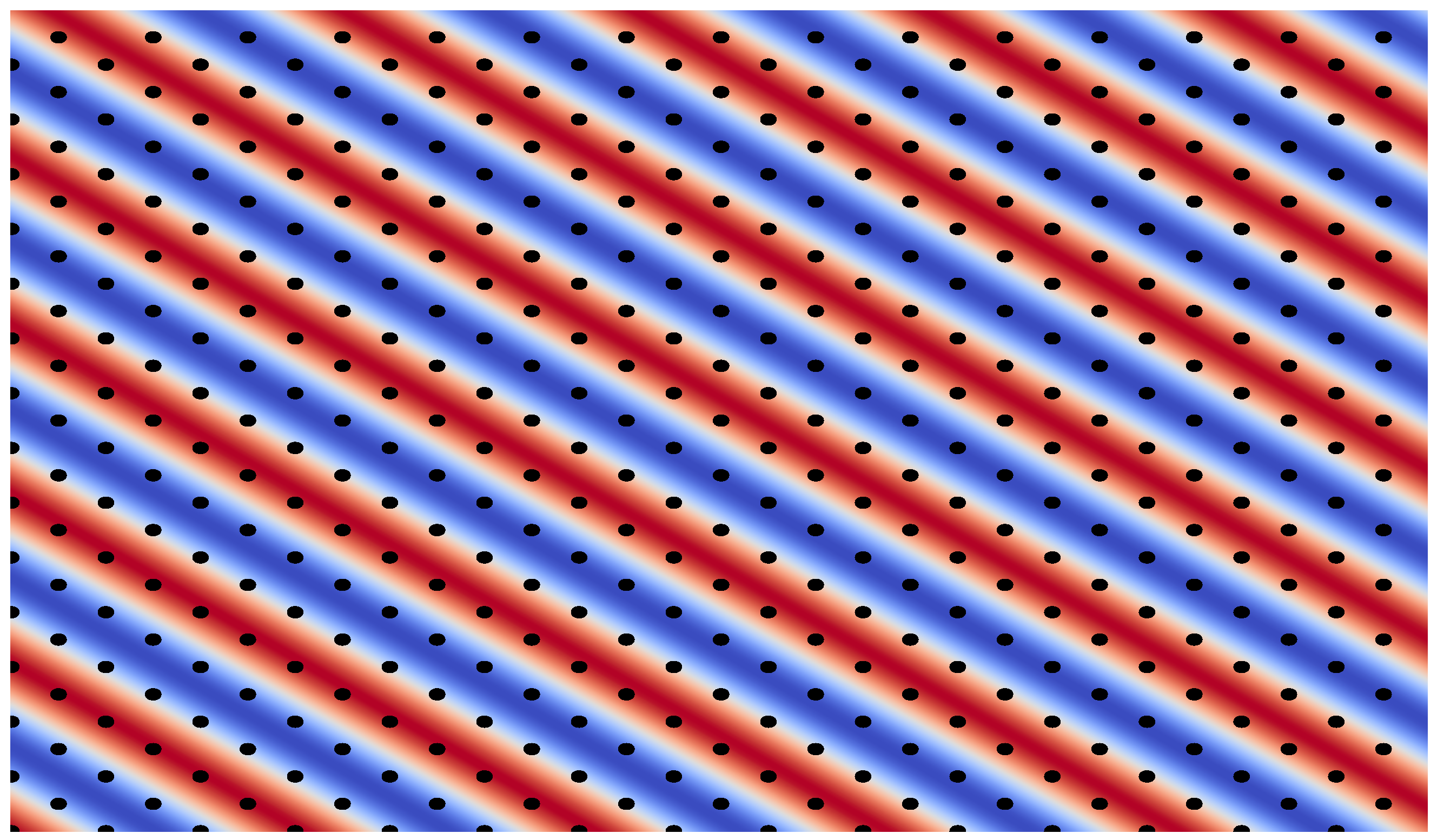
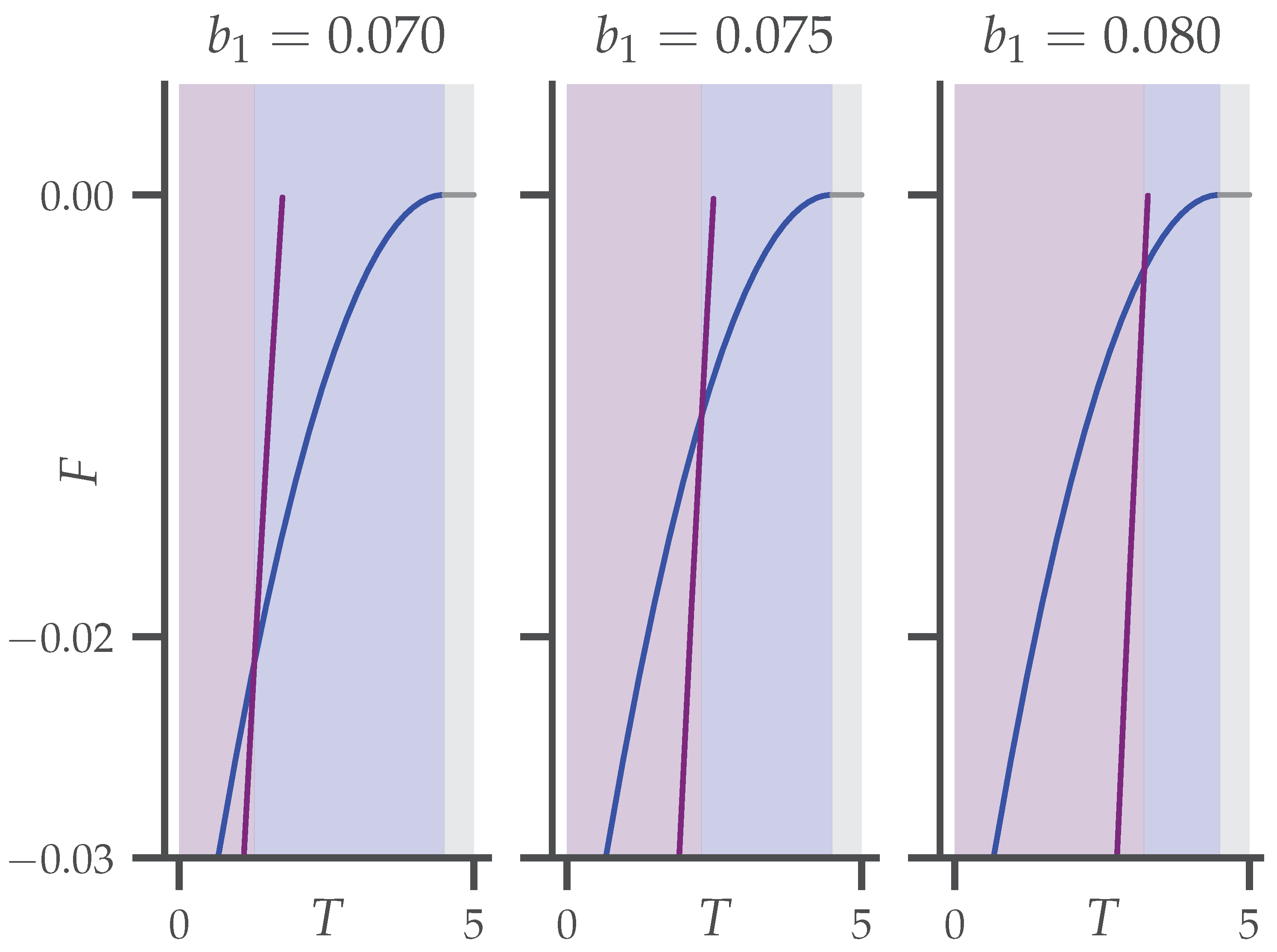
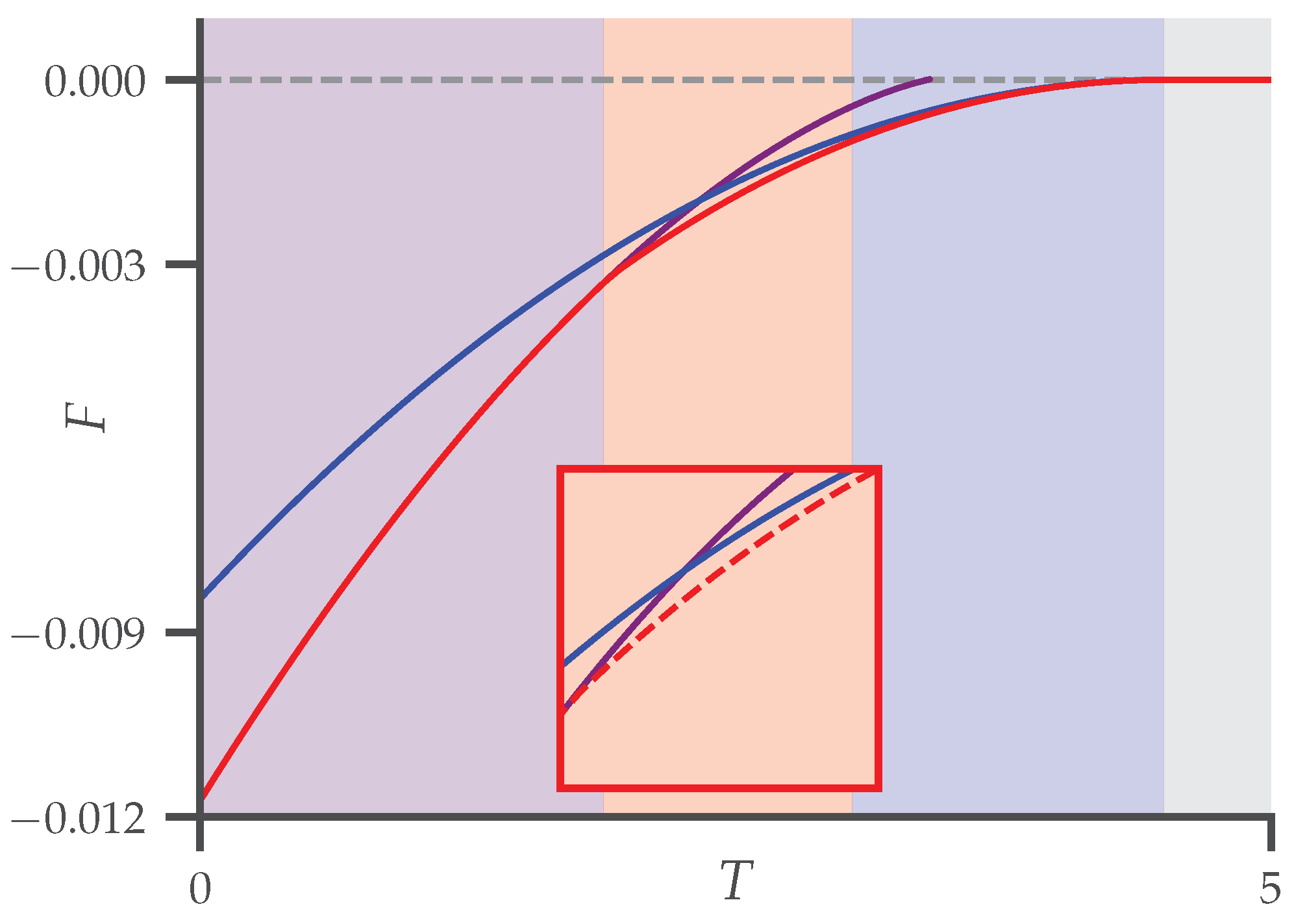
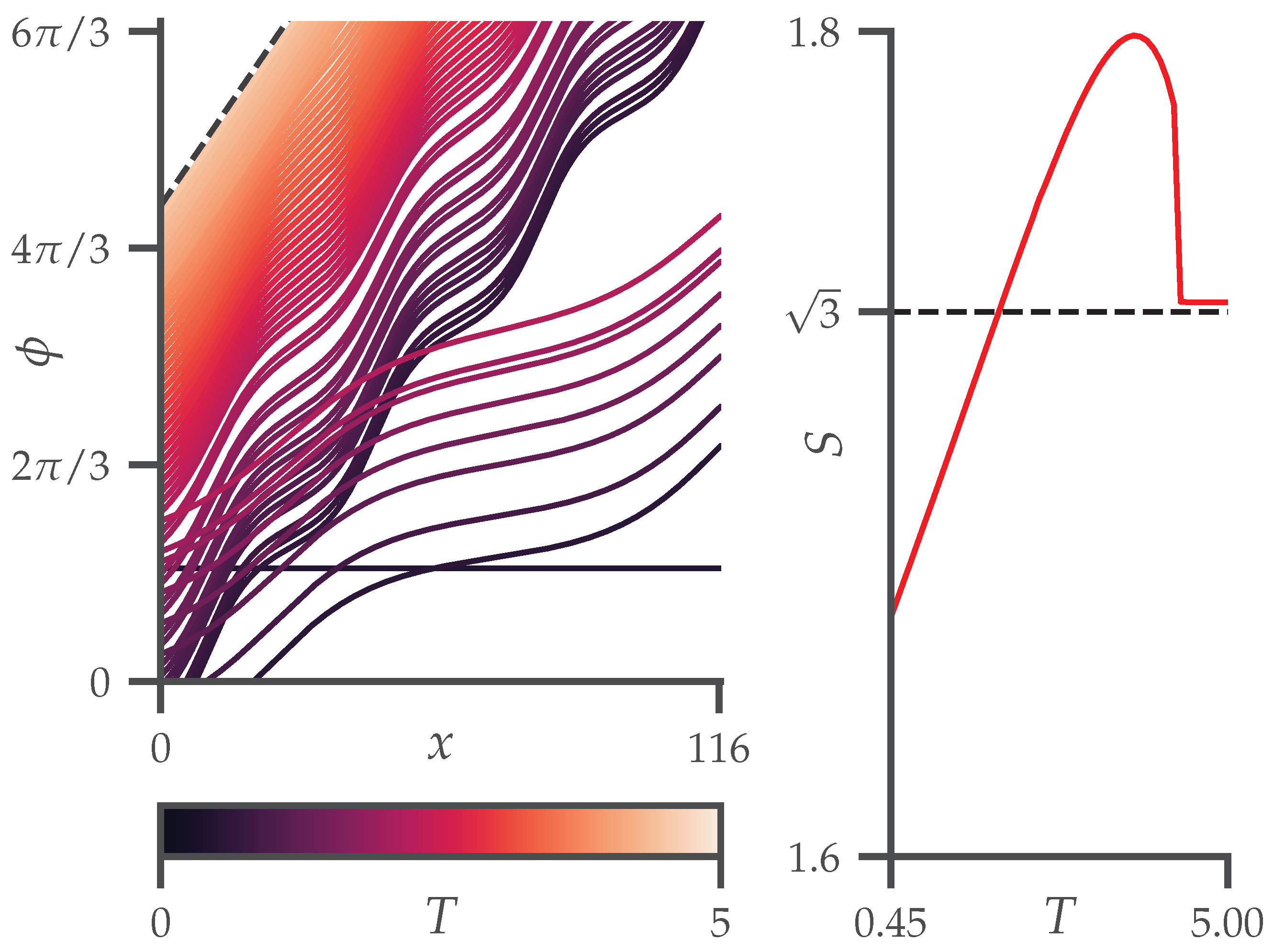
5. Discommensurations
5.1. The Equilibrium Configuration
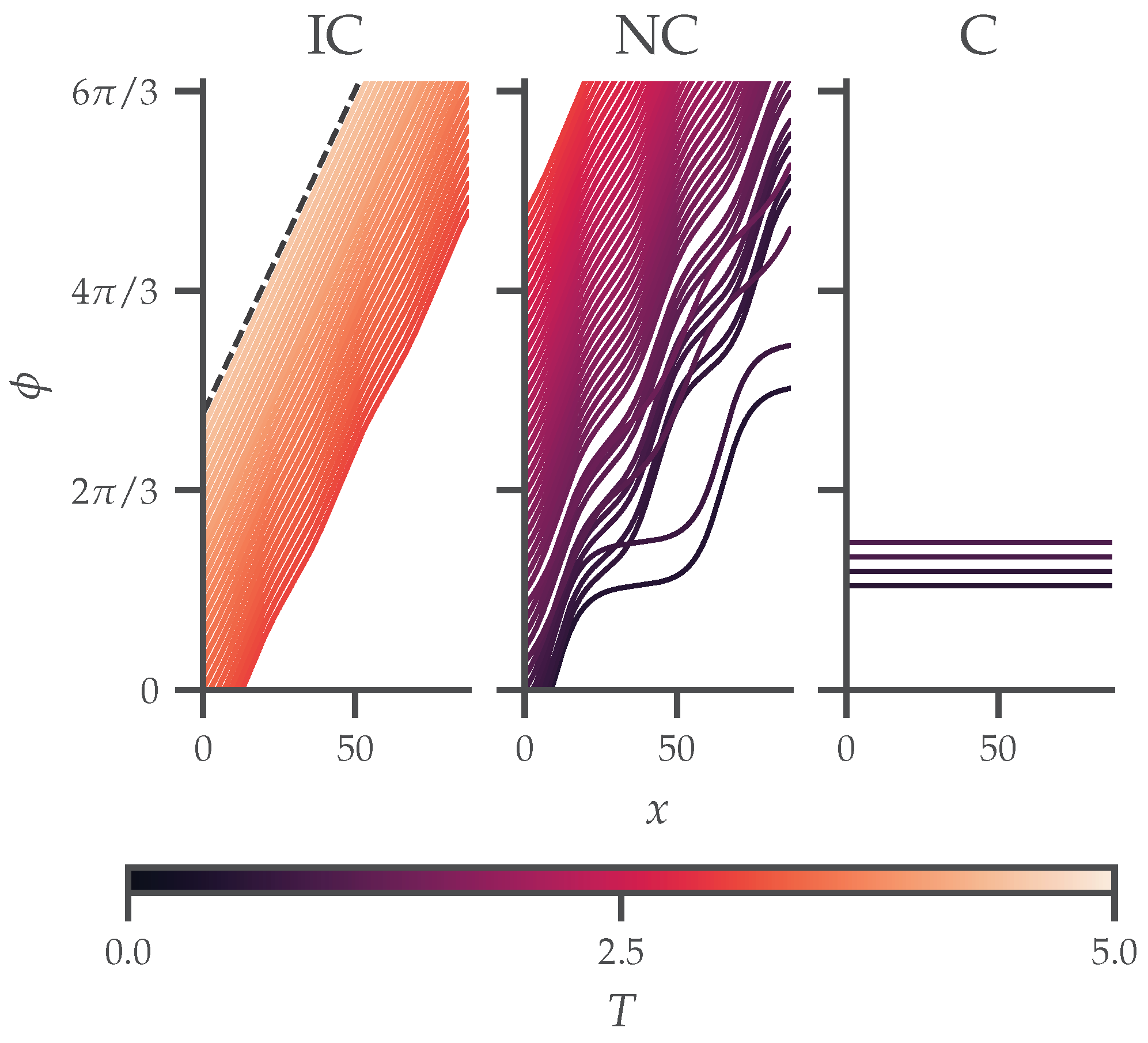
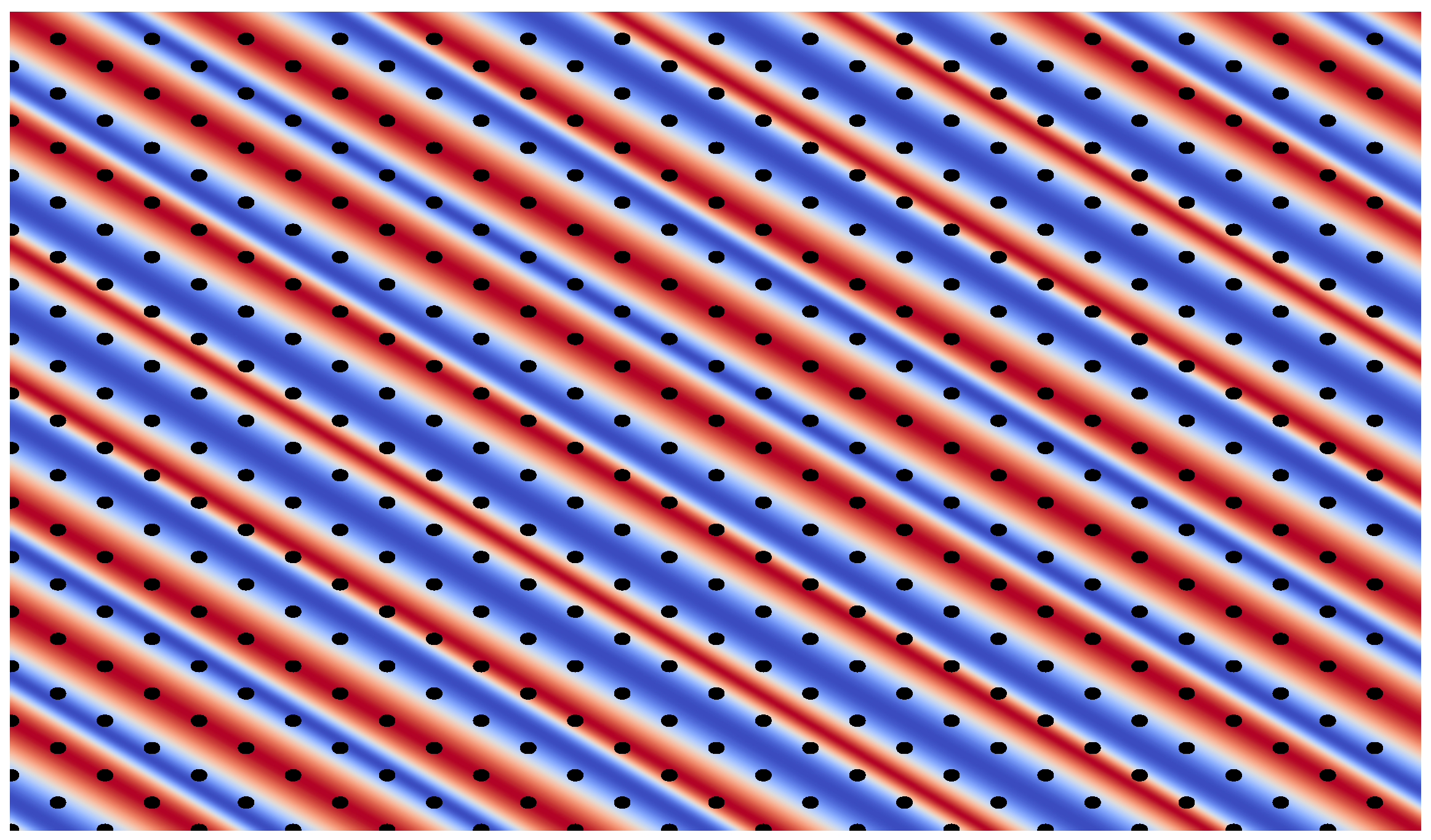
5.2. Rotation in Two Dimensions
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Silva, A.; Henke, J.; van Wezel, J. Elemental chalcogens as a minimal model for combined charge and orbital order. Phys. Rev. B 2018, 97, 045151. [Google Scholar] [CrossRef]
- Kakehashi, Y.; Kakehashi, Y. Antiferromagnetism and spin density waves. In Modern Theory of Magnetism in Metals and Alloys; Springer: Berlin, Germany, 2013; pp. 149–180. [Google Scholar]
- Chang, J.; Blackburn, E.; Holmes, A.; Christensen, N.B.; Larsen, J.; Mesot, J.; Liang, R.; Bonn, D.; Hardy, W.; Watenphul, A.; et al. Direct observation of competition between superconductivity and charge density wave order in yba2cu3o6.67. Nat. Phys. 2012, 8, 871–876. [Google Scholar] [CrossRef]
- Ghiringhelli, G.; Tacon, M.L.; Minola, M.; Blanco-Canosa, S.; Mazzoli, C.; Brookes, N.; Luca, G.D.; Frano, A.; Hawthorn, D.; He, F.; et al. Long-range incommensurate charge fluctuations in (y, nd) ba2cu3o6+ x. Science 2012, 337, 821–825. [Google Scholar] [CrossRef]
- Achkar, A.; He, F.; Sutarto, R.; McMahon, C.; Zwiebler, M.; Hücker, M.; Gu, G.; Liang, R.; Bonn, D.; Hardy, W.; et al. Orbital symmetry of charge-density-wave order in la1. 875ba0. 125cuo4 and yba2cu3o6.67. Nat. Mater. 2016, 15, 616–620. [Google Scholar] [CrossRef]
- Rosenthal, E.P.; Andrade, E.F.; Arguello, C.J.; Fernandes, R.M.; Xing, L.Y.; Wang, X.; Jin, C.; Millis, A.J.; Pasupathy, A.N. Visualization of electron nematicity and unidirectional antiferroic fluctuations at high temperatures in nafeas. Nat. Phys. 2014, 10, 225–232. [Google Scholar] [CrossRef]
- Shimojima, T.; Malaeb, W.; Nakamura, A.; Kondo, T.; Kihou, K.; Lee, C.-H.; Iyo, A.; Eisaki, H.; Ishida, S.; Nakajima, M.; et al. Antiferroic electronic structure in the nonmagnetic superconducting state of the iron-based superconductors. Sci. Adv. 2017, 3, e1700466. [Google Scholar] [CrossRef]
- Hervieu, M.; Barnabé, A.; Martin, C.; Maignan, A.; Damay, F.; Raveau, B. Evolution of charge ordering in manganites. Eur. Phys. J.-Condens. Matter Complex Syst. 1999, 8, 31–41. [Google Scholar] [CrossRef]
- Baggari, I.E.; Savitzky, B.H.; Admasu, A.S.; Kim, J.; Cheong, S.-W.; Hovden, R.; Kourkoutis, L.F. Nature and evolution of incommensurate charge order in manganites visualized with cryogenic scanning transmission electron microscopy. Proc. Natl. Acad. Sci. USA 2018, 115, 1445–1450. [Google Scholar] [CrossRef]
- Cao, Y.; Wang, Z.; Park, S.Y.; Yuan, Y.; Liu, X.; Nikitin, S.M.; Akamatsu, H.; Kareev, M.; Middey, S.; Meyers, D.; et al. Artificial two-dimensional polar metal at room temperature. Nat. Commun. 2018, 9, 1547. [Google Scholar] [CrossRef]
- Peierls, R.E. Quantum Theory of Solids; Oxford University Press: Oxford, UK, 1955. [Google Scholar]
- Peierls, R. More Surprises in Theoretical Physics; Princeton University Press: Princeton, NJ, USA, 1991. [Google Scholar]
- Landau, L. On the Theory of Phase Transitions II, Phys. Z. Soviet 545 (1937). the English Translations of Landau’s Papers Can Be Found in “Collected Papers of LD Landau”, by D. Ter Haar; Gordon and Breach: New York, NY, USA, 1965. [Google Scholar]
- McMillan, W. Landau theory of charge-density waves in transition-metal dichalcogenides. Phys. Rev. B 1975, 12, 1187. [Google Scholar] [CrossRef]
- Ginzburg, V.; Landau, L. On the theory of superconductivity. Zh. Eksp. Teor. Fiz. 1950, 20, 1064. [Google Scholar]
- Hohenberg, P.C.; Krekhov, A.P. An introduction to the Ginzburg–Landau theory of phase transitions and nonequilibrium patterns. Phys. Rep. 2015, 572, 1–42. [Google Scholar] [CrossRef]
- Pásztor, Á.; Scarfato, A.; Barreteau, C.; Giannini, E.; Renner, C. Dimensional crossover of the charge density wave transition in thin exfoliated vse2. 2D Mater. 2017, 4, 041005. [Google Scholar] [CrossRef]
- Manzeli, S.; Ovchinnikov, D.; Pasquier, D.; Yazyev, O.V.; Kis, A. 2D transition metal dichalcogenides. Nat. Rev. Mater. 2017, 2, 17033. [Google Scholar] [CrossRef]
- Campi, G.; Bianconi, A.; Ricci, A. Nanoscale phase separation of incommensurate and quasi-commensurate spin stripes in low temperature spin glass of la2- xsrxnio4. Condens. Matter 2021, 6, 45. [Google Scholar] [CrossRef]
- Leininger, P.; Chernyshov, D.; Bosak, A.; Berger, H.; Inosov, D. Competing charge density waves and temperature-dependent nesting in 2 h-tase 2. Phys. Rev. B 2011, 83, 233101. [Google Scholar] [CrossRef]
- Johannes, M.; Mazin, I.; Howells, C. Fermi-surface nesting and the origin of the charge-density wave in nb se 2. Phys. Rev. B 2006, 73, 205102. [Google Scholar] [CrossRef]
- Johannes, M.; Mazin, I. Fermi surface nesting and the origin of charge density waves in metals. Phys. Rev. B 2008, 77, 165135. [Google Scholar] [CrossRef]
- Zhu, X.; Cao, Y.; Zhang, J.; Plummer, E.; Guo, J. Classification of charge density waves based on their nature. Proc. Natl. Acad. Sci. USA 2015, 112, 2367–2371. [Google Scholar] [CrossRef]
- Bosak, A.; Souliou, S.-M.; Faugeras, C.; Heid, R.; Molas, M.R.; Chen, R.-Y.; Wang, N.-L.; Potemski, M.; Tacon, M.L. Evidence for nesting-driven charge density wave instabilities in the quasi-two-dimensional material laagsb 2. Phys. Rev. Res. 2021, 3, 033020. [Google Scholar] [CrossRef]
- Rossnagel, K.; Rotenberg, E.; Koh, H.; Smith, N.; Kipp, L. Fermi surface, charge-density-wave gap, and kinks in 2 h- tase 2. Phys. Rev. B 2005, 72, 121103. [Google Scholar] [CrossRef]
- Flicker, F.; van Wezel, J. Charge order from orbital-dependent coupling evidenced by nbse2. Nat. Commun. 2015, 6, 7034. [Google Scholar] [CrossRef] [PubMed]
- Flicker, F.; van Wezel, J. Charge order in nbse2. Phys. Rev. B 2016, 94, 235135. [Google Scholar] [CrossRef]
- Henke, J.; Flicker, F.; Laverock, J.; van Wezel, J. Charge order from structured coupling in vse2. SciPost Phys. 2020, 9, 056. [Google Scholar] [CrossRef]
- McMillan, W.L. Theory of discommensurations and the commensurate-incommensurate charge-density-wave phase transition. Phys. Rev. B 1976, 14, 1496. [Google Scholar] [CrossRef]
- McMillan, W. Microscopic model of charge-density waves in 2 h- ta se 2. Phys. Rev. B 1977, 16, 643. [Google Scholar] [CrossRef]
- Nakanishi, K.; Shiba, H. Domain-like incommensurate charge-density-wave states and the first-order incommensurate-commensurate transitions in layered tantalum dichalcogenides. i. 1t-polytype. J. Phys. Soc. Jpn. 1977, 43, 1839–1847. [Google Scholar] [CrossRef]
- Nakanishi, K.; Shiba, H. Domain-like incommensurate charge-density-wave states and the first-order incommensurate-commensurate transitions in layered tantalum dichalcogenides. ii. 2h-polytype. J. Phys. Soc. Jpn. 1978, 44, 1465–1473. [Google Scholar] [CrossRef]
- Bak, P. Commensurate phases, incommensurate phases and the devil’s staircase. Rep. Prog. Phys. 1982, 45, 587. [Google Scholar] [CrossRef]
- Wu, X.L.; Lieber, C.M. Hexagonal domain-like charge density wave phase of tas2 determined by scanning tunneling microscopy. Science 1989, 243, 1703–1705. [Google Scholar] [CrossRef]
- Scruby, C.; Williams, P.; Parry, G. The role of charge density waves in structural transformations of 1t tas2. Philos. Mag. 1975, 31, 255–274. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mertens, L.; van den Brink, J.; Wezel, J.v. Two-Dimensional Discommensurations: An Extension to McMillan’s Ginzburg–Landau Theory. Condens. Matter 2023, 8, 100. https://doi.org/10.3390/condmat8040100
Mertens L, van den Brink J, Wezel Jv. Two-Dimensional Discommensurations: An Extension to McMillan’s Ginzburg–Landau Theory. Condensed Matter. 2023; 8(4):100. https://doi.org/10.3390/condmat8040100
Chicago/Turabian StyleMertens, Lotte, Jeroen van den Brink, and Jasper van Wezel. 2023. "Two-Dimensional Discommensurations: An Extension to McMillan’s Ginzburg–Landau Theory" Condensed Matter 8, no. 4: 100. https://doi.org/10.3390/condmat8040100
APA StyleMertens, L., van den Brink, J., & Wezel, J. v. (2023). Two-Dimensional Discommensurations: An Extension to McMillan’s Ginzburg–Landau Theory. Condensed Matter, 8(4), 100. https://doi.org/10.3390/condmat8040100






