Thermodynamics in Stochastic Conway’s Game of Life
Abstract
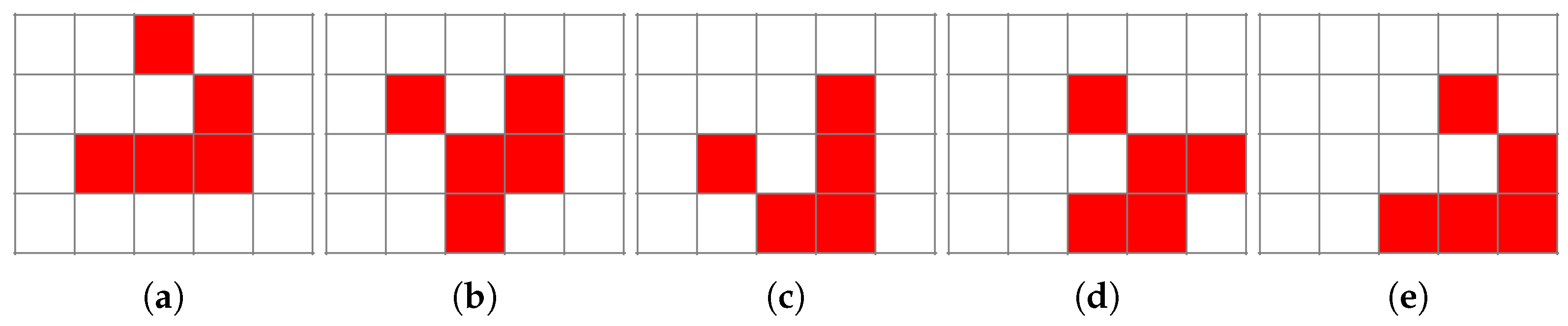
1. Introduction to Classical Conway’s Game of Life (CCGoL)
- 1.
- If a dead cell has exactly 3 neighbors, it comes alive in the next cycle.
- 2.
- If a living cell has 2 or 3 neighbors, it survives in the next cycle.
- 3.
- If a cell has a different number of neighbors than stated above, it will be dead in the next cycle.
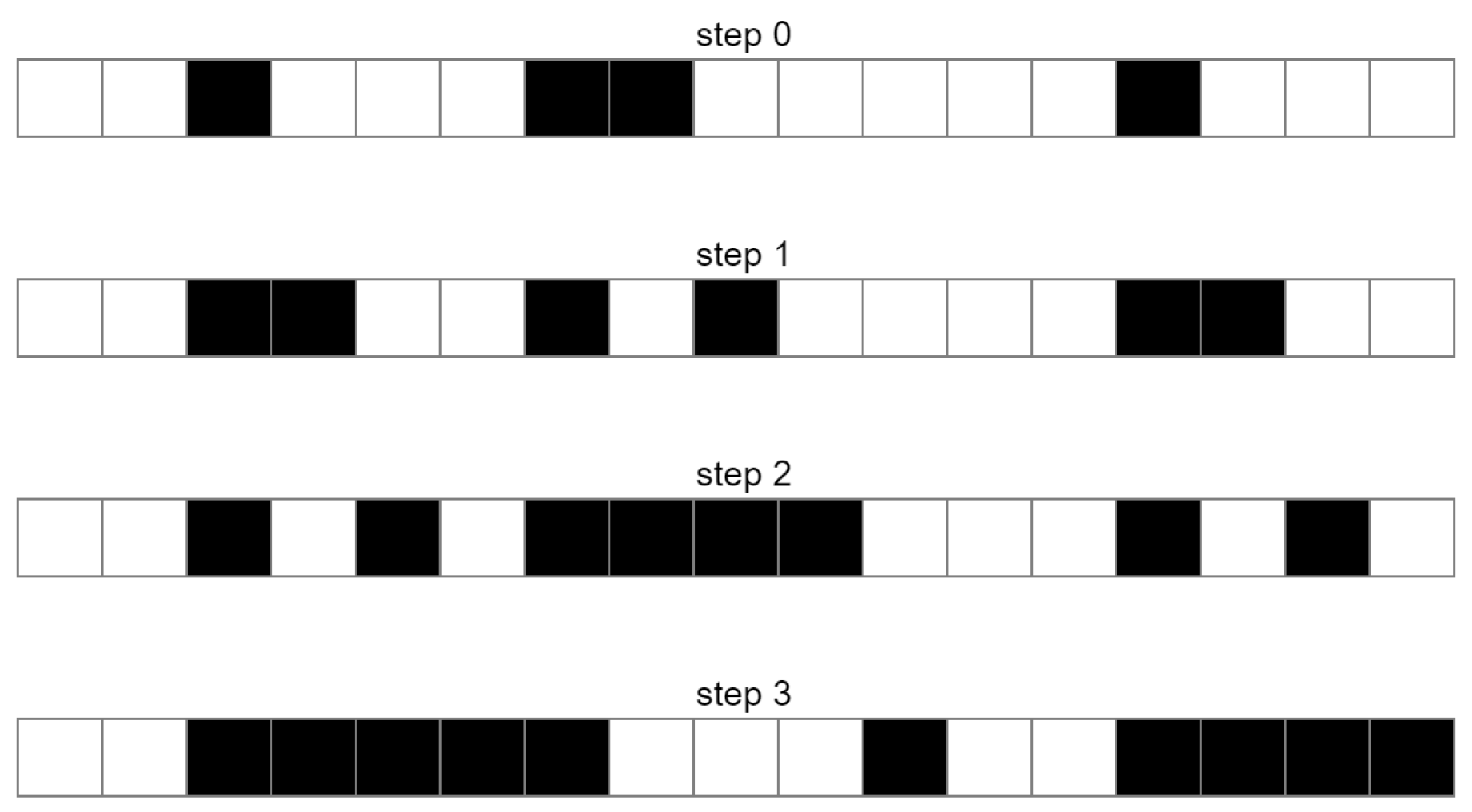
2. Introduction to Stochastic Classical Conway’s Game of Life (SCCGoL)
3. Generalization of Stochastic Classical Conway’s Game of Life (SCCGoL) to the Case of Competing Cellular Automaton Species
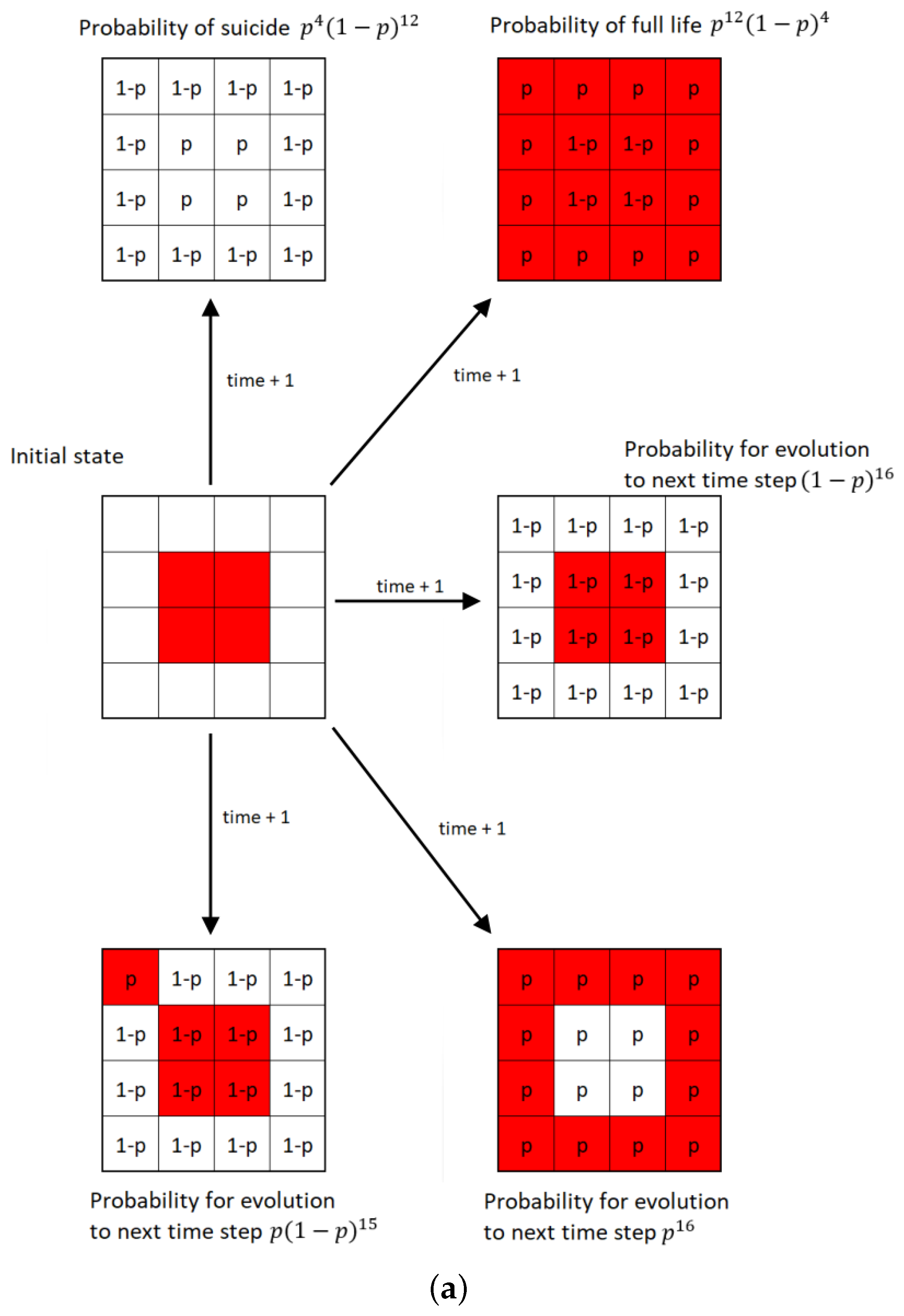
4. Methodology of Describing SCCGoL Dynamics Using Tools of Classical Statistical Physics
5. Numerical Analysis of SCCGoL Dynamics with Methodology of Classical Statistical Physics
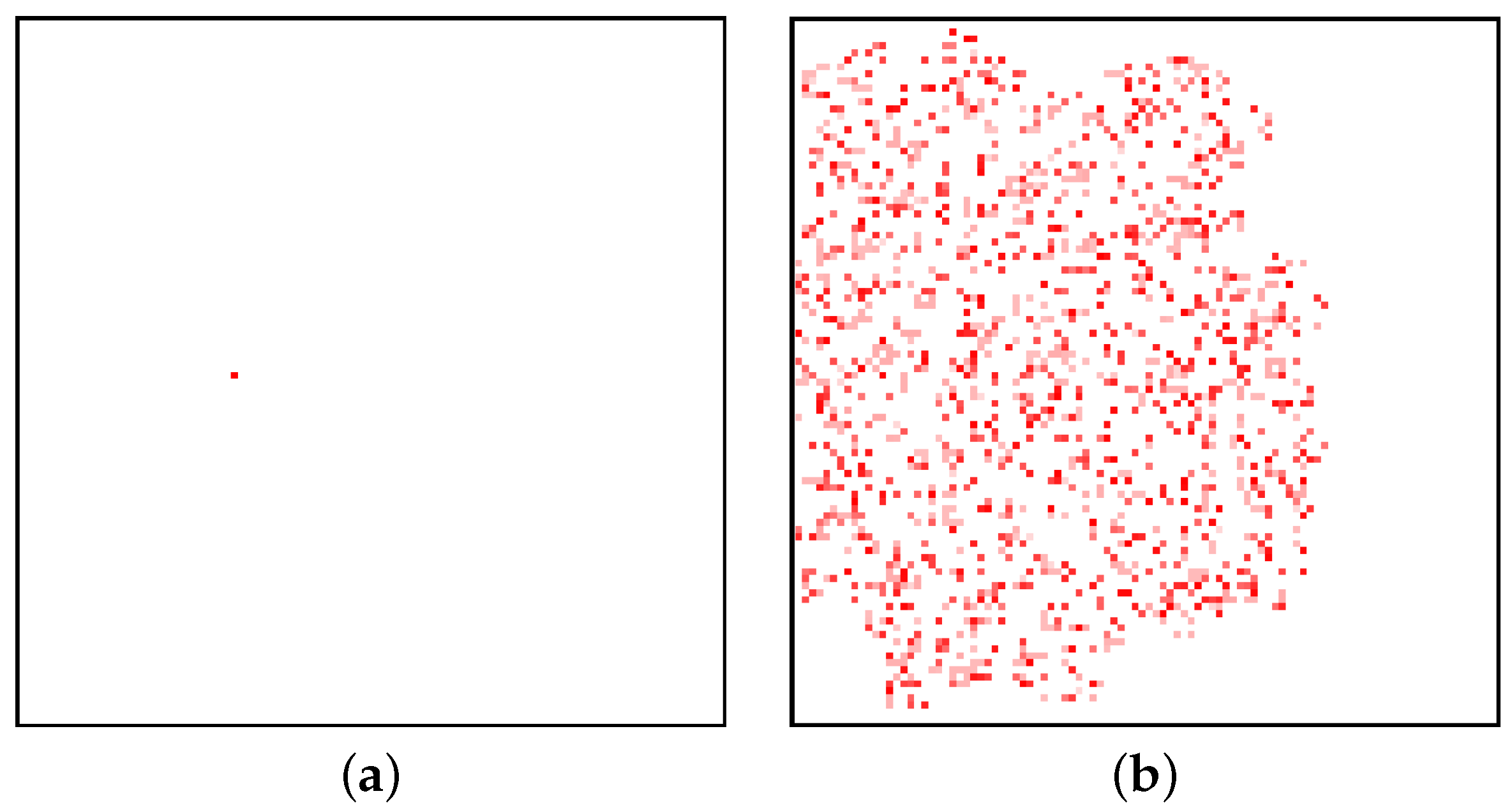
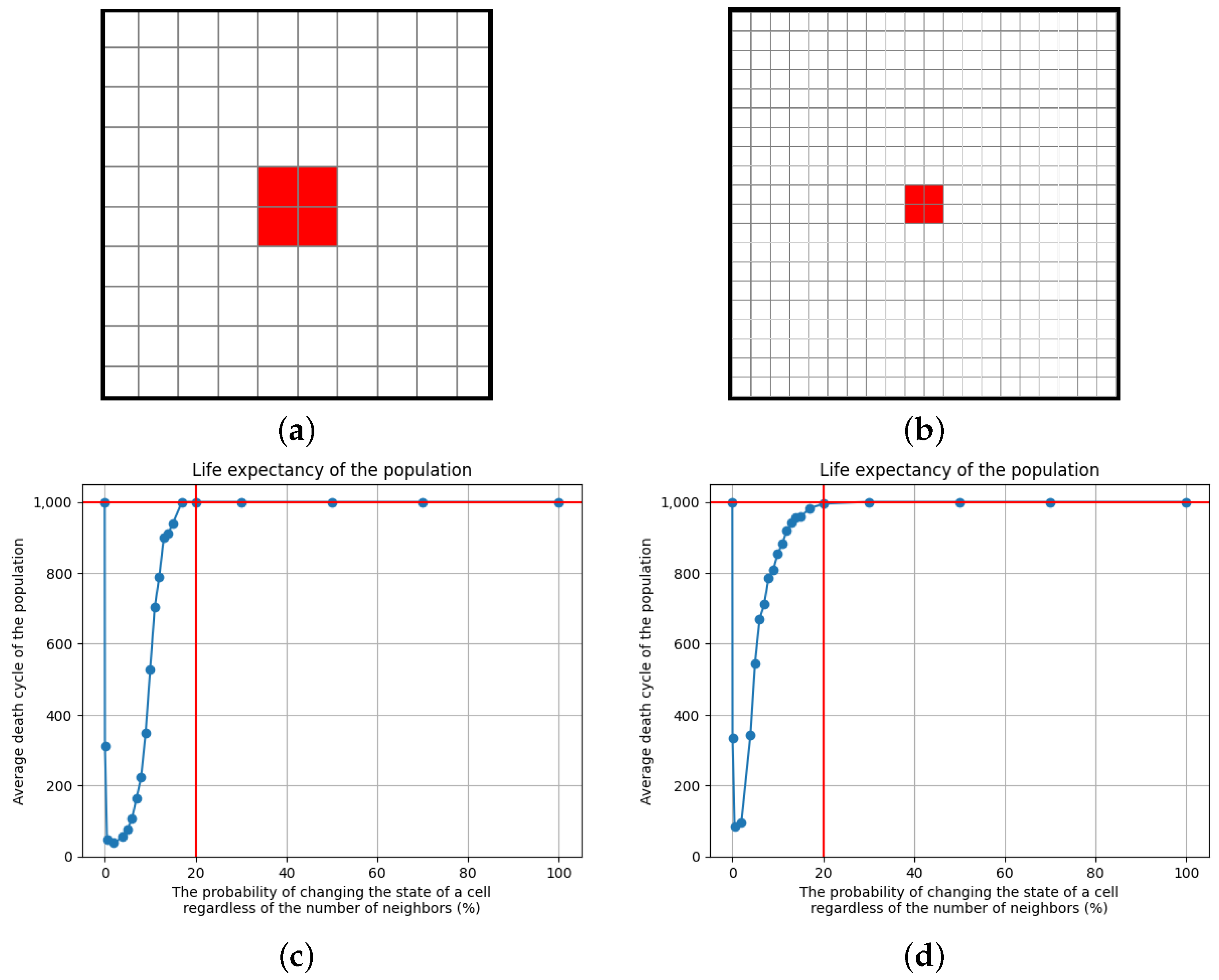
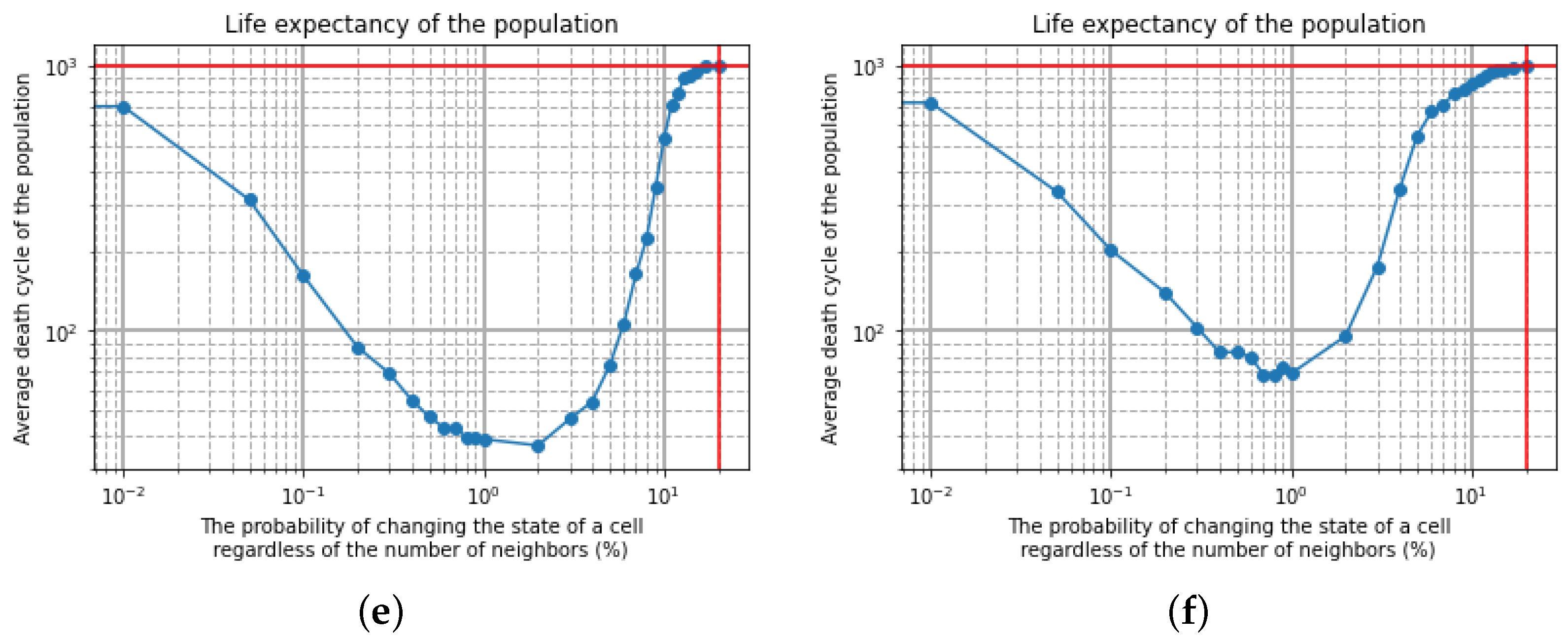
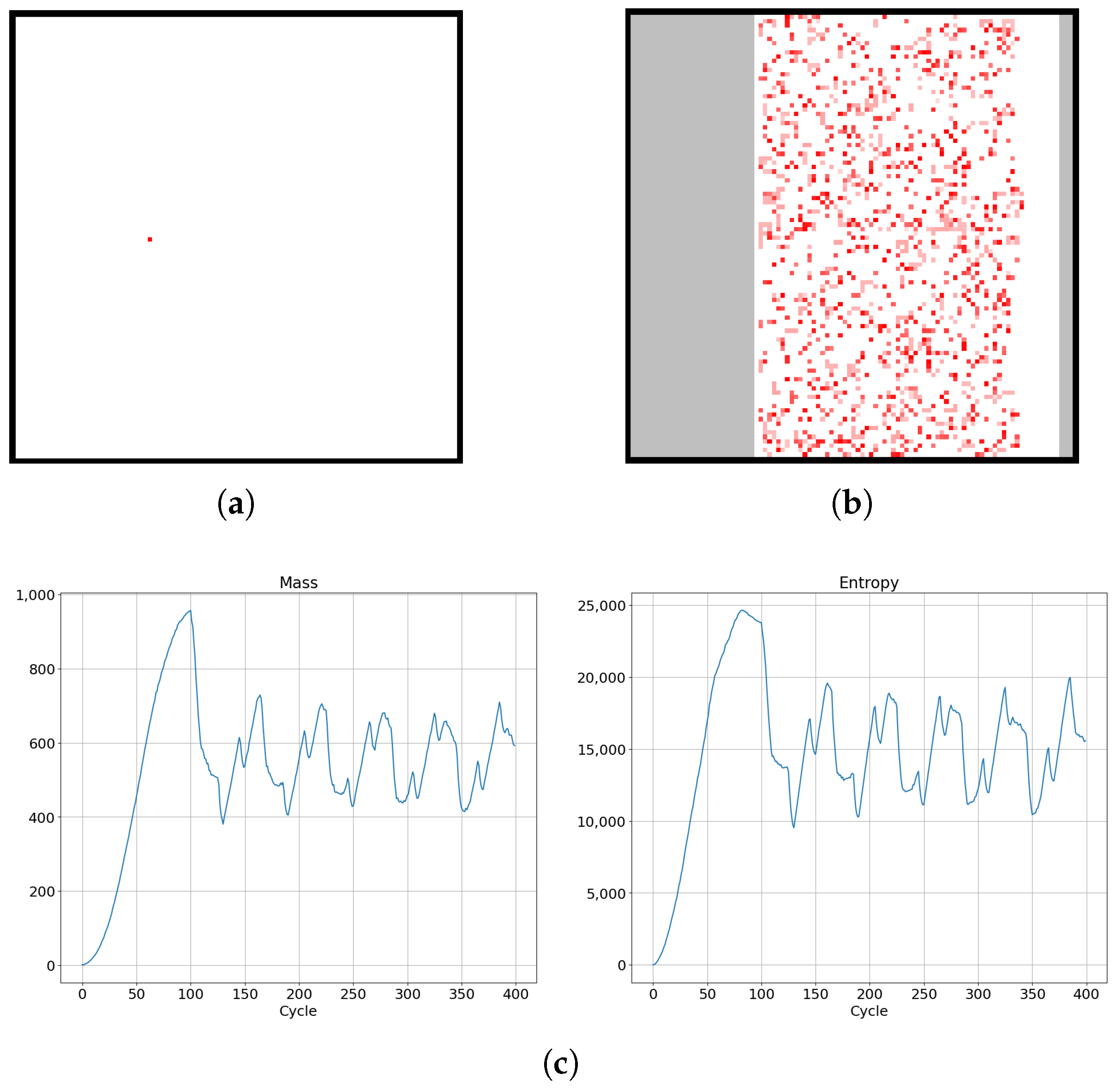
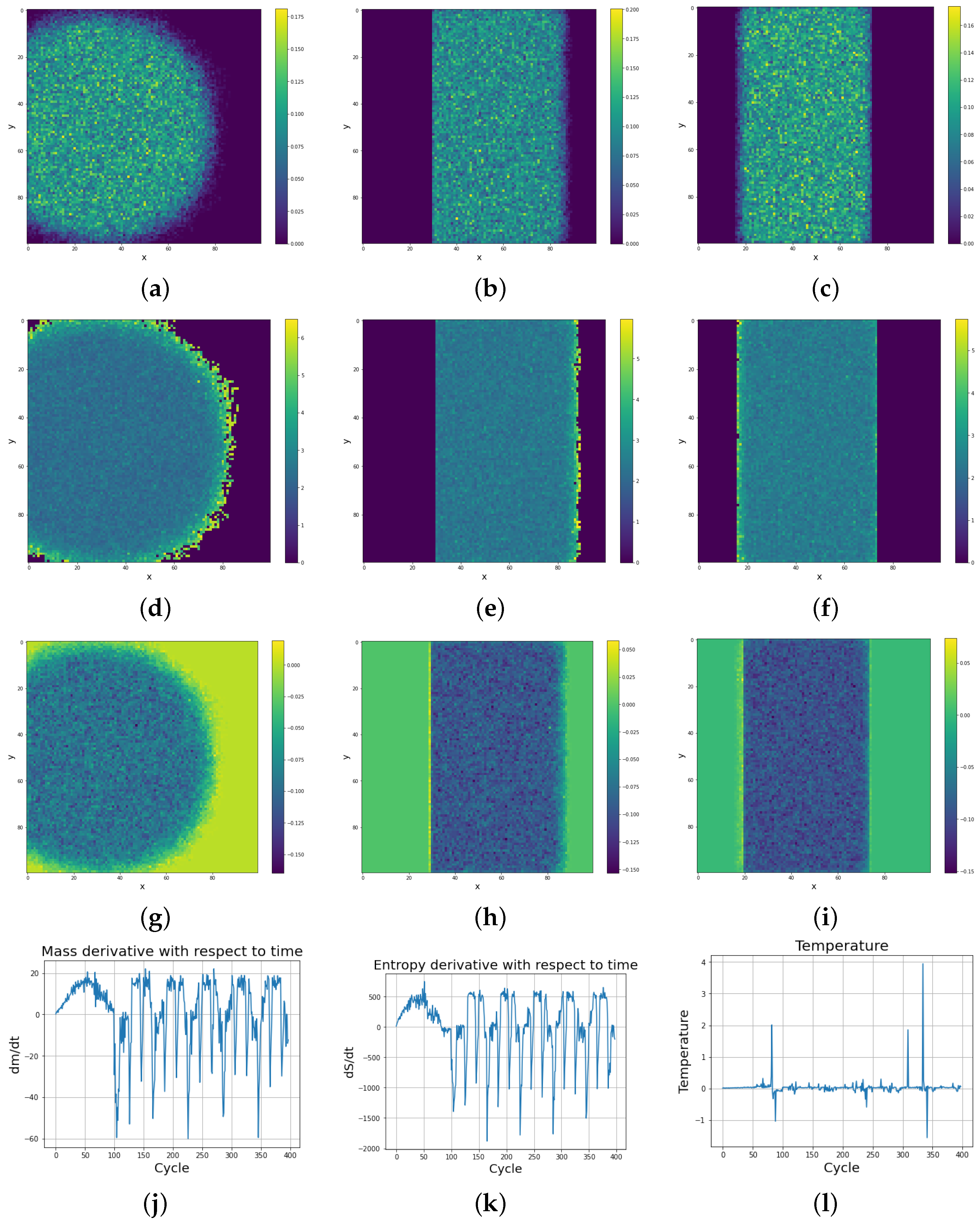
6. Numerical Study of One-Species Cellular Automata with Various Boundary Conditions
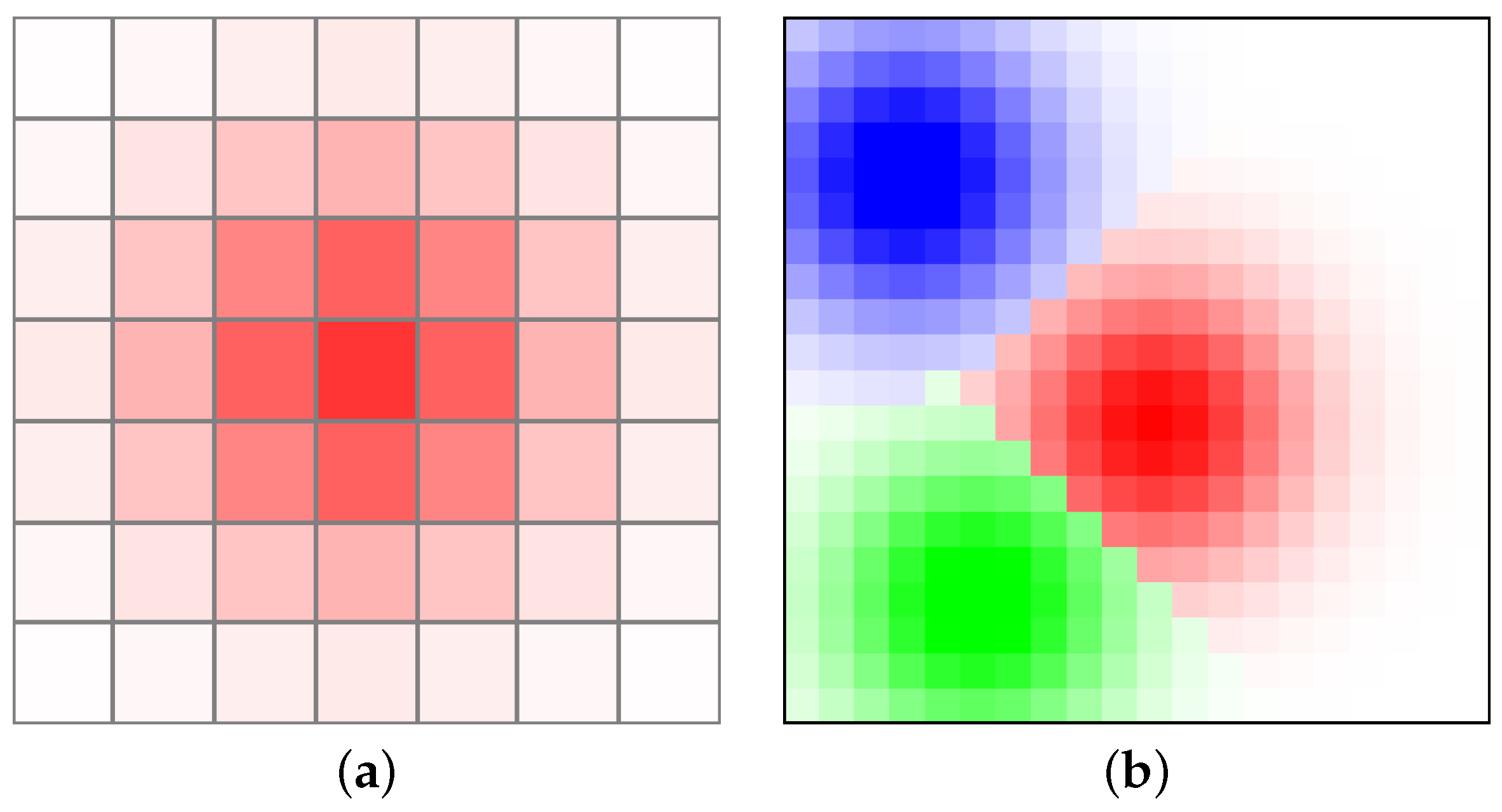
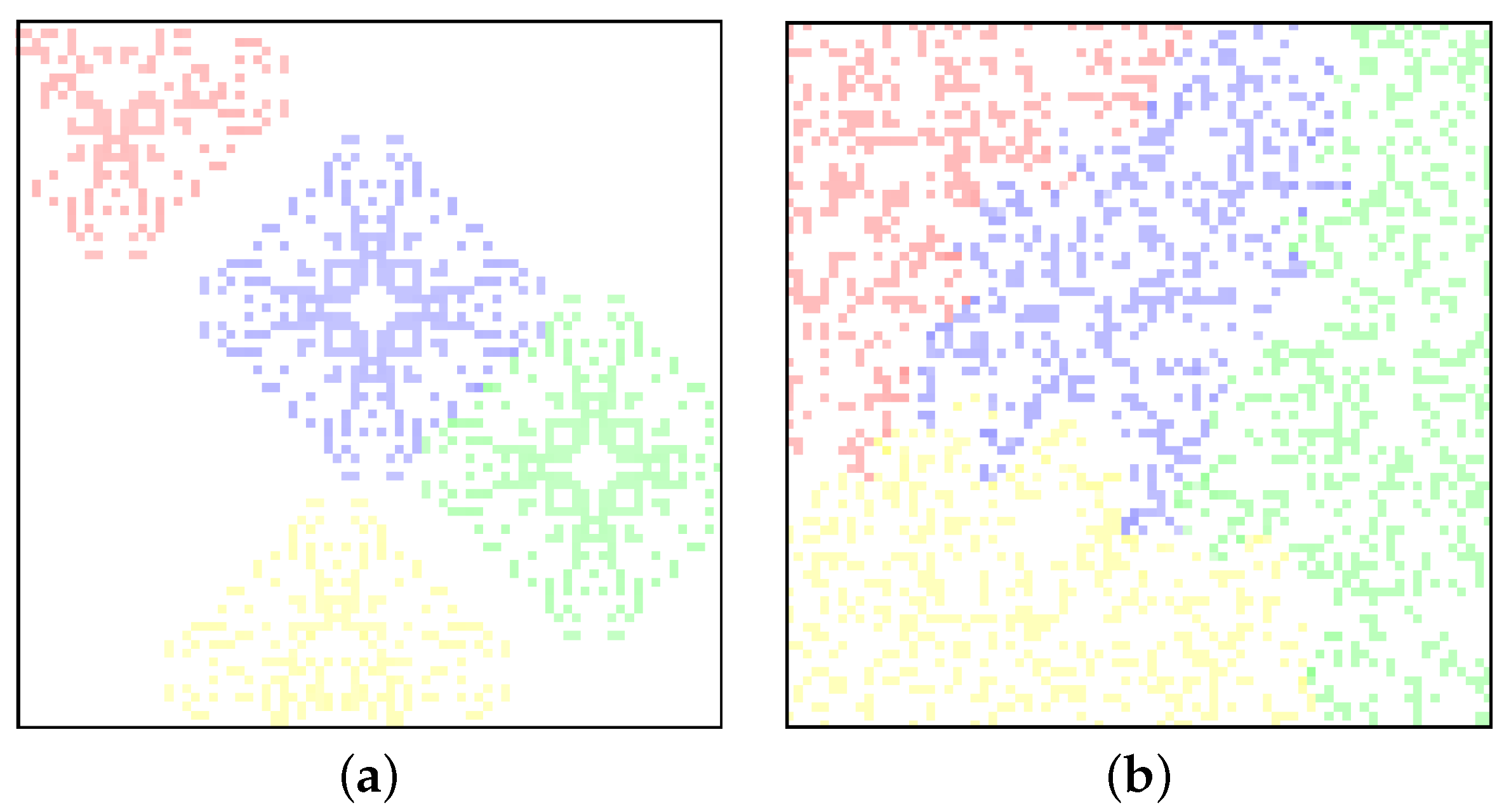
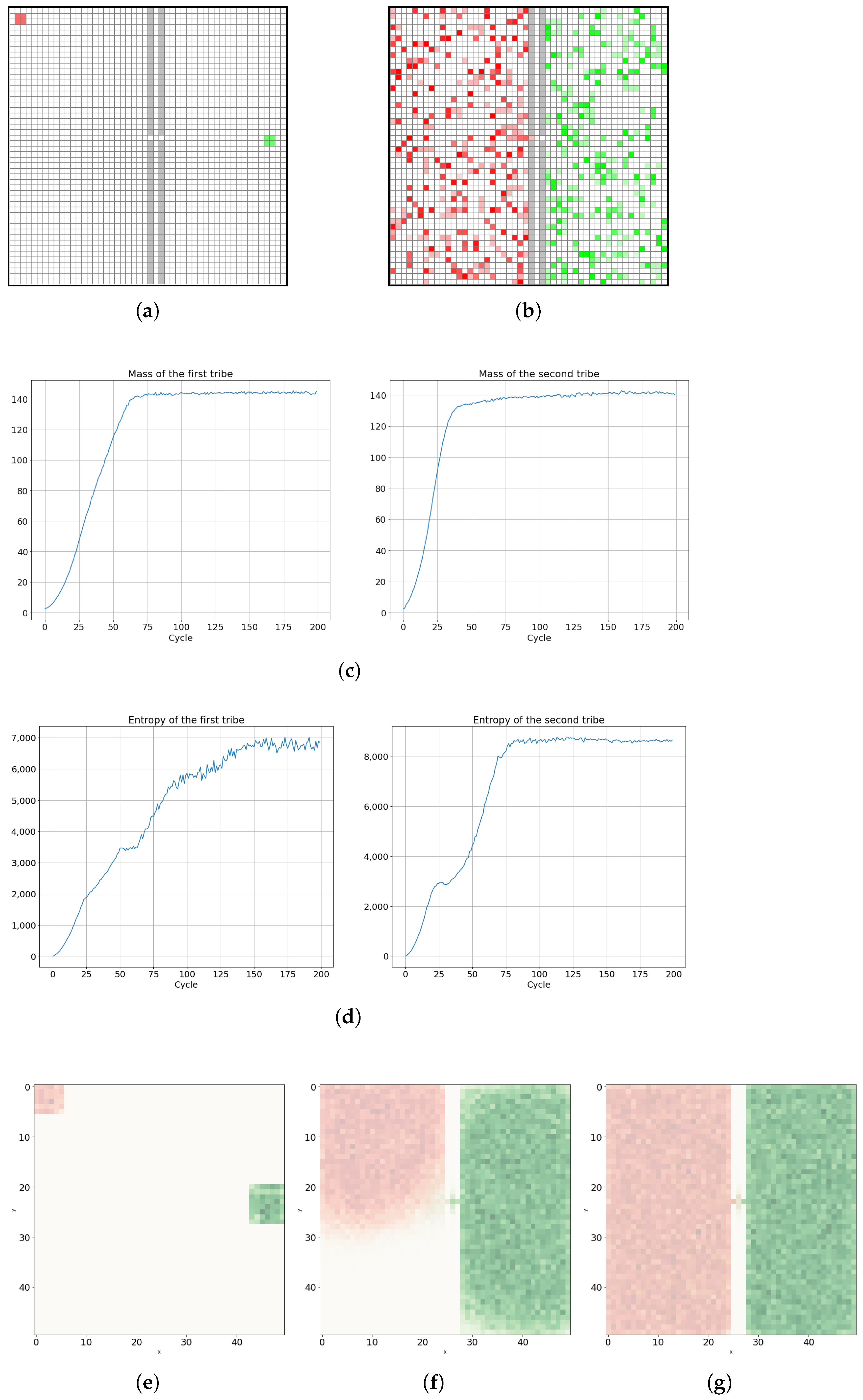
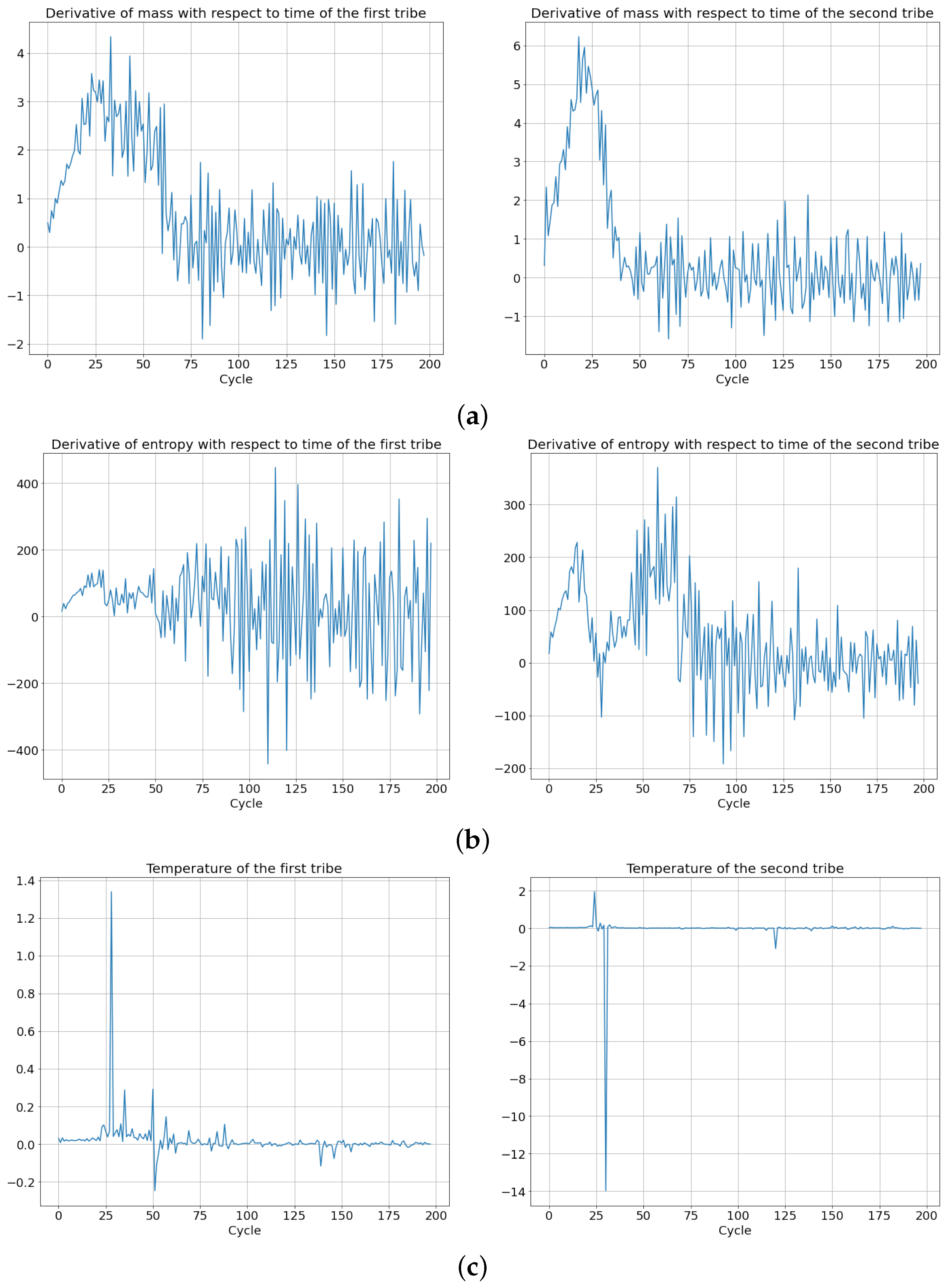
7. Numerical Study of Two-Species Cellular Automata in Perturbative Interaction by Narrow Constriction
8. Behavior of One-Species Cellular Automata with Cycle Movement of Barriers Mimicking Thermodynamical Cycle
9. Conclusions and Future Perspectives
- 1.
- 2.
- 3.
- 4.
- 5.
- 6.
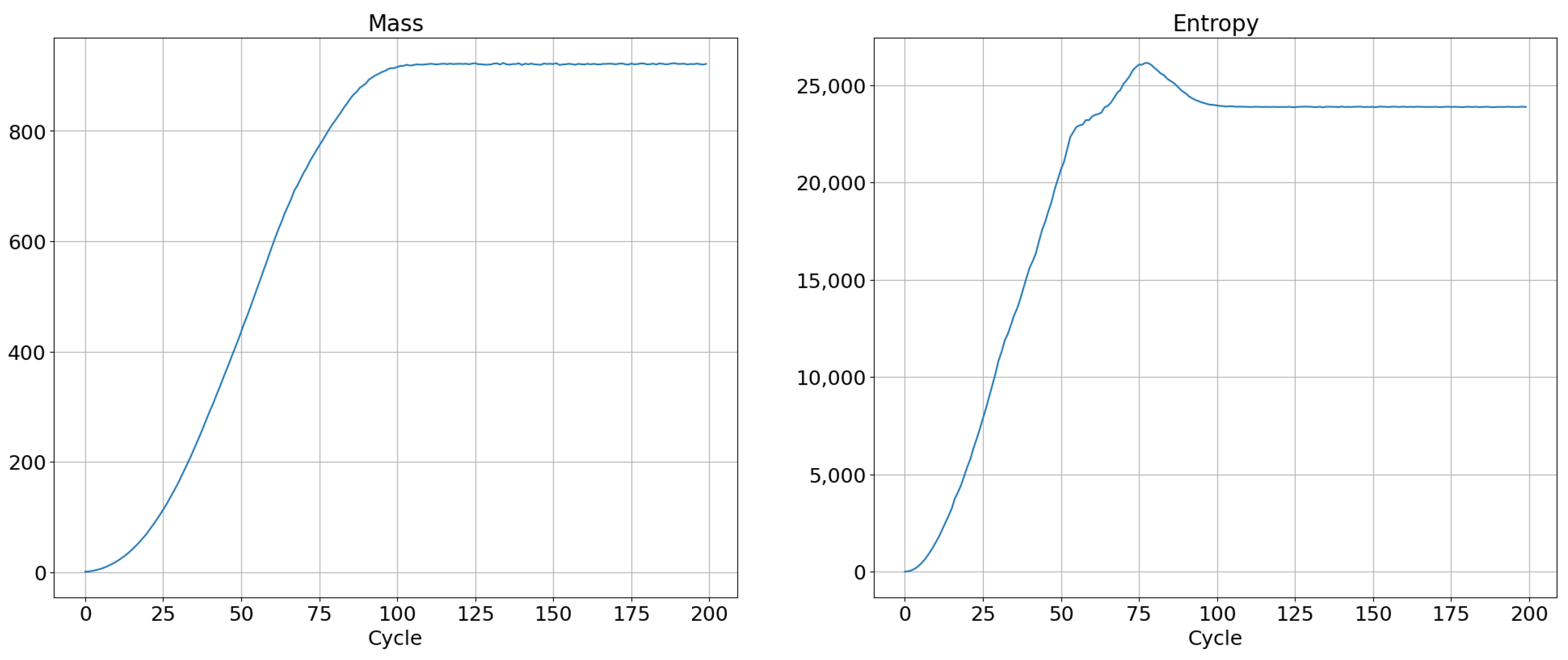
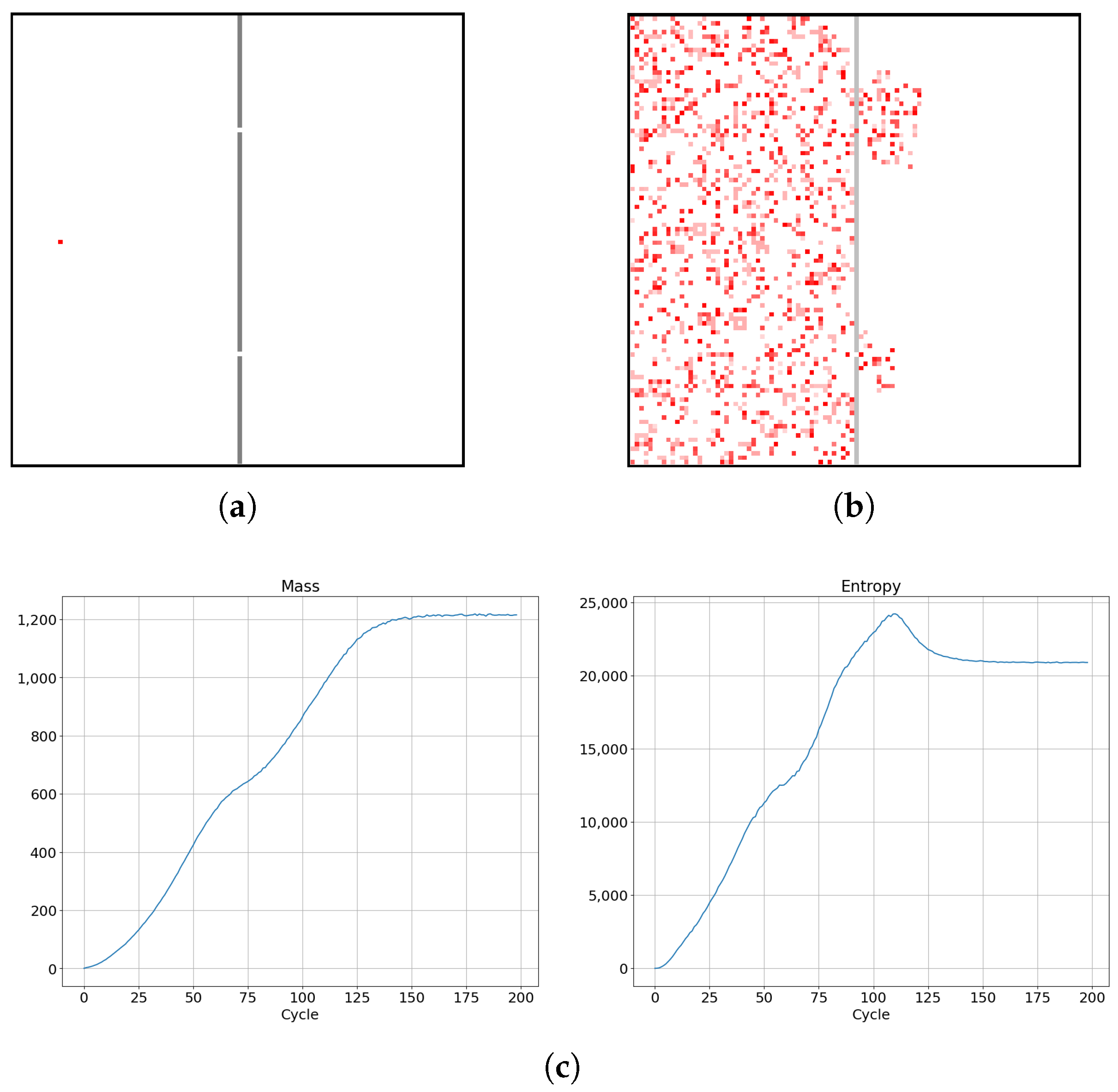
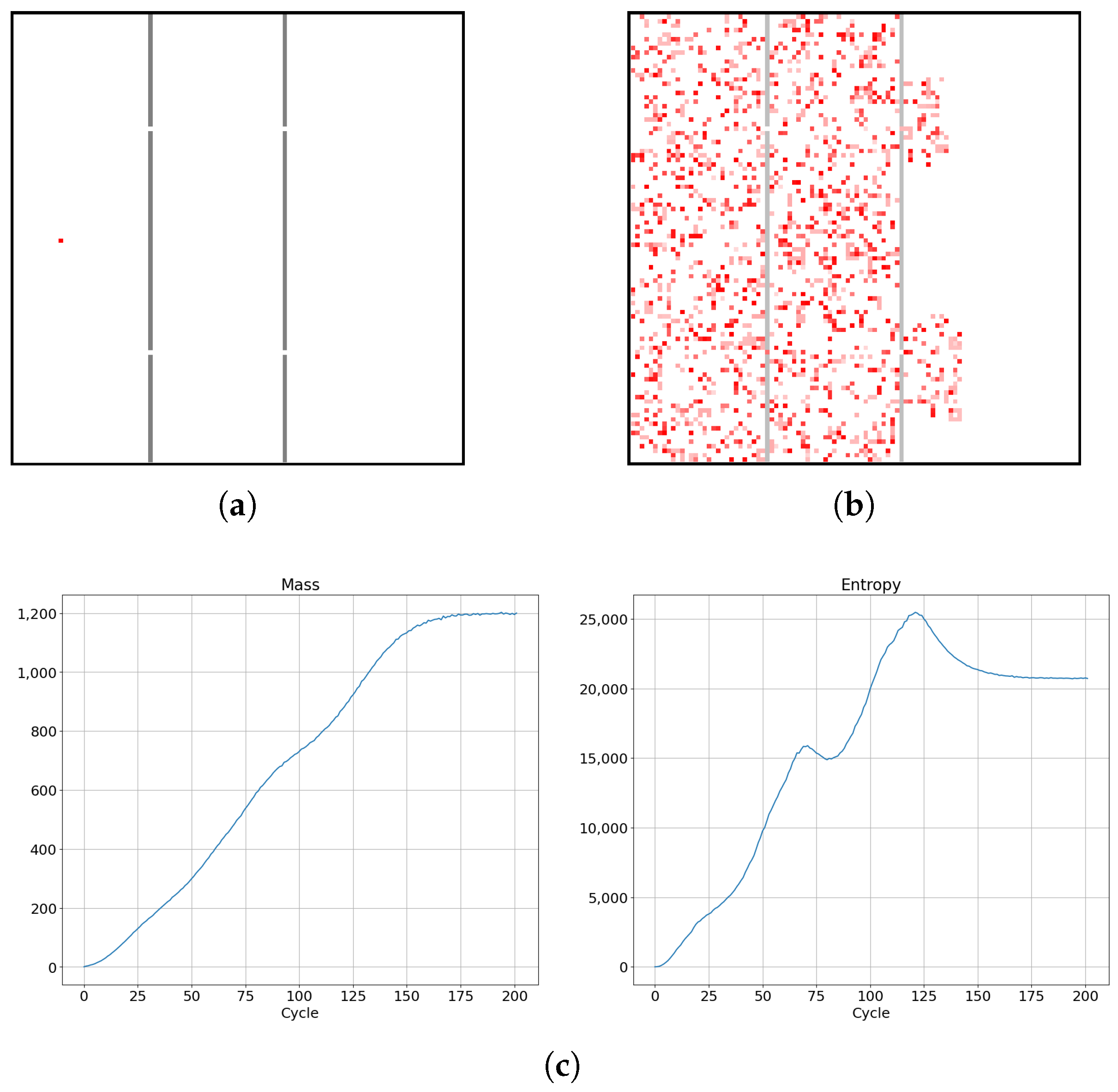
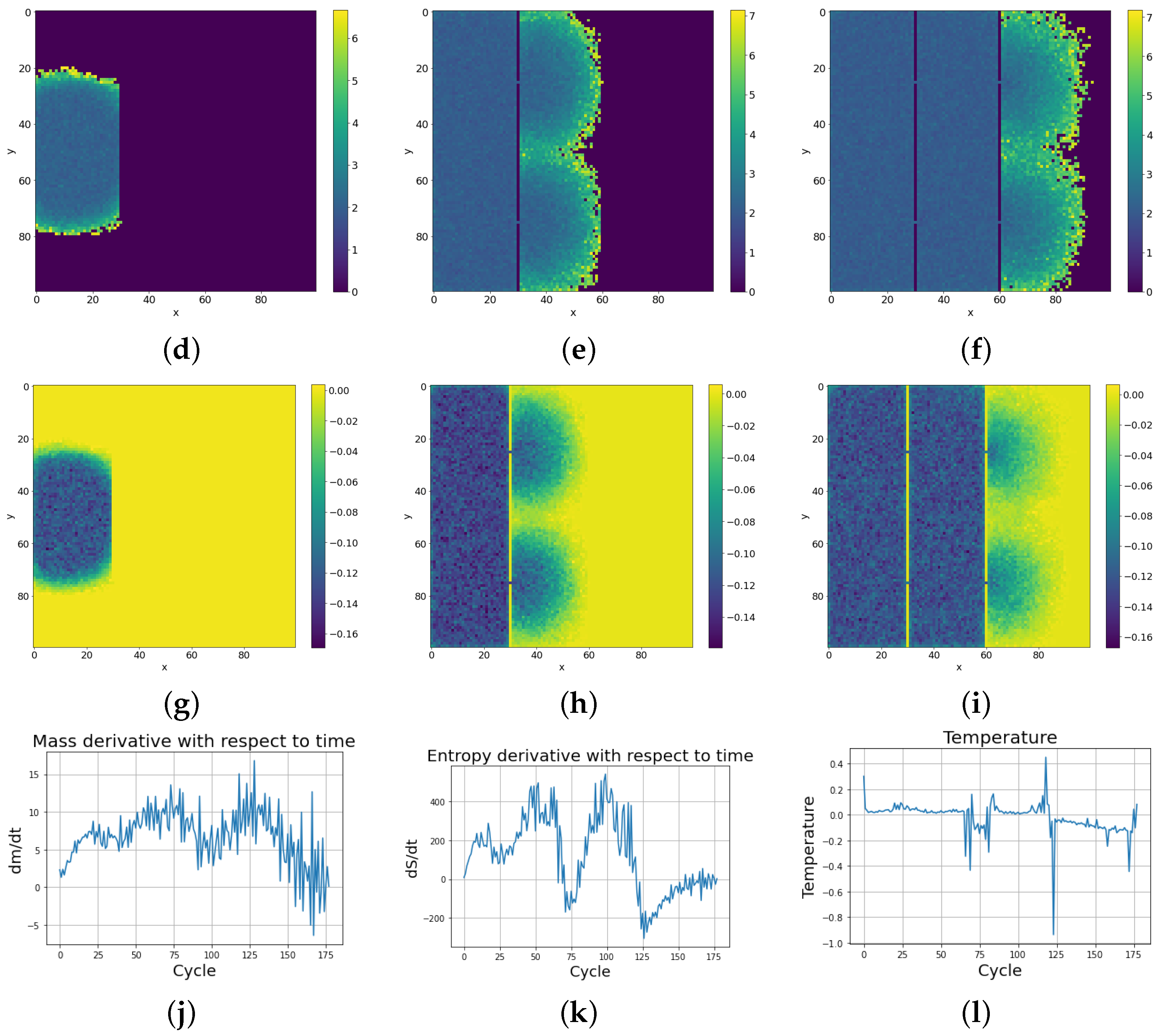
- Identification of a brief Shannon entropy peak that later minimizes and saturates in SGoL (Figure 9 and Figure 12). Monotonicity in increase of entropy is twice briefly interrupted by a small decline, which is associated with automaton cells “colliding” with the barriers and experiencing a brief propagation slowdown (Figure 14).
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Berto, F.; Tagliabue, J. Cellular Automata. In The Stanford Encyclopedia of Philosophy, Spring 2022 ed.; Zalta, E.N., Ed.; Metaphysics Research Lab, Stanford University: Stanford, CA, USA, 2022. [Google Scholar]
- Wolfram, S. Statistical mechanics of cellular automata. Rev. Mod. Phys. 1983, 55, 601. [Google Scholar] [CrossRef]
- Gardner, M. Mathematical Games—The fantastic combinations of John Conway’s new solitaire game “life”. Sci. Am. 1970, 223, 120–123. [Google Scholar] [CrossRef]
- Bandyopadhyay, P.S.; Grunska, N.; Dcruz, D.; Greenwood, M.C. Are Scientific Models of Life Testable? A Lesson from Simpson’s Paradox. Sci 2021, 3, 2. [Google Scholar] [CrossRef]
- Peitgen, H.O.; Jürgens, H.; Saupe, D. Chaos and Fractals; Springer: Cham, Switzerland, 1983. [Google Scholar]
- Aguilera-Venegas, G.; Galán-García, J.L.; Egea-Guerrero, R.; Galán-García, M.Á.; Rodríguez-Cielos, P.; Padilla-Domínguez, Y.; Galán-Luque, M. A probabilistic extension to Conway’s Game of Life. Adv. Comput. Math. 2019, 45, 2111–2121. [Google Scholar] [CrossRef]
- Vandevelde, S.; Vennekens, J. ProbLife: A Probabilistic Game of Life. arXiv 2022, arXiv:2201.09521. [Google Scholar]
- Shannon, C. A Mathematical Theory of Communication. Bell Syst. Tech. J. 1948, 27, 379–423. [Google Scholar] [CrossRef]
- Shannon, C. A Mathematical Theory of Communication. Bell Syst. Tech. J. 1948, 27, 623–656. [Google Scholar] [CrossRef]
- Velazquez Abad, L. Principles of classical statistical mechanics: A perspective from the notion of complementarity. Ann. Phys. 2012, 327, 1682–1693. [Google Scholar] [CrossRef]
- Mishin, Y. Thermodynamic Theory of Equilibrium Fluctuations; Elsevier: Amsterdam, The Netherlands, 2015. [Google Scholar]
- Feynman, R.P. Statistical Mechanics; Westview Press: Boulder, CO, USA, 1972. [Google Scholar]
- Huang, K. Introduction to Statistical Physics; CRC Press: Boca Raton, FL, USA, 2001. [Google Scholar]
- Huang, K. Statistical Mechanics; John Wiley & Sons: New York, NY, USA, 1963. [Google Scholar]
- Baez, J.C.; Pollard, B.S. Quantropy. arXiv 2013, arXiv:1311.0813. [Google Scholar] [CrossRef]
- Abe, S.; Okuyama, S. Similarity between quantum mechanics and thermodynamics: Entropy, temperature, and Carnot cycle. Phys. Rev. E Stat. Nonlin. Soft Matter Phys. 2011, 83, 021121. [Google Scholar] [CrossRef] [PubMed]
- Pomorski, K. Equivalence between Classical Epidemic Model and Quantum Tight-Binding Model; Springer: Cham, Switzerland, 2022. [Google Scholar]
- Pomorski, K. Equivalence between finite state stochastic machine, non-dissipative and dissipative tight-binding and Schroedinger model. Math. Comput. Simul. 2023, 209, 362–407. [Google Scholar] [CrossRef]
- Kotula, D.; Pomorski, K. Thermodynamics of Stochastic Conway Game of Life; ShanghaiAI Lectures. 2022. Available online: https://youtu.be/kLOB9VlF-R4 (accessed on 1 January 2023).
- Flitney, A.P.; Abbott, D. A Semi-quantum Version of the Game of Life. In Advances in Dynamic Games: Applications to Economics, Finance, Optimization, and Stochastic Control; Nowak, A.S., Szajowski, K., Eds.; Birkhäuser Boston: Boston, MA, USA, 2005; pp. 667–679. [Google Scholar]






Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pomorski, K.; Kotula, D. Thermodynamics in Stochastic Conway’s Game of Life. Condens. Matter 2023, 8, 47. https://doi.org/10.3390/condmat8020047
Pomorski K, Kotula D. Thermodynamics in Stochastic Conway’s Game of Life. Condensed Matter. 2023; 8(2):47. https://doi.org/10.3390/condmat8020047
Chicago/Turabian StylePomorski, Krzysztof, and Dariusz Kotula. 2023. "Thermodynamics in Stochastic Conway’s Game of Life" Condensed Matter 8, no. 2: 47. https://doi.org/10.3390/condmat8020047
APA StylePomorski, K., & Kotula, D. (2023). Thermodynamics in Stochastic Conway’s Game of Life. Condensed Matter, 8(2), 47. https://doi.org/10.3390/condmat8020047










