Study of Interacting Heisenberg Antiferromagnet Spin-1/2 and 1 Chains
Abstract
:1. Introduction
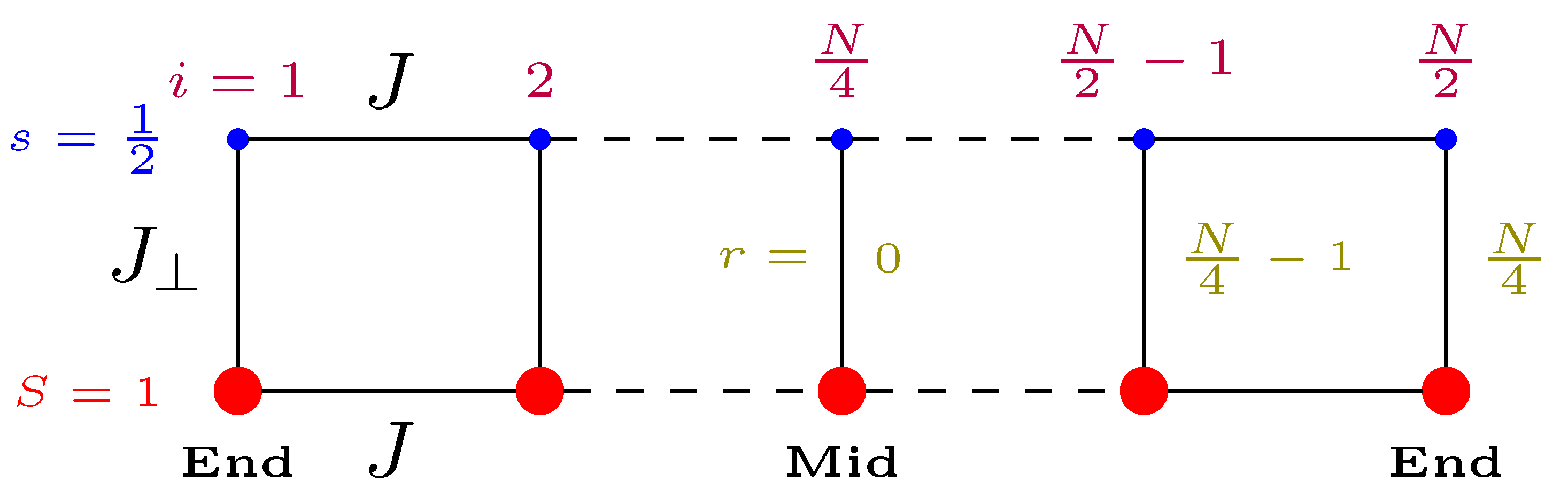
2. Model Hamiltonian and Numerical Method
3. Results
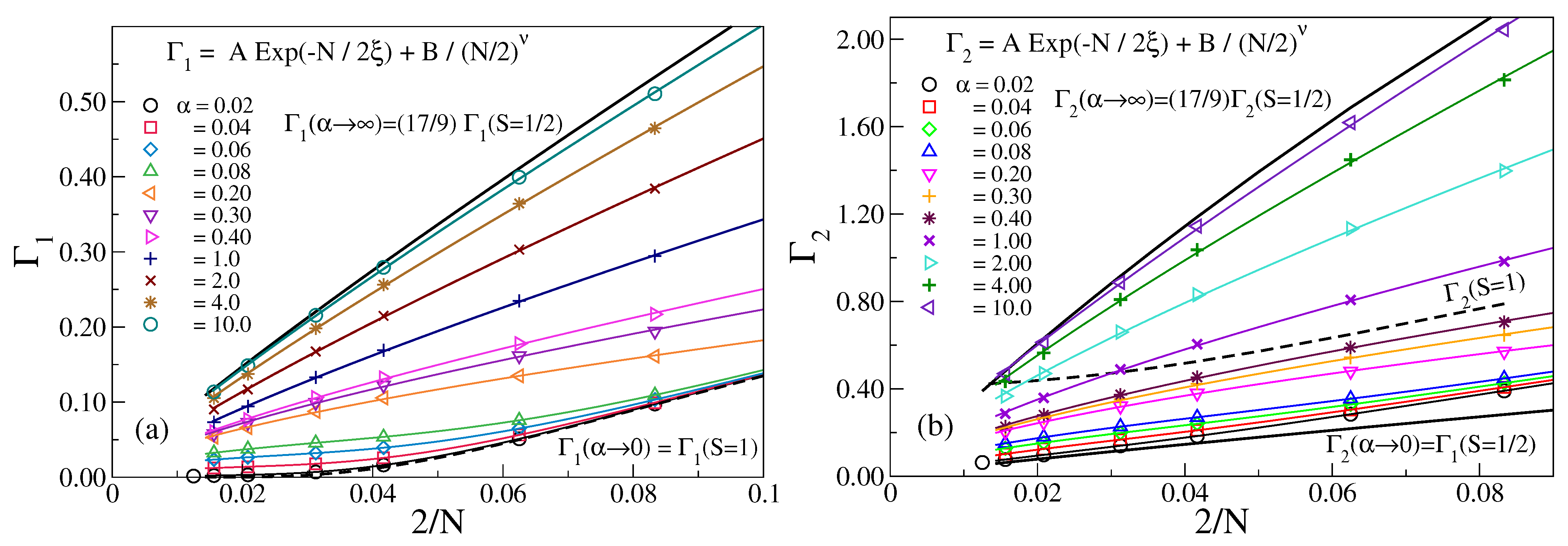
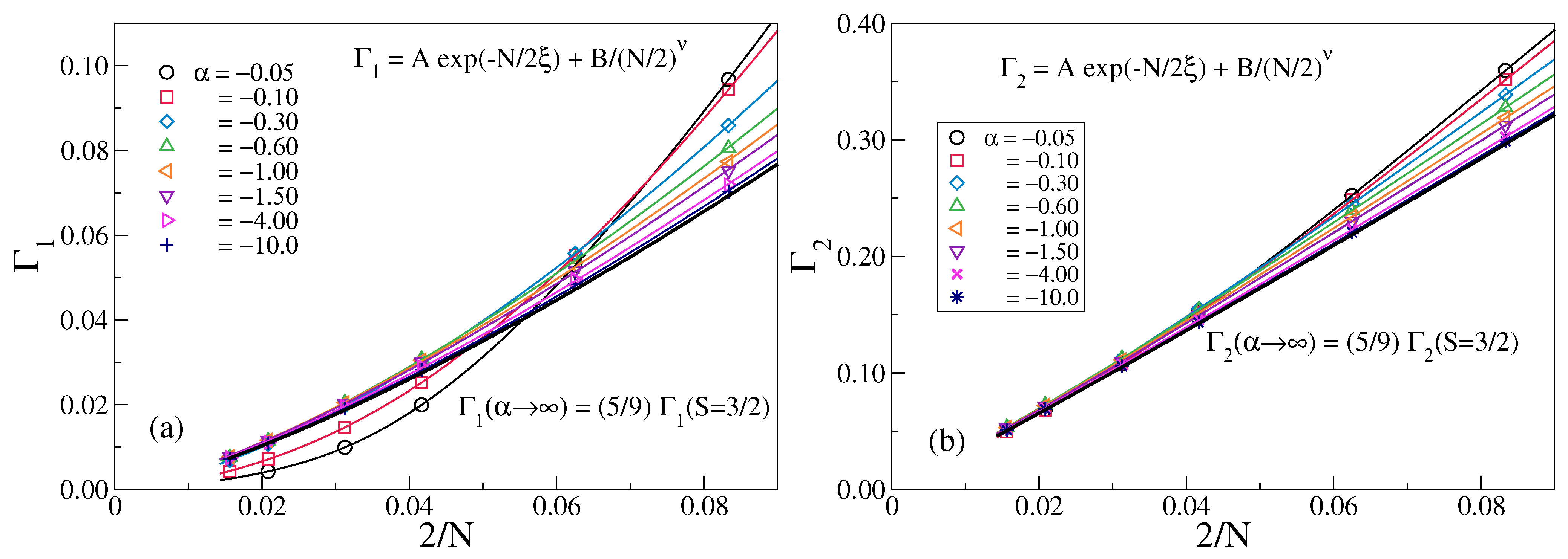
3.1. Energy Gaps
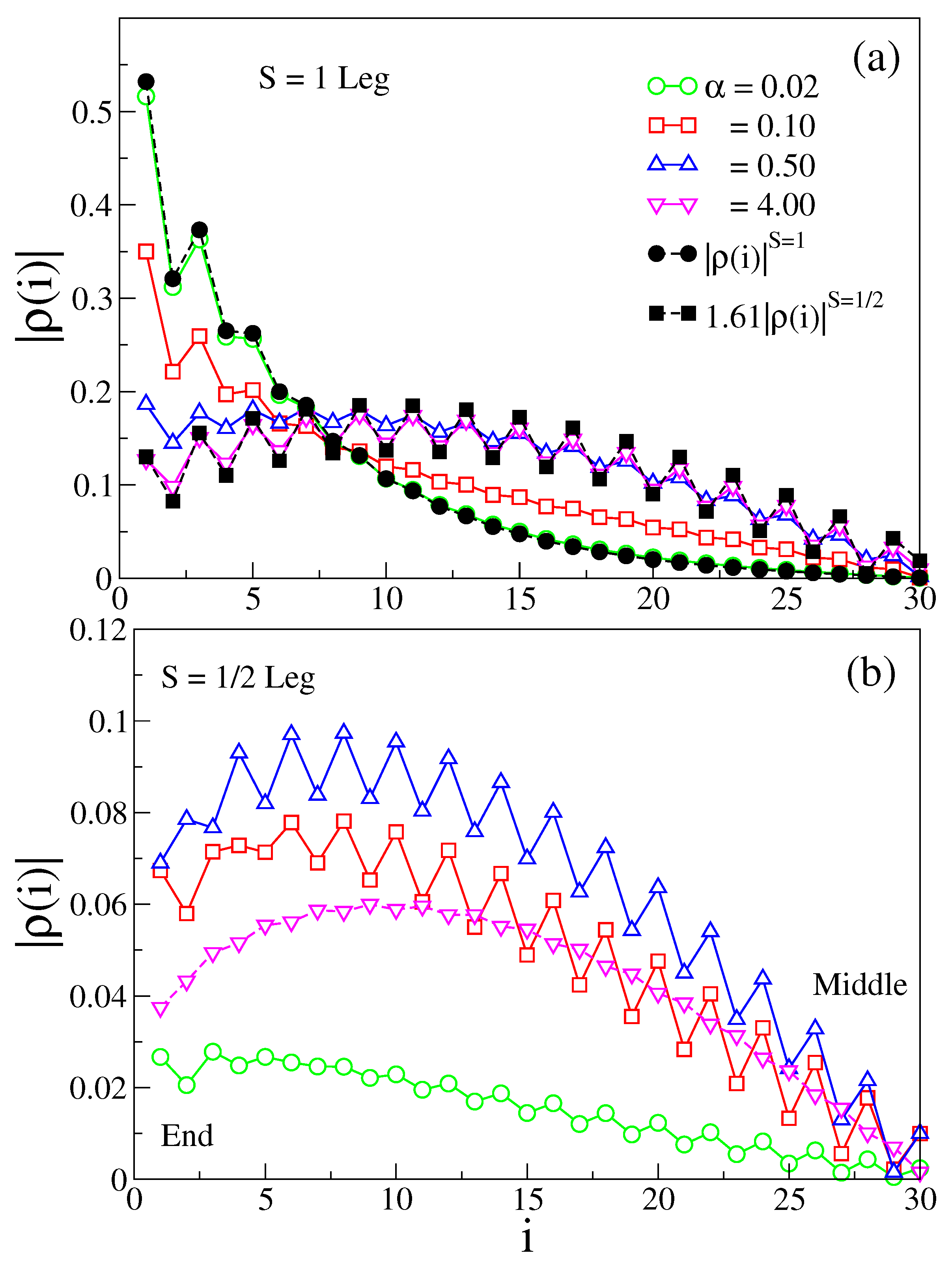
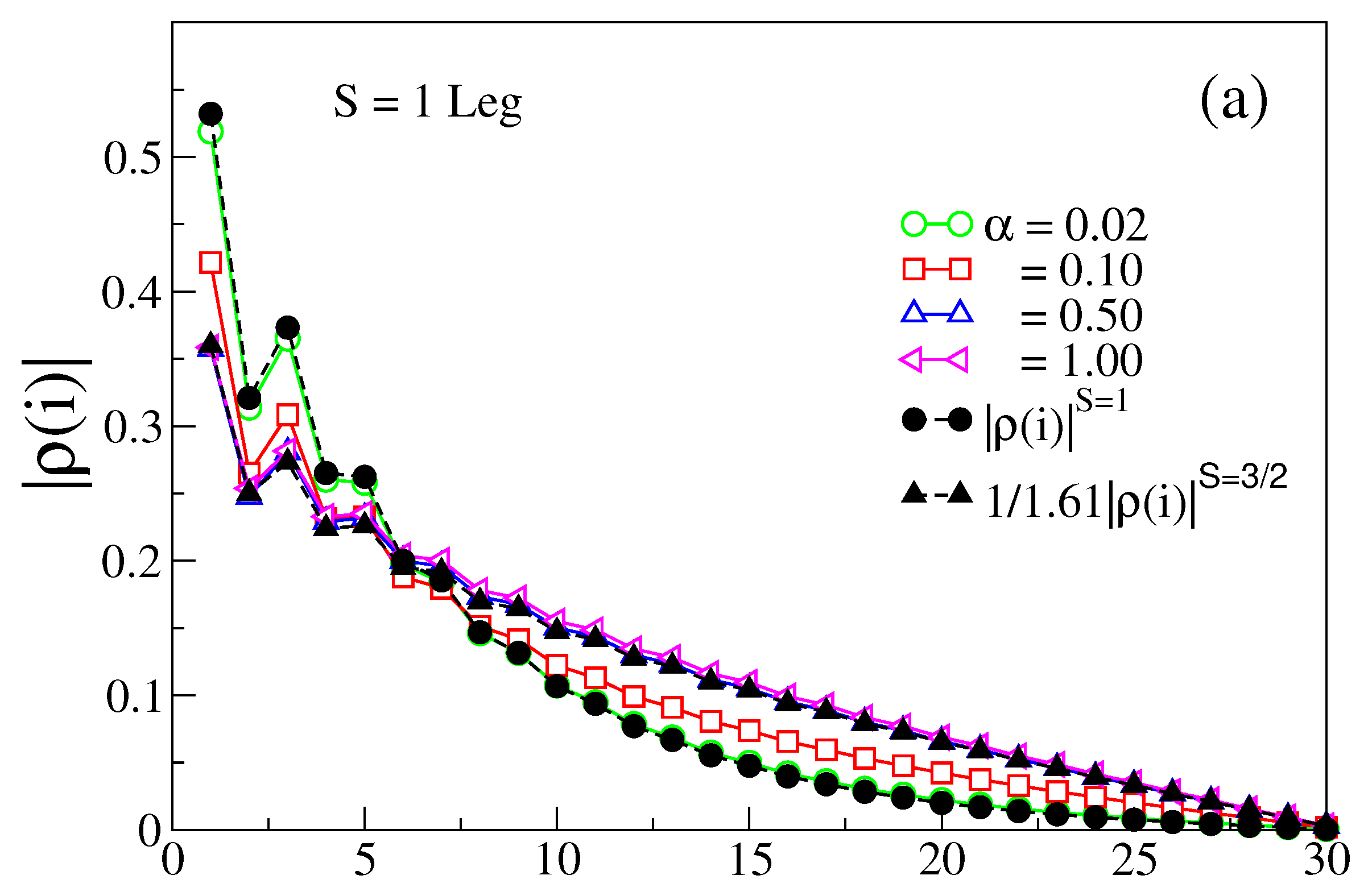
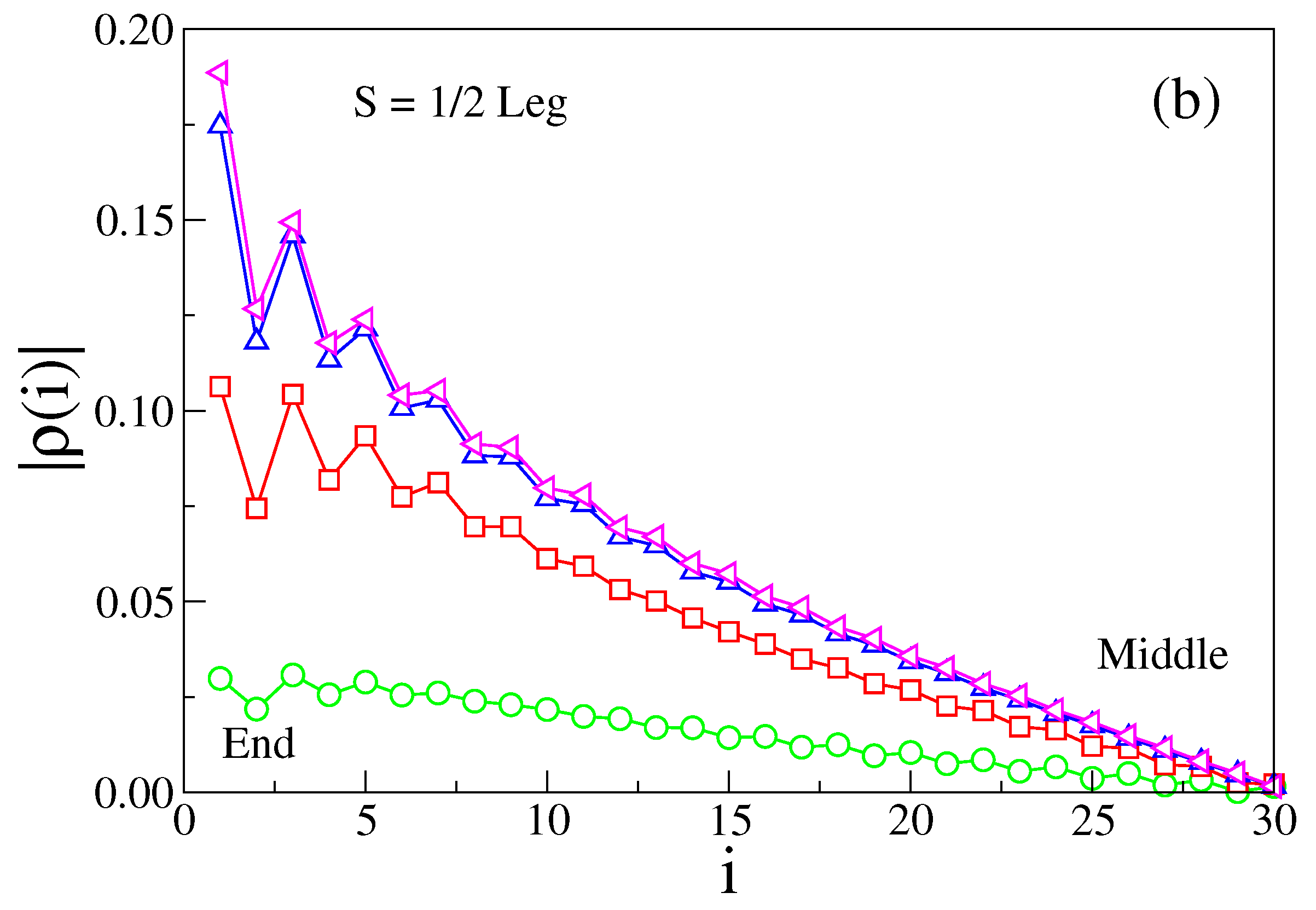
3.2. Spin Densities
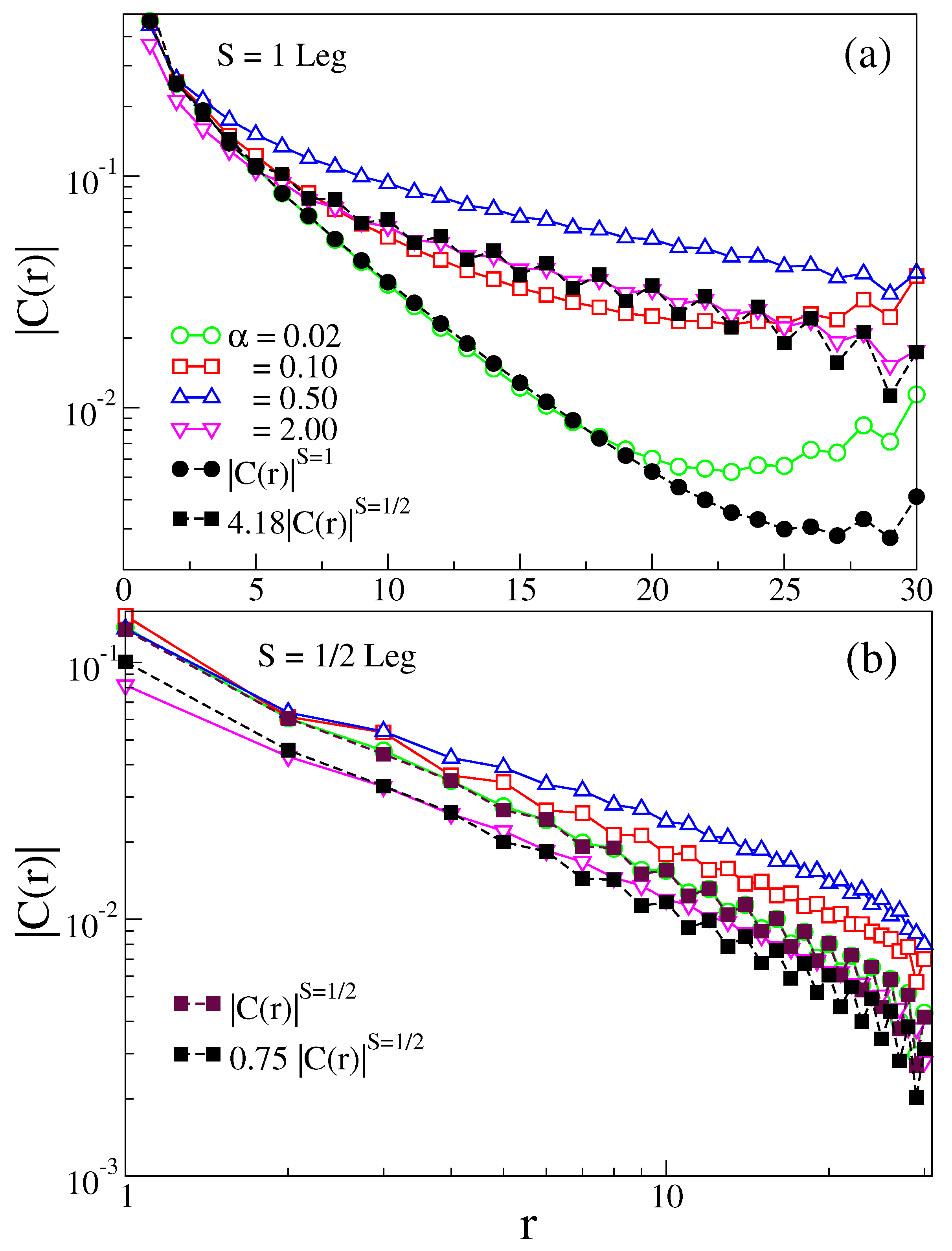
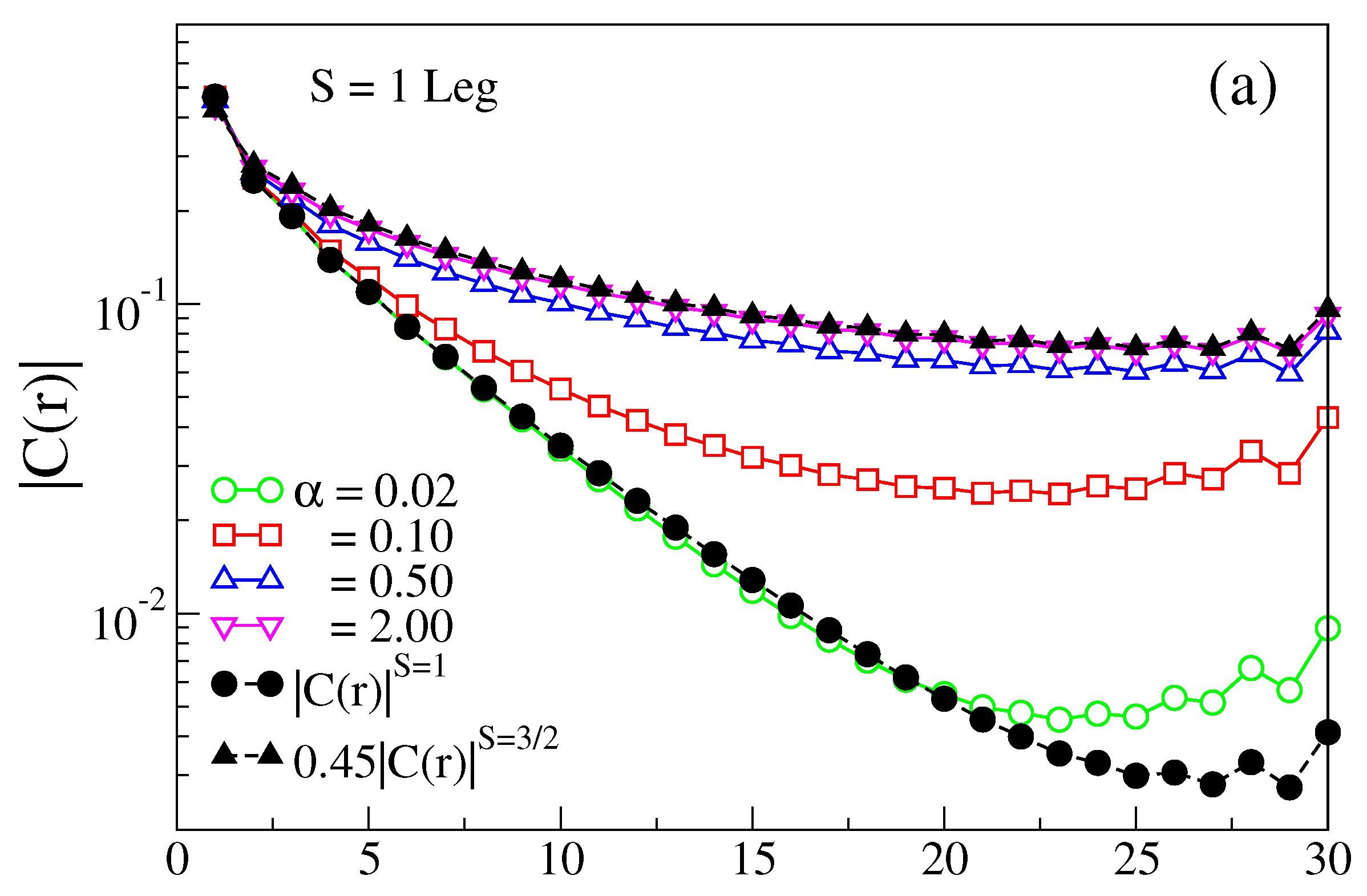
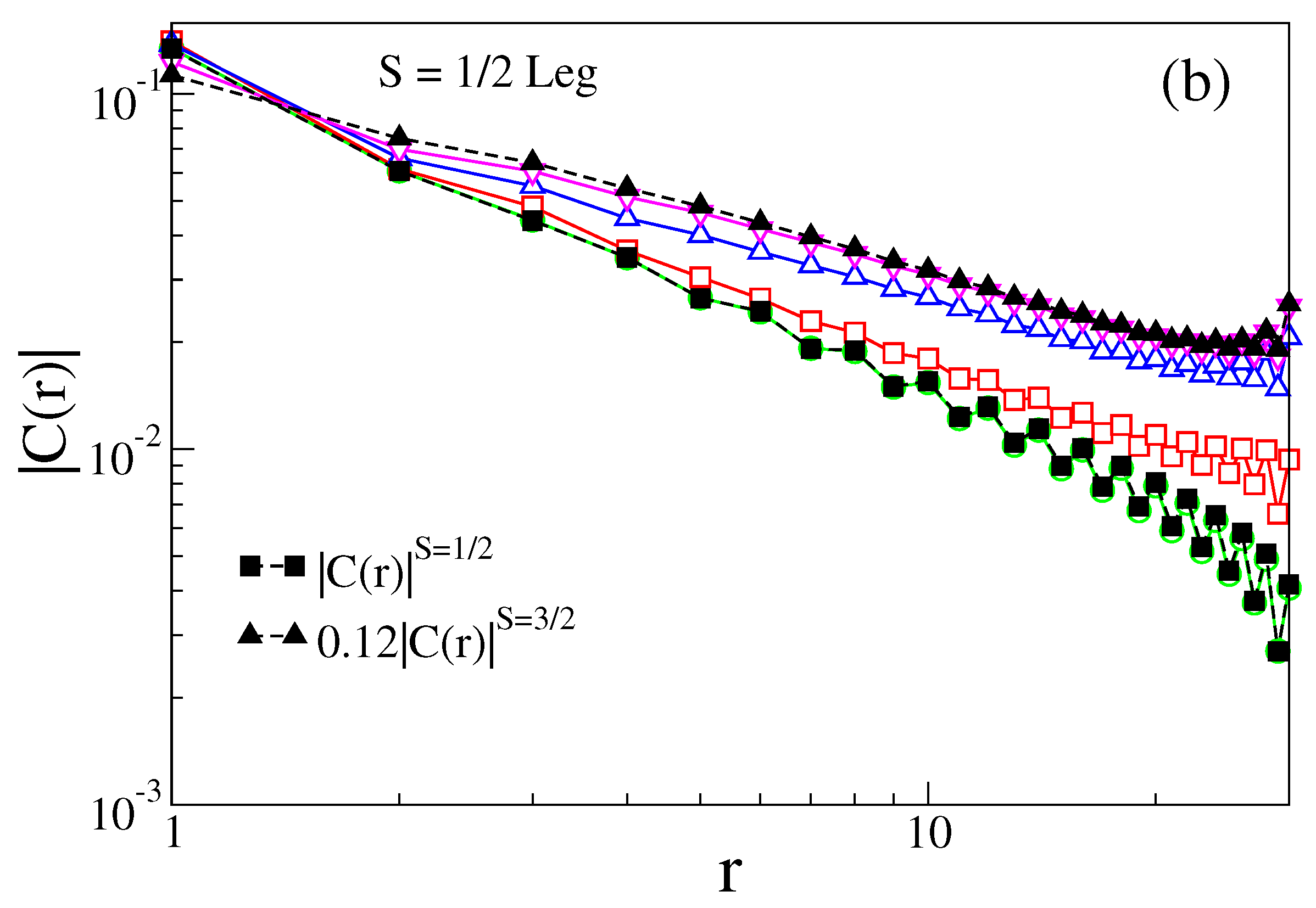
3.3. Spin–Spin Correlations
4. Effective Hamiltonian in Strong Coupling Limit
5. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| HAF | Heisenberg Antiferromagnet |
| gs | Ground State |
| AFM | Antiferromagnetic |
| FM | Ferromagnetic |
| DMRG | Density Matrix Renormalization Group |
| OBC | Open Boundary Condition |
| VBS | Valance Bond Solid |
References
- Bethe, H. Zur Theorie der Metalle. Z. Fur Phys. 1931, 71, 205–226. [Google Scholar] [CrossRef]
- Hulthén, L. Über das Austauschproblem eines Kristalls. Ark. Mat. Astron. Fys. 1938, 26, 106. [Google Scholar]
- Majumdar, C.K.; Ghosh, D.K. On Next-Nearest-Neighbor Interaction in Linear Chain. I. J. Math. Phys. 1969, 10, 1388–1398. [Google Scholar] [CrossRef]
- Majumdar, C.K.; Ghosh, D.K. On Next-Nearest-Neighbor Interaction in Linear Chain. II. J. Math. Phys. 1969, 10, 1399–1402. [Google Scholar] [CrossRef]
- Hamada, T.; Kane, J.i.; Nakagawa, S.i.; Natsume, Y. Exact Solution of Ground State for Uniformly Distributed RVB in One-Dimensional Spin-1/2 Heisenberg Systems with Frustration. J. Phys. Soc. Jpn. 1988, 57, 1891–1894. [Google Scholar] [CrossRef]
- Chubukov, A.V. Chiral, nematic, and dimer states in quantum spin chains. Phys. Rev. B 1991, 44, 4693–4696. [Google Scholar] [CrossRef]
- Tonegawa, T.; Hikihara, T.; Okamoto, K.; Furuya, S.C.; Sakai, T. Ground-State Phase Diagram of an Anisotropic S = 1/2 Ladder with Different Leg Interactions. J. Phys. Soc. Jpn. 2018, 87, 104002. [Google Scholar] [CrossRef]
- Chitra, R.; Pati, S.; Krishnamurthy, H.R.; Sen, D.; Ramasesha, S. Density-matrix renormalization-group studies of the spin-1/2 Heisenberg system with dimerization and frustration. Phys. Rev. B 1995, 52, 6581–6587. [Google Scholar] [CrossRef] [Green Version]
- Kumar, M.; Ramasesha, S.; Soos, Z.G. Bond-order wave phase, spin solitons, and thermodynamics of a frustrated linear spin- Heisenberg antiferromagnet. Phys. Rev. B 2010, 81, 054413. [Google Scholar] [CrossRef] [Green Version]
- Kumar, M.; Soos, Z.G.; Sen, D.; Ramasesha, S. Modified density matrix renormalization group algorithm for the zigzag spin- chain with frustrated antiferromagnetic exchange: Comparison with field theory at large J2/J1. Phys. Rev. B 2010, 81, 104406. [Google Scholar] [CrossRef] [Green Version]
- Kumar, M.; Parvej, A.; Soos, Z.G. Level crossing, spin structure factor and quantum phases of the frustrated spin-1/2 chain with first and second neighbor exchange. J. Phys. Condens. Matter 2015, 27, 316001. [Google Scholar] [CrossRef] [PubMed]
- Soos, Z.G.; Parvej, A.; Kumar, M. Numerical study of incommensurate and decoupled phases of spin-1/2 chains with isotropic exchange J1, J2 between first and second neighbors. J. Phys. Condens. Matter 2016, 28, 175603. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Pati, S.K.; Ramasesha, S.; Sen, D. Low-lying excited states and low-temperature properties of an alternating spin-1–spin-1/2 chain: A density-matrix renormalization-group study. Phys. Rev. B 1997, 55, 8894–8904. [Google Scholar] [CrossRef] [Green Version]
- Ivanov, N.B.; Richter, J.; Schollwöck, U. Frustrated quantum Heisenberg ferrimagnetic chains. Phys. Rev. B 1998, 58, 14456–14461. [Google Scholar] [CrossRef] [Green Version]
- Haldane, F. Continuum dynamics of the 1-D Heisenberg antiferromagnet: Identification with the O(3) nonlinear sigma model. Phys. Lett. A 1983, 93, 464–468. [Google Scholar] [CrossRef]
- Haldane, F.D.M. Nonlinear Field Theory of Large-Spin Heisenberg Antiferromagnets: Semiclassically Quantized Solitons of the One-Dimensional Easy-Axis Néel State. Phys. Rev. Lett. 1983, 50, 1153–1156. [Google Scholar] [CrossRef]
- Moreo, A. Ground-state properties of Heisenberg spin chains. Phys. Rev. B 1987, 35, 8562–8565. [Google Scholar] [CrossRef]
- Affleck, I.; Gepner, D.; Schulz, H.J.; Ziman, T. Critical behaviour of spin-s Heisenberg antiferromagnetic chains: Analytic and numerical results. J. Phys. A 1989, 22, 511. [Google Scholar] [CrossRef]
- Affleck, I.; Kennedy, T.; Lieb, E.H.; Tasaki, H. Valence bond ground states in isotropic quantum antiferromagnets. Commun. Math. Phys. 1988, 115, 477–528. [Google Scholar] [CrossRef]
- White, S.R.; Huse, D.A. Numerical renormalization-group study of low-lying eigenstates of the antiferromagnetic S = 1 Heisenberg chain. Phys. Rev. B 1993, 48, 3844–3852. [Google Scholar] [CrossRef]
- Dagotto, E.; Rice, T.M. Surprises on the Way from One- to Two-Dimensional Quantum Magnets: The Ladder Materials. Science 1996, 271, 618–623. [Google Scholar] [CrossRef]
- Allen, D.; Sénéchal, D. Spin-1 ladder: A bosonization study. Phys. Rev. B 2000, 61, 12134–12142. [Google Scholar] [CrossRef] [Green Version]
- Todo, S.; Matsumoto, M.; Yasuda, C.; Takayama, H. Plaquette-singlet solid state and topological hidden order in a spin-1 antiferromagnetic Heisenberg ladder. Phys. Rev. B 2001, 64, 224412. [Google Scholar] [CrossRef] [Green Version]
- Heilmann, I.U.; Shirane, G.; Endoh, Y.; Birgeneau, R.J.; Holt, S.L. Neutron study of the line-shape and field dependence of magnetic excitations in CuCl2·2N(C5D5). Phys. Rev. B 1978, 18, 3530–3536. [Google Scholar] [CrossRef]
- Hutchings, M.T.; Milne, J.M.; Ikeda, H. Spin wave energy dispersion in KCuF3: A nearly one-dimensional spin-1/2antiferromagnet. J. Phys. C 1979, 12, L739–L744. [Google Scholar] [CrossRef]
- Umegaki, I.; Tanaka, H.; Kurita, N.; Ono, T.; Laver, M.; Niedermayer, C.; Rüegg, C.; Ohira-Kawamura, S.; Nakajima, K.; Kakurai, K. Spinon, soliton, and breather in the spin-12 antiferromagnetic chain compound KCuGaF6. Phys. Rev. B 2015, 92, 174412. [Google Scholar] [CrossRef] [Green Version]
- Buyers, W.J.L.; Morra, R.M.; Armstrong, R.L.; Hogan, M.J.; Gerlach, P.; Hirakawa, K. Experimental evidence for the Haldane gap in a spin-1 nearly isotropic, antiferromagnetic chain. Phys. Rev. Lett. 1986, 56, 371–374. [Google Scholar] [CrossRef]
- Renard, J.P.; Verdaguer, M.; Regnault, L.P.; Erkelens, W.A.C.; Rossat-Mignod, J.; Stirling, W.G. Presumption for a Quantum Energy Gap in the Quasi-One-DimensionalS= 1 Heisenberg Antiferromagnet Ni(C2H8N2)2NO2(ClO4). EPL 1987, 3, 945–952. [Google Scholar] [CrossRef]
- Tsujii, H.; Honda, Z.; Andraka, B.; Katsumata, K.; Takano, Y. High-field phase diagram of the Haldane-gap antiferromagnet Ni(C5H14N2)2N3(PF6). Phys. Rev. B 2005, 71, 014426. [Google Scholar] [CrossRef] [Green Version]
- Sakai, T.; Tonegawa, T.; Okamoto, K. Quantum magnetization plateau of an anisotropic mixed spin chain. J. Phys. Conf. Ser. 2006, 51, 163. [Google Scholar] [CrossRef]
- Lisnyi, B.; Strečka, J. Exactly solved mixed spin-(1,1/2) Ising–Heisenberg diamond chain with a single-ion anisotropy. J. Magn. Magn. Mater. 2015, 377, 502–510. [Google Scholar] [CrossRef]
- Miller, J.S.; Drillon, M. Magnetism: Molecules to Materials IV; John Wiley & Sons: New York, NY, USA, 2002; p. 148. [Google Scholar]
- Lieb, E.; Mattis, D. Ordering Energy Levels of Interacting Spin Systems. J. Math. Phys. 1962, 3, 749–751. [Google Scholar] [CrossRef]
- Hirjibehedin, C.F.; Lutz, C.P.; Heinrich, A.J. Spin Coupling in Engineered Atomic Structures. Science 2006, 312, 1021–1024. [Google Scholar] [CrossRef] [PubMed]
- Mishra, S.; Catarina, G.; Wu, F.; Ortiz, R.; Jacob, D.; Eimre, K.; Ma, J.; Pignedoli, C.A.; Feng, X.; Ruffieux, P.; et al. Observation of fractional edge excitations in nanographene spin chains. Nature 2021, 598, 287–292. [Google Scholar] [CrossRef] [PubMed]
- Sompet, P.; Hirthe, S.; Bourgund, D.; Chalopin, T.; Bibo, J.; Koepsell, J.; Bojović, P.; Verresen, R.; Pollmann, F.; Salomon, G.; et al. Realizing the symmetry-protected Haldane phase in Fermi–Hubbard ladders. Nature 2022, 606, 484–488. [Google Scholar] [CrossRef]
- Lou, J.; Chen, C.; Qin, S. Low-energy properties and magnetization plateaus in a two-leg mixed spin ladder. Phys. Rev. B 2001, 64, 144403. [Google Scholar] [CrossRef] [Green Version]
- White, S.R. Density matrix formulation for quantum renormalization groups. Phys. Rev. Lett. 1992, 69, 2863–2866. [Google Scholar] [CrossRef]
- Hallberg, K.A. New trends in density matrix renormalization. Adv. Phys. 2006, 55, 477–526. [Google Scholar] [CrossRef] [Green Version]
- Schollwöck, U. The density-matrix renormalization group. Rev. Mod. Phys. 2005, 77, 259–315. [Google Scholar] [CrossRef] [Green Version]
- Affleck, I.; Kennedy, T.; Lieb, E.H.; Tasaki, H. Rigorous results on valence-bond ground states in antiferromagnets. Phys. Rev. Lett. 1987, 59, 799–802. [Google Scholar] [CrossRef]
- Dey, D.; Kumar, M.; Soos, Z.G. Boundary-induced spin-density waves in linear Heisenberg antiferromagnetic spin chains with S≥1. Phys. Rev. B 2016, 94, 144417. [Google Scholar] [CrossRef]
- Laukamp, M.; Martins, G.B.; Gazza, C.; Malvezzi, A.L.; Dagotto, E.; Hansen, P.M.; López, A.C.; Riera, J. Enhancement of antiferromagnetic correlations induced by nonmagnetic impurities: Origin and predictions for NMR experiments. Phys. Rev. B 1998, 57, 10755–10769. [Google Scholar] [CrossRef] [Green Version]
- Giamarchi, T.; Schulz, H.J. Correlation functions of one-dimensional quantum systems. Phys. Rev. B 1989, 39, 4620–4629. [Google Scholar] [CrossRef] [PubMed]
- Wada, A.H.O.; Hoyos, J.A. Adaptive density matrix renormalization group study of the disordered antiferromagnetic spin- Heisenberg chain. Phys. Rev. B 2022, 105, 104205. [Google Scholar] [CrossRef]
| A | B | |||
|---|---|---|---|---|
| 0 | − | |||
| 0 | − | |||
| 0 | − |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Maiti, D.; Dey, D.; Kumar, M. Study of Interacting Heisenberg Antiferromagnet Spin-1/2 and 1 Chains. Condens. Matter 2023, 8, 17. https://doi.org/10.3390/condmat8010017
Maiti D, Dey D, Kumar M. Study of Interacting Heisenberg Antiferromagnet Spin-1/2 and 1 Chains. Condensed Matter. 2023; 8(1):17. https://doi.org/10.3390/condmat8010017
Chicago/Turabian StyleMaiti, Debasmita, Dayasindhu Dey, and Manoranjan Kumar. 2023. "Study of Interacting Heisenberg Antiferromagnet Spin-1/2 and 1 Chains" Condensed Matter 8, no. 1: 17. https://doi.org/10.3390/condmat8010017
APA StyleMaiti, D., Dey, D., & Kumar, M. (2023). Study of Interacting Heisenberg Antiferromagnet Spin-1/2 and 1 Chains. Condensed Matter, 8(1), 17. https://doi.org/10.3390/condmat8010017






