Moiré-like Superlattice Generated van Hove Singularities in a Strained CuO2 Double Layer
Abstract
1. Introduction
2. Geometry of the System
3. Tight-Binding Model of Mismatched CuO Bilayer
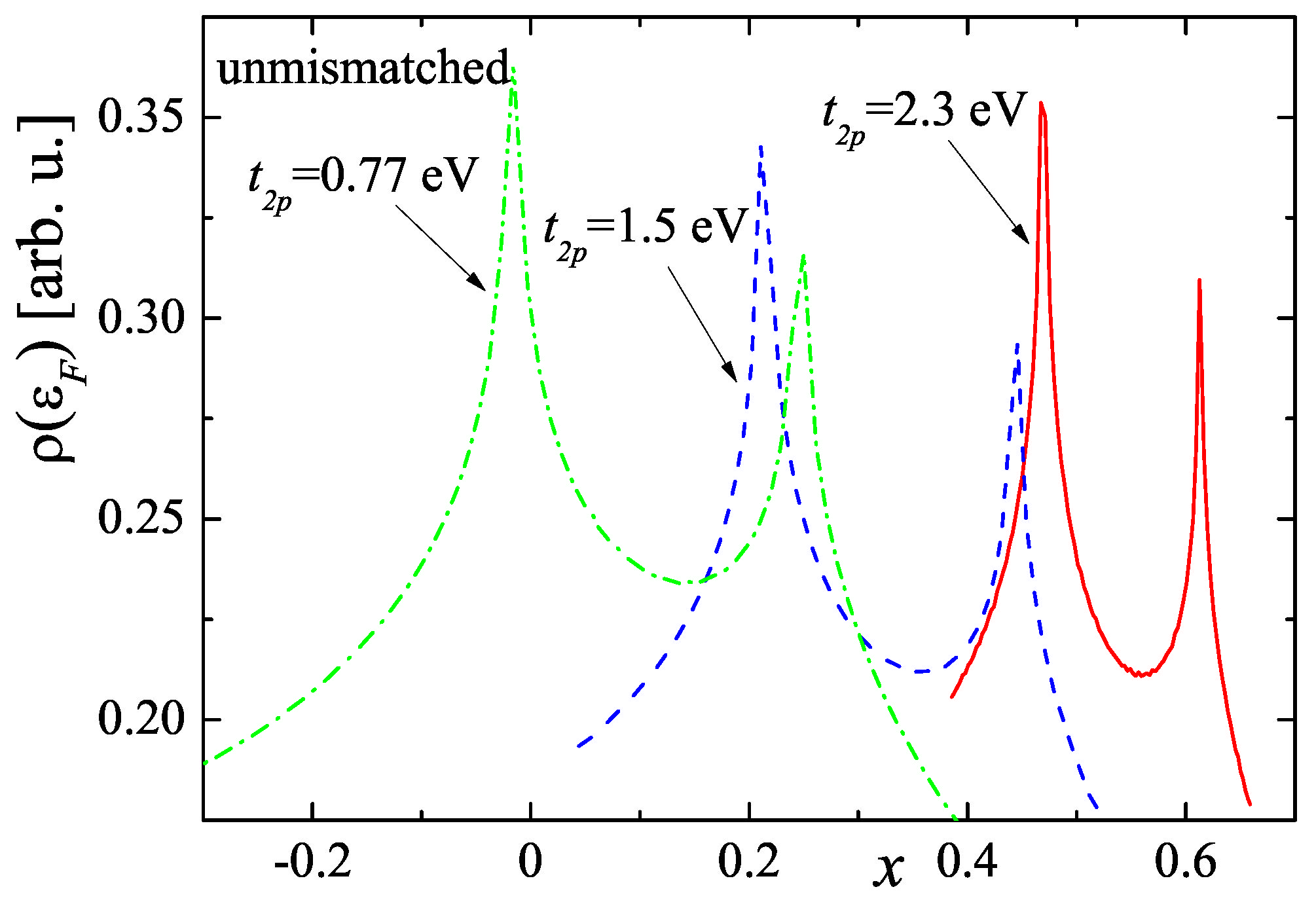
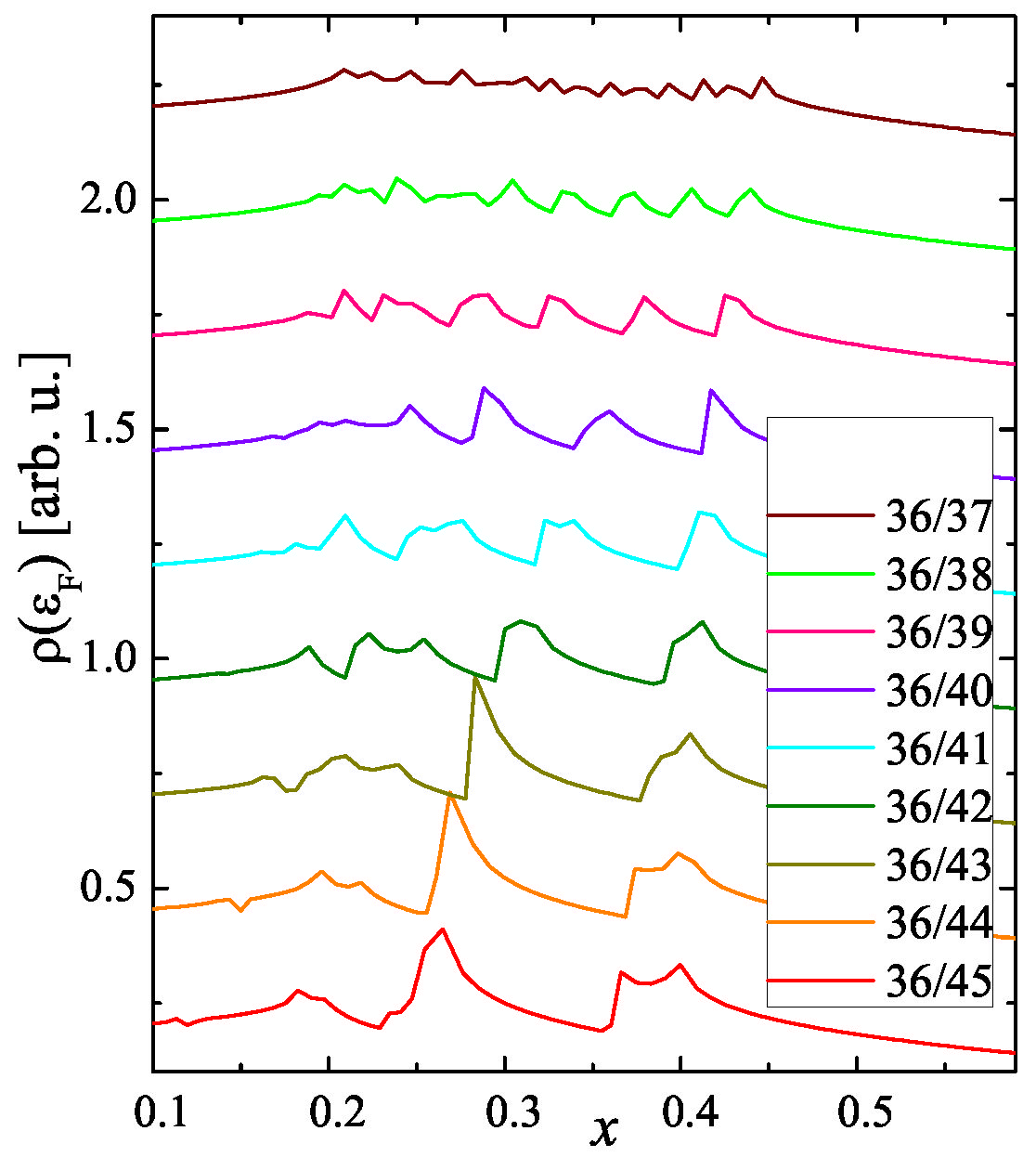
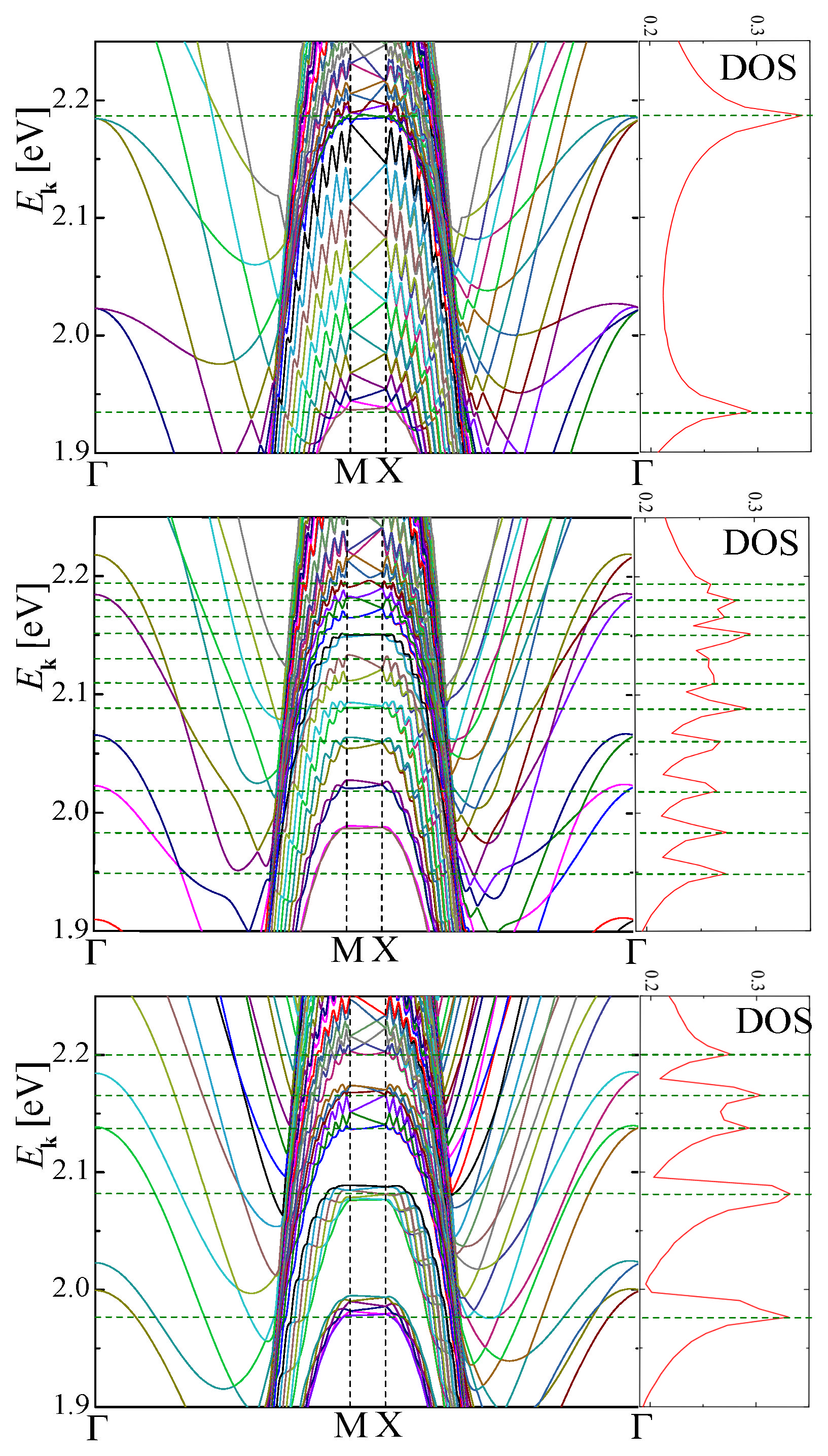
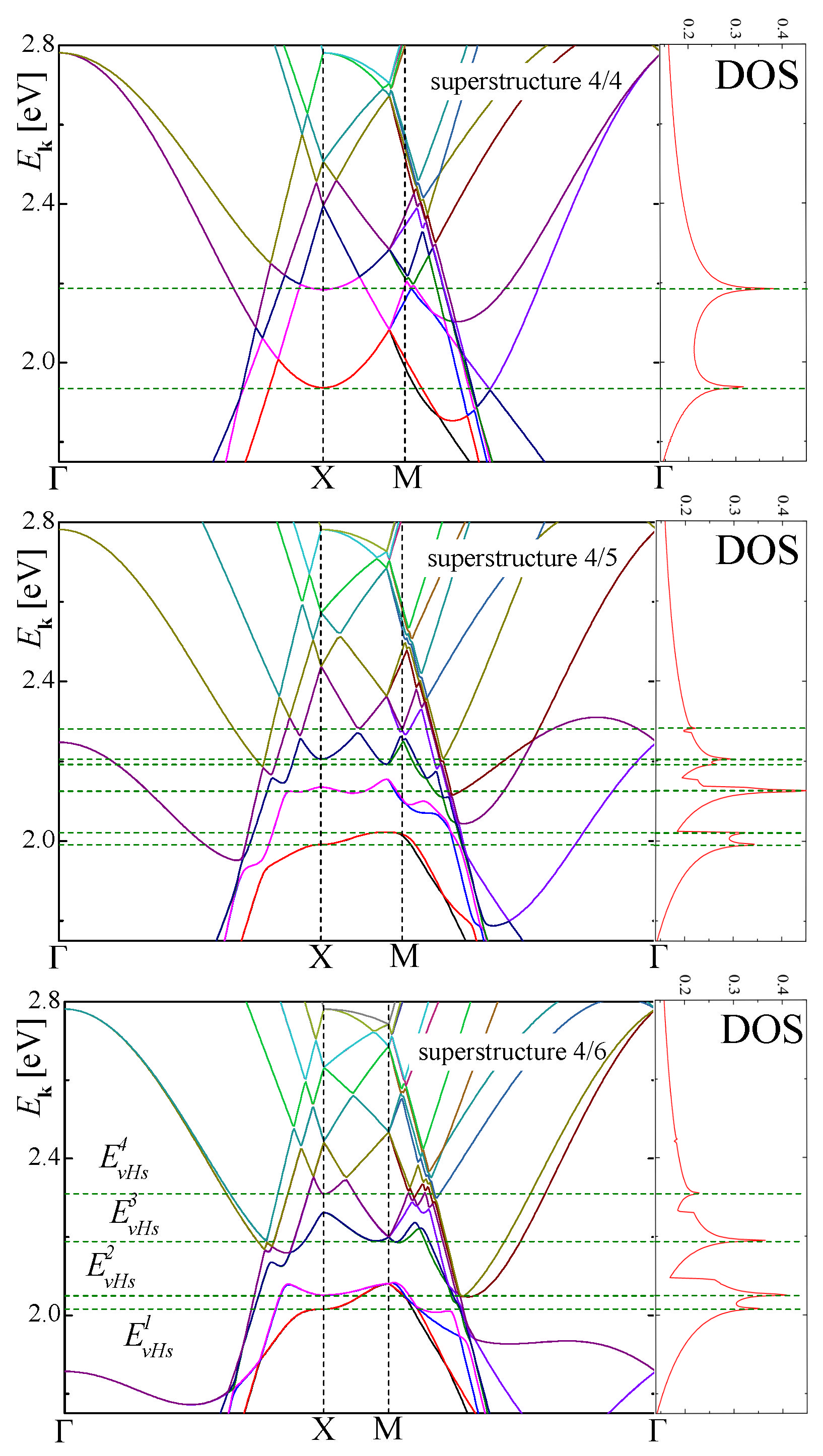
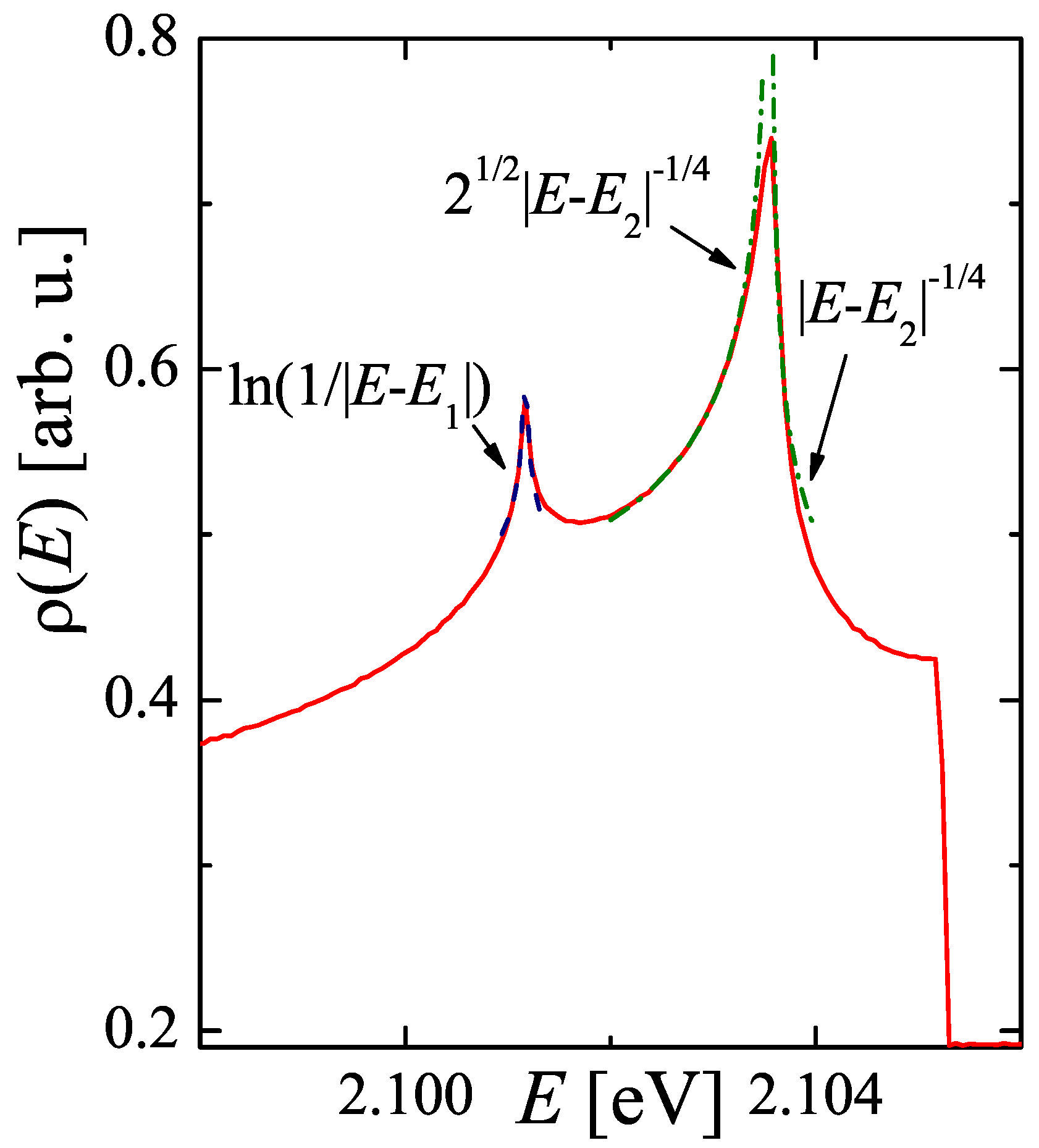
4. Results
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| DOS | density of states |
| VHS | van Hove singularities |
| QCP | quasi-commensurate phase |
| BSCCO | BiSrCaCuO |
| BSCYCO | BiSrCaYCuO |
| BZ | Brilouin zone |
| CDW | charge density wave |
| HEPs | high entropy perovskites |
| STM | scanning tunnel microscopy |
| BCS | Bardeen–Cooper–Schrieffer |
| BEC | Bose–Einstein condensate |
References
- Liu, Y.; Weiss, N.O.; Duan, X.; Cheng, H.C.; Huang, Y.; Duan, X. Van der Waals heterostructures and devices. Nat. Rev. Mater. 2016, 1, 16042. [Google Scholar] [CrossRef]
- Rozhkov, A.; Sboychakov, A.; Rakhmanov, A.; Nori, F. Electronic properties of graphene-based bilayer systems. Phys. Rep. 2016, 648, 1–104. [Google Scholar] [CrossRef]
- Weston, A.; Zou, Y.; Enaldiev, V.; Summerfield, A.; Clark, N.; Zólyomi, V.; Graham, A.; Yelgel, C.; Magorrian, S.; Zhou, M.; et al. Atomic reconstruction in twisted bilayers of transition metal dichalcogenides. Nat. Nanotechnol. 2020, 15, 592–597. [Google Scholar] [CrossRef]
- Zhao, W.M.; Zhu, L.; Nie, Z.; Li, Q.Y.; Wang, Q.W.; Dou, L.G.; Hu, J.G.; Xian, L.; Meng, S.; Li, S.C. Moiré enhanced charge density wave state in twisted 1T-TiTe2/1T-TiSe2 heterostructures. Nat. Mater. 2021, 21, 284–289. [Google Scholar] [CrossRef]
- Tong, Q.; Yu, H.; Zhu, Q.; Wang, Y.; Xu, X.; Yao, W. Topological mosaics in moiré superlattices of van der Waals heterobilayers. Nat. Phys. 2017, 13, 356–362. [Google Scholar] [CrossRef]
- Ren, Y.N.; Zhang, Y.; Liu, Y.W.; He, L. Twistronics in graphene-based van der Waals structures. Chin. Phys. B 2020, 29, 117303. [Google Scholar] [CrossRef]
- Krockenberger, Y.; Ikeda, A.; Yamamoto, H. Atomic stripe formation in infinite-layer cuprates. ACS Omega 2021, 6, 21884–21891. [Google Scholar] [CrossRef] [PubMed]
- Park, H.; Oh, J.; Song, B.; Kang, B. Structural suitability of GdFeO3 as a magnetic buffer layer for GdBa2Cu3O7-x superconducting thin films. Progr. Supercond. Cryog. 2021, 23, 14–18. [Google Scholar] [CrossRef]
- Conradson, S.D.; Geballe, T.H.; Jin, C.Q.; Cao, L.P.; Gauzzi, A.; Karppinen, M.; Baldinozzi, G.; Li, W.M.; Gilioli, E.; Jiang, J.M.; et al. Nonadiabatic coupling of the dynamical structure to the superconductivity in YSr2Cu2.75Mo0.25O7.54 and Sr2CuO3.3. Proc. Natl. Acad. Sci. USA 2020, 117, 33099–33106. [Google Scholar] [CrossRef]
- Sederholm, L.; Conradson, S.D.; Geballe, T.H.; Jin, C.Q.; Gauzzi, A.; Gilioli, E.; Karppinen, M.; Baldinozzi, G. Extremely overdoped superconducting cuprates via high pressure oxygenation methods. Condens. Matter 2021, 6, 50. [Google Scholar] [CrossRef]
- Jang, H.; Asano, S.; Fujita, M.; Hashimoto, M.; Lu, D.H.; Burns, C.A.; Kao, C.C.; Lee, J.S. Superconductivity-insensitive order at q∼1/4 in electron-doped cuprates. Phys. Rev. X 2017, 7, 041066. [Google Scholar] [CrossRef]
- Bianconi, A.; Saini, N.L.; Lanzara, A.; Missori, M.; Rossetti, T.; Oyanagi, H.; Yamaguchi, H.; Oka, K.; Ito, T. Determination of the local lattice distortions in the CuO2 plane of La1.85Sr0.15CuO4. Phys. Rev. Lett. 1996, 76, 3412–3415. [Google Scholar] [CrossRef] [PubMed]
- Saini, N.; Oyanagi, H.; Ito, T.; Scagnoli, V.; Filippi, M.; Agrestini, S.; Campi, G.; Oka, K.; Bianconi, A. Temperature dependent local Cu-O displacements from underdoped to overdoped La-Sr-Cu-O superconductor. Eur. Phys. J. B 2003, 36, 75–80. [Google Scholar] [CrossRef]
- Bianconi, A.; Lusignoli, M.; Saini, N.L.; Bordet, P.; Kvick, Å.; Radaelli, P.G. Stripe structure of the CuO2 plane in Bi2Sr2CaCu2O8+y by anomalous X-ray diffraction. Phys. Rev. B 1996, 54, 4310–4314. [Google Scholar] [CrossRef]
- Bianconi, A.; Saini, N.L.; Rossetti, T.; Lanzara, A.; Perali, A.; Missori, M.; Oyanagi, H.; Yamaguchi, H.; Nishihara, Y.; Ha, D.H. Stripe structure in the CuO2 plane of perovskite superconductors. Phys. Rev. B 1996, 54, 12018–12021. [Google Scholar] [CrossRef]
- Di Castro, D.; Bianconi, G.; Colapietro, M.; Pifferi, A.; Saini, N.; Agrestini, S.; Bianconi, A. Temperature dependent local Cu-O displacements from underdoped to overdoped La-Sr-Cu-O superconductor. Eur. Phys. J. B 2000, 18, 617–624. [Google Scholar] [CrossRef]
- Bianconi, A.; Bianconi, G.; Caprara, S.; Castro, D.D.; Oyanagi, H.; Saini, N.L. The stripe critical point for cuprates. J. Phys : Condens. Matter 2000, 12, 10655–10666. [Google Scholar] [CrossRef]
- Agrestini, S.; Saini, N.; Bianconi, G.; Bianconi, A. The strain of CuO2 lattice: The second variable for the phase diagram of cuprate perovskites. J. Phys. A Math. Gen. 2003, 36, 9133–9142. [Google Scholar] [CrossRef]
- Wiegers, G. Misfit layer compounds: Structures and physical properties. Prog. Solid State Chem. 1996, 24, 1–139. [Google Scholar] [CrossRef]
- Janssen, T.; Janner, A.; Looijenga-Vos, A.; de Wolff, P. Chapter in 9.8 Incommensurate and Commensurate Modulated Structures. In International Tables for Crystallography, Mathematical, Physical and Chemical Tables; IUCr Series; Prince, E., Ed.; Wiley: New York, NY, USA, 2004; Volume C. [Google Scholar]
- Etrillard, J.; Bourges, P.; Lin, C.T. Incommensurate composite structure of the superconductor Bi2Sr2CaCu2O8+δ. Phys. Rev. B 2000, 62, 150–153. [Google Scholar] [CrossRef]
- Grebille, D.; Leligny, H.; Pérez, O. Comment on “Incommensurate composite structure of the superconductor Bi2Sr2CaCu2O8+δ”. Phys. Rev. B 2001, 64, 106501. [Google Scholar] [CrossRef]
- Bianconi, A. On the Fermi liquid coupled with a generalized Wigner polaronic CDW giving high Tc superconductivity. Solid State Commun. 1994, 91, 1–5. [Google Scholar] [CrossRef]
- Beskrovnyy, A.; Jirák, Z. Structural modulation in Bi2Sr2Ca0.4Y0.6Cu2O8+δ. J. Phys. Conf. Ser. 2012, 340. [Google Scholar] [CrossRef]
- Pérez, O.; Leligny, H.; Grebille, D.; Grenêche, J.M.; Labbé, P.; Groult, D.; Raveau, B. Disorder phenomena in the incommensurate compound Bi2+xSr3-xFe2O9+δ. Phys. Rev. B 1997, 55, 1236–1246. [Google Scholar] [CrossRef]
- Sedykh, V.; Shekhtman, V.; Smirnova, I.; Bagautdinov, B.; Suvorov, E.; Dubovitskii, A. About specific features of the structure modulation in the Bi-ferrate compounds isostructural with Bi2Sr2CaCu2O8. Phys. C 2003, 390, 311–320. [Google Scholar] [CrossRef]
- Lambert, S.; Leligny, H.; Grebille, D. Three forms of the misfit layered cobaltite [Ca2CoO3][CoO2]1.62. A 4D structural investigation. J. Solid State Chem. 2001, 160, 322–331. [Google Scholar] [CrossRef]
- Grebille, D.; Muguerra, H.; Pérez, O.; Guilmeau, E.; Rousselière, H.; Funahashi, R. Superspace crystal symmetry of thermoelectric misfit cobalt oxides and predicted structural models. Acta Cryst. B 2007, 63, 373–383. [Google Scholar] [CrossRef]
- Kim, J.Y.; Kim, J.I.; Choi, S.M.; Soo Lim, Y.; Seo, W.S.; Jin Hwang, H. Nanostructured thermoelectric cobalt oxide by exfoliation/restacking route. J. Appl. Phys. 2012, 112, 113705. [Google Scholar] [CrossRef]
- Gavrichkov, V.A.; Shan’ko, Y.; Zamkova, N.G.; Bianconi, A. Is there any hidden symmetry in the stripe structure of perovskite high-temperature superconductors? J. Phys. Chem. Lett. 2019, 10, 1840–1844. [Google Scholar] [CrossRef]
- Mazza, A.R.; Gao, X.; Rossi, D.J.; Musico, B.L.; Valentine, T.W.; Kennedy, Z.; Zhang, J.; Lapano, J.; Keppens, V.; Moore, R.G.; et al. Searching for superconductivity in high entropy oxide Ruddlesden–Popper cuprate films. J. Vac. Sci. Tech. A 2022, 40, 013404. [Google Scholar] [CrossRef]
- Kaneko, S.; Shimizu, Y.; Akiyama, K.; Ito, T.; Mitsuhashi, M.; Ohya, S.; Saito, K.; Funakubo, H.; Yoshimoto, M. Modulation derived satellite peaks in X-ray reciprocal mapping on bismuth cuprate superconductor film. Appl. Phys. Lett. 2004, 85, 2301–2303. [Google Scholar] [CrossRef]
- Poccia, N.; Campi, G.; Fratini, M.; Ricci, A.; Saini, N.L.; Bianconi, A. Spatial inhomogeneity and planar symmetry breaking of the lattice incommensurate supermodulation in the high-temperature superconductor Bi2Sr2CaCu2O8+y. Phys. Rev. B 2011, 84, 100504. [Google Scholar] [CrossRef]
- Poccia, N.; Zhao, S.Y.F.; Yoo, H.; Huang, X.; Yan, H.; Chu, Y.S.; Zhong, R.; Gu, G.; Mazzoli, C.; Watanabe, K.; et al. Spatially correlated incommensurate lattice modulations in an atomically thin high-temperature Bi2.1Sr1.9CaCu2.0O8+y superconductor. Phys. Rev. Mater. 2020, 4, 114007. [Google Scholar] [CrossRef]
- Zhang, Y.; Hu, C.; Hu, Y.; Zhao, L.; Ding, Y.; Sun, X.; Liang, A.; Zhang, Y.; He, S.; Liu, D.; et al. In situ carrier tuning in high temperature superconductor Bi2Sr2CaCu2O8+δ by potassium deposition. Sci. Bull. 2016, 61, 1037–1043. [Google Scholar] [CrossRef][Green Version]
- Bianconi, A.; Poccia, N. Superstripes and complexity in high-temperature superconductors. J. Supercond. Novel Magn. 2012, 25, 1403–1412. [Google Scholar] [CrossRef]
- Bianconi, A.; Longa, S.D.; Missori, M.; Pettiti, I.; Pompa, M.; Soldatov, A. Structure of the different Cu sites in the corrugated CuO2 plane in high Tc superconductors. Jpn. J. Appl. Phys. 1993, 32, 578. [Google Scholar] [CrossRef]
- Alldredge, J.; Lee, J.; McElroy, K.; Wang, M.; Fujita, K.; Kohsaka, Y.; Taylor, C.; Eisaki, H.; Uchida, S.; Hirschfeld, P.; et al. Evolution of the electronic excitation spectrum with strongly diminishing hole density in superconducting Bi2Sr2CaCu2O8+δ. Nat. Phys. 2008, 4, 319–326. [Google Scholar] [CrossRef]
- Pasupathy, A.N.; Pushp, A.; Gomes, K.K.; Parker, C.V.; Wen, J.; Xu, Z.; Gu, G.; Ono, S.; Ando, Y.; Yazdani, A. Electronic origin of the inhomogeneous pairing interaction in the high-Tc superconductor Bi2Sr2CaCu2O8+δ. Science 2008, 320, 196–201. [Google Scholar] [CrossRef][Green Version]
- Gomes, K.K.; Pasupathy, A.N.; Pushp, A.; Ono, S.; Ando, Y.; Yazdani, A. Visualizing pair formation on the atomic scale in the high-Tc superconductor Bi2Sr2CaCu2O8+δ. Nature 2007, 447, 569–572. [Google Scholar] [CrossRef]
- Božović, I.; He, X.; Wu, J.; Bollinger, A. Dependence of the critical temperature in overdoped copper oxides on superfluid density. Nature 2016, 536, 309–311. [Google Scholar] [CrossRef]
- He, Y.; Chen, S.D.; Li, Z.X.; Zhao, D.; Song, D.; Yoshida, Y.; Eisaki, H.; Wu, T.; Chen, X.H.; Lu, D.H.; et al. Superconducting fluctuations in overdoped Bi2Sr2CaCu2O8+δ. Phys. Rev. X 2021, 11, 031068. [Google Scholar] [CrossRef]
- Drozdov, I.K.; Pletikosić, I.; Kim, C.K.; Fujita, K.; Gu, G.; Davis, J.C.S.; Johnson, P.; Božović, I.; Valla, T. Phase diagram of Bi2Sr2CaCu2O8+δ revisited. Nat. Commun. 2018, 9, 5210. [Google Scholar] [CrossRef]
- Li, X.; Ding, Y.; He, C.; Ruan, W.; Cai, P.; Ye, C.; Hao, Z.; Zhao, L.; Zhou, X.; Wang, Q.; et al. Quasiparticle interference and charge order in a heavily overdoped non-superconducting cuprate. New J. Phys. 2018, 20, 063041. [Google Scholar] [CrossRef]
- Maier, T.A.; Karakuzu, S.; Scalapino, D.J. Overdoped end of the cuprate phase diagram. Phys. Rev. Res. 2020, 2, 033132. [Google Scholar] [CrossRef]
- Kagan, M.Y.; Kugel, K.I.; Rakhmanov, A.L. Electronic phase separation: Recent progress in the old problem. Phys. Rep. 2021, 916, 1–105. [Google Scholar] [CrossRef]
- Rakhmanov, A.L.; Kugel, K.I.; Kagan, M.Y.; Rozhkov, A.V.; Sboychakov, A.O. Inhomogeneous electron states in the systems with imperfect nesting. JETP Lett. 2017, 105, 806–817. [Google Scholar] [CrossRef]
- Kugel, K.I.; Rakhmanov, A.L.; Sboychakov, A.O.; Poccia, N.; Bianconi, A. Model for phase separation controlled by doping and the internal chemical pressure in different cuprate superconductors. Phys. Rev. B 2008, 78, 165124. [Google Scholar] [CrossRef]
- Bianconi, A.; Poccia, N.; Sboychakov, A.O.; Rakhmanov, A.L.; Kugel, K.I. Intrinsic arrested nanoscale phase separation near a topological Lifshitz transition in strongly correlated two-band metals. Supercond. Sci. Technol. 2015, 28, 024005. [Google Scholar] [CrossRef]
- Kugel, K.I.; Rakhmanov, A.L.; Sboychakov, A.O.; Kusmartsev, F.V.; Poccia, N.; Bianconi, A. A two-band model for the phase separation induced by the chemical mismatch pressure in different cuprate superconductors. Supercond. Sci. Technol. 2008, 22, 014007. [Google Scholar] [CrossRef]
- Massidda, S.; Yu, J.; Freeman, A. Electronic structure and properties of Bi2Sr2CaCu2O8, the third high-Tc superconductor. Phys. C 1988, 152, 251–258. [Google Scholar] [CrossRef]
- Nokelainen, J.; Lane, C.; Markiewicz, R.S.; Barbiellini, B.; Pulkkinen, A.; Singh, B.; Sun, J.; Pussi, K.; Bansil, A. Ab initio description of the Bi2Sr2CaCu2O8+δ electronic structure. Phys. Rev. B 2020, 101, 214523. [Google Scholar] [CrossRef]
- Hewat, E.; Capponi, J.; Marezio, M. A model for the superstructure of Bi2Sr2CaCu2O8.2. Phys. C 1989, 157, 502–508. [Google Scholar] [CrossRef]
- Bak, P. Commensurate phases, incommensurate phases and the devil’s staircase. Rep. Prog. Phys. 1982, 45, 587–629. [Google Scholar] [CrossRef]
- Andersen, O.; Liechtenstein, A.; Jepsen, O.; Paulsen, F. LDA energy bands, low-energy Hamiltonians, t′, t′′t⊥(k), and J⊥. J. Phys. Chem. Solids 1995, 56, 1573–1591. [Google Scholar] [CrossRef]
- Slater, J.C.; Koster, G.F. Simplified LCAO method for the periodic potential problem. Phys. Rev. 1954, 94, 1498–1524. [Google Scholar] [CrossRef]
- Pavarini, E.; Dasgupta, I.; Saha-Dasgupta, T.; Jepsen, O.; Andersen, O.K. Band-Structure Trend in Hole-Doped Cuprates and Correlation with Tc max. Phys. Rev. Lett. 2001, 87, 047003. [Google Scholar] [CrossRef]
- Yuan, N.F.Q.; Isobe, H.; Fu, L. Magic of high-order van Hove singularity. Nat. Commun. 2019, 10, 5769. [Google Scholar] [CrossRef]
- Isobe, H.; Fu, L. Supermetal. Phys. Rev. Res. 2019, 1, 033206. [Google Scholar] [CrossRef]
- Classen, L.; Chubukov, A.V.; Honerkamp, C.; Scherer, M.M. Competing orders at higher-order Van Hove points. Phys. Rev. B 2020, 102, 125141. [Google Scholar] [CrossRef]
- Guerci, D.; Simon, P.; Mora, C. Higher-order Van Hove singularity in magic-angle twisted trilayer graphene. Phys. Rev. Res. 2022, 4, L012013. [Google Scholar] [CrossRef]
- Lopes dos Santos, J.M.B.; Peres, N.M.R.; Castro Neto, A.H. Continuum model of the twisted graphene bilayer. Phys. Rev. B 2012, 86, 155449. [Google Scholar] [CrossRef]
- Sboychakov, A.O.; Rakhmanov, A.L.; Rozhkov, A.V.; Nori, F. Electronic spectrum of twisted bilayer graphene. Phys. Rev. B 2015, 92, 075402. [Google Scholar] [CrossRef]
- Mazziotti, M.V.; Valletta, A.; Raimondi, R.; Bianconi, A. Multigap superconductivity at an unconventional Lifshitz transition in a three-dimensional Rashba heterostructure at the atomic limit. Phys. Rev. B 2021, 103, 024523. [Google Scholar] [CrossRef]
- Perali, A.; Bianconi, A.; Lanzara, A.; Saini, N.L. The gap amplification at a shape resonance in a superlattice of quantum stripes: A mechanism for high Tc. Solid State Commun. 1996, 100, 181–186. [Google Scholar] [CrossRef]
- Perali, A.; Innocenti, D.; Valletta, A.; Bianconi, A. Anomalous isotope effect near a 2.5 Lifshitz transition in a multi-band multi-condensate superconductor made of a superlattice of stripes. Supercond. Sci. Technol. 2012, 25, 124002. [Google Scholar] [CrossRef]
- Chen, Y.; Shanenko, A.A.; Perali, A.; Peeters, F.M. Superconducting nanofilms: Molecule-like pairing induced by quantum confinement. J. Phys. Condens. Matter 2012, 24, 185701. [Google Scholar] [CrossRef]
- Salasnich, L.; Shanenko, A.A.; Vagov, A.A.; Aguiar, J.A.; Perali, A. Screening of pair fluctuations in superconductors with coupled shallow and deep bands: A route to higher-temperature superconductivity. Phys. Rev. B 2019, 100, 064510. [Google Scholar] [CrossRef]
- Agrestini, S.; Metallo, C.; Filippi, M.; Simonelli, L.; Campi, G.; Sanipoli, C.; Liarokapis, E.; De Negri, S.; Giovannini, M.; Saccone, A.; et al. Substitution of Sc for Mg in MgB2: Effects on transition temperature and Kohn anomaly. Phys. Rev. B 2004, 70, 134514. [Google Scholar] [CrossRef]
- Bianconi, A.; Innocenti, D.; Valletta, A.; Perali, A. Shape resonances in superconducting gaps in a 2DEG at oxide-oxide interface. J. Phys. Conf. Ser. 2014, 529, 012007. [Google Scholar] [CrossRef]
- Mazziotti, M.V.; Raimondi, R.; Valletta, A.; Campi, G.; Bianconi, A. Resonant multi-gap superconductivity at room temperature near a Lifshitz topological transition in sulfur hydrides. J. Appl. Phys. 2021, 130, 173904. [Google Scholar] [CrossRef]
- Mazziotti, M.V.; Jarlborg, T.; Bianconi, A.; Valletta, A. Room temperature superconductivity dome at a Fano resonance in superlattices of wires. EPL (Europhys. Lett.) 2021, 134, 17001. [Google Scholar] [CrossRef]
- Tromp, W.O.; Benschop, T.; Ge, J.F.; Battist, I.; Bastiaans, K.M.; Chatzopoulos, D.; Vervloet, A.; Smit, S.; van Heumen, E.; Golden, M.S.; et al. Puddle formation, persistent gaps, and non-mean-field breakdown of superconductivity in overdoped (Pb, Bi)2Sr2CuO6+δ. arXiv 2022, arXiv:2205.09740. [Google Scholar]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sboychakov, A.O.; Kugel, K.I.; Bianconi, A. Moiré-like Superlattice Generated van Hove Singularities in a Strained CuO2 Double Layer. Condens. Matter 2022, 7, 50. https://doi.org/10.3390/condmat7030050
Sboychakov AO, Kugel KI, Bianconi A. Moiré-like Superlattice Generated van Hove Singularities in a Strained CuO2 Double Layer. Condensed Matter. 2022; 7(3):50. https://doi.org/10.3390/condmat7030050
Chicago/Turabian StyleSboychakov, Artem O., Kliment I. Kugel, and Antonio Bianconi. 2022. "Moiré-like Superlattice Generated van Hove Singularities in a Strained CuO2 Double Layer" Condensed Matter 7, no. 3: 50. https://doi.org/10.3390/condmat7030050
APA StyleSboychakov, A. O., Kugel, K. I., & Bianconi, A. (2022). Moiré-like Superlattice Generated van Hove Singularities in a Strained CuO2 Double Layer. Condensed Matter, 7(3), 50. https://doi.org/10.3390/condmat7030050






