From SrTiO3 to Cuprates and Back to SrTiO3: A Way Along Alex Müller’s Scientific Career
Abstract
1. Introduction
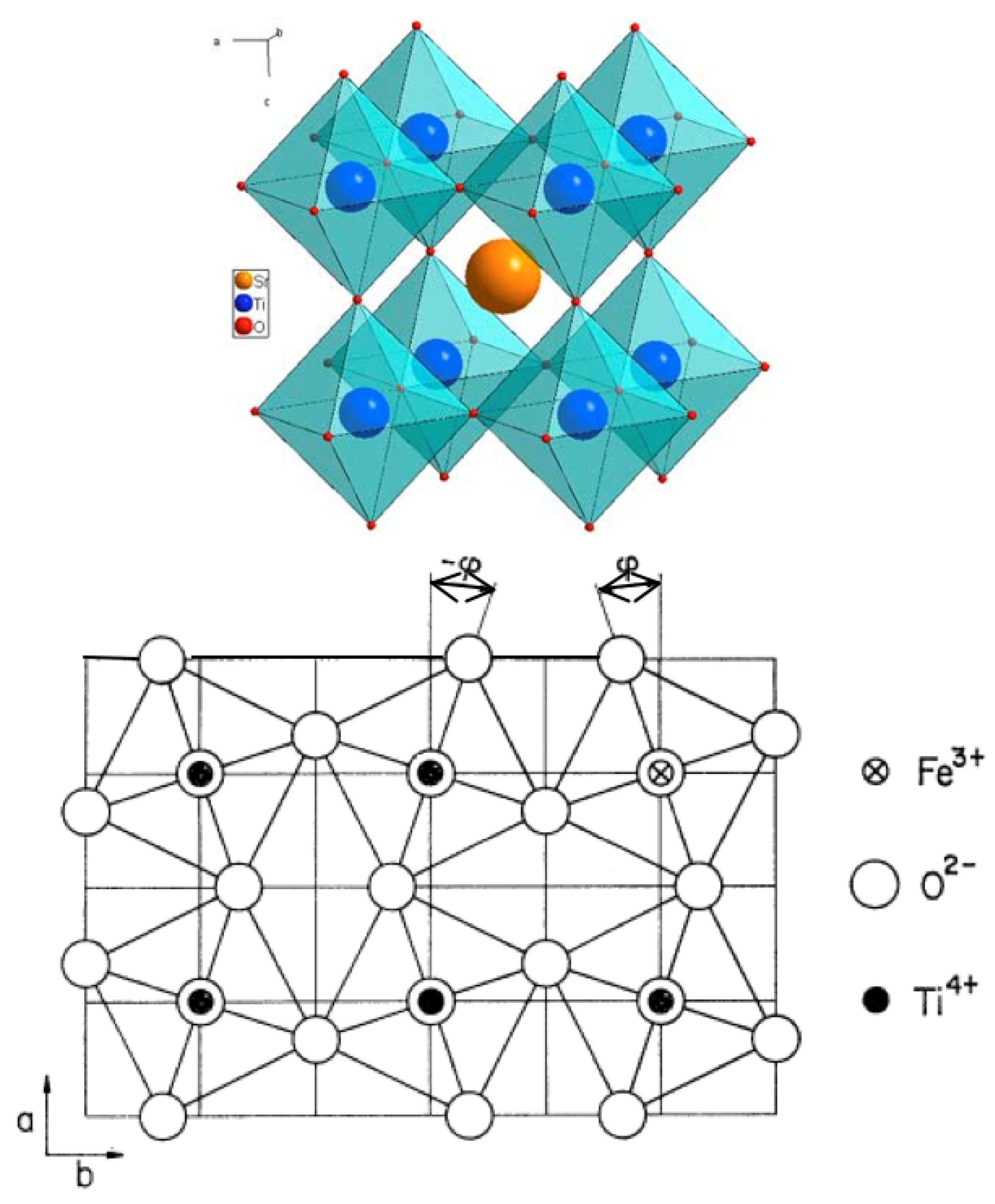
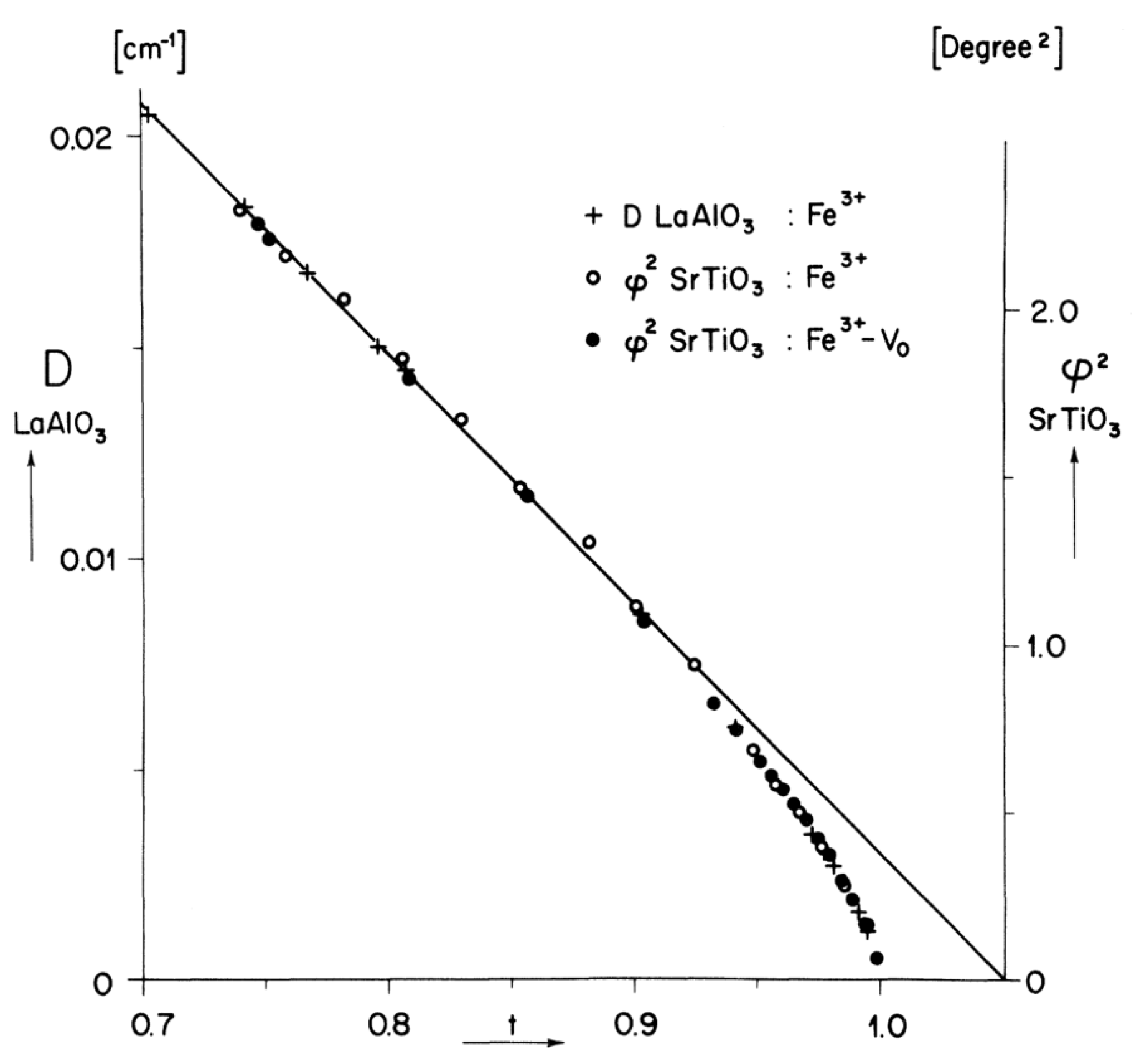
2. Polar and Rotational Instabilities in Perovskite Oxides
3. High-Temperature Superconductivity in Cuprates
3.1. Discovery of Superconductivity in the Cuprates
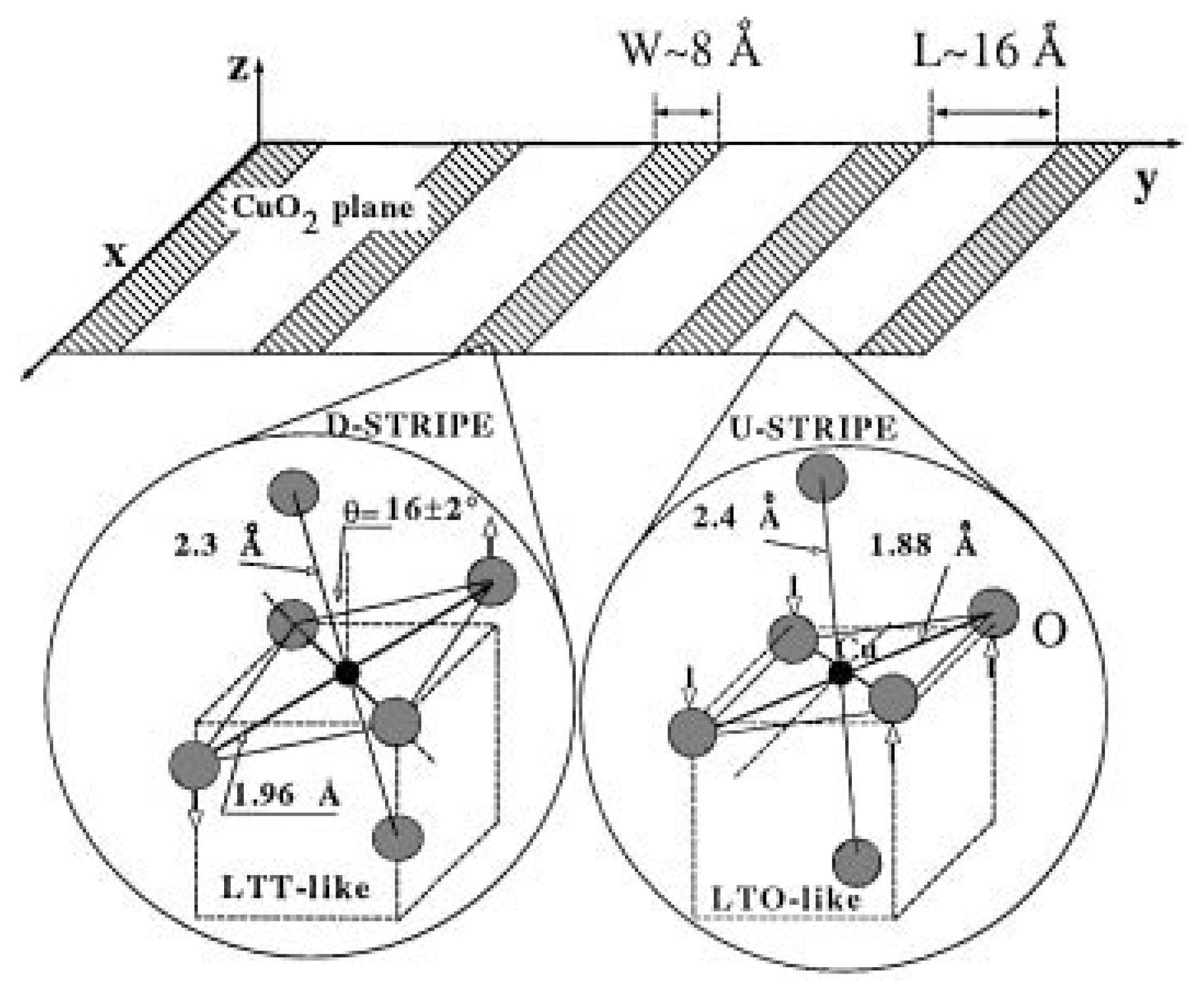
3.2. Essential Heterogeneities and Mixed Order Parameters in Cuprate Superconductors
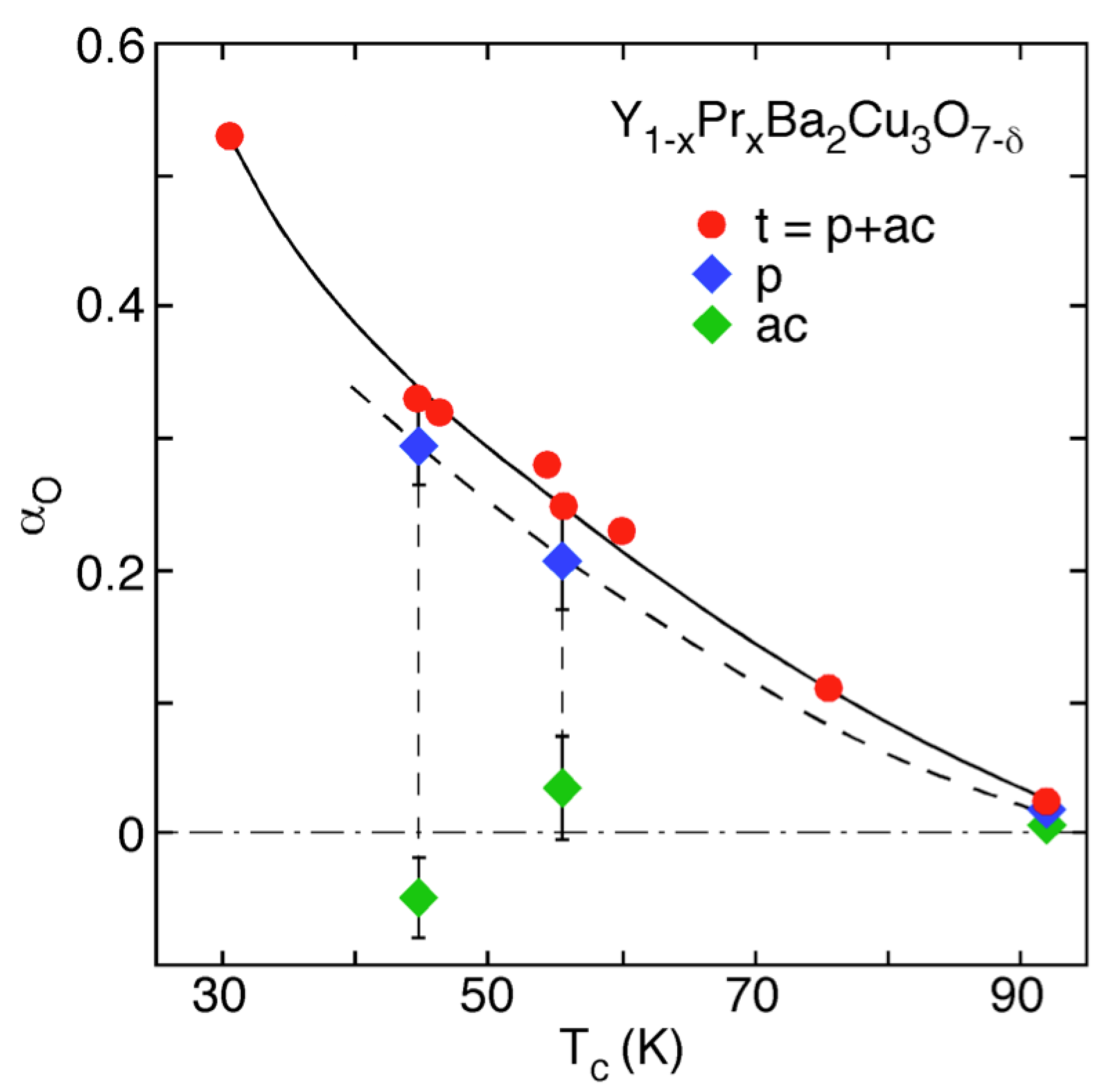
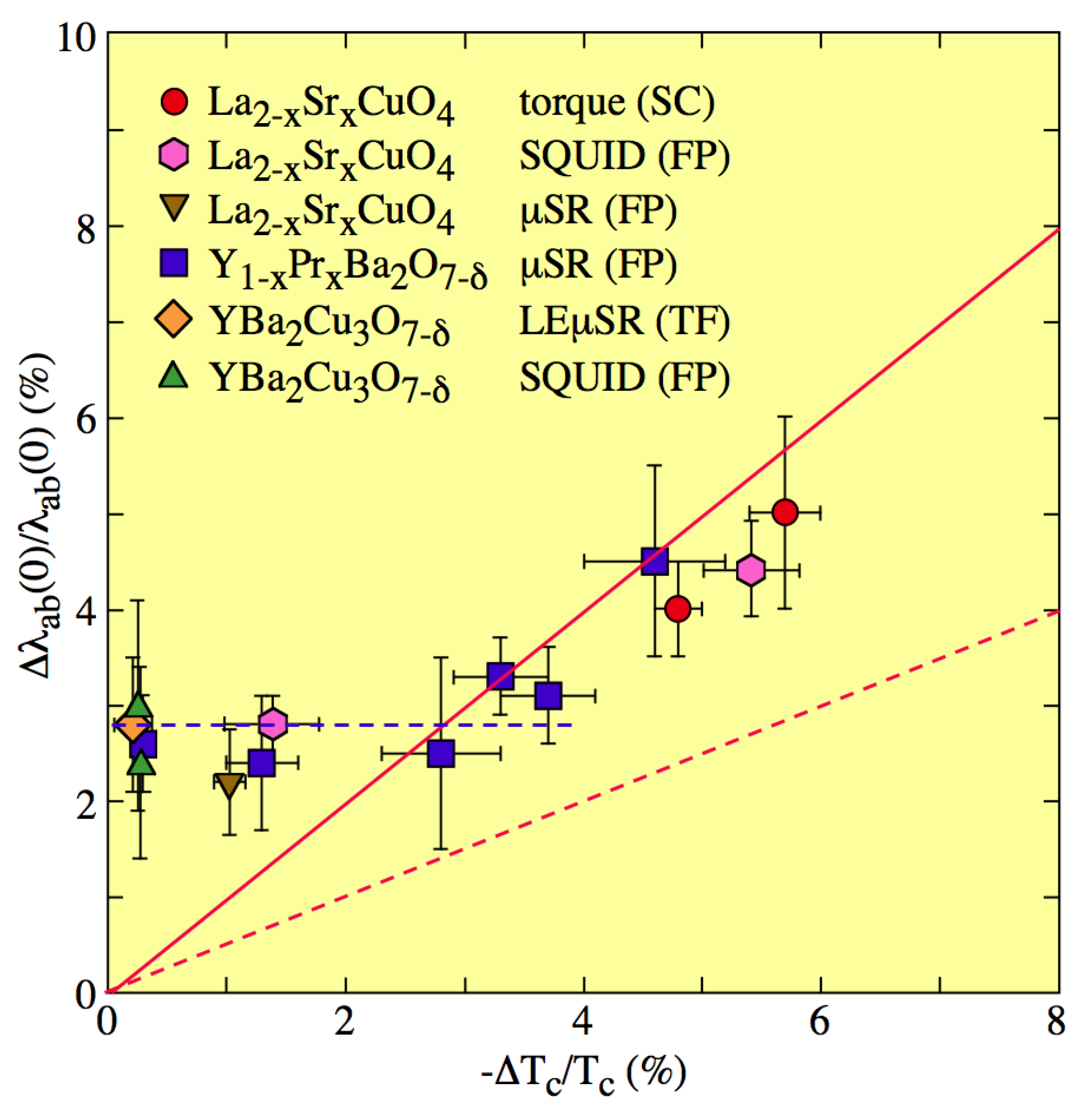
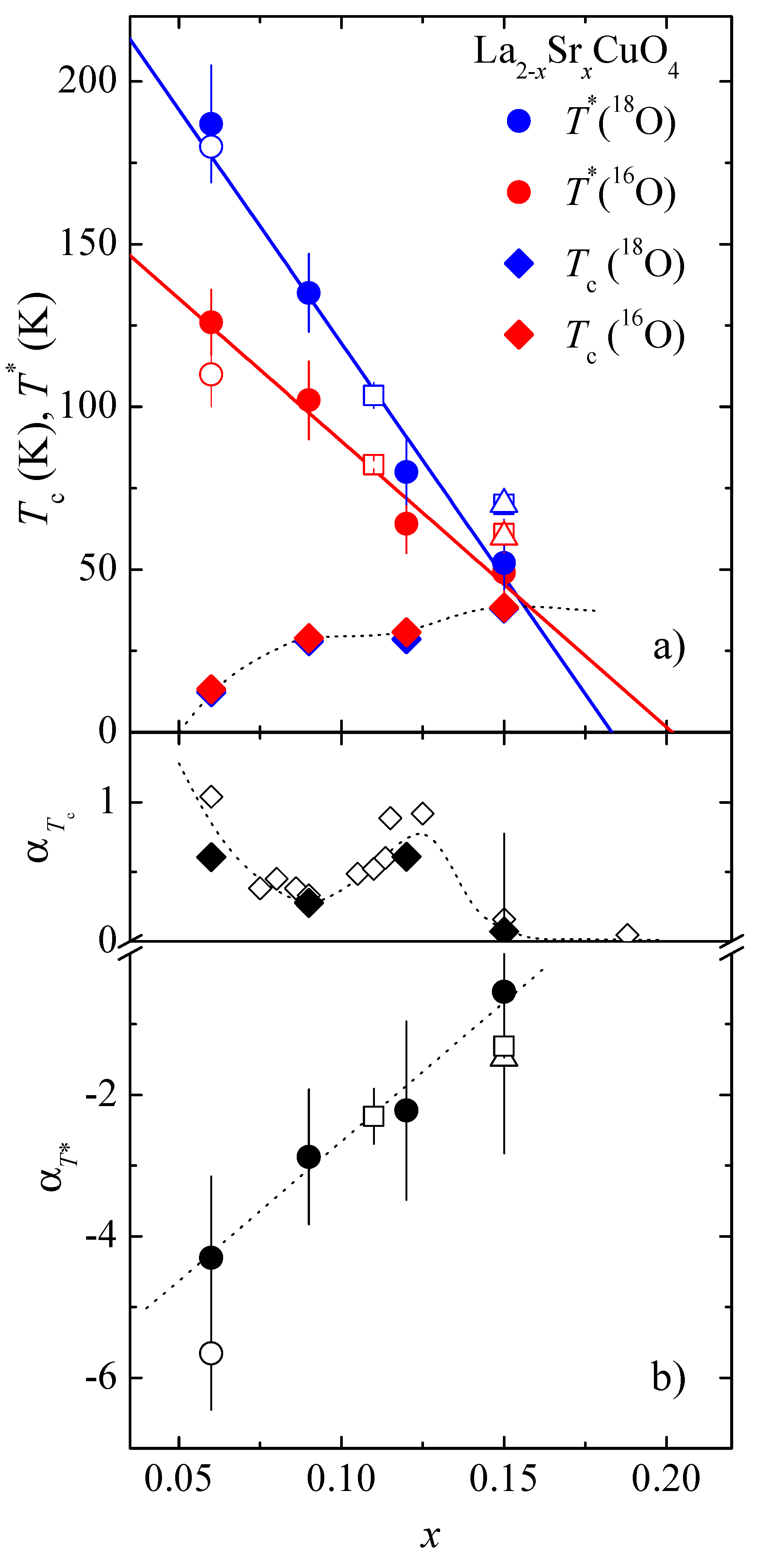
3.3. Unconventional Isotope Effects in Cuprate Superconductors
4. SrTiO3—An Exotic Low Carrier Density Superconductor
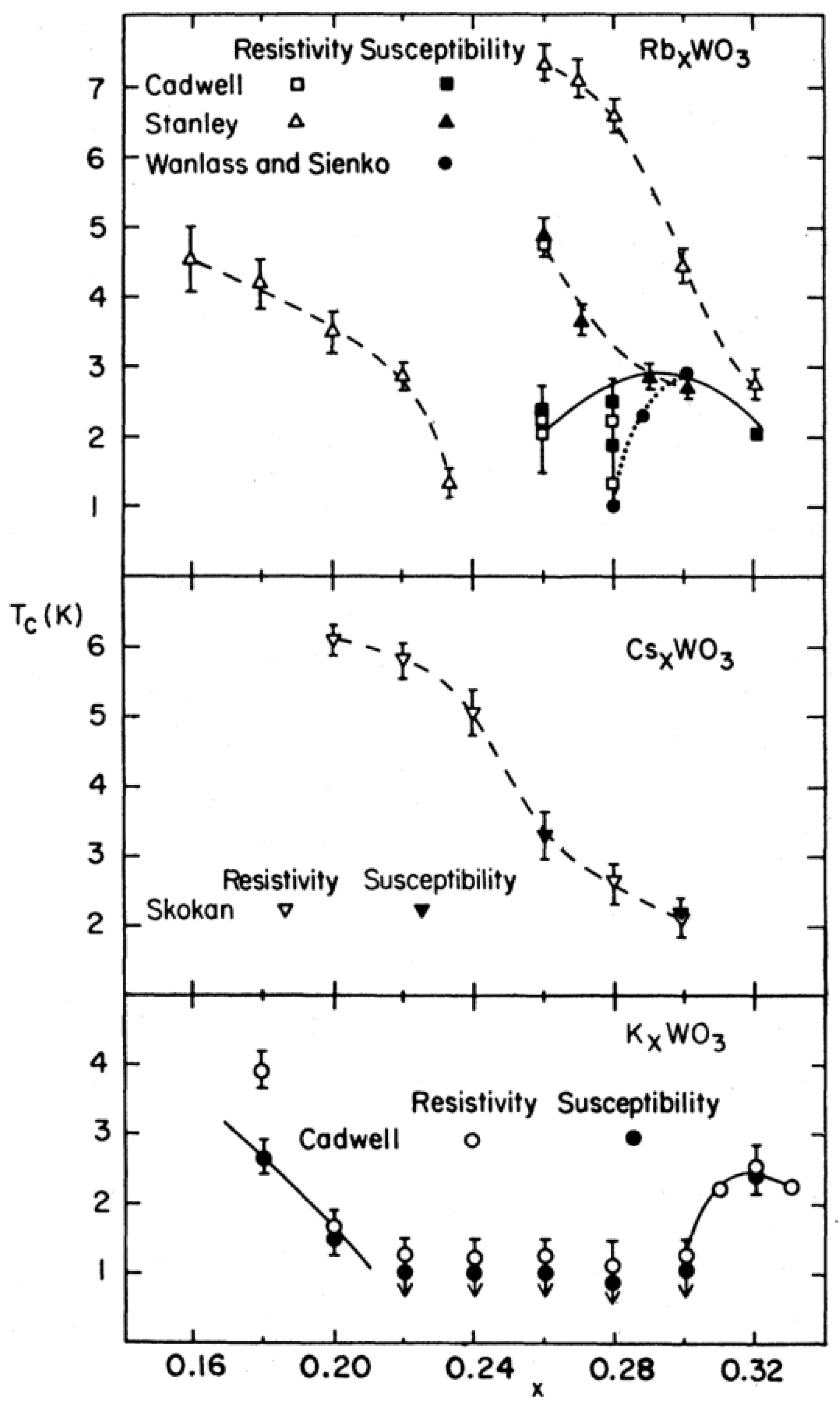
5. Another Rather Mysterious Perovskite: WO3
6. Concluding Remarks
- Thank you very much, Alex! -
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Lines, M.E.; Glass, A.M. Principles and Applications of Ferroelectrics and Related Materials; Clarendon Press: Oxford, UK, 1977. [Google Scholar]
- Cochran, W. Crystal stability and the theory of ferroelectricity. Adv. Phys. 1960, 9, 387–423. [Google Scholar] [CrossRef]
- Anderson, P.W. Concepts in Solids; Benjamin: New York, NY, USA, 1963. [Google Scholar]
- Cowley, R.A. Lattice dynamics and phase transitions of strontium titanate. Phys. Rev. 1964, 134, A981–A997. [Google Scholar] [CrossRef]
- Müller, K.A. Paramagnetic Resonance of Fe3+ in SrTiO3 single crystals. Helv. Phys. Acta 1958, 31, 173–204. [Google Scholar]
- Geller, S.; Bala, V.B. Crystallographic studies of perovskite-like compounds. II. Rare earth alluminates. Acta Cryst. 1957, 9, 1019–1025. [Google Scholar] [CrossRef]
- Müller, K.A.; Berlinger, W.; Waldner, F. Characteristic Structural Phase Transitions in Perovskite-Type Compounds. Phys. Rev. Lett. 1968, 21, 814–817. [Google Scholar] [CrossRef]
- Müller, K.A.; Kool, T.W. Properties of Perovskites and Other Oxides; World Scientific Publishing: Singapore, 2010. [Google Scholar]
- Höchli, U.; Müller, K.A. Observation of the Jahn-Teller splitting of three-valent d7 ions via Orbach relaxation. Phys. Rev. Lett. 1964, 12, 730–733. [Google Scholar] [CrossRef]
- Cochran, W.; Zia, A. Structure and dynamics of perovskite-type crystals. Phys. Stat. Sol. 1968, 25, 273–283. [Google Scholar] [CrossRef]
- Fleury, P.A.; Scott, J.F.; Worlock, J.M. Soft Phonon Modes and the 110 K Phase Transition in SrTiO3. Phys. Rev. Lett. 1968, 21, 16–19. [Google Scholar] [CrossRef]
- Müller, K.A.; Berlinger, W.; Rubins, R.S. Observation of Two Charged States of a Nickel-Oxygen Vacancy Pair in SrTiO3 by Paramagnetic Resonance. Phys. Rev. 1969, 186, 361–371. [Google Scholar] [CrossRef]
- Anderson, P.W. Model for the electronic structure of amorphous semiconductors. Phys. Rev. Lett. 1975, 34, 953–956. [Google Scholar] [CrossRef]
- Street, R.A.; Mott, N.F. States in the Gap in Glassy Semiconductors. Phys. Rev. Lett. 1975, 35, 1293–1296. [Google Scholar] [CrossRef]
- Chakraverty, B.K. Possibility of insulator to superconductor phase transition. J. Phys. Lett. 1979, 40, 99–100. [Google Scholar] [CrossRef]
- Müller, K.A.; Berlinger, W. Static critical exponents at structural phase transitions. Phys. Rev. Lett. 1971, 26, 13–16. [Google Scholar] [CrossRef]
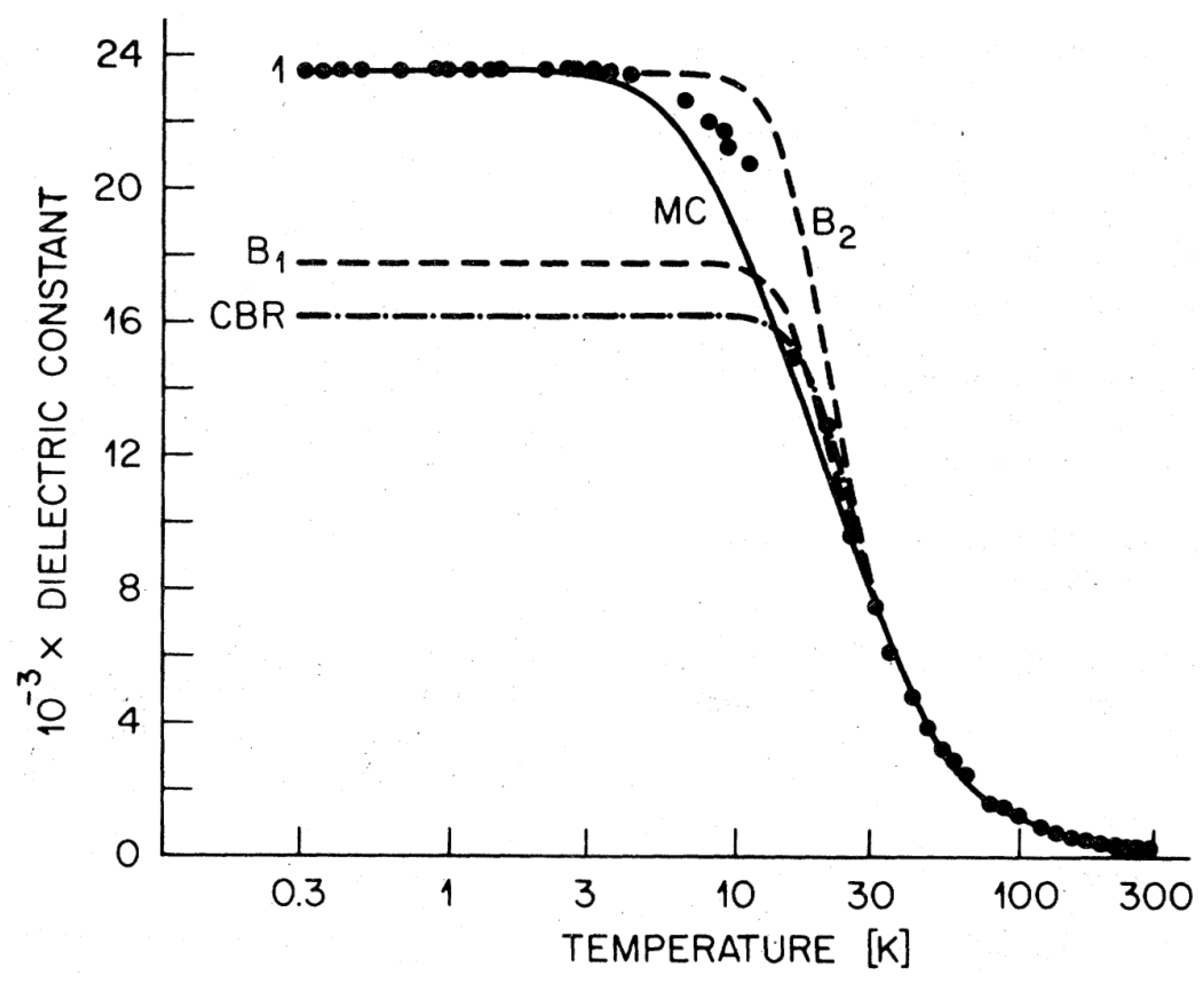
- Müller, K.A.; Burkard, H. SrTiO3: An intrinsic quantum paraelectric below 4 K. Phys. Rev. B 1979, 19, 3593–3602. [Google Scholar] [CrossRef]
- Kremer, R.K.; Bussmann-Holder, A.; Keller, H.; Haunschild, R. The Crucial Things in Science often Happen Quite Unexpectedly - Das Entscheidende in der Wissenschaft Geschieht of Ganz Unerwartet (K. Alex Müller). Condens. Matter 2020, 5, 43. [Google Scholar] [CrossRef]
- Schooley, F.; Hosler, W.R.; Cohen, M.L. Superconductivity in semiconducting SrTiO3. Phys. Rev. Lett. 1964, 12, 474–475. [Google Scholar] [CrossRef]
- Appel, J. Soft-mode superconductivity in SrTiO3-x. Phys. Rev. 1969, 180, 508–516. [Google Scholar] [CrossRef]
- Cohen, M.L. Superconductivity in many-valley semiconductors and in semimetals. Phys. Rev. 1964, 134, A511–A521. [Google Scholar] [CrossRef]
- Binnig, G.; Baratoff, A.; Hoenig, H.E.; Bednorz, J.G. Two-band superconductivity in Nb-doped SrTiO3. Phys. Rev. Lett. 1980, 45, 1352–1355. [Google Scholar] [CrossRef]
- Suhl, H.; Matthias, B.T.; Walker, L.R. Bardeen-Cooper-Schrieffer Theory of Superconductivity in the Case of Overlapping Bands. Phys. Rev. Lett. 1959, 3, 552–555. [Google Scholar] [CrossRef]
- Moskalenko, V. Superconductivity in metals with overlapped energy bands. Fiz. Metal. Metalloved. 1959, 8, 503–513. [Google Scholar]
- Gor’kov, L.P. Phonon mechanism in the most dilute superconductor n-type SrTiO3. Proc. Natl. Acad. Sci. USA 2016, 113, 4646–4651. [Google Scholar] [CrossRef]
- Thiemann, M.; Beutel, M.H.; Dressel, M.; Lee-Hone, N.R.; Broun, D.M.; Fillis-Tserakis, E.; Boschker, H.; Mannhart, J.; Scheffler, M. Single-Gap Superconductivity and Dome of Superfluid Density in Nb-Doped SrTiO3. Phys. Rev. Lett. 2018, 120, 37002. [Google Scholar] [CrossRef] [PubMed]
- Collignon, C.; Lin, X.; Rischau, C.W.; Fauqué, B.; Behnia, K. Metallicity and Superconductivity in Doped Strontium Titanate. Annual Rev. Cond. Mat. Phys. 2019, 10, 25–44. [Google Scholar] [CrossRef]
- For a recent review see: Gastiasoro, M.N.; Ruhman, J.; Fernandes, R.M. Superconductivity in dilute SrTiO3: A review. Ann. Phys. 2020, 417, 168107. [Google Scholar] [CrossRef]
- Scheerer, G.; Boselli, M.; Pulmannova, D.; Rischau, C.W.; Waelchli, A.; Gariglio, S.; Giannini, E.; van der Marel, D.; Triscone, J.M. Ferroelectricity, Superconductivity, and SrTiO3—Passions of K.A. Müller. Condens. Matter 2020, 5, 60. [Google Scholar] [CrossRef]
- Hemberger, J.; Lunkenheimer, P.; Viana, R.; Böhmer, R.; Loidl, A. Electric-field-dependent dielectric constant and nonlinear susceptibility in SrTiO3. Phys. Rev. B 1995, 52, 13159–13162. [Google Scholar] [CrossRef]
- Samara, G.A. Pressure and Temperature Dependences of the Dielectric Properties of the Perovskites BaTiO3 and SrTiO3. Phys. Rev. 1966, 151, 378–386. [Google Scholar] [CrossRef]
- Berre, B.; Fossheim, K.; Müller, K.A. Critical attenuation of the soft mode in SrTiO3. Phys. Rev. Lett. 1969, 23, 589–591. [Google Scholar] [CrossRef]
- Bussmann-Holder, A.; Bilz, H.; Bäuerle, D.; Wagner, D. A polarizability model for the ferroelectric mode in semiconducting SrTiO3. Z. Physik B 1981, 41, 353–355. [Google Scholar] [CrossRef]
- Bednorz, J.G.; Müller, K.A. Sr1-xCaxTiO3: An XY Quantum Ferroelectric with Transition to Randomness. Phys. Rev. Lett. 1984, 52, 2289–2292. [Google Scholar] [CrossRef]
- Itoh, M.; Wang, R.; Inaguma, Y.; Yamaguchi, T.; Shan, Y.-J.; Nakamura, T. Ferroelectricity Induced by Oxygen Isotope Exchange in Strontium Titanate Perovskite. Phys. Rev. Lett. 1999, 82, 3540–3543. [Google Scholar] [CrossRef]
- Bussmann-Holder, A.; Bishop, A.R. Incomplete ferroelectricity in SrTi18O3. Eur. Phys. J. B 2006, 53, 279–282. [Google Scholar] [CrossRef]
- Shigenari, T.; Abe, K. Raman Spectra of Soft Modes of SrTiO3. Ferroelectrics 2010, 369, 117–126. [Google Scholar] [CrossRef]
- Kleemann, W.; Dec, J.; Tkach, A.; Vilarinho, P.M. SrTiO3—Glimpses of an Inexhaustible Source of Novel Solid State Phenomena. Condens. Matter 2020, 5, 58. [Google Scholar] [CrossRef]
- Ravel, B.; Stern, E.A.; Verdinskii, R.I.; Kraizman, V. Local structure and the phase transitions of BaTiO3. Ferroelectrics 1998, 206–207, 407–430. [Google Scholar] [CrossRef]
- Zalar, B.; Laguta, V.V.; Blinc, R. NMR Evidence for the Coexistence of Order-Disorder and Displacive Components in Barium Titanate. Phys. Rev. Lett. 2003, 90, 037601. [Google Scholar] [CrossRef]
- Harada, J.; Axe, J.D.; Shirane, G. Neutron-Scattering Study of Soft Modes in Cubic BaTiO3. Phys. Rev. B 1971, 4, 155–162. [Google Scholar] [CrossRef]
- Völkel, G.; Müller, K.A. Order-disorder in the low-temperature phase of BaTiO3. Phys. Rev. B 2007, 76, 094105. [Google Scholar] [CrossRef]
- Bishop, A.R. A Lattice Litany for Transition Metal Oxides. Condens. Matter 2020, 5, 46. [Google Scholar] [CrossRef]
- Kool, T.W. Meetings with a Remarkable Man, Alex Müller—The Professor of SrTiO3. Condens. Matter 2020, 5, 44. [Google Scholar] [CrossRef]
- Müller, K.A. Essential Heterogeneities in Hole-Doped Cuprate Superconductors. In Superconductivity in Complex Systems; Müller, K.A., Bussman-Holder, A., Eds.; Structure and Bonding 114; Springer: Berlin/Heidelberg, Germany, 2005; pp. 1–11. [Google Scholar]
- Deutscher, G.; Fenichel, H.; Gershenson, M.; Grünbaum, E.; Ovadyahu, Z. Transition to zero dimensionality in granular aluminum superconducting films. J. Low Temp. Phys. 1973, 10, 231–243. [Google Scholar] [CrossRef]
- Deutscher, G.; Müller, K.A. Origin of superconductive glassy state and extrinsic critical currents in high-Tc oxide. Phys. Rev. Lett. 1987, 59, 1745–1748. [Google Scholar] [CrossRef] [PubMed]
- Deutscher, G. The role of the Short Coherence Length in Unconventional Superconductors. Condens. Matter 2020, 5, 77. [Google Scholar] [CrossRef]
- Bednorz, J.G.; Müller, K.A. Possible High Tc Superconductivity in the Ba-La-Cu-O Sysyem. Z. Phys. B 1986, 64, 189–193. [Google Scholar] [CrossRef]
- Bednorz, J.G.K. Alexander Müller Nobel Lecture. Available online: https://www.nobelprize.org/prizes/physics/1987/muller/lecture/ (accessed on 29 May 2020).
- Müller, K.A.; Bednorz, J.G. The Discovery of a Class of High-Temperature Superconductors. Science 1987, 237, 1133–1139. [Google Scholar] [CrossRef] [PubMed]
- Bednorz, J.G.; Müller, K.A. Perovskite-type oxides—The new approach to high-Tc superconductivity. Rev. Mod. Phys. 1988, 60, 585–600. [Google Scholar] [CrossRef]
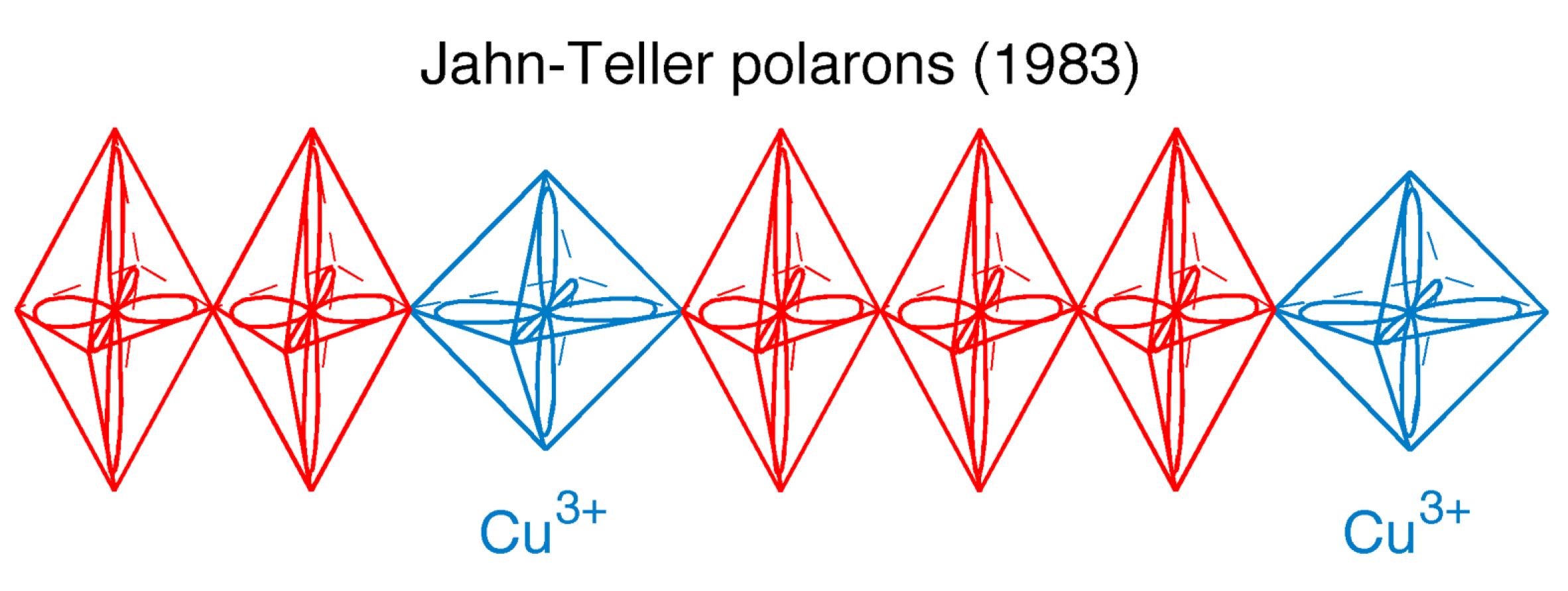
- Höck, K.-H.; Nickisch, H.; Thomas, H. Jahn-Teller effect in itinerant electron systems: The Jahn-Teller polaron. Helv. Phys. Acta 1983, 56, 237–243. [Google Scholar]
- Bianconi, A.; Saini, N.L.; Lanzara, A.; Missori, M.; Rossetti, T.; Oyanagi, H.; Yamaguchi, H.; Oka, K.; Ito, T. Determination of the Local Lattice Distortions in the CuO2 Plane of La1.85Sr0.15CuO4. Phys. Rev. Lett. 1996, 76, 3412–3415. [Google Scholar] [CrossRef]
- Müller, K.A. On the superconductivity in hole doped cuprates. J. Phys. Condens. Matter 2007, 19, 251002. [Google Scholar] [CrossRef]
- Müller, K.A. The Unique Properties of Superconductivity in Cuprates. J. Supercond. Nov. Magn. 2014, 27, 2163–2179. [Google Scholar] [CrossRef]
- Müller, K.A. The Polaronic Basis for High-Temperature Superconductivity. J. Supercond. Nov. Magn. 2017, 30, 3007–3018. [Google Scholar] [CrossRef]
- Müller, K.A. Possible coexistence of s- and d-wave condensates in copper oxide superconductors. Nature 1995, 377, 133–135. [Google Scholar] [CrossRef]
- Müller, K.A.; Keller, H. s and d Wave Symmetry Components in High-Temperature Cuprate Superconductors. In High-Tc Superconductivity 1996: Ten Years after the Discovery; Kaldis, E., Liarokapis, E., Müller, K.A., Eds.; Kluwer Academic Publishers: Dordrecht, The Netherlands, 1997; pp. 7–29. [Google Scholar]
- Wollman, D.A.; Van Harlingen, D.J.; Lee, W.C.; Ginsberg, D.M.; Leggett, A.J. Experimental determination of the superconducting pairing state in YBCO from the phase coherence of YBCO-Pb dc SQUIDs. Phys. Rev. Lett. 1993, 71, 2134–2137. [Google Scholar] [CrossRef]
- Tsuei, C.C.; Kirtley, J.R.; Chi, C.C.; Yu-Jahnes, L.S.; Gupta, A.; Shaw, T.; Sun, J.Z.; Ketchen, M.B. Pairing Symmetry and Flux Quantization in a Tricrystal Superconducting Ring of YBa2Cu3O7-δ. Phys. Rev. Lett. 1994, 73, 593–596. [Google Scholar] [CrossRef]
- Brawner, D.A.; Ott, H.R. Evidence for an unconventional superconducting order parameter in YBa2Cu3O6.9. Phys. Rev. B 1994, 50, 6530(R)–6533(R). [Google Scholar] [CrossRef]
- Khasanov, R.; Shengelaya, A.; Maisuradze, A.; La Mattina, F.; Bussmann-Holder, A.; Keller, H.; Müller, K.A. Experimental evidence for two gaps in the high-temperature La1.83Sr0.17CuO4 superconductor. Phys. Rev. Lett. 2007, 98, 057007. [Google Scholar] [CrossRef]
- Khasanov, R.; Strässle, S.; Di Castro, D.; Masui, T.; Miyasaka, S.; Tajima, S.; Bussmann-Holder, A.; Keller, H. Multiple gap symmetries for the order parameter of cuprate superconductors from penetration depth measurements. Phys. Rev. Lett. 2007, 99, 237601. [Google Scholar] [CrossRef]
- Khasanov, R.; Shengelaya, A.; Karpinski, J.; Bussmann-Holder, A.; Keller, H.; Müller, K.A. s-wave symmetry along the c-axis and s+d in-plane superconductivity in bulk YBa2Cu4O8. J. Supercond. Nov. Magn. 2008, 21, 81–85. [Google Scholar] [CrossRef]
- Bussmann-Holder, A.; Khasanov, R.; Shengelaya, A.; Maisuradze, A.; La Mattina, F.; Keller, H.; Müller, K.A. Mixed order parameter symmetries in cuprate superconductors. Europhys. Lett. 2007, 77, 27002. [Google Scholar] [CrossRef][Green Version]
- Keller, H.; Bussmann-Holder, A.; Müller, K.A. Jahn-Teller physics and high-Tc superconductivity. Mater. Today 2008, 11, 38–46. [Google Scholar] [CrossRef]
- Zimmermann, P.; Keller, H.; Lee, S.L.; Savić, I.M.; Warden, M.; Zech, D.; Cubitt, R.; Forgan, E.M.; Kaldis, E.; Karpinski, J.; et al. Muon-spin rotation studies of the temperature dependence of the magnetic penetration depth in the YBa2Cu3Ox family and related compounds. Phys. Rev. B 1995, 52, 541–552. [Google Scholar] [CrossRef] [PubMed]
- Müller, K.A. On the macroscopic s- and d-wave symmetry in cuprate superconductors. Philos. Mag. Lett. 2002, 82, 279–288. [Google Scholar] [CrossRef]
- Iachello, F. A model of cuprate superconductors based on the analogy with atomic nuclei. Philos. Mag. Lett. 2002, 82, 289–295. [Google Scholar] [CrossRef]
- Khasanov, R.; Shengelaya, A.; Brütsch, R.; Keller, H. Suppression of the s-wave Order Parameter Near the Surface of the Infinite-Layer Electron-Doped Cuprate Superconductor Sr0.9La0.1Cu2. Condens. Matter 2020, 5, 50. [Google Scholar] [CrossRef]
- Batlogg, B.; Cava, R.J.; Jayaraman, A.; van Dover, R.B.; Kourouklis, G.A.; Sunshine, S.; Murphy, D.W.; Rupp, L.W.; Chen, H.S.; White, A.; et al. Isotope Effect in the High-Tc Superconductors Ba2YCu3O7 and Ba2EuCu3O7. Phys. Rev. Lett. 1987, 58, 2333–2336. [Google Scholar] [CrossRef]
- Franck, J.P.; Jung, J.; Mohamed, A.K.; Gygax, S.; Sproule, G.I. Observation of an oxygen isotope effect in superconducting (Y1-xPrx)Ba2Cu3O7-δ. Phys. Rev. B 1991, 44, 5318–5321. [Google Scholar] [CrossRef] [PubMed]
- Franck, J.P. Experimental studies of the isotope effect in high temperature superconductors. In Physical Properties of High Temperature Superconductors IV; Ginsberg, D.M., Ed.; World Scientific: Singapore, 1994; pp. 189–293. [Google Scholar]
- Müller, K.A. On the oxygen isotope effect and apex anharmonicity in high-Tc cuprates. Z. Phys. B Condens. Matter 1990, 80, 193–201. [Google Scholar] [CrossRef]
- Zhao, G.M.; Conder, K.; Keller, H.; Müller, K.A. Oxygen isotope effects in La2-xSrxCuO4: Evidence for polaronic charge carriers and their condensation. J. Phys. Condens. Matter 1998, 10, 9055–9066. [Google Scholar] [CrossRef]
- Zhao, G.M.; Keller, H.; Conder, K. Unconventional isotope effects in the high-temperature cuprate superconductors. J. Phys. Condens. Matter 2001, 13, R569–R587. [Google Scholar] [CrossRef]
- Keller, H. Unconventional Isotope Effects in Cuprate Superconductors. In Superconductivity in Complex Systems; Müller, K.A., Bussmann-Holder, A., Eds.; Springer: Berlin/Heidelberg, Germany, 2005; pp. 143–169. [Google Scholar]
- Conder, K.; Furrer, A.; Pomjakushina, E. A Retrospective of Materials Synthesis at the Paul Scherrer Institut (PSI). Condens. Matter 2020, 5, 55. [Google Scholar] [CrossRef]
- Zech, D.; Keller, H.; Conder, K.; Kaldis, E.; Liarokapis, E.; Poulakis, N.; Müller, K.A. Site-selective oxygen isotope effect in optimally doped YBa2Cu3O6+x. Nature 1994, 371, 681–683. [Google Scholar] [CrossRef]
- Zhao, G.; Ager, J.W., III; Morris, D.E. Site dependence of large oxygen isotope effect in Y0.7Pr0.3Ba2Cu3O6.97. Phys. Rev. B 1996, 54, 14982–14985. [Google Scholar] [CrossRef] [PubMed]
- Khasanov, R.; Shengelaya, A.; Morenzoni, E.; Angst, M.; Conder, K.; Savić, I.M.; Lampakis, D.; Liarokapis, E.; Tatsi, A.; Keller, H. Site-selective oxygen isotope effect on the magnetic field penetration depth in underdoped Y0.6Pr0.4Ba2Cu3O7-δ. Phys. Rev. B 2003, 68, 220506(R). [Google Scholar] [CrossRef]
- Bussmann-Holder, A.; Genzel, L.; Bishop, A.R.; Simon, A. The role of apical oxygen in superconducting cuprates. Philos. Mag. B 1997, 75, 463–469. [Google Scholar] [CrossRef]
- Khasanov, R.; Shengelaya, A.; Di Castro, D.; Morenzoni, E.; Maisuradze, A.; Savić, I.M.; Conder, K.; Pomjakushina, E.; Bussmann-Holder, A.; Keller, H. Oxygen isotope effect on the superconducting transition and magnetic states within the phase diagram of Y1-xPrxBa2Cu3O7-δ. Phys. Rev. Lett. 2008, 101, 077001. [Google Scholar] [CrossRef] [PubMed]
- Guguchia, Z.; Khasanov, R.; Bendele, M.; Pomjakushina, E.; Conder, K.; Shengelaya, A.; Keller, H. Negative Oxygen Isotope Effect on the Static Spin Stripe Order in Superconducting La2-xBaxCuO4 (x=1/8) Observed by Muon-Spin Rotation. Phys. Rev. Lett. 2014, 113, 057002. [Google Scholar] [CrossRef]
- Alexandrov, A.S.; Mott, N.F. Spin and charge bipolaron kinetics of high Tc superconductors. Int. J. Mod. Phys. B 1994, 8, 2075–2109. [Google Scholar] [CrossRef]
- Zhao, G.; Morris, D.E. Observation of a possible oxygen isotope effect on the effective mass of carriers in YBa2Cu3O6.94. Phys. Rev. B 1995, 51, 16487–16490. [Google Scholar] [CrossRef]
- Zhao, G.M.; Hunt, M.B.; Keller, H.; Müller, K.A. Evidence for polaronic supercarriers in the copper oxide superconductors La2-xSrxCuO4. Nature 1997, 385, 236–239. [Google Scholar] [CrossRef]
- Hofer, J.; Conder, K.; Sasagawa, T.; Zhao, G.M.; Willemin, M.; Keller, H.; Kishio, K. Oxygen-isotope effect on the in-plane penetration depth in underdoped La2-xSrxCuO4 single crystals. Phys. Rev. Lett. 2000, 84, 4192–4195. [Google Scholar] [CrossRef] [PubMed]
- Khasonov, R.; Shengelaya, A.; Conder, K.; Morenzoni, E.; Savić, I.M.; Keller, H. The oxygen-isotope effect on the in-plane penetration depth in underdoped Y1-xPrxBa2Cu3O7-δ as revealed by muon-spin rotation. J. Phys. Condens. Matter 2003, 15, L17–L23. [Google Scholar] [CrossRef]
- Jackson, T.J.; Riseman, T.M.; Forgan, E.M.; Glückler, H.; Prokscha, T.; Morenzoni, E.; Pleines, M.; Niedermayer, C.; Schatz, G.; Luetkens, H.; et al. Depth-Resolved Profile of the Magnetic Field beneath the Surface of a Superconductor with a Few nm Resolution. Phys. Rev. Lett. 2000, 84, 4958–4961. [Google Scholar] [CrossRef] [PubMed]
- Khasanov, R.; Eshchenko, D.G.; Luetkens, H.; Morenzoni, E.; Prokscha, T.; Suter, A.; Garifinov, N.; Mali, M.; Roos, J.; Conder, K.; et al. Direct observation of the oxygen isotope effect on the in-plane magnetic field penetration depth in optimally doped YBa2Cu3O7-δ. Phys. Rev. Lett. 2004, 92, 057602. [Google Scholar] [CrossRef] [PubMed]
- Uemura, Y.J.; Luke, G.M.; Sternlieb, B.J.; Brewer, J.H.; Carolan, J.F.; Hardy, W.N.; Kadono, R.; Kempton, J.R.; Kiefl, R.F.; Kreitzman, S.R.; et al. Universal Correlations between Tc and ns/m* (Carrier Density over Effective Mass) in High-Tc Cuprate Superconductors. Phys. Rev. Lett. 1989, 62, 2317–2320. [Google Scholar] [CrossRef]
- Uemura, Y.J.; Le, L.P.; Luke, G.M.; Sternlieb, B.J.; Wu, W.D.; Brewer, J.H.; Riseman, T.M.; Seaman, C.L.; Maple, M.B.; Ishikawa, M.; et al. Basic similarities among cuprate, bismuthate, organic, Chevrel-phase, and heavy-fermion superconductors shown by penetration-depth measurements. Phy. Rev. Lett. 1991, 66, 2665–2668. [Google Scholar] [CrossRef]
- Weyeneth, S.; Müller, K.A. Oxygen Isotope Effect in Cuprates Results from Polaron-induced Superconductivity. J. Supercond. Nov. Magn. 2011, 24, 1235–1239. [Google Scholar] [CrossRef][Green Version]
- Kresin, V.Z.; Wolf, S.A. Microscopic model for the isotope effect in high-Tc oxides. Phys. Rev. B 1994, 49, 3652–3654. [Google Scholar] [CrossRef]
- Bill, A.; Kresin, V.Z.; Wolf, S.A. Isotope effect for the penetration depth in superconductors. Phys. Rev. B 1998, 57, 10814–10824. [Google Scholar] [CrossRef]
- Schneider, T.; Keller, H. Universal trends in extreme type-II superconductors. Phys. Rev. Lett. 1992, 69, 3374–3377. [Google Scholar] [CrossRef]
- Bendele, M.; von Rohr, F.; Gugchia, Z.; Pomjakushina, E.; Conder, K.; Bianconi, A.; Simon, A.; Bussmann-Holder, A.; Keller, H. Evidence for strong lattice effects as revealed from huge unconventional oxygen isotope effects on the pseudogap temperature in La2-xSrxCuO4. Phys. Rev. B 2017, 95, 014514. [Google Scholar] [CrossRef]
- Lanzara, A.; Zhao, G.; Saini, N.L.; Bianconi, A.; Conder, K.; Keller, H.; Müller, K.A. Oxygen-isotope effect of the charge-stripe ordering temperature in La2-xSrxCuO4 from X-ray absorption spectroscopy. J. Phys. Condens. Matter 1999, 11, L541–L546. [Google Scholar] [CrossRef]
- Rubio Temprano, D.; Mesot, J.; Janssen, S.; Conder, K.; Furrer, A.; Mutka, H.; Müller, K.A. Large Isotope Effect on the Pseudogap in the High-Temperature Superconductor HoBa2Cu4O8. Phys. Rev. Lett. 2000, 84, 1990–1993. [Google Scholar] [CrossRef] [PubMed]
- Rubio Temprano, D.; Furrer, A.; Conder, K.; Mutka, H. A neutron crystal-field study of the pseudogap in the underdoped high Tc superconductor HoBa2Cu418O8. Physica B 2000, 276–278, 762–763. [Google Scholar] [CrossRef]
- Rubio Temprano, D.; Mesot, J.; Janssen, S.; Conder, K.; Furrer, A.; Sokolov, A.; Trounov, V.; Kazakov, S.M.; Karpinski, J.; Müller, K.A. Large copper isotope effect on the pseudogap in the high-temperature superconductor HoBa2Cu4O8. Eur. Phys. J. B 2001, 19, 5–8. [Google Scholar] [CrossRef]
- Rubio Temprano, D.; Conder, K.; Furrer, A.; Mutka, H.; Trounov, V.; Müller, K.A. Oxygen and copper isotope effects on the pseudogap in the high-temperature superconductor La1.81Ho0.04Sr0.15CuO4 studied by neutron crystal-field spectroscopy. Phys. Rev. B 2002, 66, 184506. [Google Scholar] [CrossRef]
- Häfliger, P.S.; Podlesnyak, A.; Conder, K.; Pomjakushina, E.; Furrer, A. Pseudogap of the high-temperature superconductor La1.96-xSrxHo0.04CuO4 as observed by neutron crystal-field spectroscopy. Phys. Rev. B 2006, 74, 184520. [Google Scholar] [CrossRef]
- Varma, C.M. Non-Fermi-liquid states and pairing instability of a general model of copper oxide metals. Phys. Rev. B 1997, 55, 14554–14580. [Google Scholar] [CrossRef]
- Li, Y.; Balédent, V.; Barisić, N.; Cho, Y.; Fauqué, B.; Sidis, Y.; Yu, G.; Zhao, X.; Bourges, P.; Greven, M. Unusual magnetic order in the pseudogap region of the superconductor HgBa2CuO4+δ. Nature 2008, 455, 372–375. [Google Scholar] [CrossRef]
- Bussmann-Holder, A.; Keller, H. Polaron Effects in High-Temperature Cuprate Superconductors. In Polarons in Advanced Materials; Alexandrov, S.A., Ed.; Springer: Dordrecht, The Netherlands, 2007; pp. 599–621. [Google Scholar]
- Zhang, C.J.; Oyanagi, H. Local lattice instability and superconductivity in La1.85Sr0.15Cu1-xMxO4 (M=Mn, Ni, and Co). Phys. Rev. B 2009, 79, 064521. [Google Scholar] [CrossRef]
- Bussmann-Holder, A.; Simon, A.; Keller, H.; Bishop, A.R. Polaron signatures in the phonon dispersion of high-temperature superconducting copper oxides. Eur. Phys. Lett. 2013, 101, 47004. [Google Scholar] [CrossRef]
- Ohtomo, A.; Hwang, H.Y. A high-mobility electron gas at the LaAlO3/SrTiO3 heterointerface. Nature 2004, 427, 423–426. [Google Scholar] [CrossRef] [PubMed]
- Reyren, N.; Thiel, S.; Caviglia, A.D.; Hammerl, G.; Richter, C.; Schneider, C.W.; Kopp, T.; Rüetschi, A.-S.; Jaccard, D.; Gabay, M.; et al. Superconducting interfaces between insulating oxides. Science 2007, 317, 1196–1199. [Google Scholar] [CrossRef] [PubMed]
- Brinkman, A.; Huijben, M.; van Zalk, M.; Huijben, J.; Zeitler, U.; Maan, J.C.; van der Wiel, W.; Rijnders, D.; Blank, D.H.A.; Hilgenkamp, H. Magnetic effects at the interface between non-magnetic oxides. Nat. Mater. 2007, 6, 493–496. [Google Scholar] [CrossRef] [PubMed]
- Mannhart, J.; Schlom, D.G. Oxide interfaces—An opportunity for electronics. Science 2010, 327, 1607–1611. [Google Scholar] [CrossRef]
- Zubko, P.; Gariglio, S.; Gabay, M.; Ghosez, P.; Triscone, J.-M. Interface physics in complex oxide heterostructures. Ann. Rev. Cond. Mat. Phys. 2011, 2, 141–156. [Google Scholar] [CrossRef]
- Hwang, H.Y.; Iwasa, Y.; Kawasaki, M.; Keimer, B.; Nagaosa, N.; Tokura, Y. Emergent phenomena at oxide interfaces. Nat. Mater. 2012, 11, 103–113. [Google Scholar] [CrossRef]
- Lin, X.; Zhu, Z.; Fauqué, B.; Behnia, K. Fermi surface of the most dilute superconductor. Phys. Rev. X 2013, 3, 021002. [Google Scholar] [CrossRef]
- Edge, J.M.; Kedem, Y.; Aschauer, U.; Spaldin, N.A.; Balatsky, A.V. Quantum critical origin of the superconducting dome in SrTiO3. Phys. Rev. Lett. 2015, 115, 247002. [Google Scholar] [CrossRef]
- Bussmann-Holder, A.; Beige, H.; Völkel, G. Precursor effects, broken local symmetry, and coexistence of order-disorder and displacive dynamics in perovskite ferroelectrics. Phys. Rev. B 2009, 79, 18411. [Google Scholar] [CrossRef]
- Egami, T.; Billinge, S.J.L. Underneath the Bragg Peaks. In Structural Analysis of Complex Materials; Pergamon Press: New York, NY, USA, 2012. [Google Scholar]
- Sato, K.; Miyanaga, T.; Ikeda, S.; Diop, D. XAFS Study of Local Structure Change in Perovskite Titanates. Physica Scripta 2005, T115, 359–361. [Google Scholar] [CrossRef]
- Hou, D.; Zhao, C.; Paterson, A.R.; Li, S.; Jones, J.L. Local structures of perovskite dielectrics and ferroelectrics via pair distribution function analyses. J. Eur. Ceramic Soc. 2017, 38, 971–987. [Google Scholar] [CrossRef]
- Bilz, H.; Benedek, G.; Bussmann-Holder, A. Theory of ferroelectricity: The polarizability model. Phys. Rev. B 1987, 35, 4840–4848. [Google Scholar] [CrossRef] [PubMed]
- Bussmann-Holder, A.; Büttner, H. Ferroelectricity in oxides. Nature 1992, 360, 541. [Google Scholar] [CrossRef]
- Bussmann-Holder, A. The polarizability model for ferroelectricity in perovskite oxides. J. Phys. Condens. Matter 2012, 24, 273202. [Google Scholar] [CrossRef]
- Bussmann-Holder, A.; Roleder, K.; Ko, J.-H. What makes the difference in perovskite titanates? J. Phys. Chem. Solids 2018, 117, 148–157. [Google Scholar] [CrossRef]
- Bussmann-Holder, A.; Keller, H.; Simon, A.; Bihlmayer, G.; Roleder, K.; Szot, K. Unconventional Co-Existence of Insulating Nano-Regions and Conducting Filaments in Reduced SrTiO3: Mode Softening, Local Piezoelectricity, and Metallicity. Crystals 2020, 10, 437. [Google Scholar] [CrossRef]
- Calvani, P.; Capizzi, M.; Donato, F.; Lupi, S.; Maselli, P.; Peschiaroli, D. Observation of a midinfrared band in SrTiO3-y. Phys. Rev. B 1993, 47, 8917–8922. [Google Scholar] [CrossRef]
- Waser, R.; Dittmann, R.; Staikov, G.; Szot, K. Redox-Based Resistive Switching Memories—Nanoionic Mechanisms, Prospects, and Challenges. Adv. Mater. 2009, 21, 2632–2663. [Google Scholar] [CrossRef]
- Wrana, D.; Rodenbücher, C.; Bełza, W.; Szot, K.; Krok, F. In situ study of redox processes on the surface of SrTiO3 single crystals. Appl. Surf. Sci. 2018, 432, 46–52. [Google Scholar] [CrossRef]
- Rodenbücher, C.; Menzel, S.; Wrana, D.; Gensch, T.; Korte, C.; Krok, F.; Szot, K. Current channeling along extended defects during electroreduction of SrTiO3. arXiv 2019, arXiv:1910.02748. [Google Scholar] [CrossRef] [PubMed]
- Szot, K.; Speier, W.; Bihlmayer, G.; Waser, R. Switching the electrical resistance of individual dislocations in single-crystalline SrTiO3. Nat. Mater. 2006, 5, 312–320. [Google Scholar] [CrossRef] [PubMed]
- Gao, P.; Yang, S.; Ishikawa, R.; Li, N.; Feng, B.; Kumamoto, A.; Shibata, N.; Yu, P.; Ikuhara, Y. Atomic-Scale Measurement of Flexoelectric Polarization at SrTiO3 Dislocations. Phys. Rev. Lett. 2018, 120, 267601. [Google Scholar] [CrossRef] [PubMed]
- Bussmann-Holder, A.; Keller, H.; Simon, A.; Bianconi, A. Multi-Band Superconductivity and the Steep Band/Flat Band Scenario. Condens. Matter 2019, 4, 91. [Google Scholar] [CrossRef]
- Cadwell, L.H.; Morris, R.C.; Moulton, W.G. Normal and superconducting properties of KxWO3. Phys. Rev. B 1981, 23, 2219–2223. [Google Scholar] [CrossRef]
- Wiseman, P.J.; Dickens, P.G. Neutron diffraction studies of cubic tungsten bronzes. J. Solid State Chem. 1976, 17, 91–100. [Google Scholar] [CrossRef]
- Brusetti, R.; Bordet, P.; Bossy, J.; Schober, H.; Eibl, S. Superconductivity in the tungsten bronze RbxWO3 (0.20 ≤ x ≤ 0.33) in connection with its structure, electronic density of states, and phonon density of states. Phys. Rev. B 2007, 76, 174511. [Google Scholar] [CrossRef]
- Lee, K.S.; Seo, D.K.; Whangbo, M.H. Electronic Band Structure Study of the Anomalous Electrical and Superconducting Properties of Hexagonal Alkali Tungsten Bronzes AxWO3 (A = K, Rb, Cs). J. Am. Chem. Soc. 1997, 119, 4043–4049. [Google Scholar] [CrossRef]
- Bousquet, E.; Hamdi, H.; Aguado-Puente, P.; Salje, E.K.H.; Artacho, E.; Ghosez, P. First-principles characterization of single-electron polaron in WO3. Phys. Rev. Res. 2020, 2, 012052. [Google Scholar] [CrossRef]
- Schirmer, O.F.; Salje, E. Conducting bi-polarons in low-temperature crystalline WO3-x. J. Phys. C 1980, 13, 1067–1072. [Google Scholar] [CrossRef]
- Schirmer, O.F.; Salje, E. The W5+ polaron in crystalline low temperature WO3 ESR and optical absorption. Solid State Commun. 1980, 33, 333–336. [Google Scholar] [CrossRef]
- Reich, S.; Tsabba, Y. Possible nucleation of a 2D superconducting phase on WO3 single crystals surface doped with Na+. Eur. Phys. J. B 1999, 1, 1–4. [Google Scholar] [CrossRef]
- Shengelaya, A.; Reich, S.; Tsabba, Y.; Müller, K.A. Electron spin resonance and magnetic susceptibility suggest superconductivity in Na doped WO3 samples. Eur. Phys. J. B 1999, 12, 13–15. [Google Scholar] [CrossRef]
- Shengelaya, A.; Conder, K.; Müller, K.A. Signatures of filamentary superconductivity up to 94 K in tungsten oxide WO2.9. J. Supercond. Nov. Magn. 2020, 33, 301–306. [Google Scholar] [CrossRef]
- Shengelaya, A.; Mattina, F.L.; Conder, K. Unconventional Transport Properties of Reduced Tungsten Oxide WO2.9. Condens. Matter 2020, 5, 63. [Google Scholar] [CrossRef]
- Salje, E.K.H. Polaronic States and Superconductivity in WO3-x. Condens. Matter 2020, 5, 32. [Google Scholar] [CrossRef]
- Kochelaev, B.I.; Safina, A.M.; Shengelaya, A.; Keller, H.; Müller, K.A.; Conder, K. Three-Spin-Polarons and Their Elastic Interaction in Cuprates. Mod. Phys. Lett. B 2003, 17, 415–421. [Google Scholar] [CrossRef]

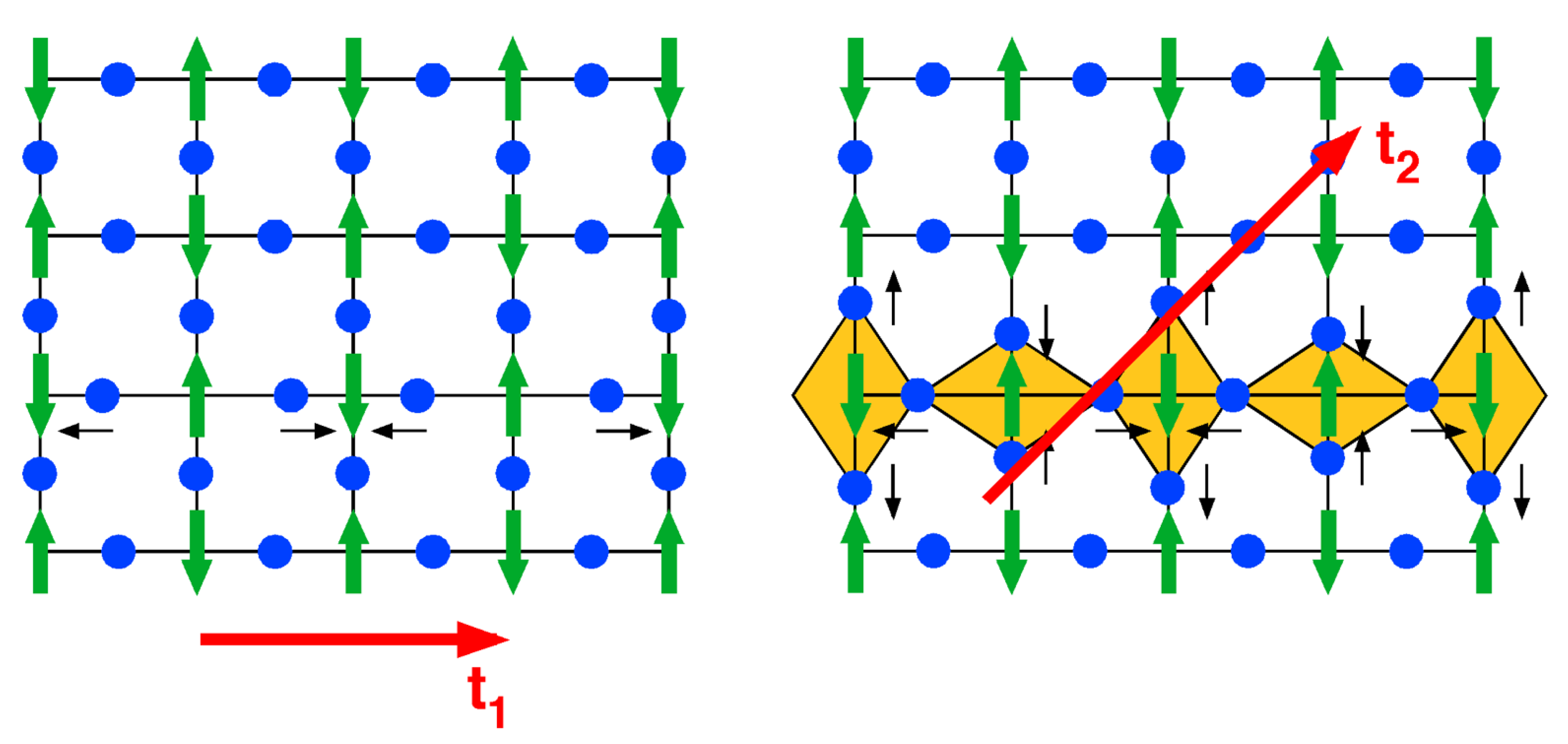

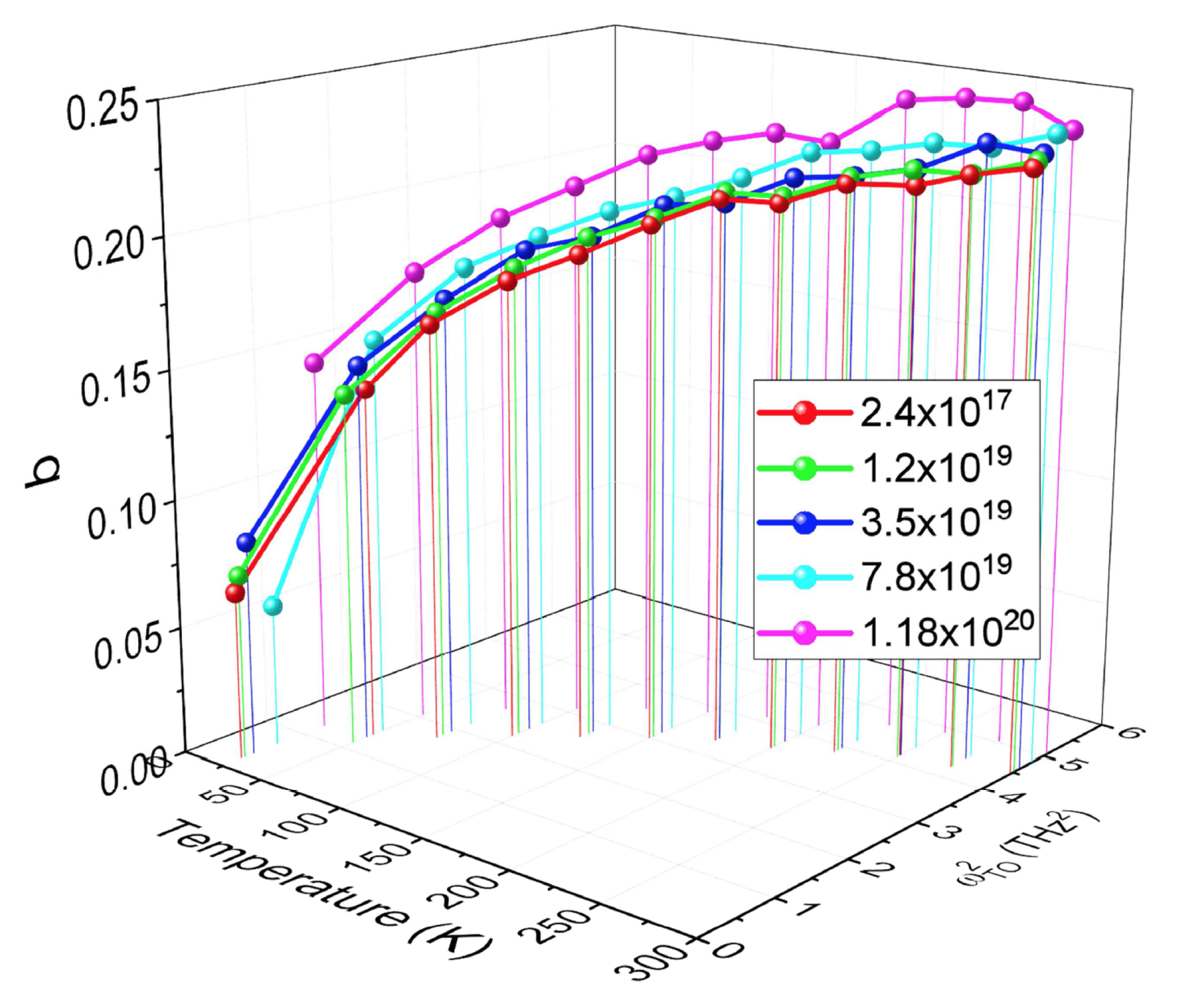
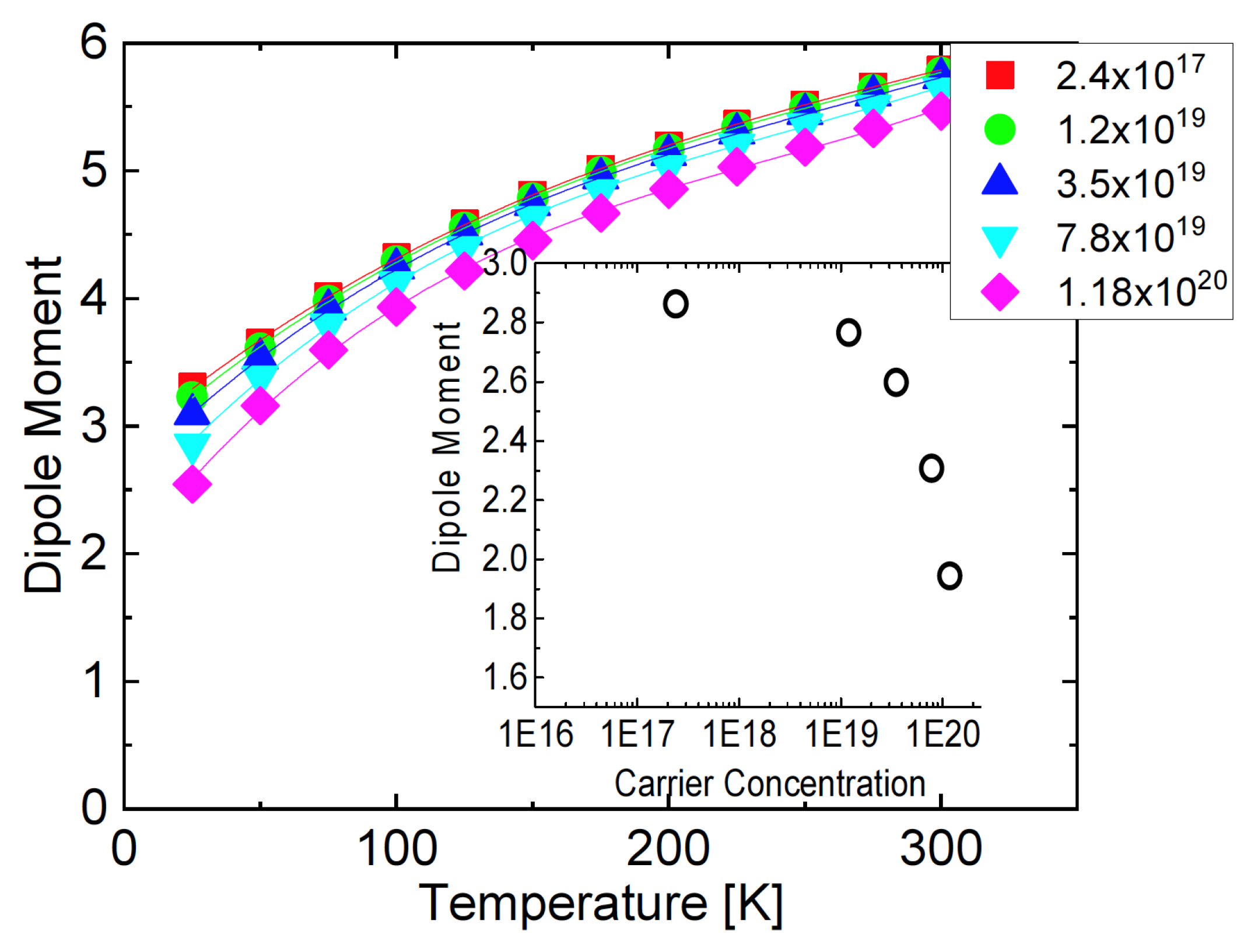
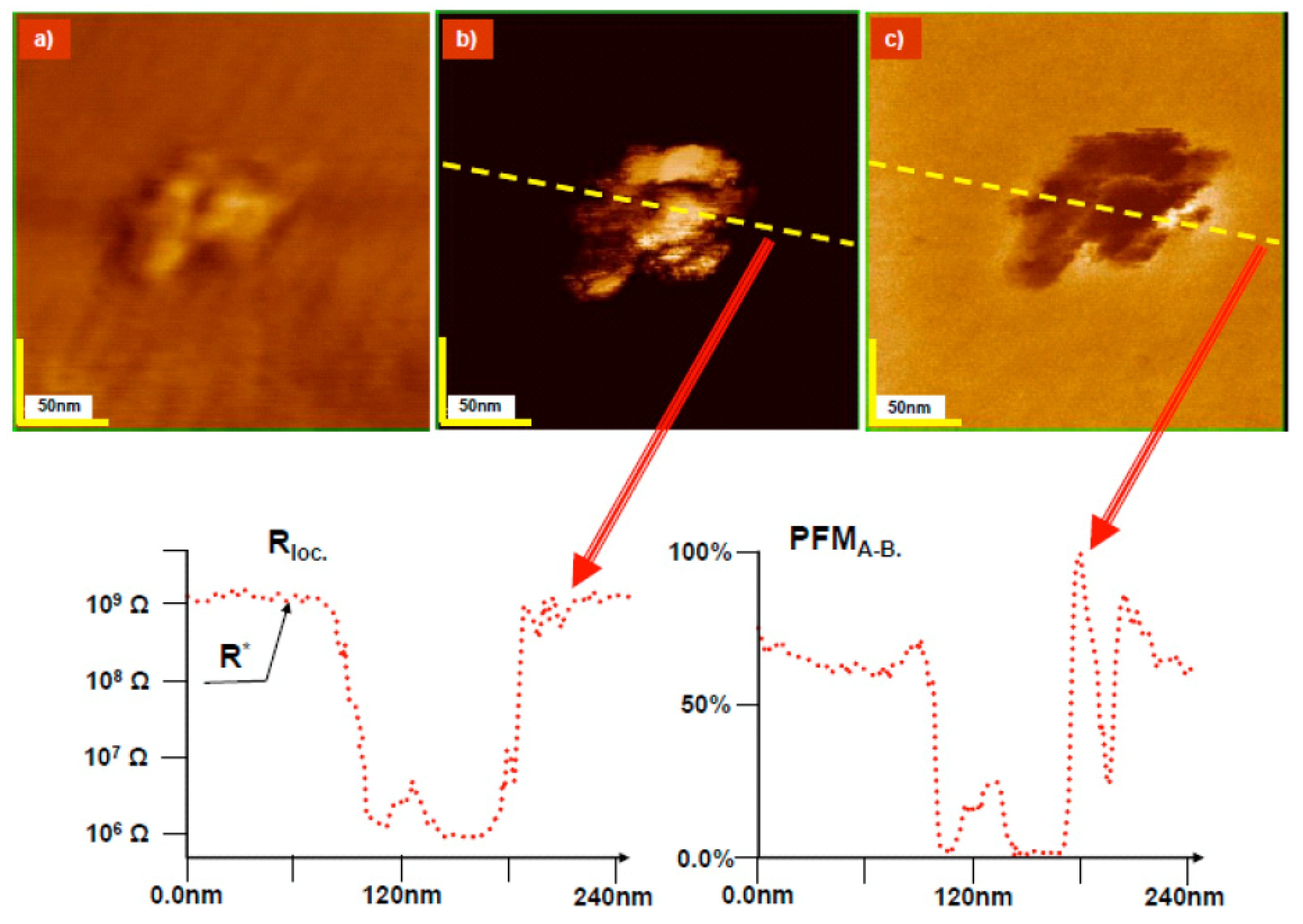


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bussmann-Holder, A.; Keller, H. From SrTiO3 to Cuprates and Back to SrTiO3: A Way Along Alex Müller’s Scientific Career. Condens. Matter 2021, 6, 2. https://doi.org/10.3390/condmat6010002
Bussmann-Holder A, Keller H. From SrTiO3 to Cuprates and Back to SrTiO3: A Way Along Alex Müller’s Scientific Career. Condensed Matter. 2021; 6(1):2. https://doi.org/10.3390/condmat6010002
Chicago/Turabian StyleBussmann-Holder, Annette, and Hugo Keller. 2021. "From SrTiO3 to Cuprates and Back to SrTiO3: A Way Along Alex Müller’s Scientific Career" Condensed Matter 6, no. 1: 2. https://doi.org/10.3390/condmat6010002
APA StyleBussmann-Holder, A., & Keller, H. (2021). From SrTiO3 to Cuprates and Back to SrTiO3: A Way Along Alex Müller’s Scientific Career. Condensed Matter, 6(1), 2. https://doi.org/10.3390/condmat6010002





