The Ancient Romans’ Route to Charge Density Waves in Cuprates
Abstract
:1. Introduction
2. The Electron Phase Separation Era
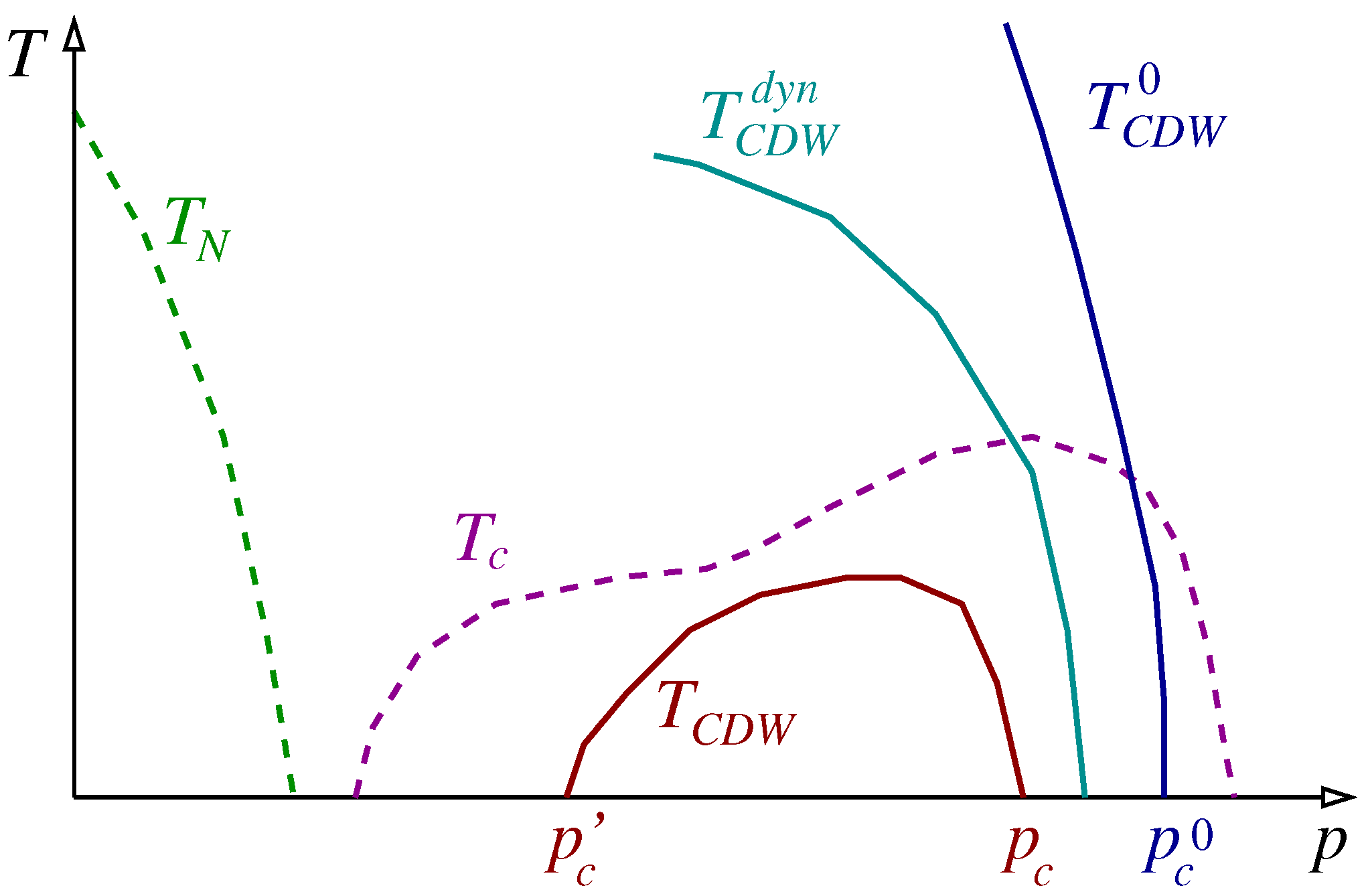
3. The Charge Density Wave Era
4. Looking for Fingerprints: Angle Resolved Photoemission Spectroscopy
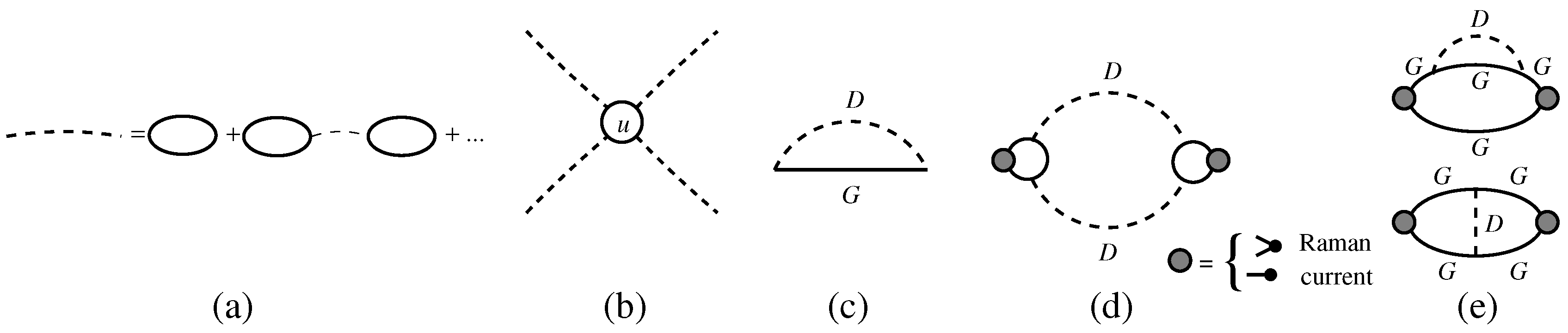
5. Looking for Fingerprints: Transport, Optics, Raman Spectroscopy
6. Other Fingerprints
7. The Resonant X-Ray Scattering Era
8. Concluding Remarks
Funding
Acknowledgments
Conflicts of Interest
References
- Plakida, N. High-Temperature Cuprate Superconductors - Experiment, Theory, and Applications; Springer Series in Solid-State Sciences; Springer: Berlin/Heidelberg, Germany, 2010; Volume 166. [Google Scholar]
- Raimondi, R.; Castellani, C.; Grilli, M.; Bang, Y.; Kotliar, G. Charge collective modes and dynamic pairing in the three-band Hubbard model. II. Strong-coupling limit. Phys. Rev. B 1993, 47, 3331. [Google Scholar] [CrossRef] [PubMed]
- Castellani, C.; Di Castro, C.; Grilli, M. Singular Quasiparticle Scattering in the Proximity of Charge Instabilities. Phys. Rev. Lett. 1995, 75, 4650. [Google Scholar] [CrossRef] [PubMed]
- Andergassen, S.; Caprara, S.; Di Castro, C.; Grilli, M. Anomalous isotopic effect near the charge-ordering quantum criticality. Phys. Rev. Lett. 2001, 87, 056401. [Google Scholar] [CrossRef] [PubMed]
- Caprara, S.; Di Castro, C.; Seibold, G.; Grilli, M. Dynamical charge density waves rule the phase diagram of cuprates. Phys. Rev. B 2017, 95, 224511. [Google Scholar] [CrossRef] [Green Version]
- Sachdev, S.; Ye, J. Universal quantum-critical dynamics of two-dimensional antiferromagnets. Phys. Rev. Lett. 1992, 69, 2411. [Google Scholar] [CrossRef]
- Monthoux, P.; Balatsky, A.V.; Pines, D. Weak-coupling theory of high-temperature superconductivity in the antiferromagnetically correlated copper oxides. Phys. Rev. B 1992, 46, 14803. [Google Scholar] [CrossRef]
- Sokol, A.; Pines, D. Toward a unified magnetic phase diagram of the cuprate superconductors. Phys. Rev. Lett. 1993, 71, 2813. [Google Scholar] [CrossRef] [PubMed]
- Monthoux, P.; Pines, D. Nearly antiferromagnetic Fermi-liquid description of magnetic scaling and spin-gap behavior. Phys. Rev. B 1994, 50, 16015. [Google Scholar] [CrossRef]
- Abanov, A.; Chubukov, A.; Schmalian, J. Quantum-critical theory of the spin-fermion model and its application to cuprates: Normal state analysis. Adv. Phys. 2003, 52, 119. [Google Scholar] [CrossRef]
- Anderson, W. The Resonating Valence Bond State in La2CuO4 and Superconductivity. Science 1987, 235, 1196. [Google Scholar] [CrossRef]
- Cancrini, N.; Caprara, S.; Castellani, C.; Di Castro, C.; Grilli, M.; Raimondi, R. Phase separation and superconductivity in the Kondo-like spin-hole coupled model. Europhys. Lett. 1991, 14, 597. [Google Scholar] [CrossRef]
- Müller, K.A.; Benedek, G. Phase Separation in Cuprate Superconductors; World Scientific: Singapore, 1993; p. 125. [Google Scholar]
- Bianconi, A. The instability close to the 2D generalized Wigner polaron crystal density: A possible pairing mechanism indicated by a key experiment. Phys. C 1994, 235–240, 269. [Google Scholar] [CrossRef]
- Caprara, S.; Grilli, M. Three Band t-J Model: A Systematic Large-N Analysis. Phys. Rev. B 1994, 49, 6971. [Google Scholar] [CrossRef] [PubMed]
- Caprara, S.; Di Castro, C.; Grilli, M. Magnetic and charge-transfer phase separation in the three band t-J model. Phys. Rev. B 1995, 51, 9286. [Google Scholar] [CrossRef]
- Caprara, S.; Castellani, C.; Di Castro, C.; Grilli, M. Phase separation and superconductivity in strongly interacting electron systems. Phys. C 1994, 235–240, 2155. [Google Scholar] [CrossRef]
- Jorgensen, J.D.; Dabrowski, B.; Pei, S.; Hinks, D.G.; Soderholm, L.; Morosin, B.; Schirber, J.E.; Venturini, E.L.; Ginley, D.S. Superconducting phase of La2CuO4+δ: A superconducting composition resulting from phase separation. Phys. Rev. B 1988, 38, 11337. [Google Scholar] [CrossRef] [PubMed]
- Bianconi, A.; Saini, N.L.; Lanzara, A.; Missori, M.; Rossetti, T.; Oyanagi, H.; Yamaguchi, H.; Oka, K.; Ito, T. Determination of the Local Lattice Distortions in the CuO2 plane of La1.85Sr0.15CuO4. Phys. Rev. Lett. 1996, 76, 3412. [Google Scholar] [CrossRef]
- Bianconi, A.; Lusignoli, M.; Saini, N.L.; Bordet, P.; Kvik, Å.; Radaelli, P.G. Stripe structure of the CuO2 plane in Bi2Sr2CaCu2O8+y. by anomalous X-ray diffraction. Phys. Rev. B 1996, 54, 4310. [Google Scholar] [CrossRef] [PubMed]
- Bianconi, A.; Saini, N.L.; Rossetti, T.; Lanzara, A.; Perali, A.; Missori, M.; Oyanagi, H.; Yamaguchi, H.; Nishihara, Y.; Ha, D.H. Stripe structure in the CuO2 plane of perovskite superconductors. Phys. Rev. B 1996, 54, 12018. [Google Scholar] [CrossRef] [PubMed]
- Caprara, S.; Castellani, C.; Di Castro, C.; Grilli, M.; Sadori, A. The Stripe-Quantum-Critical-Point as a key to the physics of the cuprates. Journale de Physique IV (Colloques) 1999, 9, 329–332. [Google Scholar] [CrossRef]
- Caprara, S.; Castellani, C.; Di Castro, C.; Grilli, M.; Perali, A. Charge and spin inhomogeneity as a key to the physics of the high-Tc cuprates. Phys. B 2000, 280, 196. [Google Scholar] [CrossRef]
- Di Castro, C.; Benfatto, L.; Caprara, S.; Castellani, C.; Grilli, M. The physics of the stripe quantum critical point. in the superconducting cuprates. Phys. C 2000, 341, 1715–1718. [Google Scholar] [CrossRef]
- Bianconi, A.; Bianconi, G.; Caprara, S.; Di Castro, D.; Oyanagi, H.; Saini, N.L. The stripe critical point for cuprates. J. Phys. Cond. Matt. 2000, 12, 10655. [Google Scholar] [CrossRef]
- Caprara, S.; Castellani, C.; Di Castro, C.; Grilli, M.; Perali, A.; Sulpizi, M. The stripe-phase Quantum-Critical-Point scenario for high-Tc superconductors. In Proceedings of the Conference Stripes 98, Rome, Italy, 4–7 June 1998; p. 45. [Google Scholar]
- Di Castro, C.; Grilli, M.; Caprara, S. Strong correlation, electron-phonon interaction and critical fluctuations: isotope effect, pseudogap formation, and phase diagram of the cuprates. J. Phys. Chem. Solids 2002, 63, 2219. [Google Scholar] [CrossRef]
- Caprara, S.; Di Castro, C.; Werner, P.; Zwerger, W. Vertex corrections near the stripe phase. Phys. Rev. Lett. 2002, 88, 066403. [Google Scholar] [CrossRef]
- Caprara, S. Reduction of the charge-density-wave amplitude in a strongly correlated system. J. Phys. Cond. Matt. 1998, 10, 5389. [Google Scholar] [CrossRef]
- Caprara, S.; Sulpizi, M.; Bianconi, A.; Di Castro, C.; Grilli, M. Single-particle properties of a model for coexisting charge and spin quasi-critical fluctuations coupled to electrons. Phys. Rev. B 1999, 59, 14980. [Google Scholar] [CrossRef]
- Saini, N.L.; Avila, J.; Bianconi, A.; Lanzara, A.; Asensio, M.C.; Tajima, S.; Gu, G.D.; Koshizuka, N. Topology of the pseudogap and shadow bands in Bi2Sr2CaCu2O8+δ at optimum doping. Phys. Rev. Lett. 1997, 79, 3467. [Google Scholar] [CrossRef]
- Bianconi, A.; Saini, N.L.; Valletta, A.; Lanzara, A.; Avila, J.; Asensio, M.C.; Tajima, S.; Gu, G.D.; Koshizuka, N. The Fermi surface of a high-Tc superconductor at optimum doping by angle-scanning photoemission spectroscopy. J. Phys. Chem. Solids. 1998, 59, 1884. [Google Scholar] [CrossRef]
- Saini, N.L.; Avila, J.; Asensio, M.C.; Tajima, S.; Gu, G.D.; Koshizuka, N.; Lanzara, A.; Bianconi, A. Evidence for a second one-dimensional set of states shedding light on the normal phase of high-Tc superconductors. Phys. Rev. B 1998, 57, R11101. [Google Scholar] [CrossRef]
- Saini, N.L.; Lanzara, A.; Bianconi, A.; Avila, J.; Asensio, M.C.; Tajima, S.; Gu, G.D.; Koshizuka, N. Antiferromagnetic and superconducting correlations in the pseudogap state of high-Tc cuprates. In Proceedings of the Second International Conference Stripes and High-Tc Superconductivity, Rome, Italy, 2–6 June 1998. [Google Scholar]
- Caprara, S.; Perali, A.; Sulpizi, M. Shadow bands, gap and pseudogaps in high-Tc superconductors. J. Superconduct. 1999, 12, 71. [Google Scholar] [CrossRef]
- Caprara, S.; Di Castro, C.; Grilli, M.; Perali, A.; Sulpizi, M. Fermi surface and gap parameter in high-Tc superconductors: The stripe quantum critical point scenario. Phys. C 1999, 317–318, 230–237. [Google Scholar] [CrossRef]
- Caprara, S.; Di Castro, C.; Grilli, M. Single-particle spectra near a stripe instability. Phys. B 2000, 284–288, 983. [Google Scholar] [CrossRef]
- Graf, J.; Gweon, G.-H.; McElroy, K.; Zhou, S.Y.; Jozwiak, C.; Rotenberg, E.; Bill, A.; Sasagawa, T.; Eisaki, H.; Uchida, S.; et al. Universal high energy anomaly in the angle-resolved photoemission spectra of high temperature superconductors: Possible evidence of spinon and holon branches. Phys. Rev. Lett. 2007, 98, 067004. [Google Scholar] [CrossRef]
- Chang, J.; Pailhés, S.; Shi, M.; Manson, M.; Claesson, T.; Tjernberg, O.; Voigt, J.; Perez, V.; Patthey, L.; Momono, N.; et al. When low- and high-energy electronic responses meet in cuprate superconductors. Phys. Rev. B 2007, 75, 224508. [Google Scholar] [CrossRef] [Green Version]
- Mazza, G.; Grilli, M.; Di Castro, C.; Caprara, S. Evidence for phonon-like charge and spin fluctuations from an analysis of angle-resolved photoemission spectra of La2−xSrxCuO4 superconductors. Phys. Rev. B 2013, 87, 014511. [Google Scholar] [CrossRef]
- Caprara, S.; Grilli, M. Single-particle spectra and Fermi surface near a stripe instability. Journale de Physique IV (Colloques) 1999, 9, Pr10–337. [Google Scholar] [CrossRef]
- Benfatto, L.; Caprara, S.; Perali, A. The pseudogap state in high-Tc superconductors. Phys. A 2000, 280, 185. [Google Scholar] [CrossRef]
- Benfatto, L.; Caprara, S.; Di Castro, C. Gap and pseudogap evolution within the charge-ordering scenario for superconducting cuprates. Eur. Phys. J. B 2000, 17, 95. [Google Scholar] [CrossRef]
- Benfatto, L.; Caprara, S. Gap and pseudogap evolution in underdoped cuprates. Int. J. Mod. Phys. B 2000, 14, 3006. [Google Scholar] [CrossRef]
- Caprara, S.; Grilli, M.; Di Castro, C.; Seibold, G. Pseudogap and (an)isotropic scattering in the fluctuating charge-density wave phase of cuprates. J. Superconduct. Novel Magn. 2017, 30, 25. [Google Scholar] [CrossRef]
- Caprara, S.; Di Castro, C.; Grilli, M.; Suppa, D. Charge-fluctuation contribution to the Raman response in superconducting cuprates. Phys. Rev. Lett. 2005, 95, 117004. [Google Scholar] [CrossRef] [PubMed]
- Tassini, L.; Venturini, F.; Zhang, Q.-M.; Hackl, R.; Kikugawa, N.; Fujita, T. Dynamical Properties of Charged Stripes in La2−xSrxCuO4. Phys. Rev. Lett. 2005, 95, 117002. [Google Scholar] [CrossRef] [PubMed]
- Di Castro, C.; Grilli, M.; Caprara, S.; Suppa, D. Charge critical fluctuations in cuprates: Isotope effect, pseudogap, conductivity and Raman spectroscopy. J. Phys. Chem. Solids. 2006, 67, 160. [Google Scholar] [CrossRef]
- Grilli, M.; Caprara, S.; Di Castro, C.; Suppa, D. Spectroscopic evidences of quantum critical charge fluctuations in cuprates. Phys. C Superconduct. 2007, 460–462, 1103. [Google Scholar] [CrossRef]
- Caprara, S.; Di Castro, C.; Enss, T.; Grilli, M. Low-energy signatures of charge and spin fluctuations in Raman and optical spectra of the cuprates. J. Phys. Chem. Solids. 2008, 69, 2155. [Google Scholar] [CrossRef]
- Caprara, S.; Di Castro, C.; Enss, T.; Grilli, M. On the contribution of nearly-critical spin and charge collective modes to the Raman spectra of high-Tc cuprates. J. Magn. Magn. Mater. 2009, 321, 686. [Google Scholar] [CrossRef]
- Grilli, M.; Caprara, S.; Di Castro, C.; Enss, T.; Hackl, R.; Muschler, B.; Prestel, W. Spectral signatures of critical charge and spin fluctuations in cuprates. Phys. B 2009, 404, 3070. [Google Scholar] [CrossRef]
- Caprara, S.; Di Castro, C.; Muschler, B.; Prestel, W.; Hackl, R.; Lambacher, M.; Erb, A.; Komiya, S.; Ando, Y.; Grilli, M. Extracting the dynamical effective interaction and competing order from an analysis of Raman spectra of the high-temperature La2−xSrxCuO4 superconductor. Phys. Rev. B 2011, 84, 054508. [Google Scholar] [CrossRef]
- Fanfarillo, L.; Mori, M.; Campetella, M.; Grilli, M.; Caprara, S. Glue function of optimally and overdoped cuprates from inversion of the Raman spectra. J. Phys. Cond. Matt. 2016, 28, 065701. [Google Scholar] [CrossRef] [Green Version]
- Caprara, S.; Di Castro, C.; Fratini, S.; Grilli, M. Anomalous optical absorption in the normal state of overdoped cuprates near the charge-ordering instability. Phys. Rev. Lett. 2002, 88, 147001. [Google Scholar] [CrossRef]
- Caprara, S.; Di Castro, C.; Grilli, M. Collective transport and optical absorption near the stripe criticality. J. Magn. Magn Mater. 2004, 272–276, 134. [Google Scholar] [CrossRef]
- Caprara, S.; Grilli, M.; Di Castro, C.; Enss, T. Optical conductivity near finite-wavelength quantum criticality. Phys. Rev. B 2007, 75, 140505(R). [Google Scholar] [CrossRef]
- Capati, M.; Caprara, S.; Di Castro, C.; Grilli, M.; Seibold, G.; Lorenzana, J. Electronic polymers and soft-matter-like broken symmetries in underdoped cuprates. Nat. Commun. 2015, 6, 7691. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kivelson, S.A.; Fradkin, E.; Emery, V.J. Electronic liquid-crystal phases of a doped Mott insulator. Nature 1998, 393, 550. [Google Scholar] [CrossRef]
- Hinkov, V.; Haug, D.; Fauqué, B.; Bourges, P.; Sidis, Y.; Ivanov, A.; Bernhard, C.; Lin, C.T.; Keimer, B. Electronic liquid crystal state in the high-temperature superconductor YBa2Cu3O6.45. Science 2008, 319, 597. [Google Scholar] [CrossRef]
- Daou, R.; Chang, J.; LeBoeuf, D.; Cyr-Choinière, O.; Laliberté, F.; Doiron-Leyraud, N.; Ramshaw, B.J.; Liang, R.; Bonn, D.A.; Hardy, W.N.; et al. Broken rotational symmetry in the pseudogap phase of a high-Tc superconductor. Nature 2010, 463, 519. [Google Scholar] [CrossRef]
- Mesaros, A.; Fujita, K.; Eisaki, H.; Uchida, S.; Davis, J.C.; Sachdev, S.; Zaanen, J.; Lawler, M.J.; Kim, E.-A. Topological Defects Coupling Smectic Modulations to Intra–Unit-Cell Nematicity in Cuprates. Science 2011, 333, 426. [Google Scholar] [CrossRef] [PubMed]
- Lawler, M.J.; Fujita, K.; Lee, J.; Schmidt, A.R.; Kohsaka, Y.; Kim, C.K.; Eisaki, H.; Uchida, S.; Davis, J.C.; Sethna, J.P.; et al. Intra-unit-cell electronic nematicity of the high-Tc copper-oxide pseudogap states. Nature 2010, 466, 347. [Google Scholar] [CrossRef] [PubMed]
- Caprara, S.; Colonna, M.; Di Castro, C.; Hackl, R.; Muschler, B.; Tassini, L.; Grilli, M. Signatures of nematic quantum critical fluctuations in the Raman spectra of lightly doped cuprates. Phys. Rev. B 2015, 91, 205115. [Google Scholar] [CrossRef] [Green Version]
- Perali, A.; Castellani, C.; Di Castro, C.; Grilli, M. d-wave superconductivity near charge instabilities. Phys. Rev. B 1996, 54, 16216. [Google Scholar] [CrossRef] [PubMed]
- Sangiovanni, G.; Capone, M.; Caprara, S.; Castellani, C.; Di Castro, C.; Grilli, M. Doping-driven transition to a time-reversal breaking state in the phase diagram of the cuprates. Phys. Rev. B 2003, 67, 174507. [Google Scholar] [CrossRef]
- Sangiovanni, G.; Capone, M.; Caprara, S. Time reversal breaking superconducting state in the phase diagram of the cuprates. Int. J. Mod. Phys. B 2003, 17, 614. [Google Scholar] [CrossRef]
- Caprara, S.; Grilli, M.; Leridon, B.; Lesueur, J. Extended paraconductivity regime in underdoped cuprates. Phys. Rev. B 2005, 72, 104509. [Google Scholar] [CrossRef] [Green Version]
- Leridon, B.; Caprara, S.; Vanacken, J.; Moshchalkov, V.V.; Attanasi, A.; Grilli, M.; Lorenzana, J. Protected superconductive pairing at the boundaries of charge-density-wave domains. arXiv 2019, arXiv:1905.05606. [Google Scholar]
- Wu, T.; Mayaffre, H.; Krämer, S.; Horvatić, M.; Berthier, C.; Hardy, W.N.; Liang, R.; Bonn, D.A.; Julien, M.-H. Magnetic-field-induced charge-stripe order in the high-temperature superconductor YBa2Cu3Oy. Nature 2011, 477, 191. [Google Scholar] [CrossRef] [PubMed]
- Wu, T.; Mayaffre, H.; Krämer, S.; Horvatić, M.; Berthier, C.; Kuhns, P.L.; Reyes, A.P.; Liang, R.; Hardy, W.N.; Bonn, D.A.; et al. Emergence of charge order from the vortex state of a high-temperature superconductor. Nat. Commun. 2013, 4, 2113. [Google Scholar] [CrossRef] [PubMed]
- Caprara, S.; Grilli, M.; Lorenzana, J.; Leridon, B. Competing order and double-step superconducting transition in La2−xSrxCuO4. 2019; in preparation. [Google Scholar]
- Campi, G.; Di Castro, D.; Bianconi, G.; Agrestini, S.; Saini, N.L.; Oyanagi, H.; Bianconi, A. Photo-Induced phase transition to a striped polaron crystal in cuprates. Phase Trans. 2002, 75, 927–933. [Google Scholar] [CrossRef]
- Campi, G.; Ricci, A.; Poccia, N.; Fratini, M.; Bianconi, A. X-Rays Writing/Reading of charge density waves in the CuO2 plane of a simple cuprate superconductor. Condense. Matt. 2017, 2, 26. [Google Scholar] [CrossRef]
- Kusmartsev, F.V.; Di Castro, D.; Bianconi, G.; Bianconi, A. Transformation of strings into an inhomogeneous phase of stripes and itinerant carriers. Phys. Lett. A 2000, 275, 118–123. [Google Scholar] [CrossRef]
- Campi, G.; Bianconi, A. A case of complex matter: coexistence of multiple phase separations in cuprates. In Symmetry and Heterogeneity in High Temperature Superconductors; Springer: Dordrecht, The Netherlands, 2006; pp. 147–156. [Google Scholar]
- Innocenti, D.; Ricci, A.; Poccia, N.; Campi, G.; Fratini, M.; Bianconi, A. A model for liquid-striped liquid phase separation in liquids of anisotropic polarons. J. Superconduct. Novel Magn. 2009, 22, 529–533. [Google Scholar] [CrossRef]
- Campi, G.; Innocenti, D.; Bianconi, A. CDW and similarity of the Mott insulator-to-metal transition in cuprates with the gas-to-liquid-liquid transition in supercooled water. J. Superconduct. Novel Magn. 2015, 28, 1355–1363. [Google Scholar] [CrossRef]
- Campi, G.; Bianconi, A. High-Temperature superconductivity in a hyperbolic geometry of complex matter from nanoscale to mesoscopic scale. J. Superconduct. Novel Magn. 2016, 29, 627–631. [Google Scholar] [CrossRef]
- Ghiringhelli, G.; Le Tacon, M.; Minola, M.; Blanco-Canosa, S.; Mazzoli, C.; Brookes, N.B.; De Luca, G.M.; Frano, A.; Hawthorn, D.G.; He, F.; et al. Long-range incommensurate charge fluctuations in (Y,Nd)Ba2Cu3O6+x. Science 2012, 337, 821. [Google Scholar] [CrossRef] [PubMed]
- Chang, J.; Blackburn, E.; Holmes, A.T.; Christensen, N.B.; Larsen, J.; Mesot, J.; Liang, R.; Bonn, D.A.; Hardy, W.N.; Watenphul, A.; et al. Direct observation of competition between superconductivity and charge density wave order in YBa2Cu3O6.67. Nat. Phys. 2012, 8, 871. [Google Scholar] [CrossRef]
- Comin, R.; Frano, A.; Yee, M.M.; Yoshida, Y.; Eisaki, H.; Schierle, E.; Weschke, E.; Sutarto, R.; He, F.; Soumyanarayanan, A.; et al. Charge order driven by Fermi-arc instability in Bi2Sr2−xLaxCuO6+δ. Science 2014, 343, 390. [Google Scholar] [CrossRef]
- Blanco-Canosa, S.; Frano, A.; Schierle, E.; Porras, J.; Loew, T.; Minola, M.; Bluschke, M.; Weschke, E.; Keimer, B.; Le Tacon, M. Resonant X-ray scattering study of charge-density- wave correlations in YBa2Cu3O6+x. Phys. Rev. B 2014, 90, 054513. [Google Scholar] [CrossRef]
- Peng, Y.Y.; Fumagalli, R.; Ding, Y.; Minola, M.; Caprara, S.; Betto, D.; De Luca, G.M.; Kummer, K.; Lefranois, E.; Salluzzo, M.; et al. Reentrant charge order in overdoped (Bi,Pb)2.12Sr1.88CuO6+δ outside the pseudogap regime. Nat. Mater. 2018, 17, 697. [Google Scholar] [CrossRef] [PubMed]
- Arpaia, R.; Caprara, S.; Fumagalli, R.; De Vecchi, G.; Peng, Y.Y.; Andersson, E.; Betto, D.; De Luca, G.M.; Brookes, N.B.; Lombardi, F.; et al. Dynamical charge density fluctuations pervading the phase diagram of a Cu-based high-Tc superconductor. arXiv 2019, arXiv:1809.04949. [Google Scholar]
- Campi, G.; Bianconi, A.; Poccia, N.; Bianconi, G.; Barba, L.; Arrighetti, G.; Innocenti, D.; Karpinski, J.; Zhigadlo, N.D.; Kazakov, S.M.; et al. Inhomogeneity of charge-density-wave order and quenched disorder in a high-Tc superconductor. Nature 2015, 525, 359. [Google Scholar] [CrossRef]
- Seibold, G.; Arpaia, R.; Peng, Y.Y.; Fumagalli, R.; Braicovich, L.; Di Castro, C.; Grilli, M.; Ghiringhelli, G.; Caprara, S. Marginal Fermi Liquid behaviour from charge density fluctuations in cuprates. arXiv 2019, arXiv:1905.10232. [Google Scholar]
© 2019 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Caprara, S. The Ancient Romans’ Route to Charge Density Waves in Cuprates. Condens. Matter 2019, 4, 60. https://doi.org/10.3390/condmat4020060
Caprara S. The Ancient Romans’ Route to Charge Density Waves in Cuprates. Condensed Matter. 2019; 4(2):60. https://doi.org/10.3390/condmat4020060
Chicago/Turabian StyleCaprara, Sergio. 2019. "The Ancient Romans’ Route to Charge Density Waves in Cuprates" Condensed Matter 4, no. 2: 60. https://doi.org/10.3390/condmat4020060
APA StyleCaprara, S. (2019). The Ancient Romans’ Route to Charge Density Waves in Cuprates. Condensed Matter, 4(2), 60. https://doi.org/10.3390/condmat4020060




