Operando XAFS and XRD Study of a Prussian Blue Analogue Cathode Material: Iron Hexacyanocobaltate
Abstract
1. Introduction
2. Results
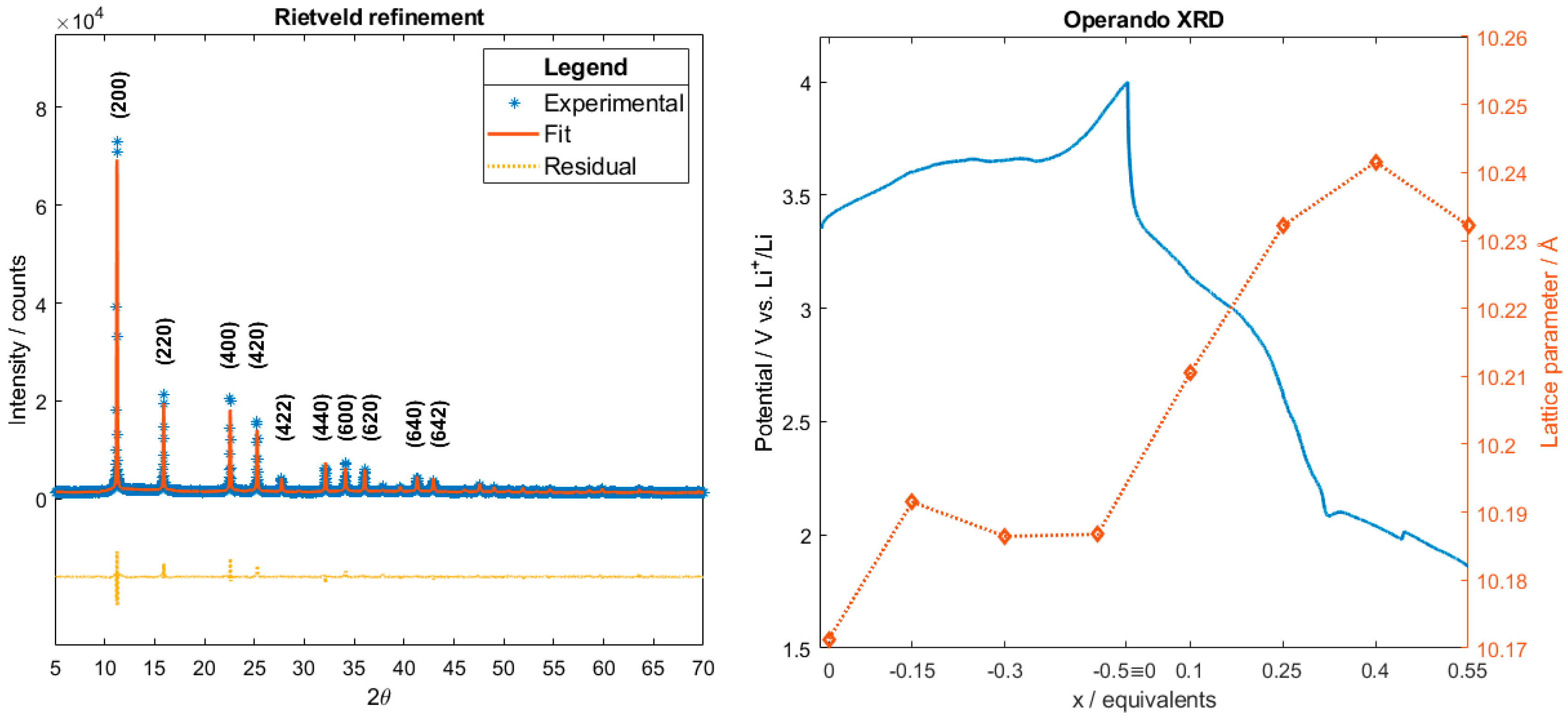
2.1. XRD Data Analysis
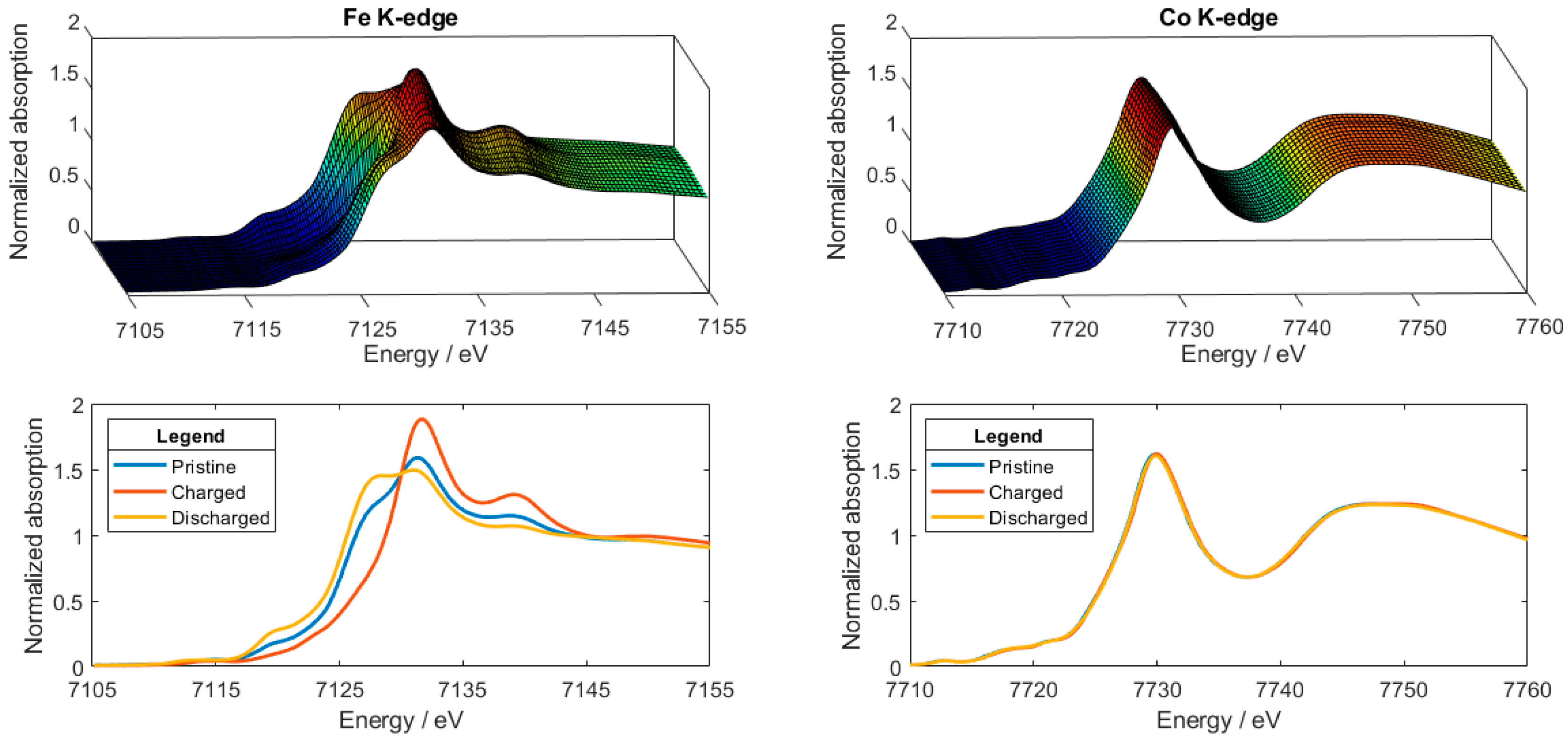
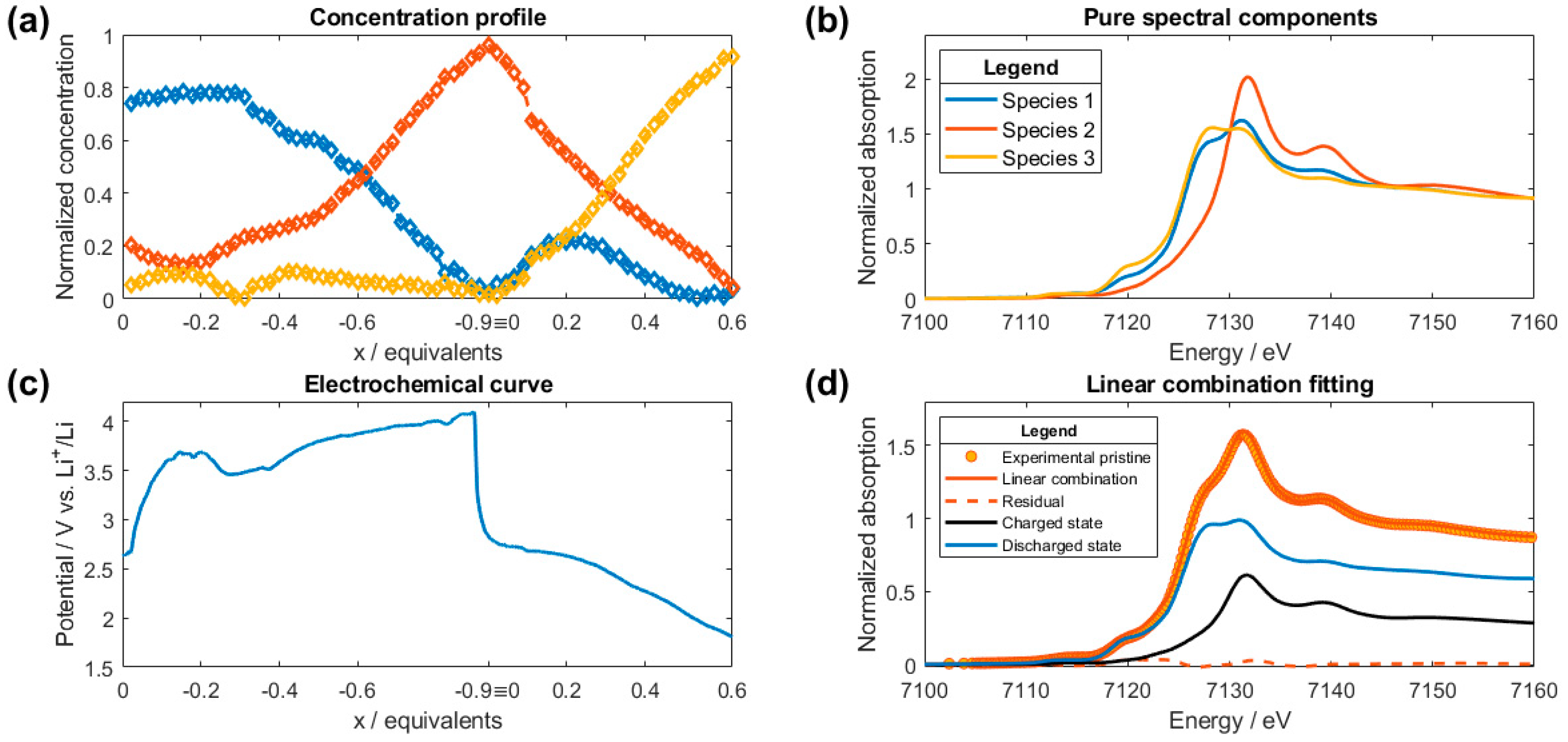
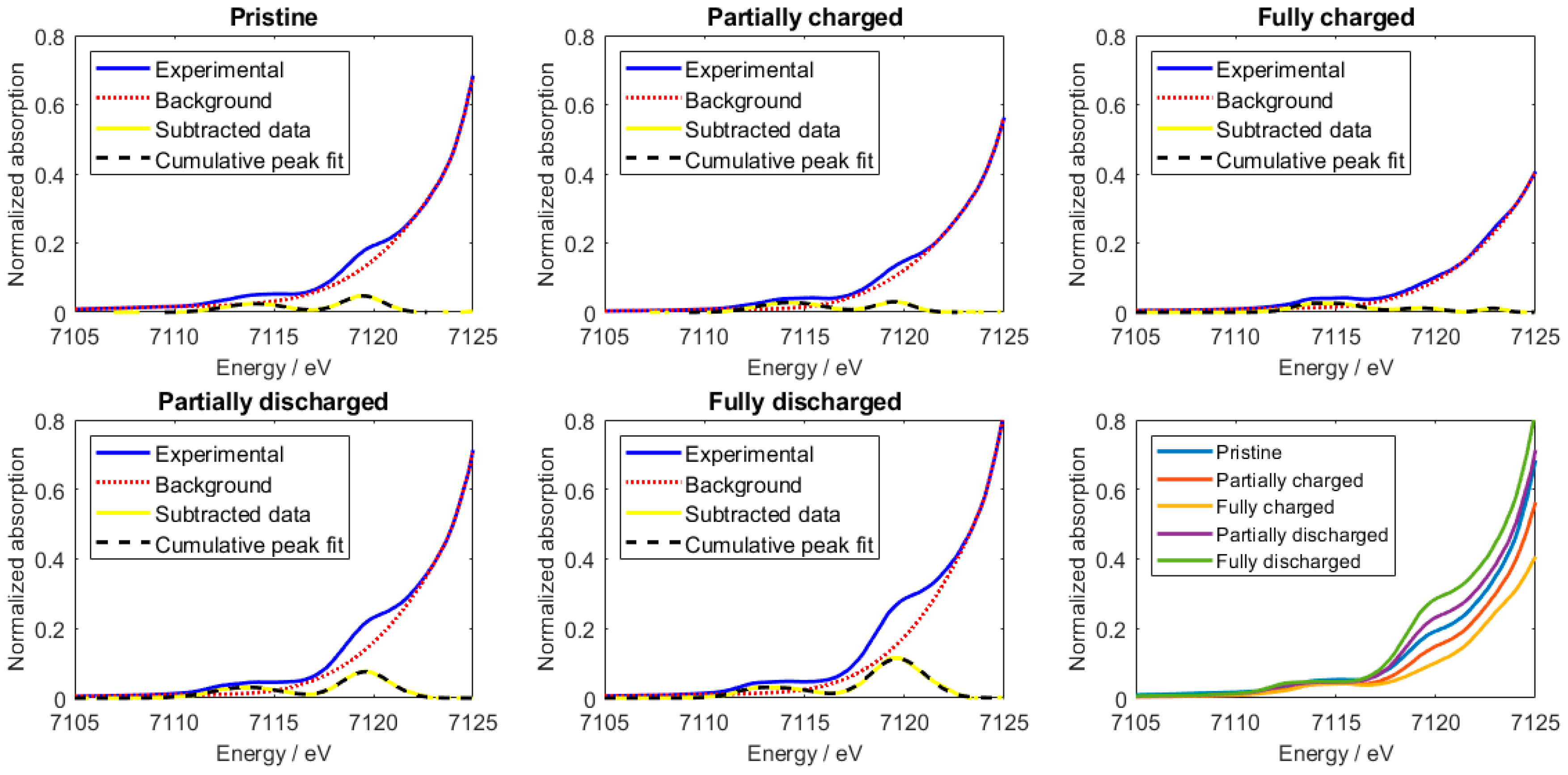
2.2. XANES Data Analysis
2.3. EXAFS Data Analysis
3. Discussion and Conclusions
4. Materials and Methods
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Chu, S.; Majumdar, A. Opportunities and challenges for a sustainable energy future. Nature 2012, 488, 294–303. [Google Scholar] [CrossRef] [PubMed]
- Goodenough, J.B.; Kim, Y. Challenges for rechargeable Li batteries. Chem. Mater. 2010, 22, 587–603. [Google Scholar] [CrossRef]
- Sun, H.; Wang, J.G.; Zhang, Y.; Hua, W.; Li, Y.; Liu, H. Ultrafast lithium energy storage enabled by interfacial construction of interlayer-expanded MoS2/N-doped carbon nanowires. J. Mater. Chem. A 2018, 6, 13419–13427. [Google Scholar] [CrossRef]
- Liu, H.; Wang, J.G.; Hua, W.; Wang, J.; Nan, D.; Wei, C. Scale-up production of high-tap-density carbon/MnOx/carbon nanotube microcomposites for Li-ion batteries with ultrahigh volumetric capacity. Chem. Eng. J. 2018, 354, 220–227. [Google Scholar] [CrossRef]
- Goodenough, J.B.; Manthiram, A. A perspective on electrical energy storage. MRS Commun. 2014, 4, 135–142. [Google Scholar] [CrossRef]
- Goodenough, J.B. Electrochemical energy storage in a sustainable modern society. Energy Environ. Sci. 2014, 7, 14–18. [Google Scholar] [CrossRef]
- Wessells, C.D.; Peddada, S.V.; McDowell, M.T.; Huggins, R.A.; Cui, Y. The Effect of Insertion Species on Nanostructured Open Framework Hexacyanoferrate Battery Electrodes. J. Electrochem. Soc. 2012, 159, A98–A103. [Google Scholar] [CrossRef]
- Moritomo, Y.; Urase, S.; Shibata, T. Enhanced battery performance in manganese hexacyanoferrate by partial substitution. Electrochim. Acta 2016, 210, 963–969. [Google Scholar] [CrossRef]
- Song, J.; Wang, L.; Lu, Y.; Liu, J.; Guo, B.; Xiao, P.; Lee, J.J.; Yang, X.Q.; Henkelman, G.; Goodenough, J.B. Removal of Interstitial H2O in Hexacyanometallates for a Superior Cathode of a Sodium-Ion Battery. J. Am. Chem. Soc. 2015, 137, 2658–2664. [Google Scholar] [CrossRef] [PubMed]
- Wessells, C.D.; Peddada, S.V.; Huggins, R.A.; Cui, Y. Nickel hexacyanoferrate nanoparticle electrodes for aqueous sodium and potassium ion batteries. Nano Lett. 2011, 11, 5421–5425. [Google Scholar] [CrossRef] [PubMed]
- Eftekhari, A. Potassium secondary cell based on Prussian blue cathode. J. Power Sources 2004, 126, 221–228. [Google Scholar] [CrossRef]
- Liu, S.; Pan, G.L.; Li, G.R.; Gao, X.P. Copper hexacyanoferrate nanoparticles as cathode material for aqueous Al-ion batteries. J. Mater. Chem. A 2015, 3, 959–962. [Google Scholar] [CrossRef]
- Giorgetti, M.; Scavetta, E.; Berrettoni, M.; Tonelli, D. Nickel hexacyanoferrate membrane as a coated wire cation-selective electrode. Analyst 2001, 126, 2168–2171. [Google Scholar] [CrossRef] [PubMed]
- Giorgetti, M.; Tonelli, D.; Berrettoni, M.; Aquilanti, G.; Minicucci, M. Copper hexacyanoferrate modified electrodes for hydrogen peroxide detection as studied by X-ray absorption spectroscopy. J. Solid State Electrochem. 2014, 18, 965–973. [Google Scholar] [CrossRef]
- Sato, O.; Iyoda, T.; Fujishima, A.; Hashimoto, K. Photoinduced magnetization of a cobalt-iron cyanide. Science 1996, 272, 704–705. [Google Scholar] [CrossRef] [PubMed]
- Bueno, P.R.; Giménez-Romero, D.; Ferreira, F.F.; Setti, G.O.; Garcia-Jareño, J.J.; Agrisuelas, J.; Vicente, F. Electrochromic switching mechanism of iron hexacyanoferrates molecular compounds: The role of Fe2+(CN)6 vacancies. J. Phys. Chem. C 2009, 113, 9916–9920. [Google Scholar] [CrossRef]
- Neff, V.D. Some Performance Characteristics of a Prussian Blue Battery. J. Electrochem. Soc. 1985, 132, 1382. [Google Scholar] [CrossRef]
- Wang, J.-G.; Zhang, Z.; Zhang, X.; Yin, X.; Li, X.; Liu, X.; Kang, F.; Wei, B. Cation exchange formation of prussian blue analogue submicroboxes for high-performance Na-ion hybrid supercapacitors. Nano Energy 2017, 39, 647–653. [Google Scholar] [CrossRef]
- Wang, J.-G.; Zhang, Z.; Liu, X.; Wei, B. Facile synthesis of cobalt hexacyanoferrate/graphene nanocomposites for high-performance supercapacitor. Electrochim. Acta 2017, 235, 114–121. [Google Scholar] [CrossRef]
- Chen, R.; Tanaka, H.; Kawamoto, T.; Asai, M.; Fukushima, C.; Kurihara, M.; Watanabe, M.; Arisaka, M.; Nankawa, T. Preparation of a film of copper hexacyanoferrate nanoparticles for electrochemical removal of cesium from radioactive wastewater. Electrochem. Commun. 2012, 25, 23–25. [Google Scholar] [CrossRef]
- Ventura, M.; Mullaliu, A.; Ciurduc, D.E.; Zappoli, S.; Giuli, G.; Tonti, D.; Enciso, E.; Giorgetti, M. Thin layer films of copper hexacyanoferrate: Structure identification and analytical applications. J. Electroanal. Chem. 2018, 827, 10–20. [Google Scholar] [CrossRef]
- Ciabocco, M.; Cancemi, P.; Saladino, M.L.; Caponetti, E.; Alduina, R.; Berrettoni, M. Synthesis and antibacterial activity of iron-hexacyanocobaltate nanoparticles. J. Biol. Inorg. Chem. 2018, 23, 385–398. [Google Scholar] [CrossRef] [PubMed]
- Lee, Y.S.; Shin, W.K.; Kannan, A.G.; Koo, S.M.; Kim, D.W. Improvement of the Cycling Performance and Thermal Stability of Lithium-Ion Cells by Double-Layer Coating of Cathode Materials with Al2O3 Nanoparticles and Conductive Polymer. ACS Appl. Mater. Interfaces 2015, 7, 13944–13951. [Google Scholar] [CrossRef] [PubMed]
- Mullaliu, A.; Aquilanti, G.; Conti, P.; Plaisier, J.R.; Fehse, M.; Stievano, L.; Giorgetti, M. Copper Electroactivity in Prussian Blue-Based Cathode Disclosed by Operando XAS. J. Phys. Chem. C 2018, 122, 15868–15877. [Google Scholar] [CrossRef]
- Wessells, C.D.; Huggins, R.A.; Cui, Y. Copper hexacyanoferrate battery electrodes with long cycle life and high power. Nat. Commun. 2011, 2, 550. [Google Scholar] [CrossRef] [PubMed]
- Allan, D.R.; Collins, S.P.; Evans, G.; Hall, D.; McAuley, K.; Owen, R.L.; Sorensen, T.; Tang, C.C.; von Delft, F.; Wagner, A.; et al. Status of the crystallography beamlines at Diamond Light Source. Eur. Phys. J. Plus 2015, 130, 1–20. [Google Scholar] [CrossRef]
- Renman, V.; Ojwang, D.O.; Valvo, M.; Gómez, C.P.; Gustafsson, T.; Svensson, G. Structural-electrochemical relations in the aqueous copper hexacyanoferrate-zinc system examined by synchrotron X-ray diffraction. J. Power Sources 2017, 369, 146–153. [Google Scholar] [CrossRef]
- Ojwang, D.O.; Grins, J.; Wardecki, D.; Valvo, M.; Renman, V.; Häggström, L.; Ericsson, T.; Gustafsson, T.; Mahmoud, A.; Hermann, R.P.; et al. Structure Characterization and Properties of K-Containing Copper Hexacyanoferrate. Inorg. Chem. 2016, 55, 5924–5934. [Google Scholar] [CrossRef] [PubMed]
- Giorgetti, M. A Review on the Structural Studies of Batteries and Host Materials by X-Ray Absorption Spectroscopy. ISRN Mater. Sci. 2013, 2013, 938625. [Google Scholar] [CrossRef]
- Buchholz, D.; Li, J.; Passerini, S.; Aquilanti, G.; Wang, D.; Giorgetti, M. X-ray Absorption Spectroscopy Investigation of Lithium-Rich, Cobalt-Poor Layered-Oxide Cathode Material with High Capacity. ChemElectroChem 2015, 2, 85–97. [Google Scholar] [CrossRef]
- Giorgetti, M.; Stievano, L. X-ray Absorption Spectroscopy Study of Battery Materials, 1st ed.; InTech: Rijeka, Croatia, 2017; ISBN 978-953-51-3014-7. [Google Scholar]
- Conti, P.; Zamponi, S.; Giorgetti, M.; Berrettoni, M.; Smyrl, W.H. Multivariate Curve Resolution Analysis for Interpretation of Dynamic Cu K-Edge X-ray Absorption Spectroscopy Spectra for a Cu Doped V2O5 Lithium Battery. Anal. Chem. 2010, 82, 3629–3635. [Google Scholar] [CrossRef] [PubMed]
- Mullica, D.F.; Oliver, J.D.; Milligan, W.O.; Hills, F.W. Ferrous hexacyanocobaltate dodecahydrate. Inorg. Nucl. Chem. Lett. 1979, 15, 361–365. [Google Scholar] [CrossRef]
- Adak, S.; Daemen, L.L.; Hartl, M.; Williams, D.; Summerhill, J.; Nakotte, H. Thermal expansion in 3d-metal Prussian Blue Analogs—A survey study. J. Solid State Chem. 2011, 184, 2854–2861. [Google Scholar] [CrossRef]
- Aquilanti, G.; Giorgetti, M.; Dominko, R.; Stievano, L.; Arčon, I.; Novello, N.; Olivi, L. Operando characterization of batteries using x-ray absorption spectroscopy: Advances at the beamline XAFS at synchrotron Elettra. J. Phys. D Appl. Phys. 2017, 50, 074001. [Google Scholar] [CrossRef]
- Giorgetti, M.; Mignani, A.; Aquilanti, G.; Conti, P.; Fehse, M.; Stievano, L. Structural and electronic studies of metal hexacyanoferrates based cathodes for Li rechargeable batteries. J. Phys. Conf. Ser. 2016, 712, 012127. [Google Scholar] [CrossRef]
- De Juan, A.; Jaumot, J.; Tauler, R. Multivariate Curve Resolution (MCR). Solving the mixture analysis problem. Anal. Methods 2014, 6, 4964–4976. [Google Scholar] [CrossRef]
- Berrettoni, M.; Giorgetti, M.; Zamponi, S.; Conti, P.; Ranganathan, D.; Zanotto, A.; Saladino, M.L.; Caponetti, E. Synthesis and characterization of nanostructured cobalt hexacyanoferrate. J. Phys. Chem. C 2010, 114, 6401–6407. [Google Scholar] [CrossRef]
- Giorgetti, M.; Aquilanti, G.; Ciabocco, M.; Berrettoni, M. Anatase-driven charge transfer involving a spin transition in cobalt iron cyanide nanostructures. Phys. Chem. Chem. Phys. 2015, 17, 22519–22522. [Google Scholar] [CrossRef] [PubMed]
- Hayakawa, K.; Hatada, K.; D’Angelo, P.; Della Longa, S.; Natoli, C.R.; Benfatto, M. Full quantitative multiple-scattering analysis of X-ray absorption spectra: Application to potassium hexacyanoferrate(II) and–(III) complexes. J. Am. Chem. Soc. 2004, 126, 15618–15623. [Google Scholar] [CrossRef] [PubMed]
- Bianconi, A.; Dell’Ariccia, M.; Durham, P.J.; Pendry, J.B. Multiple-scattering resonances and structural effects in the x-ray-absorption near-edge spectra of FeII and FeIII hexacyanide complexes. Phys. Rev. B 1982, 26, 6502–6508. [Google Scholar] [CrossRef]
- Kosugi, N.; Yokoyama, T.; Kuroda, H. Polarization dependence of XANES of square-planar Ni(CN)42− ion. A comparison with octahedral Fe(CN)64− and Fe(CN)63− ions. Chem. Phys. 1986, 104, 449–453. [Google Scholar] [CrossRef]
- Giorgetti, M.; Berrettoni, M. Structure of Fe/Co/Ni hexacyanoferrate as probed by multiple edge X-ray absorption spectroscopy. Inorg. Chem. 2008, 47, 6001–6008. [Google Scholar] [CrossRef] [PubMed]
- Hannauer, J.; Scheers, J.; Fullenwarth, J.; Fraisse, B.; Stievano, L.; Johansson, P. The Quest for Polysulfides in Lithium-Sulfur Battery Electrolytes: An Operando Confocal Raman Spectroscopy Study. Chem. Phys. Chem. 2015, 16, 2755–2759. [Google Scholar] [CrossRef] [PubMed]
- Rebuffi, L.; Plaisier, J.R.; Abdellatief, M.; Lausi, A.; Scardi, A.P. Mcx: A synchrotron radiation beamline for X-ray diffraction line profile analysis. Z. Anorg. Allg. Chem. 2014, 640, 3100–3106. [Google Scholar] [CrossRef]
- Rodríguez-Carvajal, J. Recent advances in magnetic structure determination by neutron powder diffraction. Phys. B Phys. Condens. Matter 1993, 192, 55–69. [Google Scholar] [CrossRef]
- Yao, M.; Kuratani, K.; Kojima, T.; Takeichi, N.; Senoh, H.; Kiyobayashi, T. Indigo carmine: An organic crystal as a positive-electrode material for rechargeable sodium batteries. Sci. Rep. 2014, 4, 3650. [Google Scholar] [CrossRef] [PubMed]
- Momma, K.; Izumi, F. VESTA 3 for three-dimensional visualization of crystal, volumetric and morphology data. J. Appl. Crystallogr. 2011, 44, 1272–1276. [Google Scholar] [CrossRef]
- Ravel, B.; Newville, M. ATHENA, ARTEMIS, HEPHAESTUS: Data analysis for X-ray absorption spectroscopy using IFEFFIT. J. Synchrotron Radiat. 2005, 12, 537–541. [Google Scholar] [CrossRef] [PubMed]
- Filipponi, A.; Di Cicco, A.; Natoli, C.R. X-ray-absorption spectroscopy and n-body distribution functions in condensed matter. I. Theory. Phys. Rev. B 1995, 52, 15122–15134. [Google Scholar] [CrossRef]
- Filipponi, A.; Di Cicco, A. X-ray-absorption spectroscopy and n-body distribution functions in condensed matter. II. Data analysis and applications. Phys. Rev. B 1995, 52, 15135–15149. [Google Scholar] [CrossRef]
- Giorgetti, M.; Berrettoni, M.; Filipponi, A.; Kulesza, P.J.; Marassi, R. Evidence of four-body contributions in the EXAFS spectrum of Na2Co[Fe(CN)6]. Chem. Phys. Lett. 1997, 275, 108–112. [Google Scholar] [CrossRef]
- Giorgetti, M.; Guadagnini, L.; Tonelli, D.; Minicucci, M.; Aquilanti, G. Structural characterization of electrodeposited copper hexacyanoferrate films by using a spectroscopic multi-technique approach. Phys. Chem. Chem. Phys. 2012, 14, 5527–5537. [Google Scholar] [CrossRef] [PubMed]
- Hedin, L.; Lundqvist, B.I.; Lundqvist, S. Local exchange-correlation potentials. Solid State Commun. 1971, 9, 537–541. [Google Scholar] [CrossRef]
- Krause, M.O.; Oliver, J.H. Natural widths of atomic K and L levels, K α X-ray lines and several K L L Auger lines. J. Phys. Chem. Ref. Data 1979, 8, 329–338. [Google Scholar] [CrossRef]
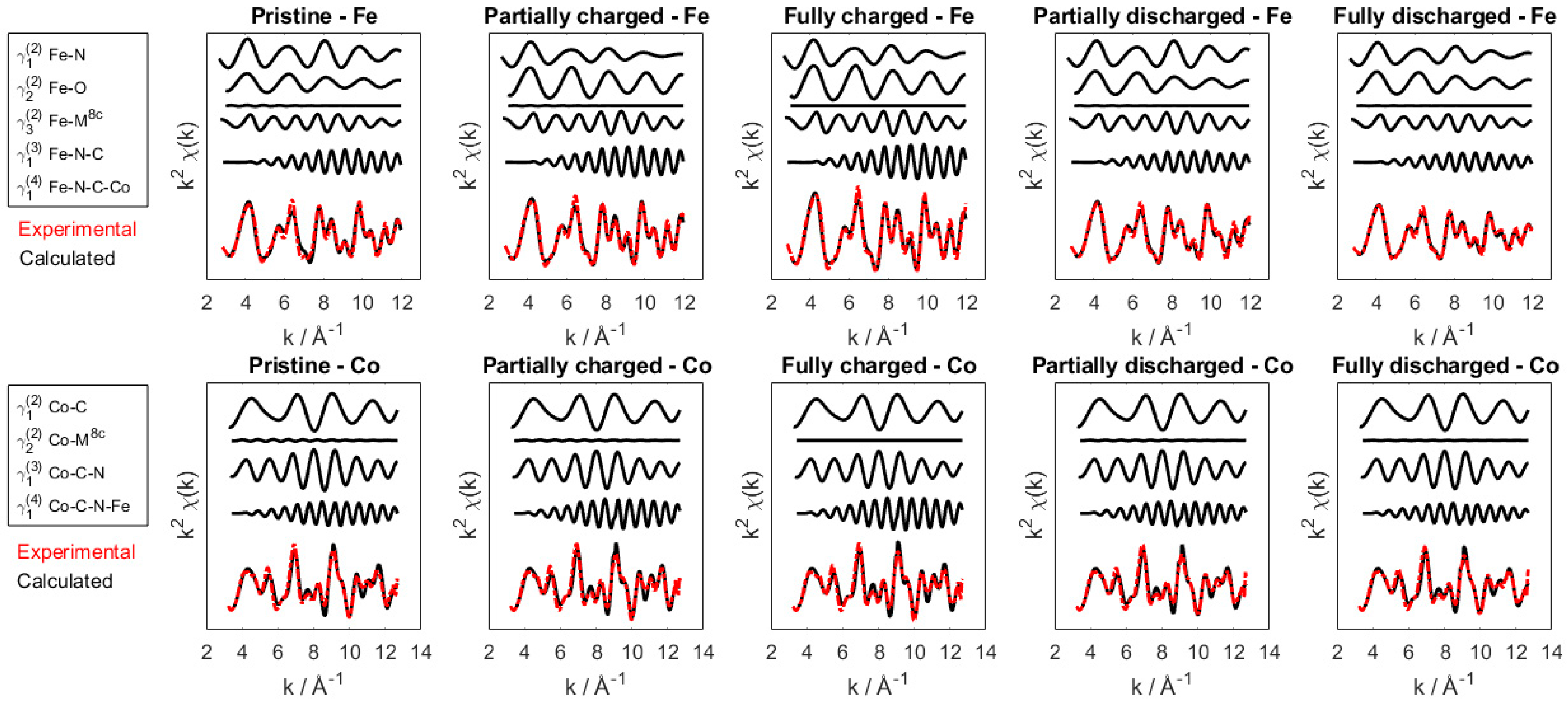
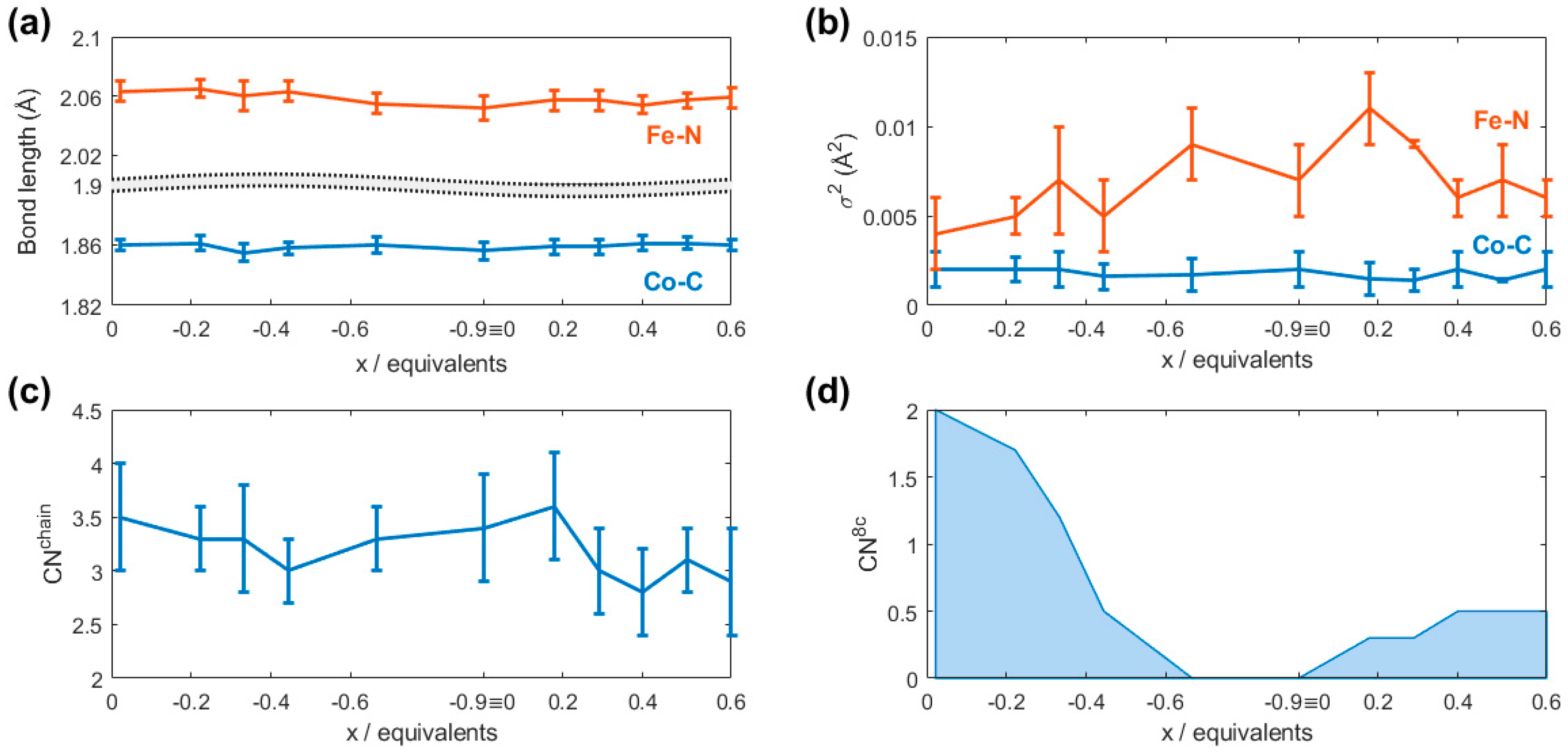
| Powder | Pristine | C10 | C15 | C20 | C30 | C42 | C50 | C55 | C60 | C65 | C70 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Co-C (N)/Å | 1.862(3) | 1.860(4) | 1.861(5) | 1.855(6) | 1.858(4) | 1.860(5) | 1.856(6) | 1.859(5) | 1.859(5) | 1.861(5) | 1.861(4) | 1.860(4) |
| σ2 Co-C/Å2 | 0.0016(5) | 0.002(1) | 0.0020(7) | 0.002(1) | 0.0016(7) | 0.0017(9) | 0.002(1) | 0.002(1) | 0.0015(9) | 0.0014(6) | 0.002(1) | 0.0014(7) |
| C≡N/Å | 1.187(5) | 1.185(5) | 1.187(7) | 1.18(1) | 1.18(1) | 1.182(7) | 1.184(7) | 1.183(6) | 1.182(6) | 1.184(6) | 1.184(7) | 1.186(6) |
| σ2 C≡N/Å2 | 0.007(2) | 0.005(2) | 0.0013(6) | 0.005(2) | 0.003(1) | 0.002(1) | 0.002(1) | 0.006(2) | 0.005(2) | 0.0012(4) | 0.004(2) | 0.004(1) |
| Fe-N/Å | 2.077(8) | 2.063(7) | 2.065(6) | 2.06(1) | 2.063(7) | 2.055(7) | 2.052(8) | 2.057(7) | 2.057(7) | 2.054(6) | 2.057(5) | 2.059(7) |
| σ2 Fe-N/Å2 | 0.007(2) | 0.004(2) | 0.005(1) | 0.007(3) | 0.005(2) | 0.009(2) | 0.007(2) | 0.011(2) | 0.009(2) | 0.006(1) | 0.007(2) | 0.006(1) |
| Fe-O/Å | 2.09(2) | 2.02(1) | 2.02(2) | 2.03(1) | 2.036(8) | 2.015(9) | 2.00(1) | 2.008(8) | 2.009(9) | 2.02(1) | 2.02(2) | 2.02(1) |
| σ2 Fe-O/Å2 | 0.006(3) | 0.005(3) | 0.004(2) | 0.004(2) | 0.002(1) | 0.003(2) | 0.003(2) | 0.002(1) | 0.003(1) | 0.006(2) | 0.004(1) | 0.005(2) |
| σ2 Co-C-N/deg2 | 4(3) | 4(3) | 4(2) | 4(3) | 4(3) | 4(3) | 4(3) | 4(3) | 5(4) | 4(3) | 4(3) | 5(4) |
| σ2 Fe-N-C/deg2 | 9(6) | 10(8) | 10(8) | 3(2) | 10(9) | 10(8) | 9(8) | 9(8) | 9(8) | 9(8) | 9(8) | 9(8) |
| E0 (Fe)/eV | 7121.4(6) | 7123.4 | 7123.2 | 7123.7 | 7124.2 | 7124.3 | 7124.9 | 7124.3 | 7123.7 | 7123.5 | 7122.9 | 7122.4 |
| E0 (Co)/eV | 7717.8(6) | 7717.5 | 7718.4 | 7717.2 | 7717.3 | 7717.4 | 7717.2 | 7717.3 | 7717.3 | 7717.4 | 7717.4 | 7717.4 |
| CNchain | 3.5(3) | 3.5(5) | 3.3(3) | 3.3(5) | 3.0(3) | 3.3(3) | 3.4(5) | 3.6(5) | 3.0(4) | 2.8(4) | 3.1(3) | 2.9(5) |
| CN3 (Fe-O) | 1.8(5) | 1.9(3) | 2.0(4) | 2.2(3) | 2.2(3) | 2.7(3) | 2.9(4) | 2.6(3) | 2.6(3) | 2.4(3) | 2.0(3) | 2.3(4) |
| CN8c (M8c = K) 1 | FIX 2 | FIX 2 | 1.7 | 1.2 | 0.5 | 0 | 0 | 0.3 | 0.3 | 0.5 | 0.5 | 0.5 |
| S02 (Fe) | 0.66(4) | 0.65(4) | 0.66(3) | 0.66(4) | 0.66(3) | 0.65(3) | 0.66(3) | 0.65(3) | 0.66(3) | 0.66(3) | 0.69(6) | 0.65(3) |
| S02 (Co) | 0.71(3) | 0.76(4) | 0.77(4) | 0.73(3) | 0.72(3) | 0.74(3) | 0.73(4) | 0.78(4) | 0.72(4) | 0.72(3) | 0.73(3) | 0.72(3) |
| a/2/Å 2 | 5.12 | 5.1 | 5.11 | 5.1 | 5.1 | 5.09 | 5.09 | 5.1 | 5.1 | 5.1 | 5.1 | 5.1 |
| 106 χ2-like residual | 3.63 | 5.83 | 5.91 | 5.8 | 5.72 | 6.5 | 7.22 | 6.02 | 5.34 | 4.95 | 4.98 | 4.44 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mullaliu, A.; Conti, P.; Aquilanti, G.; Plaisier, J.R.; Stievano, L.; Giorgetti, M. Operando XAFS and XRD Study of a Prussian Blue Analogue Cathode Material: Iron Hexacyanocobaltate. Condens. Matter 2018, 3, 36. https://doi.org/10.3390/condmat3040036
Mullaliu A, Conti P, Aquilanti G, Plaisier JR, Stievano L, Giorgetti M. Operando XAFS and XRD Study of a Prussian Blue Analogue Cathode Material: Iron Hexacyanocobaltate. Condensed Matter. 2018; 3(4):36. https://doi.org/10.3390/condmat3040036
Chicago/Turabian StyleMullaliu, Angelo, Paolo Conti, Giuliana Aquilanti, Jasper Rikkert Plaisier, Lorenzo Stievano, and Marco Giorgetti. 2018. "Operando XAFS and XRD Study of a Prussian Blue Analogue Cathode Material: Iron Hexacyanocobaltate" Condensed Matter 3, no. 4: 36. https://doi.org/10.3390/condmat3040036
APA StyleMullaliu, A., Conti, P., Aquilanti, G., Plaisier, J. R., Stievano, L., & Giorgetti, M. (2018). Operando XAFS and XRD Study of a Prussian Blue Analogue Cathode Material: Iron Hexacyanocobaltate. Condensed Matter, 3(4), 36. https://doi.org/10.3390/condmat3040036







