Using Observed Residual Error Structure Yields the Best Estimates of Individual Growth Parameters
Abstract
1. Introduction
2. Materials and Methods
2.1. Modelling
- (a)
- Additive:
- (b)
- Multiplicative:
- (e)
- Observed [20]:
2.2. Criterion to Select the Best Error Structure
2.3. Confidence Intervals for Parameters
2.4. Growth Performance (Phi Prime) ϕ′
3. Results
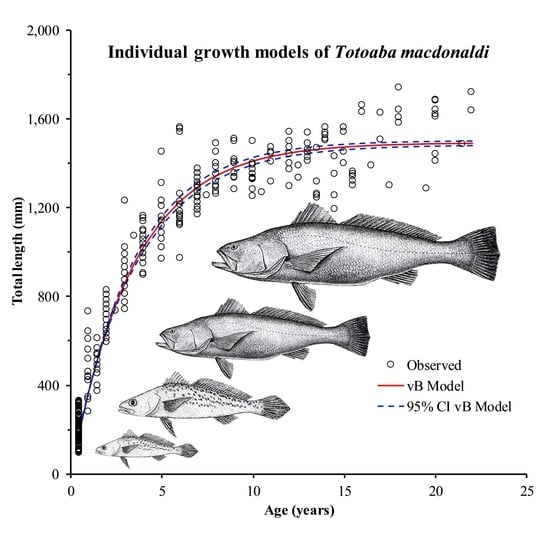
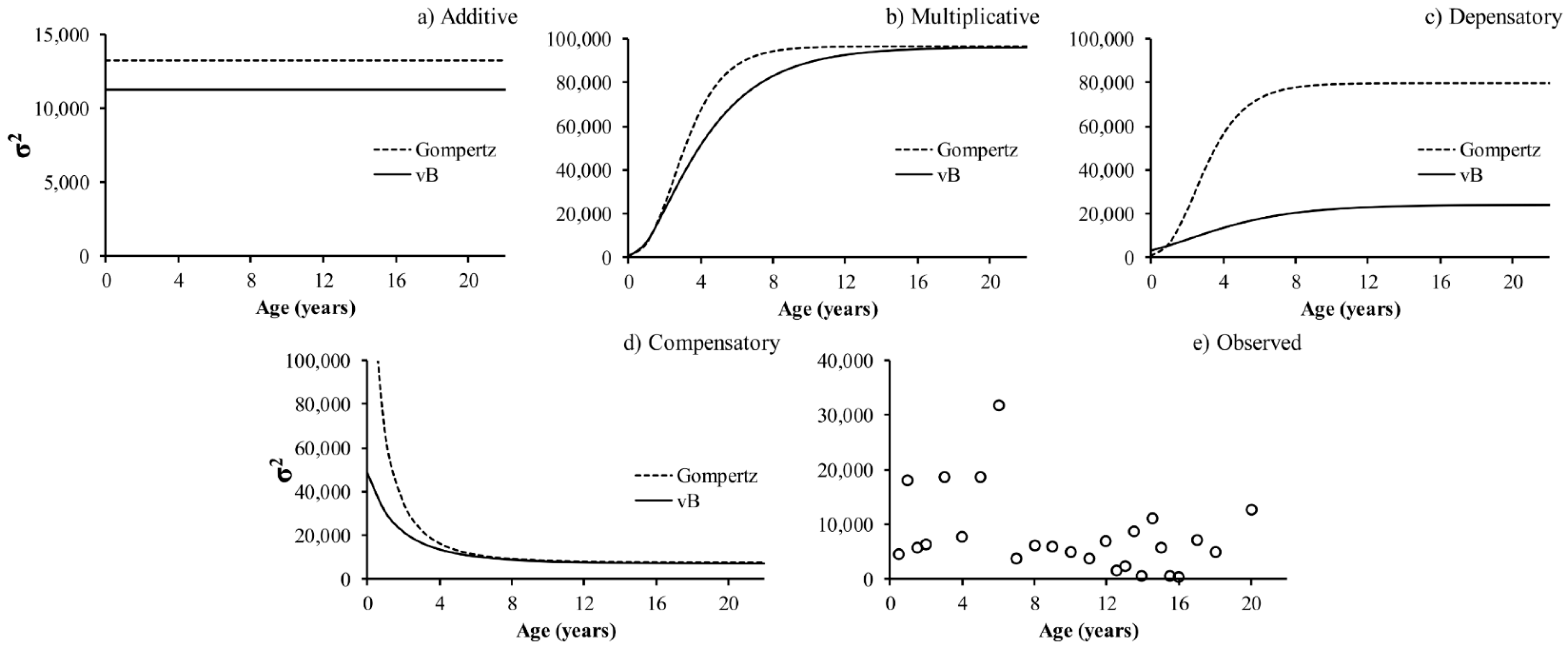
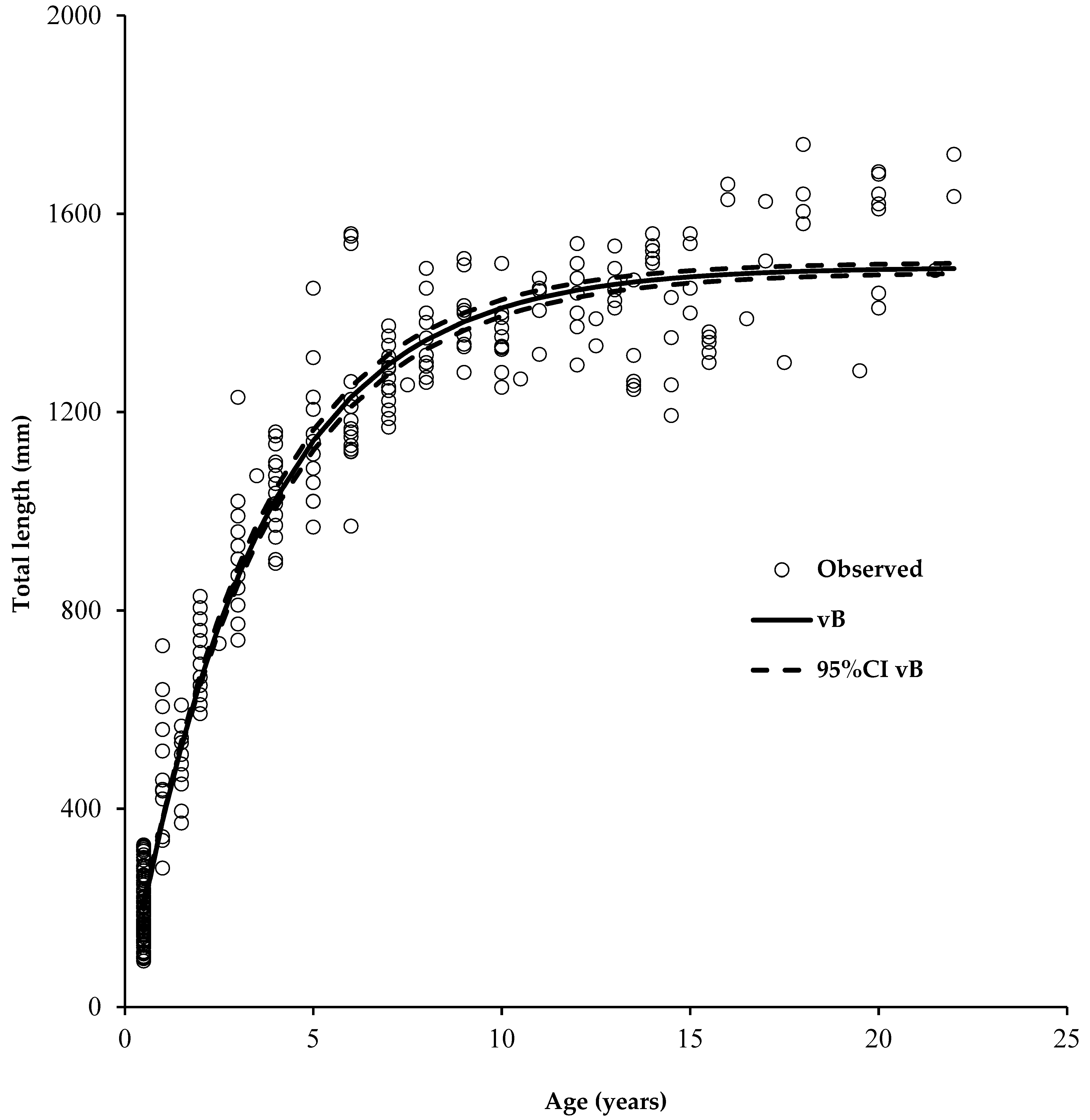
3.1. Observed Variability of Length-Age Data
3.2. Error Structure Used in Both Growth Models
3.3. Estimated Parameter Values for Different Error Structures
3.4. Computed ϕ′ Values
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
References
- Hutchinson, C.E.; TenBrink, T.T. Age determination of the yellow Irish lord: Management implications as a result of new estimates of maximum age. N. Am. J. Fish. Manag. 2011, 31, 1116–1122. [Google Scholar] [CrossRef]
- Lee, L.; Atkinson, D.; Hirst, A.G.; Cornell, S.J. A new framework for growth curve fitting based on the von Bertalanffy growth function. Sci. Rep. 2020, 10, 7953. [Google Scholar] [CrossRef] [PubMed]
- Lorenzen, K. A simple von Bertalanffy model for density-dependent growth in extensive aquaculture, with an application to common carp (Cyprinus carpio). Aquaculture 1996, 142, 191–205. [Google Scholar] [CrossRef]
- De Graaf, G.; Prein, M. Fitting growth with the von Bertalanffy growth function: A comparison of three approaches of multivariate analysis of fish growth in aquaculture experiments. Aquac. Res. 2005, 36, 100–109. [Google Scholar] [CrossRef]
- Brunel, T.; Dickey-Collas, M. Effects of temperature and population density on von Bertalanffy growth parameters in Atlantic herring: A macro-ecological analysis. Mar. Ecol. Prog. Ser. 2010, 405, 15–28. [Google Scholar] [CrossRef]
- Martínez-Jerónimo, F. Description of the individual growth of Daphnia magna (Crustacea: Cladocera) through the von Bertalanffy growth equation. Effect of photoperiod and temperature. J. Limnol. 2012, 13, 65–71. [Google Scholar] [CrossRef]
- Hilborn, R.; Walters, C.J. Quantitative Fish Stock Assessment: Choice, Dynamics and Uncertainty, 1st ed.; Chapman and Hall: New York, NY, USA, 1992; pp. 410–428. [Google Scholar]
- Von Bertalanffy, L. A quantitative theory of organic growth. Hum. Biol. 1938, 10, 181–213. [Google Scholar]
- Majkowski, J.; Uchmanski, J. Theoretical foundations of individual growth equations in animals. Pol. Ecol. Stud. 1980, 6, 7–31. [Google Scholar]
- Katsanevakis, S. Modelling fish growth: Model selection, multi-model inference and model selection uncertainty. Fish. Res. 2006, 81, 229–235. [Google Scholar] [CrossRef]
- Winsor, C.P. The Gompertz curve as a growth curve. Proc. Natl. Acad. Sci. USA 1932, 18, 1–8. [Google Scholar] [CrossRef]
- Gompertz, B. On the nature of the function expressive of the law of human mortality, and on a new mode of determining the value of life contingencies. Philos. Trans. R. Soc. Lond. 1825, 115, 513–583. [Google Scholar]
- Haddon, M. Modelling and Quantitative Methods in Fisheries, 2nd ed.; Chapman and Hall/CRC: London, UK, 2011. [Google Scholar]
- Burnham, K.P.; Anderson, D.R. Model Selection and Multi-Model Inference: A Practical Information-Theoretic Approach; Springer: New York, NY, USA, 2002. [Google Scholar]
- Flinn, S.A.; Midway, S.R. Trends in Growth Modeling in Fisheries Science. Fishes 2021, 6, 1. [Google Scholar] [CrossRef]
- Zhu, C.; Dong, S.L.; Wang, F. The interaction of salinity and the Na/K ratio in seawater on growth, nutrient retention and food conversion of Litopenaeus vannamei juveniles. J. Shellfish Res. 2006, 25, 107–112. [Google Scholar] [CrossRef]
- Myung, I.J. Tutorial on maximum likelihood estimation. J. Math. Psychol. 2003, 47, 90–100. [Google Scholar] [CrossRef]
- Schwarz, G. Estimating the dimension of a model. Ann. Stat. 1978, 6, 461–464. [Google Scholar] [CrossRef]
- Shono, H. Efficiency of the finite correction of Akaike’s information criteria. Fish. Sci. 2000, 66, 608–610. [Google Scholar] [CrossRef]
- Ratkowsky, D.A. Handbook of Nonlinear Regression Models; Marcel Dekker Inc.: New York, NY, USA, 1990. [Google Scholar]
- Sainsbury, K.J. Effect of individual variability on the von Bertalanffy growth equation. Can. J. Fish. Aquat. Sci. 1980, 37, 241–247. [Google Scholar] [CrossRef]
- Schnute, J.; Fournier, D. A new approach to length-frequency analysis: Growth structure. Can. J. Fish. Aquat. Sci. 1980, 37, 1337–1351. [Google Scholar] [CrossRef]
- Letcher, B.H.; Coombs, J.A.; Nislow, K.H. Maintenance of phenotypic variation: Repeatability, heritability and size-dependent processes in a wild brook trout population. Evol. Appl. 2011, 4, 602–615. [Google Scholar] [CrossRef] [PubMed]
- Batts, L.; Minto, C.; Gerritsen, H.; Brophy, D. Estimating growth parameters and growth variability from length frequency data using hierarchical mixture models. ICES J. Mar. Sci. 2019, 76, 2150–2163. [Google Scholar] [CrossRef]
- Restrepo, V.R.; Diaz, G.A.; Walter, J.F.; Neilson, J.D.; Campana, S.E.; Secor, D.; Wingate, R.L. Updated estimate of the growth curve of western Atlantic bluefin tuna. Aquat. Living Resour. 2010, 23, 335–342. [Google Scholar] [CrossRef]
- Luquin-Covarrubias, M.A.; Morales-Bojórquez, E.; González-Peláez, S.S.; Hidalgo-de la Toba, J.Á.; Lluch-Cota, D.B. Modeling of growth depensation of geoduck clam Panopea globosa based on a multimodel inference approach. J. Shellfish Res. 2016, 35, 379–387. [Google Scholar] [CrossRef]
- Félix-Ortiz, J.A.; Aragón-Noriega, E.A.; Castañeda-Lomas, N.; Rodríguez-Domínguez, G.; Valenzuela-Quiñónez, W.; Castillo-Vargasmachuca, S. Individual growth analysis of the Pacific yellowlegs shrimp Penaeus californiensis via multi-criteria approach. Lat. Am. J. Aquat. Res. 2020, 48, 768–778. [Google Scholar] [CrossRef]
- Gilbert, C.H. A preliminary report on the fishes collected by the streamer “Albatross” on the Pacific coast of North America during the year 1889, with descriptions of twelve new genera and ninety-two new species. Proc. US. Natl. Mus. 1891, 13, 49–126. [Google Scholar] [CrossRef]
- De Anda-Montañez, J.A.; García de León, F.J.; Zenteno-Savín, T.; Balart-Páez, E.; Méndez-Rodríguez, L.C.; Bocanegra-Castillo, N.; Martínez-Delgado, M.E. Estado de Salud y Estatus de Conservación de la(s) Población(es) de Totoaba (Totoaba Macdonaldi) en el Golfo de California: Una Especie en Peligro de Extinción; Informe Final, SNIB-CONABIO. Proyecto Núm. hK050; Centro de Investigaciones Biológicas del Noroeste, S.C.: La Paz, Mexico, 2013; Available online: http://www.conabio.gob.mx/institucion/proyectos/resultados/InfHK050.pdf (accessed on 3 February 2021).
- Barrera-Guevara, J.C. The conservation of Totoaba macdonaldi (Gilbert), (Pisces: Sciaenidae), in the Gulf of California, Mexico. J. Fish. Biol. 1990, 37, 201–202. [Google Scholar] [CrossRef]
- Valenzuela-Quiñonez, F. Genética y Dinámica Poblacional de la Totoaba (Totoaba macdonaldi Gilbert, 1891) en el Golfo de California. Ph.D. Thesis, Centro de Investigaciones Biológicas del Noroeste, S.C., La Paz, Mexico, 22 May 2014. [Google Scholar]
- Valenzuela-Quiñonez, F.; Arreguín-Sánchez, F.; Salas-Márquez, S.; García-de León, F.J.; Garza, J.C.; Román-Rodríguez, M.J.; de-Anda-Montañez, J.A. Critically endangered totoaba Totoaba macdonaldi: Signs of recovery and potential threats after a population collapse. Endanger. Species Res. 2015, 29, 1–11. [Google Scholar] [CrossRef]
- Valenzuela-Quiñonez, F.; de Anda-Montañez, J.A.; Gilbert-Horvath, E.; Garza, J.C.; García-De León, F.J. Panmixia in a critically endangered fish: The totoaba (Totoaba macdonaldi) in the Gulf of California. J. Hered. 2016, 107, 496–503. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Cisneros-Mata, M.Á. Evaluación de la población de Totoaba macdonaldi; Instituto Nacional de Pesca y Acuacultura: Ciudad de México, Mexico, 2020. [Google Scholar]
- Pauly, D.; Munro, L. Once more on growth comparison in fish and vertebrates. Fishbyte 1984, 2, 21. [Google Scholar]
- Nakashima, F. Cynoscion macdonaldi, Gilbert. Copeia 1916, 37, 85–86. [Google Scholar]
- Berdegué, J. La pesquería de totoaba (Cynoscion macdonaldi) en San Felipe, Baja California. Rev. Soc. Mex. Hist. Nat. 1955, 16, 45–78. [Google Scholar]
- Román-Rodríguez, M.; Hammann, M.G. Age and growth of totoaba, Totoaba macdonaldi (Sciaenidae) in the upper Gulf of California. Fish. Bull. 1997, 95, 620–628. [Google Scholar]
- Castillo-Vargasmachuca, S.G.; Ponce-Palafox, J.T.; Arámbul-Muñoz, E.; Rodríguez-Domínguez, G.; Aragón-Noriega, E.A. The spotted rose snapper (Lutjanus guttatus Steindachner 1869) farmed in marine cages: Review of growth models. Rev. Aquac. 2018, 10, 376–384. [Google Scholar] [CrossRef]
- Casella, G.; Berger, R.L. Statistical Inference, 2nd ed.; Duxbury Press: Pacific Grove, CA, USA, 2002. [Google Scholar]
- Venzon, D.J.; Moolgavkar, S.H. A method for computing profile-likelihood-based confidence intervals. Appl. Stat. 1988, 37, 7–94. [Google Scholar] [CrossRef]
- Ragonese, S.; Vitale, S.; Mazzola, S.; Pagliarino, E.; Bianchini, M.L. Behavior of some growth performance indexes for exploited Mediterranean hake. Acta Adriat. 2012, 53, 105–122. [Google Scholar]
- Pedrín-Osuna, O.; Córdova-Murueta, J.H.; Delgado-Marchena, M. Crecimiento y mortalidad de la totoaba, Totoaba macdonaldi, del Alto Golfo de California. Cienc. Pesq. 2001, 15, 131–140. [Google Scholar]
- Flanagan, C.A. Study of the feasibility of modeling the totoaba fishery of the northern Gulf of California with preliminary estimation of some critical parameters. Univ. Ariz. Biol. Sci. Rep. 1973, 249, 1–58. [Google Scholar]
- Molina-Valdez, D.; Cisneros-Mata, M.Á.; Urías-Sotomayor, R.; Cervantes-Valle, C.; Márquez-Tiburcio, M.Á. Prospección y Evaluación de la Totoaba (Totoaba macdonaldi) en el Golfo de California; Informe Final al Consejo Nacional de Ciencia y Tecnología de México. Instituto Nacional de la Pesca: Ciudad de Mexico, Mexico, 1988; Available from the third author. [Google Scholar]
- Cisneros-Mata, M.Á.; Montemayor-López, G.; Román-Rodríguez, M.J. Life history and conservation of Totoaba macdonaldi. Conserv. Biol. 1995, 9, 806–814. [Google Scholar] [CrossRef]
- Nyeste, K.; Kati, S.; Nagy, S.A.; Antal, L. Growth features of the Amur sleeper, Perccottus glenii (Actinopterygii: Perciformes: Odontobutidae), in the invaded Carpathian Basin, Hungary. Acta Ichthyol. Piscat. 2017, 47, 33–40. [Google Scholar] [CrossRef][Green Version]
- Chen, Y.; Fournier, D. Impacts of atypical data on Bayesian inference and robust Bayesian approach in fisheries. Can. J. Fish. Aquat. Sci. 1999, 56, 1525–1533. [Google Scholar] [CrossRef]
- Shelton, A.O.; Mangel, M. Estimating von Bertalanffy parameters with individual and environmental variations in growth. J. Biol. Dyn. 2012, 6, 3–30. [Google Scholar] [CrossRef] [PubMed]
- Pérez-Arvizu, E.M.; Aragón-Noriega, E.A.; Espinosa-Carreón, T.L. Variabilidad estacional de la clorofila a y su respuesta a condiciones El Niño y La Niña en el norte del Golfo de California. Rev. Biol. Mar. Ocean. 2013, 48, 131–141. [Google Scholar] [CrossRef]
| Case | n | θj | BIC | Wj |
|---|---|---|---|---|
| von Bertalanffy model | ||||
| Additive | 256 | 4 | 3136 | 0 |
| Multiplicative | 256 | 4 | 3298 | 0 |
| Depensatory | 256 | 4 | 3141 | 0 |
| Compensatory | 256 | 4 | 3217 | 0 |
| Observed | 256 | 29 | 2393 | 1.00 |
| Gompertz model | ||||
| Additive | 256 | 4 | 3178 | 0 |
| Multiplicative | 256 | 4 | 3325 | 0 |
| Depensatory | 256 | 4 | 3320 | 0 |
| Compensatory | 256 | 4 | 3317 | 0 |
| Observed | 256 | 29 | 2479 | 0 |
| Error Structure | (mm) | k/kg (year−1) | to/t1 (years) |
|---|---|---|---|
| von Bertalanffy model | |||
| Additive | (1466, 1484, 1502) | (0.29, 0.30, 0.31) | (−0.08, 0.0, 0.03) |
| Multiplicative | (1416, 1451, 1488) | (0.32, 0.33, 0.34) | (0.06, 0.07, 0.09) |
| Depensatory | (1450, 1470, 1489) | (0.30, 0.31, 0.33) | (−0.04, 0.0, 0.04) |
| Compensatory | (1488, 1499, 1515) | (0.27, 0.27, 0.29) | (−0.26, −0.17, −0.08) |
| Observed | (1482, 1492, 1502) | (0.28, 0.29, 0.30) | (− 0.06, 0.0, 0.03) |
| Gompertz model | |||
| Additive | (1420, 1439, 1456) | (0.46, 0.49, 0.52) | (1.58, 1.66, 1.75) |
| Multiplicative | (1340, 1373, 1409) | (0.66, 0.68, 0.70) | (1.44, 1.48, 1.51) |
| Depensatory | (1303, 1327, 1369) | (0.64, 0.67, 0.70) | (1.36, 1.40, 1.43) |
| Compensatory | (1461, 1477, 1494) | (0.33, 0.35, 0.37) | (1.07, 1.21, 1.35) |
| Observed | (1455, 1464, 1473) | (0.45, 0.47, 0.49) | (1.75, 1.81, 1.87) |
| Sampling Period | N | R | K (year−1) | (mm) | ϕ′ | ϕ′ Anomaly | Source |
|---|---|---|---|---|---|---|---|
| 1962 | 68 | 565–1586 | 0.17 | 1730 | 3.707 | −0.151 | [44] |
| 1985 | 244 | 1300–1930 | 0.12 | 2030 | 3.694 | −0.164 | [45] |
| 1986–1991 | 101 | 1000–1850 | 0.23 | 1290 | 3.583 | −0.275 | [38] |
| 1916–1994 | 1549 | 76–1540 | 0.15 | 1670 | 3.622 | −0.236 | [46] |
| 1983–1993 | 811 | 700–1890 | 0.17 | 2060 | 3.858 | 0.000 | [43] |
| 2010–2011 | 235 | 280–1860 | 0.22 | 1430 | 3.653 | −0.205 | [29] |
| 2010–2011 | 360 | 280–1860 | 0.13 | 1800 | 3.624 | −0.234 | [31] |
| 2010–2019 | 256 | 93–1685 | 0.29 | 1492 | 3.795 | −0.048 | This study’s best-fit (vB) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Curiel-Bernal, M.V.; Aragón-Noriega, E.A.; Cisneros-Mata, M.Á.; Sánchez-Velasco, L.; Jiménez-Rosenberg, S.P.A.; Parés-Sierra, A. Using Observed Residual Error Structure Yields the Best Estimates of Individual Growth Parameters. Fishes 2021, 6, 35. https://doi.org/10.3390/fishes6030035
Curiel-Bernal MV, Aragón-Noriega EA, Cisneros-Mata MÁ, Sánchez-Velasco L, Jiménez-Rosenberg SPA, Parés-Sierra A. Using Observed Residual Error Structure Yields the Best Estimates of Individual Growth Parameters. Fishes. 2021; 6(3):35. https://doi.org/10.3390/fishes6030035
Chicago/Turabian StyleCuriel-Bernal, Marcelo V., E. Alberto Aragón-Noriega, Miguel Á. Cisneros-Mata, Laura Sánchez-Velasco, S. Patricia A. Jiménez-Rosenberg, and Alejandro Parés-Sierra. 2021. "Using Observed Residual Error Structure Yields the Best Estimates of Individual Growth Parameters" Fishes 6, no. 3: 35. https://doi.org/10.3390/fishes6030035
APA StyleCuriel-Bernal, M. V., Aragón-Noriega, E. A., Cisneros-Mata, M. Á., Sánchez-Velasco, L., Jiménez-Rosenberg, S. P. A., & Parés-Sierra, A. (2021). Using Observed Residual Error Structure Yields the Best Estimates of Individual Growth Parameters. Fishes, 6(3), 35. https://doi.org/10.3390/fishes6030035