Long-Term Stability of Gradient Characteristics Warrants Model-Based Correction of Diffusion Weighting Bias
Abstract
:1. Introduction
2. Materials and Methods
2.1. DWI Phantom
2.2. MR Imaging Protocol
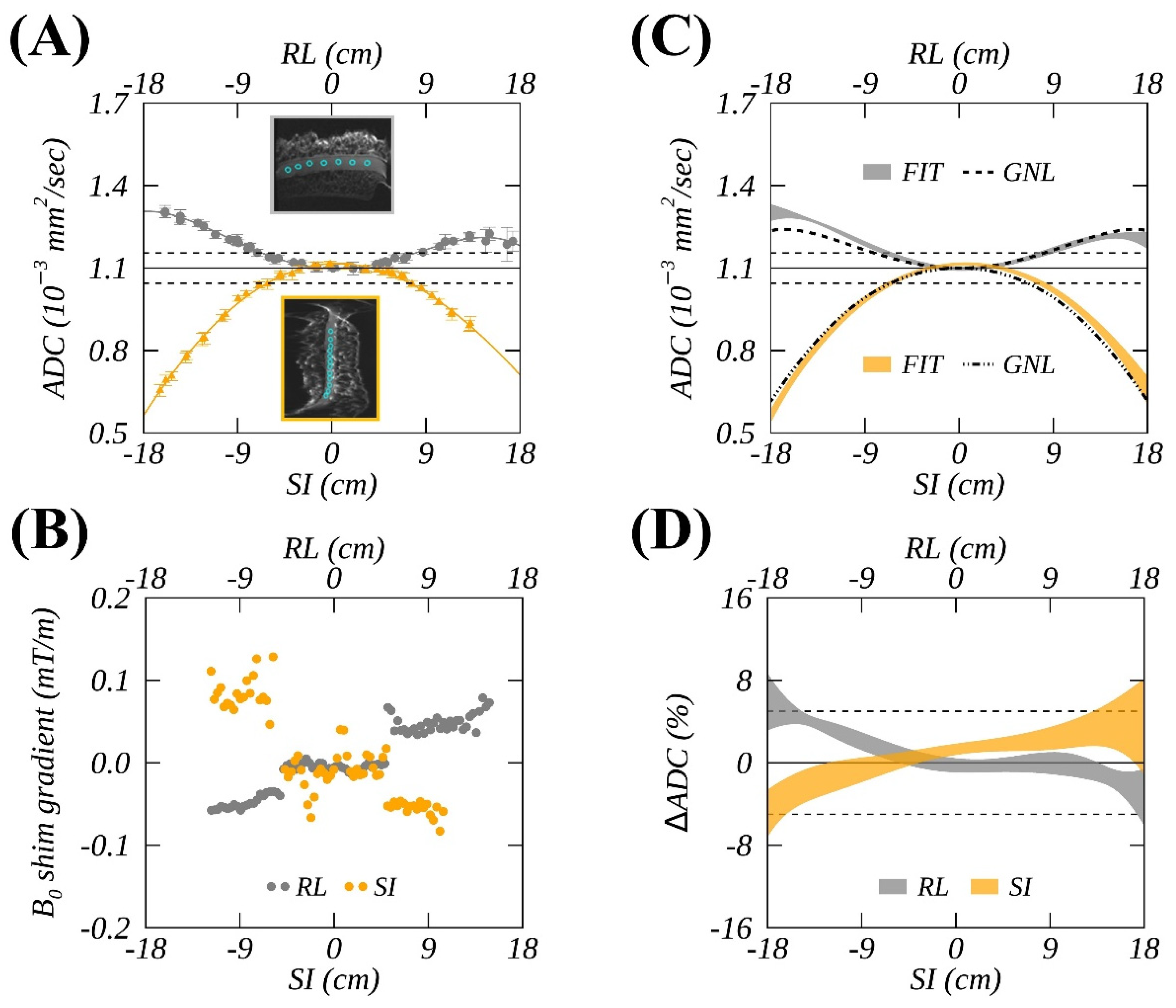
2.3. Spatial ADC Measurements
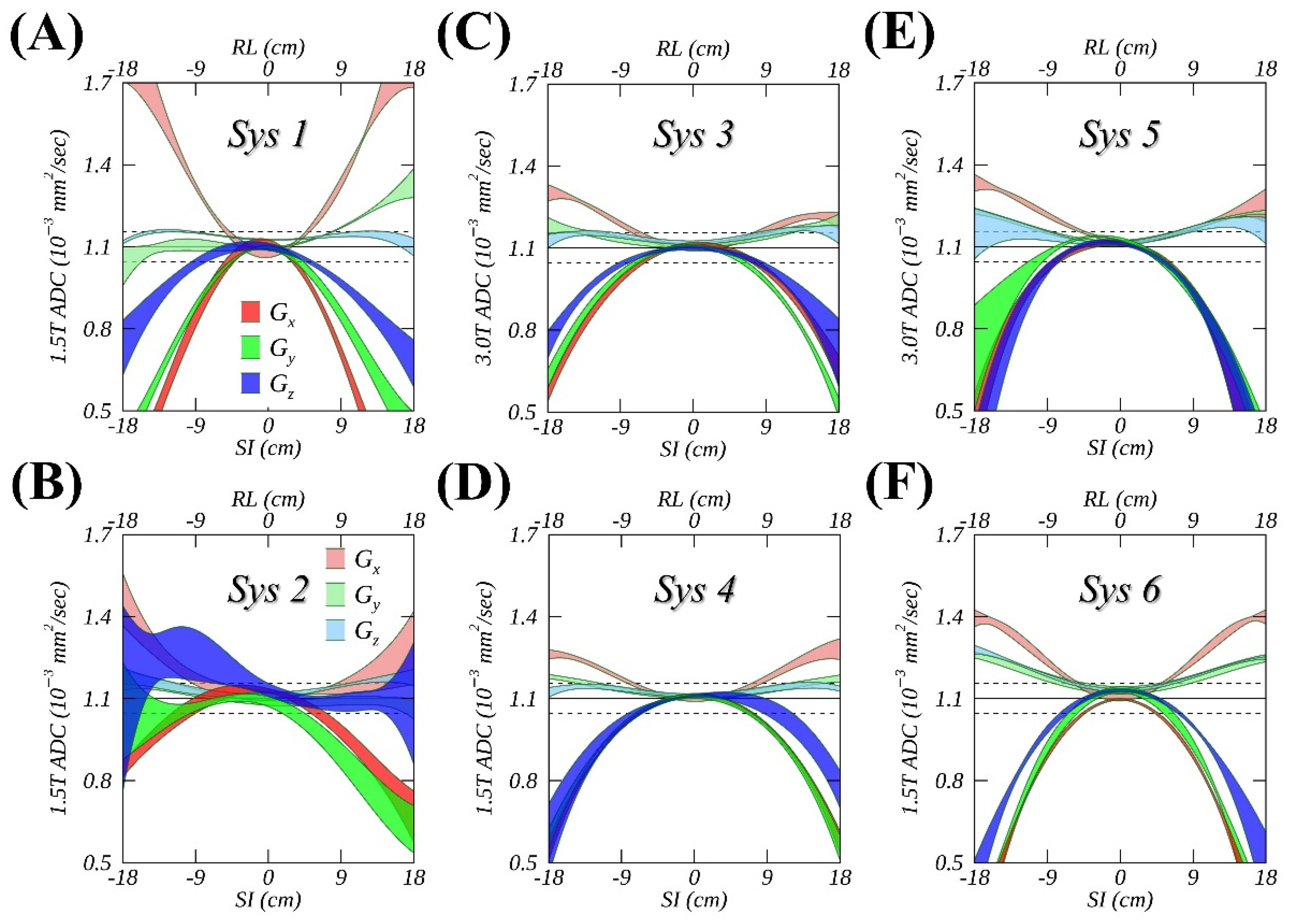
2.4. Theoretical Spatial ADC Based on GNL Model
2.5. Data Analysis
3. Results
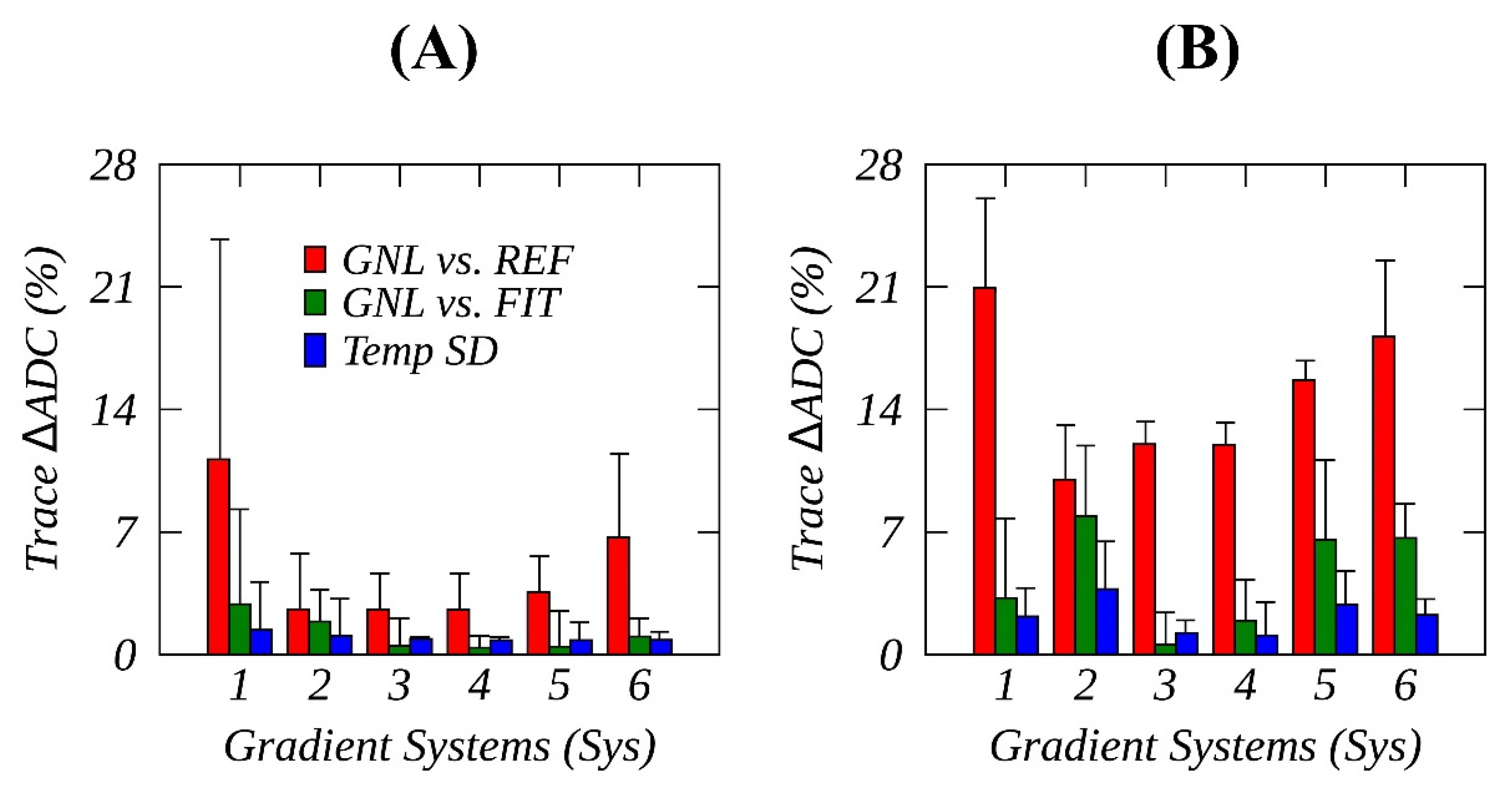
3.1. Temporal Variations in Spatial ADC Measurements
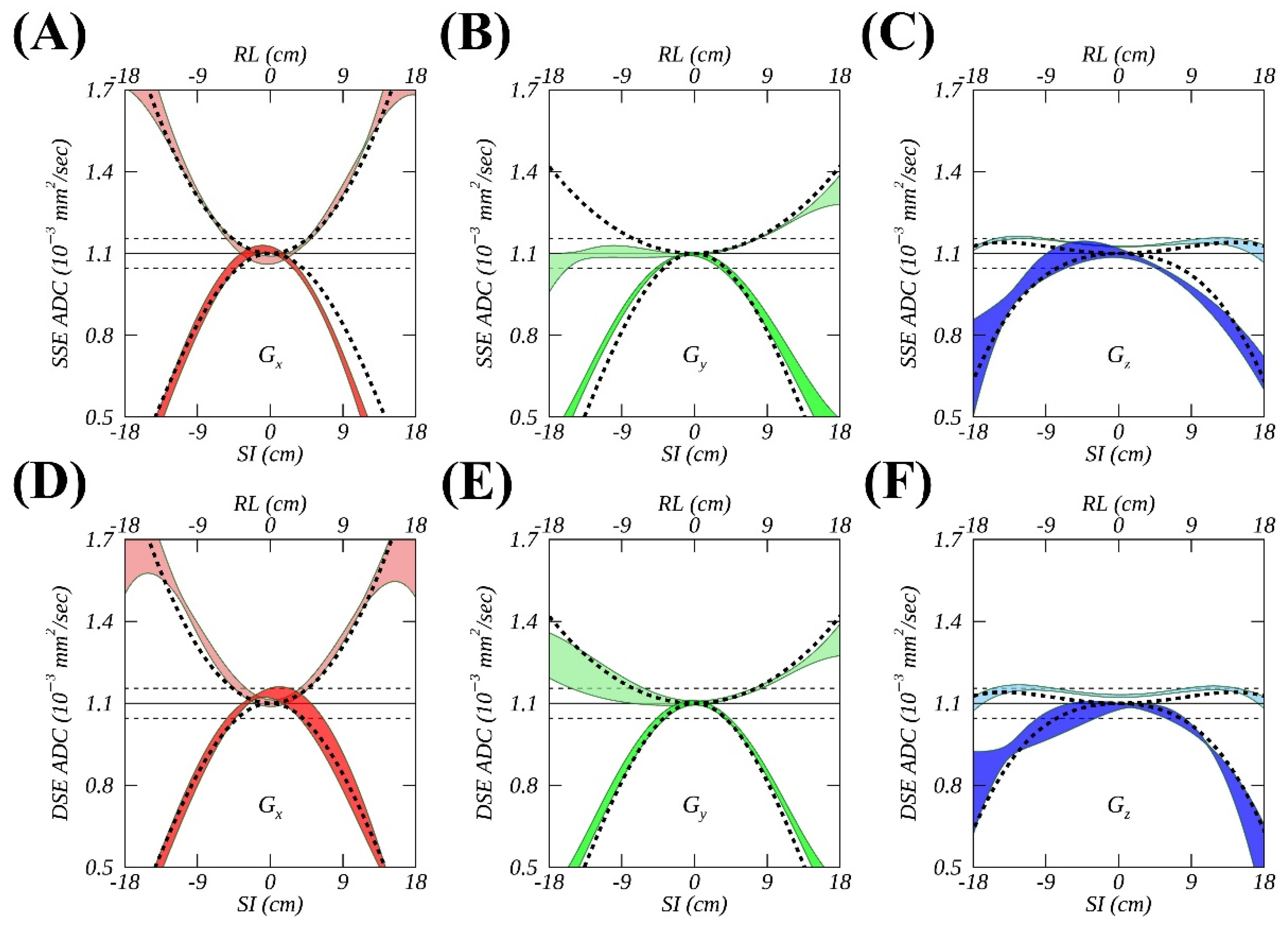
3.2. ADC Measurements from SSE and DSE
3.3. RMS Comparison of ADC Measurements
4. Discussion
5. Conclusions
6. Patents
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bammer, R.; Markl, M.; Barnett, A.; Acar, B.; Alley, M.T.; Pelc, N.J.; Glover, G.H.; Moseley, M.E. Analysis and generalized correction of the effect of spatial gradient field distortions in diffusion-weighted imaging. Magn. Reson. Med. 2003, 50, 560–569. [Google Scholar] [CrossRef] [PubMed]
- Tan, E.T.; Marinelli, L.; Slavens, Z.W.; King, K.F.; Hardy, C.J. Improved correction for gradient nonlinearity effects in diffusion-weighted imaging. J. Magn. Reson. Imaging 2013, 38, 448–453. [Google Scholar] [CrossRef] [PubMed]
- Malyarenko, D.I.; Ross, B.D.; Chenevert, T.L. Analysis and correction of gradient nonlinearity bias in apparent diffusion coefficient measurements. Magn. Reson. Med. 2014, 71, 1312–1323. [Google Scholar] [CrossRef] [PubMed]
- Barkovich, E.J.; Shankar, P.R.; Westphalen, A.C. A systematic review of the existing prostate imaging reporting and data system version 2 (PI-RADSv2) literature and subset meta-analysis of PI-RADSv2 categories stratified by Gleason scores. Am. J. Roentgenol. 2019, 212, 847–854. [Google Scholar] [CrossRef]
- Partridge, S.C.; Zhang, Z.; Newitt, D.C.; Gibbs, J.E.; Chenevert, T.L.; Rosen, M.A.; Bolan, P.J.; Marques, H.S.; Romanoff, J.; Cimino, L.; et al. Diffusion-weighted MRI Findings Predict Pathologic Response in Neoadjuvant Treatment of Breast Cancer: The ACRIN 6698 Multicenter Trial. Radiology 2018, 289, 618–627. [Google Scholar] [CrossRef]
- DeSouza, N.; Winfield, J.; Waterton, J.C.; Weller, A.; Papoutsaki, M.-V.; Doran, S.; Collins, D.; Fournier, L.; Sullivan, D.; Chenevert, T. Implementing diffusion-weighted MRI for body imaging in prospective multicentre trials: Current considerations and future perspectives. Eur. Radiol. 2018, 28, 1118–1131. [Google Scholar] [CrossRef]
- Newitt, D.C.; Tan, E.T.; Wilmes, L.J.; Chenevert, T.L.; Kornak, J.; Marinelli, L.; Hylton, N. Gradient nonlinearity correction to improve apparent diffusion coefficient accuracy and standardization in the american college of radiology imaging network 6698 breast cancer trial. J. Magn. Reson. Imaging 2015, 42, 908–919. [Google Scholar] [CrossRef]
- Barnes, A.; Alonzi, R.; Blackledge, M.; Charles-Edwards, G.; Collins, D.J.; Cook, G.; Coutts, G.; Goh, V.; Graves, M.; Kelly, C. UK quantitative WB-DWI technical workgroup: Consensus meeting recommendations on optimisation, quality control, processing and analysis of quantitative whole-body diffusion-weighted imaging for cancer. Br. J. Radiol. 2018, 91, 20170577. [Google Scholar] [CrossRef] [PubMed]
- Mulkern, R.V.; Ricci, K.I.; Vajapeyam, S.; Chenevert, T.L.; Malyarenko, D.I.; Kocak, M.; Poussaint, T.Y. Pediatric brain tumor consortium multisite assessment of apparent diffusion coefficient z-axis variation assessed with an ice-water phantom. Acad. Radiol. 2015, 22, 363–369. [Google Scholar] [CrossRef]
- Malyarenko, D.I.; Newitt, D.; Wilmes, L.J.; Tudorica, A.; Helmer, K.G.; Arlinghaus, L.R.; Jacobs, M.A.; Jajamovich, G.; Taouli, B.; Yankeelov, T.E.; et al. Demonstration of nonlinearity bias in the measurement of the apparent diffusion coefficient in multicenter trials. Magn. Reson. Med. 2016, 75, 1312–1323. [Google Scholar] [CrossRef]
- Mesri, H.Y.; David, S.; Viergever, M.A.; Leemans, A. The adverse effect of gradient nonlinearities on diffusion MRI: From voxels to group studies. NeuroImage 2020, 205, 116127. [Google Scholar] [CrossRef] [PubMed]
- Fedeli, L.; Belli, G.; Ciccarone, A.; Coniglio, A.; Esposito, M.; Giannelli, M.; Mazzoni, L.N.; Nocetti, L.; Sghedoni, R.; Tarducci, R. Dependence of apparent diffusion coefficient measurement on diffusion gradient direction and spatial position–A quality assurance intercomparison study of forty-four scanners for quantitative diffusion-weighted imaging. Phys. Med. 2018, 55, 135–141. [Google Scholar] [CrossRef] [PubMed]
- Malyarenko, D.; Newitt, D.; Amouzandeh, G.; Wilmes, L.J.; Tan, E.T.; Marinelli, L.; Deveraj, A.; Peeters, J.; vom Endt, A.; Hylton, N.; et al. Retrospective correction of ADC for gradient nonlinearity errors in multi-center breast DWI trials: ACRIN6698 multiplatform feasibility study. Tomography 2020, 6, 86–92. [Google Scholar] [CrossRef] [PubMed]
- Pang, Y.; Malyarenko, D.I.; Amouzandeh, G.; Barberi, E.; Cole, M.; Vom Endt, A.; Peeters, J.; Tan, E.T.; Chenevert, T.L. Empirical validation of gradient field models for an accurate ADC measured on clinical 3T MR systems in body oncologic applications. Phys. Med. 2021, 86, 113–120. [Google Scholar] [CrossRef] [PubMed]
- Janke, A.; Zhao, H.; Cowin, G.J.; Galloway, G.J.; Doddrell, D.M. Use of spherical harmonic deconvolution methods to compensate for nonlinear gradient effects on MRI images. Magn. Reson. Med. 2004, 52, 115–122. [Google Scholar] [CrossRef] [PubMed]
- Wang, D.; Doddrell, D.M. Geometric distortion in structural magnetic resonance imaging. Curr. Med. Imaging 2005, 1, 49–60. [Google Scholar] [CrossRef]
- Barnett, A.S.; Irfanoglu, M.O.; Landman, B.; Rogers, B.; Pierpaoli, C. Mapping gradient nonlinearity and miscalibration using diffusion-weighted MR images of a uniform isotropic phantom. Magn. Reson. Med. 2021, 86, 3259–3273. [Google Scholar] [CrossRef] [PubMed]
- Chenevert, T.L.; Galban, C.J.; Ivancevic, M.K.; Rohrer, S.E.; Londy, F.J.; Kwee, T.C.; Meyer, C.R.; Johnson, T.D.; Rehemtulla, A.; Ross, B.D. Diffusion coefficient measurement using a temperature-controlled fluid for quality control in multicenter studies. J. Magn. Reson. Imaging 2011, 34, 983–987. [Google Scholar] [CrossRef] [PubMed]
- Lee, Y.; Kettinger, A.O.; Wilm, B.J.; Deichmann, R.; Weiskopf, N.; Lambert, C.; Pruessmann, K.P.; Nagy, Z. A comprehensive approach for correcting voxel-wise b-value errors in diffusion MRI. Magn. Reson. Med. 2020, 83, 2173–2184. [Google Scholar] [CrossRef]
- Holz, M.; Heil, S.R.; Sacco, A. Temperature-dependent self-diffusion coefficients of water and six selected molecular liquids for calibration in accurate H-1 NMR PFG measurements. Phys. Chem. Chem. Phys. 2000, 2, 4740–4742. [Google Scholar] [CrossRef]
- Tadic, T.; Jaffray, D.A.; Stanescu, T. Harmonic analysis for the characterization and correction of geometric distortion in MRI. Med. Phys. 2014, 41, 112303. [Google Scholar] [CrossRef] [PubMed]
- Rohde, G.K.; Barnett, A.S.; Basser, P.J.; Marenco, S.; Pierpaoli, C. Comprehensive approach for correction of motion and distortion in diffusion-weighted MRI. Magn. Reson. Med. 2004, 51, 103–114. [Google Scholar] [CrossRef] [PubMed]
- Pang, Y.; Malyarenko, D.; Schar, M.; Wilmes, L.J.; Newitt, D.; Jacobs, M.; Chenevert, T.L. Characterization of B0 Shim-Induced Bias in Diffusion Weighting Gradients. In Proceedings of the ISMRM’25 Proceedings, Honolulu HI, USA, 22–27 April 2017; p. 3360. [Google Scholar]
- Barnhart, H.X.; Barboriak, D.P. Applications of the repeatability of quantitative imaging biomarkers: A review of statistical analysis of repeat data sets. Transl. Oncol. 2009, 2, 231–235. [Google Scholar] [CrossRef] [PubMed]
- Chenevert, T.L.; Malyarenko, D.I. Use of 2D image registration parameters for correction of eddy-current magnetizationdensity ADC errors in presence of gradient nonlinearity. In Proceedings of the ISMRM’28 Proceedings, Sydney, Australia, 8–14 August 2020; p. 4355, (virtual due to COVID). [Google Scholar]
| Sys | Grad | RL | SI | ||||
|---|---|---|---|---|---|---|---|
| REF | EXP | TMP | REF | EXP | TMP | ||
| 1 | x | 23.7 | 1.9 | 4.2 | 26.1 | 7.8 | 3.8 |
| y | 8.7 | 8.3 | 1.3 | 24.7 | 4.2 | 2.3 | |
| z | 1.3 | 1.2 | 0.6 | 12.2 | 3.4 | 2.5 | |
| t | 11.2 | 2.9 | 1.4 | 20.9 | 3.2 | 2.2 | |
| 2 | x | 5.8 | 3.7 | 3.2 | 13.0 | 5.6 | 2.5 |
| y | 2.3 | 2.5 | 1.4 | 13.1 | 11.9 | 4.2 | |
| z | 0.4 | 2.5 | 1.1 | 3.9 | 7.0 | 6.5 | |
| t | 2.6 | 1.9 | 1.1 | 10.0 | 7.9 | 3.7 | |
| 3 | x | 4.6 | 2.1 | 1.0 | 13.3 | 2.1 | 1.3 |
| y | 2.1 | 0.7 | 1.0 | 13.2 | 2.4 | 1.1 | |
| z | 1.1 | 0.6 | 0.9 | 9.6 | 0.6 | 2.0 | |
| t | 2.6 | 0.5 | 0.9 | 12.0 | 0.6 | 1.2 | |
| 4 | x | 4.6 | 1.1 | 1.0 | 13.2 | 1.5 | 0.9 |
| y | 2.1 | 0.2 | 0.8 | 13.1 | 1.4 | 1.1 | |
| z | 1.1 | 0.2 | 0.8 | 9.6 | 4.3 | 3.0 | |
| t | 2.6 | 0.4 | 0.8 | 12.0 | 1.9 | 1.1 | |
| 5 | x | 5.6 | 2.5 | 1.0 | 16.8 | 7.6 | 3.8 |
| y | 3.7 | 0.7 | 0.5 | 16.8 | 4.1 | 3.3 | |
| z | 1.6 | 1.1 | 1.8 | 13.5 | 11.1 | 4.8 | |
| t | 3.6 | 0.5 | 0.8 | 15.7 | 6.6 | 2.9 | |
| 6 | x | 11.5 | 2.1 | 1.2 | 22.5 | 5.1 | 1.5 |
| y | 5.3 | 1.8 | 1.3 | 22.2 | 6.8 | 3.2 | |
| z | 3.3 | 1 | 0.4 | 9.7 | 8.6 | 2.6 | |
| t | 6.7 | 1 | 0.8 | 18.2 | 6.7 | 2.3 | |
| Grad | x | y | z | t | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| RMSD | REF | EXP | TMP | REF | EXP | TMP | REF | EXP | TMP | REF | EXP | TMP | |
| RL | Median | 5.6 | 2.1 | 1 | 3.7 | 0.7 | 1 | 1.3 | 1 | 0.8 | 3.6 | 0.5 | 0.8 |
| Min | 4.6 | 1.1 | 1 | 2.1 | 0.2 | 0.5 | 1.1 | 0.2 | 0.4 | 2.6 | 0.4 | 0.8 | |
| Max | 23.7 | 2.5 | 4.2 | 8.7 | 8.3 | 1.3 | 3.3 | 1.2 | 1.8 | 11.2 | 2.9 | 1.4 | |
| SI | Median | 16.8 | 5.1 | 1.5 | 16.8 | 4.1 | 2.3 | 9.7 | 4.3 | 2.6 | 15.7 | 3.2 | 2.2 |
| Min | 13.2 | 1.5 | 0.9 | 13.1 | 1.4 | 1.1 | 9.6 | 0.6 | 2 | 12 | 0.6 | 1.1 | |
| Max | 26.1 | 7.8 | 3.8 | 24.7 | 6.8 | 3.3 | 13.5 | 11.1 | 4.8 | 20.9 | 6.7 | 2.9 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pang, Y.; Malyarenko, D.I.; Wilmes, L.J.; Devaraj, A.; Tan, E.T.; Marinelli, L.; Endt, A.v.; Peeters, J.; Jacobs, M.A.; Newitt, D.C.; et al. Long-Term Stability of Gradient Characteristics Warrants Model-Based Correction of Diffusion Weighting Bias. Tomography 2022, 8, 364-375. https://doi.org/10.3390/tomography8010030
Pang Y, Malyarenko DI, Wilmes LJ, Devaraj A, Tan ET, Marinelli L, Endt Av, Peeters J, Jacobs MA, Newitt DC, et al. Long-Term Stability of Gradient Characteristics Warrants Model-Based Correction of Diffusion Weighting Bias. Tomography. 2022; 8(1):364-375. https://doi.org/10.3390/tomography8010030
Chicago/Turabian StylePang, Yuxi, Dariya I. Malyarenko, Lisa J. Wilmes, Ajit Devaraj, Ek T. Tan, Luca Marinelli, Axel vom Endt, Johannes Peeters, Michael A. Jacobs, David C. Newitt, and et al. 2022. "Long-Term Stability of Gradient Characteristics Warrants Model-Based Correction of Diffusion Weighting Bias" Tomography 8, no. 1: 364-375. https://doi.org/10.3390/tomography8010030
APA StylePang, Y., Malyarenko, D. I., Wilmes, L. J., Devaraj, A., Tan, E. T., Marinelli, L., Endt, A. v., Peeters, J., Jacobs, M. A., Newitt, D. C., & Chenevert, T. L. (2022). Long-Term Stability of Gradient Characteristics Warrants Model-Based Correction of Diffusion Weighting Bias. Tomography, 8(1), 364-375. https://doi.org/10.3390/tomography8010030






