Coniferous Trees as Bioinspiration for Designing Long Reinforced Prestressed Concrete Columns
Abstract
1. Introduction
2. Analysis and Abstraction of the Biological Model
2.1. Biomimetics and Trees
2.2. Dynamics and Damping
2.3. Morphology and Deformation Capacity
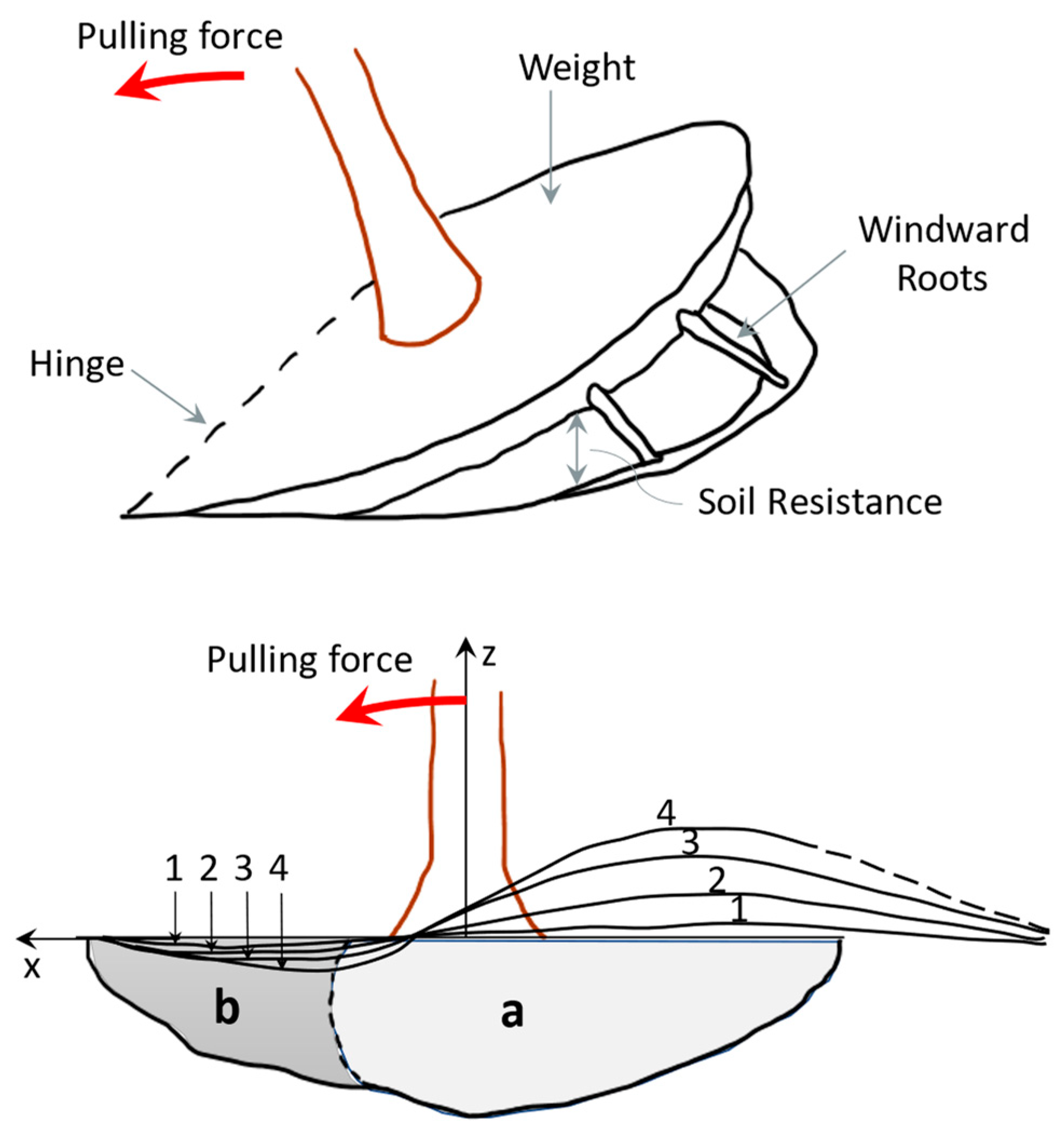
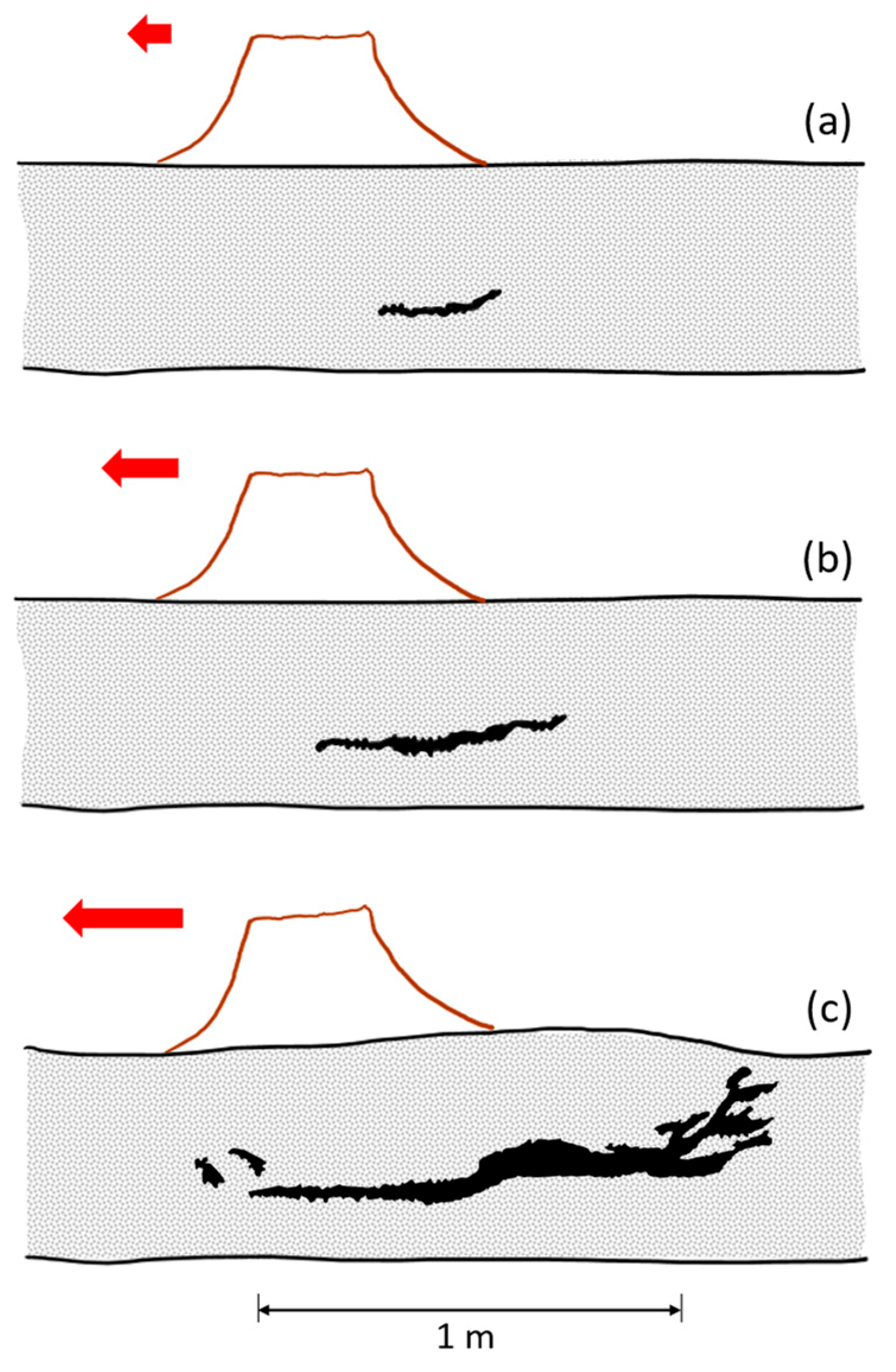
2.4. The Root System: Reaction Forces (Tree–Ground Anchorage Forces)
- Structure and mechanical properties of the roots;
- Spatial distribution and way of anchoring of the roots;
- The structure and physical/mechanical properties of the soil, of which moisture plays an essential role;
- The interaction between the roots and the surrounding soil.
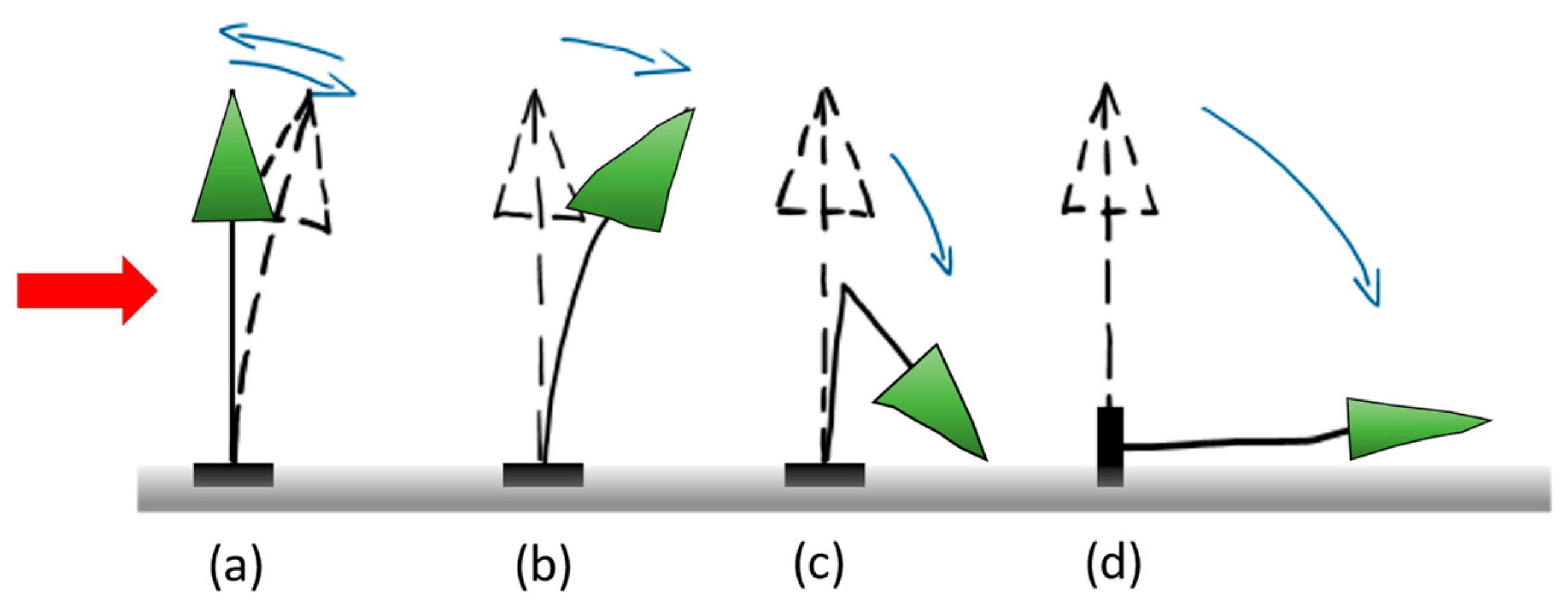
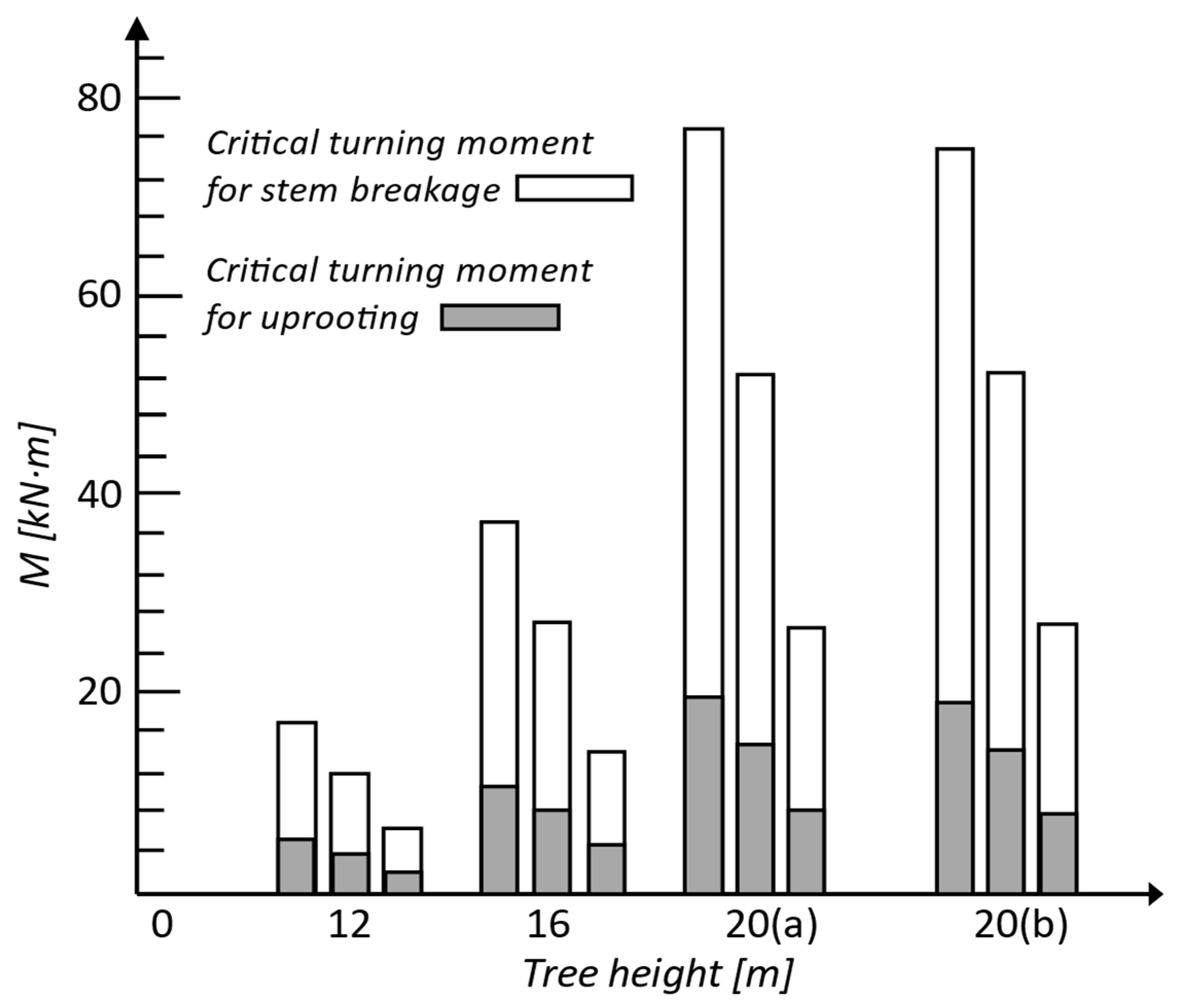
2.5. Failure of Trees under Wind Action
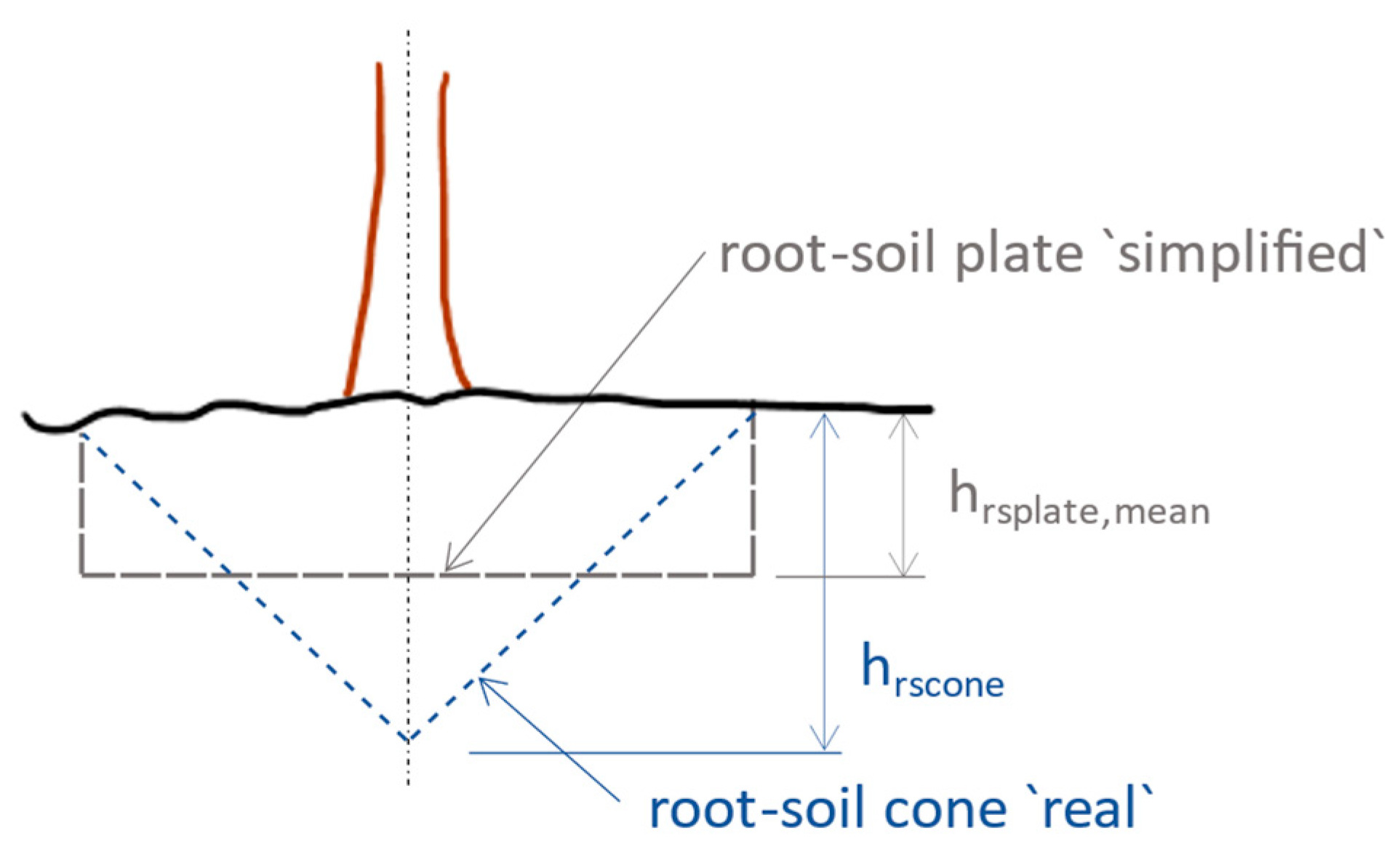
2.6. The Biomechanical Model of Conifers
- Elevation, consisting of stump, trunk, and crown;
- Foundation, consisting of roots and related soil, called the root–soil system.
2.7. Study Site
3. Design Methodology Transfer from Coniferous Trees to Load-Bearing Structures
3.1. Steps from Biomimetics to Know-How Transfer
3.2. Step 1: Identifying the Structural Characteristics of Coniferous Forest Trees
- Trees are three-dimensional structures statically determined;
- From the building statics’ perspective, trees are vertical cantilevers;
- In a tree, the values of internal forces due to its own weight are minimal in relation to the external forces caused by wind and/or snow;
- All the elements of a tree are made of the same material, but the chemical composition, density, and mechanical properties can vary, and the load-bearing capacity varies along the element depending on the size of the applying force in that cross-section;
- Trees are believed to have a minimum mass structure with elements optimized for function and shape;
- The lack of mechanical ductility of the trees is compensated by greater flexibility and damping;
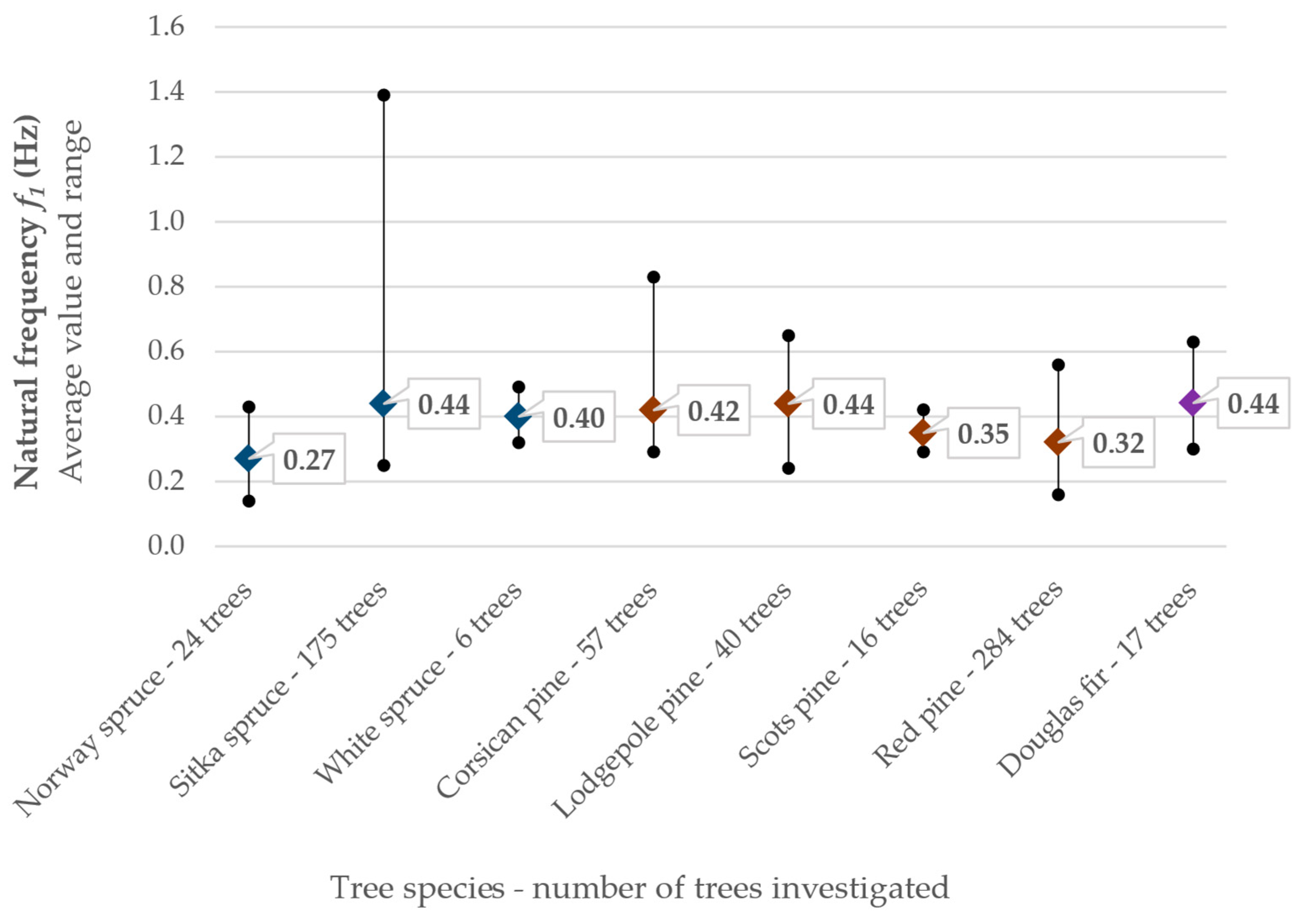
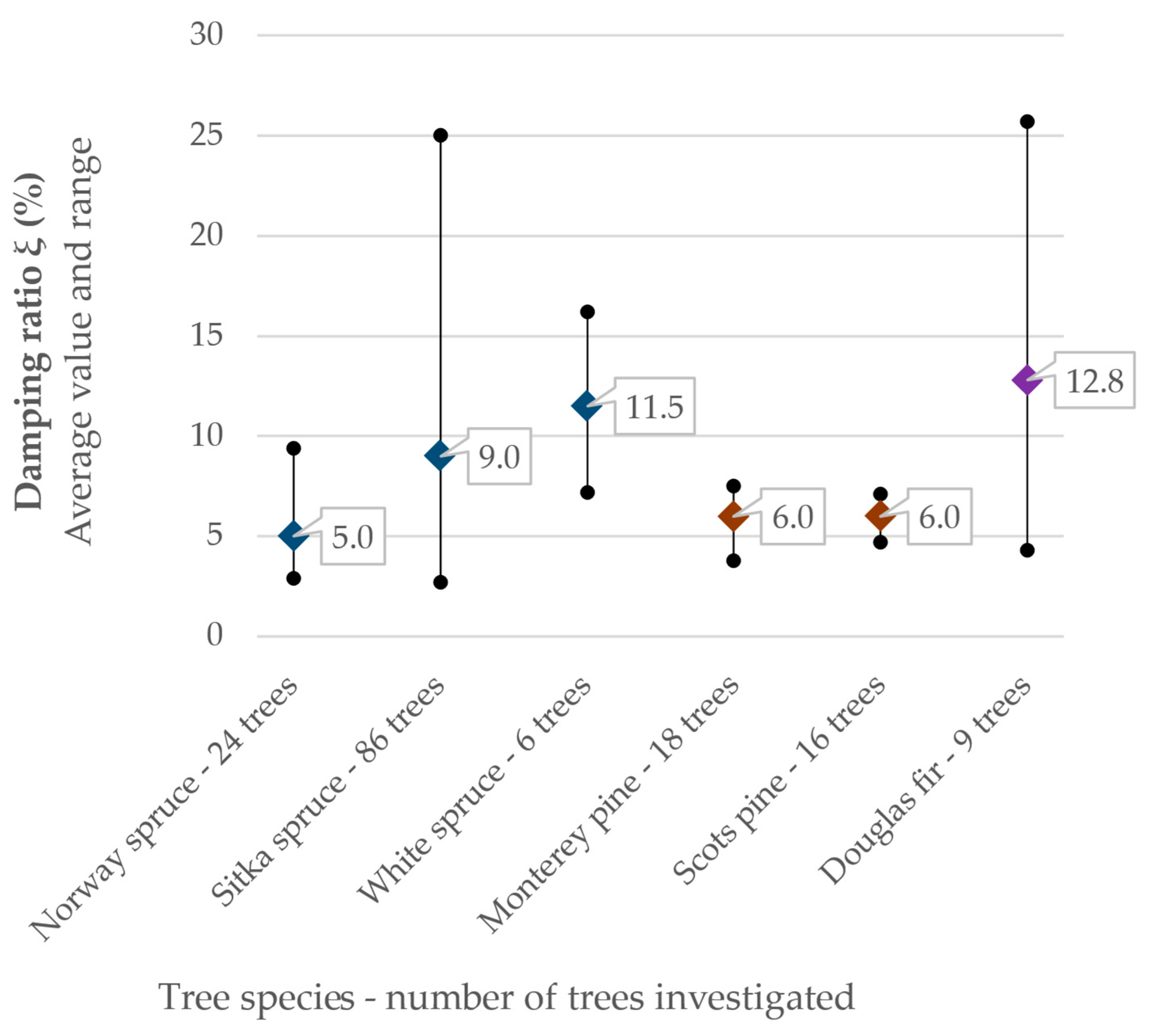
- The average fraction of the critical damping, ξ, lies between 5% and 12.8%;
- Trees maintain relatively large lateral displacements in extreme wind conditions;
- Tree joints can have a quasi-plastic response to extreme loads;
- Tree joints are endowed with a higher tenacity than that of the trunk and branches;
- Trees are systems with several degrees of freedom and with high damping;
- Trees in the same stand, although they have different heights, have the same natural frequency;
- Due to the high damping capacity and the multitude of independently vibrating elements (leaves and branches), trees rarely enter resonance;
- Tree trunks are naturally prestressed in both directions, longitudinally and circumferentially;
- Tree roots are thus designed to deform and uplift to a certain extent to prevent permanent damage to the base of the trunk.
- The slenderness of the spruce trunk is 5 times higher than that of the columns for low seismicity regions (ag = 0.10 g) and 10 times higher than that of the columns for high seismicity regions (ag = 0.30 g);
- The ratio between the weight of the crown and that of the stem (Wcrown/Wstem = 0.5) is 24 times smaller than the ratio of roof’s total loads (including self-weight) and column weight (Wroof/Wcolumn = 12) for areas with low seismicity and 8 times smaller (Wroof/Wcolumn = 4) for areas with high seismicity;
- The natural period of vibration for trees is between 10.0 and 2.0 s, while for a single-story warehouse it is between 2.3 and 0.7 s;
- The alternation of synchronous and asynchronous oscillations of the branches with the effect of dissipating the energy induced by wind or earthquake actions contrast with the movement of the roof beams connected to the column;
- The root system is a hybrid between a shallow and a deep foundation (with individual footing and ground anchors), while for reinforced concrete columns such a solution would be too expensive, being used especially for special structures such as towers for wind turbines. In general, nowadays, the common solutions used for the foundations of the columns in single-story warehouses are either a shallow foundation as individual footing or a deep foundation with individual footing sitting on piles.
4. Features of the Biological Role Model Meant to Be Abstracted and Later Transferred
- Structural applicability (geometric similarities and use and behavior of materials);
- Functional similarity (similar loading conditions and similar climatic actions);
- Similar structural response (behaving in the same way under comparable external actions);
- Cost efficient (being as profitable as possible in terms of material and energy consumption and production costs).
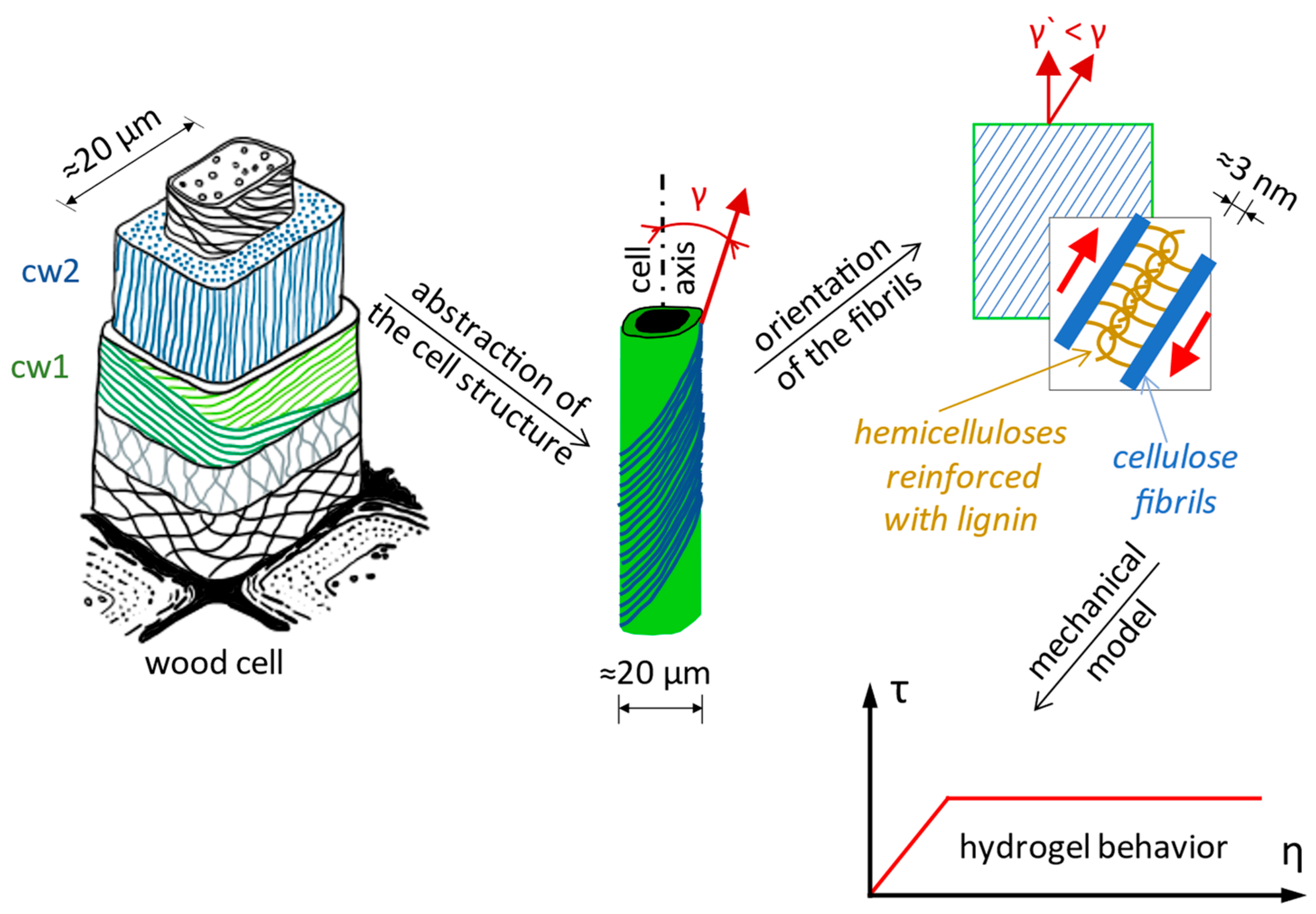
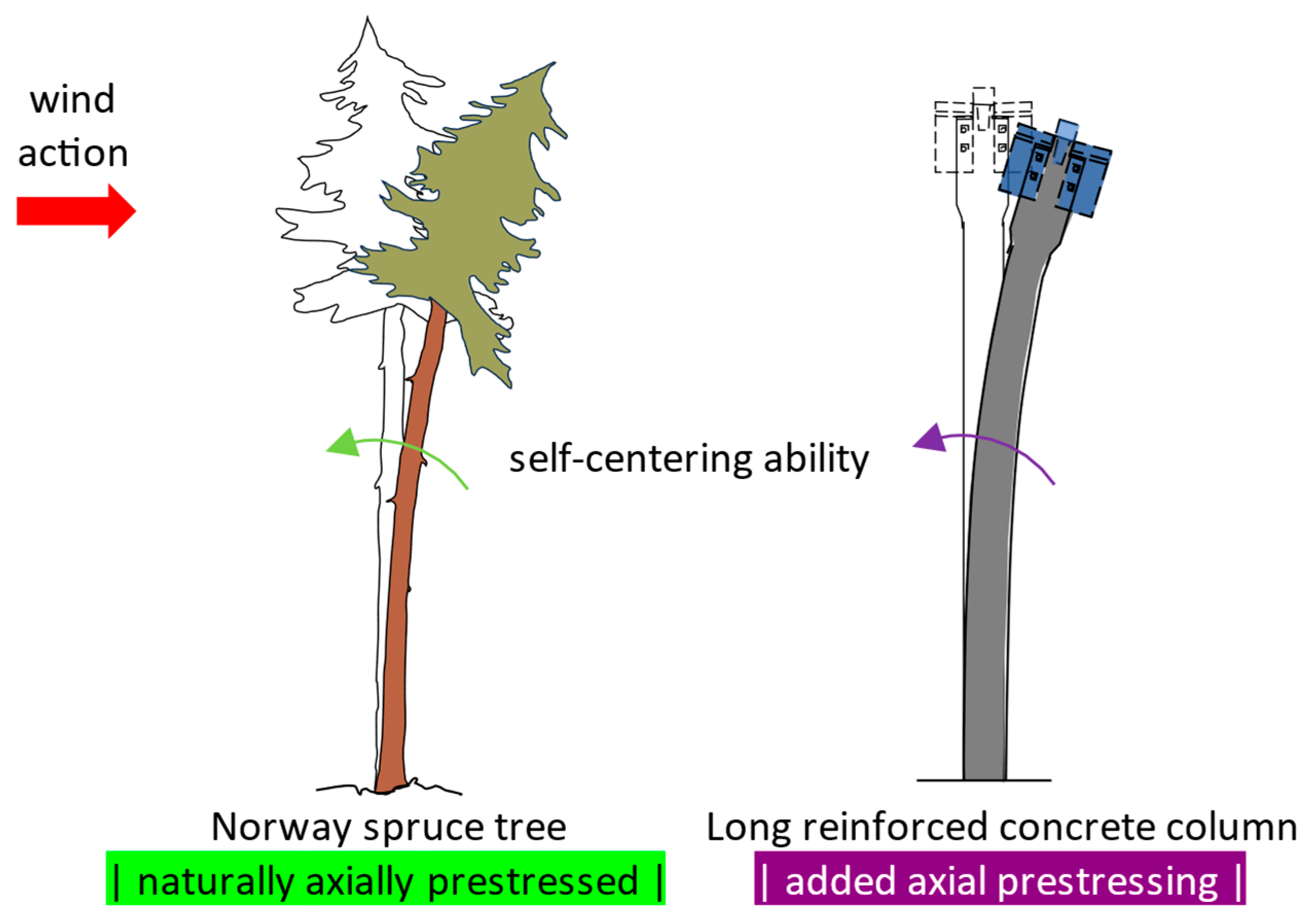
- At the macro-level, longitudinal prestressing for gaining increased flexural stiffness and self-centering capacity (Figure 14). The technical implementation consists in using prestressed unbonded steel strands inside the reinforced concrete column;
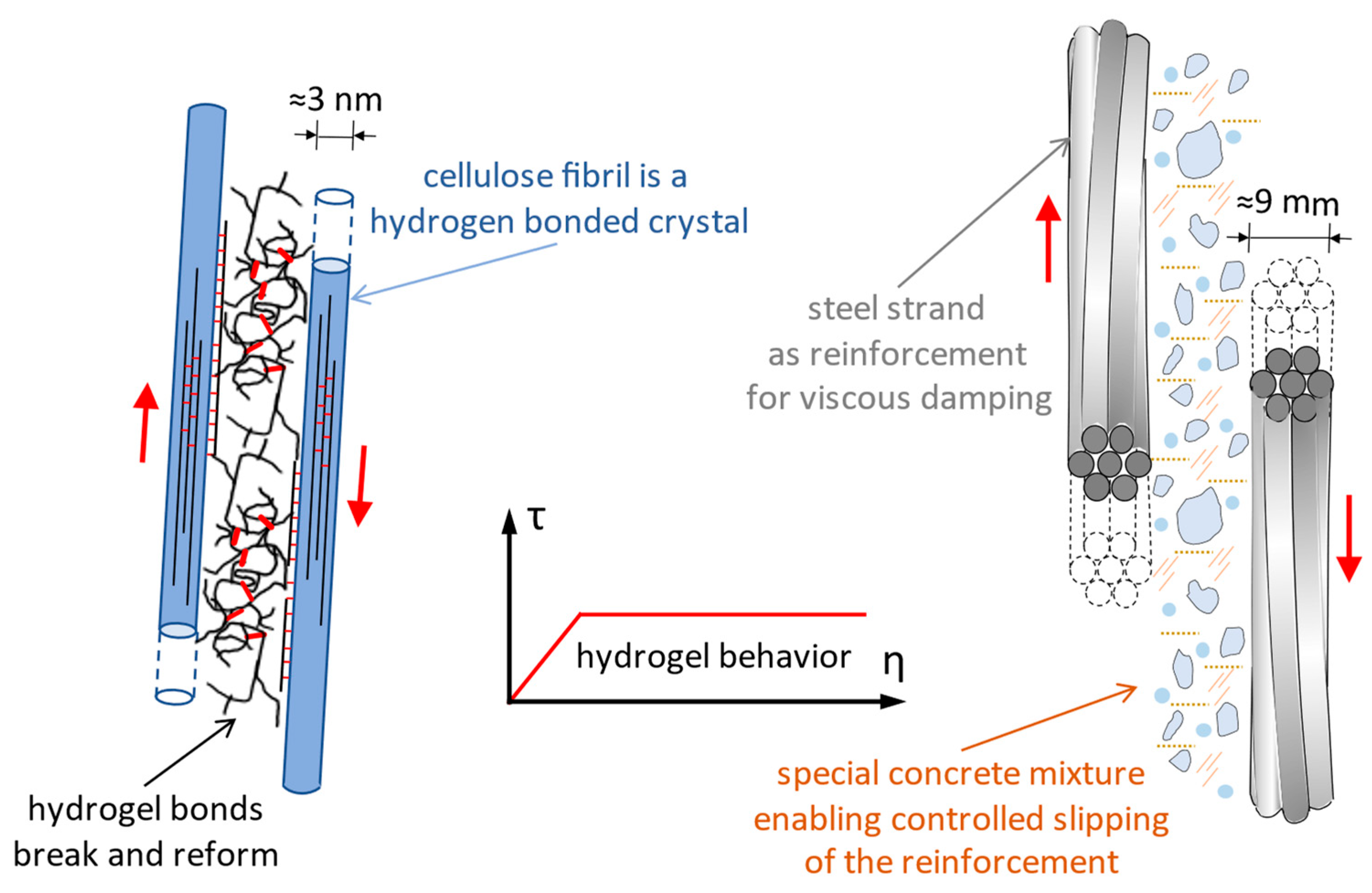
- At the meso-level, viscoelastic damping through sliding of the cellulose fibrils with shearing of the hemicellulose and lignin matrix between them (Figure 15). The technical implementation is solved by greatly upscaling the fibrils (diameter of ≈3 nm) embedded into a matrix of hemicellulose and lignin and substituting them with steel strands (diameter of ≈9 mm) embodied in a concrete mixture with lignin and hemicellulose content. Thus, it targets a controlled bond slip of the steel strands when in tension or in compression.
5. Results and Discussion
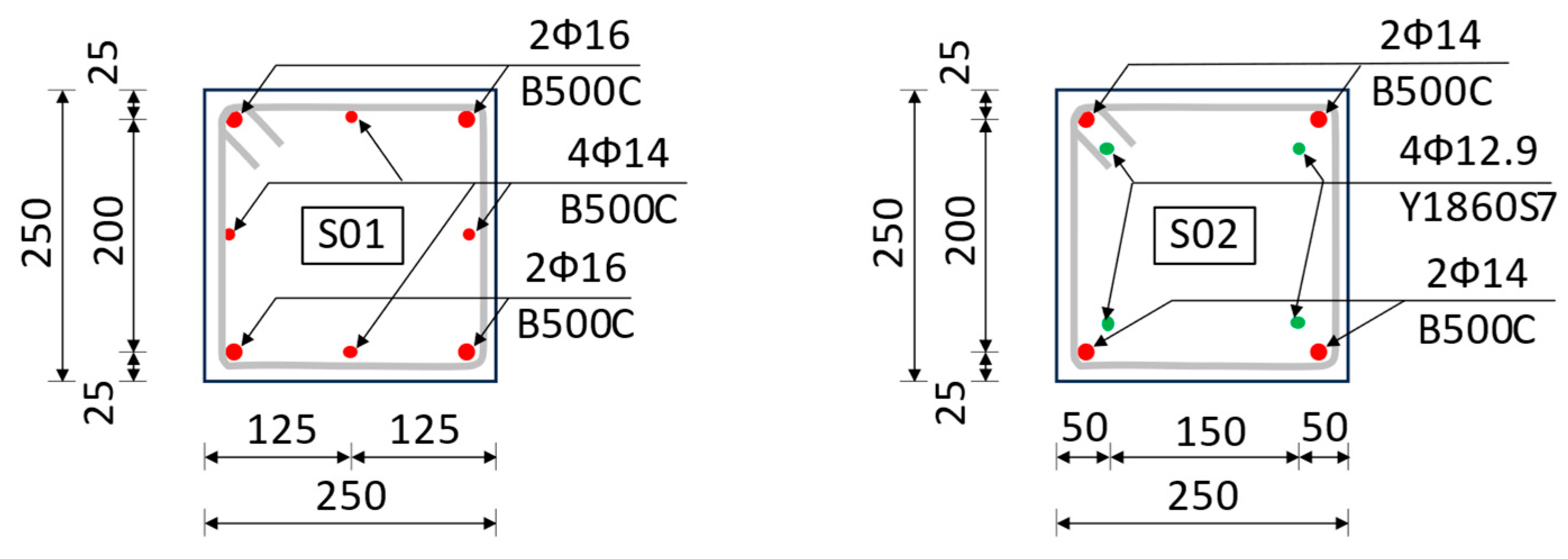
5.1. Experimental Study on the Influence of Centric Prestressing in Long Reinforced Concrete Columns
- For the same lateral force of 24 kN (approx. 80% of the failure force), the reinforced concrete column (S01) had an average lateral displacement of 426 mm (≈13.3% drift) compared with the prestressed reinforced concrete column (S02), which had an average lateral displacement of 289 mm (≈9.0% drift), which means an increase in stiffness of almost 50%;
- Section S02 was less ductile than S01, the energy dissipation capacity being reduced by about 40%. This was caused by the much lower ultimate elongation of the prestressing steel (2.2%) than that of the reinforcing steel (7.5%), collaborated with the uninterrupted adhesion (full bond) of the active reinforcement along the entire length of the column;
- The prestressed elements had self-centering capacity;
- The use of centrically prestressed reinforced concrete columns for single-story warehouses was efficient to reduce lateral displacements at the top of the building (through longitudinal prestressing, the bending stiffness associated with large bending moments was reduced, Figure 17). Cracking of the concrete occurred much later compared with reinforced concrete members without prestressing but, at the same time, a reduced value of the behavior factor must be considered in the seismic design depending on the displacement ductility factor (µδ = Δu/Δc) and on the real curvature ductility factor (µθ = ϕu/ϕc) of the cross-section.
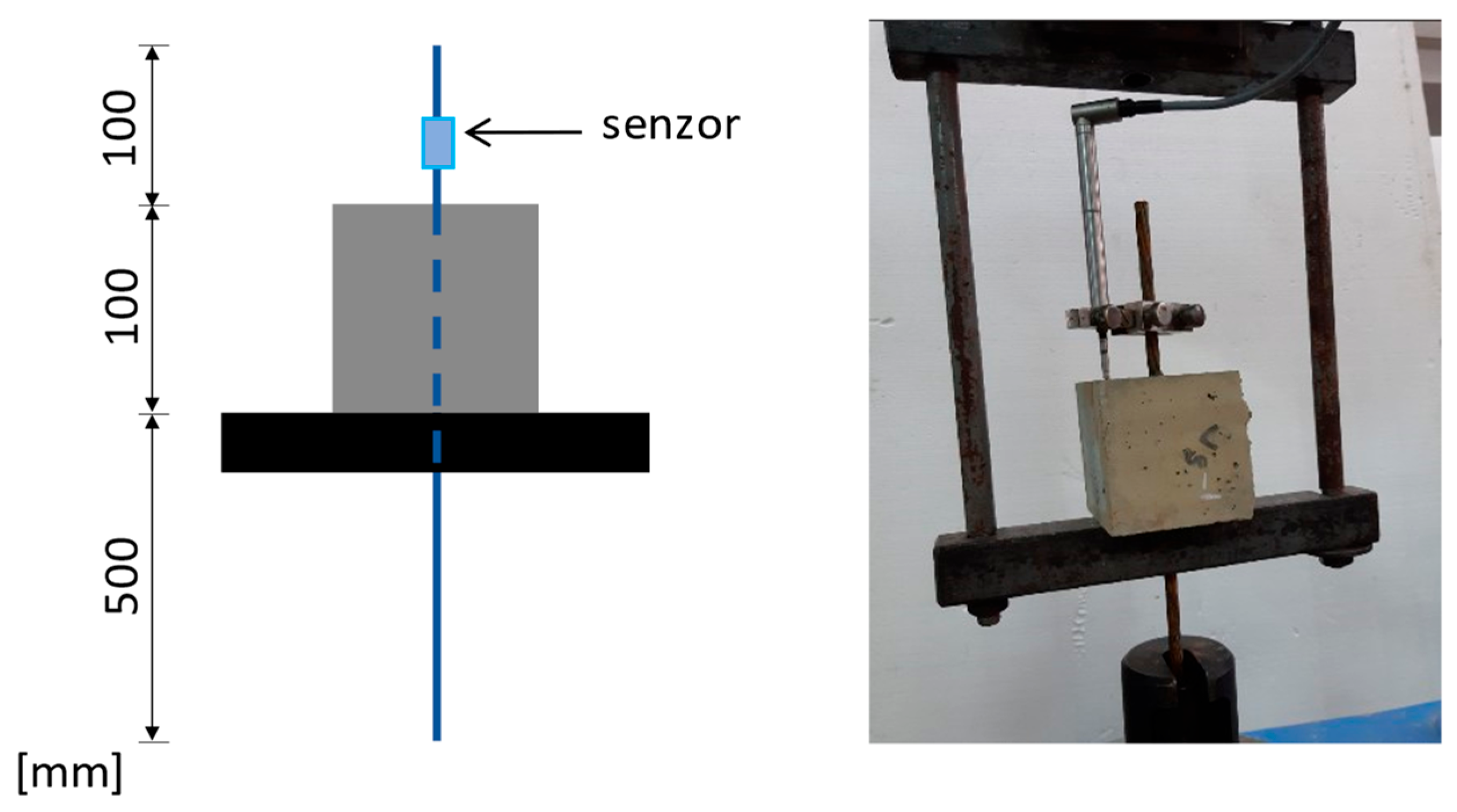
5.2. Experimental Study on the Influence of the Walnut Shell on the Bond of the Reinforcement in Concrete
6. Conclusions
- Reaching viscoelastic damping is assured by using concrete with the addition of hemicellulose and lignin and/or some longitudinal reinforcements with an integrated friction mechanism along their length;
- Supplementary damping results from the interaction of the soil foundation, such as the controlled uplifting of the foundation (solution studied on onshore concrete towers for wind turbines);
- Centric longitudinal unbonded post-tensioning of the concrete columns increases bending stiffness and enables self-centering capacity;
- Designing the structures of neighboring buildings so that the natural frequency of each is equal (this way the seismic joints will have a minimum width), as revealed by the on-site measurements of the Norway spruce trees in the Bilbor region.
- Additional studies on transversal prestressing of columns to increase the degree of concrete core confinement and the rotation capacity at the base of the column;
- Experimental tests for evaluating the viscoelastic damping after cyclic loading and unloading;
- Durability and ageing tests on the special concrete mixture containing biomass (lignin and hemicelluloses);
- Checking the hydrogel behavior on cracked concrete samples;
- Full scale experiments on concrete columns integrating both mimicked features: self-centering ability and viscoelastic damping, with bonded and unbonded strands at the same time.
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kiss, Z. Îmbinări Rigide Pentru Structuri în Cadre din Beton Armat, Amplasate în Zone Seismice. Rev. AICPS 2018, 126–141. Available online: https://www.revistaconstructiilor.eu/index.php/2018/07/01/imbinari-rigide-pentru-structuri-in-cadre-din-beton-armat-amplasate-in-zone-seismice/ (accessed on 6 January 2022).
- Kiss, Z.; Balint, K.; Mocsary, B.; Toader, N. Soluții de Fundare and Tipuri de Infrastructuri Pentru Clădiri din Beton Armat and Precomprimat. Rev. Română Geotehnică Fundații 2013, 1, 29–32. Available online: https://srgf.ro/wp-content/uploads/2022/01/RRGF-2013-1.pdf (accessed on 6 January 2022).
- Toader, N.; Sobek, W.; Nickel, K.G. Energy Absorption in Functionally Graded Concrete. J. Bionic Eng. 2017, 14, 369–378. [Google Scholar] [CrossRef]
- Klang, K.; Bauer, G.; Toader, N.; Lauer, C.; Termin, K.; Schmier, S.; Kovaleva, D.; Haase, W.; Berthold, C.; Nickel, K.G.; et al. (Chapter 7) Plants and Animals as Source of Inspiration for Energy Dissipation in Load Bearing Systems and Facades. In Biomimetic Research for Architecture and Building Construction. Biologically-Inspired Systems; Springer: Berlin/Heidelberg, Germany, 2016; pp. 109–133. [Google Scholar] [CrossRef]
- Grigorian, M. Biomimicry and theory of structures-design methodology transfer from trees to moment frames. J. Bionic Eng. 2014, 11, 638–648. [Google Scholar] [CrossRef]
- Grudnicki, F. Bazele Stabilităţii Arborilor Forestieri (“The Basis of Stability of Forest Trees”); Editura Universităţii “Ştefan cel Mare”: Suceava, Romania, 2003. [Google Scholar]
- Grudnicki, F. Coeficientul de Zveltețe și Stabilitatea Individuală a Arborilor de Molid. Bucov. For. 2004, 12, 75–87. Available online: http://bucovina-forestiera.ro/BF%20old%20site/Bucovina%20forestiera/www.bucovina-forestiera.ro/arhiva/2004/12(1-2)/coeficientul-de-zveltete-si-stabilitatea-individuala-a-arborilor-de-molid.pdf (accessed on 6 January 2022).
- Grudnicki, F. Contribuții la Biomecanica Formei Arborilor (Contributions to the Biomechanics of Tree Shape). Analele Univ. “Ștefan Cel Mare” Suceava Ser. Silvicutură 1994, I, 39–48. [Google Scholar]
- Grudnicki, F. Stabilitatea Molizilor la Acțiunea Vântului (“The Stability of Norway Spruce to Wind Action”). Analele Univ. “Ștefan Cel Mare” Suceava. Secțiunea Silvicultură. Ser. Nouă 2004, 1, 23–36. Available online: http://www.silvic.usv.ro/anale/as_2004_1/as_grudnicki_2004_1.pdf (accessed on 6 January 2022).
- Comitetul European de Standardizare, Proiectarea Structurilor de Beton. Partea 1-1: Reguli Generale and Reguli Pentru Clădiri; Asociația de Standardizare din România (ASRO): Bruxelles, Belgium, 2004. [Google Scholar]
- Emil, M. Berechnung von Eingespannten Gewölben; Rascher: Zürich, Switzerland, 1907. [Google Scholar]
- Emil, M. Bemessungstafeln für das Entwerfen von Eisenbetonbauten; Wittwer: Stuttgart, Germany, 1938. [Google Scholar]
- Fritz, L. Vorlesungen über Massivbau. Teil 1 mit Eduard Mönning: Grundlagen zur Bemessung im Stahlbetonbau, 3rd ed.; Springer: Berlin/Heidelberg, Germany, 1984. [Google Scholar]
- Fritz, L. Vorlesungen über Massivbau. Teil 2 mit Eduard Mönning: Sonderfälle der Bemessung im Stahlbetonbau, 3rd ed.; Springer: Berlin/Heidelberg, Germany, 1986. [Google Scholar]
- Fritz, L. Vorlesungen über Massivbau. Teil 3 mit Eduard Mönning: Grundlagen zum Bewehren im Stahlbetonbau; Springer: Berlin/Heidelberg, Germany, 1977. [Google Scholar]
- Fritz, L. Vorlesungen über Massivbau. Teil 4: Nachweis der Gebrauchsfähigkeit: Rissebeschränkung, Formänderungen, Momentenumlagerung u. Bruchlinientheorie im Stahlbetonbau, 2nd ed.; Springer: Berlin/Heidelberg, Germany, 1978. [Google Scholar]
- Freyssinet, E. Une Révolution Dans la Technique du Béton; Eyrolles: Paris, France, 1936. [Google Scholar]
- Fritz, L. Teil 5: Spannbeton; Springer: Berlin/Heidelberg, Germany, 1980. [Google Scholar]
- Toader, T.-N.; Kiss, Z.; Țere, S.-G.; Puskás, A. An Analytical Design Approach for Post-Tensioned Concrete Slabs with Unguided Tendons. In Proceedings of the Computational Civil Engineering Empower the Digital Transition in the Construction World, Iași, Romania, 27–29 May 2021; Available online: https://iopscience.iop.org/article/10.1088/1757-899X/1141/1/012013 (accessed on 6 January 2022).
- Toader, N.; Haase, W.; Sobek, W. Energy Absorption in Functionally Graded Concrete under Compression. Bul. Institutului Politeh. Iasi—Secția Construcții Arhit. 2018, 64, 9–23. Available online: http://www.bipcons.ce.tuiasi.ro/Content/ArticleInformation.php?ArticleID=654 (accessed on 6 January 2022).
- Nickel, K.G.; Klang, K.L.C.; Toader, N.; Sobek, W. The potential of improving building construction materials by a biomimetic approach. In Proceedings of the 10th International Conference on Emerging Materials and Nanotechnology, Vancouver, BC, Canada, 27–29 July 2017. [Google Scholar] [CrossRef]
- Jan, K.; Ulrich, S.; Thomas, S. Baubionik—Biologie Beflügelt Architektur. Subcapitolul: Gradientenbeton; Naturkunde Museum Stuttgart: Stuttgart, Germany, 2017. [Google Scholar]
- de Langre, E. Effects of wind on plants. Annu. Rev. Fluid Mech. 2008, 40, 141–168. [Google Scholar] [CrossRef]
- James, K.R.; Dahle, G.A.; Grabosky, J.; Kane, B.; Detter, A. Tree Biomechanics Literature Review: Dynamics. Arboric. Urban For. 2014, 40, 1–15. [Google Scholar] [CrossRef]
- Spatz, H.C.; Brüchert, F. Basic biomechanics of self-supporting plants: Wind and gravitational loads on a Norway spruce tree. For. Ecol. Manag. 2000, 135, 33–44. [Google Scholar] [CrossRef]
- Vogel, S. Blowing in the Wind: Storm-Resisting Features of the Design of Trees. J. Arboric. 1996, 22, 92–98. Available online: https://joa.isa-arbor.com/request.asp?JournalID=1&ArticleID=2715&Type=2 (accessed on 6 January 2022). [CrossRef]
- Miller, L. Structural Dynamics and Resonance in Plants with Nonlinear Stiffness. J. Theor. Biol. 2005, 234, 511–524. [Google Scholar] [CrossRef] [PubMed]
- James, K.R.; Moore, J.R.; Slater, D.; Dahle, G. Tree biomechanics. CAB Rev. 2017, 12, 1–11. [Google Scholar] [CrossRef]
- Niklas, K.J. Plant Biomechanics: An Engineering Approach to Plant Form and Function; University of Chicago Press: Chicago, IL, USA, 1992. [Google Scholar]
- Lindström, H.; Evans, J.; Verril, S. Influence of Cambial Age and Growth Condition on Microfibril Angle in Young Norway Spruce [Picea abies (L.) Karst]. Holzforforschung 1998, 52, 573–581. Available online: https://www.fpl.fs.usda.gov/documnts/pdf1998/linds98a.pdf (accessed on 6 January 2022). [CrossRef]
- Lichtenegger, H.; Reiterer, A.; Stanzl-Tschegg, S.; Fratzl, P. Variation of cellulose microfibril angles in softwoods and hardwoods—A possible strategy of mechanical optimization. J. Struct. Biol. 1999, 128, 257–269. [Google Scholar] [CrossRef] [PubMed]
- Reiterer, A.; Lichtenegger, H.; Tschegg, S.; Fratzl, P. Experimental evidence for a mechanical function of the cellulose microfibril angle in wood cell walls. Philos. Mag. A 1999, 79, 2173–2184. [Google Scholar] [CrossRef]
- Brüchert, F.; Becker, F.; Speck, T. The mechanics of Norway Spruce [Picea abies (L.) Karst]: Mechanical properties of standing trees from different thinning regimes. For. Ecol. Manag. 2000, 135, 45–62. [Google Scholar] [CrossRef]
- Lundström, T.; Stoffel, M.; Stöckli, V. Fresh-Stem Bending of Silver Fir and Norway Spruce. Tree Physiol. 2008, 28, 355–366. Available online: https://academic.oup.com/treephys/article-pdf/28/3/355/4659859/28-3-355.pdf (accessed on 6 January 2022). [CrossRef] [PubMed]
- Speck, T.; Burgert, I. Plant Stems: Functional Design and Mechanics. Annu. Rev. Mater. Res. 2011, 41, 169–193. [Google Scholar] [CrossRef]
- Moore, J.R.; Maguire, D.A. Natural sway frequencies and damping ratios of trees: Concepts, review and synthesis of previous studies. Trees—Struct. Funct. 2004, 18, 195–203. [Google Scholar] [CrossRef]
- Milne, R. Dynamics of swaying Picea sitchensis. Tree Physiol. 1991, 9, 383–399. [Google Scholar] [CrossRef]
- Flesch, T.; Wilson, J.D. Wind and remnant tree sway in forest cutblocks. II. Relating measured tree sway to wind statistics. Agric. For. Meteorol. 1999, 93, 243–258. [Google Scholar] [CrossRef]
- Jonsson, M.J.; Foetzki, A.; Kalberer, M.; Lundström, T.; Ammann, W.; Stöckli, V. Natural frequencies and damping ratios of Norway spruce (Picea abies (L.) Karst) growing on subalpine forested slopes. Trees 2007, 21, 541–548. [Google Scholar] [CrossRef]
- Mayhead, G.J. Sway Periods of Forest Trees. Scott. For. 1973, 27, 19–23. Available online: https://link.springer.com/article/10.1007/BF00123529 (accessed on 6 January 2022).
- Mayhead, G.J.; Gardiner, J.B.H.; Durrant, D.W. A Report on the Physical Properties of Conifers in Relation to Plantation Stability; Research and Development Branch: Edinburgh, Scotland, 1975. [Google Scholar]
- Holbo, H.R.; Corbett, T.C.; Horton, P.J. Aeromechanical behaviour of selected Douglas-fir. Agric. Meteorol. 1980, 21, 81–91. [Google Scholar] [CrossRef]
- Peltola, H.; Kellomaki, S.; Hassinen, A.; Lemittinen, M.; Aho, J. Swaying of trees as caused by wind: Analysis of field measurements. Silva Fenn. 1993, 27, 113–126. [Google Scholar] [CrossRef][Green Version]
- Hassinen, A.; Lemettinen, M.; Peltola, H.; Kellomäki, S.; Gardiner, B. A prism-based system for monitoring the swaying of trees under wind loading. Agric. For. Meteorol. 1998, 90, 187–194. [Google Scholar] [CrossRef]
- Petty, J.A.; Swain, C. Factors influencing stem breakage of conifers in high winds. Forestry 1985, 58, 75–84. [Google Scholar] [CrossRef]
- Scannell, B. Quantification of the Interactive Motions of the Atmospheric Surface Layer and a Conifer Canopy. Ph.D. Thesis, Cranfield Institute of Technology, Bedford, UK, 1984. [Google Scholar]
- Blevins, R. Formulas for Natural Frequency and Mode Shape; Van Nostrand Reinhold Co.: New York, NY, USA, 1979. [Google Scholar]
- Spatz, H.-C.; Theckes, B. Oscillation damping in trees. Plant Sci. 2013, 207, 66–71. [Google Scholar] [CrossRef] [PubMed]
- Moore, J.R.; Maguire, D.A. Natural sway frequencies and damping ratios of trees: Influence of crown structure. Trees 2005, 19, 363–373. [Google Scholar] [CrossRef]
- Blackburn, P.; Miller, K.F.; Petty, J.A. An assessment of the static and dynamic factors involved in windthrow. Forestry 1988, 61, 29–43. [Google Scholar] [CrossRef]
- Gardiner, B.A. Mathematical modelling of the static and dynamic characteristics of plantation trees. In Mathematical Modelling of Forest Ecosystems; Franke, J., Roeder, A., Eds.; Sauerlander’s Verlag: Frankfurt am Main, Germany, 1992; pp. 40–61. [Google Scholar]
- Gardiner, B.A.; Stacey, G.R.; Belcher, R.E.; Wood, C.J. Field and wind tunnel assessments and the implications of respacing and thinning for tree stability. Forestry 1997, 70, 233–252. [Google Scholar] [CrossRef]
- Sugden, M.J. Tree sway period—A possible new parameter for crown classification and stand competition. For. Chron. 1962, 38, 336–344. [Google Scholar] [CrossRef]
- Loo, S.P. Aerodynamic Characteristics of a Flexible Structure (Tree). Master’s Thesis, University of Canterbury, Christchurch, New Zealand, 1975. [Google Scholar]
- James, K.R. A Study of Branch Dynamics on an Open Grown Tree. Arboric. Urban For. 2014, 40, 125–134. Available online: https://joa.isa-arbor.com/request.asp?JournalID=1&ArticleID=3318&Type=2 (accessed on 6 January 2022). [CrossRef]
- Sellier, D.; Fourcaud, T. Crown Structure and Wood Properties: Influence on Tree Sway and Response to High Winds. Am. J. Bot. 2009, 26, 885–896. Available online: https://www.jstor.org/stable/27733419 (accessed on 6 January 2022). [CrossRef]
- Telewski, F. Wind-Induced Physiological and Developmental Responses in Trees; Published in Coutts MP; Wind and Trees; Grace, J., Ed.; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]
- Nicoll, B.C.; Gardiner, B.A.R.B.; Peace, A.J. Anchorage of Coniferous Trees in Relation to Species, Soil Type, and Rooting Depth. Can. J. For. Res. 2006, 36, 1871–1883. Available online: https://cdnsciencepub.com/doi/10.1139/x06-072 (accessed on 6 January 2022). [CrossRef]
- Nicoll, B.; Gardiner, B.; Peace, A. Improvements in anchorage provided by the acclimation of forest trees to wind stress. Forestry 2008, 81, 389–398. [Google Scholar] [CrossRef]
- Telewski, F. Is windswept tree growth negative thigmotropism? Plant Sci. 2012, 184, 20–28. [Google Scholar] [CrossRef]
- Badel, E.; Ewers, F.; Cochard, H.; Telewski, F. Acclimation of mechanical and hydraulic functions in trees: Impact of the thigmomorphogenetic process. Front. Plant Sci. 2015, 6, 266. [Google Scholar] [CrossRef]
- Shigo, A. A New Tree Biology Dictionary; Shigo and Trees Associates: Snohomish, WA, USA, 1986. [Google Scholar]
- Blankenhorn, P. Wood: Sawn Materials. Encyclopedia of Materials: Science and Technology; Elsevier Ltd.: Pergamon, Turkey, 2001. [Google Scholar]
- McDonald, A.; Donaldson, L. Constituents of wood. Encyclopedia of Materials: Science and Technology; Elsevier Ltd.: Pergamon, Turkey, 2001. [Google Scholar]
- Yamamoto, H.; Ruelle, J.; Arakawa, Y.; Yoshida, M.; Clair, B.; Gril, J. Origin of the characteristic hygro-mechanical properties of the gelatinous layer in tension wood from Kunugi oak (Quercus acutissima). Wood Sci. Technol. 2010, 44, 149–163. [Google Scholar] [CrossRef]
- Fratzl, P.; Weinkamer, R. Nature’s hierarchical materials. Prog. Mater. Sci. 2007, 52, 1263–1334. [Google Scholar] [CrossRef]
- Gibson, L.J. Biomechanics of cellular solids. J. Biomech. 2005, 38, 377–399. [Google Scholar] [CrossRef] [PubMed]
- Fengel, D.; Wegener, G. Wood: Chemistry, Ultrastructure, Reactions; De Gruyter: Berlin, Germany, 1989. [Google Scholar]
- Keckes, J.; Burgert, I.; Fruhmann, K.; Muller, M.; Kolln, K.; Hamilton, M.; Burghammer, M.; Roth, S.V.; Stanzl-Tschegg, S.; Fratzl, P. Cell-wall recovery after irreversible deformation of wood. Nat. Mater. 2003, 2, 810–814. [Google Scholar] [CrossRef] [PubMed]
- Koehler, L.; Spatz, H. Micromechanics of Plant Tissues beyond the Linear-Elastic Range. Planta 2002, 215, 33–40. Available online: https://link.springer.com/content/pdf/10.1007/s00425-001-0718-9.pdf (accessed on 6 January 2022). [CrossRef] [PubMed]
- Fratzl, P.; Burgert, I.; Gupta, H. On the role of interface polymers for the mechanics of natural polymeric composites. Phys. Chem. Chem. Phys. 2004, 6, 5575–5579. [Google Scholar] [CrossRef]
- Dargahi, M.; Newson, T.; Moore, J.R. A Numerical Approach to Estimate Natural Frequency of Trees with Variable Properties. Forests 2020, 11, 915–935. [Google Scholar] [CrossRef]
- Coutts, M.P. Components of tree stability in Sitka spruce on peaty gley soil. Forestry 1986, 59, 173–197. [Google Scholar] [CrossRef]
- Giurgiu, V.; Decei, I. Biometria Arborilor din România; Editura Snagov: București, Romania, 1997. [Google Scholar]
- Lundström, T.; Jonsson, M.J.; Kalberer, M. The root–soil system of Norway spruce subjected to turning moment: Resistance as a function of rotation. Plant Soil 2007, 300, 35–49. [Google Scholar] [CrossRef]
- Mayer, H. Wind induced tree sways. Trees 1987, 1, 195–206. [Google Scholar] [CrossRef]
- Hale, S.; Gardiner, B.; Wellpott, A.; Nicoll, B.; Achim, A. Wind loading of trees: Influence of tree size and competition. Eur. J. For. Res. 2010, 131, 203–217. [Google Scholar] [CrossRef]
- Duryea, M.; Kampf, E.; Littel, R.; Rodriguez-Pedraza, C. Hurricanes and the Urban Forest II: Effects on Tropical. Arboric. Urban For. 2007, 33, 98–112. Available online: https://hort.ifas.ufl.edu/treesandhurricanes/documents/pdf/EffectsOnTropicalAndSubtropicalTreeSpecies.pdf (accessed on 6 January 2022). [CrossRef]
- Kane, B. Tree failure following a wind storm in Brewster, Massachusetts, USA. Urban For. Urban Green. 2008, 7, 15–23. [Google Scholar] [CrossRef]
- Peltola, H.; Kellomäki, S.; Väisänen, H.; Ikonen, V.-P. A Mechanistic Model for Assessing the Risk of Wind and Snow Damage to Single Trees and Stands of Scots Pine, Norway Spruce, and Birch. Can. J. For. Res. 1999, 29, 647–661. Available online: https://cdnsciencepub.com/doi/10.1139/x99-029 (accessed on 6 January 2022). [CrossRef]
- Kellomäki, S.; Peltola, H. Silvicultural Strategies for Predicting Damage to Forests from Wind, Fire and Snow: Integrating Tree, Site and Stand Properties with Geographical Information Systems and Regional Environmental Models to Evaluate Options for Forest Management; European Community: Brussels, Belgium, 1998. [Google Scholar]
- Morgan, J.; Cannell, M.G.R. Shape of tree stems: A re-examination of the uniform stress hypothesis. Tree Physiol. 1994, 14, 49–62. [Google Scholar] [CrossRef]
- Sunley, J.-G. Grade Stresses for Structural Timbers. For. Prod. Res. (Bull.) 1968, 47, 1–18. Available online: https://catalogue.nla.gov.au/catalog/940485 (accessed on 6 January 2022).
- Petty, J.A.; Worrell, R. Stability of coniferous tree stems in relation to damage by snow. Forestry 1981, 54, 115–128. [Google Scholar] [CrossRef]
- Jones, H.G. Plants and Microclimate. A Quantitative Approach to Environmental Plant Physiology; Cambridge University Press: Cambridge, UK, 1983. [Google Scholar] [CrossRef]
- Peltola, H.; Kellomäki, S. A mechanistic model for calculating windthrow and stem breakage of Scots pines at stand edge. Silva Fenn. 1993, 27, 99–111. [Google Scholar] [CrossRef]
- Neild, S.A.; Wood, C.J. Estimating stem and root-anchorage flexibility in trees. Tree Physiol. 1999, 19, 141–151. [Google Scholar] [CrossRef] [PubMed]
- Popa, A.; Ilieș, N.M. Fundații; Casa Cărții de Știință: Cluj-Napoca, Romania, 2013; pp. 87–88. [Google Scholar]
- Jonsson, M.J.M.J.; Foetzki, A.; Kalberer, M.; Lundström, T.; Ammann, W.; Stöckli, V. Root-soil rotation stiffness of Norway spruce (Picea abies (L.) Karst) growing on subalpine forested slopes. Plant Soil 2006, 285, 267–277. [Google Scholar] [CrossRef]
- Vogel, S.; Davis, K.K. Cat’s Paws and Capults: Mechanical Worlds of Nature and People; W. W. Norton & Company: New York, NY, USA, 2000. [Google Scholar]
- Cattano, C.; Nikou, T.; Klotz, L. Teaching systems: Thinking and biomimicry to civil engineering students. J. Prof. Issues Eng. Educ. Pract. 2011, 137, 176–182. [Google Scholar] [CrossRef]
- Knippers, J.; Speck, T. Design and Construction Principles in Nature and Architecture. Bioinspir. Biomim. 2012, 7, 015002. Available online: https://iopscience.iop.org/article/10.1088/1748-3182/7/1/015002/meta (accessed on 6 January 2022). [CrossRef] [PubMed]
- Benyus, J. Biomimicry; William Morrow and Company: New York, NY, USA, 1997. [Google Scholar]
- Xing, D.; Chen, W. Systematic method of applying structural characteristics of natural organisms to mechanical structures. Trans. Tianjin Univ. 2011, 17, 303–307. [Google Scholar] [CrossRef]
- Aviation Weather Center (AWC). Wood Frame Construction Manual Workbook: Design of Wood Frame Buildings for High Wind, Snow and Seismic Loadings; AWC: Virginia, NV, USA, 2005. [Google Scholar]
- Ennos, R. Trees: Magnificent Structures; The Natural History Museum: London, UK, 2001. [Google Scholar]
- Grudnicki, F. Biomecanica Sistemelor Radicelare ale Arborilor Forestieri (“Biomechanics of Root Systems of Forest Trees”). Rev.-Bucov. For. 2003, 11, 39–48. Available online: http://bucovina-forestiera.ro/BF%20old%20site/Bucovina%20forestiera/www.bucovina-forestiera.ro/arhiva/2003/11(1)/biomecanica-sistemelor-radicelare-ale-arborilor-forestieri.pdf (accessed on 6 January 2022).
- Schmier, S.; Bold, G.; Buck, G.; Klang, K.; Lauer, C.; Toader, N.; Gericke, O.; Haase, W.; Schäfer, I.; Schmauder, S.; et al. Hohe Belastungen sicher überstehen. In Bionisch Bauen; Birkhäuser: Berlin, Germany; Boston, MA, USA, 2019; pp. 54–73. [Google Scholar] [CrossRef]
- Ostertag, A.; Toader, T.-N.; Bertsche, B.; Sobek, W. System-Safety in the Application of Adaptive Load-Bearing Structures. In Proceedings of the Annual Reliability and Maintainability Symposium, Orlando, FL, USA, 28–31 January 2019; IEEE Proceedings. Available online: https://ieeexplore.ieee.org/document/8769000 (accessed on 6 January 2022).
- Chrițescu, M. Utilizarea Precomprimării la Elemente Prefabricate Lungi din Beton, Solicitate Preponderent la Compresiune cu Încovoiere. Ph.D. Thesis, UTCN, Cluj-Napoca, Romania, 2016. [Google Scholar]
- Chirițescu, M.; Kiss, Z.; Mureșan, R.-M. A finite element numerical simulation on different types of prestressed concrete columns. In Proceedings of the 13th International Scientific Conference VSU 2013, Sofia, Bulgaria, 22–24 June 2013. [Google Scholar]
- Chirițescu, M.; Kiss, Z.; Mureșan, R.-M. An experimental study on a series of three types of prestressed concrete columns. In Proceedings of the 13th International Scientific Conference VS 2013, Sofia, Bulgaria, 22–24 June 2013. [Google Scholar]
- Kovacs, B. Un Algoritm de Calcul Pentru Stâlpi din Beton Precomprimat. Master’s Thesis, UTCN, Cluj-Napoca, Romania, 2022. [Google Scholar]
- Kovacs, B.; Toader, T.-N. An automated Algorithm for the Sectional Analysis of Reinforced and Prestressed Concrete Columns. J. Appl. Eng. Sci. 2022, 12, 61–70. Available online: https://sciendo.com/fr/article/10.2478/jaes-2022-0010 (accessed on 6 January 2022). [CrossRef]
- Rozian, D. Influența Ligninei Asupra Rezistenței la Compresiune a Betonului și Asupra Aderenței Armăturii în Beton. Master’s Thesis, UTCN, Cluj-Napoca, Romania, 2022. [Google Scholar]
- Jahanban-Esfahlan, A.; Ostadrahimi, A.; Tabibiazar, M.; Amarowicz, R. A comprehensive review on the chemical constituents and functional uses of walnut (Juglans spp.) husk. Int. J. Mol. Sci. 2019, 20, 3920. [Google Scholar] [CrossRef] [PubMed]
- Sareena, C.; Sreejith, M.; Ramesan, M.; Purushothaman, E. Biodegradation behaviour of natural rubber composites reinforced with natural resource fillers—Monitoring by soil burial test. J. Reinf. Plast. Compos. 2014, 33, 412–442. [Google Scholar] [CrossRef]
- Kiss, Z.; Balint, K.; Toader, N.; Nagy, Z. A long span structure in Romania. In Proceedings of the IABSE Symposium: Long Span Bridges and Roofs—Development, Design and Implementation, Kolkata, India, 24–27 September 2013. [Google Scholar] [CrossRef]
- Toader, T.-N.; Schmeer, D.; Sobek, W. Concept for an Onshore Tower Structure Made of UHPFRC Segments for Wind Turbines. Acta Tech. Napoc. Civ. Eng. Archit. 2019, 62, 5–25. Available online: https://oldconstructii.utcluj.ro/ActaCivilEng/download/atn/ATN2019(1)_1.pdf (accessed on 6 January 2022).
- Toader, N.; Kiss, Z. Experimental Tests on a Precast Concrete Frame Structure Subjected to Lateral Loads. Bul. Institutului Politeh. Din Iasi—Secția Construcții. Arhit. 2014, 54, 110–119. Available online: http://www.bipcons.ce.tuiasi.ro/Archive/475.pdf (accessed on 6 January 2022).
- Maier, M.; Siegel, D.; Thoben, K.D.; Niebuhr, N.; Hamm, C. Transfer of natural micro structures to bionic lightweight design proposals. J. Bionic Eng. 2013, 11, 469–478. [Google Scholar] [CrossRef]
- American Institute of Steel Construction (AISC). Seismic Provisions for Structural Steel Buildings; AISC: Chicago, IL, USA, 2005. [Google Scholar]
- National Research Council Canada. National Building Code of Canada; National Research Council Canada: Ottawa, ON, Canada, 1995. [Google Scholar]
- Grigorian, M.; Grigorian, C. Performance Control: New Elastic-Plastic Design Procedure for Earthquake Resisting Moment Frames. J. Struct. Eng. 2012, 138, 812–821. Available online: https://ascelibrary.org/doi/abs/10.1061/%28ASCE%29ST.1943-541X.0000515 (accessed on 6 January 2022). [CrossRef]
- CEN European Comission for Standardization. Eurocode 8: Design of Structures for Earthquake Resistance. Part 1: General Rules, Seismic Actions and Rules for Buildings; CENELEC: Bruxelles, Belgium, 2006. [Google Scholar]
- MDRAP. P100-1/2013. Cod de Proiectare Seismică—Partea I—Prevederi Pentru Clădiri; Monitorul Oficial al României: București, Romania, 2019. [Google Scholar]








| Genus/Species | Number of Trees Investigated | Modulus of Elasticity of Green Wood, Eg (GPa) | Density, ρ (kg/m3) | The Ratio of Crown Weight to Stem Weight, Wcrown/Wstem |
|---|---|---|---|---|
| Spruce (Picea spp.) | ||||
| Norway spruce (Picea abies) | 32 | 6.23 | 598 | 0.32 |
| Sitka spruce (Picea sitchensis) | 175 | 7.53 | 447 | 0.50 |
| White spruce (Picea glauca) | 6 | 7.40 | 466 | 0.34 |
| Pine (Pinus spp.) | ||||
| Corsican pine (Pinus nigra) | 57 | 8.70 | 657 | 0.34 |
| Lodgepole pine (Pinus contorta) | 40 | 6.90 | 487 | 0.33 |
| Scots pine (Pinus sylvestris) | 20 | 7.33 | 700 | 0.29 |
| Red pine (Pinus resinosa) | 300 | 8.80 | 410 | 0.22 |
| Douglas fir (Pseudotsuga spp.) | ||||
| Douglas fir (Pseudotsuga menziesii) | 17 | 9.83 | 583 | 0.16 |
| No. | Height (m) | Diameter at Base (cm) | DBH (cm) | hrsplate (cm) | drsplate1 (m) | drsplate2 (m) | MR_rs (kN·m) | MR_stem (kN·m) | MR_stem/MR_rs (-) |
|---|---|---|---|---|---|---|---|---|---|
| 1 | 29.0 | 41 | 36 | 25 | 3.4 | 2.7 | 34.2 | 140.2 | 4.1 |
| 2 | 29.0 | 42 | 36 | 30 | 3.5 | 1.8 | 37.2 | 140.2 | 3.8 |
| 3 | 28.5 | 38 | 32 | 27 | 2.3 | 1.6 | 16.3 | 98.4 | 6.0 |
| 4 | 30.0 | 43 | 38 | 34 | 3.4 | 2.4 | 57.3 | 164.8 | 2.9 |
| 5 | 30.5 | 45 | 39 | 29 | 3.2 | 2.0 | 33.5 | 178.2 | 5.3 |
| 6 | 31.0 | 51 | 42 | 30 | 3.5 | 2.4 | 46.1 | 222.6 | 4.8 |
| 7 | 30.5 | 50 | 41 | 26 | 3.4 | 2.5 | 34.7 | 207.0 | 6.0 |
| 8 | 30.0 | 43 | 39 | 29 | 3.2 | 2.1 | 34.8 | 178.2 | 5.1 |
| 9 | 27.5 | 36 | 32 | 20 | 2.0 | 1.4 | 6.8 | 98.4 | 14.5 |
| 10 | 29.0 | 40 | 38 | 24 | 3.0 | 2.3 | 23.8 | 164.8 | 6.9 |
| 11 | 29.5 | 41 | 38.5 | 30 | 3.2 | 2.4 | 41.6 | 171.4 | 4.1 |
| 12 | 28.0 | 39 | 37 | 29 | 3.0 | 1.8 | 28.5 | 152.2 | 5.3 |
| 13 | 27.0 | 38 | 34 | 26 | 2.8 | 1.7 | 20.2 | 118.1 | 5.9 |
| 14 | 28.0 | 37 | 32 | 28 | 2.1 | 1.7 | 16.7 | 98.4 | 5.9 |
| 15 | 31.0 | 42 | 38 | 30 | 2.4 | 2.1 | 26.8 | 164.8 | 6.1 |
| 16 | 30.0 | 43 | 38.5 | 30 | 3.1 | 2.2 | 37.2 | 171.4 | 4.6 |
| 17 | 29.0 | 36 | 34 | 28 | 2.2 | 1.7 | 17.6 | 118.1 | 6.7 |
| 18 | 28.5 | 41 | 36 | 30 | 3.0 | 1.8 | 30.5 | 140.2 | 4.6 |
| 19 | 29.0 | 40 | 36 | 27 | 3.3 | 2.5 | 36.1 | 140.2 | 3.9 |
| 20 | 30.5 | 41 | 39 | 34 | 3.5 | 2.6 | 63.3 | 178.2 | 2.8 |
| 21 | 30.0 | 48 | 40 | 30 | 3.4 | 2.6 | 47.7 | 192.3 | 4.0 |
| 22 | 31.0 | 50 | 42 | 26 | 3.6 | 2.7 | 39.5 | 222.6 | 5.6 |
| 23 | 27.0 | 35 | 32 | 25 | 2.2 | 1.6 | 13.3 | 98.4 | 7.4 |
| 24 | 27.0 | 37 | 34 | 28 | 2.7 | 2.0 | 25.5 | 118.1 | 4.6 |
| 25 | 30.0 | 40 | 37 | 32 | 3.2 | 2.2 | 44.0 | 152.2 | 3.5 |
| 26 | 28.0 | 36 | 33 | 30 | 3.1 | 2.0 | 34.5 | 108.0 | 3.1 |
| 27 | 30.0 | 50 | 42 | 34 | 3.4 | 2.2 | 53.4 | 222.6 | 4.2 |
| 28 | 30.0 | 48 | 40 | 27 | 3.1 | 2.6 | 34.9 | 192.3 | 5.5 |
| 29 | 28.5 | 42 | 37 | 29 | 2.8 | 2.1 | 29.7 | 152.2 | 5.1 |
| 30 | 30.5 | 51 | 42 | 32 | 3.5 | 2.5 | 54.3 | 222.6 | 4.1 |
| Modulus of Elasticity MPa | Characteristic Strength (fck, fpk, fyk) MPa | Ultimate Strain (εcu, εpu, εsu) [–] | |
|---|---|---|---|
| Concrete C60/75 | 39,000 | 60 | 0.3% |
| Prestressing steel Y1860S7 | 199,000 | 1860 | 2.2% |
| Reinforcement B500C | 205,000 | 500 | 7.5% |
| Specimen | Concrete Grade | Maximum Value of Pull-Out Force kN | Average Value for Maximum Force kN | |
|---|---|---|---|---|
| V3 | V3–1 | C32/40 | 21.39 | 19.88 |
| V3–2 | 21.9 | |||
| V3–3 | 16.35 | |||
| V4 | V4–1 | C35/45 | 16.98 | 17.43 |
| V4–2 | 16.92 | |||
| V4–3 | 18.39 | |||
| V5 | V5–1 | C35/45 | 15.33 | 17.2 |
| V5–2 | 18.81 | |||
| V5–3 | 17.46 | |||
| V6 | V6–1 | C35/45 | 14.25 | 12.06 |
| V6–2 | N/A | |||
| V6–3 | 9.87 | |||
| Compound | Walnut Shell [106] | Peanut Shell [107] |
|---|---|---|
| Ash | 3.4% | 3.8% |
| Lignin | 50.3% | 36.1% |
| Hemicellulose | 22.4% | 5.6% |
| Cellulose | 23.9% | 44.8% |
| Ingredient | Type | Amount |
|---|---|---|
| CEM I 52.5R | Cement | 1155 g |
| Water | Water | 533 g |
| Dry aggregates | Source: river | |
| Sand | 0–2 mm | 2767 g |
| Fine gravel | 2–8 mm | 1320 g |
| Medium gravel | 8–16 mm | 1921 g |
| Sika Plastiment BV 440 | Plasticizer (lignosulfonate based) | 13.3 g |
| Walnut (Juglans regia) shell powder | 0.063–0.125 mm | 11.5 g |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Toader, T.-N.; Mircea, C.G.-R.; Truta, A.M.; Constantinescu, H. Coniferous Trees as Bioinspiration for Designing Long Reinforced Prestressed Concrete Columns. Biomimetics 2024, 9, 165. https://doi.org/10.3390/biomimetics9030165
Toader T-N, Mircea CG-R, Truta AM, Constantinescu H. Coniferous Trees as Bioinspiration for Designing Long Reinforced Prestressed Concrete Columns. Biomimetics. 2024; 9(3):165. https://doi.org/10.3390/biomimetics9030165
Chicago/Turabian StyleToader, Traian-Nicu, Călin G.-R. Mircea, Alina M. Truta, and Horia Constantinescu. 2024. "Coniferous Trees as Bioinspiration for Designing Long Reinforced Prestressed Concrete Columns" Biomimetics 9, no. 3: 165. https://doi.org/10.3390/biomimetics9030165
APA StyleToader, T.-N., Mircea, C. G.-R., Truta, A. M., & Constantinescu, H. (2024). Coniferous Trees as Bioinspiration for Designing Long Reinforced Prestressed Concrete Columns. Biomimetics, 9(3), 165. https://doi.org/10.3390/biomimetics9030165