Implementation of Underwater Electric Field Communication Based on Direct Sequence Spread Spectrum (DSSS) and Binary Phase Shift Keying (BPSK) Modulation
Abstract
1. Introduction
2. Theory of Underwater Electric Field Communication
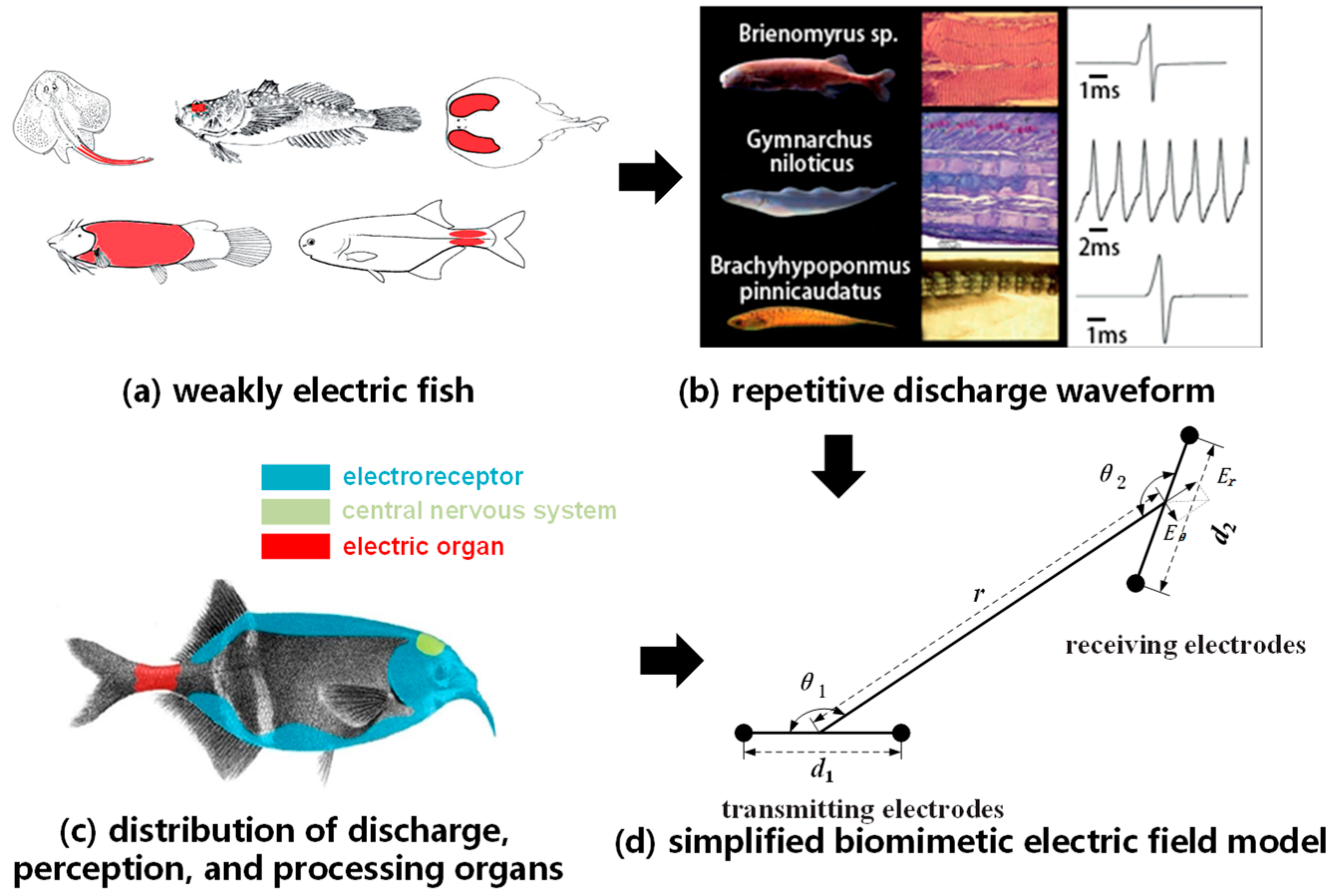
2.1. Analysis of Electric Field Sensing Mechanism of Weak Electric Fish
2.2. Electric Field Communication Model
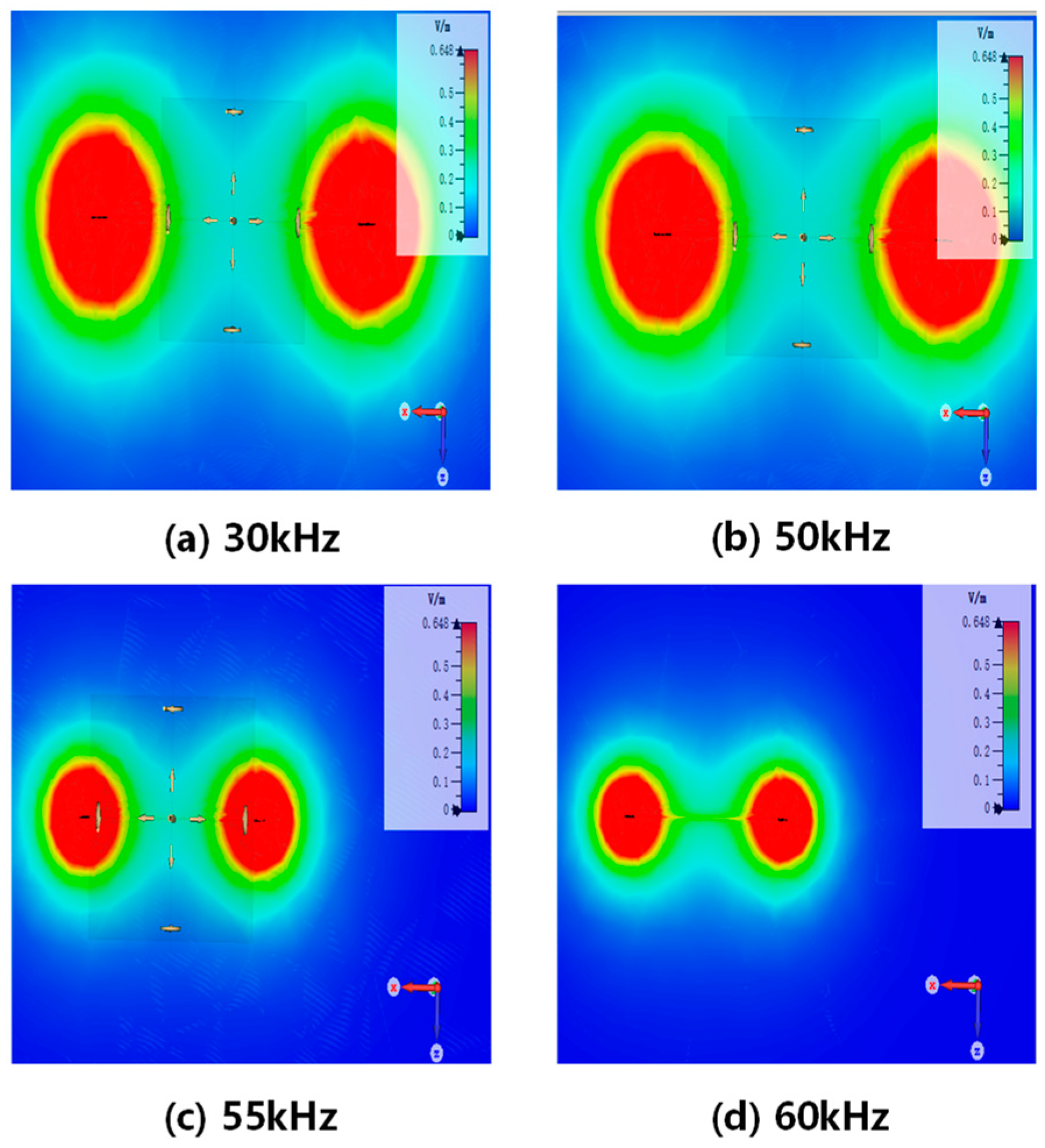
2.3. Electric Dipole Field Strength
3. DSSS in Electric Field Communication
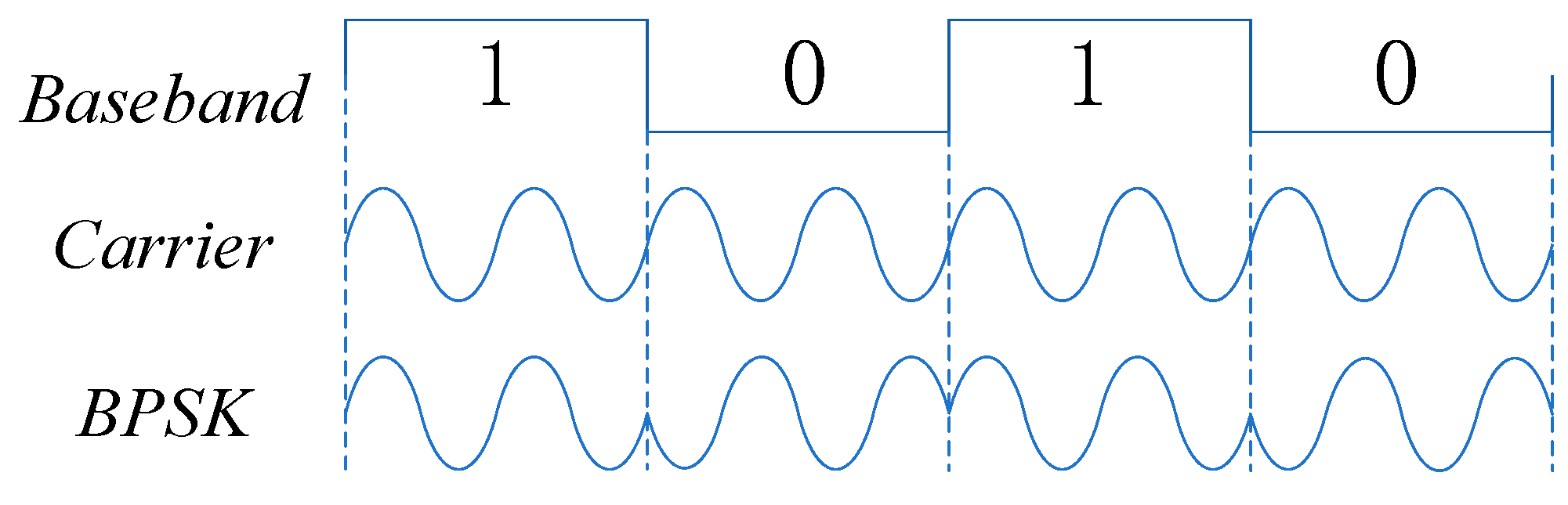
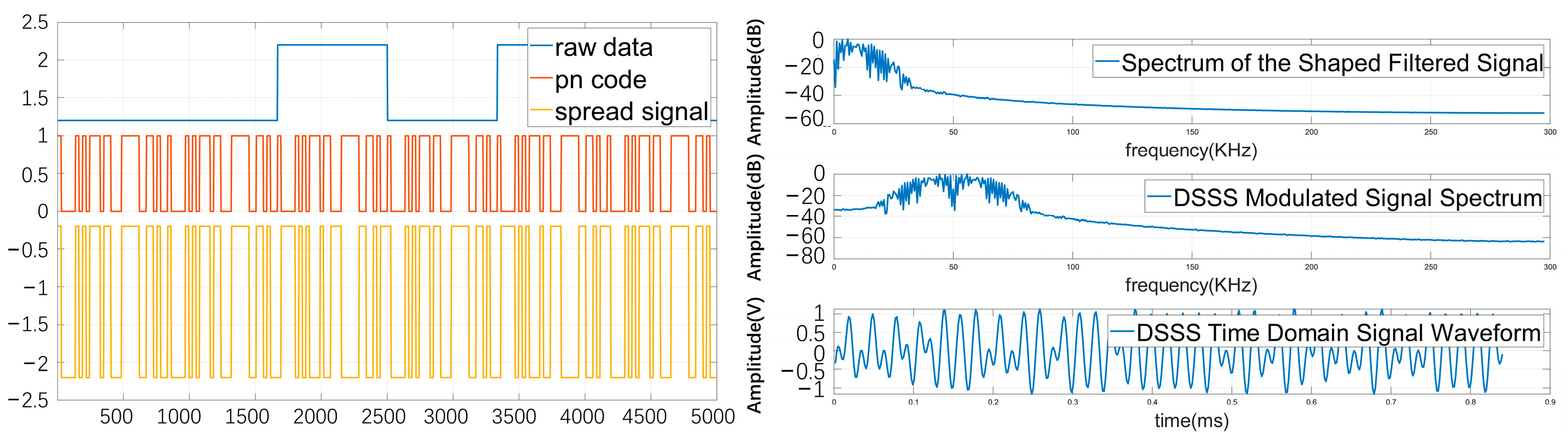
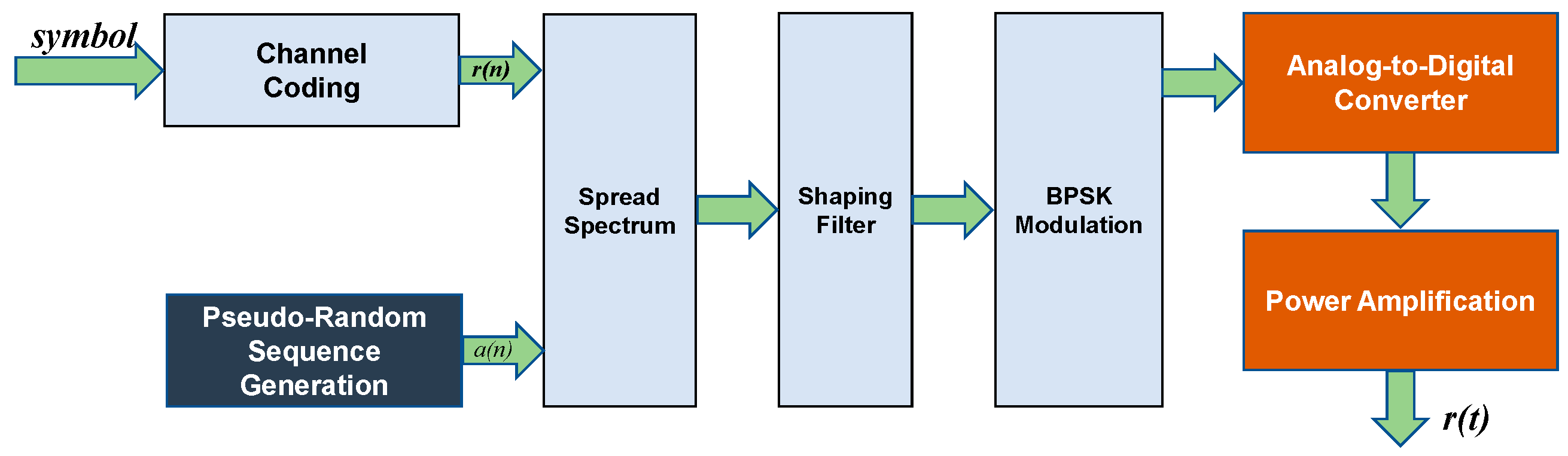
3.1. The Modulation of DSSS BPSK Signals
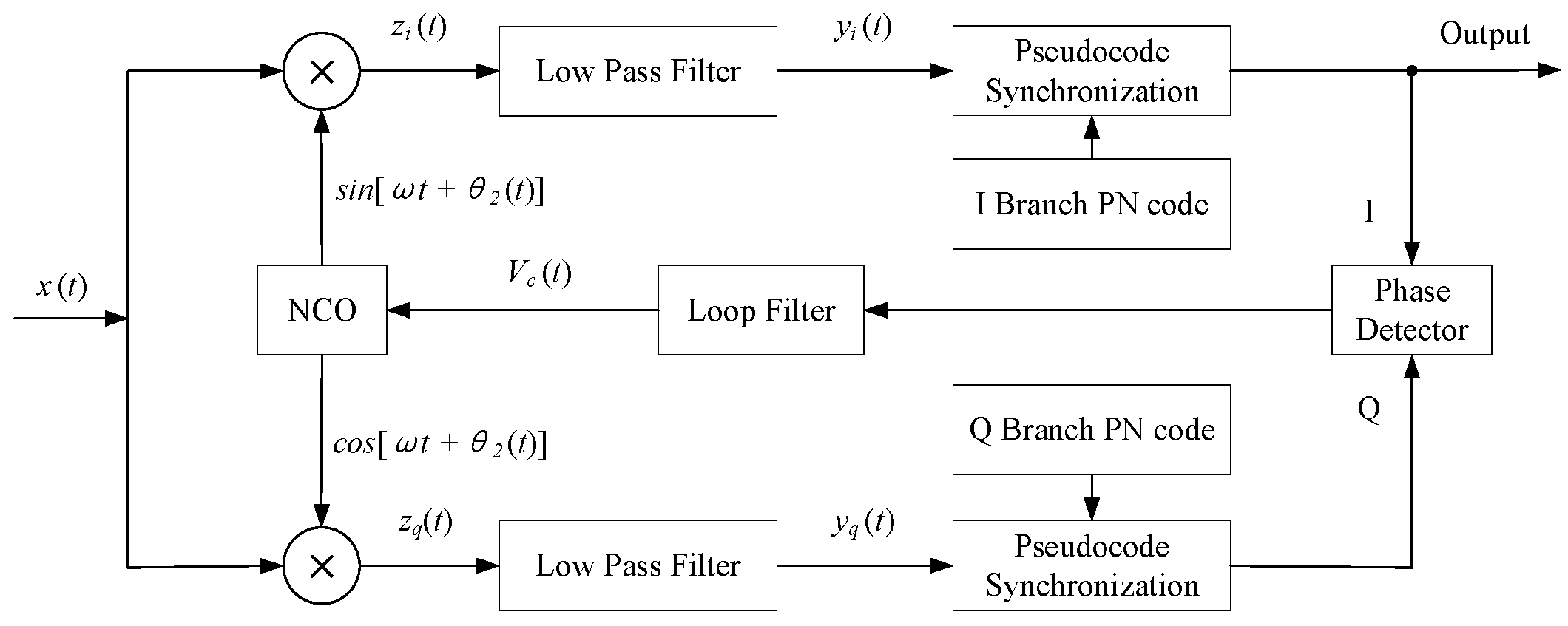
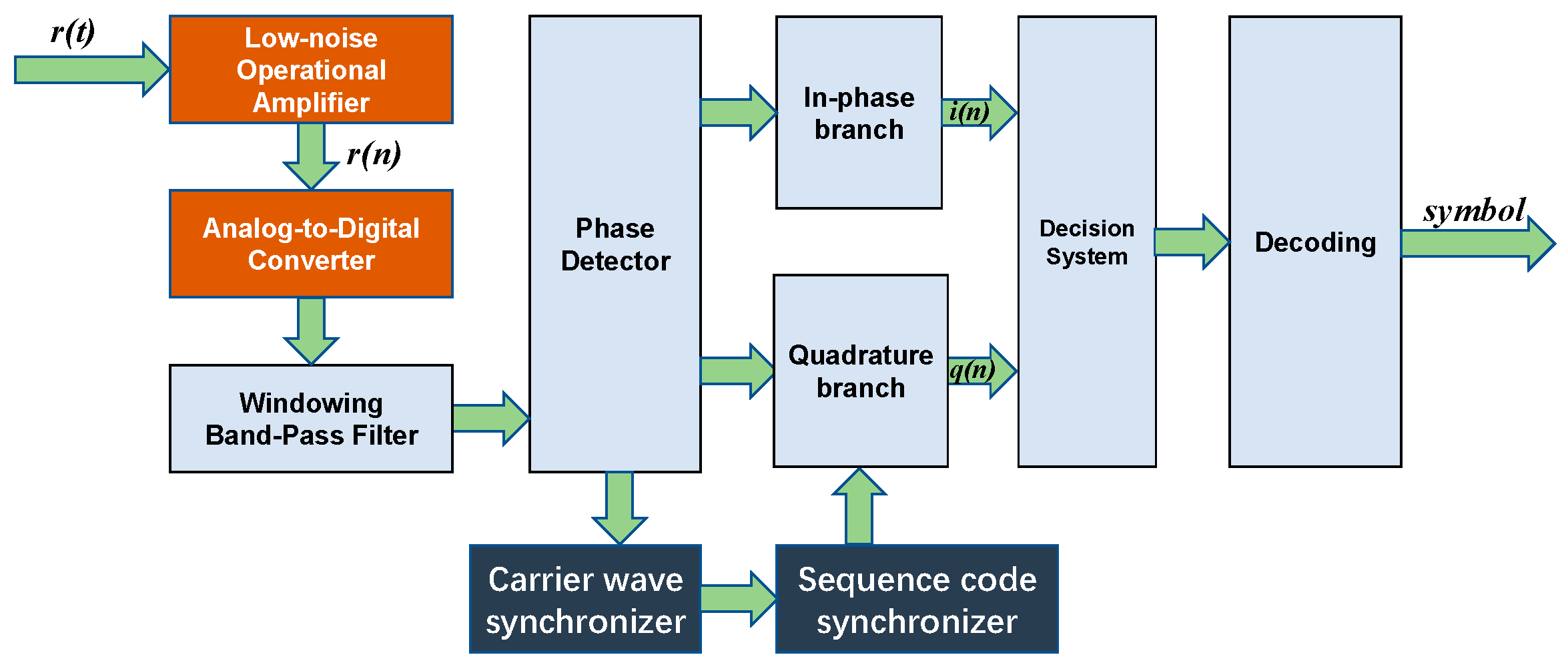
3.2. The Demodulation of DSSS BPSK Signals
3.3. Design of the Electric Field Communication System
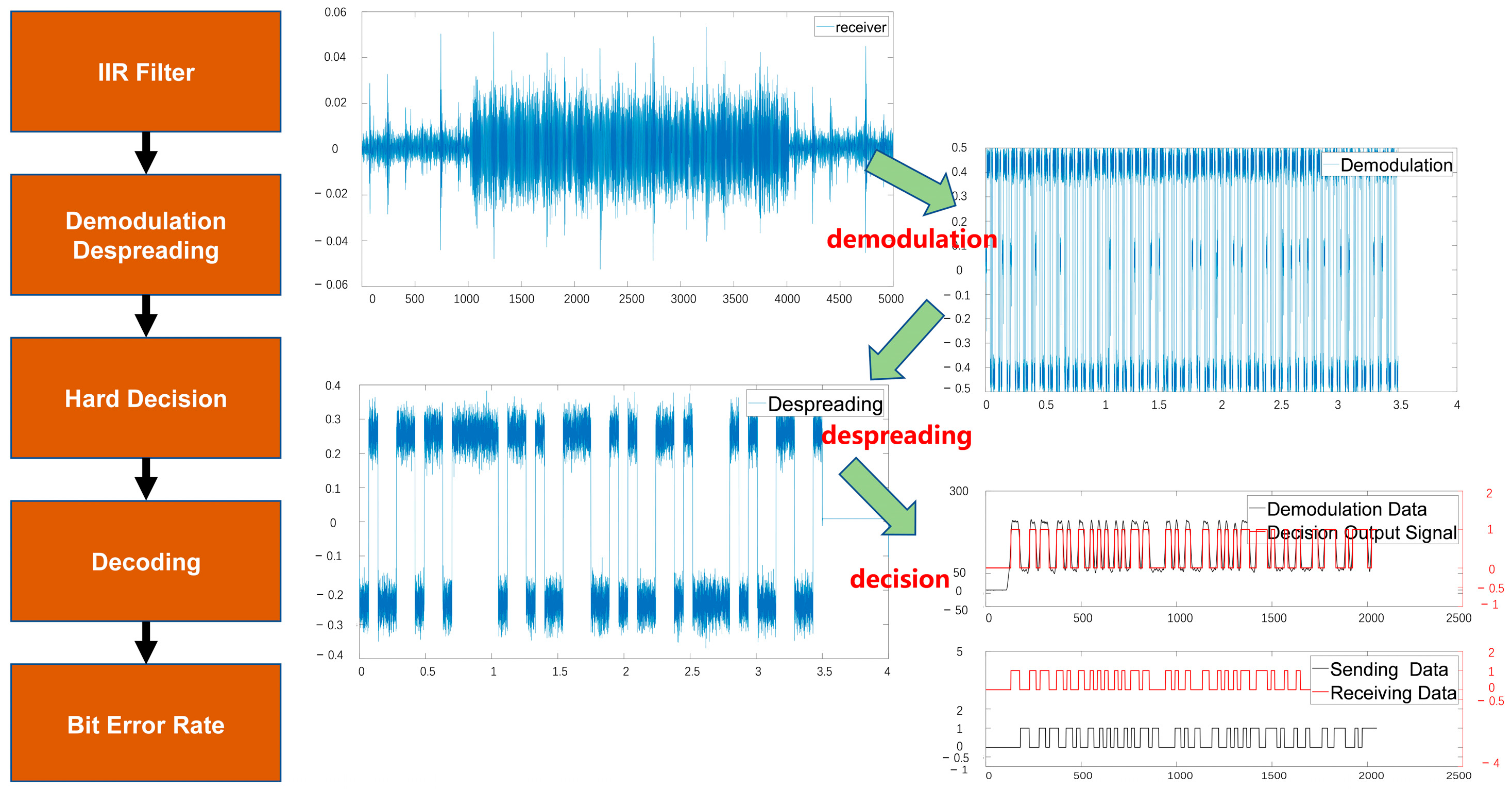
4. Experimental Verification
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Song, H.C. Long-range acoustic communication in deep water using a towed array: Beam diversity. In Proceedings of the 2012 Oceans—Yeosu, Yeosu, Republic of Korea, 21–24 May 2012; p. 4. [Google Scholar]
- Kilfoyle, D.B.; Baggeroer, A.B. The state of the art in underwater acoustic telemetry. IEEE J. Ocean. Eng. 2000, 25, 4–27. [Google Scholar] [CrossRef]
- Zia, M.Y.I.; Poncela, J.; Otero, P. State-of-the-art underwater acoustic communication modems: Classifications, analyses and design challenges. Wirel. Pers. Commun. 2021, 116, 1325–1360. [Google Scholar] [CrossRef]
- Zeng, Z.; Fu, S.; Zhang, H.; Dong, Y.; Cheng, J. A Survey of Underwater Optical Wireless Communications. IEEE Commun. Surv. Tutor. 2017, 19, 204–238. [Google Scholar] [CrossRef]
- Zhu, S.; Chen, X.; Liu, X.; Zhang, G.; Tian, P. Recent progress in and perspectives of underwater wireless optical communication. Prog. Quantum Electron. 2020, 73, 100274. [Google Scholar] [CrossRef]
- Aissaoui, A.; Hacini, L. Enhancing UWOC link performance using a hybrid OFDM/SAC-OCDMA system. Opt. Quantum Electron. 2024, 56, 28. [Google Scholar] [CrossRef]
- Scapin, A. Electrocommunication for weakly electric fish. Inverse Probl. Imaging 2020, 14, 97–115. [Google Scholar] [CrossRef]
- Ariel Caputi, A. The bioinspiring potential of weakly electric fish. Bioinspiration Biomim. 2017, 12, 025004. [Google Scholar] [CrossRef]
- Joe, J.; Toh, S.H. Digital underwater communication using electric current method. In Proceedings of the OCEANS 2007—Europe, Aberdeen, UK, 18–21 June 2007; pp. 1160–1163. [Google Scholar]
- Kim, C.W.; Lee, E.; Syed, N.A.A. Channel characterization for underwater electric conduction communications systems. In Proceedings of the OCEANS 2010 MTS/IEEE SEATTLE, Seattle, WA, USA, 20–23 September 2010; pp. 1–6. [Google Scholar]
- Friedman, J.K. Electrostatic Transconduction for Underwater Communication and Imaging. Ph.D. Thesis, University of California, Los Angeles, CA, USA, 2009. [Google Scholar]
- Friedman, J.K. Biomimetic Electrostatics for Submerged Oceanic Sensing, Communication, and Coordination; University of California: Los Angeles, CA, USA, 2012. [Google Scholar]
- Rauf, M.; Aamir, M.; Khan, A.M. A Prospect of Efficient radio-frequency based underwater Wireless Data Transfer. In Proceedings of the 2020 Global Conference on Wireless and Optical Technologies (GCWOT), Malaga, Spain, 6–8 October 2020; pp. 1–5. [Google Scholar]
- Rauf, M.; Jamil, A.; Dawood Idrees, M.; Ansari, A.; Sami, A. Surface Waves Analysis of Efficient Underwater Radio-Based Wireless Link. Math. Probl. Eng. 2021, 2021, 4186036. [Google Scholar] [CrossRef]
- Makinwa, S.; van der Laan, D. Communication System for a Deepsea Robot: Analysis of Underwater Communication Using Electric Quasi-Static Fields. Bachelor Thesis, Delft University of Technology, Delft, The Netherlands, 2022. [Google Scholar]
- Zhao, H.; Xu, M.; Shu, M.; An, J.; Ding, W.; Liu, X.; Wang, S.; Zhao, C.; Yu, H.; Wang, H. Underwater wireless communication via TENG-generated Maxwell’s displacement current. Nat. Commun. 2022, 13, 3325. [Google Scholar] [CrossRef] [PubMed]
- Donati, E.; Worm, M.; Mintchev, S.; van der Wiel, M.; Benelli, G.; von der Emde, G.; Stefanini, C. Investigation of Collective Behaviour and Electrocommunication in the Weakly Electric Fish, Mormyrus rume, through a biomimetic Robotic Dummy Fish. Bioinspiration Biomim. 2016, 11, 066009. [Google Scholar] [CrossRef] [PubMed]
- Lebastard, V.; Boyer, F.; Lanneau, S. Reactive underwater object inspection based on artificial electric sense. Bioinspiration Biomim. 2016, 11, 045003. [Google Scholar] [CrossRef] [PubMed]
- Liu, Y.; Ning, P.; Dai, H.; Liu, A. Randomized Differential DSSS: Jamming-Resistant Wireless Broadcast Communication. In Proceedings of the Conference on IEEE INFOCOM, San Diego, CA, USA, 14–19 March 2010. [Google Scholar]
- Al-Heeti, M.M.; Fadhel, M.A. Comparative Analysis for Different Digital Modulation Techniques. J. Southwest Jiaotong Univ. 2020, 55. [Google Scholar] [CrossRef]
- Paillier, L.; Le Bidan, R.; Conan, J.-M.; Artaud, G.; Vedrenne, N.; Jaouën, Y. Space-ground coherent optical links: Ground receiver performance with adaptive optics and digital phase-locked loop. J. Light. Technol. 2020, 38, 5716–5727. [Google Scholar] [CrossRef]
- Hirokawa, T.; Pinna, S.; Hosseinzadeh, N.; Maharry, A.; Andrade, H.; Liu, J.; Meissner, T.; Misak, S.; Movaghar, G.; Valenzuela, L.A. Analog coherent detection for energy efficient intra-data center links at 200 Gbps per wavelength. J. Light. Technol. 2020, 39, 520–531. [Google Scholar] [CrossRef]
- Zhang, C.; Wang, J.; Yin, C.; Zhang, B.; Liu, R.; Xi, L.; Li, M. Research progress of optical phase-locked loop. Infrared Laser Eng. 2022, 51, 20210156. [Google Scholar]
- Cao, C.; Hu, N.; Xiong, S.; Xia, J.; Hou, Q. Phase Noise Characteristics of Remote Amplification for Fiber-Optic Hydrophone System. Acta Opt. Sin. 2023, 43, 1106001. [Google Scholar] [CrossRef]
- Kochańska, I.; Schmidt, J. Simulation of direct-sequence spread spectrum data transmission system for reliable underwater acoustic communications. Vib. Phys. Syst. 2019, 30, 1–8. [Google Scholar]
- Li, J.; Yang, B.; Ye, D.; Wang, L.; Fu, K.; Piao, J.; Wang, Y. A real-time, full-duplex system for underwater wireless optical communication: Hardware structure and optical link model. IEEE Access 2020, 8, 109372–109387. [Google Scholar] [CrossRef]
- Fuada, S.; Adiono, T.; Pradana, A. Employing LM13700 as AGC for mobile visible light communication system. Int. J. Electr. Electron. Eng. Telecommun. 2020, 9, 88–93. [Google Scholar] [CrossRef]
- Lu, T.; Hu, Q.; Xu, D.; Feng, X.; Zhang, Y. Design and Verification of Underwater Electric Field Communication System Based Spread Spectrum Techniques. In Proceedings of the International Conference on Autonomous Unmanned Systems, Xi’an, China, 23–25 September 2022; pp. 1421–1431. [Google Scholar]




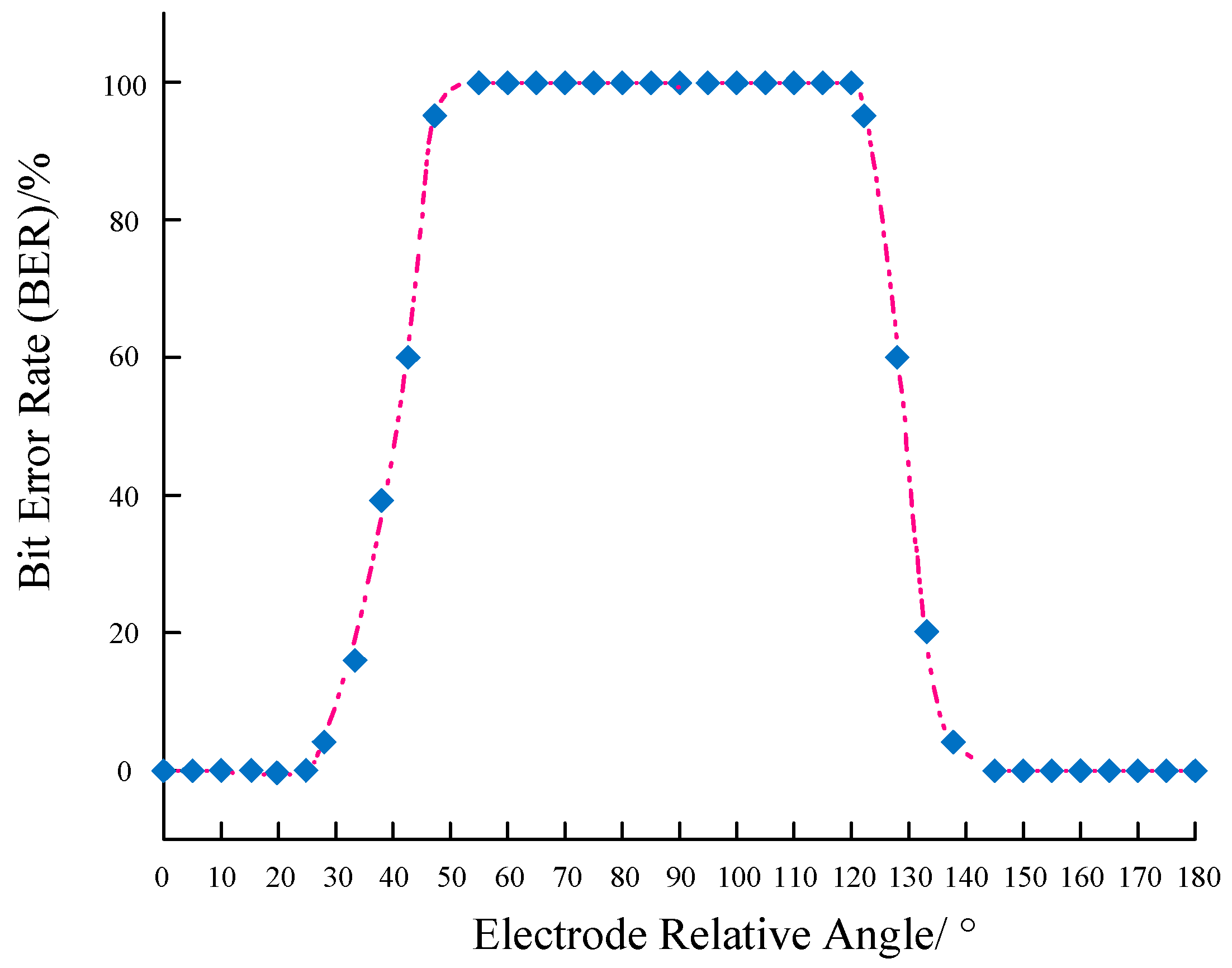
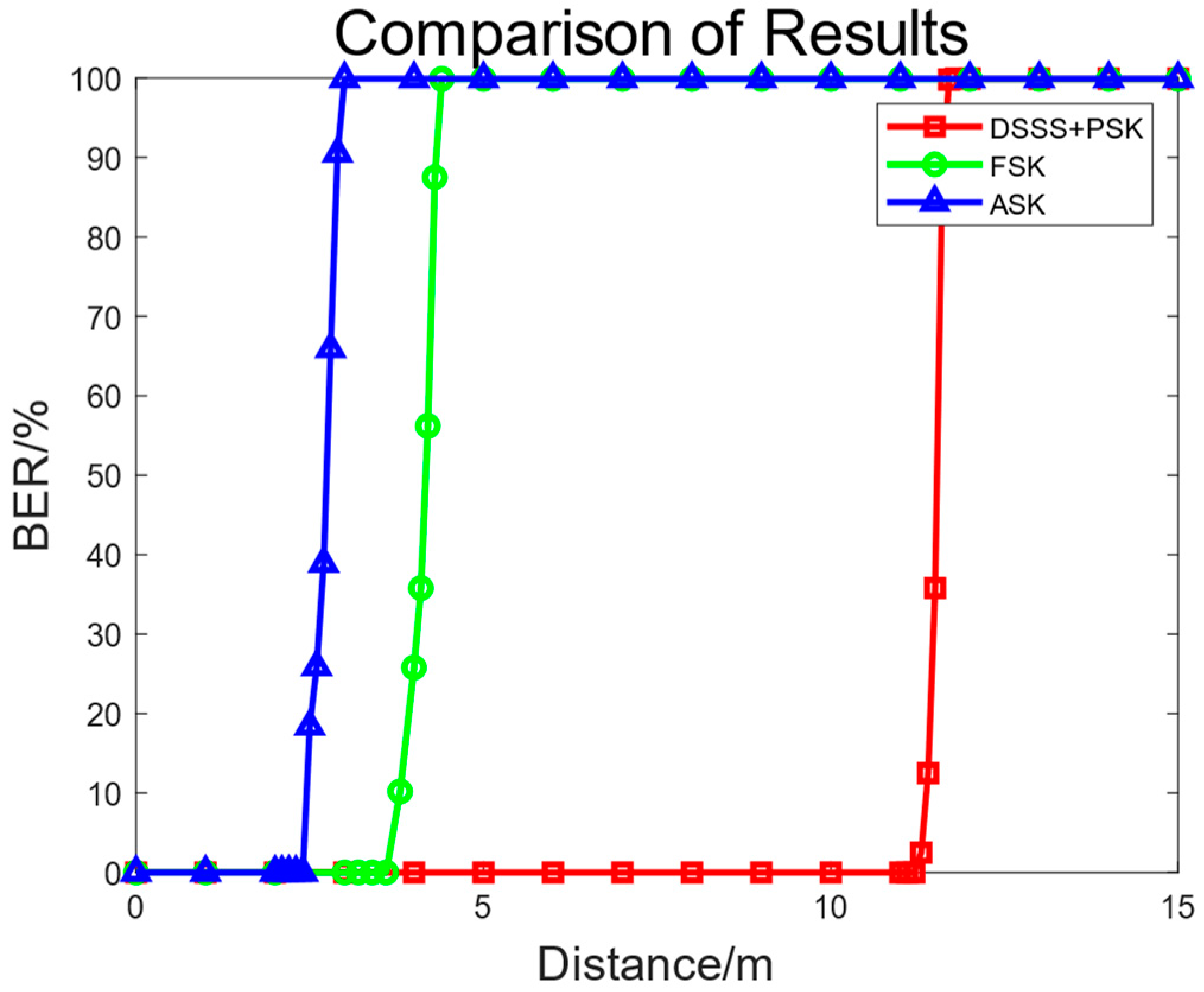
| Parameter | Value |
|---|---|
| Model dimensions | 800 m × 800 m × 1000 m |
| Electrical conductivity | |
| Relative permittivity | |
| Relative permeability | |
| Electric dipole size | 15 mm × 15 mm × 0.2 mm |
| Distance between electric dipoles | 1 m |
| Current between electric dipoles | 0.5 A |
| Modulation | The Coherent Demodulation BER (Pe) | The Non-Coherent Demodulation BER (Pe) |
|---|---|---|
| 2ASK | ||
| 2FSK | ||
| 2PSK (BPSK) |
| Parameter | Value |
|---|---|
| Original signal rate | Rb = 1200 bps |
| Fifth Order primitive polynomial | polynomial = [1 0 0 1 0 1] |
| Pseudocode sequence length | LPN = 31 |
| Pseudocode rate | Rc = LPN × Rb = 37,200 chip/s |
| Sampling frequency | fs = 8Rc = 297,600 Hz |
| Carrier frequency | fc = 50 KHz |
| Shaping filter roll-off factor | α = 0.8 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Y.; Zhao, Z.; Feng, X.; Zhao, T.; Hu, Q. Implementation of Underwater Electric Field Communication Based on Direct Sequence Spread Spectrum (DSSS) and Binary Phase Shift Keying (BPSK) Modulation. Biomimetics 2024, 9, 103. https://doi.org/10.3390/biomimetics9020103
Zhang Y, Zhao Z, Feng X, Zhao T, Hu Q. Implementation of Underwater Electric Field Communication Based on Direct Sequence Spread Spectrum (DSSS) and Binary Phase Shift Keying (BPSK) Modulation. Biomimetics. 2024; 9(2):103. https://doi.org/10.3390/biomimetics9020103
Chicago/Turabian StyleZhang, Yuzhong, Zhenyi Zhao, Xinglong Feng, Tianyi Zhao, and Qiao Hu. 2024. "Implementation of Underwater Electric Field Communication Based on Direct Sequence Spread Spectrum (DSSS) and Binary Phase Shift Keying (BPSK) Modulation" Biomimetics 9, no. 2: 103. https://doi.org/10.3390/biomimetics9020103
APA StyleZhang, Y., Zhao, Z., Feng, X., Zhao, T., & Hu, Q. (2024). Implementation of Underwater Electric Field Communication Based on Direct Sequence Spread Spectrum (DSSS) and Binary Phase Shift Keying (BPSK) Modulation. Biomimetics, 9(2), 103. https://doi.org/10.3390/biomimetics9020103





