Biomechanical and Sensory Feedback Regularize the Behavior of Different Locomotor Central Pattern Generators
Abstract
1. Introduction
2. Methods
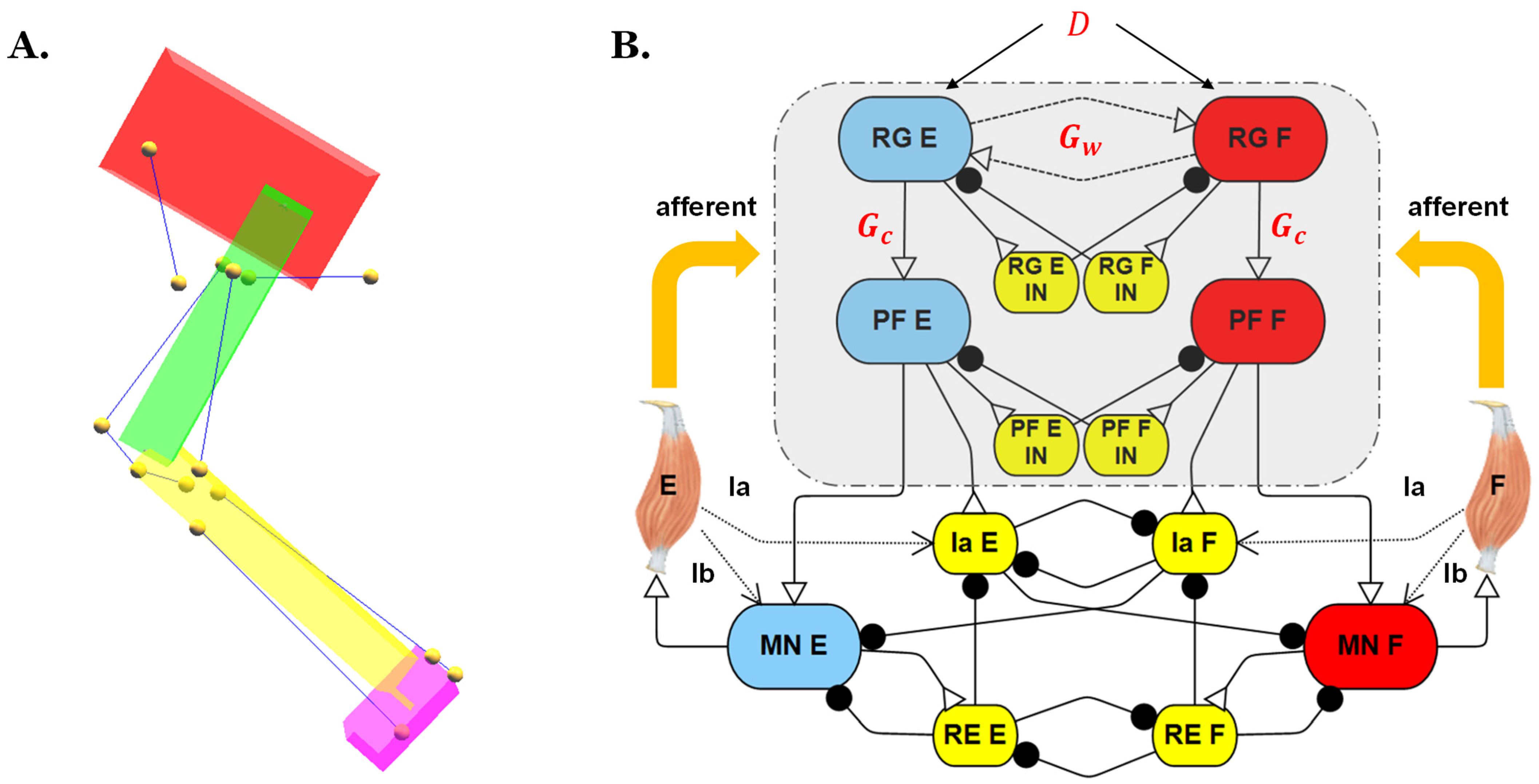
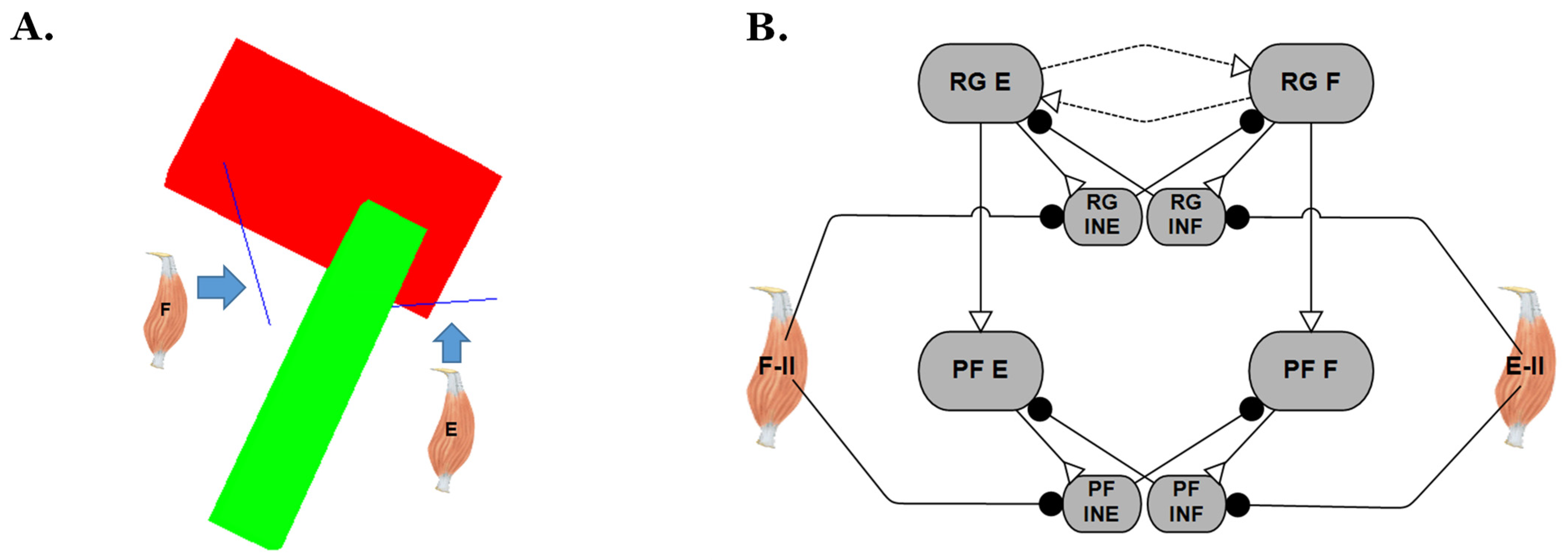
2.1. Neuromechanical Model
2.2. Neural Model
2.3. Bifurcation Parameter
2.4. Overview of Analysis
3. Results
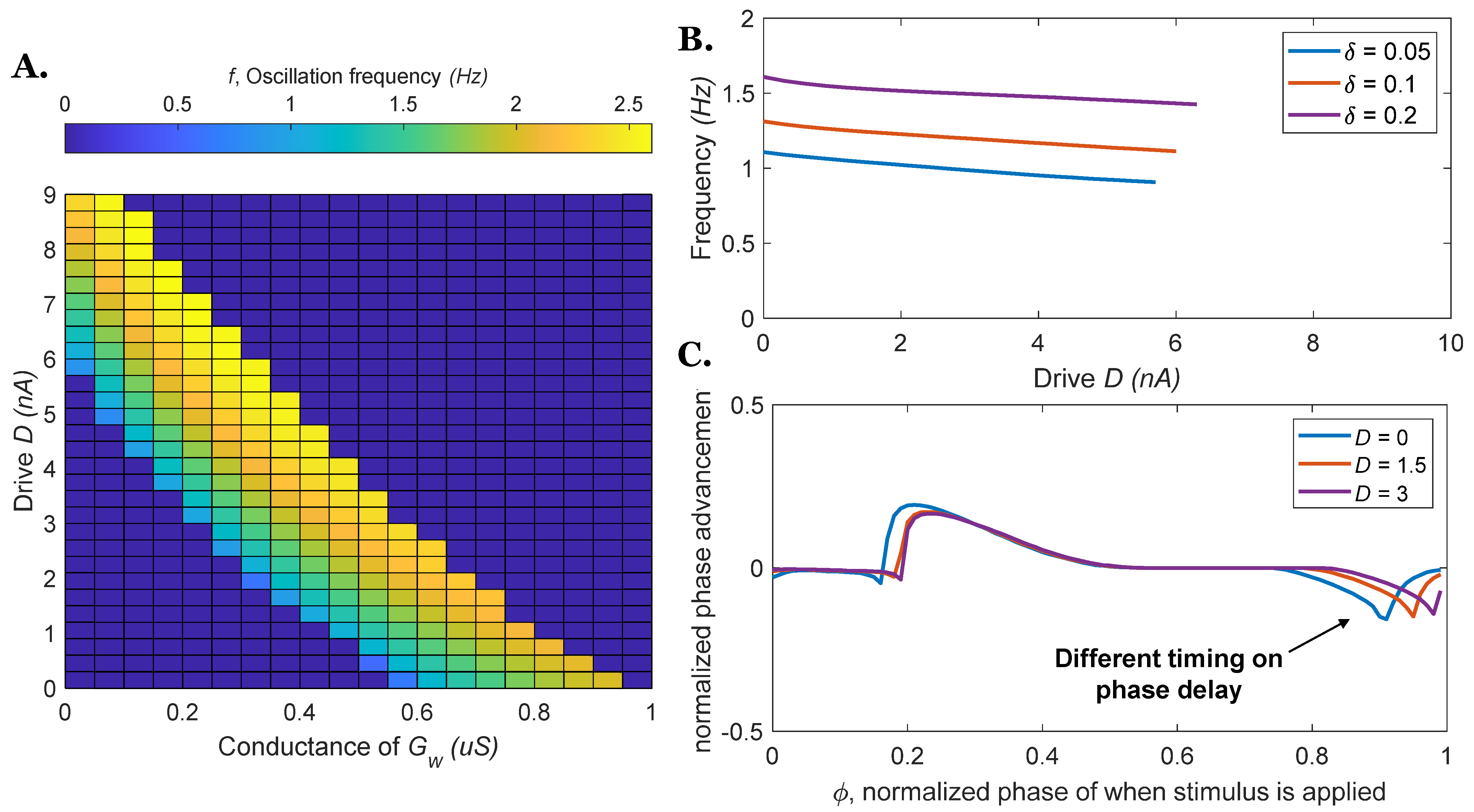
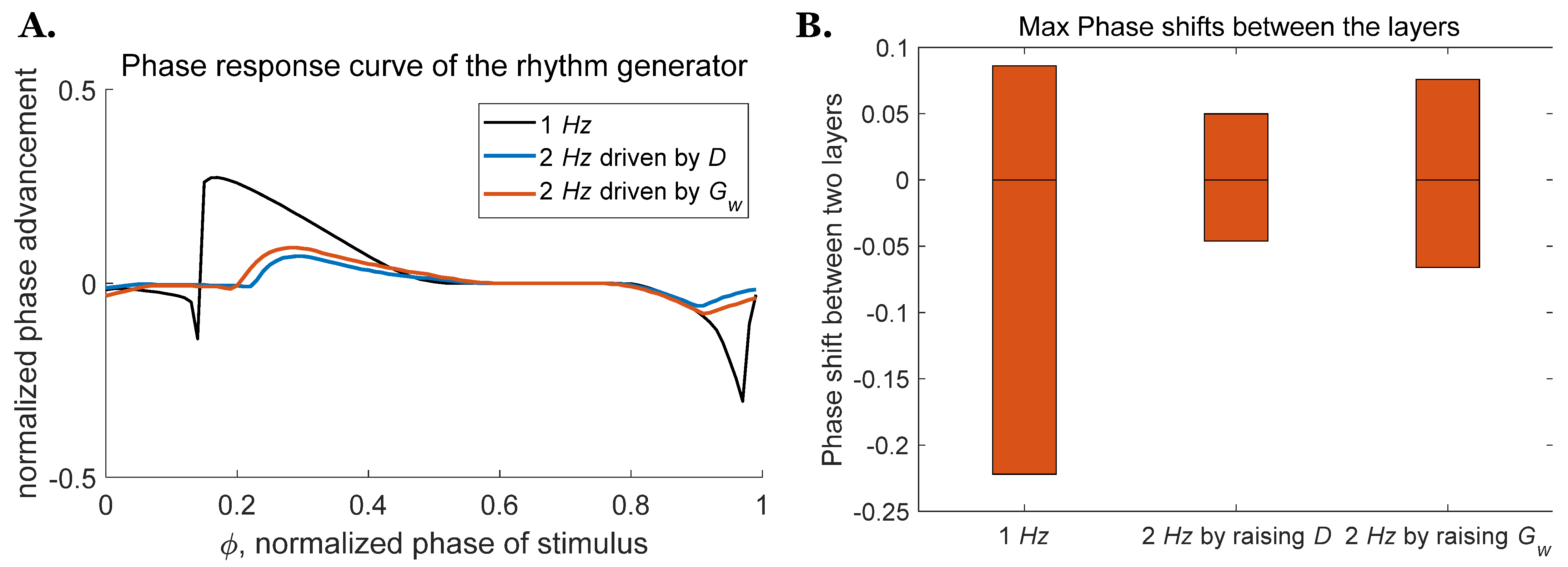
3.1. The Effect of Variations in the Strength of Reciprocal Excitatory Connections and Descending Drive on the Performance of the Rhythm-Generating Layer
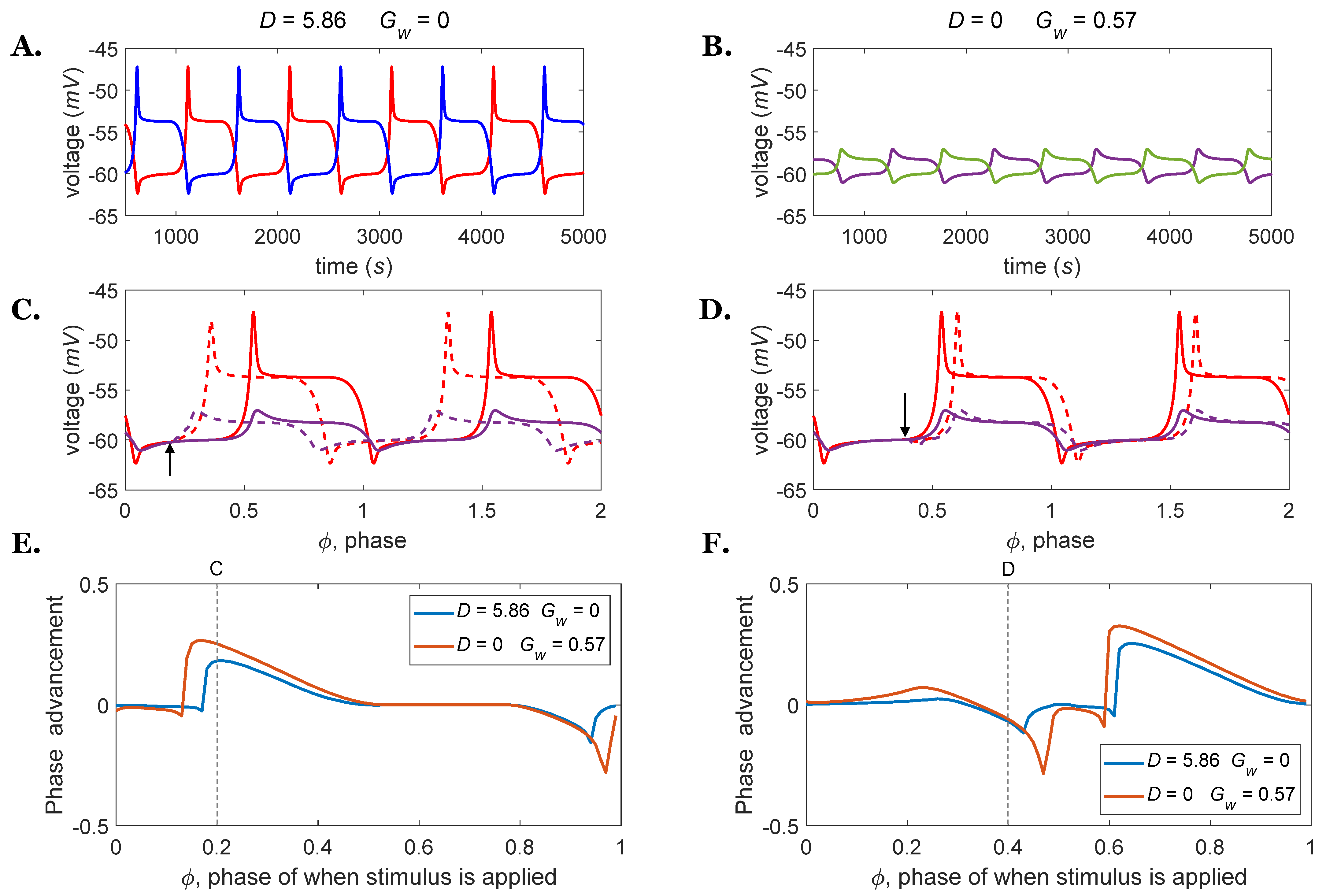
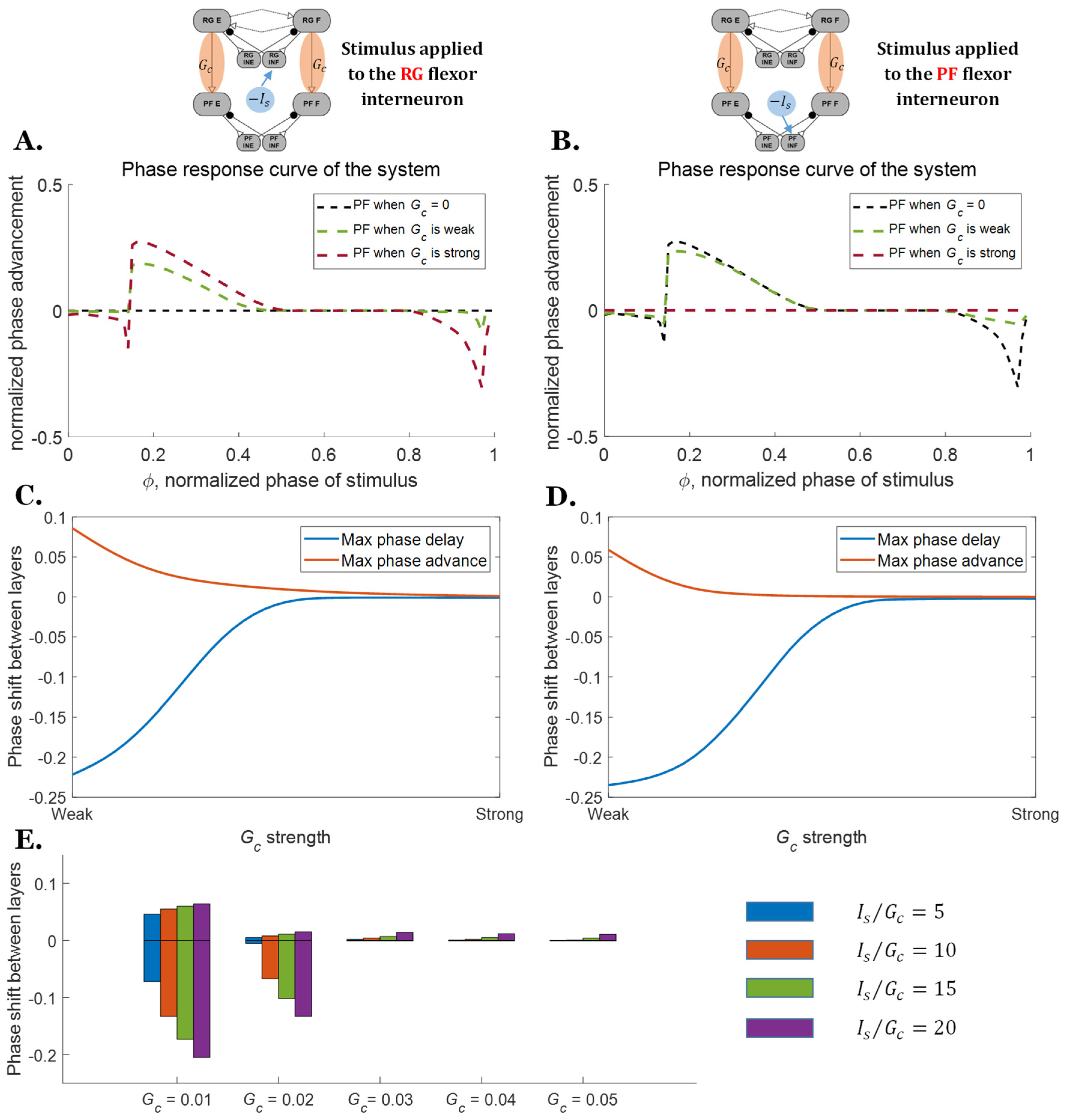
3.2. The Effect of Variations in the Connection Strength between Rhythm Generating and Pattern Formation Layers
3.2.1. Effect of Signal Transmission Strength between Two Layers
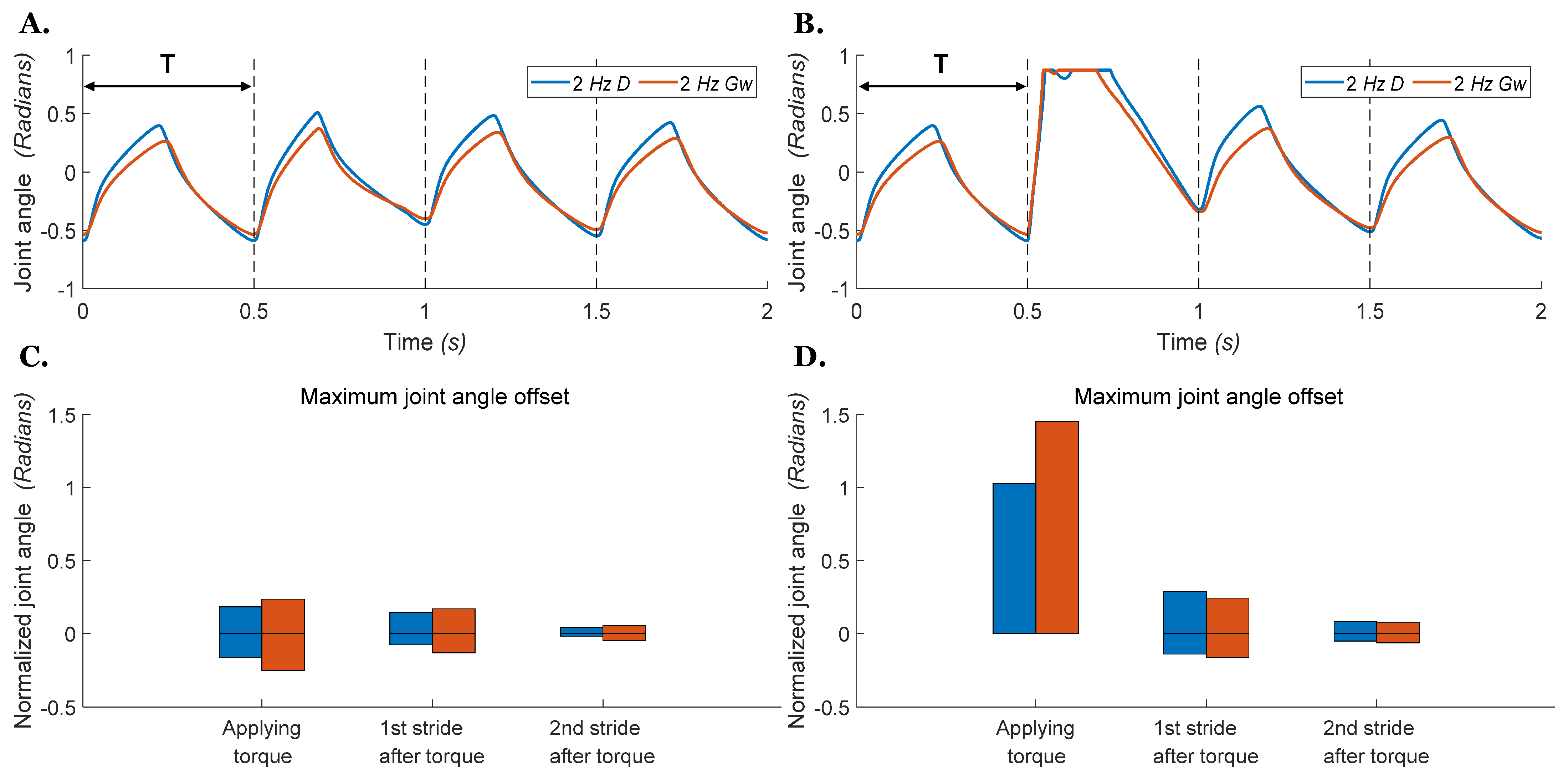
3.2.2. Speed Modulation
3.3. Performance of Two-Layer CPG with Simulated Mechanical Model
4. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
Appendix A
| Neuron | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| RG | 5 | 1 | −60 | 1.5 | 0.5 | −0.6 | −60 | 0.35 | 1 | 0.2 | −40 | 2 |
| PF | 5 | 1 | −60 | 1.5 | 0.5 | −0.6 | −60 | 0.35 | 1 | 0.2 | −40 | 2 |
| MN | 5 | 1 | −100 | 0 | - | - | - | - | - | - | - | - |
| IN | 5 | 1 | −60 | 0 | - | - | - | - | - | - | - | - |
| Synapse | ||||||
|---|---|---|---|---|---|---|
| RG to IN | 1.625 | - | - | −40 | −60 | −25 |
| IN to RG | 1.625 | - | - | −70 | −60 | −25 |
| Between RG | 0.01 | - | - | −40 | −65 | −40 |
| PF to IN | 1.613 | - | - | −40 | −60 | −25 |
| IN to PF | 1.613 | - | - | −70 | −60 | −25 |
| RG to PF | 0.1 | - | - | −40 | −60 | −40 |
| PF to MN | - | - | - | −10 | −60 | −50 |
| Hip | - | 2.546 | 3.722 | - | −60 | −40 |
| Knee | - | 1.6 | 2 | - | −60 | −40 |
| Ankle | - | 3.2 | 4.5 | - | −60 | −40 |
| PF to Ia | 0.5 | - | - | −40 | −60 | −55 |
| Between Ia | 0.5 | - | - | −70 | −60 | −40 |
| Ia to MN | 2 | - | - | −100 | −60 | −40 |
| MN to RE | 0.5 | - | - | −40 | −100 | −10 |
| Between R | 0.5 | - | - | −70 | −60 | −40 |
| R to MN | 0.5 | - | - | −100 | −60 | −40 |
| R to Ia | 0.5 | - | - | −70 | −60 | −40 |
References
- Forssberg, H.; Grillner, S.; Halbertsma, J.; Rossignol, S. The Locomotion of the Low Spinal Cat. II. Interlimb Coordination. Acta Physiol. Scand. 1980, 108, 283–295. [Google Scholar] [CrossRef] [PubMed]
- Frigon, A.; Hurteau, M.-F.; Thibaudier, Y.; Leblond, H.; Telonio, A.; D’Angelo, G. Split-Belt Walking Alters the Relationship between Locomotor Phases and Cycle Duration across Speeds in Intact and Chronic Spinalized Adult Cats. J. Neurosci. 2013, 33, 8559–8566. [Google Scholar] [CrossRef] [PubMed]
- Frigon, A.; Thibaudier, Y.; Hurteau, M.-F. Modulation of Forelimb and Hindlimb Muscle Activity during Quadrupedal Tied-Belt and Split-Belt Locomotion in Intact Cats. Neuroscience 2015, 290, 266–278. [Google Scholar] [CrossRef] [PubMed]
- Grillner, S.; McCLELLAN, A.; Sigvardt, K.; Wallén, P.; Wilén, M. Activation of NMDA-Receptors Elicits “Fictive Locomotion” in Lamprey Spinal Cord in Vitro. Acta Physiol. Scand. 1981, 113, 549–551. [Google Scholar] [CrossRef] [PubMed]
- Brown, T.G. On the Nature of the Fundamental Activity of the Nervous Centres; Together with an Analysis of the Conditioning of Rhythmic Activity in Progression, and a Theory of the Evolution of Function in the Nervous System. J. Physiol. 1914, 48, 18–46. [Google Scholar] [CrossRef]
- Hägglund, M.; Dougherty, K.J.; Borgius, L.; Itohara, S.; Iwasato, T.; Kiehn, O. Optogenetic Dissection Reveals Multiple Rhythmogenic Modules Underlying Locomotion. Proc. Natl. Acad. Sci. USA 2013, 110, 11589–11594. [Google Scholar] [CrossRef]
- Ekeberg, Ö. A Combined Neuronal and Mechanical Model of Fish Swimming. Biol. Cybern. 1993, 69, 363–374. [Google Scholar] [CrossRef]
- Szczecinski, N.S.; Brown, A.E.; Bender, J.A.; Quinn, R.D.; Ritzmann, R.E. A Neuromechanical Simulation of Insect Walking and Transition to Turning of the Cockroach Blaberus Discoidalis. Biol. Cybern. 2014, 108, 1–21. [Google Scholar] [CrossRef]
- Hunt, A.J.; Szczecinski, N.S.; Andrada, E.; Fischer, M.; Quinn, R.D. Using Animal Data and Neural Dynamics to Reverse Engineer a Neuromechanical Rat Model. In Proceedings of the Biomimetic and Biohybrid Systems; Wilson, S.P., Verschure, P.F.M.J., Mura, A., Prescott, T.J., Eds.; Springer International Publishing: Cham, Switzerland, 2015; pp. 211–222. [Google Scholar]
- Daun, S.; Rubin, J.E.; Rybak, I.A. Control of Oscillation Periods and Phase Durations in Half-Center Central Pattern Generators: A Comparative Mechanistic Analysis. J. Comput. Neurosci. 2009, 27, 3–36. [Google Scholar] [CrossRef]
- Spardy, L.E.; Markin, S.N.; Shevtsova, N.A.; Prilutsky, B.I.; Rybak, I.A.; Rubin, J.E. A Dynamical Systems Analysis of Afferent Control in a Neuromechanical Model of Locomotion: I. Rhythm Generation. J. Neural Eng. 2011, 8, 065003. [Google Scholar] [CrossRef]
- Ausborn, J.; Snyder, A.C.; Shevtsova, N.A.; Rybak, I.A.; Rubin, J.E. State-Dependent Rhythmogenesis and Frequency Control in a Half-Center Locomotor CPG. J. Neurophysiol. 2018, 119, 96–117. [Google Scholar] [CrossRef]
- Fujiki, S.; Aoi, S.; Tsuchiya, K.; Danner, S.M.; Rybak, I.A.; Yanagihara, D. Phase-Dependent Response to Afferent Stimulation During Fictive Locomotion: A Computational Modeling Study. Front. Neurosci. 2019, 13, 1288. [Google Scholar] [CrossRef]
- Hunt, A.; Szczecinski, N.; Quinn, R. Development and Training of a Neural Controller for Hind Leg Walking in a Dog Robot. Front. Neurorobot. 2017, 11, 18. [Google Scholar] [CrossRef]
- Ijspeert, A.J.; Crespi, A.; Ryczko, D.; Cabelguen, J.-M. From Swimming to Walking with a Salamander Robot Driven by a Spinal Cord Model. Science 2007, 315, 1416–1420. [Google Scholar] [CrossRef]
- Kimura, H.; Fukuoka, Y.; Cohen, A.H. Adaptive Dynamic Walking of a Quadruped Robot on Natural Ground Based on Biological Concepts. Int. J. Robot. Res. 2016, 26, 475–490. [Google Scholar] [CrossRef]
- Szczecinski, N.S.; Chrzanowski, D.M.; Cofer, D.W.; Terrasi, A.S.; Moore, D.R.; Martin, J.P.; Ritzmann, R.E.; Quinn, R.D. Introducing MantisBot: Hexapod Robot Controlled by a High-Fidelity, Real-Time Neural Simulation. In Proceedings of the 2015 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Hamburg, Germany, 28 September–3 October 2015; IEEE: Hamburg, Germany, 2015; pp. 3875–3881. [Google Scholar]
- McCrea, D.A.; Rybak, I.A. Organization of Mammalian Locomotor Rhythm and Pattern Generation. Brain Res. Rev. 2008, 57, 134–146. [Google Scholar] [CrossRef]
- Grillner, S.; Zangger, P. On the Central Generation of Locomotion in the Low Spinal Cat. Exp. Brain Res. 1979, 34, 241–261. [Google Scholar] [CrossRef]
- Rybak, I.A.; Shevtsova, N.A.; Lafreniere-Roula, M.; McCrea, D.A. Modelling Spinal Circuitry Involved in Locomotor Pattern Generation: Insights from Deletions during Fictive Locomotion. J. Physiol. 2006, 577, 617–639. [Google Scholar] [CrossRef]
- Deng, K.; Szczecinski, N.S.; Arnold, D.; Andrada, E.; Fischer, M.S.; Quinn, R.D.; Hunt, A.J. Neuromechanical Model of Rat Hindlimb Walking with Two-Layer CPGs. Biomimetics 2019, 4, 21. [Google Scholar] [CrossRef]
- Markin, S.N.; Klishko, A.N.; Shevtsova, N.A.; Lemay, M.A.; Prilutsky, B.I.; Rybak, I.A. A Neuromechanical Model of Spinal Control of Locomotion. In Neuromechanical Modeling of Posture and Locomotion; Prilutsky, B.I., Edwards, D.H., Eds.; Springer Series in Computational Neuroscience; Springer: New York, NY, USA, 2016; pp. 21–65. ISBN 978-1-4939-3267-2. [Google Scholar]
- Shevtsova, N.A. Two-Level Model of Mammalian Locomotor CPG. In Encyclopedia of Computational Neuroscience; Jaeger, D., Jung, R., Eds.; Springer: New York, NY, USA, 2013; pp. 1–21. ISBN 978-1-4614-7320-6. [Google Scholar]
- Cowley, K.C.; Schmidt, B.J. Effects of Inhibitory Amino Acid Antagonists on Reciprocal Inhibitory Interactions during Rhythmic Motor Activity in the in Vitro Neonatal Rat Spinal Cord. J. Neurophysiol. 1995, 74, 1109–1117. [Google Scholar] [CrossRef]
- Beato, M.; Nistri, A. Interaction Between Disinhibited Bursting and Fictive Locomotor Patterns in the Rat Isolated Spinal Cord. J. Neurophysiol. 1999, 82, 2029–2038. [Google Scholar] [CrossRef] [PubMed]
- Zhang, J.; Lanuza, G.M.; Britz, O.; Wang, Z.; Siembab, V.C.; Zhang, Y.; Velasquez, T.; Alvarez, F.J.; Frank, E.; Goulding, M. V1 and V2b Interneurons Secure the Alternating Flexor-Extensor Motor Activity Mice Require for Limbed Locomotion. Neuron 2014, 82, 138–150. [Google Scholar] [CrossRef] [PubMed]
- Pinsker, H.M. Aplysia Bursting Neurons as Endogenous Oscillators. I. Phase-Response Curves for Pulsed Inhibitory Synaptic Input. J. Neurophysiol. 1977, 40, 527–543. [Google Scholar] [CrossRef]
- Ayers, J.L.; Selverston, A.I. Monosynaptic Entrainment of an Endogenous Pacemaker Network: A Cellular Mechanism for von Holst’s Magnet Effect. J. Comp. Physiol. 1979, 129, 5–17. [Google Scholar] [CrossRef]
- Szczecinski, N.S.; Hunt, A.J.; Quinn, R.D. Design Process and Tools for Dynamic Neuromechanical Models and Robot Controllers. Biol. Cybern. 2017, 111, 105–127. [Google Scholar] [CrossRef]
- Tamura, D.; Aoi, S.; Funato, T.; Fujiki, S.; Senda, K.; Tsuchiya, K. Contribution of Phase Resetting to Adaptive Rhythm Control in Human Walking Based on the Phase Response Curves of a Neuromusculoskeletal Model. Front. Neurosci. 2020, 14, 17. [Google Scholar] [CrossRef]
- Serrancolí, G.; Alessandro, C.; Tresch, M.C. The Effects of Mechanical Scale on Neural Control and the Regulation of Joint Stability. Int. J. Mol. Sci. 2021, 22, 2018. [Google Scholar] [CrossRef]
- Cofer, D.; Cymbalyuk, G.; Reid, J.; Zhu, Y.; Heitler, W.J.; Edwards, D.H. AnimatLab: A 3D Graphics Environment for Neuromechanical Simulations. J. Neurosci. Methods 2010, 187, 280–288. [Google Scholar] [CrossRef]
- McVea, D.A.; Donelan, J.M.; Tachibana, A.; Pearson, K.G. A Role for Hip Position in Initiating the Swing-to-Stance Transition in Walking Cats. J. Neurophysiol. 2005, 94, 3497–3508. [Google Scholar] [CrossRef]
- Pearson, K.G. Role of Sensory Feedback in the Control of Stance Duration in Walking Cats. Brain Res. Rev. 2008, 57, 222–227. [Google Scholar] [CrossRef]
- Akay, T.; Tourtellotte, W.G.; Arber, S.; Jessell, T.M. Degradation of Mouse Locomotor Pattern in the Absence of Proprioceptive Sensory Feedback. Proc. Natl. Acad. Sci. USA 2014, 111, 16877–16882. [Google Scholar] [CrossRef] [PubMed]
- Wilson, H.R.; Cowan, J.D. Excitatory and Inhibitory Interactions in Localized Populations of Model Neurons. Biophys. J. 1972, 12, 1–24. [Google Scholar] [CrossRef] [PubMed]
- Szczecinski, N.S.; Quinn, R.D.; Hunt, A.J. Extending the Functional Subnetwork Approach to a Generalized Linear Integrate-and-Fire Neuron Model. Front. Neurorobotics 2020, 14, 577804. [Google Scholar] [CrossRef] [PubMed]
- Clarke, K.A.; Parker, A.J. A Quantitative Study of Normal Locomotion in the Rat. Physiol. Behav. 1986, 38, 345–351. [Google Scholar] [CrossRef]
- Takakusaki, K. Functional Neuroanatomy for Posture and Gait Control. J. Mov. Disord. 2017, 10, 1–17. [Google Scholar] [CrossRef]
- Cullins, M.J.; Gill, J.P.; McManus, J.M.; Lu, H.; Shaw, K.M.; Chiel, H.J. Sensory Feedback Reduces Individuality by Increasing Variability within Subjects. Curr. Biol. 2015, 25, 2672–2676. [Google Scholar] [CrossRef]
- Matrangola, S.L.; Madigan, M.L. The Effects of Obesity on Balance Recovery Using an Ankle Strategy. Hum. Mov. Sci. 2011, 30, 584–595. [Google Scholar] [CrossRef]
- Brown, I.E.; Loeb, G.E. A Reductionist Approach to Creating and Using Neuromusculoskeletal Models. In Biomechanics and Neural Control of Posture and Movement; Winters, J.M., Crago, P.E., Eds.; Springer: New York, NY, USA, 2000; pp. 148–163. ISBN 978-1-4612-2104-3. [Google Scholar]
- Tytell, E.; Holmes, P.; Cohen, A. Spikes Alone Do Not Behavior Make: Why Neuroscience Needs Biomechanics. Curr. Opin. Neurobiol. 2011, 21, 816–822. [Google Scholar] [CrossRef]
- Loeb, G.E.; Brown, I.E.; Cheng, E.J. A Hierarchical Foundation for Models of Sensorimotor Control. Exp. Brain Res. 1999, 126, 1–18. [Google Scholar] [CrossRef]
- Deng, K.; Szczecinski, N.S.; Hunt, A.J.; Chiel, H.J.; Quinn, R.D. Kinematic and Kinetic Analysis of a Biomechanical Model of Rat Hind Limb with Biarticular Muscles. In Proceedings of the Biomimetic and Biohybrid Systems; Vouloutsi, V., Mura, A., Tauber, F., Speck, T., Prescott, T.J., Verschure, P.F.M.J., Eds.; Springer International Publishing: Cham, Switzerland, 2020; pp. 55–67. [Google Scholar]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Deng, K.; Hunt, A.J.; Szczecinski, N.S.; Tresch, M.C.; Chiel, H.J.; Heckman, C.J.; Quinn, R.D. Biomechanical and Sensory Feedback Regularize the Behavior of Different Locomotor Central Pattern Generators. Biomimetics 2022, 7, 226. https://doi.org/10.3390/biomimetics7040226
Deng K, Hunt AJ, Szczecinski NS, Tresch MC, Chiel HJ, Heckman CJ, Quinn RD. Biomechanical and Sensory Feedback Regularize the Behavior of Different Locomotor Central Pattern Generators. Biomimetics. 2022; 7(4):226. https://doi.org/10.3390/biomimetics7040226
Chicago/Turabian StyleDeng, Kaiyu, Alexander J. Hunt, Nicholas S. Szczecinski, Matthew C. Tresch, Hillel J. Chiel, C. J. Heckman, and Roger D. Quinn. 2022. "Biomechanical and Sensory Feedback Regularize the Behavior of Different Locomotor Central Pattern Generators" Biomimetics 7, no. 4: 226. https://doi.org/10.3390/biomimetics7040226
APA StyleDeng, K., Hunt, A. J., Szczecinski, N. S., Tresch, M. C., Chiel, H. J., Heckman, C. J., & Quinn, R. D. (2022). Biomechanical and Sensory Feedback Regularize the Behavior of Different Locomotor Central Pattern Generators. Biomimetics, 7(4), 226. https://doi.org/10.3390/biomimetics7040226






