Bio-Inspired Nanomembranes as Building Blocks for Nanophotonics, Plasmonics and Metamaterials
Abstract
1. Introduction
2. Materials and Methods
3. Photonic Crystals Based on Nanomembranes
3.1. Introductory Remarks
3.2. Photonic Crystal Nanomembranes
3.3. Some Practical Applications
4. Nanomembrane Plasmonics
4.1. Introductory Remarks
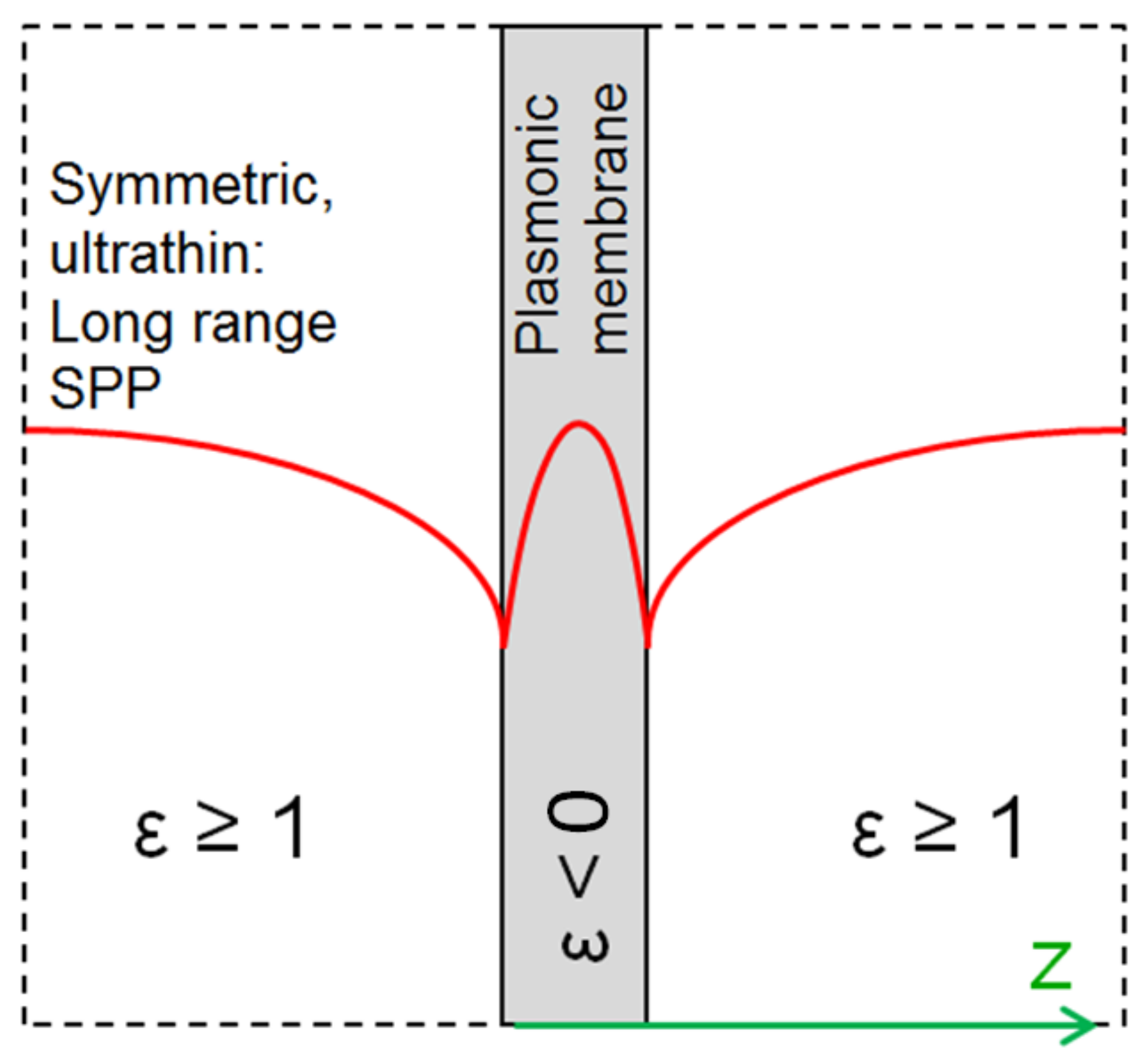
4.2. Long Range Surface Plasmon Polaritons
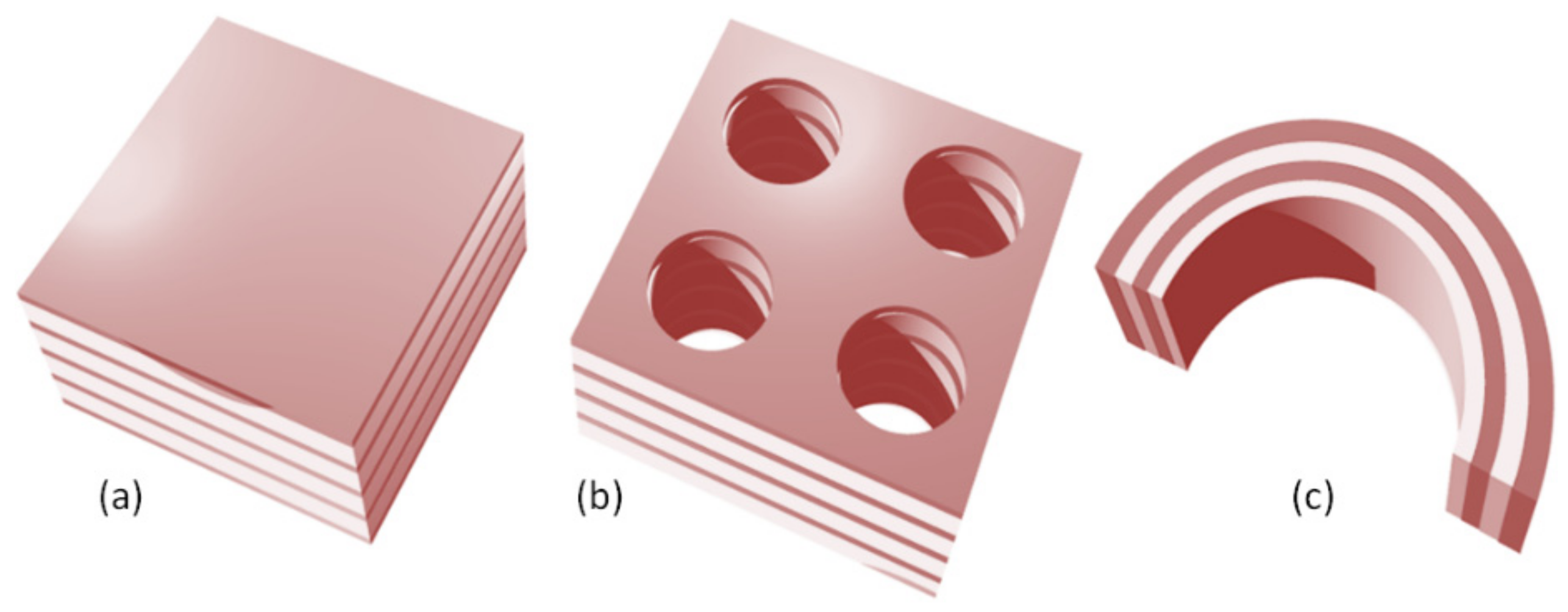
4.3. Subwavelength Plasmonic Crystals
4.4. Applications of Nanomembrane-Based Plasmonics
5. Membrane Metamaterials
5.1. Introductory Remarks and Basic Concepts
5.2. Metasurfaces
5.3. Low-Loss Metasurfaces
5.4. Hyperbolic Metasurfaces
5.5. Applications of Metasurfaces
6. Planar Nanoantennas on Nanomembranes
6.1. Introductory Remarks
6.2. Some Applications
7. Nanomembranes in General Photonics
7.1. Introductory Remarks
7.2. Applications
8. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Conflicts of Interest
References
- Feynman, R. There’s Plenty of Room at the Bottom. In Feynman and Computation; CRC Press: Boca Raton, FL, USA, 2019; pp. 63–76. [Google Scholar] [CrossRef]
- Binns, C. Introduction to Nanoscience and Nanotechnology; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2021. [Google Scholar] [CrossRef]
- Siontorou, C.G.; Nikoleli, G.-P.; Nikolelis, D.P.; Karapetis, S.K. Artificial lipid membranes: Past, present, and future. Membranes 2017, 7, 38. [Google Scholar] [CrossRef] [PubMed]
- Chen, J.-Y.; Hou, J.-L. Controllable synthetic ion channels. Org. Chem. Front. 2018, 5, 1728–1736. [Google Scholar] [CrossRef]
- Rabinowitz, J.; Cohen, C.; Shepard, K.L. An Electrically Actuated, Carbon-Nanotube-Based Biomimetic Ion Pump. Nano Lett. 2020, 20, 1148–1153. [Google Scholar] [CrossRef]
- Ying, Y.-L.; Hu, Z.-L.; Zhang, S.; Qing, Y.; Fragasso, A.; Maglia, G.; Meller, A.; Bayley, H.; Dekker, C.; Long, Y.-T. Nanopore-based technologies beyond DNA sequencing. Nat. Nanotechnol. 2022, 17, 1136–1146. [Google Scholar] [CrossRef] [PubMed]
- Jovanovic-Talisman, T.; Zilman, A. Protein transport by the nuclear pore complex: Simple biophysics of a complex biomachine. Biophys. J. 2017, 113, 6–14. [Google Scholar] [CrossRef] [PubMed]
- Supramaniam, P.; Ces, O.; Salehi-Reyhani, A. Microfluidics for Artificial Life: Techniques for Bottom-Up Synthetic Biology. Micromachines 2019, 10, 299. [Google Scholar] [CrossRef]
- Jakšić, Z.; Jakšić, O. Biomimetic Nanomembranes: An Overview. Biomimetics 2020, 5, 24. [Google Scholar] [CrossRef]
- Ciganė, U.; Palevičius, A.; Janušas, G. Review of nanomembranes: Materials, fabrications and applications in tissue engineering (bone and skin) and drug delivery systems. J. Mater. Sci. 2021, 56, 13479–13498. [Google Scholar] [CrossRef]
- Schuller, J.A.; Barnard, E.S.; Cai, W.; Jun, Y.C.; White, J.S.; Brongersma, M.L. Plasmonics for extreme light concentration and manipulation. Nat. Mater. 2010, 9, 193–204. [Google Scholar] [CrossRef]
- Ozbay, E. Plasmonics: Merging Photonics and Electronics at Nanoscale Dimensions. Science 2006, 311, 189–193. [Google Scholar] [CrossRef]
- Lim, D.K.; Jeon, K.S.; Kim, H.M.; Nam, J.M.; Suh, Y.D. Nanogap-engineerable Raman-active nanodumbbells for single-molecule detection. Nat. Mater. 2010, 9, 60–67. [Google Scholar] [CrossRef]
- Schwartz, B.T.; Piestun, R. Total external reflection from metamaterials with ultralow refractive index. J. Opt. Soc. Am. B 2003, 20, 2448–2453. [Google Scholar] [CrossRef]
- Choi, M.; Lee, S.H.; Kim, Y.; Kang, S.B.; Shin, J.; Kwak, M.H.; Kang, K.Y.; Lee, Y.H.; Park, N.; Min, B. A terahertz metamaterial with unnaturally high refractive index. Nature 2011, 470, 369–373. [Google Scholar] [CrossRef]
- Smith, D.R.; Pendry, J.B.; Wiltshire, M.C.K. Metamaterials and Negative Refractive Index. Science 2004, 305, 788–792. [Google Scholar] [CrossRef]
- Cai, W.; Shalaev, V. Optical Metamaterials: Fundamentals and Applications; Springer: Dordrecht, The Netherlands, 2009. [Google Scholar] [CrossRef]
- Wang, X.-Y.; Li, Z.-Z.; Ding, S.-N. A signal amplification of near-infrared electrochemiluminescence immunosensor for SFTSV determination based on SiO2 photonic crystals nanomembrane. Sens. Actuators B Chem. 2022, 358, 131493. [Google Scholar] [CrossRef]
- Baffou, G.; Cichos, F.; Quidant, R. Applications and challenges of thermoplasmonics. Nat. Mater. 2020, 19, 946–958. [Google Scholar] [CrossRef]
- Zhao, X.; Lu, M. (Eds.) Nanophotonics in Biomedical Engineering; Springer: Singapore, 2021. [Google Scholar] [CrossRef]
- Zhang, S.; Wong, C.L.; Zeng, S.; Bi, R.; Tai, K.; Dholakia, K.; Olivo, M. Metasurfaces for biomedical applications: Imaging and sensing from a nanophotonics perspective. Nanophotonics 2021, 10, 259–293. [Google Scholar] [CrossRef]
- Huang, K.; Li, Z.; Lin, J.; Han, G.; Huang, P. Two-dimensional transition metal carbides and nitrides (MXenes) for biomedical applications. Chem. Soc. Rev. 2018, 47, 5109–5124. [Google Scholar] [CrossRef]
- Jia, P.; Zuber, K.; Guo, Q.; Gibson, B.C.; Yang, J.; Ebendorff-Heidepriem, H. Large-area freestanding gold nanomembranes with nanoholes. Mater. Horiz. 2019, 6, 1005–1012. [Google Scholar] [CrossRef]
- Matović, J.; Jakšić, Z. Simple and reliable technology for manufacturing metal-composite nanomembranes with giant aspect ratio. Microelectron. Eng. 2009, 86, 906–909. [Google Scholar] [CrossRef]
- Yoshikawa, T.; Gao, F.; Zuerbig, V.; Giese, C.; Nebel, C.E.; Ambacher, O.; Lebedev, V. Pinhole-free ultra-thin nanocrystalline diamond film growth via electrostatic self-assembly seeding with increased salt concentration of nanodiamond colloids. Diam. Relat. Mater. 2016, 63, 103–107. [Google Scholar] [CrossRef]
- Stauss, S.; Terashima, K. Diamondoids: Synthesis, Properties, and Applications; CRC Press: Boca Raton, FL, USA, 2017. [Google Scholar] [CrossRef]
- Karan, S.; Samitsu, S.; Peng, X.; Kurashima, K.; Ichinose, I. Ultrafast viscous permeation of organic solvents through diamond-like carbon nanosheets. Science 2012, 335, 444–447. [Google Scholar] [CrossRef] [PubMed]
- Yang, Y.; Hillmann, R.; Qi, Y.; Korzetz, R.; Biere, N.; Emmrich, D.; Westphal, M.; Büker, B.; Hütten, A.; Beyer, A. Ultrahigh Ionic Exclusion through Carbon Nanomembranes. Adv. Mater. 2020, 32, 1907850. [Google Scholar] [CrossRef] [PubMed]
- Rogers, J.A.; Ahn, J.-H. (Eds.) Silicon Nanomembranes: Fundamental Science and Applications; John Wiley & Sons: Weinheim, Germany, 2016. [Google Scholar] [CrossRef]
- Rogers, J.A.; Lagally, M.; Nuzzo, R.G. Synthesis, assembly and applications of semiconductor nanomembranes. Nature 2011, 477, 45–53. [Google Scholar] [CrossRef] [PubMed]
- Zhou, Q.; Xia, G.; Du, M.; Lu, Y.; Xu, H. Scotch-tape-like exfoliation effect of graphene quantum dots for efficient preparation of graphene nanosheets in water. Appl. Surf. Sci. 2019, 483, 52–59. [Google Scholar] [CrossRef]
- Wang, J.; Bo, W.; Ding, Y.; Wang, X.; Mu, X. Optical, optoelectronic, and photoelectric properties in moiré superlattices of twist bilayer graphene. Mater. Today Phys. 2020, 14, 100238. [Google Scholar] [CrossRef]
- Anasori, B.; Lukatskaya, M.R.; Gogotsi, Y. 2D metal carbides and nitrides (MXenes) for energy storage. Nat. Rev. Mater. 2017, 2, 16098. [Google Scholar] [CrossRef]
- Vendamme, R.; Onoue, S.Y.; Nakao, A.; Kunitake, T. Robust free-standing nanomembranes of organic/inorganic interpenetrating networks. Nat. Mater. 2006, 5, 494–501. [Google Scholar] [CrossRef]
- Weber, M.; Kim, J.-H.; Lee, J.-H.; Kim, J.-Y.; Iatsunskyi, I.; Coy, E.; Drobek, M.; Julbe, A.; Bechelany, M.; Kim, S.S. High-performance nanowire hydrogen sensors by exploiting the synergistic effect of Pd nanoparticles and metal–organic framework membranes. ACS Appl. Mater. Interfaces 2018, 10, 34765–34773. [Google Scholar] [CrossRef]
- Xie, W.; Lee, J.-H. Dynamics of Entangled Networks in Ultrafast Perforation of Polystyrene Nanomembranes. Macromolecules 2020, 53, 1701–1705. [Google Scholar] [CrossRef]
- Salvatore, S. Optical Metamaterials by Block Copolymer Self-Assembly; Springer: Cham, Switzerland, 2014. [Google Scholar] [CrossRef]
- Drude, P. The Theory of Optics; Dover Publications: Mineola, NY, USA, 2005. [Google Scholar]
- Madou, M.J. Fundamentals of Microfabrication and Nanotechnology; CRC Press: Boca Raton, FL, USA, 2018; Volume 1–3. [Google Scholar] [CrossRef]
- Jakšić, Z.; Matovic, J. Functionalization of Artificial Freestanding Composite Nanomembranes. Materials 2010, 3, 165–200. [Google Scholar] [CrossRef]
- Wang, P.; Zheng, Y.; Chen, X.; Huang, C.; Kartashov, Y.V.; Torner, L.; Konotop, V.V.; Ye, F. Localization and delocalization of light in photonic moiré lattices. Nature 2020, 577, 42–46. [Google Scholar] [CrossRef]
- Carr, S.; Massatt, D.; Fang, S.; Cazeaux, P.; Luskin, M.; Kaxiras, E. Twistronics: Manipulating the electronic properties of two-dimensional layered structures through their twist angle. Phys. Rev. B 2017, 95, 075420. [Google Scholar] [CrossRef]
- Yuan, N.F.Q.; Isobe, H.; Fu, L. Magic of high-order van Hove singularity. Nat. Commun. 2019, 10, 5769. [Google Scholar] [CrossRef]
- Zheludev, N.I.; Plum, E. Reconfigurable nanomechanical photonic metamaterials. Nat. Nanotechnol. 2016, 11, 16–22. [Google Scholar] [CrossRef]
- Gallinet, B.; Butet, J.; Martin, O.J.F. Numerical methods for nanophotonics: Standard problems and future challenges. Laser Photonics Rev. 2015, 9, 577–603. [Google Scholar] [CrossRef]
- Achanta, V.G. Plasmonic quasicrystals. Prog. Quantum Electron. 2015, 39, 1–23. [Google Scholar] [CrossRef]
- Chen, Q.; Wu, S.; Zhang, L.; Zhou, H.; Fan, W.; Tan, C.S. Transferable single-layer GeSn nanomembrane resonant-cavity-enhanced photodetectors for 2 μm band optical communication and multi-spectral short-wave infrared sensing. Nanoscale 2022, 14, 7341–7349. [Google Scholar] [CrossRef]
- Aouassa, M.; Franzò, G.; M’Ghaieth, R.; Chouaib, H. Direct growth and size tuning of InAs/GaAs quantum dots on transferable silicon nanomembranes for solar cells application. J. Mater. Sci. Mater. Electron. 2021, 32, 18251–18263. [Google Scholar] [CrossRef]
- Kang, J.; Tok, J.B.-H.; Bao, Z. Self-healing soft electronics. Nat. Electron. 2019, 2, 144–150. [Google Scholar] [CrossRef]
- Song, J.-K.; Do, K.; Koo, J.H.; Son, D.; Kim, D.-H. Nanomaterials-based flexible and stretchable bioelectronics. MRS Bull. 2019, 44, 643–656. [Google Scholar] [CrossRef]
- Zong, Y.; Zhang, X.; Wu, Y.; Wang, Y.; Liu, C.; Xu, B.; Huang, G.; Cui, J.; Mei, Y. Nanomembrane folding origami: Geometry control and micro-machine applications. Prog. Nat. Sci. Mater. Int. 2021, 31, 865–871. [Google Scholar] [CrossRef]
- Ferro, L.M.M.; Merces, L.; de Camargo, D.H.S.; Bof Bufon, C.C. Ultrahigh-Gain Organic Electrochemical Transistor Chemosensors Based on Self-Curled Nanomembranes. Adv. Mater. 2021, 33, 2101518. [Google Scholar] [CrossRef] [PubMed]
- Puri, N.; Gupta, A.; Mishra, A. Recent advances on nano-adsorbents and nanomembranes for the remediation of water. J. Clean. Prod. 2021, 322, 129051. [Google Scholar] [CrossRef]
- Yablonovitch, E. Inhibited spontaneous emission in solid-state physics and electronics. Phys. Rev. Lett. 1987, 58, 2059–2062. [Google Scholar] [CrossRef]
- Lourtioz, J.M.; Benisty, H.; Berger, V.; Gerard, J.-M.; Maystre, D.; Tchelnokov, A. Photonic Crystals: Towards Nanoscale Photonic Devices, 2nd ed.; Springer: Berlin/Heidelberg, Germany, 2008. [Google Scholar] [CrossRef]
- Soukoulis, C.M. (Ed.) Photonic Crystals and Light Localization in the 21st Century; Springer: Dordrecht, The Netherlands, 2012. [Google Scholar] [CrossRef]
- Sharabi, Y.; Dikopoltsev, A.; Lustig, E.; Lumer, Y.; Segev, M. Spatiotemporal photonic crystals. Optica 2022, 9, 585–592. [Google Scholar] [CrossRef]
- Yeh, P.; Yariv, A.; Hong, C.-S. Electromagnetic propagation in periodic stratified media. I. General theory. J. Opt. Soc. Am. 1977, 67, 423–438. [Google Scholar] [CrossRef]
- Li, Z.-Y.; Lin, L.-L. Photonic band structures solved by a plane-wave-based transfer-matrix method. Phys. Rev. E 2003, 67, 046607. [Google Scholar] [CrossRef]
- Dörfler, W.; Lechleiter, A.; Plum, M.; Schneider, G.; Wieners, C. Photonic Crystals: Mathematical Analysis and Numerical Approximation; Springer: Basel, Switzerland, 2011; Volume 42. [Google Scholar] [CrossRef]
- Zhang, X.; De-Eknamkul, C.; Gu, J.; Boehmke, A.L.; Menon, V.M.; Khurgin, J.; Cubukcu, E. Guiding of visible photons at the ångström thickness limit. Nat. Nanotechnol. 2019, 14, 844–850. [Google Scholar] [CrossRef]
- Vuković, S.M.; Miret, J.J.; Zapata-Rodriguez, C.J.; Jakšić, Z. Oblique surface waves at an interface between a metal-dielectric superlattice and an isotropic dielectric. Phys. Scr. 2012, 014041. [Google Scholar] [CrossRef]
- Vuković, S.M.; Shadrivov, I.V.; Kivshar, Y.S. Surface Bloch waves in metamaterial and metal-dielectric superlattices. Appl. Phys. Lett. 2009, 95, 041902. [Google Scholar] [CrossRef]
- Notomi, M.; Shinya, A.; Mitsugi, S.; Kuramochi, E.; Ryu, H.Y. Waveguides, resonators and their coupled elements in photonic crystal slabs. Opt. Express 2004, 12, 1551–1561. [Google Scholar] [CrossRef]
- Wang, X.; Wang, Z.; Dong, H.; Saggau, C.N.; Tang, H.; Tang, M.; Liu, L.; Baunack, S.; Bai, L.; Liu, J.; et al. Collective Coupling of 3D Confined Optical Modes in Monolithic Twin Microtube Cavities Formed by Nanomembrane Origami. Nano Lett. 2022, 22, 6692–6699. [Google Scholar] [CrossRef]
- Nada, M.Y.; Capolino, F. Exceptional point of sixth-order degeneracy in a modified coupled-resonator optical waveguide. J. Opt. Soc. Am. B 2020, 37, 2319–2328. [Google Scholar] [CrossRef]
- Gadhwal, R.; Kaushik, P.; Devi, A. A review on 1D photonic crystal based reflective optical limiters. Crit. Rev. Solid State Mater. Sci. 2022, 1–19. [Google Scholar] [CrossRef]
- Chung, C.-J.; Xu, X.; Wang, G.; Pan, Z.; Chen, R.T. On-chip optical true time delay lines featuring one-dimensional fishbone photonic crystal waveguide. Appl. Phys. Lett. 2018, 112, 071104. [Google Scholar] [CrossRef]
- Zhang, C.; ElAfandy, R.; Han, J. Distributed Bragg Reflectors for GaN-Based Vertical-Cavity Surface-Emitting Lasers. Appl. Sci. 2019, 9, 1593. [Google Scholar] [CrossRef]
- Wu, S.; Yang, W.; Meng, Z.; Li, L.; Zhang, S. Photonic Crystals Assembled by SiO2@Ni/TiO2 for Photocatalytic Reduction of CO2. Catal. Lett. 2020, 150, 3598–3607. [Google Scholar] [CrossRef]
- Limonov, M.F.; Rybin, M.V.; Poddubny, A.N.; Kivshar, Y.S. Fano resonances in photonics. Nat. Photonics 2017, 11, 543–554. [Google Scholar] [CrossRef]
- Bekele, D.; Yu, Y.; Yvind, K.; Mork, J. In-Plane Photonic Crystal Devices using Fano Resonances. Laser Photonics Rev. 2019, 13, 1900054. [Google Scholar] [CrossRef]
- Zhou, W.; Zhao, D.; Shuai, Y.-C.; Yang, H.; Chuwongin, S.; Chadha, A.; Seo, J.-H.; Wang, K.X.; Liu, V.; Ma, Z.; et al. Progress in 2D photonic crystal Fano resonance photonics. Prog. Quantum Electron. 2014, 38, 1–74. [Google Scholar] [CrossRef]
- Lin, C.-Y.; Subbaraman, H.; Hosseini, A.; Wang, A.X.; Zhu, L.; Chen, R.T. Silicon nanomembrane based photonic crystal waveguide array for wavelength-tunable true-time-delay lines. Appl. Phys. Lett. 2012, 101, 051101. [Google Scholar] [CrossRef]
- Jakšić, Z. Micro and Nanophotonics for Semiconductor Infrared Detectors: Towards an Ultimate Uncooled Device; Springer International Publishing: Cham, Switzerland, 2014. [Google Scholar] [CrossRef]
- Pan, R.; Guo, Q.; Li, G.; Song, E.; Huang, G.; An, Z.; Di, Z.; Mei, Y. Schottky Barrier Modulation in Surface Nanoroughened Silicon Nanomembranes for High-Performance Optoelectronics. ACS Appl. Mater. Interfaces 2018, 10, 41497–41503. [Google Scholar] [CrossRef] [PubMed]
- Moein, T.; Gailevičius, D.; Katkus, T.; Ng, S.H.; Lundgaard, S.; Moss, D.J.; Kurt, H.; Mizeikis, V.; Staliūnas, K.; Malinauskas, M.; et al. Optically-thin broadband graphene-membrane photodetector. Nanomaterials 2020, 10, 407. [Google Scholar] [CrossRef] [PubMed]
- Kim, M.; Park, J.; Zhou, W.; Ma, Z. Flexible Photodetectors with Nanomembranes and Nanowires. In Inorganic Flexible Optoelectronics; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2019; pp. 79–116. [Google Scholar] [CrossRef]
- Paiella, R.; Lagally, M.G. Optical Properties of Tensilely Strained Ge Nanomembranes. Nanomaterials 2018, 8, 407. [Google Scholar] [CrossRef]
- Lai, W.-C.; Chakravarty, S.; Zou, Y.; Chen, R.T. Silicon nano-membrane based photonic crystal microcavities for high sensitivity bio-sensing. Opt. Lett. 2012, 37, 1208–1210. [Google Scholar] [CrossRef]
- Wang, X.-Y.; Zhu, K.-D.; Zhu, J.; Ding, S.-N. Photonic crystal of polystyrene nanomembrane: Signal amplification and low triggered potential electrochemiluminescence for tetracycline detection. Anal. Chem. 2021, 93, 2959–2967. [Google Scholar] [CrossRef]
- Fenzl, C.; Hirsch, T.; Wolfbeis, O.S. Photonic crystals for chemical sensing and biosensing. Angew. Chem. Int. Ed. 2014, 53, 3318–3335. [Google Scholar] [CrossRef]
- Shen, P.; Zhang, Y.; Cai, Z.; Liu, R.; Xu, X.; Li, R.; Wang, J.-J.; Yang, D.A. Three-dimensional/two-dimensional photonic crystal hydrogels for biosensing. J. Mater. Chem. C 2021, 9, 5840–5857. [Google Scholar] [CrossRef]
- Kini Manjeshwar, S.; Elkhouly, K.; Fitzgerald, J.M.; Ekman, M.; Zhang, Y.; Zhang, F.; Wang, S.M.; Tassin, P.; Wieczorek, W. Suspended photonic crystal membranes in AlGaAs heterostructures for integrated multi-element optomechanics. Appl. Phys. Lett. 2020, 116, 264001. [Google Scholar] [CrossRef]
- Lu, T.-W.; Wu, C.-C.; Lee, P.-T. 1D photonic crystal strain sensors. ACS Photonics 2018, 5, 2767–2772. [Google Scholar] [CrossRef]
- Chen, W.; Ren, W.; Cheng, M.; Liu, X.; Zhang, X.; Zhang, M. Strain induced photonic topological insulator. Phys. Lett. A 2022, 447, 128299. [Google Scholar] [CrossRef]
- Zhang, R.; Wang, Q.; Zheng, X. Flexible mechanochromic photonic crystals: Routes to visual sensors and their mechanical properties. J. Mater. Chem. C 2018, 6, 3182–3199. [Google Scholar] [CrossRef]
- Rindorf, L.; Bang, O. Sensitivity of photonic crystal fiber grating sensors: Biosensing, refractive index, strain, and temperature sensing. J. Opt. Soc. Am. B 2008, 25, 310–324. [Google Scholar] [CrossRef]
- Fang, Y.; Leo, S.-Y.; Ni, Y.; Wang, J.; Wang, B.; Yu, L.; Dong, Z.; Dai, Y.; Basile, V.; Taylor, C.; et al. Reconfigurable Photonic Crystals Enabled by Multistimuli-Responsive Shape Memory Polymers Possessing Room Temperature Shape Processability. ACS Appl. Mater. Interfaces 2017, 9, 5457–5467. [Google Scholar] [CrossRef]
- Maier, S.A. Plasmonics: Fundamentals and Applications; Springer: New York, NY, USA, 2007. [Google Scholar] [CrossRef]
- Maier, S.A. (Ed.) World Scientific Handbook of Metamaterials and Plasmonics; World Scientific Publishing Company Pte Limited: Singapore, 2018; Volume 1–4. [Google Scholar] [CrossRef]
- Barnes, W.L.; Dereux, A.; Ebbesen, T.W. Surface plasmon subwavelength optics. Nature 2003, 424, 824–830. [Google Scholar] [CrossRef]
- Boltasseva, A.; Atwater, H.A. Low-Loss Plasmonic Metamaterials. Science 2011, 331, 290–291. [Google Scholar] [CrossRef]
- West, P.R.; Ishii, S.; Naik, G.V.; Emani, N.K.; Shalaev, V.; Boltasseva, A. Searching for better plasmonic materials. Laser Photonics Rev. 2010, 4, 795–808. [Google Scholar] [CrossRef]
- Jiang, C.; Markutsya, S.; Pikus, Y.; Tsukruk, V.V. Freely suspended nanocomposite membranes as highly sensitive sensors. Nat. Mater. 2004, 3, 721–728. [Google Scholar] [CrossRef]
- Perkin, E.; Gubala, V. Nanomaterials—On the Brink of Revolution? Or the Endless Pursuit of Something Unattainable? Chem. Int. 2017, 39, 10–13. [Google Scholar] [CrossRef]
- Baburin, A.S.; Kalmykov, A.S.; Kirtaev, R.V.; Negrov, D.V.; Moskalev, D.O.; Ryzhikov, I.A.; Melentiev, P.N.; Rodionov, I.A.; Balykin, V.I. Toward a theoretically limited SPP propagation length above two hundred microns on an ultra-smooth silver surface. Opt. Mater. Express 2018, 8, 3254–3261. [Google Scholar] [CrossRef]
- Berini, P. Long-range surface plasmon polaritons. Adv. Opt. Photonics 2009, 1, 484–588. [Google Scholar] [CrossRef]
- Berini, P. Plasmon-polariton waves guided by thin lossy metal films of finite width: Bound modes of symmetric structures. Phys. Rev. B 2000, 61, 10484–10503. [Google Scholar] [CrossRef]
- Jakšić, Z.; Vuković, S.M.; Buha, J.; Matovic, J. Nanomembrane-Based Plasmonics. J. Nanophotonics 2011, 5, 051818. [Google Scholar] [CrossRef]
- Jung, D.; Lim, C.; Shim, H.J.; Kim, Y.; Park, C.; Jung, J.; Han, S.I.; Sunwoo, S.-H.; Cho, K.W.; Cha, G.D.; et al. Highly conductive and elastic nanomembrane for skin electronics. Science 2021, 373, 1022–1026. [Google Scholar] [CrossRef]
- Shvets, G.; Urzhumov, Y.A. Electric and magnetic properties of sub-wavelength plasmonic crystals. J. Opt. A Pure Appl. Opt. 2005, 7, S23–S31. [Google Scholar] [CrossRef]
- Bauer, C.; Kobiela, G.; Giessen, H. 2D quasiperiodic plasmonic crystals. Sci. Rep. 2012, 2, 681. [Google Scholar] [CrossRef]
- Ebbesen, T.W.; Lezec, H.J.; Ghaemi, H.F.; Thio, T.; Wolff, P.A. Extraordinary optical transmission through sub-wavelength hole arrays. Nature 1998, 391, 667–669. [Google Scholar] [CrossRef]
- Ai, B.; Yu, Y.; Möhwald, H.; Wang, L.; Zhang, G. Resonant Optical Transmission through Topologically Continuous Films. ACS Nano 2014, 8, 1566–1575. [Google Scholar] [CrossRef]
- Abdulhalim, I.; Zourob, M.; Lakhtakia, A. Surface plasmon resonance for biosensing: A mini-review. Electromagnetics 2008, 28, 214–242. [Google Scholar] [CrossRef]
- Assad, O.N.; Gilboa, T.; Spitzberg, J.; Juhasz, M.; Weinhold, E.; Meller, A. Light-enhancing plasmonic-nanopore biosensor for superior single-molecule detection. Adv. Mater. 2017, 29, 1605442. [Google Scholar] [CrossRef]
- Taylor, A.B.; Zijlstra, P. Single-Molecule Plasmon Sensing: Current Status and Future Prospects. ACS Sensors 2017, 2, 1103–1122. [Google Scholar] [CrossRef]
- Garoli, D.; Yamazaki, H.; Maccaferri, N.; Wanunu, M. Plasmonic Nanopores for Single-Molecule Detection and Manipulation: Toward Sequencing Applications. Nano Lett. 2019, 19, 7553–7562. [Google Scholar] [CrossRef]
- Kim, S.; Seo, J.; Park, H.H.; Kim, N.; Oh, J.-W.; Nam, J.-M. Plasmonic Nanoparticle-Interfaced Lipid Bilayer Membranes. Acc. Chem. Res. 2019, 52, 2793–2805. [Google Scholar] [CrossRef]
- Ferhan, A.R.; Yoon, B.K.; Jeon, W.-Y.; Cho, N.-J. Biologically interfaced nanoplasmonic sensors. Nanoscale Adv. 2020, 2, 3103–3114. [Google Scholar] [CrossRef]
- Seo, J.; Kim, S.; Park, H.H.; Choi, D.Y.; Nam, J.-M. Nano-bio-computing lipid nanotablet. Sci. Adv. 2019, 5, eaau2124. [Google Scholar] [CrossRef]
- Fang, Y.; Sun, M. Nanoplasmonic waveguides: Towards applications in integrated nanophotonic circuits. Light Sci. Appl. 2015, 4, e294. [Google Scholar] [CrossRef]
- Guo, X.; Ma, Y.; Wang, Y.; Tong, L. Nanowire plasmonic waveguides, circuits and devices. Laser Photonics Rev. 2013, 7, 855–881. [Google Scholar] [CrossRef]
- Yu, L.; Liang, Y.; Chu, S.; Gao, H.; Wang, Q.; Peng, W. Freestanding bilayer plasmonic waveguide coupling mechanism for ultranarrow electromagnetic-induced transparency band generation. Sci. Rep. 2021, 11, 1437. [Google Scholar] [CrossRef]
- Rogez, B.; Marmri, Z.; Thibaudau, F.; Baffou, G. Thermoplasmonics of metal layers and nanoholes. APL Photonics 2021, 6, 101101. [Google Scholar] [CrossRef]
- Politano, A.; Cupolillo, A.; Di Profio, G.; Arafat, H.A.; Chiarello, G.; Curcio, E. When plasmonics meets membrane technology. J. Phys. Condens. Matter 2016, 28, 363003. [Google Scholar] [CrossRef]
- Farid, M.U.; Kharraz, J.A.; An, A.K. Plasmonic Titanium Nitride Nano-enabled Membranes with High Structural Stability for Efficient Photothermal Desalination. ACS Appl. Mater. Interfaces 2021, 13, 3805–3815. [Google Scholar] [CrossRef] [PubMed]
- Luo, Y.; Jiang, X.; Liu, L.; Si, G. Recent advances in plasmonic nanolithography. Nanosci. Nanotechnol. Lett. 2018, 10, 1–12. [Google Scholar] [CrossRef]
- Polman, A.; Atwater, H.A. Photonic design principles for ultrahigh-efficiency photovoltaics. Nat. Mater. 2012, 11, 174–177. [Google Scholar] [CrossRef] [PubMed]
- Morawiec, S.; Mendes, M.J.; Priolo, F.; Crupi, I. Plasmonic nanostructures for light trapping in thin-film solar cells. Mater. Sci. Semicond. Process. 2019, 92, 10–18. [Google Scholar] [CrossRef]
- Endo-Kimura, M.; Kowalska, E. Plasmonic Photocatalysts for Microbiological Applications. Catalysts 2020, 10, 824. [Google Scholar] [CrossRef]
- Abed, J.; Rajput, N.S.; Moutaouakil, A.E.; Jouiad, M. Recent Advances in the Design of Plasmonic Au/TiO2 Nanostructures for Enhanced Photocatalytic Water Splitting. Nanomaterials 2020, 10, 2260. [Google Scholar] [CrossRef]
- Qi, G.; Diao, X.; Hou, S.; Kong, J.; Jin, Y. Label-Free SERS Detection of Protein Damage in Organelles under Electrostimulation with 2D AuNPs-based Nanomembranes as Substrates. Anal. Chem. 2022, 94, 14931–14937. [Google Scholar] [CrossRef]
- King, M.E.; Fonseca Guzman, M.V.; Ross, M.B. Material strategies for function enhancement in plasmonic architectures. Nanoscale 2022, 14, 602–611. [Google Scholar] [CrossRef]
- Fu, R.; Shi, Q.; Yong, Z.; Lu, Y.; Cheng, W. Two-Dimensional Plasmonic Nanoassemblies: Fabrication, Properties, and Applications. In World Scientific Reference on Plasmonic Nanomaterials; World Scientific: Singapore, 2021; Volume 22, pp. 351–407. [Google Scholar] [CrossRef]
- Knust, S.; Kreft, D.; Hillmann, R.; Meyer, A.; Viefhues, M.; Reimann, P.; Anselmetti, D. Measuring DNA Translocation Forces through MoS2-Nanopores with Optical Tweezers. Mater. Today Proc. 2017, 4, S168–S173. [Google Scholar] [CrossRef]
- Walker, Z.J.; Wells, T.; Belliston, E.; Romney, S.; Walker, S.B.; Sampad, M.J.; Saiduzzaman, S.M.; Losakul, R.; Schmidt, H.; Hawkins, A.R. Optofluidic Particle Manipulation Platform with Nanomembrane. Micromachines 2022, 13, 721. [Google Scholar] [CrossRef]
- Oh, S.-H.; Altug, H.; Jin, X.; Low, T.; Koester, S.J.; Ivanov, A.P.; Edel, J.B.; Avouris, P.; Strano, M.S. Nanophotonic biosensors harnessing van der Waals materials. Nat. Commun. 2021, 12, 3824. [Google Scholar] [CrossRef]
- Chen, C.; Li, C.; Min, S.; Guo, Q.; Xia, Z.; Liu, D.; Ma, Z.; Xia, F. Ultrafast Silicon Nanomembrane Microbolometer for Long-Wavelength Infrared Light Detection. Nano Lett. 2021, 21, 8385–8392. [Google Scholar] [CrossRef]
- Azzam, S.I.; Kildishev, A.V.; Ma, R.-M.; Ning, C.-Z.; Oulton, R.; Shalaev, V.M.; Stockman, M.I.; Xu, J.-L.; Zhang, X. Ten years of spasers and plasmonic nanolasers. Light Sci. Appl. 2020, 9, 90. [Google Scholar] [CrossRef]
- Cao, Y.; Nallappan, K.; Xu, G.; Skorobogatiy, M. Add drop multiplexers for terahertz communications using two-wire waveguide-based plasmonic circuits. Nat. Commun. 2022, 13, 4090. [Google Scholar] [CrossRef]
- Xu, C.; Li, C.; Jin, Y. Plasmonic Tunnel Diode and Photodetector based on Layer-Stacked AuNP-Nanomembrane/p-Si Heterojunction. Adv. Electron. Mater. 2022, 8, 2101251. [Google Scholar] [CrossRef]
- Chen, Y.; Yin, Y.; Ma, L.; Schmidt, O.G. Recent Progress on Optoplasmonic Whispering-Gallery-Mode Microcavities. Adv. Opt. Mater. 2021, 9, 2100143. [Google Scholar] [CrossRef]
- Yin, Y.; Pang, J.; Wang, J.; Lu, X.; Hao, Q.; Saei Ghareh Naz, E.; Zhou, X.; Ma, L.; Schmidt, O.G. Graphene-Activated Optoplasmonic Nanomembrane Cavities for Photodegradation Detection. ACS Appl. Mater. Interfaces 2019, 11, 15891–15897. [Google Scholar] [CrossRef]
- Fu, R.; Lu, Y.; Cheng, W. Soft Plasmonics: Design, Fabrication, Characterization, and Applications. Adv. Opt. Mater. 2022, 10, 2101436. [Google Scholar] [CrossRef]
- Veselago, V.G. The electrodynamics of substances with simultaneously negative values of mu and epsilon. Sov. Phys. Uspekhi 1968, 10, 509–514. [Google Scholar] [CrossRef]
- Ramakrishna, S.A. Physics of negative refractive index materials. Rep. Prog. Phys. 2005, 68, 449. [Google Scholar] [CrossRef]
- Sakoda, K. (Ed.) Electromagnetic Metamaterials; Springer: Singapore, 2019. [Google Scholar] [CrossRef]
- Ziolkowski, R.W.; Kipple, A.D. Causality and double-negative metamaterials. Phys. Rev. E 2003, 68, 026615. [Google Scholar] [CrossRef] [PubMed]
- Cummer, S.A.; Christensen, J.; Alù, A. Controlling sound with acoustic metamaterials. Nat. Rev. Mater. 2016, 1, 16001. [Google Scholar] [CrossRef]
- Li, Y.; Li, W.; Han, T.; Zheng, X.; Li, J.; Li, B.; Fan, S.; Qiu, C.-W. Transforming heat transfer with thermal metamaterials and devices. Nat. Rev. Mater. 2021, 6, 488–507. [Google Scholar] [CrossRef]
- Neshev, D.; Aharonovich, I. Optical metasurfaces: New generation building blocks for multi-functional optics. Light Sci. Appl. 2018, 7, 58. [Google Scholar] [CrossRef]
- Meinzer, N.; Barnes, W.L.; Hooper, I.R. Plasmonic meta-atoms and metasurfaces. Nat. Photonics 2014, 8, 889–898. [Google Scholar] [CrossRef]
- Jakšić, Z.; Smiljanić, M.M.; Vasiljević-Radović, D.; Obradov, M.; Radulović, K.; Tanasković, D.; Krstajić, P.M. Field localization control in aperture-based plasmonics by Boolean superposition of primitive forms at deep subwavelength scale. Opt. Quantum Electron. 2016, 48, 225. [Google Scholar] [CrossRef]
- Ding, F.; Pors, A.; Bozhevolnyi, S.I. Gradient metasurfaces: A review of fundamentals and applications. Rep. Prog. Phys. 2018, 81, 026401. [Google Scholar] [CrossRef]
- Shvartsburg, A.B.; Maradudin, A.A. Waves in Gradient Metamaterials; World Scientific: Singapore, 2013. [Google Scholar] [CrossRef]
- Ren, Y.; Lu, Y.; Zang, T.; Wang, Y.; Dai, Y.; Wang, P. Multi-mode resonance properties of two-dimensional metal-dielectric-metal fishnet metasurface at visible wavelengths. Opt. Express 2017, 25, 28417–28426. [Google Scholar] [CrossRef]
- Jakšić, Z.; Vuković, S.M.; Matovic, J.; Tanasković, D. Negative Refractive Index Metasurfaces for Enhanced Biosensing. Materials 2010, 4, 1–36. [Google Scholar] [CrossRef]
- Valentine, J.; Zhang, S.; Zentgraf, T.; Ulin-Avila, E.; Genov, D.A.; Bartal, G.; Zhang, X. Three-dimensional optical metamaterial with a negative refractive index. Nature 2008, 455, 376–379. [Google Scholar] [CrossRef]
- Brown, J. Artificial dielectrics having refractive indices less than unity. Proc. IEEE 1953, 100, 51–62. [Google Scholar]
- Wuttig, M.; Bhaskaran, H.; Taubner, T. Phase-change materials for non-volatile photonic applications. Nat. Photonics 2017, 11, 465–476. [Google Scholar] [CrossRef]
- Jahani, S.; Jacob, Z. All-dielectric metamaterials. Nat. Nanotechnol. 2016, 11, 23–36. [Google Scholar] [CrossRef]
- Bi, K.; Wang, Q.; Xu, J.; Chen, L.; Lan, C.; Lei, M. All-Dielectric Metamaterial Fabrication Techniques. Adv. Opt. Mater. 2021, 9, 2001474. [Google Scholar] [CrossRef]
- Drachev, V.P.; Podolskiy, V.A.; Kildishev, A.V. Hyperbolic metamaterials: New physics behind a classical problem. Opt. Express 2013, 21, 15048–15064. [Google Scholar] [CrossRef]
- Poddubny, A.; Iorsh, I.; Belov, P.; Kivshar, Y. Hyperbolic metamaterials. Nat. Photonics 2013, 7, 948–957. [Google Scholar] [CrossRef]
- Smolyaninov, I.I. Hyperbolic Metamaterials; IOP Publishing: Bristol, UK, 2018. [Google Scholar] [CrossRef][Green Version]
- High, A.A.; Devlin, R.C.; Dibos, A.; Polking, M.; Wild, D.S.; Perczel, J.; De Leon, N.P.; Lukin, M.D.; Park, H. Visible-frequency hyperbolic metasurface. Nature 2015, 522, 192–196. [Google Scholar] [CrossRef]
- Hu, G.; Krasnok, A.; Mazor, Y.; Qiu, C.-W.; Alù, A. Moiré Hyperbolic Metasurfaces. Nano Lett. 2020, 20, 3217–3224. [Google Scholar] [CrossRef]
- Smolyaninov, I.I. Photonic Hyper-Crystals. In Hyperbolic Metamaterials; Morgan & Claypool Publishers: San Rafael, CA, USA, 2018; pp. 4-1–4-11. [Google Scholar] [CrossRef]
- Ebrahimi Meymand, R.; Soleymani, A.; Granpayeh, N. All-optical AND, OR, and XOR logic gates based on coherent perfect absorption in graphene-based metasurface at terahertz region. Opt. Commun. 2020, 458, 124772. [Google Scholar] [CrossRef]
- Liu, C.; Ma, Q.; Luo, Z.J.; Hong, Q.R.; Xiao, Q.; Zhang, H.C.; Miao, L.; Yu, W.M.; Cheng, Q.; Li, L.; et al. A programmable diffractive deep neural network based on a digital-coding metasurface array. Nat. Electron. 2022, 5, 113–122. [Google Scholar] [CrossRef]
- Joo, W.-J.; Kyoung, J.; Esfandyarpour, M.; Lee, S.-H.; Koo, H.; Song, S.; Kwon, Y.-N.; Song, S.H.; Bae, J.C.; Jo, A.; et al. Metasurface-driven OLED displays beyond 10,000 pixels per inch. Science 2020, 370, 459–463. [Google Scholar] [CrossRef] [PubMed]
- Kim, I.; Yoon, G.; Jang, J.; Genevet, P.; Nam, K.T.; Rho, J. Outfitting Next Generation Displays with Optical Metasurfaces. ACS Photonics 2018, 5, 3876–3895. [Google Scholar] [CrossRef]
- Kim, G.; Kim, S.; Kim, H.; Lee, J.; Badloe, T.; Rho, J. Metasurface-empowered spectral and spatial light modulation for disruptive holographic displays. Nanoscale 2022, 14, 4380–4410. [Google Scholar] [CrossRef] [PubMed]
- Arbabi, A.; Arbabi, E.; Kamali, S.M.; Horie, Y.; Han, S.; Faraon, A. Miniature optical planar camera based on a wide-angle metasurface doublet corrected for monochromatic aberrations. Nat. Commun. 2016, 7, 13682. [Google Scholar] [CrossRef]
- Miyata, M.; Nakajima, M.; Hashimoto, T. Compound-eye metasurface optics enabling a high-sensitivity, ultra-thin polarization camera. Opt. Express 2020, 28, 9996–10014. [Google Scholar] [CrossRef]
- Wang, Z.; Zhang, H.; Zhao, H.; Cui, T.J.; Li, L. Intelligent electromagnetic metasurface camera: System design and experimental results. Nanophotonics 2022, 11, 2011–2024. [Google Scholar] [CrossRef]
- Huo, P.; Song, M.; Zhu, W.; Zhang, C.; Chen, L.; Lezec, H.J.; Lu, Y.; Agrawal, A.; Xu, T. Photorealistic full-color nanopainting enabled by a low-loss metasurface. Optica 2020, 7, 1171–1172. [Google Scholar] [CrossRef]
- Huang, L.; Zhang, S.; Zentgraf, T. Metasurface holography: From fundamentals to applications. Nanophotonics 2018, 7, 1169–1190. [Google Scholar] [CrossRef]
- Yang, Q.; Kruk, S.; Xu, Y.; Wang, Q.; Srivastava, Y.K.; Koshelev, K.; Kravchenko, I.; Singh, R.; Han, J.; Kivshar, Y.; et al. Mie-Resonant Membrane Huygens’ Metasurfaces. Adv. Funct. Mater. 2020, 30, 1906851. [Google Scholar] [CrossRef]
- Chen, W.T.; Khorasaninejad, M.; Zhu, A.Y.; Oh, J.; Devlin, R.C.; Zaidi, A.; Capasso, F. Generation of wavelength-independent subwavelength Bessel beams using metasurfaces. Light Sci. Appl. 2017, 6, e16259. [Google Scholar] [CrossRef]
- Eleftheriades, G.V.; Kim, M.; Ataloglou, V.G.; Dorrah, A.H. Prospects of Huygens’ Metasurfaces for Antenna Applications. Engineering 2022, 11, 21–26. [Google Scholar] [CrossRef]
- Krasnok, A.E.; Maksymov, I.S.; Denisyuk, A.I.; Belov, P.A.; Miroshnichenko, A.E.; Simovski, C.R.; Kivshar, Y.S. Optical nanoantennas. Phys. Uspekhi 2013, 56, 539. [Google Scholar] [CrossRef]
- Alu, A.; Engheta, N. Theory, modeling and features of optical nanoantennas. IEEE Trans. Antennas Propag. 2013, 61, 1508–1517. [Google Scholar] [CrossRef]
- Biagioni, P.; Huang, J.-S.; Hecht, B. Nanoantennas for visible and infrared radiation. Rep. Prog. Phys. 2012, 75, 024402. [Google Scholar] [CrossRef]
- Kildishev, A.V.; Boltasseva, A.; Shalaev, V.M. Planar photonics with metasurfaces. Science 2013, 339, 12320091–12320096. [Google Scholar] [CrossRef]
- Sharma, A.; Singh, V.; Bougher, T.L.; Cola, B.A. A carbon nanotube optical rectenna. Nat. Nanotechnol. 2015, 10, 1027–1032. [Google Scholar] [CrossRef]
- Hamied, F.M.A.; Mahmoud, K.R.; Hussein, M.; Obayya, S.S.A. Design and analysis of a nano-rectenna based on multi-insulator tunnel barrier for solar energy harvesting. Opt. Quantum Electron. 2022, 54, 144. [Google Scholar] [CrossRef]
- Zhang, Z.; Liu, P.; Lu, W.; Bai, P.; Zhang, B.; Chen, Z.; Maier, S.A.; Gómez Rivas, J.; Wang, S.; Li, X. High-Q collective Mie resonances in monocrystalline silicon nanoantenna arrays for the visible light. Fundam. Res. 2022, in press. [CrossRef]
- Alam, M.S.; Karim, F.; Zhao, C. Single-molecule detection at high concentrations with optical aperture nanoantennas. Nanoscale 2016, 8, 9480–9487. [Google Scholar] [CrossRef]
- Buch, Z.; Schmid, S. Design considerations of gold nanoantenna dimers for plasmomechanical transduction. Opt. Express 2022, 30, 5294–5303. [Google Scholar] [CrossRef] [PubMed]
- Wang, S.; Le-Van, Q.; Peyronel, T.; Ramezani, M.; Van Hoof, N.; Tiecke, T.G.; Gómez Rivas, J. Plasmonic Nanoantenna Arrays as Efficient Etendue Reducers for Optical Detection. ACS Photonics 2018, 5, 2478–2485. [Google Scholar] [CrossRef]
- Chekini, A.; Sheikhaei, S.; Neshat, M. An infrared energy harvesting device using planar cross bowtie nanoantenna arrays and diode-less rectification based on electron field emission. J. Mod. Opt. 2020, 67, 1348–1364. [Google Scholar] [CrossRef]
- Pacheco-Peña, V.; Alves, R.; Navarro-Cía, M. Hidden Symmetries in Bowtie Nanocavities and Diabolo Nanoantennas. ACS Photonics 2019, 6, 2014–2024. [Google Scholar] [CrossRef]
- Briones, E.; Cuadrado, A.; Briones, J.; Díaz de León, R.; Martínez-Antón, J.C.; McMurtry, S.; Hehn, M.; Montaigne, F.; Alda, J.; González, F.J. Seebeck nanoantennas for the detection and characterization of infrared radiation. Opt. Express 2014, 22, A1538–A1546. [Google Scholar] [CrossRef] [PubMed]
- Zhao, H.; Gao, H.; Cao, T.; Li, B. Efficient full-spectrum utilization, reception and conversion of solar energy by broad-band nanospiral antenna. Opt. Express 2018, 26, A178–A191. [Google Scholar] [CrossRef]
- Yang, Z.-J.; Zhao, Q.; Xiao, S.; He, J. Engineering two-wire optical antennas for near field enhancement. Photonics Nanostruct. 2017, 25, 72–76. [Google Scholar] [CrossRef]
- Tok, R.U.; Ow-Yang, C.W.; Şendur, K. Unidirectional broadband radiation of honeycomb plasmonic antenna array with broken symmetry. Opt. Express 2011, 19, 22731–22742. [Google Scholar] [CrossRef]
- Seitl, L.; Laible, F.; Dickreuter, S.; Gollmer, D.A.; Kern, D.P.; Fleischer, M. Miniaturized fractal optical nanoantennas defined by focused helium ion beam milling. Nanotechnology 2020, 31, 075301. [Google Scholar] [CrossRef]
- Maksymov, I.S.; Staude, I.; Miroshnichenko, A.E.; Kivshar, Y.S. Optical Yagi-Uda nanoantennas. Nanophotonics 2012, 1, 65–81. [Google Scholar] [CrossRef]
- Fan, J.R.; Wu, W.G.; Chen, Z.J.; Zhu, J.; Li, J. Three-dimensional cavity nanoantennas with resonant-enhanced surface plasmons as dynamic color-tuning reflectors. Nanoscale 2017, 9, 3416–3423. [Google Scholar] [CrossRef]
- Ali, F.; Aksu, S. A Narrow-Band Multi-Resonant Metamaterial in Near-IR. Materials 2020, 13, 5140. [Google Scholar] [CrossRef]
- Sugimoto, H.; Fujii, M. Broadband Dielectric–Metal Hybrid Nanoantenna: Silicon Nanoparticle on a Mirror. ACS Photonics 2018, 5, 1986–1993. [Google Scholar] [CrossRef]
- Ni, X.; Emani, N.K.; Kildishev, A.V.; Boltasseva, A.; Shalaev, V.M. Broadband Light Bending with Plasmonic Nanoantennas. Science 2012, 335, 427-427. [Google Scholar] [CrossRef]
- Ren, Z.; Zhang, Z.; Wei, J.; Dong, B.; Lee, C. Wavelength-multiplexed hook nanoantennas for machine learning enabled mid-infrared spectroscopy. Nat. Commun. 2022, 13, 3859. [Google Scholar] [CrossRef]
- Li, N.; Lai, Y.; Lam, S.H.; Bai, H.; Shao, L.; Wang, J. Directional Control of Light with Nanoantennas. Adv. Opt. Mater. 2021, 9, 2001081. [Google Scholar] [CrossRef]
- Sortino, L.; Zotev, P.G.; Mignuzzi, S.; Cambiasso, J.; Schmidt, D.; Genco, A.; Aßmann, M.; Bayer, M.; Maier, S.A.; Sapienza, R.; et al. Enhanced light-matter interaction in an atomically thin semiconductor coupled with dielectric nano-antennas. Nat. Commun. 2019, 10, 5119. [Google Scholar] [CrossRef]
- Barreda, A.; Hell, S.; Weissflog, M.A.; Minovich, A.; Pertsch, T.; Staude, I. Metal, dielectric and hybrid nanoantennas for enhancing the emission of single quantum dots: A comparative study. J. Quant. Spectrosc. Radiat. Transf. 2021, 276, 107900. [Google Scholar] [CrossRef]
- McGurn, A. Nanophotonics; Springer: Cham, Switzerland, 2018. [Google Scholar] [CrossRef]
- Pérez, D.; Gasulla, I.; Das Mahapatra, P.; Capmany, J. Principles, fundamentals, and applications of programmable integrated photonics. Adv. Opt. Photonics 2020, 12, 709–786. [Google Scholar] [CrossRef]
- López, A.; Pérez, D.; DasMahapatra, P.; Capmany, J. Auto-routing algorithm for field-programmable photonic gate arrays. Opt. Express 2020, 28, 737–752. [Google Scholar] [CrossRef]
- Zhang, M.; Wang, C.; Kharel, P.; Zhu, D.; Lončar, M. Integrated lithium niobate electro-optic modulators: When performance meets scalability. Optica 2021, 8, 652–667. [Google Scholar] [CrossRef]
- Baranov, D.G.; Xiao, Y.; Nechepurenko, I.A.; Krasnok, A.; Alù, A.; Kats, M.A. Nanophotonic engineering of far-field thermal emitters. Nat. Mater. 2019, 18, 920–930. [Google Scholar] [CrossRef] [PubMed]
- Marin-Palomo, P.; Kemal, J.N.; Karpov, M.; Kordts, A.; Pfeifle, J.; Pfeiffer, M.H.P.; Trocha, P.; Wolf, S.; Brasch, V.; Anderson, M.H.; et al. Microresonator-based solitons for massively parallel coherent optical communications. Nature 2017, 546, 274–279. [Google Scholar] [CrossRef] [PubMed]
- Ge, F.; Han, X.; Xu, J. Strongly Coupled Systems for Nonlinear Optics. Laser Photonics Rev. 2021, 15, 2000514. [Google Scholar] [CrossRef]
- Gong, T.; Corrado, M.R.; Mahbub, A.R.; Shelden, C.; Munday, J.N. Recent progress in engineering the Casimir effect—Applications to nanophotonics, nanomechanics, and chemistry. Nanophotonics 2021, 10, 523–536. [Google Scholar] [CrossRef]
- Zhou, X.; Kulkova, I.; Lund-Hansen, T.; Hansen, S.L.; Lodahl, P.; Midolo, L. High-efficiency shallow-etched grating on GaAs membranes for quantum photonic applications. Appl. Phys. Lett. 2018, 113, 251103. [Google Scholar] [CrossRef]
- Shadmani, A.; Thomas, R.A.; Liu, Z.; Papon, C.; Heck, M.J.R.; Volet, N.; Scholz, S.; Wieck, A.D.; Ludwig, A.; Lodahl, P.; et al. Integration of GaAs waveguides on a silicon substrate for quantum photonic circuits. Opt. Express 2022, 30, 37595–37602. [Google Scholar] [CrossRef]
- Jiang, P.; Balram, K.C. Suspended gallium arsenide platform for building large scale photonic integrated circuits: Passive devices. Opt. Express 2020, 28, 12262–12271. [Google Scholar] [CrossRef]
- Wang, J.; Yin, Y.; Yang, Y.-D.; Hao, Q.; Tang, M.; Wang, X.; Saggau, C.N.; Karnaushenko, D.; Yan, X.; Huang, Y.-Z.; et al. Deterministic Yet Flexible Directional Light Emission from Spiral Nanomembrane Cavities. ACS Photonics 2019, 6, 2537–2544. [Google Scholar] [CrossRef]
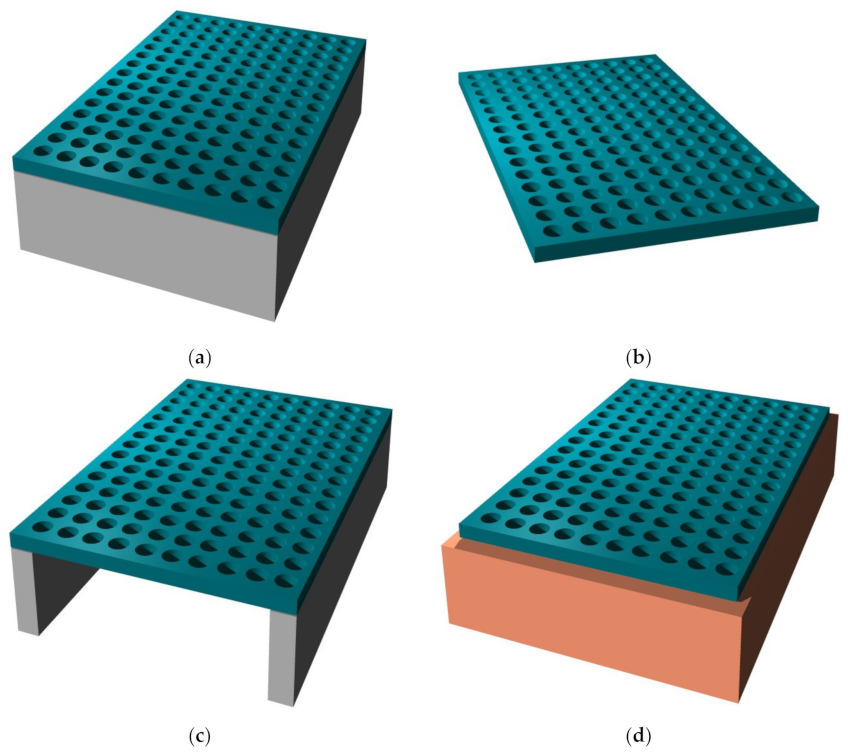

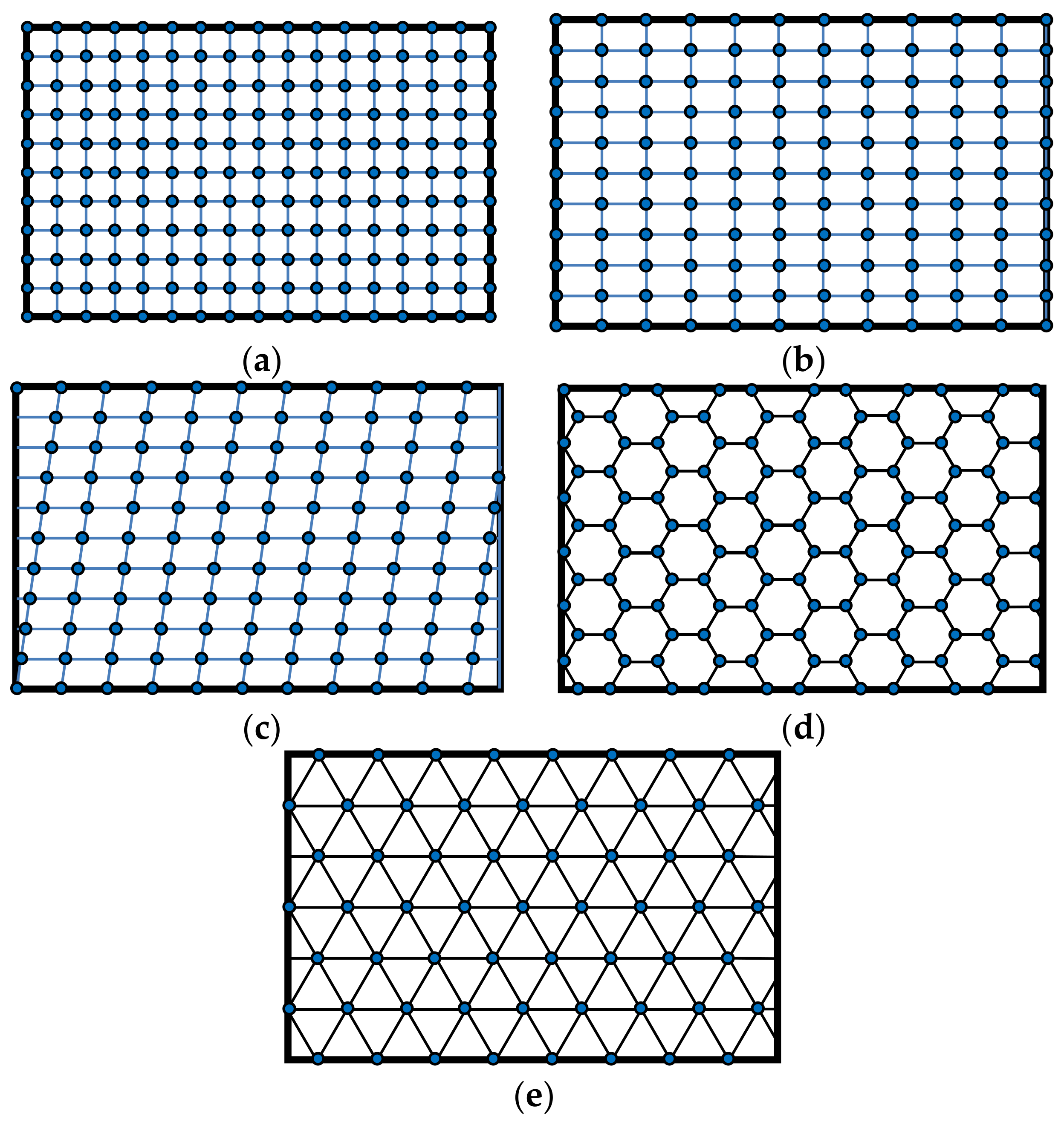
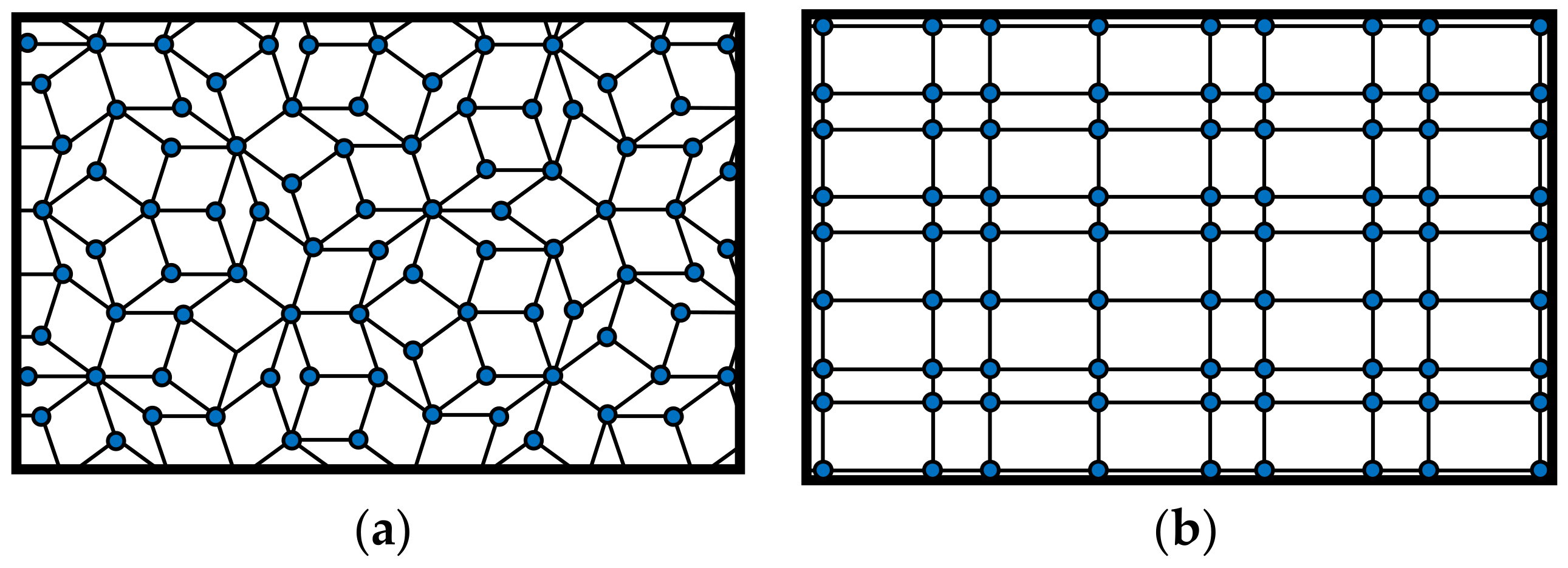
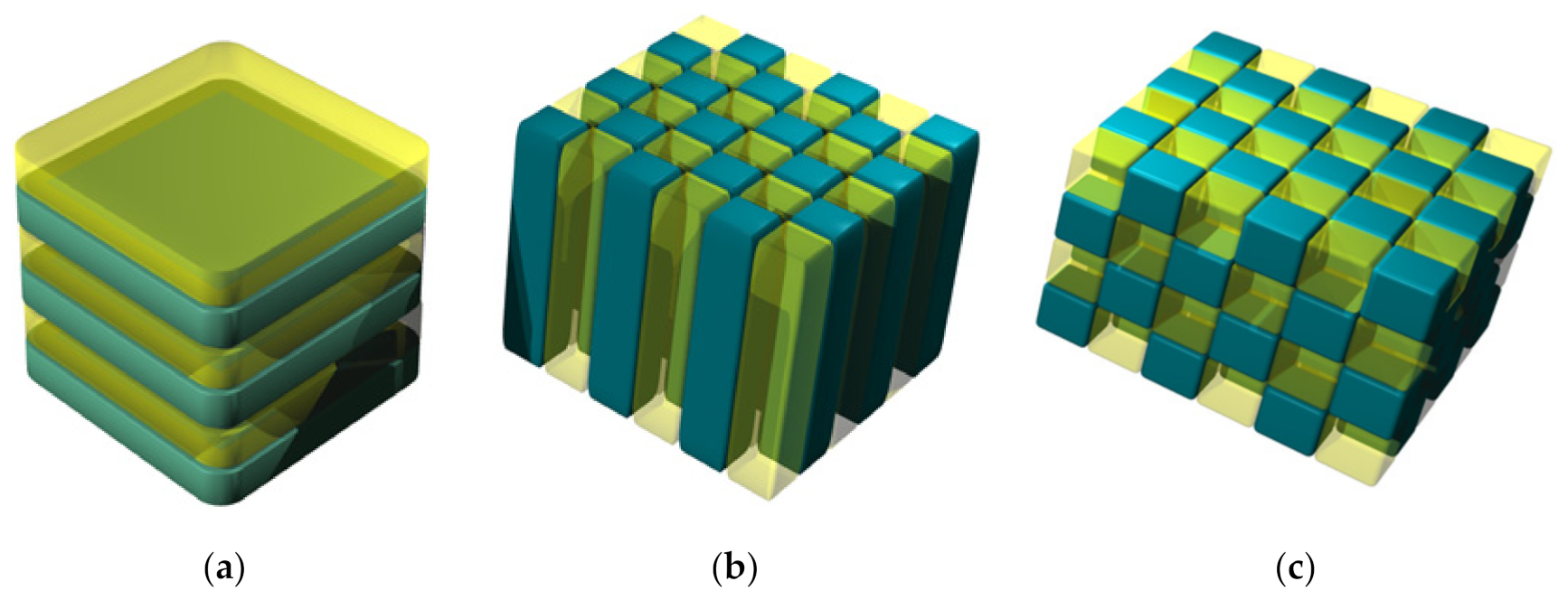




| Synthetic Nanomembranes for Nano-Optics | Types of Nanomembranes |
|---|---|
| Inorganic | Pure metal (e.g., gold, chromium) [23] Metal composites (mixed matrix) and alloys (e.g., Cr + Si) [24] |
| Diamond (nanocrystallite sheets) [25] Diamondoids (adamantane, tetramantane) [26] Diamond-like carbon (DLC) nanomembranes (hard carbon allotrope) [27] Carbon nanomembranes–cross-linked carbon precursors [28] | |
| Single element semiconductors (silicon, germanium) [29] Compound semiconductors [30] | |
| Freestanding monatomic sheets (e.g., graphene, borophene) [31] Twisted bilayers-moiré structures (e.g., graphene bilayers) [32] Freestanding inorganic monomolecular sheets (e.g., MXenes) [33] | |
| Organic-inorganic hybrids | Interpenetrated structures (e.g., polyacrylate interpenetrated with ZrO2, SiO2) [34] Metal–organic frameworks (metal ions or ion clusters and organic molecules) [35] |
| Organic (polymer-based) | Single-polymer (pure)–e.g., polyester, polystyrene [36] Copolymer (2 or more different polymers blended) [37] |
| ε, μ | Type of Waves | Natural/Artificial | Name |
|---|---|---|---|
| ε < 0 μ > 0 | Evanescent | Metals Drude-type free electron conductors | ENG (epsilon-negative) |
| ε > 0 μ > 0 | Propagating | Lossless dielectrics Low-loss semiconductors | PRM (positive refractive index materials) |
| ε < 0 μ < 0 | Propagating | Artificial | DNG (NRM) (double-negative materials) (negative refractive index) |
| ε > 0 μ < 0 | Evanescent | frequencies below GHz: gyrotropic magnetics optical frequencies: artificial | MNG (magnetic permeability negative) |
| ε ≈ 0 μ ≈ 1 | Evanescent | Artificial | ENZ (near-zero permittivity) (near-zero refractive index) |
| ε >> 0 μ > 0 | Propagating | Artificial | EVL (permittivity very large) (refractive index very large) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jakšić, Z.; Obradov, M.; Jakšić, O. Bio-Inspired Nanomembranes as Building Blocks for Nanophotonics, Plasmonics and Metamaterials. Biomimetics 2022, 7, 222. https://doi.org/10.3390/biomimetics7040222
Jakšić Z, Obradov M, Jakšić O. Bio-Inspired Nanomembranes as Building Blocks for Nanophotonics, Plasmonics and Metamaterials. Biomimetics. 2022; 7(4):222. https://doi.org/10.3390/biomimetics7040222
Chicago/Turabian StyleJakšić, Zoran, Marko Obradov, and Olga Jakšić. 2022. "Bio-Inspired Nanomembranes as Building Blocks for Nanophotonics, Plasmonics and Metamaterials" Biomimetics 7, no. 4: 222. https://doi.org/10.3390/biomimetics7040222
APA StyleJakšić, Z., Obradov, M., & Jakšić, O. (2022). Bio-Inspired Nanomembranes as Building Blocks for Nanophotonics, Plasmonics and Metamaterials. Biomimetics, 7(4), 222. https://doi.org/10.3390/biomimetics7040222








