A Symmetric Three Degree of Freedom Tensegrity Mechanism with Dual Operation Modes for Robot Actuation
Abstract
1. Introduction
- A robust and light weight linkage: The mechanism is mass efficient as a linkage to stably connect two bodies of a robot while providing fault tolerance of its elements, making it suited for planetary exploration, mass sensitive scenarios and applications in harsh environments.
- Dual mode operation: The mechanism can operate fully passive or actuated, without losing its structural integrity. This enables the robot to adapt to different scenarios to save energy when necessary.
- Good manipulability: The mechanism is agile and easy to control compared with existing tensegrity implementations. It is suitable for applications requiring a large degree of deformation and accurate estimation of state.
- Easy combination with conventional robot frameworks: The mechanism realises a clean transition between tensegrity and conventional structures, enabling a rapid attachment and detachment to target robots, and with only minor interference with the payload of the conventional segment.
2. Materials and Methods
2.1. Design Concept
- Minimal detrimental antagonistic forces within the structure;
- Minimal intrusion of tensegrity structure into the conventional structure;
- Clean segmentation between tensegrity and conventional structure;
- Three degrees of rotational freedom; and
- Optimised power efficiency.
2.2. Numerical Analysis
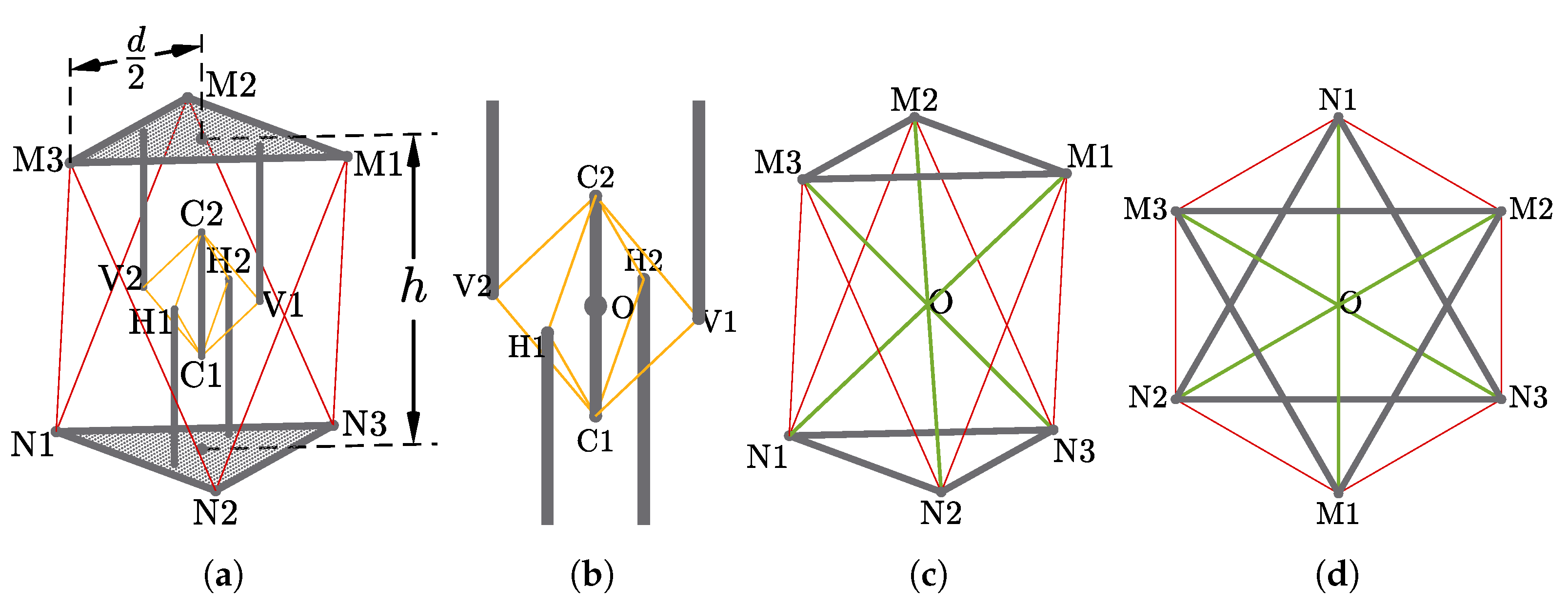
2.2.1. Universal Joint-Like Passive Substructure
2.2.2. Parallel Manipulator-Like Actuated Connection
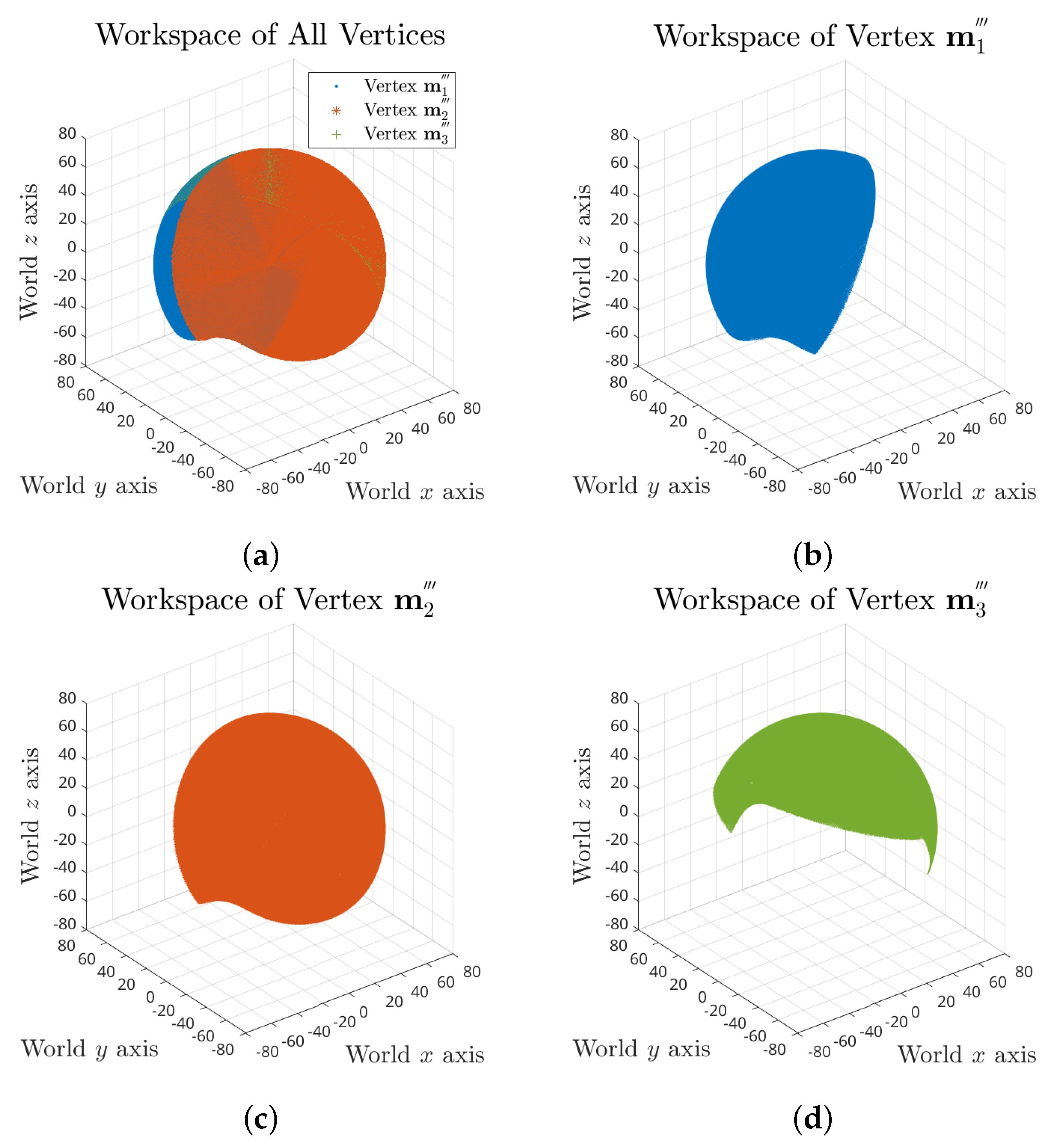
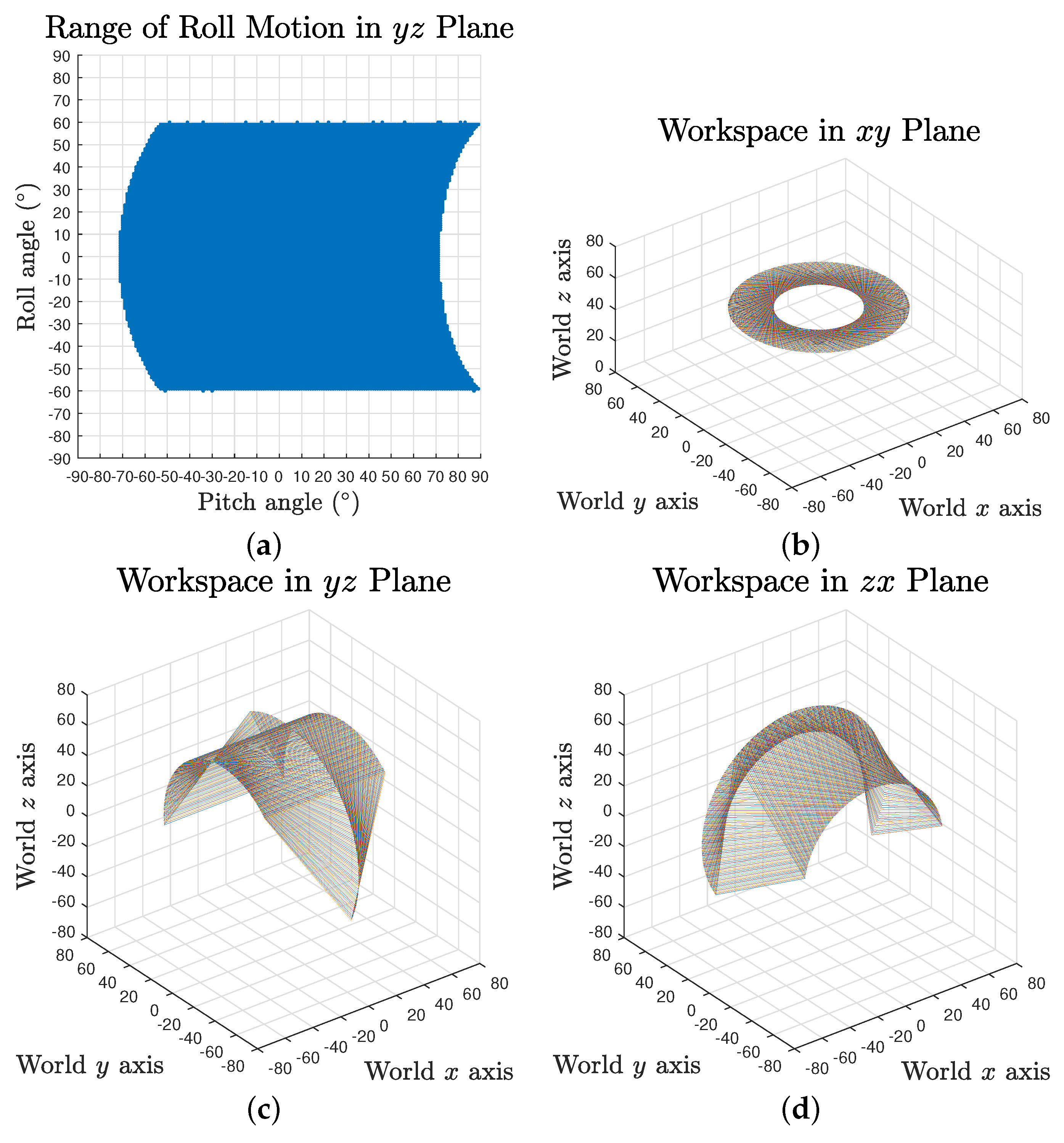
2.2.3. Workspace of the Tensegrity Mechanism
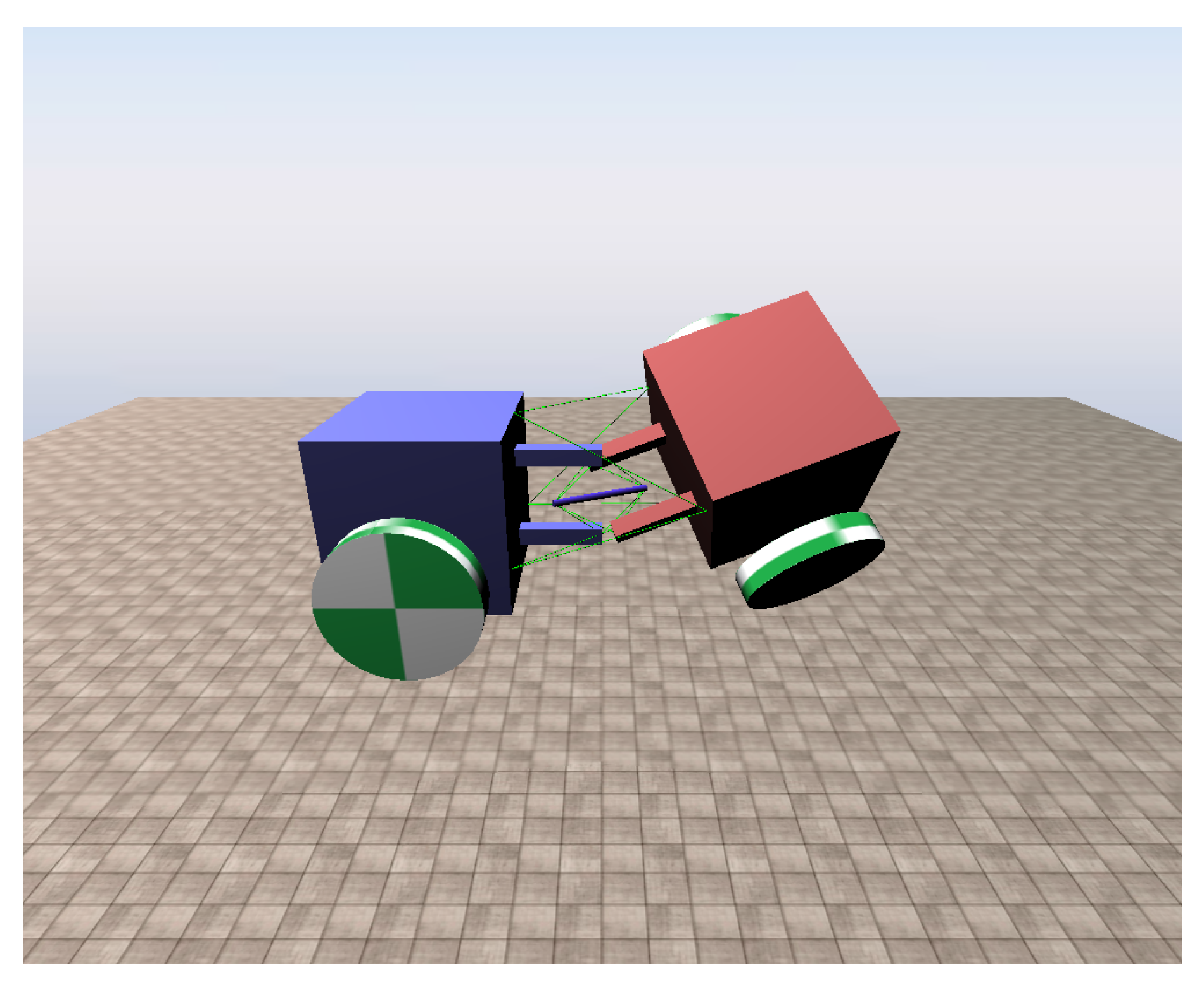
2.3. Simulation Methods
2.3.1. FEM Cable Based Simulation
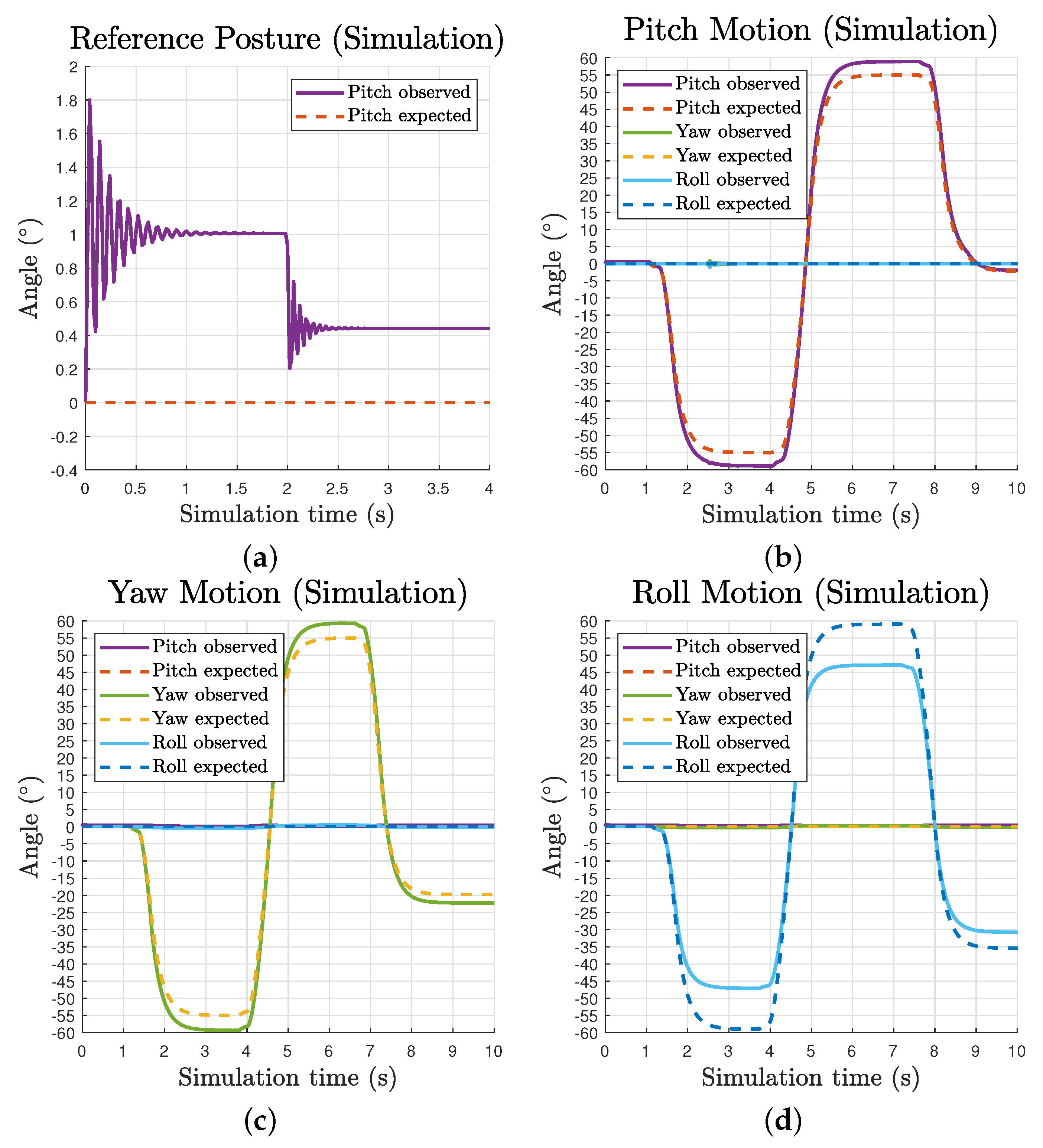
2.3.2. Quaternion Based Motion Control
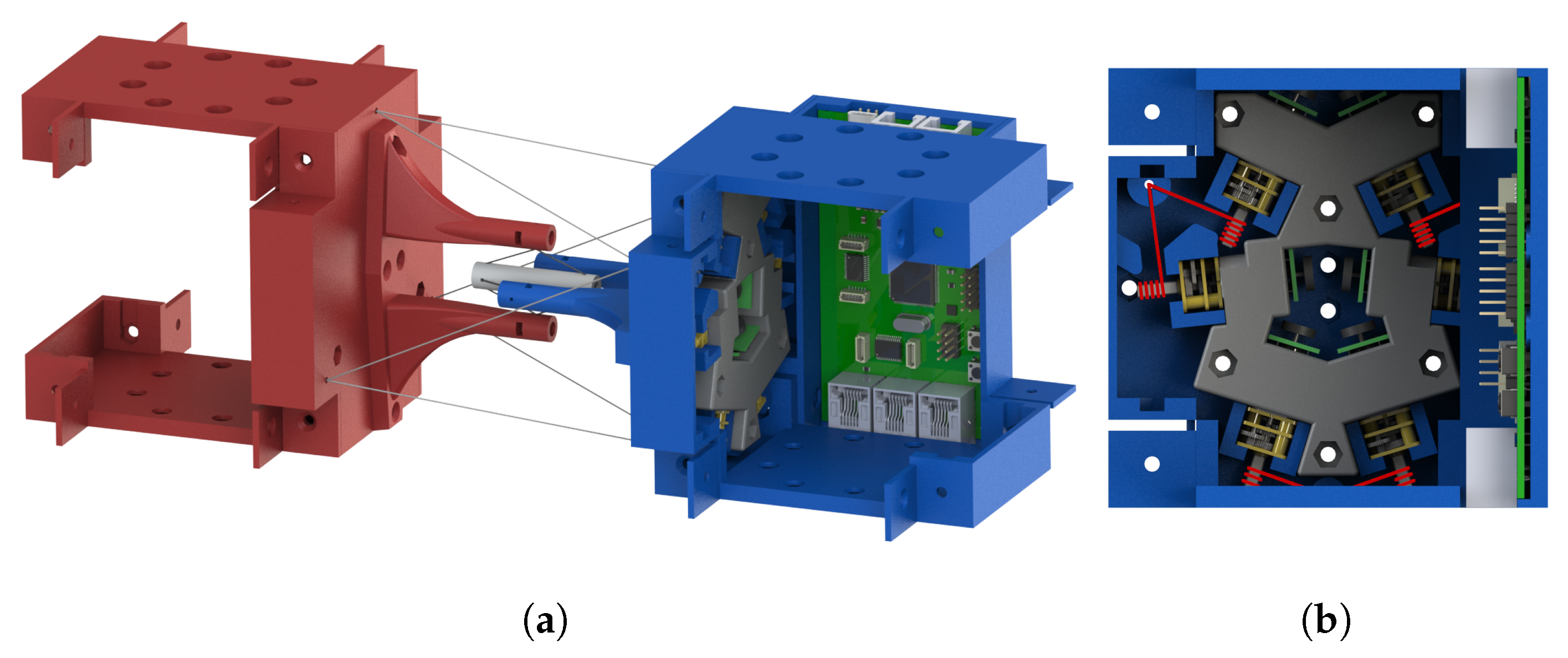
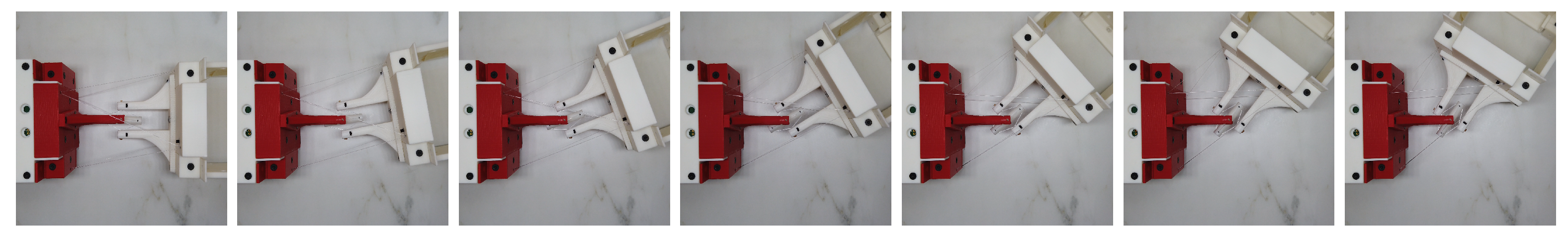
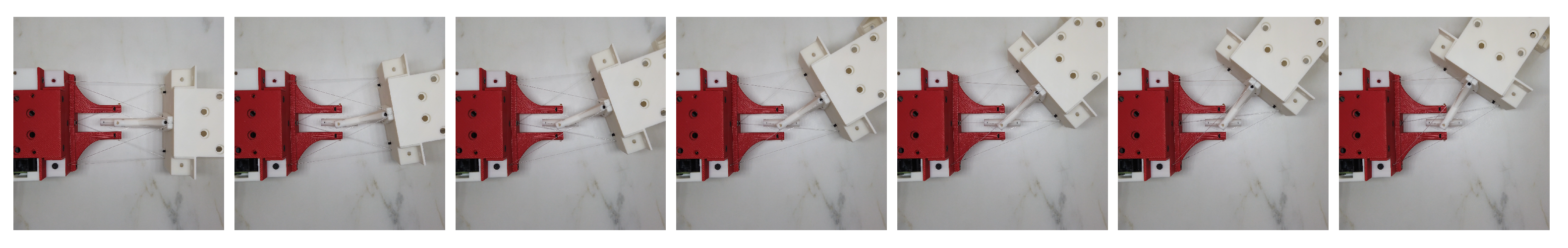
2.4. Prototype Configuration
3. Results
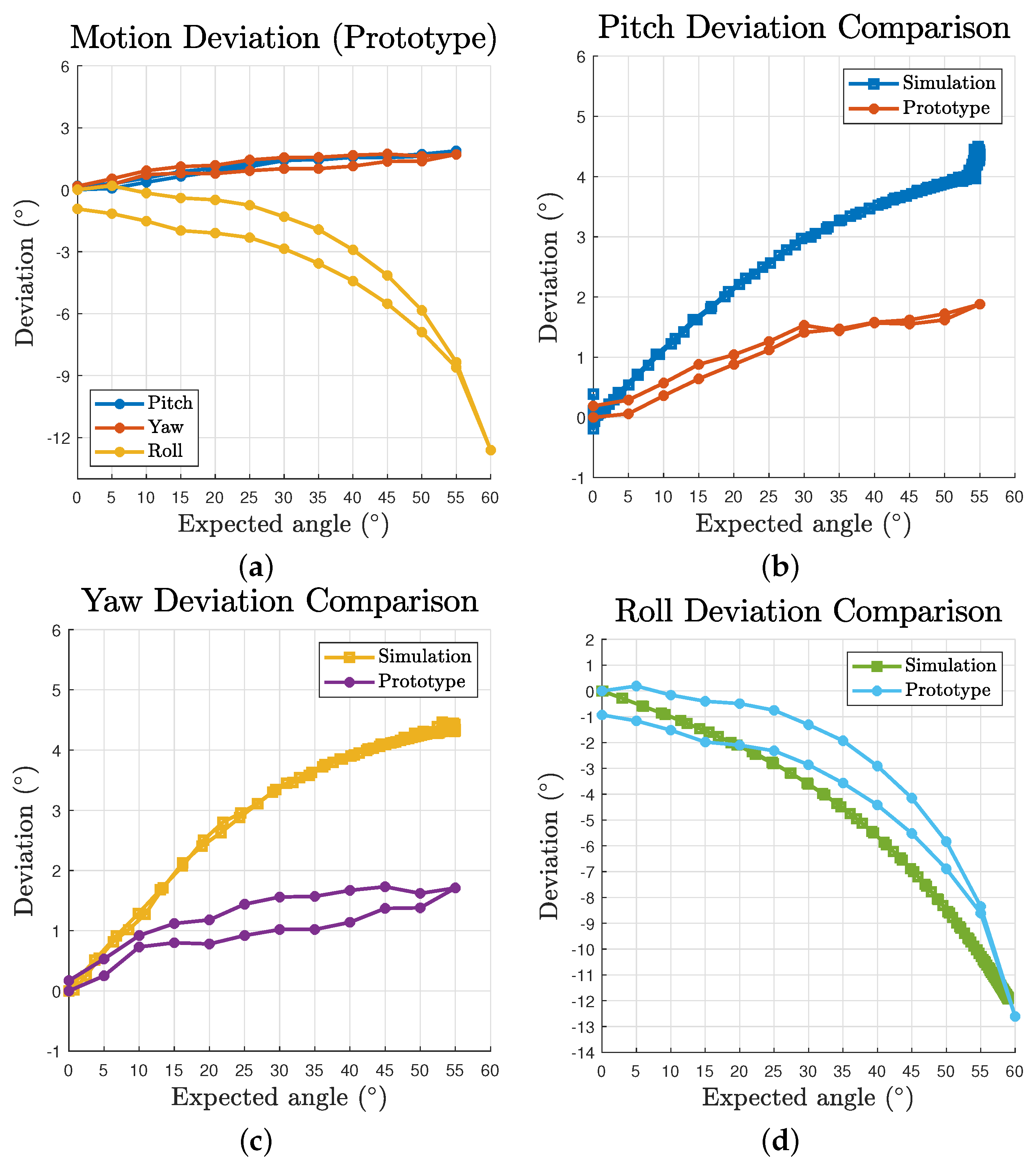
3.1. Numerical Analysis Results
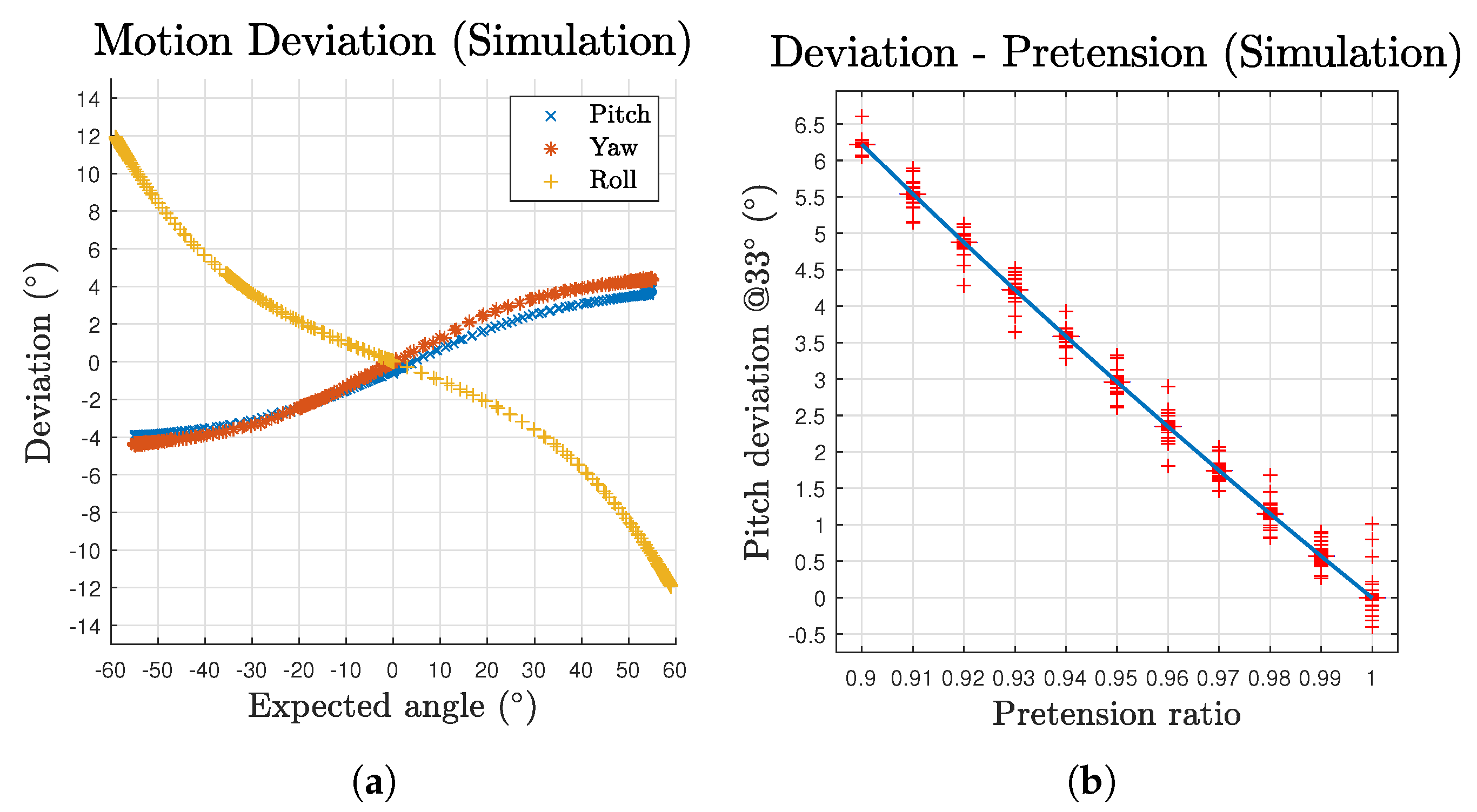
3.2. Simulation Results
3.3. Prototype Test Results
4. Conclusions and Future Work
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Michell, A.G.M. LVIII. The limits of economy of material in frame-structures. London Edinburgh Dublin Philos. Mag. J. Sci. 1904, 8, 589–597. [Google Scholar] [CrossRef]
- Buckminster Fuller, R.; Marks, R. The Dymaxion World of Buckminster Fuller; Anchor Books: Garden City, NY, USA, 1973. [Google Scholar]
- Komendera, E. A Survey of the Computational Modeling and Control of Tensegrity Robots. Ph.D. Thesis, Prelim Exam in Computational Modeling of Complex Systems, University of Colorado, Boulder, CO, USA, 2012. [Google Scholar]
- Skelton, R.; Adhikari, R.; Pinaud, J.P.; Chan, W.; Helton, J. An introduction to the mechanics of tensegrity structures. In Proceedings of the 40th IEEE Conference on Decision and Control (Cat. No.01CH37228), Orlando, FL, USA, 4–7 December 2001; Volume 5, pp. 4254–4259. [Google Scholar] [CrossRef]
- Chen, L.; Cera, B.; Zhu, E.L.; Edmunds, R.; Rice, F.; Bronars, A.; Tang, E.; Malekshahi, S.R.; Romero, O.; Agogino, A.K.; et al. Inclined surface locomotion strategies for spherical tensegrity robots. In Proceedings of the 2017 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Vancouver, BC, Canada, 24–28 September 2017; pp. 4976–4981. [Google Scholar]
- Friesen, J.M.; Glick, P.; Fanton, M.; Manovi, P.; Xydes, A.; Bewley, T.; Sunspiral, V. The second generation prototype of a Duct Climbing Tensegrity robot, DuCTTv2. In Proceedings of the 2016 IEEE International Conference on Robotics and Automation, Stockholm, Sweden, 16–21 May 2016; pp. 2123–2128. [Google Scholar]
- Tietz, B.R.; Carnahan, R.W.; Bachmann, R.J.; Quinn, R.D.; SunSpiral, V. Tetraspine: Robust terrain handling on a tensegrity robot using central pattern generators. In Proceedings of the 2013 IEEE/ASME International Conference on Advanced Intelligent Mechatronics, Wollongong, Australia, 9–12 July 2013; pp. 261–267. [Google Scholar]
- Paul, C.; Valero-Cuevas, F.J.; Lipson, H. Design and control of tensegrity robots for locomotion. IEEE Trans. Rob. 2006, 22, 944–957. [Google Scholar] [CrossRef]
- Zappetti, D.; Mintchev, S.; Shintake, J.; Floreano, D. Bio-inspired Tensegrity Soft Modular Robots. In Biomimetic and Biohybrid Systems; Springer: Berlin, Germany, 2017; pp. 497–508. [Google Scholar]
- Böhm, V.; Zimmermann, K. Vibration-driven mobile robots based on single actuated tensegrity structures. In Proceedings of the 2013 IEEE International Conference on Robotics and Automation, Karlsruhe, Germany, 6–10 May 2013; pp. 5475–5480. [Google Scholar]
- Kaufhold, T.; Schale, F.; Böhm, V.; Zimmermann, K. Indoor Locomotion Experiments of a Spherical Mobile Robot Based on a Tensegrity Structure with Curved Compressed Members. In Proceedings of the IEEE/ASME International Conference on Advanced Intelligent Mechatronics, AIM, Munich, Germany, 3–7 July 2017; pp. 523–528. [Google Scholar]
- Hustig-Schultz, D.; SunSpiral, V.; Teodorescu, M. Morphological design for controlled tensegrity quadruped locomotion. In Proceedings of the 2016 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Daejeon, Korea, 9–14 October 2016; pp. 4714–4719. [Google Scholar]
- Sabelhaus, A.P. Tensegrity Spines for Quadruped Robots. Ph.D. Thesis, UC Berkeley, Berkeley, CA, USA, 2019. [Google Scholar]
- Mirletz, B.T.; Park, I.W.; Flemons, T.E.; Agogino, A.K.; Quinn, R.D.; SunSpiral, V. Design and control of modular spine-like tensegrity structures. In Proceedings of the 6th World Conference of the International Association for Structural Control and Monitoring (6WCSCM), Barcelona, Spain, 15–17 July 2014; pp. 4–10. [Google Scholar]
- Bruce, J.; Caluwaerts, K.; Iscen, A.; Sabelhaus, A.P.; SunSpiral, V. Design and evolution of a modular tensegrity robot platform. In Proceedings of the 2014 IEEE International Conference on Robotics and Automation (ICRA), Hong Kong, China, 31 May–7 June 2014; pp. 3483–3489. [Google Scholar]
- Carre no, F.; Post, M.A. Design of a novel wheeled tensegrity robot: A comparison of tensegrity concepts and a prototype for travelling air ducts. Robot. Biomimetics 2018, 5, 1–24. [Google Scholar] [CrossRef] [PubMed]
- Lessard, S.; Bruce, J.; Jung, E.; Teodorescu, M.; SunSpiral, V.; Agogino, A. A lightweight, multi-axis compliant tensegrity joint. In Proceedings of the 2016 IEEE International Conference on Robotics and Automation (ICRA), Stockholm, Sweden, 16–21 May 2016; pp. 630–635. [Google Scholar]
- Lessard, S.; Castro, D.; Asper, W.; Chopra, S.D.; Baltaxe-Admony, L.B.; Teodorescu, M.; Sunspiral, V.; Agogino, A. A bio-inspired tensegrity manipulator with multi-DOF, structurally compliant joints. In Proceedings of the 2016 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Daejeon, Korea, 9–14 October 2016; pp. 5515–5520. [Google Scholar]
- Ramadoss, V.; Sagar, K.; Ikbal, M.S.; Calles, J.H.L.; Zoppi, M. HEDRA: A Bio-Inspired Modular Tensegrity Soft Robot with Polyhedral Parallel Modules. arXiv 2011, arXiv:2011.14240. Available online: https://arxiv.org/pdf/2011.14240.pdf (accessed on 16 December 2020).
- Naribole, S.; Anil, R.K.; Chakraborty, G. Design, Mathematical Modelling and Analysis of Externally Actuated Somersaulting Tensegrity Spine. In Proceedings of the 2020 6th International Conference on Mechatronics and Robotics Engineering (ICMRE), Barcelona, Spain, 12–15 February 2020; pp. 135–139. [Google Scholar]
- Friesen, J.M.; Dean, J.L.; Bewley, T.; Sunspiral, V. A Tensegrity-Inspired Compliant 3-DOF Compliant Joint. In Proceedings of the 2018 IEEE International Conference on Robotics and Automation (ICRA), Brisbane, Australia, 21–25 May 2018; pp. 3301–3306. [Google Scholar]
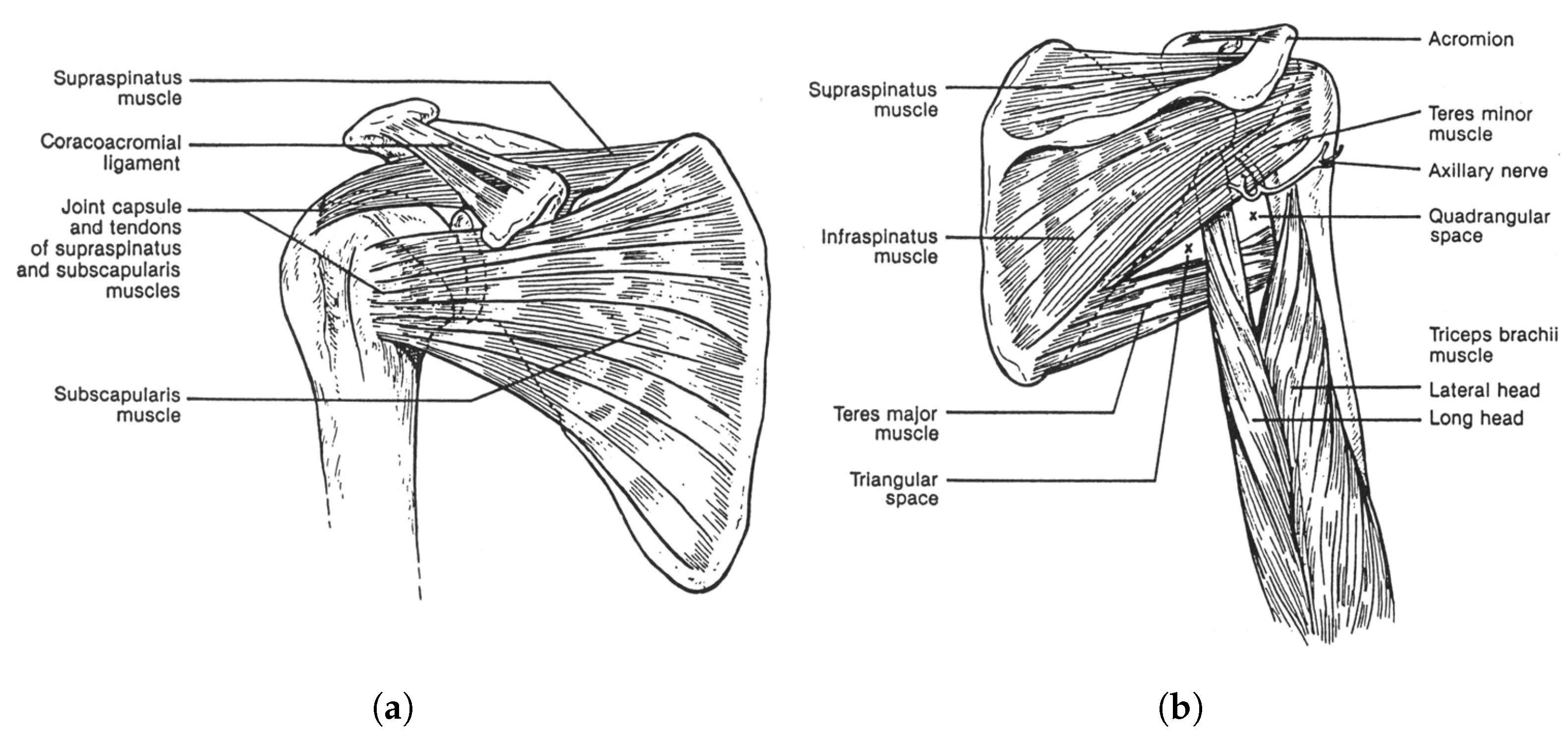
- Pratt, N.E. Anatomy and biomechanics of the shoulder. J. Hand Ther. 1994, 7, 65–76. [Google Scholar] [CrossRef]
- Post, M.A.; Austin, J. Knowledge-Based Self-Reconfiguration And Self-Aware Demonstration For Modular Satellite Assembly. In Proceedings of the 10th International Workshop on Satellite Constellations & Formation Flying, Glasgow, Scotland, 16–19 July 2019. [Google Scholar]
- Pham, C.B.; Yeo, S.H.; Yang, G.; Kurbanhusen, M.S.; Chen, I.M. Force-closure workspace analysis of cable-driven parallel mechanisms. Mech. Mach. Theory 2006, 41, 53–69. [Google Scholar] [CrossRef]
- Tasora, A.; Serban, R.; Mazhar, H.; Pazouki, A.; Melanz, D.; Fleischmann, J.; Taylor, M.; Sugiyama, H.; Negrut, D. Chrono: An Open Source Multi-physics Dynamics Engine. In High Performance Computing in Science and Engineering; Springer: Berlin, Germany, 2016; pp. 19–49. [Google Scholar]
- Taylor, M.; Serban, R. Validation of Basic Modeling Elements in Chrono. Technical Report. 2015. Available online: https://sbel.wiscweb.wisc.edu/wp-content/uploads/sites/569/2018/05/TR-2015-05.pdf (accessed on 24 January 2021).
- SunSpiral, V.; Agogino, A.; Atkinson, D. Super Ball Bot—Structures for Planetary Landing and Exploration, NIAC Phase 2 Final Report. 2015. Available online: https://www.nasa.gov/sites/default/files/atoms/files/niac_sunspiral_superballbot_phaseii_finalreport_tagged.pdf (accessed on 29 April 2020).
- Recuero, A.; Negrut, D. Validation of a Gradient-Deficient ANCF Beam Element in Chrono: FEA. Technical Report. 2016. Available online: https://www.projectchrono.org/assets/validations/FEA/ancfBeamValidation.pdf (accessed on 24 January 2021).

| Cable | ||||||
|---|---|---|---|---|---|---|
| ✓ | ✓ | ✓ | ✓ | ✓ | × | |
| / | ✓ | ✓ | × | ✓ | ✓ | |
| / | / | ✓ | ✓ | × | ✓ | |
| / | / | / | ✓ | ✓ | ✓ | |
| / | / | / | / | ✓ | ✓ | |
| / | / | / | / | / | ✓ |
| Motion | Pitch | Yaw | Roll |
|---|---|---|---|
| Half complete difference () | 1.25 | 0.65 | −0.77 |
| Full complete difference () | 1.42 | 0.52 | −0.79 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, T.; Post, M.A. A Symmetric Three Degree of Freedom Tensegrity Mechanism with Dual Operation Modes for Robot Actuation. Biomimetics 2021, 6, 30. https://doi.org/10.3390/biomimetics6020030
Wang T, Post MA. A Symmetric Three Degree of Freedom Tensegrity Mechanism with Dual Operation Modes for Robot Actuation. Biomimetics. 2021; 6(2):30. https://doi.org/10.3390/biomimetics6020030
Chicago/Turabian StyleWang, Tianyuan, and Mark A. Post. 2021. "A Symmetric Three Degree of Freedom Tensegrity Mechanism with Dual Operation Modes for Robot Actuation" Biomimetics 6, no. 2: 30. https://doi.org/10.3390/biomimetics6020030
APA StyleWang, T., & Post, M. A. (2021). A Symmetric Three Degree of Freedom Tensegrity Mechanism with Dual Operation Modes for Robot Actuation. Biomimetics, 6(2), 30. https://doi.org/10.3390/biomimetics6020030







