The Design, Modeling, and Experiment of a Novel Diving-Beetle-Inspired Paddling Propulsion Robot
Abstract
1. Introduction
2. Design of Bionic Paddling Robots
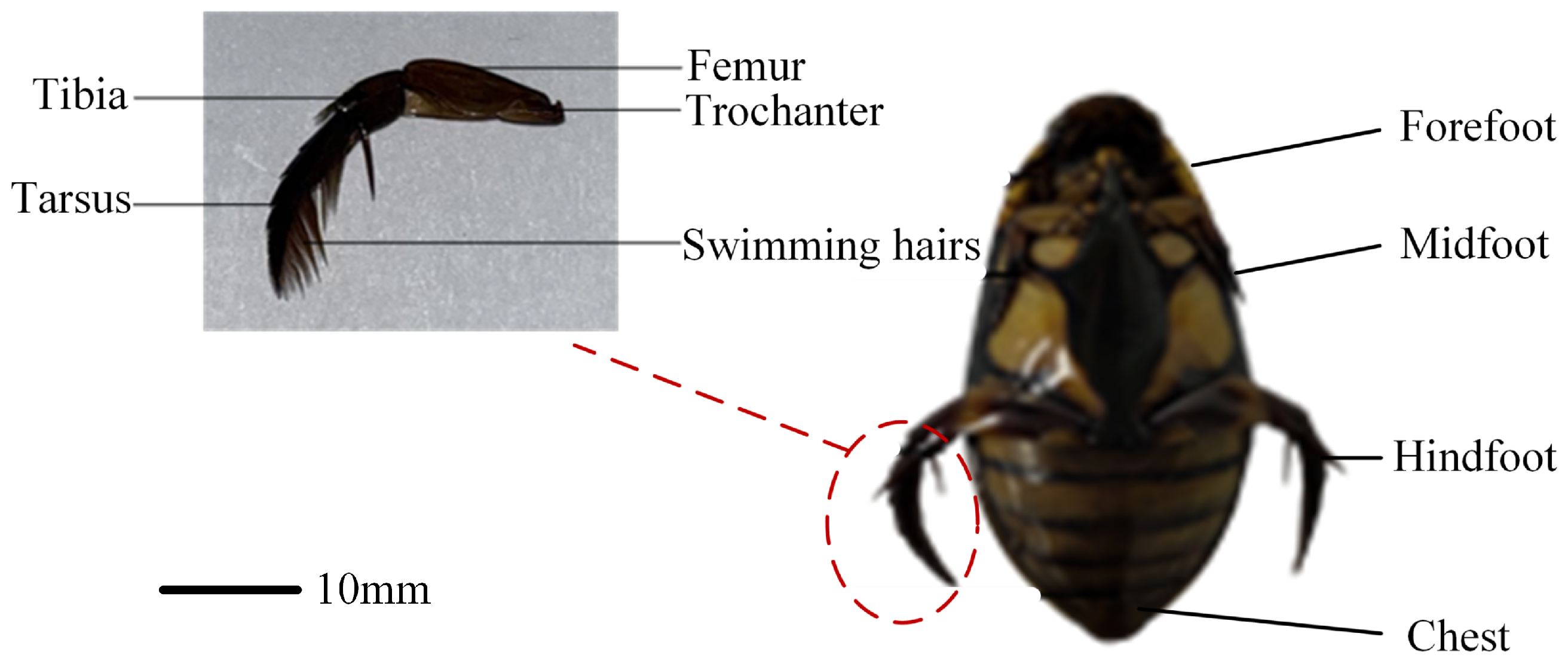
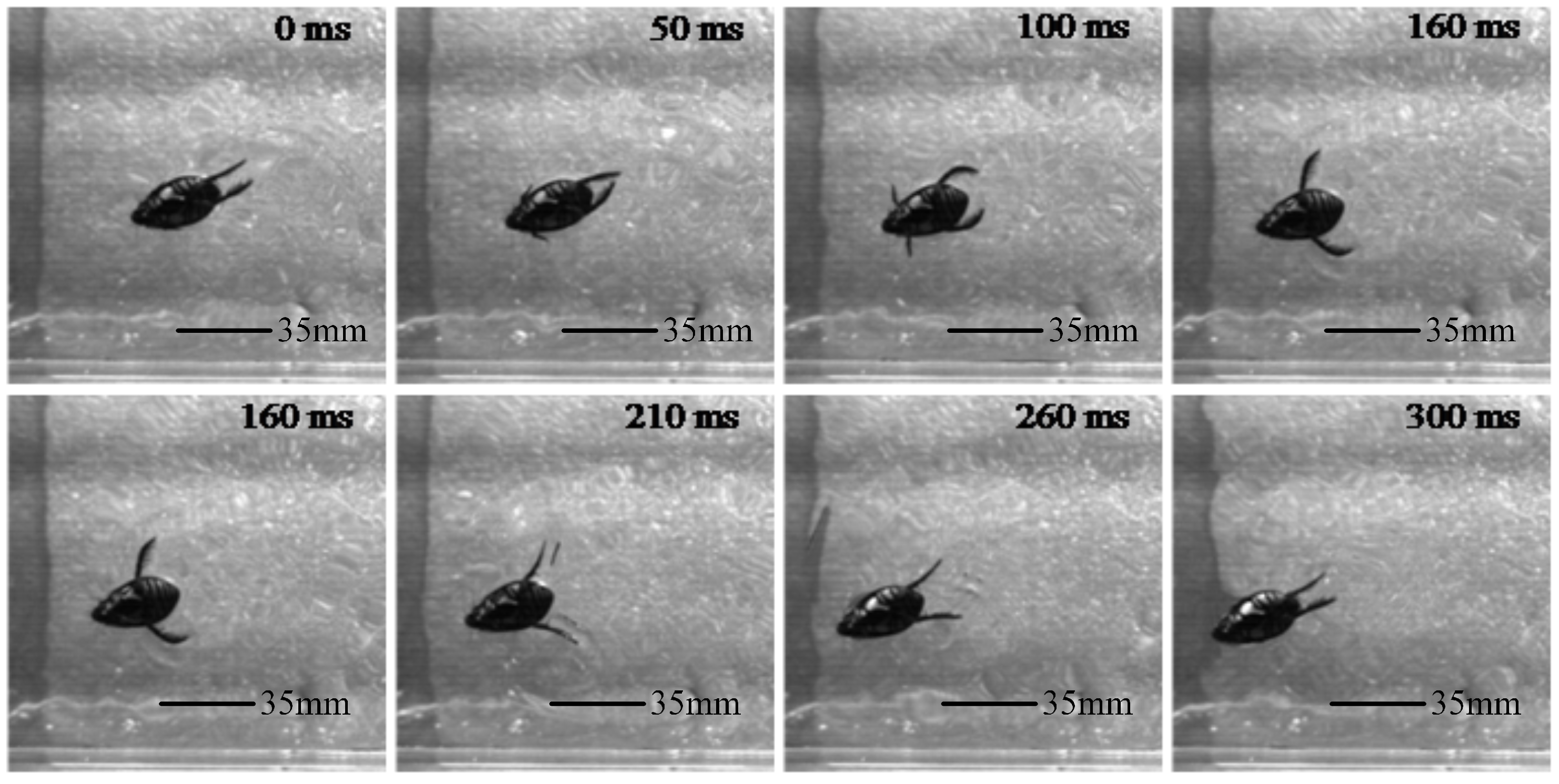
2.1. Diving Beetle Motion Characterization
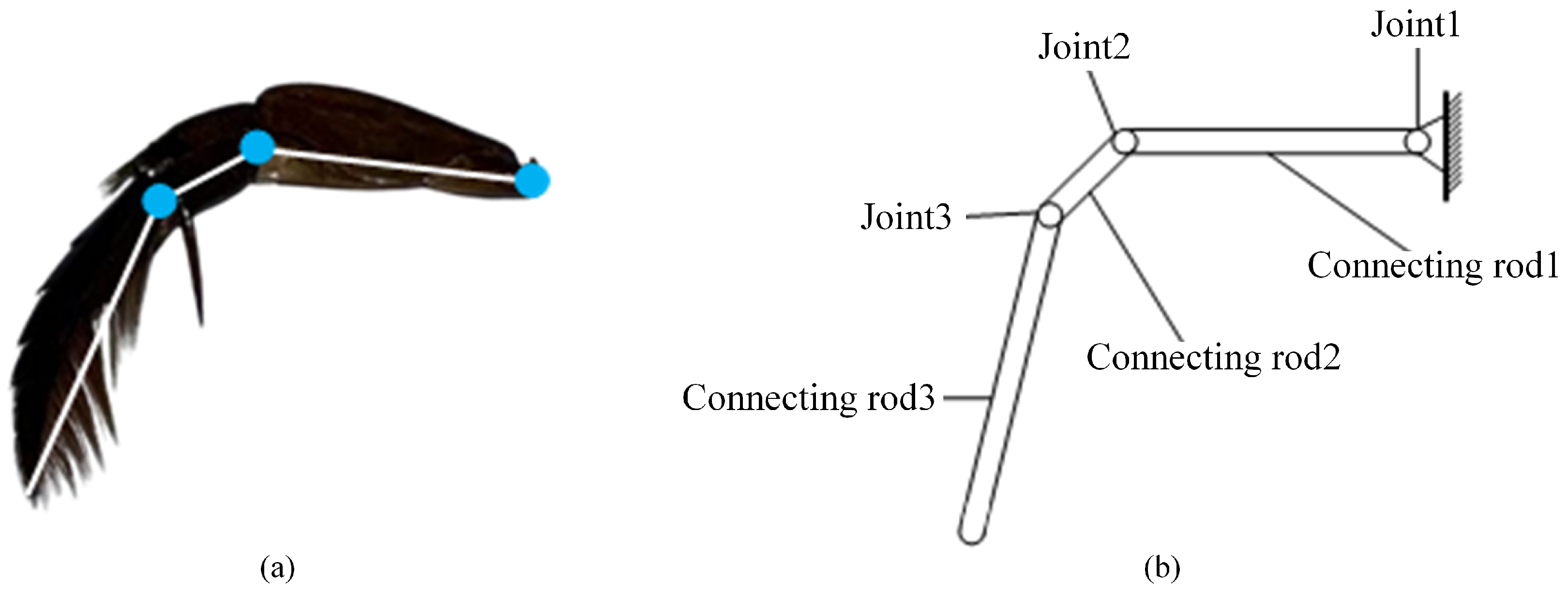
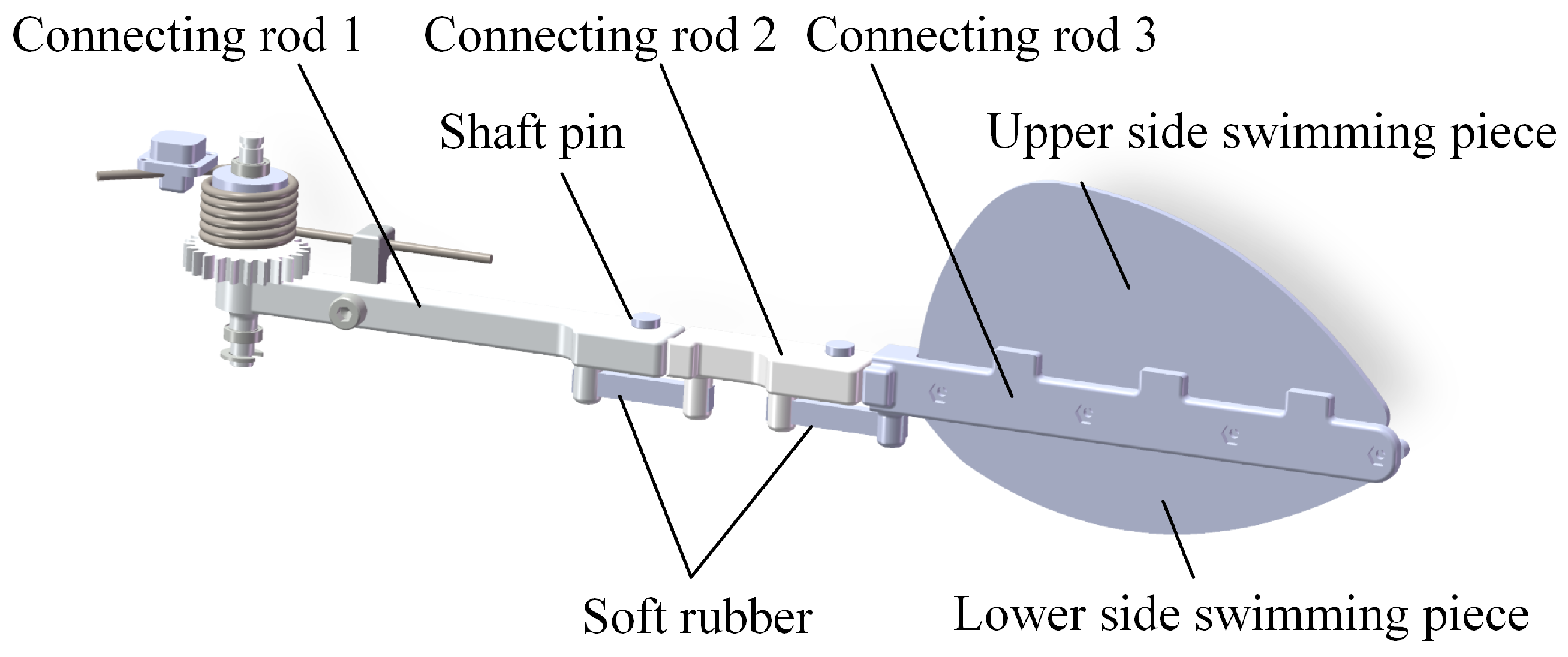
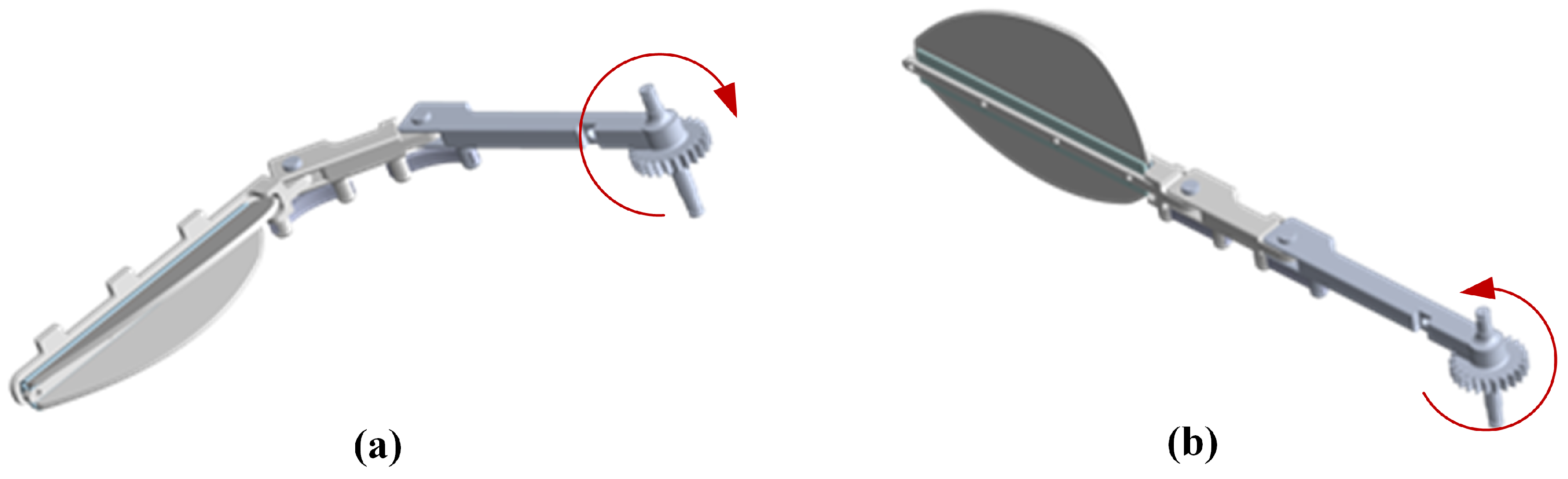
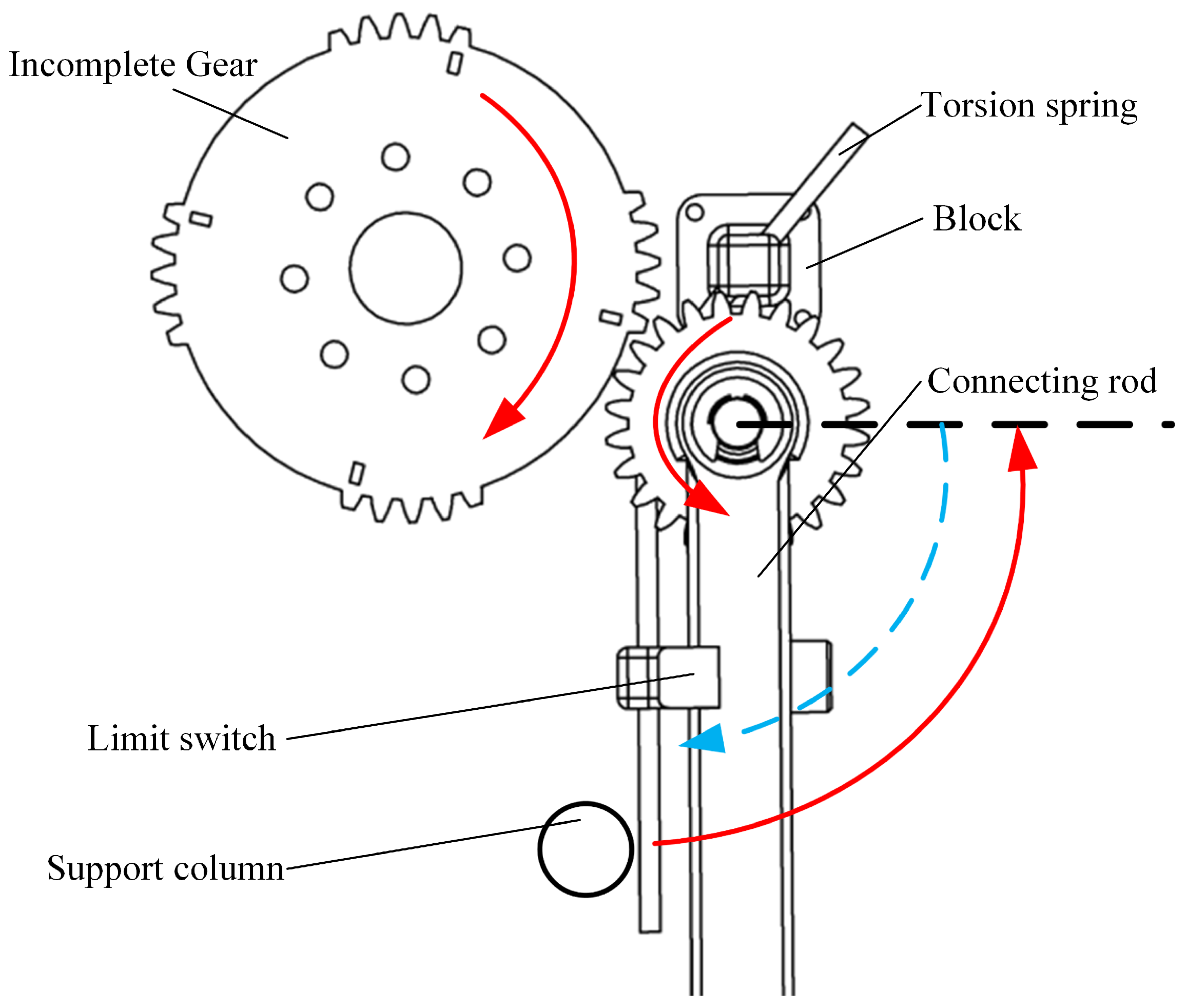
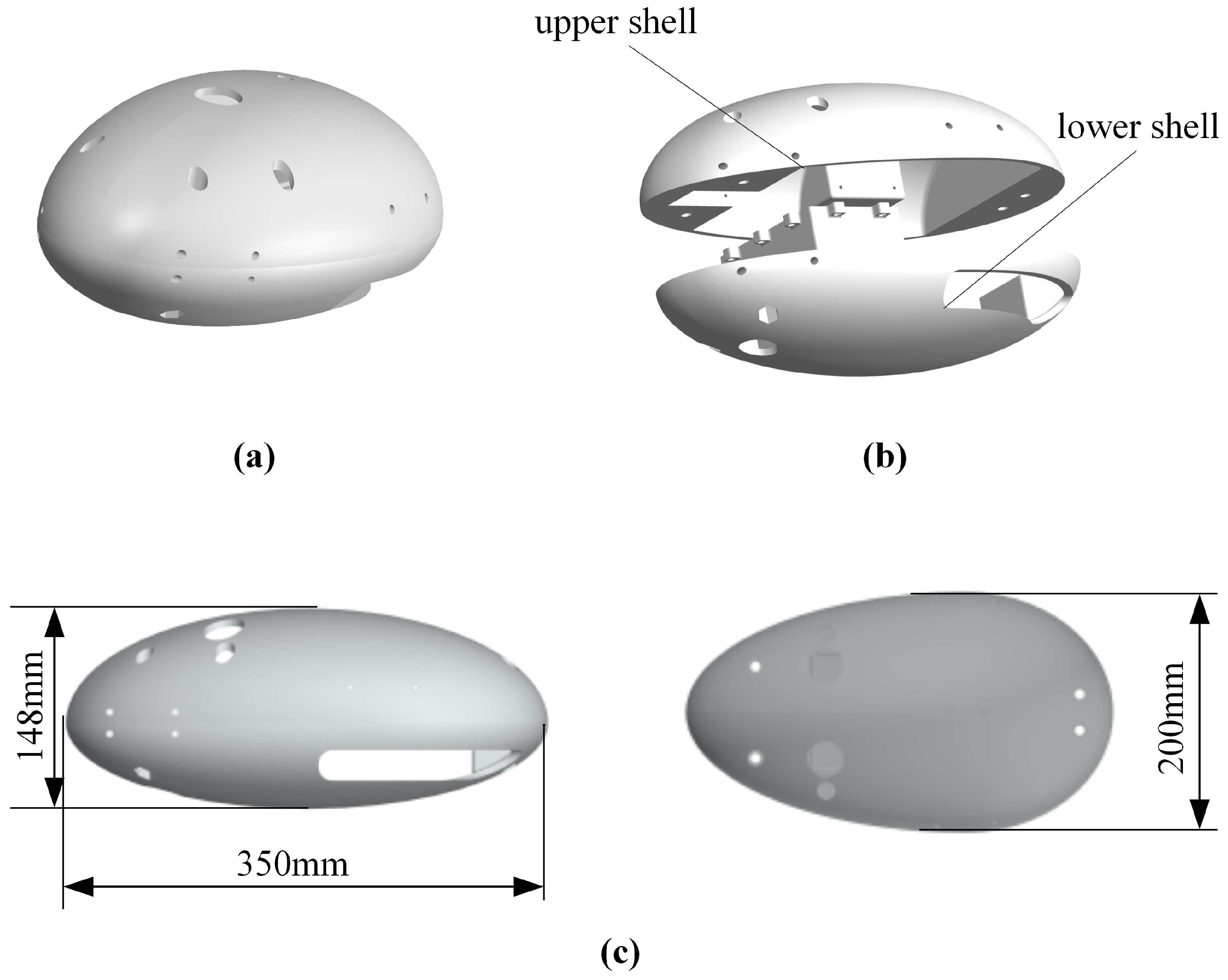
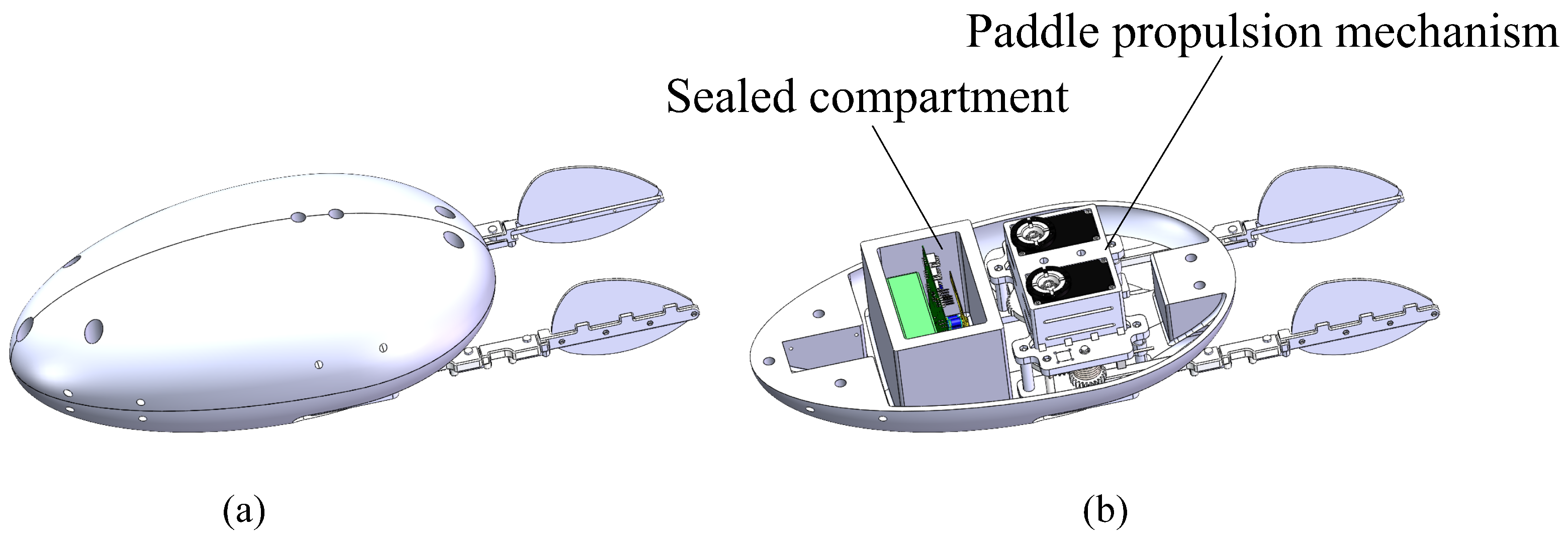
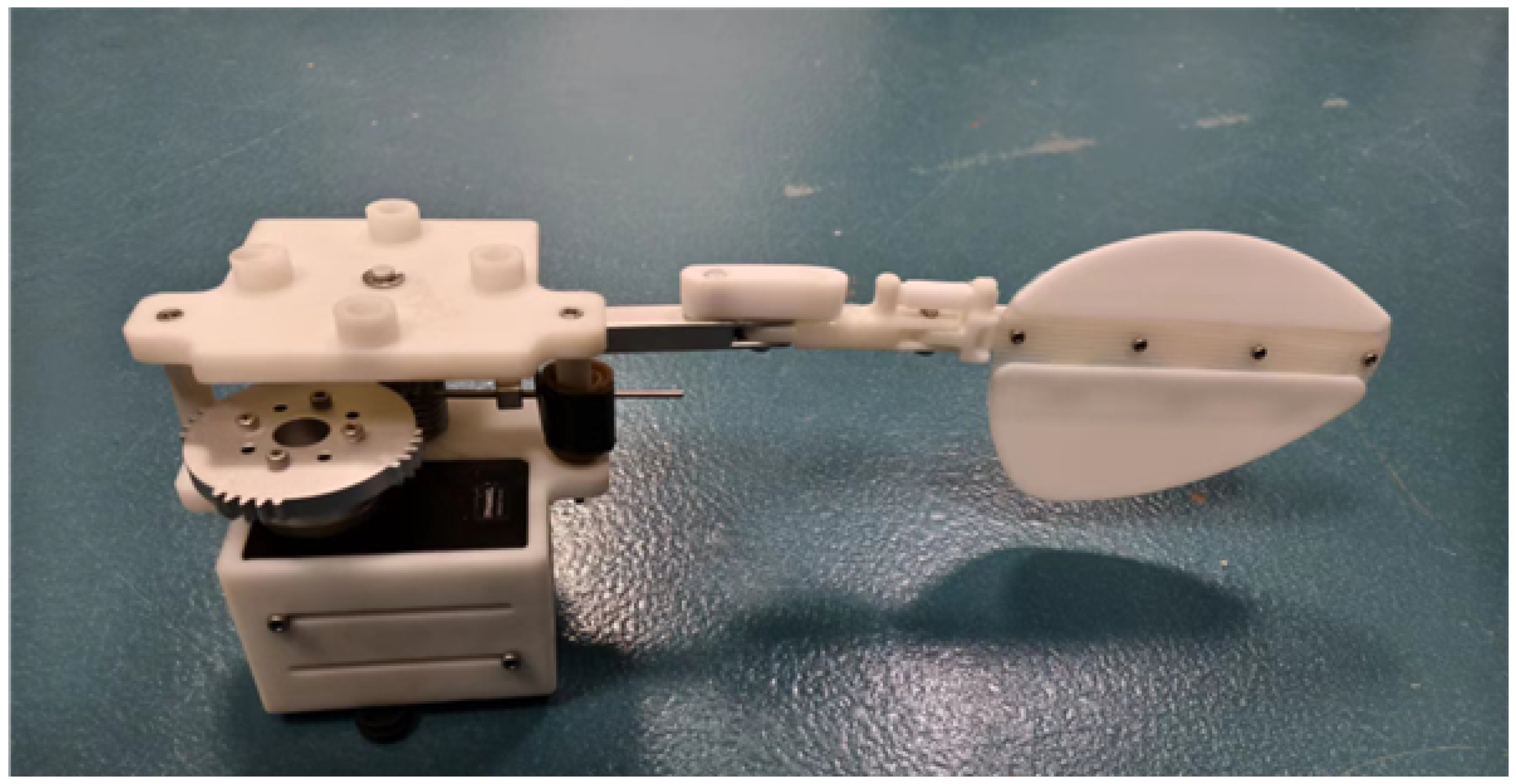
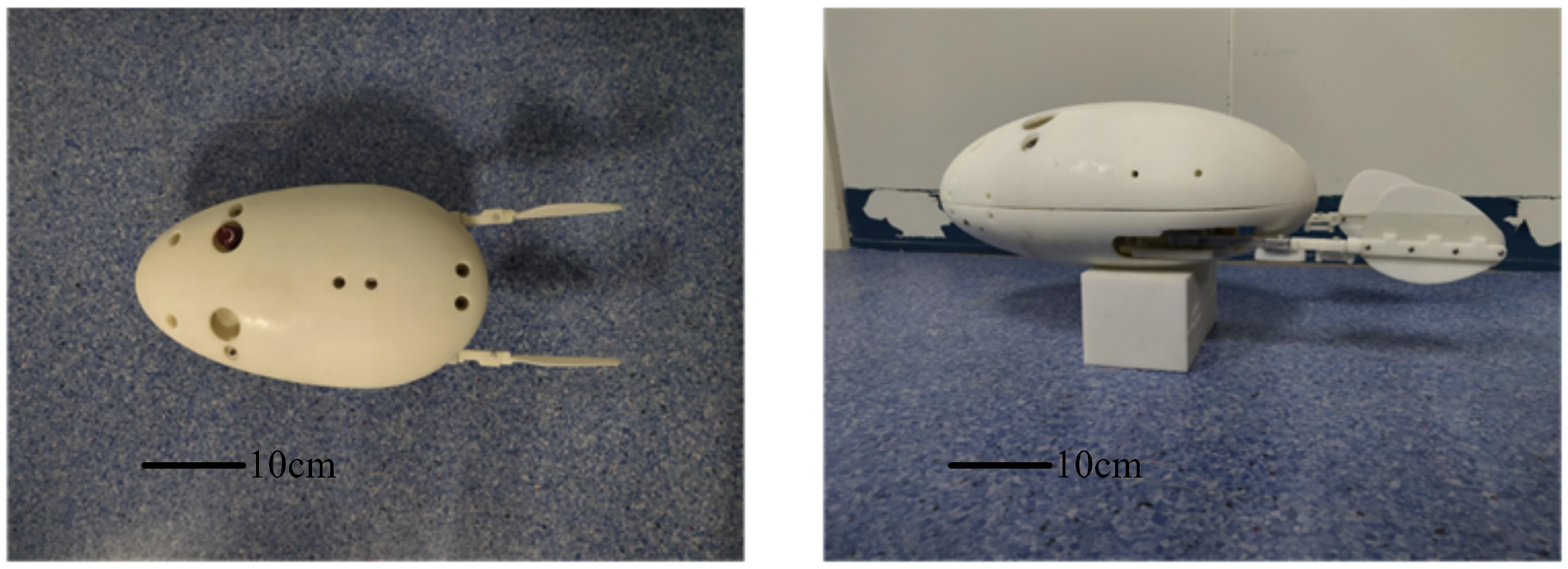
2.2. The Structural Design of a Bionic Paddling Robot
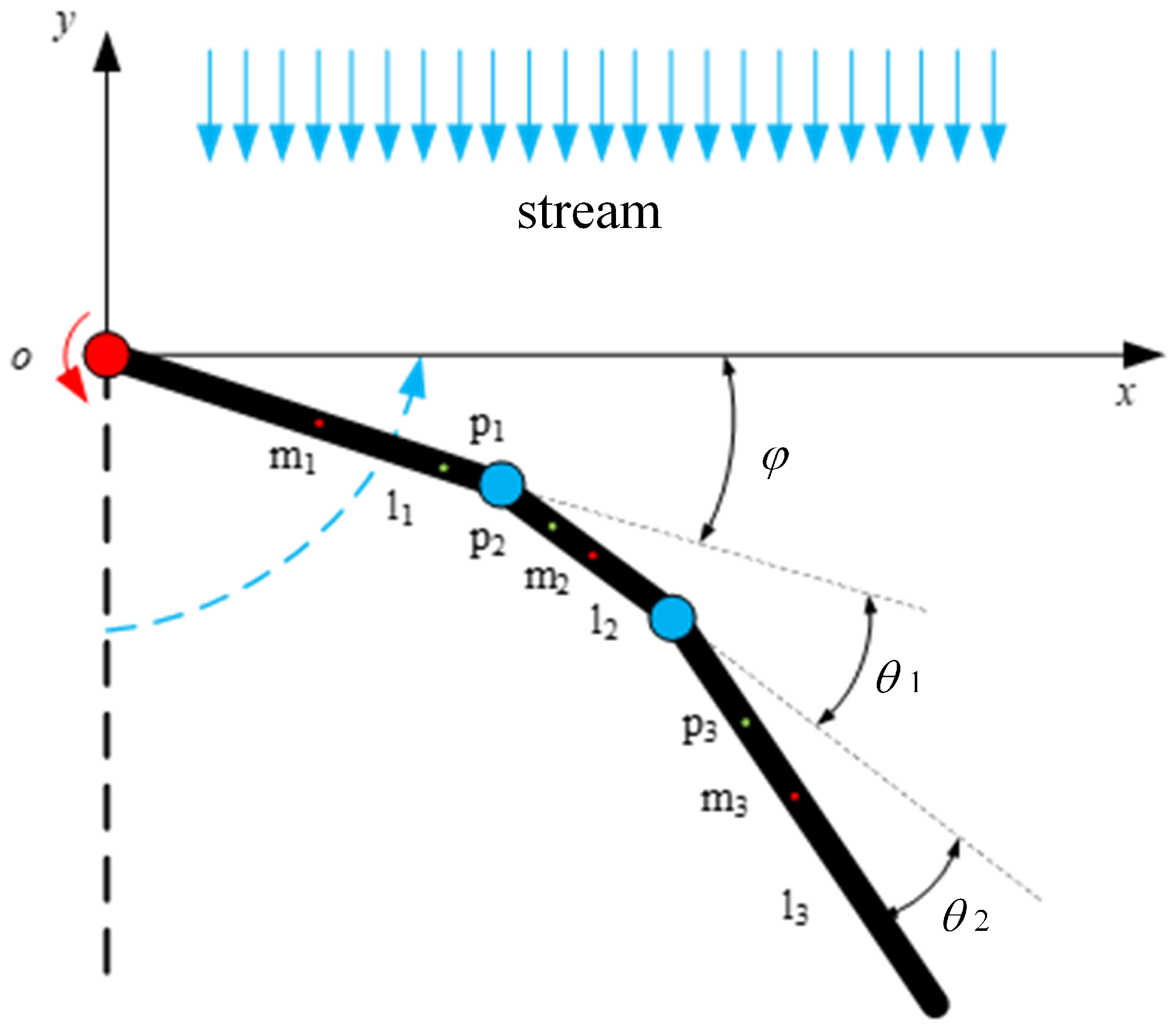
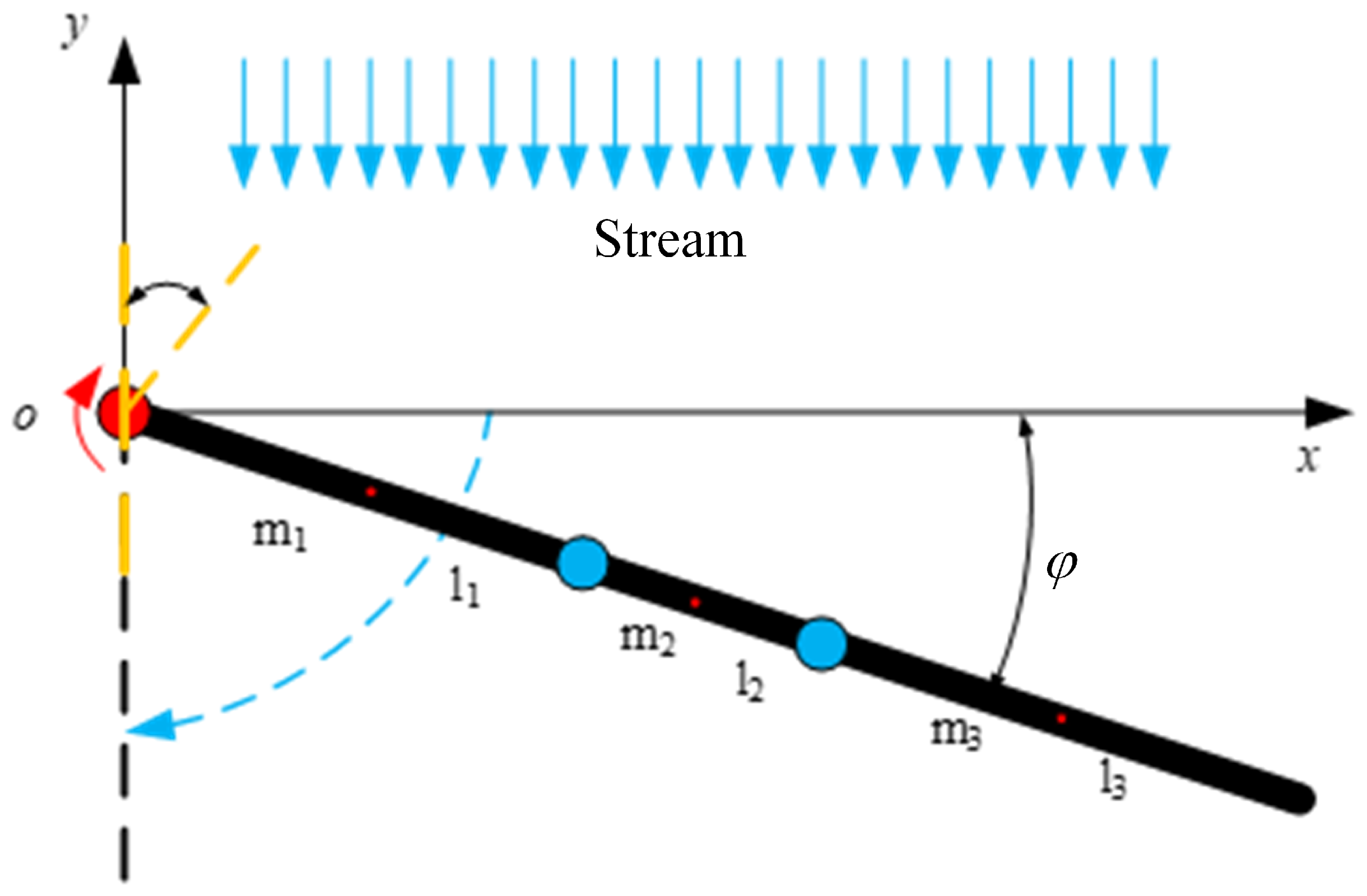
3. Dynamic Modeling
3.1. Return Phase
3.2. Stroke Phase
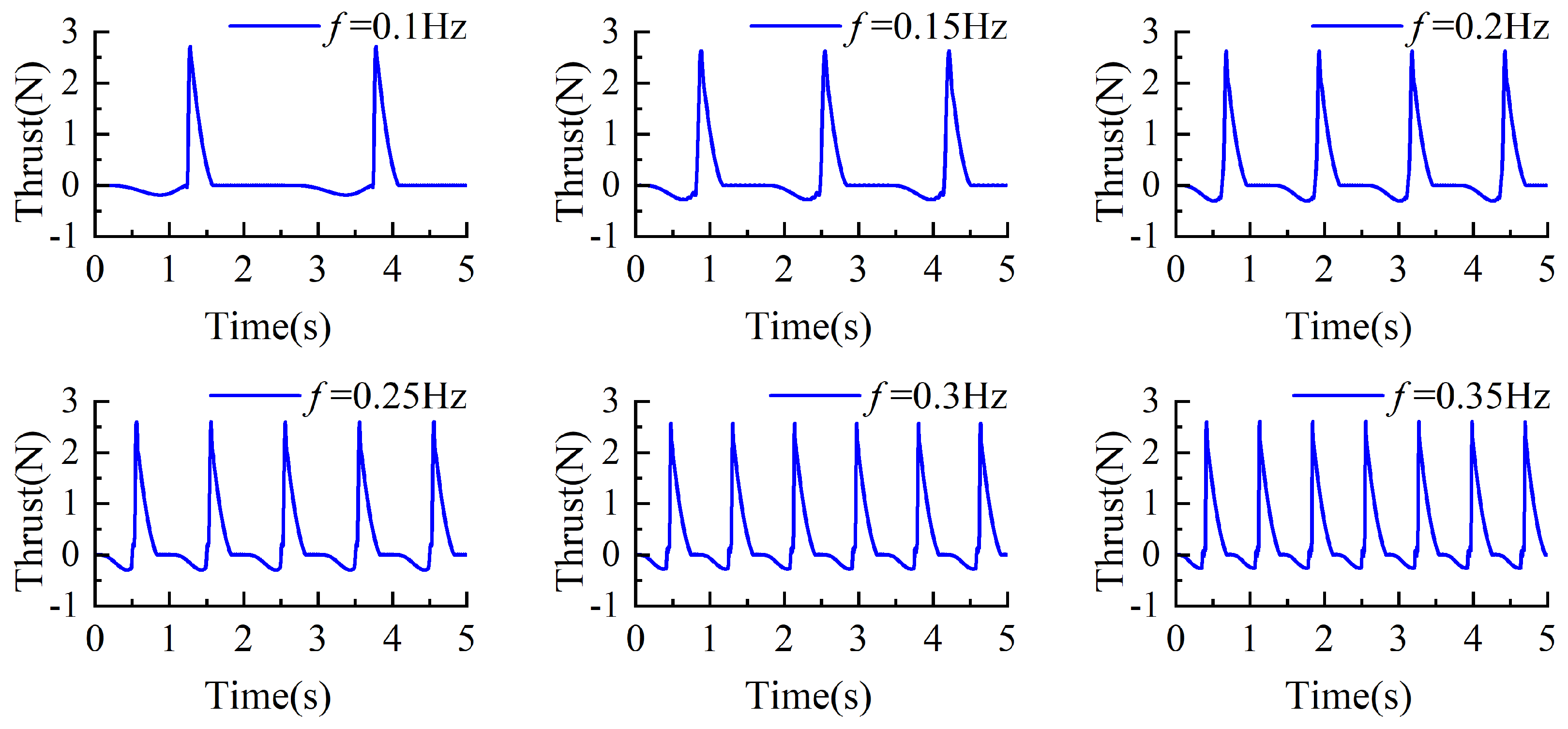
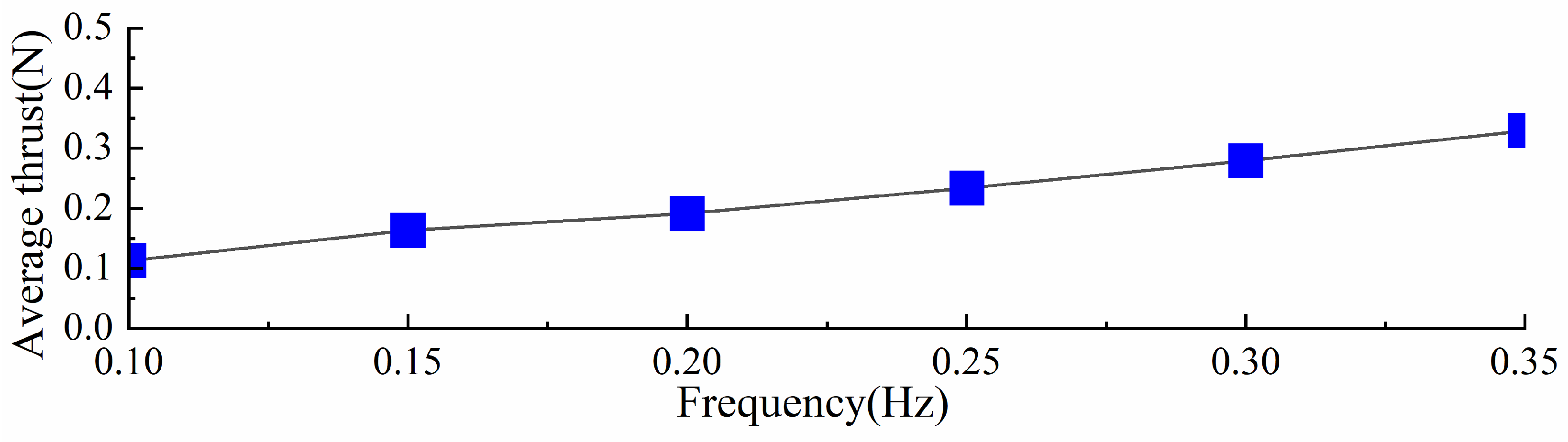
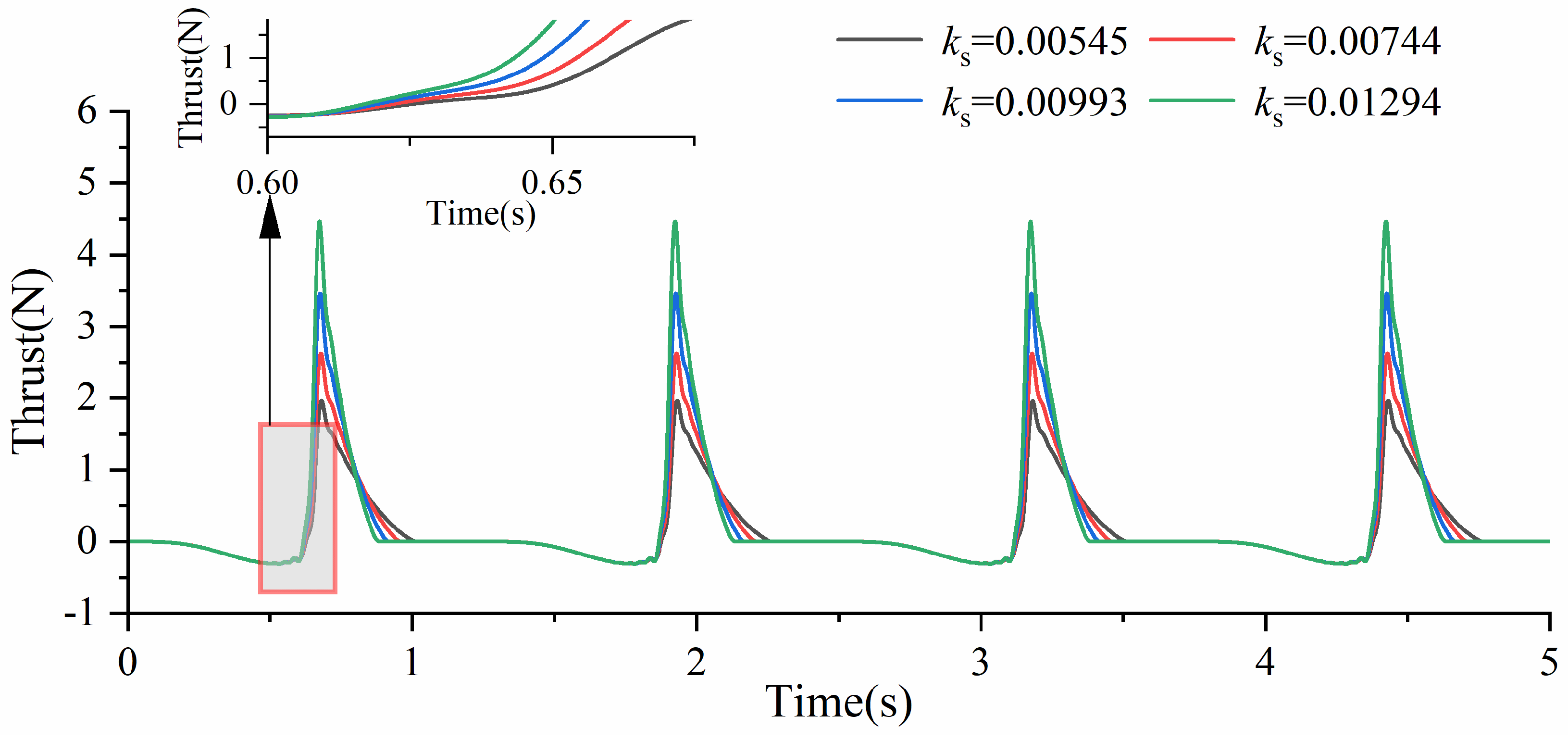
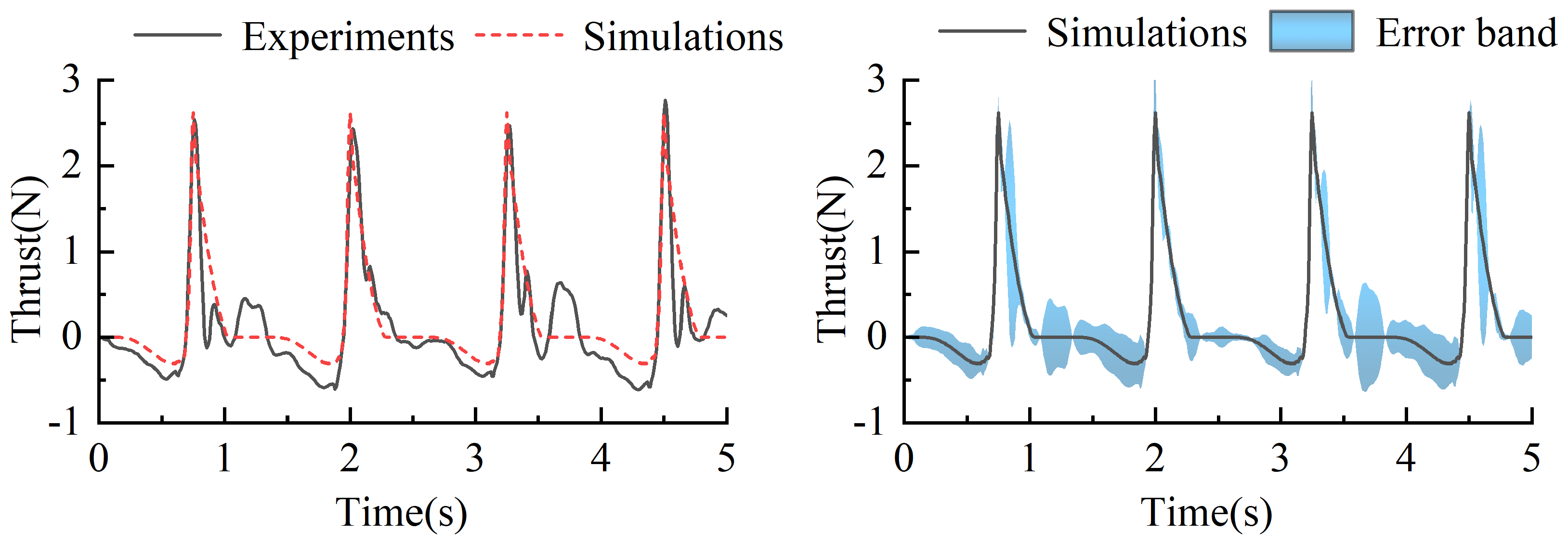
3.3. Simulation Data Analysis
4. Experiments and Results
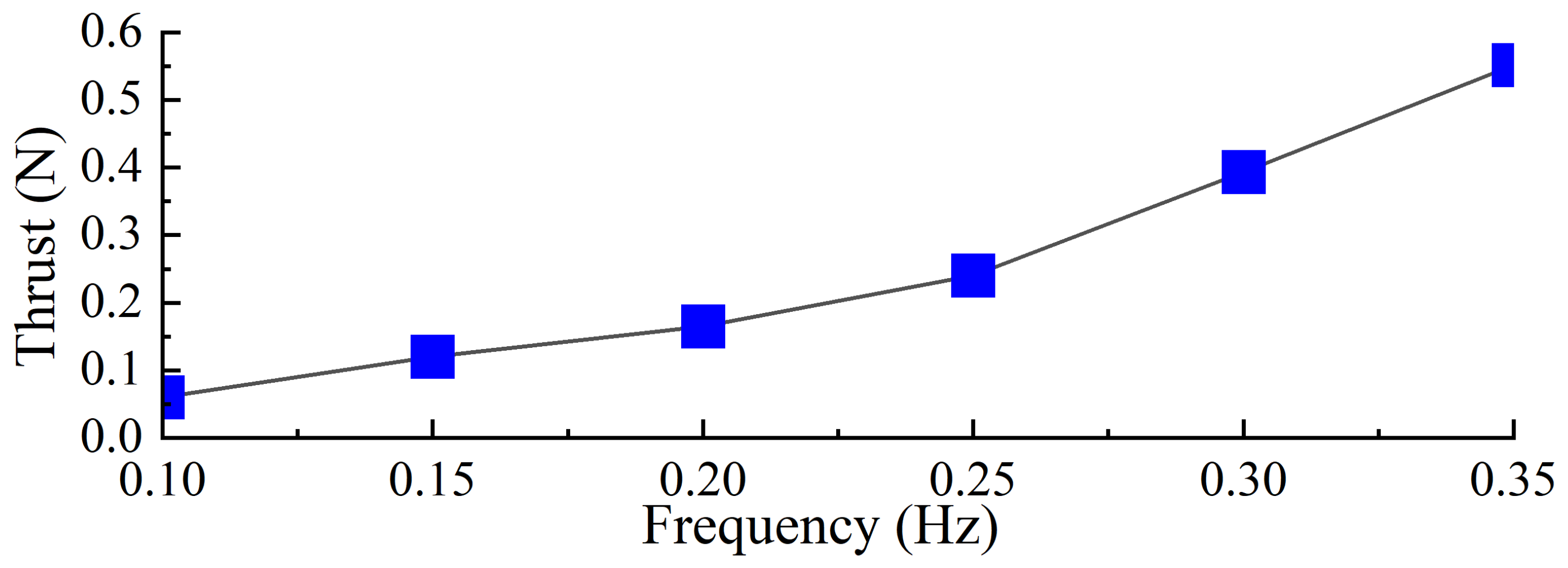
4.1. Trials and Analysis of Unilateral Paddling Propulsion Mechanism Thrust Test
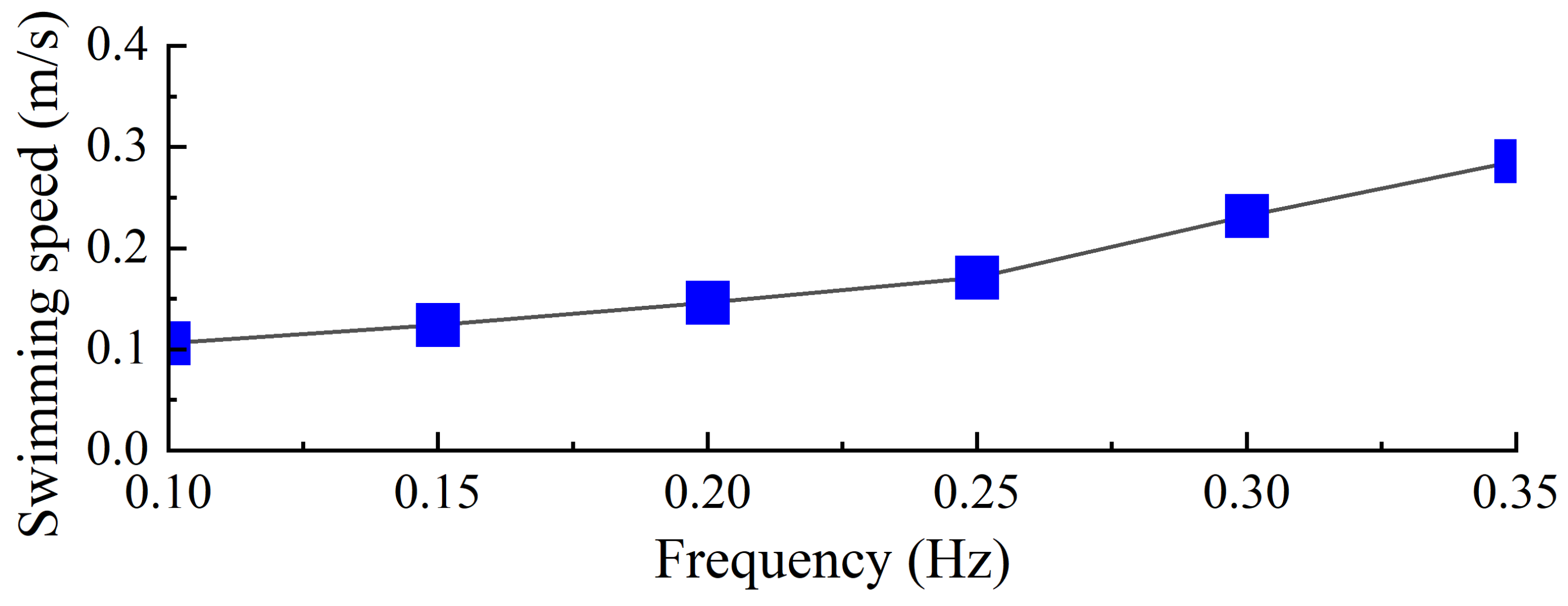
4.2. Swimming Test and Analysis of Paddling Propulsion Robots
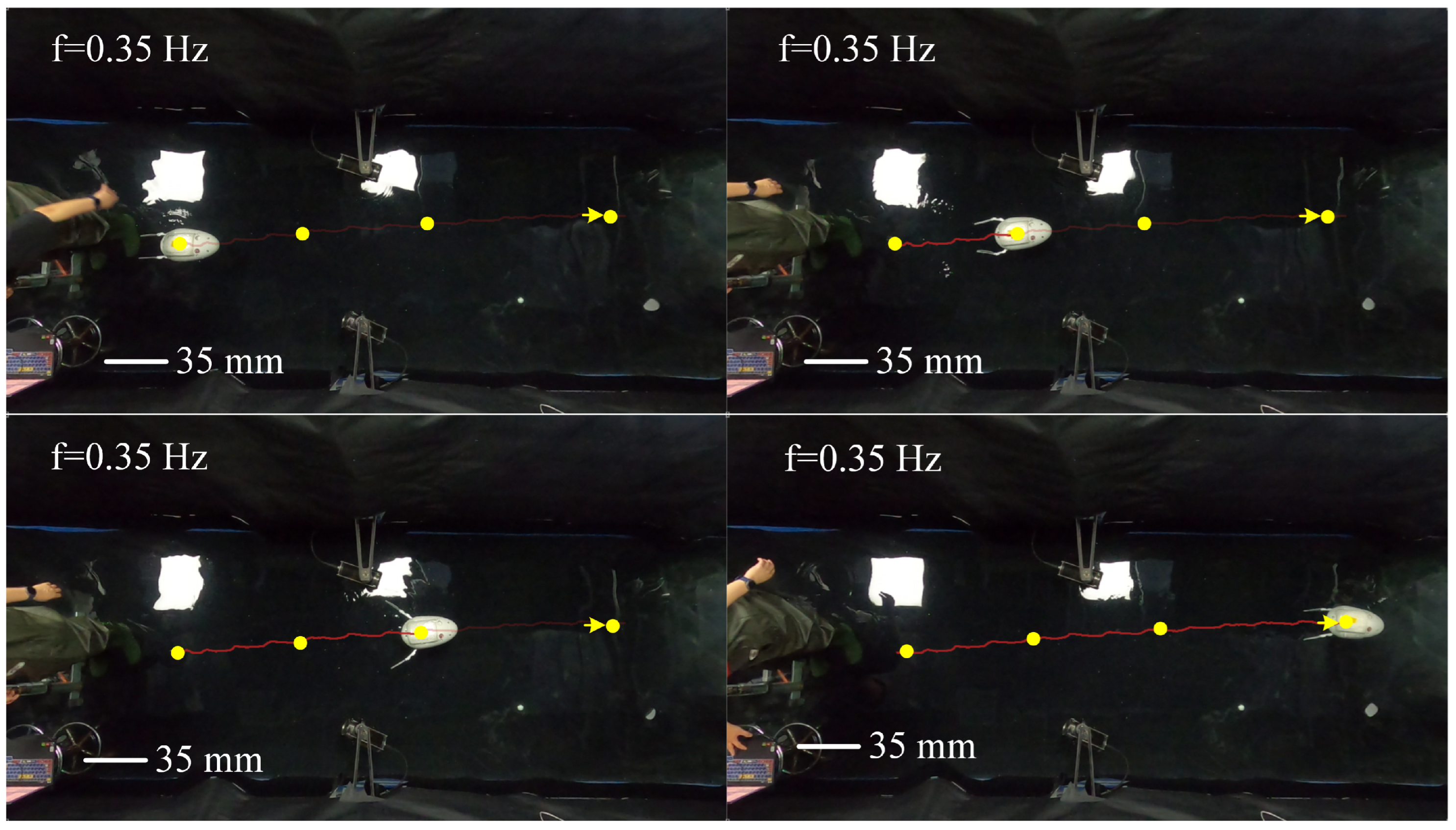
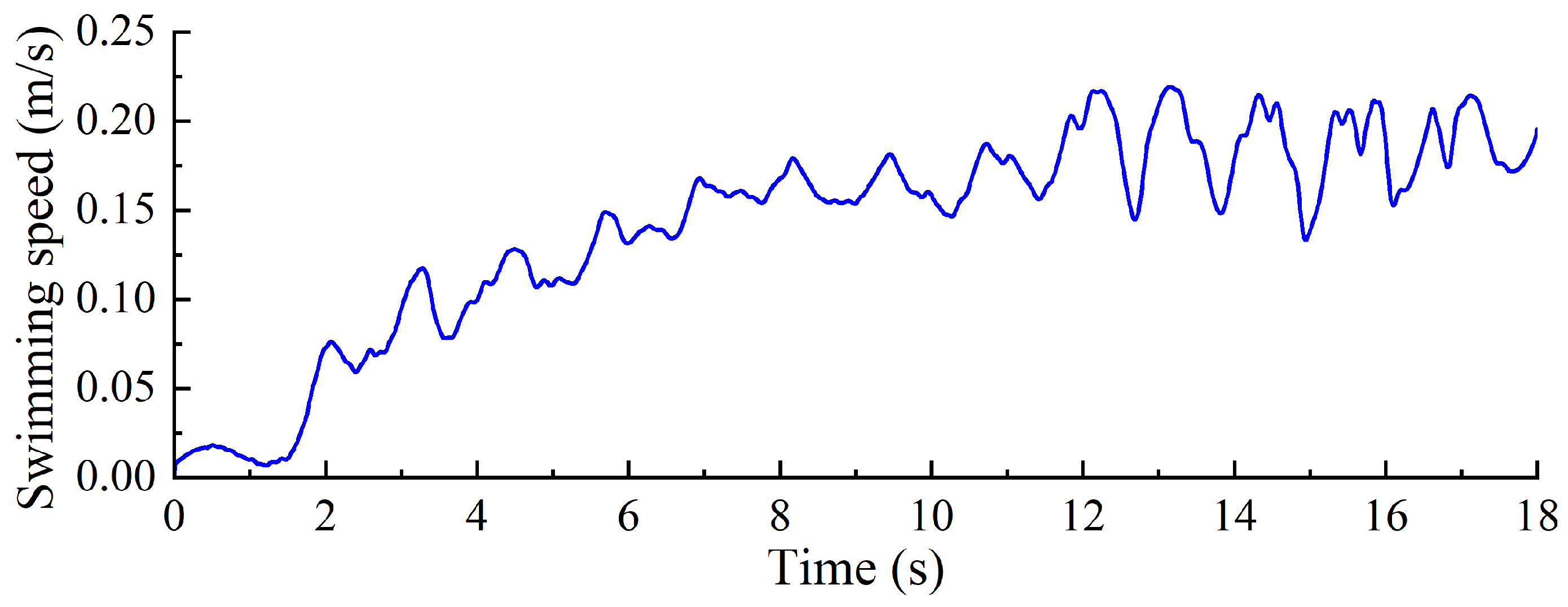
4.2.1. Forward Swimming Experiment
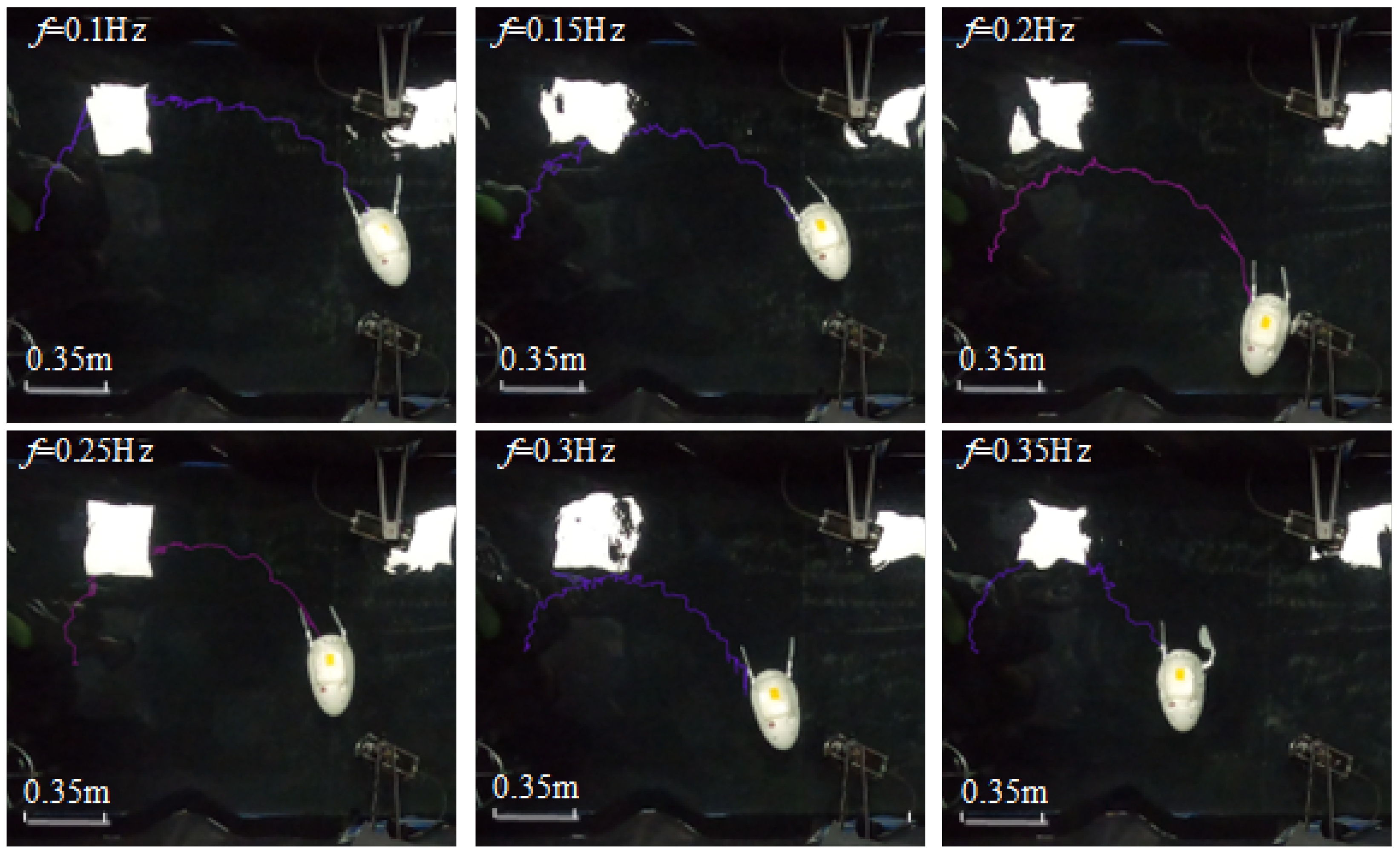
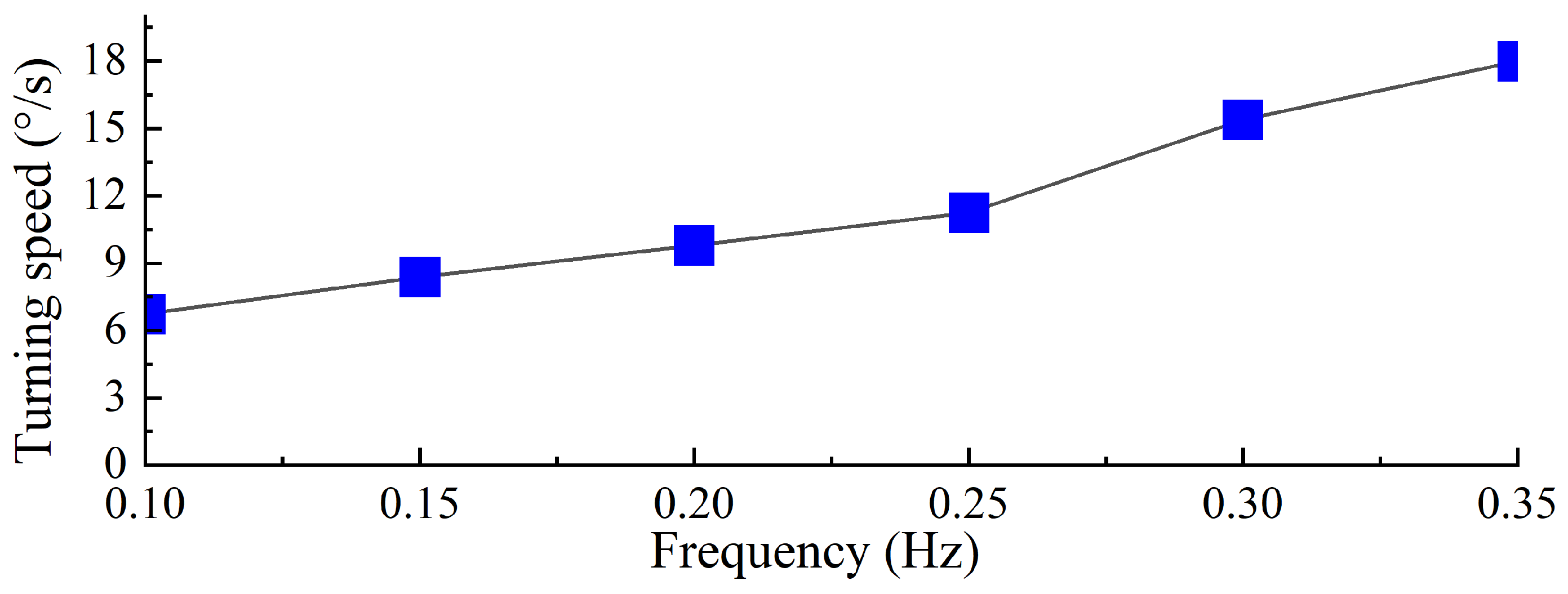
4.2.2. Turning Swimming Experiment
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Shao, H.; Dong, B.; Zheng, C.; Li, T.; Zuo, Q.; Xu, Y.; Fang, H.; He, K.; Xie, F. Thrust Improvement of a Biomimetic Robotic Fish by Using a Deformable Caudal Fin. Biomimetics 2022, 7, 113. [Google Scholar] [CrossRef] [PubMed]
- Zheng, C.; Ding, J.; Dong, B.; Lian, G.; He, K.; Xie, F. How Non-Uniform Stiffness Affects the Propulsion Performance of a Biomimetic Robotic Fish. Biomimetics 2022, 7, 187. [Google Scholar] [CrossRef]
- Chen, G.; Xu, Y.; Yang, C.; Yang, X.; Hu, H.; Chai, X.; Wang, D. Design and Control of a Novel Bionic Mantis Shrimp Robot. IEEE/ASME Trans. Mechatron. 2023, 28, 3376–3385. [Google Scholar] [CrossRef]
- Costa, D.; Palmieri, G.; Palpacelli, M.C.; Scaradozzi, D.; Callegari, M. Design of a Carangiform Swimming Robot through a Multiphysics Simulation Environment. Biomimetics 2020, 5, 46. [Google Scholar] [CrossRef] [PubMed]
- Aragaki, D.; Nishimura, T.; Sato, R.; Ming, A. Biomimetic Soft Underwater Robot Inspired by the Red Muscle and Tendon Structure of Fish. Biomimetics 2023, 8, 133. [Google Scholar] [CrossRef] [PubMed]
- Bianchi, G.; Lanzetti, L.; Mariana, D.; Cinquemani, S. Bioinspired Design and Experimental Validation of an Aquatic Snake Robot. Biomimetics 2024, 9, 87. [Google Scholar] [CrossRef]
- Xing, J.; Jin, W.; Yang, K.; Howard, I. A Bionic Piezoelectric Robotic Jellyfish With a Large Deformation Flexure Hinge. IEEE Trans. Ind. Electron. 2023, 70, 12596–12605. [Google Scholar] [CrossRef]
- Yu, Z.; Li, K.; Ji, Y.; Yang, S.X. Fast Motion Performance of a Bionic Ray Robot With Serial Pectoral Fins. IEEE Robot. Autom. Lett. 2023, 8, 7218–7225. [Google Scholar] [CrossRef]
- Kim, T.; Kim, J.; Yu, S.C. Development of Bioinspired Multimodal Underwater Robot “HERO-BLUE” for Walking, Swimming, and Crawling. IEEE Trans. Robot. 2024, 40, 1421–1438. [Google Scholar] [CrossRef]
- Nauwelaerts, S.; Ramsay, J.; Aerts, P. Morphological correlates of aquatic and terrestrial locomotion in a semi-aquatic frog, Rana esculenta: No evidence for a design conflict. J. Anat. 2007, 210, 304–317. [Google Scholar] [CrossRef]
- Wang, M.; Zang, X.z.; Fan, J.z.; Zhao, J. Biological Jumping Mechanism Analysis and Modeling for Frog Robot. J. Bionic Eng. 2008, 5, 181–188. [Google Scholar] [CrossRef]
- Tang, Y.; Qin, L.; Li, X.; Chew, C.M.; Zhu, J. A frog-inspired swimming robot based on dielectric elastomer actuators. In Proceedings of the 2017 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Vancouver, BC, Canada, 24–28 September 2017; IEEE: Piscataway, NJ, USA, 2017; pp. 2403–2408. [Google Scholar] [CrossRef]
- Fan, J.; Wang, S.; Yu, Q.; Zhu, Y. Swimming Performance of the Frog-Inspired Soft Robot. Soft Robot. 2020, 7, 615–626. [Google Scholar] [CrossRef] [PubMed]
- Chen, G.; Ti, X.; Shi, L.; Hu, H. Design of Beaver-like Hind Limb and Analysis of Two Swimming Gaits for Underwater Narrow Space Exploration. J. Intell. Robot. Syst. 2022, 104, 65. [Google Scholar] [CrossRef]
- Qi, D.; Zhang, C.; Wu, Z.; Shen, C.; Yue, Y.; Ren, L.; Yang, L. Dynamics and hydrodynamic efficiency of diving beetle while swimming. Heliyon 2023, 9, e14200. [Google Scholar] [CrossRef]
- Glaeser, G.; Nachtigall, W. Signaling, Swimming, Flying, Exploding. In The Evolution and Function of Biological Macrostructures; Springer: Berlin/Heidelberg, Germany, 2019; pp. 82–109. [Google Scholar] [CrossRef]
- Sudo, S.; Yano, T.; Kan, Y.; Yamada, Y.; Tsuyuki, K. Swimming behavior of small diving beetles. J. Adv. Sci. 2006, 18, 46–49. [Google Scholar] [CrossRef]
- Kwak, B.; Bae, J. Toward Fast and efficient mobility in aquatic environment: A robot with compliant swimming appendages inspired by a water beetle. J. Bionic Eng. 2017, 14, 260–271. [Google Scholar] [CrossRef]
- Kwak, B.; Lee, D.; Bae, J. Efficient Drag-based Swimming using Articulated Legs with Micro Hair Arrays Inspired by a Water Beetle. In Proceedings of the 2019 2nd IEEE International Conference on Soft Robotics (RoboSoft), Seoul, Republic of Korea, 14–18 April 2019; pp. 795–800. [Google Scholar] [CrossRef]
- Yu, J.; Wang, M.; Dong, H.; Zhang, Y.; Wu, Z. Motion Control and Motion Coordination of Bionic Robotic Fish: A Review. J. Bionic Eng. 2018, 15, 579–598. [Google Scholar] [CrossRef]
- Bergsten, J.; Miller, K.B. Diving Beetles of the World: Systematics and Biology of the Dytiscidae; Johns Hopkins University Press: Baltimore, MA, USA, 2016. [Google Scholar]
- Xu, Z.; Lenaghan, S.C.; Reese, B.E.; Jia, X.; Zhang, M. Experimental Studies and Dynamics Modeling Analysis of the Swimming and Diving of Whirligig Beetles (Coleoptera: Gyrinidae). PLoS Comput. Biol. 2012, 8, e1002792. [Google Scholar] [CrossRef]
- Macías, M.M.; García-Ortiz, J.H.; Oliveira, T.F.; Brasil Junior, A.C.P. Numerical Investigation of Dimensionless Parameters in Carangiform Fish Swimming Hydrodynamics. Biomimetics 2024, 9, 45. [Google Scholar] [CrossRef]
- Xia, M.; Zhu, Q.; Yin, Q.; Lu, Z.; Zhu, Y.; Luo, Z. Hydrodynamic Simulation and Experiment of a Self-Adaptive Amphibious Robot Driven by Tracks and Bionic Fins. Biomimetics 2024, 9, 580. [Google Scholar] [CrossRef]
- Zhong, Y.; Wang, Q.; Yang, J.; Wang, C. Design, Modeling, and Experiment of Underactuated Flexible Gliding Robotic Fish. IEEE/ASME Trans. Mechatron. 2024, 29, 2266–2276. [Google Scholar] [CrossRef]
- Triantafyllou, M.S.; Triantafyllou, G.S.; Yue, D.K.P. Hydrodynamics of Fishlike Swimming. Annu. Rev. Fluid Mech. 2000, 32, 33–53. [Google Scholar] [CrossRef]
- Gong, Y.; Wang, M.; Zhao, Q.; Wang, R.; Zuo, L.; Zheng, X.; Gao, H. Investigating the Influence of Counterflow Regions on the Hydrodynamic Performance of Biomimetic Robotic Fish. Biomimetics 2024, 9, 452. [Google Scholar] [CrossRef] [PubMed]
- Hong, Z.; Wu, Z.; Wang, Q.; Li, J.; Zhong, Y. A Novel Variable-Stiffness Tail Based on Layer-Jamming for Robotic Fish. Adv. Intell. Syst. 2024, 6, 2400189. [Google Scholar] [CrossRef]
- Zhong, Y.; Li, Z.; Du, R. A Novel Robot Fish With Wire-Driven Active Body and Compliant Tail. IEEE/ASME Trans. Mechatron. 2017, 22, 1633–1643. [Google Scholar] [CrossRef]
- Bonomo, C.; Fortuna, L.; Giannone, P.; Graziani, S.; Strazzeri, S. A resonant force sensor based on ionic polymer metal composites. Smart Mater. Struct. 2007, 17, 015014. [Google Scholar] [CrossRef]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ding, J.; Li, J.; Lan, T.; He, K.; Zuo, Q. The Design, Modeling, and Experiment of a Novel Diving-Beetle-Inspired Paddling Propulsion Robot. Biomimetics 2025, 10, 182. https://doi.org/10.3390/biomimetics10030182
Ding J, Li J, Lan T, He K, Zuo Q. The Design, Modeling, and Experiment of a Novel Diving-Beetle-Inspired Paddling Propulsion Robot. Biomimetics. 2025; 10(3):182. https://doi.org/10.3390/biomimetics10030182
Chicago/Turabian StyleDing, Jiang, Jingyu Li, Tianbo Lan, Kai He, and Qiyang Zuo. 2025. "The Design, Modeling, and Experiment of a Novel Diving-Beetle-Inspired Paddling Propulsion Robot" Biomimetics 10, no. 3: 182. https://doi.org/10.3390/biomimetics10030182
APA StyleDing, J., Li, J., Lan, T., He, K., & Zuo, Q. (2025). The Design, Modeling, and Experiment of a Novel Diving-Beetle-Inspired Paddling Propulsion Robot. Biomimetics, 10(3), 182. https://doi.org/10.3390/biomimetics10030182






