Phase Retardation Analysis in a Rotated Plane-Parallel Plate for Phase-Shifting Digital Holography
Abstract
:1. Introduction
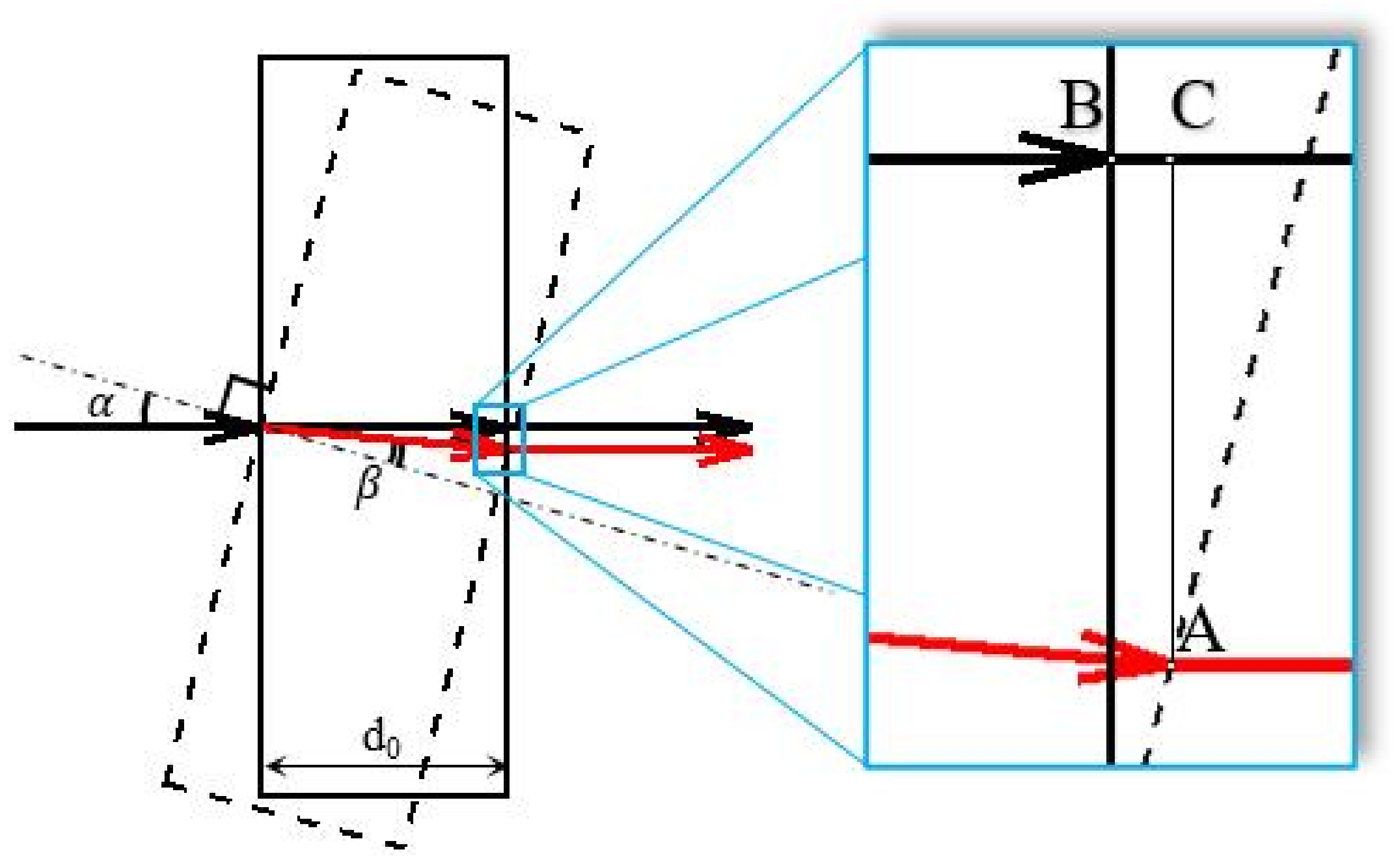
2. Phase Retardation in a Rotated Plane-Parallel Plate
3. Phase Retardation Analysis
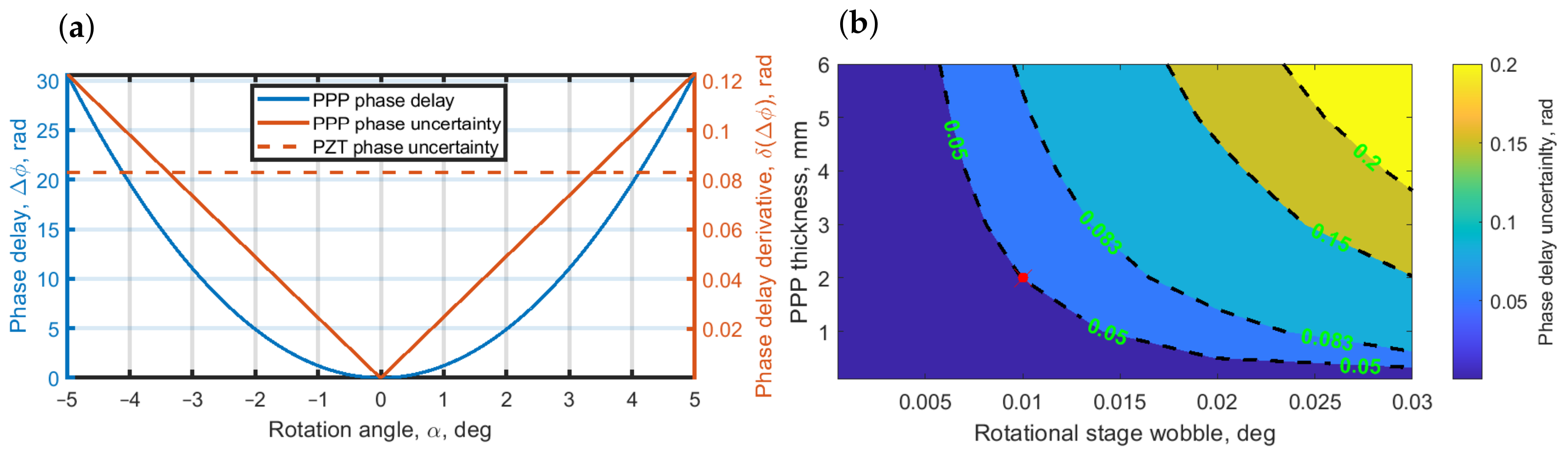
4. Uncertainty Estimation
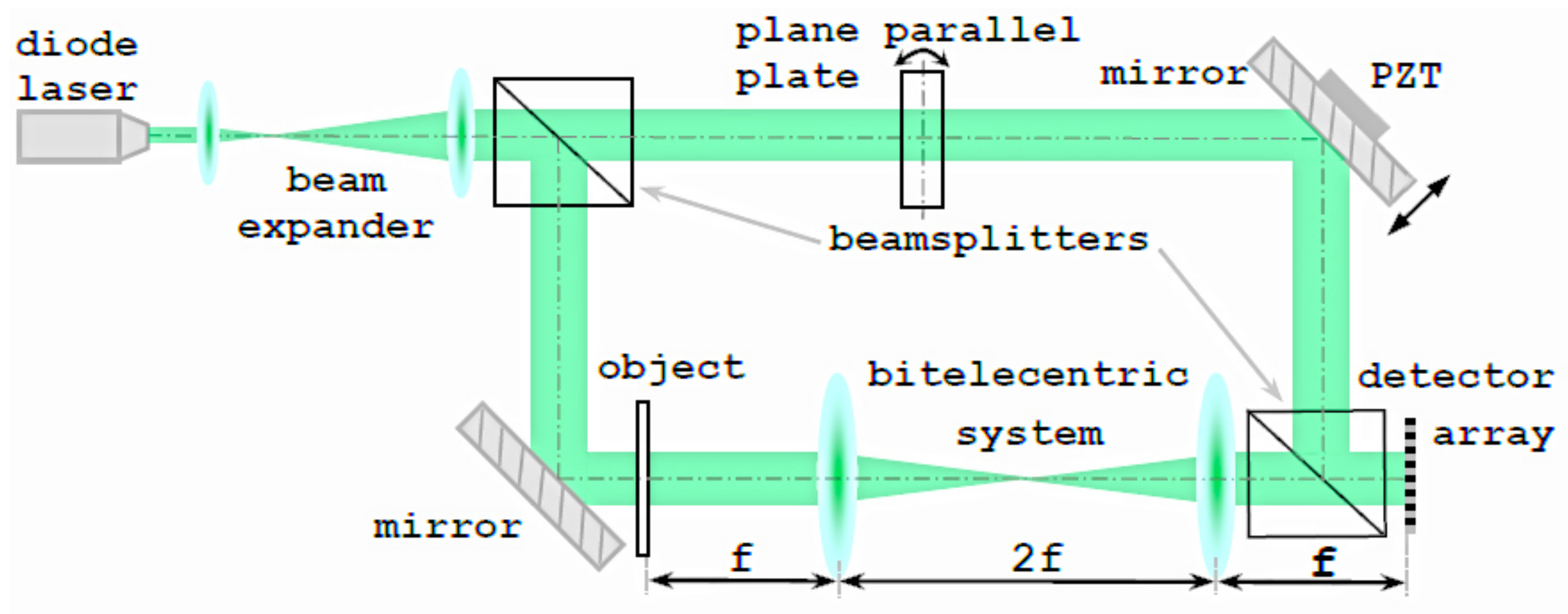
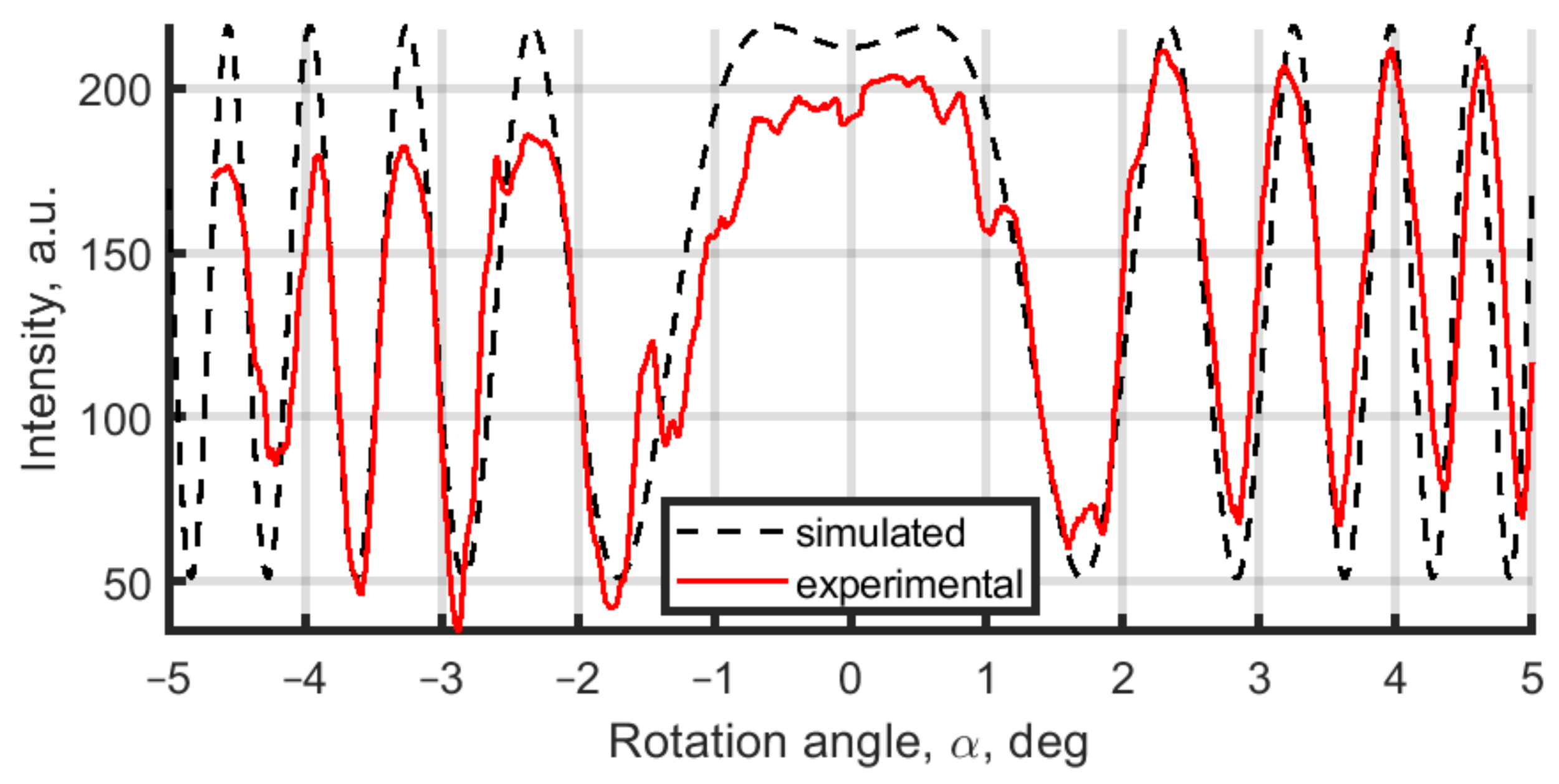
5. Experimental Verification
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| PSDH | Phase-shifting digital holography |
| PPP | Plane-parallel plate |
| PZT | Piezoelectric translator |
| MTS | Motorized translation stage |
| SLM | Spatial light modulator |
References
- Bruning, J.H.; Herriott, D.R.; Gallagher, J.E.; Rosenfeld, D.P.; White, A.D.; Brangaccio, D.J. Digital Wavefront Measuring Interferometer for Testing Optical Surfaces and Lenses. Appl. Opt. 1974, 13, 2693–2703. [Google Scholar] [CrossRef] [PubMed]
- Boyle, W.S.; Smith, G.E. Charge coupled semiconductor devices. Bell Syst. Tech. J. 1970, 49, 587–593. [Google Scholar]
- Hariharan, P.; Oreb, B.F.; Eiju, T. Digital phase-shifting interferometry: A simple error-compensating phase calculation algorithm. Appl. Opt. 1987, 26, 2504. [Google Scholar] [CrossRef]
- Yamaguchi, I.; Zhang, T. Phase-shifting digital holography. Opt. Lett. 1997, 22, 1268. [Google Scholar] [CrossRef] [PubMed]
- De Groot, P.J.; Deck, L.L.; Su, R.; Osten, W. Contributions of holography to the advancement of interferometric measurements of surface topography. Light Adv. Manuf. 2022, 3, 1. [Google Scholar] [CrossRef]
- Khonina, S.; Khorin, P.G.; Serafimovich, P.G.; Dzyuba, A.P.; Georgieva, A.O.; Petrov, N.V. Analysis of the wavefront aberrations based on neural networks processing of the interferograms with a conical reference beam. Appl. Phys. B 2022, 128, 1–16. [Google Scholar] [CrossRef]
- Latychevskaia, T.; Formanek, P.; Koch, C.; Lubk, A. Off-axis and inline electron holography: Experimental comparison. Ultramicroscopy 2010, 110, 472–482. [Google Scholar] [CrossRef] [Green Version]
- Yamaguchi, I.; Zhang, T. Phase-shifting digital holography. In Digital Holography and Three-Dimensional Display; Springer Series in Optical Sciences; Springer: Boston, MA, USA, 1997; Volume 22, pp. 1268–1270. [Google Scholar] [CrossRef]
- Kim, M.K. Digital Holographic Microscopy; Springer Series in Optical Sciences; Springer: New York, NY, USA, 2011; Volume 162. [Google Scholar] [CrossRef] [Green Version]
- Guo, P.; Devaney, A.J. Digital microscopy using phase-shifting digital holography with two reference waves. Opt. Lett. 2004, 29, 857–859. [Google Scholar] [CrossRef]
- Yamaguchi, I.; Kato, J.; Ohta, S.; Mizuno, J. Image formation in phase-shifting digital holography and applications to microscopy. Appl. Opt. 2001, 40, 6177–6186. [Google Scholar]
- Huang, P.S.; Zhang, S. Fast three-step phase-shifting algorithm. Appl. Opt. 2006, 45, 5086–5091. [Google Scholar] [CrossRef]
- Takaki, Y.; Kawai, H.; Ohzu, H. Hybrid holographic microscopy free of conjugate and zero-order images. Appl. Opt. 1999, 38, 4990–4996. [Google Scholar] [CrossRef] [PubMed]
- Meng, X.F.; Cai, L.Z.; Xu, X.F.; Yang, X.L.; Shen, X.X.; Dong, G.Y.; Wang, Y.R. Two-step phase-shifting interferometry and its application in image encryption. Opt. Lett. 2006, 31, 1414–1416. [Google Scholar] [CrossRef] [PubMed]
- Wang, Z.; Han, B. Advanced iterative algorithm for phase extraction of randomly phase-shifted interferograms. Opt. Lett. 2004, 29, 1671–1673. [Google Scholar]
- Xu, X.F.; Cai, L.Z.; Wang, Y.R.; Meng, X.F.; Sun, W.J.; Zhang, H.; Cheng, X.C.; Dong, G.Y.; Shen, X.X. Simple direct extraction of unknown phase shift and wavefront reconstruction in generalized phase-shifting interferometry: Algorithm and experiments. Opt. Lett. 2008, 33, 776–778. [Google Scholar] [CrossRef] [PubMed]
- Xia, P.; Wang, Q.; Ri, S. Random phase-shifting digital holography based on a self-calibrated system. Opt. Express 2020, 28, 19988–19996. [Google Scholar] [CrossRef]
- Nomura, T.; Murata, S.; Nitanai, E.; Numata, T. Phase-shifting digital holography with a phase difference between orthogonal polarizations. Appl. Opt. 2006, 45, 4873–4877. [Google Scholar] [CrossRef]
- Xia, P.; Ri, S.; Inoue, T.; Awatsuji, Y.; Matoba, O. Dynamic phase measurement of a transparent object by parallel phase-shifting digital holography with dual polarization imaging cameras. Opt. Lasers Eng. 2021, 141, 106583. [Google Scholar]
- Lai, S.; King, B.; Neifeld, M.A. Wave front reconstruction by means of phase-shifting digital in-line holography. Opt. Commun. 2000, 173, 155–160. [Google Scholar]
- Griffin, D.W. Phase-shifting shearing interferometer. Opt. Lett. 2001, 26, 140. [Google Scholar] [CrossRef]
- Cazac, V.; Achimova, E.; Abashkin, V.; Prisacar, A.; Loshmanschii, C.; Meshalkin, A.; Egiazarian, K. Polarization holographic recording of vortex diffractive optical elements on azopolymer thin films and 3D analysis via phase-shifting digital holographic microscopy. Opt. Express 2021, 29, 9217. [Google Scholar] [CrossRef]
- Wang, Z.; Millet, L.; Mir, M.; Ding, H.; Unarunotai, S.; Rogers, J.; Gillette, M.U.; Popescu, G. Spatial light interference microscopy (SLIM). Opt. Express 2011, 19, 1016. [Google Scholar] [CrossRef] [PubMed]
- Tahara, T.; Endo, Y. Multiwavelength-selective phase-shifting digital holography without mechanical scanning. Appl. Opt. 2019, 58, G218–G225. [Google Scholar] [CrossRef] [PubMed]
- Wyant, J.; Shagam, R. Use of electronic phase measurement techniques in optical testing. In Proceedings of the ICO-11 Conference, Madrid, Spain, 10–17 September 1978. [Google Scholar]
- Creath, K. V Phase-Measurement Interferometry Techniques. Prog. Opt. 1988, 26, 349–393. [Google Scholar] [CrossRef]
- Nicola, S.D.; Ferraro, P.; Finizio, A.; Pierattini, G. Wave front reconstruction of Fresnel off-axis holograms with compensation of aberrations by means of phase-shifting digital holography. Opt. Lasers Eng. 2002, 37, 331–340. [Google Scholar] [CrossRef]
- Meneses-Fabian, C.; Rivera-Ortega, U. Phase-Shifting Interferometry by Amplitude Modulation. In Interferometry; Padron, I., Ed.; IntechOpen: Rijeka, Croatia, 2012; Chapter 8. [Google Scholar] [CrossRef] [Green Version]
- Wang, S. Dual Transverse Electro-Optic Modulator in Optical Interferometric Systems. Ph.D. Thesis, Technische Universität München, München, Germany, 2020. [Google Scholar]
- Belashov, A.V.; Petrov, N.V. Improvement of rough surfaces height map reconstruction accuracy in tilt angle illumination digital holography. Opt. Eng. 2020, 59, 1. [Google Scholar] [CrossRef]
- Guo, C.S.; Zhang, L.; Wang, H.T.; Liao, J.; Zhu, Y.Y. Phase-shifting error and its elimination in phase-shifting digital holography. Opt. Lett. 2002, 27, 1687–1689. [Google Scholar] [CrossRef]
- Cordero, R.R.; Molimard, J.; Martínez, A.; Labbe, F. Uncertainty analysis of temporal phase-stepping algorithms for interferometry. Opt. Commun. 2007, 275, 144–155. [Google Scholar] [CrossRef]
- Schwider, J.; Burow, R.; Elssner, K. Digital wave-front measuring interferometry: Some systematic error sources. Appl. Opt. 1983, 22, 3421–3432. [Google Scholar]
- De Groot, P. Phase-shift calibration errors in interferometers with spherical Fizeau cavities. Appl. Opt. 1995, 34, 2856–2863. [Google Scholar] [CrossRef]
- Zhang, H.; Lalor, M.; Burton, D. Error-compensating algorithms in phase-shifting interferometry: A comparison by error analysis. Opt. Lasers Eng. 1999, 31, 381–400. [Google Scholar]
- Guzhov, V.I.; Il’yinykh, S.P.; Khaidukov, D.S.; Vagizov, A.R. Eliminating phase-shift errors in interferometry. Optoelectron. Instrum. Data Process. 2011, 47, 76–80. [Google Scholar] [CrossRef]
- Guzhov, V.I.; Ilinykh, S.P.; Emelyanov, V.A.; Marchenko, I.O. Eliminating the effect of non-linear distortion of profile fringes for structured illumination method. In Proceedings of the 2016 11th International Forum on Strategic Technology, IFOST 2016, Novosibirsk, Russia, 1–3 June 2016; pp. 535–537. [Google Scholar] [CrossRef]
- Kakue, T.; Itoh, S.; Xia, P.; Tahara, T.; Awatsuji, Y.; Nishio, K.; Ura, S.; Kubota, T.; Matoba, O. Single-shot femtosecond-pulsed phase-shifting digital holography. Opt. Express 2012, 20, 20286. [Google Scholar] [CrossRef] [PubMed]
- Hasegawa, S.; Hayasaki, Y. Dynamic control of spatial wavelength dispersion in holographic femtosecond laser processing. Opt. Lett. 2014, 39, 478. [Google Scholar] [CrossRef]
- Petrov, N.V.; Putilin, S.E.; Chipegin, A.A. Time-resolved image plane off-axis digital holography. Appl. Phys. Lett. 2017, 110, 161107. [Google Scholar] [CrossRef]
- Lesnichii, V.V.; Petrov, N.V.; Cheremkhin, P.A. A technique of measuring spectral characteristics of detector arrays in amateur and professional photocameras and their application for problems of digital holography. Opt. Spectrosc. 2013, 115, 557–566. [Google Scholar] [CrossRef]
- Cheremkhin, P.A.; Lesnichii, V.V.; Petrov, N.V. Use of spectral characteristics of DSLR cameras with Bayer filter sensors. J. Phys. Conf. Ser. 2014, 536, 012021. [Google Scholar] [CrossRef]
- Cheremkhin, P.; Shevkunov, I.; Petrov, N. Multiple-wavelength Color Digital Holography for Monochromatic Image Reconstruction. Phys. Procedia 2015, 73, 301–307. [Google Scholar] [CrossRef] [Green Version]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shevkunov, I.; Petrov, N.V. Phase Retardation Analysis in a Rotated Plane-Parallel Plate for Phase-Shifting Digital Holography. J. Imaging 2022, 8, 87. https://doi.org/10.3390/jimaging8040087
Shevkunov I, Petrov NV. Phase Retardation Analysis in a Rotated Plane-Parallel Plate for Phase-Shifting Digital Holography. Journal of Imaging. 2022; 8(4):87. https://doi.org/10.3390/jimaging8040087
Chicago/Turabian StyleShevkunov, Igor, and Nikolay V. Petrov. 2022. "Phase Retardation Analysis in a Rotated Plane-Parallel Plate for Phase-Shifting Digital Holography" Journal of Imaging 8, no. 4: 87. https://doi.org/10.3390/jimaging8040087
APA StyleShevkunov, I., & Petrov, N. V. (2022). Phase Retardation Analysis in a Rotated Plane-Parallel Plate for Phase-Shifting Digital Holography. Journal of Imaging, 8(4), 87. https://doi.org/10.3390/jimaging8040087






