Optical Imaging of Magnetic Particle Cluster Oscillation and Rotation in Glycerol
Abstract
1. Introduction
2. Materials and Methods
2.1. Magnetic Particle Solution
2.2. Magnetic Particle Characterization
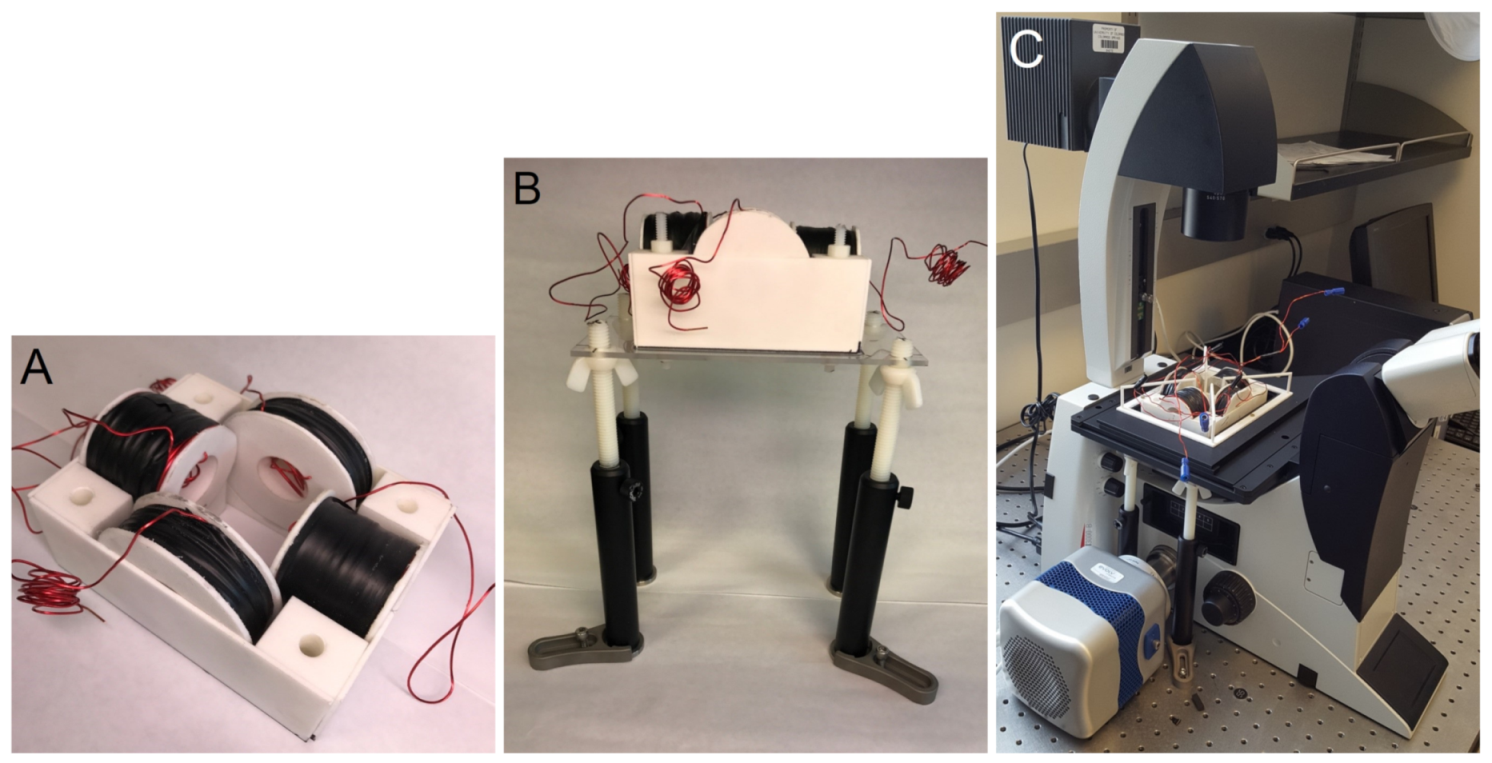
2.3. Wire Coil Microscope Insert
2.4. Magnetic Particle Cluster Imaging
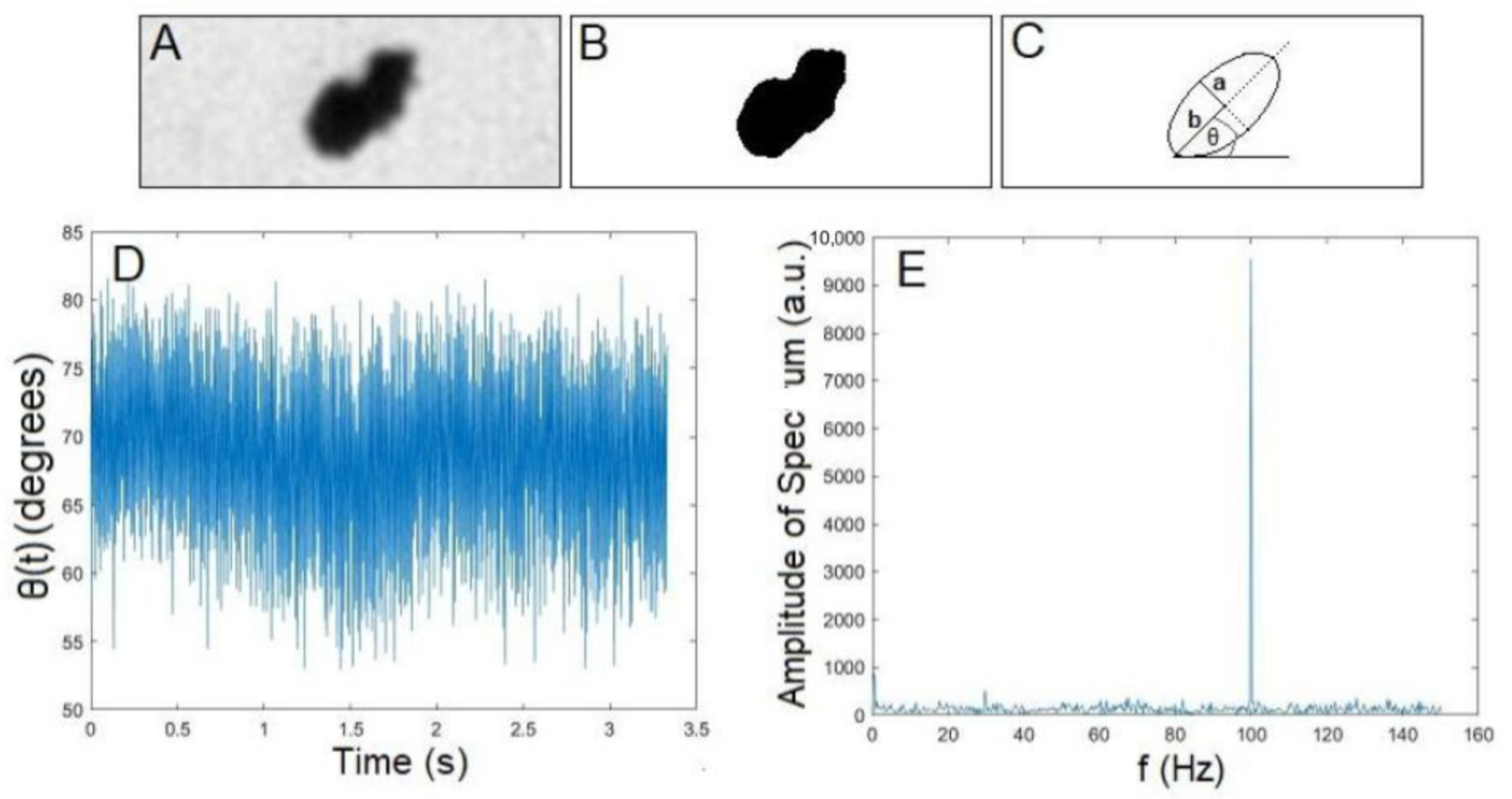
2.5. Image Analysis
2.6. Theoretical Model
3. Results
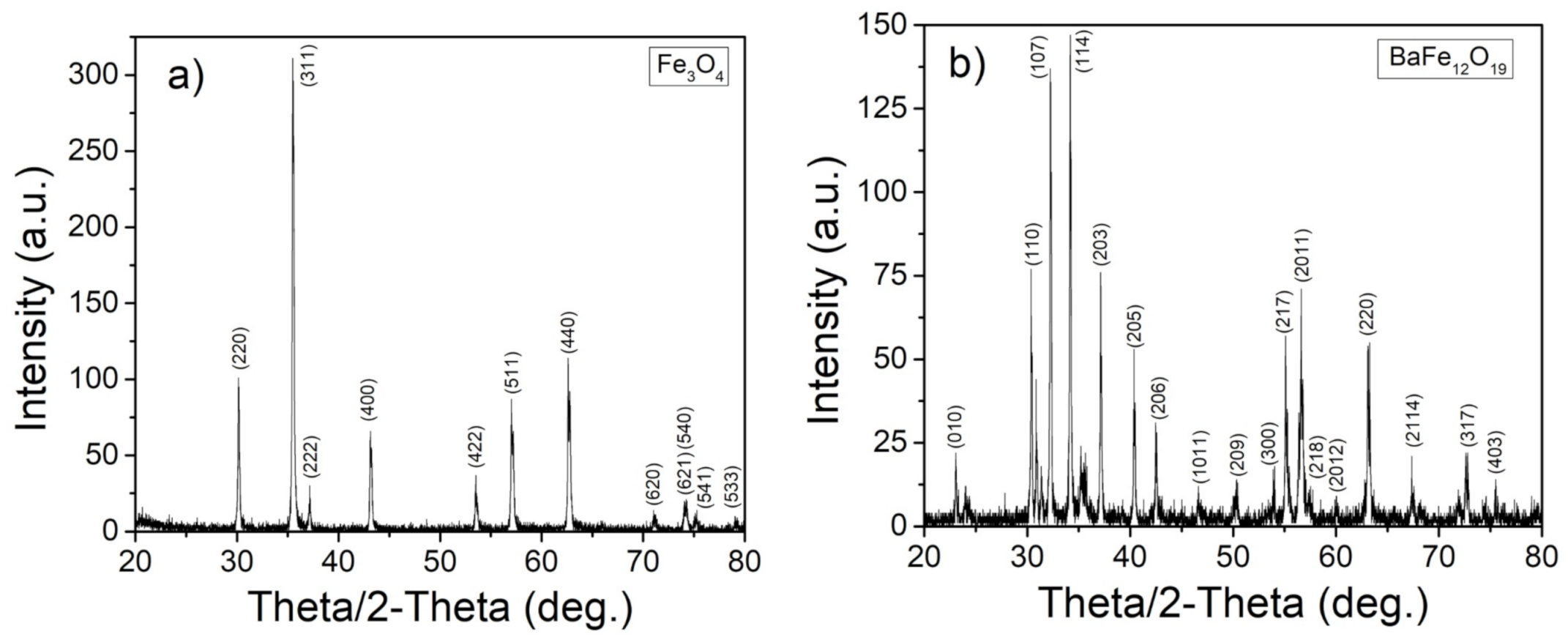
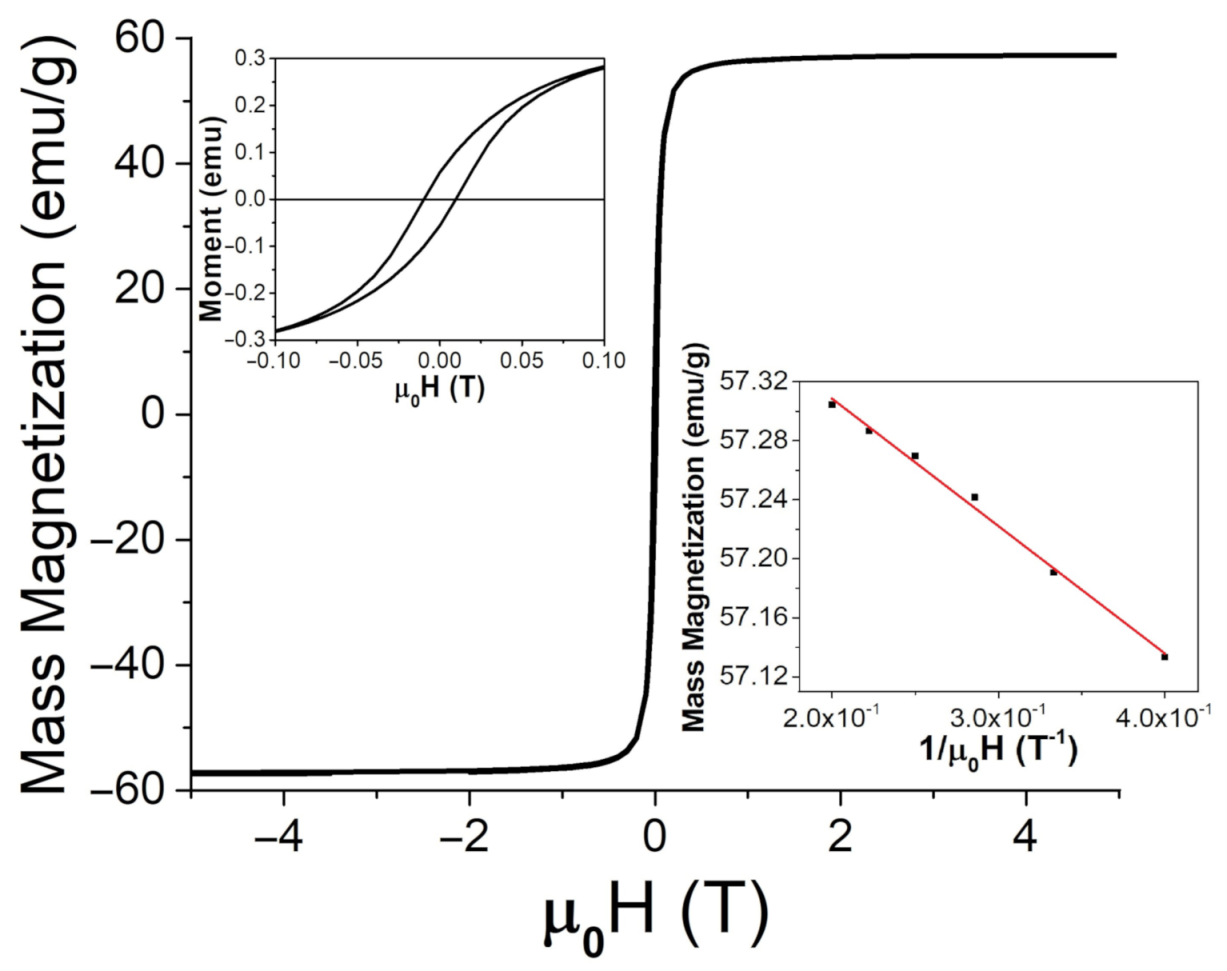
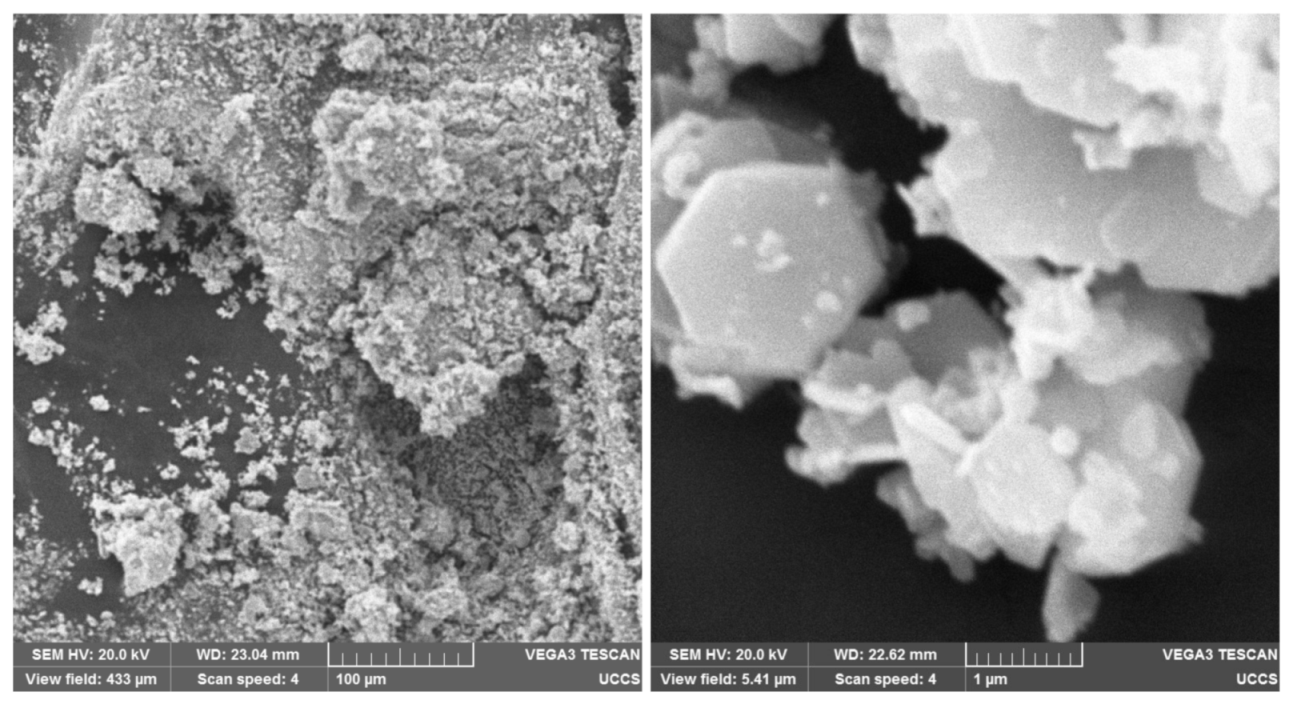
3.1. Magnetic Particle Characterization
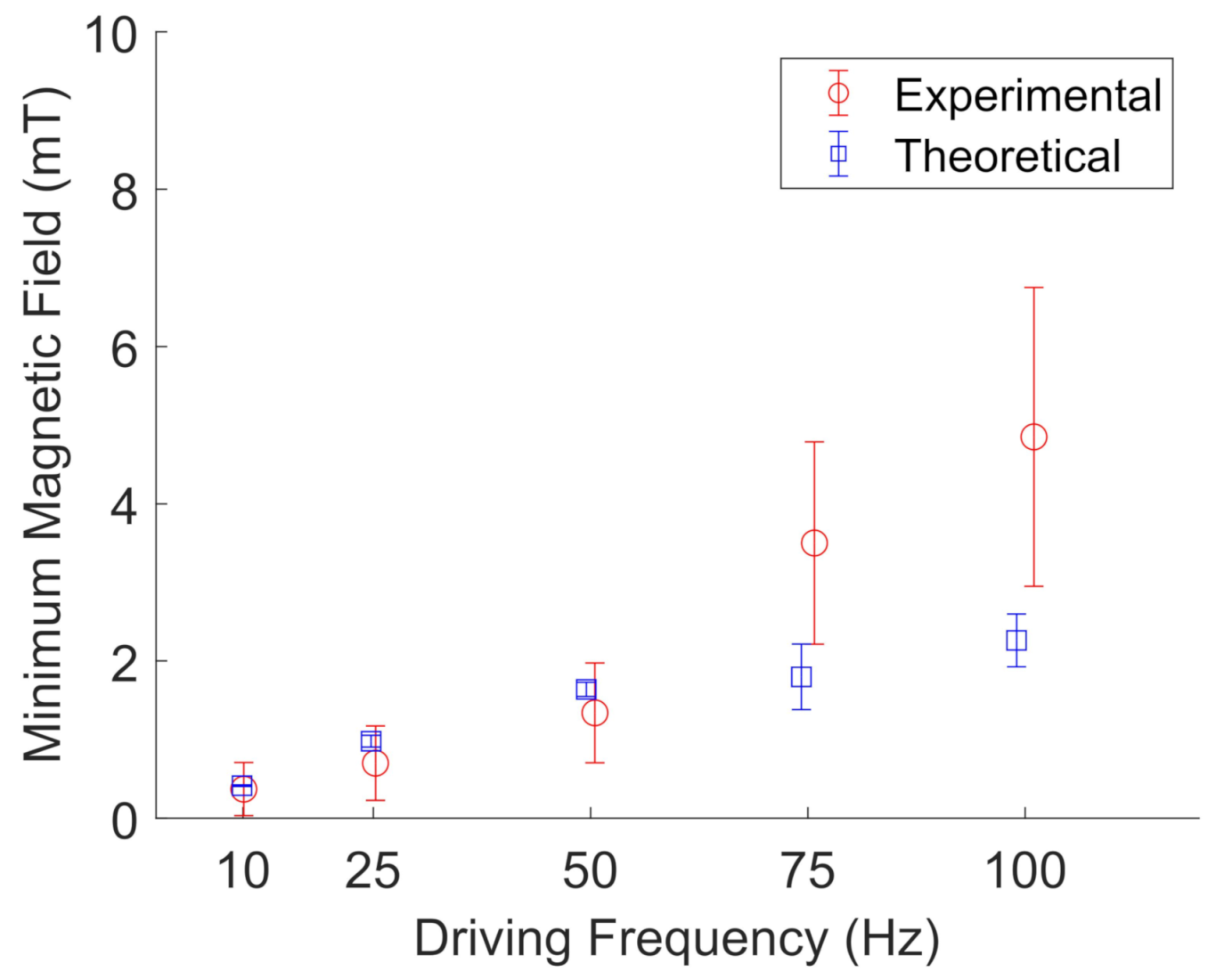
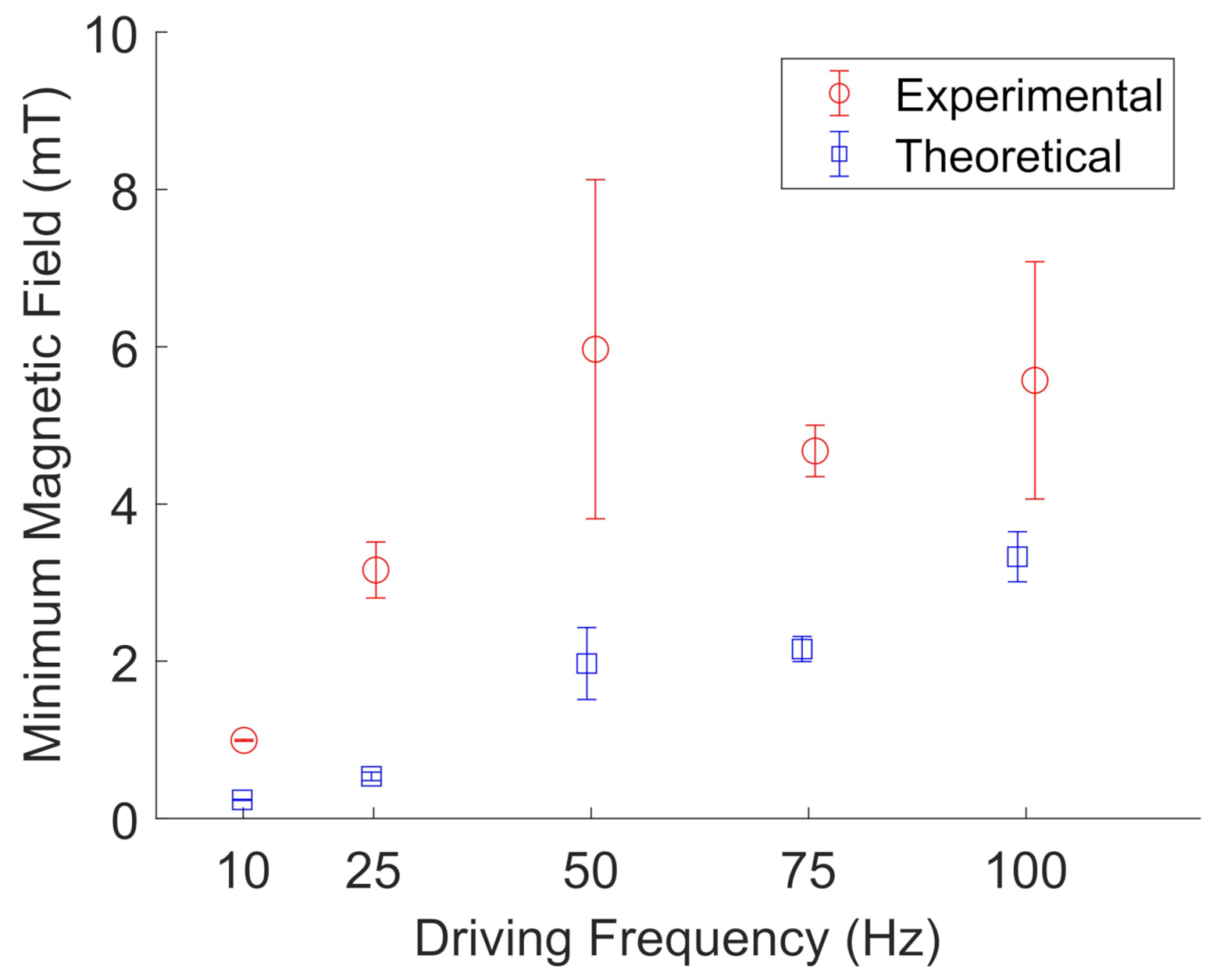
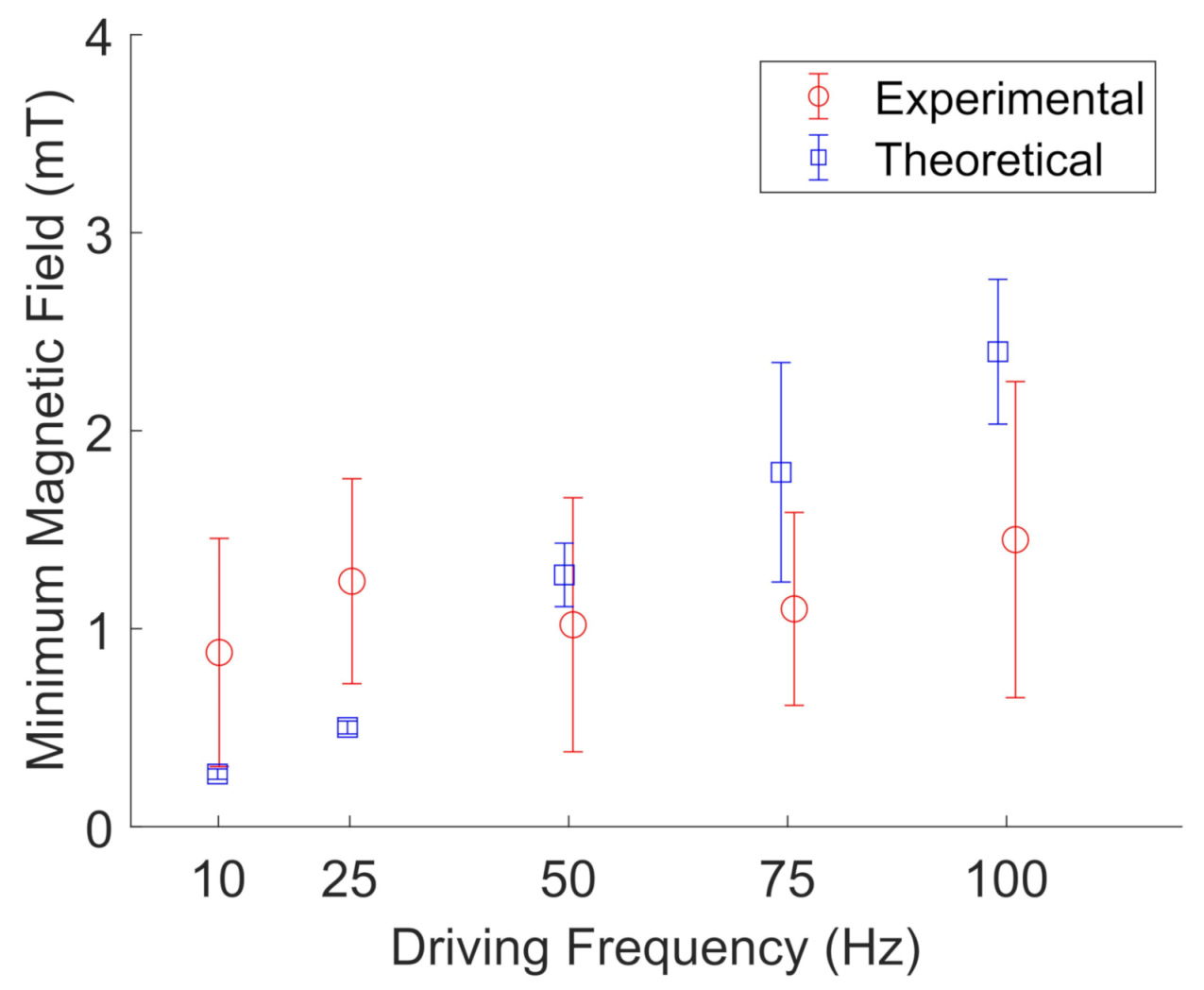
3.2. Minimum Magnetic Field Measurements
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Disease Control and Prevention (CDC). Most Recent National Asthma Data. 2020. Available online: https://www.cdc.gov/asthma/most_recent_national_asthma_data.htm (accessed on 20 May 2020).
- SreeHarsha, N.; Venugopala, K.; Nair, A.; Roopashree, T.; Attimarad, M.; Hiremath, J.; Al-Dhubiab, B.; Ramnarayanan, C.; Shinu, P.; Handral, M.; et al. An Efficient, Lung-Targeted, Drug-Delivery System to Treat Asthma Via Microparticles. Drug Des. Dev. Ther. 2019, 13, 4389–4403. [Google Scholar] [CrossRef] [PubMed]
- Howarth, P. Small Airways and Asthma: An Important Therapeutic Target? Am. J. Respir. Crit. Care Med. 1998, 157, S173. [Google Scholar] [CrossRef] [PubMed]
- Kirch, J.; Schneider, A.; Abou, B.; Hopf, A.; Schaefer, U.F.; Schneider, M.; Schall, C.; Wagner, C.; Lehr, C.M. Optical tweezers reveal relationship between microstructure and nanoparticle penetration of pulmonary mucus. Proc. Natl. Acad. Sci. USA 2012, 109, 18355–18360. [Google Scholar] [CrossRef] [PubMed]
- Singh, A.P.; Biswas, A.; Shukla, A.; Maiti, P. Targeted therapy in chronic diseases using nanomaterial-based drug delivery vehicles. Signal Transduct. Target. Ther. 2019, 33. [Google Scholar] [CrossRef]
- Economou, E.; Marinelli, S.; Smith, M.; Routt, A.; Kravets, V.; Chu, H.; Spendier, K.; Celinski, Z. Magnetic Nanodrug Delivery Through the Mucus Layer of Air-Liquid Interface Cultured Primary Normal Human Tracheobronchial Epithelial Cells. BioNanoScience 2016, 6. [Google Scholar] [CrossRef]
- Lai, J.C.; Tang, C.C.; Hong, C.Y. Size-dependent motion of bio-functionalized magnetic nanoparticle clusters under a rotating magnetic field. J. Nanopart. Res. 2013, 15, 1–8. [Google Scholar] [CrossRef]
- Du, X.; Chen, K.; Kuriyavar, S.; Kopke, R.D.; Grady, B.P.; Bourne, D.H.; Dormer, K.J. Magnetic targeted delivery of dexamethasone acetate across the round window membrane in guinea pigs. Otol. Neurotol. 2013, 34. [Google Scholar] [CrossRef]
- Hu, B.; Dobson, J.; El Haj, A.J. Control of smooth muscle α-actin (SMA) up-regulation in HBMSCs using remote magnetic particle mechano-activation. Nanomed. Nanotechnol. Biol. Med. 2014, 10, 45–55. [Google Scholar] [CrossRef]
- Price, P.M.; Mahmoud, W.E.; Al-Ghamdi, A.A.; Bronstein, L.M. Magnetic Drug Delivery: Where the Field Is Going. Front. Chem. 2018, 6, 619. [Google Scholar] [CrossRef]
- Patra, J.K.; Das, G.; Fraceto, L.F.; Campos, E.V.R.; Rodriguez-Torres, M.D.P.; Acosta-Torres, L.S.; Diaz-Torres, L.A.; Grillo, R.; Swamy, M.K.; Sharma, S.; et al. Nano based drug delivery systems: Recent developments and future prospects. J. Nanobiotechnol. 2018, 16, 1–33. [Google Scholar] [CrossRef]
- Babincova, M.; Babinec, P. Magnetic drug delivery and targeting: Principles and applications. Biomed. Pap. 2009, 153, 243–250. [Google Scholar] [CrossRef]
- Ruuge, E.K.; Rusetski, A.N. Magnetic fluids as drug carriers: Targeted transport of drugs by a magnetic field. J. Magn. Magn. Mater. 1993, 122, 335–339. [Google Scholar] [CrossRef]
- Kumar, C.S.; Mohammad, F. Magnetic nanomaterials for hyperthermia-based therapy and controlled drug delivery. Adv. Drug Deliv. Rev. 2011, 63. [Google Scholar] [CrossRef]
- Marszałł, M. Application of Magnetic Nanoparticles in Pharmaceutical Sciences. Pharm. Res. 2010, 28. [Google Scholar] [CrossRef]
- Scherer, C.; Figueiredo, N.A.M. Ferrofluids: Properties and applications. Braz. J. Phys. 2005, 35, 718–727. [Google Scholar] [CrossRef]
- Martirosyan, K.; Galstyan, E.; Hossain, S.; Wang, Y.J.; Litvinov, D. Barium hexaferrite nanoparticles: Synthesis and magnetic properties. Mater. Sci. Eng. B 2011, 176, 8–13. [Google Scholar] [CrossRef]
- Kim, D.H.; Rozhkova, E.A.; Ulasov, I.V.; Bader, S.D.; Rajh, T.; Lesniak, M.S.; Novosad, V. Biofunctionalized magnetic-vortex microdiscs for targeted cancer-cell destruction. Nat. Mater. 2010, 9, 165–171. [Google Scholar] [CrossRef]
- Dobson, J. Magnetic nanoparticles for drug delivery. Drug Dev. Res. 2006, 67, 55–60. [Google Scholar] [CrossRef]
- Denkbaş, E.B.; Çelik, E.; Erdal, E.; Kavaz, D.; Akbal, Ö.; Kara, G.; Bayram, C. Magnetically based nanocarriers in drug delivery. Nanobiomater. Drug Deliv. Appl. Nanobiomater. 2016, 285–331. [Google Scholar] [CrossRef]
- Abedin, M.R.; Umapathi, S.; Mahendrakar, H.; Laemthong, T.; Coleman, H.; Muchangi, D.; Santra, S.; Nath, M.; Barua, S. Polymer coated gold-ferric oxide superparamagnetic nanoparticles for theranostic applications. J. Nanobiotechnol. 2018, 16. [Google Scholar] [CrossRef]
- Golovin, Y.I.; Gribanovsky, S.L.; Golovin, D.Y.; Zhigachev, A.O.; Klyachko, N.L.; Majouga, A.G.; Sokolsky, M.; Kabanov, A.V. The dynamics of magnetic nanoparticles exposed to non-heating alternating magnetic field in biochemical applications: Theoretical study. J. Nanoparticle Res. 2017, 19, 1–14. [Google Scholar] [CrossRef]
- Shen, Y.; Wu, C.; Uyeda, T.Q.P.; Plaza, G.R.; Liu, B.; Han, Y.; Lesniak, M.S.; Cheng, Y. Elongated Nanoparticle Aggregates in Cancer Cells for Mechanical Destruction with Low Frequency Rotating Magnetic Field. Theranostics 2017, 7, 1735–1748. [Google Scholar] [CrossRef]
- Dieckhoff, J.; Schilling, M.; Ludwig, F. Fluxgate based detection of magnetic nanoparticle dynamics in a rotating magnetic field. Appl. Phys. Lett. 2011, 99, 112501. [Google Scholar] [CrossRef]
- Lai, S.K.; Wang, Y.Y.; Wirtz, D.; Hanes, J. Micro- and macrorheology of mucus. Adv. Drug Deliv. Rev. 2009. [Google Scholar] [CrossRef]
- Zhou, Z.; Miller, H.; Wollman, A.J.; Leake, M.C. Developing a new biophysical tool to combine magneto-optical tweezers with super-resolution fluorescence microscopy. Photonics 2015, 2, 758–772. [Google Scholar] [CrossRef]
- Edelstein, A.; Tsuchida, M.; Amodaj, N.; Pinkard, H.; Vale, R.; Stuurman, N. Advanced methods of microscope control using μmanager software. J. Biol. Methods 2014, 1, e10. [Google Scholar] [CrossRef]
- Edelstein, A.; Amodaj, N.; Hoover, K.; Vale, R.; Stuurman, N. Computer Control of Microscopes Using μmanager. Curr. Protoc. Mol. Biol. 2010, 92, 14–20. [Google Scholar] [CrossRef]
- Chin, C.; Gramoll, N.K. Fluids eBook: Viscosity. Available online: https://www.ecourses.ou.edu/cgi-bin/ebook.cgi?doc=&topic=fl&chap_sec=01.3&page=case_sol (accessed on 20 May 2020).
- Calculate Density and Viscosity of Glycerol/Water Mixtures. Available online: http://www.met.reading.ac.uk/~sws04cdw/viscosity_calc (accessed on 20 May 2020).
- Issa, B.; Obaidat, I.M.; Albiss, B.A.; Haik, Y. Magnetic nanoparticles: Surface effects and properties related to biomedicine applications. Int. J. Mol. Sci. 2013, 14, 21266–21305. [Google Scholar] [CrossRef]
- Ruíz-Baltazar, A.; Esparza, R.; Rosas, G.; Perez-Campos, R. Effect of the Surfactant on the Growth and Oxidation of Iron Nanoparticles. J. Nanomater. 2015, 2015, 240948. [Google Scholar] [CrossRef]
- Tan, G.; Chen, X. Structure and multiferroic properties of barium hexaferrite ceramics. J. Magn. Magn. Mater. 2013, 327, 87–90. [Google Scholar] [CrossRef]
- Chikazumi, S. Physics of Ferromagnetism; Oxford University Press on Demand: Oxford, UK, 1997; p. 197. [Google Scholar]
- Hu, P.; Chang, T.; Chen, W.J.; Deng, J.; Li, S.L.; Zuo, Y.G.; Kang, L.; Yang, F.; Hostetter, M.; Volinsky, A.A. Temperature effects on magnetic properties of Fe3O4 nanoparticles synthesized by the sol-gel explosion-assisted method. J. Alloys Compd. 2019, 773, 605–611. [Google Scholar] [CrossRef]
- Structure and magnetic properties of BaFe11.9In0.1O19hexaferrite in a wide temperature range. J. Alloys Compd. 2016, 689, 383–393. [CrossRef]
- Makovec, D.; Komelj, M.; Dražić, G.; Belec, B.; Goršak, T.; Gyergyek, S.; Lisjak, D. Incorporation of Sc into the structure of barium-hexaferrite nanoplatelets and its extraordinary finite-size effect on the magnetic properties. Acta Mater. 2019, 172, 84–91. [Google Scholar] [CrossRef]
- Shaker, S.; Chidurala, S.; Tumma, B. Decolorization of Congo red from Aqueous Solutions using Fe3O4 Nanoparticles. Adv. Biores. 2016, 7, 37–43. [Google Scholar] [CrossRef]
- Shannon, C. Communication in the Presence of Noise. Proc. IRE 1949, 37, 10–21. [Google Scholar] [CrossRef]
- Taylor, J.R. Classical Mechanics; University Science Books: Melville, NY, USA, 2005. [Google Scholar]
- Lisjak, D.; Ovtar, S. The Alignment of Barium Ferrite Nanoparticles from Their Suspensions in Electric and Magnetic Fields. J. Phys. Chem. B 2013, 117, 1644–1650. [Google Scholar] [CrossRef]






| Particle Cluster Area (m) | 10 Hz | 20 Hz | 40 Hz | 60 Hz | 100 Hz |
|---|---|---|---|---|---|
| BaP Oscillation | 225 +/− 345 | 220 +/− 273 | 420 +/− 297 | 453 +/− 236 | 369 +/− 254 |
| FeNP Oscillation | 42 +/− 22 | 160 +/− 57 | 169 +/− 38 | 78 +/− 59 | 105 +/− 43 |
| BaP Rotation | 392 +/− 289 | 594 +/− 220 | 258 +/− 145 | 360 +/− 245 | 359 +/− 417 |
| FeNP Rotation | 568 +/− 280 | 826 +/− 138 | 375 +/− 161 | 218 +/− 61 | 162 +/− 161 |
| Particle Cluster Diameter (m) | 10 Hz | 20 Hz | 40 Hz | 60 Hz | 100 Hz |
|---|---|---|---|---|---|
| BaP Oscillation | 17.3 +/− 7.7 | 22.9 +/− 4.6 | 24.2 +/− 11.2 | 23.4 +/− 11.2 | 23.4 +/− 11.2 |
| FeNP Oscillation | 7.3 +/− 1.9 | 14.7 +/− 2.5 | 14.7 +/− 1.7 | 9.9 +/− 3.8 | 11.6 +/− 2.3 |
| BaP Rotation | 21.6 +/− 13.6 | 22.7 +/− 11.1 | 25.5 +/− 7.2 | 20.7 +/− 8.2 | 32.2 +/− 10.3 |
| FeNP Rotation | 26.9 +/− 6.6 | 32.4 +/− 2.7 | 21.6 +/− 7.8 | 16.3 +/− 18.1 | 14.4 +/− 7.1 |
| Particle Cluster Area (m) | 10 Hz | 25 Hz | 50 Hz | 75 Hz | 100 Hz |
|---|---|---|---|---|---|
| BaP Oscillation | 273 +/− 250 | 426 +/− 174 | 537 +/− 407 | 509 +/− 432 | 516 +/− 442 |
| FeNP Oscillation | 398 +/− 229 | 187 +/− 85 | 51 +/− 3 | 183 +/− 127 | 51 +/− 2 |
| BaP Rotation | 82 +/− 62 | 320 +/− 11 | 315 +/− 9 | 181 +/− 81 | 154 +/− 34 |
| FeNP Rotation | 400 +/− 10 | 349 +/− 33 | 464 +/− 82 | 433 +/− 2 | 452 +/− 4 |
| Particle Cluster Diameter (m) | 10 Hz | 20 Hz | 40 Hz | 60 Hz | 100 Hz |
|---|---|---|---|---|---|
| BaP Oscillation | 17.3 +/− 7.7 | 22.9 +/− 4.6 | 24.2 +/− 11.2 | 23.4 +/− 11.2 | 23.4 +/− 11.2 |
| FeNP Oscillation | 22.5 +/− 6.5 | 15.4 +/− 3.5 | 18.7 +/− 4.9 | 15.2 +/− 5.3 | 8.1 +/− 0.2 |
| BaP Rotation | 10.2 +/− 3.9 | 20.2 +/− 0.3 | 20.0 +/− 0.3 | 15.2 +/− 3.4 | 14.0 +/− 1.5 |
| FeNP Rotation | 22.6 +/− 0.3 | 21.1 +/− 1.0 | 24.3 +/− 2.1 | 23.4 +/− 0.1 | 24.0 +/− 0.1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gassen, R.; Thompkins, D.; Routt, A.; Jones, P.; Smith, M.; Thompson, W.; Couture, P.; Bozhko, D.A.; Celinski, Z.; Camley, R.E.; et al. Optical Imaging of Magnetic Particle Cluster Oscillation and Rotation in Glycerol. J. Imaging 2021, 7, 82. https://doi.org/10.3390/jimaging7050082
Gassen R, Thompkins D, Routt A, Jones P, Smith M, Thompson W, Couture P, Bozhko DA, Celinski Z, Camley RE, et al. Optical Imaging of Magnetic Particle Cluster Oscillation and Rotation in Glycerol. Journal of Imaging. 2021; 7(5):82. https://doi.org/10.3390/jimaging7050082
Chicago/Turabian StyleGassen, River, Dennis Thompkins, Austin Routt, Philippe Jones, Meghan Smith, William Thompson, Paul Couture, Dmytro A. Bozhko, Zbigniew Celinski, Robert E. Camley, and et al. 2021. "Optical Imaging of Magnetic Particle Cluster Oscillation and Rotation in Glycerol" Journal of Imaging 7, no. 5: 82. https://doi.org/10.3390/jimaging7050082
APA StyleGassen, R., Thompkins, D., Routt, A., Jones, P., Smith, M., Thompson, W., Couture, P., Bozhko, D. A., Celinski, Z., Camley, R. E., Hagen, G. M., & Spendier, K. (2021). Optical Imaging of Magnetic Particle Cluster Oscillation and Rotation in Glycerol. Journal of Imaging, 7(5), 82. https://doi.org/10.3390/jimaging7050082






