Time-of-Flight Neutron Imaging on IMAT@ISIS: A New User Facility for Materials Science
Abstract
1. Introduction
2. TOF Imaging and Instrument Description
2.1. TOF Imaging
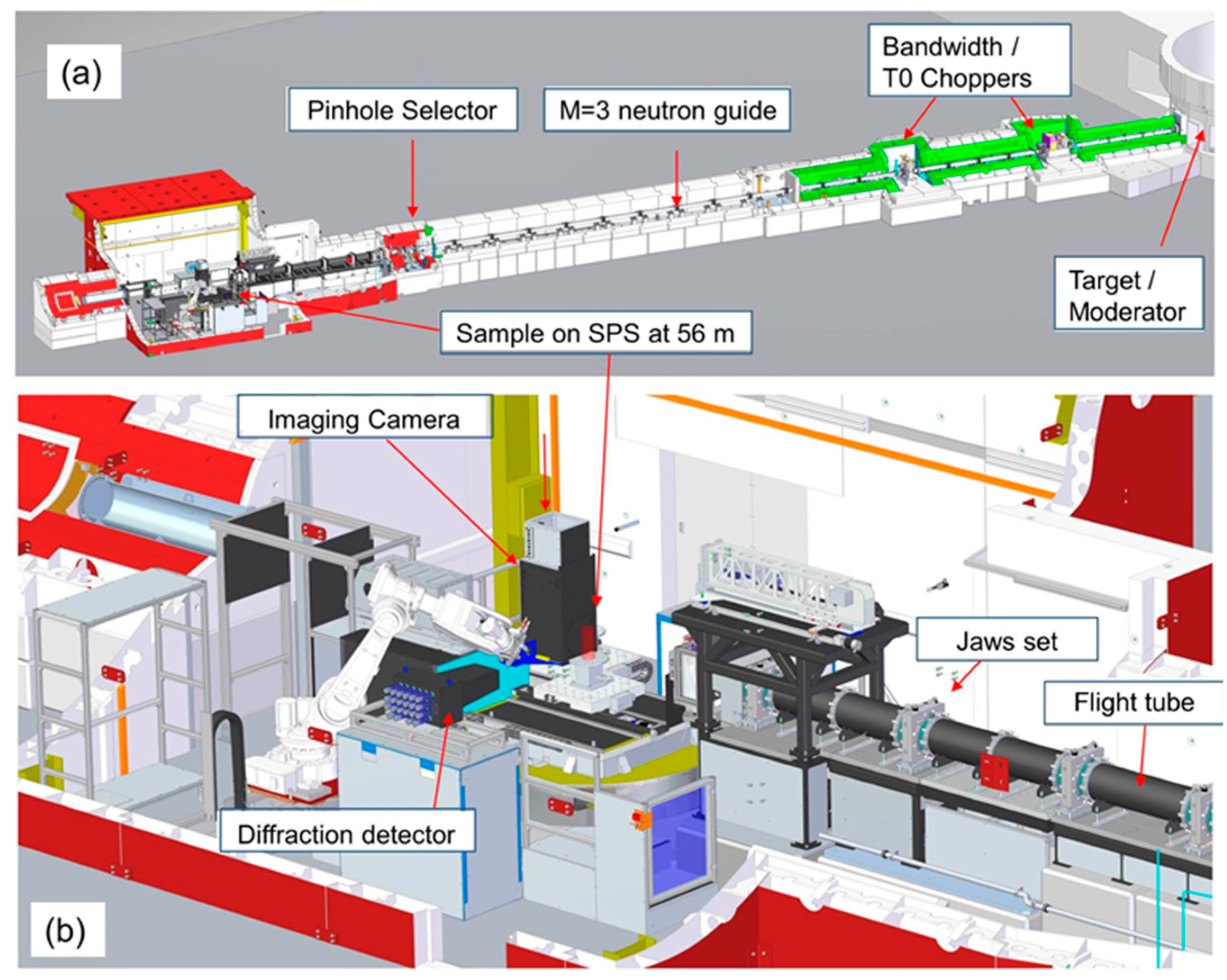
2.2. IMAT Instrument
2.2.1. Source and Choppers
2.2.2. Beamline Components
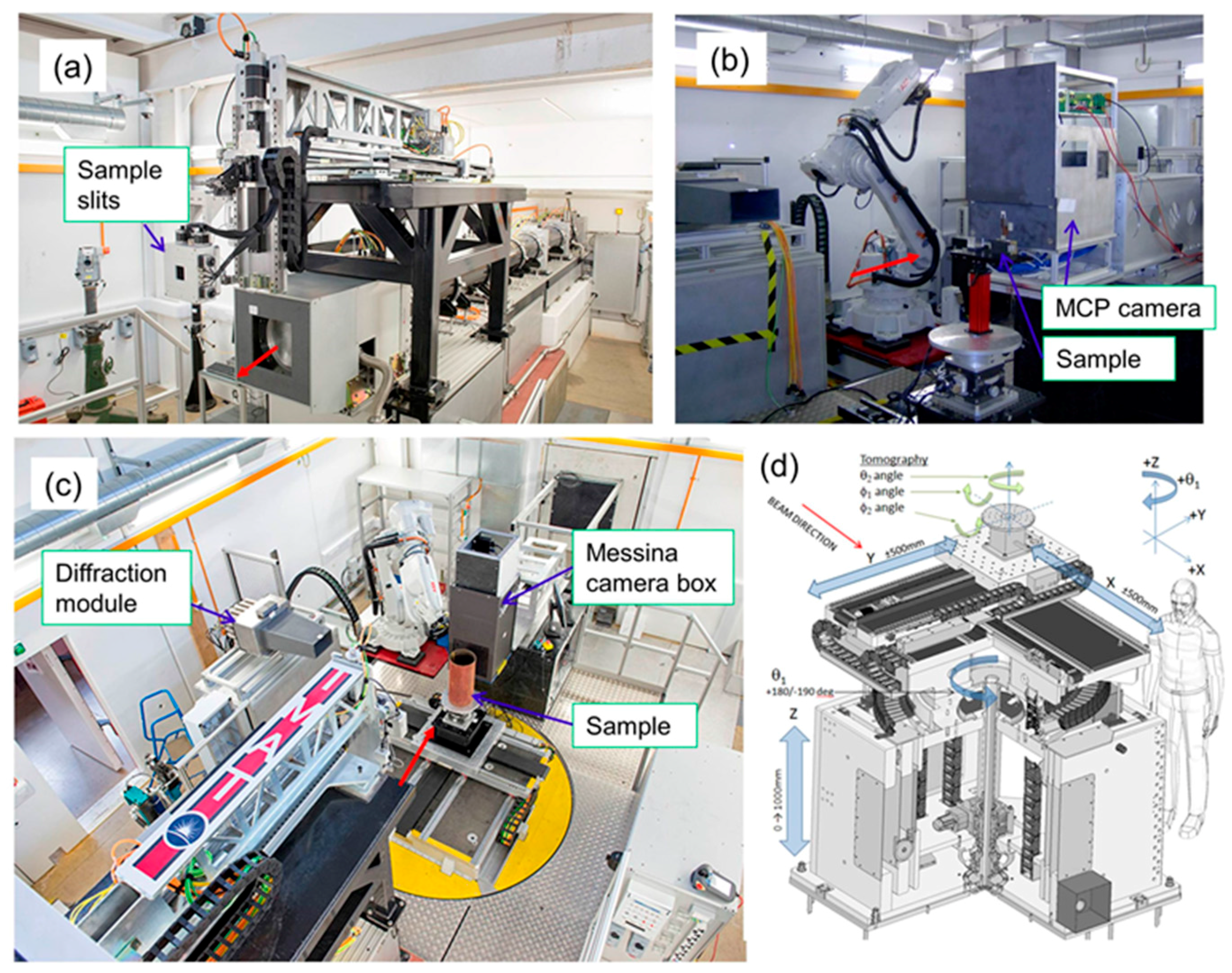
2.2.3. IMAT Cameras
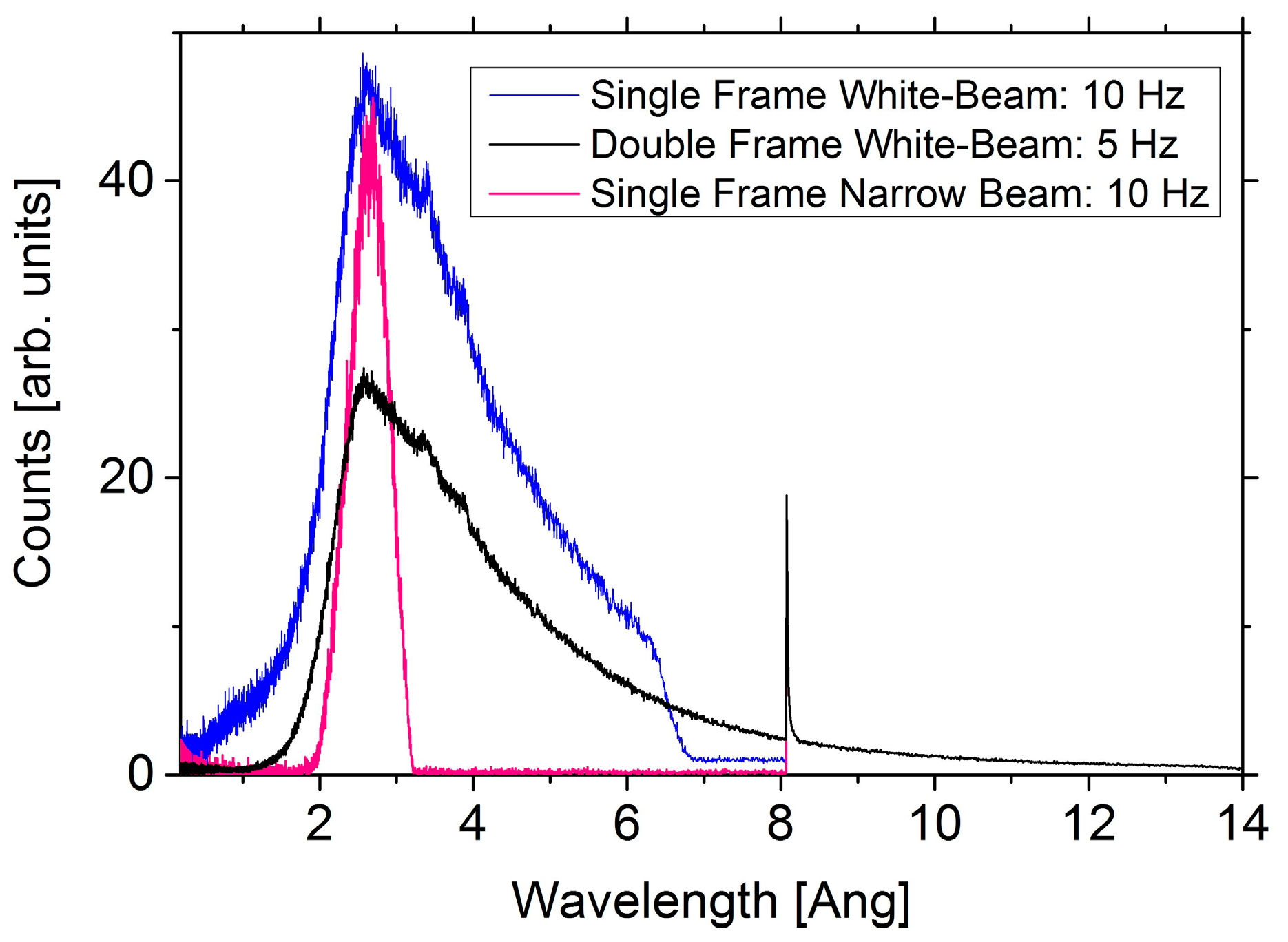
2.3. IMAT Spectrum and Energy-Selection
2.4. Instrument Parameters
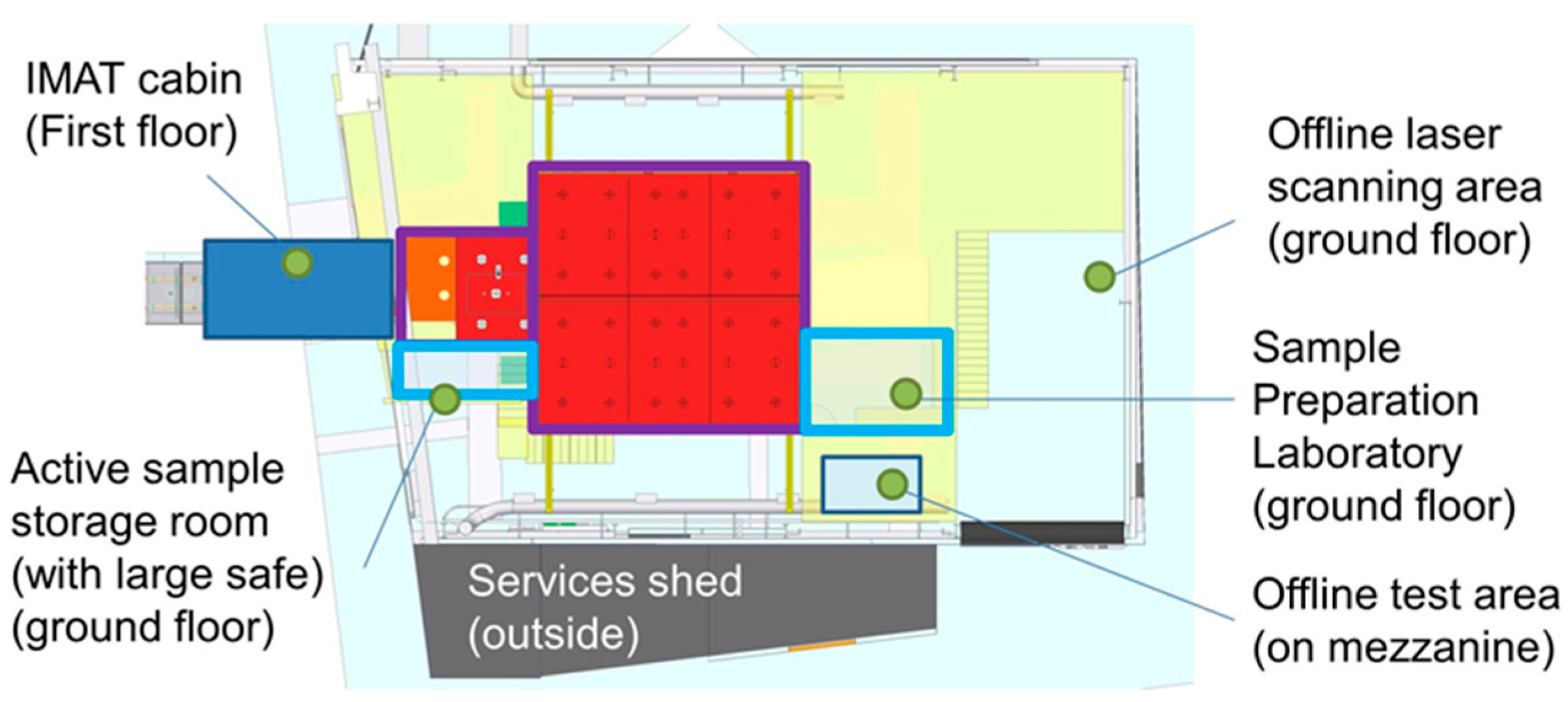
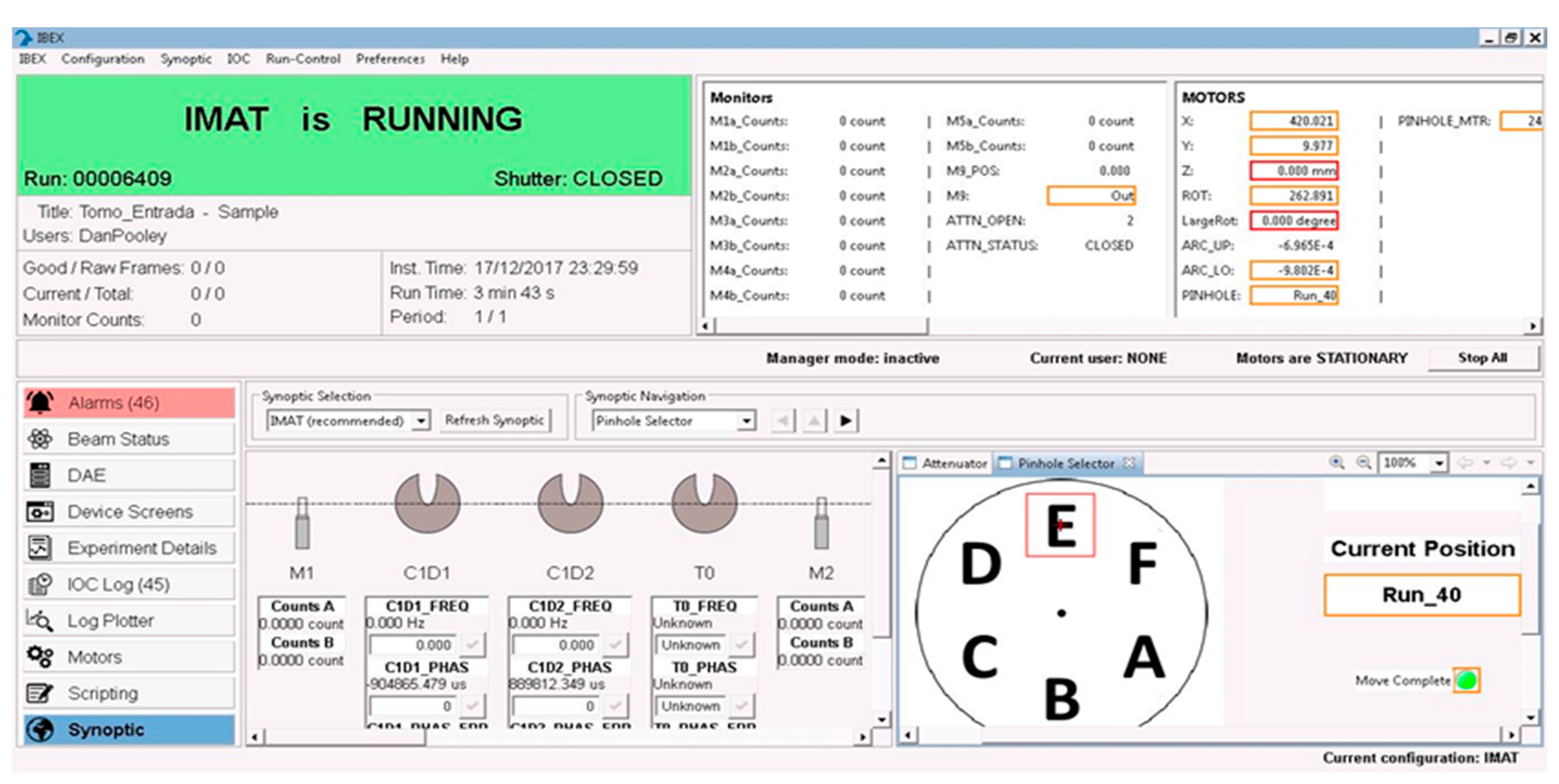
2.5. Infrastructure and Software
- IMAT cabin: With work stations for instrument control and image analysis, and links to the ISIS data archive;
- a small chemistry/sample preparation laboratory;
- a large safe in lockable room for storing large samples, and valuable samples and objects. The room can be used for sample storage before and after irradiation, i.e., for radio-activated samples and equipment;
- an offline-testing area for sample environment, in particular for mechanical loading rigs;
- a hydraulic system to deliver 210 bar hydraulic pressure at 90 lpm flow rate to two manifold stations - one positioned at the sample area and another station at the offline-testing area.
- a services shed outside the IMAT extension for hydraulic pumps, He gas compressors for cold heads, vacuum pumps;
- an offline laser scanning area
3. Demonstration of Bragg Edge Analysis on IMAT
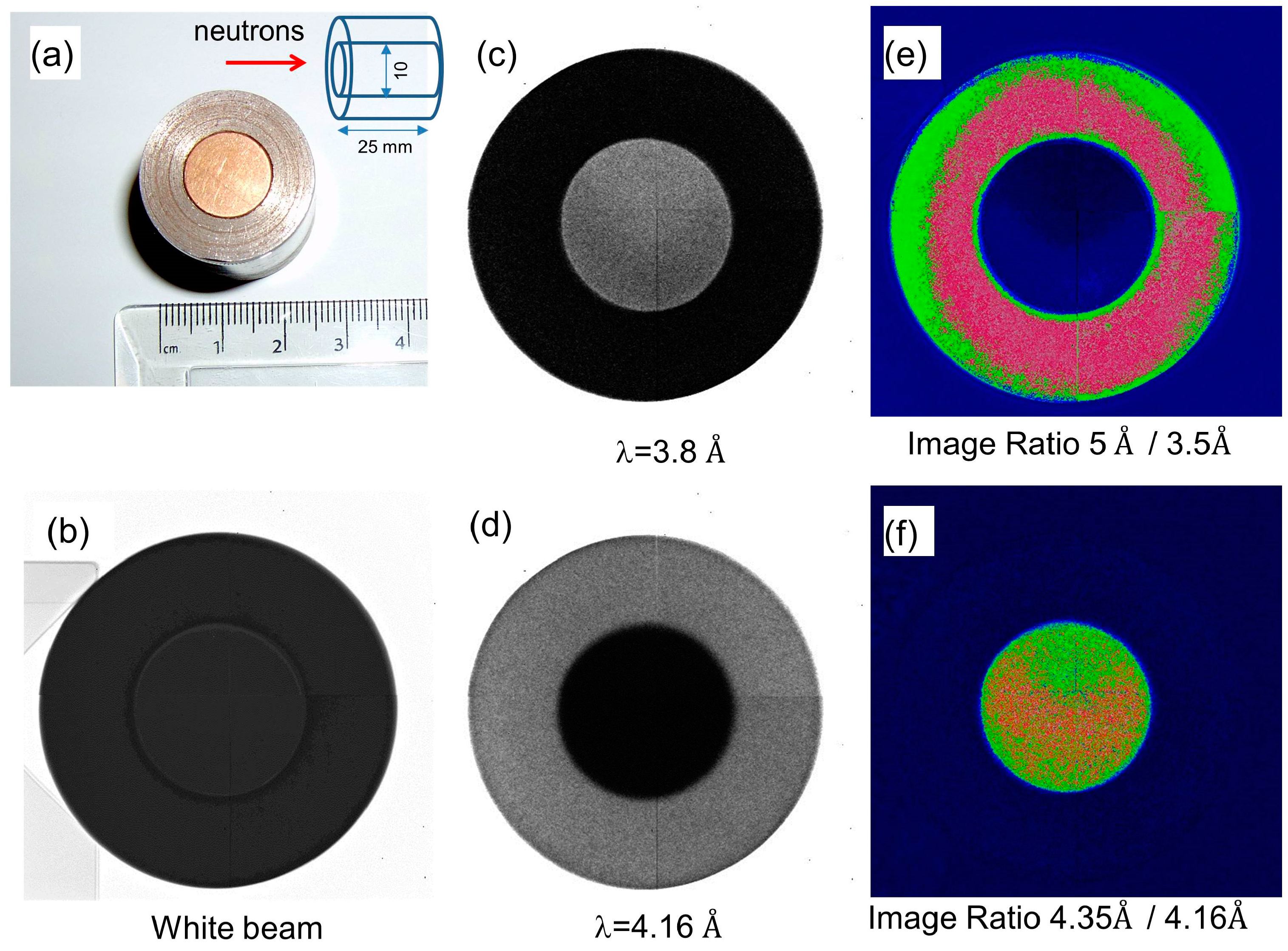
3.1. Sample Description
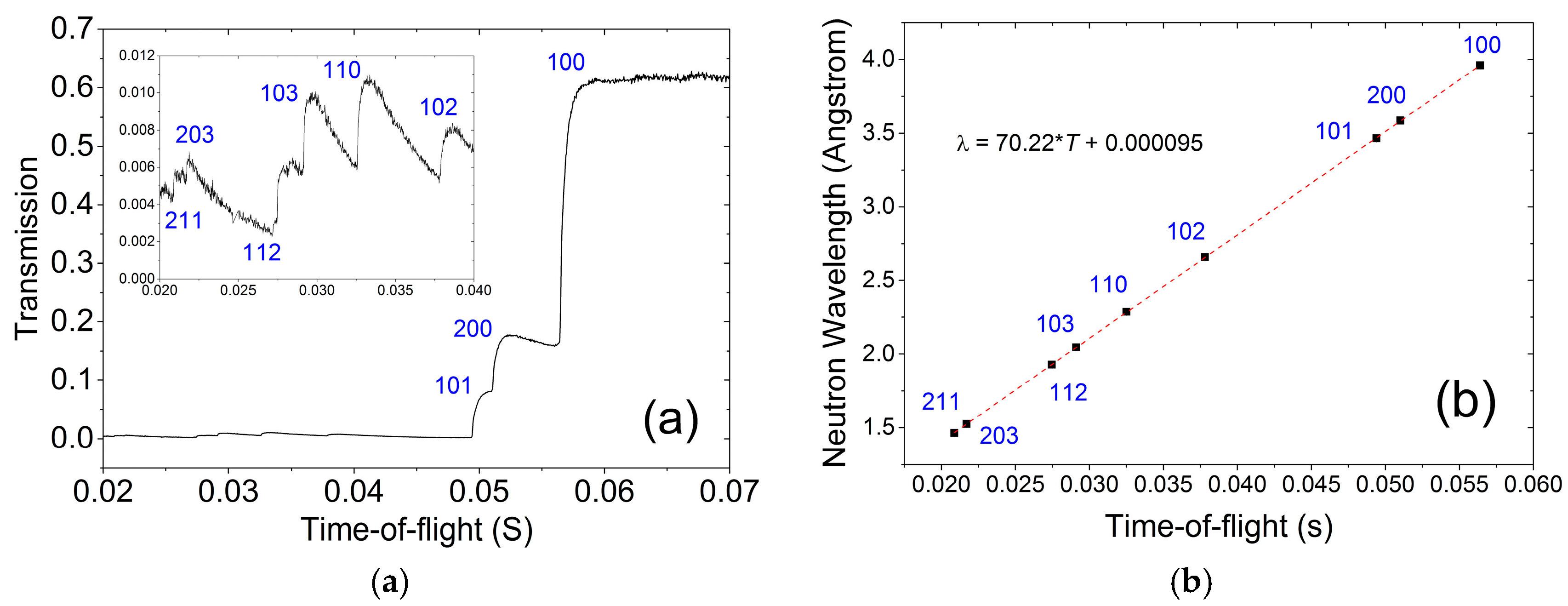
3.2. Flight Path Calibration
3.3. Data Collection
3.3.1. Sample Alignment and Camera Preparation
- -
- The IMAT disk choppers were set to 10 Hz, and dephased by 20 ms to define a TOF range of 32–115 ms, corresponding to a wavelength band of about 2.2–8 Å. The same delay of 20 ms was set for the MCP detector (to be added to the TOFmin and TOFmax values in Table 4). The MCP system used a 10 Hz trigger signal from the choppers.
- -
- The beam size was set to 35 × 35 mm2 to fully illuminate the MCP active sensor.
- -
- The pinhole was set to 40 mm diameter; i.e., the L/D was 245 [29]; the part of the sample furthest away (45 mm) from the sensor determines the best resolution of 45/245 = 180 μm.
- -
- The expected positions of Bragg edges can be found in crystallographic databases. There are tools available to calculate Bragg edge spectra taking the structure information, and neutron absorption and neutron scattering cross sections into account e.g., [55].
- -
- The TOF ranges and the time bins were set in the MCP detector interface (by editing ShutterValues.txt). Three MCP readouts were chosen between 32 and 115 ms, to reduce event overlap (see below) whilst avoiding having Fe and Cu Bragg edges coinciding with readout gaps. Table 4 contains calculated edge positions of copper and ferritic steel, and a list of shutters used for the experiment: three TOF ranges (shutters) with time bins of 40.96, 20.48, 40.96 μs were defined.
- -
- M5 and the sample slits were moved out of the beam.
3.3.2. Open-Beam Data and Dark Current Images
3.3.3. Sample Scan
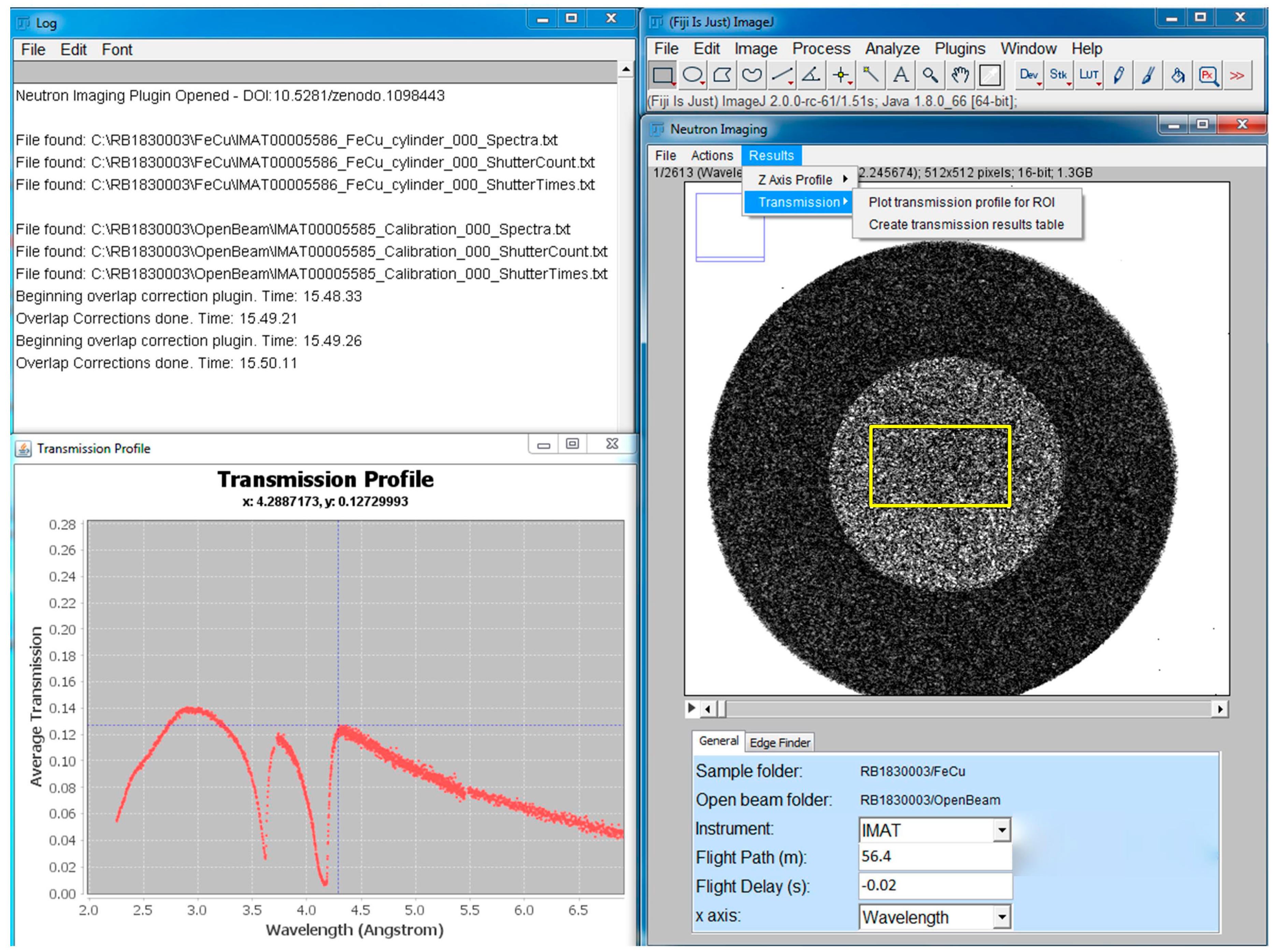
3.3.4. Inspecting the Data
3.4. Correction Procedures
3.4.1. Flat Fielding
3.4.2. MCP Related Corrections
3.5. Basic Image Analysis
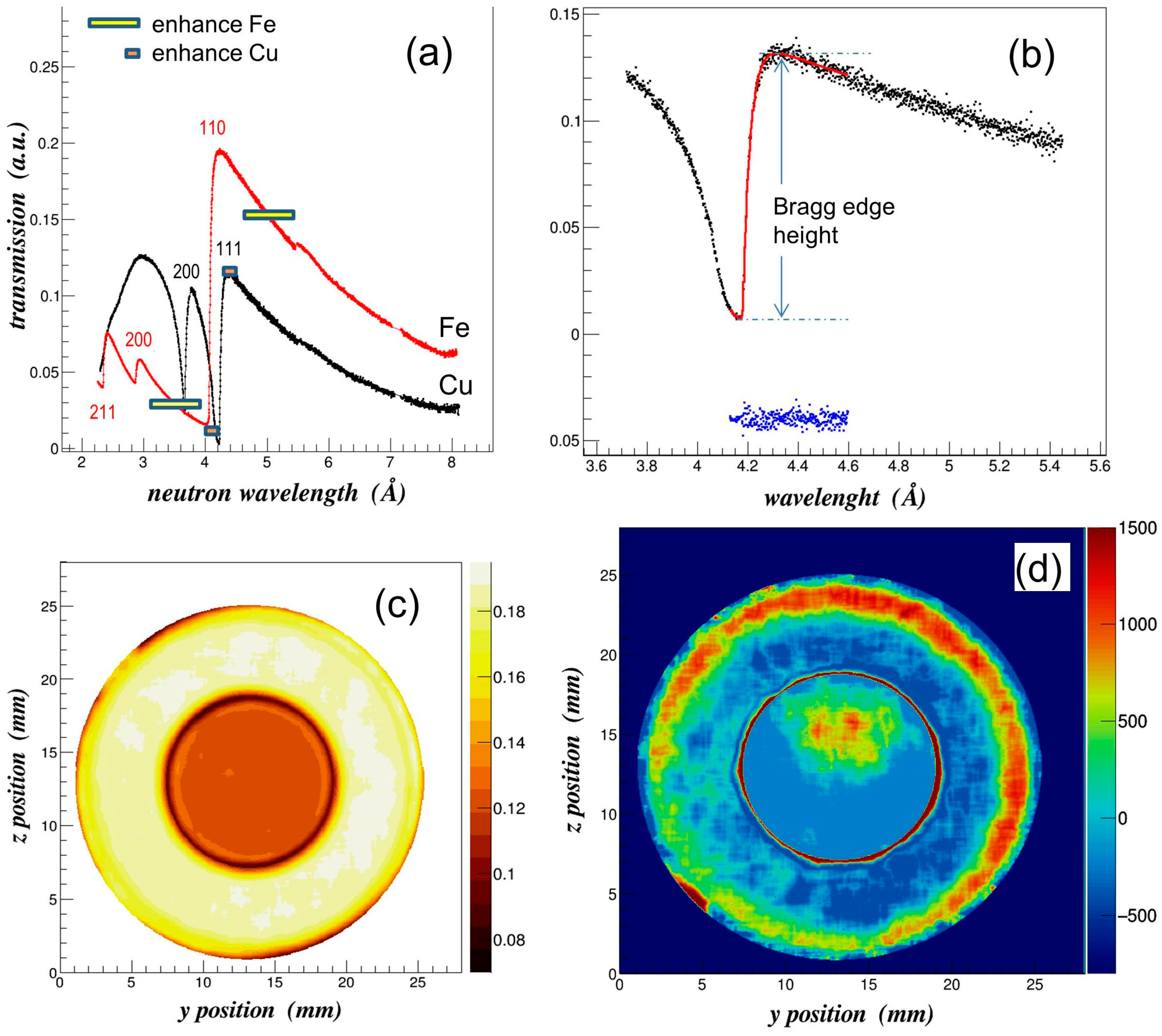
3.6. Bragg Edge Mapping
3.7. Tomographic Reconstruction
3.8. Discussion
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Kockelmann, W.; Frei, G.; Lehmann, E.H.; Vontobel, P.; Santisteban, J.R. Energy-selective neutron transmission imaging at a pulsed source. Nucl. Instrum. Meth. 2007, A57, 421–434. [Google Scholar] [CrossRef]
- Shinohara, T.; Kai, T.; Oikawa, K.; Segawa, M.; Harada, M.; Nakatani, T.; Ooi, M.; Aizawa, K.; Sato, H.; Kamiyama, T.; et al. Final design of the energy-resolved neutron imaging system RADEN at J-PARC. J. Phys. Conf. Ser. 2016, 746, 012007. [Google Scholar] [CrossRef]
- Strobl, M. Future prospects of imaging at spallation neutron sources. Nucl. Instrum. Meth. 2009, A604, 646–652. [Google Scholar] [CrossRef]
- Strobl, M.; Kardjilov, N.; Hilger, A.; Penumadu, D.; Manke, I. Advanced neutron imaging methods with a potential to benefit from pulsed sources. Nucl. Instrum. Meth. 2011, A651, 57–61. [Google Scholar] [CrossRef]
- Kabra, S.; Kelleher, J.; Kockelmann, W.; Gutmann, M.; Tremsin, A.S. Energy-dispersive neutron imaging and diffraction of magnetically driven twins in a Ni2MnGa single crystal magnetic shape memory alloy. J. Phys. Conf. Ser. 2016, 746, 012056. [Google Scholar] [CrossRef]
- Tremsin, A.S.; Yau, T.Y.; Kockelmann, W. Non-destructive examination of loads in regular and self-locking Spiralock® threads through energy-resolved neutron imaging. Strain 2016, 52, 548–558. [Google Scholar] [CrossRef]
- Sato, H.; Shiota, Y.; Morooka, S.; Todaka, Y.; Adachi, N.; Sadamatsu, S.; Oikawa, K.; Harada, M.; Zang, S.; Su, Y.; et al. Inverse pole figure mapping of bulk crystalline grains in a polycrystalline steel plate by pulsed neutron Bragg-dip transmission imaging. J. Appl. Cryst. 2017, 50, 1601–1610. [Google Scholar] [CrossRef]
- Makowska, M.G.; Strobl, M.; Lauridsen, E.M.; Kabra, S.; Kockelmann, W.; Tremsin, A.; Frandsen, H.L.; Theil Kuhn, L. In situ time-of-flight neutron imaging of NiO–YSZ anode support reduction under influence of stress. J. Appl. Cryst. 2016, 49, 1674–1681. [Google Scholar] [CrossRef]
- Festa, G.; Perelli Cippo, E.; Di Martino, D.; Cattaneo, R.; Senesi, R.; Andreani, C.; Schooneveld, E.; Kockelmann, W.; Rhodes, N.; Scherillo, A.; et al. Neutron resonance transmission imaging for 3D elemental mapping at the ISIS spallation neutron source. J. Anal. At. Spectrom. 2015, 30, 745–750. [Google Scholar] [CrossRef]
- Tremsin, A.S.; Vogel, S.C.; Mocko, M.; Bourke, M.A.M.; Yuan, V.; Nelson, R.O.; Brown, D.W.; Feller, W.B. Non-destructive studies of fuel rodlets by neutron resonance absorption radiography and thermal neutron radiography. J. Nucl. Mater. 2013, 440, 633–646. [Google Scholar] [CrossRef]
- Kai, T.; Maekawa, F.; Oshita, H.; Sato, H.; Shinohara, T.; Ooi, M.; Harada, M.; Uno, S.; Otomo, T.; Kamiyama, T.; et al. Visibility estimation for neutron resonance absorption radiography using a pulsed neutron source. Phys. Procedia 2013, 43, 111–120. [Google Scholar] [CrossRef]
- Tremsin, A.S.; Kockelmann, W.; Pooley, D.E.; Feller, W.B. Spatially resolved remote measurement of temperature by neutron resonance absorption. Nucl. Instrum. Meth. A 2015, 803, 15–23. [Google Scholar] [CrossRef]
- Shinohara, T.; Hiroi, K.; Su, Y.; Kai, T.; Nakatani, T.; Oikawa, K.; Segawa, M.; Hayashida, H.; Parker, J.D.; Matsumoto, Y.; et al. Polarization analysis for magnetic field imaging at RADEN in J-PARC/MLF. J. Phys. Conf. Ser. 2017, 862, 012025. [Google Scholar] [CrossRef]
- Cereser, A.; Strobl, M.; Hall, S.A.; Steuwer, A.; Kiyanagi, Y.; Tremsin, A.S.; Knudsen, E.B.; Shinohara, T.; Willendrup, P.K.; Bastos da Silva Fanta, A.; et al. Time-of-Flight Three Dimensional Neutron Diffraction in Transmission Mode for Mapping Crystal Grain Structures. Sci. Rep. 2017, 7, 9561. [Google Scholar] [CrossRef] [PubMed]
- Strobl, M.; Betz, B.; Harti, R.P.; Hilger, A.; Kardjilov, N.; Manke, I.; Gruenzweig, C. Wavelength-dispersive dark-field contrast: Micrometre structure resolution in neutron imaging with gratings. J. Appl. Cryst. 2016, 49, 569–573. [Google Scholar] [CrossRef]
- Vogel, S. A Rietveld-Approach for the Analysis of Neutron Time-of-Flight Transmission Data. Ph.D. Thesis, Christian Albrechts Universität, Kiel, Germany, 2000. [Google Scholar]
- Santisteban, J.R.; Edwards, L.; Steuwer, A.; Withers, P.J. Time-of-flight neutron transmission diffraction. J. Appl. Crystallogr. 2001, 34, 289–297. [Google Scholar] [CrossRef]
- Santisteban, J.R.; Vicente-Alvarez, M.A.; Vizcaino, P.; Banchik, A.D.; Vogel, S.C.; Tremsin, A.S.; Vallerga, J.V.; McPhate, J.B.; Lehmann, E.; Kockelmann, W. Texture imaging of zirconium based components by total neutron cross-section experiments. J. Nucl. Mater. 2012, 425, 218–227. [Google Scholar] [CrossRef]
- Tremsin, A.S.; Ganguly, S.; Meco, S.M.; Pardal, G.R.; Shinohara, T.; Feller, W.B. Investigation of dissimilar metal welds by energy resolved neutron imaging. J. Appl. Cryst. 2016, 49, 1130–1140. [Google Scholar] [CrossRef] [PubMed]
- Vitucci, G.; Minniti, T.; Di Martino, D.; Musa, M.; Gori, L.; Micieli, D.; Kockelmann, W.; Watanabe, K.; Tremsin, A.S.; Gorini, G. Energy-resolved neutron tomography of an unconventional cultured pearl at a pulsed spallation source using a microchannel plate camera. Microchem. J. 2018, 137, 473–479. [Google Scholar] [CrossRef]
- Hendriks, J.N.; Gregg, A.W.T.; Wensrich, C.M.; Tremsin, A.S.; Shinohara, T.; Meylan, M.; Kisi, E.H.; Luzin, V.; Kirsten, O. Bragg-edge elastic strain tomography for in situ systems from energy-resolved neutron transmission imaging. Phys. Rev. Mater. 2017, 1, 053802. [Google Scholar] [CrossRef]
- Lionheart, W.R.B.; Withers, P.J. Diffraction tomography of strain. Inverse Probl. 2015, 31, 045005. [Google Scholar] [CrossRef]
- Kardjilov, N.; Manke, I.; Hilger, A.; Williams, S.; Strobl, M.; Woracek, R.; Boin, M.; Lehmann, E.; Penumadu, D.; Banhart, J. Neutron Bragg edge mapping of weld seams. Int. J. Mater. Res. 2012, 103, 151–154. [Google Scholar] [CrossRef]
- Woracek, R.; Penumadu, D.; Kardjilov, N.; Hilger, A.; Boin, M.; Banhart, J.; Manke, I. 3D Mapping of Crystallographic Phase Distribution using Energy-Selective Neutron Tomography. Adv. Mater. 2014, 26, 4069–4073. [Google Scholar] [CrossRef] [PubMed]
- Minniti, T.; Kockelmann, W.; Burca, G.; Kelleher, J.F.; Kabra, S.; Zhang, S.Y.; Pooley, D.E.; Schooneveld, E.M.; Mutamba, Q.; Sykora, J.; et al. Materials analysis opportunities on the new neutron imaging facility IMAT@ISIS. J. Instrum. 2016, 11, C03014. [Google Scholar] [CrossRef]
- Kockelmann, W.; Burca, G.; Kelleher, J.F.; Kabra, S.; Zhang, S.Y.; Rhodes, N.J.; Schooneveld, E.M.; Sykora, J.; Pooley, D.E.; Nightingale, J.B.; et al. Status of the neutron imaging and diffraction instrument IMAT. Phys. Procedia 2015, 69, 71–78. [Google Scholar] [CrossRef]
- Strobl, M. The Scope of the Imaging Instrument Project ODIN at ESS. Phys. Procedia 2015, 69, 18–26. [Google Scholar] [CrossRef]
- Bilheux, H.; Herwig, K.; Keener, S.; Davis, L. Overview of the conceptual design of the future VENUS neutron imaging beam line at the Spallation Neutron Source. Phys. Procedia 2015, 69, 55–59. [Google Scholar] [CrossRef]
- Minniti, T.; Watanabe, K.; Burca, G.; Pooley, D.E.; Kockelmann, W. Characterization of the new neutron imaging and materials science facility IMAT. Nucl. Instrum. Meth. A 2018, A888, 184–195. [Google Scholar] [CrossRef]
- Vallance, C.; Brouard, M.; Lauer, A.; Slater, C.S.; Halford, E.; Winter, B.; King, S.J.; Lee, J.W.L.; Pooley, D.E.; Sedgwick, I.; et al. Fast sensor for time-of-flight imaging applications. Phys. Chem. Chem. Phys. 2014, 16, 383–395. [Google Scholar] [CrossRef] [PubMed]
- Pooley, D.E.; Lee, J.W.L.; Brouard, M.; John, J.J.; Kockelmann, W.; Rhodes, N.J.; Schooneveld, E.M.; Sedgwick, I.; Turchetta, R.; Vallance, C. Development of the GP2 Detector: Modification of the PImMS CMOS Sensor for Energy-Resolved Neutron Radiography. IEEE Trans. Nucl. Sci. 2017, 64, 2970–2981. [Google Scholar] [CrossRef]
- Tremsin, A.S.; Vallerga, J.V.; McPhate, J.B.; Siegmund, O.H.W.; Raffanti, R. High Resolution Photon Counting with MCP-Timepix Quad Parallel Readout Operating at > 1 KHz Frame Rates. IEEE Trans. Nucl. Sci. 2013, 60, 578–585. [Google Scholar] [CrossRef]
- Parker, J.D.; Harada, M.; Hattori, K.; Iwaki, S.; Kabuki, S.; Kishimoto, Y.; Kubo, H.; Kurosawa, S.; Matsuoka, Y.; Miuchi, K.; et al. Spatial resolution of a µPIC−based neutron imaging detector. Nucl. Instrum. Meth. 2013, A726, 155–161. [Google Scholar] [CrossRef]
- Finocchiaro, V.; Aliotta, F.; Tresoldi, D.; Ponterio, R.C.; Vasi, C.S.; Salvato, G. The autofocusing system of the IMAT neutron camera. Rev. Sci. Instrum. 2013, 84, 093701. [Google Scholar] [CrossRef] [PubMed]
- Watanabe, K.; Minniti, T.; Kockelmann, W.; Dalgliesh, R.; Burca, G.; Tremsin, A.S. Characterization of a neutron sensitive MCP/Timepix detector for quantitative image analysis at a pulsed neutron source. Nucl. Instrum. Meth. 2017, A861, 55–63. [Google Scholar] [CrossRef]
- Akeroyd, F.A.; Baker, K.V.L.; Clarke, M.J.; Holt, J.R.; Howells, G.D.; Keymer, D.P.; Löhnert, T.; Moreton-Smith, C.M.; Oram, D.E.; Potter, A.; et al. IBEX—An EPICS based control system for the ISIS pulsed neutron and muon source. In Proceedings of the International Conference on Advanced Neutron Sources (ICANS-XII), Oxford, UK, 27–31 March 2018. in press. [Google Scholar]
- EPICS: Experimental Physics and Industrial Control System URL. Available online: http://www.aps.anl.gov/epics/ (accessed on 2 January 2018).
- EPICS OPI. Available online: http://controlsystemstudio.org/ (accessed on 2 January 2018).
- Uno, S.; Uchida, T.; Sekimoto, M.; Murakami, T.; Miyama, K.; Shoji, M.; Nakano, E.; Koike, T.; Morita, K.; Sato, H.; et al. Two-dimensional Neutron Detector with GEM and its Applications. Phys. Procedia 2012, 26, 142–152. [Google Scholar] [CrossRef]
- Turecek, D.; Holy, T.; Jakubek, J.; Pospisil, S.; Vykydal, Z. Pixelman: A multi-platform data acquisition and processing software package for Medipix2, Timepix and Medipix3 detectors. J. Instrum. 2011, 6, C01046. [Google Scholar] [CrossRef]
- Koennecke, M.; Akeroyd, F.A.; Bernstein, H.J.; Brewster, A.S.; Campbell, S.I.; Clausen, B.; Cottrell, S.; Hoffmann, J.U.; Jemian, P.R.; Maennicke, D.; et al. The NeXus data format. J. Appl. Cryst. 2015, 48, 301–305. [Google Scholar] [CrossRef] [PubMed]
- NeXus Application De_nition NXtomo URL. Available online: http://download.nexusformat.org/sphinx/classes/applications/NXtomo.html (accessed on 2 January 2018).
- Minniti, T. BEATRIX TOF Analysis Code. Private communication 2017.
- Tremsin, A.S. TOF Analysis Code. Private communication 2017.
- Sato, H.; Watanabe, K.; Kiyokawa, K.; Kiyanagi, R.; Hara, K.Y.; Kamiyama, T.; Furusaka, M.; Shinohara, T.; Kiyanagi, Y. Further improvement of the RITS code for pulsed neutron Bragg-edge transmission imaging. Phys. Procedia 2017, 88, 322–330. [Google Scholar] [CrossRef]
- Available online: https://octopusimaging.eu/ (accessed on 2 January 2018).
- Guersoy, D.; Carlo, F.D.; Xiao, X.; Jacobsen, C. Tomopy: A framework for the analysis of synchrotron tomographic data. J. Synchrotron Radiat. 2014, 21, 1188–1193. [Google Scholar] [CrossRef] [PubMed]
- AstraToolbox URL. Available online: https://github.com/astra-toolbox/astra-toolbox/ (accessed on 2 January 2018).
- Available online: https://www.volumegraphics.com/en/products/vgstudio-max.html (accessed on 2 January 2018).
- Available online: https://www.fei.com/software/amira-avizo/ (accessed on 2 January 2018).
- Mantid 2015 Manipulation and Analysis Toolkit for Instrument Data. Mantid Project. Available online: http://dx.doi.org/10.5286/SOFTWARE/MANTID3.5 (accessed on 2 January 2018).
- Savu: Tomography Reconstructon Pipeline. Available online: https://github.com/DiamondLightSource/Savu (accessed on 2 January 2018).
- Atwood, R.C.; Bodey, A.J.; Price, S.W.T.; Basham, M.; Drakopoulos, M. A high-throughput system for high-quality tomographic reconstruction of large datasets at Diamond Light Source. Philos. Trans. R. Soc. A 2015, 373, 20140398. [Google Scholar] [CrossRef] [PubMed]
- Steuwer, A.; Withers, P.J.; Santisteban, J.R.; Edwards, L. Using pulsed neutron transmission for crystalline phase imaging and analysis. J. Appl. Phys. 2005, 97, 074903. [Google Scholar] [CrossRef]
- Boin, M. NXS: A program library for neutron cross section calculations. J. Appl. Crystallogr. 2012, 45, 603–607. [Google Scholar] [CrossRef]
- Burca, G.; Kelleher, J.; Peacock, A.; Hayes, M.; Deliry, P. An integrated tool for Bragg Edge analysis at ISIS. In Proceedings of the NEUWAVE-8 Workshop, Abingdon UK, 12–15 June 2016. [Google Scholar]
- Tremsin, A.S.; Vallerga, J.V.; McPhate, J.B.; Siegmund, O.H.W. Optimization of Timepix count rate capabilities for the applications with a periodic input signal. J. Instrum. 2014, 9, C05026. [Google Scholar] [CrossRef]
- Vitucci, G.; Minniti, T.; Tremsin, A.S.; Kockelmann, W.; Gorini, G. Investigation of image distortion due to MCP electronic readout misalignment and correction via customized GUI application. J. Instrum. 2018. under review. [Google Scholar]
- Micieli, D.; Minniti, T.; Formoso, V.; Kockelmann, W.; Gorini, G. A comparative study of reconstruction methods applied to neutron tomography. J. Instrum. 2017. under review. [Google Scholar]


| Camera/Detector Type | Camera/Detector Parameters | Energy-Selection Options and Special Features |
|---|---|---|
| Berkeley MCP (Timepix 2) |
| |
| Field of View (mm2) | 28 × 28 | |
| Pixel Size (μm) | 55 | |
| Number of Pixels | 512 × 512 | |
| Number of Time Bins | 3100 | |
| Smallest Time Bin (ns) | ~10 | |
| Registers per pixel | 1 | |
| Detection efficiency | up to 40% for cold neutrons | |
| Oxford-ISIS GP2 |
| |
| Field of View (mm2) | 22.7 × 22.7 | |
| Pixel Size (μm) | 70 | |
| Number of Pixels | 324 × 324 | |
| Number of Time Bins | 4096 | |
| Smallest Time Bin (ns) | 12.5 | |
| Registers per pixel | 4 | |
| Detection efficiency | 7.5% at 2.5 Å | |
| Messina Tomography |
| |
| Camera Box | ||
| Field of View (mm2) | 60 × 60–210 × 210 | |
| Effective Pixel Size (2048) (μm) | 29–103 | |
| ANDOR Ikon-L 936 CCD | 2048 × 2048; cooled (i) | |
| ANDOR Zyla sCMOS 4.2 | 2048 × 2048 (ii) | |
| Plus | ||
| ANDOR iStar DH712 CCD | 512 × 512; gatable (iii) |
| Camera/Detector Type | Camera/Detector Parameters | Energy-Selection |
|---|---|---|
| Berkeley MCP (Timepix 3) | See MCP above; sparsified readout; 10,000 time bins; enlarged field of view, e.g., 28 × 110 mm2 |
|
| Tiled MCP | ||
| Tiled Oxford-ISIS GP2 | See GP2 above; enlarged field of view, e.g., 22 × 194 mm2 |
|
| nGEM gas electron multiplier (e.g., [39 ]) |
| |
| Field of View (mm2) | 100 × 100 | |
| Number of pixels | 125 × 125 | |
| Effective Pixel Size (μm) | ~800 | |
| Additional options for Messina Optical Tomography Box |
| |
| Gated CCD or CMOS | ||
| Field of View (mm2) | 60 × 60–210 × 210 | |
| Number of pixels | 2048 × 2048 | |
| Effective Pixel Size (μm) | 29–103 | |
| High Frame Rate Camera | 105 frames per second; field of view e.g., ~30 × 30 mm2 |
|
| High-Resolution Camera |
| |
| Field of View (mm2) | 30 × 30 | |
| Number of Pixels | 4096 × 4096; cooled | |
| Effective Pixel Size (μm) | 7.3 | |
| Neutron Source | 10 Hz Pulsed Source | ||
|---|---|---|---|
| Neutron spectrum | Cold spectrum, with a maximum at 2.6 Å; 100 × 100 mm2 view onto the LH2-moderator @ 18 K | ||
| Neutron transport | 44 m, m = 3, straight supermirror guide with square (95 × 95 mm2) cross section | ||
| Single/double frame bandwidth | 6 Å/12 Å | ||
| Flight path to pinhole | 46 m | ||
| Flight path to sample | 56 m (centre of sample positioning system) | ||
| L/D | L/D: 2000, 1000, 500, 250, 125 (nominal) L/D: …, 1150, 510, 245, … (measured) | ||
| Maximum neutron flux | 3.8 107 n/cm2/s (measured, for 100 × 100 mm2 pinhole) | ||
| Maximum field of view @ 56 m | 185 × 185 mm2 | ||
| Wavelength resolution | Δλ/λ < 0.4% (<2 Å) (measured) Δλ/λ < 0.8% (>2 Å) (measured) | ||
| Spatial resolution and data collection time (ballpark numbers, for L/D ~ 250) | |||
| White-beam | Pink-beam | TOF (Bragg edge) | |
| δλ/λ ~ 25% | δλ/λ < 0.8% | ||
| Collection time (hours) | 4–8 | 8–16 | 2–6 |
| Spatial resolution (μm) | 50 (3D) | 100 (3D) | 200 (2D) |
| Field of view (μm) | 200 × 200 | 200 × 200 | 20 × 20 |
| Calculated Bragg Edge Positions: | |||||
|---|---|---|---|---|---|
| (hkl) (Cu) | λ (A) | T (μs) | (hkl)(Fe) | λ (A) | T (μs) |
| (1 1 1) | 4.174 | 59,465 | (1 1 0) | 4.051 | 57,710 |
| (2 0 0) | 3.615 | 51,500 | (2 0 0) | 2.865 | 40,815 |
| (2 2 0) | 2.556 | 36,410 | (2 1 1) | 2.339 | 33,320 |
| (3 1 1) | 2.180 | 31,060 | (2 2 0) | 2.026 | 28,860 |
| TOFmin | TOFmax | n | Time bin | number of time slices | |
| (s) | (s) | (μs) | |||
| 12 × 10−3 | 32.68 × 10−3 | 8 | 40.96 | 504 | |
| 33 × 10−3 | 57.68 × 10−3 | 9 | 20.48 | 1205 | |
| 58 × 10−3 | 95 × 10−3 | 8 | 40.96 | 903 | |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kockelmann, W.; Minniti, T.; Pooley, D.E.; Burca, G.; Ramadhan, R.; Akeroyd, F.A.; Howells, G.D.; Moreton-Smith, C.; Keymer, D.P.; Kelleher, J.; et al. Time-of-Flight Neutron Imaging on IMAT@ISIS: A New User Facility for Materials Science. J. Imaging 2018, 4, 47. https://doi.org/10.3390/jimaging4030047
Kockelmann W, Minniti T, Pooley DE, Burca G, Ramadhan R, Akeroyd FA, Howells GD, Moreton-Smith C, Keymer DP, Kelleher J, et al. Time-of-Flight Neutron Imaging on IMAT@ISIS: A New User Facility for Materials Science. Journal of Imaging. 2018; 4(3):47. https://doi.org/10.3390/jimaging4030047
Chicago/Turabian StyleKockelmann, Winfried, Triestino Minniti, Daniel E. Pooley, Genoveva Burca, Ranggi Ramadhan, Freddie A. Akeroyd, Gareth D. Howells, Chris Moreton-Smith, David P. Keymer, Joe Kelleher, and et al. 2018. "Time-of-Flight Neutron Imaging on IMAT@ISIS: A New User Facility for Materials Science" Journal of Imaging 4, no. 3: 47. https://doi.org/10.3390/jimaging4030047
APA StyleKockelmann, W., Minniti, T., Pooley, D. E., Burca, G., Ramadhan, R., Akeroyd, F. A., Howells, G. D., Moreton-Smith, C., Keymer, D. P., Kelleher, J., Kabra, S., Lee, T. L., Ziesche, R., Reid, A., Vitucci, G., Gorini, G., Micieli, D., Agostino, R. G., Formoso, V., ... Nightingale, J. (2018). Time-of-Flight Neutron Imaging on IMAT@ISIS: A New User Facility for Materials Science. Journal of Imaging, 4(3), 47. https://doi.org/10.3390/jimaging4030047










