Mechanical Behaviour of Stainless Steels under Dynamic Loading: An Investigation with Thermal Methods
Abstract
:1. Introduction and State of Art: Infrared Detection Based Approaches
1.1. Thermal Methods for Assessing Crack Propagation in Material
1.2. Thermal Methods for Fatigue Limit Assessment
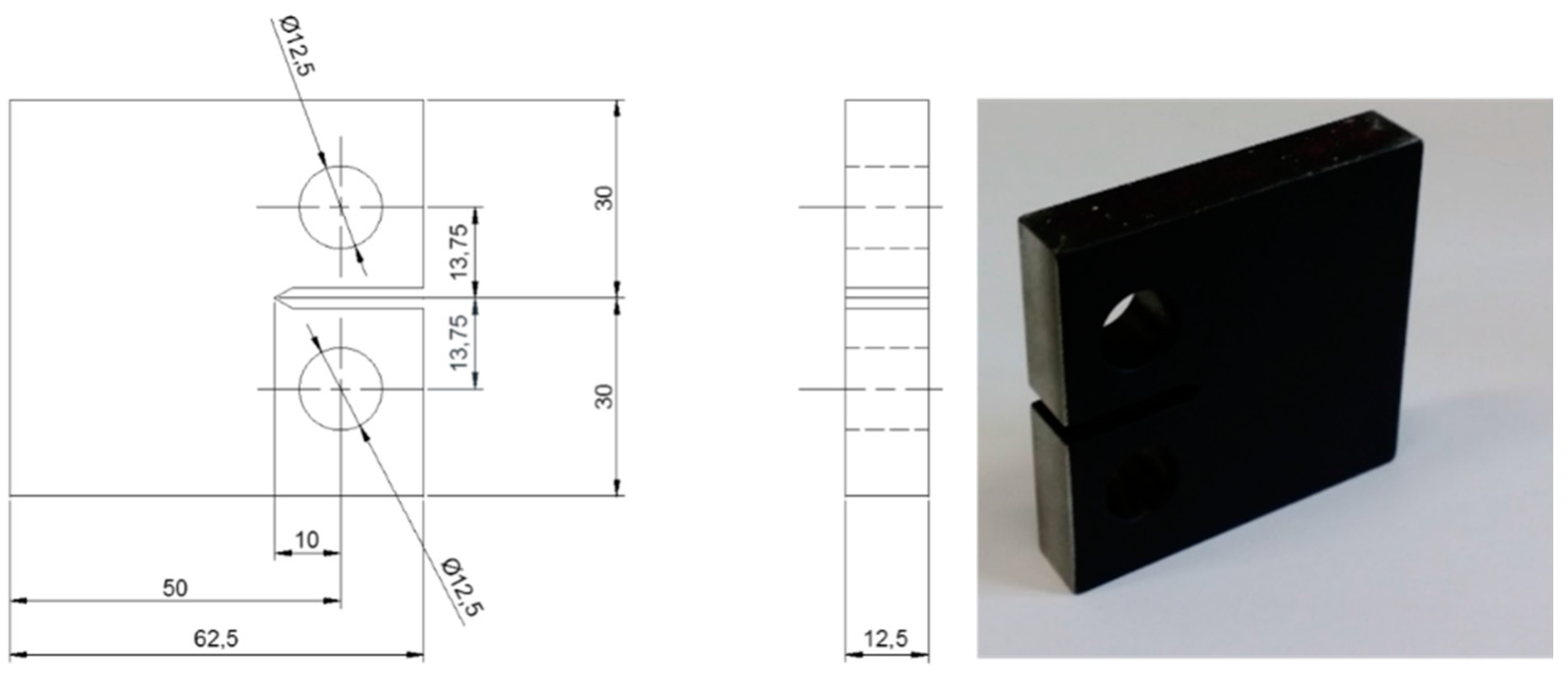
2. Material and Methods
- The Infrared camera used was FLIR IR X6540 SC (Wilsonville, OR, USA) with an InSb detector (640 × 512 pixels) and a full-frame acquisition rate of 123 Hz.
- MTS model 370 servo-hydraulic fatigue machine with a 100 kN capacity.
2.1. Fracture Mechanics Test Procedure
2.2. Fatigue Test Procedure
3. Data Processing
- The acquisition of the thermographic sequence of approximately 1000 frames acquired in 10 s.
- The signal analysis for assessing φ, S2 pixel by pixel matrix.
- Applying a Median 2D-smoothing on the data matrix obtained by IRTA® software,
- Reducing data matrix to refer the analysis only to the gauge length area (for fatigue tests). The same data matrix has been chosen for φ, S2 parameters.
- Evaluating the standard deviation of signal of the φ, S2 for application of the threshold method [4] to assess the fatigue limit of material.
4. Results and Discussions
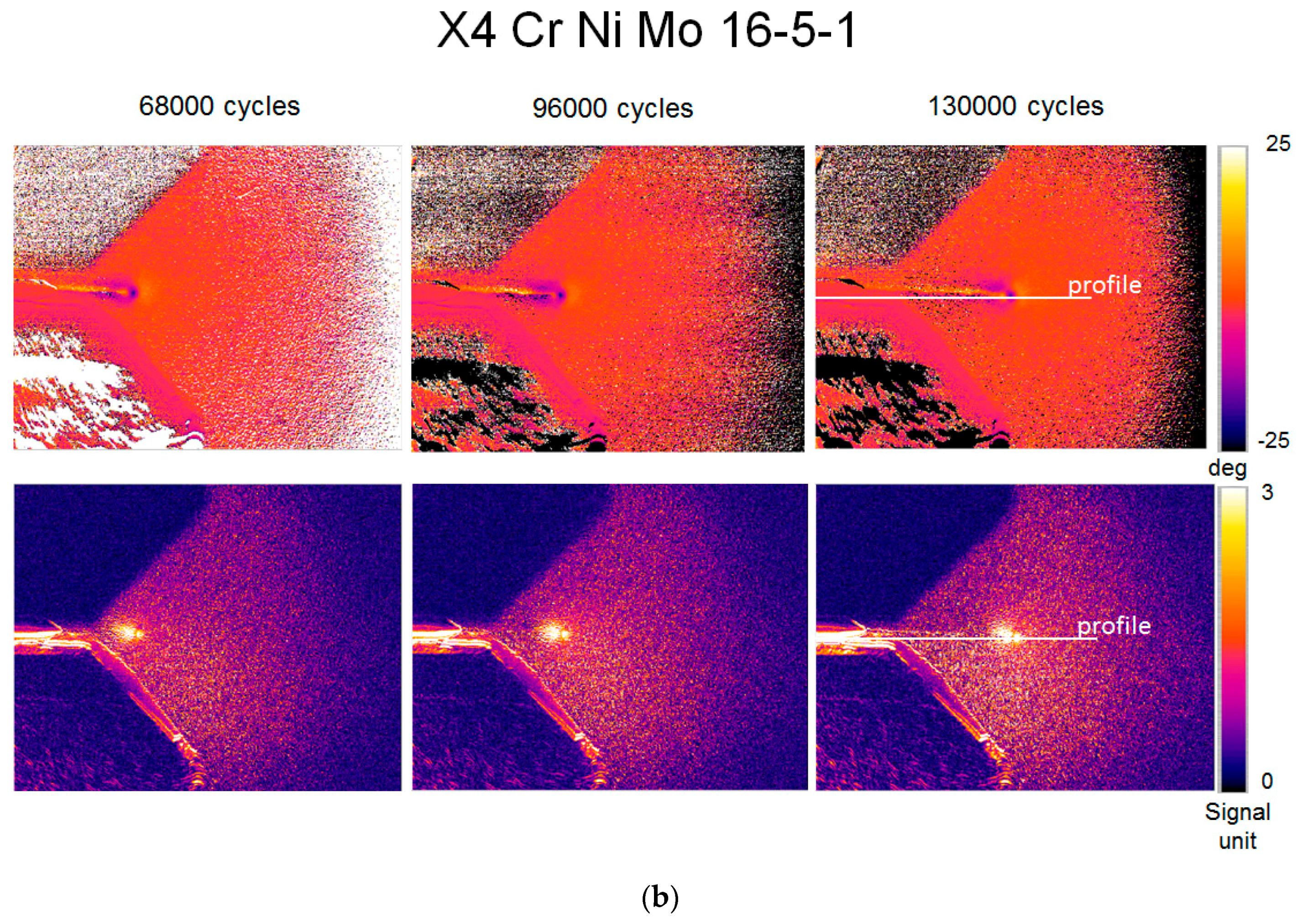
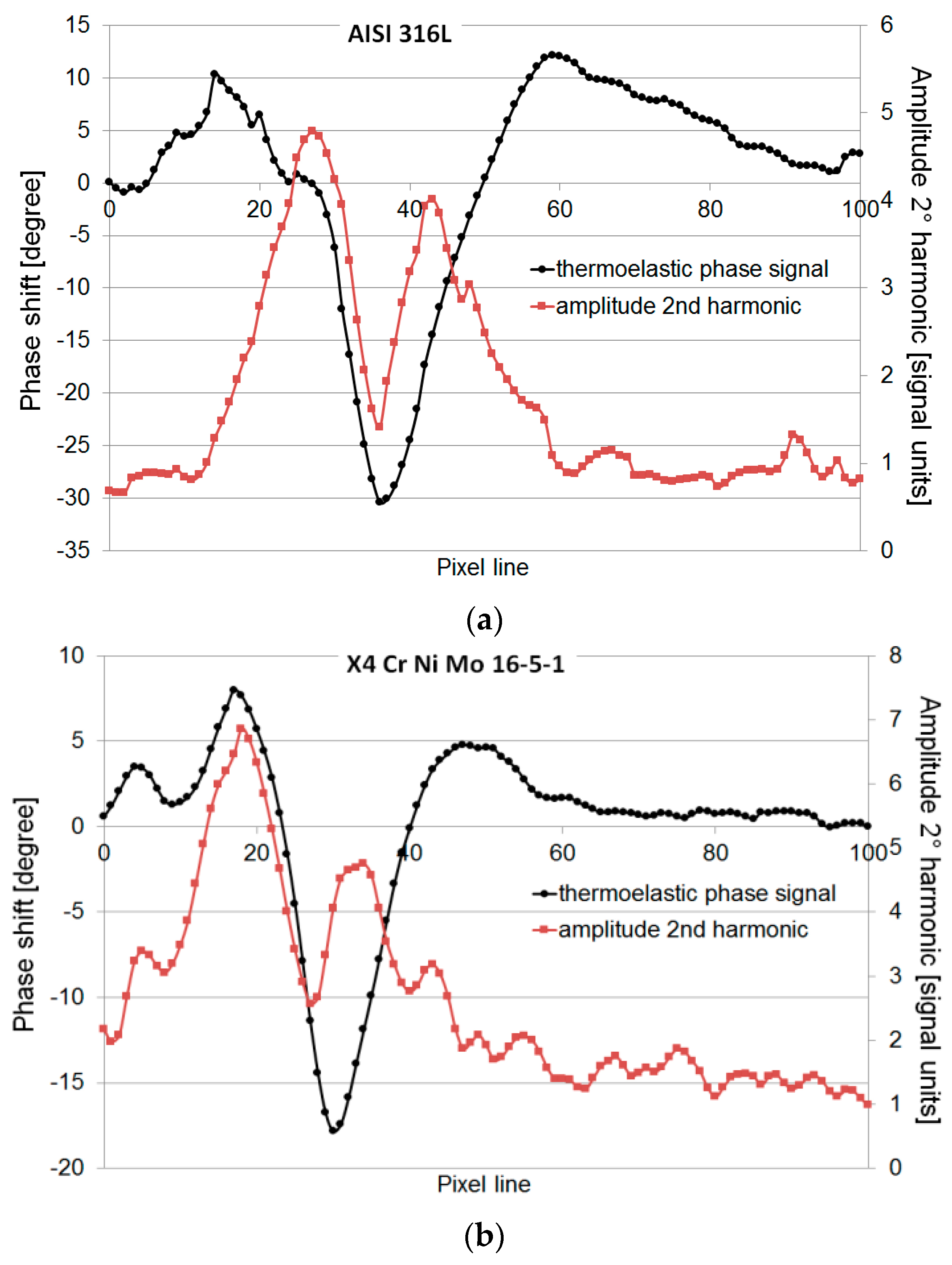
4.1. Fracture Mechanics: Crack Propagation by Studying Dissipative Heat Source
- to determine the crack tip of both materials;
- to highlight different phase shift intervals for AISI316L and X4 Cr Ni Mo 16-5-1 steels.
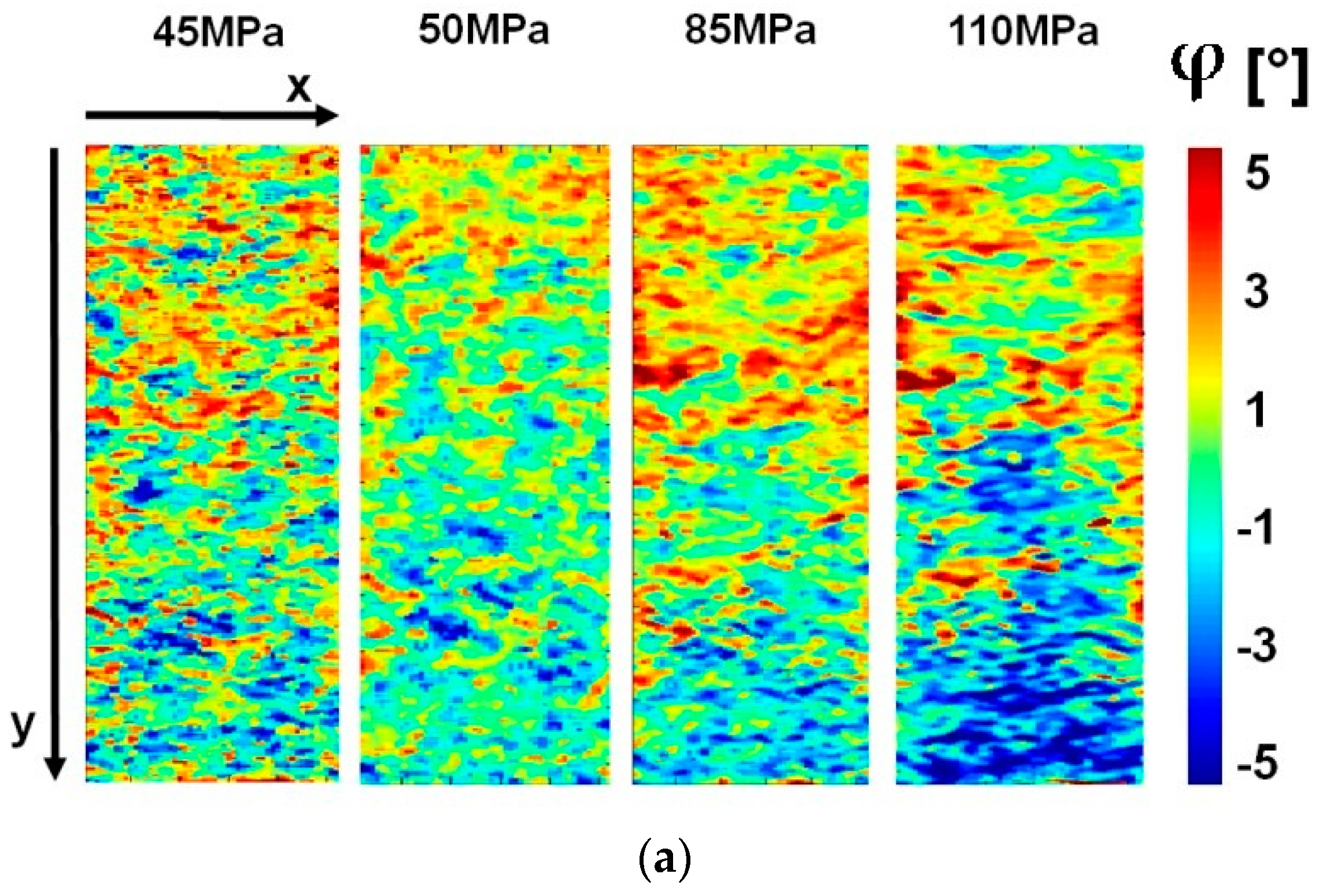
4.2. FATIGUE: Damage Process Assessment
4.3. FATIGUE: Fatigue Limit Evaluation
5. Conclusions
- their capability of assessing and monitoring damage behaviour, they provide more information than other methods;
- the simple algorithm performed, the adopted method provides a less time consuming test and data analysis;
- the simple sample preparation, requiring only the application of a thin removable layer of matt black on the surface, the proposed method may be applied successfully on real components during their operating life, without damaging or wasting them.
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Luong, M.P. Infrared Thermographic scanning of fatigue in metals. Nucl. Eng. Des. 1995, 158, 363–373. [Google Scholar] [CrossRef]
- La Rosa, G.; Risitano, A. Thermographic methodology for the rapid determination of the fatigue limit of materials and mechanical components. Int. J. Fatigue 2000, 22, 65–73. [Google Scholar] [CrossRef]
- Galietti, U.; Palumbo, D. Application of Thermal Methods for Characterization of Steel Welded Joints; EPJ Web of Conferences 2010; EDP Sciences: Les Ulis, France, 2010; Volume 6. [Google Scholar]
- De Finis, R.; Palumbo, D.; Ancona, F.; Galietti, U. Fatigue limit evaluation of various martensitic stainless steels with new robust thermographic data analysis. Int. J. Fatigue 2015, 74, 88–96. [Google Scholar] [CrossRef]
- Harwood, N.; Cummings, W. Thermoelastic Stress Analysis; CRC Press: Bristol, PA, USA, 1991. [Google Scholar]
- Stanley, P. Applications and potential of thermoelastic stress analysis. J. Mater. Process. Technol. 1997, 64, 359–370. [Google Scholar] [CrossRef]
- Dulieu-Barton, J.M. Introduction to thermoelastic stress analysis. Strain 1999, 35, 35–39. [Google Scholar] [CrossRef]
- Pitarresi, G.; Patterson, E.A. A review of the general theory of thermoelastic stress analysis. J. Strain Anal. Eng. Des. 2003, 38, 405–413. [Google Scholar] [CrossRef]
- Wang, W.J.; Dulieu-Barton, J.M.; Li, Q. Assessment of non-adiabatic behaviour in thermoelastic stress analysis of small scale components. Exp. Mech. 2010, 50, 449–461. [Google Scholar] [CrossRef]
- Palumbo, D.; Galietti, U. Data Correction for Thermoelastic Stress Analysis on Titanium Components. Exp. Mech. 2016. [Google Scholar] [CrossRef]
- Enke, N.F.; Sandor, B.I. Cyclic plasticity analysis by differential infrared thermography. In Proceedings of the VII International Congress on Experimental Mechanics, Cincinnati, OH, USA, 7 June 1988; pp. 830–835.
- Sakagami, T.; Kubo, S.; Tamura, E.; Nishimura, T. Identification of plastic-zone based on double frequency lock-in thermographic temperature measurement. In Proceedings of the International Conference of Fracture ICF11, Torino, Italy, 20–25 March 2005.
- Krapez, J.K.; Pacou, D.; Gardette, G. Lock-In Thermography and Fatigue Limit of Metals. In Proceedings of the Quantitative Infrared Thermography (QIRT), Reims, France, 18–21 July 2000.
- Palumbo, D.; Galietti, U. Characterization of Steel Welded Joints by Infrared Thermographic Methods. Quant. Infrared Thermogr. J. 2014, 11, 29–42. [Google Scholar] [CrossRef]
- Kim, K.S.; Jung, H.C.; Akhter, N.; Chang, H.S.; Kim, M.K.; Kim, D.S.; Jung, D.W. Thermoelastic Stress Analysis of a Fatigue Specimen using the Lock-in Infrared Thermography. In Proceedings of the 7th International Conference on NDE in Relation to Structural Integrity for Nuclear and Pressurized Components, Yokohama, Japan, 12–15 May 2009.
- Shiozawa, D.; Inagawa, T.; Washio, T.; Sakagami, T. Fatigue limit estimation of stainless steels with new dissipated energy data analysis. Procedia Struct. Integr. 2016, 2, 2091–2096. [Google Scholar] [CrossRef]
- Talemi, R.; Chhith, S.; De Waele, W. On effect of pre-bending process on low cycle fatigue behaviour of high strength steel using lock-in thermography. Procedia Struct. Integr. 2016, 2, 3135–3142. [Google Scholar] [CrossRef]
- Li, X.D.; Zhang, H.; Wu, D.L.; Liu, X.; Liu, J.Y. Adopting lock-in infrared thermography technique for rapid determination of fatigue limit of aluminum alloy riveted component and affection to determined result caused by initial stress. Int. J. Fatigue 2012, 36, 18–23. [Google Scholar] [CrossRef]
- Fan, J.; Guo, X.; Wu, C. A new application of the infrared thermography for fatigue evaluation and damage assessment. Int. J. Fatigue 2012, 44, 1–7. [Google Scholar] [CrossRef]
- Kim, W.T.; Choi, M.J.; Huh, Y.H.; Eom, S.J. Measurement of Thermal Stress And Prediction of Fatigue for Sts Using Lock-In Thermography. In Proceedings of the 12th A-PCNDT 2006—Asia-Pacific Conference on NDT, Auckland, New Zealand, 5–10 November 2006.
- Galietti, U.; Palumbo, D.; de Finis, R.; Ancona, F. Fatigue Damage Evaluation with New Thermal Methods. In Proceedings of the 3th International Workshop On Advanced Infrared Technology And Applications, Tourin, Italy, 11–14 September 2013.
- Palumbo, D.; Galietti, U. Thermoelastic Phase Analysis (TPA): A new method for fatigue behaviour analysis of steels. Fatigue Fract. Eng. Mater. Struct. 2016. [Google Scholar] [CrossRef]
- Fedorova, A.Y.U.; Bannikov, M.V.; Plekhov, O.A.; Plekhova, E.V. Infrared thermography study of the fatigue crack propagation. Frattura ed Integrità Strutturale 2012, 21, 46–53. [Google Scholar]
- Diaz, F.A.; Patterson, E.A.; Yates, R.A. Some improvements in the analysis of fatigue cracks using thermoelasticity. Int. J. Fatigue 2004, 26, 365–387. [Google Scholar] [CrossRef]
- Diaz, F.A.; Patterson, E.A.; Yates, R.A. Application of thermoelastic stress analysis for the experimental evaluation of the effective stress intensity factor. Frattura ed Integrità Strutturale 2013, 25, 109–117. [Google Scholar]
- Tomlinson, R.A.; Olden, E.J. Thermoelasticity for the analysis of crack tip stress fields—A review. Strain 1999, 7, 35–49. [Google Scholar] [CrossRef]
- Tomlinson, R.A.; Patterson, E.A. Examination of Crack Tip Plasticity Using Thermoelastic Stress Analysis. In Thermomechanics and Infra-Red Imaging; Society for Experimental Mechanics Series; Springer: New York, NY, USA, 2011; Volume 7, pp. 123–130. [Google Scholar]
- Diaz, F.A.; Patterson, E.A.; Yates, R.A. Differential Thermography Reveals Crack Tip Behaviour? In Proceedings of the SEM Annual Conference on Experimental and Applied Mechanics Society for Experimental Mechanics, Portland, OR, USA, 6–9 June 2005; pp. 1413–1419.
- Ancona, F.; de Finis, R.; Demelio, G.P.; Galietti, U.; Palumbo, D. Study of the plastic behavior around the crack tip by means of thermal methods. In Proceedings of the Structural Integrity Procedia of the 21st European Conference on Fracture, ECF21, Catania, Italy, 20–24 June 2016.
- Ancona, F.; Palumbo, D.; de Finis, R.; Demelio, G.P.; Galietti, U. Automatic procedure for evaluating the Paris Law of martensitic and austenitic steels by means of thermal methods. Eng. Fract. Mech. 2016. [Google Scholar] [CrossRef]
- Galietti, U.; Palumbo, D.; De Finis, R.; Ancona, F. Fatigue Damage Evaluation of Martensitic Stainless Steel by Means of Thermal Methods. In Proceedings of the National Conference IGF XXII, Rome, Italy, 1–3 July 2013; pp. 80–91.
- Bar, J. Determination of dissipated Energy in Fatigue Crack Propagation Experiments with Lock-in Thermography and Heat Flow Measurements. Procedia Struct. Integr. 2016, 2, 2105–2112. [Google Scholar] [CrossRef]
- Morabito, A.E.; Chrysochoos, A.; Dattoma, V.; Galietti, U. Analysis of heat sources accompanying the fatigue of 2024 T3 aluminium alloys. Int. J. Fatigue 2007, 29, 977–984. [Google Scholar] [CrossRef]
- Meneghetti, G. Analysis of the Fatigue Strength of a Stainless Steel Based on the Energy Dissipation. Int. J. Fatigue 2007, 29, 81–94. [Google Scholar] [CrossRef]
- Boulanger, T.; Chrysochoos, A.; Mabru, C.; Galtier, C. Calorimetric analysis of dissipative and thermoelastic effects associated with the fatigue behavior of steels. Int. J. Fatigue 2006, 26, 221–229. [Google Scholar] [CrossRef]
- Matweb. Available online: http://www.matweb.com/ (accessed on 4 November 2016).
- Industeel Belgium, ArcelorMittal. Technical Data Sheet VIRGO 39—Ed 28.07. 2003. Available online: http://www.industeel.info/products/stainless-steels/ferritic-martensitic/virgo-39/ (accessed on 4 November 2016).
- ASTM E 647–00. Standard Test Method for Measurement of Fatigue Crack Growth Rates, 2000. Available online: http://www.astm.org/Standards/E647 (accessed on 4 November 2016).
- ASTM E E466-96. Standard Practice for Conducting Force Controlled Constant Amplitude Axial Fatigue Tests of Metallic Materials. 1996. Available online: http://www.astm.org/DATABASE.CART/HISTORICAL/E466-96.htm (accessed on 4 November 2016).
- Delpueyo, D.; Balandraud, X.; Grédiac, M. Applying infrared thermography to analyse martensitic microstructures in a Cu–Al–Be shape-memory alloy subjected to a cyclic loading. Mater. Sci. Eng. A Struct. 2011, 528, 8249–8258. [Google Scholar] [CrossRef]
- Arghavani, J.; Auricchio, F.; Naghdabadi, R.; Reali, A.; Sohrabpour, S. A 3-D phenomenological constitutive model for shape memory alloys under multiaxial loadings. Int. J. Plast. 2010, 26, 976–991. [Google Scholar] [CrossRef]
- Kan, Q.; Kang, G. Constitutive model for uniaxial transformation ratchetting of super-elastic NiTi shape memory alloy at room temperature. Int. J. Plast. 2009, 26, 441–465. [Google Scholar] [CrossRef]
- Ortin, J.; Planes, A. Thermodynamics of thermoelastic martensitic transformations. Acta Metall. 1989, 37, 1433–1441. [Google Scholar] [CrossRef]
- Pan, H.; Thamburaja, P.; Chau, F.S. Multi-axial behavior of shape memory alloys undergoing martensitic reorientation and detwinning. Int. J. Plast. 2007, 23, 711–732. [Google Scholar] [CrossRef]
- Juvinall, R.; Marshek, K. Fundamental of Machine Component Design; Edizioni ETS: Pisa, Italy, 1994. [Google Scholar]




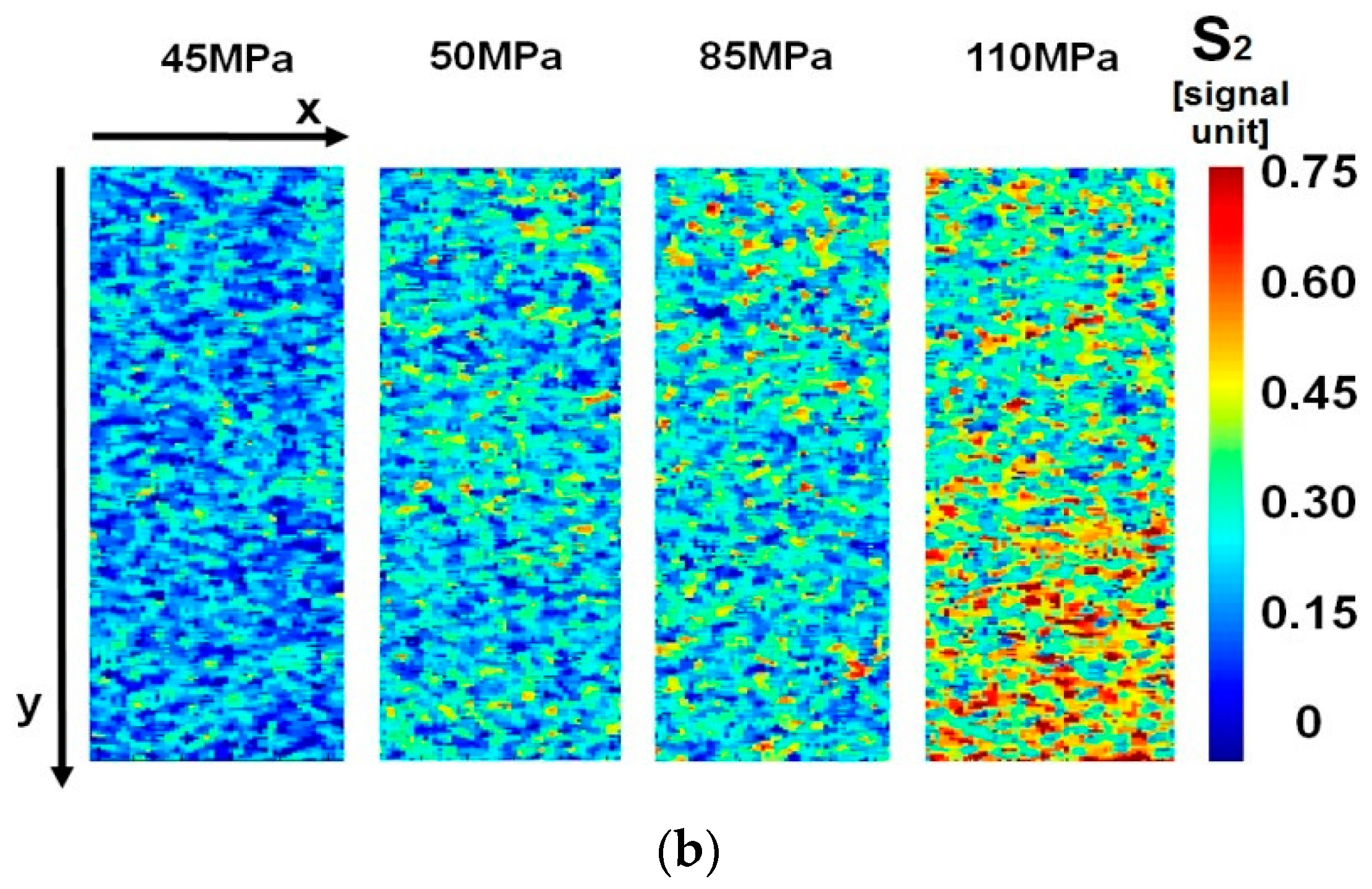
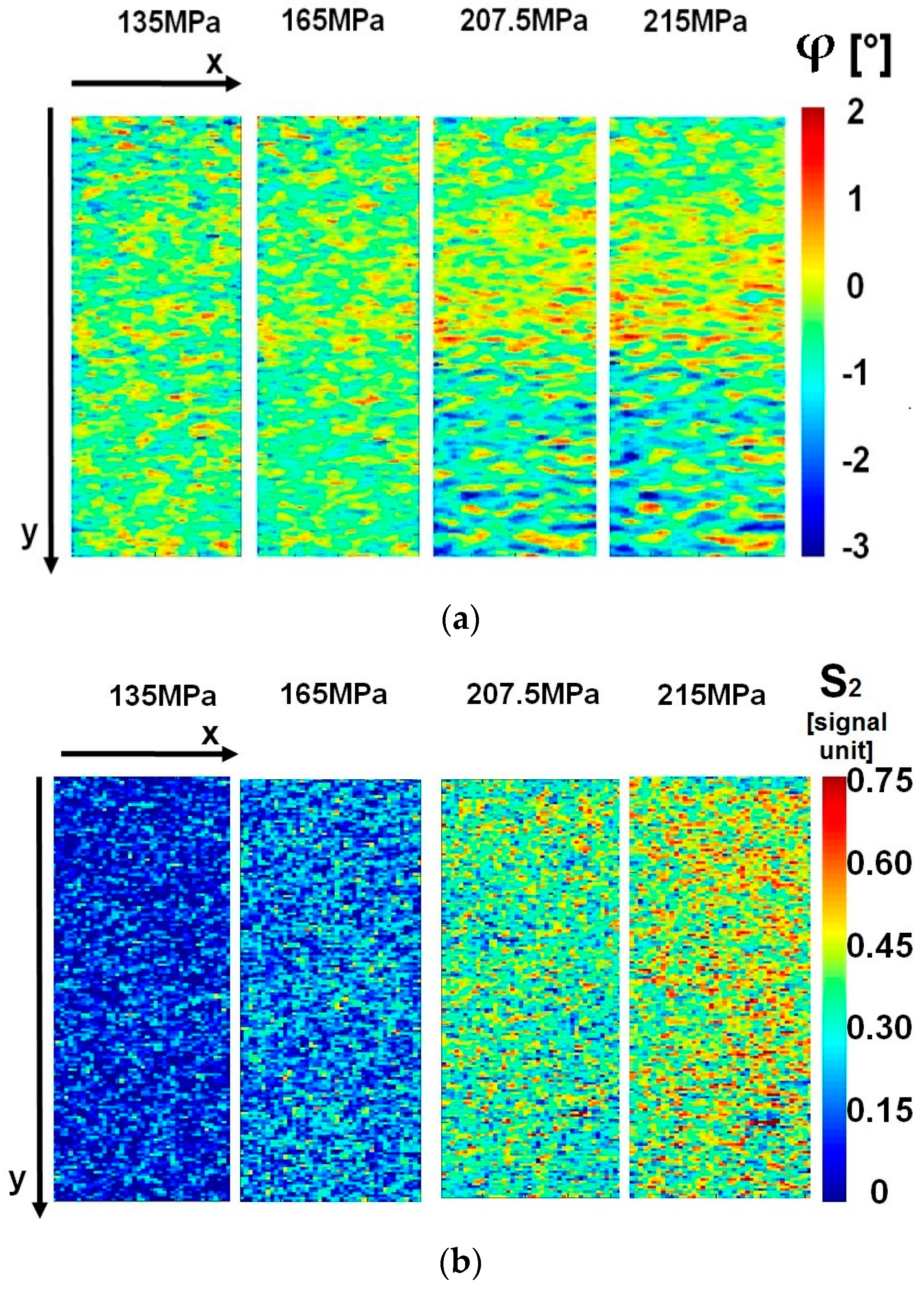

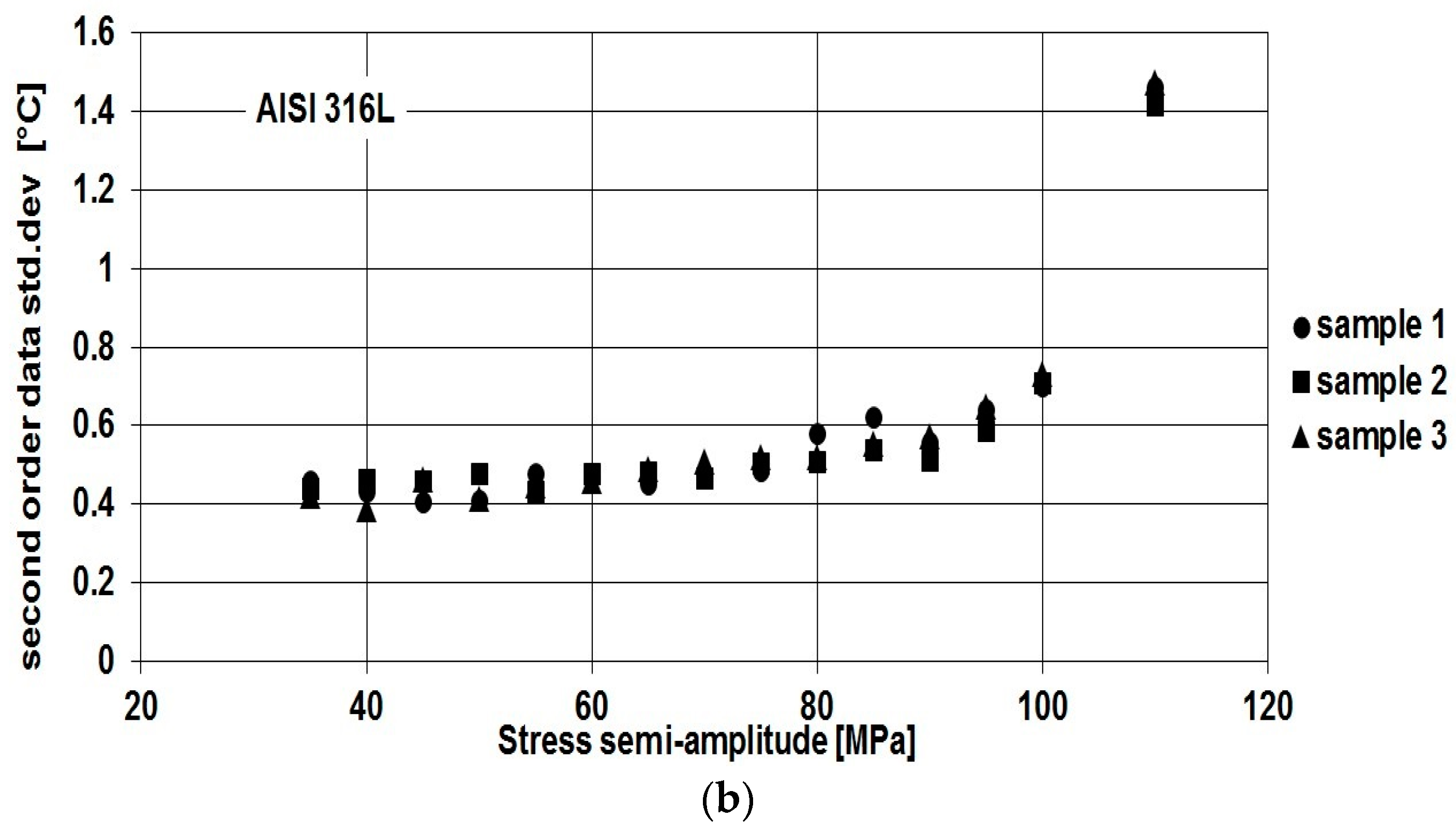
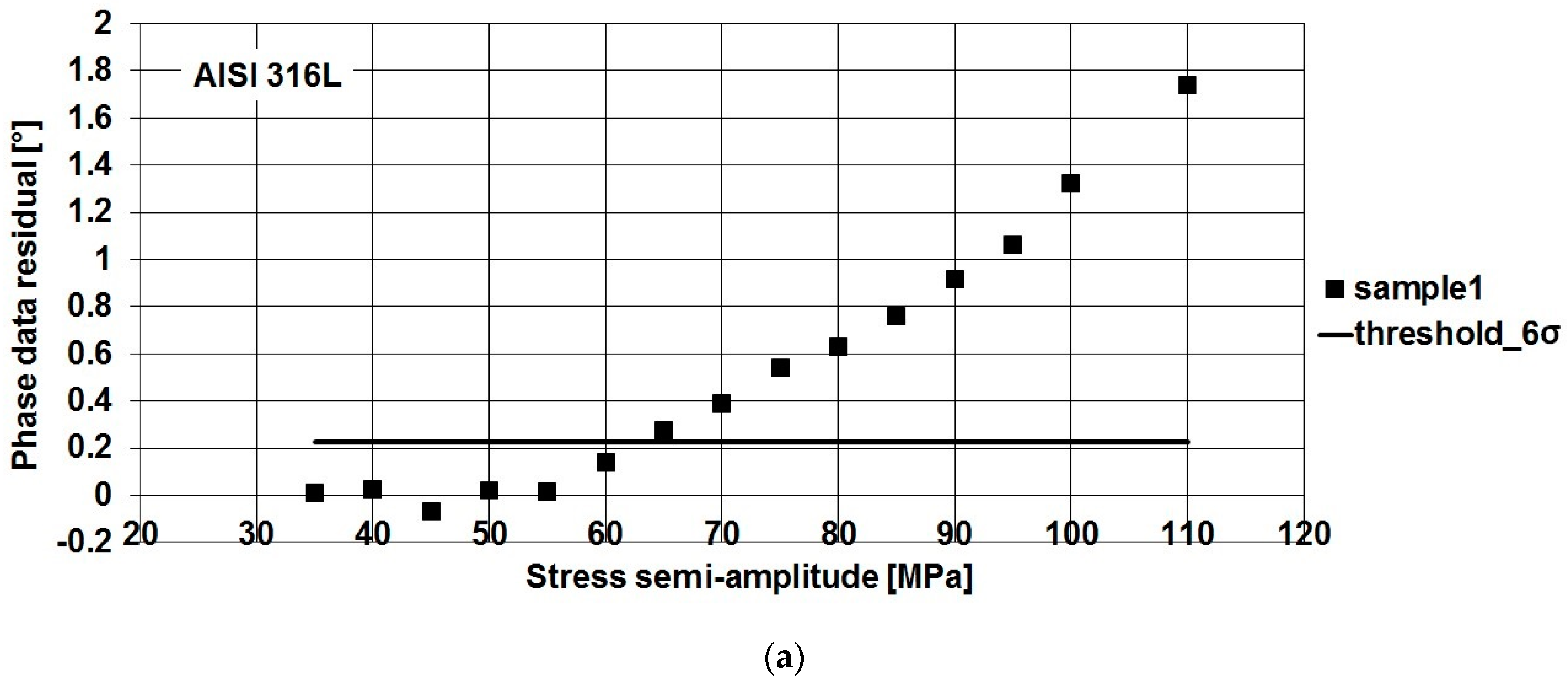

| Material | ΔP (kN) |
|---|---|
| X4 Cr-Ni-Mo 16-5-1 | 12.4 |
| AISI 316L | 9.90 |
| (a) | ||
| Loading Level | Δσ/2 (MPa) | Δσ (MPa) |
| 1 | 35.00 | 70.00 |
| 2 | 40.00 | 80.00 |
| 3 | 45.00 | 90.00 |
| 4 | 50.00 | 100.00 |
| 5 | 55.00 | 110.00 |
| 6 | 60.00 | 120.00 |
| 7 | 65.00 | 130.00 |
| 8 | 70.00 | 140.00 |
| 9 | 75.00 | 150.00 |
| 10 | 80.00 | 160.00 |
| 11 | 85.00 | 170.00 |
| 12 | 90.00 | 180.00 |
| 13 | 95.00 | 190.00 |
| 14 | 100.00 | 200.00 |
| 15 | 110.00 | 220.00 |
| (b) | ||
| Loading Level | Δσ/2 (MPa) | Δσ (MPa) |
| 1 | 25.00 | 50.00 |
| 2 | 45.00 | 90.00 |
| 3 | 65.00 | 130.00 |
| 4 | 85.00 | 170.00 |
| 5 | 105.00 | 210.00 |
| 6 | 120.00 | 240.00 |
| 7 | 135.00 | 270.00 |
| 8 | 150.00 | 300.00 |
| 9 | 165.00 | 330.00 |
| 10 | 180.00 | 360.00 |
| 11 | 200.00 | 400.00 |
| 12 | 207.50 | 415.00 |
| 13 | 215.00 | 430.00 |
| 14 | 225.00 | 450.00 |
| 15 | 250.00 | 500.00 |
| Sample | AISI 316L 1 | X4 Cr Ni Mo 16-5-1 1 | ||
|---|---|---|---|---|
| φ | S2 | φ | S2 | |
| 1 | 65.00 | 95.00 | 180.00 | 180.00 |
| 2 | 75.00 | 95.00 | 165.00 | 190.00 |
| 3 | 90.00 | 100.00 | 190.00 | 185.00 |
| MEAN | 76.67 | 96.67 | 178.33 | 185.00 |
| Standard Deviation. | 12.58 | 2.89 | 12.58 | 5.00 |
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
De Finis, R.; Palumbo, D.; Galietti, U. Mechanical Behaviour of Stainless Steels under Dynamic Loading: An Investigation with Thermal Methods. J. Imaging 2016, 2, 32. https://doi.org/10.3390/jimaging2040032
De Finis R, Palumbo D, Galietti U. Mechanical Behaviour of Stainless Steels under Dynamic Loading: An Investigation with Thermal Methods. Journal of Imaging. 2016; 2(4):32. https://doi.org/10.3390/jimaging2040032
Chicago/Turabian StyleDe Finis, Rosa, Davide Palumbo, and Umberto Galietti. 2016. "Mechanical Behaviour of Stainless Steels under Dynamic Loading: An Investigation with Thermal Methods" Journal of Imaging 2, no. 4: 32. https://doi.org/10.3390/jimaging2040032
APA StyleDe Finis, R., Palumbo, D., & Galietti, U. (2016). Mechanical Behaviour of Stainless Steels under Dynamic Loading: An Investigation with Thermal Methods. Journal of Imaging, 2(4), 32. https://doi.org/10.3390/jimaging2040032








