Simulation and Optimization of Lithium-Ion Battery Thermal Management System Integrating Composite Phase Change Material, Flat Heat Pipe and Liquid Cooling
Abstract
1. Introduction
2. Physical Model and Mathematical Formulation
2.1. Physical Model of Battery Modules
2.2. Mathematical Formulation
2.3. Boundary and Initial Conditions
3. Model Verification and Comparison
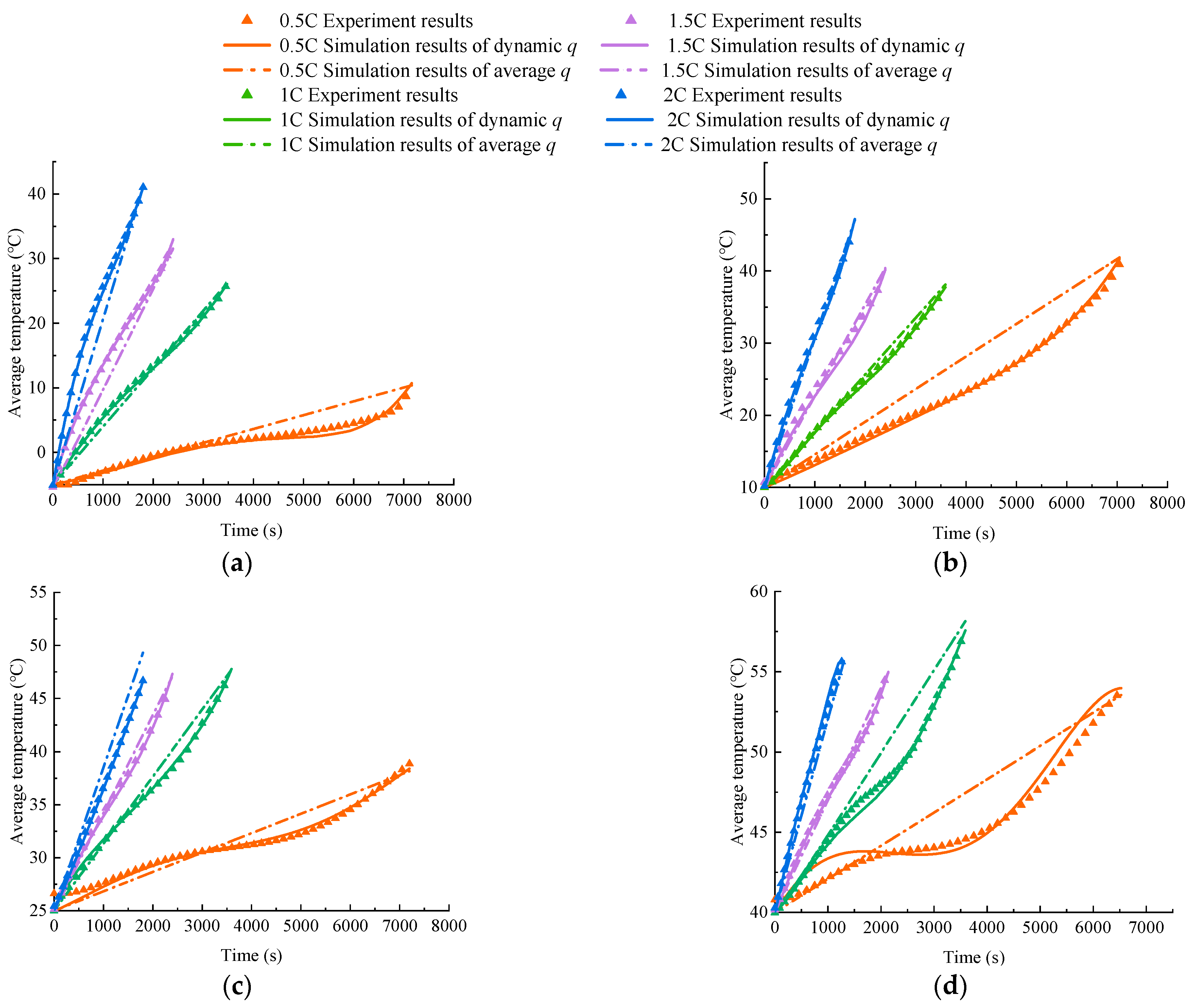
3.1. Verification of Heat Generation Rate
3.2. Result Comparison of Battery Modules
4. Scheme Design and Parameter Discussion
4.1. Scheme Design
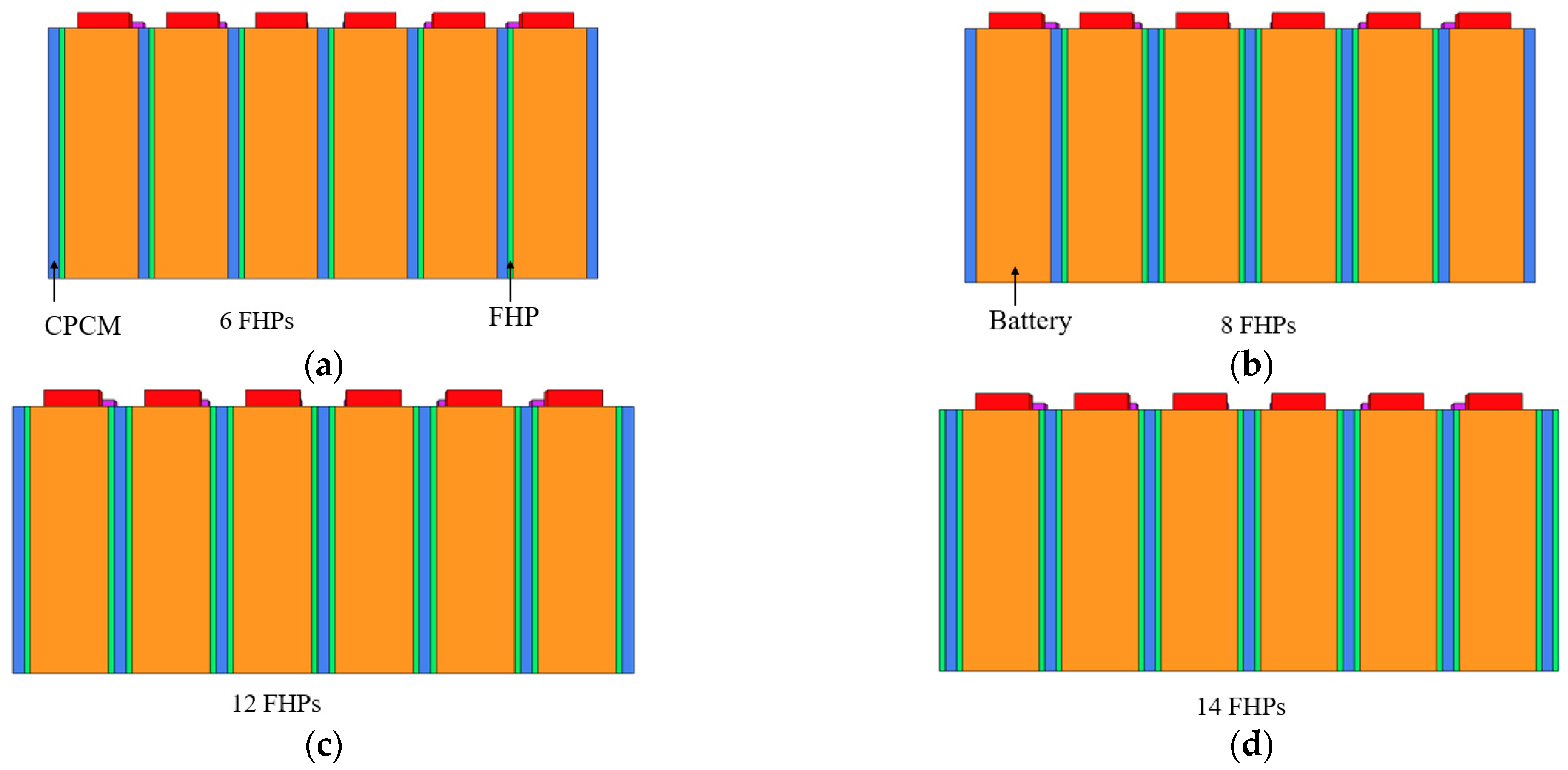
4.2. Effect of FHP Number
4.3. Effect of Coolant Temperature
4.4. Effect of Coolant Pipe Number
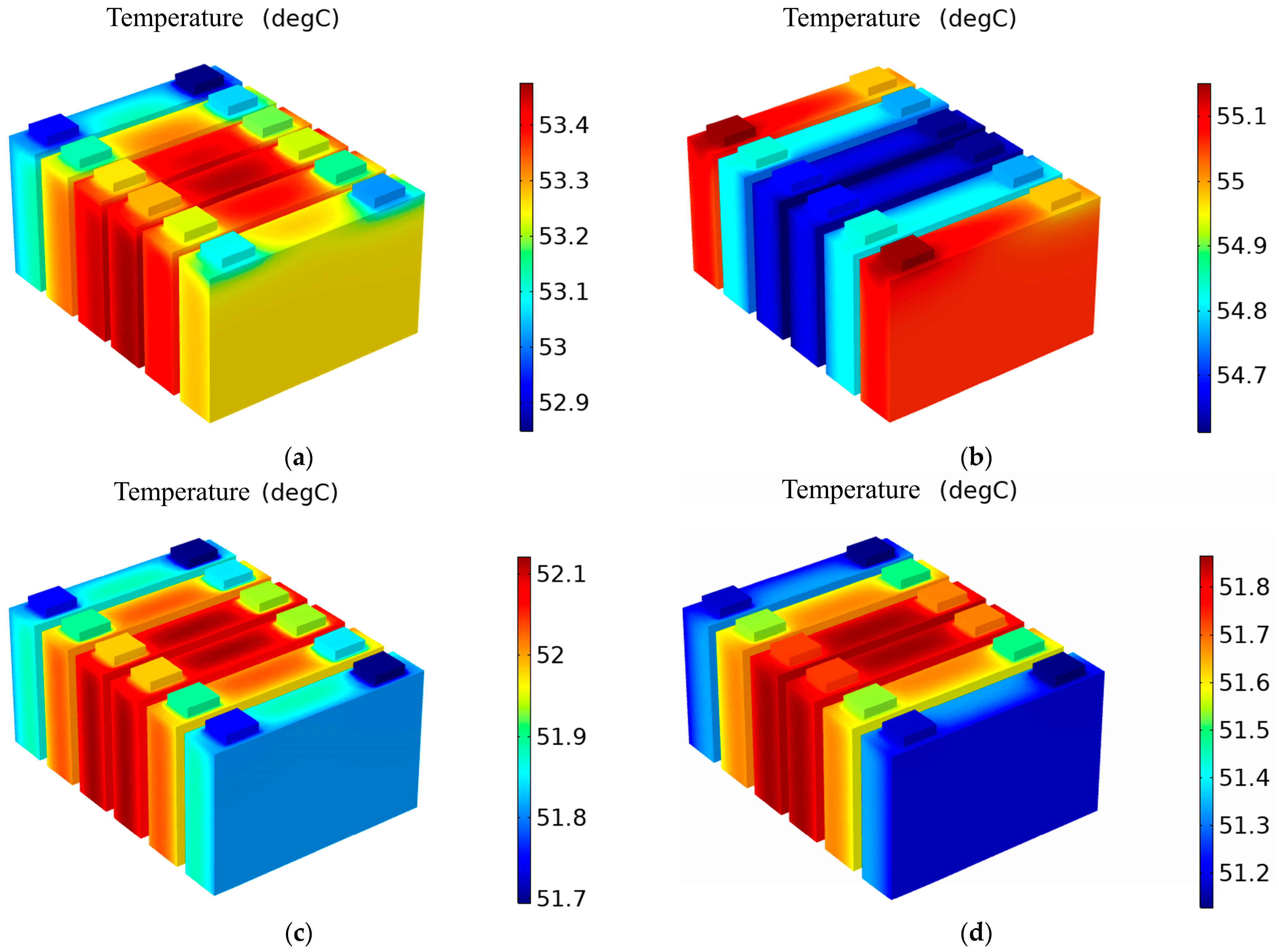
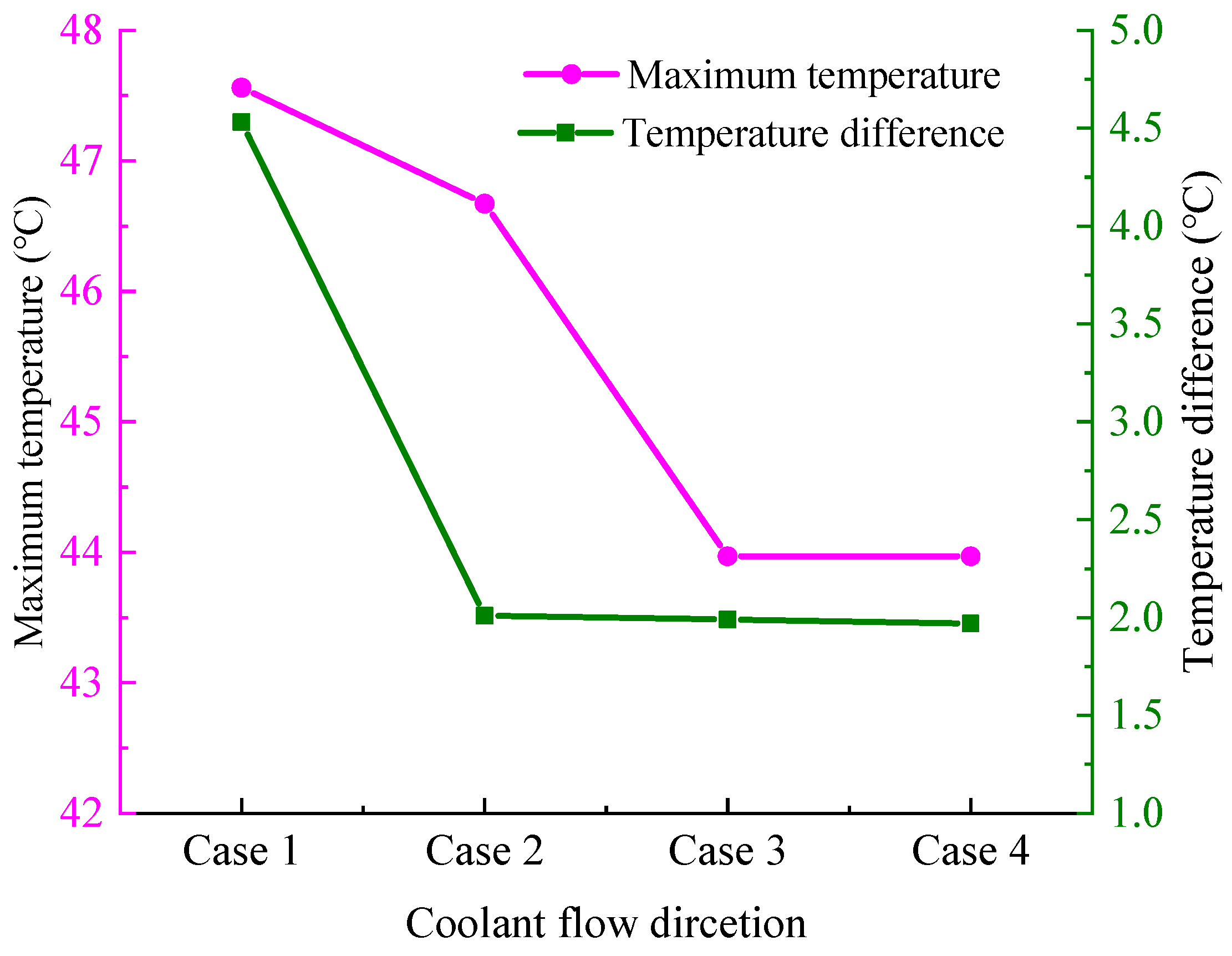
4.5. Effect of Coolant Direction
5. Optimization Based on Orthogonal Design Method
5.1. Orthogonal Design
5.2. Results and Discussion
5.2.1. Range Analysis
5.2.2. Variance Analysis
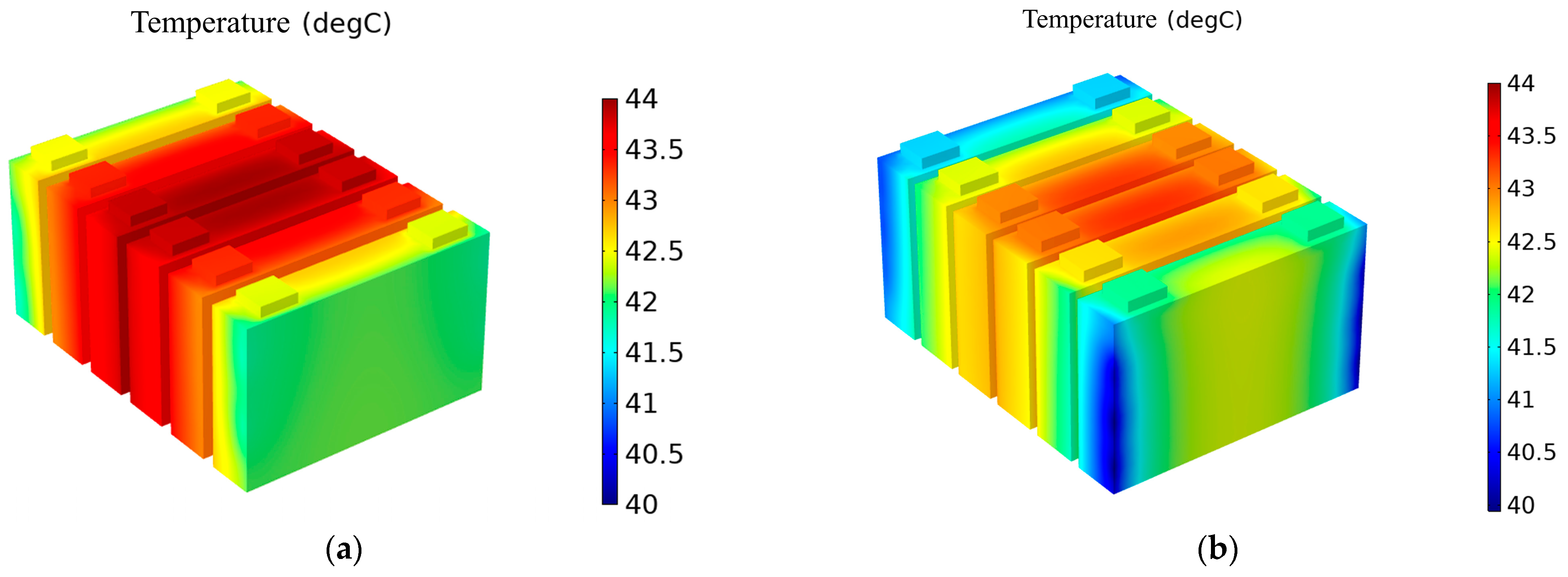
5.2.3. Optimal Solution Analysis
6. Conclusions
- (1)
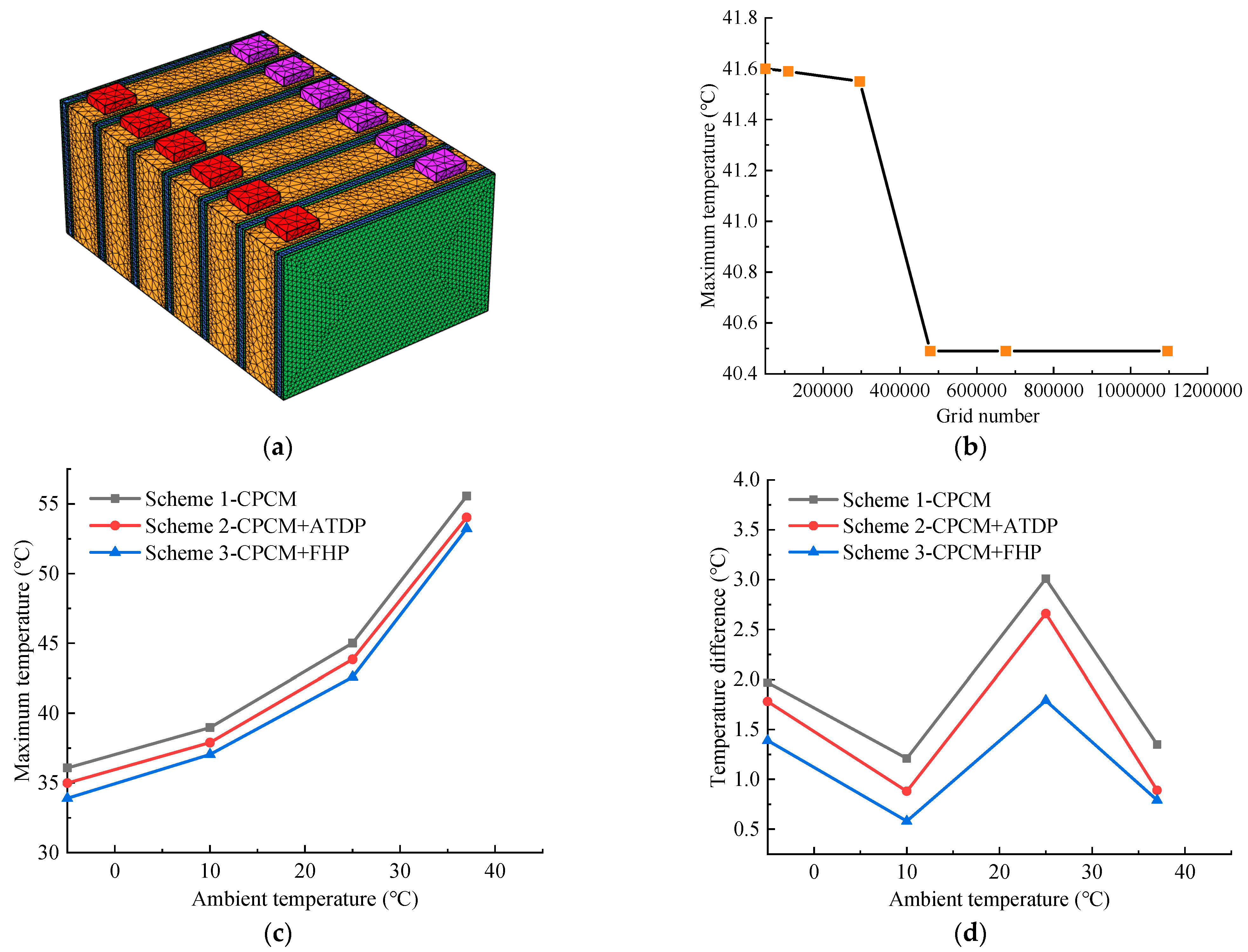
- It was found that Scheme 3 could control the maximum temperature and temperature difference of the battery module to a certain extent compared to Scheme 1 and Scheme 2, regardless of the discharge rate or environmental temperature. However, it was unsuitable for high discharge rates and high environmental temperatures.
- (2)
- A BTMS, combined with liquid cooling with CPCM–FHP, can significantly reduce the maximum temperature of the battery module, with a decrease in the coolant temperature and an increase in the number of cooling pipes. Moreover, the maximum temperature and temperature difference of the battery module was lower with a counter flow direction than with a parallel flow.
- (3)
- The number of FHPs, cooling pipes, and the coolant temperature were selected for multi-factor and multi-level analysis in the orthogonal design method. The optimal parameter combinations, with an FHP number of 6, a coolant temperature of 37 °C, and a cooling pipe number of 12, were obtained to achieve the best thermal performance of the BTMS. The maximum temperature and temperature difference were kept at 43.17 °C and 3.36 °C, respectively, under the maximum discharge rate of 2C and the high-temperature environment of 37 °C. The optimal solution analysis of the optimal scheme was conducted further, proving the effectiveness and rationality of selecting the optimal parameter combination.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Li, A.; Yuen, A.C.Y.; Wang, W.; Chen, T.B.Y.; Lai, C.S.; Yang, W.; Wu, W.; Chan, Q.N.; Kook, S.; Yeoh, G.H. Integration of computational fluid dynamics and artificial neural network for optimization design of battery thermal management system. Batteries 2022, 8, 69. [Google Scholar] [CrossRef]
- Yamanaka, T.; Kihara, D.; Takagishi, Y.; Yamaue, T. Multi-Physics equivalent circuit models for a cooling system of a lithium ion battery pack. Batteries 2020, 6, 44. [Google Scholar] [CrossRef]
- Chen, W.; Hou, S.; Shi, J.; Han, P.; Liu, B.; Wu, B.; Lin, X. Numerical analysis of novel air-based li-ion battery thermal management. Batteries 2022, 8, 128. [Google Scholar] [CrossRef]
- Ling, Z.; Wang, F.; Fang, X.; Gao, X.; Zhang, Z. A hybrid thermal management system for lithium ion batteries combining phase change materials with forced-air cooling. Appl. Energy 2015, 148, 403–409. [Google Scholar] [CrossRef]
- Xie, Y.; Tang, J.; Shi, S.; Xing, Y.; Wu, H.; Hu, Z.; Wen, D. Experimental and numerical investigation on integrated thermal management for lithium-ion battery pack with composite phase change materials. Energy Convers. Manag. 2017, 154, 562–575. [Google Scholar] [CrossRef]
- Jiang, G.; Huang, J.; Liu, M.; Cao, M. Experiment and simulation of thermal management for a tube-shell Li-ion battery pack with composite phase change material. Appl. Therm. Eng. 2017, 120, 1–9. [Google Scholar] [CrossRef]
- Anqi, A.; Li, C.; Dhahad, H.; Sharma, K.; Attia, E.-A.; Abdelrahman, A.; Mohammed, A.G.; Alamri, S.; Rajhi, A.A. Effect of combined air cooling and nano enhanced phase change materials on thermal management of lithium-ion batteries. J. Energy Storage 2022, 52, 104906. [Google Scholar] [CrossRef]
- Rao, Z.; Wang, Q.; Huang, C. Investigation of the thermal performance of phase change material/mini-channel coupled battery thermal management system. Appl. Energy 2016, 164, 659–669. [Google Scholar] [CrossRef]
- Bai, F.; Chen, M.; Song, W.; Yu, Q.; Li, Y.; Feng, Z.; Ding, Y. Investigation of thermal management for lithium-ion pouch battery module based on phase change slurry and mini channel cooling plate. Energy 2019, 167, 561–574. [Google Scholar] [CrossRef]
- Ling, Z.; Cao, J.; Zhang, W.; Zhang, Z.; Fang, X.; Gao, X. Compact liquid cooling strategy with phase change materials for Li-ion batteries optimized using response surface methodology. Appl. Energy 2018, 228, 777–788. [Google Scholar] [CrossRef]
- Abdelkareem, M.; Maghrabie, H.; Abo-Khalil, A.; Adhari, O.H.K.; Sayed, E.T.; Radwan, A.; Elsaid, K.; Wilberforce, T.; Olabi, A.G. Battery thermal management systems based on nanofluids for electric vehicles. J. Energy Storage 2022, 50, 104385. [Google Scholar] [CrossRef]
- Abbas, S.; Ramadan, Z.; Park, C. Thermal performance analysis of compact-type simulative battery module with paraffin as phase-change material and flat plate heat pipe. Int. J. Heat Mass Transf. 2021, 173, 121269. [Google Scholar] [CrossRef]
- Wang, Q.; Rao, Z.; Huo, Y.; Wang, S. Thermal performance of phase change material/oscillating heat pipe-based battery thermal management system. Int. J. Therm. Sci. 2016, 102, 9–16. [Google Scholar] [CrossRef]
- Wayan, S.; Muhamad, A.; Made, A. The application of battery thermal management system based on heat pipes and phase change materials in the electric bike. J. Energy Storage 2022, 56, 106014. [Google Scholar]
- Khan, M.; Dhahad, H.; Alamri, S.; Ali, E.A.; Sharma, K. Air cooled lithium-ion battery with cylindrical cell in phase change material filled cavity of different shapes. J. Energy Storage 2022, 50, 104573. [Google Scholar] [CrossRef]
- Faizan, M.; Pati, S.; Randive, P. Implications of novel cold plate design with hybrid cooling on thermal management of fast discharging lithium-ion battery. J. Energy Storage 2022, 53, 105051. [Google Scholar] [CrossRef]
- Yuan, Q.; Xu, X.; Tong, G.; Ding, H. Effect of coupling phase change materials and heat pipe on performance enhancement of Li-ion battery thermal management system. Int. J. Energy Res. 2020, 45, 5399–5411. [Google Scholar] [CrossRef]
- Elnaggar, M.; Abdullah, M.; Munusamy, S. Experimental and numerical studies of finned L-shape heat pipe for notebook PC cooling. IEEE Trans. Compon. Packag. Manuf. Technol. 2013, 3, 978–988. [Google Scholar] [CrossRef]
- Chen, W. Research on Heat Control System for Vehicular Power Battery Pack Based on Heat Pipe Technology. Master’s thesis, South China University of Technology, Guangzhou, China, 2014. [Google Scholar]
- Wei, K. Structure Design and Themal Analysis of Power Battery Thermal Management Based on Flat Heat Pipes. Master’s thesis, Beijing Jiaotong University, Beijing, China, 2019. [Google Scholar]
- Li, R. Study on the Heat Dissipation Performance of Power Battery Liquid Cooling Thermal Management System of Electric Vehicle Based on Flat Heat Pipe. Master’s thesis, Jiangsu University, Zhenjiang, China, 2019. [Google Scholar]
- Xu, X.; Zhang, H.; Cao, L.; Yi, Z.; Li, P.; Guo, H. Heat generation and surrogate model for large-capacity nickel-rich prismatic lithium-ion battery as against 18650 battery. J. Loss Prev. Process Ind. 2022, 77, 104783. [Google Scholar] [CrossRef]
- Zhang, H.; Wu, X.; Wu, Q.; Xu, S. Experimental investigation of thermal performance of large-sized battery module using hybrid PCM and bottom liquid cooling configuration. Appl. Therm. Eng. 2019, 159, 113968. [Google Scholar] [CrossRef]
- Song, L.; Zhang, H.; Yang, C. Thermal analysis of conjugated cooling configurations using phase change material and liquid cooling techniques for a battery module. Int. J. Heat Mass Transf. 2019, 133, 827–841. [Google Scholar] [CrossRef]
- COMSOL Multiphysics®. Available online: https://www.comsol.com (accessed on 16 June 2023).
- Xin, Q.; Yang, T.; Zhang, H.; Yang, J.; Zeng, J.; Xiao, J. Experimental and numerical study of lithium-ion battery thermal management system using composite phase change material and liquid cooling. J. Energy Storage 2023, in press. [Google Scholar]
- Liu, H.; Wei, Z.; He, W.; Zhao, J. Thermal issues about Li-ion batteries and recent progress in battery thermal management systems: A review. Energy Convers. Manag. 2017, 150, 304–330. [Google Scholar] [CrossRef]
- Wang, Q.; Ping, P.; Zhao, X.; Chu, G.; Sun, J.; Chen, C. Thermal runaway caused fire and explosion of lithium ion battery. J. Power Sources 2012, 208, 210–224. [Google Scholar] [CrossRef]
- Paul, S.; Diegelmann, C.; Kabza, H.; Tillmetz, W. Analysis of ageing inhomogeneities in lithium-ion battery systems. J. Power Sources 2013, 239, 642–650. [Google Scholar] [CrossRef]
- Yang, X.; Tan, S.; Liu, J. Thermal management of Li-ion battery with liquid metal. Energy Convers. Manag. 2016, 117, 577–585. [Google Scholar] [CrossRef]
- Chen, K.; Song, M.; Wei, W.; Wang, S. Structure optimization of parallel air-cooled battery thermal management system with U-type flow for cooling efficiency improvement. Energy 2018, 145, 603–613. [Google Scholar] [CrossRef]
- Xie, J.; Zang, M.; Wang, S.; Ge, Z. Optimization investigation on the liquid cooling heat dissipation structure for the lithium-ion battery package in electric vehicles. Proc. Inst. Mech. Eng. Part D J. Automob. Eng. 2017, 231, 1735–1750. [Google Scholar] [CrossRef]
- Li, N. Design and Optimization of Heat Dissipation Structure for Thermal Management System of Lithium Ion Batteries. Master’s thesis, Southwest Jiaotong University, Chengdou, China, 2019. [Google Scholar]
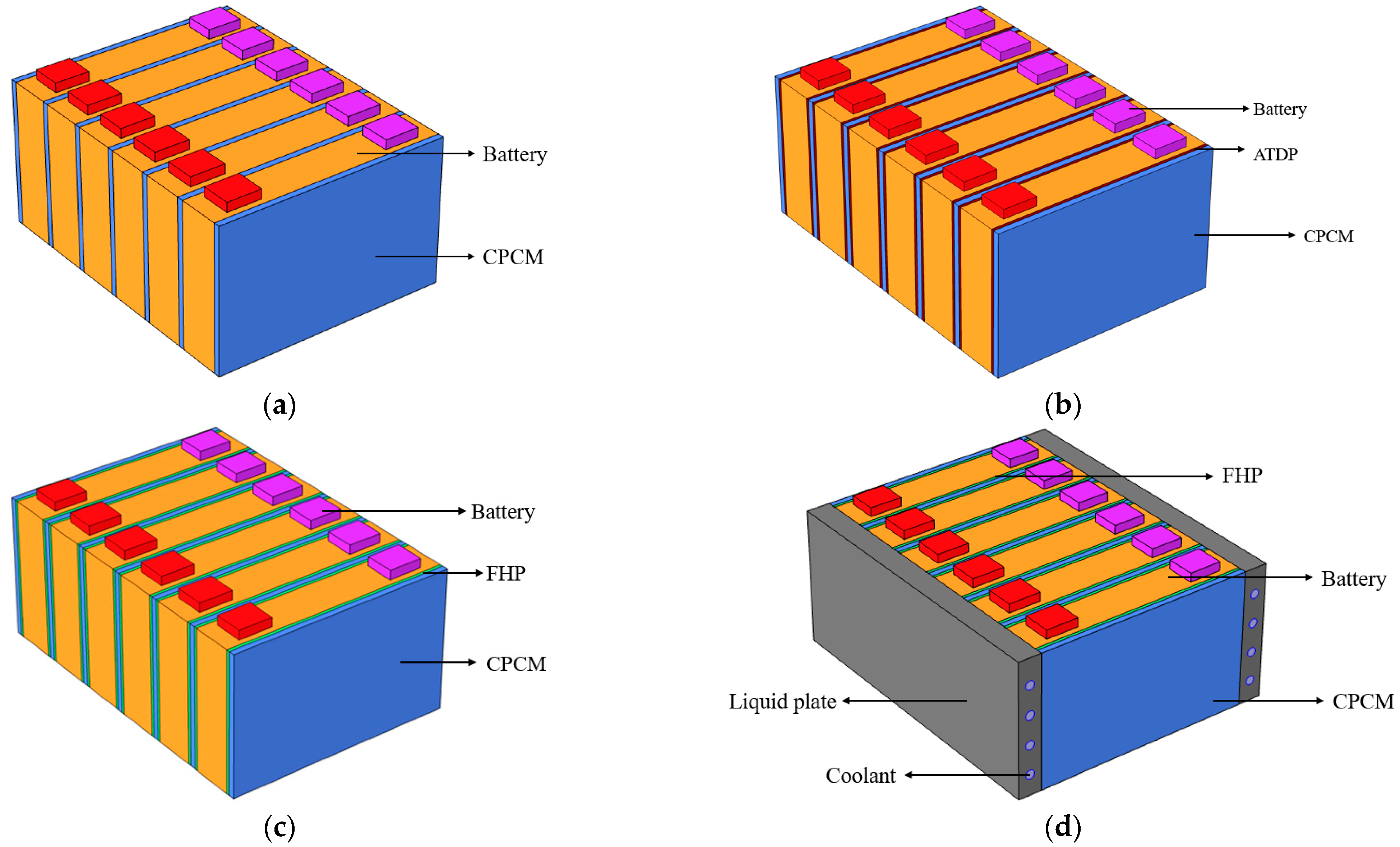






| Design Schemes | Cooling Methods |
|---|---|
| Scheme 1 | Only 7 CPCMs |
| Scheme 2 | 7 CPCMs and 12 ATDPs |
| Scheme 3 | 7 CPCMs and 12 FHPs |
| Scheme 4 | 7 CPCMs, 12 FHPs, and liquid cooling |
| Materials | Density (kg/m3) | Specific Heat Capacity (J/(kg·K)) | Thermal Conductivity (W/(m·K)) |
|---|---|---|---|
| Battery | 2300 | 1072 | 18.5, 18.5, 1.5 |
| Aluminum | 2719 | 871 | 202.4 |
| Water | 998.2 | 4128 | 0.6 |
| Heat pipe | 8978 | 381 | 6000 |
| CPCM | 1000 | 2000 | 1.2 |
| Level | A (FHP Number) | B (Coolant Temperature), °C | C (Coolant Pipe Number) |
|---|---|---|---|
| 1 | 6 | 25 | 6 |
| 2 | 8 | 30 | 8 |
| 3 | 12 | 33 | 10 |
| 4 | 14 | 37 | 12 |
| Test | A (FHP Number) | B (Coolant Temperature) | C (Coolant Pipe Number) | Tmax (°C) | ΔT (°C) |
|---|---|---|---|---|---|
| 1 | 6 | 25 | 6 | 37.98 | 5.50 |
| 2 | 6 | 30 | 8 | 39.11 | 4.24 |
| 3 | 6 | 33 | 10 | 40.09 | 3.21 |
| 4 | 6 | 37 | 12 | 43.17 | 3.36 |
| 5 | 8 | 25 | 8 | 37.60 | 5.98 |
| 6 | 8 | 30 | 6 | 41.63 | 5.89 |
| 7 | 8 | 33 | 12 | 40.35 | 4.13 |
| 8 | 8 | 37 | 10 | 45.15 | 4.58 |
| 9 | 12 | 25 | 10 | 34.29 | 3.97 |
| 10 | 12 | 30 | 12 | 37.60 | 3.01 |
| 11 | 12 | 33 | 6 | 41.79 | 3.27 |
| 12 | 12 | 37 | 8 | 44.18 | 2.26 |
| 13 | 14 | 25 | 12 | 33.48 | 3.62 |
| 14 | 14 | 30 | 10 | 38.23 | 3.35 |
| 15 | 14 | 33 | 8 | 40.42 | 2.63 |
| 16 | 14 | 37 | 6 | 45.03 | 2.77 |
| Serial Number | A (FHP Number) | B (Coolant Temperature) | C (Coolant Pipe Number) | e1 | e2 |
|---|---|---|---|---|---|
| Mean value 1 | 40.09 | 35.84 | 41.61 | 40.19 | 40.29 |
| Mean value 2 | 41.18 | 39.14 | 40.33 | 39.88 | 39.69 |
| Mean value 3 | 39.47 | 40.66 | 39.44 | 40.09 | 39.85 |
| Mean value 4 | 39.29 | 44.38 | 38.65 | 39.88 | 40.20 |
| Range | 1.89 | 8.55 | 2.96 | 0.31 | 0.59 |
| Serial Number | A (FHP Number) | B (Coolant Temperature) | C (Coolant Pipe Number) | e1 | e2 |
|---|---|---|---|---|---|
| Mean value 1 | 4.08 | 4.77 | 4.36 | 3.81 | 3.93 |
| Mean value 2 | 5.15 | 4.12 | 3.78 | 3.93 | 3.78 |
| Mean value 3 | 3.13 | 3.31 | 3.78 | 3.74 | 3.75 |
| Mean value 4 | 3.09 | 3.32 | 3.653 | 3.96 | 3.99 |
| Range | 2.05 | 1.53 | 0.83 | 0.22 | 0.25 |
| Factor | Sum of Squares of Deviations | Degree of Freedom | Ratio of F | Critical Value of F | Significance |
|---|---|---|---|---|---|
| A (FHP number) | 8.79 | 3 | 14.27 | 4.76 | * |
| B (Coolant temperature) | 150.83 | 3 | 245.05 | 4.76 | *** |
| C (Coolant pipe number) | 19.31 | 3 | 31.37 | 4.76 | ** |
| Error | 1.23 | 6 |
| Factor | Sum of Squares of Deviations | Degree of Freedom | Ratio of F | Critical Value of F | Significance |
|---|---|---|---|---|---|
| A (FHP number) | 11.30 | 3 | 77.38 | 4.76 | *** |
| B (Coolant temperature) | 6.31 | 3 | 43.19 | 4.76 | ** |
| C (Coolant pipe number) | 1.48 | 3 | 10.14 | 4.76 | * |
| Error | 0.29 | 6 |
| Design Schemes | Tmax (°C) | ΔT (°C) |
|---|---|---|
| A4B1C4 | 33.48 | 3.62 |
| A3B4C2 | 44.18 | 2.26 |
| A1B4C4 | 43.17 | 3.36 |
| Parameter | Effect to Tmax | Effect to ΔT |
|---|---|---|
| 0.08 | 0.22 | |
| 4.37 | −0.61 | |
| −1.36 | −0.33 | |
| (Estimated) | 43.10 °C | 3.14 °C |
| A1B4C4 (Optimal) | 43.17 °C | 3.36 °C |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xin, Q.; Yang, T.; Zhang, H.; Zeng, J.; Xiao, J. Simulation and Optimization of Lithium-Ion Battery Thermal Management System Integrating Composite Phase Change Material, Flat Heat Pipe and Liquid Cooling. Batteries 2023, 9, 334. https://doi.org/10.3390/batteries9060334
Xin Q, Yang T, Zhang H, Zeng J, Xiao J. Simulation and Optimization of Lithium-Ion Battery Thermal Management System Integrating Composite Phase Change Material, Flat Heat Pipe and Liquid Cooling. Batteries. 2023; 9(6):334. https://doi.org/10.3390/batteries9060334
Chicago/Turabian StyleXin, Qianqian, Tianqi Yang, Hengyun Zhang, Juan Zeng, and Jinsheng Xiao. 2023. "Simulation and Optimization of Lithium-Ion Battery Thermal Management System Integrating Composite Phase Change Material, Flat Heat Pipe and Liquid Cooling" Batteries 9, no. 6: 334. https://doi.org/10.3390/batteries9060334
APA StyleXin, Q., Yang, T., Zhang, H., Zeng, J., & Xiao, J. (2023). Simulation and Optimization of Lithium-Ion Battery Thermal Management System Integrating Composite Phase Change Material, Flat Heat Pipe and Liquid Cooling. Batteries, 9(6), 334. https://doi.org/10.3390/batteries9060334








