Time-Resolved and Robust Lithium Plating Detection for Automotive Lithium-Ion Cells with the Potential for Vehicle Application
Abstract
:1. Introduction
2. Methods/Experimental
3. Results
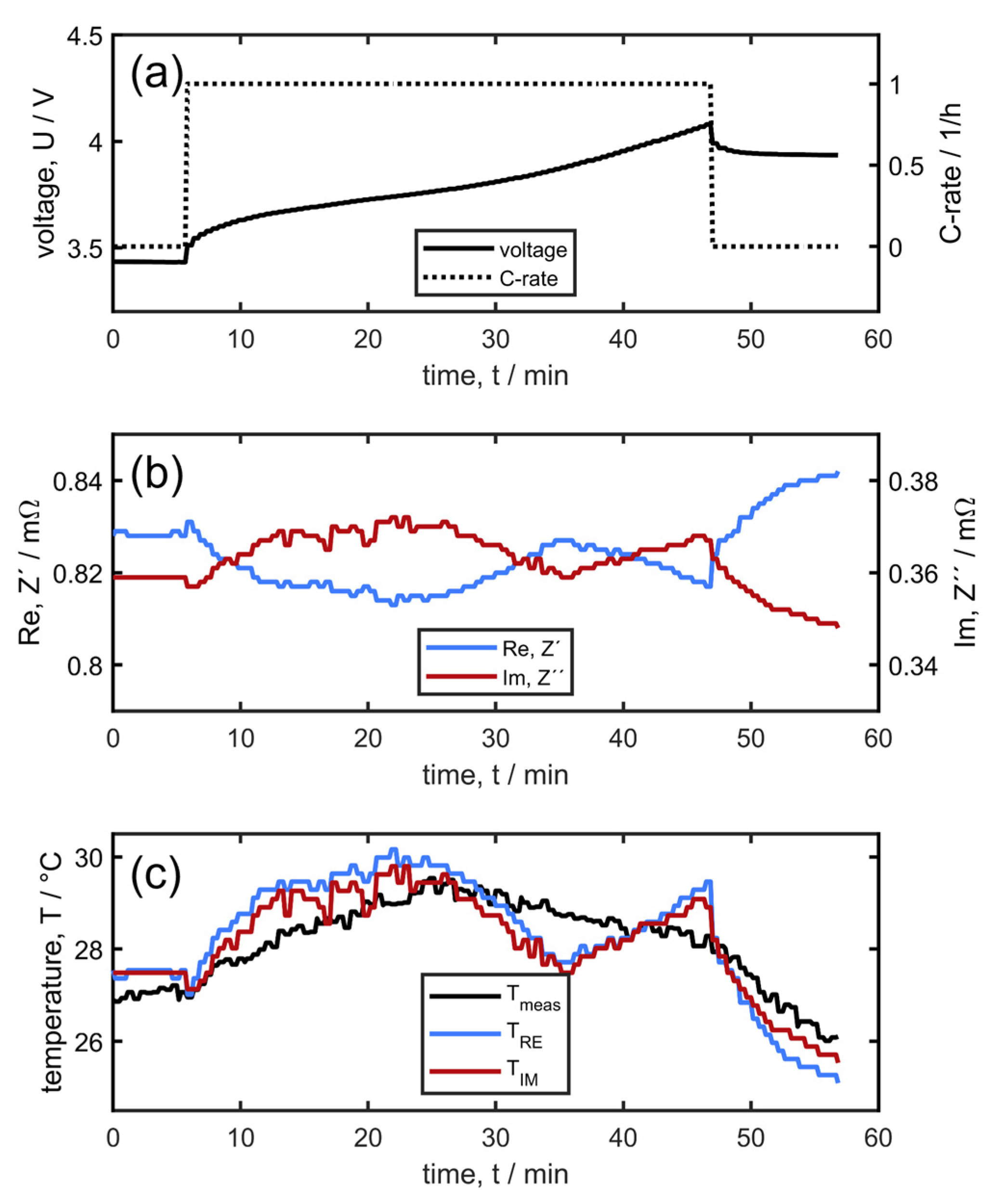
- For the case of 2.5C-CCCV (light blue), the beginning of a significant increase IN ΔTRe−Im coincides with the onset of plating detected by the differential voltage;
- For the case of 2C-CCCV ΔTRe−Im., even increases before the peak in the differential voltage can be detected;
- For the case of 1.5-CCCV, only minor plating could be observed and ΔTRe−Im seems not to be sensitive enough to detect that degree of plating.
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- IEA. IEA, Transport Sector CO2 Emissions by Mode in the Sustainable Development Scenario, 2000–2030; IEA: Paris, France, 2020. [Google Scholar]
- Le Quéré, C.; Andrew, R.M.; Friedlingstein, P.; Sitch, S.; Hauck, J.; Pongratz, J.; Pickers, P.A.; Korsbakken, J.I.; Peters, G.P.; Canadell, J.G.; et al. Global Carbon Budget 2018. Earth Syst. Sci. Data 2018, 10, 2141–2194. [Google Scholar] [CrossRef] [Green Version]
- Bieker, G. A global comparison of the life-cycle greenhouse gas emissions of combustion engine and electric passenger cars. The international council on clean transportation. Communications 2021, 49, 847129–102. [Google Scholar]
- Petzl, M.; Danzer, M.A. Nondestructive detection, characterization, and quantification of lithium plating in commercial lithium-ion batteries. J. Power Sources 2014, 254, 80–87. [Google Scholar] [CrossRef]
- Lin, H.-P.; Chua, D.; Salomon, M.; Shiao, H.-C.; Hendrickson, M.; Plichta, E.; Slane, S. Low-Temperature Behavior of Li-Ion Cells. Electrochem. Solid State Lett. 2001, 4, A71–A73. [Google Scholar] [CrossRef]
- Schindler, S.; Bauer, M.; Petzl, M.; Danzer, M.A. Voltage relaxation and impedance spectroscopy as in-operandomethods for the detection of lithium plating on graphitic anodes incommercial lithium-ion cells. J. Power Sources 2016, 304, 170–180. [Google Scholar] [CrossRef]
- von Lüders, C.; Zinth, V.; Erhard, S.V.; Osswald, P.J.; Hofmann, M.; Gilles, R.; Jossen, A. Lithium plating in lithium-ion batteries investigated by voltage relaxation and in situ neutron diffraction. J. Power Sources 2017, 342, 17–23. [Google Scholar] [CrossRef]
- Smart, M.C.; Ratnakumar, B.V. Effects of Electrolyte Composition on Lithium Plating inLithium-Ion Cells. J. Electrochem. Soc. 2011, 158, 379–389. [Google Scholar] [CrossRef]
- Smart, M.C.; Ratnakumar, B.V.; Whitcanack, L.; Chin, K.; Rodriguez, M.; Surampudi, S. Performance characteristics of lithium ion cells at low temperatures—IEEE Aerospace and Electronics Systems Magazine. IEEE AESS Syst. Mag. 2002, 17, 16–20. [Google Scholar] [CrossRef]
- Uhlmann, C.; Illig, J.; Ender, M.; Schuster, R.; Ivers-Tiffée, E. In situ detection of lithium metal plating on graphite in ex-perimentalcells. J. Power Sources 2015, 279, 428–438. [Google Scholar] [CrossRef]
- Konz, Z.M.; McShane, E.J.; McCloskey, B.D. Detecting the Onset of Lithium Plating and Monitoring Fast Charging Per-formance with Voltage Relaxation. ACS Energy Lett. 2020, 5, 1750–1757. [Google Scholar] [CrossRef]
- Katzer, F.; Jahn, L.; Hahn, M.; Danzer, M.A. Model-based lithium deposition detection method using differential voltage analysis. J. Power Sources 2021, 512, 230449. [Google Scholar] [CrossRef]
- Katzer, F.; Mößle, P.; Schamel, M.; Danzer, M.A. Adaptive fast charging control using impedance-based detection of lith-ium. J. Power Sources 2023, 555, 232354. [Google Scholar] [CrossRef]
- Chen, X.; Li, L.; Liu, M.; Huang, T.; Yu, A. Detection of lithium plating in lithium-ion batteries by distribution of relaxation times. J. Power Sources 2021, 496, 229867. [Google Scholar] [CrossRef]
- Downie, L.E.; Krause, L.J.; Burns, J.C.; Jensen, L.D.; Chevrier, V.; Dahna, J.R. In Situ Detection of Lithium Plating on Graphite Electrodes by Electrochemical Calorimetry. J. Electrochem. Soc. 2013, 160, A588–A594. [Google Scholar] [CrossRef]
- Burns, J.C.; Stevens, D.A.; Dahn, J.R. In-Situ Detection of Lithium Plating Using High Precision Coulometry. J. Electrochem. Soc. 2015, 162, A959–A964. [Google Scholar] [CrossRef]
- Smith, A.J.; Burns, J.C.; Trussler, S.; Dahn, J.R. Precision Measurements of the Coulombic Efficiency of Lithium-Ion Batteries and of Electrode Materials for Lithium-Ion Batteries. J. Electrochem. Soc. 2010, 157, A196–A202. [Google Scholar] [CrossRef]
- Adam, A.; Knobbe, E.; Wandt, J.; Kwade, A. Application of the differential charging voltage analysis to determine the onset of lithium-plating during fast charging of lithium-ion cells. J. Power Sources 2021, 495, 229794. [Google Scholar] [CrossRef]
- Adam, A.; Wandt, J.; Knobbe, E.; Bauer, G.; Kwade, A. Fast-Charging of Automotive Lithium-Ion Cells: In-Situ Lithi-um-Plating Detection and Comparison of Different Cell Designs. J. Electrochem. Soc. 2020, 167, 130503. [Google Scholar] [CrossRef]
- Birkenmaier, C.; Bitzer, B.; Harzheim, M.; Hintennach, A.; Schleid, T. Lithium Plating on Graphite Negative Electrodes: Innovative Qualitative and Quantitative Investigation Methods. J. Electrochem. Soc. 2015, 162, A2646–A2650. [Google Scholar] [CrossRef]
- Bauer, M.; Wachtler, M.; Stöwe, H.; Persson, J.V.; Danzer, M.A. Understanding the dilation and dilation relaxation behavior of graphite-based lithium-ion cells. J. Power Sources 2016, 317, 93–102. [Google Scholar] [CrossRef]
- Rieger, B.; Schuster, S.; Erhard, S.; Osswald, P.; Rheinfeld, A.; Willmann, C.; Jossen, A. Multi-directional laser scanning as innovative method to detect local cell damage during fast charging of lithium-ion cells. J. Energy Storage 2016, 8, 1–5. [Google Scholar] [CrossRef]
- Daubinger, P.; Ebert, F.; Hartmann, S.; Giffin, G.A. Impact of electrochemical and mechanical interactions on lithium-ion battery performance investigated by operando dilatometry. J. Power Sources 2021, 488, 229457. [Google Scholar] [CrossRef]
- Bitzer, B.; Gruhle, A. A new method for detecting lithium plating by measuring the cell thickness. J. Power Sources 2014, 262, 297–302. [Google Scholar] [CrossRef]
- Spingler, F.B.; Wittmann, W.; Sturm, J.; Rieger, B.; Jossen, A. Optimum fast charging of lithium-ion pouch cells based on local volume expansion criteria. J. Power Sources 2018, 393, 152–160. [Google Scholar] [CrossRef]
- Zinth, V.; von Lüders, C.; Hofmann, M.; Hattendorff, J.; Buchberger, I.; Erhard, S.; Rebelo-Kornmeier, J.; Jossen, A.; Gilles, R. Lithium plating in lithium-ion batteries at sub-ambient temperatures investigated by in situ neutron diffraction. J. Power Sources 2014, 271, 152–159. [Google Scholar] [CrossRef]
- McShane, E.J.; Colclasure, A.M.; Brown, D.E.; Konz, Z.M.; Smith, K.; McCloskey, B.D. Quantification of Inactive Lithium and Solid–Electrolyte Interphase Species on Graphite Electrodes after Fast Charging. ACS Energy Lett. 2020, 5, 2045–2051. [Google Scholar] [CrossRef]
- Wandt, J.; Jakes, P.; Granwehr, J.; Eichel, R.-A.; Gasteiger, H.A. Quantitative and time-resolved detection of lithium plating on graphite anodes in lithium ion batteries. Mater. Today 2017, 21, 231–240. [Google Scholar] [CrossRef]
- Wandt, J.; Marino, C.; Gasteiger, H.A.; Jakes, P.; Eichel, R.-A.; Granwehr, J. Operando electron paramagnetic resonance spectroscopy—Formation of mossy lithium on lithium anodes during charge–discharge cycling. Energy Environ. Sci. 2015, 8, 1358–1367. [Google Scholar] [CrossRef] [Green Version]
- Niemöller, A.; Jakes, P.; Eichel, R.-A.; Granwehr, J. EPR Imaging of Metallic Lithium and its Application to Dendrite Lo-calisation in Battery Separators. Sci. Rep. 2018, 8, 14331. [Google Scholar] [CrossRef] [Green Version]
- Koleti, U.R.; Dinh, T.Q.; Marco, J. A new on-line method for lithium plating detection in lithium-ion batteries. J. Power Sources 2020, 451, 227798. [Google Scholar] [CrossRef]
- Koseoglou, M.; Tsioumas, E.; Ferentinou, D.; Jabbour, N.; Papagiannis, D.; Mademlis, C. Lithium plating detection using dynamic electrochemical impedance spectroscopy in lithium-ion batteries. J. Power Sources 2021, 512, 230508. [Google Scholar] [CrossRef]
- Dotoli, M.; Milo, E.; Giuliano, M.; Rocca, R.; Nervi, C.; Baricco, M.; Ercole, M.; Sgroi, M.F. Detection of Lithium Plating in Li-Ion Cell Anodes Using Realistic Automotive Fast-Charge Profiles. Batteries 2021, 7, 46. [Google Scholar] [CrossRef]
- Dotoli, M.; Milo, E.; Giuliano, M.; Tiozzo, A.; Baricco, M.; Nervi, C.; Ercole, M.; Sgroi, M.F. Development of an Innovative Procedure for Lithium Plating. Batteries 2022, 8, 88. [Google Scholar] [CrossRef]
- Haussmann, P.; Melbert, J. Internal Cell Temperature Measurement and Thermal Modeling of Lithium Ion Cells for Au-tomotive Applications by Means of Electrochemical Impedance Spectroscopy. SAE Int. J. Altern. Powertrains 2017, 6, 261–270. [Google Scholar] [CrossRef]
- Brown, D.E.; McShane, E.J.; Konz, Z.M.; Knudsen, K.B.; McCloskey, B.D. Detecting onset of lithium plating during fast charging of Li-ion batteries using operando electrochemical impedance spectroscopy. Cell Rep. Phys. Sci. 2021, 2, 100589. [Google Scholar] [CrossRef]
- Wandt, J.; Adam, A.; Schardax, C.; Schmidt, J.P. Verfahren zum Detektieren von Lithium-Plating in Einer Lithiumionenzelle Sowie Lithiumionenbatterie. Germany Patent DE102021103949A, 25 August 2022. [Google Scholar]
- Landinger, T.; Schwarzberger, G.; Hofer, G.; Rose, M.; Jossen, A. Power Line Communications for Automotive High Voltage Battery Systems: Channel Modeling and Coexistence Study with Battery Monitoring. Energies 2021, 14, 1851. [Google Scholar] [CrossRef]
- Schmidt, J.P.; Arnold, S.; Loges, A.; Werner, D.; Wetzel, T.; Ivers-Tiffée, E. Measurement of the internal cell temperature via impedance: Evaluation and application of a new method. J. Power Sources 2013, 243, 110–117. [Google Scholar] [CrossRef]
- Beelen, H.; Raijmakers, L.; Donkers, M.; Notten, P.; Bergveld, H. A comparison and accuracy analysis of impedance-based temperature estimation methods for Li-ion batteries. Appl. Energy 2016, 175, 128–140. [Google Scholar] [CrossRef]
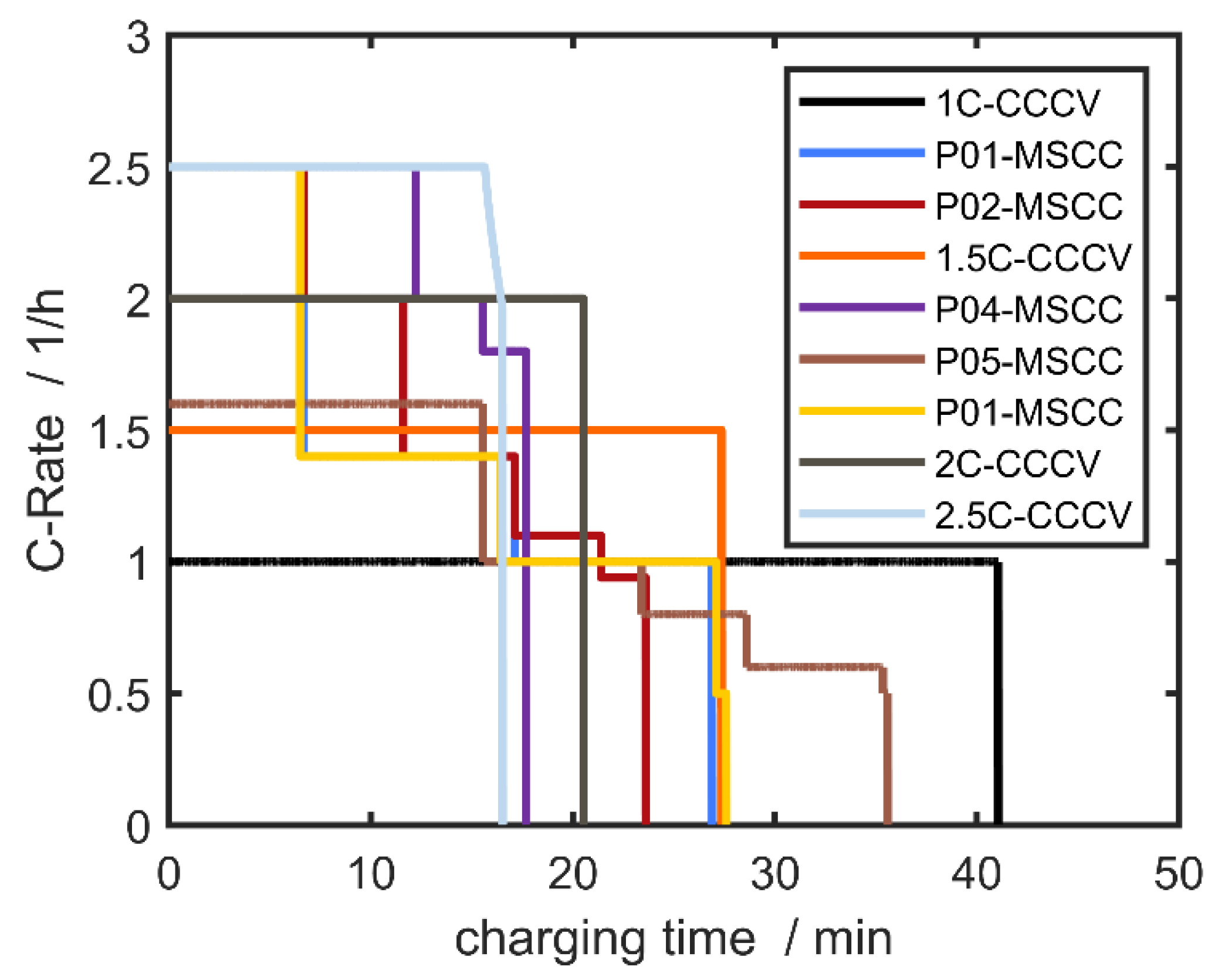


| Profiles | Charging Time (10% to 80% SOC) | Lithium Plating |
|---|---|---|
| 1C-CCCV | 41 min | No |
| P01-MSCC | 29 min | No |
| P02-MSCC | 25 min | No |
| P03-CCCA | 23 min | No |
| 1.5C-CCCV | 28 min | Traces |
| P04-MSCC | 19 min | Yes |
| P05-MSCC | 39 min | No |
| 2C-CCCV | 21 min | Yes |
| 2.5C-CCCV | 17 min | Yes |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Schmidt, J.P.; Adam, A.; Wandt, J. Time-Resolved and Robust Lithium Plating Detection for Automotive Lithium-Ion Cells with the Potential for Vehicle Application. Batteries 2023, 9, 97. https://doi.org/10.3390/batteries9020097
Schmidt JP, Adam A, Wandt J. Time-Resolved and Robust Lithium Plating Detection for Automotive Lithium-Ion Cells with the Potential for Vehicle Application. Batteries. 2023; 9(2):97. https://doi.org/10.3390/batteries9020097
Chicago/Turabian StyleSchmidt, Jan P., Alexander Adam, and Johannes Wandt. 2023. "Time-Resolved and Robust Lithium Plating Detection for Automotive Lithium-Ion Cells with the Potential for Vehicle Application" Batteries 9, no. 2: 97. https://doi.org/10.3390/batteries9020097
APA StyleSchmidt, J. P., Adam, A., & Wandt, J. (2023). Time-Resolved and Robust Lithium Plating Detection for Automotive Lithium-Ion Cells with the Potential for Vehicle Application. Batteries, 9(2), 97. https://doi.org/10.3390/batteries9020097






