Performance Analysis of the Liquid Cooling System for Lithium-Ion Batteries According to Cooling Plate Parameters
Abstract
:1. Introduction
2. Methods and Simulation
2.1. Simulation Model
2.2. Governing Equations
2.3. Initial and Boundary Conditions
2.4. Validation of Simulation Model
3. Results and Discussion
3.1. Effect of the Inlet Flow Rate of Cooling Fluid
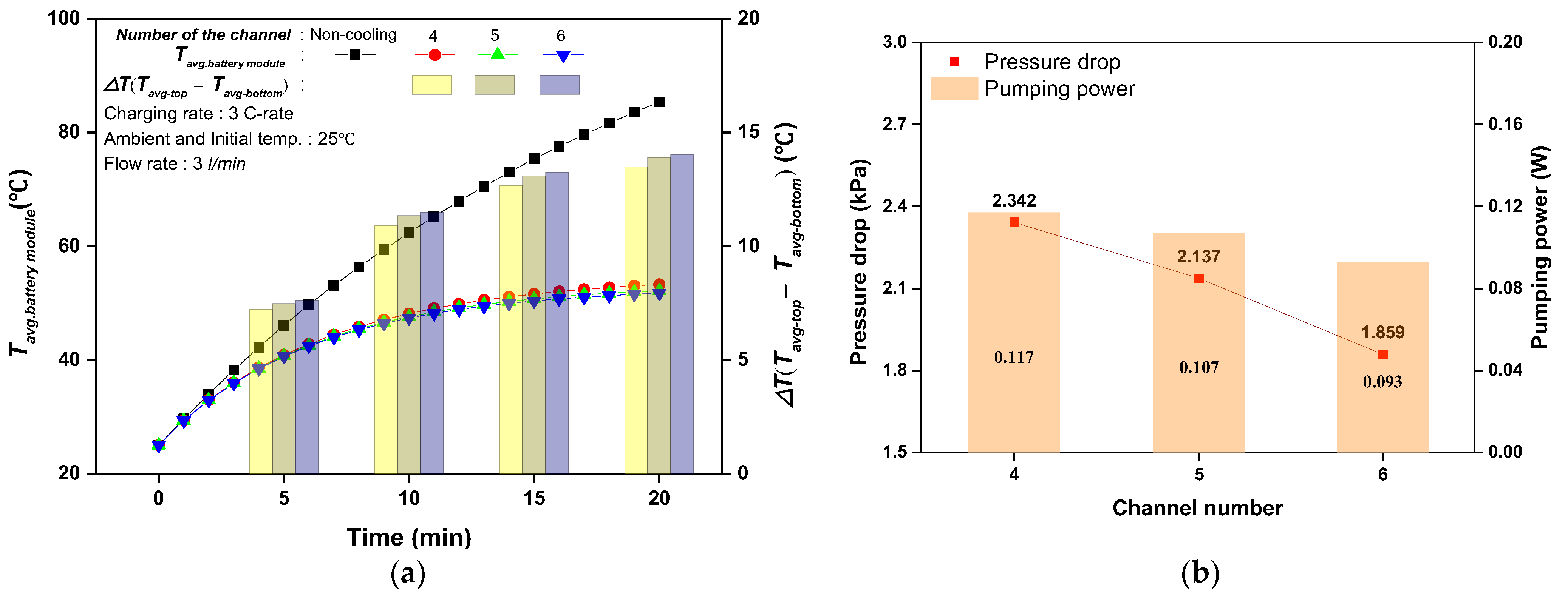
3.2. Effect of Channel Width and Number
3.3. Result of the Orthogonal Analysis
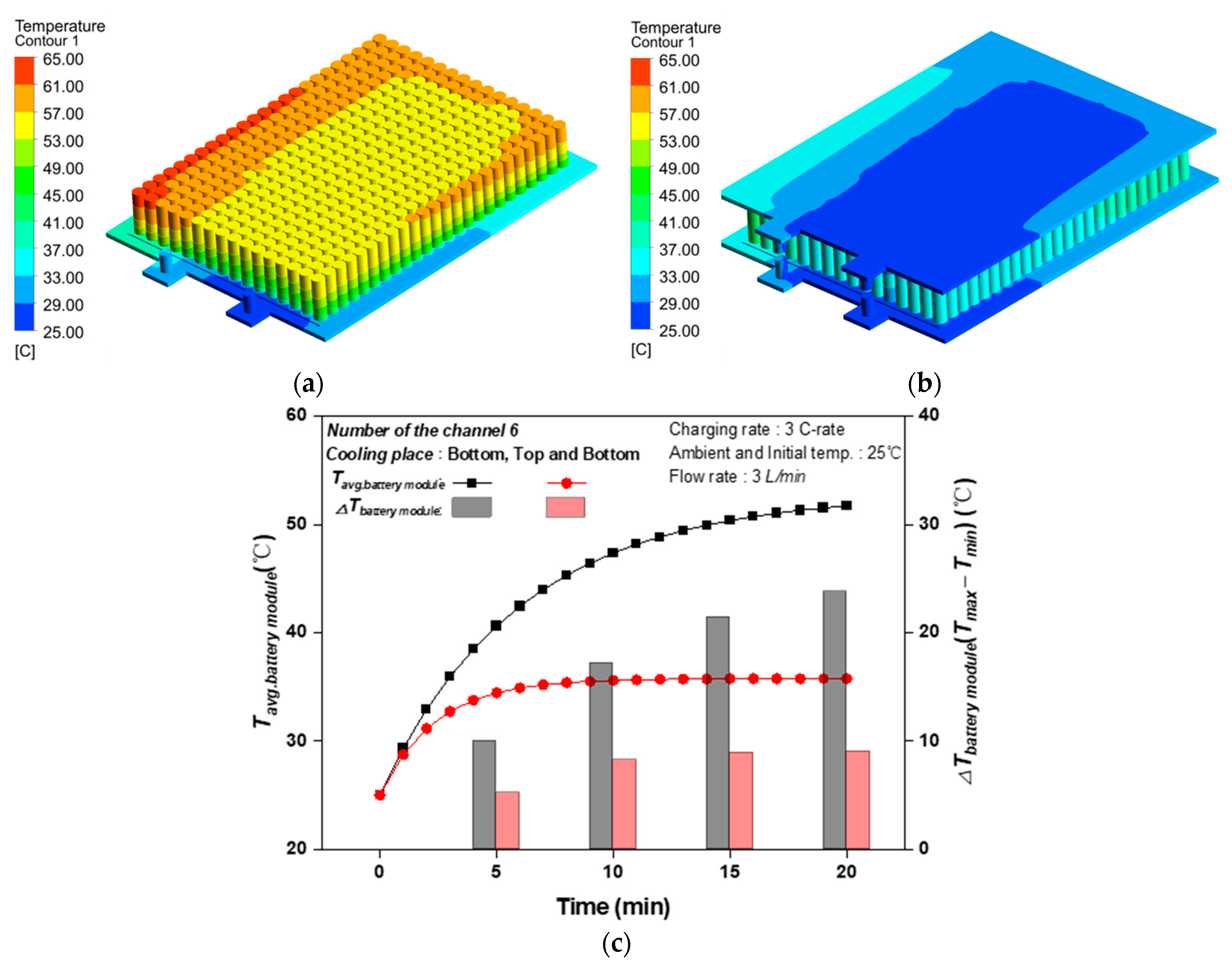
3.4. Result of Cooling Performance by Adapting an Improved Cooling System
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| LIB | Lithium-ion battery |
| EV | Electric vehicle |
| HEV | Hybrid electric vehicle |
| BTMS | Battery management system |
| PCM | Phase change material |
| TCS | Thermally conductive structure |
| L1 | Top width of cooling channel (mm) |
| L2 | Bottom width of cooling channel (mm) |
| h1 | Height of cooling channel (mm) |
| Qgen | Total heating value of battery cells (W) |
| Qir | Charge/discharge irreversible heat (W) |
| Qre | Charge/discharge reversible heat |
| I | Current (A) |
| R | Resistance (Ω) |
| Tcell | Battery cell temperature (°C) |
| UOCV | Open circuit voltage (V) |
| Wpump | Pumping power (W) |
| ΔP | Inlet and outlet pressure difference (kPa) |
| Qw | Flow rate of water (L/min) |
| Q1 | Flow rate (L/min) |
| Tavg | Average temperature of battery module ((°C) |
| ΔTavg | Average temperature difference of battery module ((°C) |
| K1 | Smaller condition result |
| K2 | Standard condition result |
| K3 | Larger condition result |
| Ri | Influence range |
| * | Standard condition |
References
- Falih, H.; Hamed, A.J.; Khalifa, A.H.N. Techno-Economic Assessment of a Hybrid Connected PV Solar System. Int. J. Air-Cond. Refrig. 2022, 30, 1–15. [Google Scholar] [CrossRef]
- Liu, X.; Chen, Z.; Zhang, C.; Wu, J. A Novel Temperature-Compensated Model for Power Li-Ion Batteries with Dual-Particle-Filter State of Charge Estimation. Appl. Energy 2014, 123, 263–272. [Google Scholar] [CrossRef]
- Zhang, K.; An, Y.; Wei, C.; Qian, Y.; Zhang, Y.; Feng, J. High-Safety and Dendrite-Free Lithium Metal Batteries Enabled by Building a Stable Interface in a Nonflammable Medium-Concentration Phosphate Electrolyte. ACS Appl. Mater. Interfaces 2021, 13, 50869–50877. [Google Scholar] [CrossRef]
- Fang, Y.; Ye, F.; Zhu, Y.; Li, K.; Shen, J.; Su, L. Experimental Investigation on System Performances and Transient Response of a Pumped Two-Phase Battery Cooling System Using R1233zd. Energy Rep. 2020, 6, 238–247. [Google Scholar] [CrossRef]
- Pinson, M.B.; Bazant, M.Z. Theory of SEI Formation in Rechargeable Batteries: Capacity Fade, Accelerated Aging and Lifetime Prediction. J. Electrochem. Soc. 2013, 160, A243–A250. [Google Scholar] [CrossRef]
- Ramadass, P.; Haran, B.; White, R.; Popov, B.N. Capacity Fade of Sony 18650 Cells Cycled at Elevated Temperatures: Part, I. Cycling Performance. J. Power Sources 2002, 112, 606–613. [Google Scholar] [CrossRef]
- Wu, M.S.; Chiang, P.C.J. High-Rate Capability of Lithium-Ion Batteries after Storing at Elevated Temperature. Electrochim. Acta 2007, 52, 3719–3725. [Google Scholar] [CrossRef]
- Ping, P.; Wang, Q.; Huang, P.; Sun, J.; Chen, C. Thermal Behaviour Analysis of Lithium-Ion Battery at Elevated Temperature Using Deconvolution Method. Appl. Energy 2014, 129, 261–273. [Google Scholar] [CrossRef]
- Giuliano, M.R.; Prasad, A.K.; Advani, S.G. Experimental Study of an Air-Cooled Thermal Management System for High Capacity Lithium–Titanate Batteries. J. Power Sources 2012, 216, 345–352. [Google Scholar] [CrossRef]
- Wang, H.; Ma, L. Thermal Management of a Large Prismatic Battery Pack Based on Reciprocating Flow and Active Control. Int. J. Heat. Mass. Transf. 2017, 115, 296–303. [Google Scholar] [CrossRef]
- Fan, Y.; Bao, Y.; Ling, C.; Chu, Y.; Tan, X.; Yang, S. Experimental Study on the Thermal Management Performance of Air Cooling for High Energy Density Cylindrical Lithium-Ion Batteries. Appl. Therm. Eng. 2019, 155, 96–109. [Google Scholar] [CrossRef]
- Yu, X.; Lu, Z.; Zhang, L.; Wei, L.; Cui, X.; Jin, L. Experimental Study on Transient Thermal Characteristics of Stagger-Arranged Lithium-Ion Battery Pack with Air Cooling Strategy. Int. J. Heat. Mass. Transf. 2019, 143, 118576. [Google Scholar] [CrossRef]
- Zhou, H.; Zhou, F.; Xu, L.; Kong, J. QingxinYang Thermal Performance of Cylindrical Lithium-Ion Battery Thermal Management System Based on Air Distribution Pipe. Int. J. Heat. Mass. Transf. 2019, 131, 984–998. [Google Scholar] [CrossRef]
- Liu, H.; Wei, Z.; He, W.; Zhao, J. Thermal Issues about Li-Ion Batteries and Recent Progress in Battery Thermal Management Systems: A Review. Energy Convers. Manag. 2017, 150, 304–330. [Google Scholar] [CrossRef]
- Dubey, P.; Pulugundla, G.; Srouji, A.K. Direct Comparison of Immersion and Cold-Plate Based Cooling for Automotive Li-Ion Battery Modules. Energies 2021, 14, 1259. [Google Scholar] [CrossRef]
- Liu, J.; Fan, Y.; Wang, J.; Tao, C.; Chen, M. A Model-Scale Experimental and Theoretical Study on a Mineral Oil-Immersed Battery Cooling System. Renew. Energy 2022, 201, 712–723. [Google Scholar] [CrossRef]
- Kim, M.; Ham, J.; Shin, D.; Cho, H. Comparison of Cooling Performance in a Cylindrical Battery with Single-Phase Direct Contact Cooling under Various Operating Conditions. Batteries 2022, 8, 195. [Google Scholar] [CrossRef]
- Roe, C.; Feng, X.; White, G.; Li, R.; Wang, H.; Rui, X.; Li, C.; Zhang, F.; Null, V.; Parkes, M.; et al. Immersion Cooling for Lithium-Ion Batteries—A Review. J. Power Sources 2022, 525, 231094. [Google Scholar] [CrossRef]
- Pulugundla, G.; Dubey, P.; Srouji, A. Time-Accurate CFD Analysis of Liquid Cold Plates for Efficient Thermal Performance of Electric Vehicle Li-Ion Battery Modules. SAE Tech. Pap. 2019, 2019, 01-0500. [Google Scholar] [CrossRef]
- Lai, Y.; Wu, W.; Chen, K.; Wang, S.; Xin, C. A Compact and Lightweight Liquid-Cooled Thermal Management Solution for Cylindrical Lithium-Ion Power Battery Pack. Int. J. Heat. Mass. Transf. 2019, 144, 118581. [Google Scholar] [CrossRef]
- Zhou, H.; Zhou, F.; Zhang, Q.; Wang, Q.; Song, Z. Thermal Management of Cylindrical Lithium-Ion Battery Based on a Liquid Cooling Method with Half-Helical Duct. Appl. Therm. Eng. 2019, 162, 114257. [Google Scholar] [CrossRef]
- Zhao, C.; Cao, W.; Dong, T.; Jiang, F. Thermal Behavior Study of Discharging/Charging Cylindrical Lithium-Ion Battery Module Cooled by Channeled Liquid Flow. Int. J. Heat. Mass. Transf. 2018, 120, 751–762. [Google Scholar] [CrossRef]
- Qian, Z.; Li, Y.; Rao, Z. Thermal Performance of Lithium-Ion Battery Thermal Management System by Using Mini-Channel Cooling. Energy Convers. Manag. 2016, 126, 622–631. [Google Scholar] [CrossRef]
- Lv, Y.; Zhou, D.; Yang, X.; Liu, X.; Li, X.; Zhang, G. Experimental Investigation on a Novel Liquid-Cooling Strategy by Coupling with Graphene-Modified Silica Gel for the Thermal Management of Cylindrical Battery. Appl. Therm. Eng. 2019, 159, 113885. [Google Scholar] [CrossRef]
- Wu, W.; Yang, X.; Zhang, G.; Chen, K.; Wang, S. Experimental Investigation on the Thermal Performance of Heat Pipe-Assisted Phase Change Material Based Battery Thermal Management System. Energy Convers. Manag. 2017, 138, 486–492. [Google Scholar] [CrossRef]
- Nelson, P.; Dees, D.; Amine, K.; Henriksen, G. Modeling Thermal Management of Lithium-Ion PNGV Batteries. J. Power Sources 2002, 110, 349–356. [Google Scholar] [CrossRef]
- Kim, M. Performance Analysis of a Direct Contact Battery Cooling System Using R134a. Master’s Thesis, Chosun University, Gwangju, Republic of Korea, 2023. [Google Scholar]
- Yadav, V.; Baghel, K.; Kumar, R.; Kadam, S.T. Numerical Investigation of Heat Transfer in Extended Surface Microchannels. Int. J. Heat. Mass. Transf. 2016, 93, 612–622. [Google Scholar] [CrossRef]
- Panchal, S.; Khasow, R.; Dincer, I.; Agelin-Chaab, M.; Fraser, R.; Fowler, M. Numerical Modeling and Experimental Investigation of a Prismatic Battery Subjected to Water Cooling. Numer. Heat. Transf. Part A Appl. 2017, 71, 626–637. [Google Scholar] [CrossRef]
- Sheng, L.; Zhang, Z.; Su, L.; Zhang, H.; Zhang, H.; Li, K.; Fang, Y.; Ye, W. A Calibration Calorimetry Method to Investigate the Thermal Characteristics of a Cylindrical Lithium-Ion Battery. Int. J. Therm. Sci. 2021, 165, 106891. [Google Scholar] [CrossRef]
- Wang, J.; Liu, X.; Liu, F.; Liu, Y.; Wang, F.; Yang, N. Numerical Optimization of the Cooling Effect of the Bionic Spider-Web Channel Cold Plate on a Pouch Lithium-Ion Battery. Case Stud. Therm. Eng. 2021, 26, 101124. [Google Scholar] [CrossRef]
- An, S.J.; Li, J.; Daniel, C.; Mohanty, D.; Nagpure, S.; Wood, D.L. The State of Understanding of the Lithium-Ion-Battery Graphite Solid Electrolyte Interphase (SEI) and Its Relationship to Formation Cycling. Carbon 2016, 105, 52–76. [Google Scholar] [CrossRef]
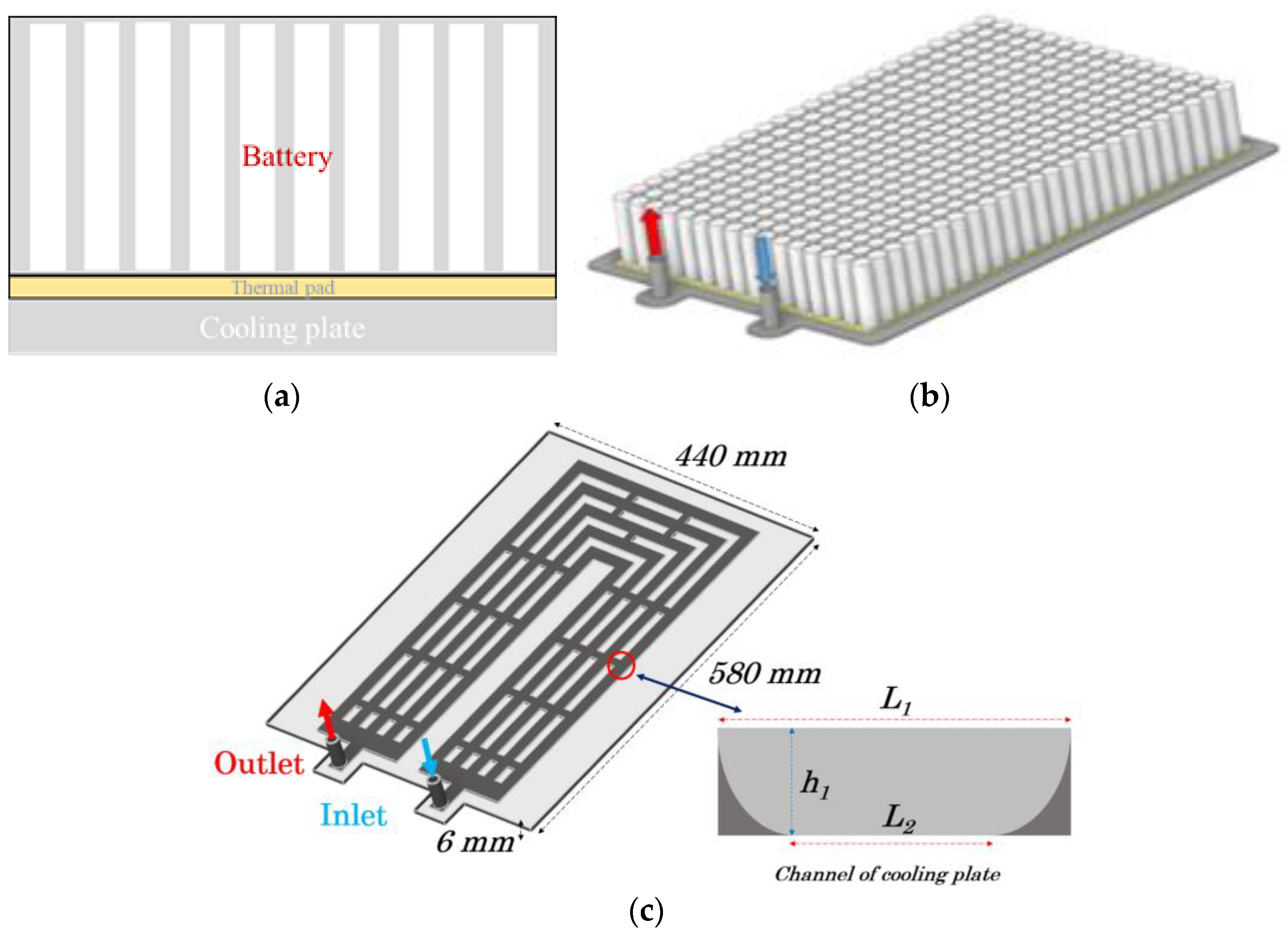
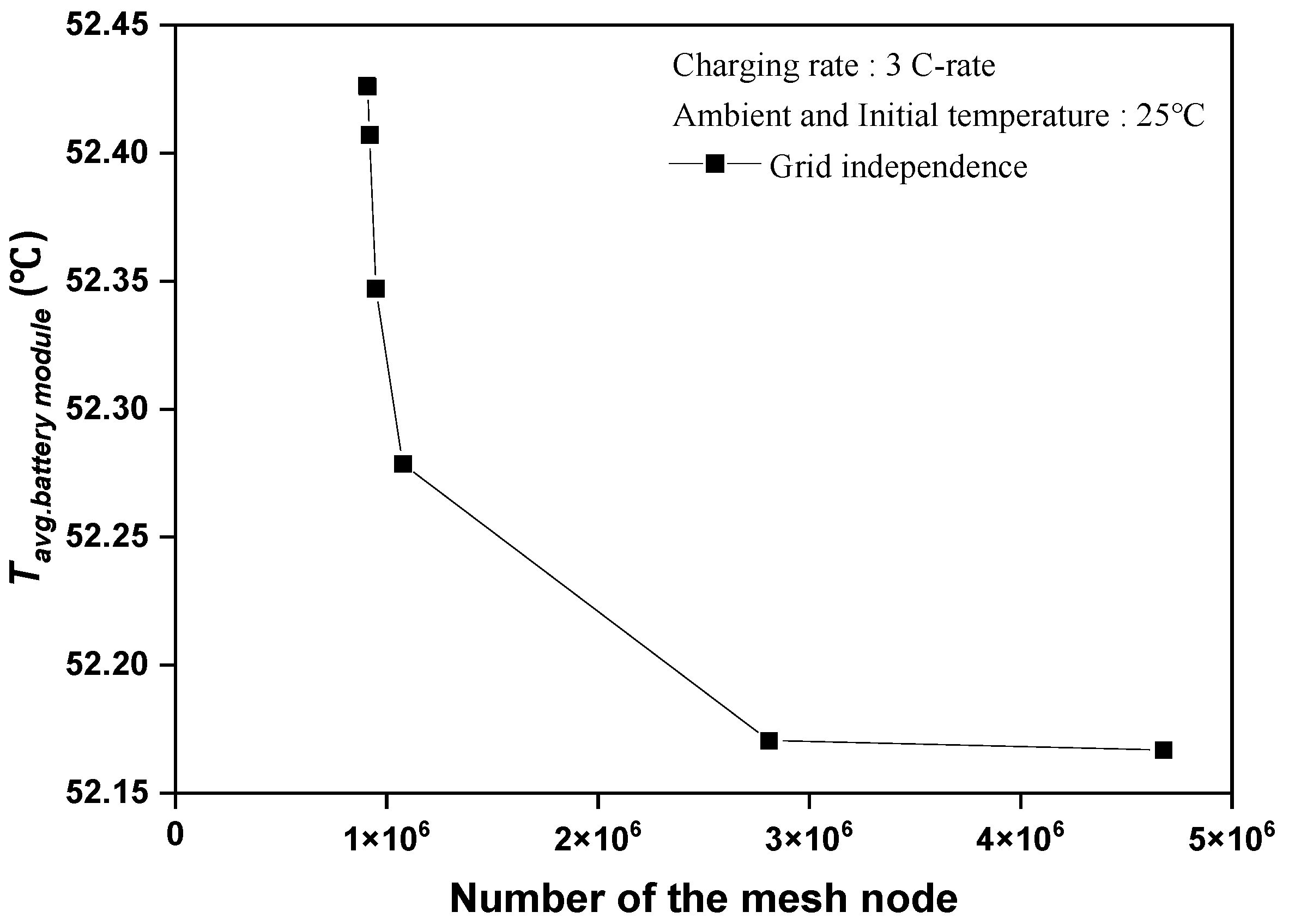

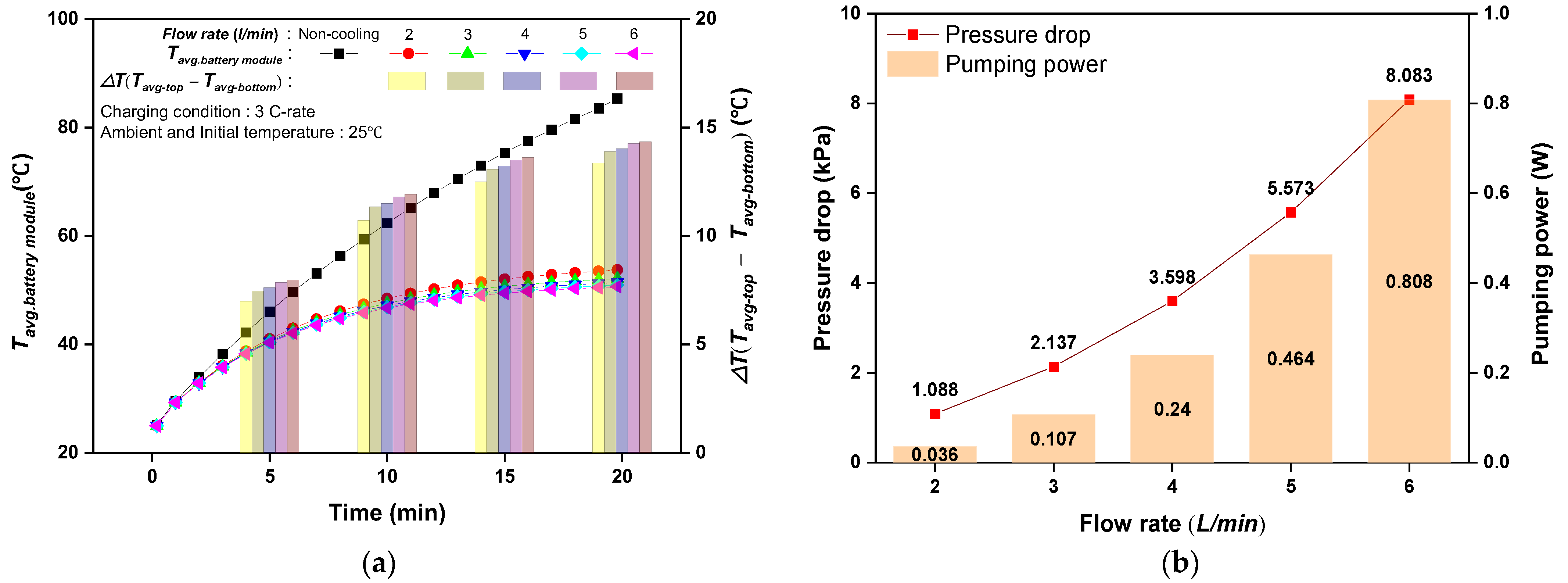
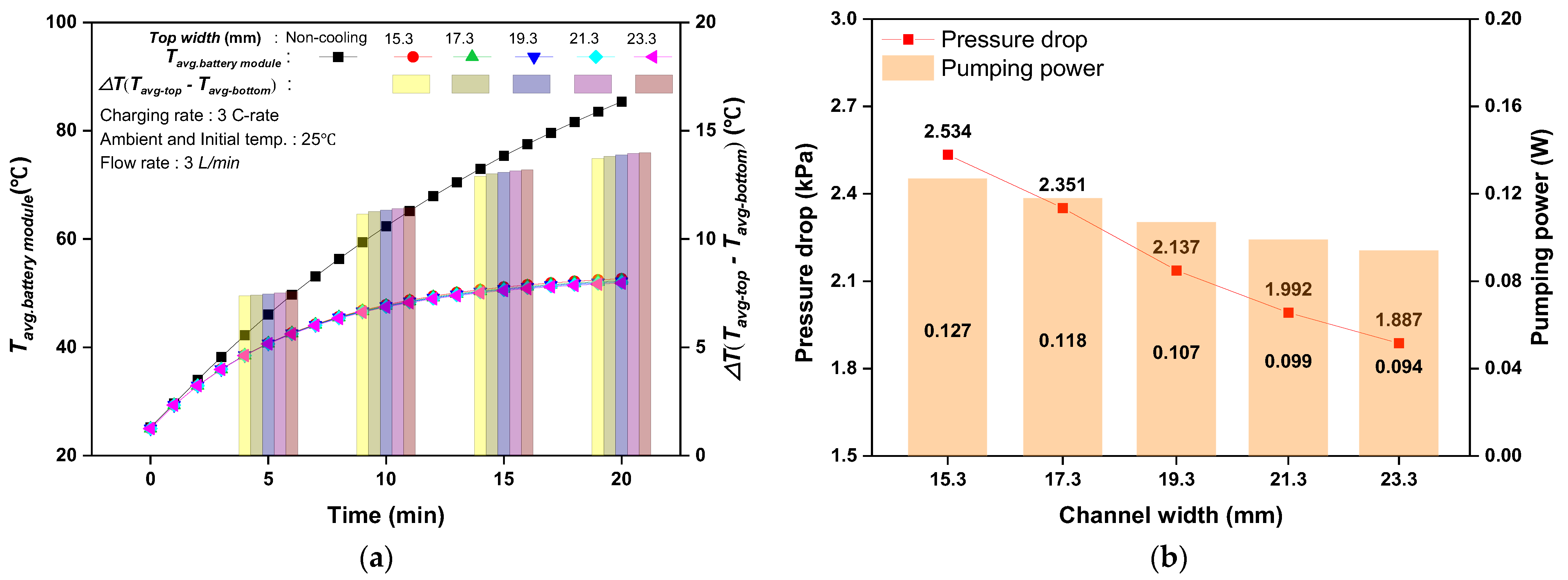
| Parameter | Specification |
|---|---|
| Diameter (mm) | 21 |
| Height (mm) | 70 |
| Weight (g) | 67.5 |
| Nominal capacity (Ah) | 4.95 |
| Nominal voltage (V) | 3.69 |
| Charge cut-off voltage (V) | 4.25 |
| Parameter | Specification |
|---|---|
| Density (kg/m3) | 765.33 + 1.8142T − 0.0035T2 |
| Specific heat (J/kg∙K) | 28070 − 281.7T + 1.25T2 − 2.48 × 10−3T3 + 1.857 × 10−6T4 |
| Thermal conductivity (W/m∙K) | −0.5752 + 6.397 × 10−3T − 8.151 × 10−6T2 |
| Viscosity (Pa·s) | 9.67 × 10−2 − 8.207 × 10−4T + 2.344 × 10−6T2 − 2.244 × 10−9T3 |
| Parameters | Range | |
|---|---|---|
| Boundary condition and flow rate | Ambient temperature (°C) | 25 |
| Initial temperature (°C) | 25 | |
| Battery volumetric heat generation (W/m3) | 225,000 | |
| Heat transfer coefficient (W/m2∙K) | 10 | |
| Flow rate (L/min) | 2, 3 *, 4, 5, 6 | |
| Channel shape | Top (L1)/Bottom width (L2) (mm) | 15.3/8.1, 17.3/10.1, 19.3/12.1 *, 21.3/14.1, 23.3/16.1 |
| Height (h1) (mm) | 3.6 | |
| Number of the channel (n1) | 4, 5 *, 6 |
| [°C] | [°C] | [W] | ||
|---|---|---|---|---|
| 53.77 | 13.37 | 0.04 | ||
| 52.17 | 13.88 | 0.11 | ||
| 51.43 | 14.02 | 0.24 | ||
| 2.34 | 0.65 | 0.20 | ||
| 52.36 | 13.82 | 0.12 | ||
| 52.17 | 13.88 | 0.11 | ||
| 52.00 | 13.94 | 0.10 | ||
| 0.36 | 0.12 | 0.02 | ||
| 53.27 | 13.48 | 0.12 | ||
| 52.17 | 13.88 | 0.11 | ||
| 51.72 | 14.04 | 0.09 | ||
| 1.55 | 0.56 | 0.03 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
You, N.; Ham, J.; Shin, D.; Cho, H. Performance Analysis of the Liquid Cooling System for Lithium-Ion Batteries According to Cooling Plate Parameters. Batteries 2023, 9, 538. https://doi.org/10.3390/batteries9110538
You N, Ham J, Shin D, Cho H. Performance Analysis of the Liquid Cooling System for Lithium-Ion Batteries According to Cooling Plate Parameters. Batteries. 2023; 9(11):538. https://doi.org/10.3390/batteries9110538
Chicago/Turabian StyleYou, Nayoung, Jeonggyun Ham, Donghyeon Shin, and Honghyun Cho. 2023. "Performance Analysis of the Liquid Cooling System for Lithium-Ion Batteries According to Cooling Plate Parameters" Batteries 9, no. 11: 538. https://doi.org/10.3390/batteries9110538
APA StyleYou, N., Ham, J., Shin, D., & Cho, H. (2023). Performance Analysis of the Liquid Cooling System for Lithium-Ion Batteries According to Cooling Plate Parameters. Batteries, 9(11), 538. https://doi.org/10.3390/batteries9110538









