Modeling and Experimental Investigation of the Interaction between Pressure-Dependent Aging and Pressure Development Due to the Aging of Lithium-Ion Cells
Abstract
:1. Introduction
1.1. Motivation and Background
1.2. Contribution and Organization
- (1)
- explicit characterization methods that apply the pressure to the cell. The pressure is adjusted independently or almost independently of the aging and charge state of the cell from the external.
- (2)
- implicit characterization methods are characterized by the fact that the pressure arises as a result of cell expansion.
2. Theoretical Modeling
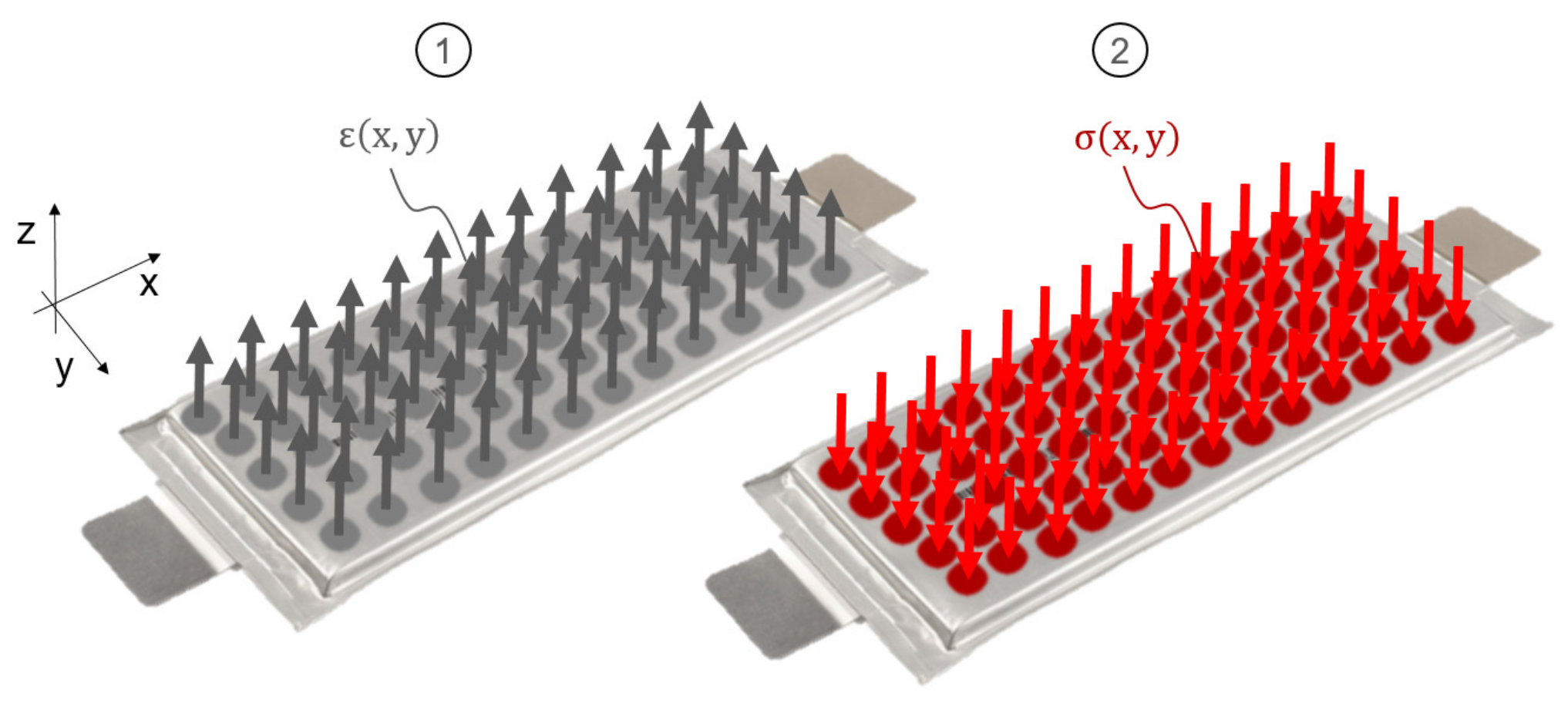
2.1. Modeling of Irreveribeles Cell Swelling
2.2. Consideration of Ealstomachnics
2.3. Characterization Method
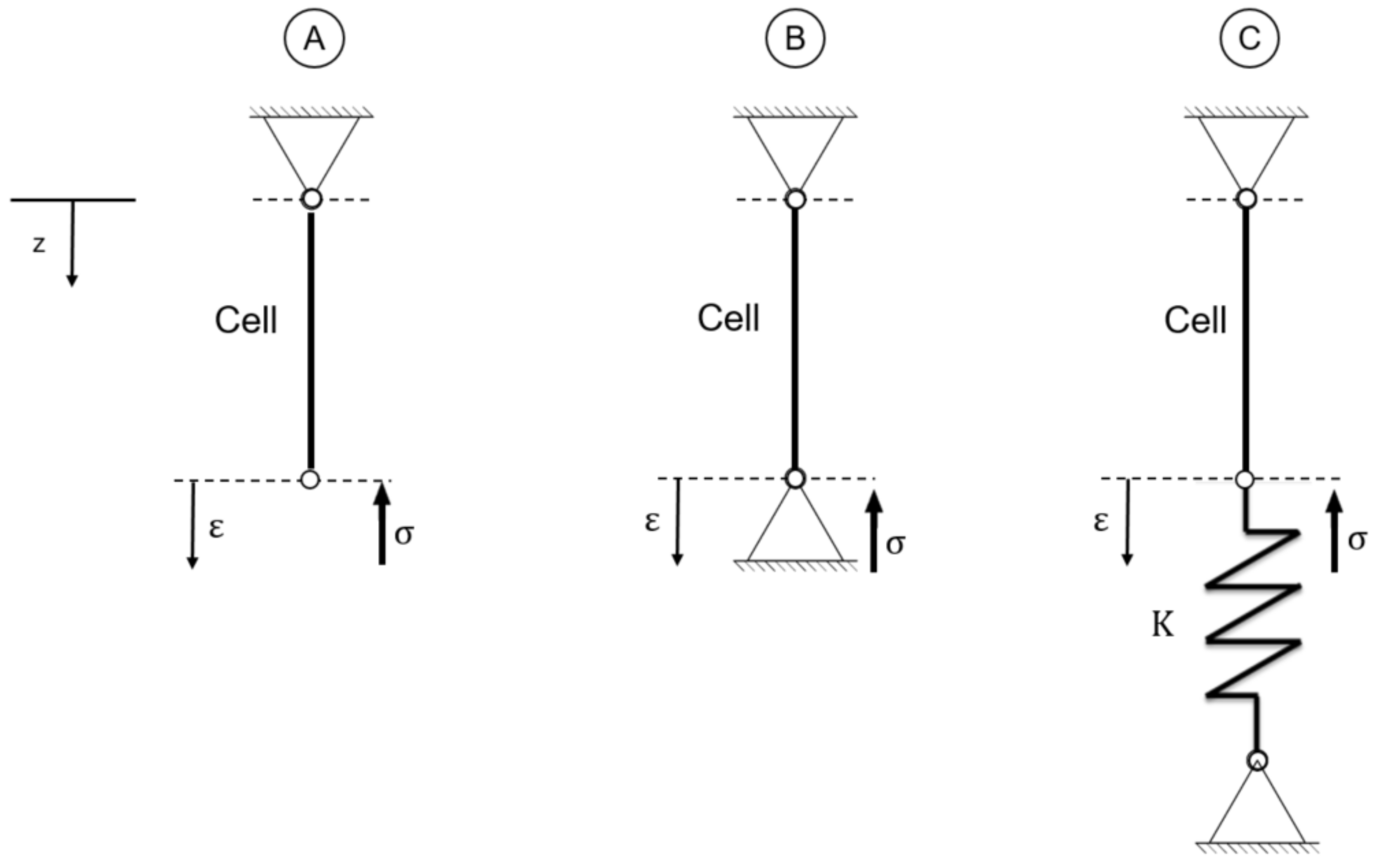
2.3.1. Constant Force Scenario
2.3.2. Constant Gap Scenario
2.3.3. Constant Stiffness Scenario
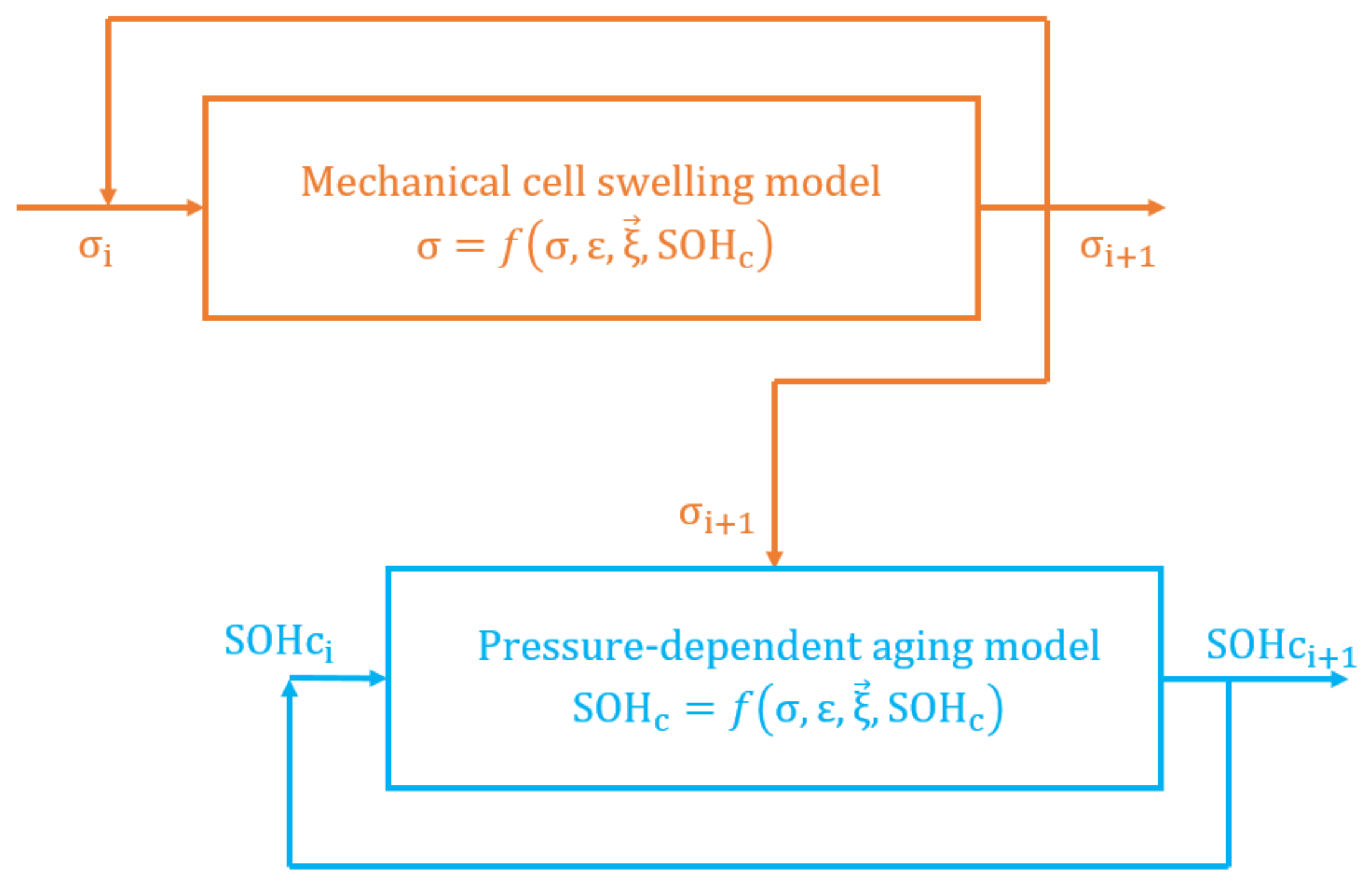
2.4. Aging Model
2.5. Solution of Model Equations
3. Test Setup
3.1. Test Procedure
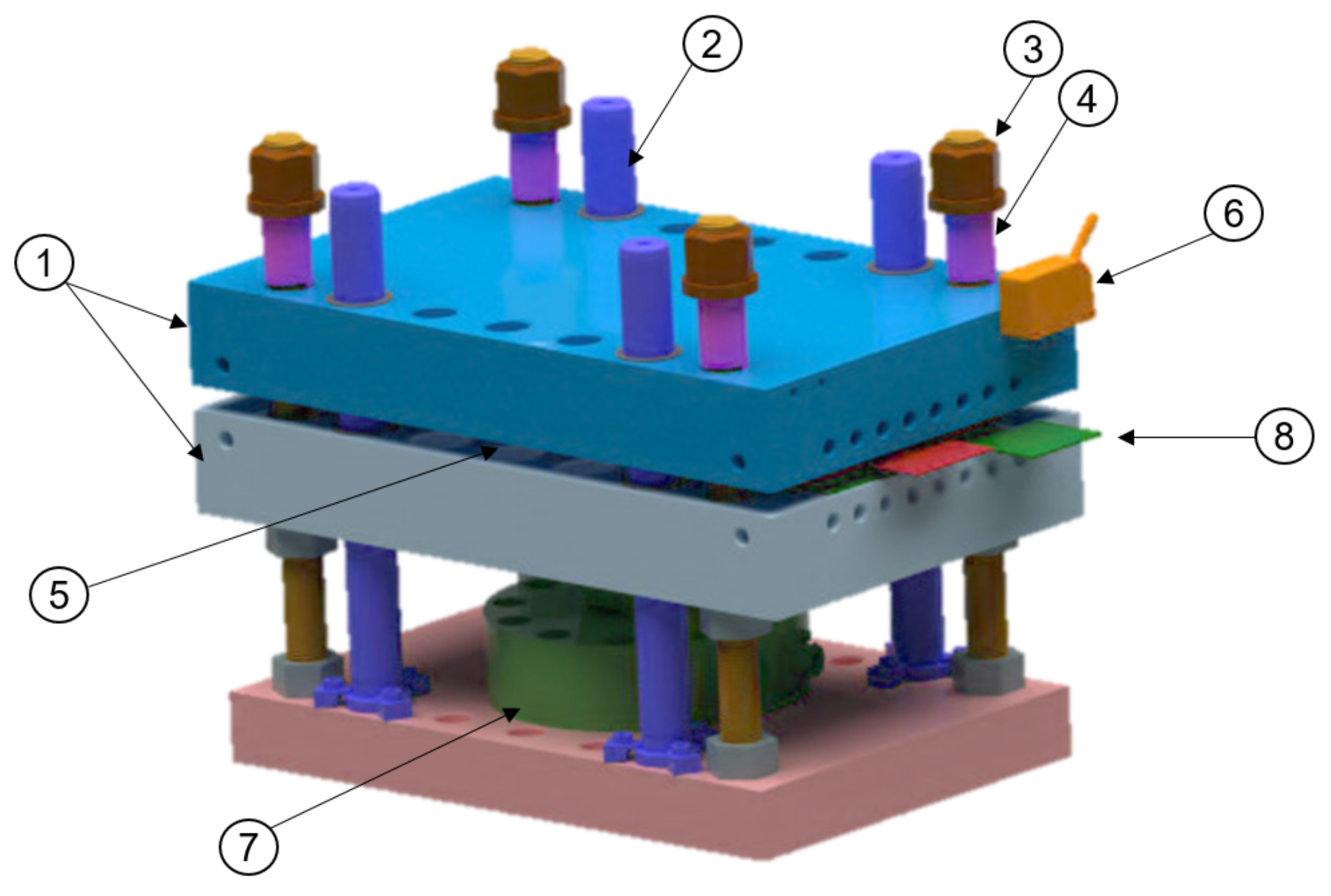
3.2. Measurement Setup
3.3. Test Matrix and Cycling
4. Evaluation, Modeling and Parameterization
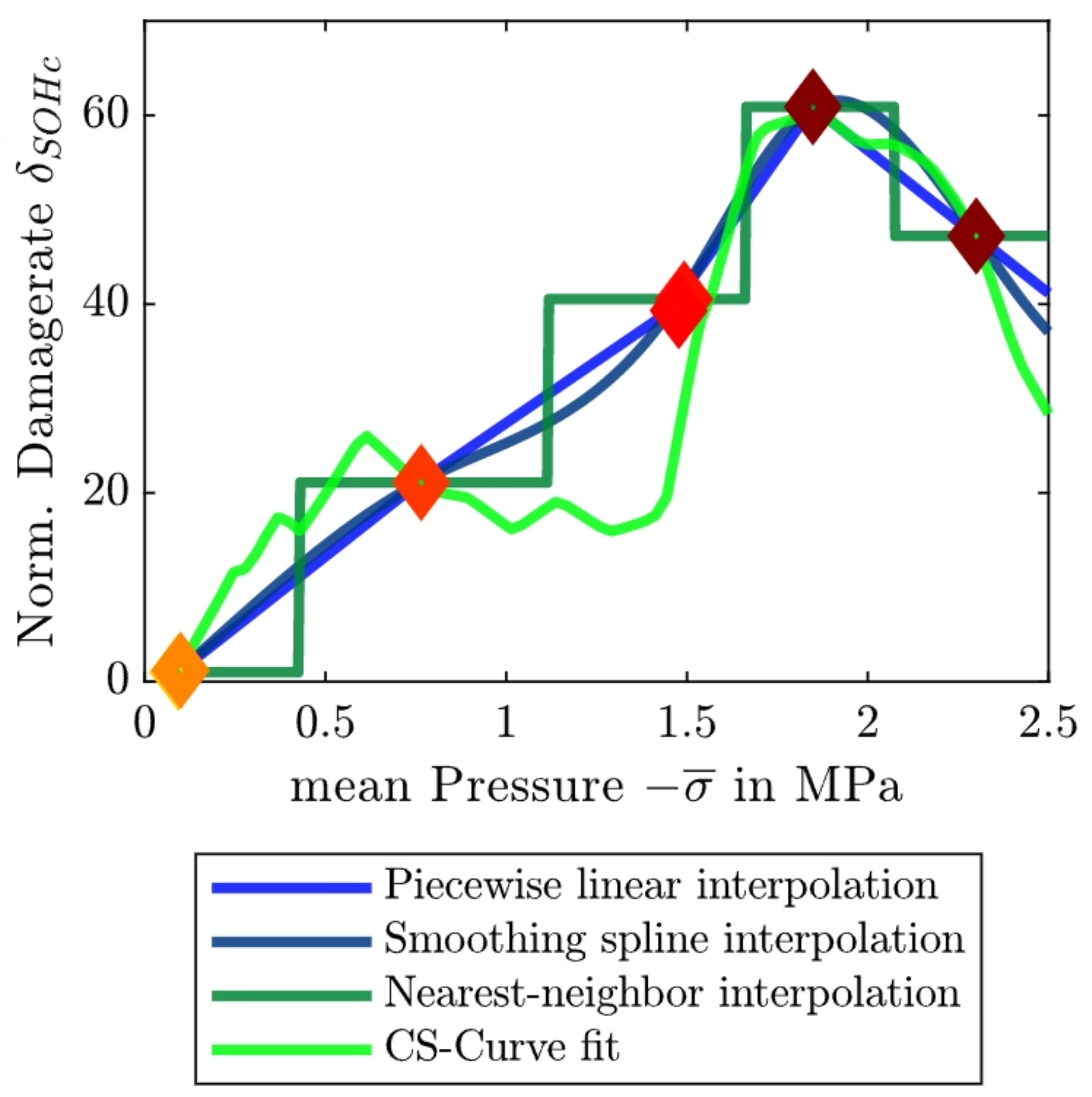
4.1. Aging Model Parameterization via Constant Force Measurements
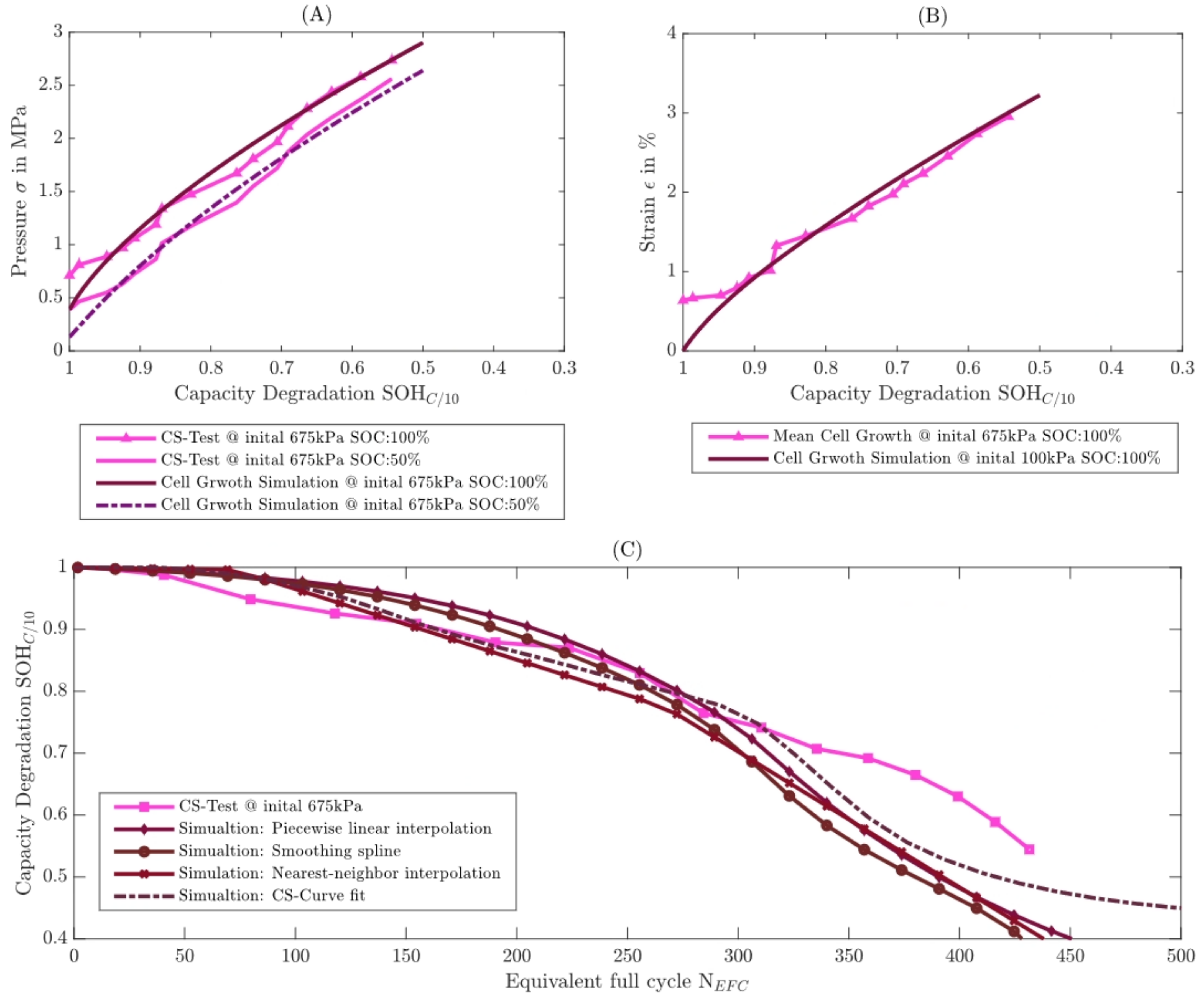
- Piecewise linear interpolation
- Smoothing spline
- Nearest-neighbor interpolation.
4.2. Mechanical Model Parameterization of Irreversible Cell Growth
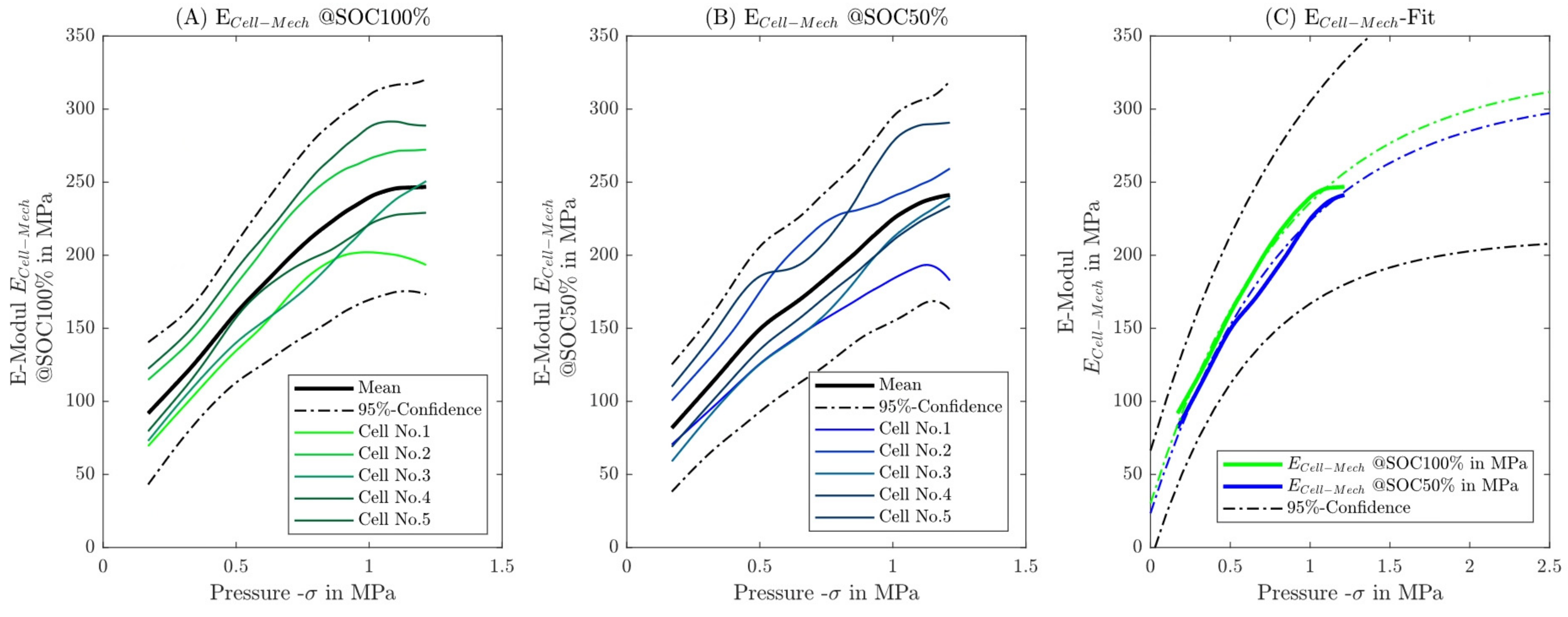
4.3. Mechanical Model Parameterization of Cell Stiffness
4.3.1. Elastomechanical Cell Stiffness
4.3.2. Electrochemical Cell Stiffness
4.3.3. Total Cell Stiffness
4.4. Modeling for Linear Full Cell Element
4.5. Consideration of the Boundary Conditions from the Measurement Setup
- Optimal central placement of the cell
- Homogeneous cell surface
- Optimal homogeneous cell aging and cell expansion
- Homogeneous pressure application and distribution by threaded rods.
| Algorithm 1: Recursive numerical solution algorithms |
| Initialization ) |
| Force-Prediction-Step: |
| Displacement-Correction-Step: |
5. Simulation and Validation
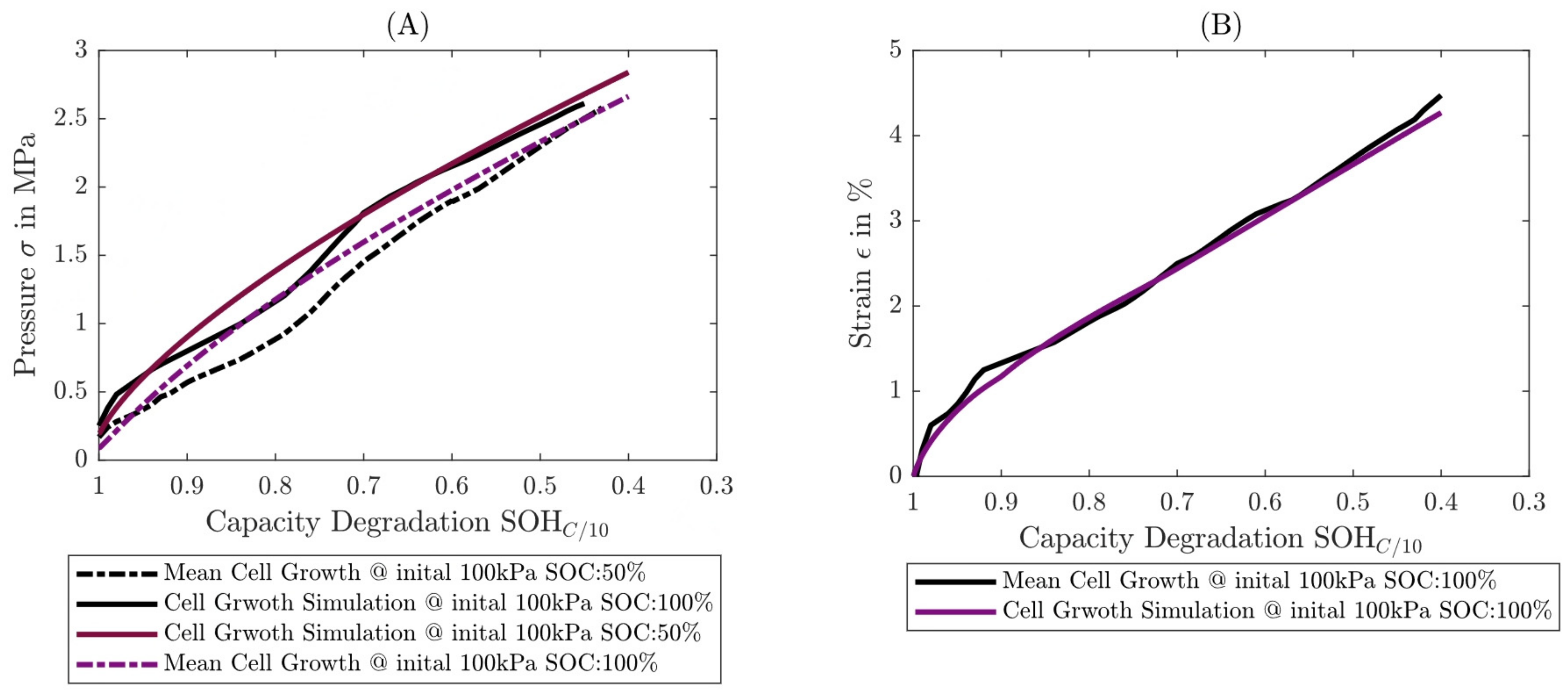
5.1. Comparison of the Simulation with the Validation Measurement I
5.2. Comparison of the Simulation with the Validation Measurement II
6. Discussion and Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Brand, M.J.; Schuster, S.F.; Bach, T.; Fleder, E.; Stelz, M.; Gläser, S.; Müller, J.; Sextl, G.; Jossen, A. Effects of vibrations and shocks on lithium-ion cells. J. Power Sources 2015, 288, 62–69. [Google Scholar] [CrossRef]
- Pistorio, F.; Clerici, D.; Mocera, F. Review on the Experimental Characterization of Fracture in Active Material for Lithium-Ion Batteries. Energies 2022, 15, 9168. [Google Scholar] [CrossRef]
- Jones, E.M.C.; Çapraz, Ö.Ö.; White, S.R.; Sottos, N.R. Reversible and Irreversible Deformation Mechanisms of Composite Graphite Electrodes in Lithium-Ion Bateries. J. Electrochem. Soc. 2016, 163, A1965. [Google Scholar] [CrossRef]
- Daubinger, P.; Schelter, M.; Petersohn, R.; Nagler, F.; Hartmann, S.; Herrmann, M.; Giffin, G.A. Impact of Bracing on Large Format Prismatic Lithium-Ion Battery Cells during Aging. Adv. Energy Mater. 2022, 12, 2102448. [Google Scholar] [CrossRef]
- Mussa, A.S.; Klett, M.; Lindbergh, G.; Lindström, R.W. Effects of external pressure on the performance and ageing of single-layer lithium-ion pouch cells. J. Power Sources 2018, 385, 18–26. [Google Scholar] [CrossRef]
- Deich, T.; Storch, M.; Steiner, K.; Bund, A. Effects of module stiffness and initial compression on lithium-ion cell aging. J. Power Sources 2021, 506, 230163. [Google Scholar] [CrossRef]
- Cannarella, J.; Arnold, C.B. Stress evolution and capacity fade in constrained lithium-ion pouch cells. J. Power Sources 2014, 245, 745–751. [Google Scholar] [CrossRef]
- Mohtat, P.; Lee, S.; Siegel, J.B.; Stefanopoulou, A.G. Reversible and Irreversible Expansion of Lithium-Ion Batteries under a Wide Range of Stress Factors. J. Electrochem. Soc. 2021, 168, 100520. [Google Scholar] [CrossRef]
- Grimsmann, F.; Brauchle, F.; Gerbert, T.; Gruhle, A.; Parisi, J.; Knipper, M. Impact of different aging mechanisms on the thickness change and the quick-charge capability of lithium-ion cells. J. Energy Storage 2017, 14, 158–162. [Google Scholar] [CrossRef]
- Hahn, S.; Theil, S.; Kroggel, J.; Birke, K.P. Pressure Prediction Modeling and Validation for Lithium-Ion Pouch Cells in Buffered Module Assemblies. J. Energy Storage 2021, 40, 102517. [Google Scholar] [CrossRef]
- Louli, A.J.; Li, J.; Trussler, S.; Fell, C.R.; Dahn, J.R. Volume, pressure and thickness evolution of li-ion pouch cells with silicon-composite negative electrodes. J. Electrochem. Soc. 2017, 164, A2689. [Google Scholar] [CrossRef]
- Pegel, H.; von Kessel, O.; Heugel, P.; Deich, T.; Tübke, J.; Birke, K.P.; Sauer, D.U. Volume and thickness change of NMC811|SiOx-graphite large-format lithium-ion cells: From pouch cell to active material level. J. Power Sources 2022, 537, 231443. [Google Scholar] [CrossRef]
- Spingler, F.B.; Kücher, S.; Phillips, R.; Moyassari, E.; Jossen, A. Electrochemically Stable In Situ Dilatometry of NMC, NCA and Graphite Electrodes for Lithium-Ion Cells Compared to XRD Measurements. J. Electrochem. Soc. 2021, 168, 040515. [Google Scholar] [CrossRef]
- Aufschläger, A.; Kücher, S.; Kraft, L.; Spingler, F.; Niehoff, P.; Jossen, A. High precision measurement of reversible swelling and electrochemical performance of flexibly compressed 5Ah NMC622/graphite lithium-ion pouch cells. J. Energy Storage 2023, 59, 106483. [Google Scholar] [CrossRef]
- Cannarella, J.; Arnold, C.B. State of health and charge measurements in lithium-ion batteries using mechanical stress. J. Power Sources 2014, 269, 7–14. [Google Scholar] [CrossRef]
- Sauerteig, D.; Ivanov, S.; Reinshagen, H.; Bund, A. Reversible and irreversible dilation of lithium-ion battery electrodes investigated by in-situ dilatometry. J. Power Sources 2017, 342, 939–946. [Google Scholar] [CrossRef]
- Ivanov, S.; Sauerteig, D.; Dimitrova, A.; Krischok, S.; Bund, A. Irreversible dilation of graphite composite anodes influenced by vinylene carbonate. J. Power Sources 2020, 457, 228020. [Google Scholar] [CrossRef]
- Louli, A.J.; Ellis, L.D.; Dahn, J.R. Operando Pressure Measurements Reveal Solid Electrolyte Interphase Growth to Rank Li-Ion Cell Performance. Joule 2018, 3, 745–761. [Google Scholar] [CrossRef]
- Bitzer, B.; Gruhle, A. A new method for detecting lihium plating by measuring the cell thickness. J. Power Sources 2014, 262, 297–302. [Google Scholar] [CrossRef]
- Adam, A.; Wandt, J.; Knobbe, E.; Bauer, G.; Kwade, A. Fast-Charging of Automotive Lithium-Ion Cells: In-Situ Lithium-Plating Detection and Comparison of Different Cell Designs. J. Electrochem. Soc. 2020, 167, 130503. [Google Scholar] [CrossRef]
- Zhao, Y.; Spingler, F.B.; Patel, Y.; Offer, G.J.; Jossen, A. Localized Swelling Inhomogeneity Detection in Lithium Ion Cells Using Multi-Dimensional Laser Scanning. J. Electrochem. Soc. 2019, 166, A27–A34. [Google Scholar] [CrossRef]
- Li, J.; Dozier, A.K.; Li, Y.; Yang, F.; Cheng, Y.-T. Crack Pattern Formation in Thin Film Lithium-Ion Battery Electrodes. J. Electrochem. Soc. 2011, 158, A689–A694. [Google Scholar] [CrossRef]
- Vetter, J.; Novák, P.; Wagner, M.R.; Veit, C.; Möller, K.C.; Besenhard, J.O.; Winter, M.; Wohlfahrt-Mehrens, M.; Vogler, C.; Hammouche, A. Ageing mechanisms in lithium-ion batteries. J. Power Sources 2005, 147, 269–281. [Google Scholar] [CrossRef]
- Li, T.; Yuan, X.-Z.; Zhang, L.; Song, D.; Shi, K.; Bock, C. Degradation Mechanisms and Mitigation Strategies of Nickel-Rich NMC-Based Lithium-Ion Batteries. Electrochem. Energy Rev. 2020, 3, 43–80. [Google Scholar] [CrossRef]
- Lin, N.; Jia, Z.; Wang, Z.; Zhao, H.; Ai, G.; Song, X.; Bai, Y.; Battaglia, V.; Sun, C.; Qiao, J.; et al. Understanding the crack formation of graphite particles in cycled commercial lithium-ion batteries by focused ion beam–scanning electron microscopy. J. Power Sources 2017, 365, 235–239. [Google Scholar] [CrossRef]
- Leonard, A.; Planden, B.; Lukow, K.; Morrey, D. Investigation of constant stack pressure on lithium-ion battery performance. J. Energy Storage 2023, 72, 108422. [Google Scholar] [CrossRef]
- Attia, P.M.; Bills, A.; Planella, F.B.; Dechent, P.; Dos Reis, G.; Dubarry, M.; Gasper, P.; Gilchrist, R.; Greenbank, S.; Howey, D.; et al. Review—“Knees” in Lithium-Ion Battery Aging Trajectories. J. Electrochem. Soc. 2022, 169, 060517. [Google Scholar] [CrossRef]
- Bach, T.C.; Schuster, S.F.; Fleder, E.; Müller, J.; Brand, M.J.; Lorrmann, H.; Jossen, A.; Sextl, G. Nonlinear aging of cylindrical lithium-ion cells linked to heterogeneous compression. J. Energy Storage 2016, 5, 212. [Google Scholar] [CrossRef]
- Müller, V.; Scurtu, R.-G.; Memm, M.; Danzer, M.A.; Wohlfahrt-Mehrens, M. Study of the influence of mechanical pressure on the performance and aging of Lithium-ion battery cells. J. Power Sources 2019, 440, 227148. [Google Scholar] [CrossRef]
- Deich, T.; Hahn, S.L.; Both, S.; Birke, K.P.; Bund, A. Validation of an actively-controlled pneumatic press to simulate automotive module stiffness for mechanically representative lithium-ion cell aging. J. Energy Storage 2020, 28, 101192. [Google Scholar] [CrossRef]
- Jeong, J.; Kwak, E.; Kim, J.-H.; Oh, K.-Y. Novel active management of compressive pressure on a lithium-ion battery using a phase transition actuator. Energy Rep. 2022, 8, 10762–10775. [Google Scholar] [CrossRef]
- Rieger, B.; Schlueter, S.; Erhard, S.V.; Schmalz, J.; Reinhart, G.; Jossen, A. Multi-scale investigation of thickness changes in a commercial pouch type lithium-ion battery. J. Energy Storage 2016, 6, 213–221. [Google Scholar] [CrossRef]
- Ebert, F.; Spielbauer, M.; Bruckmoser, M.; Lienkamp, M. Simulation of spatial strain inhomogeneities in lithium-ion-cells due to electrode dilation dependent on internal and external cell structures. J. Energy Storage 2022, 49, 104143. [Google Scholar] [CrossRef]
- Daubinger, P.; Göttlinger, M.; Hartmann, S.; Giffin, G.A. Consequences of Different Pressures and Electrolytes on the Irreversible Expansion of Lithium Metal Half Cells. Batter. Supercaps 2023, 6, e202200452. [Google Scholar] [CrossRef]
- von Kessel, O.; Deich, T.; Hahn, S.; Brauchle, F.; Vrankovic, D.; Soczka-Guth, T.; Birke, K.P. Mechanical impedance as a tool for electromechanical investigation and equivalent modeling of lithium-ion batteries. J. Power Sources 2021, 508, 230337. [Google Scholar] [CrossRef]
- Sauerteig, D.; Hanselmann, N.; Arzberger, A.; Reinshagen, H.; Ivanov, S.; Bund, A. Electrochemical-mechanical coupled modeling and parameterization of swelling and ionic transport in lithium-ion batteries. J. Power Sources 2018, 378, 235–247, ISSN 0378-7753. [Google Scholar] [CrossRef]
- Feiler, S.; Daubinger, P.; Gold, L.; Hartmann, S.; Giffin, G.A. Interplay between Elastic and Electrochemical Properties during Active Material Transitions and Aging of a Lithium-Ion Battery. Batter. Supercaps 2023, 6, e202200518. [Google Scholar] [CrossRef]
- Fill, A.; Avdyli, A.; Birke, K.P. Online Data-based Cell State Estimation of a Lithium-Ion Battery. In Proceedings of the 2020 2nd IEEE International Conference on Industrial Electronics for Sustainable Energy Systems (IESES), Cagliari, Italy, 1–3 September 2020; pp. 351–356. [Google Scholar]
- Avdyli, A.; Fill, A.; Birke, K.P. Statistical approach for continuous internal resistance estimation of lithium ion cells under dynamic loads. In Proceedings of the 2022 IEEE 21st Mediterranean Electrotechnical Conference (MELECON), Palermo, Italy, 14–16 June 2022; pp. 189–194. [Google Scholar]

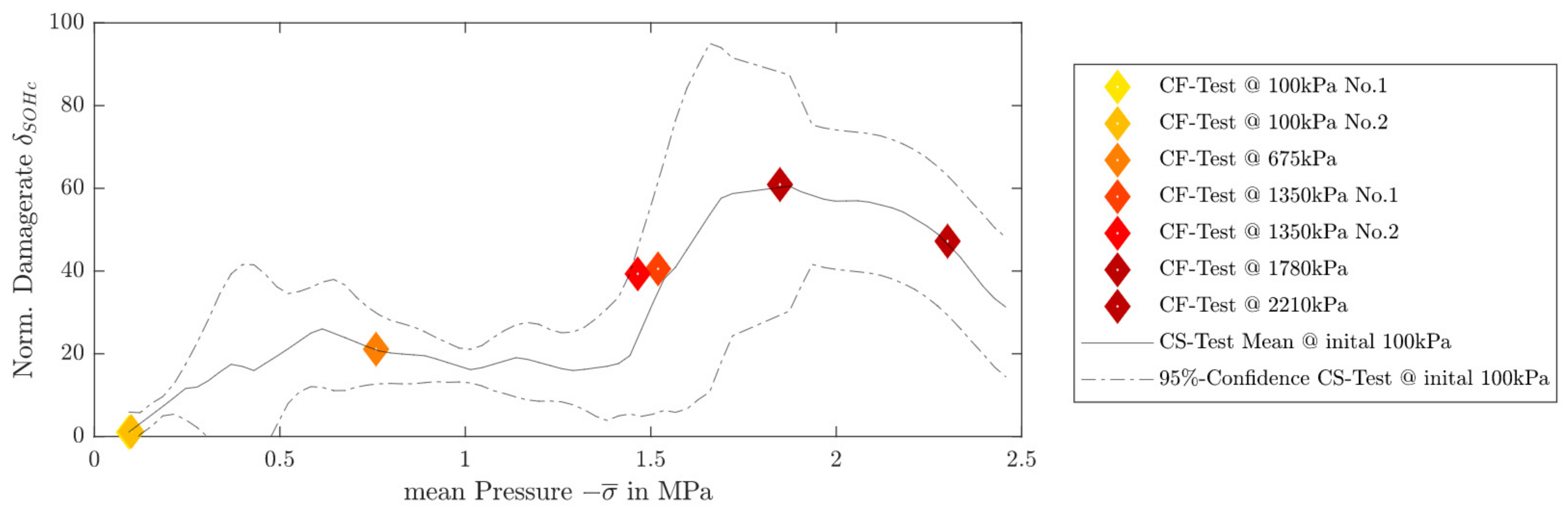
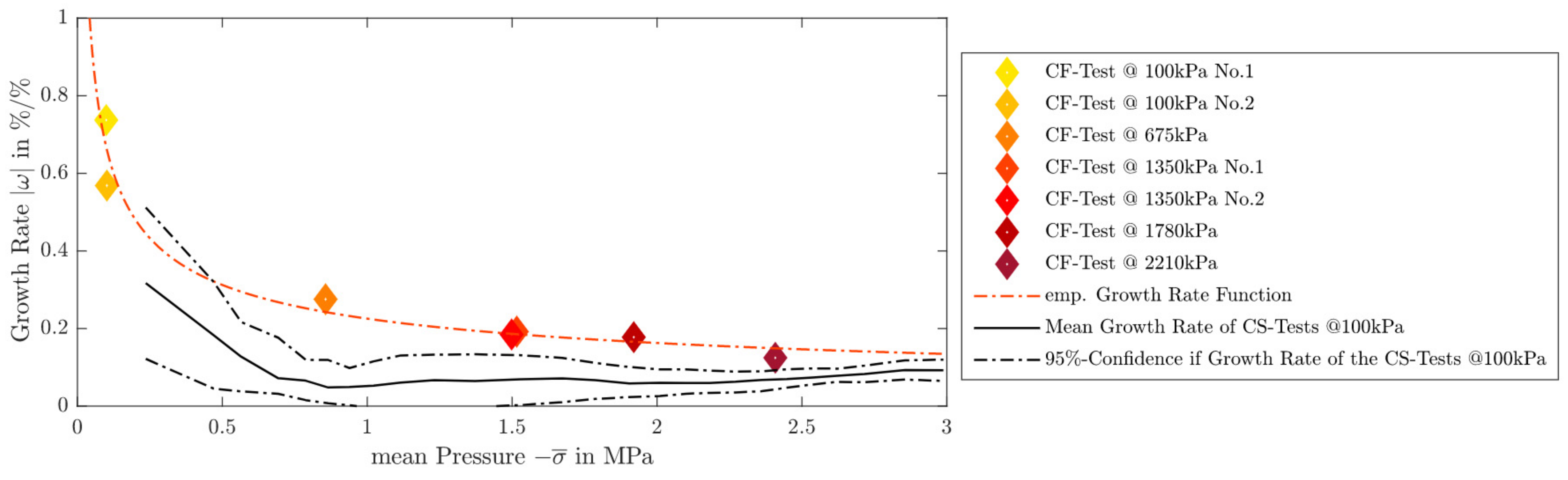


| Preload Condition @SOC30% | Charge-Profile | Discharge-Profile | Temperature | DOD | n |
|---|---|---|---|---|---|
| 100 kPa | 1.3 C | 1.3 C | 35 °C | 100% | 2 |
| 675 kPa | 1.3 C | 1.3 C | 35 °C | 100% | 1 |
| 1350 kPa | 1.3 C | 1.3 C | 35 °C | 100% | 1 |
| 1780 kPa | 1.3 C | 1.3 C | 35 °C | 100% | 1 |
| 2210 kPa | 1.3 C | 1.3 C | 35 °C | 100% | 1 |
| Preload Condition @SOC30% | Charge-Profile | Discharge-Profile | Temperature | DOD | n |
|---|---|---|---|---|---|
| 100 kPa | 1.3 C | 1.3 C | 35 °C | 100% | 3 |
| Parameter | SOC = 50% | SOC = 100% | Unit |
|---|---|---|---|
| α | 288.5 | 297.1 | MPa |
| 0.8403 | 0.8474 | MPa | |
| γ | 23.86 | 30.22 | MPa |
| Measurement Series | Preload Condition @SOC30% | Charge-Profile | Discharge-Profile | Temperature | DOD | n |
|---|---|---|---|---|---|---|
| I | 100 kPa | 1.3 C | 1.3 C | 35 °C | 100% | 3 |
| II | 675 kPa | 1.3 C | 1.3 C | 35 °C | 100% | 1 |
| Interpolation Method | σstd | 95%-CL | 99%-CL | |
|---|---|---|---|---|
| Piecewise linear interpolation | 18.55 | 13.52 | 26.5 | 34.83 |
| Smoothing spline interpolation | 16.62 | |||
| Nearst-neighbor interpolation | 53.83 | |||
| CS-Curve fit | 10.57 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Avdyli, A.; Fill, A.; Birke, K.P. Modeling and Experimental Investigation of the Interaction between Pressure-Dependent Aging and Pressure Development Due to the Aging of Lithium-Ion Cells. Batteries 2023, 9, 484. https://doi.org/10.3390/batteries9100484
Avdyli A, Fill A, Birke KP. Modeling and Experimental Investigation of the Interaction between Pressure-Dependent Aging and Pressure Development Due to the Aging of Lithium-Ion Cells. Batteries. 2023; 9(10):484. https://doi.org/10.3390/batteries9100484
Chicago/Turabian StyleAvdyli, Arber, Alexander Fill, and Kai Peter Birke. 2023. "Modeling and Experimental Investigation of the Interaction between Pressure-Dependent Aging and Pressure Development Due to the Aging of Lithium-Ion Cells" Batteries 9, no. 10: 484. https://doi.org/10.3390/batteries9100484
APA StyleAvdyli, A., Fill, A., & Birke, K. P. (2023). Modeling and Experimental Investigation of the Interaction between Pressure-Dependent Aging and Pressure Development Due to the Aging of Lithium-Ion Cells. Batteries, 9(10), 484. https://doi.org/10.3390/batteries9100484








