Experimental Study of Heat Generation Rate during Discharge of LiFePO4 Pouch Cells of Different Nominal Capacities and Thickness
Abstract
1. Introduction
2. Purpose of the Present Investigation
3. Experimental
3.1. Test Procedure
3.2. Problem Formulation
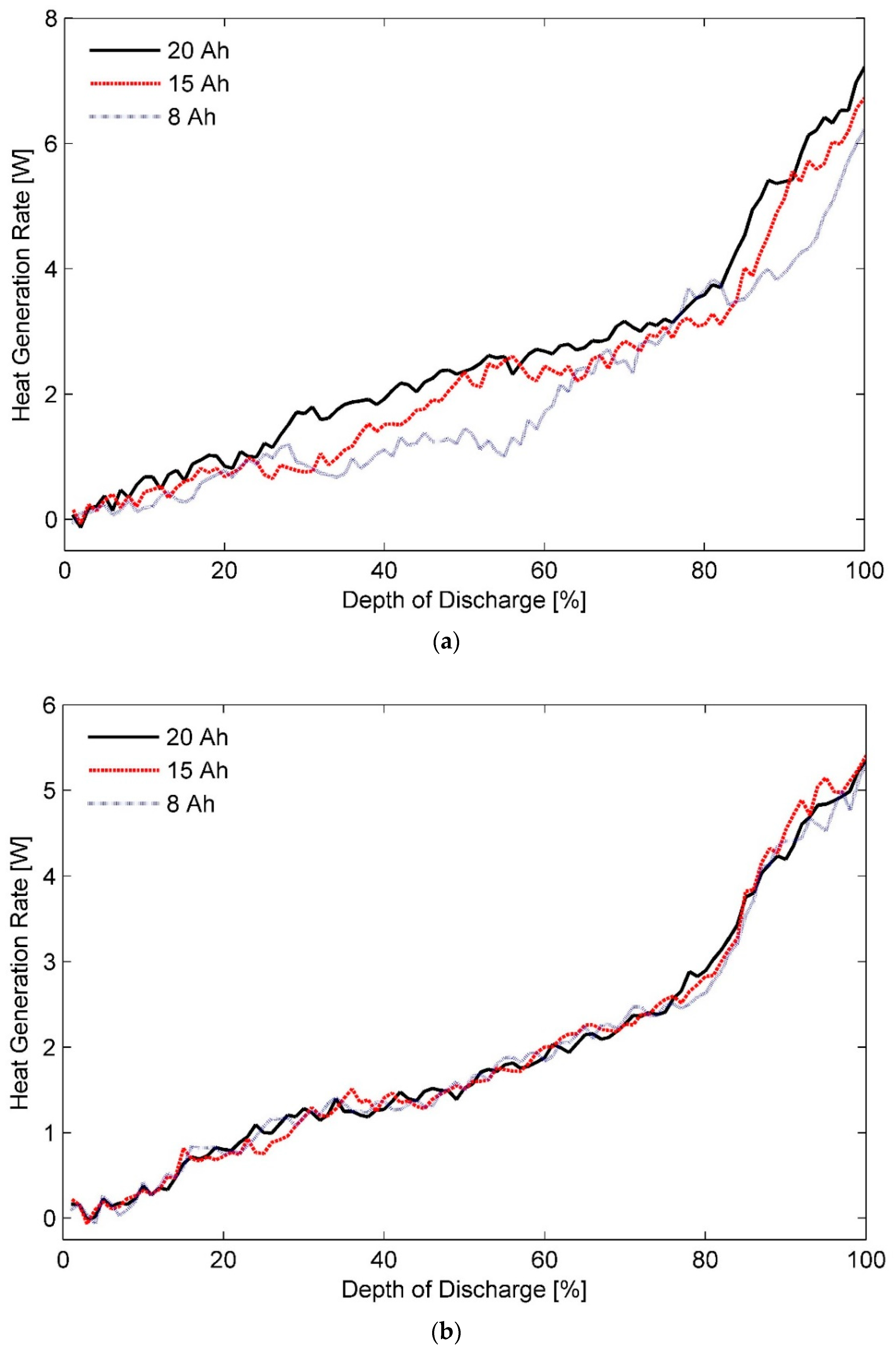
4. Results and Discussion
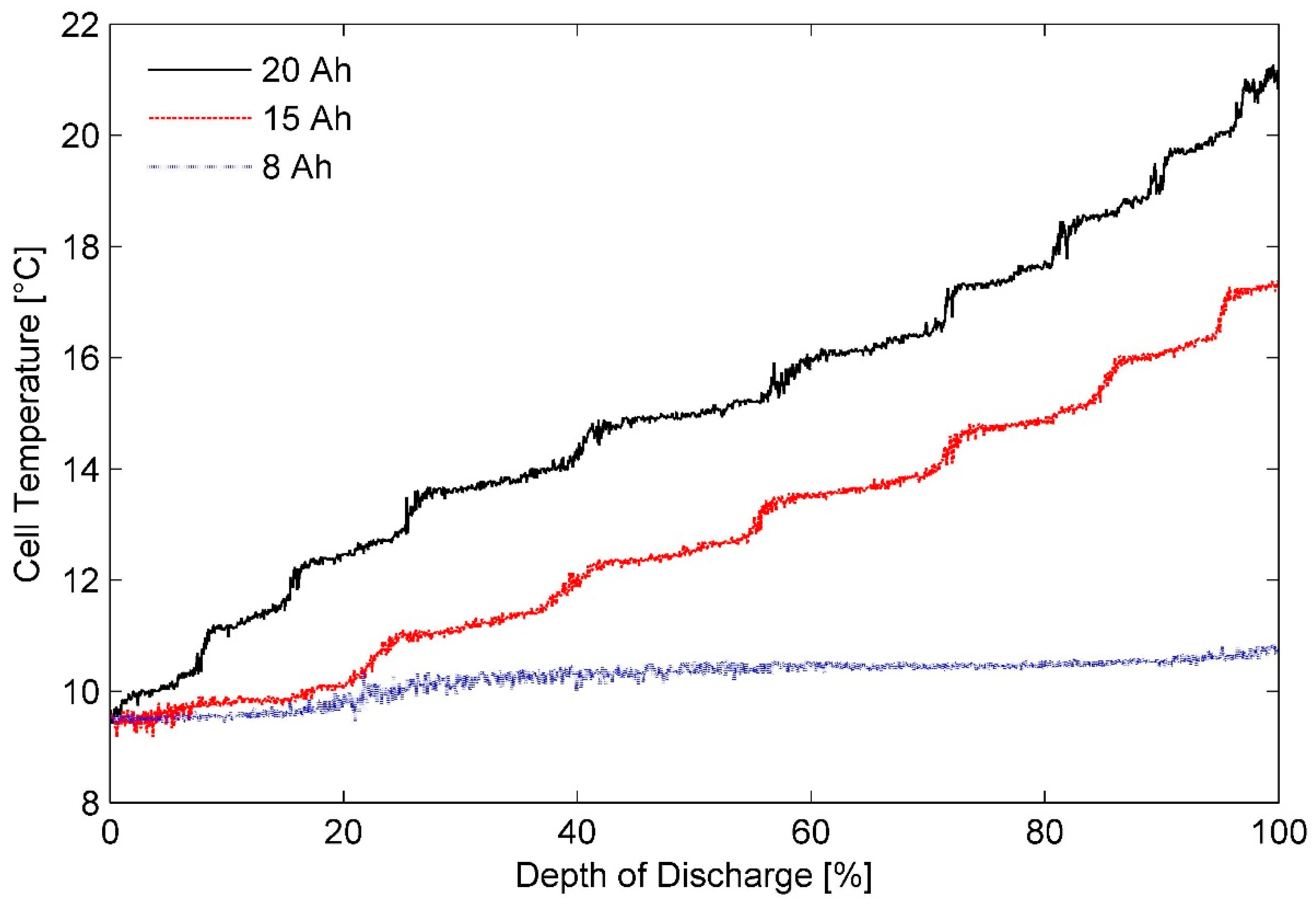
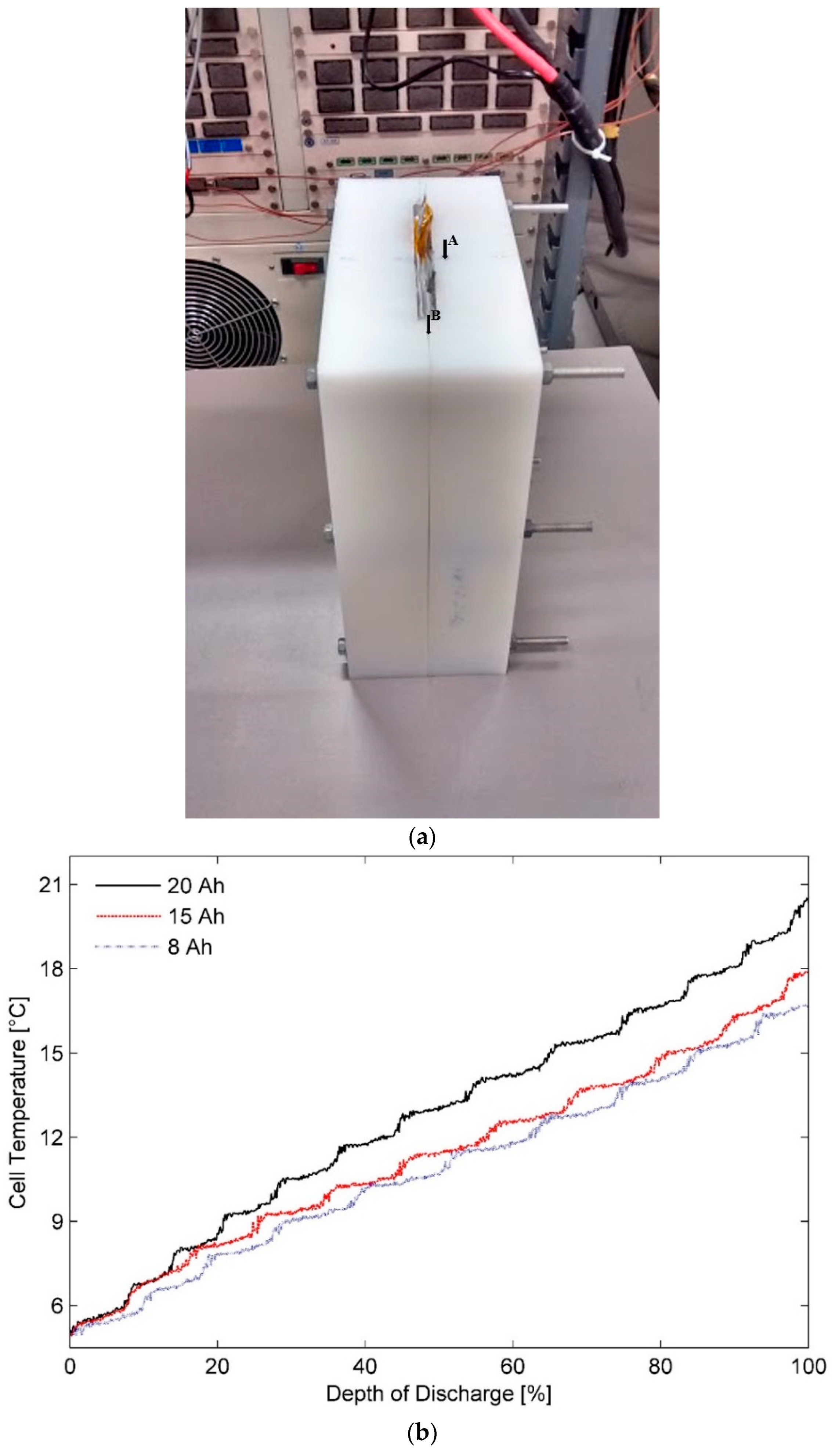
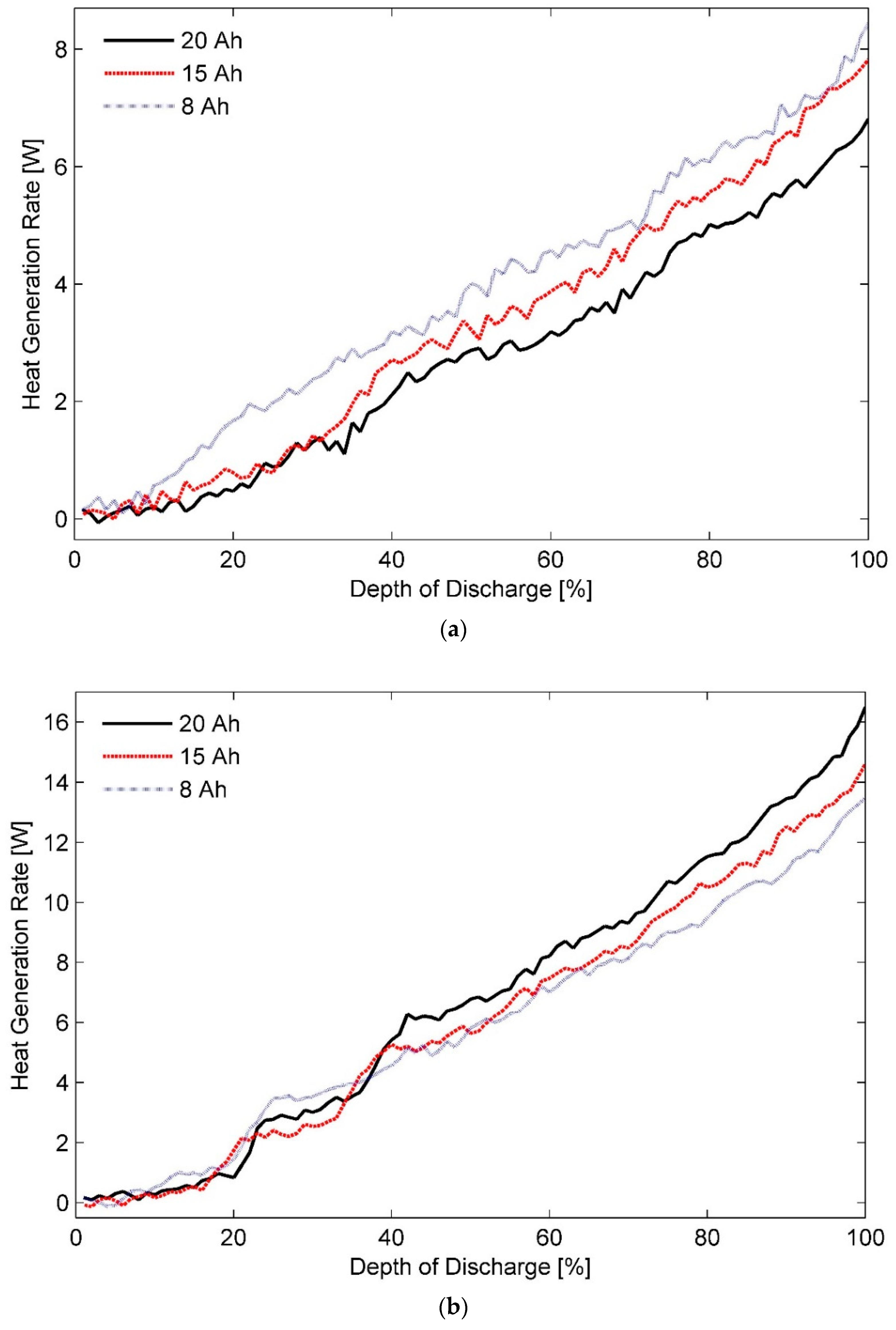
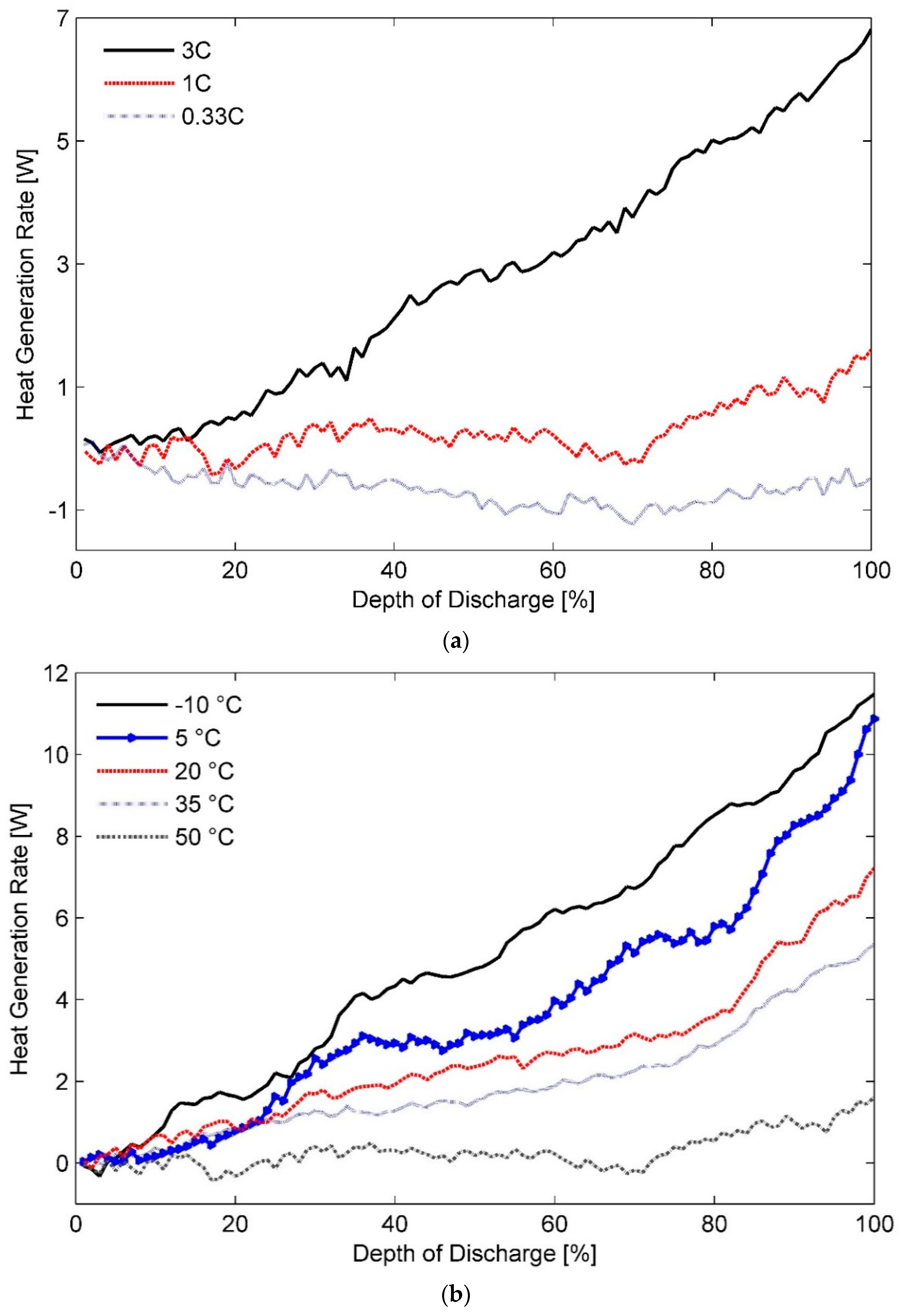
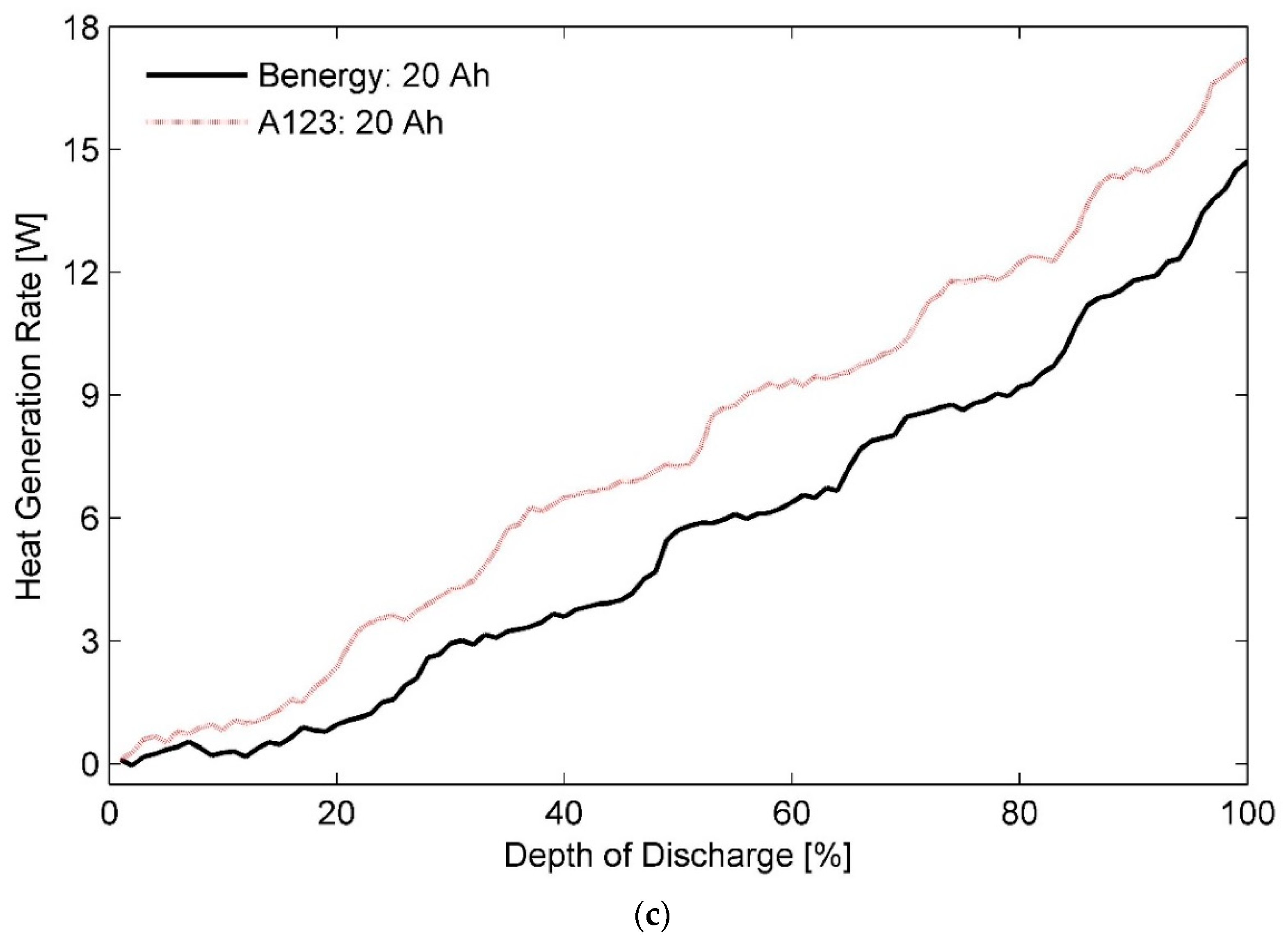
4.1. Effect of Temperature
- Resistance offered to diffusion of Li-ions by the solution phase of the porous electrode;
- Resistance presented by the cell separator to Li-ion diffusion;
- Resistance provided by the solid electrolyte interphase to Li-ions;
- Resistance of the solid phase of porous electrode to transfer of electrons across it;
- Resistivity of the current collectors.
- Thick battery cells for EVs that are to be driven in low ambient temperatures, and
- Thin cells for EVs that could be used in ambient temperatures higher than 35 °C.
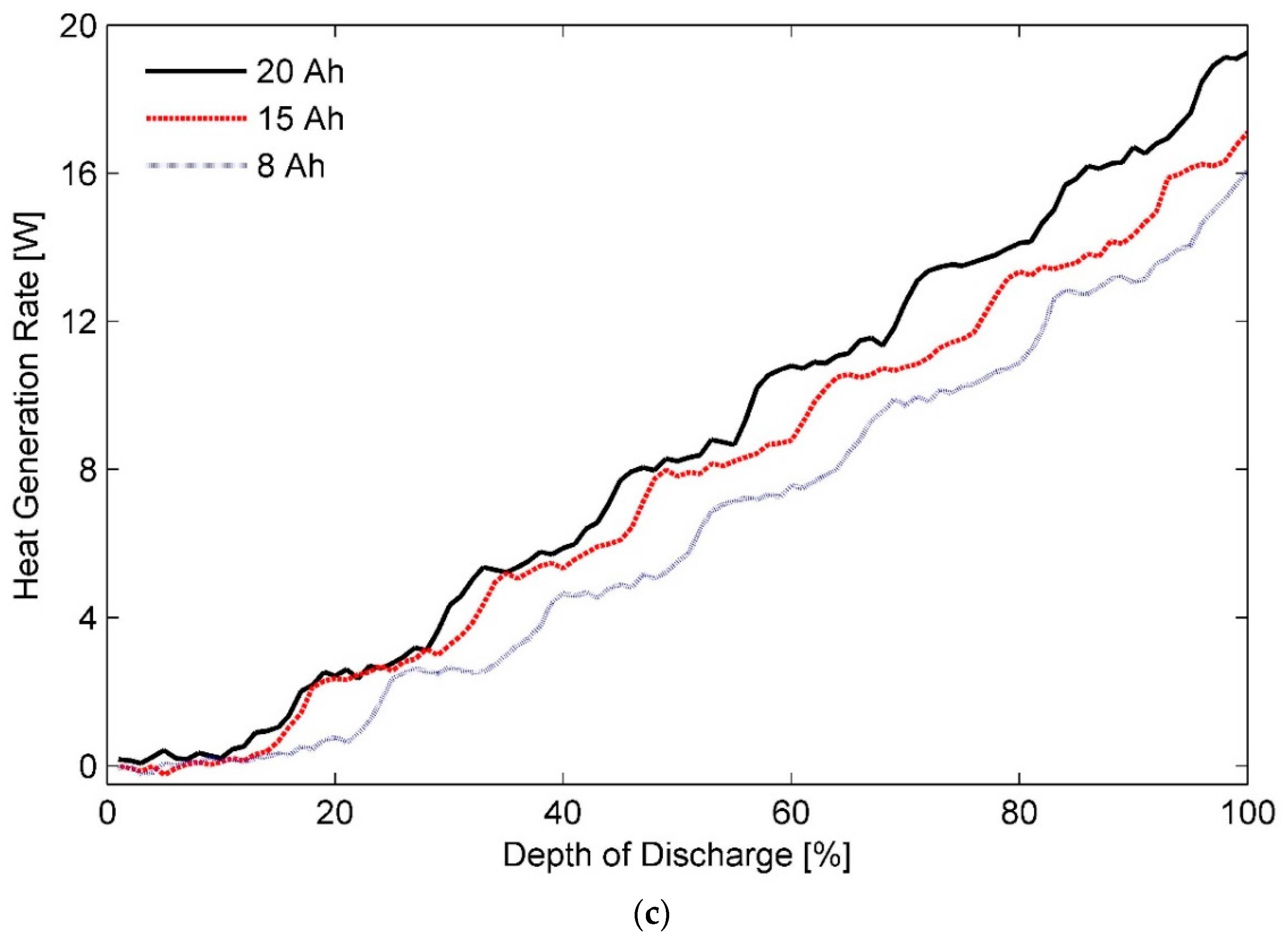
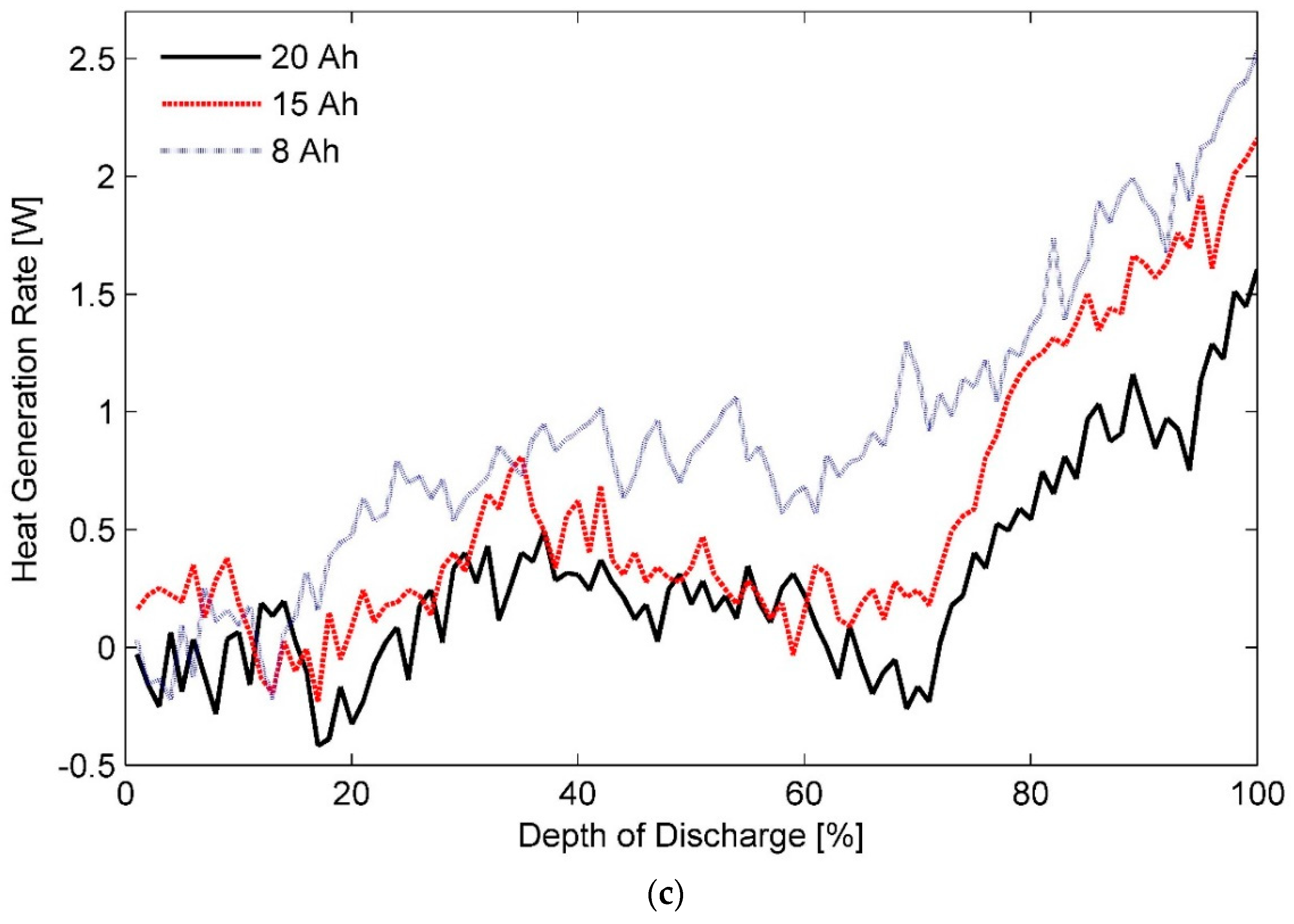
4.2. Comments on the Presence of a ‘Second Discharge Plateau’
4.3. Effect of Discharge Rate
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Tie, S.F.; Tan, C.W. A review of energy sources and energy management system in electric vehicles. Renew. Sustain. Energy Rev. 2013, 20, 82. [Google Scholar] [CrossRef]
- Rothgang, S.; Baumhöfer, T.; van Hoek, H.; Lange, T.; De Doncker, R.W.; Sauer, D.U. Modular battery design for reliable, flexible and multi-technology energy storage systems. Appl. Energy 2015, 137, 931–937. [Google Scholar] [CrossRef]
- Arora, S.; Shen, W.; Kapoor, A. Review of mechanical design and strategic placement technique of a robust battery pack for electric vehicles. Renew. Sustain. Energy Rev. 2016, 60, 1319–1331. [Google Scholar] [CrossRef]
- Arora, S.; Kapoor, A.; Shen, W. Application of Robust Design Methodology to Battery Packs for Electric Vehicles: Identification of Critical Technical Requirements for Modular Architecture. Batteries 2018, 4, 30. [Google Scholar] [CrossRef]
- Kulkarni, A.; Kapoor, A.; Arora, S. Battery Packaging and System Design for an Electric Vehicle. SAE Int. 2015. [Google Scholar] [CrossRef]
- Gauthier, M.; Carney, T.J.; Grimaud, A.; Giordano, L.; Pour, N.; Chang, H.H.; Fenning, D.P.; Lux, S.F.; Paschos, O.; Bauer, C.; et al. Electrode-Electrolyte Interface in Li-Ion Batteries: Current Understanding and New Insights. J. Phys. Chem. Lett. 2015, 6, 4653–4672. [Google Scholar] [CrossRef] [PubMed]
- Liu, P.; Wang, J.; Hicks-Garner, J.; Sherman, E.; Soukiazian, S.; Verbrugge, M.; Tataria, H.; Musser, J.; Finamore, P. Aging Mechanisms of LiFePO4 batteries deduced by electrochemical and structural analyses. J. Electrochem. Soc. 2010, 157, A499–A507. [Google Scholar] [CrossRef]
- Amine, K.; Liu, J.; Belharouak, I. High-temperature storage and cycling of C-LiFePO4/graphite Li-ion cells. Electrochem. Commun. 2005, 7, 669–673. [Google Scholar] [CrossRef]
- Biensan, P.; Simon, B.; Peres, J.P.; De Guibert, A.; Broussely, M.; Bodet, J.M.; Perton, F. On safety of lithium-ion cells. J. Power Sources 1999, 81–82, 906–912. [Google Scholar] [CrossRef]
- Ramadass, P.; Haran, B.; White, R.; Popov, B.N. Capacity fade of Sony 18650 cells cycled at elevated temperatures: Part I. Cycling performance. J. Power Sources 2002, 112, 606–613. [Google Scholar] [CrossRef]
- Maleki, H.; Deng, G.; Anani, A.; Howard, J. Thermal stability studies of Li-ion cells and components. J. Electrochem. Soc. 1999, 146, 3224–3229. [Google Scholar] [CrossRef]
- Arora, S.; Shen, W.; Kapoor, A. Designing a Robust Battery Pack for Electric Vehicles Using a Modified Parameter Diagram. SAE Tech. Paper 2015. [Google Scholar] [CrossRef]
- Arora, S.; Kapoor, A.; Shen, W. A novel thermal management system for improving discharge/charge performance of Li-ion battery packs under abuse. J. Power Sources 2018, 378, 759–775. [Google Scholar] [CrossRef]
- Arora, S.; Tammi, K. A Hybrid Thermal Management System with Negative Parasitic Losses for Electric Vehicle Battery Packs. In Proceedings of the ASME 2018 International Mechanical Engineering Congress and Exposition, Pittsburgh, PA, USA, 9–15 November 2018. [Google Scholar]
- Santhanagopalan, S.; Zhang, Q.; Kumaresan, K.; White, R.E. Parameter estimation and life modeling of lithium-ion cells. J. Electrochem. Soc. 2008, 155, A345–A353. [Google Scholar] [CrossRef]
- Yang, H.; Amiruddin, S.; Bang, H.; Sun, Y.; Prakash, J. A review of Li-Ion cell chemistries and their potential use in hybrid electric vehicles. J. Ind. Eng. Chem. 2006, 12, 12–38. [Google Scholar]
- Arora, S.; Kapoor, A. Mechanical Design and Packaging of Battery Packs for Electric Vehicles. In Behaviour of Lithium-Ion Batteries in Electric Vehicles: Battery Health, Performance, Safety, and Cost; Pistoia, G., Liaw, B., Eds.; Springer International Publishing: Cham, Switzerland, 2018; pp. 175–200. [Google Scholar]
- Ji, Y.; Wang, C.Y. Heating strategies for Li-ion batteries operated from subzero temperatures. Electrochim. Acta 2013, 107, 664–674. [Google Scholar] [CrossRef]
- Arora, S. Selection of thermal management system for modular battery packs of electric vehicles: A review of existing and emerging technologies. J. Power Sources 2018, 400, 621–640. [Google Scholar] [CrossRef]
- Chen, K.; Unsworth, G.; Li, X. Measurements of heat generation in prismatic Li-ion batteries. J. Power Sources 2014, 261, 28–37. [Google Scholar] [CrossRef]
- Bandhauer, T.M.; Garimella, S.; Fuller, T.F. Temperature-dependent electrochemical heat generation in a commercial lithium-ion battery. J. Power Sources 2014, 247, 618–628. [Google Scholar] [CrossRef]
- Lu, W.; Prakash, J. In situ measurements of heat generation in a Li/mesocarbon microbead half-cell. J. Electrochem. Soc. 2003, 150, A262–A266. [Google Scholar] [CrossRef]
- Bandhauer, T.M.; Garimella, S.; Fuller, T. A Critical Review of Thermal Issues in Lithium-Ion Batteries. J. Electrochem. Soc. 2011, 158, R1–R25. [Google Scholar] [CrossRef]
- Bang, H.; Yang, H.; Sun, Y.K.; Prakash, J. In situ studies of LixMn2O4 and Li xAl0.17Mn1.83O3.97S0.03 cathode by IMC. J. Electrochem. Soc. 2005, 152, A421–A428. [Google Scholar] [CrossRef]
- Kim, J.S.; Prakash, J.; Selman, J.R. Thermal characteristics of LixMn2O4 spinel. Electrochem. Sol. State Lett. 2001, 4, A141–A144. [Google Scholar] [CrossRef]
- Kobayashi, Y.; Kihira, N.; Takei, K.; Miyashiro, H.; Kumai, K.; Terada, N.; Ishikawa, R. Electrochemical and calorimetric approach to spinel lithium manganese oxide. J. Power Sources 1999, 81–82, 463–466. [Google Scholar] [CrossRef]
- Kobayashi, Y.; Miyashiro, H.; Kumai, K.; Takei, K.; Iwahori, T.; Uchida, I. Precise electrochemical calorimetry of LiCoO2/graphite lithium-ion cell understanding thermal behavior and estimation of degradation mechanism. J. Electrochem. Soc. 2002, 149, A978–A982. [Google Scholar] [CrossRef]
- Lu, W.; Belharouak, I.; Park, S.H.; Sun, Y.K.; Amine, K. Isothermal calorimetry investigation of Li1+xMn2-yAlzO4 spinel. Electrochim. Acta 2007, 52, 5837–5842. [Google Scholar] [CrossRef]
- Onda, K.; Kameyama, H.; Hanamoto, T.; Ito, K. Experimental study on heat generation behavior of small lithium-ion secondary batteries. J. Electrochem. Soc. 2003, 150, A285–A291. [Google Scholar] [CrossRef]
- Saito, Y.; Kanari, K.; Takano, K. Thermal studies of a lithium-ion battery. J. Power Sources 1997, 68, 451–454. [Google Scholar] [CrossRef]
- Song, L.; Evans, J.W. Electrochemical-thermal model of lithium polymer batteries. J. Electrochem. Soc. 2000, 147, 2086–2095. [Google Scholar] [CrossRef]
- Yang, H.; Prakash, J. Determination of the reversible and irreversible heats of a LiNi 0.8Co0.15Al0.05O2/natural graphite cell using electrochemical-calorimetric technique. J. Electrochem. Soc. 2004, 151, A1222–A1229. [Google Scholar] [CrossRef]
- Thomas, K.E.; Newman, J. Heats of mixing and of entropy in porous insertion electrodes. J. Power Sources 2003, 119–121, 844–849. [Google Scholar] [CrossRef]
- Xiao, M.; Choe, S.-Y. Theoretical and experimental analysis of heat generations of a pouch type LiMn2O4/carbon high power Li-polymer battery. J. Power Sources 2013, 241, 46–55. [Google Scholar] [CrossRef]
- Schuster, E.; Ziebert, C.; Melcher, A.; Rohde, M.; Seifert, H.J. Thermal behavior and electrochemical heat generation in a commercial 40 Ah lithium ion pouch cell. J. Power Sources 2015, 286, 580–589. [Google Scholar] [CrossRef]
- Lin, C.; Xu, S.; Li, Z.; Li, B.; Chang, G.; Liu, J. Thermal analysis of large-capacity LiFePO4 power batteries for electric vehicles. J. Power Sources 2015, 294, 633–642. [Google Scholar] [CrossRef]
- Drake, S.J.; Martin, M.; Wetz, D.A.; Ostanek, J.K.; Miller, S.P.; Heinzel, J.M.; Jain, A. Heat generation rate measurement in a Li-ion cell at large C-rates through temperature and heat flux measurements. J. Power Sources 2015, 285, 266–273. [Google Scholar] [CrossRef]
- Arora, S.; Shen, W.; Kapoor, A. Critical analysis of open circuit voltage and its effect on estimation of irreversible heat for Li-ion pouch cells. J. Power Sources 2017, 350, 117–126. [Google Scholar] [CrossRef]
- Arora, S.; Shen, W.; Kapoor, A. Neural network based computational model for estimation of heat generation in LiFePO4 pouch cells of different nominal capacities. Comput. Chem. Eng. 2017, 101, 81–94. [Google Scholar] [CrossRef]
- Worldwide C. 2016 Chevrolet Spark Specifications. 2016. Available online: https://media.chevrolet.com/media/us/en/chevrolet/vehicles/spark-ev/2016 (accessed on 10 November 2019).
- Worldwide C. 2015 Chevrolet Spark Specifications. 2015. Available online: https://media.gm.com/media/us/en/chevrolet/vehicles/spark-ev/2015.html (accessed on 10 November 2019).
- Authority, G. 2017 Chevrolet Bolt EV. 2016. Available online: https://media.chevrolet.com/media/us/en/chevrolet/vehicles/bolt-ev/2017 (accessed on 10 November 2019).
- Cole, J. New Specs for 2015 e-Golf Released By VW, Launches Later This Year. 2014. Available online: http://insideevs.com/volkswagen-releases-details-2015-e-golf/ (accessed on 10 November 2019).
- Rovito, M. 2015 VW e-Golf Ushers in an Era of Interchangeable Drivetrains for Every Volkswagen Model; CHARGED—Electric Vehicles Magazine, Isentropic Media, 2015. Available online: https://chargedevs.com/features/the-2015-vw-e-golf-ushers-in-an-era-of-interchangeable-drivetrains-for-every-volkswagen-model/ (accessed on 10 November 2019).
- Cole, J. 2017 Volkswagen e-Golf: 35.8 kWh Battery, 124 Mile/200km Range (Update). Inside EVs. 2016. Available online: http://insideevs.com/2017-volkswagen-e-golf-35-8-kwh-battery-124-miles200km-real-world-range/ (accessed on 10 November 2019).
- Buchmann, I. BU-1003: Electric Vehicle (EV); Cadex Electronics Inc., 2016. Available online: http://batteryuniversity.com/learn/article/electric_vehicle_ev (accessed on 10 November 2019).
- Idaho National Laboratory. 2013 Ford Focus Electric Advanced Vehicle Testing—Baseline Testing Results. 2015. Available online: https://www.energy.gov/sites/prod/files/2015/02/f19/fact2013fordfocus.pdf (accessed on 10 November 2019).
- Ford Motor Company. FOCUS 2016—Electric Engine Specifications. 2016. Available online: http://www.ford.com/cars/focus/specifications/engine/ (accessed on 10 November 2019).
- Roper, L.D. Mercedes B-Class ED 2015. 2016. Available online: http://www.roperld.com/Science/MercedesBClassED.htm (accessed on 10 November 2019).
- Huffman, J.P. 2014 BMW i3 vs. 2014 Mercedes-Benz B-Class Electric Drive. Car and Driver. 8 September 2014. Available online: https://www.caranddriver.com/reviews/comparison-test/a15110584 (accessed on 10 November 2019).
- Lofton, B. Kia Soul EV Battery System. EV Hangar. 2016. Available online: https://www.evhangar.com/system/battery-systems/sys-batt-kia-soul-ev/ (accessed on 10 November 2019).
- Hope, J. 2019 Kia Soul EV Specifications. 2019. Available online: https://www.kiamedia.com/us/en/models/soul-ev/2019/specifications (accessed on 10 November 2019).
- Moloughney, T. 2017 BMW i3 Specs Revealed with Some Surprises. The Electric BMW i3. 2016. Available online: https://insideevs.com/news/331741/2017-bmw-i3-specs-revealed-with-some-surprises/ (accessed on 10 November 2019).
- Blanco, S. Updated 2017 BMW i3 Goes 114 Miles with New 33-kWh Battery; AOL Inc., 2016. Available online: https://www.autoblog.com/2016/05/01/updated-bmw-i3-114-miles-new-33-kwh-battery (accessed on 10 November 2019).
- Mitsubishi Motors North America Inc. Main Drive Battery; Mitsubishi Motors, 2016. Available online: http://www.mitsubishicars.com/imiev/specifications (accessed on 10 November 2019).
- Idaho National Laboratory. BEV Battery Testing Results 2012 Mitsubishi iMiev—VIN 4550; Advanced Vehicle Testing Activity, 2014. Available online: https://www.energy.gov/sites/prod/files/2015/02/f19/batteryiMiev4550.pdf (accessed on 10 November 2019).
- EVSpecifications. 2019 Nissan Leaf S Plus - Specification and Price. 2019. Available online: https://www.evspecifications.com/en/model/68ad67 (accessed on 10 November 2019).
- Kane, M. Here is the Nissan LEAF e+62 kWh battery: Video. InsideEVs. 2019. Available online: https://insideevs.com/news/342009/here-is-the-nissan-leaf-e-62-kwh-battery-video/ (accessed on 10 November 2019).
- McCullough, J.P. Calorimetry of Non-Reacting Systems Prepared Under the Sponsorship of the International Union of Pure and Applied Chemistry Commission on Thermodynamics and the Thermochemistry; Scott, D.W., Ed.; Elsevier Science: Burlington, NJ, USA, 2013. [Google Scholar]
- Reeve, T.H. The Method of Fundamental Solutions for Some Direct and Inverse Problems; University of Birmingham: Birmingham, UK, 2013. [Google Scholar]
- Alifanov, O.M. Inverse Heat Transfer Problems; Springer Science & Business Media: Berlin, Germany, 2012. [Google Scholar]
- Alifanov, O.M.; Artyukhin, E.A.; Rumyantsev, S.V. Extreme Methods for Solving Ill-Posed Problems with Applications to Inverse Heat Transfer Problems; Begell House: New York, NY, USA, 1995. [Google Scholar]
- Jarny, Y.; Ozisik, M.; Bardon, J. A general optimization method using adjoint equation for solving multidimensional inverse heat conduction. Int. J. Heat Mass Transf. 1991, 34, 2911–2919. [Google Scholar] [CrossRef]
- Beck, J.; Blackwell, B.; Haji-Sheikh, A. Comparison of some inverse heat conduction methods using experimental data. Int. J. Heat Mass Transf. 1996, 39, 3649–3657. [Google Scholar] [CrossRef]
- Lesnic, D.; Elliott, L.; Ingham, D. Application of the boundary element method to inverse heat conduction problems. Int. J. Heat Mass Transf. 1996, 39, 1503–1517. [Google Scholar] [CrossRef]
- Huang, C.-H.; Wang, S.-P. A three-dimensional inverse heat conduction problem in estimating surface heat flux by conjugate gradient method. Int. J. Heat Mass Transf. 1999, 42, 3387–3403. [Google Scholar] [CrossRef]
- Beck, J.V.; Blackwell, B.; Clair, C.R.S., Jr. Inverse Heat Conduction: Ill-Posed Problems: James Beck. 1985. Available online: https://www.thermalfluidscentral.org/e-books/book-intro.php?b=44 (accessed on 10 November 2019).
- GatesEnergyProducts. Section 3—Sealed Nickel-Cadmium Cells and Batteries. Rechargeable Batteries Applications Handbook; Newton: Newnes, NSW, Australia, 1998; pp. 35–151. [Google Scholar]
- Gu, H. Mathematical analysis of a Zn/NiOOH cell. J. Electrochem. Soc. 1983, 130, 1459–1464. [Google Scholar] [CrossRef]
- Arora, S. Design of a Modular Battery Pack for Electric Vehicles. Ph.D. Thesis, Swinburne University of Technology, Melbourne, Australia, 2017. [Google Scholar]
- Ahlberg, E.; Palmqvist, U.; Simic, N.; Sjövall, R. Capacity loss in Ni–Cd pocket plate batteries. The origin of the second voltage plateau. J. Power Sources 2000, 85, 245–253. [Google Scholar] [CrossRef]
- Barnard, R.; Crickmore, G.T.; Lee, J.A.; Tye, F.L. The cause of residual capacity in nickel oxyhydroxide electrodes. J. Appl. Electrochem. 1980, 10, 61–70. [Google Scholar] [CrossRef]
- Leger, C.; Tessier, C.; Ménétrier, M.; Denage, C.; Delmas, C. Investigation of the second discharge plateau of the β (III)-NiOOH/β (II)-Ni (OH) 2 system. J. Electrochem. Soc. 1999, 146, 924–932. [Google Scholar] [CrossRef]
- Motupally, S.; Jain, M.; Srinivasan, V.; Weidner, J.W. The role of oxygen at the second discharge plateau of nickel hydroxide. J. Electrochem. Soc. 1998, 145, 34–39. [Google Scholar] [CrossRef]
- Mao, Z.; De Vidts, P.; White, R.E.; Newman, J. Theoretical analysis of the discharge performance of a NiOOH/H 2 cell. J. Electrochem. Soc. 1994, 141, 54–64. [Google Scholar] [CrossRef]
- Finley, T.D. Battery Degradation Modeling For Vehicle Applications. 2014. Available online: https://uwspace.uwaterloo.ca/handle/10012/8830 (accessed on 10 November 2019).
- Zaghib, K.; Mauger, A.; Groult, H.; Goodenough, J.B.; Julien, C.M. Advanced electrodes for high power Li-ion batteries. Materials 2013, 6, 1028–1049. [Google Scholar] [CrossRef] [PubMed]
- Wang, W.L.; Jin, E.M.; Gu, H.-B. Electrochemical Performance of Lithium Iron Phosphate by Adding Graphite Nanofiber for Lithium Ion Batteries. Trans. Electr. Electron. Mater. 2012, 13, 121–124. [Google Scholar] [CrossRef]
- Wu, W.; Xiao, X.; Huang, X. The effect of battery design parameters on heat generation and utilization in a Li-ion cell. Electrochim. Acta 2012, 83, 227–240. [Google Scholar] [CrossRef]
- Churikov, A.V.; Ivanishchev, A.V.; Ivanishcheva, I.A.; Sycheva, V.O.; Khasanova, N.R.; Antipov, E.V. Determination of lithium diffusion coefficient in LiFePO4 electrode by galvanostatic and potentiostatic intermittent titration techniques. Electrochim. Acta 2010, 55, 2939–2950. [Google Scholar] [CrossRef]
- Arora, S. A Novel Technique for Estimation of the Solid Electrolyte Interphase Film Resistance for Li-Ion Batteries. In Proceedings of the ASME 2018 International Mechanical Engineering Congress and Exposition, Pittsburgh, PA, USA, 9–15 November 2019. [Google Scholar]
- Laffont, L.; Delacourt, C.; Gibot, P.; Wu, M.Y.; Kooyman, P.; Masquelier, C.; Tarascon, J.M. Study of the LiFePO4/FePO4 Two-Phase System by High-Resolution Electron Energy Loss Spectroscopy. Chem. Mater. 2006, 18, 5520–5529. [Google Scholar] [CrossRef]



| Vehicle | Pack Capacity (kWh) | Cell Capacity (Ah) | Reference |
|---|---|---|---|
| Chevrolet Spark EV 2016 | 19 | 23.75 | [40,41] |
| Chevrolet Bolt | 60 | 50 | [42] |
| VW e-Golf 2015 | 24.2 | 25 | [43,44] |
| VW e-Golf 2017 | 35.8 | 37 | [45] |
| Tesla Model S | 60 and 90 | 3.4 | [46] |
| Ford Focus Electric | 23 | 15 | [47,48] |
| Mercedes-Benz B-class ED | 36 | 3.4 | [49,50] |
| Kia Soul EV | 30 | 75 | [51,52] |
| BMW i3 2017 | 33 | 94 | [53,54] |
| Mitsubishi iMiEV | 16 | 50 | [55,56] |
| Nissan LEAF S Plus | 62 | 56.3 | [57,58] |
| Battery Cell | Model Number | Height (mm) | Width (mm) | Thickness (mm) |
|---|---|---|---|---|
| 8 Ah | BHK-1280A5 | 105 | 80 | 12 |
| 15 Ah | BHK-11A8F5 | 155 | 108 | 11 |
| 20 Ah | BHK-85C0M7 | 227 | 120 | 9 |
| A123 20 Ah | AMP20M1HD-A | 227 | 160 | 7.25 |
| Discharge Rate | Nominal Capacity (Ah) | Ambient Temperature (°C) | ||||
|---|---|---|---|---|---|---|
| −10 | 5 | 20 | 35 | 50 | ||
| 0.33C | 8 | −0.16 to 4.42 | −0.98 to 2.20 | −0.75 to 1.46 | −0.81 to 0.04 | −0.75 to 0.13 |
| 15 | −0.11 to 4.84 | −0.63 to 2.52 | −0.65 to 1.97 | −0.85 to 0.14 | −0.89 to 0.16 | |
| 20 | −0.01 to 5.19 | −1.36 to 2.82 | −0.37 o 2.23 | −0.84 to 0.13 | −1.24 to 0.12 | |
| 1C | 8 | −0.07 to 10.46 | 0.10 to 8.73 | −0.07 to 6.23 | −0.06 to 5.32 | −0.22 to 2.53 |
| 15 | −0.25 to 11.05 | −0.18 to 10.02 | −0.06 to 6.72 | −0.07 to 5.41 | −0.23 to 2.16 | |
| 20 | −0.32 to 11.85 | 0.03 to 10.87 | −0.13 to 7.22 | −0.01 to 5.35 | −0.42 to 1.60 | |
| 3C | 8 | _ | −0.24 to 16.08 | −0.12 to 13.46 | −0.07 to 10.13 | 0.09 to 8.43 |
| 15 | _ | −0.24 to 17.11 | −0.14 to 14.58 | 0.06 to 10.22 | 0 to 7.82 | |
| 20 | _ | 0.07 to 19.27 | 0.10 to 16.49 | 0.06 to 10.32 | −0.06 to 6.81 | |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Arora, S.; Kapoor, A. Experimental Study of Heat Generation Rate during Discharge of LiFePO4 Pouch Cells of Different Nominal Capacities and Thickness. Batteries 2019, 5, 70. https://doi.org/10.3390/batteries5040070
Arora S, Kapoor A. Experimental Study of Heat Generation Rate during Discharge of LiFePO4 Pouch Cells of Different Nominal Capacities and Thickness. Batteries. 2019; 5(4):70. https://doi.org/10.3390/batteries5040070
Chicago/Turabian StyleArora, Shashank, and Ajay Kapoor. 2019. "Experimental Study of Heat Generation Rate during Discharge of LiFePO4 Pouch Cells of Different Nominal Capacities and Thickness" Batteries 5, no. 4: 70. https://doi.org/10.3390/batteries5040070
APA StyleArora, S., & Kapoor, A. (2019). Experimental Study of Heat Generation Rate during Discharge of LiFePO4 Pouch Cells of Different Nominal Capacities and Thickness. Batteries, 5(4), 70. https://doi.org/10.3390/batteries5040070






