Study on the Effect of Air Velocity and Duct Area on the Heat Dissipation of Lithium-Ion Batteries
Abstract
:1. Introduction
2. Modeling and Simulation Methods
2.1. Establishment of Battery Air Cooling Model
2.2. Battery Heat Generation Model
- The materials inside the battery are isotropic, and they have uniform physical properties, the same density, and the same physicochemical properties everywhere.
- The chemical reactions inside the battery are idealized as simple electrochemical reactions, ignoring the complex multiphase reactions and electrochemical catalysis inside the battery.
- The current density and internal heat generation in the battery are uniformly distributed during charging and discharging.
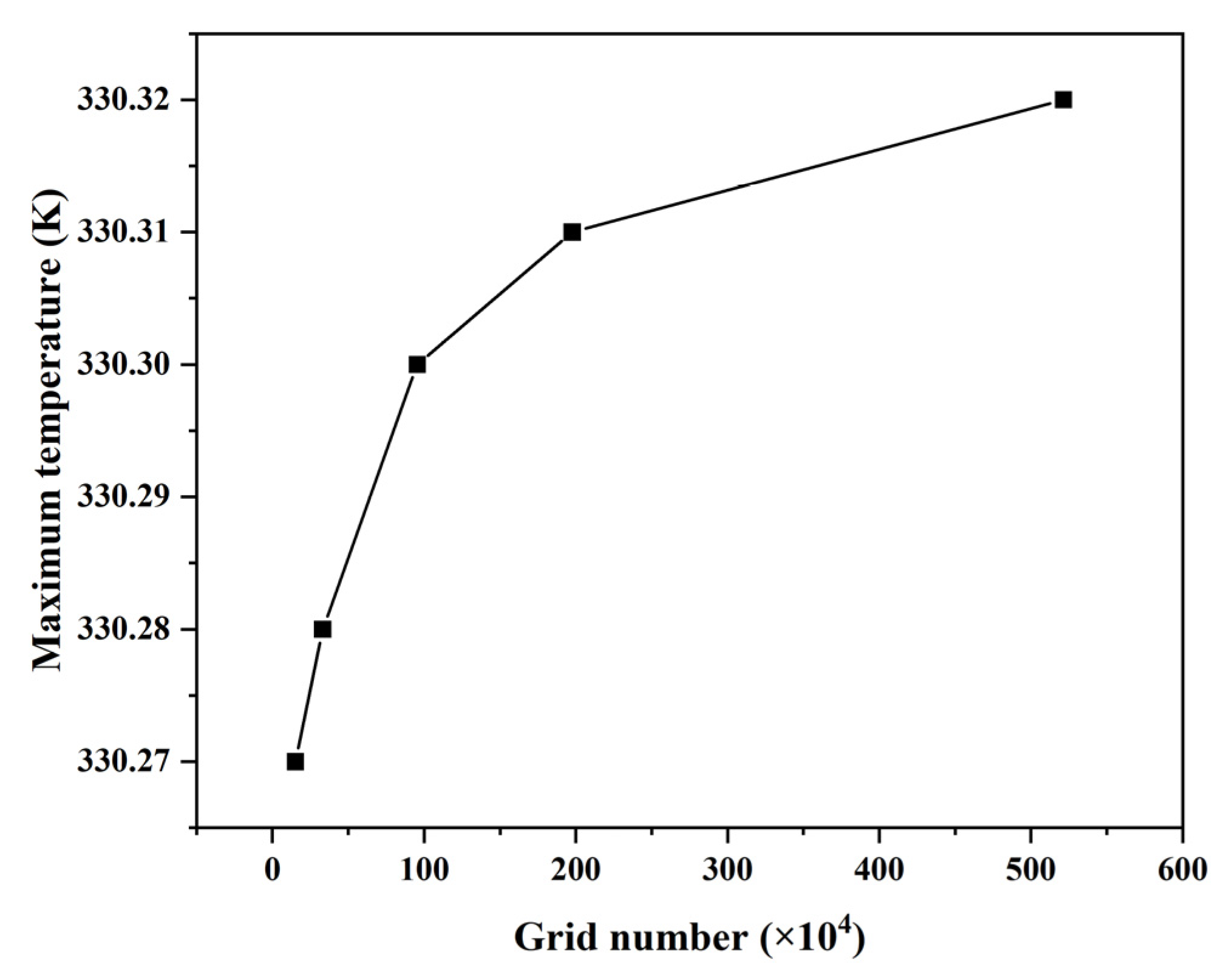
2.3. Simulation Method
3. Results and Discussion
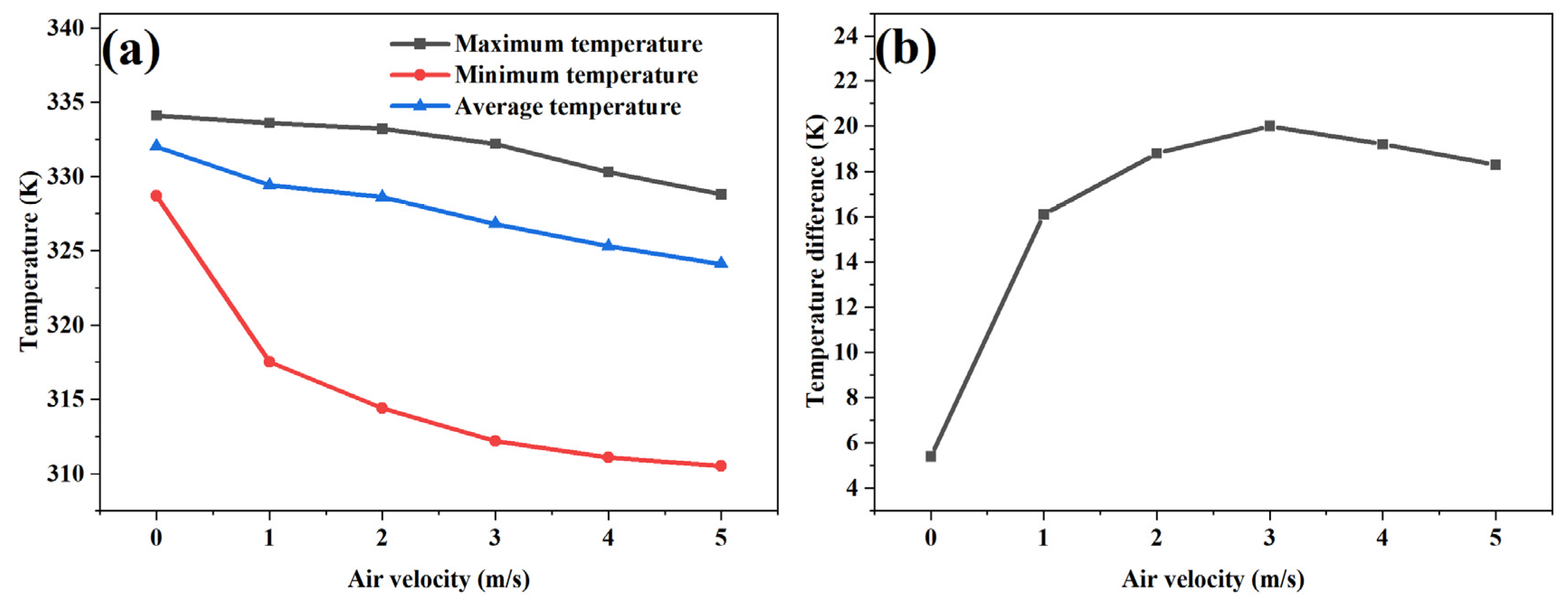
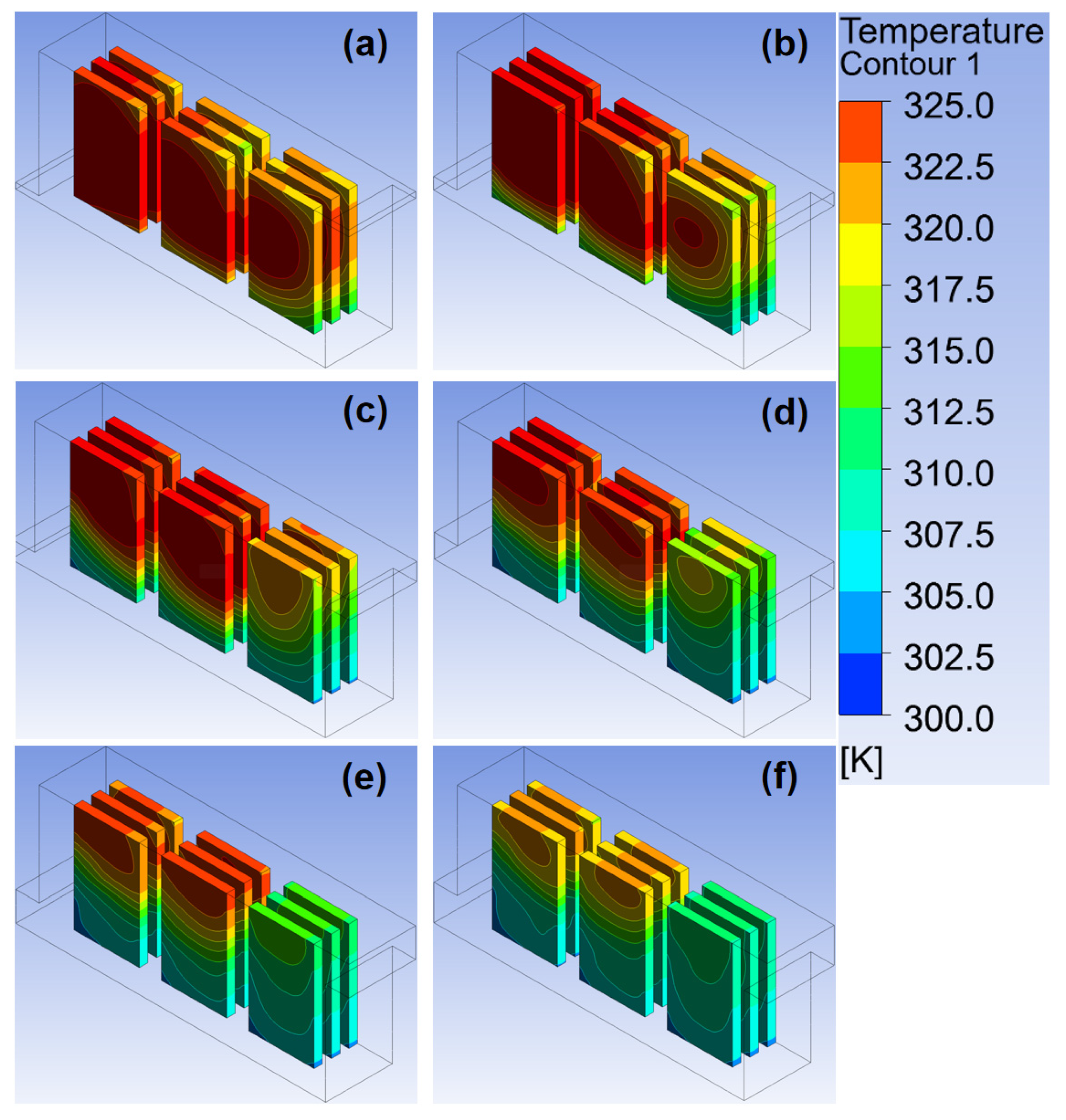
3.1. Effect of Inlet Air Velocity on Battery Temperature
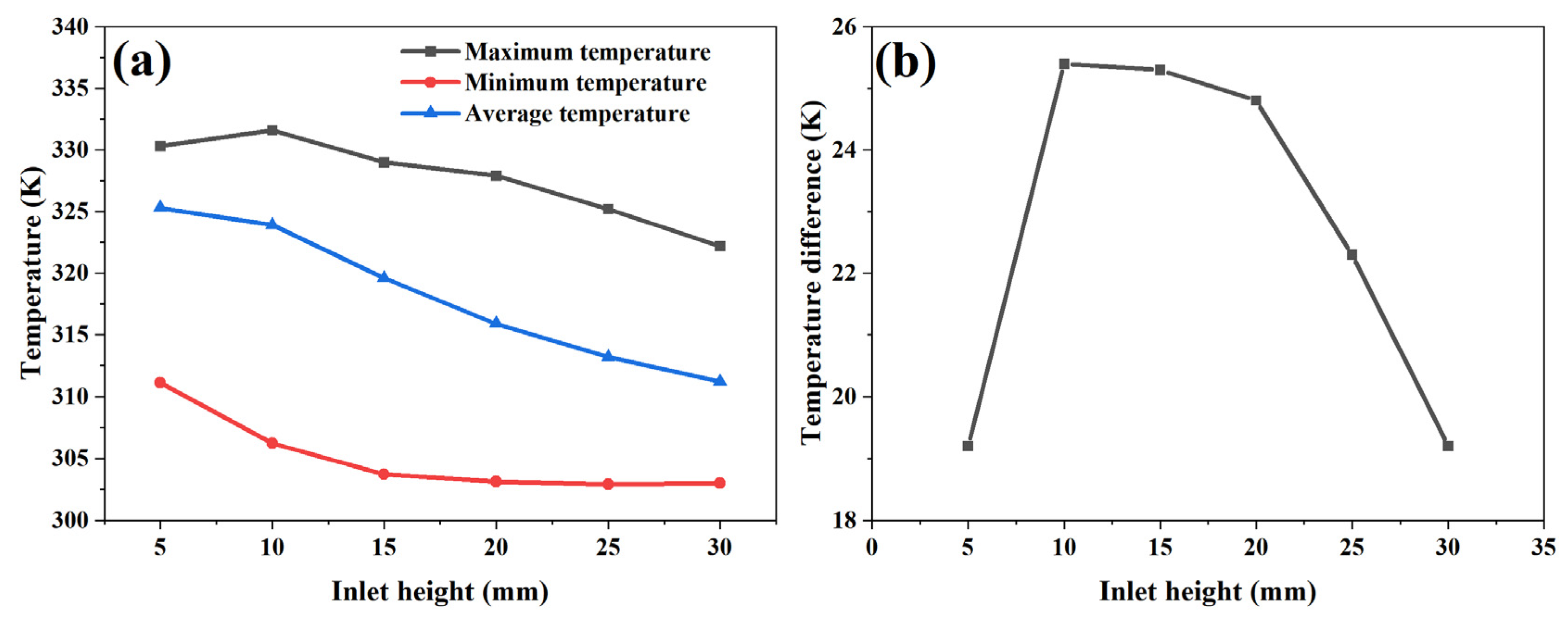
3.2. Effect of Inlet Size on the Temperature of Battery Pack
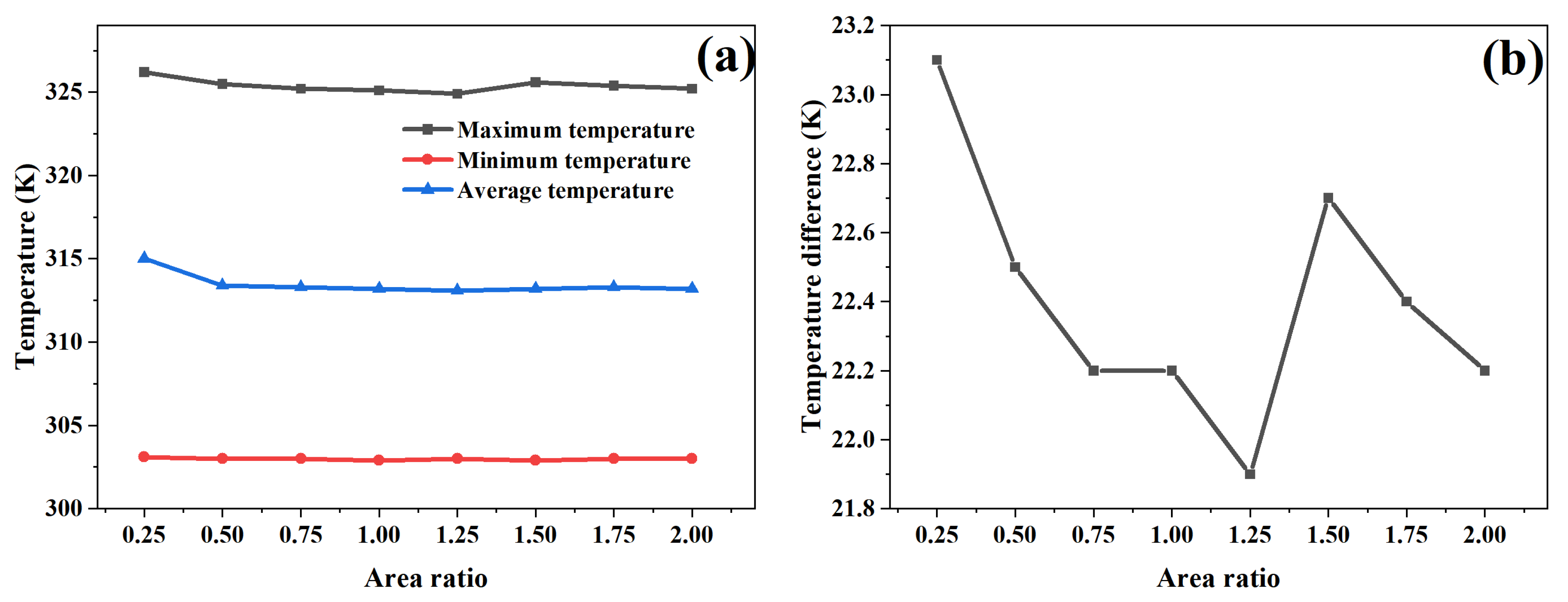
3.3. Effect of Inlet and Outlet Area Ratio on Heat Dissipation of Battery
4. Conclusions
Future Work and Design Adaptability
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Wang, Y.; Feng, X.; Huang, W.; He, X.; Wang, L.; Ouyang, M. Challenges and opportunities to mitigate the catastrophic thermal runaway of high-energy batteries. Adv. Energy Mater. 2023, 13, 2203841. [Google Scholar] [CrossRef]
- Chen, X.; Zhang, X.; Wang, H.; Jia, J.; Xie, S.; Zhi, M.; Fu, J.; Sun, Q. Influence of ambient pressure and heating power on the thermal runaway features of the lithium-ion battery. J. Electrochem. Energy Convers. Storage 2021, 18, 021014. [Google Scholar] [CrossRef]
- Thakur, A.K.; Sathyamurthy, R.; Velraj, R.; Saidur, R.; Pandey, A.; Ma, Z.; Singh, P.; Hazra, S.K.; Sharshir, S.W.; Prabakaran, R.; et al. A state-of-the art review on advancing battery thermal management systems for fast-charging. Appl. Therm. Eng. 2023, 226, 120303. [Google Scholar] [CrossRef]
- Huang, Y.; Wei, C.; Fang, Y. Numerical investigation on optimal design of battery cooling plate for uneven heat generation conditions in electric vehicles. Appl. Therm. Eng. 2022, 211, 118476. [Google Scholar] [CrossRef]
- Yang, C.; Xi, H.; Wang, M. Structure optimization of air cooling battery thermal management system based on lithium-ion battery. J. Energy Storage 2023, 59, 106538. [Google Scholar] [CrossRef]
- Mo, C.; Xie, J.; Zhang, G.; Zou, Z.; Yang, X. All-climate battery thermal management system integrating units-assembled phase change material module with forced air convection. Energy 2024, 294, 130642. [Google Scholar] [CrossRef]
- Weragoda, D.M.; Tian, G.; Burkitbayev, A.; Lo, K.-H.; Zhang, T. A comprehensive review on heat pipe based battery thermal management systems. Appl. Therm. Eng. 2023, 224, 120070. [Google Scholar] [CrossRef]
- Zhao, Y.; Zhang, X.; Yang, B.; Cai, S. A review of battery thermal management systems using liquid cooling and PCM. J. Energy Storage 2024, 76, 109836. [Google Scholar] [CrossRef]
- Jiang, K.; Liao, G.; Jiaqiang, E.; Zhang, F.; Chen, J.; Leng, E. Thermal management technology of power lithium-ion batteries based on the phase transition of materials: A review. J. Energy Storage 2020, 32, 101816. [Google Scholar] [CrossRef]
- Mahmud, M.; Rahman, K.S.; Rokonuzzaman, M.; Habib, A.A.; Islam, M.R.; Motakabber, S.M.; Channumsin, S.; Chowdhury, S. Lithium-ion battery thermal management for electric vehicles using phase change material: A review. Results Eng. 2023, 20, 101424. [Google Scholar] [CrossRef]
- Zeng, W.; Ma, C.; Hu, S.; Li, S.; Zhang, Y. The performance investigation and optimization of reciprocating flow applied for liquid-cooling-based battery thermal management system. Energy Convers. Manag. 2023, 292, 117378. [Google Scholar] [CrossRef]
- Zhi, M.; Fan, R.; Yang, X.; Zheng, L.; Yue, S.; Liu, Q.; He, Y. Recent research progress on phase change materials for thermal management of lithium-ion batteries. J. Energy Storage 2022, 45, 103694. [Google Scholar] [CrossRef]
- Wang, Q.; Jiang, B.; Li, B.; Yan, Y. A critical review of thermal management models and solutions of lithium-ion batteries for the development of pure electric vehicles. Renew. Sustain. Energy Rev. 2016, 64, 106–128. [Google Scholar] [CrossRef]
- Kim, J.; Oh, J.; Lee, H. Review on battery thermal management system for electric vehicles. J. Appl. Therm. Eng. 2019, 149, 192–212. [Google Scholar] [CrossRef]
- Alihosseini, A.; Shafaee, M. Experimental study and numerical simulation of a Lithium-ion battery thermal management system using a heat pipe. J. Energy Storage 2021, 39, 102616. [Google Scholar] [CrossRef]
- Huang, R.; Li, Z.; Hong, W.; Wu, Q.; Yu, X. Experimental and numerical study of PCM thermophysical parameters on lithium-ion battery thermal management. Energy Rep. 2020, 6, 8–19. [Google Scholar] [CrossRef]
- Ding, Y.; Zheng, Y.; Li, S.; Dong, T.; Gao, Z.; Zhang, T.; Li, W.; Rao, S.; Xiao, Y.; Chen, Y.; et al. A review of battery thermal management methods for electric vehicles. J. Electrochem. Energy Convers. Storage 2023, 20, 021002. [Google Scholar] [CrossRef]
- Zhao, G.; Wang, X.; Negnevitsky, M.; Zhang, H. A review of air-cooling battery thermal management systems for electric and hybrid electric vehicles. J. Power Sources 2021, 501, 230001. [Google Scholar] [CrossRef]
- Behi, H.; Karimi, D.; Behi, M.; Ghanbarpour, M.; Jaguemont, J.; Sokkeh, M.A.; Gandoman, F.H.; Berecibar, M.; Van Mierlo, J. A new concept of thermal management system in Li-ion battery using air cooling and heat pipe for electric vehicles. Appl. Therm. Eng. 2020, 174, 115280. [Google Scholar] [CrossRef]
- Gao, Y.; Ji, W.; Chen, X. Numerical study on thermal management of air-cooling model for diamond, triangular and rectangular lithium-ion batteries of electric vehicles. Processes 2022, 10, 1104. [Google Scholar] [CrossRef]
- Wang, Y.-W.; Jiang, J.-M.; Chung, Y.-H.; Chen, W.-C.; Shu, C.-M. Forced-air cooling system for large-scale lithium-ion battery modules during charge and discharge processes. J. Therm. Anal. Calorim. 2019, 135, 2891–2901. [Google Scholar] [CrossRef]
- Chen, K.; Li, Z.; Chen, Y.; Long, S.; Hou, J.; Song, M.; Wang, S. Design of parallel air-cooled battery thermal management system through numerical study. Energies 2017, 10, 1677. [Google Scholar] [CrossRef]
- Mustafa, J. Effect of inlet and outlet size, battery distance, and air inlet and outlet position on the cooling of a lithium-ion battery pack and utilizing outlet air of cooling system to heat an air handling unit. J. Energy Storage 2022, 46, 103826. [Google Scholar] [CrossRef]
- Zhang, F.; Shi, Y.; He, Y.; Liu, P. Design and Optimization of an F-type Air-Cooling Structure for Lithium-Ion Battery of Electric Vehicle. Energy Technol. 2023, 11, 2300243. [Google Scholar] [CrossRef]
- Zhao, C.; Zhang, Y.; Du, X.; Zhao, J.; Hu, Y. Modeling and Analysis of the Drying Process of Lithium-Ion Battery Electrodes Based on Non-Steady-State Drying Kinetics. Processes 2023, 11, 3236. [Google Scholar] [CrossRef]
- Bernardi, D.; Pawlikowski, E.; Newman, J. A general energy balance for battery systems. J. Electrochem. Soc. 1985, 132, 5–12. [Google Scholar] [CrossRef]
- Li, W.; Xiao, M.; Peng, X.; Garg, A.; Gao, L. A surrogate thermal modeling and parametric optimization of battery pack with air cooling for Evs. Appl. Therm. Eng. 2019, 147, 90–100. [Google Scholar] [CrossRef]



| Specification [unit] | Value |
|---|---|
| Thickness [mm] | 7.3 |
| Width [mm] | 56.0 |
| Length [mm] | 92.5 |
| Densities [kg/m3] | 2776 |
| Specific heat capacity [J/(kg·K)] | 1633 |
| Radial thermal conductivity [W/(m·K)] | 0.94 |
| Axial thermal conductivity [W/(m·K)] | 2.75 |
| Tangential thermal conductivity [W/(m·K)] | 0.94 |
| Nominal voltage [V] | 3.7 |
| Nominal capacity [Ah] | 5 |
| Internal resistance [mΩ] | 60 |
| φ | 0.25 | 0.5 | 0.75 | 1 | 1.25 | 1.5 | 1.75 | 2 |
|---|---|---|---|---|---|---|---|---|
| Inlet height (mm) | 25 | 25 | 25 | 25 | 25 | 25 | 25 | 25 |
| Outlet height (mm) | 100 | 50 | 33.3 | 25 | 20 | 16.7 | 14.3 | 12.5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pan, Z.; Zhi, M.; Yuan, L.; Xu, Q.; Sun, Q.; Chen, X. Study on the Effect of Air Velocity and Duct Area on the Heat Dissipation of Lithium-Ion Batteries. Batteries 2025, 11, 43. https://doi.org/10.3390/batteries11020043
Pan Z, Zhi M, Yuan L, Xu Q, Sun Q, Chen X. Study on the Effect of Air Velocity and Duct Area on the Heat Dissipation of Lithium-Ion Batteries. Batteries. 2025; 11(2):43. https://doi.org/10.3390/batteries11020043
Chicago/Turabian StylePan, Zhiheng, Maoyong Zhi, Lei Yuan, Qinrou Xu, Qiang Sun, and Xiantao Chen. 2025. "Study on the Effect of Air Velocity and Duct Area on the Heat Dissipation of Lithium-Ion Batteries" Batteries 11, no. 2: 43. https://doi.org/10.3390/batteries11020043
APA StylePan, Z., Zhi, M., Yuan, L., Xu, Q., Sun, Q., & Chen, X. (2025). Study on the Effect of Air Velocity and Duct Area on the Heat Dissipation of Lithium-Ion Batteries. Batteries, 11(2), 43. https://doi.org/10.3390/batteries11020043





