Electrical Modeling and Characterization of Electrochemical Impedance Spectroscopy-Based Energy Storage Systems
Abstract
1. Introduction
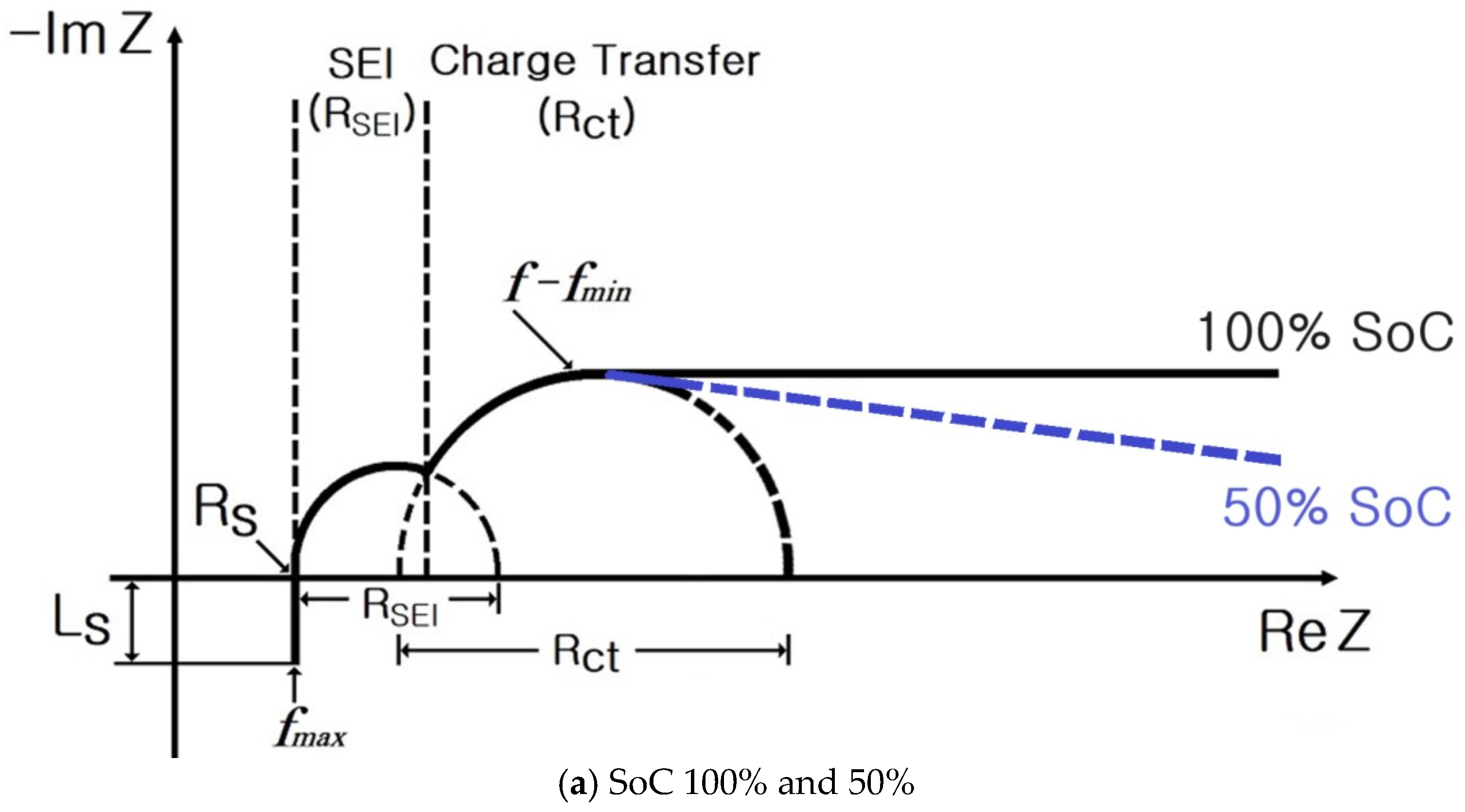
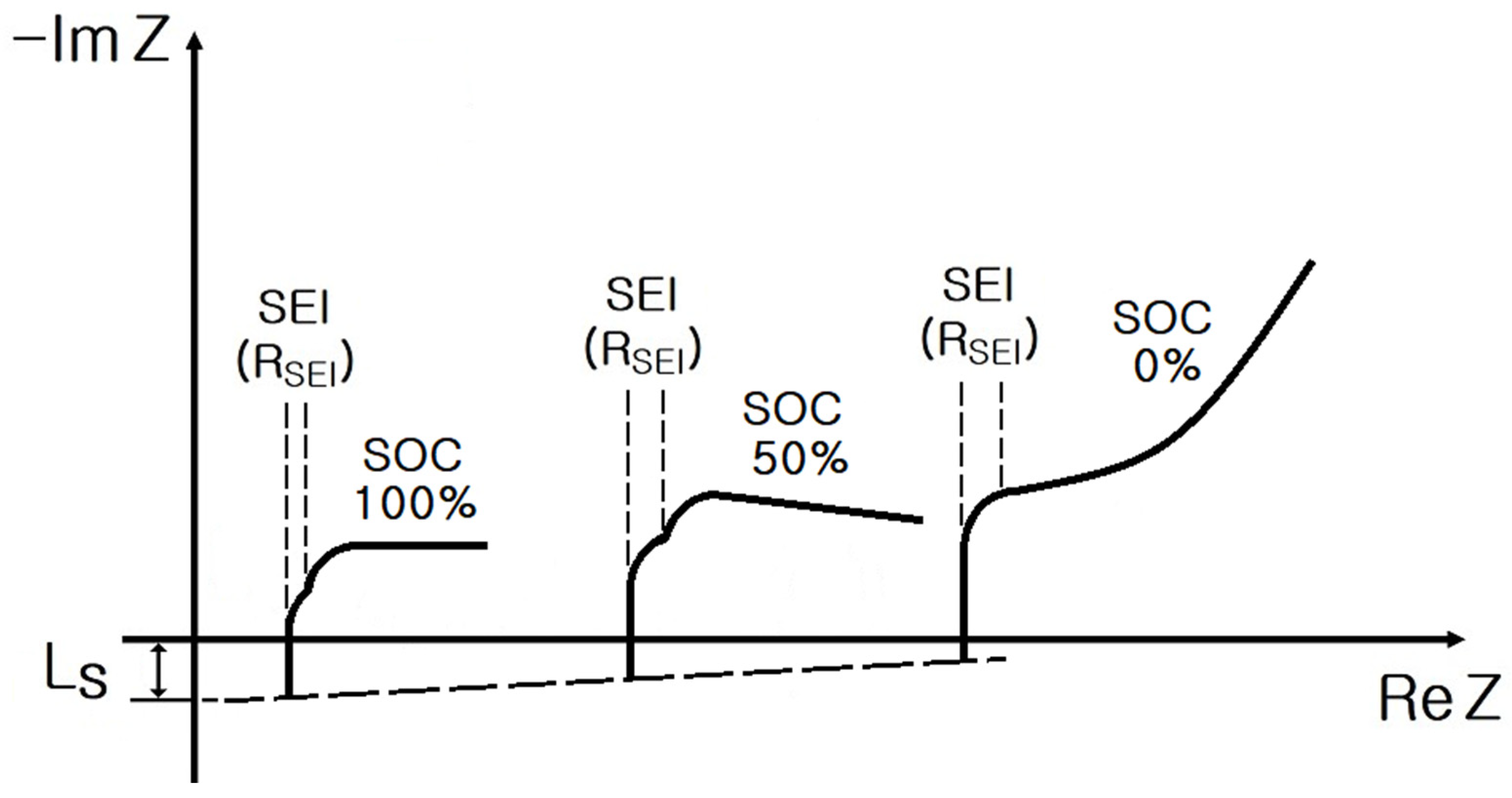
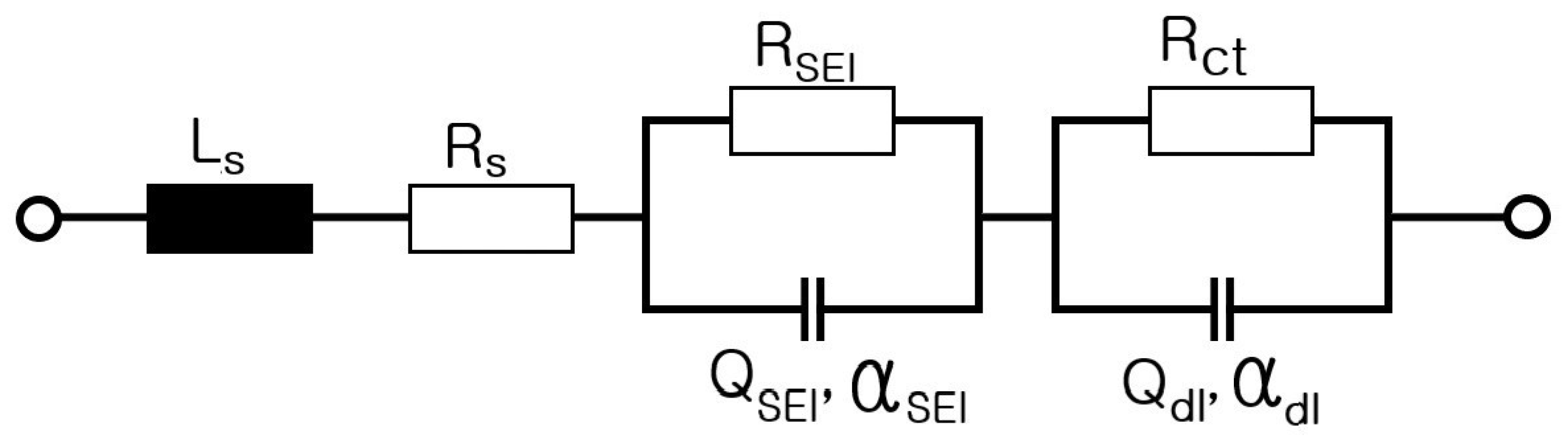
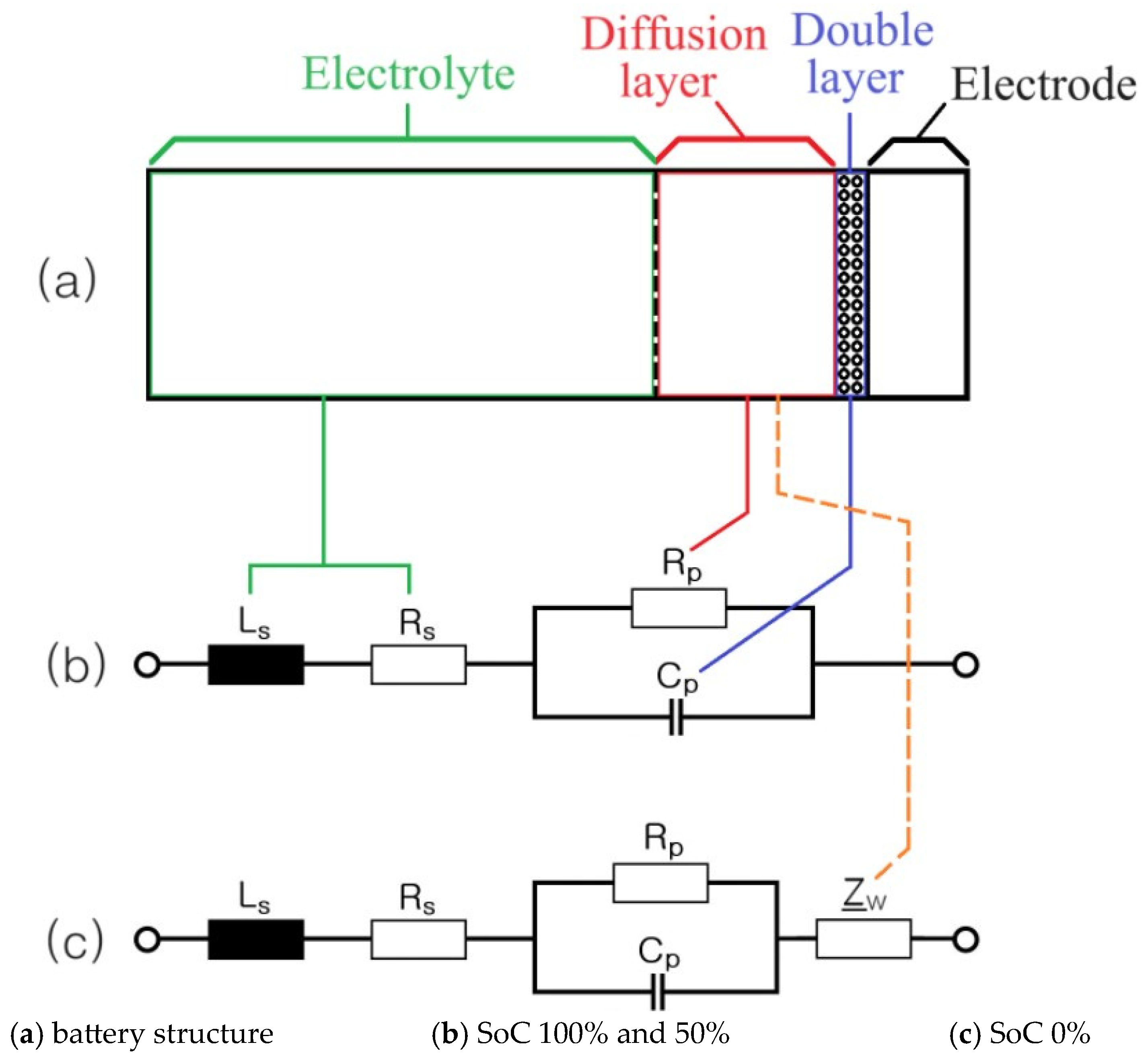
2. Impedance Spectrum of Cylindrical and Pouch-Type ESS
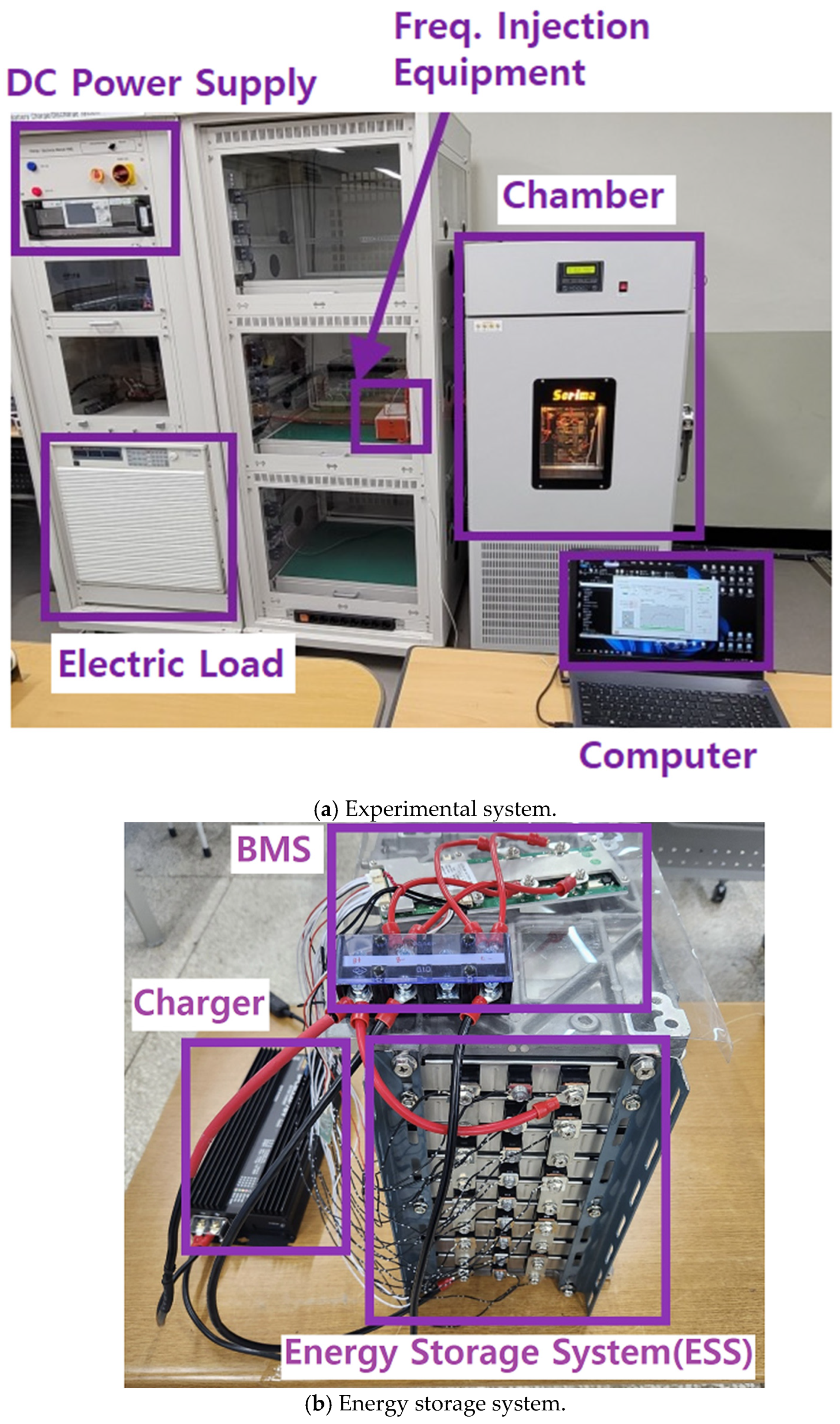
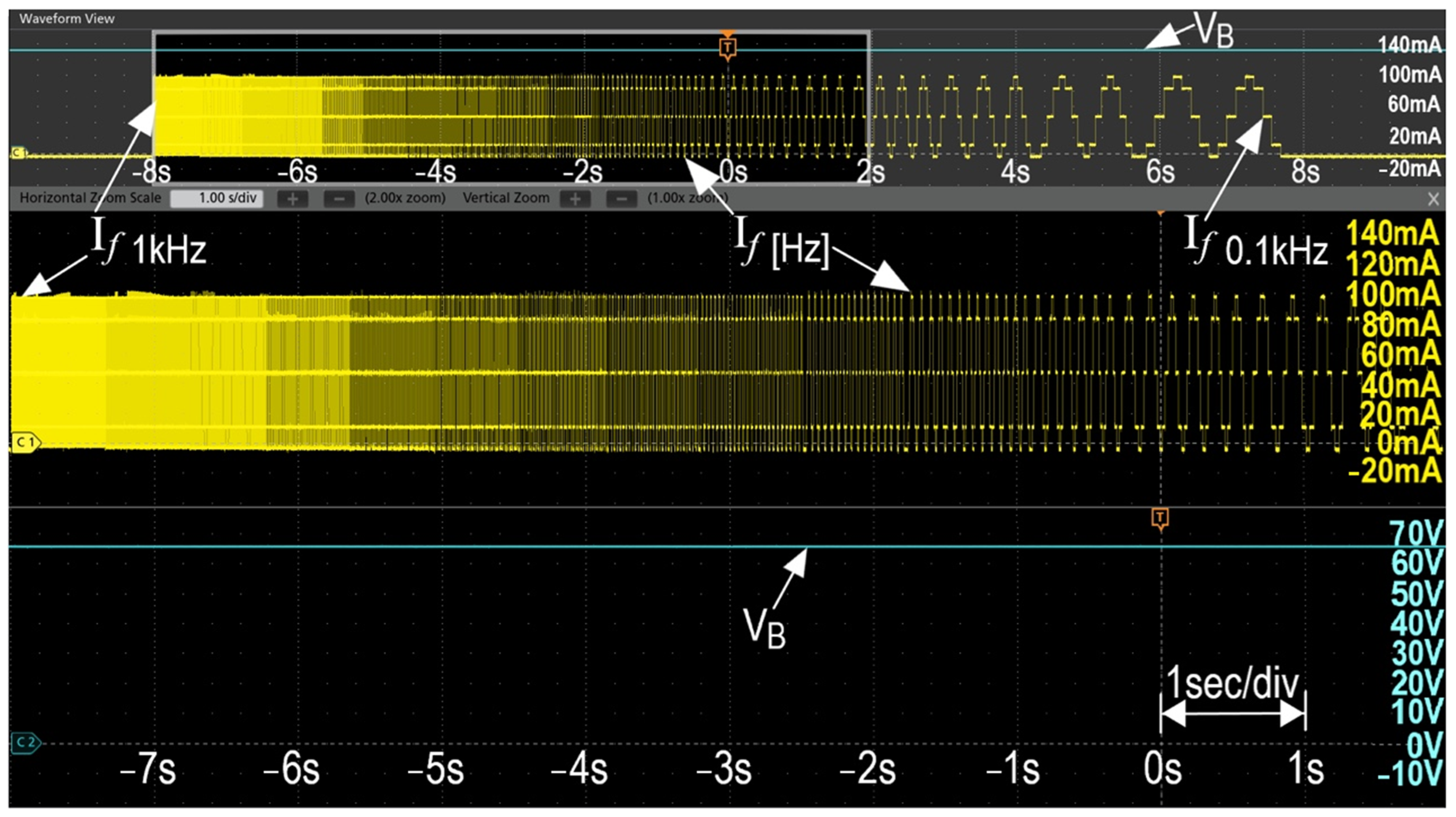
3. Experimental Equipment and Systems
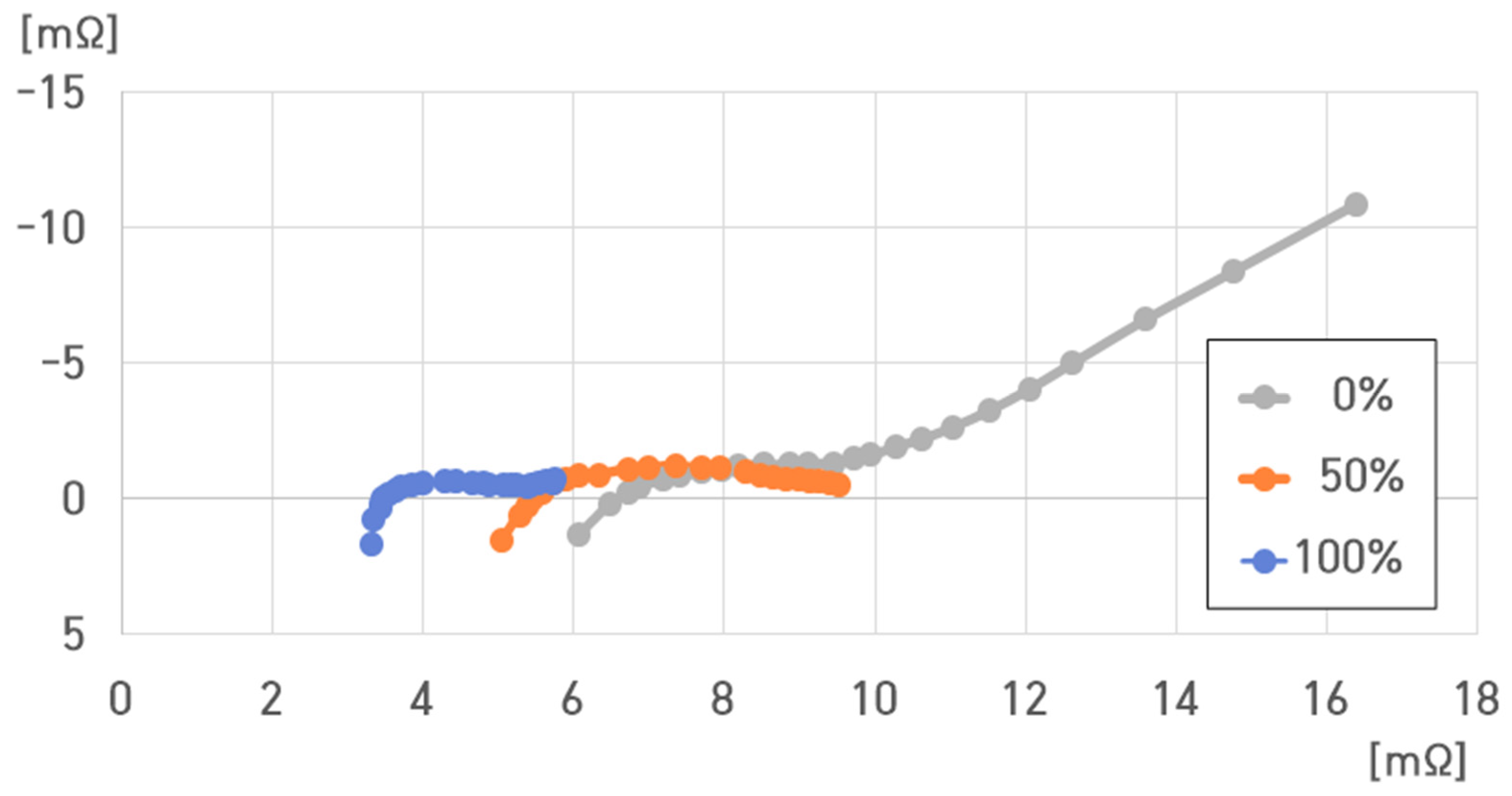
4. Impedance Characteristic Analysis of a Cell of a Pouch-Type Lithium-Ion Battery (75 Ah)
- -
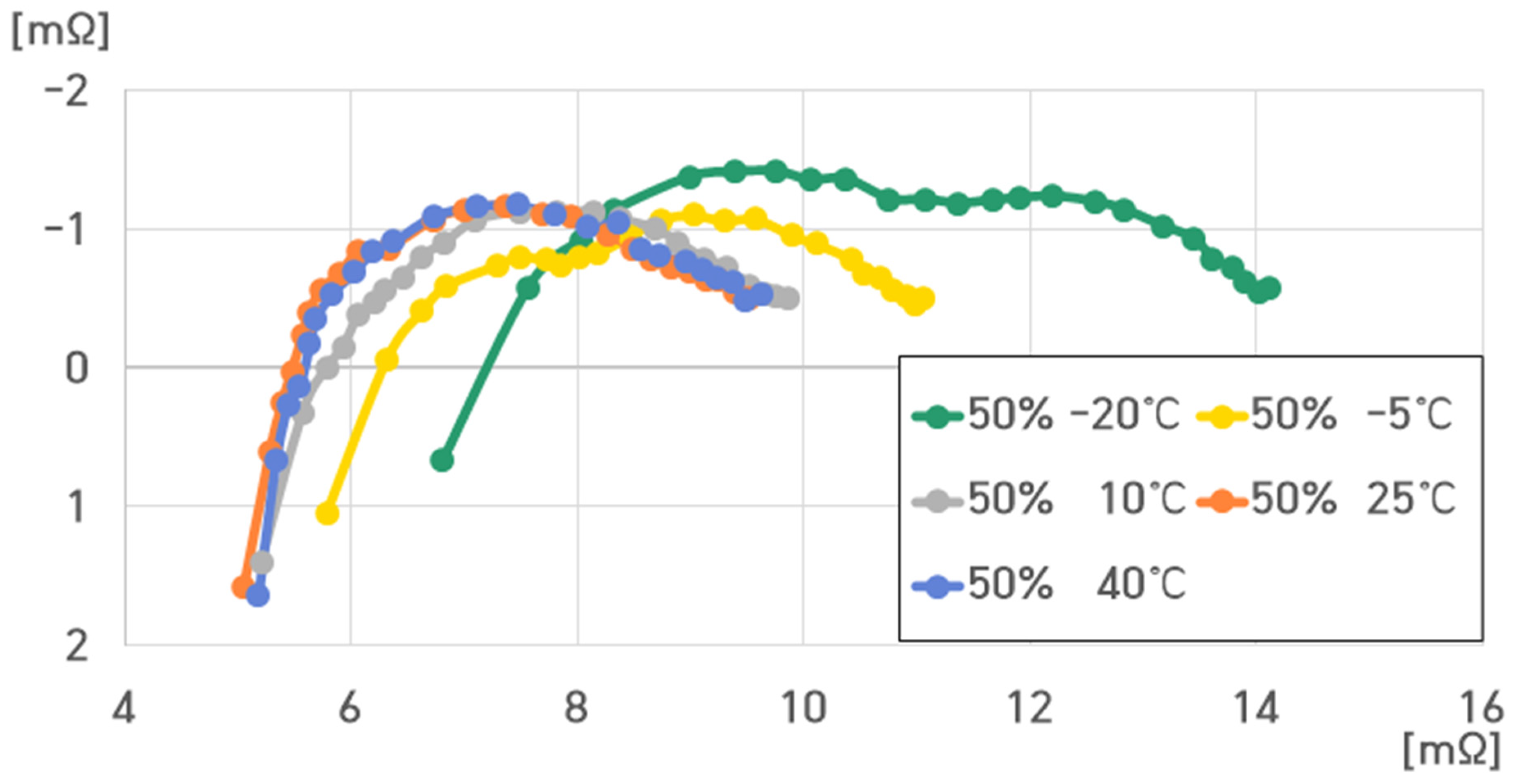
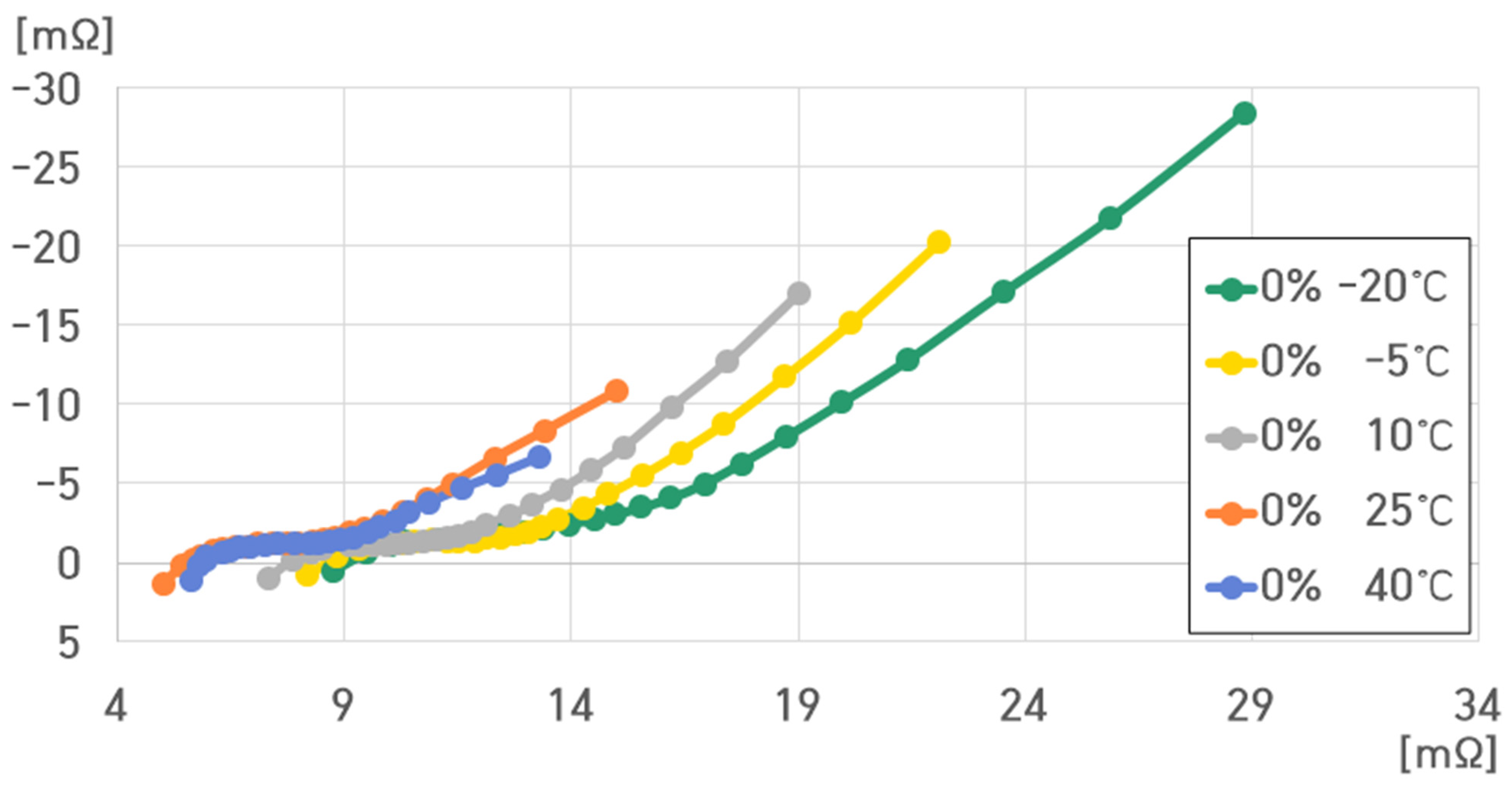
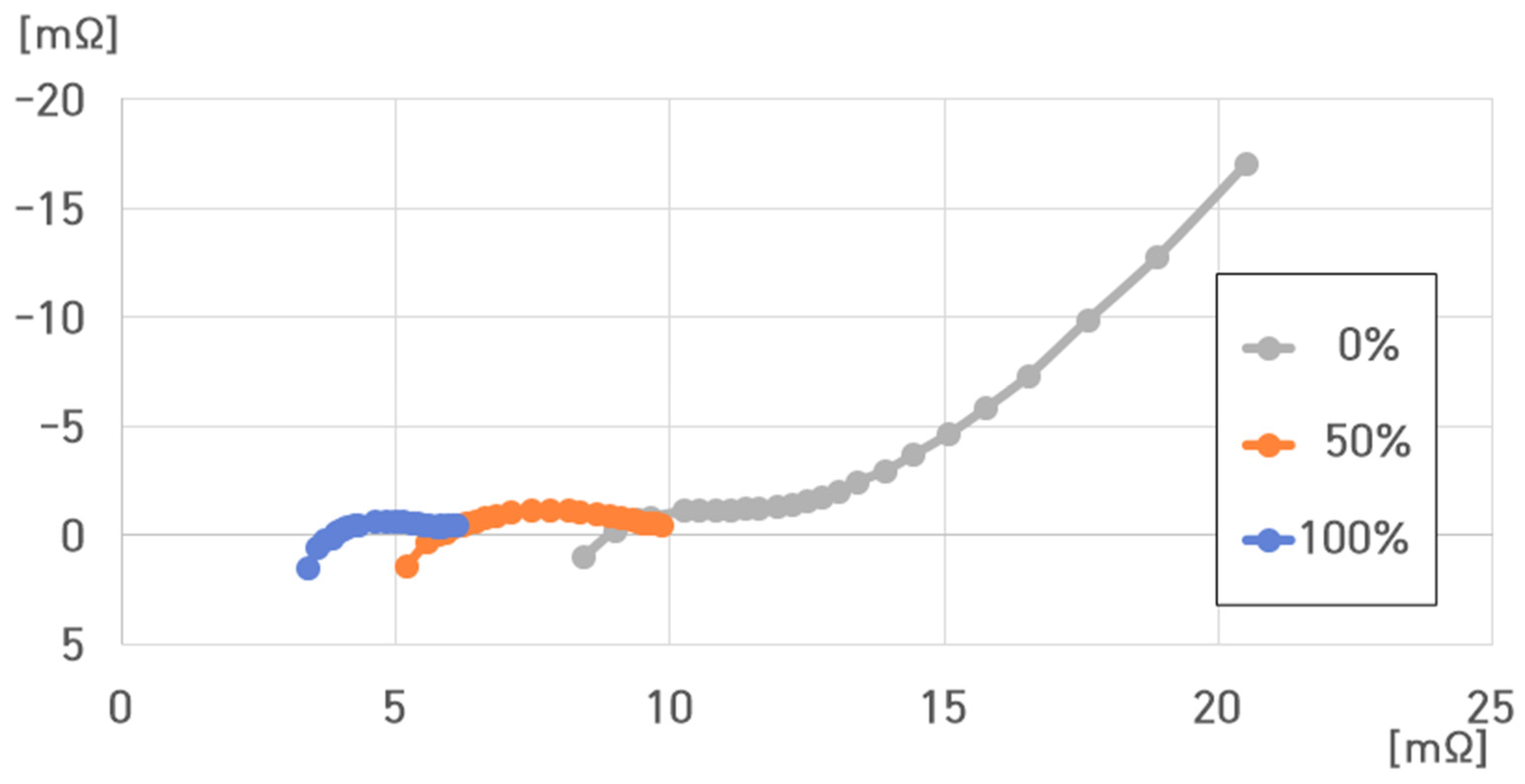
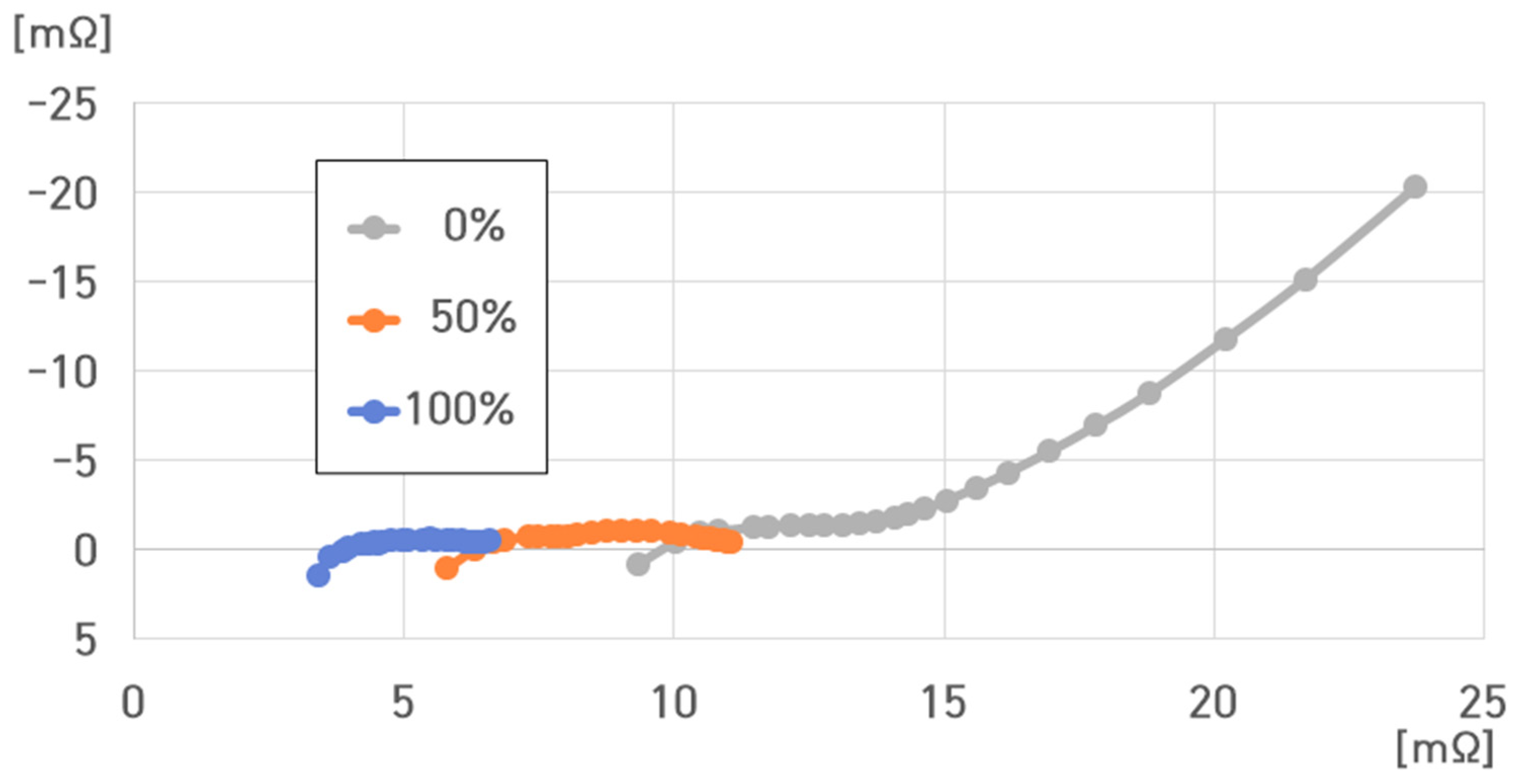
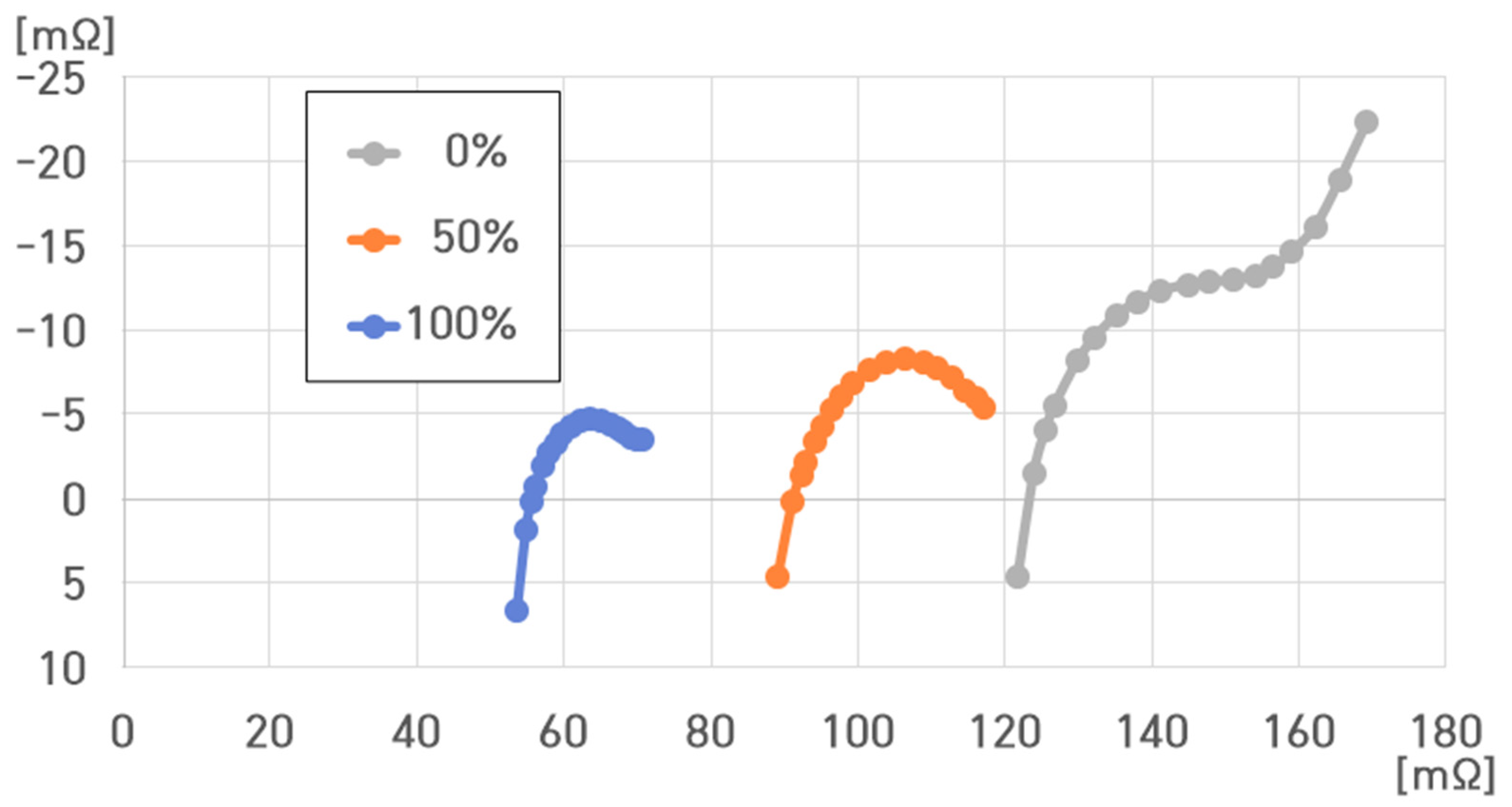
- SoC: 100%, 50%, and 0%;
- -
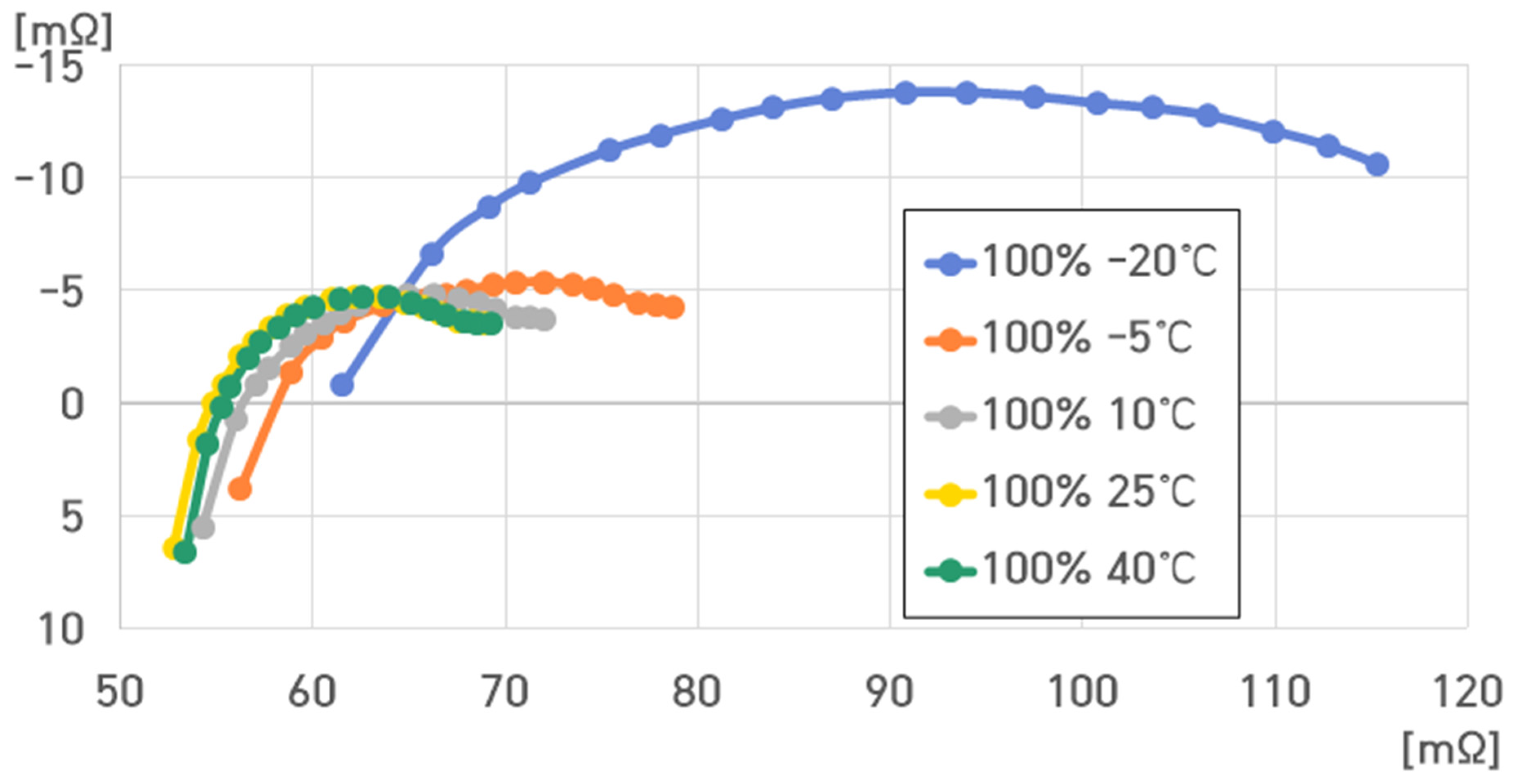
- Temperature: 40 °C, 25 °C, 10 °C, −5 °C, and −20 °C.
- (1)
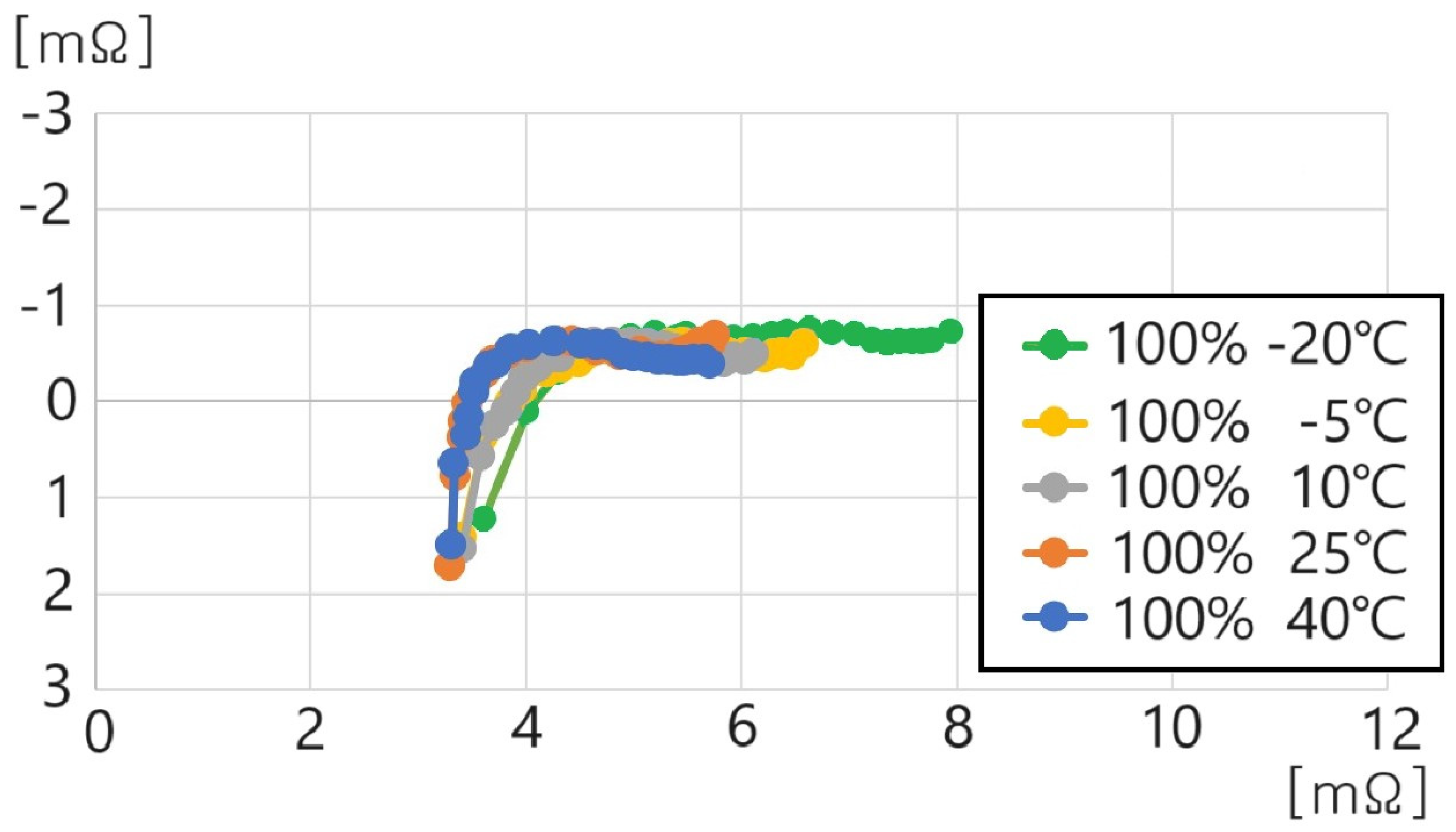
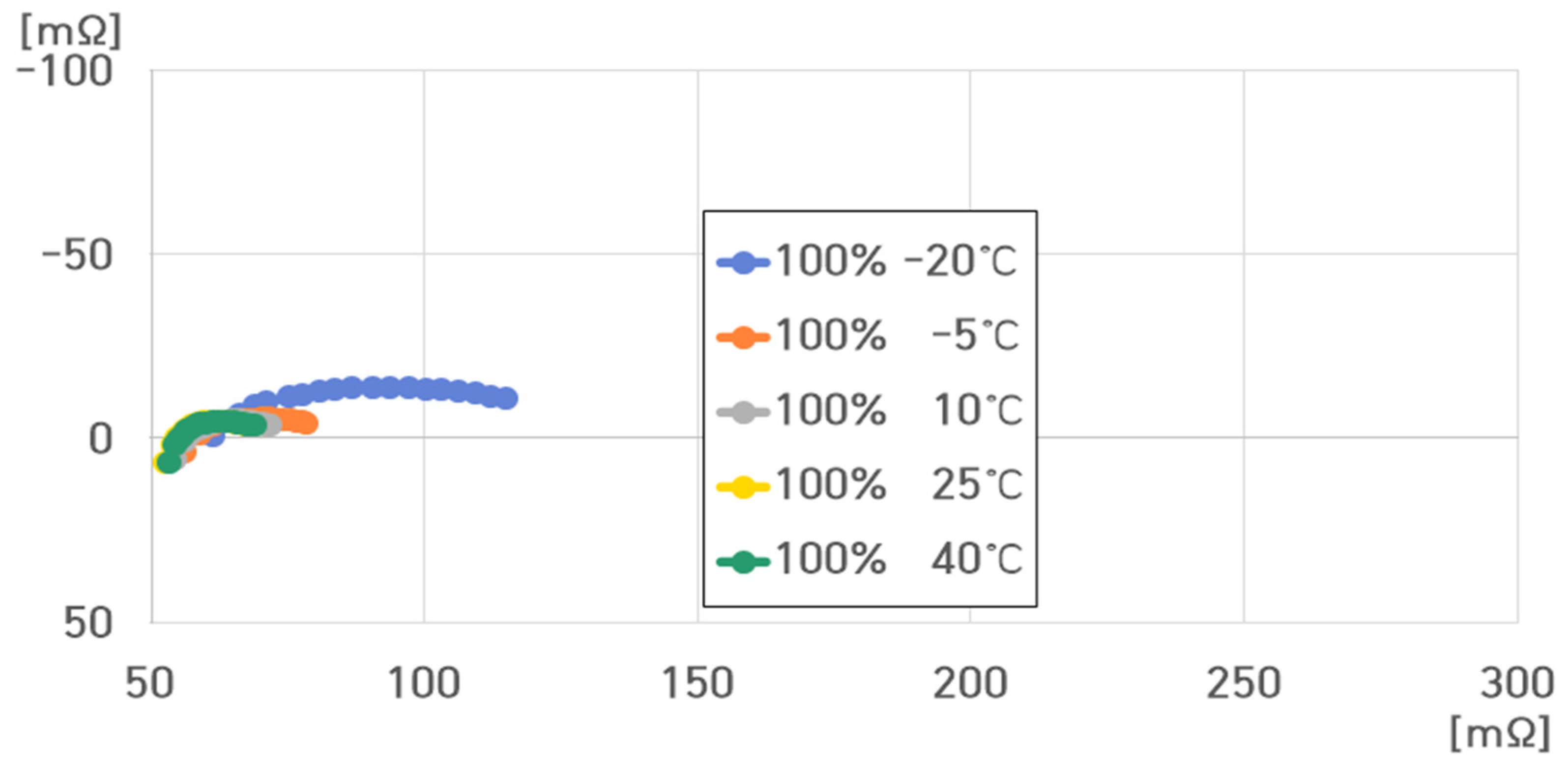
- 100% SoC, 40 °C: , , , ;
- (2)
- 100% SoC, 25 °C: , , , ;
- (3)
- 100% SoC, 10 °C: , , , ;
- (4)
- 100% SoC, −5 °C: , , , ;
- (5)
- 100% SoC, −20 °C: , , , .
- (1)
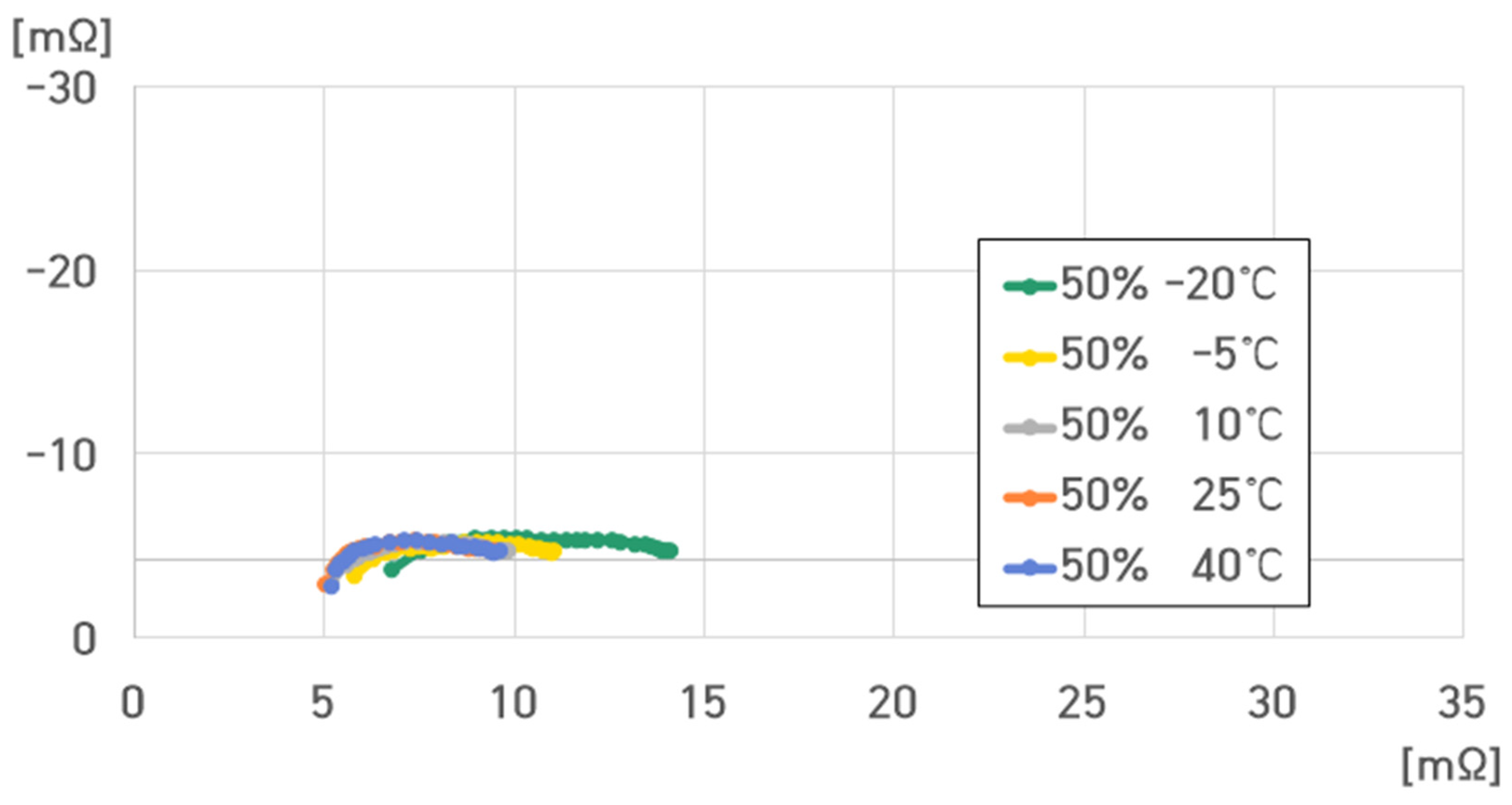
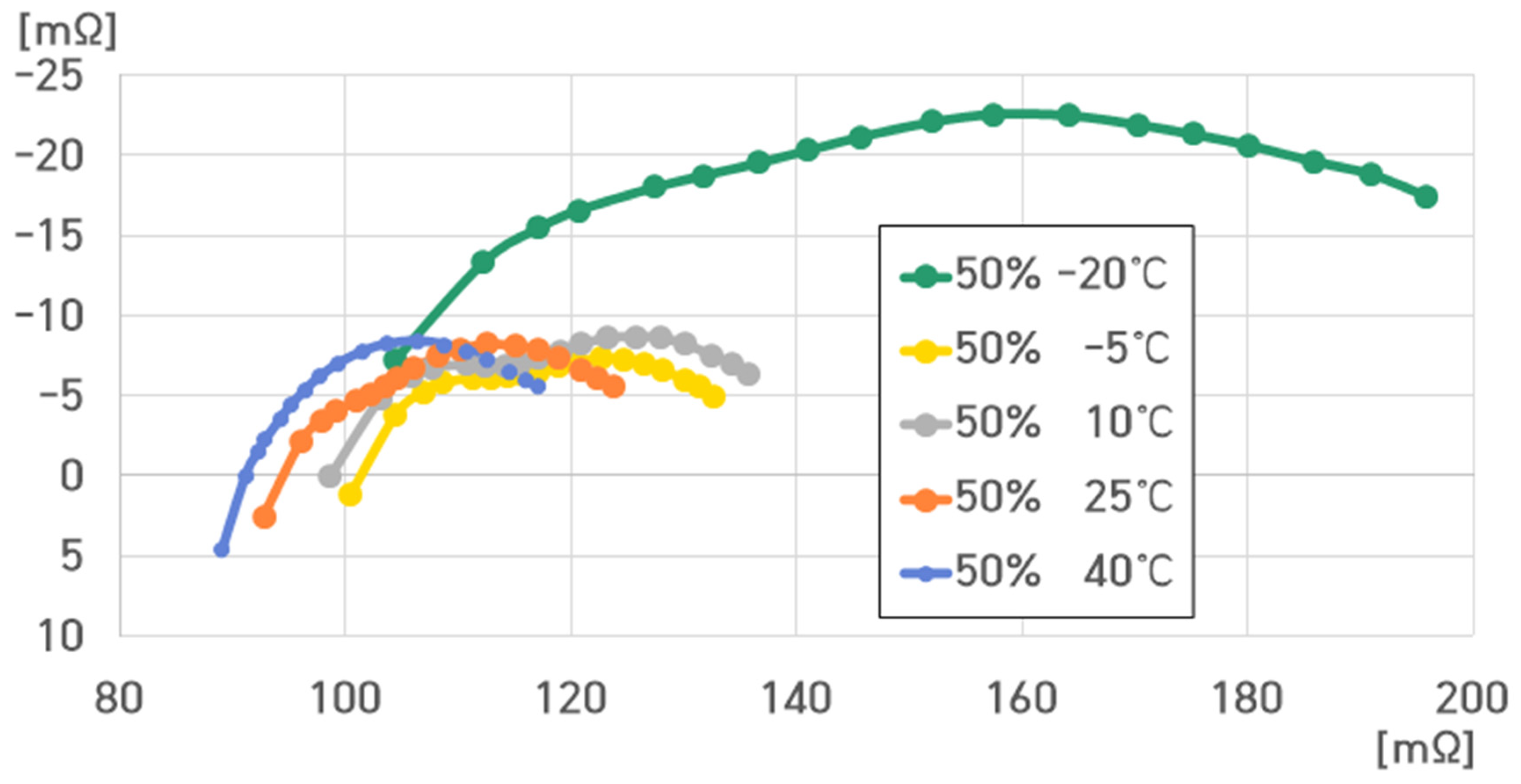
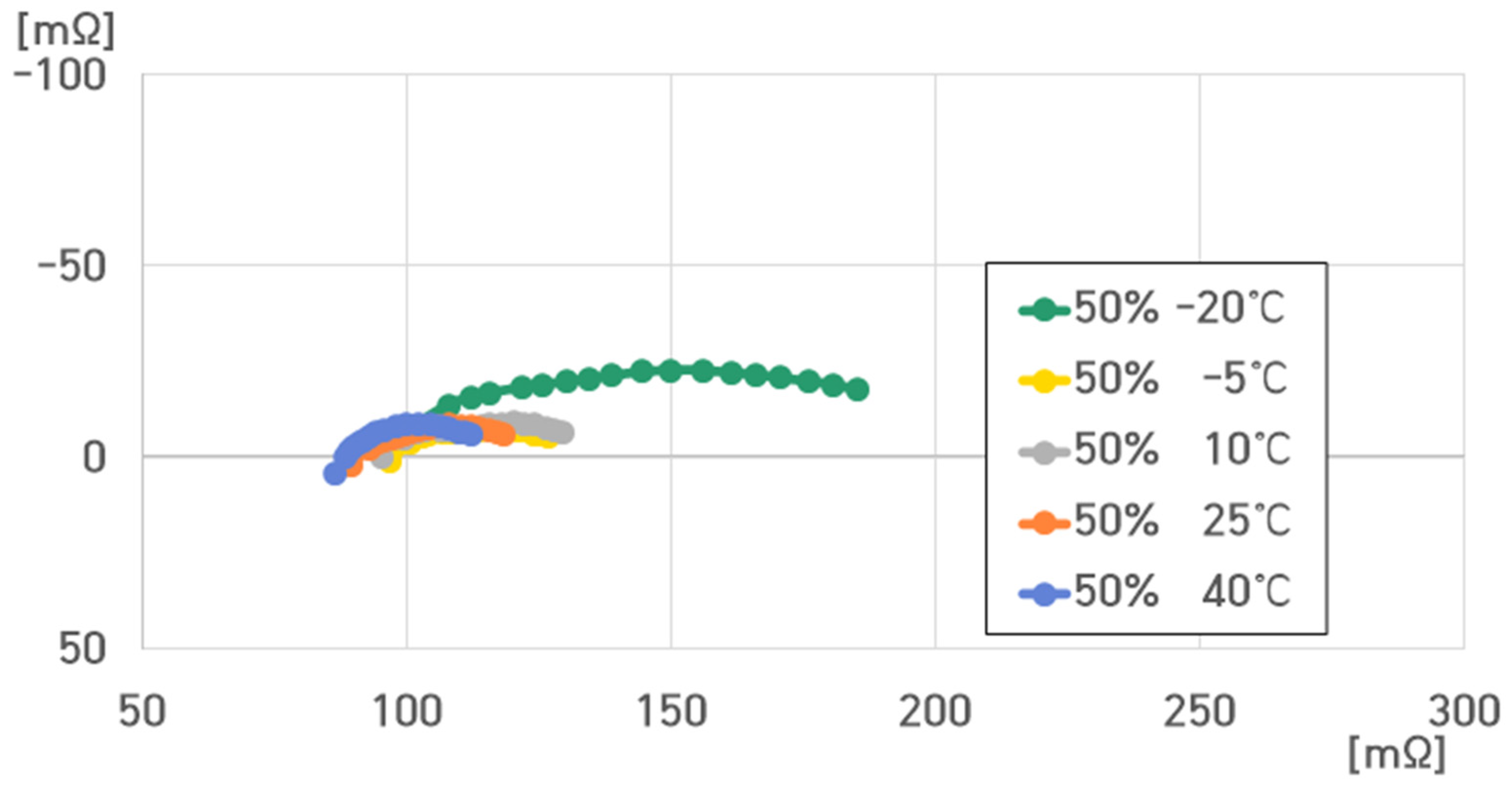
- 50% SoC, 40 °C: , , , ;
- (2)
- 50% SoC, 25 °C: , , , ;
- (3)
- 50% SoC, 10 °C: , , , ;
- (4)
- 50% SoC, −5 °C: , , , ;
- (5)
- 50% SoC, −20 °C: , , , .
- (1)
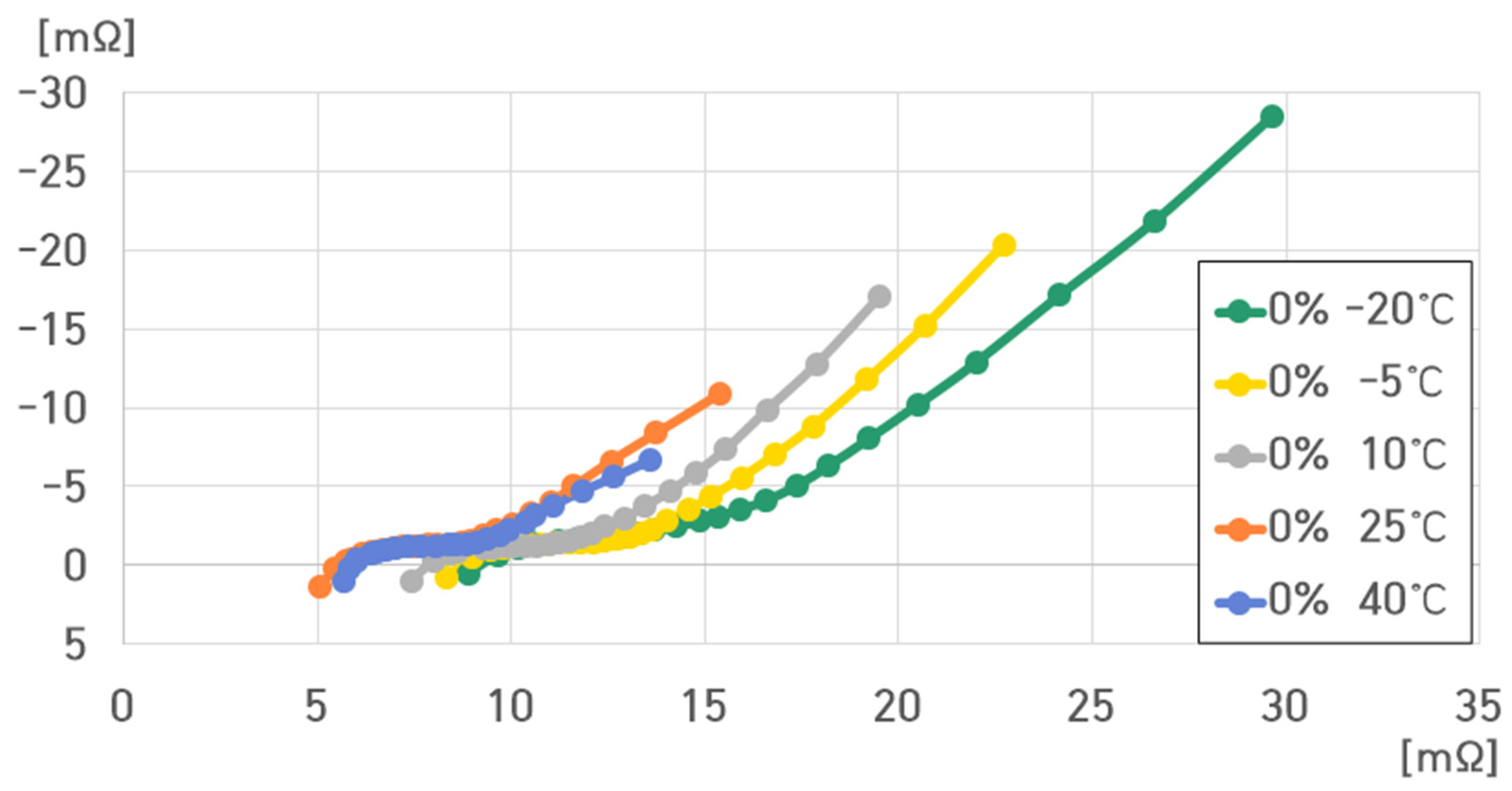
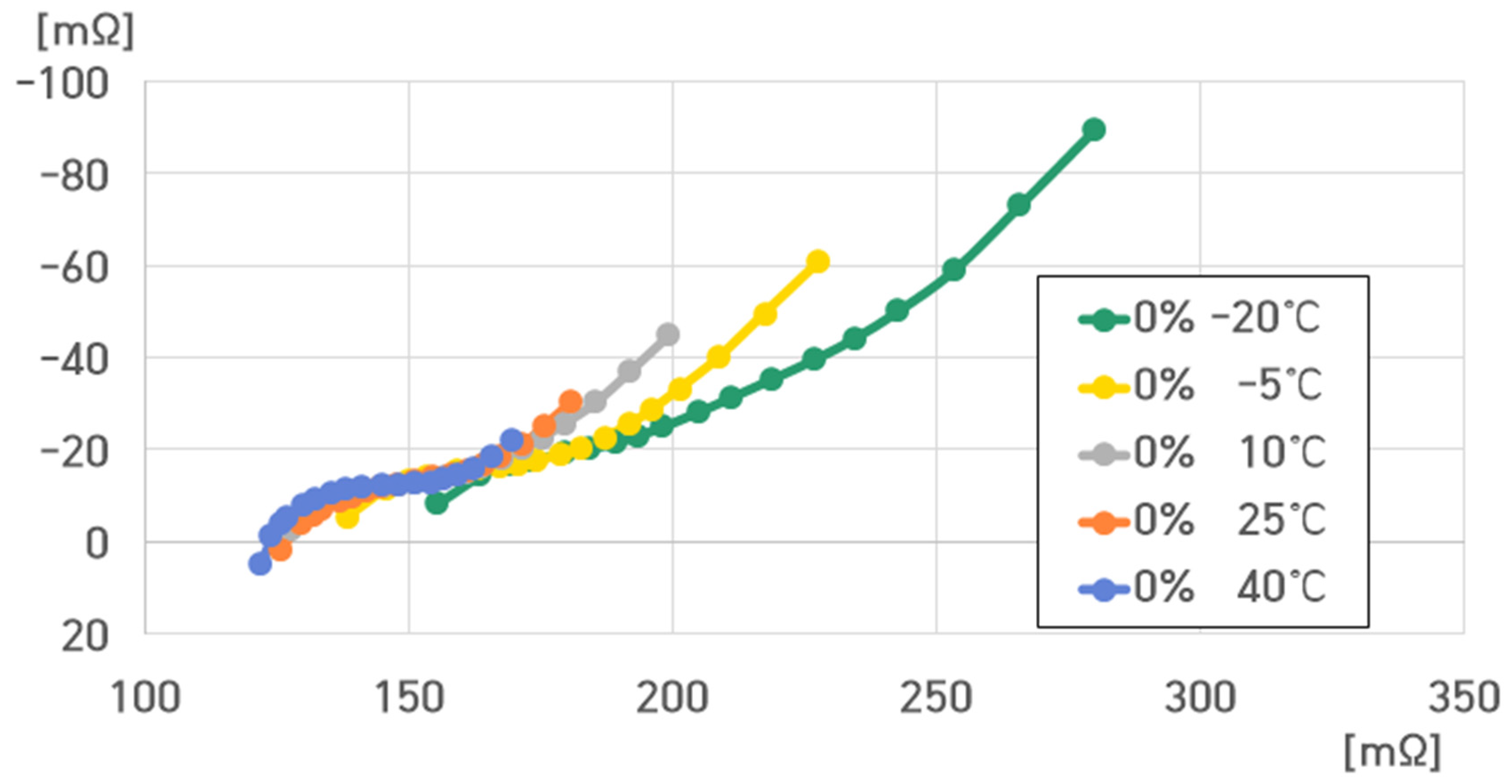
- 0% SoC, 40 °C: , , , ;
- (2)
- 0% SoC, 25 °C: , , , ;
- (3)
- 0% SoC, 10 °C: , , , ;
- (4)
- 0% SoC, −5 °C: , , , ;
- (5)
- 0% SoC, −20 °C: , , , .
- (1)
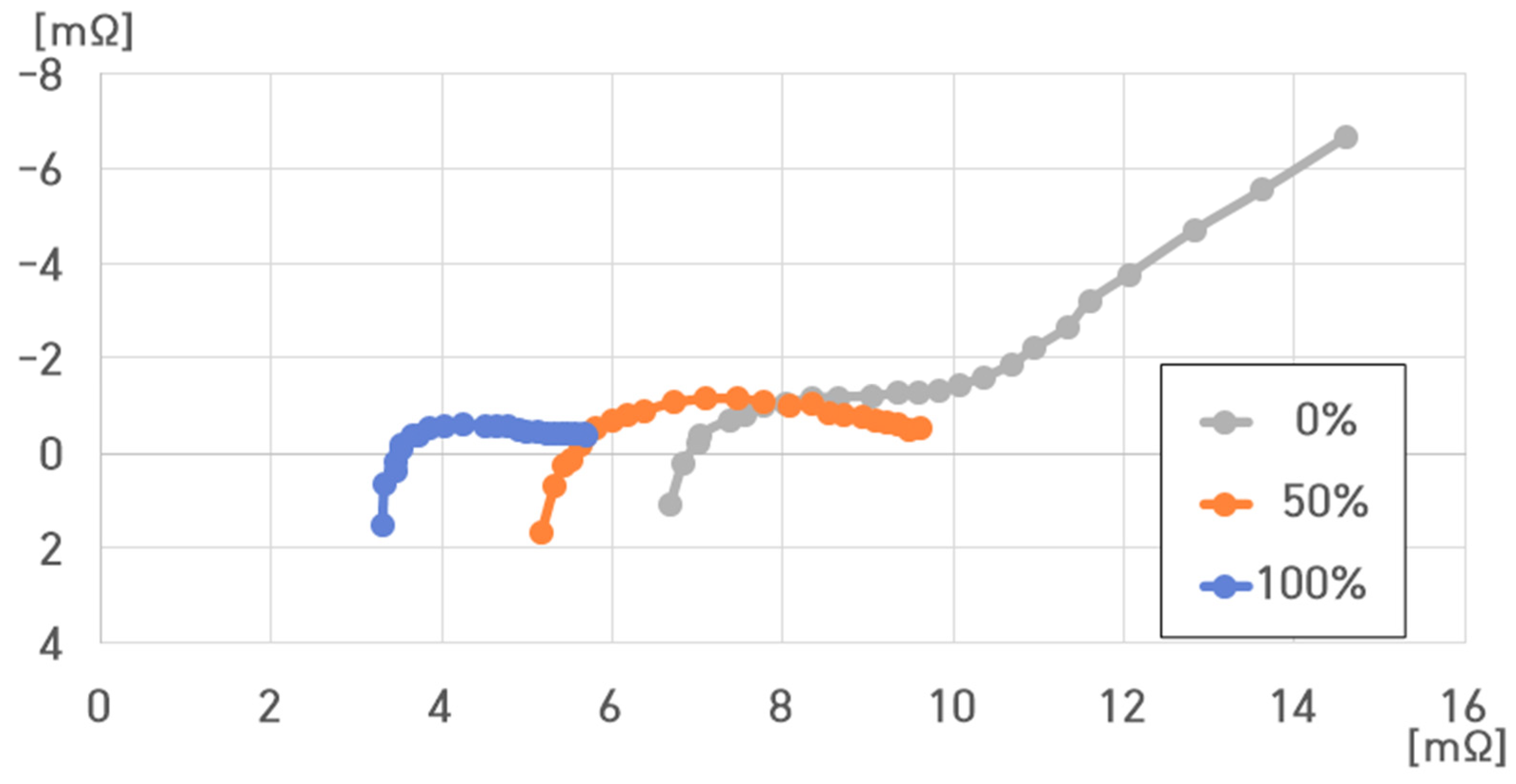
- 40 °C, 100% SoC: , , , ;
- (2)
- 40 °C, 50% SoC: , , , ;
- (3)
- 40 °C, 0% SoC: , , , .
- (1)
- 25 °C, 100% SoC: , , , ;
- (2)
- 25 °C, 50% SoC: , , , ;
- (3)
- 25 °C, 0% SoC: , , , .
- (1)
- 10 °C, 100% SoC: , , ,
- (2)
- 10 °C, 50% SoC: , , , ;
- (3)
- 10 °C, 0% SoC: , , , .
- (1)
- −5 °C, 100% SoC: , , , ;
- (2)
- −5 °C, 50% SoC: , , , ;
- (3)
- −5 °C, 0% SoC: , , , .
- (1)
- −20 °C, 100% SoC: , , , ;
- (2)
- −20 °C, 50% SoC: , , , ;
- (3)
- −20 °C, 0% SoC: , , , .
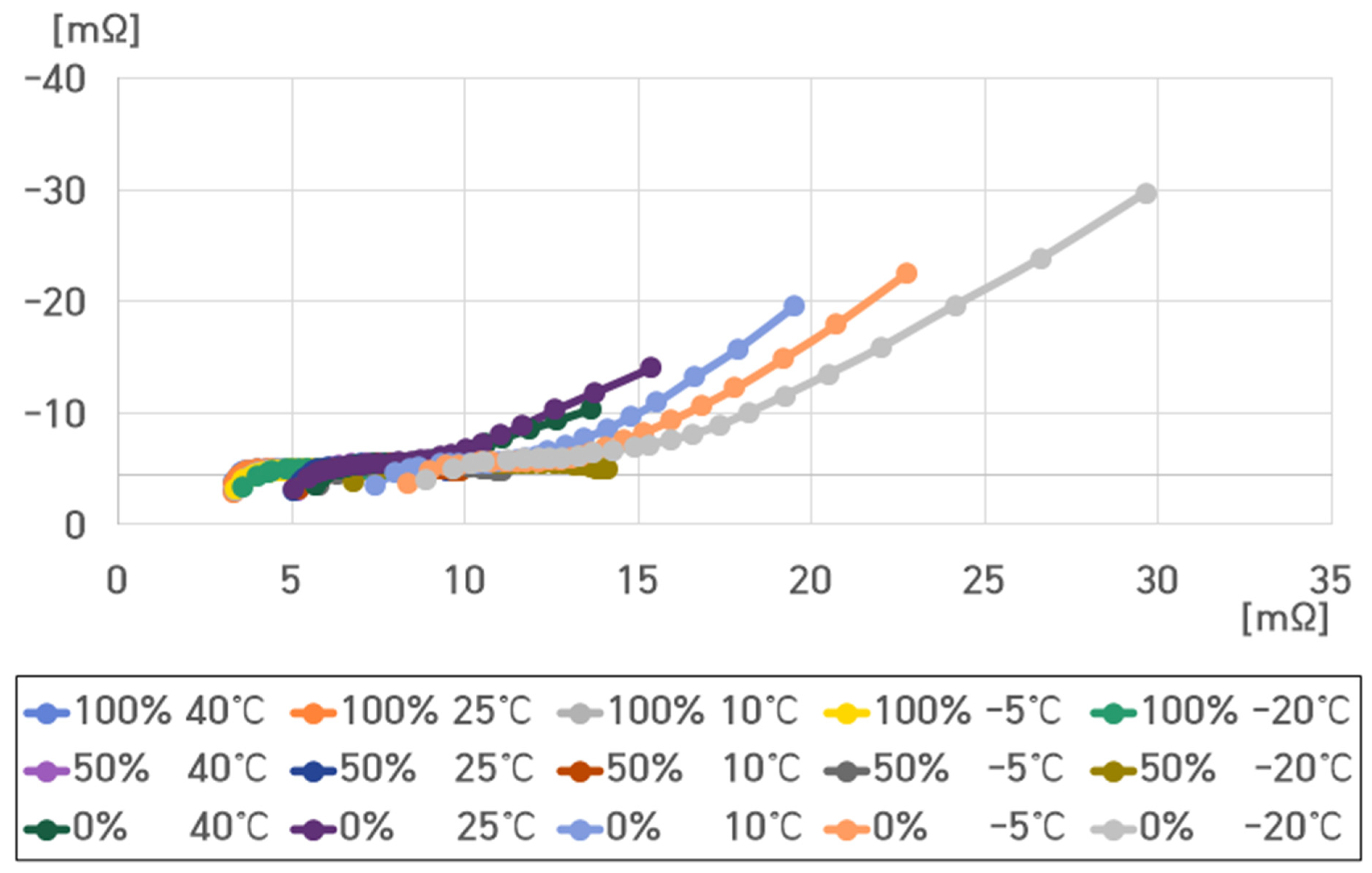
5. Analysis of Impedance Characteristics of 16 Cells of 4.4 kWh ESS
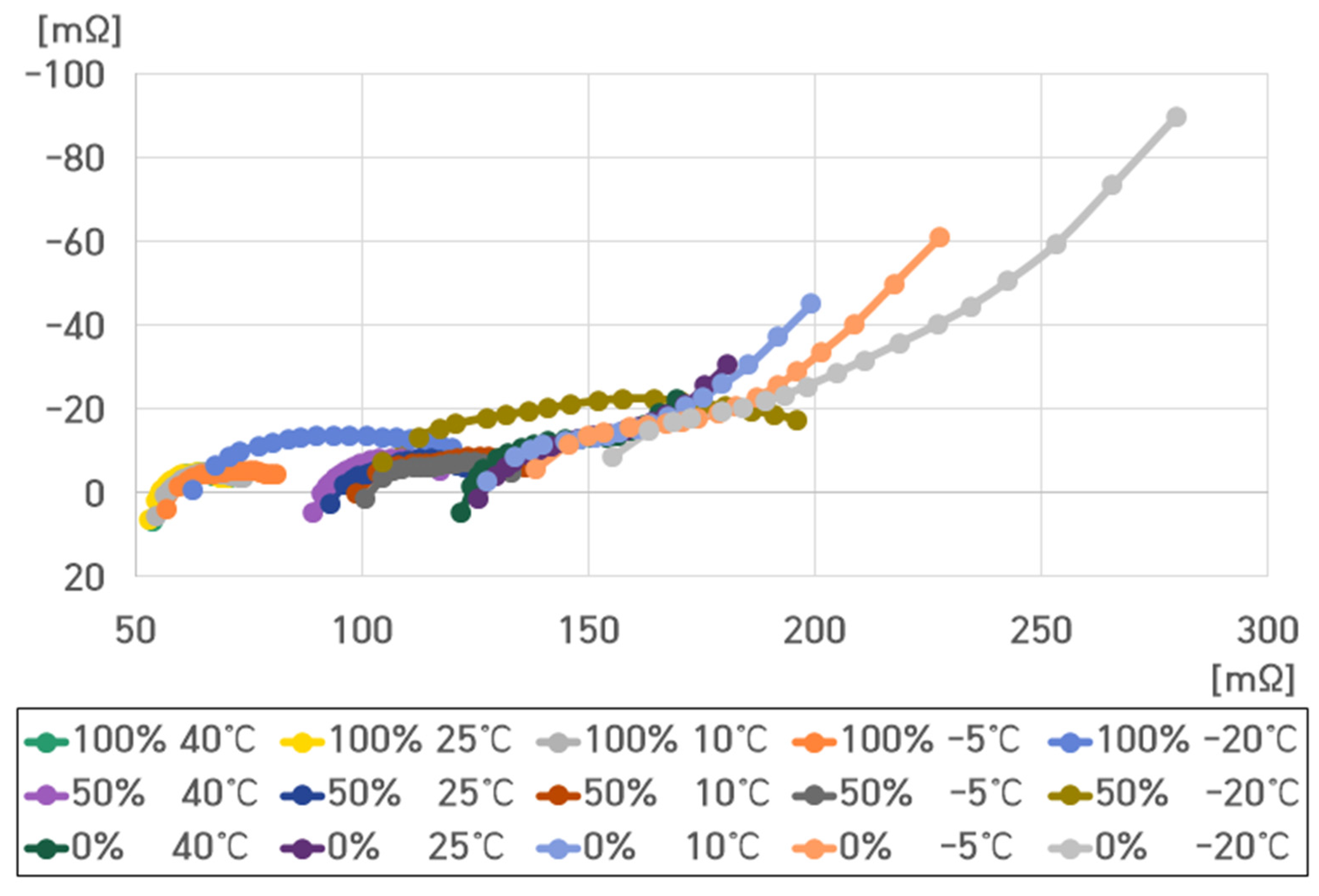
- (1)
- 100% SoC, 40 °C: , , , ;
- (2)
- 100% SoC, 25 °C: , , , ;
- (3)
- 100% SoC, 10 °C: , , , ;
- (4)
- 100% SoC, −5 °C: , , , ;
- (5)
- 100% SoC, −20 °C: , , , .
- (1)
- 50% SoC, 40 °C: , , , ;
- (2)
- 50% SoC, 25 °C: , , , ;
- (3)
- 50% SoC, 10 °C: , , , ;
- (4)
- 50% SoC, −5 °C: , , , ;
- (5)
- 50% SoC, −20 °C: , , , .
- (1)
- 0% SoC, 40 °C: , , , ;
- (2)
- 0% SoC, 25 °C: , , , ;
- (3)
- 0% SoC, 10 °C: , , , ;
- (4)
- 0% SoC, −5 °C: , , , ;
- (5)
- 0% SoC, −20 °C: , , , .
- (1)
- 40 °C, 100% SoC: , , , ;
- (2)
- 40 °C, 50% SoC: , , , ;
- (3)
- 40 °C, 0% SoC: , , , .
- (1)
- 25 °C, 100% SoCs: , , , ;
- (2)
- 25 °C, 50% SoC: , , , ;
- (3)
- 25 °C, 0% SoC: , , , .
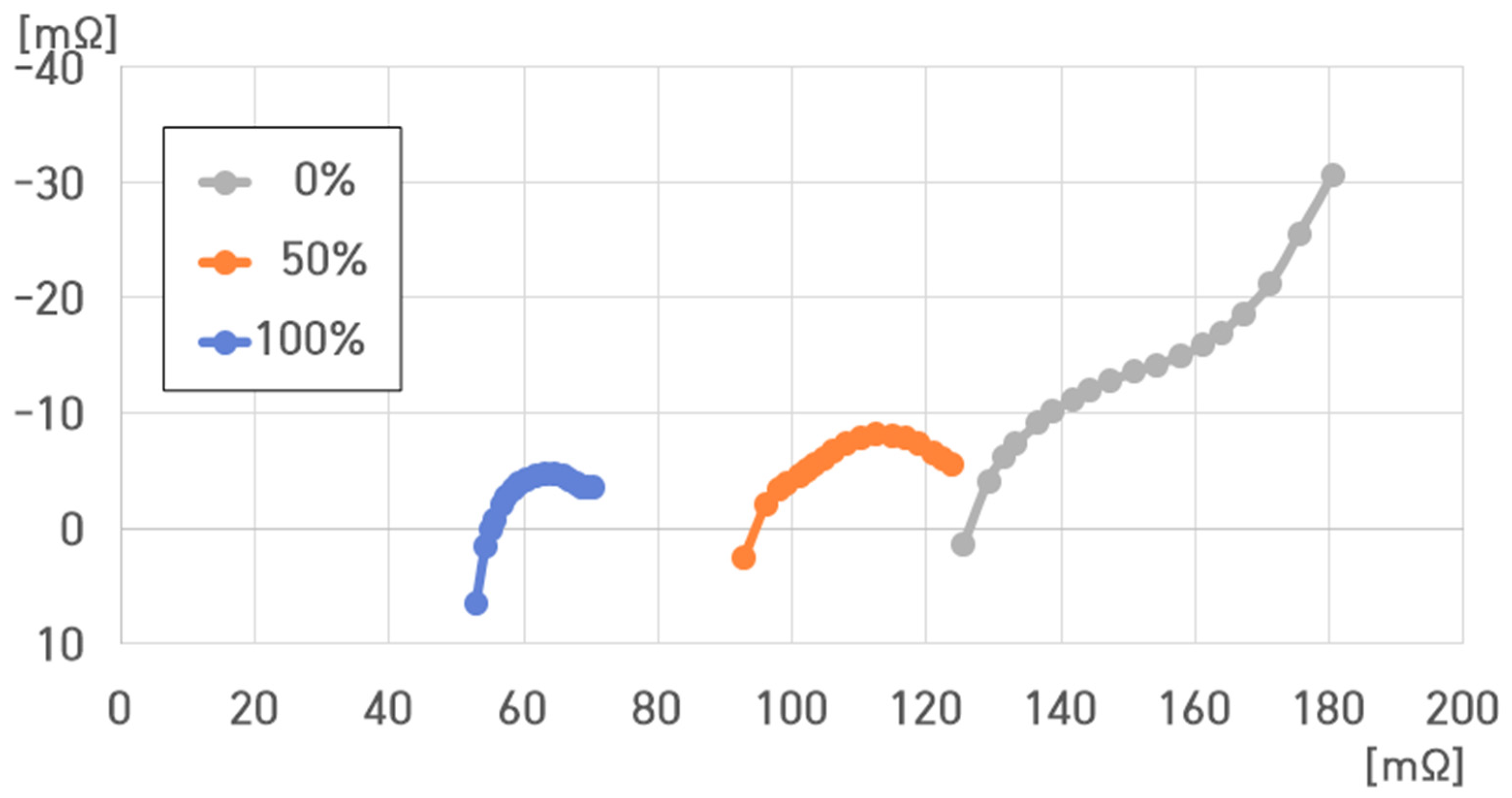
- (1)
- 10 °C, 100% SoC: , , , ;
- (2)
- 10 °C, 50% SoC: , , , ;
- (3)
- 10 °C, 0% SoC: , , , .
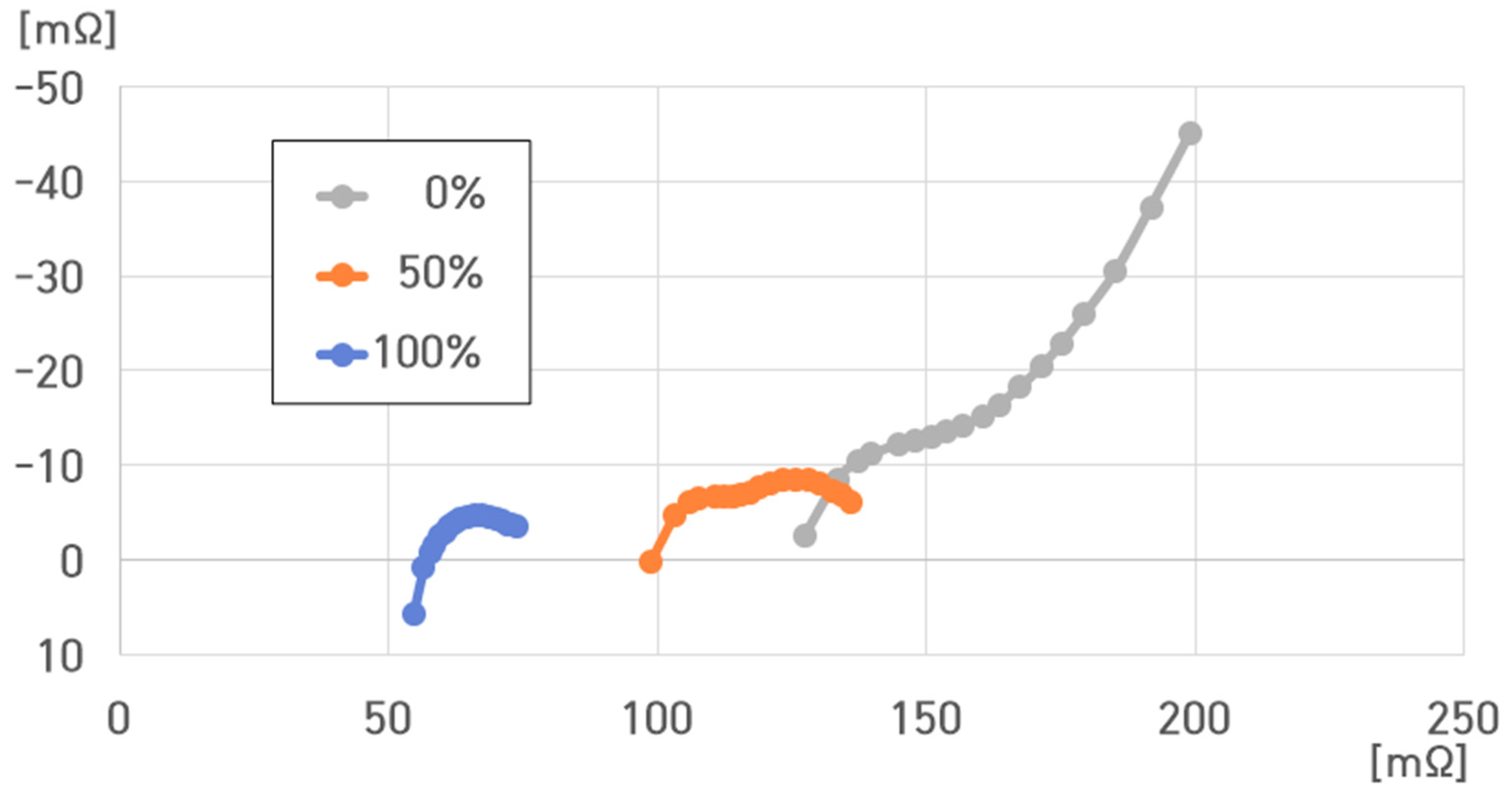
- (1)
- −5 °C, 100% SoC: , , , ;
- (2)
- −5 °C, 50% SoC: , , , ;
- (3)
- −5 °C, 0% SoC: , , , .
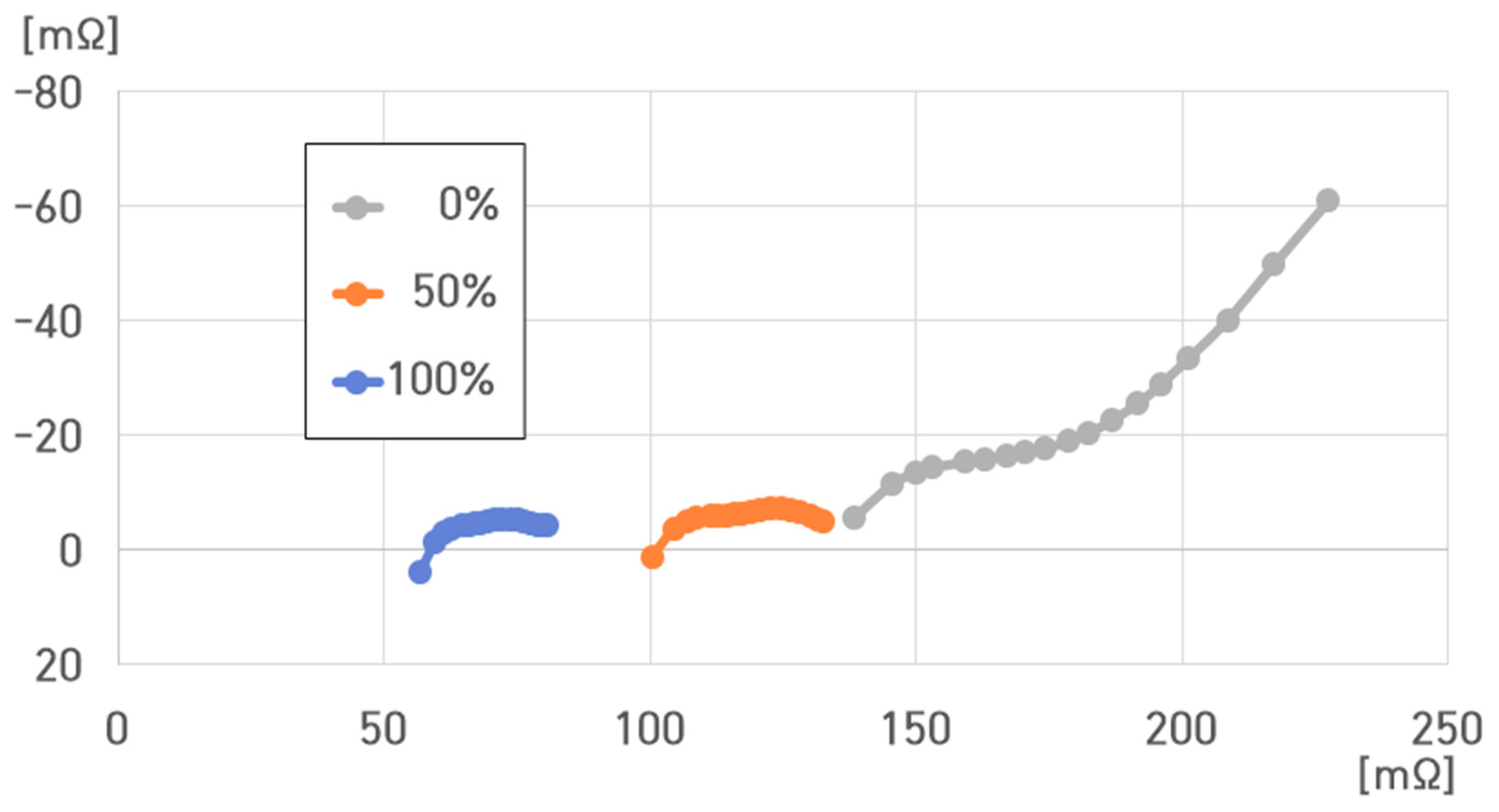
- (1)
- −20 °C, 100% SoC: , , , ;
- (2)
- −20 °C, 50% SoC: , , , ;
- (3)
- −20 °C, 0% SoC: , , , .
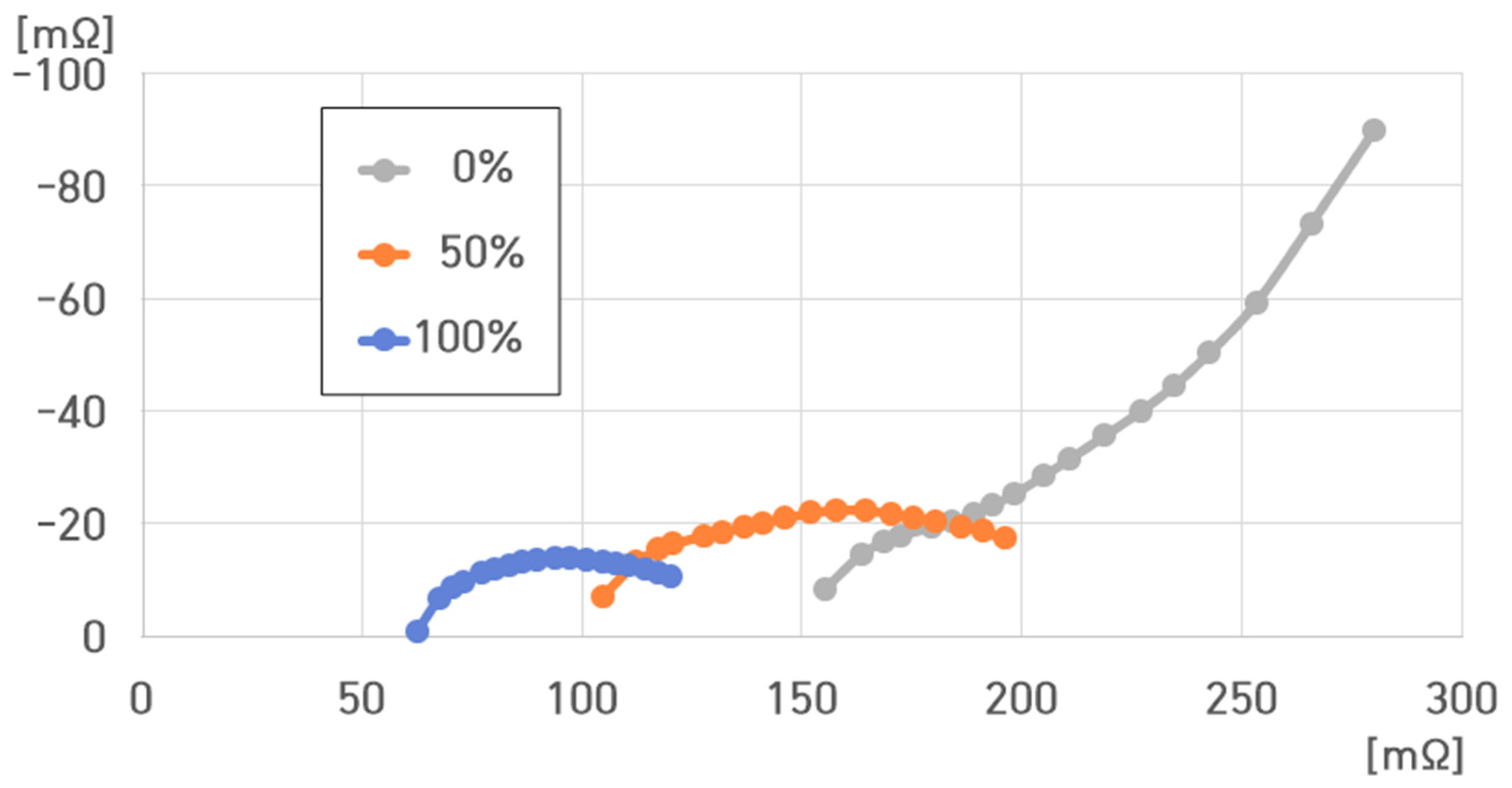
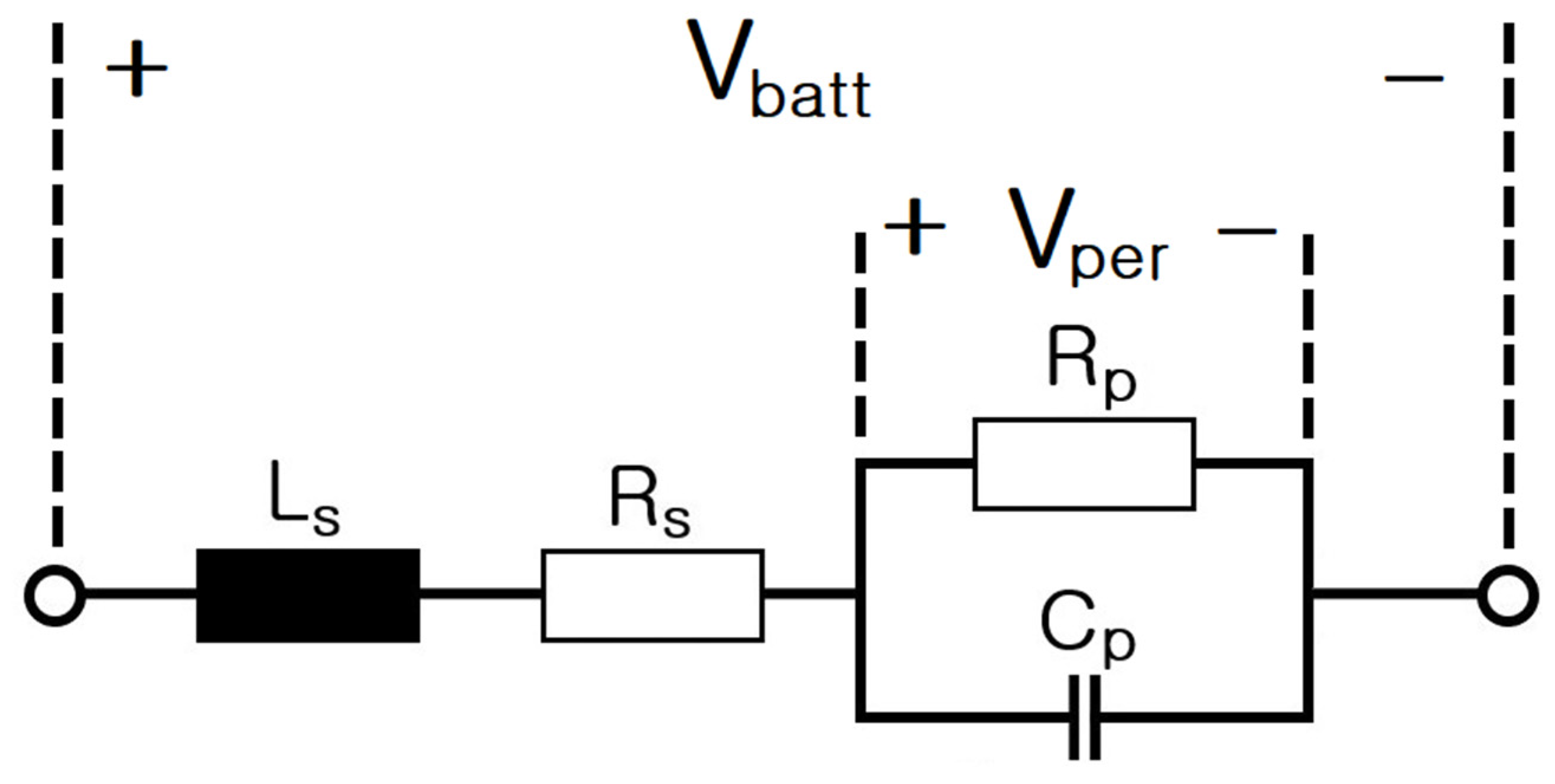
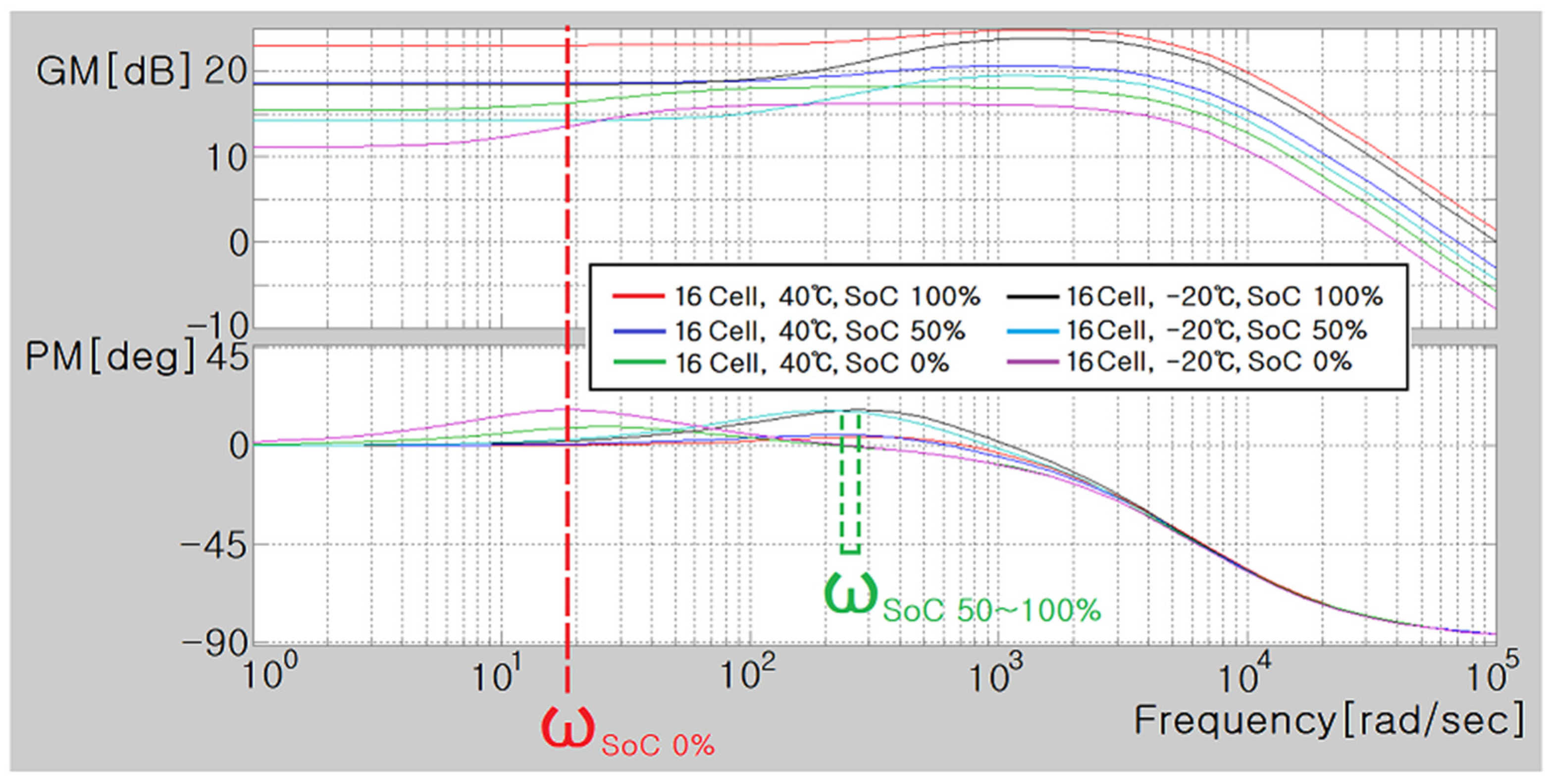
6. Modeling of ESS and Analysis of Electrical Characteristics According to Frequency
- (1)
- 1 Cell, 40 °C, 100% SoC: ;
- (2)
- 1 Cell, 40 °C, 50% SoC: ;
- (3)
- 1 Cell, 40 °C, 0% SoC: ;
- (4)
- 1 Cell, −20 °C, 100% SoC: ;
- (5)
- 1 Cell, −20 °C, 50% SoC: ;
- (6)
- 1 Cell, −20 °C, 0% SoC: .
- (1)
- 16 Cell, 40 °C, 100% SoC: ;
- (2)
- 16 Cell, 40 °C, 50% SoC: ;
- (3)
- 16 Cell, 40 °C, 0% SoC: ;
- (4)
- 16 Cell, −20 °C, 100% SoC: ;
- (5)
- 16 Cell, −20 °C, 50% SoC: ;
- (6)
- 16 Cell, −20 °C, 0% SoC: .
7. Conclusions
- ∎
- 100% SoC of One Cell (75 Ah)
- (1)
- Series resistance range: ;
- (2)
- Parallel resistance range: ;
- (3)
- Parallel capacitor range: ;
- (4)
- Series inductor range: .
- ∎
- 50% SoC of One Cell (75 Ah)
- (1)
- Series resistance range: ;
- (2)
- Parallel resistance range: ;
- (3)
- Parallel capacitor range: ;
- (4)
- Series inductor range: .
- ∎
- 0% SoC of One Cell (75 Ah)
- (1)
- Series resistance range: ;
- (2)
- Parallel resistance range: ;
- (3)
- Parallel capacitor range: ;
- (4)
- Series inductor range: .
- ∎
- 100% SoC of the 16-cell 4.4 kWh ESS
- (1)
- Series resistance range: ;
- (2)
- Parallel resistance range: ;
- (3)
- Parallel capacitor range: ;
- (4)
- Series inductor range: .
- ∎
- 50% SoC of the 16-cell 4.4 kWh ESS
- (1)
- Series resistance range: ;
- (2)
- Parallel resistance range: ;
- (3)
- Parallel capacitor range: ;
- (4)
- Series inductor range: .
- ∎
- 0% SoC of the 16-cell 4.4 kWh ESS
- (1)
- Series resistance range: ;
- (2)
- Parallel resistance range: ;
- (3)
- Parallel capacitor range: ;
- (4)
- Series inductor range: .
- (1)
- Analysis of the changes in the impedance characteristics of lithium-ion cells during failures and abnormalities;
- (2)
- Applicability of impedance spectroscopy to large-capacity ESSs of tens of kWh or several MWh;
- (3)
- In impedance frequency injection, an analysis of the effective frequency rather than continuous frequencies from 1 kHz to 0.1 Hz;
- (4)
- Methods for applying impedance spectra technology for BMS and ways to maintain battery safety.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Ding, Y.L.; Cano, Z.P.; Yu, A.; Lu, J.; Chen, Z. Automotive Li-Ion Batteries: Current Status and Future Perspectives. Electrochem. Energy Rev. 2019, 2, 1–28. [Google Scholar] [CrossRef]
- Komsiyska, L.; Buchberger, T.; Diehl, S.; Ehrensberger, M.; Hanzl, C.; Hartmann, C.; Hölzle, M.; Kleiner, J.; Lewerenz, M.; Liebhart, B.; et al. Critical Review of Intelligent Battery Systems: Challenges, Implementation, and Potential for Electric Vehicles. Energies 2021, 14, 5989. [Google Scholar] [CrossRef]
- Sun, P.; Bisschop, R.; Niu, H.; Huang, X. A Review of Battery Fires in Electric Vehicles. Fire Technol. 2020, 56, 1361–1410. [Google Scholar] [CrossRef]
- Lu, L.; Han, X.; Li, J.; Hua, J.; Ouyang, M. A Review on the Key Issues for Lithium-ion Battery Management in Electric Vehicles. J. Power Sources 2013, 226, 272–288. [Google Scholar] [CrossRef]
- Kong, L.; Li, C.; Jiang, J.; Pecht, M.G. Li-Ion Battery Fire Hazards and Safety Strategies. Energies 2018, 11, 2191. [Google Scholar] [CrossRef]
- Kim, S.W.; Park, S.G.; Lee, E.J. Assessment of the Explosion Risk during Lithium-ion Battery Fires. J. Loss Pervention Process Ind. 2022, 80, 104851. [Google Scholar] [CrossRef]
- Troxler, Y.; Wu, B.; Marinescu, M.; Yufit, V.; Patel, Y.; Marquis, A.J.; Brandon, N.P.; Offer, G.J. The Effect of Thermal Gradients on the Performance of Lithium-ion Batteries. J. Power Sources 2014, 247, 1018–1025. [Google Scholar] [CrossRef]
- Larsson, F.; Andersson, P.; Blomqvist, P.; Lorén, A.; Mellander, B.E. Characteristics of Lithium-ion Batteries during Fire Tests. J. Power Sources 2014, 271, 414–420. [Google Scholar] [CrossRef]
- Arora, S.; Shen, W.; Kapoor, A. Neural Network based Computational Model for Estimation of Heat Generation in LiFePO4 Pouch Cells of Different Nominal Capacities. Comput. Chem. Eng. 2017, 101, 81–94. [Google Scholar] [CrossRef]
- Cui, X.; Zeng, J.; Zhang, H.; Yang, J.; Qiao, J.; Li, J.; Li, W. Optimization of the Lumped Parameter Thermal Model for Hard-cased Li-ion Batteries. J. Energy Storage 2020, 32, 101758. [Google Scholar] [CrossRef]
- Kleiner, J.; Komsiyska, L.; Elger, G.; Endisch, C. Thermal Modelling of a Prismatic Lithium-ion Cell in a Battery Electric Vehicle Environment: Influences of the Experimental Validation Setup. Energies 2020, 13, 62. [Google Scholar] [CrossRef]
- Wang, X.; Wei, X.; Chen, Q.; Zhu, J.; Dai, H. Lithium-ion Battery Temperature Online Estimation based on Fast Impedance Calculation. J. Energy Storage 2019, 26, 100952. [Google Scholar] [CrossRef]
- Peng, P.; Jiang, F. Thermal Safety of Lithium-ion Batteries with Various Cathode Materials: A Numerical Study. Int. J. Heat Mass Transf. 2016, 103, 1008–1016. [Google Scholar] [CrossRef]
- Li, J.; Sun, D.; Jin, X.; Shi, W.; Sun, C. Lithium-ion Battery Overcharging Thermal Characteristics Analysis and an Impedance-Based Electro-Thermal Coupled Model Simulation. Appl. Energy 2019, 254, 113574. [Google Scholar] [CrossRef]
- Belov, D.; Yang, M.H. Failure Mechanism of Li-ion Battery at Overcharge Conditions. J. Solid State Electrochem. 2007, 12, 885–894. [Google Scholar] [CrossRef]
- Chan, H.L.; Sutanto, D. A New Battery Model for use with Battery Energy Storage Systems and Electric Vehicles Power Systems. In Proceedings of the IEEE Power Engineering Society Conference, Singapore, 23–27 January 2000; pp. 470–475. [Google Scholar] [CrossRef]
- Schmid, M.; Vögele, U.; Endisch, C. A Novel Matrix-vector-based Framework for Modeling and Simulation of Electric Vehicle Battery Packs. J. Energy Storage 2020, 32, 101736. [Google Scholar] [CrossRef]
- Chen, M.; Rincón-Mora, G.A. Accurate Electrical Battery Model Capable of Predicting Runtime and I–V Performance. IEEE Trans. Energy Convers. 2006, 21, 504–511. [Google Scholar] [CrossRef]
- Bugryniec, P.J.; Davidson, J.N.; Brown, S.F. Advanced Abuse Modelling of Li-ion Cells—A Novel Description of Cell Pressurisation and Simmering Reactions. J. Power Sources 2020, 474, 228396. [Google Scholar] [CrossRef]
- Xiong, R.; Sun, F.; Chen, Z.; He, H. A Data-driven Multi-scale Extended Kalman Filtering Based Parameter and State Estimation Approach of Lithium-ion Polymer Battery in Electric Vehicles. Appl. Energy 2014, 113, 463–476. [Google Scholar] [CrossRef]
- Vergori, E.; Mocera, F.; Somà, A. Battery Modelling and Simulation Using a Programmable Testing Equipment. Computers 2018, 7, 20. [Google Scholar] [CrossRef]
- Ziyad, M.S.; Margaret, A.C.; William, A.L. A Mathematical Model for Lead-Acid Batteries. IEEE Trans. Energy Convers. 1992, 7, 93–98. [Google Scholar] [CrossRef]
- Margaret, A.C.; Ziyad, M.S. Determination of Lead-Acid Battery Capacity via Mathematical Modeling Techniques. IEEE Trans. Energy Convers. 1992, 7, 442–446. [Google Scholar] [CrossRef]
- Mahon, P.J.; Paul, G.L.; Keshishian, S.M.; Vassallo, A.M. Measurement and Modelling of the High-power performance of Carbon-based Supercapacitors. J. Power Sources 2000, 91, 68–76. [Google Scholar] [CrossRef]
- Gauchia, L.; Castaño, S.; Sanz, J. New Approach to Supercapacitor Testing and Dynamic Modelling. In Proceedings of the IEEE Vehicle Power and Propulsion Conference, Lille, France, 1–3 September 2010; pp. 1–5. [Google Scholar] [CrossRef]
- Buller, S.; Karden, E.; Kok, D.; Doncker, R.W.D. Modeling the Dynamic Behavior of Supercapacitors Using Impedance Spectroscopy. IEEE Trans. Ind. App. 2002, 38, 1622–1626. [Google Scholar] [CrossRef]
- Buller, S.; Thele, M.; Doncker, R.W.D.; Karden, E. Impedance-Based Simulation Models of Supercapacitors and Li-Ion Batteries for Power Electronic Applications. IEEE Trans. Ind. App. 2005, 41, 742–747. [Google Scholar] [CrossRef]
- Bae, J.Y. Electrical Modeling and Impedance Spectra of Lithium-Ion Batteries and Supercapacitors. Batteries 2023, 9, 160. [Google Scholar] [CrossRef]
- Karden, E.; Buller, S.; Doncker, R.W.D. A Frequency-domain Approach to Dynamical Modeling of Electrochemical Power Sources. Electrochim. Acta 2002, 47, 2347–2356. [Google Scholar] [CrossRef]
- Stroe, D.I.; Swierczynski, M.; Stroe, A.I.; Knap, V.; Teodorescu, R.; Andreasen, S.J. Evaluation of Different Methods for Measuring the Impedance of Lithium-Ion Batteries during Ageing. In Proceedings of the International Conference on Ecological Vehicles and Renewable Energies (EVER), Monte Carlo, Monaco, 31 March–2 April 2015. [Google Scholar] [CrossRef]
- Varnosfaderani, M.A.; Strickland, D. Online Impedance Spectroscopy Estimation of a Battery. In Proceedings of the International Conference on European Conference on Power Electronics and Applications (ECCE Europe), Karlsruhe, Germany, 5–9 September 2016; pp. 1–10. [Google Scholar] [CrossRef]
- Stroe, D.I.; Swierczynski, M.; Stan, A.I.; Knap, V.; Teodorescu, R.; Andreasen, S.J. Diagnosis of Lithium-Ion Batteries State-of-Health based on Electrochemical Impedance Spectroscopy Technique. In Proceedings of the IEEE Transactions on Energy Conversion Congress and Exposition (ECCE), Pittsburgh, PA, USA, 14–18 September 2014; pp. 4576–4582. [Google Scholar] [CrossRef]
- Maheshwari, A.; Heck, M.; Santarelli, M. Cycle Aging Studies of Lithium Nickel Manganese Cobalt Oxide-based Batteries Using Electrochemical Impedance Spectroscopy. Electrochim. Acta 2018, 273, 335–348. [Google Scholar] [CrossRef]
- Buller, S.; Thele, M.; Karden, E.; Doncker, R.W.D. Impedance-based Non-linear Dynamic Battery Modeling for Automotive Applications. J. Power Sources 2003, 113, 422–430. [Google Scholar] [CrossRef]
- Sihvo, J.; Stroe, D.I.; Messo, T.; Roinila, T. A Fast Approach for Battery Impedance Identification Using Pseudo Random Sequence (PRS) Signals. IEEE Trans. Power Electron. 2020, 35, 2548–2557. [Google Scholar] [CrossRef]
- Macdonald, J.R. Impedance Spectroscopy. Ann. Biomed. Eng. 1992, 20, 289–305. [Google Scholar] [CrossRef] [PubMed]
- Santoni, F.; Angelis, A.D.; Moschitta, A.; Carbone, P. Digital Impedance Emulator for Battery Measurement System Calibration. Sensors 2021, 21, 7377. [Google Scholar] [CrossRef] [PubMed]
- Gheem, E.V.; Pintelon, R.; Vereecken, J.; Schoukens, J.; Hubin, A.; Verboven, P.; Blajiev, O. Electrochemical Impedance Spectroscopy in the Presence of Nonlinear Distortions and Non-stationary Behaviour Part I: Theory and Validation. Electrochim. Acta 2004, 49, 4753–4762. [Google Scholar] [CrossRef]
- Stroe, D.I.; Knap, V.; Swierczynski, M.; Schaltz, E. Electrochemical Impedance Spectroscopy-based Electric Circuit Modeling of Lithium-Sulfur Batteries during Discharging State. IEEE Trans. Ind. Appl. 2019, 55, 631–637. [Google Scholar] [CrossRef]
- Deng, Z.; Zhang, Z.; Lai, Y.; Liu, J.; Li, J.; Liu, Y. Electrochemical Impedance Spectroscopy Study of a Lithium/Sulfur Battery: Modeling and Analysis of Capacity Fading. J. Electrochem. Soc. 2013, 160, A553–A558. [Google Scholar] [CrossRef]
- Lee, J.H.; Choi, W.J. Novel State-of-Charge Estimation Method for Lithium Polymer Batteries Using Electrochemical Impedance Spectroscopy. J. Power Electron. 2011, 11, 237–243. [Google Scholar] [CrossRef]
- Franke-Lang, R.; Kowa, J. Analysis of Electrochemical Impedance Spectroscopy on Zinc-Air Batteries Using the Distribution of Relaxation Times. Batteries 2021, 7, 56. [Google Scholar] [CrossRef]
- Olarte, J.; Ilarduya, J.M.; Zulueta, E.; Ferret, R.; Fernández-Gámiz, U.; Lopez-Guede, J.M. A Battery Management System with EIS Monitoring of Life Expectancy for Lead–Acid Batteries. Electronics 2021, 10, 1228. [Google Scholar] [CrossRef]
- Oldenburger, M.; Bedürftig, B.; Gruhle, A.; Grimsmann, F.; Richter, E.; Findeisen, R.; Hintennach, A. Investigation of the low frequency warburg impedance of li-ion cells by frequency domain measurements. J. Energy Storage 2019, 21, 272–280. [Google Scholar] [CrossRef]
- Samuel, C.M.; Paul, G. An Impedance Model based on a Transmission Line Circuit and a Frequency Dispersion Warburg Component for the Study of EIS in Li-Ion Batteries. J. Electroanal. Chem. 2020, 871, 114305. [Google Scholar] [CrossRef]
- Barreras, J.V.; Fleischer, C.; Christensen, A.E.; Swierczynski, M.; Schaltz, E.; Andreasen, S.J.; Sauer, D.U. An Advanced HIL Simulation Battery Model for Battery Management System Testing. IEEE Trans. Ind. Appl. 2016, 52, 5086–5099. [Google Scholar] [CrossRef]
- Liebhart, B.; Komsiyska, L.; Endisch, C. Passive Impedance Spectroscopy for Monitoring Lithium-ion Battery Cells during Vehicle Operation. J. Power Sources 2020, 449, 227297. [Google Scholar] [CrossRef]
- Kim, J.H.; Kowal, J. Development of a Matlab/Simulink Model for Monitoring Cell State-of-Health and State-of-Charge via Impedance of Lithium-ion Battery Cells. Batteries 2022, 8, 8. [Google Scholar] [CrossRef]
- Guha, A.; Patra, A. Online Estimation of the Electrochemical Impedance Spectrum and Remaining Useful Life of Lithium-ion Batteries. IEEE Trans. Instrum. Meas. 2018, 67, 1836–1849. [Google Scholar] [CrossRef]
- Babaeiyazdi, I.; Rezaei-Zare, A.; Shokrzadeh, S. State of Charge Prediction of EV Li-ion Batteries Using EIS: A Machine Learning Approach. Energy 2021, 223, 120116. [Google Scholar] [CrossRef]
- Koleti, U.R.; Dinh, T.Q.; Marco, J. A New On-line Method for Lithium Plating Detection in Lithium-ion Batteries. J. Power Sources 2020, 451, 227798. [Google Scholar] [CrossRef]
- Crescentini, M.; De Angelis, A.; Ramilli, R.; De Angelis, G.; Tartagni, M.; Moschitta, A.; Traverso, P.A.; Carbone, P. Online EIS and Diagnostics on Lithium-Ion Batteries by Means of Low-Power Integrated Sensing and Parametric Modeling. IEEE Trans. Instrum. Meas. 2021, 70, 2001711. [Google Scholar] [CrossRef]
- Galeotti, M.; Cinà, L.; Giammanco, C.; Cordiner, S.; Di Carlo, A. Performance Analysis and SOH (State of Health) Evaluation of Lithium Polymer Batteries Through Electrochemical Impedance Spectroscopy. Energy 2015, 89, 678–686. [Google Scholar] [CrossRef]
- Hasan, R.; Scott, J. Impedance Measurement of Batteries Under Load. In Proceedings of the IEEE International Instrumentation and Measurement Technology Conference (I2MTC), Auckland, New Zealand, 20–23 May 2019. [Google Scholar] [CrossRef]
- Lee, J.M.; Nam, O.Y.; Cho, B.H. Li-ion Battery SOC Estimation Method based on the Reduced Order Extended Kalman Filtering. J. Power Sources 2007, 174, 9–15. [Google Scholar] [CrossRef]
- Wei, Z.; Zhao, J.; Zou, C.; Lim, T.M.; Tseng, K.J. Comparative Study of Methods for Integrated Model Identification and State of Charge Estimation of Lithium-ion Battery. J. Power Sources 2018, 402, 189–197. [Google Scholar] [CrossRef]
- Kim, J.H.; Shin, J.W.; Chun, C.Y.; Cho, B.H. Stable Configuration of a Li-Ion Series Battery Pack Based on a Screening Process for Improved Voltage/SOC Balancing. IEEE Trans. Power Electron. 2012, 27, 411–424. [Google Scholar] [CrossRef]
- Wang, Y.; Zhao, L.; Cheng, J.; Zhou, J.; Wang, S. A State of Charge Estimation Method of Lithium-Ion Battery Based on Fused Open Circuit Voltage Curve. Appl. Sci. 2020, 10, 1264. [Google Scholar] [CrossRef]
- Chiang, Y.H.; Sean, W.Y.; Ke, J.C. Online Estimation of Internal Resistance and Open-circuit Voltage of Lithium-ion Batteries in Electric Vehicles. J. Power Sources 2011, 196, 3921–3932. [Google Scholar] [CrossRef]
- Wei, Z.; Zou, C.; Leng, F.; Soong, B.H.; Tseng, K.J. Online Model Identification and State-of-Charge Estimate for Lithium-Ion Battery with a Recursive Total Least Squares-Based Observer. IEEE Trans. Ind. Electron. 2018, 65, 1336–1346. [Google Scholar] [CrossRef]
- Rivera-Barrera, J.; Muñoz-Galeano, N.; Sarmiento-Maldonado, H. SoC Estimation for Lithium-ion Batteries: Review and Future Challenges. Electronics 2017, 6, 102. [Google Scholar] [CrossRef]
- Alvi, M.J.; Zafar, A.; Nengroo, S.H.; Hussain, S.; Alvi, M.J.; Kim, H.J. Towards a Smarter Battery Management System for Electric Vehicle Applications: A Critical Review of Lithium-ion Battery State of Charge Estimation. Energies 2019, 12, 446. [Google Scholar] [CrossRef]
- Jang, J.H.; Yoo, J.Y. Impedance-based and Circuit-parameter-based Battery Models for HEV Power Systems. Int. J. Automot. Technol. 2008, 9, 615–623. [Google Scholar] [CrossRef]
- Richardson, R.R.; Ireland, P.T.; Howey, D.A. Battery Internal Temperature Estimation by Combined Impedance and Surface Temperature Measurement. J. Power Sources 2014, 265, 254–261. [Google Scholar] [CrossRef]
- Gogona, R.; Pinson, M.B.; Bazant, M.Z.; Sarma, S.E. Internal Resistance Matching for Parallel-connected Lithium-ion Cells and Impacts on Battery Pack Cycle Life. J. Power Sources 2014, 252, 8–13. [Google Scholar] [CrossRef]









Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bai, L.; Bae, J.-Y. Electrical Modeling and Characterization of Electrochemical Impedance Spectroscopy-Based Energy Storage Systems. Batteries 2024, 10, 263. https://doi.org/10.3390/batteries10080263
Bai L, Bae J-Y. Electrical Modeling and Characterization of Electrochemical Impedance Spectroscopy-Based Energy Storage Systems. Batteries. 2024; 10(8):263. https://doi.org/10.3390/batteries10080263
Chicago/Turabian StyleBai, Lei, and Jin-Yong Bae. 2024. "Electrical Modeling and Characterization of Electrochemical Impedance Spectroscopy-Based Energy Storage Systems" Batteries 10, no. 8: 263. https://doi.org/10.3390/batteries10080263
APA StyleBai, L., & Bae, J.-Y. (2024). Electrical Modeling and Characterization of Electrochemical Impedance Spectroscopy-Based Energy Storage Systems. Batteries, 10(8), 263. https://doi.org/10.3390/batteries10080263







