Numerical Modeling of a Low-Cobalt All-Solid-State Cell with Ceramic Electrolyte Using a Deformable Geometry
Abstract
1. Introduction
2. Methods
2.1. Governing Equations
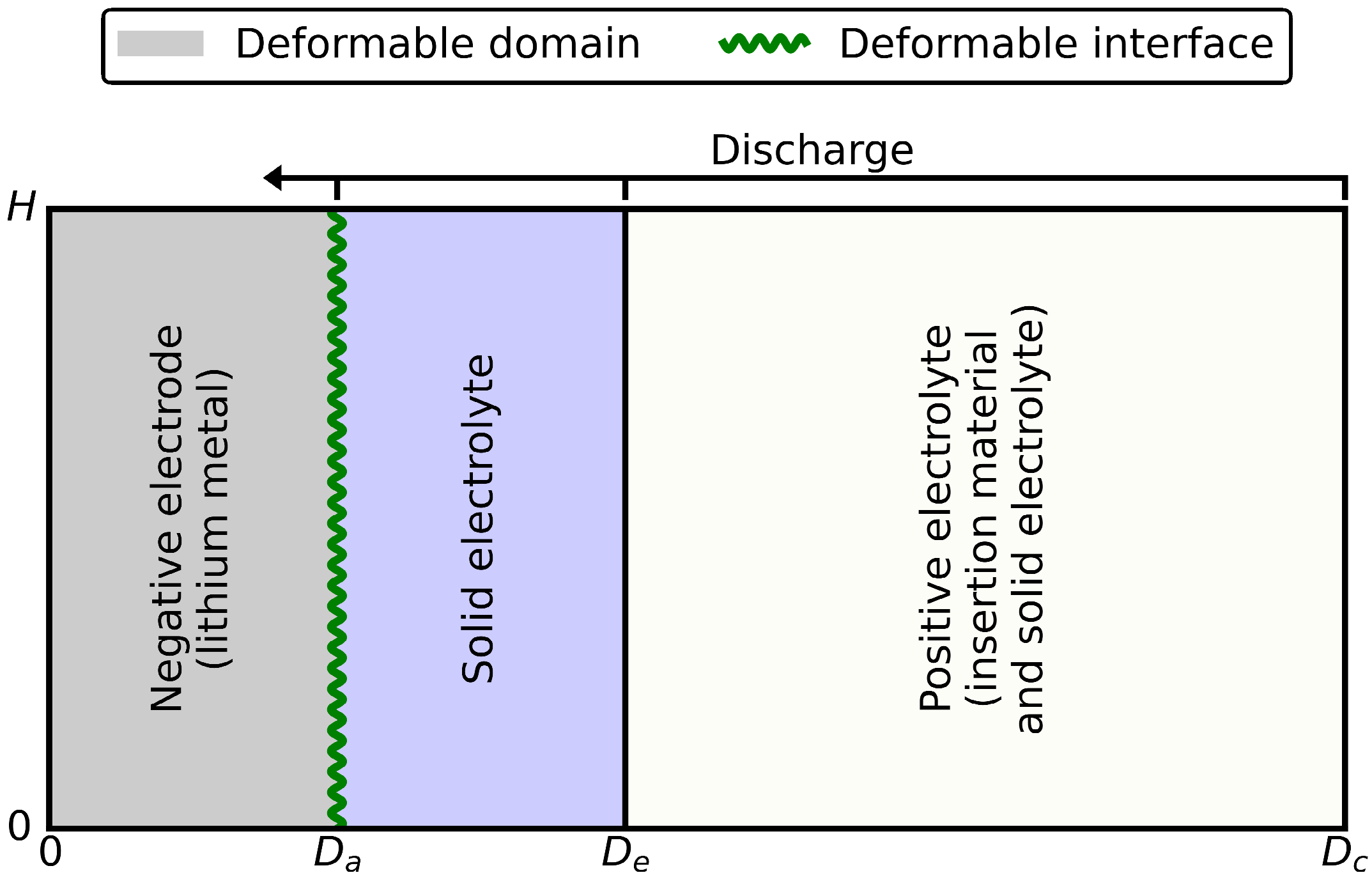
2.2. Deformation of the Negative Electrode
2.3. Polarization Decomposition and Evaluation
2.4. Simulation
3. Results and Discussion
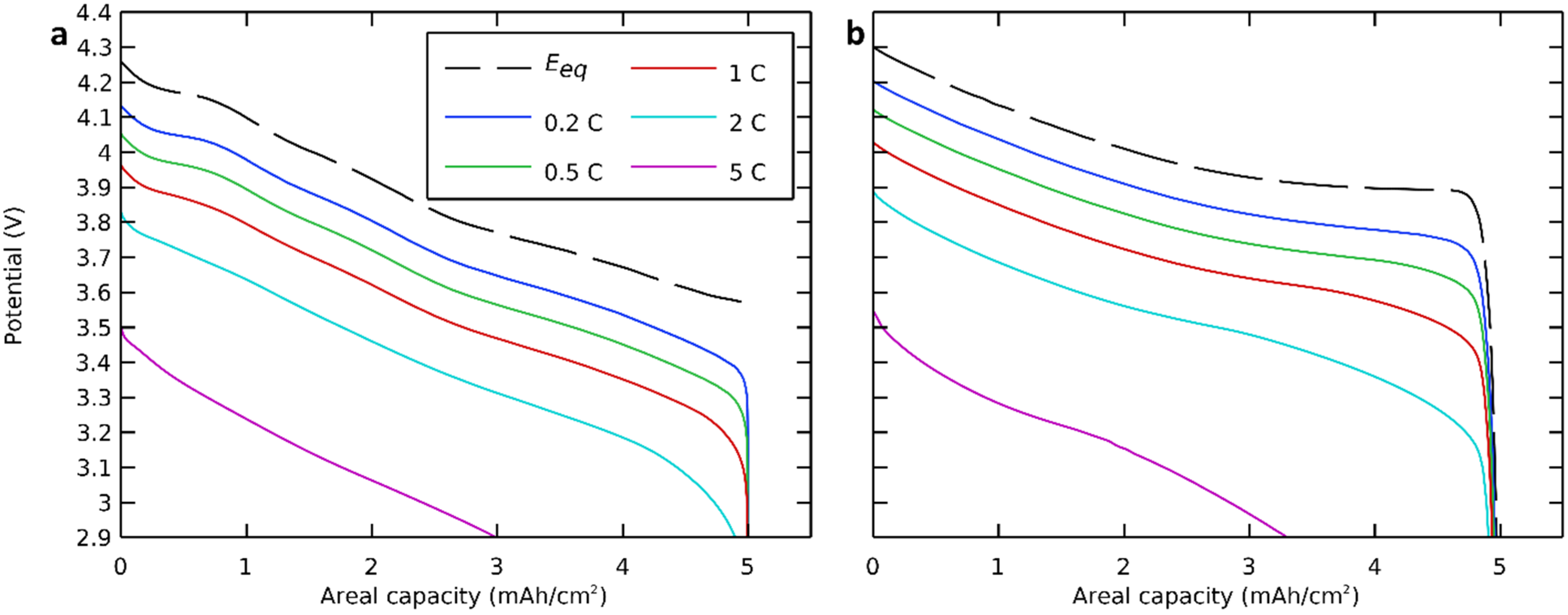
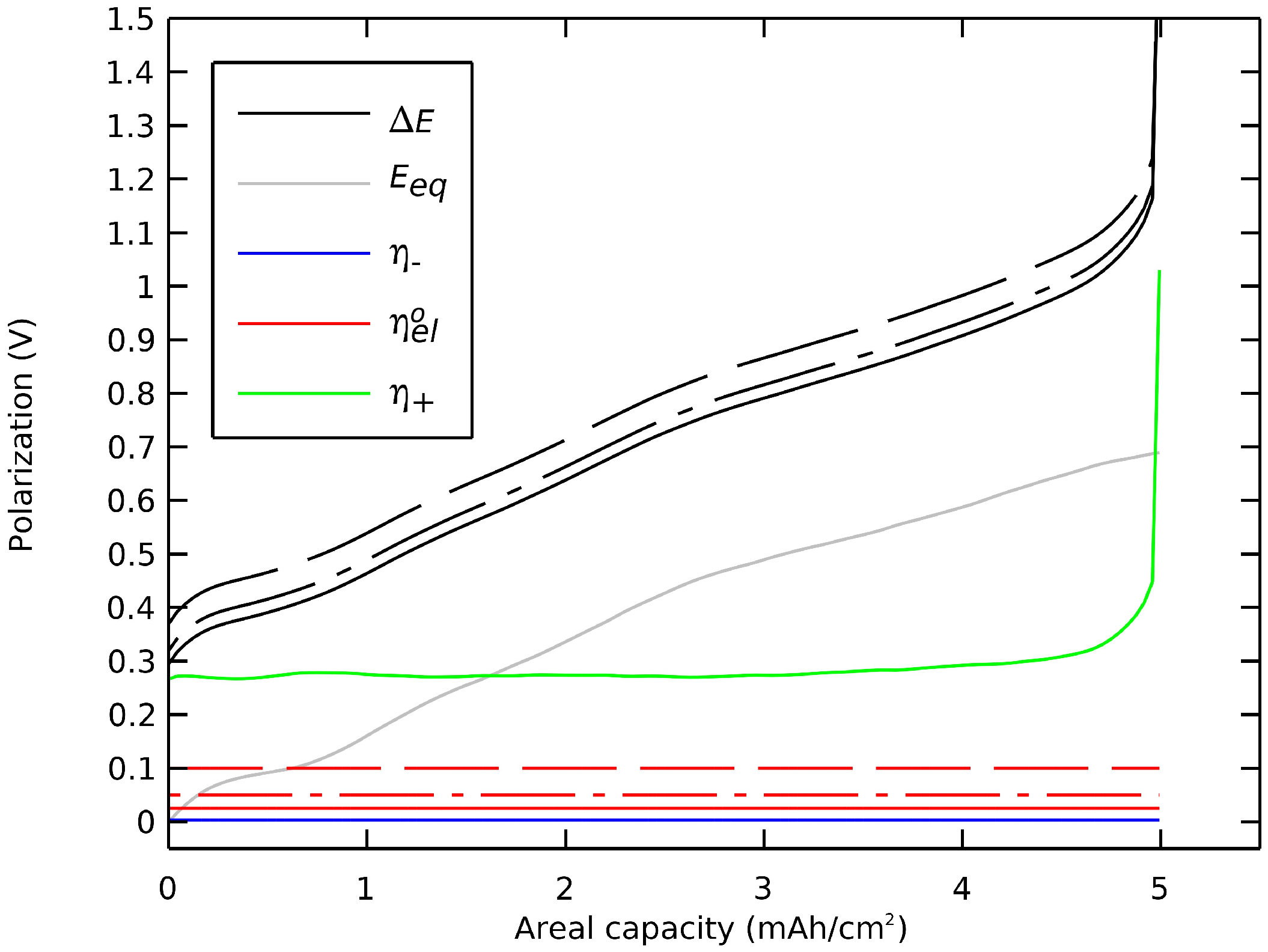
3.1. Discharge Rate
3.2. Deformation of the Negative Electrode
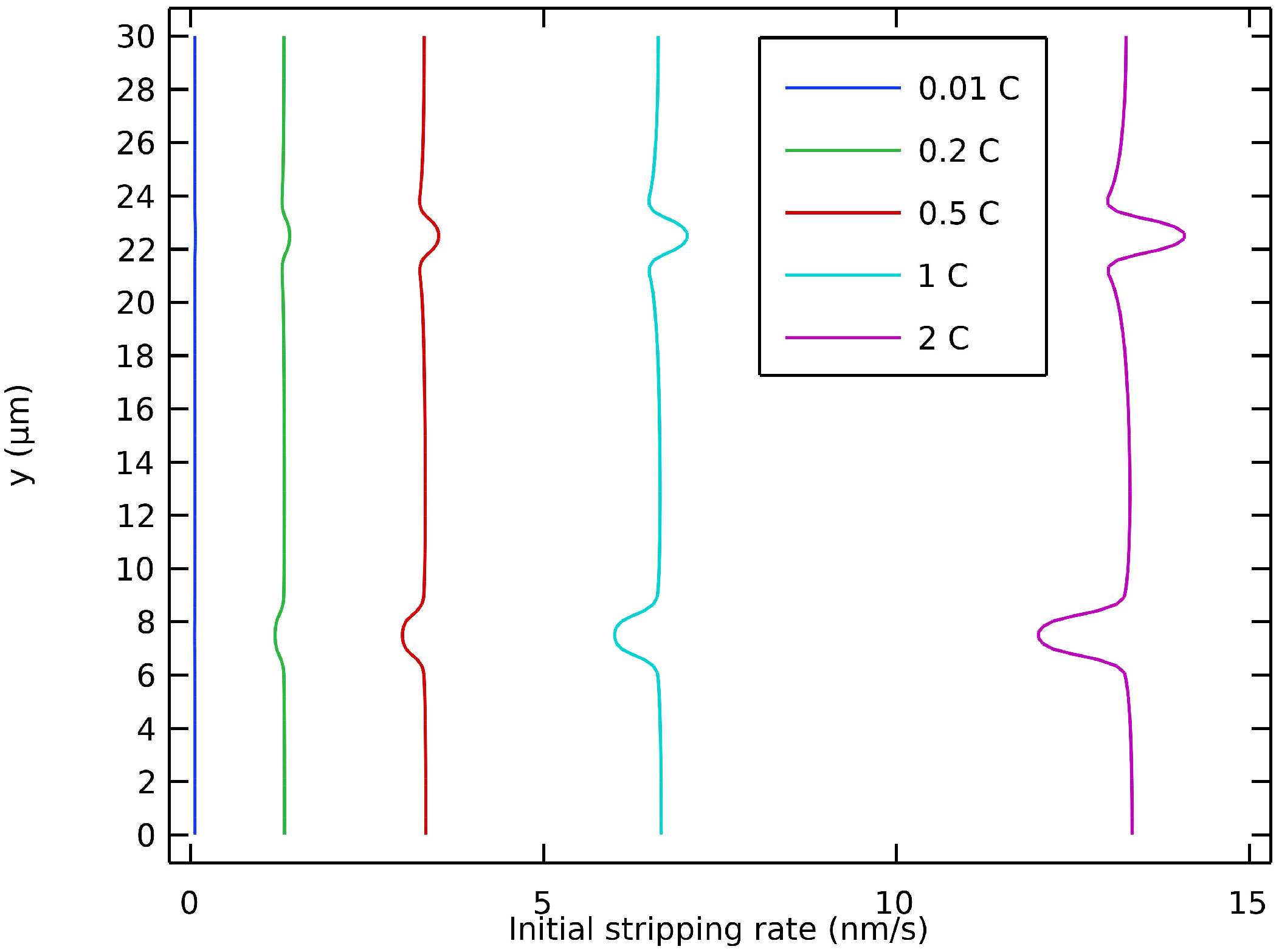
3.3. Electrostripping of Geometric Defects in the Anodic Interface
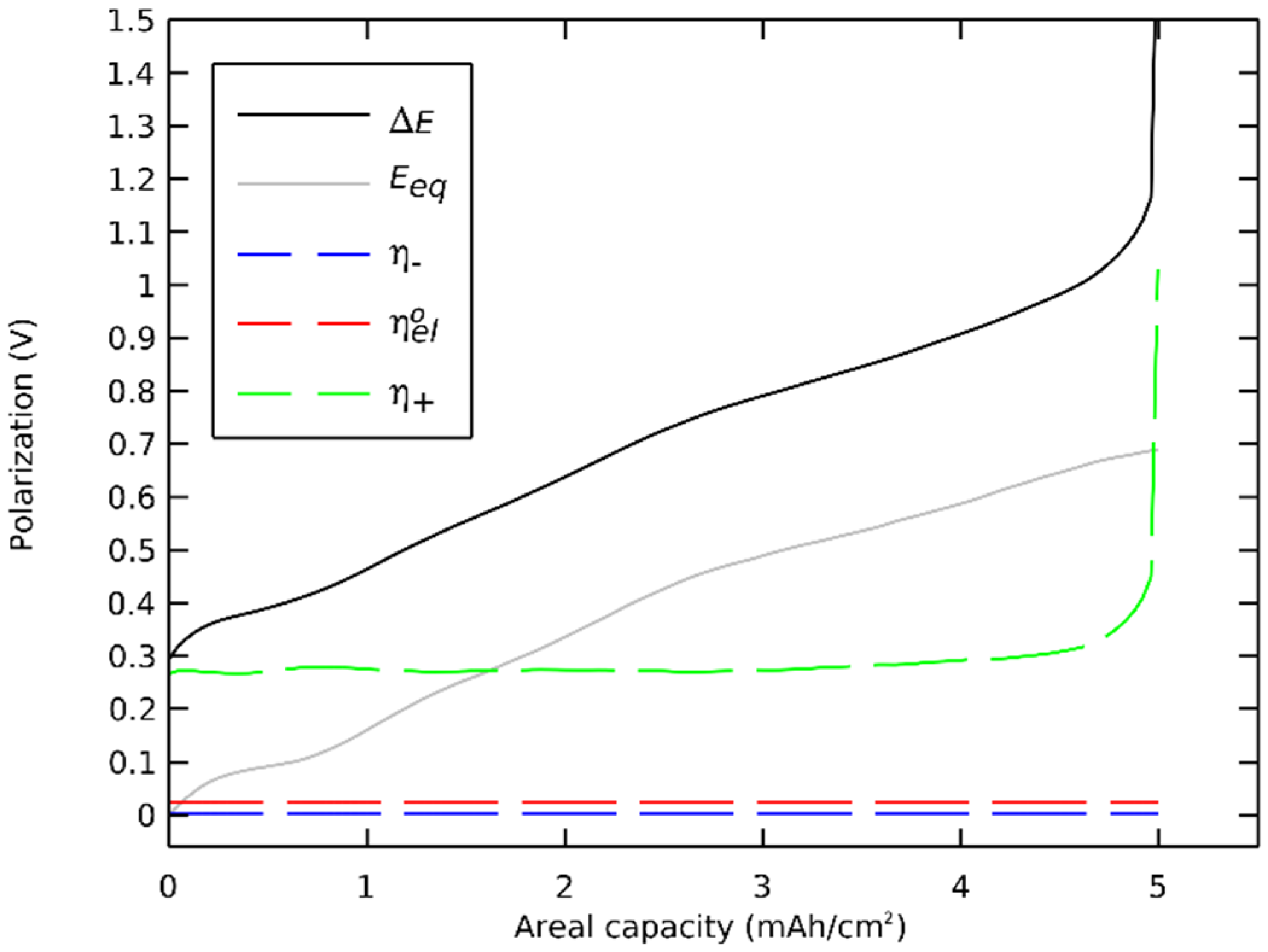
3.4. Decomposition of Polarizations
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Xu, W.; Wang, J.; Ding, F.; Chen, X.; Nasybulin, E.; Zhang, Y.; Zhang, J.G. Lithium metal anodes for rechargeable batteries. Energy Environ. Sci. 2014, 7, 513–537. [Google Scholar] [CrossRef]
- Lin, D.; Liu, Y.; Cui, Y. Reviving the lithium metal anode for high-energy batteries. Nat. Nanotechnol. 2017, 12, 194–206. [Google Scholar] [CrossRef] [PubMed]
- Manthiram, A.; Yu, X.; Wang, S. Lithium battery chemistries enabled by solid-state electrolytes. Nat. Rev. Mater. 2017, 2, 16103. [Google Scholar] [CrossRef]
- Sun, C.; Liu, J.; Gong, Y.; Wilkinson, D.P.; Zhang, J. Recent advances in all-solid-state rechargeable lithium batteries. Nano Energy 2017, 33, 363–386. [Google Scholar] [CrossRef]
- Wang, Z.; Liu, J.; Wang, M.; Shen, X.; Qian, T.; Yan, C. Toward safer solid-state lithium metal batteries: A review. Nanoscale Adv. 2020, 2, 1828–1836. [Google Scholar] [CrossRef]
- Li, C.; Wang, Z.-Y.; He, Z.-J.; Li, Y.-J.; Mao, J.; Dai, K.-H.; Yan, C.; Zheng, J.-C. An advance review of solid-state battery: Challenges, progress and prospects. Sustain. Mater. Technol. 2021, 29, e00297. [Google Scholar] [CrossRef]
- Zaman, W.; Hatzell, K.B. Processing and manufacturing of next generation lithium-based all solid-state batteries. Curr. Opin. Solid State. Mater. Sci. 2022, 26, 101003. [Google Scholar] [CrossRef]
- Wang, R.; Cui, W.; Chu, F.; Wu, F. Lithium metal anodes: Present and future. J. Energy Chem. 2020, 48, 145–159. [Google Scholar] [CrossRef]
- Abada, S.; Marlair, G.; Lecocq, A.; Petit, M.; Sauvant-Moynot, V.; Huet, F. Safety focused modeling of lithium-ion batteries: A review. J. Power Sources 2016, 306, 178–192. [Google Scholar] [CrossRef]
- Zhao, Y.; Stein, P.; Bai, Y.; Al-Siraj, M.; Yang, Y.; Xu, B.X. A review on modeling of electro-chemo-mechanics in lithium-ion batteries. J. Power Sources 2019, 413, 259–283. [Google Scholar] [CrossRef]
- Bistri, D.; Afshar, A.; Di Leo, C.V. Modeling the chemo-mechanical behavior of all-solid-state batteries: A review. Meccanica 2021, 56, 1523–1554. [Google Scholar] [CrossRef]
- Doyle, M.; Fuller, T.F.; Newman, J. Modeling of galvanostatic charge and discharge of the lithium/polymer/insertion cell. J. Electrochem. Soc. 1993, 140, 1526. [Google Scholar] [CrossRef]
- Fuller, T.F.; Doyle, M.; Newman, J. Simulation and optimization of the dual lithium ion insertion cell. J. Electrochem. Soc. 1994, 141, 1. [Google Scholar] [CrossRef]
- Newman, J.; Tiedemann, W. Porous-electrode theory with battery applications. AIChE J. 1975, 21, 25–41. [Google Scholar] [CrossRef]
- Danilov, D.L.; Niessen, R.A.H.; Notten, P.H.L. Modeling all-solid-state Li-ion batteries. J. Electrochem. Soc. 2010, 158, A215. [Google Scholar] [CrossRef]
- Chen, Z.; Danilov, D.L.; Raijmakers, L.H.; Chayambuka, K.; Jiang, M.; Zhou, L.; Zhou, J.; Eichel, R.-A.; Notten, P.H. Overpotential analysis of graphite-based Li-ion batteries seen from a porous electrode modeling perspective. J. Power Sources 2021, 509, 230345. [Google Scholar] [CrossRef]
- Wang, Z.; Danilov, D.L.; Eichel, R.A.; Notten, P.H. Modeling the Resistance of Thin-Film Current Collectors in Thin-Film Batteries. J. Electrochem. Soc. 2022, 170, 020514. [Google Scholar] [CrossRef]
- Xiong, R.; Yu, Y.; Chen, S.; Li, M.; Li, L.; Zhou, M.; Zhang, W.; Yan, B.; Li, D.; Yang, H.; et al. Overpotential decomposition enabled decoupling of complex kinetic processes in battery electrodes. J. Power Sources 2023, 553, 232296. [Google Scholar] [CrossRef]
- Fabre, S.D.; Guy-Bouyssou, D.; Bouillon, P.; Le Cras, F.; Delacourt, C. Charge/discharge simulation of an all-solid-state thin-film battery using a one-dimensional model. J. Electrochem. Soc. 2011, 159, A104. [Google Scholar] [CrossRef]
- N’Dri, N.; Megahed, M.; Fabre, S.D. Thermo-Mechanical Simulation of Solid Microbatteries. ECS Trans. 2012, 40, 205. [Google Scholar] [CrossRef]
- Nesro, M.S.; Elfadel, I.M. Simplified multiphysics model for all-solid-state microbatteries. In Proceedings of the 2013 COMSOL Conference in Rotterdam, Rotterdam, The Netherlands, 24–28 October 2013. [Google Scholar]
- Ferrese, A.; Newman, J. Modeling lithium movement over multiple cycles in a lithium-metal battery. J. Electrochem. Soc. 2014, 161, A948. [Google Scholar] [CrossRef]
- Vyroubal, P.; Maxa, J.; Kazda, T.; Vondrák, J. Computational modeling of lithium ion battery. ECS Trans. 2014, 63, 99. [Google Scholar] [CrossRef]
- Vyroubal, P.; Maxa, J.; Kazda, T.; Vondrák, J. The Finite Element Method in Electrochemistry–Modelling of the Lithium-Ion Battery. ECS Trans. 2014, 48, 289. [Google Scholar] [CrossRef]
- Bates, A.; Mukherjee, S.; Schuppert, N.; Son, B.; Kim, J.G.; Park, S. Modeling and simulation of 2D lithium-ion solid state battery. Int. J. Energy Res. 2015, 39, 1505–1518. [Google Scholar] [CrossRef]
- Vyroubal, P.; Kazda, T.; Maxa, J.; Vondrák, J.; Sedlaříková, M.; Tichý, J.; Cipín, R. 3D Modelling and study of electrochemical characteristics and thermal stability of commercial accumulator by simulation methods. Int. J. Electrochem. Sci. 2016, 11, 1938–1950. [Google Scholar] [CrossRef]
- Mendoza, H.; Roberts, S.A.; Brunini, V.E.; Grillet, A.M. Mechanical and electrochemical response of a LiCoO2 cathode using reconstructed microstructures. Electrochim. Acta 2016, 190, 1–15. [Google Scholar] [CrossRef]
- Taleghani, S.T.; Marcos, B.; Zaghib, K.; Lantagne, G. A study on the effect of porosity and particles size distribution on Li-ion battery performance. J. Electrochem. Soc. 2017, 164, E3179. [Google Scholar] [CrossRef]
- Kashkooli, A.G.; Amirfazli, A.; Farhad, S.; Lee, D.U.; Felicelli, S.; Park, H.W.; Feng, K.; De Andrade, V.; Chen, Z. Representative volume element model of lithium-ion battery electrodes based on X-ray nano-tomography. J. Appl. Electrochem. 2017, 47, 281–293. [Google Scholar] [CrossRef]
- Taleghani, S.T.; Marcos, B.; Lantagne, G. Modeling and simulation of a commercial graphite–LiFePO4 cell in a full range of C-rates. J. Appl. Electrochem. 2018, 48, 1389–1400. [Google Scholar] [CrossRef]
- Wolff, N.; Röder, F.; Krewer, U. Model based assessment of performance of lithium-ion batteries using single ion conducting electrolytes. Electrochim. Acta 2018, 284, 639–646. [Google Scholar] [CrossRef]
- Kazemi, N.; Danilov, D.L.; Haverkate, L.; Dudney, N.J.; Unnikrishnan, S.; Notten, P.H. Modeling of all-solid-state thin-film Li-ion batteries: Accuracy improvement. Solid State Ion. 2019, 334, 111–116. [Google Scholar] [CrossRef]
- Xu, S.; Chen, K.H.; Dasgupta, N.P.; Siegel, J.B.; Stefanopoulou, A.G. Evolution of dead lithium growth in lithium metal batteries: Experimentally validated model of the apparent capacity loss. J. Electrochem. Soc. 2019, 166, A3456. [Google Scholar] [CrossRef]
- Raijmakers, L.H.J.; Danilov, D.L.; Eichel, R.A.; Notten, P.H.L. An advanced all-solid-state Li-ion battery model. Electrochim. Acta 2020, 330, 135147. [Google Scholar] [CrossRef]
- Kim, J.; Mohanty, S.K.; Yoo, H.D. Modeling ionic intercalation and solid-state diffusion using typical descriptors of batteries. J. Appl. Electrochem. 2021, 51, 703–713. [Google Scholar] [CrossRef]
- Yue, F.; Xia, Q.; Gong, Y.; Wang, M.; Xia, H.; Huang, X. A fully coupled electrochemical-mechanical-thermal model of all-solid-state thin-film Li-ion batteries. J. Power Sources 2022, 539, 231614. [Google Scholar] [CrossRef]
- Li, W.; Bao, Z.; Wang, J.; Du, Q.; Jiao, K. Comparative simulation of thin-film and bulk-type all-solid-state batteries under adiabatic and isothermal conditions. Appl. Therm. Eng. 2023, 223, 119957. [Google Scholar] [CrossRef]
- Quarti, M.; Bayer, A.; Bessler, W.G. Trade-off between energy density and fast-charge capability of lithium-ion batteries: A model-based design study of cells with thick electrodes. Electrochem. Sci. Adv. 2023, 3, e2100161. [Google Scholar] [CrossRef]
- Murugan, R.; Thangadurai, V.; Weppner, W. Fast lithium ion conduction in garnet-type Li7La3Zr2O12. Angew. Chem. Int. Ed. 2007, 46, 7778–7781. [Google Scholar] [CrossRef] [PubMed]
- Ohta, S.; Kobayashi, T.; Asaoka, T. High lithium ionic conductivity in the garnet-type oxide Li7−XLa3(Zr2−X, NbX)O12 (X = 0–2). J. Power Sources 2011, 196, 3342–3345. [Google Scholar] [CrossRef]
- Buschmann, H.; Dölle, J.; Berendts, S.; Kuhn, A.; Bottke, P.; Wilkening, M.; Heitjans, P.; Senyshyn, A.; Ehrenberg, H.; Lotnyk, A.; et al. Structure and dynamics of the fast lithium ion conductor “Li7La3Zr2O12”. Phys. Chem. Chem. Phys. 2011, 13, 19378–19392. [Google Scholar] [CrossRef]
- Buschmann, H.; Berendts, S.; Mogwitz, B.; Janek, J. Lithium metal electrode kinetics and ionic conductivity of the solid lithium ion conductors “Li7La3Zr2O12” and Li7−xLa3Zr2−xTaxO12 with garnet-type structure. J. Power Sources 2012, 206, 236–244. [Google Scholar] [CrossRef]
- Rangasamy, E.; Wolfenstine, J.; Sakamoto, J. The role of Al and Li concentration on the formation of cubic garnet solid electrolyte of nominal composition Li7La3Zr2O12. Solid State Ion. 2012, 206, 28–32. [Google Scholar] [CrossRef]
- Thangadurai, V.; Narayanan, S.; Pinzaru, D. Garnet-type solid-state fast Li ion conductors for Li batteries: Critical review. Chem. Soc. Rev. 2014, 43, 4714–4727. [Google Scholar] [CrossRef] [PubMed]
- Thangadurai, V.; Pinzaru, D.; Narayanan, S.; Baral, A.K. Fast solid-state Li ion conducting garnet-type structure metal oxides for energy storage. J. Phys. Chem. Lett. 2015, 6, 292–299. [Google Scholar] [CrossRef] [PubMed]
- Samson, A.J.; Hofstetter, K.; Bag, S.; Thangadurai, V. A bird’s-eye view of Li-stuffed garnet-type Li7La3Zr2O12 ceramic electrolytes for advanced all-solid-state Li batteries. Energy Environ. Sci. 2019, 12, 2957–2975. [Google Scholar] [CrossRef]
- Sun, H.; Kang, S.; Cui, L. Prospects of LLZO type solid electrolyte: From material design to battery application. Chem. Eng. J. 2023, 454, 140375. [Google Scholar] [CrossRef]
- Horii, M.; Christianson, R.J.; Mutha, H.; Bachman, J.C. Modeling the effect of electrolyte microstructure on conductivity and solid-state Li-ion battery performance. J. Power Sources 2022, 528, 231177. [Google Scholar] [CrossRef]
- Kravchyk, K.V.; Karabay, D.T.; Kovalenko, M.V. On the feasibility of all-solid-state batteries with LLZO as a single electrolyte. Sci. Rep. 2022, 12, 1177. [Google Scholar] [CrossRef]
- Kuganathan, N.; Rushton, M.J.; Grimes, R.W.; Kilner, J.A.; Gkanas, E.I.; Chroneos, A. Self-diffusion in garnet-type Li7La3Zr2O12 solid electrolytes. Sci. Rep. 2021, 11, 451. [Google Scholar] [CrossRef]
- COMSOL Multiphysics® v. 6.1; COMSOL AB: Stockholm, Sweden, 2022; Available online: http://www.comsol.com (accessed on 16 August 2023).
- Wang, A.A.; O’kane, S.E.J.; Planella, F.B.; Le Houx, J.; O’Regan, K.; Zyskin, M.; Edge, J.S.; Monroe, C.; Cooper, S.; Howey, D.A.; et al. of parameterisation and a novel database (LiionDB) for continuum Li-ion battery models. Prog. Energy 2022, 4, 032004. [Google Scholar] [CrossRef]
- Swiderska-Mocek, A.; Lewandowski, A. Kinetics of Li-ion transfer reaction at LiMn2O4, LiCoO2, and LiFePO4 cathodes. J. Solid State Electrochem. 2017, 21, 1365–1372. [Google Scholar] [CrossRef]
- Chen, C.H.; Planella, F.B.; O’regan, K.; Gastol, D.; Widanage, W.D.; Kendrick, E. Development of experimental techniques for parameterization of multi-scale lithium-ion battery models. J. Electrochem. Soc. 2020, 167, 080534. [Google Scholar] [CrossRef]
- Golozar, M.; Paolella, A.; Demers, H.; Bessette, S.; Lagacé, M.; Bouchard, P.; Guerfi, A.; Gauvin, R.; Zaghib, K. In situ observation of solid electrolyte interphase evolution in a lithium metal battery. Commun. Chem 2019, 2, 131. [Google Scholar] [CrossRef]
- Tang, M.; Albertus, P.; Newman, J. Two-dimensional modeling of lithium deposition during cell charging. J. Electrochem. Soc. 2009, 156, A390. [Google Scholar] [CrossRef]
- Sturm, J.; Rheinfeld, A.; Zilberman, I.; Spingler, F.B.; Kosch, S.; Frie, F.; Jossen, A. Modeling and simulation of inhomogeneities in a 18650 nickel-rich, silicon-graphite lithium-ion cell during fast charging. J. Power Sources 2019, 412, 204–223. [Google Scholar] [CrossRef]
- Vishnugopi, B.S.; Naik, K.G.; Kawakami, H.; Ikeda, N.; Mizuno, Y.; Iwamura, R.; Kotaka, T.; Aotani, K.; Tabuchi, Y.; Mukherjee, P.P. Asymmetric contact loss dynamics during plating and stripping in solid-state batteries. Adv. Energy Mater. 2023, 13, 2203671. [Google Scholar] [CrossRef]
- Carmona, E.A.; Albertus, P. Modeling how interface geometry and mechanical stress affect Li metal/solid electrolyte current distributions. J. Electrochem. Soc. 2023, 170, 020524. [Google Scholar] [CrossRef]




| Symbol | Definition | Unit |
|---|---|---|
| Lithium concentration | mol m−3 | |
| Lithium concentration averaged on electrode length | mol m−3 | |
| Lithium concentration averaged on particles radius | mol m−3 | |
| Minimal lithium concentration in the active material of the positive electrode | mol m−3 | |
| Maximal lithium concentration in the active material of the positive electrode | mol m−3 | |
| Reference lithium concentration in the active material of the positive electrode | mol m−3 | |
| Diffusion coefficient of lithium in the active material of the positive electrode | m2 s−1 | |
| Cell potential | V | |
| Equilibrium cell potential | V | |
| Faraday constant | C per equivalent | |
| Current density | A m−2 | |
| Current density at the negative electrode interface | A m−2 | |
| Current density at the positive electrode interface | A m−2 | |
| Exchange current density at the negative electrode | A m−2 | |
| Exchange current density at the positive electrode | A m−2 | |
| Reference exchange current density at the positive electrode | A m−2 | |
| Current density in the electrolyte | A m−2 | |
| Local current density | A m−2 | |
| Current density in the negative electrode | A m−2 | |
| Current density in the active material of the positive electrode | A m−2 | |
| Thickness of the negative electrode | m | |
| Thickness of the electrolyte between the two electrodes | m | |
| Thickness of the positive electrode | m | |
| Molar mass of lithium | kg mol−1 | |
| Number of electrons transferred | Equivalent | |
| Molar flux of lithium | mol m−2 s−1 | |
| Areal capacity | mAh cm−2 | |
| Areal capacity calculated from the lithium stripped from the negative electrode | mAh cm−2 | |
| Areal capacity calculated from the lithium inserted into the positive electrode | mAh cm−2 | |
| Radius | m | |
| Particle radius of the active material | m | |
| Ideal gas constant | J mol−1 K−1 | |
| Minimal state of charge of the active material | Unitless | |
| Maximal state of charge of the active material | Unitless | |
| Time | s | |
| Temperature | K | |
| Lithium stripping rate at the anodic interface | m s−1 | |
| Anodic transfer coefficient | Unitless | |
| Cathodic transfer coefficient | Unitless | |
| Volume fraction of solid electrolyte in the positive electrode | Unitless | |
| Volume fraction of active material in the positive electrode | Unitless | |
| Polarization | V | |
| Stoichiometric coefficient | Unitless | |
| Density of lithium | kg m−3 | |
| Ionic conductivity of the solid electrolyte | S m−1 | |
| Effective ionic conductivity of the solid electrolyte | S m−1 | |
| Electronic conductivity of the negative electrode | S m−1 | |
| Electronic conductivity of the active material of the positive electrode | S m−1 | |
| Effective electronic conductivity of the active material of the positive electrode | S m−1 | |
| Electrolyte potential | V | |
| Electric potential in the negative electrode | V | |
| Electric potential in the active material of the positive electrode | V |
| Main Component | Governing Equations | Comments |
|---|---|---|
| Negative electrode | Charge conservation | |
| Ohm’s law | ||
| Lithium reduction–oxidation | ||
| Butler–Volmer equation | ||
| Single-ion electrolyte | Charge conservation | |
| Ohm’s law | ||
| Positive electrode | Charge conservation | |
| Ohm’s law | ||
| Bruggeman relationship | ||
| Charge conservation | ||
| Ohm’s law | ||
| Bruggeman relationship | ||
| Mass conservation | ||
| Fick’s first law of diffusion | ||
| Centrale symmetry condition | ||
| Diffusion–insertion flux equality | ||
| Lithium insertion in oxide (II) | ||
| Butler–Volmer equation | ||
| Adjustment of exchangecurrent density |
| Parameter | Definition | Value |
|---|---|---|
| Reference lithium concentration in LCO | 56,250 mol m−3 | |
| Reference lithium concentration in NMC811 | 50,060 mol m−3 | |
| Diffusion coefficient of lithium in the active material of the positive electrode | 5 × 10−13 m2 s−1 | |
| Height of cell for the 2D simulation | 30 µm | |
| Exchange current density at the negative electrode | 400 A m−2 | |
| Exchange current density at the positive electrode with LCO | 9.4 × 10−2 A m−2 [53] | |
| Exchange current density at the negative electrode with NMC811 | 6.4 × 10−2 A m−2 [54] | |
| Thickness of the negative electrode | 34 µm [55] | |
| Thickness of the electrolyte between the two electrodes | 50 µm | |
| Thickness of the positive electrode | ||
| Molar mass of lithium | 6.94 × 10−3 kg mol−1 | |
| Theoretical areal capacity (limited by the positive electrode) | 5 mAh cm−2 | |
| Particle radius of positive active material | 6 µm | |
| Minimal state of charge of LCO | 0.43 | |
| Maximal state of charge of LCO | 1 | |
| Minimal state of charge of NMC811 | 0.222 | |
| Maximal state of charge of NMC811 | 0.942 | |
| Temperature | 25 °C | |
| Anodic transfer coefficient | 0.5 | |
| Cathodic transfer coefficient | 0.5 | |
| Volume fraction of solid electrolyte in the positive electrode | 0.3 | |
| Volume fraction of active material in the positive electrode | 0.7 | |
| Density of lithium | 534 kg m−3 | |
| Ionic conductivity of LLZO | 0.1 S m−1 | |
| Electronic conductivity of lithium | 1.0776 × 107 S m−1 | |
| Electronic conductivity of the active material of the positive electrode | 0.1 S m−1 |
| Polarization | Order of Magnitude (V) | Polarization | Order of Magnitude (V) | ||
|---|---|---|---|---|---|
| 10−1–100 | 10−2 | ||||
| 10−2–10−1 | 10−1 | ||||
| 10−1 | 10−3–10−2 | ||||
| 10−11–10−10 | 10−2 | ||||
| 10−3 | 10−4–10−3 | ||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nadeau, D.; Roué, L.; Allard, F. Numerical Modeling of a Low-Cobalt All-Solid-State Cell with Ceramic Electrolyte Using a Deformable Geometry. Batteries 2024, 10, 250. https://doi.org/10.3390/batteries10070250
Nadeau D, Roué L, Allard F. Numerical Modeling of a Low-Cobalt All-Solid-State Cell with Ceramic Electrolyte Using a Deformable Geometry. Batteries. 2024; 10(7):250. https://doi.org/10.3390/batteries10070250
Chicago/Turabian StyleNadeau, David, Lionel Roué, and François Allard. 2024. "Numerical Modeling of a Low-Cobalt All-Solid-State Cell with Ceramic Electrolyte Using a Deformable Geometry" Batteries 10, no. 7: 250. https://doi.org/10.3390/batteries10070250
APA StyleNadeau, D., Roué, L., & Allard, F. (2024). Numerical Modeling of a Low-Cobalt All-Solid-State Cell with Ceramic Electrolyte Using a Deformable Geometry. Batteries, 10(7), 250. https://doi.org/10.3390/batteries10070250







