Driving a Molecular Spin-Peierls System into a Short Range Ordered State through Chemical Substitution
Abstract
:1. Introduction
2. Materials and Methods
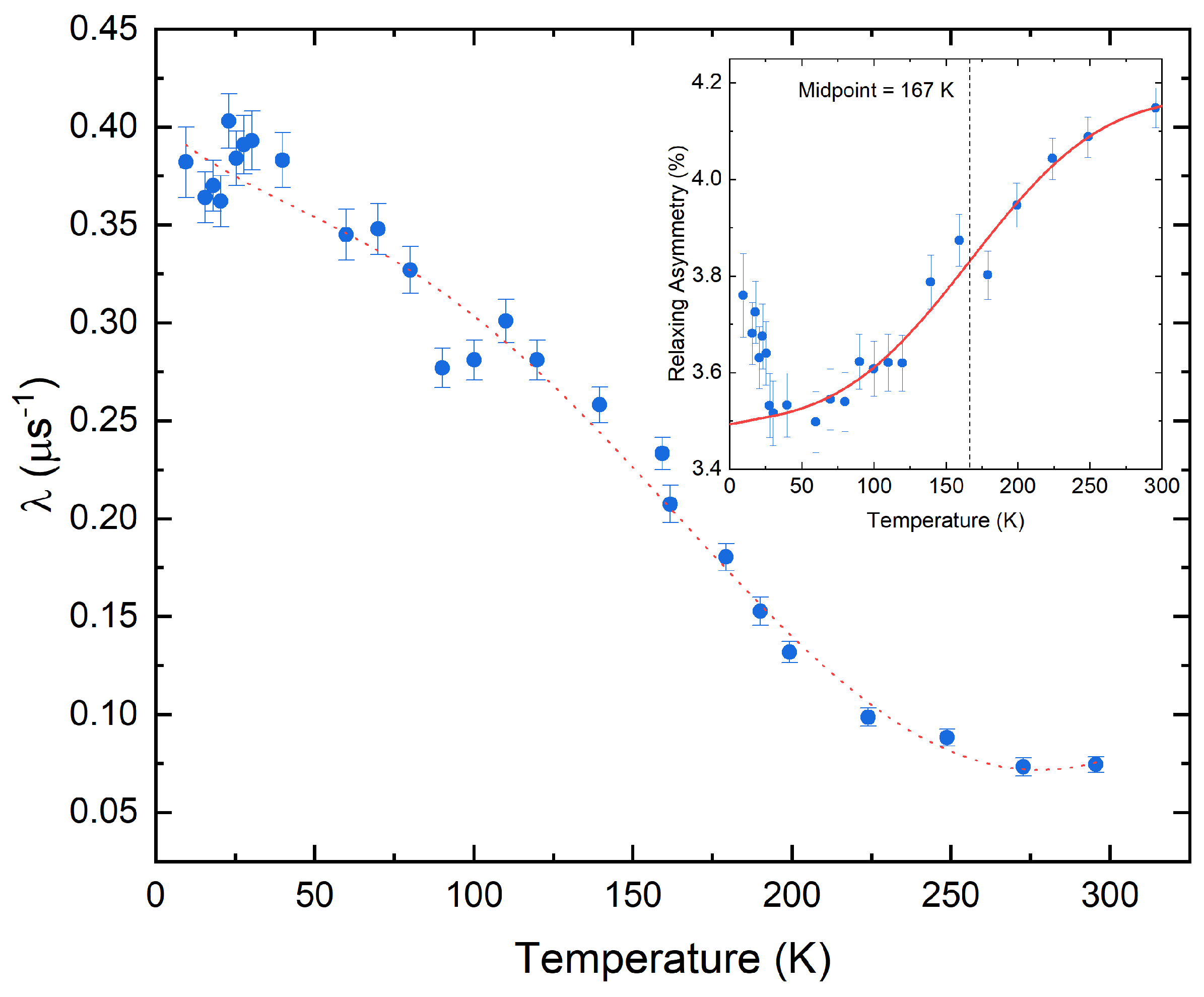
3. Results and Discussions
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wang, C.; Liu, D.; Lin, W. Metal–Organic Frameworks as A Tunable Platform for Designing Functional Molecular Materials. J. Am. Chem. Soc. 2013, 135, 13222–13234. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Coronado, E. Molecular magnetism: From chemical design to spin control in molecules, materials and devices. Nat. Rev. Mater. 2020, 5, 87–104. [Google Scholar] [CrossRef]
- Reczyński, M.; Nakabayashi, K.; Ohkoshi, S.-I. Tuning the Optical Properties of Magnetic Materials. Eur. J. Inorg. Chem. 2020, 28, 2669–2678. [Google Scholar] [CrossRef]
- McMillan, P.F. New materials from high-pressure experiments. Nat. Mater. 2002, 1, 19–25. [Google Scholar] [CrossRef] [PubMed]
- Coronado, E.; Martí-Gastaldo, C.; Navarro-Moratalla, E.; Ribera, A.; Blundell, S.J.; Baker, P.J. Coexistence of superconductivity and magnetism by chemical design. Nat. Chem. 2010, 2, 1031–1036. [Google Scholar] [CrossRef] [PubMed]
- Zhang, K.; Montigaud, V.; Cador, O.; Li, G.-P.; Guennic, B.L.; Tang, J.-K.; Wang, Y.-Y. Tuning the Magnetic Interactions in Dy(III)4 Single-Molecule Magnets. Inorg. Chem. 2018, 57, 8550–8557. [Google Scholar] [CrossRef] [Green Version]
- Yin, Z.; Zhou, Y.-L.; Zeng, M.-H.; Kurmoo, M. The concept of mixed organic ligands in metal–organic frameworks: Design, tuning and functions. Dalton Trans. 2015, 44, 5258–5275. [Google Scholar] [CrossRef]
- Xie, H.; Vignesh, K.R.; Zhang, X.; Dunbar, K.R. From spin-crossover to single molecule magnetism: Tuning magnetic properties of Co(II) bis-ferrocenylterpy cations via supramolecular interactions with organocyanide radical anion. J. Mater. Chem. C 2020, 8, 8135–8144. [Google Scholar] [CrossRef]
- Kurmoo, M. Magnetic metal–organic frameworks. Chem. Soc. Rev. 2009, 38, 1353–1379. [Google Scholar] [CrossRef]
- Vegter, J.G.; Hibma, T.; Kommandeur, J. New phase transitions in simple M-TCNQ-salts. Chem. Phys. Lett. 1969, 3, 427. [Google Scholar] [CrossRef]
- Lépine, Y.; Caillxex, A.; Larochelle, V. Potassium-tetracyanoquinodimethane (K-TCNQ): A spin-Peierls system. Phys. Rev. B 1978, 18, 3585. [Google Scholar] [CrossRef]
- Konno, M.; Ishii, T.; Saito, Y. The crystal structures of the low- and high-temperature modifications of potassium 7,7,8,8-tetracyanoquinodimethanide. Acta Cryst. 1977, B33, 7603–7770. [Google Scholar] [CrossRef]
- Tanner, D.B.; Jacobsen, C.S.; Bright, A.A.; Heeger, A.J. Infrared studies of the energy gap and electron-phonon interaction in potassium-tetracyanoquinodimethane (K-TCNQ). Phys. Rev. B 1977, 16, 3283. [Google Scholar] [CrossRef]
- Berlie, A.; Terry, I.; Szablewski, M.; Giblin, S.R. Tuneability and criticality in a three-dimensional stacked molecular system. Phys. Rev. B 2016, 93, 054422. [Google Scholar] [CrossRef] [Green Version]
- Hillier, A.D.; Blundell, S.J.; McKenzie, I.; Umegaki, I.; Shu, L.; Wright, J.A.; Prokscha, T.; Bert, F.; Shimomura, K.; Berlie, A.; et al. Muon Spin Spectroscopy. Nat. Rev. Methods Prim. 2022, 2, 4. [Google Scholar] [CrossRef]
- Blundell, S.J.; Renzi, R.D.; Lancaster, T.; Pratt, F.L. (Eds.) Muon Spectroscopy: An Introduction; Oxford University Press: Oxford, UK, 2022. [Google Scholar]
- Berlie, A.; Terry, I.; Cottrell, S.; Pratt, F.L.; Szablewski, M. Magnetic ordering of defects in a molecular spin-Peierls system. J. Phys. Condens. Matter 2017, 29, 025809. [Google Scholar] [CrossRef] [Green Version]
- Wheland, R.C.; Martin, E.L. Synthesis of Substituted 7,7,8,8-Tetracyanoquinodimethanes. J. Org. Chem. 1975, 40, 3101. [Google Scholar] [CrossRef]
- Melby, L.R.; Harder, R.J.; Hertler, W.R.; Mahler, W.; Benson, R.E.; Mochel, W.E. Substituted Quinodimethans. II. Anion-radical Derivatives and Complexes of 7,7,8,8-Tetracyanoquinodimethan. J. Am. Chem. Soc. 1962, 84, 3374–3387. [Google Scholar] [CrossRef]
- Bonner, J.C.; Fisher, M.E. Linear magentic chains with anisotropic coupling. Phys. Rev. 1964, 135, A640. [Google Scholar] [CrossRef] [Green Version]
- Berlie, A.; Terry, I.; Szablewski, M.; Giblin, S.R. Separating the ferromagnetic and glassy behavior within the metal-organic magnet Ni(TCNQ)2. Phys. Rev. B 2015, 92, 184431. [Google Scholar] [CrossRef] [Green Version]
- Blundell, S.J.; Lancaster, T.; Brooks, M.L.; Pratt, F.L.; Taliaferro, M.L.; Miller, J.S. A μSR study of the metamagnetic phase transition in the electron-transfer salt [Fe(Cp)2] [TCNQ]. Phys. B Condens. Matter 2006, 374–375, 114–117. [Google Scholar] [CrossRef]
- Berlie, A.; Terry, I.; Szablewski, M. A 3D antiferromagnetic ground state in a quasi-1D π-stacked charge-transfer system. J. Mater. Chem. C 2018, 6, 12468–12472. [Google Scholar] [CrossRef] [Green Version]
- Lovett, B.W.; Blundell, S.J.; Pratt, F.L.; Jestädt, T.; Hayes, W.; Tagaki, S.; Kurmoo, M. Spin fluctuations in the spin-Peierls compound MEM(TCNQ)2 studied using muon spin relaxation. Phys. Rev. B 2000, 61, 12241. [Google Scholar] [CrossRef]
- Frandsen, B.A.; Petersen, K.A.; Ducharme, N.A.; Shaw, A.G.; Gibson, E.J.; Winn, B.; Yan, J.; Zhang, J.; Manley, M.E.; Hermann, R.P. Spin dynamics and a nearly continuous magnetic phase transition in an entropy-stabilized oxide antiferromagnet. Phys. Rev. Mater. 2020, 4, 074405. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Berlie, A.; Terry, I.; Szablewski, M. Driving a Molecular Spin-Peierls System into a Short Range Ordered State through Chemical Substitution. Magnetochemistry 2023, 9, 150. https://doi.org/10.3390/magnetochemistry9060150
Berlie A, Terry I, Szablewski M. Driving a Molecular Spin-Peierls System into a Short Range Ordered State through Chemical Substitution. Magnetochemistry. 2023; 9(6):150. https://doi.org/10.3390/magnetochemistry9060150
Chicago/Turabian StyleBerlie, Adam, Ian Terry, and Marek Szablewski. 2023. "Driving a Molecular Spin-Peierls System into a Short Range Ordered State through Chemical Substitution" Magnetochemistry 9, no. 6: 150. https://doi.org/10.3390/magnetochemistry9060150
APA StyleBerlie, A., Terry, I., & Szablewski, M. (2023). Driving a Molecular Spin-Peierls System into a Short Range Ordered State through Chemical Substitution. Magnetochemistry, 9(6), 150. https://doi.org/10.3390/magnetochemistry9060150






