Study of Solar Energetic Particle Events with Ulysses, ACE Observations and Numerical Simulations †
Abstract
1. Introduction
2. Observations
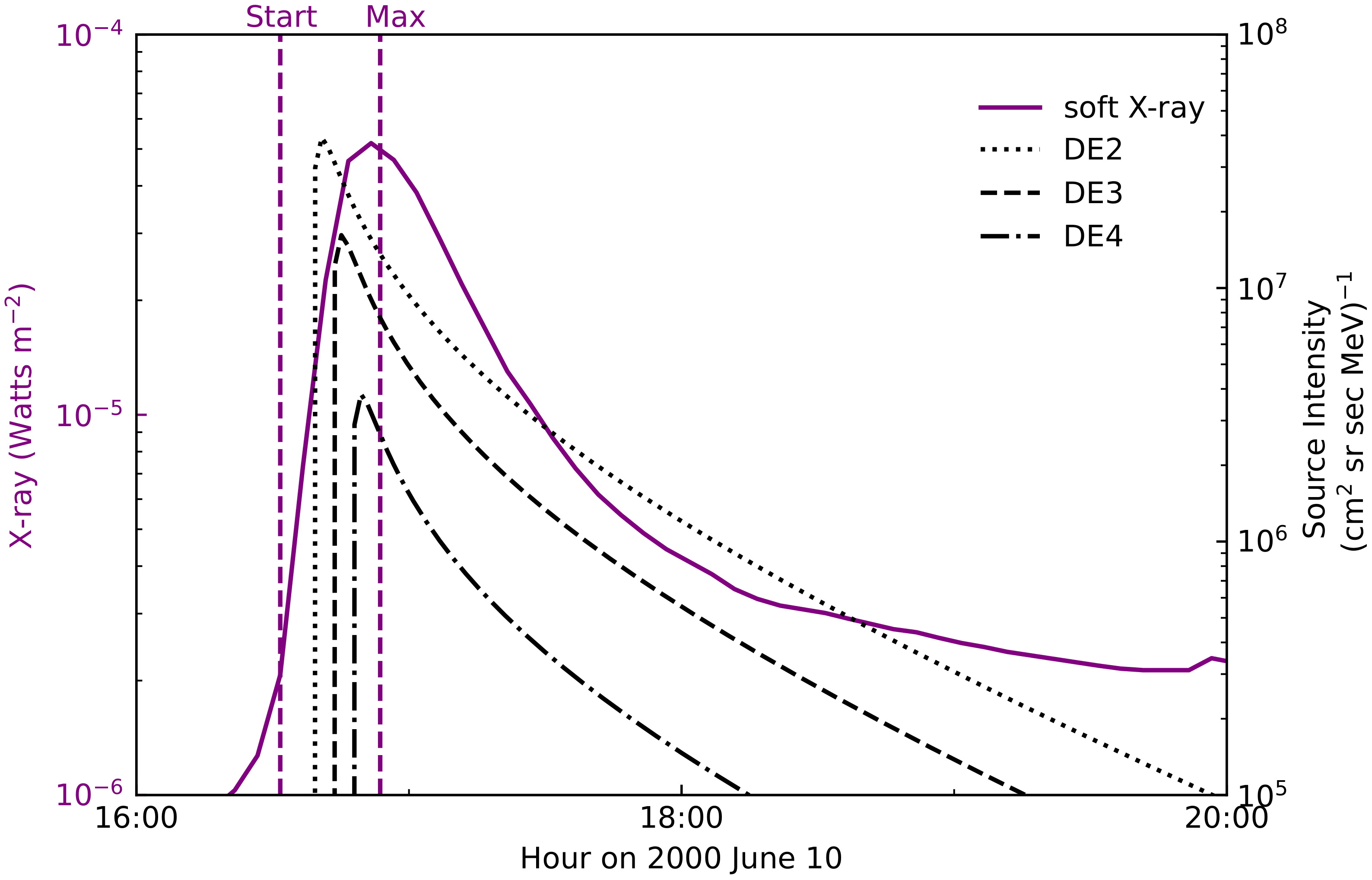
2.1. The Observation of the 10 June 2000 SEP Event
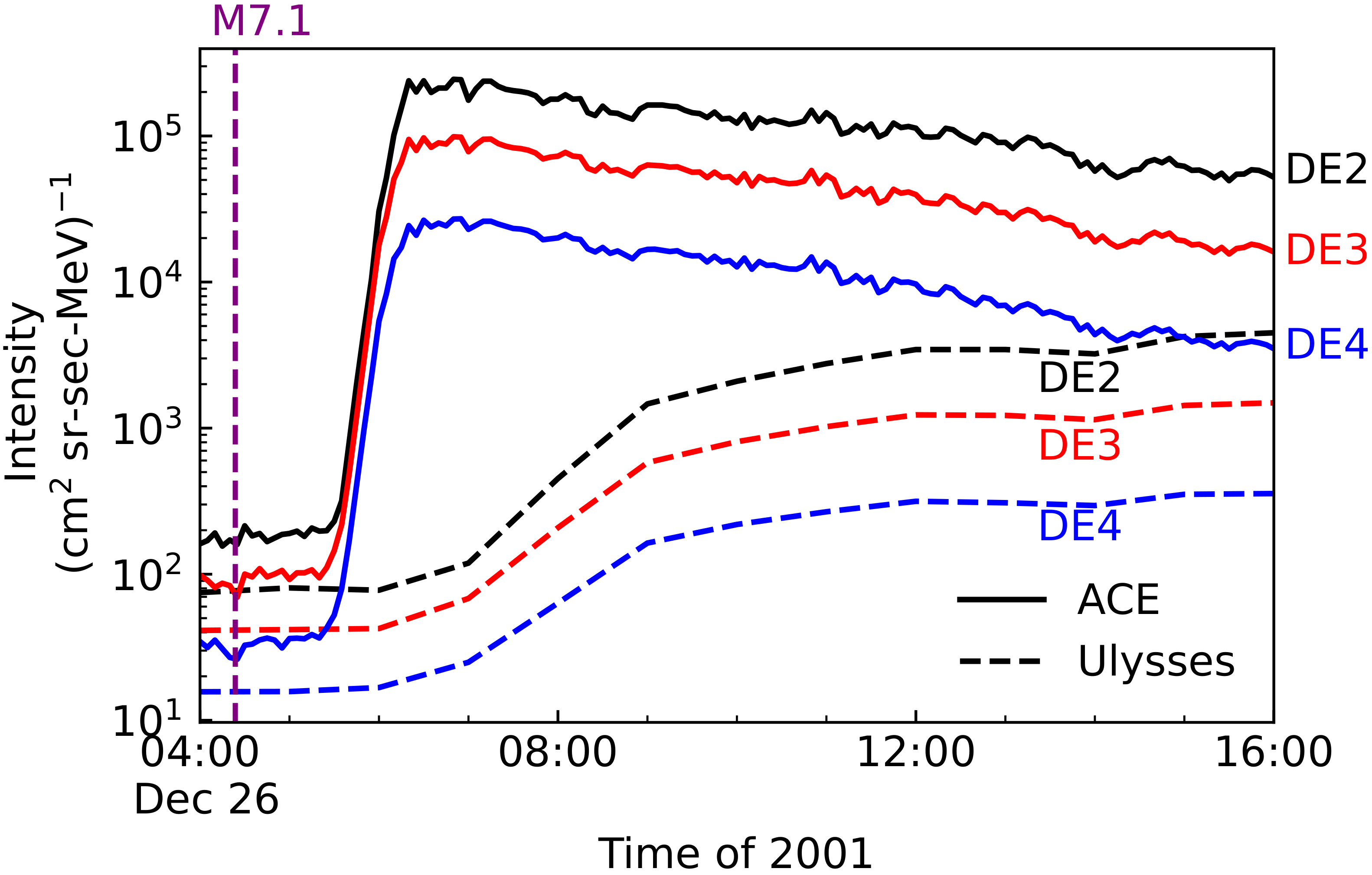
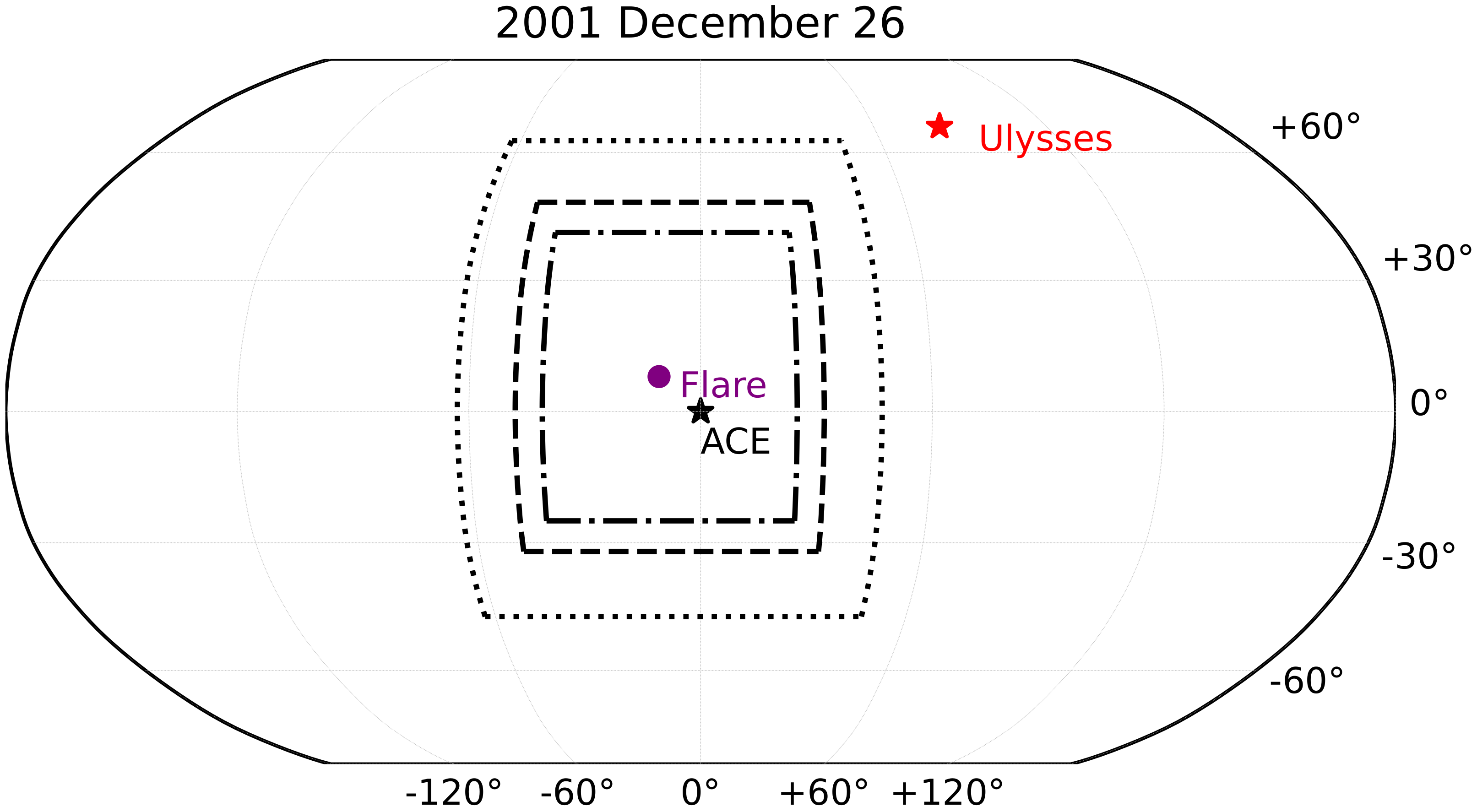
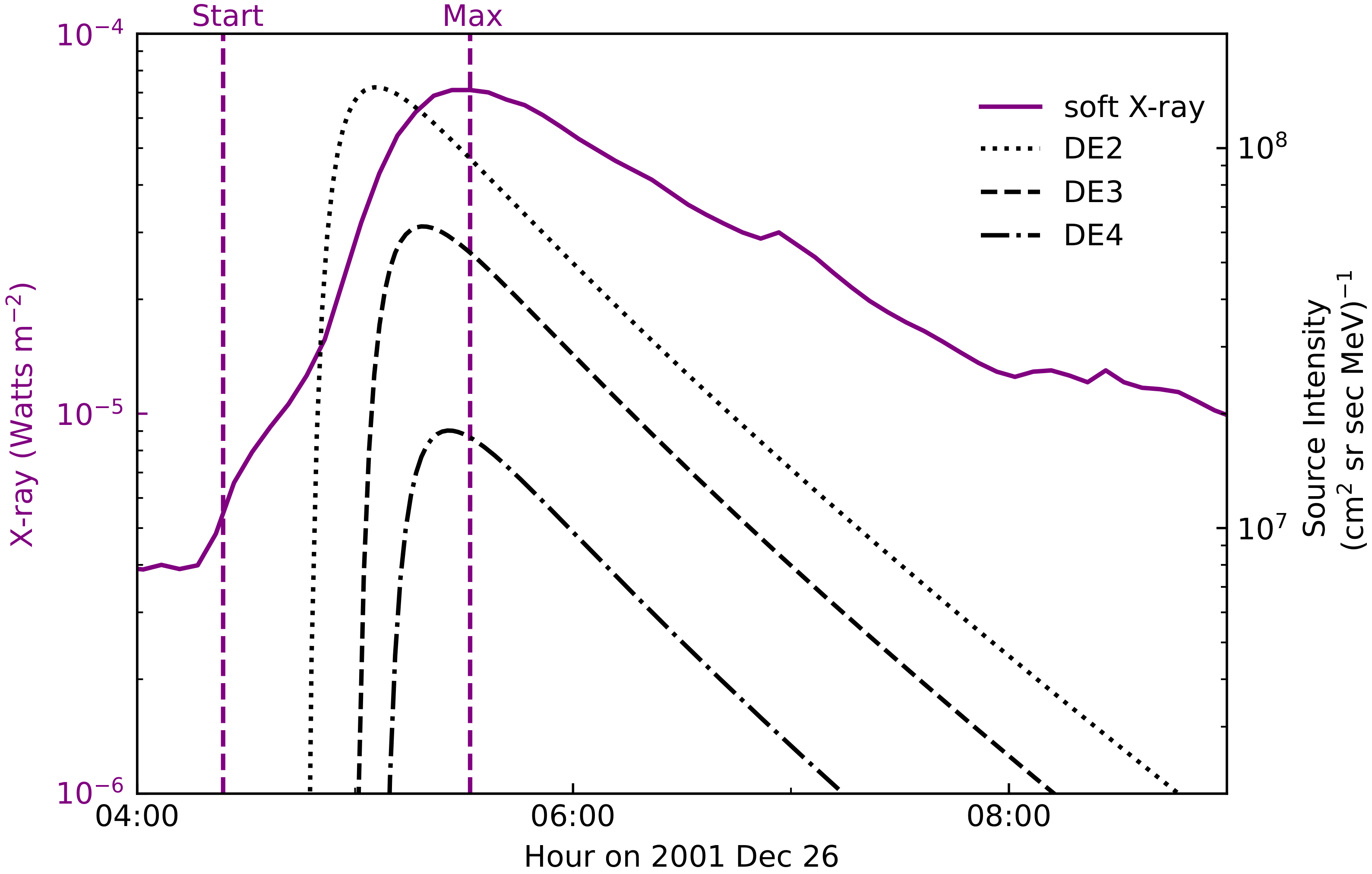
2.2. The Observation of the 26 December 2001 SEP Event
2.3. Summary of the SEP Events
3. Models
4. The SEP Simulation Results and Comparison with Observations
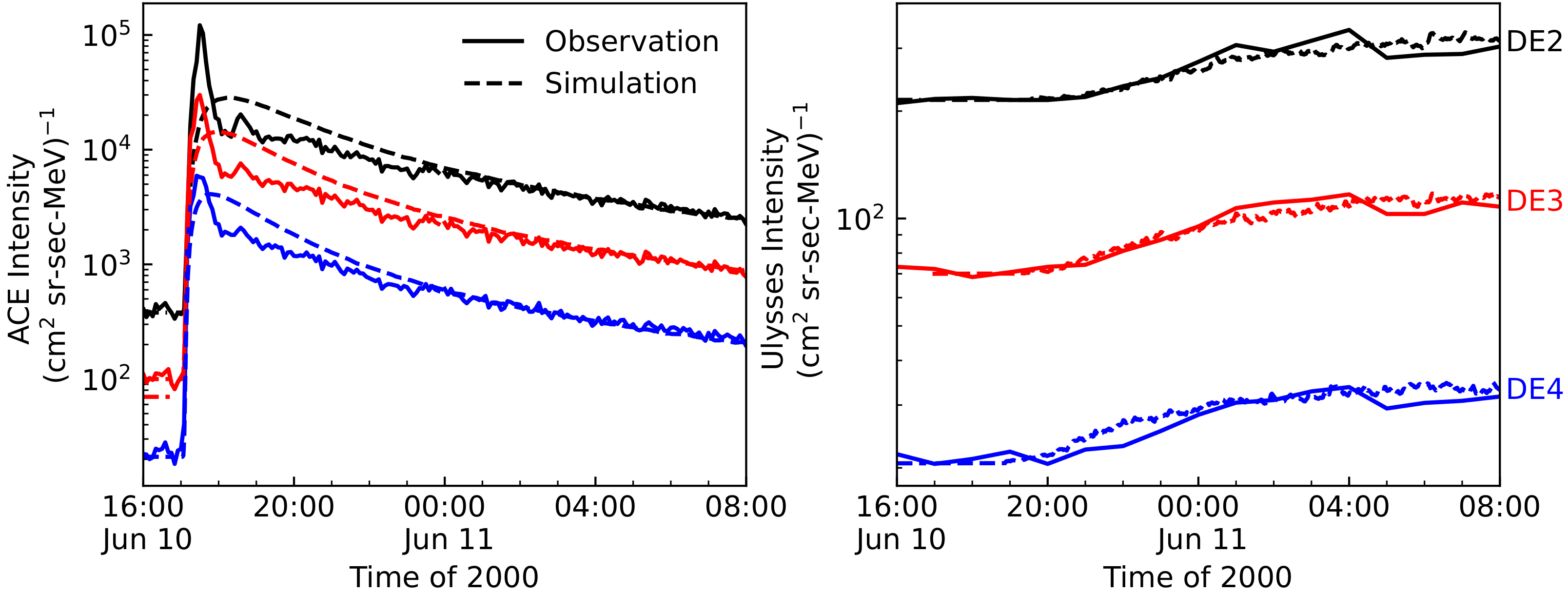
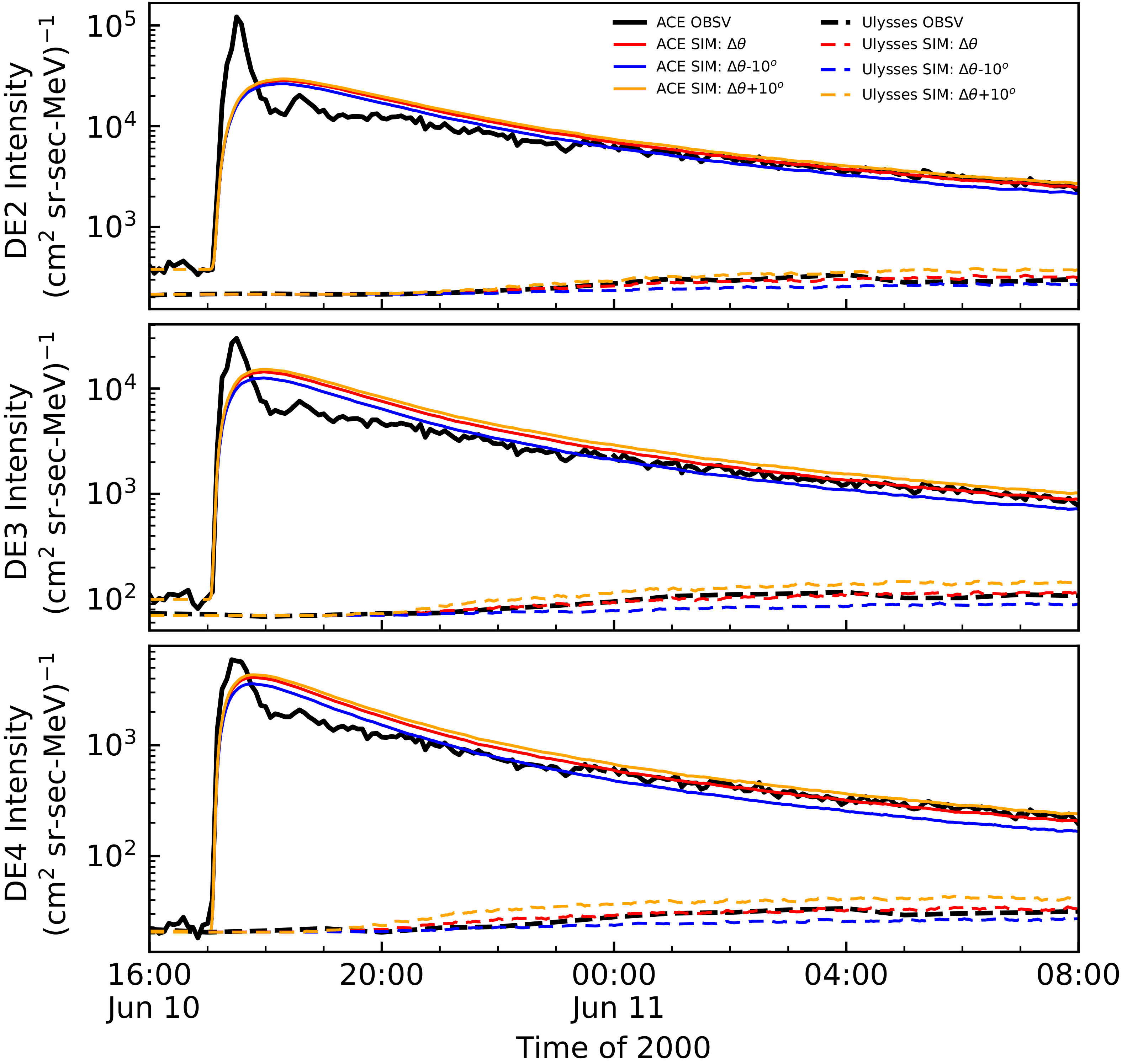
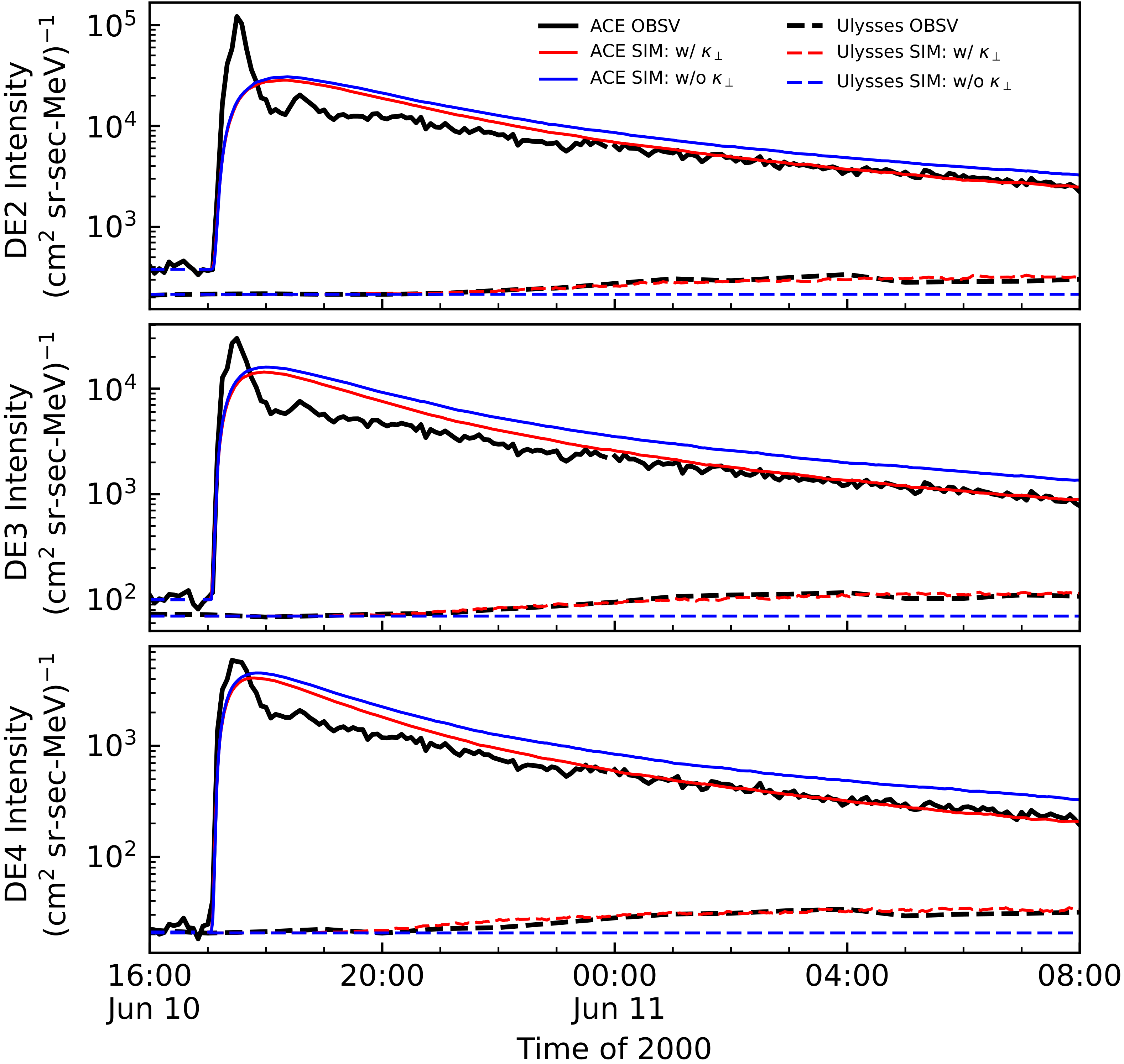
4.1. Study of the 10 June 2000 SEP Event through Simulations and a Comparison with Observations
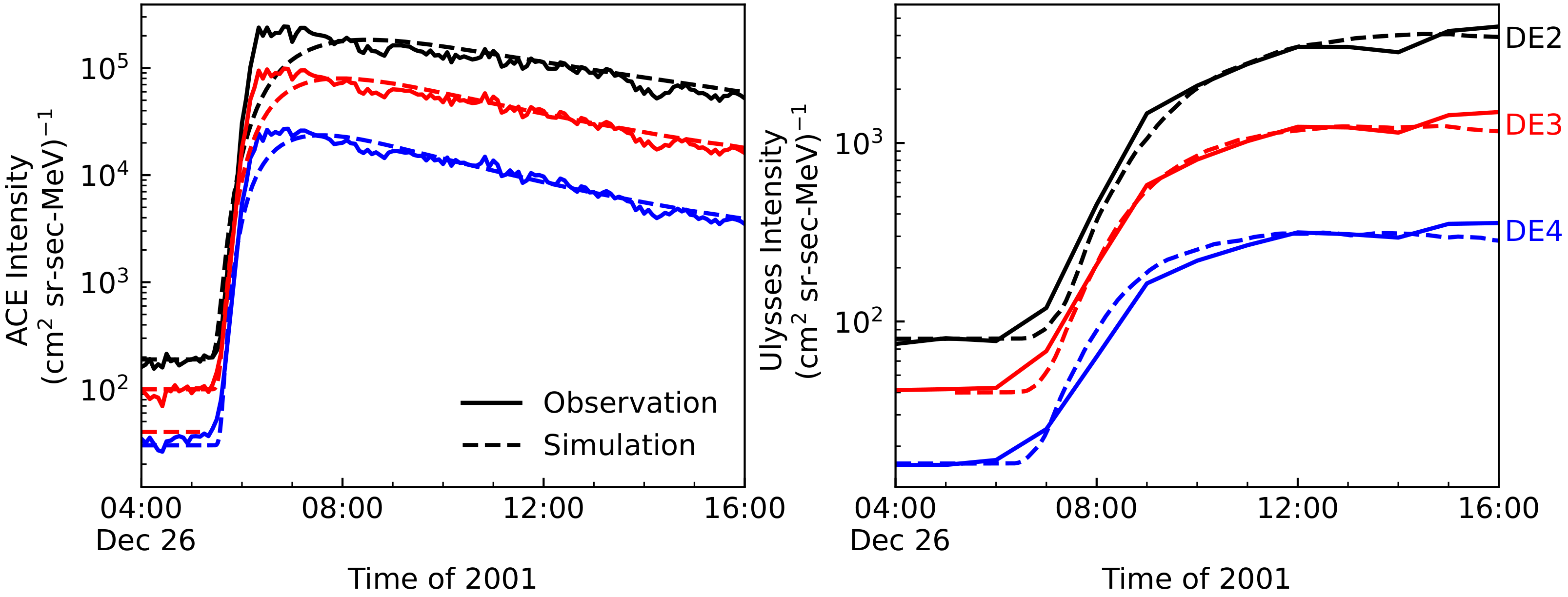
4.2. Study of the 26 December 2001 SEP Event through Simulations and a Comparison with Observations
5. Best Fit Parameters for the Particle Source and Models
6. Summary and Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Reames, D.V. Solar energetic particles: A paradigm shift. Rev. Geophys. 1995, 33, 585. [Google Scholar] [CrossRef]
- Reames, D.V. Particle acceleration at the Sun and in the heliosphere. Space Sci. Rev. 1999, 90, 413. [Google Scholar] [CrossRef]
- Cane, H.V.; von Rosenvinge, T.T.; Cohen, C.M.S.; Mewaldt, R.A. Two components in major solar particle events. Geophys. Res. Lett. 2003, 30, 8017. [Google Scholar] [CrossRef]
- Li, C.; Tang, Y.H.; Dai, Y.; Zong, W.G.; Fang, C. The acceleration characteristics of solar energetic particles in the 2000 July 14 event. Astron. Astrophys. 2007, 461, 1115–1119. [Google Scholar] [CrossRef]
- Cane, H.V.; Richardson, I.G.; von Rosenvinge, T.T. A study of solar energetic particle events of 1997-2006: Their composition and associations. J. Geophys. Res. 2010, 115, A08101. [Google Scholar] [CrossRef]
- Trottet, G.; Samwel, S.; Klein, K.L.; Dudok de Wit, T.; Miteva, R. Statistical Evidence for Contributions of Flares and Coronal Mass Ejections to Major Solar Energetic Particle Events. Sol. Phys. 2015, 290, 819. [Google Scholar] [CrossRef]
- Klein, K.L.; Dalla, S. Acceleration and Propagation of Solar Energetic Particles. Space Sci. Rev. 2017, 212, 1107–1136. [Google Scholar] [CrossRef]
- Wang, L.; Lin, R.P.; Krucker, S.; Mason, G.M. A Statistical Study of Solar Electron Events over One Solar Cycle. Astrophys. J. 2012, 759, 69. [Google Scholar] [CrossRef]
- Kahler, S.W. Solar Sources of Heliospheric Energetic Electron Events-Shocks or Flares? Space Sci. Rev. 2007, 129, 359–390. [Google Scholar] [CrossRef]
- Agueda, N.; Vainio, R.; Dalla, S.; Lario, D.; Sanahuja, B. Current Sheet Regulation of Solar Near-relativistic Electron Injection Histories. Astrophys. J. 2013, 765, 83. [Google Scholar] [CrossRef]
- Veronig, A.M.; Muhr, N.; Kienreich, I.W.; Temmer, M.; Vršnak, B. First Observations of a Dome-shaped Large-scale Coronal Extreme-ultraviolet Wave. Astrophys. J. Lett. 2010, 716, L57–L62. [Google Scholar] [CrossRef]
- Warmuth, A. Large-scale Globally Propagating Coronal Waves. Living Rev. Sol. Phys. 2015, 12, 3. [Google Scholar] [CrossRef]
- Wild, J.P. Observations of the Spectrum of High-Intensity Solar Radiation at Metre Wavelengths. II. Outbursts. Aust. J. Sci. Res. A Phys. Sci. 1950, 3, 399. [Google Scholar] [CrossRef]
- Gopalswamy, N.; Aguilar-Rodriguez, E.; Yashiro, S.; Nunes, S.; Kaiser, M.L.; Howard, R.A. Type II radio bursts and energetic solar eruptions. J. Geophys. Res. (Space Phys.) 2005, 110, A12S07. [Google Scholar] [CrossRef]
- Magdalenić, J.; Marqué, C.; Zhukov, A.N.; Vršnak, B.; Veronig, A. Flare-generated Type II Burst without Associated Coronal Mass Ejection. Astrophys. J. 2012, 746, 152. [Google Scholar] [CrossRef]
- Simnett, G.M. Solar and interplanetary particle acceleration. Advances in Space Research 2003, 31, 883–893. [Google Scholar] [CrossRef]
- Struminsky, A.B.; Grigorieva, I.Y.; Logachev, Y.I.; Sadovski, A.M. Two Phases of Solar Flares and a Stochastic Mechanism for Acceleration of Electrons and Protons. Astrophys. J. 2020, 63, 388–398. [Google Scholar] [CrossRef]
- Simnett, G.M.; Roelof, E.C.; Haggerty, D.K. The Acceleration and Release of Near-relativistic Electrons by Coronal Mass Ejections. Astrophys. J. 2002, 579, 854–862. [Google Scholar] [CrossRef]
- Simnett, G.M. Energetic Particles and Coronal Mass Ejections: A Case Study From ace and Ulysses. Sol. Phys. 2003, 213, 387–412. [Google Scholar] [CrossRef]
- Lario, D.; Decker, R.B.; Roelof, E.C.; Reisenfeld, D.B.; Sanderson, T.R. Low-energy particle response to CMEs during the Ulysses solar maximum northern polar passage. J. Geophys. Res. 2004, 109, A01107. [Google Scholar] [CrossRef]
- Dresing, N.; Kouloumvakos, A.; Vainio, R.; Rouillard, A. On the Role of Coronal Shocks for Accelerating Solar Energetic Electrons. Astrophys. J. Lett. 2022, 925, L21. [Google Scholar] [CrossRef]
- Dröge, W.; Kartavykh, Y.Y.; Dresing, N.; Klassen, A. Multi-spacecraft Observations and Transport Modeling of Energetic Electrons for a Series of Solar Particle Events in August 2010. Astrophys. J. 2016, 826, 134. [Google Scholar] [CrossRef]
- Liu, Y.; Luhmann, J.G.; Bale, S.D.; Lin, R.P. Solar Source and Heliospheric Consequences of the 2010 April 3 Coronal Mass Ejection: A Comprehensive View. Astrophys. J. 2011, 734, 84. [Google Scholar] [CrossRef]
- Richardson, I.G.; von Rosenvinge, T.T.; Cane, H.V.; Christian, E.R.; Cohen, C.M.S.; Labrador, A.W.; Leske, R.A.; Mewaldt, R.A.; Wiedenbeck, M.E.; Stone, E.C. >25 MeV Proton Events Observed by the High Energy Telescopes on the STEREO A and B Spacecraft and/or at Earth During the First ∼Seven Years of the STEREO Mission. Sol. Phys. 2014, 289, 3059–3107. [Google Scholar] [CrossRef]
- Desai, M.; Giacalone, J. Large gradual solar energetic particle events. Living Rev. Sol. Phys. 2016, 13, 3. [Google Scholar] [CrossRef] [PubMed]
- Paassilta, M.; Papaioannou, A.; Dresing, N.; Vainio, R.; Valtonen, E.; Heber, B. Catalogue of >55 MeV Wide-longitude Solar Proton Events Observed by SOHO, ACE, and the STEREOs at ∼1 AU During 2009-2016. Sol. Phys. 2018, 293, 70. [Google Scholar] [CrossRef]
- Wang, Y.; Lyu, D.; Xiao, B.X.; Qin, G.; Zhong, Y.S.; Lian, L.L. Statistical Survey of Reservoir Phenomenon in Energetic Proton Events Observed by Multiple Spacecraft. Astrophys. J. 2021, 909, 110. [Google Scholar] [CrossRef]
- Mason, G.M.; Gloeckler, G.; Hovestadt, D. Temporal variations of nucleonic abundances in solar flare energetic particle events. II - Evidence for large-scale shock acceleration. Astrophys. J. 1984, 280, 902–916. [Google Scholar] [CrossRef]
- Xu, Z.G.; Li, C.; Ding, M.D. Observations of a Coronal Shock Wave and the Production of Solar Energetic Particles. Astrophys. J. 2017, 840, 38. [Google Scholar] [CrossRef]
- Wiedenbeck, M.E.; Mason, G.M.; Cohen, C.M.S.; Nitta, N.V.; Gómez-Herrero, R.; Haggerty, D.K. Observations of Solar Energetic Particles from 3He-rich Events over a Wide Range of Heliographic Longitude. Astrophys. J. 2013, 762, 54. [Google Scholar] [CrossRef]
- Dresing, N.; Gómez-Herrero, R.; Heber, B.; Klassen, A.; Malandraki, O.; Dröge, W.; Kartavykh, Y. Statistical survey of widely spread out solar electron events observed with STEREO and ACE with special attention to anisotropies. Astron. Astrophys. 2014, 567, A27. [Google Scholar] [CrossRef]
- Bain, H.M.; Mays, M.L.; Luhmann, J.G.; Li, Y.; Jian, L.K.; Odstrcil, D. Shock Connectivity in the August 2010 and July 2012 Solar Energetic Particle Events Inferred from Observations and ENLIL Modeling. Astrophys. J. 2016, 825, 1. [Google Scholar] [CrossRef]
- Lario, D.; Haggerty, D.K.; Roelof, E.C.; Tappin, S.J.; Forsyth, R.J.; Gosling, J.T. Joint Ulysses and ACE observations of a magnetic cloud and the associated solar energetic particle event. Space Sci. Rev. 2001, 97, 277–280. [Google Scholar] [CrossRef]
- Lario, D.; Roelof, E.C.; Forsyth, R.J.; Gosling, J.T. 26-day Analysis of Energetic Ion Observations at High and Low Heliolatitudes: Ulysses and ACE. Space Sci. Rev. 2001, 97, 249–252. [Google Scholar] [CrossRef]
- Lario, D.; Decker, R.B.; Malandraki, O.E.; Lanzerotti, L.J. Influence of large-scale interplanetary structures on energetic particle propagation: September 2004 event at Ulysses and ACE. J. Geophys. Res. 2008, 113, A03105. [Google Scholar] [CrossRef]
- Malandraki, O.E.; Marsden, R.G.; Lario, D.; Tranquille, C.; Heber, B.; Mewaldt, R.A.; Cohen, C.M.S.; Lanzerotti, L.J.; Forsyth, R.J.; Elliott, H.A.; et al. Energetic Particle Observations and Propagation in the Three-dimensional Heliosphere During the 2006 December Events. Astrophys. J. 2009, 704, 469–476. [Google Scholar] [CrossRef]
- Dalla, S.; Balogh, A.; Krucker, S.; Posner, A.; Müller-Mellin, R.; Anglin, J.D.; Hofer, M.Y.; Marsden, R.G.; Sanderson, T.R.; Heber, B.; et al. Delay in solar energetic particle onsets at high heliographic latitudes. AnGeo 2003, 21, 1367–1375. [Google Scholar] [CrossRef]
- Malandraki, O.E.; Lario, D.; Lanzerotti, L.J.; Sarris, E.T.; Geranios, A.; Tsiropoula, G. October/November 2003 interplanetary coronal mass ejections: ACE/EPAM solar energetic particle observations. J. Geophys. Res. 2005, 110, A09S06. [Google Scholar] [CrossRef]
- Weygand, J.M.; Matthaeus, W.H.; Dasso, S.; Kivelson, M.G.; Kistler, L.M.; Mouikis, C. Anisotropy of the Taylor scale and the correlation scale in plasma sheet and solar wind magnetic field fluctuations. J. Geophys. Res. 2009, 114, A07213. [Google Scholar] [CrossRef]
- Weygand, J.M.; Matthaeus, W.H.; Dasso, S.; Kivelson, M.G. Correlation and Taylor scale variability in the interplanetary magnetic field fluctuations as a function of solar wind speed. J. Geophys. Res. 2011, 116, A08102. [Google Scholar] [CrossRef]
- Richardson, I.G.; Cane, H.V.; von Rosenvinge, T.T. Prompt arrival of solar energetic particles from far eastern events: The role of large-scale interplanetary magnetic field structure. J. Geophys. Res. 1991, 96, 7853–7860. [Google Scholar] [CrossRef]
- Richardson, I.G.; Cane, H.V. Particle flows observed in ejecta during solar event onsets and their implication for the magnetic field topology. J. Geophys. Res. 1996, 101, 27521–27532. [Google Scholar] [CrossRef]
- Chollet, E.E.; Mewaldt, R.A.; Cummings, A.C.; Gosling, J.T.; Haggerty, D.K.; Hu, Q.; Larson, D.; Lavraud, B.; Leske, R.A.; Opitz, A.; et al. Multipoint connectivity analysis of the May 2007 solar energeticparticle events. J. Geophys. Res. 2010, 115, 12106. [Google Scholar] [CrossRef]
- Qin, G.; Zhang, M.; Dwyer, J.R. Effect of adiabatic cooling on the fitted parallel mean free path of solar energetic particles. J. Geophys. Res. 2006, 111, A08101. [Google Scholar] [CrossRef]
- Qin, G.; Wang, Y.; Zhang, M.; Dalla, S. Transport of Solar Energetic Particles Accelerated by ICME Shocks: Reproducing the Reservoir Phenomenon. Astrophys. J. 2013, 766, 74. [Google Scholar] [CrossRef]
- Agueda, N.; Vainio, R.; Lario, D.; Sanahuja, B. Injection and Interplanetary Transport of Near-Relativistic Electrons: Modeling the Impulsive Event on 2000 May 1. Astrophys. J. 2008, 675, 1601–1613. [Google Scholar] [CrossRef]
- Agueda, N.; Lario, D.; Ontiveros, V.; Kilpua, E.; Sanahuja, B.; Vainio, R. Multi-spacecraft Study of the 8 November 2000 SEP Event: Electron Injection Histories 100° Apart. Sol. Phys. 2012, 281, 319–331. [Google Scholar] [CrossRef]
- Zhang, M.; Qin, G.; Rassoul, H. Propagation of Solar Energetic Particles in Three-Dimensional Interplanetary Magnetic Fields. Astrophys. J. 2009, 692, 109–132. [Google Scholar] [CrossRef]
- He, H.Q.; Qin, G.; Zhang, M. Propagation of Solar Energetic Particles in Three-dimensional Interplanetary Magnetic Fields: In View of Characteristics of Sources. Astrophys. J. 2011, 734, 74. [Google Scholar] [CrossRef]
- Wang, Y.; Qin, G.; Zhang, M. Effects of Perpendicular Diffusion on Energetic Particles Accelerated by the Interplanetary Coronal Mass Ejection shock. Astrophys. J. 2012, 752, 37. [Google Scholar] [CrossRef]
- Wang, Y.; Qin, G.; Zhang, M.; Dalla, S. A Numerical Simulation of Solar Energetic Particle Dropouts during Impulsive Events. Astrophys. J. 2014, 789, 157. [Google Scholar] [CrossRef]
- Dröge, W.; Kartavykh, Y.Y.; Dresing, N.; Heber, B.; Klassen, A. Wide longitudinal distribution of interplanetary electrons following the 7 February 2010 solar event: Observations and transport modeling. J. Geophys. Res. 2014, 119, 6074–6094. [Google Scholar] [CrossRef]
- Dröge, W.; Kartavykh, Y.Y.; Wang, L.; Telloni, D.; Bruno, R. Transport Modeling of Interplanetary Electrons in the 2002 October 20 Solar Particle Event. Astrophys. J. 2018, 869, 168. [Google Scholar] [CrossRef]
- Qin, G.; Wang, Y. Simulations of a Gradual Solar Energetic Particle Event Observed by Helios 1, Helios 2, and IMP 8. Astrophys. J. 2015, 809, 177. [Google Scholar] [CrossRef]
- Wang, Y.; Qin, G. Simulations of the Spatial and Temporal Invariance in the Spectra of Gradual Solar Energetic Particle Events. Astrophys. J. 2015, 806, 252. [Google Scholar] [CrossRef]
- Lario, D.; Kwon, R.Y.; Richardson, I.G.; Raouafi, N.E.; Thompson, B.J.; von Rosenvinge, T.T.; Mays, M.L.; Mäkelä, P.A.; Xie, H.; Bain, H.M.; et al. The Solar Energetic Particle Event of 2010 August 14: Connectivity with the Solar Source Inferred from Multiple Spacecraft Observations and Modeling. Astrophys. J. 2017, 838, 51. [Google Scholar] [CrossRef]
- Qin, G.; Qi, S.Y. Numerical simulation and data analysis of the 23 July 2012 SEP event observed by ACE, STEREO-A, and STEREO-B. Astron. Astrophys. 2020, 637, A48. [Google Scholar] [CrossRef]
- Dröge, W.; Kartavykh, Y.Y.; Klecker, B.; Kovaltsov, G.A. Anisotropic Three-Dimensional Focused Transport of Solar Energetic Particles in the Inner Heliosphere. J. Geophys. Res. 2010, 709, 912–919. [Google Scholar] [CrossRef]
- Dresing, N.; Gómez-Herrero, R.; Klassen, A.; Heber, B.; Kartavykh, Y.; Dröge, W.A. The Large Longitudinal Spread of Solar Energetic Particles During the 17 January 2010 Solar Event. Sol. Phys. 2012, 281, 281–300. [Google Scholar] [CrossRef]
- Wu, S.S.; Qin, G. Magnetic Cloud and Sheath in the Ground-Level Enhancement Event of 2000 July 14. I. Effects on the Solar Energetic Particles. Astrophys. J. 2020, 904, 151. [Google Scholar] [CrossRef]
- Zhao, L.L.; Zhang, M. Effects of Coronal Magnetic Field Structures on the Transport of Solar Energetic Particles. Astrophys. J. Lett. 2018, 859, L29. [Google Scholar] [CrossRef]
- Jokipii, J.R. Cosmic-Ray Propagation. I. Charged Particles in a Random Magnetic Field. Astrophys. J. 1966, 146, 480. [Google Scholar] [CrossRef]
- Matthaeus, W.H.; Qin, G.; Bieber, J.W.; Zank, G. Nonlinear Collisionless Perpendicular Diffusion of Charged Particles. Astrophys. J. 2003, 590, L53. [Google Scholar] [CrossRef]
- Dwyer, J.R.; Mason, G.M.; Mazur, J.E.; Jokipii, J.R.; von Rosenvinge, T.T.; Lepping, R.P. Perpendicular Transport of Low-Energy Corotating Interaction Region–associated Nuclei. Astrophys. J. 1997, 490, L115–L118. [Google Scholar] [CrossRef]
- Zhang, M.; Jokipii, J.R.; McKibben, R.B. Perpendicular Transport of Solar Energetic Particles in Heliospheric Magnetic Fields. Astrophys. J. 2003, 595, 493. [Google Scholar] [CrossRef]
- Qin, G.; Matthaeus, W.H.; Bieber, J.W. Subdiffusive transport of charged particles perpendicular to the large scale magnetic field. Geophys. Res. Lett. 2002, 29, 1048. [Google Scholar] [CrossRef]
- Qin, G.; Matthaeus, W.H.; Bieber, J.W. Perpendicular transport of charged particles in composite model turbulence: Recovery of diffusion. Astrophys. J. 2002, 578, L117–L120. [Google Scholar] [CrossRef]
- Qin, G. Nonlinear Parallel Diffusion of Charged Particles: Extent to the Nonlinear Guiding Center Theory. Astrophys. J. 2007, 656, 217–221. [Google Scholar] [CrossRef]
- Cane, H.V.; Reames, D.V.; von Rosenvinge, T.T. The role of interplanetary shocks in the longitude distribution of solar energetic particles. J. Geophys. Res. 1988, 93, 9555–9567. [Google Scholar] [CrossRef]
- Cliver, E.W.; Kahler, S.W.; Neidig, D.F.; Cane, H.V.; Richardson, I.G.; Kallenrode, M.B.; Wibberenz, G. Extreme “Propagation” of Solar Energetic Particles. In Proceedings of the 24th InInternational Cosmic Ray Conference, Rome, Italy, 28 August–8 September 1995; Volume 4, p. 257. [Google Scholar]
- Reames, D.V.; Barbier, L.M.; Ng, C.K. The Spatial Distribution of Particles Accelerated by Coronal Mass Ejection-driven Shocks. Astrophys. J. 1996, 466, 473. [Google Scholar] [CrossRef]
- Lario, D.; Kwon, R.Y.; Riley, P.; Raouaf, N.E. On the Link between the Release of Solar Energetic Particles Measured at Widespread Heliolongitudes and the Properties of the Associated Coronal Shocks. Astrophys. J. 2017, 847, 103. [Google Scholar] [CrossRef]
- Wang, H.; Chae, J.; Yurchyshyn, V.; Yang, G.; Steinegger, M.; Goode, P. Inter-Active Region Connection of Sympathetic Flaring on 2000 February 17. Astrophys. J. 2001, 559, 1171–1179. [Google Scholar] [CrossRef]
- Schrijver, C.J.; Title, A.M. Long-range magnetic couplings between solar flares and coronal mass ejections observed by SDO and STEREO. J. Geophys. Res. 2011, 116, A04108. [Google Scholar] [CrossRef]
- Shen, Y.; Liu, Y.; Su, J. Sympathetic Partial and Full Filament Eruptions Observed in One Solar Breakout Event. Astrophys. J. 2012, 750, 12. [Google Scholar] [CrossRef]
- Schrijver, C.J.; Title, A.M.; Yeates, A.R.; DeRosa, M.L. Pathways of Large-scale Magnetic Couplings between Solar Coronal Events. Astrophys. J. 2013, 773, 93. [Google Scholar] [CrossRef]
- Klein, K.L.; Krucker, S.; Lointier, G.; Kerdraon, A. Open magnetic flux tubes in the corona and the transport of solar energetic particles. Astron. Astrophys. 2008, 486, 589–596. [Google Scholar] [CrossRef]
- Masson, S.; Antiochos, S.K.; DeVore, C.R. A Model for the Escape of Solar-flare-accelerated Particles. Astrophys. J. 2013, 771, 82. [Google Scholar] [CrossRef]
- Shalchi, A.; Qin, G. Random Walk of Magnetic Field Lines: Analytical Theory versus Simulations. Astrophys. J. 2010, 330, 279. [Google Scholar] [CrossRef]
- Strauss, R.D.; Fichtner, H. On Aspects Pertaining to the Perpendicular Diffusion of Solar Energetic Particles. Astrophys. J. 2015, 801, 29. [Google Scholar] [CrossRef]
- Zhang, M.; Zhao, L. Precipitation and Release of Solar Energetic Particles from the Solar Coronal Magnetic Field. Astrophys. J. 2017, 846, 107. [Google Scholar] [CrossRef]
- Diaz, I.; Zhang, M.; Qin, G.; Rassoul, H. Delay in Onset Times of Solar Energetic Particles. In Proceedings of the 32nd International Cosmic Ray Conference, Beijing, China, 11–18 August 2011; Volume 10, p. 41. [Google Scholar] [CrossRef]
- Wang, Y.; Qin, G. Estimation of the release time of solar energetic particles near the Sun. Astrophys. J. 2015, 799, 111. [Google Scholar] [CrossRef]
- Qin, G.; Shalchi, A. Numerical investigation of the infulence of large turbulence scales on the parallel and perpendicular transport of cosmic rays. Adv. Space Res. 2012, 49, 1643–1652. [Google Scholar] [CrossRef]
- Matthaeus, W.H.; Goldstein, M.L.; Roberts, D.A. Evidence for the presence of quasi-two-dimensional nearly incompressible fluctuations in the solar wind. J. Geophys. Res. 1990, 95, 20673–20683. [Google Scholar] [CrossRef]
- Heras, A.M.; Sanahuja, B.; Smith, Z.K.; Detman, T.; Dryer, M. The Influence of the Large-Scale Interplanetary Shock Structure on a Low-Energy Particle Event. Astrophys. J. 1992, 391, 359. [Google Scholar] [CrossRef]
- Heras, A.M.; Sanahuja, B.; Lario, D.; Smith, Z.K.; Detman, T.; Dryer, M. Three Low-Energy Particle Events: Modeling the Influence of the Parent Interplanetary Shock. Astrophys. J. 1995, 445, 497. [Google Scholar] [CrossRef]
- Kallenrode, M.B.; Wibberenz, G. Propagation of particles injected from interplanetary shocks: A black box model and its consequences for acceleration theory and data interpretation. J. Geophys. Res. 1997, 102, 22311–22334. [Google Scholar] [CrossRef]
- Lario, D.; Sanahuja, B.; Heras, A.M. Energetic Particle Events: Efficiency of Interplanetary Shocks as 50 keV < E < 100 MeV Proton Accelerators. Astrophys. J. 1998, 509, 415–434. [Google Scholar] [CrossRef]
- Kallenrode, M.B. Shock as a black box: 2. Effects of adiabatic deceleration and convection included. J. Geophys. Res. 2001, 106, 24989–25004. [Google Scholar] [CrossRef]
- Lin, R.P. Non-relativistic Solar Electrons. Space Sci. Rev. 1974, 16, 189–256. [Google Scholar] [CrossRef]
- Lin, R.P. WIND Observations of Suprathermal Electrons in the Interplanetary Medium. Space Sci. Rev. 1998, 86, 61–78. [Google Scholar] [CrossRef]
- Haggerty, D.K.; Roelof, E.C. Impulsive Near-relativistic Solar Electron Events: Delayed Injection with Respect to Solar Electromagnetic Emission. Astrophys. J. 2002, 579, 841–853. [Google Scholar] [CrossRef]
- Ho, G.C.; Roelof, E.C.; Mason, G.M.; Lario, D.; Mazur, J.E. Onset study of impulsive solar energetic particle events. Adv. Space Res. 2003, 32, 2679–2684. [Google Scholar] [CrossRef]
- Mason, G.M. 3He-Rich Solar Energetic Particle Events. Space Sci. Rev. 2007, 130, 231–242. [Google Scholar] [CrossRef]
- Qin, G.; He, H.Q.; Zhang, M. An effect of perpendicular diffusion on the anisotropy of solar energetic particles from unconnected sources. Astrophys. J. 2011, 738, 28. [Google Scholar] [CrossRef]
- Gold, R.E.; Krimigis, S.M.; Hawkins, S.E.u.; Haggerty, D.K.; Lohr, D.A.; Fiore, E.; Armstrong, T.P.; Holland, G.; Lanzerotti, L.J. Electron, Proton, and Alpha Monitor on the Advanced Composition Explorer spacecraft. Space Sci. Rev. 1998, 86, 541–562. [Google Scholar] [CrossRef]
- Lanzerotti, L.J.; Gold, R.E.; Anderson, K.A.; Armstrong, T.P.; Lin, R.P.; Krimigis, S.M.; Pick, M.; Roelof, E.C.; Sarris, E.T.; Simnett, G.M. Heliosphere Instrument for Spectra, Composition and Anisotropy at Low Energies. Astron. Astrophys. Suppl. Ser. 1992, 92, 349–363. [Google Scholar]
- Lario, D. Heliospheric Energetic Particle Reservoirs: Ulysses and ACE 175-315 keV Electron Observations. AIP Conf. Proc. 2010, 1216, 625. [Google Scholar] [CrossRef]
- McComas, D.J.; Bame, S.J.; Barker, P.; Feldman, W.C.; Phillips, J.L.; Riley, P.; Griffee, J.W. Solar Wind Electron Proton Alpha Monitor (SWEPAM) for the Advanced Composition Explorer. Space Sci. Rev. 1998, 86, 563–612. [Google Scholar] [CrossRef]
- Bame, S.J.; McComas, D.J.; Barraclough, B.L.; Phillips, J.L.; Sofaly, K.J.; Chavez, J.C.; Goldstein, B.E.; Sakurai, R.K. The ULYSSES solar wind plasma experiment. Astron. Astrophys. Suppl. Ser. 1992, 92, 237–265. [Google Scholar]
- Qin, G.; Zhang, M.; Dwyer, J.R.; Rassoul, H.K.; Mason, G.M. The Model Dependence of Solar Energetic Particle Mean Free Paths Under Weak Scattering. Astrophys. J. 2005, 627, 562. [Google Scholar] [CrossRef]
- Agueda, N.; Klein, K.L.; Vilmer, N.; Rodríguez-Gasén, R.; Malandraki, O.E.; Papaioannou, A.; Subirȧ, M.; Sanahuja, B.; Valtonen, E.; Dröge, W.; et al. Release timescales of solar energetic particles in the low corona. Astron. Astrophys. 2014, 570, A5. [Google Scholar] [CrossRef]
- Skilling, J. Cosmic Rays in the Galaxy: Convection or Diffusion? Astrophys. J. 1971, 170, 265. [Google Scholar] [CrossRef]
- Schlicheiser, R. Cosmic Ray Astrophysics, 2nd ed.; Springer: Berlin/Heidelberg, Germany, 2002. [Google Scholar]
- Beek, J.; Wibberenz, G. Pitch Angle Distributions of Solar Energetic Particles and the Local Scattering Properties of the Interplanetary Medium. Astrophys. J. 1986, 311, 437. [Google Scholar] [CrossRef]
- Qin, G.; Shalchi, A. Pitch angle diffusion coefficients of charged particles from computer simulations. Astrophys. J. 2009, 707, 61–66. [Google Scholar] [CrossRef]
- Qin, G.; Shalchi, A. Pitch-Angle Dependent Perpendicular Diffusion of Energetic Particles Interacting With Magnetic Turbulence. Appl. Phys. Res. 2014, 6, 1–13. [Google Scholar] [CrossRef]
- Hasselmann, K.; Wibberenz, G. Scattering of charged particles by random electromagnetic fields. Z. Fur Geophys. 1968, 34, 353. [Google Scholar]
- Earl, J.A. The diffusive idealization of charged-particle transport in random magnetic fields. Astrophys. J. 1974, 193, 231. [Google Scholar] [CrossRef]
- Shalchi, A.; Bieber, J.W.; Matthaeus, W.H. Analytic Forms of the Perpendicular Diffusion Coefficient in Magnetostatic Turbulence. Astrophys. J. 2004, 604, 675. [Google Scholar] [CrossRef]
- Shalchi, A.; Li, G.; Zank, G.P. Analytic forms of the perpendicular cosmic ray diffusion coefficient for an arbitrary turbulence spectrum and applications on transport of Galactic protons and acceleration at interplanetary shocks. Astrophys. Space Sci. 2010, 325, 99. [Google Scholar] [CrossRef]
- Adhikari, L.; Zank, G.P.; Hunana, P.; Shiota, D.; Bruno, R.; Hu, Q.; Telloni, D., II. Transport of Nearly Incompressible Magnetohydrodynamic Turbulence from 1 to 75 au. Astrophys. J. 2017, 841, 85. [Google Scholar] [CrossRef]
- Zank, G.P.; Adhikari, L.; Hunana, P.; Shiota, D.; Bruno, R.; Telloni, D. Theory and Transport of Nearly Incompressible Magnetohydrodynamic Turbulence. Astrophys. J. 2017, 835, 147. [Google Scholar] [CrossRef]
- Zank, G.P.; Adhikari, L.; Zhao, L.L.; Mostafavi, P.; Zirnstein, E.J.; McComas, D.J. The Pickup Ion-mediated Solar Wind. Astrophys. J. 2017, 835, 147. [Google Scholar] [CrossRef]
- Zhao, L.L.; Adhikari, L.; Zank, G.P.; Hu, Q.; Feng, X.S. Influence of the Solar Cycle on Turbulence Properties and Cosmic-Ray Diffusion. Astrophys. J. 2018, 856, 94. [Google Scholar] [CrossRef]
- Reid, G.C. A diffusive model for the initial phase of a solar proton event. J. Geophys. Res. 1964, 69, 2659–2667. [Google Scholar] [CrossRef]
- Zhang, M. A Markov Stochastic Process Theory of Cosmic-Ray Modulation. Astrophys. J. 1999, 513, 409. [Google Scholar] [CrossRef]
- Vainio, R.; Valtonen, E.; Heber, B.; Malandraki, O.E.; Papaioannou, A.; Klein, K.L.; Afanasiev, A.; Agueda, N.; Aurass, H.; Battarbee, M.; et al. The first SEPServer event catalogue 68-MeV solar proton events observed at 1 AU in 1996–2010. J. Space Weather Space Clim. 2013, 3, A12. [Google Scholar] [CrossRef]
- Kong, F.J.; Qin, G.; Wu, S.S.; Zhang, L.H.; Wang, H.N.; Chen, T.; Sun, P. Study of Time Evolution of the Bend-over Energy in the Energetic Particle Spectrum at a Parallel Shock. Astrophys. J. 2019, 877, 97. [Google Scholar] [CrossRef]


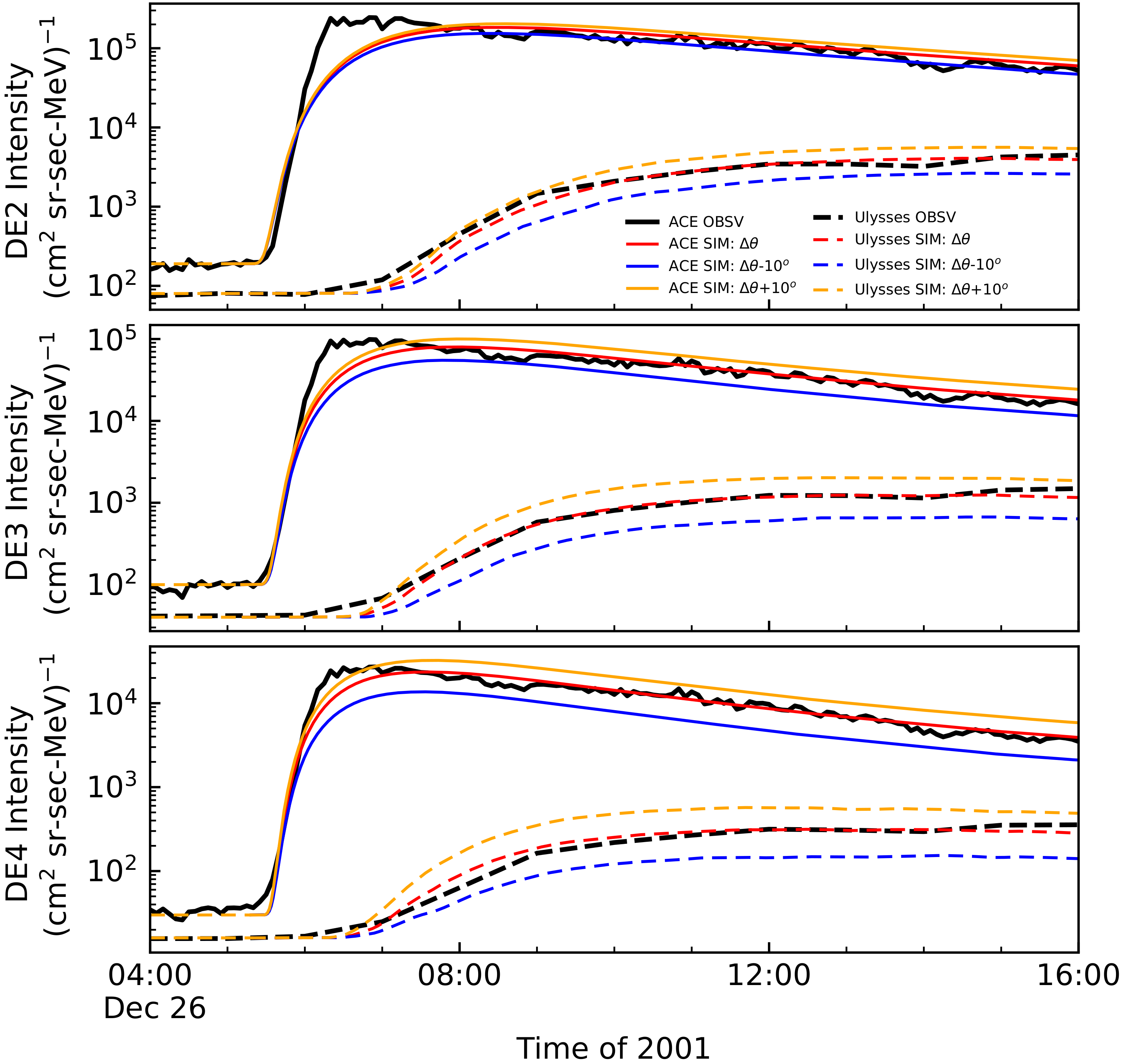
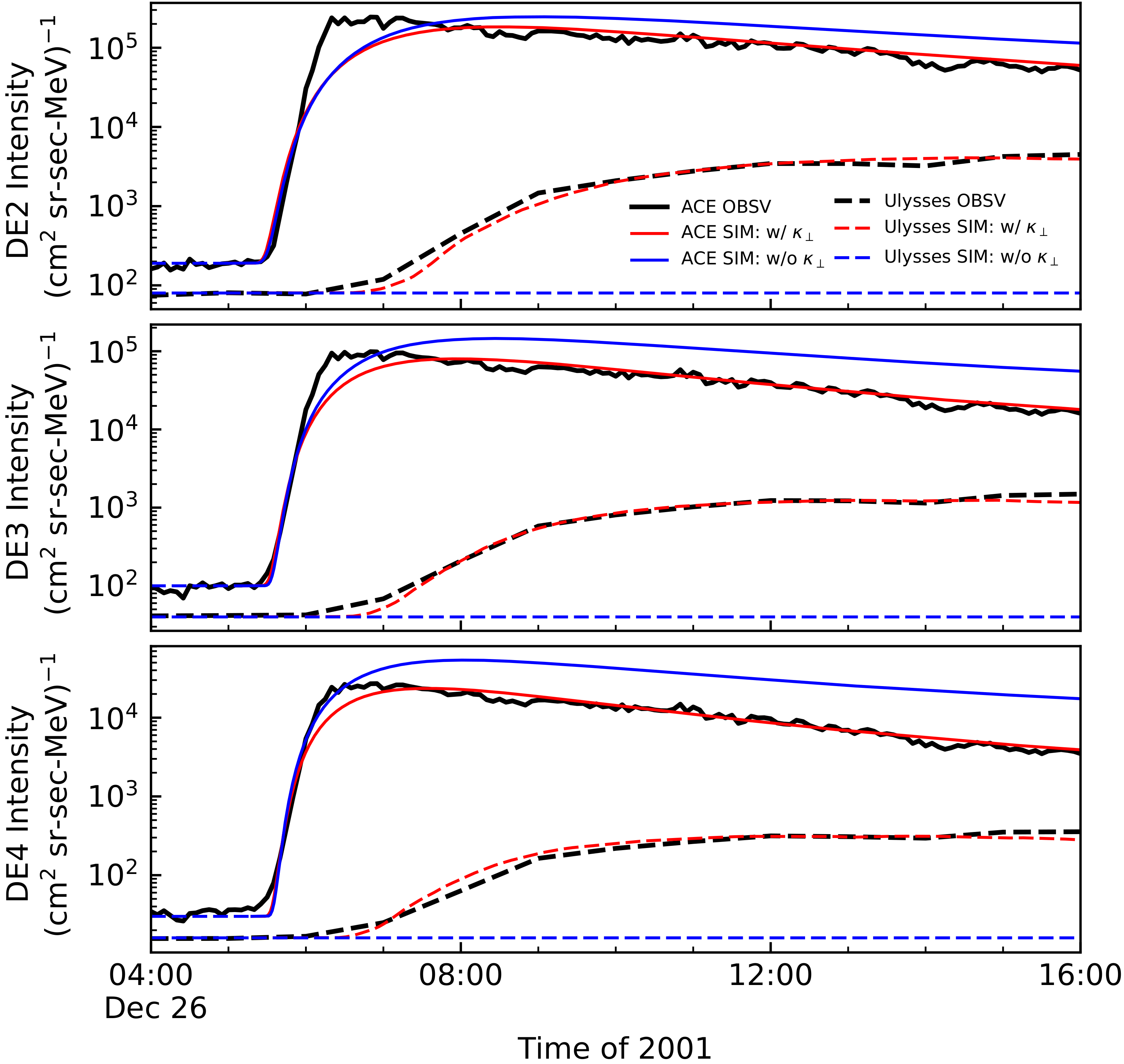

| Event Date | Flare | SXR | SXR | SXR | CME | CME | Ulysses | Ulysses | |||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Site | Class | (Watts m) | Onset | Max | Speed | Width | (km s) | (km s) | R (AU) | Loc | |
| 10 June 2000 | N22W38 | M5.2 | 5.2 | 16:40 | 17:02 | 1108 | Halo | 505 | 475 | 3.37 | S58E87 |
| 26 December 2001 | N08W54 | M7.1 | 7.1 | 04:32 | 05:40 | 1446 | 212 | 380 | 575 | 2.54 | N67W39 |
| Event Date | Channel | Peak | Peak | Ratio |
|---|---|---|---|---|
| (cm sr s MeV) | (cm sr s MeV) | |||
| DE2 | 1.21 | 3.37 | 3.59 | |
| 10 June 2000 | DE3 | 3.00 | 1.17 | 2.56 |
| DE4 | 5.92 | 3.37 | 1.76 | |
| DE2 | 2.44 | 4.50 | 5.42 | |
| 26 December 2001 | DE3 | 9.88 | 1.50 | 6.63 |
| DE4 | 2.71 | 4.00 | 7.60 |
| Event Date | Channel | ||||||
|---|---|---|---|---|---|---|---|
| (day) | (day) | () | (cm sr s MeV) | (min) | |||
| DE2 | 0.002 | 0.05 | 60 | 3.92 | 6.32 | ||
| 10 June 2000 | 0.353 | DE3 | 0.002 | 0.05 | 53 | 1.61 | 10.64 |
| DE4 | 0.002 | 0.05 | 53 | 3.47 | 14.96 | ||
| DE2 | 0.02 | 0.05 | 55 | 1.45 | 20.08 | ||
| 26 December 2001 | 0.533 | DE3 | 0.02 | 0.05 | 40 | 6.22 | 33.04 |
| DE4 | 0.02 | 0.05 | 33 | 1.81 | 40.24 |
| Event Date | Channel | (au) | ||
|---|---|---|---|---|
| DE2 | 0.220 | 0.023 | ||
| 10 June 2000 | 0.353 | DE3 | 0.245 | 0.021 |
| DE4 | 0.273 | 0.020 | ||
| DE2 | 0.097 | 0.069 | ||
| 26 December 2001 | 0.533 | DE3 | 0.108 | 0.064 |
| DE4 | 0.120 | 0.060 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lian, L.; Qin, G.; Wang, Y.; Cui, S. Study of Solar Energetic Particle Events with Ulysses, ACE Observations and Numerical Simulations. Magnetochemistry 2023, 9, 96. https://doi.org/10.3390/magnetochemistry9040096
Lian L, Qin G, Wang Y, Cui S. Study of Solar Energetic Particle Events with Ulysses, ACE Observations and Numerical Simulations. Magnetochemistry. 2023; 9(4):96. https://doi.org/10.3390/magnetochemistry9040096
Chicago/Turabian StyleLian, Lele, Gang Qin, Yang Wang, and Shuwang Cui. 2023. "Study of Solar Energetic Particle Events with Ulysses, ACE Observations and Numerical Simulations" Magnetochemistry 9, no. 4: 96. https://doi.org/10.3390/magnetochemistry9040096
APA StyleLian, L., Qin, G., Wang, Y., & Cui, S. (2023). Study of Solar Energetic Particle Events with Ulysses, ACE Observations and Numerical Simulations. Magnetochemistry, 9(4), 96. https://doi.org/10.3390/magnetochemistry9040096






