Research on Identification and Detection of Aluminum Plate Thickness Step Change Based on Electromagnetic Acoustic Resonance
Abstract
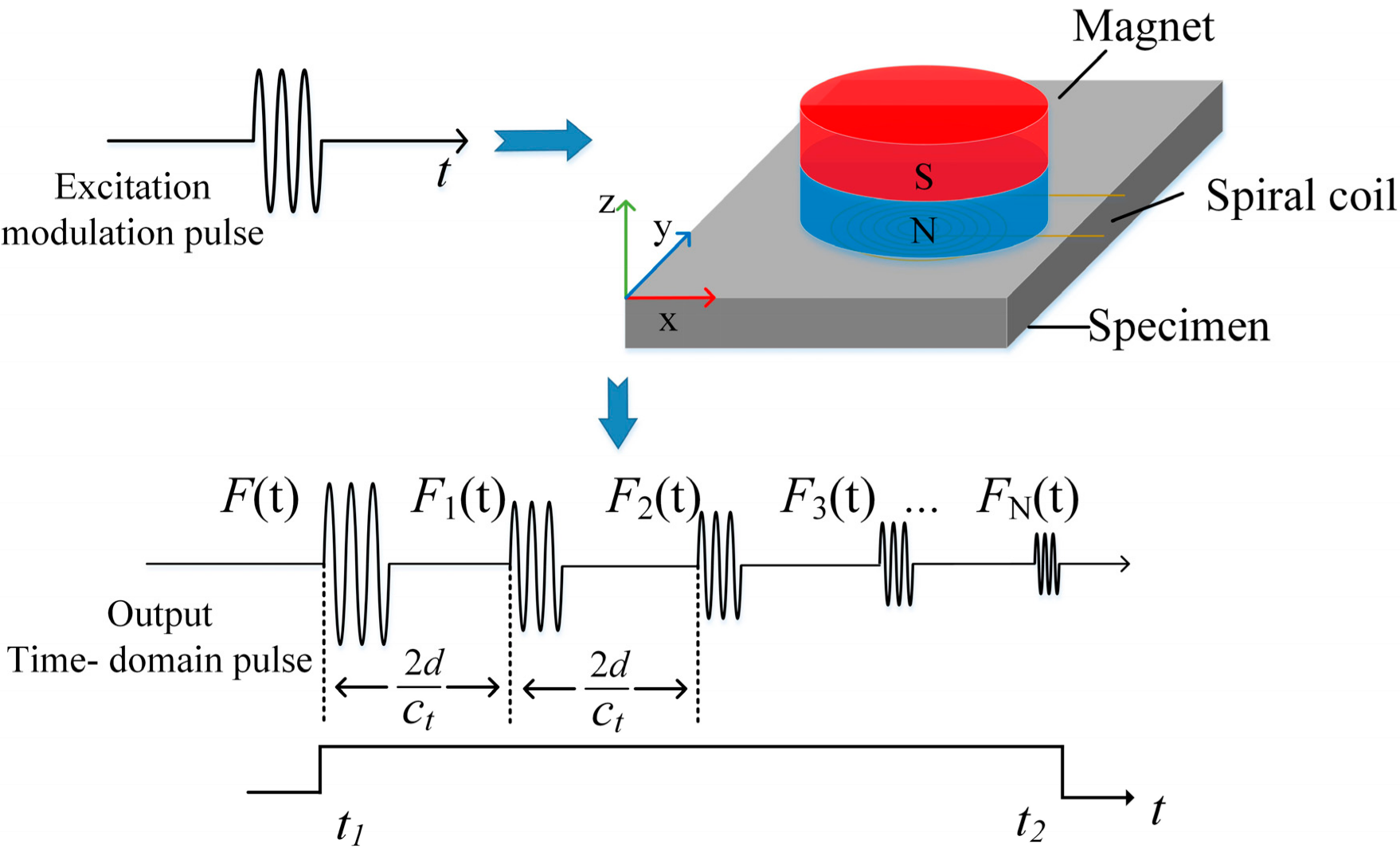
1. Introduction
2. Theory and Methods
2.1. Principle of EMAR Detection
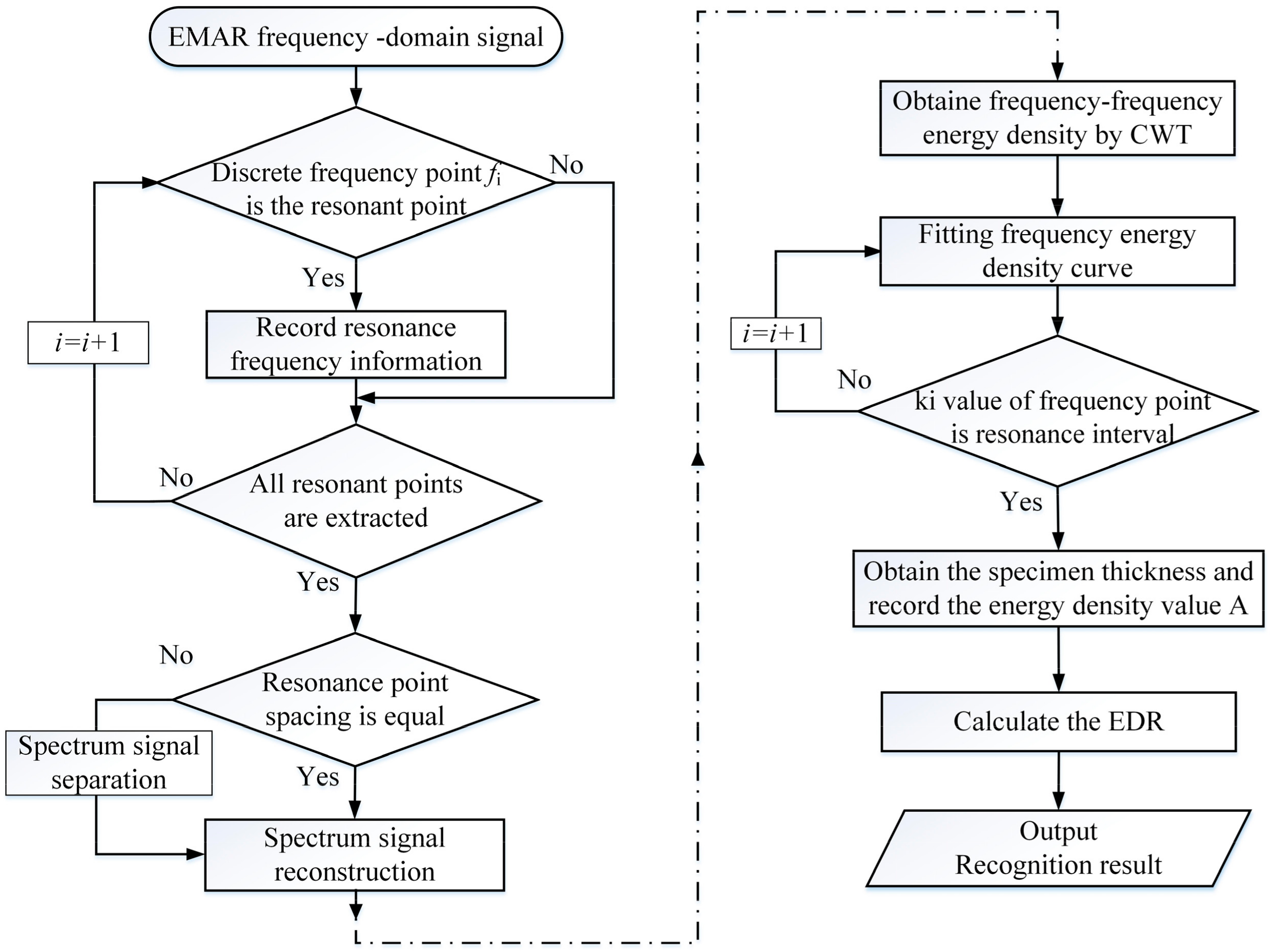
2.2. FFEDP Algorithm Theory
- (1)
- The frequency domain signal obtained by frequency scanning is defined as x(m), where m = 1, 2, …, M, M is a positive integer, and the frequency coordinate is fi (i = 1,2, …, M). The height threshold and interval threshold are set when extracting the maximum point to filter the noise signal;
- (2)
- Traverse all discrete frequency points fi obtained in step (1), extract maximum points meeting the requirements, and record their frequency values in frequency coordinates;
- (3)
- Judge whether all the frequency values corresponding to the resonant maximum point are obtained. If so, continue to step (4); if not, consider the frequency coordinate fi+1 and return to step (2);
- (4)
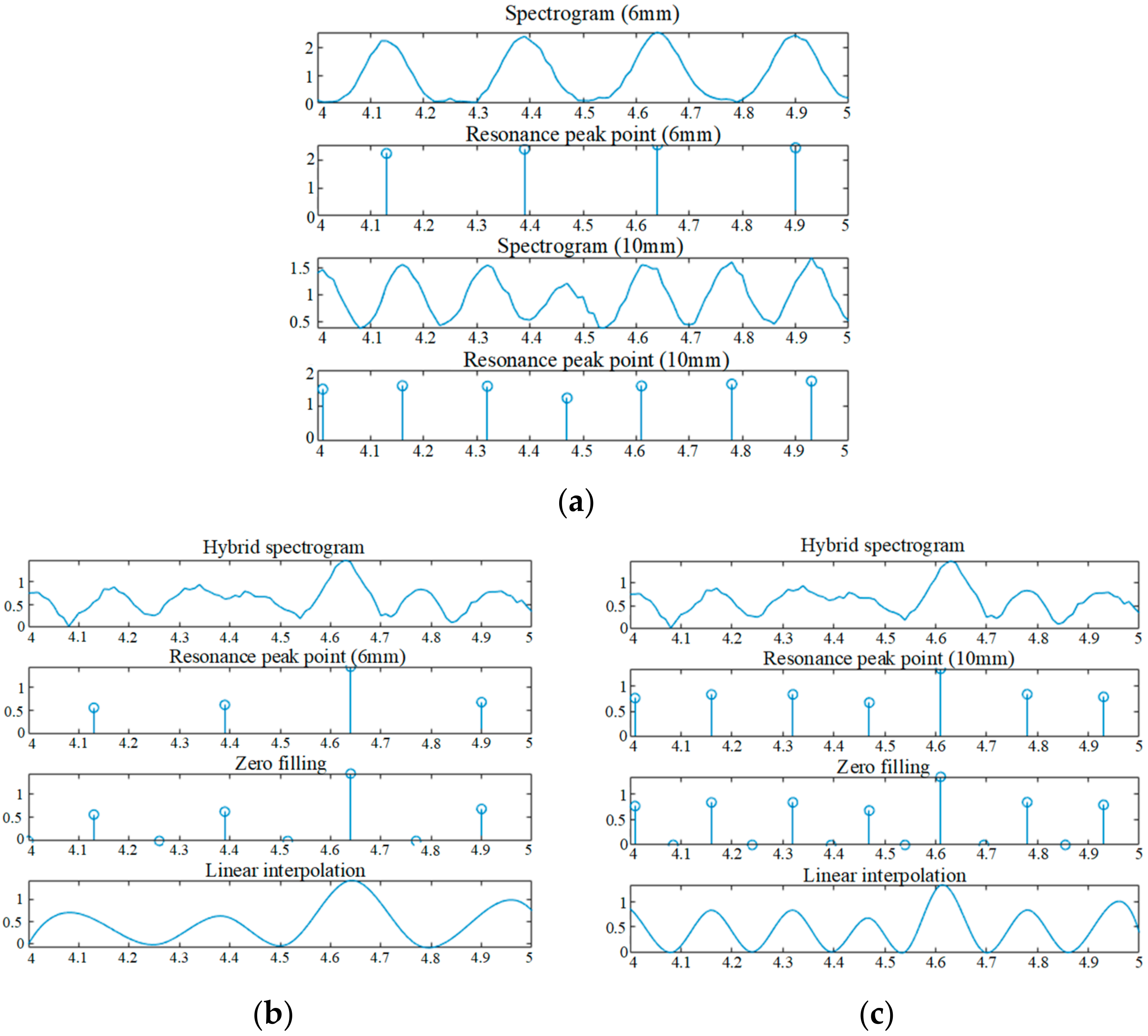
- Ensure that there are equal gaps between each frequency value in the record. If they are equal, it means that x(m) only contains information about one thickness; therefore, move on to step (6). If they differ, it means that x(m) contains information on two or more thicknesses; continue to step (5);
- (5)
- After extraction, the frequency values at the resonant sites are compared to those of standard specimens with specified thicknesses, and the resonant peak points belonging to various thicknesses are then separated;
- (6)
- Reconstruct the signal between the two groups of resonance maximum points that were previously separated, and define the separated frequency domain signal corresponding to the various thicknesses as yh (m), where h denotes the sequential number of various thicknesses;
- (7)
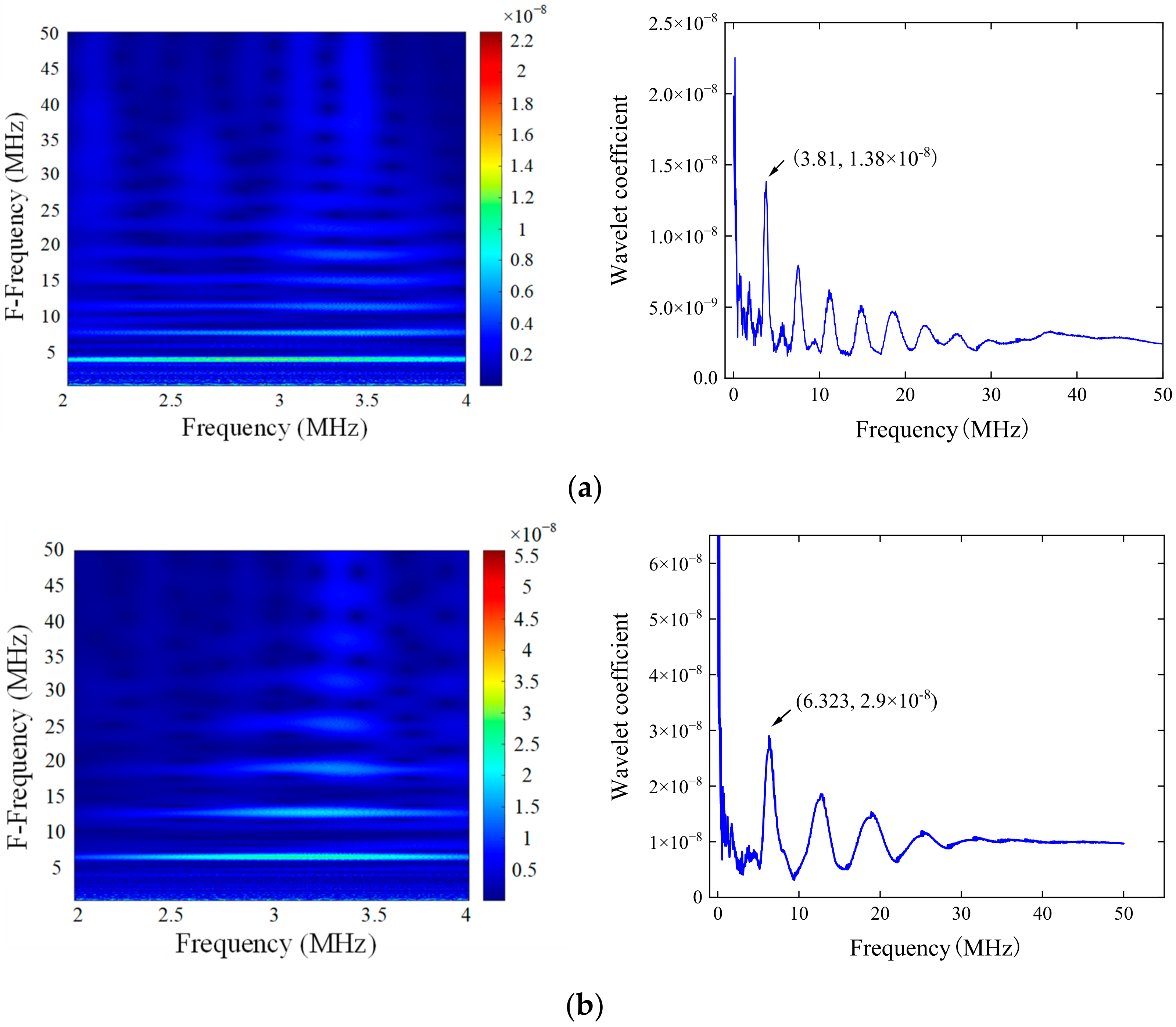
- Perform CWT to the decoupled signal yh from the previous step. The frequency of the decoupled signal is represented by the ordinate Fk (k = 1, 2, …, N), where N is a positive integer, and the abscissa fi (i = 1, 2, …, M) is produced from the transform, where M is a positive integer. E(fi, Fk) is the definition of the energy density at a collection of frequency points and F-Frequency points (fi, Fk) that have been confirmed. The CWT result of the frequency domain signal yh (m) can be expressed as follows [24]:
- (8)
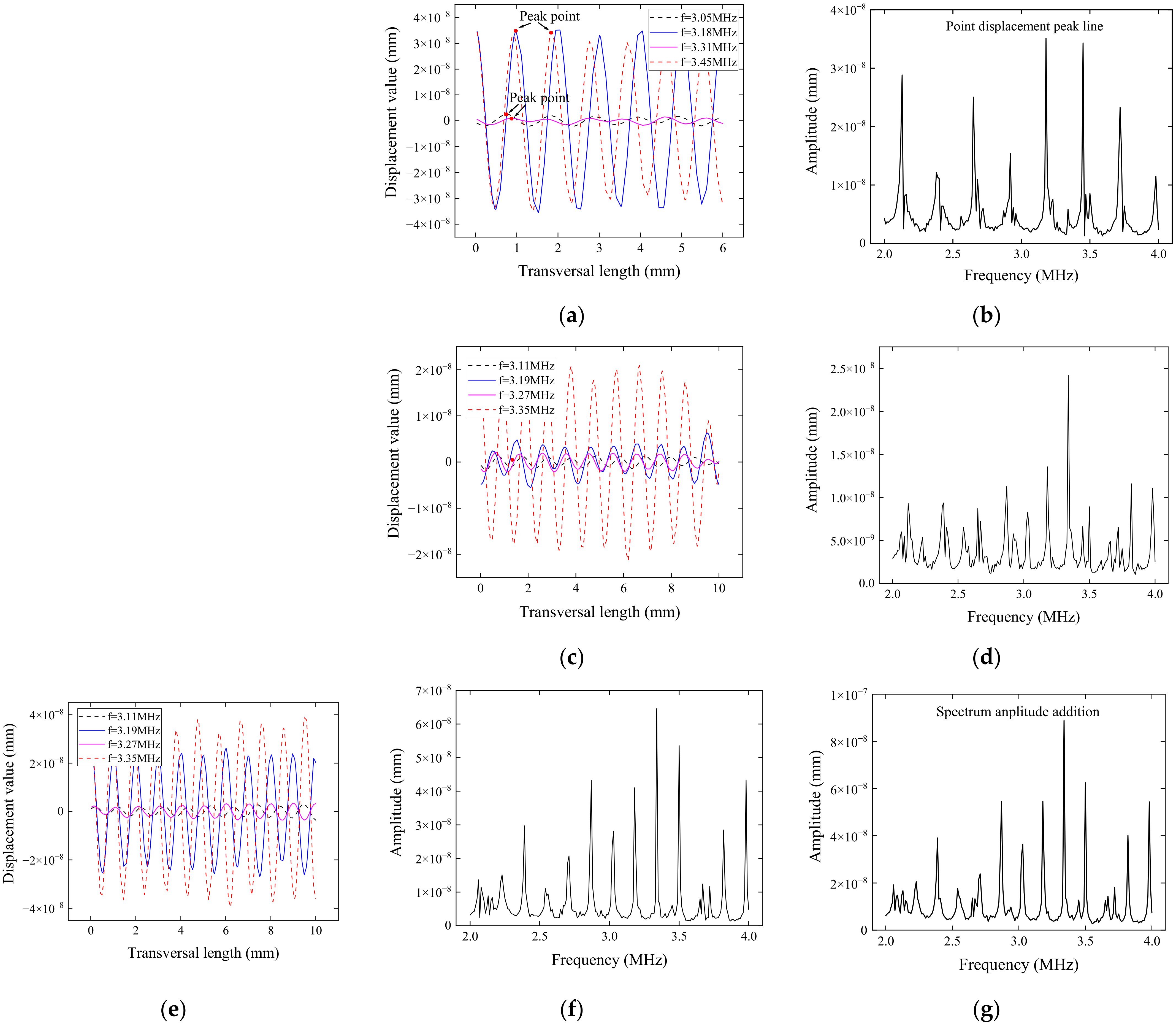
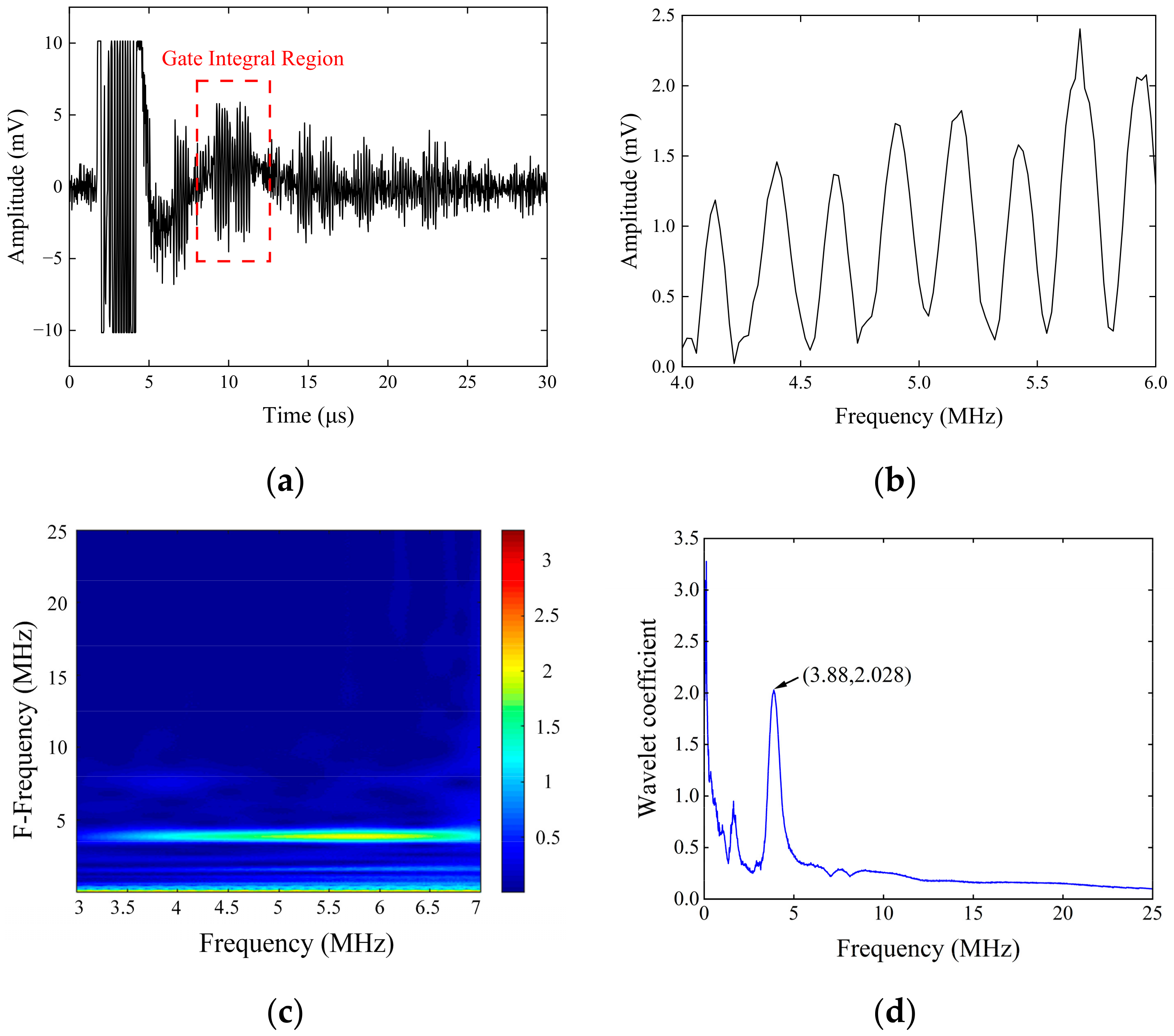
- For the F-Frequency energy density distribution data obtained in (7), the maximum F-Frequency energy density corresponding to each F-Frequency point Fk is extracted, and the distribution curve is drawn by fitting. According to the EMAR principle, the signal has the maximum F-Frequency energy density at 1/Δfh, and the reciprocal of the maximum value is the frequency interval Δf of the resonant spectrum of the original thickness;
- (9)
- Calculations are performed to determine the EDR on either side of the step surface, and the step surface is determined by examining the change in the proportion result.
3. Finite Element Simulation Analysis
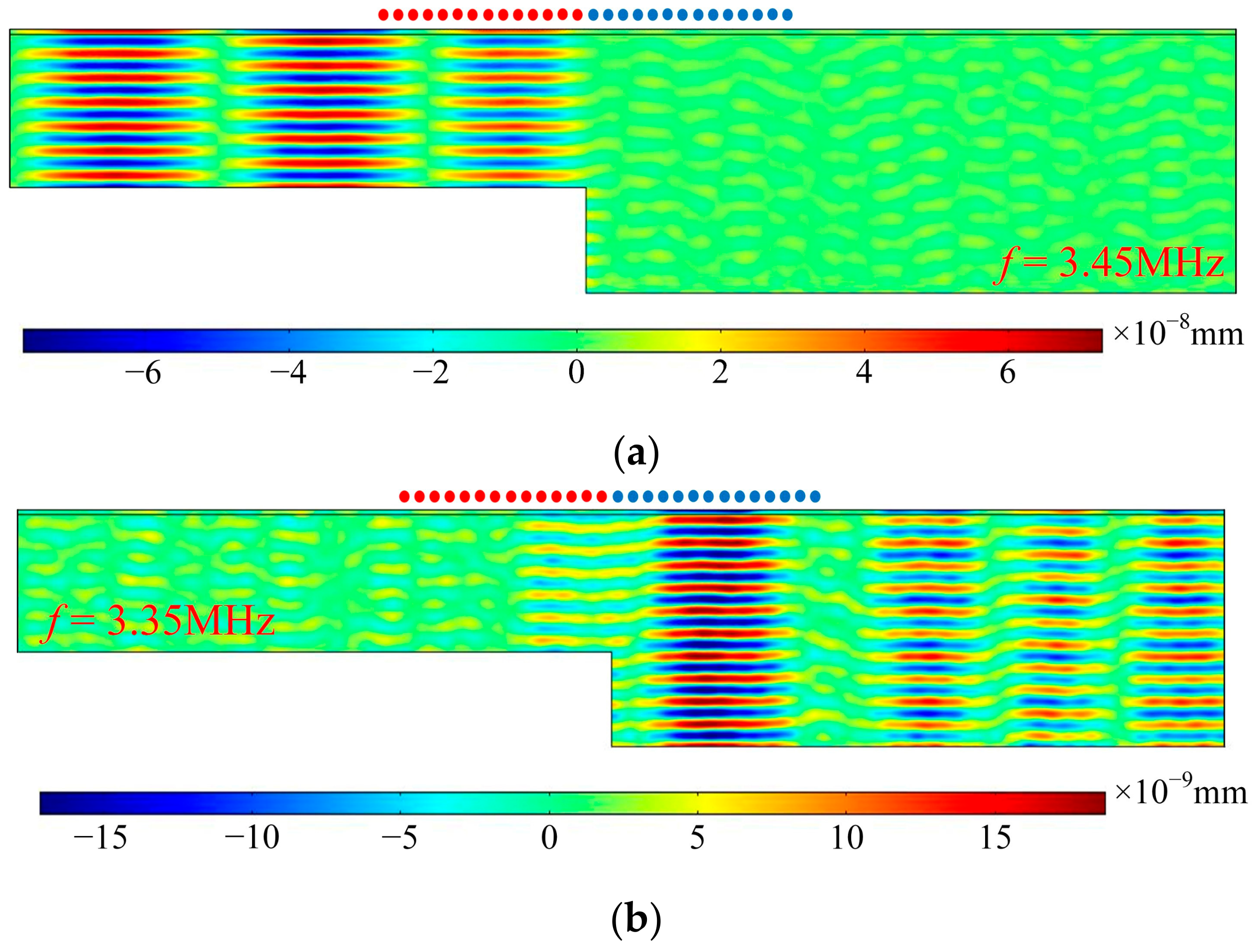
3.1. Method of Extracting Resonance Signals
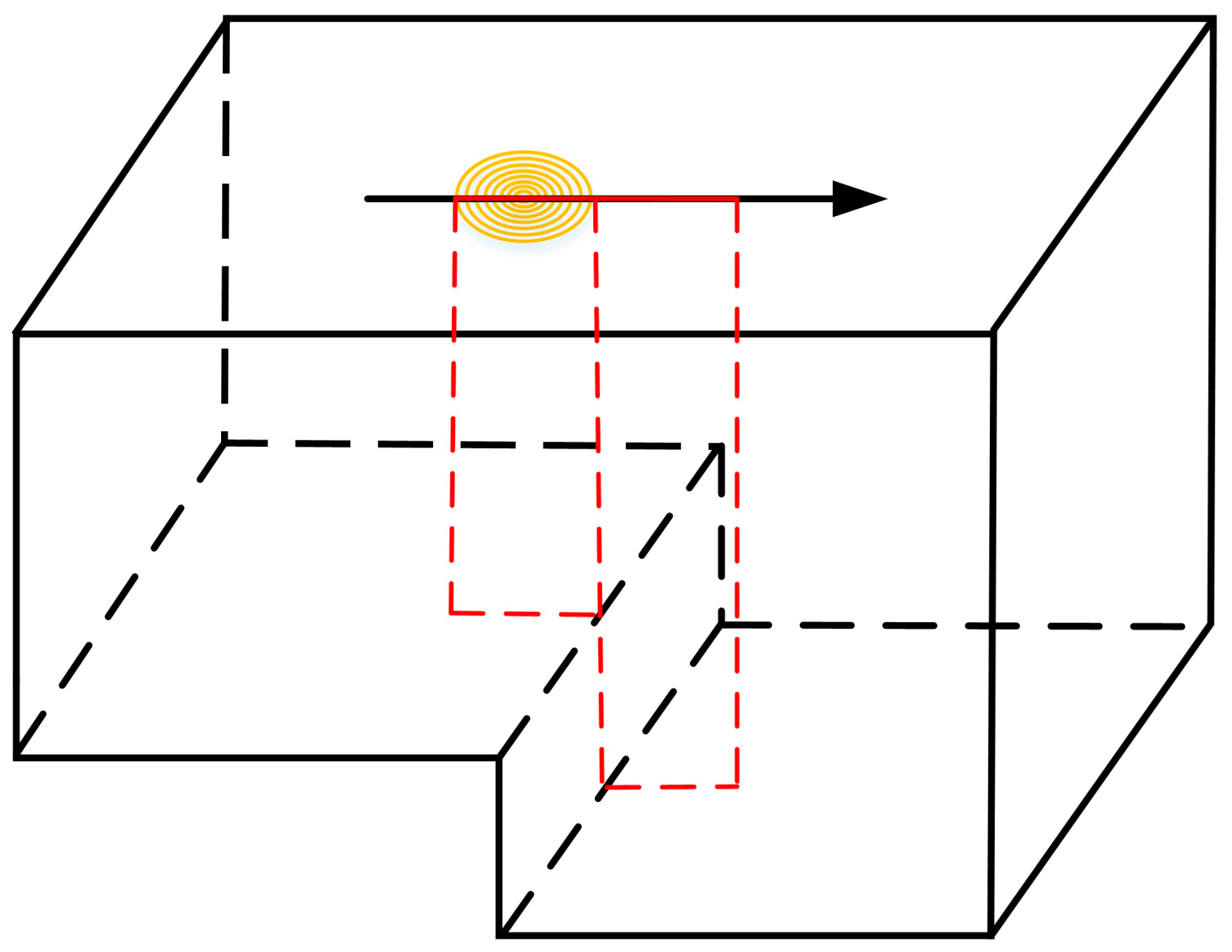
3.2. Moving Thickness Step Surface Scanning Method
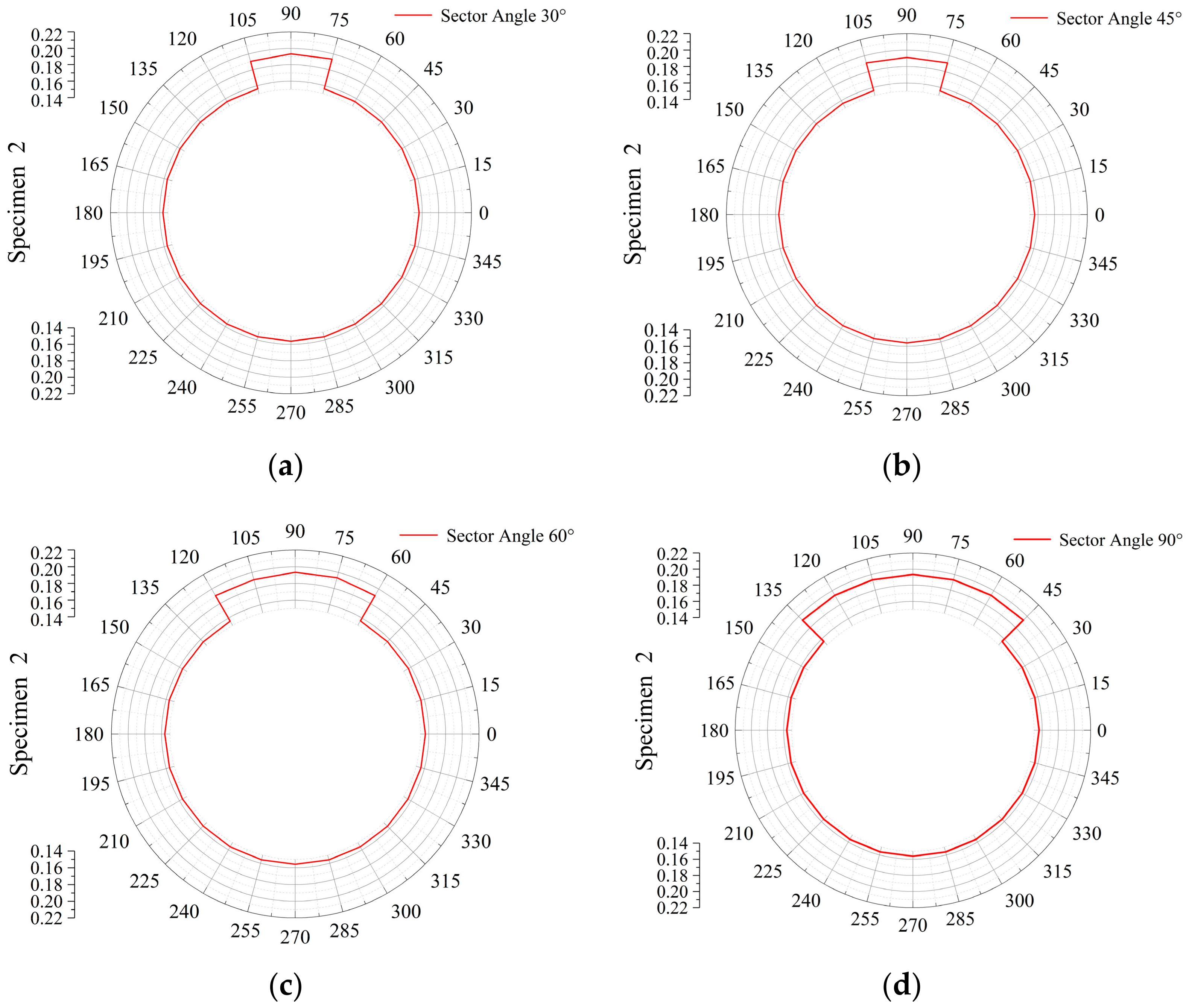
4. Experimental Results and Analysis
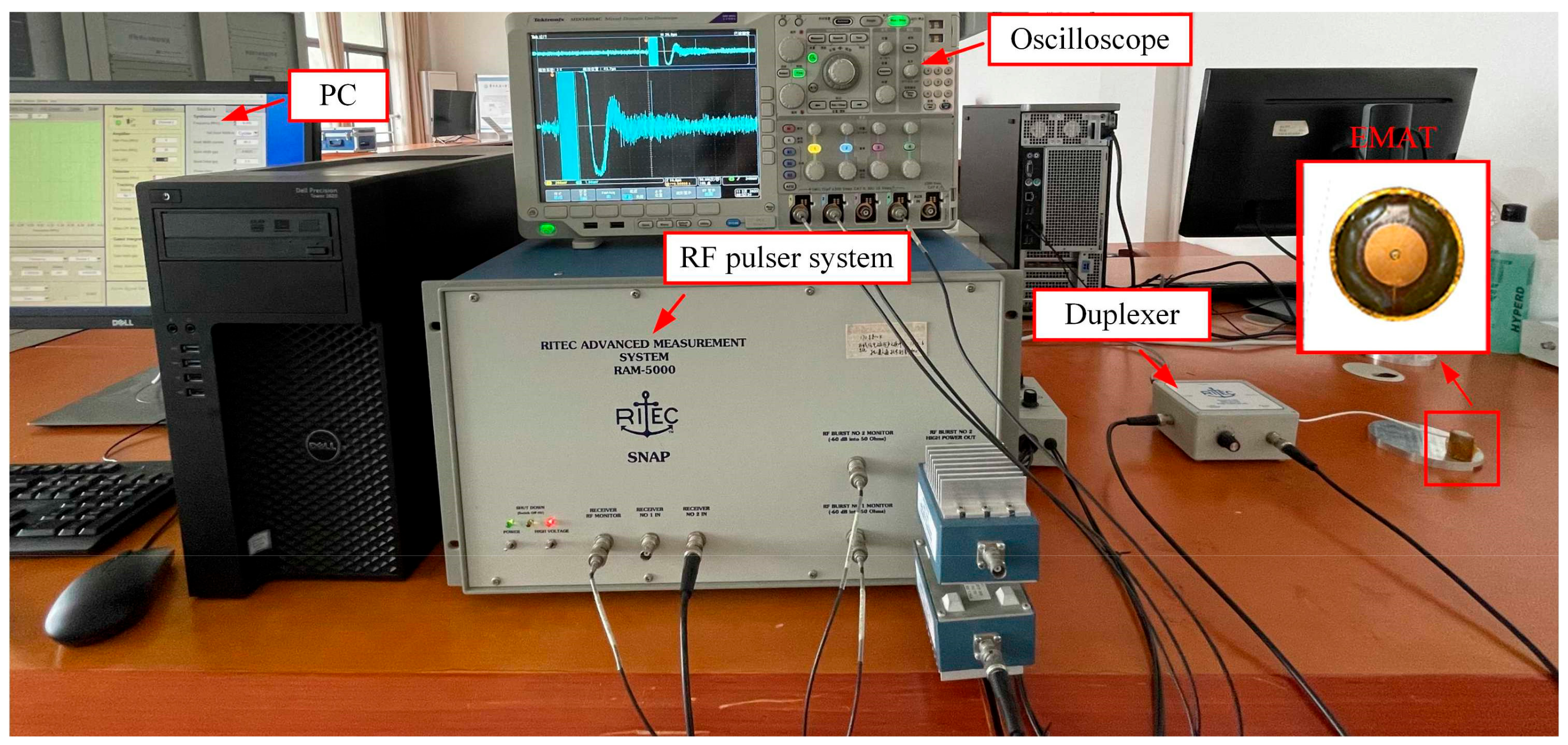
4.1. Experimental Platform
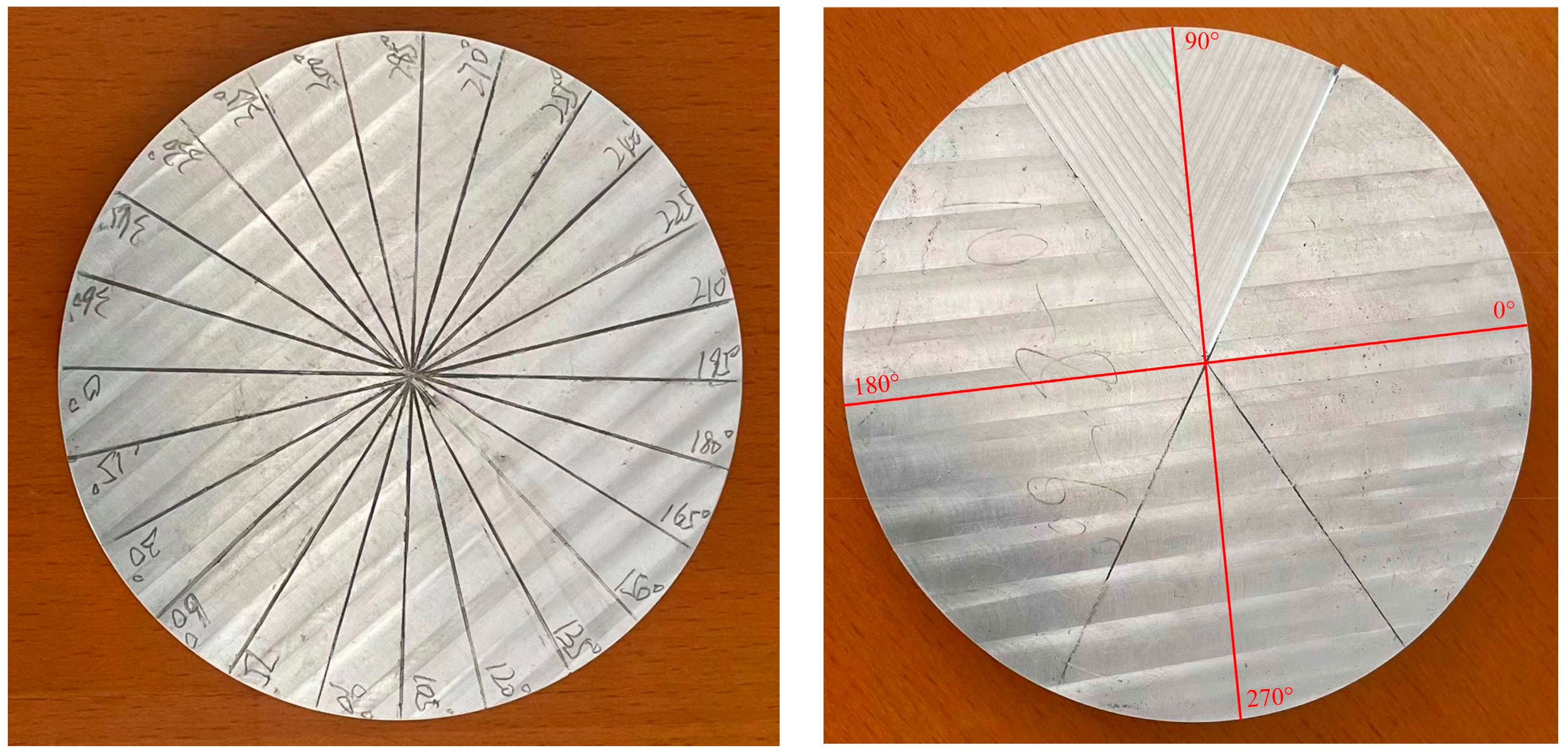
4.2. Step Specimen Moving Scan Scheme
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kasztankiewicz, A.; Gańczyk-Specjalska, K.; Zygmunt, A.; Cieślak, K.; Zakościelny, B.; Gołofit, T. Application and properties of aluminum in rocket propellants and pyrotechnics. J. Elem. 2018, 23, 321–331. [Google Scholar]
- Schubbe, J.J. Plate Thickness Variation Effects on Crack Growth Rates in 7050-T7451 Alloy Thick Plate. J. Mater. Eng. Perform. 2010, 20, 147–154. [Google Scholar] [CrossRef]
- Mihaljević, M.; Markučič, D.; Runje, B.; Keran, Z. Measurement uncertainty evaluation of ultrasonic wall thickness measurement. Measurement 2019, 137, 179–188. [Google Scholar] [CrossRef]
- Towsyfyan, H.; Biguri, A.; Boardman, R.; Blumensath, T. Successes and challenges in non-destructive testing of aircraft composite structures. Chin. J. Aeronaut. 2019, 33, 771–791. [Google Scholar] [CrossRef]
- Su, Z.; Ye, L.; Lu, Y. Guided Lamb waves for identification of damage in composite structures: A review. J. Sound Vib. 2006, 295, 753–780. [Google Scholar] [CrossRef]
- Dixon, S.; Edwards, C.; Palmer, S. High accuracy non-contact ultrasonic thickness gauging of aluminum sheet using electromagnetic acoustic transducers. Ultrasonics 2001, 39, 445–453. [Google Scholar] [CrossRef]
- Wu, Y.; Han, L.; Gong, H.; Yang, J.; Li, W. Effect of Coil Configuration on Conversion Efficiency of EMAT on 7050 Aluminum Alloy. Energies 2017, 10, 1496. [Google Scholar] [CrossRef]
- Ashigwuike, E.; Ushie, O.; Mackay, R.; Balachandran, W. A study of the transduction mechanisms of electromagnetic acoustic transducers (emats) on pipe steel materials. Sens. Actuators 2015, 229, 154–165. [Google Scholar] [CrossRef]
- Kim, Y.-K.; Park, I.-K. Evaluation of Thickness Reduction in an Aluminum Sheet using SH-EMAT. J. Korean Weld. Join. Soc. 2010, 28, 74–78. [Google Scholar] [CrossRef]
- Parra-Raad, J.; Khalili, P.; Cegla, F. Shear waves with orthogonal polarisations for thickness measurement and crack detection using emats. NDT E Int. 2020, 111, 102212. [Google Scholar] [CrossRef]
- Zhang, J.; Liu, M.; Jia, X.; Gao, R. Numerical Study and Optimal Design of the Butterfly Coil EMAT for Signal Amplitude Enhancement. Sensors 2022, 22, 4985. [Google Scholar] [CrossRef]
- Zhang, X.; Cheng, J.; Qiu, G.; Tu, J.; Song, X.; Cai, C. Shear Horizontal Circumferential Wave EMAT Design for Pipeline Inspection Based on FEM. Int. J. Appl. Electromagn. Mech. 2020, 64, 913–919. [Google Scholar] [CrossRef]
- Kang, L.; Zhang, C.; Dixon, S.; Zhao, H.; Hill, S.; Liu, M. Enhancement of ultrasonic signal using a new design of Rayleigh-wave electromagnetic acoustic transducer. NDT E Int. 2016, 86, 36–43. [Google Scholar] [CrossRef]
- Li, W.; Jiang, C.; Deng, M. Thermal damage assessment of metallic plates using a nonlinear electromagnetic acoustic resonance technique. NDT E Int. 2019, 108, 102172. [Google Scholar] [CrossRef]
- Cho, S.W.; Ji, B.; Cho, S.H. Detection of Microcrack on Thin Plates Using EMAT-Based Resonant Ultrasonic Spectroscopy. J. Korean Soc. Nondestruct. Test. 2019, 39, 1–6. [Google Scholar] [CrossRef]
- WLi, W.; Jiang, C.; Lan, Z.; Deng, M. Thermal Damage Evaluation in Nickel Plate by Nonlinear Electromagnetic Acoustic Resonance Technique. NDT E Int. 2019, 64, 835–842. [Google Scholar]
- Diguet, G.; Miyauchi, H.; Takeda, S.; Uchimoto, T.; Mary, N.; Takagi, T.; Abe, H. EMAR monitoring system applied to the thickness reduction of carbon steel in a corrosive environment. Mater. Corros. 2022, 73, 658–668. [Google Scholar] [CrossRef]
- Yusa, N.; Song, H.; Iwata, D.; Uchimoto, T.; Moroi, M. Probabilistic analysis of electromagnetic acoustic resonance signals for the detection of pipe wall thinning. Nondestruct. Test. Eval. 2019, 36, 1–16. [Google Scholar] [CrossRef]
- Liu, T.; Pei, C.; Cheng, X.; Zhou, H.; Xiao, P.; Chen, Z. Adhesive debonding inspection with a small EMAT in resonant mode. NDT E Int. 2018, 98, 110–116. [Google Scholar] [CrossRef]
- Li, Y.; Cai, Z.; Chen, L. Detection of Sloped Aluminum Plate Based on Electromagnetic Acoustic Resonance. IEEE Trans. Instrum. Meas. 2021, 71, 1–12. [Google Scholar] [CrossRef]
- Huang, S.; Sun, H.; Wang, S.; Qu, K.; Zhao, W.; Peng, L. SSWT and VMD Linked Mode Identification and Time-of-Flight Extraction of Denoised SH Guided Waves. IEEE Sens. J. 2021, 21, 14709–14717. [Google Scholar] [CrossRef]
- Ritec Inc. Operation Manual, Model Snap-0.25-7, Ritec Advanced Measurement System; Ritec Inc.: Warwick, RI, USA, 2002. [Google Scholar]
- Deng, M.; Wang, P.; Lv, X. Experimental observation of cumulative second-harmonic generation of Lamb-wave propagation in an elastic plate. J. Phys. D Appl. Phys. 2005, 38, 344–353. [Google Scholar] [CrossRef]
- Zhang, Y.; Huang, S.L.; Wang, S.; Zhao, W. Time-frequency energy density precipitation method for time-of-flight extraction of narrowband Lamb wave detection signals. Rev. Sci. Instrum. 2016, 87, 054702. [Google Scholar] [CrossRef] [PubMed]



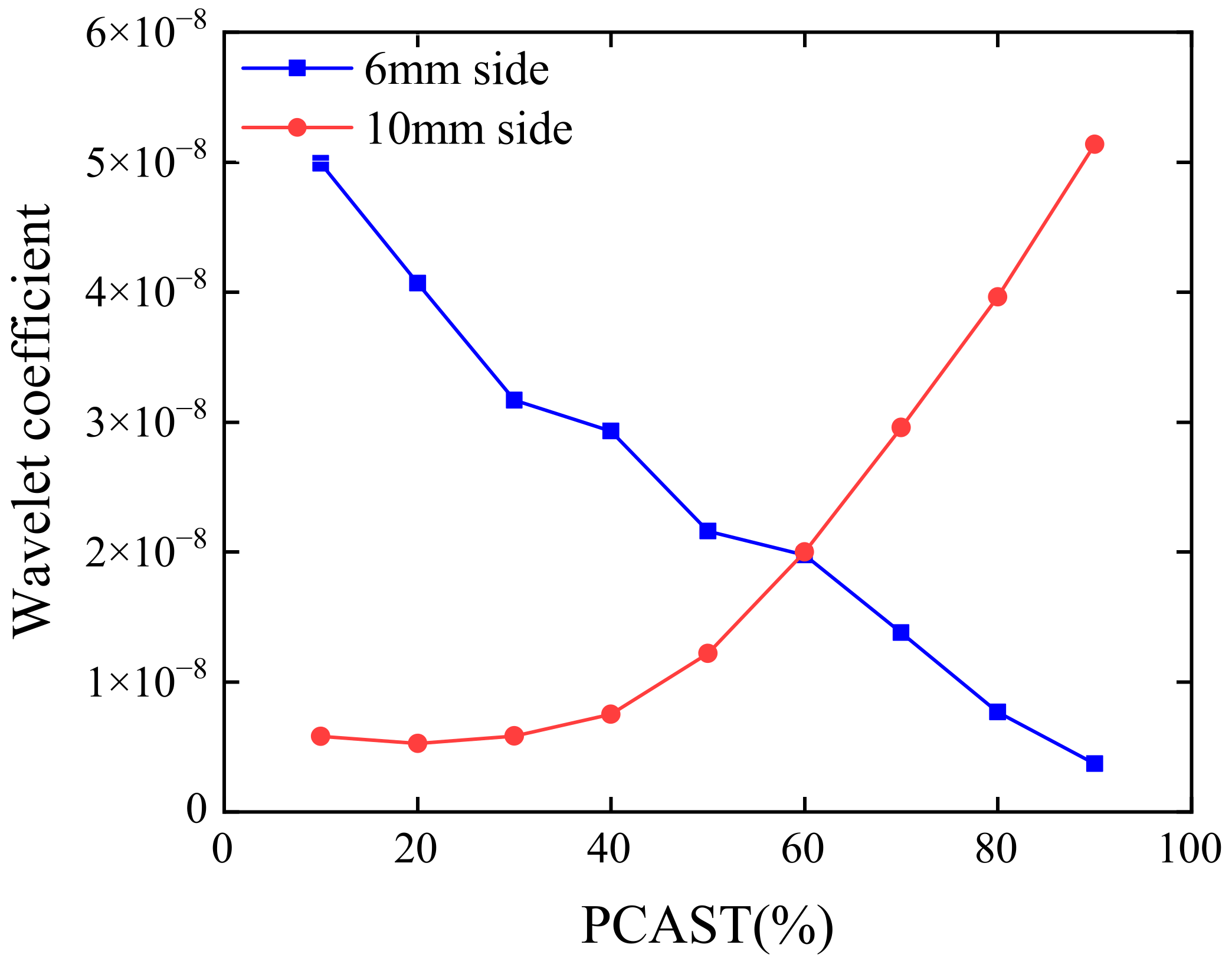
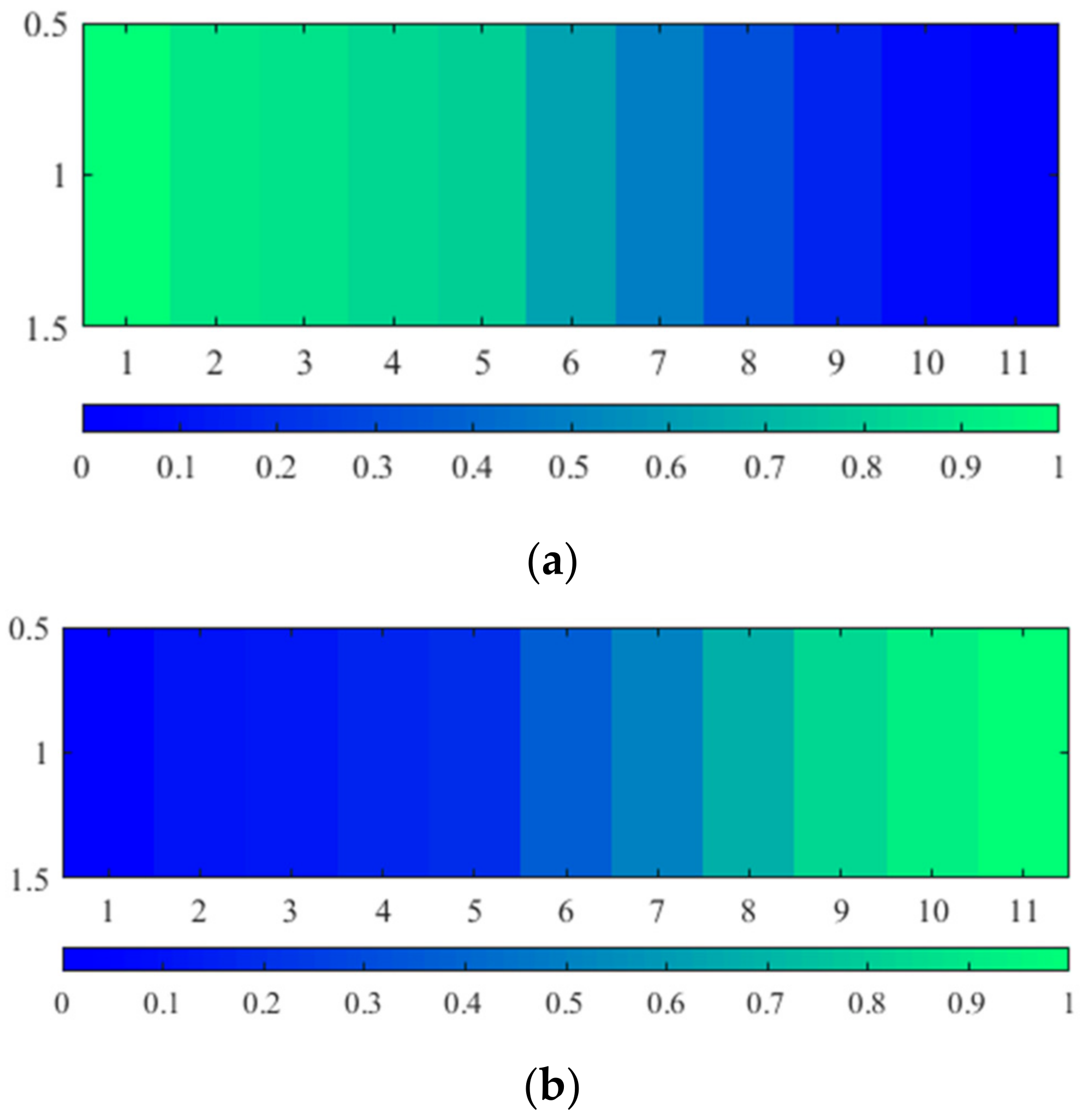



| APCIT | 0% | 10% | 20% | 30% | 40% | 50% | 60% | 70% | 80% | 90% | 100% | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| EDR | ||||||||||||
| A6 | 1 | 0.90 | 0.89 | 0.84 | 0.80 | 0.64 | 0.49 | 0.32 | 0.16 | 0.07 | 0 | |
| A10 | 0 | 0.10 | 0.11 | 0.16 | 0.20 | 0.36 | 0.51 | 0.68 | 0.84 | 0.93 | 1 | |
| Specimen Number | Large Thickness h1 | Small Thickness h2 | Sector Angle |
|---|---|---|---|
| Specimen 1 | 10 mm | 6 mm | 30°, 45°, 60°, 90° |
| Specimen 2 | 10 mm | 8 mm | 30°, 45°, 60°, 90° |
| APCIT | 0% | 10% | 20% | 30% | 40% | 50% | 60% | 70% | 80% | 90% | 100% | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| EDR | ||||||||||||
| A6 | 0 | 0.32 | 0.35 | 0.37 | 0.40 | 0.59 | 0.62 | 0.66 | 0.72 | 0.76 | 1 | |
| A10 | 1 | 0.68 | 0.65 | 0.63 | 0.60 | 0.41 | 0.38 | 0.34 | 0.28 | 0.24 | 0 | |
| APCIT | 0% | 10% | 20% | 30% | 40% | 50% | 60% | 70% | 80% | 90% | 100% | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| EDR | ||||||||||||
| A6 | 0 | 0.31 | 0.35 | 0.38 | 0.40 | 0.59 | 0.61 | 0.66 | 0.73 | 0.76 | 1 | |
| A10 | 1 | 0.69 | 0.65 | 0.62 | 0.60 | 0.41 | 0.39 | 0.34 | 0.27 | 0.24 | 0 | |
| EDR | APCIT | 0% | 10% | 20% | 30% | 40% | 50% | 60% | 70% | 80% | 90% | 100% | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| MD | |||||||||||||
| 10 mm to 8 mm | A8 | 0 | 0.33 | 0.34 | 0.36 | 0.38 | 0.46 | 0.56 | 0.63 | 0.67 | 0.69 | 1 | |
| A10 | 1 | 0.67 | 0.66 | 0.64 | 0.62 | 0.54 | 0.44 | 0.37 | 0.33 | 0.31 | 0 | ||
| 8 mm to 10 mm | A8 | 1 | 0.70 | 0.63 | 0.58 | 0.54 | 0.44 | 0.40 | 0.37 | 0.36 | 0.35 | 0 | |
| A10 | 0 | 0.30 | 0.37 | 0.42 | 0.46 | 0.56 | 0.60 | 0.63 | 0.64 | 0.65 | 1 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cai, Z.; Sun, Y.; Lu, Z.; Zhao, Q. Research on Identification and Detection of Aluminum Plate Thickness Step Change Based on Electromagnetic Acoustic Resonance. Magnetochemistry 2023, 9, 86. https://doi.org/10.3390/magnetochemistry9030086
Cai Z, Sun Y, Lu Z, Zhao Q. Research on Identification and Detection of Aluminum Plate Thickness Step Change Based on Electromagnetic Acoustic Resonance. Magnetochemistry. 2023; 9(3):86. https://doi.org/10.3390/magnetochemistry9030086
Chicago/Turabian StyleCai, Zhichao, Yihu Sun, Zhengshi Lu, and Qixiang Zhao. 2023. "Research on Identification and Detection of Aluminum Plate Thickness Step Change Based on Electromagnetic Acoustic Resonance" Magnetochemistry 9, no. 3: 86. https://doi.org/10.3390/magnetochemistry9030086
APA StyleCai, Z., Sun, Y., Lu, Z., & Zhao, Q. (2023). Research on Identification and Detection of Aluminum Plate Thickness Step Change Based on Electromagnetic Acoustic Resonance. Magnetochemistry, 9(3), 86. https://doi.org/10.3390/magnetochemistry9030086





