Dzyaloshinsky–Moriya Interaction Induced Anomalous g Behavior of Sr2IrO4 Probed by Electron Spin Resonance
Abstract
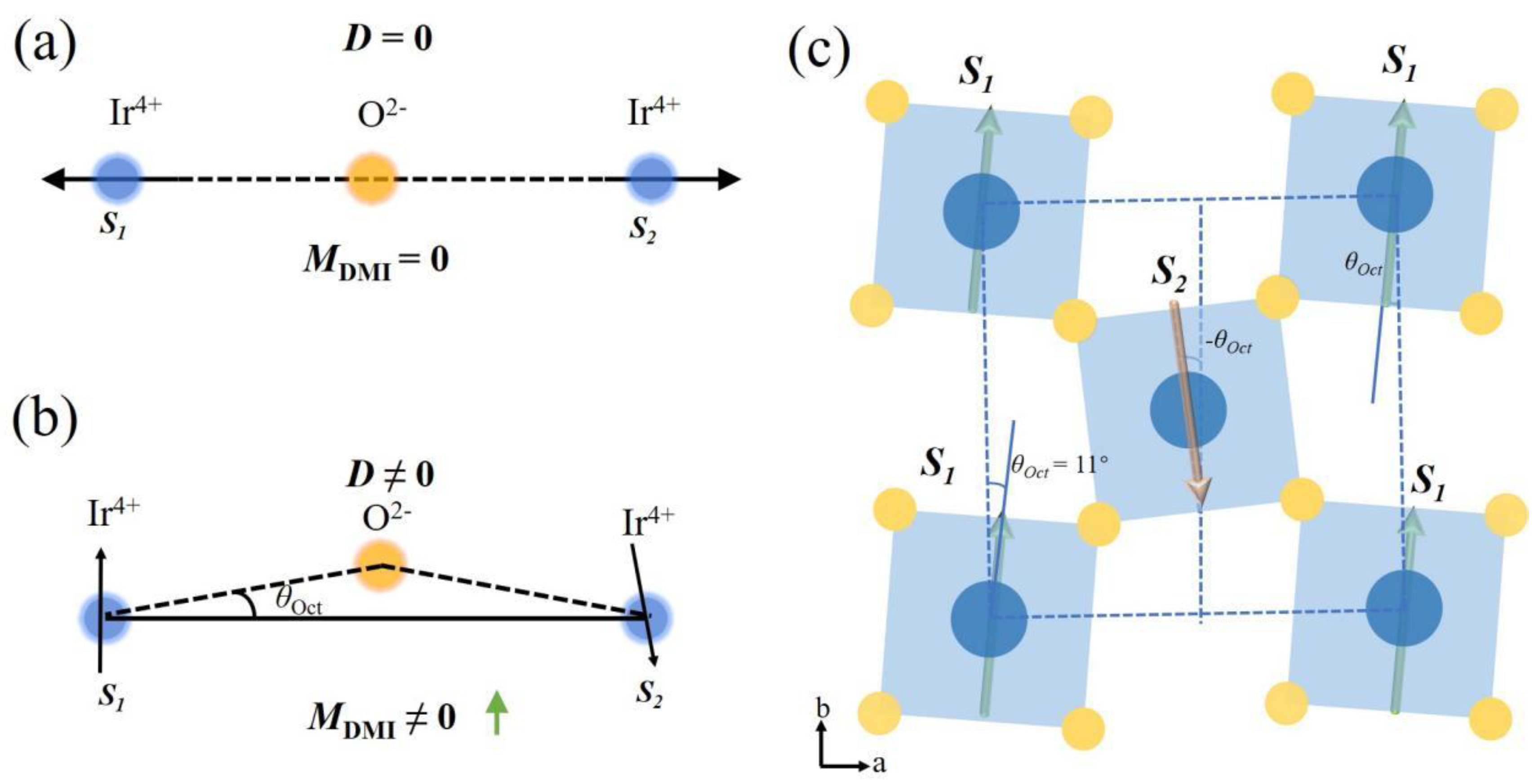
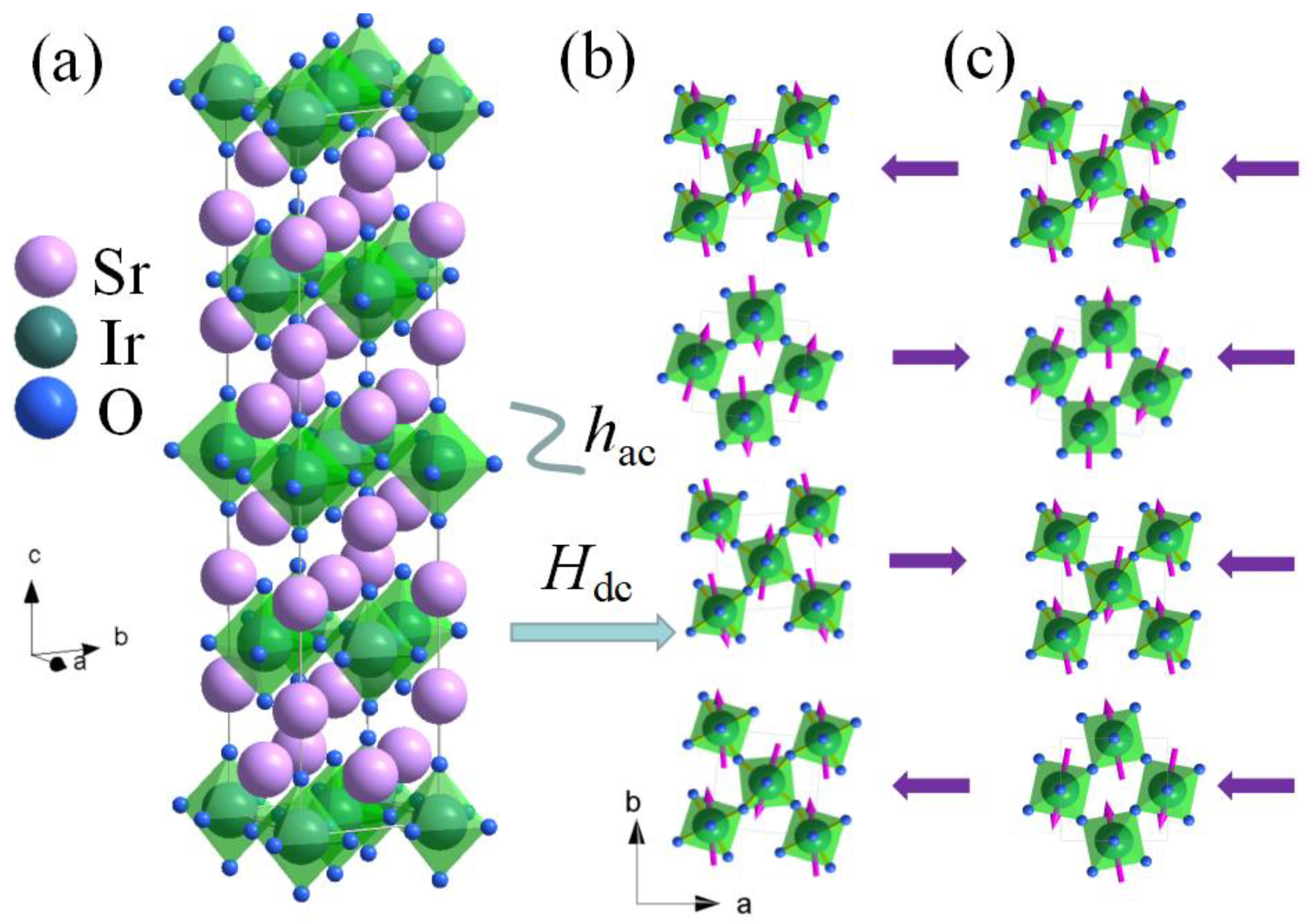
:1. Introduction
2. Experimental
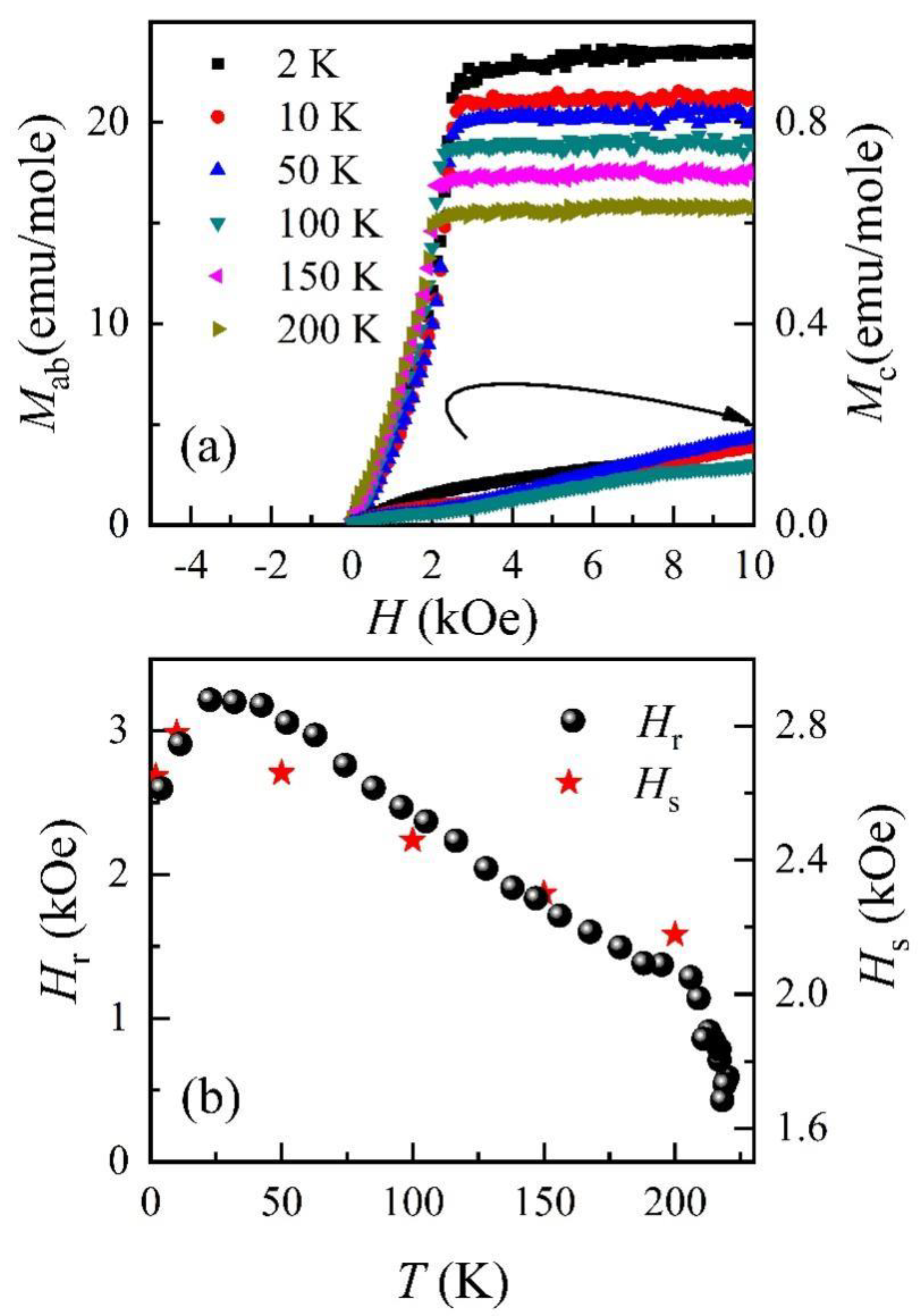
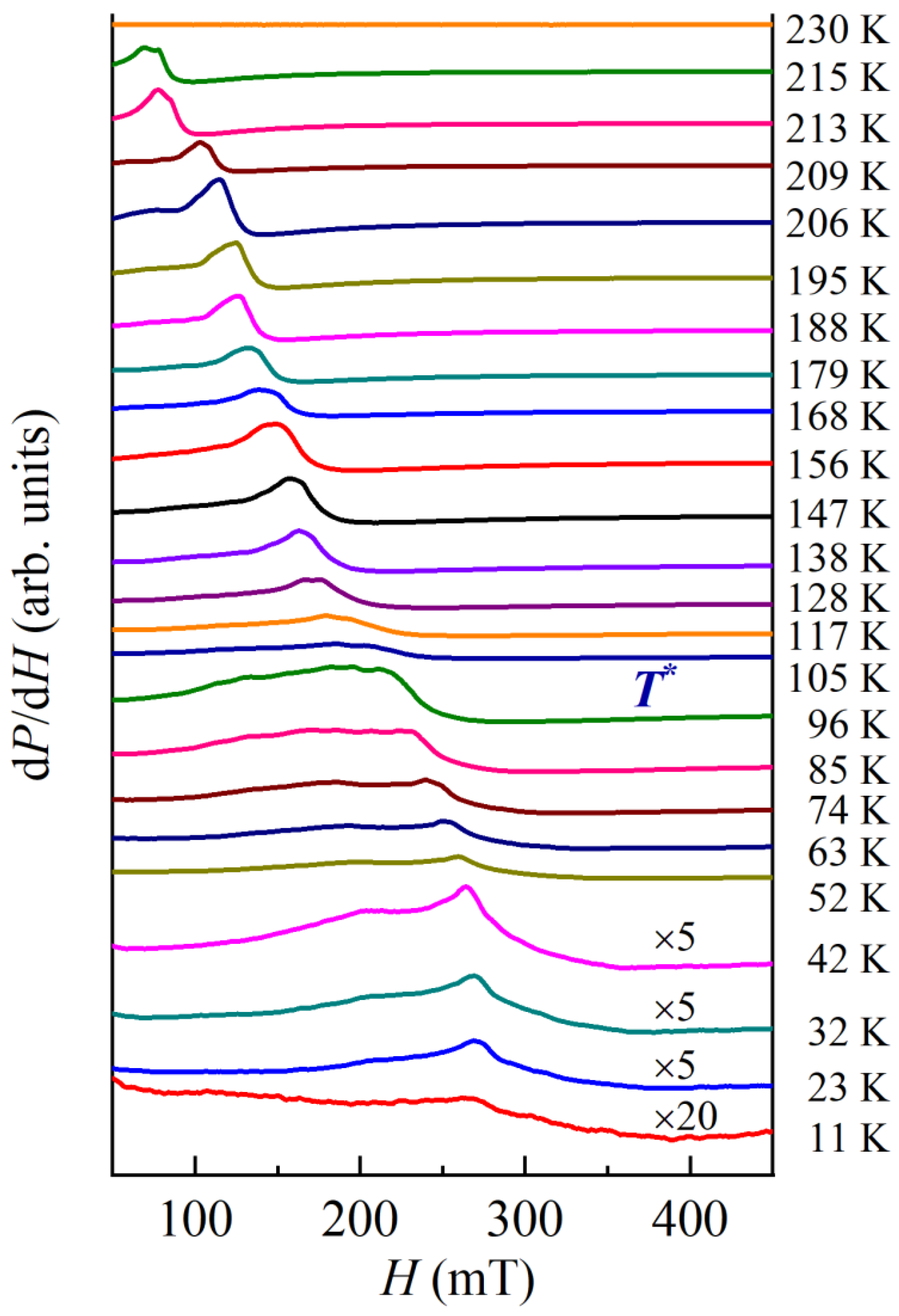
3. Results and Discussions
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Caretta, L.; Rosenberg, E.; Büttner, F.; Fakhrul, T.; Gargiani, P.; Valvidares, M.; Chen, Z.; Reddy, P.; Muller, D.A.; Ross, C.A.; et al. Interfacial Dzyaloshinskii-Moriya interaction arising from rare-earth orbital magnetism in insulating magnetic oxides. Nat. Commun. 2020, 11, 1090. [Google Scholar] [CrossRef] [PubMed]
- Ishii, K.; Jarrige, I.; Yoshida, M.; Ikeuchi, K.; Mizuki, J.; Ohashi, K.; Takayama, T.; Matsuno, J.; Takagi, H. Momentum-resolved electronic excitations in the Mott insulator Sr2IrO4 studied by resonant inelastic X-ray scattering. Phys. Rev. B 2011, 83, 115121. [Google Scholar] [CrossRef]
- Singh, V.; Pulikkotil, J. Evidence of Slater-type mechanism as origin of insulating state in Sr2IrO4. J. Phys. Condens. Matter 2019, 31, 425501. [Google Scholar] [CrossRef]
- Franke, I.; Baker, P.; Blundell, S.J.; Lancaster, T.; Hayes, W.; Pratt, F.L.; Cao, G. Measurement of the internal magnetic field in the correlated iridates Ca4IrO6, Ca5Ir3O12, Sr3Ir2O7 and Sr2IrO4. Phys. Rev. B 2011, 83, 094416. [Google Scholar] [CrossRef]
- Cetin, M.F.; Lemmens, P.; Gnezdilov, V.; Wulferding, D.; Menzel, D.; Takayama, T.; Ohashi, K.; Takagi, H. Crossover from coherent to incoherent scattering in spin-orbit dominated Sr2IrO4. Phys. Rev. B 2012, 85, 195148. [Google Scholar] [CrossRef]
- Priyamvada, J.; Leonard, F. The microscopic origin of DMI in magnetic bilayers and prediction of giant DMI in new bilayers. NPJ Comput. Mater. 2020, 6, 88. [Google Scholar]
- Jong, W.; Verstelle, J. Antisymmetric exchange interaction and spin-spin relaxation in CuCl2·2H2O. Phys. Lett. A 1972, 40, 403–404. [Google Scholar] [CrossRef]
- Liu, Q.; Kim, T.; Lee, K.; Yang, D.; Kumar, D.; Hu, F.; Yang, H. Dzyaloshinskii-Moriya Torque-Driven Resonance in Antiferromagnetic α-Fe2O3. Adv. Funct. Mater. 2023, 5, 1101–1105. [Google Scholar] [CrossRef]
- Velikov, L.; Rudashevskii, E. Antiferromagnetic resonance in hematite in the weakly ferromagnetic state. Sov. Phys. JETP 1969, 5, 836–839. [Google Scholar]
- Fink, H.; Shaltiel, D. High-Frequency Resonance of a Weak Ferromagnet: MnCO3. Phys. Rev. 1962, 12, 627–631. [Google Scholar] [CrossRef]
- Samuel, J.; Simon, F. Antiferromagnetic Resonance in Systems with Dzyaloshinsky-Moriya Coupling; Orientation Dependence in α-Fe2O3. Phys. Rev. 1964, 7, 136. [Google Scholar]
- Ge, M.; Tan, S.; Huang, Y.; Zhang, L.; Tong, W.; Pi, L.; Zhang, Y. Magnetism of insulator Sr2IrO4 with strong spin–orbit coupling. J. Magn. Magn. Mater. 2013, 345, 13–17. [Google Scholar] [CrossRef]
- Kim, B.J.; Ohsumi, H.; Komesu, T.; Sakai, S.; Morita, T.; Takagi, H.; Arima, T.H. Phase-sensitive observation of a spin-orbital Mott state in Sr2IrO4. Science 2009, 323, 1329–1332. [Google Scholar] [CrossRef]
- Hong, Y.; Jo, Y.; Choi, H.Y.; Lee, N.; Choi, Y.J.; Kang, W. Large magnetic anisotropy in canted antiferromagnetic Sr2IrO4 single crystals. Phys. Rev. B 2016, 93, 094406. [Google Scholar] [CrossRef]
- Cao, G.; Terzic, J.; Zhao, H.D.; Zheng, H.; De Long, L.E.; Riseborough, P.S. Electrical Control of Structural and Physical Properties via Strong Spin-Orbit Interactions in Sr2IrO4. Phys. Rev. Lett. 2018, 120, 017201. [Google Scholar] [CrossRef] [PubMed]
- Kim, B.J.; Jin, H.; Moon, S.J.; Kim, J.Y.; Park, B.G.; Leem, C.S.; Yu, J.; Noh, T.W.; Kim, C.; Oh, S.J.; et al. Novel Jeff = 1/2 Mott state induced by relativistic spin-orbit coupling in Sr2IrO4. Phys. Rev. Lett. 2008, 101, 076402. [Google Scholar]
- Fujiyama, S.; Ohsumi, H.; Komesu, T.; Matsuno, J.; Kim, B.J.; Takata, M.; Arima, T.; Takagi, H. Two-dimensional Heisenberg behavior of Jeff = 1/2 isospins in the paramagnetic state of the spin-orbital Mott insulator Sr2IrO4. Phys. Rev. Lett. 2012, 108, 247212. [Google Scholar] [CrossRef] [PubMed]
- Solovyev, I.V.; Mazurenko, V.V.; Katanin, A.A. Validity and limitations of the superexchange model for the magnetic properties of Sr2IrO4 and Ba2IrO4 mediated by the strong spin-orbit coupling. Phys. Rev. B 2015, 92, 235109. [Google Scholar] [CrossRef]
- Zorko, A.; Nellutla, J.; Brunel, C.; Brunel, L.C.; Bert, F.; Duc, F.; Trombe, J.C.; De Vries, M.A.; Harrison, A.; Mendels, P. Dzyaloshinsky-Moriya anisotropy in the spin-1/2 kagome compound ZnCu3(OH)6Cl2. Phys. Rev. Lett. 2008, 101, 026405. [Google Scholar] [CrossRef] [PubMed]
- Schweflinghaus, B.; Zimmermann, M.; Heide, G.; Bihlmayer, G.; Blügel, S. Role of Dzyaloshinskii-Moriya interaction for magnetism in transition-metal chains at Pt-step edges. Phys. Rev. B 2016, 94, 024403. [Google Scholar] [CrossRef]
- Wei, W.S.; He, Z.D.; Qu, Z.; Du, H.F. Dzyaloshinsky–Moriya interaction (DMI)-induced magnetic skyrmion materials. Rare Met. 2021, 40, 3076–3090. [Google Scholar] [CrossRef]
- Li, L.; Yan, L.Q.; Shi, Y.; Lu, P.; Sun, Y. Evidence and evolution of magnetic polaron in HgCr2Se4 investigated by electron spin resonance. J. Phys. Condens. Matter 2018, 30, 255804. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Yao, L.; Yao, J.; Zhu, W.; Zhang, C. Decreased Energy Gap and Enhanced Conductivity in Zn-Doped Sr2IrO4. J. Supercond. Nov. Magn. 2018, 32, 1583–1587. [Google Scholar] [CrossRef]
- Crawford, M.K.; Subramanian, M.A.; Harlow, R.L.; Fernandez-Baca, J.A.; Wang, Z.R.; Johnston, D.C. Structural and magnetic studies of Sr2IrO4. Phys. Rev. B 1994, 49, 19198–19201. [Google Scholar] [CrossRef] [PubMed]
- Sudipta, M.; Topwal, D. Complex spin glass behavior in Ga2−xFexO3. Appl. Phys. Lett. 2017, 110, 102907. [Google Scholar]
- Kanti, T.; Moessner, R. Dipolar spin glass transition in three dimensions. Phys. Rev. B 2019, 100, 064425. [Google Scholar]
- Bhatti, N.; Rawat, R.; Banerjee, A.; Pramanik, A.K. Temperature evolution of magnetic and transport behavior in 5d Mott insulator Sr2IrO4, significance of magneto-structural coupling. J. Phys. Condens. Matter 2014, 27, 016005. [Google Scholar] [CrossRef] [PubMed]
- Cao, G.; Bolivar, J.; McCall, S.; Crow, J.E.; Guertin, R.P. Weak ferromagnetism, metal-to-nonmetal transition and negative differential resistivity in single-crystal Sr2IrO4. Phys. Rev. B 1998, 57, R11039. [Google Scholar] [CrossRef]
- Wang, C.; Seinige, H.; Cao, G.; Zhou, J.S.; Goodenough, J.B.; Tsoi, M. Temperature dependence of anisotropic magnetoresistance in antiferromagnetic Sr2IrO4. J. Appl. Phys. 2015, 117, 17A310. [Google Scholar] [CrossRef]
- Ge, M.; Zhang, L.; Fan, J.; Zhang, C.; Pi, L.; Tan, S.; Zhang, Y. Critical behavior of the in-plane weak ferromagnet Sr2IrO4. Solid State Commun. 2013, 166, 60–65. [Google Scholar] [CrossRef]
- Feher, G.; Kip, A.F. Electron Spin Resonance Absorption in Metals. Phys. Rev. 1955, 98, 337–348. [Google Scholar] [CrossRef]
- Joh, K.W.; Lee, C.H.; Lee, C.E.; Jeong, Y.H. Origin of electron spin resonance in La0.7Ca0.3MnO3. Phys. Status Solidi (b) 2003, 239, 452–456. [Google Scholar] [CrossRef]
- Wei, N. Electron Spin Resonance Studies of Chalcogenide Manganese Oxides; Institute of Physics, Chinese Academy of Sciences: Beijing, China, 2008; Volume 5, pp. 30–100. [Google Scholar]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, K.; Yan, L.; Shi, Y.; Shen, B.; He, L.; Wang, F.; Lu, J.; Zhao, T.; Lu, Z. Dzyaloshinsky–Moriya Interaction Induced Anomalous g Behavior of Sr2IrO4 Probed by Electron Spin Resonance. Magnetochemistry 2023, 9, 231. https://doi.org/10.3390/magnetochemistry9110231
Wang K, Yan L, Shi Y, Shen B, He L, Wang F, Lu J, Zhao T, Lu Z. Dzyaloshinsky–Moriya Interaction Induced Anomalous g Behavior of Sr2IrO4 Probed by Electron Spin Resonance. Magnetochemistry. 2023; 9(11):231. https://doi.org/10.3390/magnetochemistry9110231
Chicago/Turabian StyleWang, Kai, Liqin Yan, Youguo Shi, Baogen Shen, Lunhua He, Fangwei Wang, Jun Lu, Tongyun Zhao, and Zunming Lu. 2023. "Dzyaloshinsky–Moriya Interaction Induced Anomalous g Behavior of Sr2IrO4 Probed by Electron Spin Resonance" Magnetochemistry 9, no. 11: 231. https://doi.org/10.3390/magnetochemistry9110231
APA StyleWang, K., Yan, L., Shi, Y., Shen, B., He, L., Wang, F., Lu, J., Zhao, T., & Lu, Z. (2023). Dzyaloshinsky–Moriya Interaction Induced Anomalous g Behavior of Sr2IrO4 Probed by Electron Spin Resonance. Magnetochemistry, 9(11), 231. https://doi.org/10.3390/magnetochemistry9110231






