Superparamagnetism of Artificial Glasses Based on Rocks: Experimental Data and Theoretical Modeling
Abstract
:1. Introduction
2. Materials and Methods
2.1. Sample Preparation
2.2. Methods for Physical, Chemical, and Magnetic Characterization
3. Results and Discussion
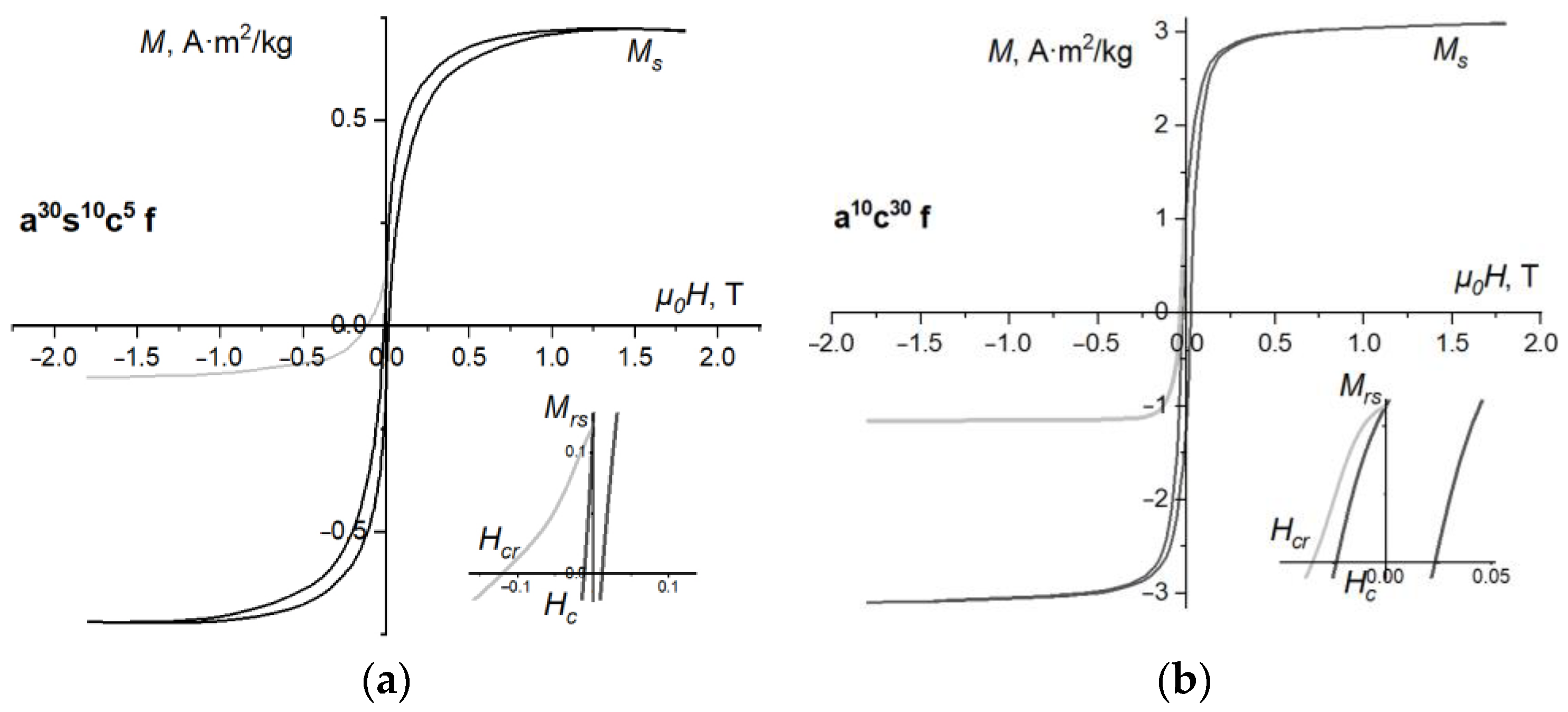
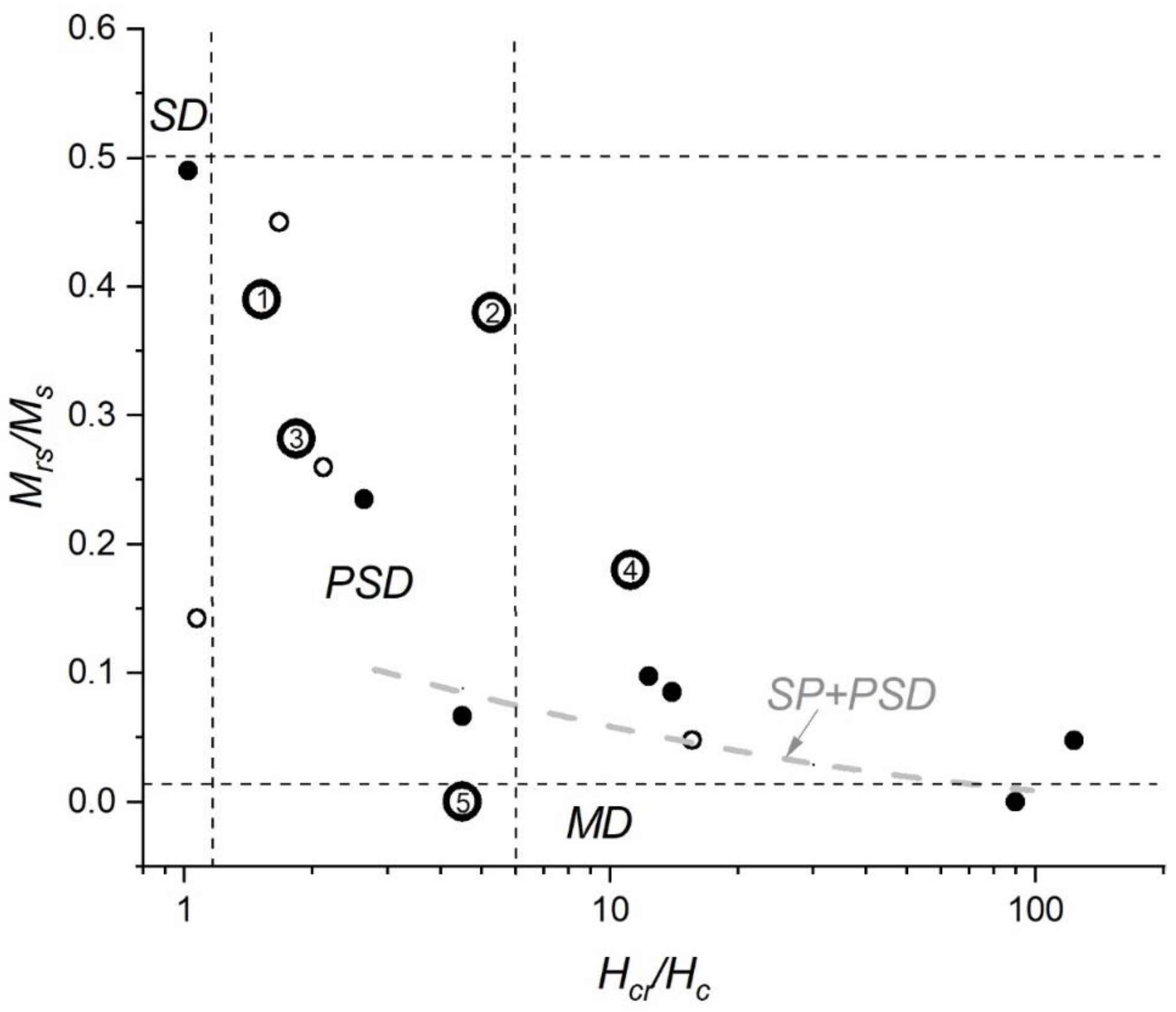
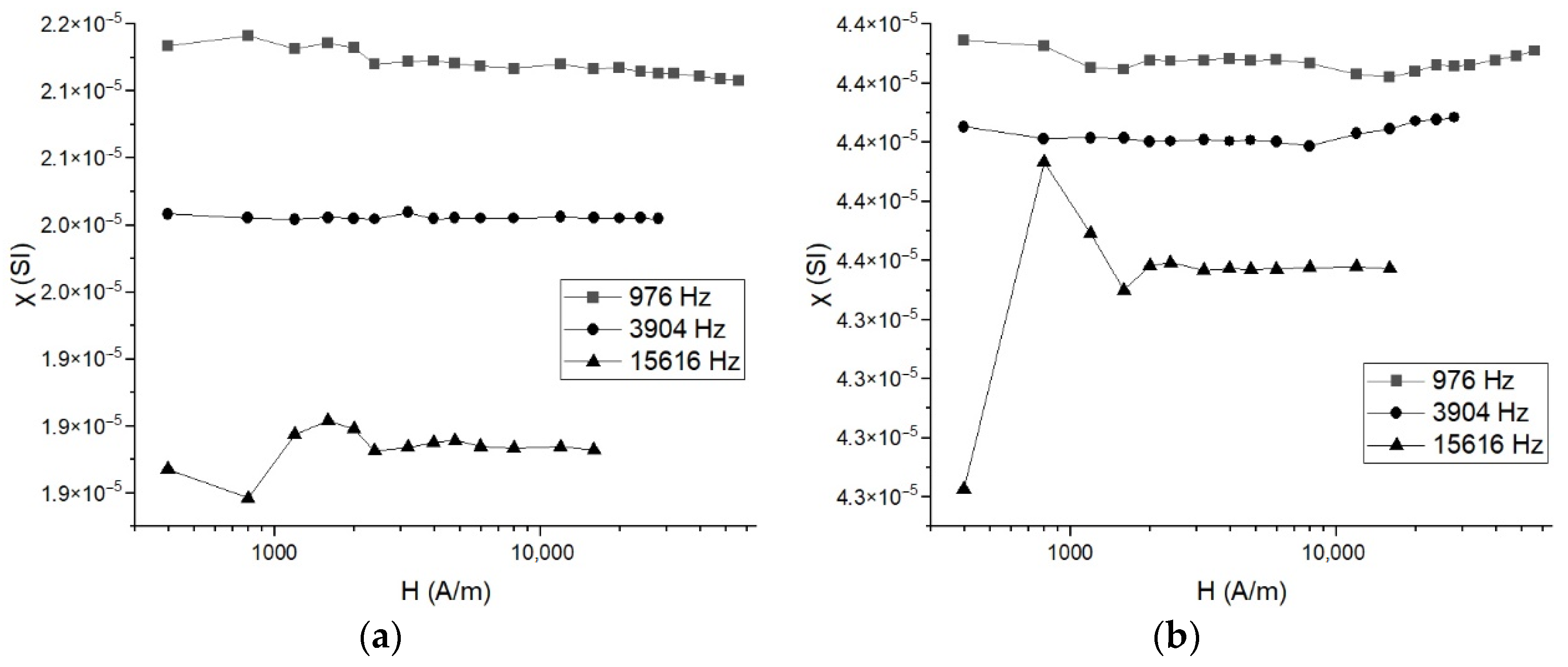
3.1. Magnetic Properties
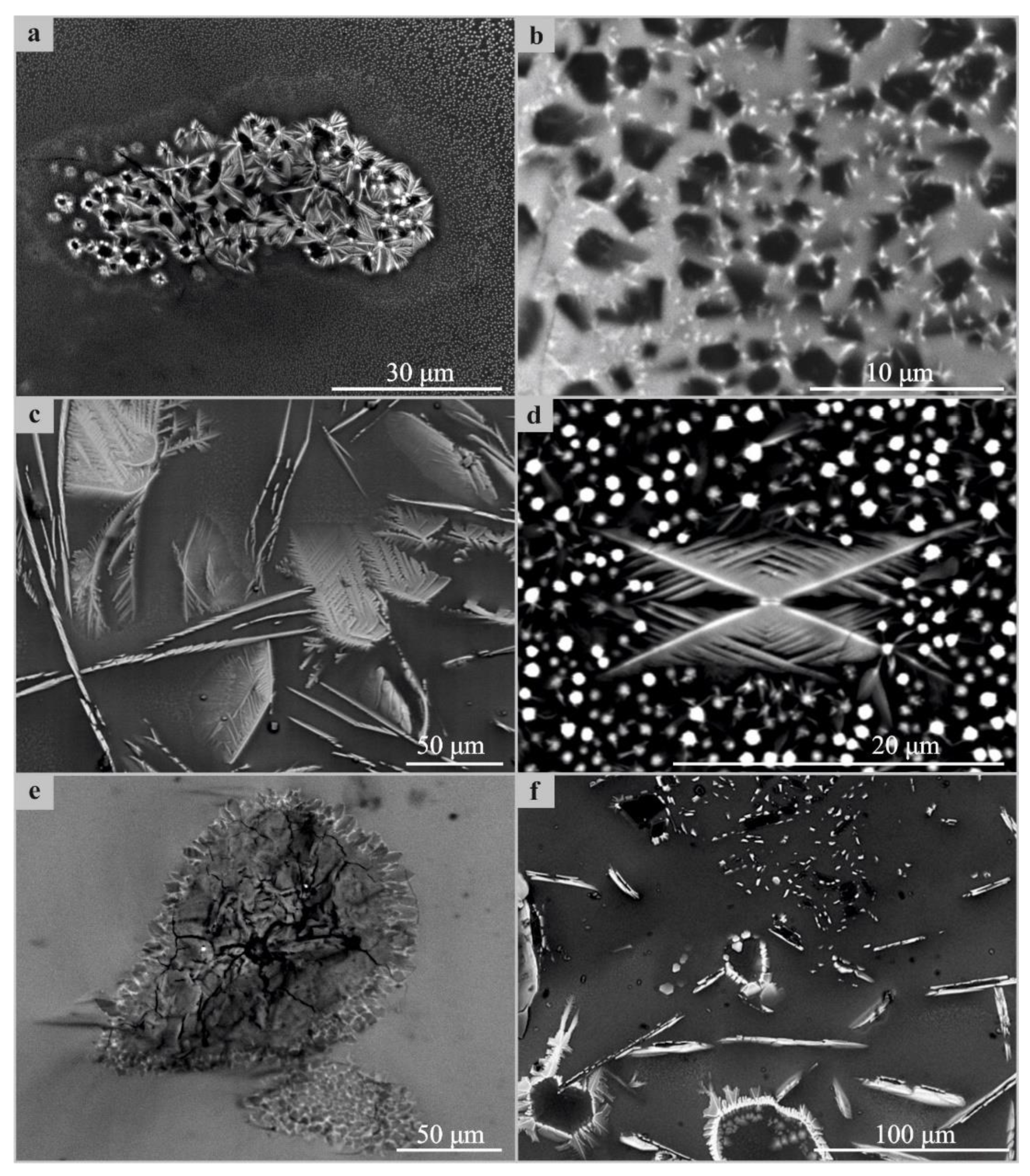
3.2. Morphology and Composition
3.3. Mössbauer Spectroscopy
3.4. Electron Spin Resonance
4. Theoretical Modeling
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A

References
- Dressler, B.O.; Reimold, W.U. Terrestrial impact melt rocks and glasses. Earth-Science Rev. 2001, 56, 205–284. [Google Scholar] [CrossRef]
- De Graaff, S.J.; Kaskes, P.; Déhais, T.; Goderis, S.; Debaille, V.; Ross, C.H.; Gulick, S.P.S.; Feignon, J.G.; Ferrière, L.; Koeberl, C.; et al. New insights into the formation and emplacement of impact melt rocks within the Chicxulub impact structure, following the 2016 IODP-ICDP Expedition 364. Bull. Geol. Soc. Am. 2022, 134, 293–315. [Google Scholar] [CrossRef]
- Feignon, J.G.; Schulz, T.; Ferrière, L.; Goderis, S.; de Graaff, S.J.; Kaskes, P.; Déhais, T.; Claeys, P.; Koeberl, C. Search for a meteoritic component within the impact melt rocks of the Chicxulub impact structure peak ring, Mexico. Geochim. Cosmochim. Acta 2022, 323, 74–101. [Google Scholar] [CrossRef]
- Bogatikov, O.A.; Petrov, O.V.; Sharpenok, L.N. Petrographic Code of Russia. Igneous, Metamorphic, Metasomatic, Impact Formations; Russian Geological Research Institute: Saint Petersburg, Russia, 2008; ISBN 9785937611062. (In Russian) [Google Scholar]
- Melosh, H.J. Impact Cratering: A Geologic Process; Oxford University Press: Oxford, UK, 1996; ISBN 0195104633. [Google Scholar]
- Batanova, A.M.; Gramenitsky, E.N.; Kotelnikov, A.R.; Plechov, P.Y.; Shchekina, T.I. Experimental and Technical Petrology; Scientific World: Moscow, Russia, 2000; ISBN 5891761203. (In Russian) [Google Scholar]
- Duchkov, A.D. Laboratory modeling of hydrate formation in rock specimens (a review). Russ. Geol. Geophys. 2017, 58, 240–252. [Google Scholar] [CrossRef]
- Wawrzyniak-Guz, K. Rock physics modelling for determination of effective elastic properties of the lower Paleozoic shale formation, North Poland. Acta Geophys. 2019, 67, 1967–1989. [Google Scholar] [CrossRef]
- Okunevich, V.S.; Bayuk, I.O. Petrophysical modeling of rocks dominik formation as the basis of interpretation of seismic data. Mosc. Univ. Bull. Ser. 4 Geol. 2022, 4, 149–156. [Google Scholar] [CrossRef]
- Fomichev, S.V.; Babievskaya, I.Z.; Dergacheva, N.P.; Noskova, O.A.; Krenev, V.A. Criteria for assessing technological properties of gabbro-basalt rocks. Theor. Found. Chem. Eng. 2012, 46, 424–428. [Google Scholar] [CrossRef]
- Krenev, V.A.; Pechenkina, E.N.; Fomichev, S.V. Gabbro–Basalt Raw Materials of Russia: Mineral Composition, Modification Methods, and Complex Use. Russ. J. Inorg. Chem. 2021, 66, 253–257. [Google Scholar] [CrossRef]
- Khater, G.A.; Abdel-Motelib, A.; El Manawi, A.W.; Abu Safiah, M.O. Glass-ceramics materials from basaltic rocks and some industrial waste. J. Non. Cryst. Solids 2012, 358, 1128–1134. [Google Scholar] [CrossRef]
- Khater, G.A.; Mahmoud, M.A. Preparation and characterization of nucleated glass-ceramics based on basaltic rocks. J. Aust. Ceram. Soc. 2017, 53, 433–441. [Google Scholar] [CrossRef]
- Kizilkanat, A.B.; Kabay, N.; Akyüncü, V.; Chowdhury, S.; Akça, A.H. Mechanical properties and fracture behavior of basalt and glass fiber reinforced concrete: An experimental study. Constr. Build. Mater. 2015, 100, 218–224. [Google Scholar] [CrossRef]
- Simon, S.; Prathap, A.; Balki, S.; Dhilip Kumar, R.G. An experimental investigation on concrete with basalt rock fibers. J. Phys. Conf. Ser. IOP 2021, 2070, 012196. [Google Scholar] [CrossRef]
- Deák, T.; Czigány, T. Chemical Composition and Mechanical Properties of Basalt and Glass Fibers: A Comparison. Text. Res. J. 2009, 79, 645–651. [Google Scholar] [CrossRef]
- Hausrath, R.L.; Longobardo, A.V. High-strength glass fibers and markets. In Fiberglass and Glass Technology: Energy-Friendly Compositions and Applications; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2010; pp. 197–225. ISBN 9781441907356. [Google Scholar]
- Höland, W.; Beall, G.H. Glass-Ceramic Technology; John Wiley & Sons: Hoboken, NJ, USA, 2012. [Google Scholar]
- Acar, V.; Cakir, F.; Alyamaç, E.; Seydibeyoğlu, M.Ö. Basalt fibers. In Fiber Technology for Fiber-Reinforced Composites; Elsevier: Amsterdam, The Netherlands, 2017; pp. 169–185. ISBN 9780081009932. [Google Scholar]
- Bretcanu, O.; Spriano, S.; Verné, E.; Cöisson, M.; Tiberto, P.; Allia, P. The influence of crystallised Fe3O4 on the magnetic properties of coprecipitation-derived ferrimagnetic glass-ceramics. Acta Biomater. 2005, 1, 421–429. [Google Scholar] [CrossRef] [PubMed]
- Zaitsev, D.D.; Gravchikova, E.A.; Kazin, P.E.; Garshev, A.V.; Tret’yakov, Y.D.; Jansen, M. Preparation of magnetic glass-ceramics through glass crystallization in the Na2O-SrO-Fe2O3-B2O3 system. Inorg. Mater. 2006, 42, 326–330. [Google Scholar] [CrossRef]
- Kushnir, S.E.; Vasil’Ev, A.V.; Zaitsev, D.D.; Kazin, P.E.; Tret’Yakov, Y.D. Synthesis of magnetoresistive glass-ceramic composites in the SrO-MnOx-SiO2-La2O3 system. J. Surf. Investig. 2008, 2, 34–36. [Google Scholar]
- Gubin, S.P.; Koksharov, Y.A.; Khomutov, G.B.; Yurkov, G.Y. Magnetic nanoparticles: Preparation, structure and properties. Usp. Khim. 2005, 74, 539–574. [Google Scholar] [CrossRef]
- Pomogailo, A.D.; Rozenberg, A.S.; Uflyand, I.E. Metal Nanoparticles in Polymers; Chemistry: Moscow, Russia, 2000; ISBN 5-7245-1107-X. (In Russian) [Google Scholar]
- Heilmann, A. Polymer Films with Embedded Metal Nanoparticles; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2003; ISBN 978-3-662-05233-4. [Google Scholar] [CrossRef]
- Sunil, D.; Sokolov, J.; Rafailovich, M.H.; Duan, X.; Gafney, H.D. Evidence for the Photodeposition of Elemental Iron in Porous Vycor Glass. Inorg. Chem. 1993, 32, 4489–4490. [Google Scholar] [CrossRef]
- Roy, S.; Roy, B.; Chakravorty, D. Magnetic properties of iron nanoparticles grown in a glass matrix. J. Appl. Phys. 1996, 79, 1642–1645. [Google Scholar] [CrossRef]
- Berger, R.; Bissey, J.C.; Kliava, J.; Daubric, H.; Estournès, C. Temperature dependence of superparamagnetic resonance of iron oxide nanoparticles. J. Magn. Magn. Mater. 2001, 234, 535–544. [Google Scholar] [CrossRef]
- Berger, R.; Kliava, J.; Bissey, J.C.; Baïetto, V. Magnetic resonance of superparamagnetic iron-containing nanoparticles in annealed glass. J. Appl. Phys. 2000, 87, 7389–7396. [Google Scholar] [CrossRef]
- Godsell, J.F.; Donegan, K.P.; Tobin, J.M.; Copley, M.P.; Rhen, F.M.F.; Otway, D.J.; Morris, M.A.; O’Donnell, T.; Holmes, J.D.; Roy, S. Magnetic properties of Ni nanoparticles on microporous silica spheres. J. Magn. Magn. Mater. 2010, 322, 1269–1274. [Google Scholar] [CrossRef]
- Henri, P.A.; Rommevaux-Jestin, C.; Lesongeur, F.; Mumford, A.; Emerson, D.; Godfroy, A.; Ménez, B. Structural iron (II) of basaltic glass as an energy source for zetaproteobacteria in an abyssal plain environment, off the mid-Atlantic ridge. Front. Microbiol. 2016, 6, 1518. [Google Scholar] [CrossRef]
- Kursawe, M.; Anselmann, R.; Hilarius, V.; Pfaff, G. Nano-particles by wet chemical processing in commercial applications. J. Sol-Gel Sci. Technol. 2005, 33, 71–74. [Google Scholar] [CrossRef]
- Wang, C.H.; Lin, X.C.; Yang, S.S.; Liu, S.Q.; Yoon, S.; Wang, Y.G. Evaluation of the thermal and rheological characteristics of minerals in coal using SiO2-Al2O3-CaO-FeOx quaternary system. Ranliao Huaxue Xuebao/J. Fuel Chem. Technol. 2016, 44, 1025–1033. [Google Scholar] [CrossRef]
- Senanayake, G.; Das, G.K. A comparative study of leaching kinetics of limonitic laterite and synthetic iron oxides in sulfuric acid containing sulfur dioxide. Hydrometallurgy 2004, 72, 59–72. [Google Scholar] [CrossRef]
- Baikousi, M.; Agathopoulos, S.; Panagiotopoulos, I.; Georgoulis, A.D.; Louloudi, M.; Karakassides, M.A. Synthesis and characterization of sol-gel derived bioactive CaO-SiO2-P2O5 glasses containing magnetic nanoparticles. J. Sol-Gel Sci. Technol. 2008, 47, 95–101. [Google Scholar] [CrossRef]
- Wang, D.; Lin, H.; Jiang, J.; Han, X.; Guo, W.; Wu, X.; Jin, Y.; Qu, F. One-pot synthesis of magnetic, macro/mesoporous bioactive glasses for bone tissue engineering. Sci. Technol. Adv. Mater. 2013, 14, 25004. [Google Scholar] [CrossRef]
- Shahar, A.; Hillgren, V.J.; Horan, M.F.; Mesa-Garcia, J.; Kaufman, L.A.; Mock, T.D. Sulfur-controlled iron isotope fractionation experiments of core formation in planetary bodies. Geochim. Cosmochim. Acta 2015, 150, 253–264. [Google Scholar] [CrossRef]
- Ahmadzadeh, M.; Marcial, J.; McCloy, J. Crystallization of iron-containing sodium aluminosilicate glasses in the NaAlSiO4-NaFeSiO4 join. J. Geophys. Res. Solid Earth 2017, 122, 2504–2524. [Google Scholar] [CrossRef]
- Abdallah, E.A.M.; Gagnon, G.A. Arsenic removal from groundwater through iron oxyhydroxide coated waste products. Can. J. Civ. Eng. 2009, 36, 881–888. [Google Scholar] [CrossRef]
- Jackson, W.E.; Farges, F.; Yeager, M.; Mabrouk, P.A.; Rossano, S.; Waychunas, G.A.; Solomon, E.I.; Brown, G.E. Multi-spectroscopic study of Fe(II) in silicate glasses: Implications for the coordination environment of Fe(II) in silicate melts. Geochim. Cosmochim. Acta 2005, 69, 4315–4332. [Google Scholar] [CrossRef]
- Berger, G.; Cathala, A.; Fabre, S.; Borisova, A.Y.; Pages, A.; Aigouy, T.; Esvan, J.; Pinet, P. Experimental exploration of volcanic rocks-atmosphere interaction under Venus surface conditions. Icarus 2019, 329, 8–23. [Google Scholar] [CrossRef]
- Isobe, H.; Yoshizawa, M. Formation of iron mineral fine particles by acidic hydrothermal alteration experiments of synthetic martian basalt. J. Mineral. Petrol. Sci. 2014, 109, 62–73. [Google Scholar] [CrossRef]
- Ward, C.R.; French, D. Determination of glass content and estimation of glass composition in fly ash using quantitative X-ray diffractometry. Fuel 2006, 85, 2268–2277. [Google Scholar] [CrossRef]
- Dyadenko, M.V.; Levitskii, I.A.; Sidorevich, A.G. Influence of the Structure of the Glasses from the BaO–La2O3–B2O3–ZrO2–TiO2–SiO2–Nb2O5 System on Their Thermal Expansion and Technological Characteristics. Glas. Phys. Chem. 2022, 48, 341–350. [Google Scholar] [CrossRef]
- Rietveld, H.M. A profile refinement method for nuclear and magnetic structures. J. Appl. Crystallogr. 1969, 2, 65–71. [Google Scholar] [CrossRef]
- Masaitis, V.L.; Danilin, A.N.; Mashchak, M.S.; Raikhlin, A.I.; Selivanovskaia, T.V.; Shadenkov, E.M. The Geology of Astroblemes; Nedra: Leningrad, Russia, 1980. (In Russian) [Google Scholar]
- Ubbelohde, A.R. Melting and crystal structure. Q. Rev. Chem. Soc. 1950, 4, 356–381. [Google Scholar] [CrossRef]
- Ubbelohde, A.R. Melting and Crystal Structure—Some Current Problems. Angew. Chem. Int. Ed. Engl. 1965, 4, 587–591. [Google Scholar] [CrossRef]
- Sobolev, R.N. The temperature range of melting of crystalline material. Dokl. Earth Sci. 2017, 473, 367–370. [Google Scholar] [CrossRef]
- Sobolev, R.N.; Mal’tsev, V.V.; Volkova, E.A. Experimental Investigation of the Melting of Minerals and Rocks. Russ. Metall. 2021, 2021, 102–108. [Google Scholar] [CrossRef]
- Masaitis, V.L. Structures and Textures of Explosive Breccias and Impactites; Nedra: Leningrad, Russia, 1983. (In Russian) [Google Scholar]
- Torre, E. Della Magnetic Hysteresis; Wiley-IEEE Press: Hoboken, NJ, USA, 2000; ISBN 9780470545195. [Google Scholar]
- Altshuler, S.A.; Kozyrev, B.M. Electron Paramagnetic Resonance in Compounds of Transition Elements; John Wiley & Sons: New York, NJ, USA, 1974; ISBN 978-0-470-02523-9. [Google Scholar]
- Day, R.; Fuller, M.; Schmidt, V.A. Hysteresis properties of titanomagnetites: Grain-size and compositional dependence. Phys. Earth Planet. Inter. 1977, 13, 260–267. [Google Scholar] [CrossRef]
- Dunlop, D.J. Theory and application of the Day plot (Mrs/Ms versus Hcr/Hc) 2. Application to data for rocks, sediments, and soils. J. Geophys. Res. 2002, 107, EPM 5-1–EPM 5-15. [Google Scholar] [CrossRef]
- Roberts, A.P.; Tauxe, L.; Heslop, D.; Zhao, X.; Jiang, Z. A Critical Appraisal of the “Day” Diagram. J. Geophys. Res. Solid Earth 2018, 123, 2618–2644. [Google Scholar] [CrossRef]
- Ralin, A.Y.; Kharitonskii, P.V. Effect of thermal fluctuations on the stability of the magnetic state of small two-phase ferrimagnetic particles. Phys. Met. Metallogr. 2002, 93, 109–114. [Google Scholar]
- Kharitonskii, P.; Zolotov, N.; Kirillova, S.; Gareev, K.; Kosterov, A.; Sergienko, E.; Yanson, S.; Ustinov, A.; Ralin, A. Magnetic granulometry, Mössbauer spectroscopy, and theoretical modeling of magnetic states of FemOn–Fem-xTixOn composites. Chin. J. Phys. 2022, 78, 271–296. [Google Scholar] [CrossRef]
- Kirshvink, J.L. (Ed.) Magnetite Biomineralization and Magnetoreception in Organisms. A New Biomagnetism; Plenum Press: New York, NY, USA, 1985. [Google Scholar]
- Eyre, J.K. Frequency dependence of magnetic susceptibility for populations of single-domain grains. Geophys. J. Int. 1997, 129, 209–211. [Google Scholar] [CrossRef]
- Egli, R. Magnetic susceptibility measurements as a function of temperature and frequency I: Inversion theory. Geophys. J. Int. 2009, 177, 395–420. [Google Scholar] [CrossRef]
- Hrouda, F. Models of frequency-dependent susceptibility of rocks and soils revisited and broadened. Geophys. J. Int. 2011, 187, 1259–1269. [Google Scholar] [CrossRef]
- Kharitonskii, P.; Bobrov, N.; Gareev, K.; Kosterov, A.; Nikitin, A.; Ralin, A.; Sergienko, E.; Testov, O.; Ustinov, A.; Zolotov, N. Magnetic granulometry, frequency-dependent susceptibility and magnetic states of particles of magnetite ore from the Kovdor deposit. J. Magn. Magn. Mater. 2022, 553, 169279. [Google Scholar] [CrossRef]
- Dearing, J.A.; Dann, R.J.L.; Hay, K.; Lees, J.A.; Loveland, P.J.; Maher, B.A.; O’Grady, K. Frequency-dependent susceptibility measurements of environmental materials. Geophys. J. Int. 1996, 124, 228–240. [Google Scholar] [CrossRef]
- Gotić, M.; Musić, S. Mössbauer, FT-IR and FE SEM investigation of iron oxides precipitated from FeSO4 solutions. J. Mol. Struct. 2007, 834–836, 445–453. [Google Scholar] [CrossRef]
- Kharitonskii, P.; Kamzin, A.; Gareev, K.; Valiullin, A.; Vezo, O.; Sergienko, E.; Korolev, D.; Kosterov, A.; Lebedev, S.; Gurylev, A.; et al. Magnetic granulometry and Mössbauer spectroscopy of FemOn–SiO2 colloidal nanoparticles. J. Magn. Magn. Mater. 2018, 461, 30–36. [Google Scholar] [CrossRef]
- Castner, T.; Newell, G.S.; Holton, W.C.; Slighter, C.P. Note on the paramagnetic resonance of iron in glass. J. Chem. Phys. 1960, 32, 668–673. [Google Scholar] [CrossRef]
- Antoni, E.; Montagne, L.; Daviero, S.; Palavit, G.; Bernard, J.L.; Wattiaux, A.; Vezin, H. Structural characterization of iron-alumino-silicate glasses. J. Non-Cryst. Solids 2004, 345–346, 66–69. [Google Scholar] [CrossRef]
- Lyutoev, V.P.; Lysiuk, A.Y.; Karlova, L.O.; Beznosikov, D.S.; Zhuk, N.A. ESR and 57Fe Mössbauer Spectroscopy Study of Fe-doped SrBi2Nb2O9. J. Sib. Fed. Univ.—Math. Phys. 2022, 15, 450–458. [Google Scholar] [CrossRef]
- Griscom, D.L. Ferromagnetic resonance of precipitated phases in natural glasses. J. Non. Cryst. Solids 1984, 67, 81–118. [Google Scholar] [CrossRef]
- Radchenko, Y.S.; Levitskii, I.A.; Ugolev, I.I. Investigation of glasses and glaze coats of composition R2O-RO-Fe2O3(FeO)-Al2O3-B2O3-SiO2 by the EPR method. J. Appl. Spectrosc. 2003, 70, 821–826. [Google Scholar] [CrossRef]
- Griscom, D.L. Electron spin resonance in glasses. J. Non. Cryst. Solids 1980, 40, 211–272. [Google Scholar] [CrossRef]
- Bodziony, T.; Guskos, N.; Typek, J.; Roslaniec, Z.; Narkiewicz, U.; Kwiatkowska, M.; Maryniak, M. Temperature dependence of the FMR spectra of Fe3O4 and Fe3C nanoparticle magnetic systems in copolymer matrices. Mater. Sci. Pol. 2005, 23, 1055–1063. [Google Scholar]
- Olin, M.; Anttila, T.; Dal Maso, M. Using a combined power law and log-normal distribution model to simulate particle formation and growth in a mobile aerosol chamber. Atmos. Chem. Phys. 2016, 16, 7067–7090. [Google Scholar] [CrossRef]
- Fujihara, A.; Tanimoto, S.; Yamamoto, H.; Ohtsuki, T. Log-normal distribution in a growing system with weighted and multiplicatively interacting particles. J. Phys. Soc. Jpn. 2018, 87, 034001. [Google Scholar] [CrossRef]
- Dunlop, D.J. Superparamagnetic and single-domain threshold sizes in magnetite. J. Geophys. Res. 1973, 78, 1780–1793. [Google Scholar] [CrossRef]
- Butler, R.F.; Banerjee, S.K. Theoretical single-domain grain size range in magnetite and titanomagnetite. J. Geophys. Res. 1975, 80, 4049–4058. [Google Scholar] [CrossRef]
- Moskowitz, B.M.; Banerjee, S.K. Grain Size Limits for Pseudosingle Domain Behavior in Magnetite: Implications for Paleomagnetism. IEEE Trans. Magn. 1979, 15, 1241–1246. [Google Scholar] [CrossRef]
- Nagy, L.; Williams, W.; Tauxe, L.; Muxworthy, A.R. From Nano to Micro: Evolution of Magnetic Domain Structures in Multidomain Magnetite. Geochem. Geophys. Geosyst. 2019, 20, 2907–2918. [Google Scholar] [CrossRef]
- Kharitonskii, P.V.; Gareev, K.G.; Ionin, S.A.; Ryzhov, V.A.; Bogachev, Y.V.; Klimenkov, B.D.; Kononova, I.E.; Moshnikov, V.A. Microstructure and magnetic state of Fe3O4-SiO2 colloidal particles. J. Magn. 2015, 20, 221–228. [Google Scholar] [CrossRef]
- Ralin, A.Y.; Kharitonskii, P.V. Magnetic Metastability of Small Inhomogeneous Ferrimagnetic Particles. Phys. Met. Metallogr. 1994, 78, 270–273. [Google Scholar]
- Kharitonskii, P.V.; Anikieva, Y.A.; Zolotov, N.A.; Gareev, K.G.; Ralin, A.Y. Micromagnetic modeling of Fe3O4−Fe3−xTixO4 composites. Phys. Solid State 2022, 64, 1311–1314. [Google Scholar] [CrossRef]
- Kharitonskiǐ, P.V. Magnetostatic interaction of superparamagnetic particles dispersed in a thin layer. Phys. Solid State 1997, 39, 162–163. [Google Scholar] [CrossRef]
- Kharitonskii, P.; Kirillova, S.; Gareev, K.; Kamzin, A.; Gurylev, A.; Kosterov, A.; Sergienko, E.; Valiullin, A.; Shevchenko, E. Magnetic Granulometry and Mössbauer Spectroscopy of Synthetic FemOn-TiO2 Composites. IEEE Trans. Magn. 2020, 56, 7200209. [Google Scholar] [CrossRef]
- Kharitonskii, P.V.; Kosterov, A.A.; Gurylev, A.K.; Gareev, K.G.; Kirillova, S.A.; Zolotov, N.A.; Anikieva, Y.A. Magnetic States of Two-Phase Synthesized FemOn–Fe3–xTixO4 Particles: Experimental and Theoretical Analysis. Phys. Solid State 2020, 62, 1691–1694. [Google Scholar] [CrossRef]
- Al’miev, A.S.; Ralin, A.Y.; Kharitonskii, P.V. Distribution functions of dipole-dipole interaction fields in dilute magnetic materials. Phys. Met. Metallogr. 1994, 78, 28–34. [Google Scholar]
- Starowicz, M.; Starowicz, P.; Żukrowski, J.; Przewoźnik, J.; Lemański, A.; Kapusta, C.; Banaś, J. Electrochemical synthesis of magnetic iron oxide nanoparticles with controlled size. J. Nanoparticle Res. 2011, 13, 7167–7176. [Google Scholar] [CrossRef]
- Roberts, A.P.; Almeida, T.P.; Church, N.S.; Harrison, R.J.; Heslop, D.; Li, Y.; Li, J.; Muxworthy, A.R.; Williams, W.; Zhao, X. Resolving the Origin of Pseudo-Single Domain Magnetic Behavior. J. Geophys. Res. Solid Earth 2017, 122, 9534–9558. [Google Scholar] [CrossRef]
- Wohlfarth, E.P. Spin glasses exhibit rock magnetism. Phys. B+C 1977, 86–88, 852–853. [Google Scholar] [CrossRef]
- Wohlfarth, E.P. The temperature dependence of the magnetic susceptibility of spin glasses. Phys. Lett. A 1979, 70, 489–491. [Google Scholar] [CrossRef]
- Néel, L. Théorie du traînage magnétique des ferromagnétiques en grains fins avec application aux terres cuites. Ann. Geophys. 1949, 5, 99–136. [Google Scholar]
- Kneller, E.F.; Luborsky, F.E. Particle size dependence of coercivity and remanence of single-domain particles. J. Appl. Phys. 1963, 34, 656–658. [Google Scholar] [CrossRef]
- Bagin, V.I.; Gendler, T.S.; Avilova, T.E. Magnetism of α-Oxides and Hydroxides of Iron; Nauka: Moscow, Russia, 1988. (In Russian) [Google Scholar]
- Dunlop, D.J.; Özdemir, Ö. Rock Magnetism: Fundamentals and Frontiers; Cambridge University Press: Cambridge, UK, 1997. [Google Scholar] [CrossRef]
- Rochette, P.; Mathé, P.E.; Esteban, L.; Rakoto, H.; Bouchez, J.L.; Liu, Q.; Torrent, J. Non-saturation of the defect moment of goethite and fine-grained hematite up to 57 Teslas. Geophys. Res. Lett. 2005, 32, 1–4. [Google Scholar] [CrossRef]
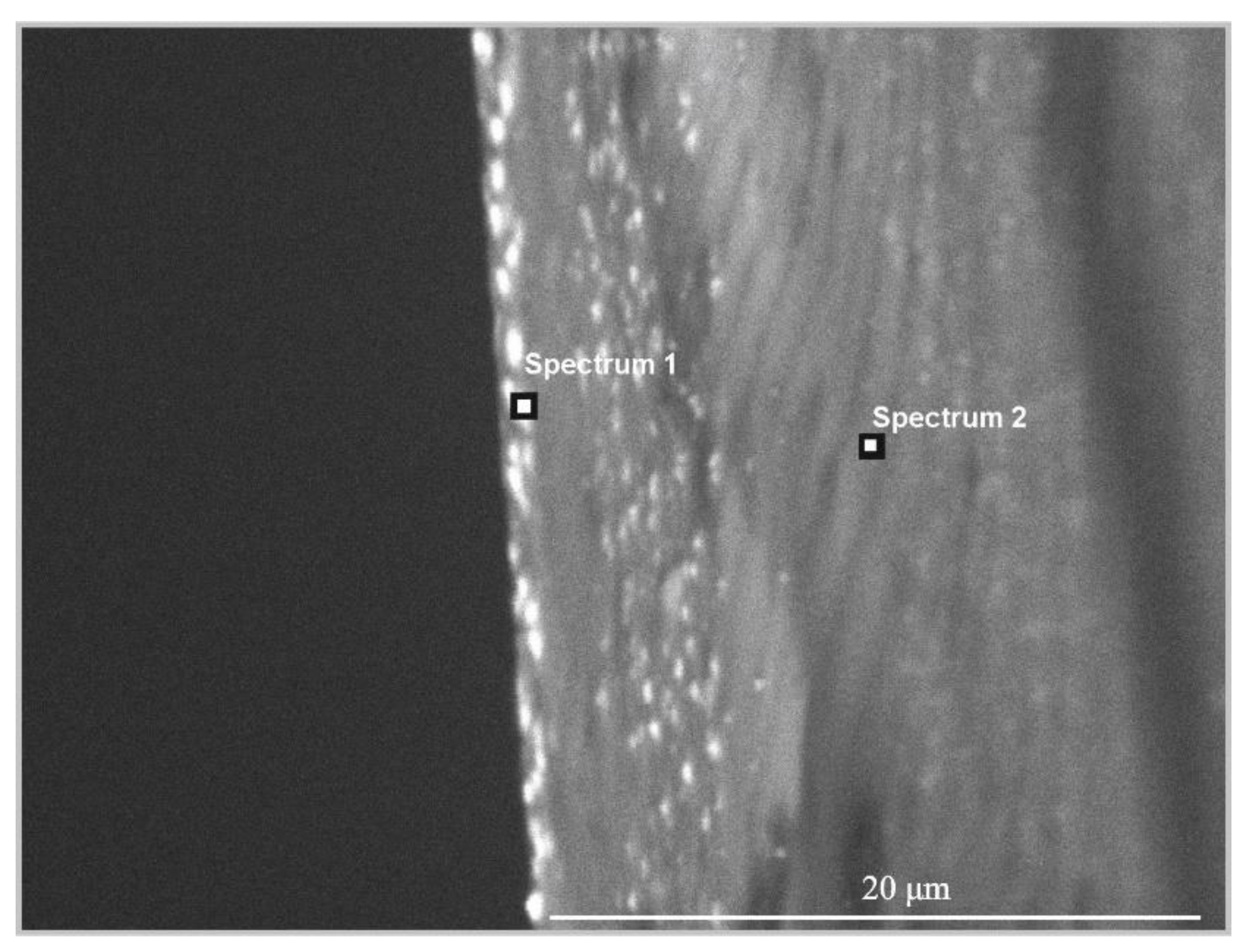

| Mineral | Content, at. % | ||
|---|---|---|---|
| Crystalline Shales | Volcanogenic–Sedimentary Rock | Psammite–Silt–Pelitic Complex | |
| Quartz | 5.3 | 18.0 | 63.7 |
| Plagioclase | 33.2 | 33.9 | 8.3 |
| K-feldspar | 2.3 | 1.0 | 5.4 |
| Pyroxene | - | 4.4 | 1.3 |
| Amphibole | 12.8 | 3.5 | - |
| Mica (muscovite/biotite) | <1 | 6.0 | 1.8 |
| Chlorite | 8.6 | 5.1 | - |
| Calcite | 17.7 | 13.2 | <1 |
| Dolomite | - | 1.3 | <1 |
| Epidote | 10.7 | - | - |
| Titanite | 6.5 | - | - |
| Smectite | 1.9 | - | - |
| Heulandite | - | - | 2.2 |
| Kaolinite | - | - | 15.5 |
| Hematite | - | 4.0 | 1.1 |
| Goethite | - | 9.8 | - |
| Rp (%) * | 5.4 | 4.1 | 2.9 |
| Sample | μ0Hc, mT | μ0Hcr, mT | Ms, A·m2/kg | Mrs, A·m2/kg | Hcr/Hc | Mrs/Ms |
|---|---|---|---|---|---|---|
| s | 13.0 | 311.0 | 0.0006 | 0.0003 | 23.92 | 0.48 |
| a | 160.0 | 513.0 | 0.0840 | 0.0110 | 3.21 | 0.13 |
| c | 17.0 | 47.0 | 0.0056 | 0.0005 | 2.76 | 0.09 |
| a w | 1.0 | 14.3 | 0.0266 | 0.0023 | 13.99 | 0.09 |
| a f | 41.7 | 652.5 | 0.2055 | 0.0099 | 15.63 | 0.05 |
| s w | 2.9 | 7.6 | 0.3050 | 0.0716 | 2.65 | 0.24 |
| s f | 18.5 | 33.9 | 4.5980 | 1.2980 | 1.83 | 0.28 |
| c w | – | – | – | – | – | – |
| c f | 47.4 | 286.5 | 0.2061 | 0.0258 | 6.04 | 0.13 |
| a20s20f | – | – | – | – | – | – |
| a20s20w | – | – | – | – | – | – |
| a30c10w | 2.0 | 9.0 | 0.0090 | 0.0006 | 4.50 | 0.07 |
| a10c30w | 0.1 | 9.0 | 0.3600 | 0.0001 | 90.00 | 0.0003 |
| a30c10f | 22.0 | 116.0 | 3.0200 | 1.1500 | 5.27 | 0.38 |
| a10c30f | 23.0 | 35.0 | 2.9590 | 1.1400 | 1.52 | 0.39 |
| s30c10w | 16.7 | 17.0 | 0.0040 | 0.0020 | 1.02 | 0.49 |
| s10c30w | 0.6 | 74.0 | 0.0210 | 0.0010 | 123.33 | 0.05 |
| s30c10f | 1.4 | 1.5 | 0.0070 | 0.0010 | 1.07 | 0.14 |
| s10c30f | 0.6 | 2.7 | 1.3850 | 0.0005 | 4.50 | 0.0004 |
| a30s10c5w | 6.0 | 74.0 | 0.0100 | 0.0010 | 12.33 | 0.10 |
| a10s30c5w | – | – | – | – | – | – |
| a30s10c5f | 12.0 | 134.0 | 0.7020 | 0.1240 | 11.17 | 0.18 |
| a10s30c5f | 60.0 | 99.9 | 0.8900 | 0.4000 | 1.67 | 0.45 |
| No. | Sample | fd (%) |
|---|---|---|
| 1 | a10c30 f | 2.0 |
| 2 | a30c10 f | 1.1 |
| 3 | sf | 6.0 |
| 4 | a30s10c5 f | 11.9 |
| 5 | s10c30 f | 7.4 |
| Element | Spectrum 1, wt % | Spectrum 2, wt % |
|---|---|---|
| O | 59.84 | 60.73 |
| Na | 1.42 | 1.31 |
| Mg | 0.79 | 1.01 |
| Al | 5.29 | 5.68 |
| Si | 23.73 | 25.77 |
| K | 0.93 | 0.80 |
| Ca | 1.57 | 1.79 |
| Fe | 6.43 | 2.90 |
| Sample | Na (1s) | Fe (2p3/2) | F (1s) | O (1s) | Ti (2p3/2) | Ca (2p) | C (1s) | Si (2p) | Al (2p) | |||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| a10c30f | 1.0 | 0.7 | 0.3 | 33.7 | 18.1 | 0.3 | 1.7 | 2.0 | 6.0 | 12.1 | 21.9 | 2.2 |
| eV | 1072.9 | 710.9 | 685.5 | 532.8 | 531.4 | 459.8 | 348.0 | 288.0 | 286.0 | 285.0 | 102.7 | 74.2 |
| a30c10f | 0.7 | 0.5 | – | 27.3 | 16.1 | 0.1 | 1.5 | 3.2 | 15.4 | 17.1 | 13.2 | 4.8 |
| eV | 1072.9 | 711.0 | – | 533.0 | 531.6 | 459.5 | 348.0 | 288.6 | 286.6 | 285.0 | 102.6 | 74.3 |
| sf | 1.6 | 1.1 | 0.4 | 30.0 | 14.4 | 0.3 | 2.4 | – | 3.1 | 13.0 | 27.1 | 5.7 |
| eV | 1072.7 | 710.9 | 685.3 | 532.6 | 531.2 | 458.9 | 347.9 | – | 286.6 | 285.0 | 102.7 | 74.2 |
| a30s10c5f | 0.6 | 1.7 | – | 33.5 | 18.1 | – | 2.3 | 2.2 | 6.5 | 12.8 | 14.7 | 7.6 |
| eV | 1072.5 | 710.8 | – | 532.6 | 531.3 | – | 348.0 | 288.5 | 286.5 | 285.0 | 102.2 | 74.0 |
| s10c30f | 1.0 | 0.8 | 0.7 | 32.6 | 14.7 | 0.3 | 1.4 | 2.4 | 5.2 | 8.3 | 25.2 | 8.1 |
| eV | 1073.1 | 710.5 | 685.3 | 533.0 | 531.7 | 459.9 | 348.4 | 288.2 | 286.6 | 285.0 | 103.1 | 74.7 |
| Na+ | Fe2+, Fe3+ | MF | SiO2 | Al2O3 | Ti4+ | Ca2+ | OCO | COC | CC, CH | Si4+ | Al3+ | |
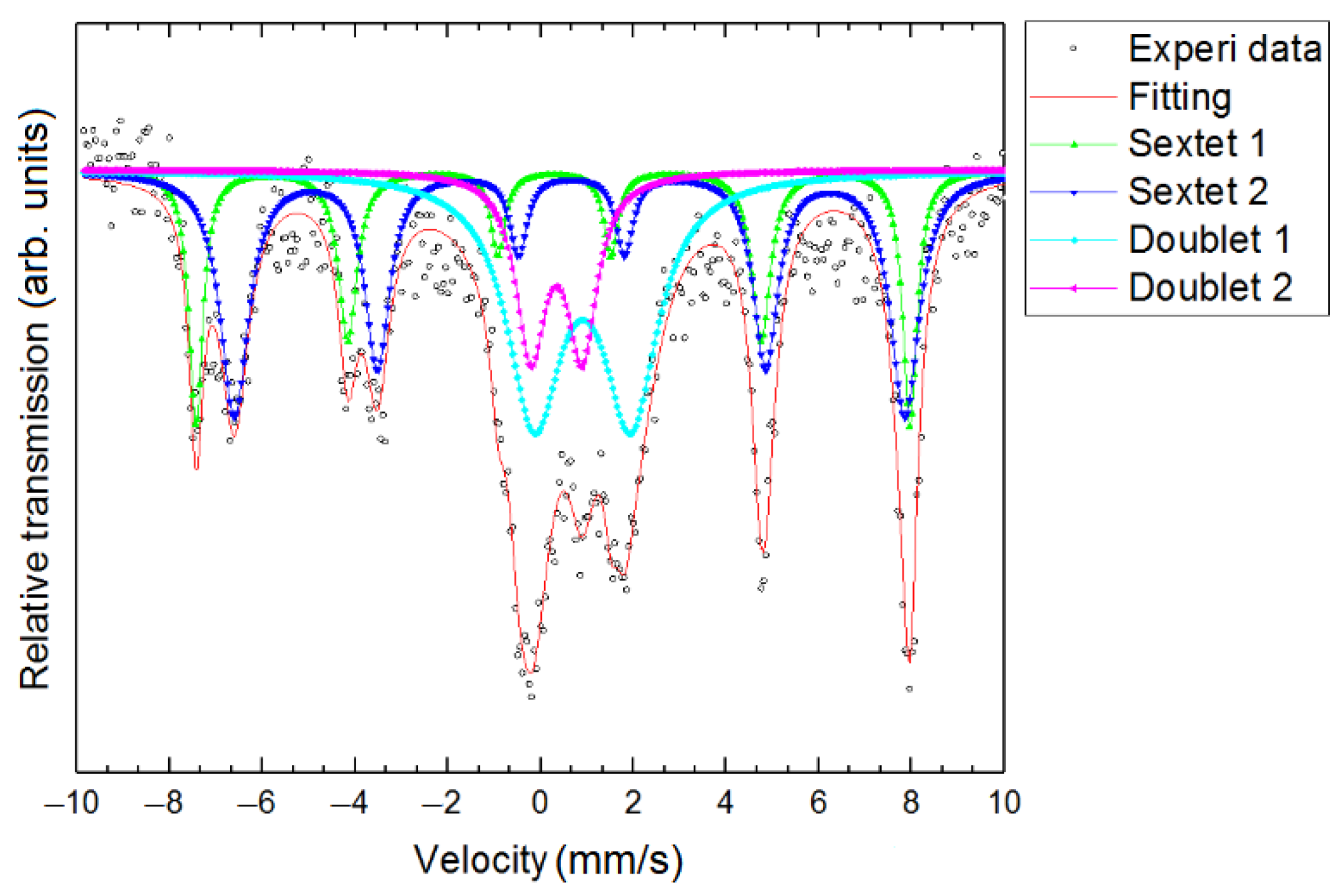
| Sample | Component | IS (mm/s) | QS (mm/s) | BHf (T) | S (%) |
|---|---|---|---|---|---|
| a10c30f | Sextet Fe(1) | 0.246 ± 0.009 | 0.017 ± 0.018 | 48.454 ± 0.081 | 17.66 |
| Sextet Fe(2) | 0.659 ± 0.019 | 0.013 ± 0.035 | 45.659 ± 0.143 | 40.39 | |
| Doublet Fe(1) | 0.863 ± 0.029 | 1.932 ± 0.052 | – | 41.95 | |
| a30c10f | Sextet Fe(1) | 0.284 ± 0.008 | 0.032 ± 0.013 | 47.843 ± 0.052 | 20.35 |
| Sextet Fe(2) | 0.648 ± 0.009 | 0.039 ± 0.016 | 44.925 ± 0.076 | 33.57 | |
| Doublet Fe(1) | 0.906 ± 0.029 | 2.081 ± 0.047 | – | 32.77 | |
| Doublet Fe(2) | 0.344 ± 0.023 | 1.104 ± 0.052 | – | 13.30 | |
| sf | Sextet Fe(1) | 0.304 ± 0.000 | 0.064 ± 0.020 | 47.430 ± 0.068 | 20.29 |
| Sextet Fe(2) | 0.645 ± 0.000 | 0.015 ± 0.028 | 44.209 ± 0.116 | 27.18 | |
| Doublet Fe(1) | 0.842 ± 0.011 | 2.288 ± 0.022 | – | 24.97 | |
| Doublet Fe(2) | 0.456 ± 0.009 | 0.867 ± 0.015 | – | 27.55 | |
| a30s10c5f | Sextet Fe(1) | 0.340 ± 0.025 | 0.101 ± 0.047 | 47.337 ± 0.151 | 3.91 |
| Sextet Fe(2) | 0.540 ± 0.022 | 0.029 ± 0.041 | 43.451 ± 0.220 | 17.09 | |
| Doublet Fe(1) | 0.872 ± 0.004 | 2.350 ± 0.006 | – | 37.51 | |
| Doublet Fe(2) | 0.463 ± 0.003 | 0.842 ± 0.005 | – | 41.49 | |
| s10c30f | Sextet Fe(1) | 0.312 ± 0.020 | 0.060 ± 0.034 | 48.020 ± 0.129 | 17.64 |
| Sextet Fe(2) | 0.663 ± 0.026 | 0.024 ± 0.043 | 44.869 ± 0.183 | 26.41 | |
| Doublet Fe(1) | 0.912 ± 0.018 | 2.155 ± 0.037 | – | 36.89 | |
| Doublet Fe(2) | 0.536 ± 0.023 | 0.832 ± 0.038 | – | 19.07 |
| Sample | Cnbsp | Cbsp | Csd | Cfpsd | Ccpsd | ME | dmean, nm |
|---|---|---|---|---|---|---|---|
| a30c10f | 0.010 | 0.075 | 0.233 | 0.656 | 0.026 | 5.35 | 33.6 |
| a10c30f | 0.020 | 0.097 | 0.237 | 0.619 | 0.027 | 3.79 | 29.7 |
| sf | 0.060 | 0.136 | 0.233 | 0.543 | 0.028 | 1.34 | 20.5 |
| s10c30f | 0.070 | 0.142 | 0.231 | 0.530 | 0.027 | 1.05 | 18.9 |
| a30s10c5f | 0.120 | 0.158 | 0.218 | 0.477 | 0.027 | 0.31 | 12.3 |
| No. | Sample | ε | Cf, 10–3 | Ms = Ms1 + Ms2 + Ms sp, A·m2/kg | Mrs = Mrs1 + Mrs2, A·m2/kg | Is eff, kA/m | Irs eff, kA/m | ||
|---|---|---|---|---|---|---|---|---|---|
| 1 | a10c30f | 0.05 | 9 | 2.96 | 1.88 + 1.02 + 0.06 | 1.14 | 0.73 + 0.41 | 465 | 286 |
| 2 | a30c10f | 0.85 | 20 | 3.02 | 0.75 + 2.20 + 0.07 | 1.15 | 0.28 + 0.87 | 203 | 167 |
| 3 | sf | 0.05 | 14 | 4.60 | 2.57 + 1.75 + 0.28 | 1.30 | 0.76 + 0.54 | 466 | 266 |
| 4 | a30s10c5f | 0.90 | 4 | 0.70 | 0.07 + 0.48 + 0.15 | 0.12 | 0.01 + 0.11 | 263 | 110 |
| 5 | s10c30f | 0.75 | 10 | 1.39 | 0.67 + 0.48 + 0.24 | 0.5 × 10–3 | (0.3 + 0.2) × 10–3 | 198 | ~1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kharitonskii, P.; Sergienko, E.; Ralin, A.; Setrov, E.; Sheidaev, T.; Gareev, K.; Ustinov, A.; Zolotov, N.; Yanson, S.; Dubeshko, D. Superparamagnetism of Artificial Glasses Based on Rocks: Experimental Data and Theoretical Modeling. Magnetochemistry 2023, 9, 220. https://doi.org/10.3390/magnetochemistry9100220
Kharitonskii P, Sergienko E, Ralin A, Setrov E, Sheidaev T, Gareev K, Ustinov A, Zolotov N, Yanson S, Dubeshko D. Superparamagnetism of Artificial Glasses Based on Rocks: Experimental Data and Theoretical Modeling. Magnetochemistry. 2023; 9(10):220. https://doi.org/10.3390/magnetochemistry9100220
Chicago/Turabian StyleKharitonskii, Petr, Elena Sergienko, Andrey Ralin, Evgenii Setrov, Timur Sheidaev, Kamil Gareev, Alexander Ustinov, Nikita Zolotov, Svetlana Yanson, and Danil Dubeshko. 2023. "Superparamagnetism of Artificial Glasses Based on Rocks: Experimental Data and Theoretical Modeling" Magnetochemistry 9, no. 10: 220. https://doi.org/10.3390/magnetochemistry9100220
APA StyleKharitonskii, P., Sergienko, E., Ralin, A., Setrov, E., Sheidaev, T., Gareev, K., Ustinov, A., Zolotov, N., Yanson, S., & Dubeshko, D. (2023). Superparamagnetism of Artificial Glasses Based on Rocks: Experimental Data and Theoretical Modeling. Magnetochemistry, 9(10), 220. https://doi.org/10.3390/magnetochemistry9100220







