Kinetics and the Effect of Thermal Treatments on the Martensitic Transformation and Magnetic Properties in Ni49Mn32Ga19 Ferromagnetic Shape Memory Ribbons
Abstract
:1. Introduction
2. Materials and Methods
3. Results
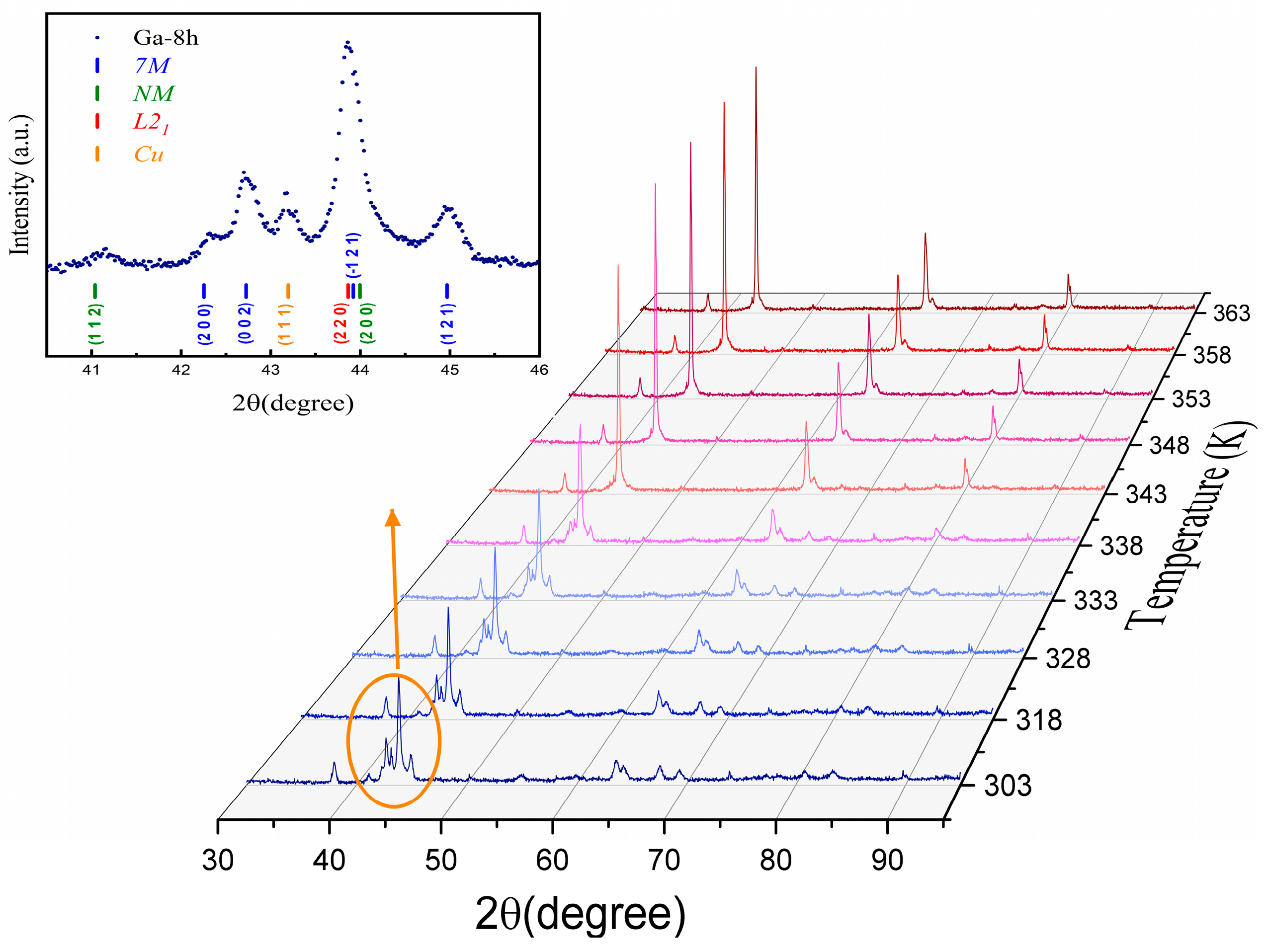
3.1. XRD
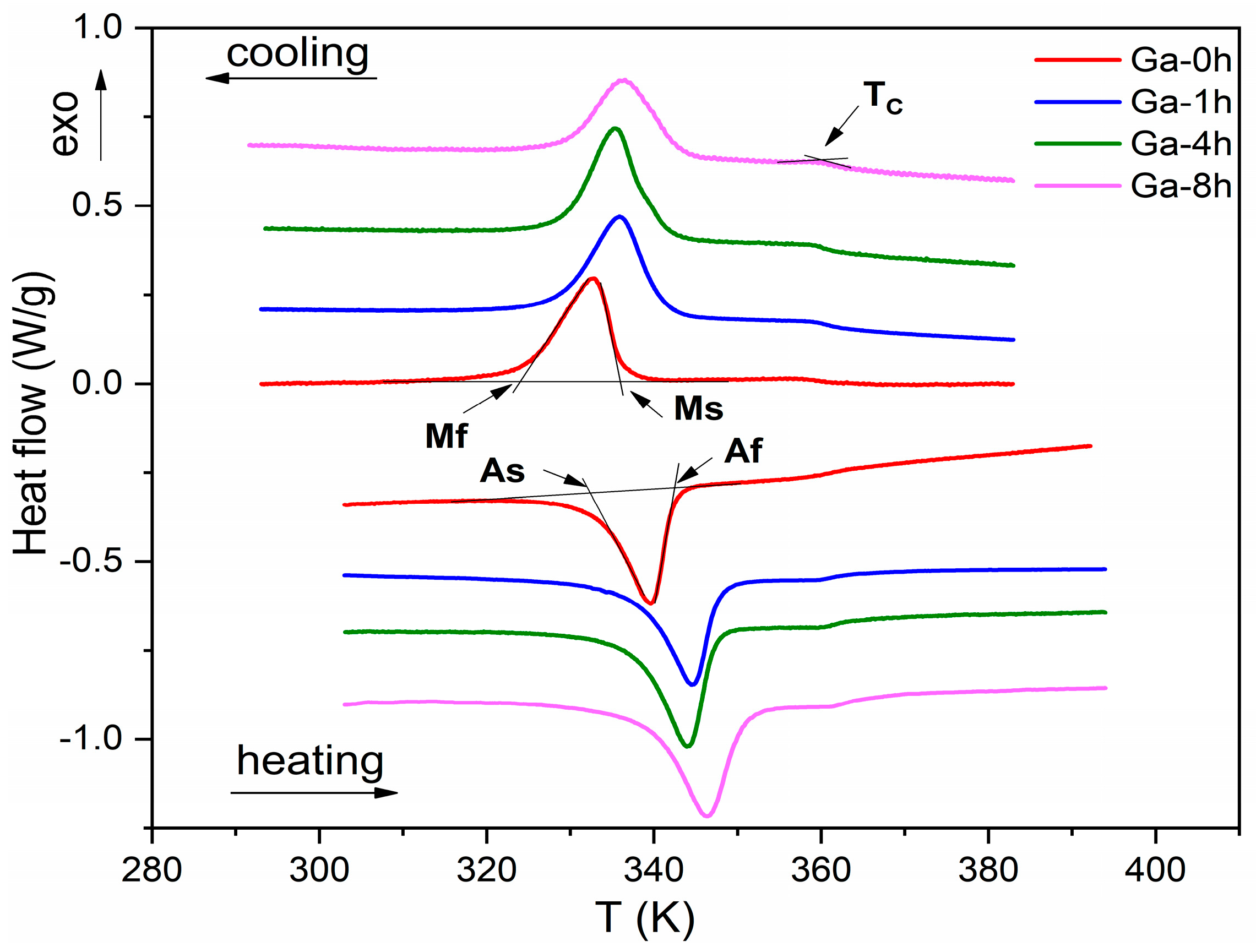
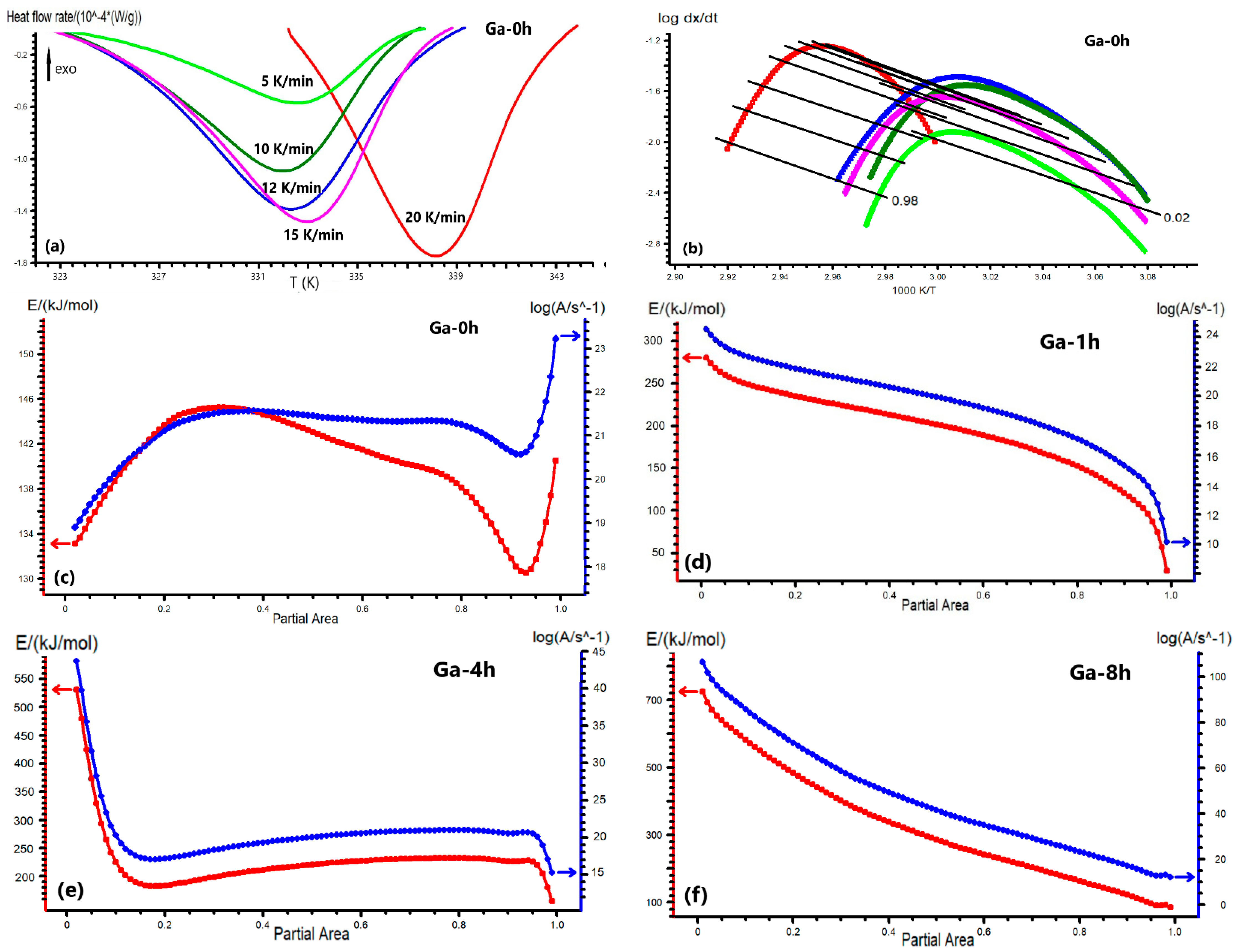
3.2. DSC and Kinetics
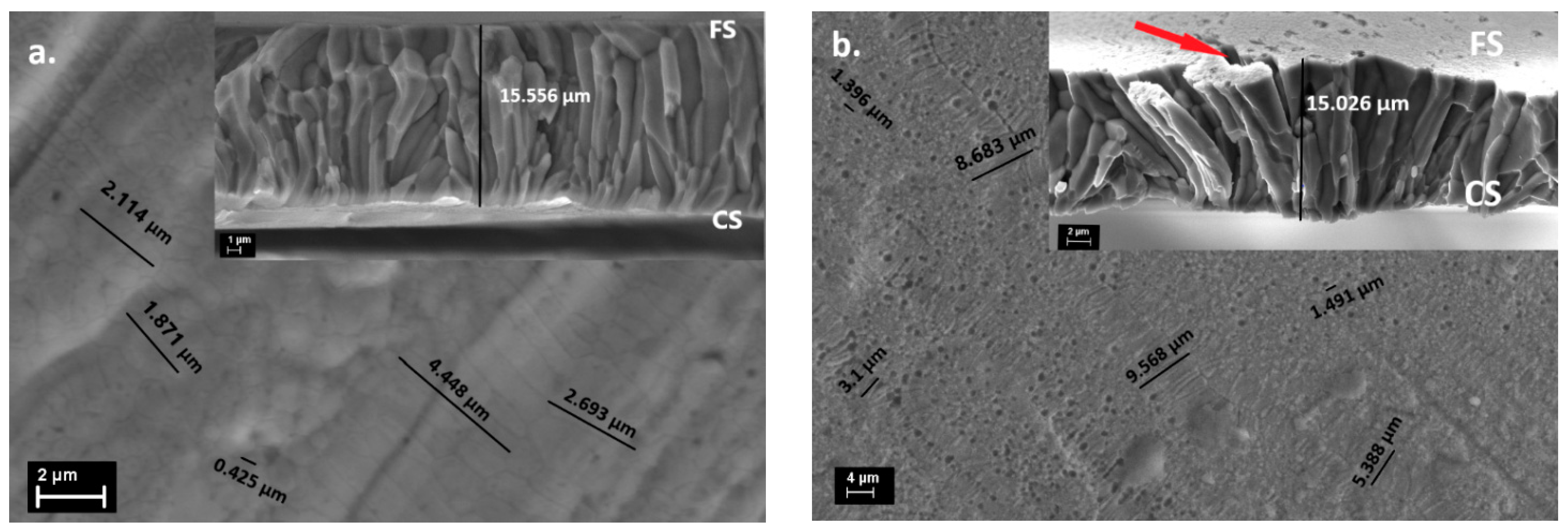
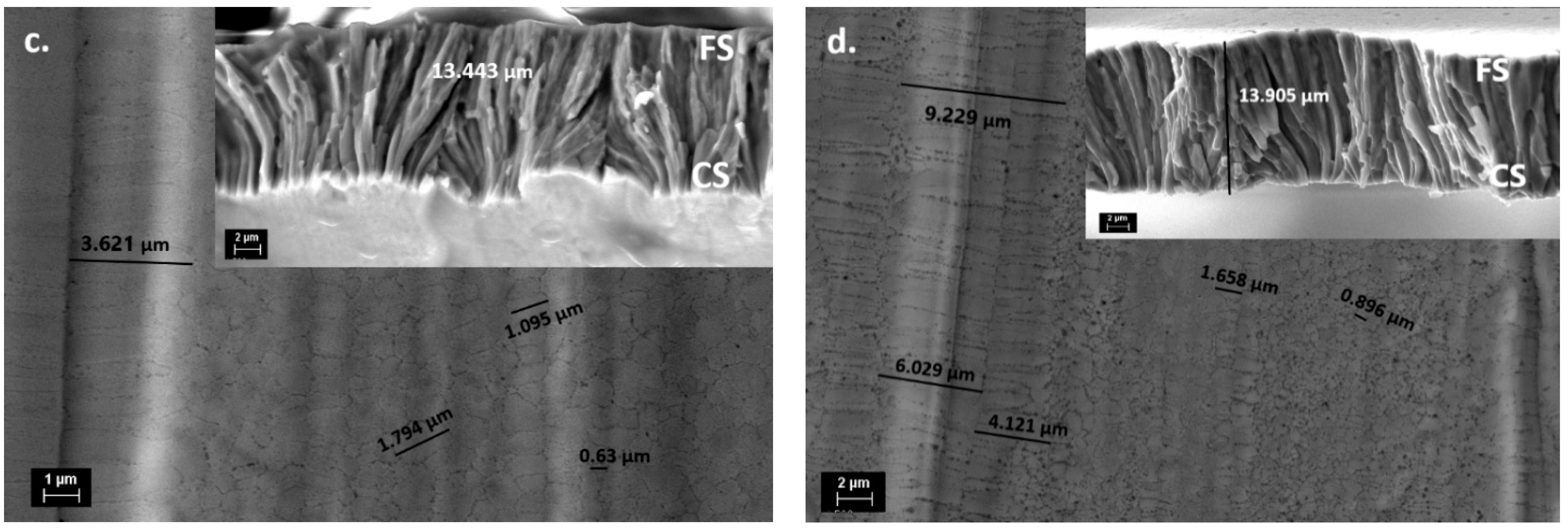
3.3. SEM
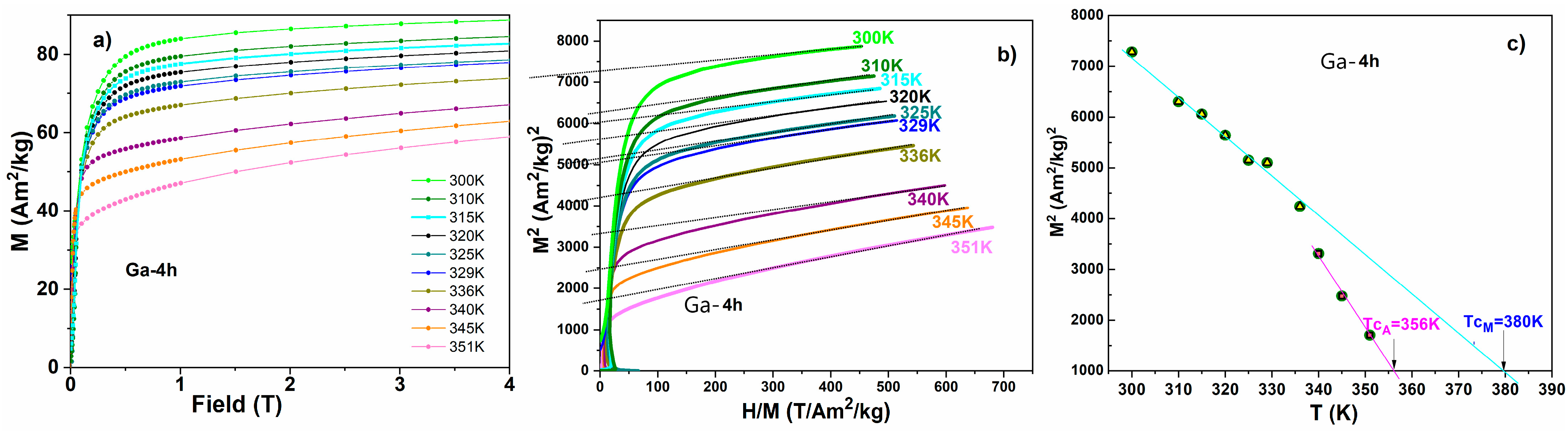
3.4. Magnetic Properties
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Nespoli, A.; Besseghini, S.; Pittaccio, S.; Villa, E.; Viscuso, S. The high potential of shape memory alloys in developing miniature mechanical devices: A review on shape memory alloy mini-actuators. Sens. Actuator A 2010, 158, 149–160. [Google Scholar] [CrossRef]
- Furuya, Y.; Shimada, H. Shape memory actuators for robotic applications. Mater. Des. 1991, 12, 21–28. [Google Scholar] [CrossRef]
- Ullakko, K.; Wendell, L.; Smith, A.; Müllner, P.; Hampikian, G. A magnetic shape memory micropump: Contact-free, and compatible with PCR and human DNA profiling. Smart Mater. Struct. 2012, 21, 115020. [Google Scholar] [CrossRef]
- Morgan, N.B. Medical shape memory alloy applications-the market and its products. Mater. Sci. Eng. A 2004, 378, 16–23. [Google Scholar] [CrossRef]
- Marioni, M.A.; O’Handley, R.C.; Allen, S.M. Pulsed magnetic field-induced actuation of Ni–Mn–Ga single crystals. Appl. Phys. Lett. 2003, 83, 3966. [Google Scholar] [CrossRef]
- Auernhammer, D.; Kohl, M.; Krevet, B.; Ohtsuka, M. Intrinsic position sensing of a Ni–Mn–Ga microactuator. Smart Mater. Struct. 2009, 18, 104016. [Google Scholar] [CrossRef]
- Hobza, A.; Patrick, C.L.; Ullakko, K.; Rafla, N.; Lindquist, P.; Müllner, P. Sensing strain with Ni-Mn-Ga. Sens. Actuators A Phys. 2018, 269, 137–144. [Google Scholar] [CrossRef]
- Songa, G.; Maa, N.; Li, H.-N. Applications of shape memory alloys in civil structures. Eng. Struct. 2006, 28, 1266–1274. [Google Scholar] [CrossRef]
- Dey, S.; Singh, S.; Roy, R.K.; Ghosh, M.; Mitra, A.; Panda, A.K. Influence of Mn incorporation for Ni on the magnetocaloric properties of rapidly solidified off-stoichiometric NiMnGa ribbons. J. Magn. Magn. Mater. 2016, 397, 342–346. [Google Scholar] [CrossRef]
- Satapathy, D.K.; Babu, P.D.; Aich, S. Magnetomechanical properties of melt-spun off stoichiometric Ni50Mn28Ga22 ribbons. J. Magn. Magn. Mater. 2021, 524, 167639. [Google Scholar] [CrossRef]
- Van Vilsteren, S.J.M.; Yarmand, H.; Ghodrat, S. Review of Magnetic Shape Memory Polymers and Magnetic Soft Materials. Magnetochemistry 2021, 7, 123. [Google Scholar] [CrossRef]
- Otsuka, K.; Wayman, C.M. Shape Memory Materials; Cambridge University Press: Cambridge, UK, 1998. [Google Scholar]
- Webster, P.J.; Ziebeck, K.R.A.; Town, S.L.; Peak, M.S. Magnetic order and phase transformation in Ni2MnGa. Philos. Mag. B 1984, 49, 295–310. [Google Scholar] [CrossRef]
- Chernenko, V.A.; Seguí, C.; Cesari, E.; Pons, J.; Kokorin, V.V. Sequence of martensitic transformations in Ni-Mn-Ga alloys. Phys. Rev. B 1998, 57, 2659–2662. [Google Scholar] [CrossRef] [Green Version]
- Zhu, J.; Tan, C.; Zhao, W.; Yang, Z.P.; Zhang, K.; Cai, W. The Crystallization Kinetics of Ni-Mn-Ga Magnetic Shape Memory Alloy Thin Films. J. Electron. Mater. 2019, 48, 2137–2143. [Google Scholar] [CrossRef]
- Pons, J.; Chernenko, V.A.; Santamarta, R.; Cesari, E. Crystal structure of martensitic phases in Ni–Mn–Ga shape memory alloys. Acta Mater. 2000, 48, 3027. [Google Scholar] [CrossRef]
- Niemann, R.; Rößler, U.K.; Gruner, M.E.; Heczko, O.; Schultz, L.; Fähler, S. The role of adaptive martensite in magnetic shape memory alloys. Adv. Eng. Mater. 2012, 14, 562–581. [Google Scholar] [CrossRef]
- Sánchez-Alarcos, V.; Pérez-Landazábal, J.I.; Recarte, V. Influence of long-range atomic order on the structural and magnetic properties of Ni-Mn-Ga ferromagnetic shape memory alloys. Mater. Sci. Forum. 2011, 684, 85. [Google Scholar]
- Heczko, O.; Svec, P.; Jankovic, D.; Ullakko, K. Magnetic properties of Ni-Mn-Ga ribbon prepared by rapid solidification. IEEE Trans. Magn. 2002, 38, 2841–2843. [Google Scholar] [CrossRef]
- Satapathy, D.K.; Biswas, S.; Aich, S. Microstructure and micro-texture evolution in rapidly solidified melt-spun Ni50Mn28Ga22 ribbons. J. Magn. Magn. Mater. 2021, 527, 167784. [Google Scholar] [CrossRef]
- Chernenko, V.A.; Cesari, E.; Pons, J.; Segui, C. Phase Transformations in Rapidly Quenched Ni–Mn–Ga Alloys. J. Mater. Research 2000, 15, 1496–1504. [Google Scholar] [CrossRef]
- Heczko, O. Magnetic shape memory effect and highly mobile twin boundaries. Mater. Sci. Techno. 2014, 30, 1559–1578. [Google Scholar] [CrossRef]
- Entel, P.; Buchelnikov, V.D.; Khovailo, V.V.; Zayak, A.T.; Adeagbo, W.A.; Gruner, M.E.; Herper, H.C.; Wassermann, E.F. Modelling the phase diagram of magnetic shape memory Heusler alloys. J. Phys. D Appl. Phys. 2006, 39, 865. [Google Scholar] [CrossRef]
- Gutiérrez, J.; Barandiarán, J.M.; Lázpita, P.; Seguí, C.; Cesari, E. Magnetic properties of a rapidly quenched Ni–Mn–Ga shape memory alloy. Sens. Actuators A. 2006, 129, 163. [Google Scholar] [CrossRef]
- Rama, R.; Gopalan, N.V.; Manivel Raja, R.; Arout Chelvane, M.; Majumdar, J.B.; Chandrasekaran, V. Magneto-structural transformation studies in melt-spun Ni–Mn–Ga ribbons. Scr. Mater. 2007, 56, 405. [Google Scholar] [CrossRef]
- Goryszka, T.; Lelatko, J.; Gorka-Kostrubiec, B.; Ochin, P.; Morawiec, H. Martensitic transformation in melt-spun Ni-Mn-Ga ribbons. Eur. Phys. J. Spec. Top. 2008, 158, 131–136. [Google Scholar] [CrossRef]
- Pasquale, M.; Sasso, C.P.; Lewis, L.H.; Giudici, L.; Lograsso, T.; Schlagel, D. Magnetostructural transition and magnetocaloric effect in Ni55Mn20Ga25 single crystals. Phys. Rev. B 2005, 72, 094435. [Google Scholar] [CrossRef] [Green Version]
- Liang, X.; Bai, J.; Gu, J.; Yan, H.; Zhang, Y.; Esling, C.; Zhao, X.; Zuo, L. Probing martensitic transformation, kinetics, elastic and magnetic properties of Ni2-xMn1.5In0.5Cox alloys. J. Mater. Sci. Techno. 2020, 44, 31–41. [Google Scholar] [CrossRef]
- Pérez-Reche, F.-J.; Stipcich, M.; Vives, E.; Mañosa, L.; Planes, A.; Morin, M. Kinetics of martensitic transitions in Cu-Al-Mn under thermal cycling: Analysis at multiple length scales. Phys. Rev. B 2004, 69, 64101. [Google Scholar] [CrossRef] [Green Version]
- Christian, J. The Theory of Transformations in Metals and Alloys; Pergamon Elsevier Science: Oxford, UK, 2002. [Google Scholar]
- Vyazovkin, S.; Dollimore, D. Linear and Nonlinear Procedures in Isoconversional Computations of the Activation Energy of Nonisothermal Reactions. Solids J. Chem. Inf. Comput. Sci. 1996, 36, 42. [Google Scholar] [CrossRef]
- Vyazovkin, S. Thermal Analysis. Anal. Chem. 2008, 80, 4301–4316. [Google Scholar] [CrossRef]
- Friedman, H. Kinetics of thermal degradation of char-forming plastics from thermogravimetry: Application to a phenolic plastic. J. Polym. Sci. C 1964, 6, 183. [Google Scholar] [CrossRef]
- Ozawa, T. A New Method of Analyzing Thermogravimetric Data. Bull. Chem. Soc. Japan 1965, 38, 1881–1886. [Google Scholar] [CrossRef] [Green Version]
- Kissinger, H. Reaction Kinetics in Differential Thermal Analysis. Anal. Chem. 1957, 29, 1702–1706. [Google Scholar] [CrossRef]
- Li, Z.; Zhang, Y.; Sáanchez-Valdés, C.F.; Sánchez Llamazares, J.L.; Esling, C.; Zhao, X.; Zuo, L. Giant magnetocaloric effect in melt-spun Ni-Mn-Ga ribbons with magneto-multi structural transformation. Appl. Phys. Lett. 2014, 104, 044101. [Google Scholar] [CrossRef] [Green Version]
- Lanska, N.; Soderberg, O.; Sozinov, A.; Ge, Y.; Ullakko, K.; Lindroos, V.K. Composition and temperature dependence of the crystal structure of Ni–Mn–Ga alloys. J. Appl. Phys. 2004, 95, 8074–8078. [Google Scholar] [CrossRef] [Green Version]
- Söderberg, O. Novel Ni-Mn-Ga Alloys and Their Magnetic Shape Memory Behaviour. PhD Thesis, Helsinki University of Technology, Espoo, Finland, 10 December 2004. [Google Scholar]
- Liu, J.; Scheerbaum, N.; Hinz, D.; Gutfleisch, O. Martensitic transformation and magnetic properties in Ni-Fe-Ga-Co magnetic shape memory alloys. Acta Mater. 2008, 56, 3177–3186. [Google Scholar] [CrossRef]
- Wang, J.; Han, Y.; Hua, H.; Wang, X.; Jiang, C. Grain size effect on the martensitic transformation of Ni50Mn25Ga17Cu8 high-temperature shape memory alloy. Intermetallics 2015, 61, 42–46. [Google Scholar] [CrossRef]
- Zhou, Z.N.; Yang, L.; Li, R.C.; Li, J.; Hu, Q.D.; Li, J.G. Martensitic transformations and kinetics in Ni-Mn-In-Mg shape memory alloys. Intermetallics 2018, 92, 49–54. [Google Scholar] [CrossRef]
- Manchón-Gordón, A.F.; Ipus, J.J.; Kowalczyk, M.; Wójcik, A.; Blázquez, J.S.; Conde, C.F.; Maziarz, W.; Švec, S.P.; Kulik, T.; Conde, A. Effect of pressure on the phase stability and magnetostructural transitions in nickel-rich NiFeGa ribbons. J. Alloys. Compd. 2020, 844, 156092. [Google Scholar] [CrossRef]
- Albertini, F.; Besseghini, S.; Paoluzi, A.; Pareti, L.; Pasquale, M.; Passaretti, F.; Sasso, C.P.; Stantero, A.; Villa, E. Structural, magnetic and anisotropic properties of Ni2MnGa melt-spun ribbons. J. Magn. Magn. Mater. 2002, 1421, 242–245. [Google Scholar] [CrossRef]
- Rajkumar, D.; Sridhara Rao, D.; Rama Rao, N.; Manivel Raja, M.; Singh, R.; Suresh, K. In-situ phase transformation studies of Ni48Mn39In13 melt-spun ribbons. Intermetallics 2012, 25, 126–130. [Google Scholar] [CrossRef]
- Heczko, O.; Fähler, S.; Vasilchikova, T.M.; Voloshok, T.N.; Klimov, K.V.; Chumlyakov, Y.I.; Vasiliev, A.N. Thermodynamic, kinetic, and magnetic properties of a Ni54Fe19Ga27 magnetic shape-memory single crystal. Phys. Rev. B 2008, 77, 174402. [Google Scholar] [CrossRef]
- Arrott, A. Criterion for Ferromagnetism from Observations of Magnetic Isotherms. Phys. Rev. 1957, 108, 1394. [Google Scholar] [CrossRef]
- Lyubina, J.; Gutfleisch, O.; Kuz’min, M.D.; Richter, M.J. La(Fe,Si)13-based magnetic refrigerants obtained by novel processing routes. Magn. Magn. Mater 2008, 320, 2252–2258. [Google Scholar] [CrossRef]
- Kübler, J.; Williams, A.R.; Sommers, C.B. Formation and coupling of magnetic moments in Heusler alloys. Phys. Rev. B 1983, 28, 1745. [Google Scholar] [CrossRef]
- Tolea, F.; Sofronie, M.; Crisan, A.D.; Enculescu, M.; Kuncser, V.; Valeanu, M. Effect of thermal treatments on the structural and magnetic transitions in melt-spun Ni-Fe-Ga-(Co) ribbons. J. Alloys. Compd. 2015, 650, 664–670. [Google Scholar] [CrossRef]
- Sofronie, M.; Tolea, F.; Kuncser, V.; Valeanu, M. Martensitic transformation and accompanying magnetic changes in Ni–Fe–Ga–Co alloys. J. Appl. Phys. 2010, 107, 113905. [Google Scholar] [CrossRef]
- Erdélyi, G.; Mehrer, H.; Imre, A.W.; Lograsso, T.A.; Schlagel, D.L. Self-diffusion in Ni2MnGa. Intermetallics 2007, 15, 1078–1083. [Google Scholar] [CrossRef]
- Ma, Y.Q.; Jiang, C.B.; Li, Y.; Xu, H.B.; Wang, C.P.; Liu, X.J. Study of Ni50+xMn25Ga25-x (x=2-11) as high-temperature shape-memory alloys. Acta Mater. 2007, 55, 1533–1541. [Google Scholar] [CrossRef]
- Xin, Y.; Li, Y.; Chai, L.; Xu, H.B. Shape memory characteristics of dual-phase Ni-Mn-Ga based high-temperature shape memory alloys. Scr. Mater. 2007, 57, 599–601. [Google Scholar] [CrossRef]
- Picornell, C.; Pons, J.; Cesari, E.; Dutkiewicz, J. Thermal characteristics of Ni–Fe–Ga–Mn and Ni–Fe–Ga–Co ferromagnetic shape memory alloys. Intermetallics 2008, 16, 751–757. [Google Scholar] [CrossRef]
- Tickle, R.; James, R.D. Magnetic and magnetomechanical properties of Ni2 MnGa. J. Magn. Magn. Mater. 1999, 195, 627–638. [Google Scholar] [CrossRef]
- Mérida, D.; Unzueta, I.; Sánchez-Alarcos, V.; Recarte, V.; Pérez-Landazábal, J.I.; García, J.A.; Plazaola, F. Vacancies mediated ordering in Ni-Mn-Ga shape memory alloys. Scr. Mater. 2022, 215, 114731. [Google Scholar] [CrossRef]
- Unzueta, I.; Sánchez-Alarcos, V.; Recarte, V.; Pérez-Landazábal, J.I.; Zabala, N.; García, J.A.; Plazaola, F. Identification of a Ni-vacancy defect in Ni-Mn-Z (Z=Ga, Sn, In): An experimental and DFT positron-annihilation study. Phys. Rev. B 2019, 99, 064108. [Google Scholar] [CrossRef]
- Mérida, D.; García, J.A.; Sánchez-Alarcos, V.; Pérez-Landazábal, J.I.; Recarte, V.; Plazaola, F. Vacancy dynamic in Ni-Mn-Ga ferromagnetic shape memory alloys. Appl. Phys. Lett. 2014, 104, 231905. [Google Scholar] [CrossRef]
- Mérida, D.; García, J.; Sánchez-Alarcos, V.; Pérez-Landazábal, J.; Recarte, V.; Plazaola, F. Characterisation and modelling of vacancy dynamics in Ni–Mn–Ga ferromagnetic shape memory alloys. J. Alloys. Compd. 2015, 639, 180. [Google Scholar] [CrossRef]


| Sample | Phase | a (Å) | b (Å) | c (Å) | β (Degree) | V (Å3) | c/a |
|---|---|---|---|---|---|---|---|
| Ga-0h | 7M (I2/m) | 4.276(3) | 5.445(7) | 4.234(1) | 90.541 | 98.597(8) | 0.990(1) |
| NM (I4/mmm) | 4.094(5) | - | 6.730(6) | - | 112.838(4) | 1.643(8) | |
| L21 (Fm-3m) | 5.839(1) | - | - | - | 199.085(8) | - | |
| Ga-1h | 7M (I2/m) | 4.267(5) | 5.447(2) | 4.227(9) | 90.536 | 98.276(8) | 0.990(7) |
| NM (I4/mmm) | 4.075(6) | - | 6.737(9) | - | 111.918(3) | 1.653(2) | |
| L21 (Fm-3m) | 5.833(4) | - | - | - | 198.497(5) | - | |
| Ga-4h | 7M (I2/m) | 4.273(4) | 5.438(4) | 4.228(6) | 90.489 | 98.272(7) | 0.989(5) |
| NM (I4/mmm) | 4.098(1) | - | 6.717(6) | - | 112.820 | 1.639(1) | |
| L21 (Fm-3m) | 5.829(6) | - | - | - | 198.118(2) | - | |
| Ga-8h | 7M (I2/m) | 4.271(4) | 5.437 | 4.228(9) | 90.501 | 98.206(3) | 0.99 |
| NM (I4/mmm) | 4.112(9) | - | 6.710(2) | - | 113.509(7) | 1.631(5) | |
| L21 (Fm-3m) | 5.833(1) | - | - | - | 198.475(6) | - |
| Sample | Ms // Mf (K) | As // Af (K) | H (J/g) | T0 (K) | TC-DSC (K) | TCM // TCA (K) |
|---|---|---|---|---|---|---|
| Ga-0h | 336 // 325 | 334 // 343 | 6.205 | 339.5 | 357.5 | 348 // 345 |
| Ga-1h | 341 // 329 | 339 // 348 | 6.84 | 344.5 | 359 | 400 // 362 |
| Ga-4h | 340 // 329 | 338 // 347 | 6.6 | 343.5 | 359.5 | 380 //356 |
| Ga-8h | 344 // 330 | 340 // 351 | 7.27 | 347.5 | 360.5 | 382 //357 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tolea, F.; Popescu, B.; Bartha, C.; Enculescu, M.; Tolea, M.; Sofronie, M. Kinetics and the Effect of Thermal Treatments on the Martensitic Transformation and Magnetic Properties in Ni49Mn32Ga19 Ferromagnetic Shape Memory Ribbons. Magnetochemistry 2023, 9, 7. https://doi.org/10.3390/magnetochemistry9010007
Tolea F, Popescu B, Bartha C, Enculescu M, Tolea M, Sofronie M. Kinetics and the Effect of Thermal Treatments on the Martensitic Transformation and Magnetic Properties in Ni49Mn32Ga19 Ferromagnetic Shape Memory Ribbons. Magnetochemistry. 2023; 9(1):7. https://doi.org/10.3390/magnetochemistry9010007
Chicago/Turabian StyleTolea, Felicia, Bogdan Popescu, Cristina Bartha, Monica Enculescu, Mugurel Tolea, and Mihaela Sofronie. 2023. "Kinetics and the Effect of Thermal Treatments on the Martensitic Transformation and Magnetic Properties in Ni49Mn32Ga19 Ferromagnetic Shape Memory Ribbons" Magnetochemistry 9, no. 1: 7. https://doi.org/10.3390/magnetochemistry9010007
APA StyleTolea, F., Popescu, B., Bartha, C., Enculescu, M., Tolea, M., & Sofronie, M. (2023). Kinetics and the Effect of Thermal Treatments on the Martensitic Transformation and Magnetic Properties in Ni49Mn32Ga19 Ferromagnetic Shape Memory Ribbons. Magnetochemistry, 9(1), 7. https://doi.org/10.3390/magnetochemistry9010007







