Analysis of Low-Temperature Magnetotransport Properties of NbN Thin Films Grown by Atomic Layer Deposition
Abstract
:1. Introduction
2. Materials and Methods
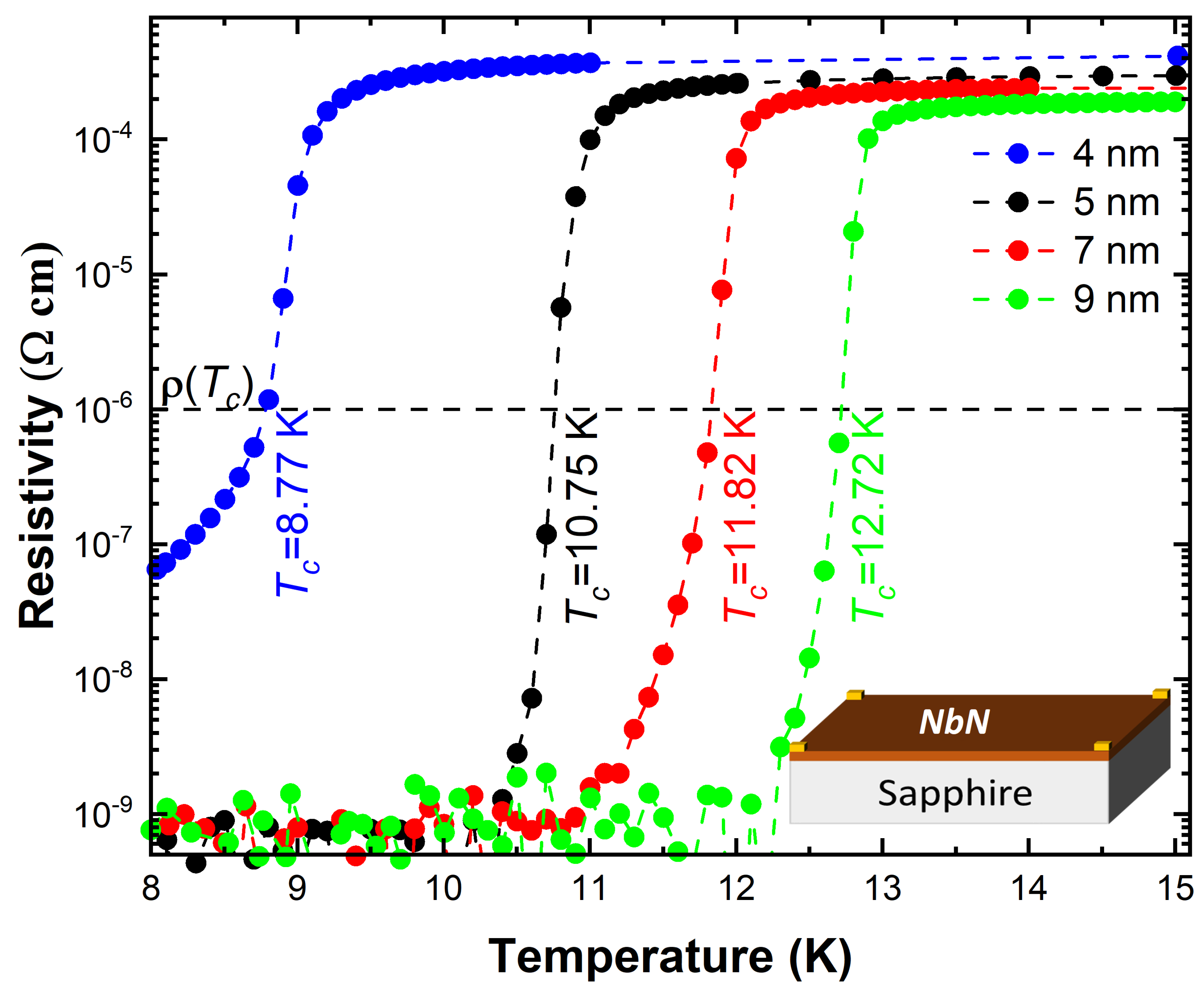
2.1. Critical Temperature
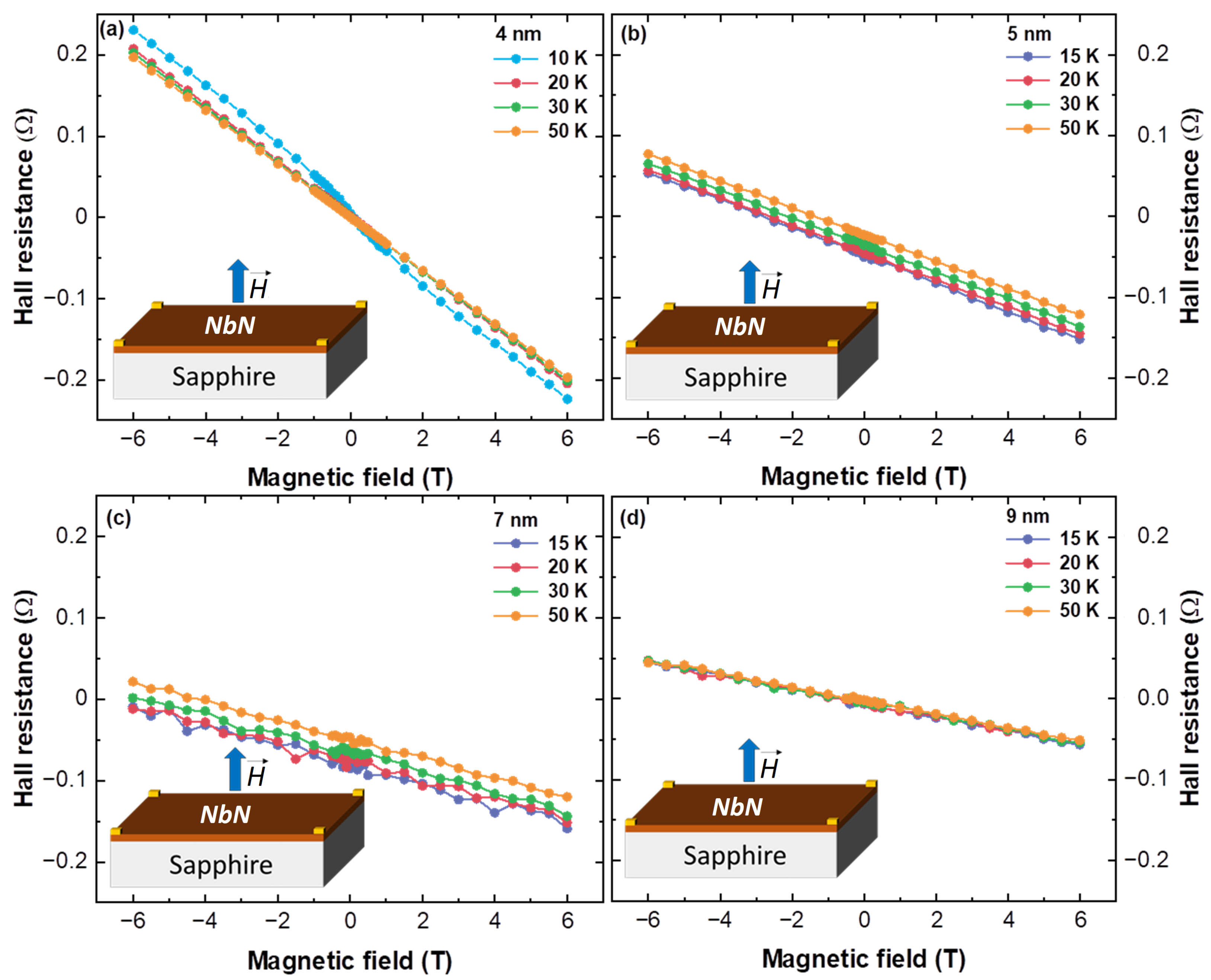
2.2. Hall Resistance
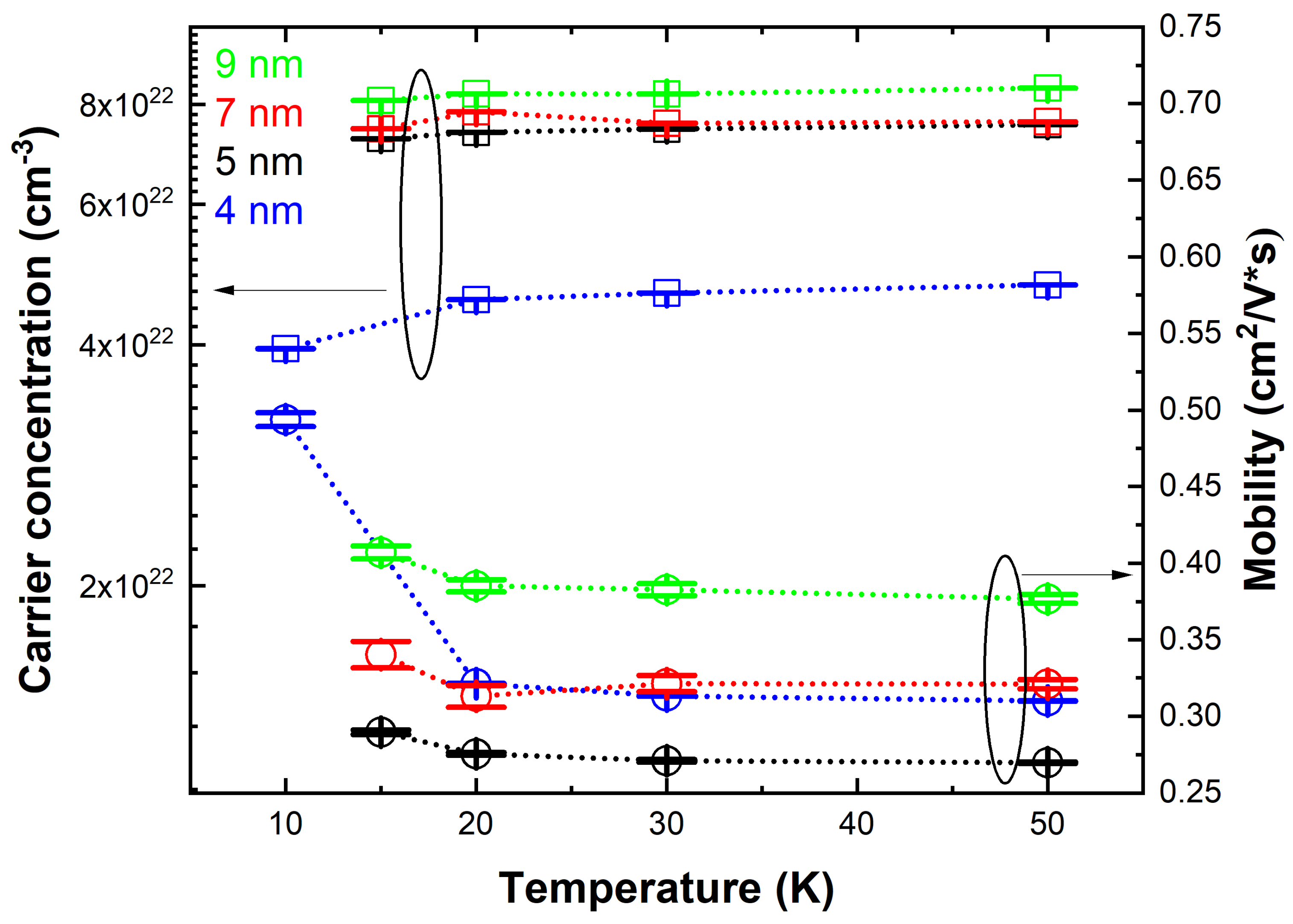
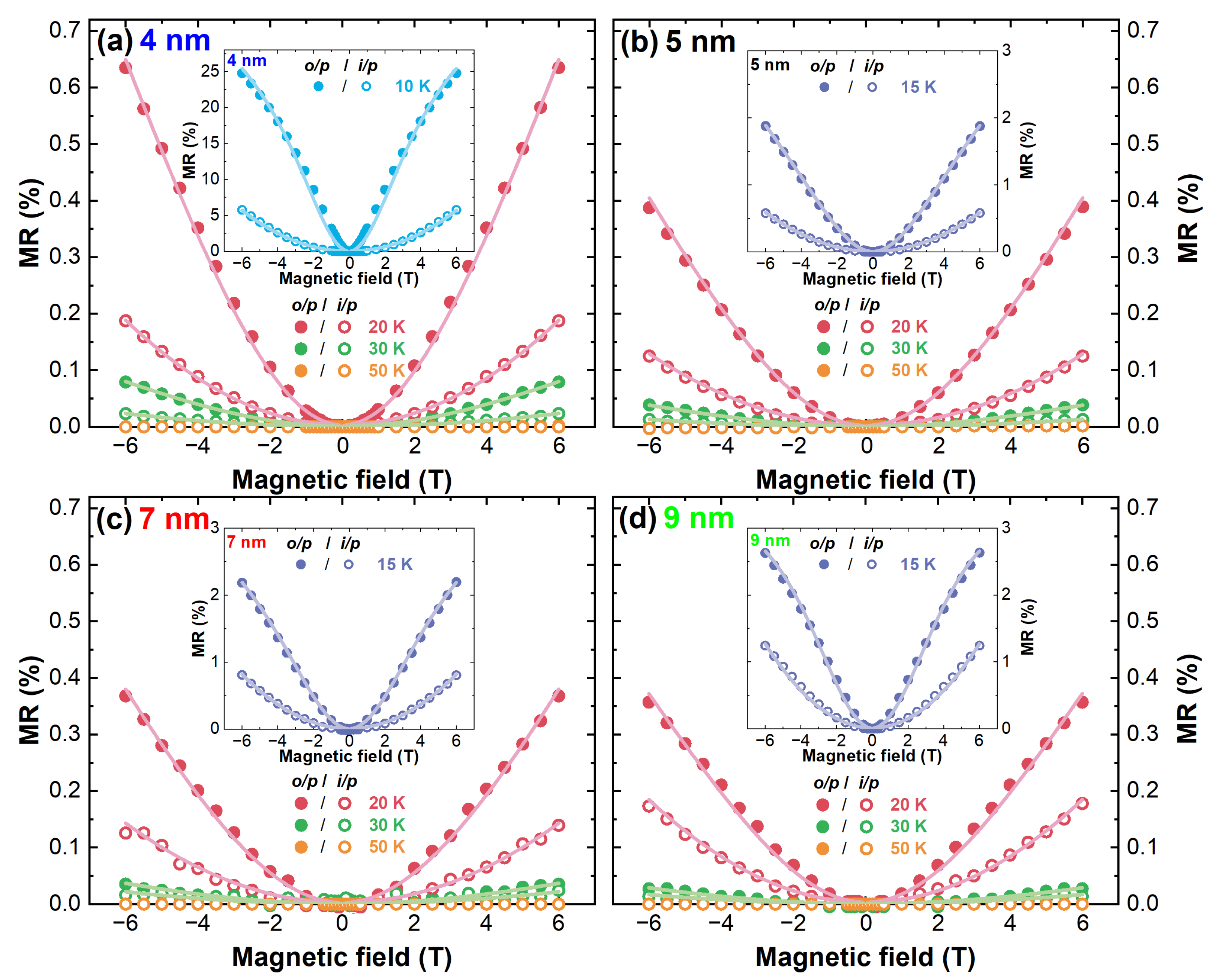
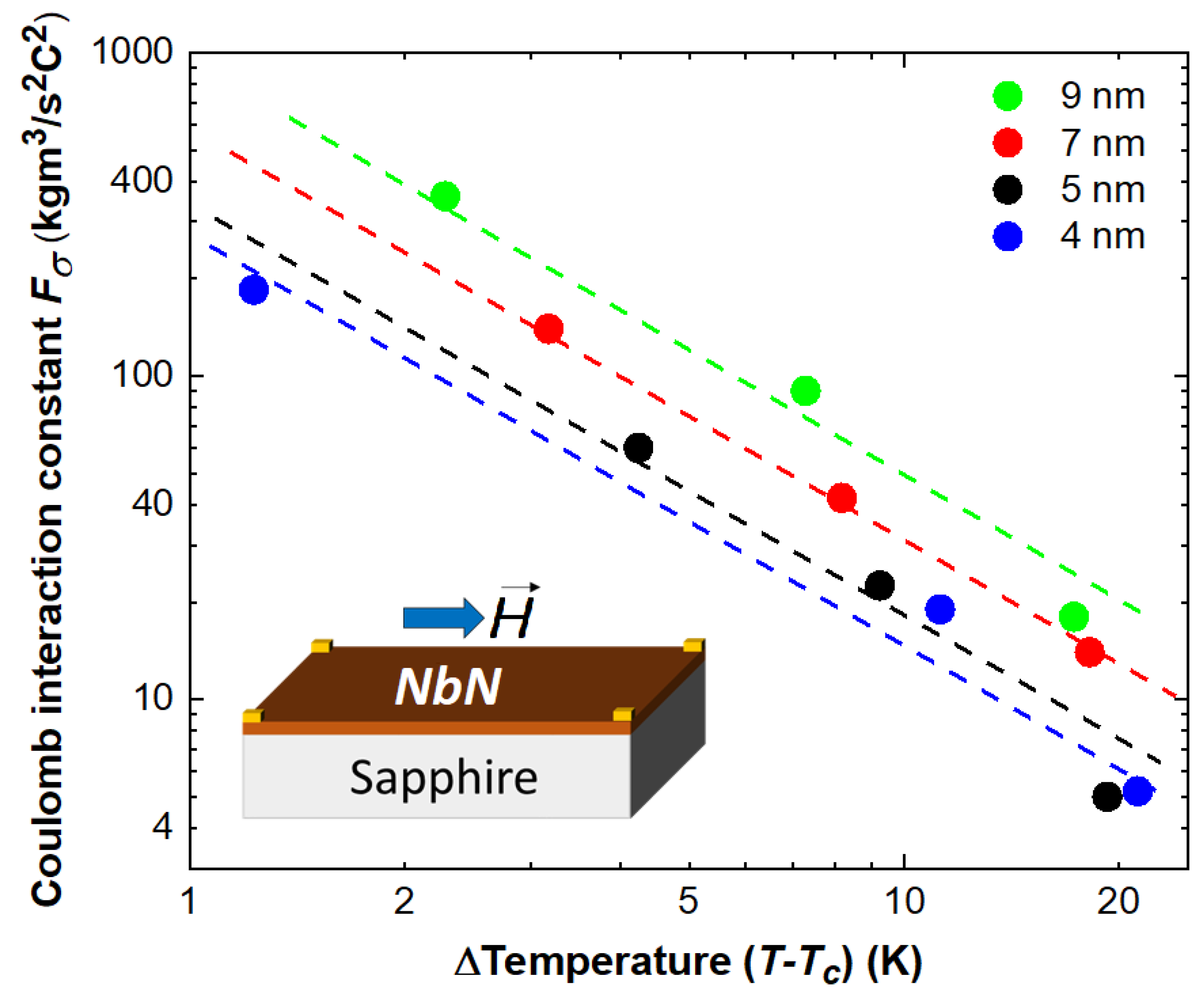
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lee, P.A.; Ramakrishnan, T.V. Disordered electronic systems. Rev. Mod. Phys. 1985, 57, 287. [Google Scholar] [CrossRef]
- Rogacheva, E.; Pavlosiuk, O.; Meriuts, A.; Shelest, T.; Sipatov, A.; Nashchekina, O.; Novak, K.; Kaczorowski, D. Quantum interference phenomena and electron–electron interaction in topological insulator Bi2Se3 thin polycrystalline films. Thin Solid Films 2022, 743, 139070. [Google Scholar] [CrossRef]
- Chatterjee, S.; Chatterjee, S.; Giri, S.; Majumdar, S. Transport properties of Heusler compounds and alloys. J. Phys. Condens. Matter 2021, 34, 013001. [Google Scholar] [CrossRef]
- Mal, B.; Banerjee, M.; Maiti, S.K. Magnetotransport in fractal network with loop sub-structures: Anisotropic effect and delocalization. Phys. Lett. A 2020, 384, 126378. [Google Scholar] [CrossRef]
- Barbosa, A.L.R.; Lima, J.R.F.; Bezerra, Í.S.F.; Lyra, M.L. Electronic transport in disordered graphene superlattices with scale-free correlated barrier spacements. Phys. E Low Dimens. Syst. Nanostruct. 2020, 124, 114210. [Google Scholar] [CrossRef]
- Xu, Q.; Hartmann, L.; Schmidt, H.; Hochmuth, H.; Lorenz, M.; Spemann, D.; Grundmann, M. s-d exchange interaction induced magnetoresistance in magnetic ZnO. Phys. Rev. B 2007, 76, 134417. [Google Scholar] [CrossRef]
- Vegesna, S.V.; Bürger, D.; Patra, R.; Dellith, J.; Abendroth, B.; Skorupa, I.; Schmidt, O.G.; Schmidt, H. Tunable large field magnetoconductance of ZnO, ZnMnO, and ZnCoO thin films. J. Appl. Phys. 2019, 125, 215305. [Google Scholar] [CrossRef]
- Shinozaki, B.; Ezaki, S.; Odou, T.; Asano, T.; Makise, K. Anomalous electron inelastic scattering rate probed via superconducting fluctuation in epitaxial NbN thin films. Phys. C Supercond. Appl. 2019, 567, 1353547. [Google Scholar] [CrossRef]
- Lo, S.T.; Lin, S.W.; Wang, Y.T.; Lin, S.D.; Liang, C.-T. Spin-orbit-coupled superconductivity. Sci. Rep. 2014, 4, 5438. [Google Scholar] [CrossRef]
- Matthias, B.T.; Geballe, T.H.; Compton, V.B. Superconductivity. Rev. Mod. Phys. 1963, 35, 1. [Google Scholar] [CrossRef]
- Linzen, S.; Ziegler, M.; Astafiev, O.V.; Schmelz, M.; Hübner, U.; Diegel, M.; Il’ichev, E.; Meyer, H.-G. Structural and electrical properties of ultrathin niobium nitride films grown by atomic layer deposition. Supercond. Sci. Technol. 2017, 30, 035010. [Google Scholar] [CrossRef]
- Ziegler, M.; Linzen, S.; Goerke, S.; Bruckner, U.; Plentz, J.; Dellith, J.; Himmerlich, A.; Himmerlich, M.; Hubner, U.; Krischok, S.; et al. Effects of Plasma Parameter on Morphological and Electrical Properties of Superconducting Nb-N Deposited by MO-PEALD. IEEE Trans. Appl. Supercond. 2017, 27, 7501307. [Google Scholar] [CrossRef]
- Knehr, E.; Ziegler, M.; Linzen, S.; Ilin, K.; Schanz, P.; Plentz, J.; Diegel, M.; Schmidt, H.; Il’ichev, E.; Siegel, M. Wafer-level uniformity of atomic-layer-deposited niobium nitride thin films for quantum devices. J. Vac. Sci. Technol. A 2021, 39, 052401. [Google Scholar] [CrossRef]
- Nazir, M.; Yang, X.; Tian, H.; Song, P.; Wang, Z.; Xiang, Z.; Guo, X.; Jin, Y.; You, L.; Zheng, D. Investigation of dimensionality in superconducting NbN thin film samples with different thicknesses and NbTiN meander nanowire samples by measuring the upper critical field. Chin. Phys. B 2020, 29, 087401. [Google Scholar] [CrossRef]
- Zhang, Q.; Wang, H.; Tang, X.; Peng, W.; Wang, Z. Superconductivity Dependence on Epitaxial NbN Film Thickness. IEEE Trans. Appl. Supercond. 2019, 29, 7500305. [Google Scholar] [CrossRef]
- Ivry, Y.; Kim, C.-S.; Dane, A.E.; Fazio, D.D.; McCaughan, A.N.; Sunter, K.A.; Zhao, Q.; Berggren, K.K. Universal scaling of the critical temperature for thin films near the superconducting-to-insulating transition. Phys. Rev. B 2014, 90, 214515. [Google Scholar] [CrossRef] [Green Version]
- Huang, S.-Y.; Qu, D.; Chien, C.-L. Chapter Three—Charge, Spin, and Heat Transport in the Proximity of Metal/Ferromagnet Interface. In Recent Advances in Magnetic Insulators—From Spintronics to Microwave Applications; Wu, M., Hoffmann, A., Eds.; Academic Press: San Diego, CA, USA, 2013; pp. 53–82. [Google Scholar]
- Burdis, M.S.; Dean, C.C. Anomalous values of interaction constants in the two-dimensional electron gas of a silicon metal-oxide-semiconductor field-effect transistor measured by parallel- and perpendicular-field magnetoconductivity. Phys. Rev. B 1988, 38, 3269–3275. [Google Scholar] [CrossRef]
- Ousset, J.C.; Askenazy, S.; Rakoto, H.; Broto, J.M. Analytic expressions of the magnetoresistance due to localization and electron-electron interaction effects—Application to the amorphous alloys La3Al and La3Ga. J. Phys. 1985, 46, 2145–2149. [Google Scholar] [CrossRef]
- Bardeen, J.; Cooper, L.N.; Schrieffer, J.R. Theory of Superconductivity. Phys. Rev. 1957, 108, 1175. [Google Scholar] [CrossRef] [Green Version]
- Zheng, X.H.; Walmsley, D.G. Coulomb repulsion and Tc in BCS theory of superconductivity. Phys. Rev. B 2005, 71, 134512. [Google Scholar] [CrossRef]
- Mattheiss, L.F. Electronic Band Structure of Niobium Nitride. Phys. Rev. B 1972, 5, 315–320. [Google Scholar] [CrossRef]
- Chockalingam, S.P.; Chand, M.; Jesudasan, J.; Tripathi, V.; Raychaudhuri, P. Superconducting properties and Hall effect of epitaxial NbN thin films. Phys. Rev. B 2008, 77, 214503. [Google Scholar] [CrossRef] [Green Version]
- Hettinger, M.; Khodas, M.; Levchenko, A. Anomalous mesoscopic kinetics in disordered superconductors. Phys. Rev. B 2019, 99, 174504. [Google Scholar] [CrossRef] [Green Version]
| Sample | Temperature | |||||
|---|---|---|---|---|---|---|
| K | kgm/sC | meV | nm | |||
| i/p and o/p | o/p | o/p | o/p | o/p | ||
| 4 nm | 10 | 185 | 1.38 | 6.0 | 185 | 3.61 |
| 20 | 19 | 1.07 | 4.6 | 1 | 2.58 | |
| 30 | 5 | 1.07 | 4.0 | 1 | 2.55 | |
| 5 nm | 15 | 60 | 1.09 | 4.0 | 60 | 3.17 |
| 20 | 22 | 0.95 | 4.0 | 1 | 3.06 | |
| 30 | 5 | 0.95 | 4.0 | 1 | 3.02 | |
| 7 nm | 15 | 140 | 1.23 | 4.8 | 140 | 3.79 |
| 20 | 42 | 0.79 | 4.8 | 1 | 3.61 | |
| 30 | 14 | 0.79 | 4.8 | 1 | 3.62 | |
| 9 nm | 15 | 360 | 1.25 | 5.2 | 360 | 4.79 |
| 20 | 90 | 0.85 | 4.9 | 23 | 4.60 | |
| 30 | 18 | 0.75 | 4.9 | 1 | 4.56 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Vegesna, S.V.; Lanka, S.V.; Bürger, D.; Li, Z.; Linzen, S.; Schmidt, H. Analysis of Low-Temperature Magnetotransport Properties of NbN Thin Films Grown by Atomic Layer Deposition. Magnetochemistry 2022, 8, 33. https://doi.org/10.3390/magnetochemistry8030033
Vegesna SV, Lanka SV, Bürger D, Li Z, Linzen S, Schmidt H. Analysis of Low-Temperature Magnetotransport Properties of NbN Thin Films Grown by Atomic Layer Deposition. Magnetochemistry. 2022; 8(3):33. https://doi.org/10.3390/magnetochemistry8030033
Chicago/Turabian StyleVegesna, Sahitya V., Sai V. Lanka, Danilo Bürger, Zichao Li, Sven Linzen, and Heidemarie Schmidt. 2022. "Analysis of Low-Temperature Magnetotransport Properties of NbN Thin Films Grown by Atomic Layer Deposition" Magnetochemistry 8, no. 3: 33. https://doi.org/10.3390/magnetochemistry8030033
APA StyleVegesna, S. V., Lanka, S. V., Bürger, D., Li, Z., Linzen, S., & Schmidt, H. (2022). Analysis of Low-Temperature Magnetotransport Properties of NbN Thin Films Grown by Atomic Layer Deposition. Magnetochemistry, 8(3), 33. https://doi.org/10.3390/magnetochemistry8030033






