AC Susceptibility Studies of Magnetic Relaxation in Mn12-Stearate SMMs on the Spherical Silica Surface
Abstract
:1. Introduction
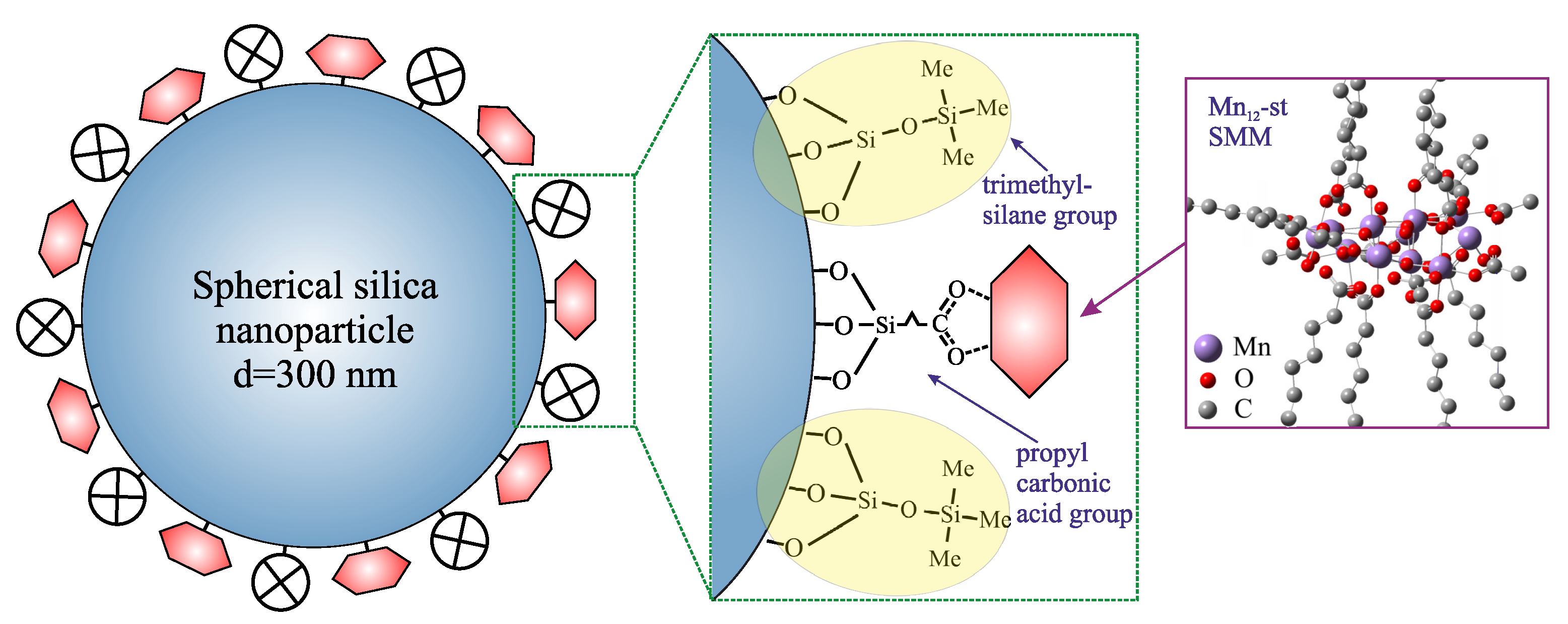
2. Materials and Methods
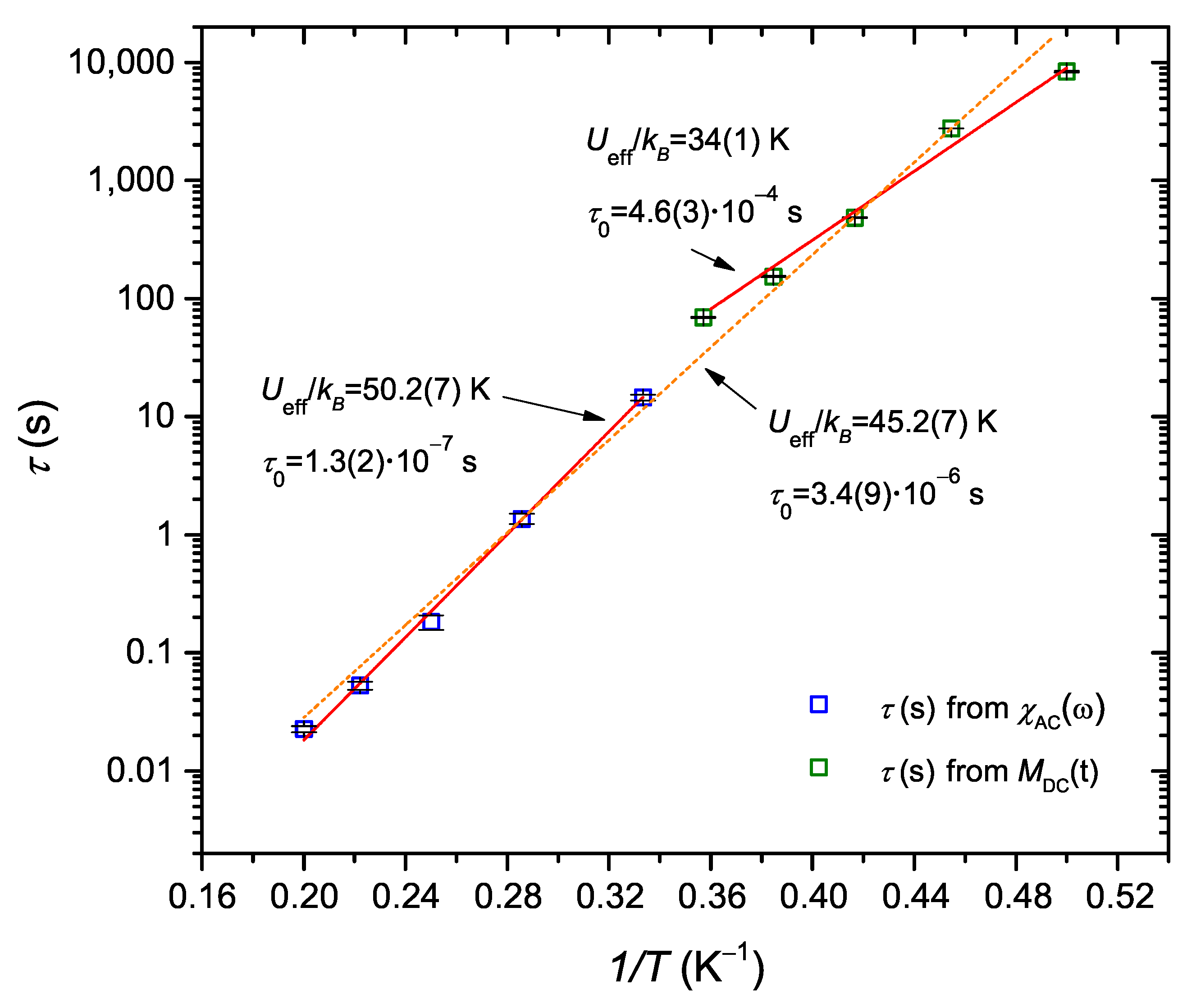
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Sessoli, R.; Gatteschi, D.; Caneschi, A.; Novak, M. Magnetic bistability in a metal-ion cluster. Nature 1993, 365, 141–143. [Google Scholar] [CrossRef]
- Christou, G.; Gatteschi, D.; Hendrickson, D.N.; Sessoli, R. Single-molecule magnets. MRS Bull. 2000, 25, 66–71. [Google Scholar] [CrossRef]
- Bartolome, S.J.; Luis, F.; Fernández, J.F. Molecular Magnets: Physics and Applications; Springer: Berlin/Heidelberg, Germany, 2014; p. 395. [Google Scholar]
- Cornia, A.; Mannini, M.; Sainctavit, P.; Sessoli, R. Chemical strategies and characterization tools for the organization of single molecule magnets on surfaces. Chem. Soc. Rev. 2011, 40, 3076–3091. [Google Scholar] [CrossRef] [PubMed]
- Cornia, A.; Fabretti, A.C.; Pacchioni, M.; Zobbi, L.; Bonacchi, D.; Caneschi, A.; Gatteschi, D.; Biagi, R.; Del Pennino, U.; De Renzi, V.; et al. Direct observation of single-molecule magnets organized on gold surfaces. Angew. Chem. Int. Ed. 2003, 42, 1645–1648. [Google Scholar] [CrossRef]
- Mannini, M.; Pineider, F.; Sainctavit, P.; Danieli, C.; Otero, E.; Sciancalepore, C.; Talarico, A.M.; Arrio, M.A.; Cornia, A.; Gatteschi, D.; et al. Magnetic memory of a single-molecule quantum magnet wired to a gold surface. Nat. Mater. 2009, 8, 194–197. [Google Scholar] [CrossRef] [PubMed]
- Voss, S.; Burgert, M.; Fonin, M.; Groth, U.; Rüdiger, U. A comparative study on the deposition of Mn 12 single molecule magnets on the Au (111) surface. Dalton Trans. 2008, 499–505. [Google Scholar] [CrossRef] [Green Version]
- Zobbi, L.; Mannini, M.; Pacchioni, M.; Chastanet, G.; Bonacchi, D.; Zanardi, C.; Biagi, R.; Del Pennino, U.; Gatteschi, D.; Cornia, A.; et al. Isolated single-molecule magnets on native gold. Chem. Commun. 2005, 12, 1640–1642. [Google Scholar] [CrossRef]
- Mannini, M.; Pineider, F.; Danieli, C.; Totti, F.; Sorace, L.; Sainctavit, P.; Arrio, M.A.; Otero, E.; Joly, L.; Cezar, J.C.; et al. Quantum tunnelling of the magnetization in a monolayer of oriented single-molecule magnets. Nature 2010, 468, 417–421. [Google Scholar] [CrossRef] [PubMed]
- Cornia, A.; Mannini, M. Single-Molecule Magnets on Surfaces. In Molecular Nanomagnets and Related Phenomena; Springer: Berlin/Heidelberg, Germany, 2014; pp. 293–331. [Google Scholar]
- Laskowski, L.; Kityk, I.; Konieczny, P.; Pastukh, O.; Schabikowski, M.; Laskowska, M. The Separation of the Mn12 Single-Molecule Magnets onto Spherical Silica Nanoparticles. Nanomaterials 2019, 9, 764. [Google Scholar] [CrossRef] [Green Version]
- Laskowska, M.; Pastukh, O.; Kuźma, D.; Laskowski, Ł. How to Control the Distribution of Anchored, Mn12–Stearate, Single-Molecule Magnets. Nanomaterials 2019, 9, 1730. [Google Scholar] [CrossRef] [Green Version]
- Laskowska, M.; Pastukh, O.; Konieczny, P.; Dulski, M.; Zalsiński, M.; Laskowski, L. Magnetic behaviour of Mn12-stearate single-molecule magnets immobilized on the surface of 300 nm spherical silica nanoparticles. Materials 2020, 13, 2624. [Google Scholar] [CrossRef]
- Pastukh, O.; Konieczny, P.; Czernia, D.; Laskowska, M.; Dulski, M.; Laskowski, Ł. Aging effect on the magnetic properties of Mn12-stearate single-molecule magnets anchored onto the surface of spherical silica nanoparticles. Mater. Sci. Eng. B 2020, 261, 114670. [Google Scholar] [CrossRef]
- Balanda, M. AC susceptibility studies of phase transitions and magnetic relaxation: Conventional, molecular and low-dimensional magnets. Acta Phys. Pol. A 2013, 124, 964–976. [Google Scholar] [CrossRef]
- Cole, K.S.; Cole, R.H. Dispersion and absorption in dielectrics I. Alternating current characteristics. J. Chem. Phys. 1941, 9, 341–351. [Google Scholar] [CrossRef] [Green Version]
- Dekker, C.; Arts, A.; De Wijn, H.; Van Duyneveldt, A.; Mydosh, J. Activated dynamics in a two-dimensional Ising spin glass: Rb 2 Cu1-xCox F4. Phys. Rev. B 1989, 40, 11243. [Google Scholar] [CrossRef] [Green Version]
- Evangelisti, M.; Bartolomé, J. Secondary magnetic relaxations in Mn12 complexes. J. Magn. Magn. Mater. 2000, 221, 99–102. [Google Scholar] [CrossRef]
- Chakov, N.E.; Soler, M.; Wernsdorfer, W.; Abboud, K.A.; Christou, G. Single-molecule magnets: Structural characterization, magnetic properties, and 19F NMR spectroscopy of a Mn12 family spanning three oxidation levels. Inorg. Chem. 2005, 44, 5304–5321. [Google Scholar] [CrossRef] [PubMed]
- Boskovic, C.; Brechin, E.K.; Streib, W.E.; Folting, K.; Bollinger, J.C.; Hendrickson, D.N.; Christou, G. Single-molecule magnets: A new family of Mn12 clusters of formula [Mn12O8X4 (O2CPh)8L6]. J. Am. Chem. Soc. 2002, 124, 3725–3736. [Google Scholar] [CrossRef] [PubMed]
- Bałanda, M.; Pełka, R.; Fitta, M.; Laskowski, Ł.; Laskowska, M. Relaxation and magnetocaloric effect in the Mn 12 molecular nanomagnet incorporated into mesoporous silica: A comparative study. RSC Adv. 2016, 6, 49179–49186. [Google Scholar] [CrossRef]
- Bagai, R.; Christou, G. The drosophila of single-molecule magnetism:[Mn12O12(O2CR)16(H2O)4]. Chem. Soc. Rev. 2009, 38, 1011–1026. [Google Scholar] [CrossRef]
- Mydosh, J.A. Spin Glasses: An Experimental Introduction; Taylor and Francis: Abingdon, UK, 1993. [Google Scholar]
- Novak, M.; Folly, W.; Sinnecker, J.; Soriano, S. Relaxation in magnetic nanostructures. J. Magn. Magn. Mater. 2005, 294, 133–140. [Google Scholar] [CrossRef]
- Laskowska, M.; Bałanda, M.; Fitta, M.; Dulski, M.; Zubko, M.; Pawlik, P.; Laskowski, Ł. Magnetic behaviour of Mn12-stearate single-molecule magnets immobilized inside SBA-15 mesoporous silica matrix. J. Magn. Magn. Mater. 2019, 478, 20–27. [Google Scholar] [CrossRef]
- Willemin, S.; Arrachart, G.; Lecren, L.; Larionova, J.; Coradin, T.; Clérac, R.; Mallah, T.; Guérin, C.; Sanchez, C. Immobilisation of single molecule magnets in mesoporous silica hosts. New J. Chem. 2003, 27, 1533–1539. [Google Scholar] [CrossRef]
- Clemente-León, M.; Coronado, E.; Forment-Aliaga, A.; Amorós, P.; Ramírez-Castellanos, J.; González-Calbet, J.M. Incorporation of Mn12 single molecule magnets into mesoporous silica. J. Mater. Chem. 2003, 13, 3089–3095. [Google Scholar] [CrossRef]
- Aulakh, D.; Bilan, H.K.; Wriedt, M. Porous substrates as platforms for the nanostructuring of molecular magnets. CrystEngComm 2018, 20, 1011–1030. [Google Scholar] [CrossRef]
- Coradin, T.; Larionova, J.; Smith, A.A.; Rogez, G.; Clérac, R.; Guérin, C.; Blondin, G.; Winpenny, R.E.; Sanchez, C.; Mallah, T. Magnetic nanocomposites built by controlled incorporation of magnetic clusters into mesoporous silicates. Adv. Mater. 2002, 14, 896–898. [Google Scholar] [CrossRef]
- Friedman, J.R.; Sarachik, M.; Tejada, J.; Ziolo, R. Macroscopic measurement of resonant magnetization tunneling in high-spin molecules. Phys. Rev. Lett. 1996, 76, 3830. [Google Scholar] [CrossRef] [PubMed]



| T (K) | (10 emu g Oe) | (10 emu g Oe) | (s) | |
|---|---|---|---|---|
| 3.0 | 3.84 (9) | 11.65 (8) | 14.55672 (4) | 0.582 (4) |
| 3.5 | 3.78 (3) | 10.51 (6) | 1.36302 (1) | 0.457 (7) |
| 4 | 3.72 (1) | 8.84 (2) | 0.18202 (2) | 0.352 (3) |
| 4.5 | 3.84 (1) | 7.69 (1) | 0.05274 (4) | 0.192 (4) |
| 5 | 4.003 (7) | 6.94 (2) | 0.0226 (1) | 0.065 (5) |
| Type of Silica Substrate | Applied SMMs | (K) | (s) | Reference |
|---|---|---|---|---|
| Spherical nanoparticles | Mn-stearate | — | ||
| SBA-15 | Mn-stearate | 55 | [25] | |
| SBA-15 | Mn-acetate | 72 | [26] | |
| SBA-type | Mn-benzonate | 63 | [29] | |
| MCM-41 | Mn-acetate | [27] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pastukh, O.; Konieczny, P.; Laskowska, M.; Laskowski, Ł. AC Susceptibility Studies of Magnetic Relaxation in Mn12-Stearate SMMs on the Spherical Silica Surface. Magnetochemistry 2021, 7, 122. https://doi.org/10.3390/magnetochemistry7090122
Pastukh O, Konieczny P, Laskowska M, Laskowski Ł. AC Susceptibility Studies of Magnetic Relaxation in Mn12-Stearate SMMs on the Spherical Silica Surface. Magnetochemistry. 2021; 7(9):122. https://doi.org/10.3390/magnetochemistry7090122
Chicago/Turabian StylePastukh, Oleksandr, Piotr Konieczny, Magdalena Laskowska, and Łukasz Laskowski. 2021. "AC Susceptibility Studies of Magnetic Relaxation in Mn12-Stearate SMMs on the Spherical Silica Surface" Magnetochemistry 7, no. 9: 122. https://doi.org/10.3390/magnetochemistry7090122
APA StylePastukh, O., Konieczny, P., Laskowska, M., & Laskowski, Ł. (2021). AC Susceptibility Studies of Magnetic Relaxation in Mn12-Stearate SMMs on the Spherical Silica Surface. Magnetochemistry, 7(9), 122. https://doi.org/10.3390/magnetochemistry7090122








