1. Introduction
Supercapacitors exhibit middle characteristics between batteries and conventional capacitors in terms of power vs energy density, placing themselves in between the two previous solutions. Their specific energy is lower than batteries, but considerable power spikes can be obtained for shorter times (a few seconds), making them an ideal profile as load-leveling systems. In the category of pseudocapacitors (a variety of supercapacitors), also the charge–discharge mechanism is intermediate between the two considered main systems, although it is still not completely defined. In fact, faradaic processes are involved, but they consist of particular pseudo-Redox reactions: they are very fast, reversible, and do not involve any bulk-phase change in the electrodes material (usually implying adsorption, chemisorption, and intercalation side processes).
Possible choices for pseudocapacitor-active electrode materials are heteroatom-modified carbon [
1,
2,
3], conducting polymers [
4,
5] and both noble [
6,
7,
8] and transition-metal oxides (preferable over the noble ones for their lower price), with these latter being particularly appreciated for their rich redox behavior, and their chemical and thermal stability [
9]. Between the transition metal, manganese oxide (
) has been reported to exhibit high specific capacitance, allowing to realize systems which range from 130 to 700 F/g in mild aqueous electrolytes [
10]. In addition, Manganese has also low toxicity and it is an abundant element on Earth’s surface. The mechanism underlying the
charge–discharge process is not completely defined yet. Among the different proposed models, a common feature is the passage between the III and the IV oxidation states of Mn, resulting in electron transfer at Mn sites. Different kinds of interfacial reactions are regarded as balancing this charge transfer. Toupin M. summarized the two main proposed solutions [
9]: the first assesses the intercalation of
protons or alkali metal Cations
(such as
) in the bulk of the material.
The second mechanism involves cations of the electrolyte solution adsorbed on the surface of
(for example,
is a plausible option in case of a
electrolyte):
These theorized solutions are consistent with the experimental dependency of the capacitance performance on the microstructure and on the thickness of the
MnO layer. In case of
thin films, specific capacitances up to 1380 F/g have been reported, in contrast with top values of only 200 F/g for bulk
and only 10 F/g for
[
11].
In the first place, this trend is explained by the larger tunnel sizes in the crystal structure of with respect to . This allows to enhance ion diffusion and provides additional adsorption sites to accept cations.
Results obtained by Pang S.C. [
10] lead to the same direction, highlighting a greater capacitive performance of
MnO thin-films electrodes after prolonged voltammetric cycling. In fact, the system tends to an ideal behavior (a rectangular shape with a quick and almost-vertical achievement of the saturation current in charge and discharge runs) as the forward and backward scans are cycled, i.e., an elevated number of cycles is performed. This is due to the transformation of the microstructure, from densely packed (at the beginning), to irregular and rough particles (50 cycles), and finally a petal-like structure (100 cycles), resulting in a macroporous morphology.
With the microstructure being a focal point of interest, in the context of electrochemical methods, an established way to affect the plating microstructure is the application of an external magnetic field. As reported by M. A. Monzon [
12] and V. Gatard [
13] the Lorentz Force arising from a static applied magnetic field can provide additional convection by affecting charged species and so increase mass transport. Therefore, the investigation on the capacitance difference between samples deposited with and without the presence of a magnet can be used to indirectly reveal the effects of the magnetic field on the
microstructure.
In this study, we report a series of experimental results highlighting the influence of the magnetic field on electrodeposition of pseudo-capacitors active material. The presence of the magnet, placed outside the electrochemical cell, but in direct contact with the lower (non-plated) side of the Si-substrate, introduces a degree of turbulence acting on the current of charged species in solution. This affects the local hydrodynamic conditions and consequently modifies nucleation and growth of the electrodeposited active material, leading to a more dense and low-defected coating.
3. Discussion
We investigated the influence of the magnet on the deposition and on the capacitive performance of an
electrode for pseudocapacitors. Potentiostatic and galvanostatic deposition curves (
Figure 1a,b) have been presented in
Section 2.1 and show a significant difference in the first 50 seconds of the procedure. The presence of the magnetic field enhances the electrochemical efficiency, leading to higher currents for equal potentials or lower potentials with the same current. The reason behind this experimental effect lies in the convection provided by the Lorentz force in the electrochemical cell, which is addressed as the magnetohydrodynamic (MHD) effect in the literature [
12]. Charged species in solution interact with the magnetic field and are so affected by an additional local perturbation, which results in a magnetic stirring action.
Equation (
1) describes the Lorentz force acting on moving charged particles (or equivalently on a current), where
is the magnetic field,
q is the charge of the species affected by
,
is the velocity, and so
is the current density. Clearly, the Lorentz force is largest when
and
are perpendicular, and null when they are parallel. Even though the axis of the magnetic field is parallel to the assumed flow of ions in our experimental set-up, local interactions are still to be accounted for because of the bending of the field’s force lines.
This kind of local interaction manifests most where
is greater, for example at the edges of the electrode and around the surface morphological asperities or growing nuclei, and leads to a vortice-like motion of the charged species with different benefits with respect to a macroscopic large-scale convection made by mechanical agitation [
12]. In fact, the most general trajectory for a charged particle in a uniform magnetic field is helical, with the axis of the helix parallel to the direction of the magnetic field [
16]. This statement is the result of the general resolution of the differential Equation (
2) (2nd equation of motion):
with the condition of the field
directed along the cartesian z-axis, as in our case (versor
). The resolution leads to trajectory parameters of Equation (
3):
is a constant (since , then the component of velocity parallel to the magnetic field is constant), R is an arbitrary constant, and is called cyclotron frequency (it’s independent of the velocity of the charged particle). Because of this helical motion, MHD causes a mass transport increase through the electrolyte by reducing the diffusion layer.
Secondly, these magnetic effects ensure a lower impact of
bubbles at the cathode surface, which are the product of hydrogen reduction parasite reaction and tend to occlude the electrode surface, deforming local current density [
12]. MHD opposes this unfavorable condition, both leading to more uniform current densities at the electrode’s interface (which traduces to smaller
bubbles) and physically sweeping those bubbles away, clearing the electroactive surface. In general, this is beneficial during electrodeposition, as the
film grows tidier and less porous. The overall efficiency increase (obtained by the presence of the magnet) is mostly evident in the early stages of the process, when the diffusion effects are still small (
Figure 1a,b). In fact, after about 50 s, the Nernst diffusion layer extends and the nature of the substrate surface changes radically, switching from flat and very conductive metallic Au to more disordered
. These latter effects increase their influence more and more as the process continues, hiding the benefits of the MHD.
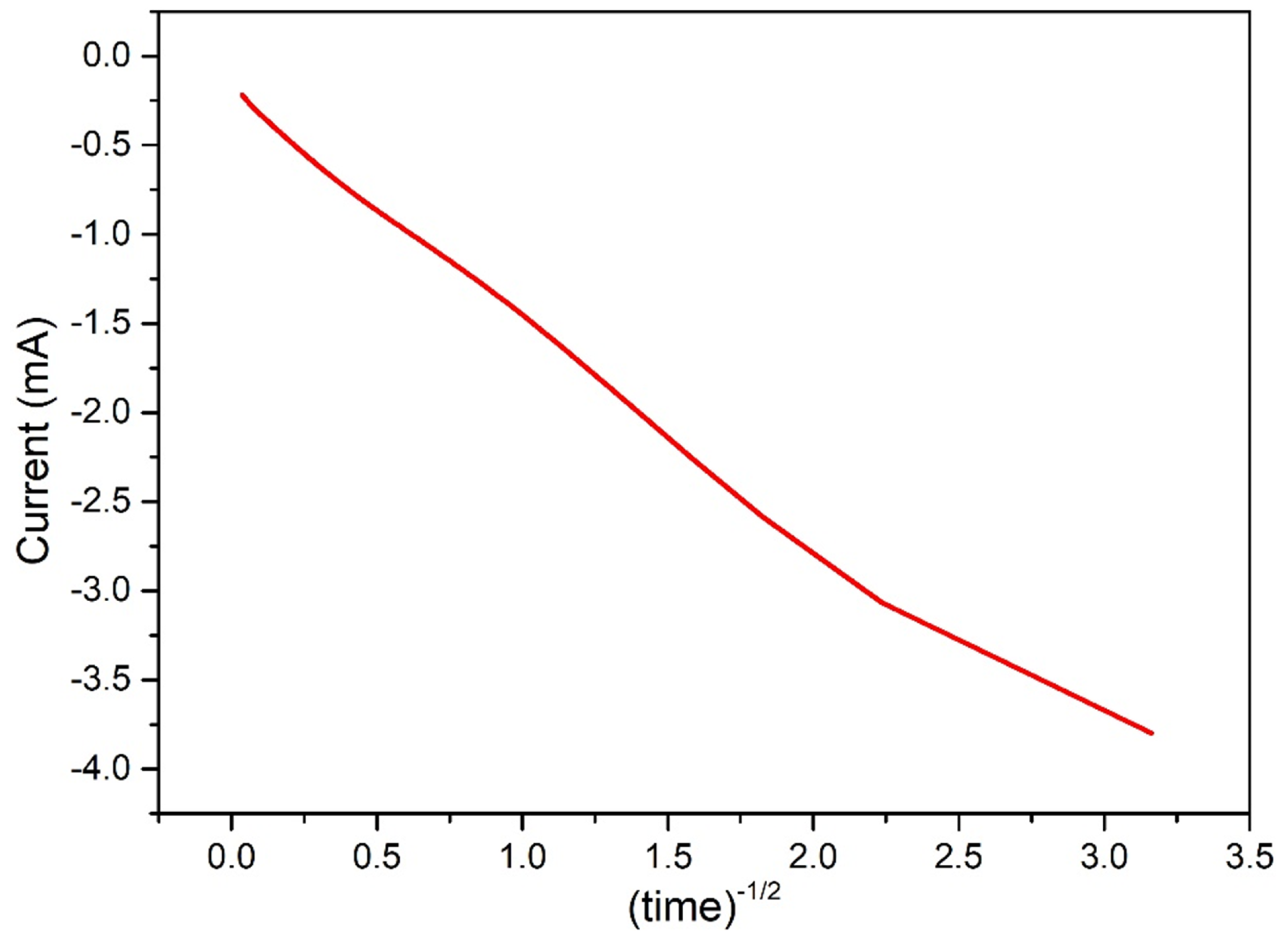
Indeed, the
deposition process can be reliably fitted by the Cottrell diffusion model, described by Equation (
4):
In order to prove this, the cathodic current obtained in the potentiostatic regime (
Figure 1a) can be plotted vs
, and a linear trend is obtained (
Figure 13). The
i-t behavior under Cottrell conditions is a marker of diffusive control over electrolysis [
17].
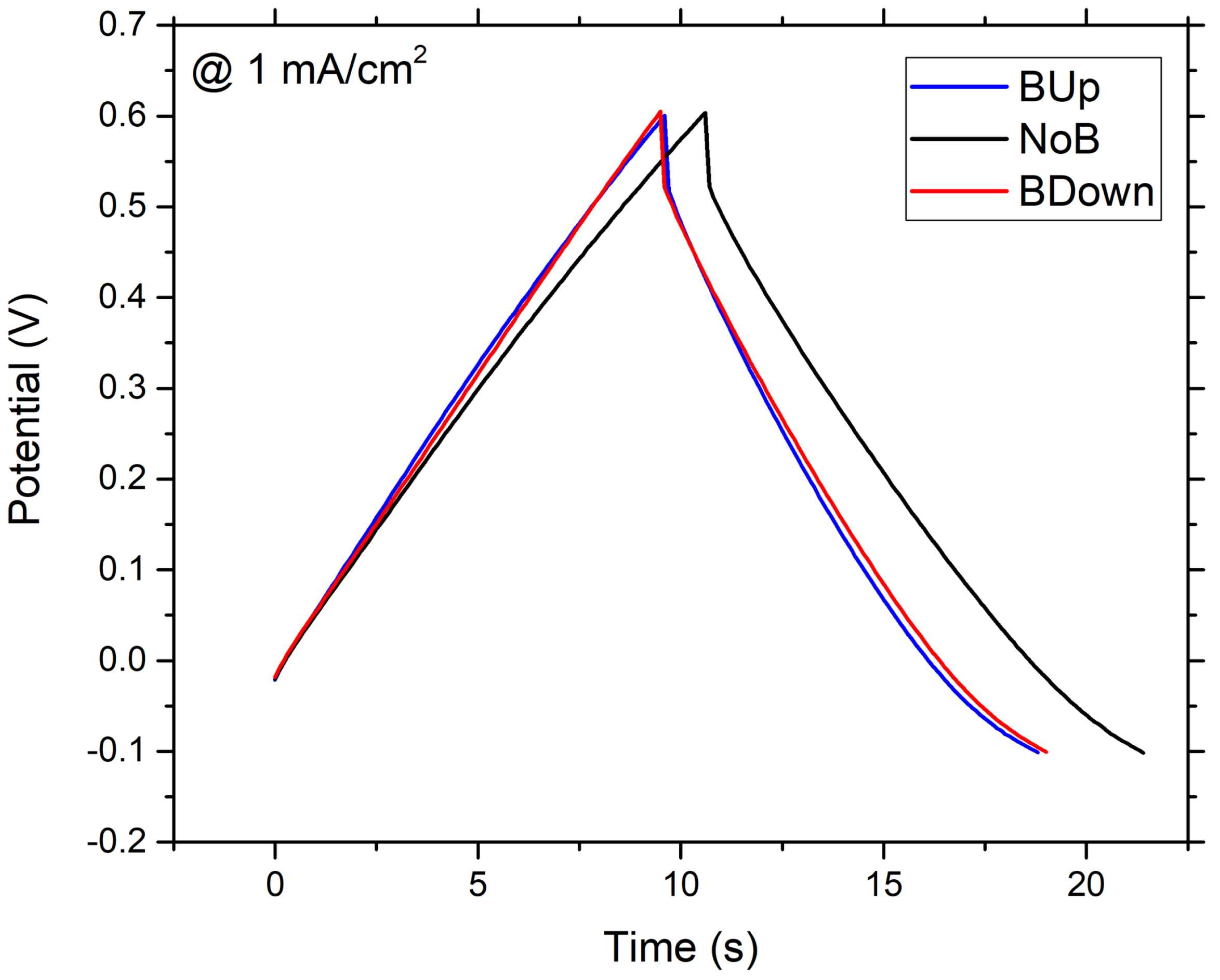
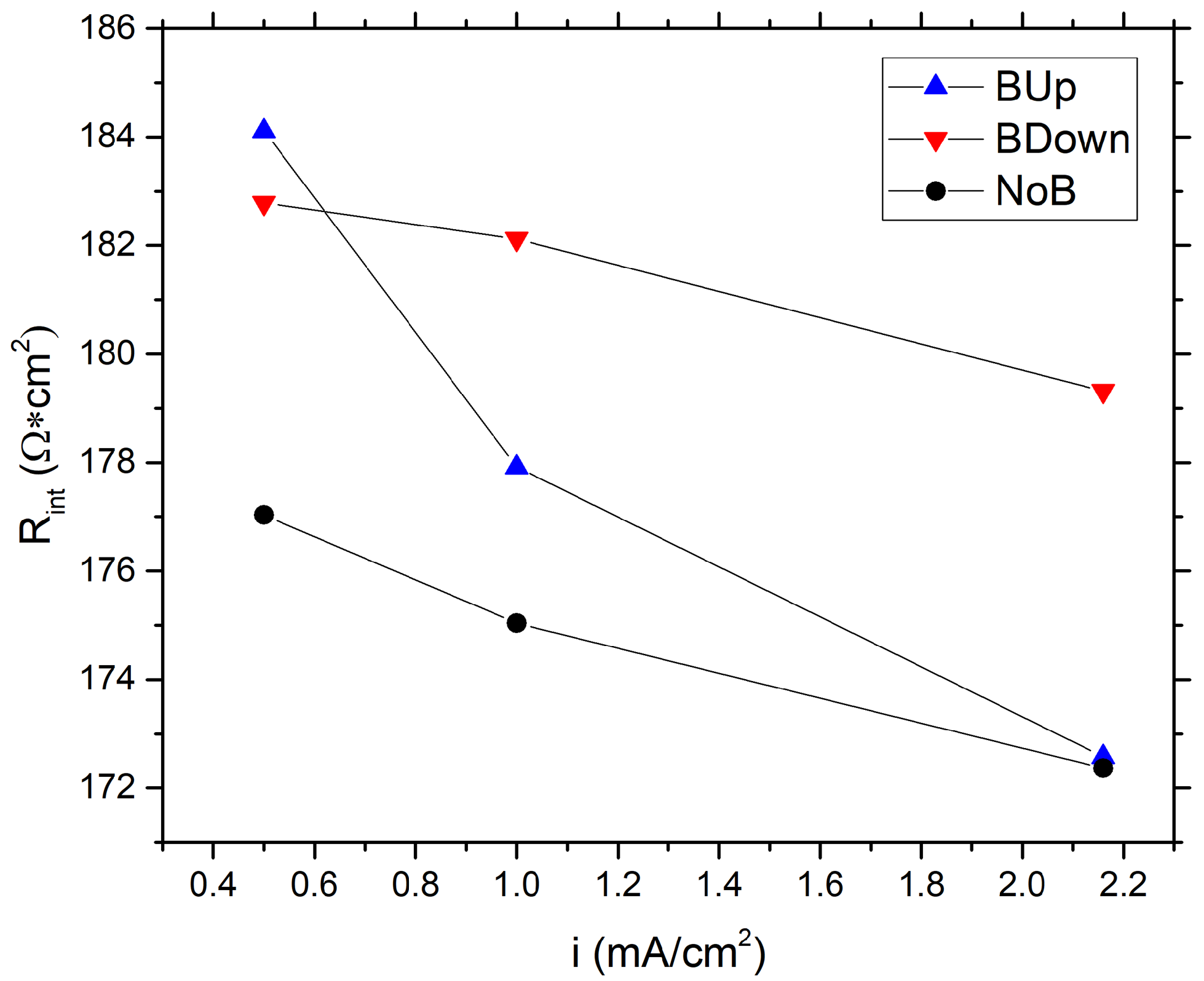
The obtained characterization results are consistent with the model proposed for the explanation of the manganese dioxide deposition. GCD analysis shows a net fade in specific capacitance for the samples BDown and BUp, which are the ones exposed to the magnetic field in the North and South direction, respectively. This decrease in capacitance is due to the microstructure that becomes more compact and uniform in presence of the magnet. Furthermore, the internal resistance of the devices follow the expected trend, with higher values for BDown and BUp and the lowest internal resistance of the NoB sample; besides, a less roughness and lower average thickness could be correlated to this result for NoB.
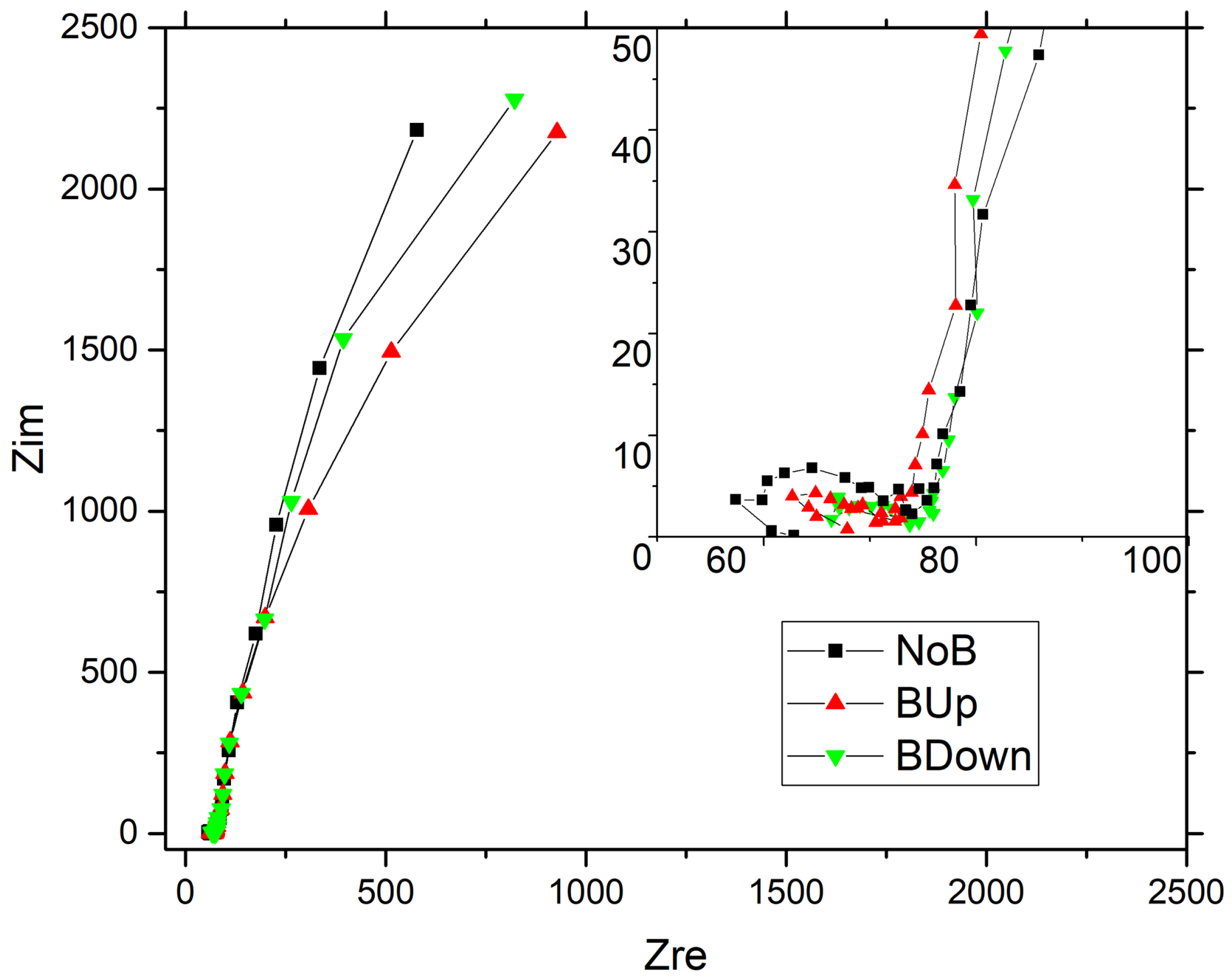
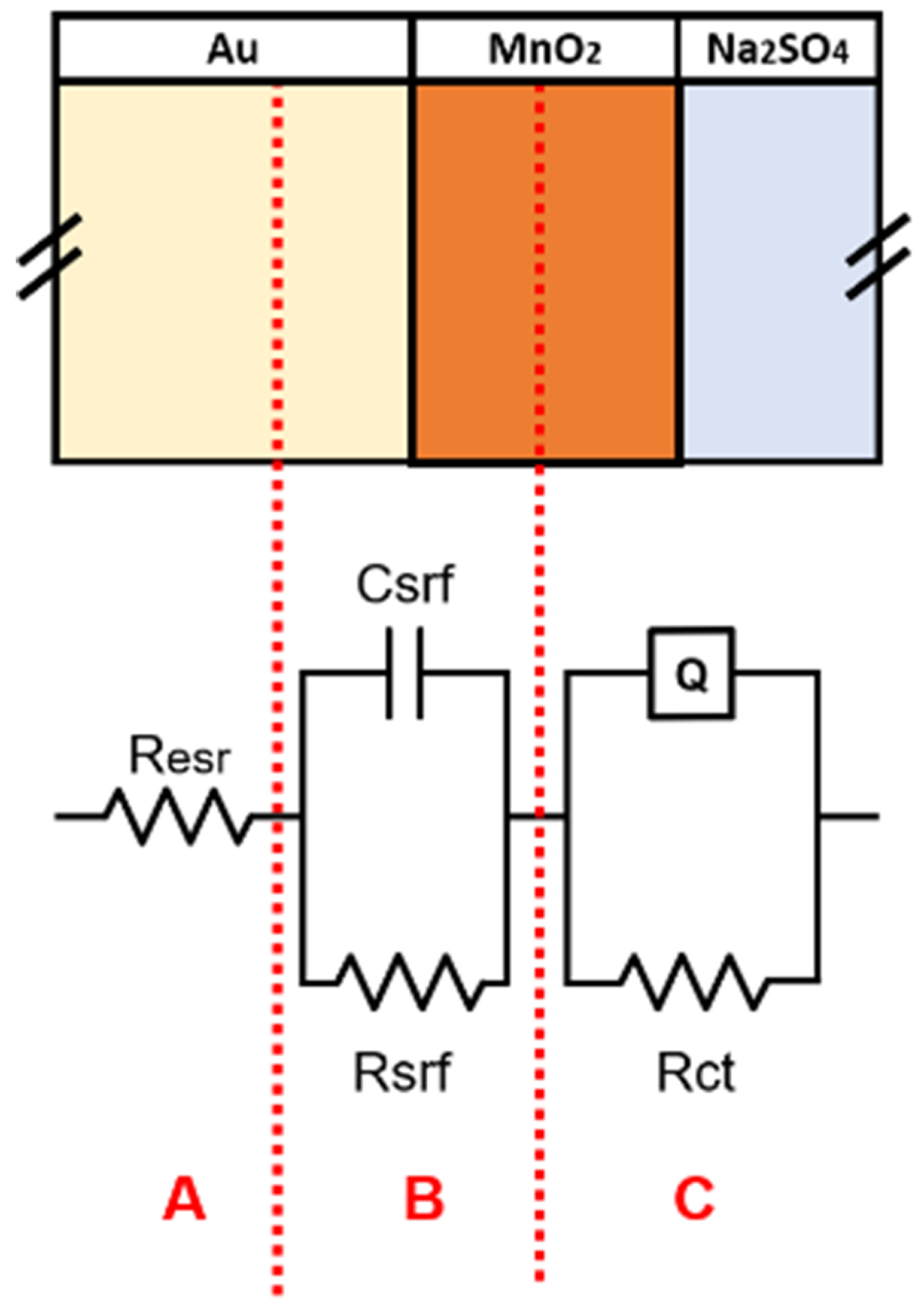
Electrochemical Impedance Spectroscopy characterization interestingly shows small differences in two separated regions; at a high frequency region, a depressed semicircle appears, associated with the interface between the active material and the substrate. NoB shows the highest
(
Table 1), while BDown and BUp show lower values closer to each other, thus indicating a possible difference in packing density (higher for samples constructed with the magnet) but also in nucleation and growing rates. The latter, in fact, are influenced by the presence of the magnetic field during the first stages of deposition and growth (first seconds of electrodeposition), and in this study, it is demonstrated a large influence of the magnet on the deposition curves. At low frequency the pseudo-capacitive response dominates the Nyquist plot, again no substantial difference exists between BDown and BUp, which present similar pseudo-capacitance and charge-transfer resistance, the latter indicating similar microstructures. The highest pseudocapacitance and charge transfer resistance is recorded for NoB(∼9 mF).
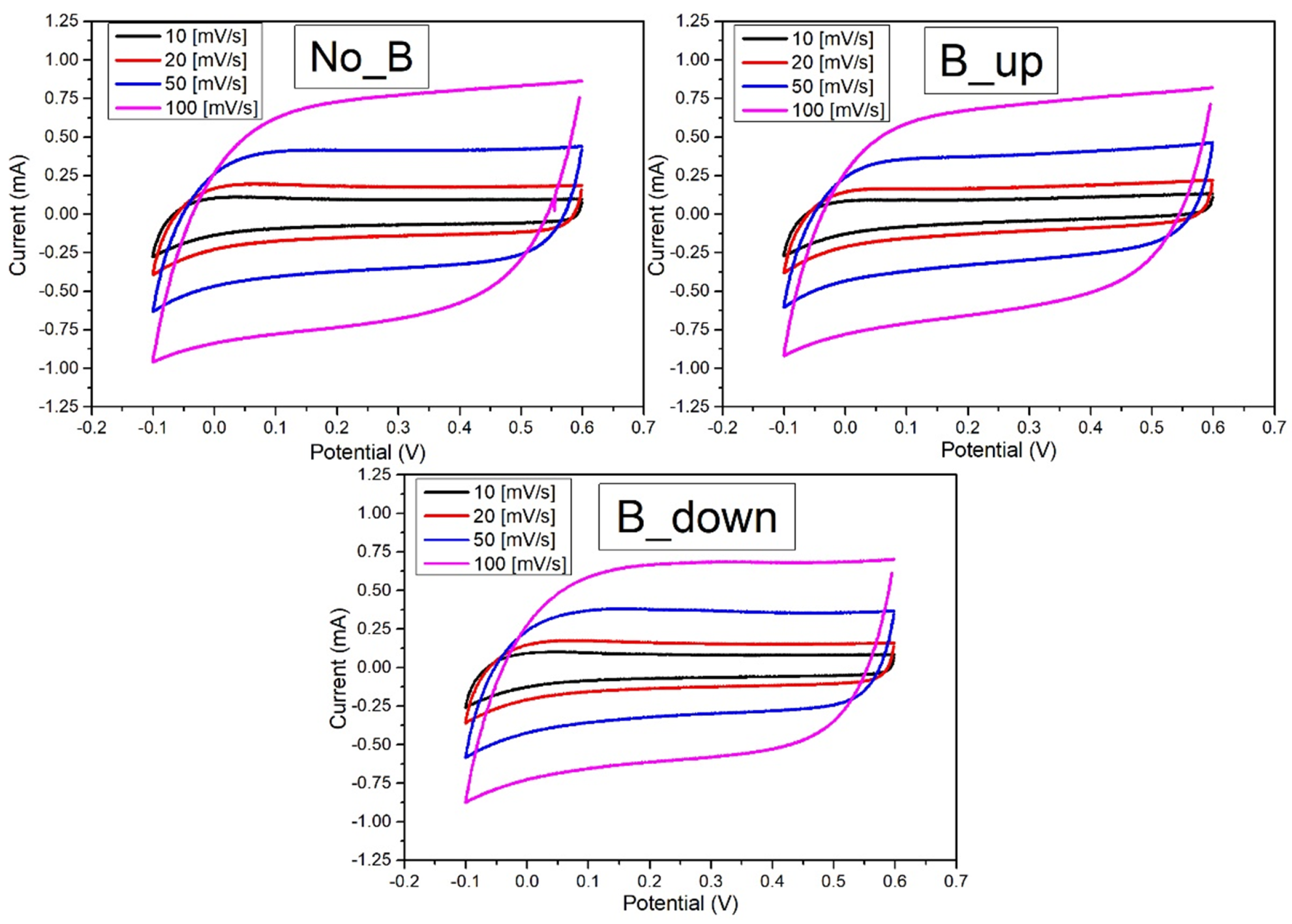
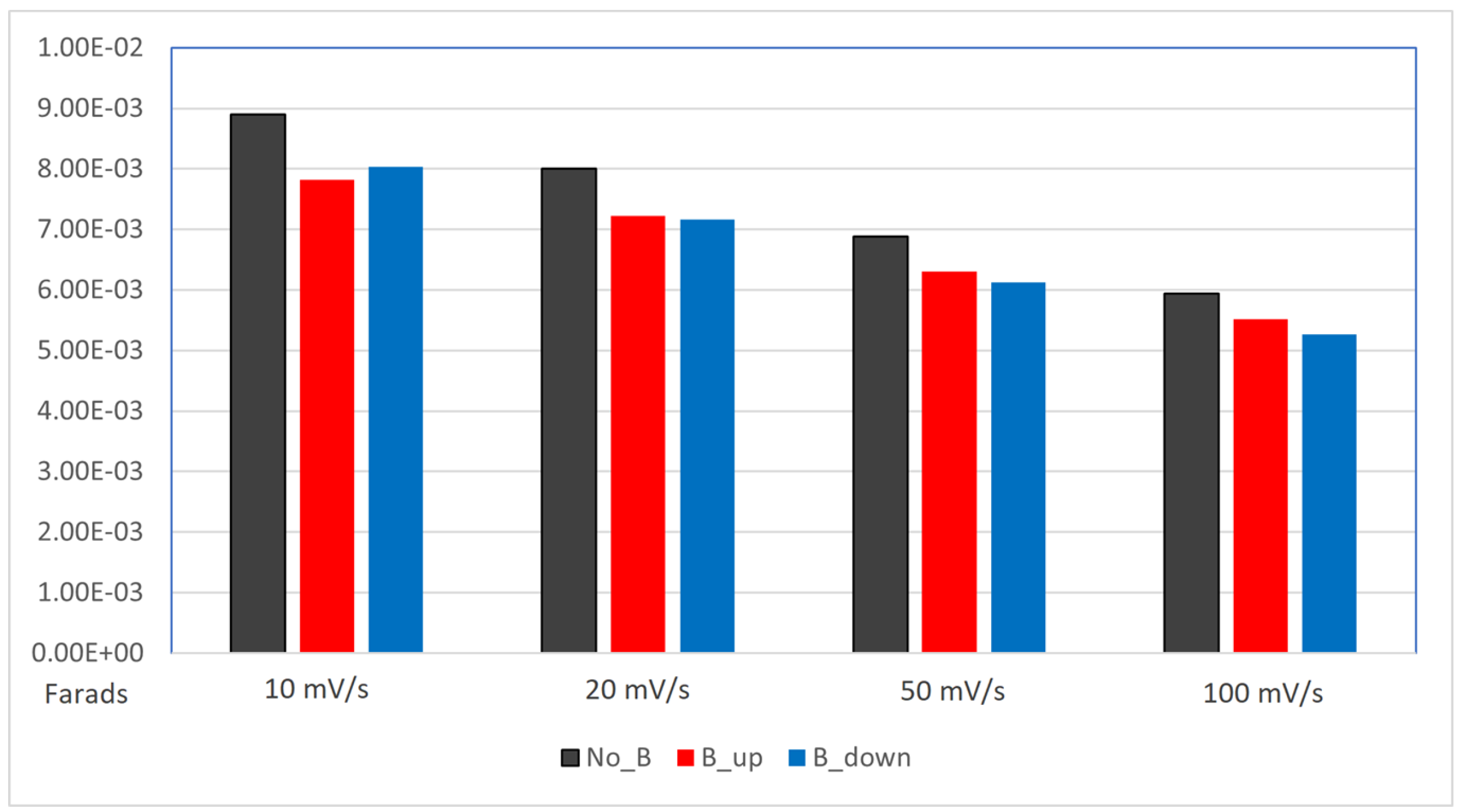
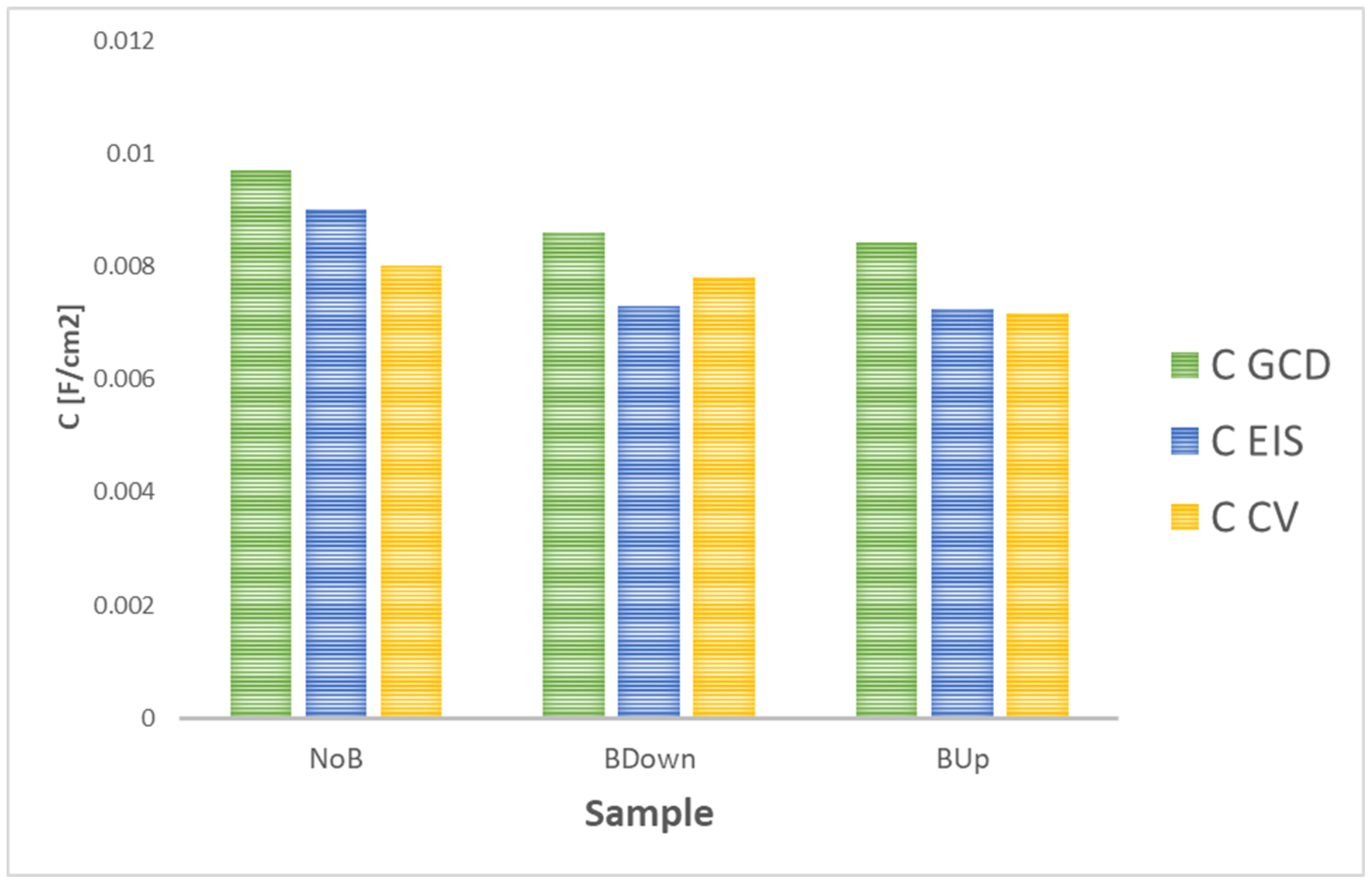
Finally, data obtained from other characterization techniques confirm the model proposed for the electrodeposition and the growth of the electrode material. CV and EIS analyses capacitance further confirm the results of GCD with the same trend, as shown in
Figure 14, where capacitance obtained at 0.5 mA/cm
current density (for GCD) and 20 mV/s scan rate (for CV) are chosen for best comparison.
The comparison of the results with values obtained with GCD tests shows a matching general trend of the capacitance values. Results are not expected to match perfectly, as they are obtained with different kind of characterization techniques; while during EIS, there is no polarization of the sample and the voltage tends to be changed sinusoidally in a pseudo-equilibrium state, in galvanostatic charge–discharge, the charge accumulation and charge transfer phenomena are forced by the imposition of a constant current. Finally, in the CV test, the potential is swept between two extreme values at different scan rates. In addition, in this work, GCD are performed after a series of preliminary characterization tests, as CV, so an increase in capacitance is expected as typical behavior of the manganese dioxide [
10].
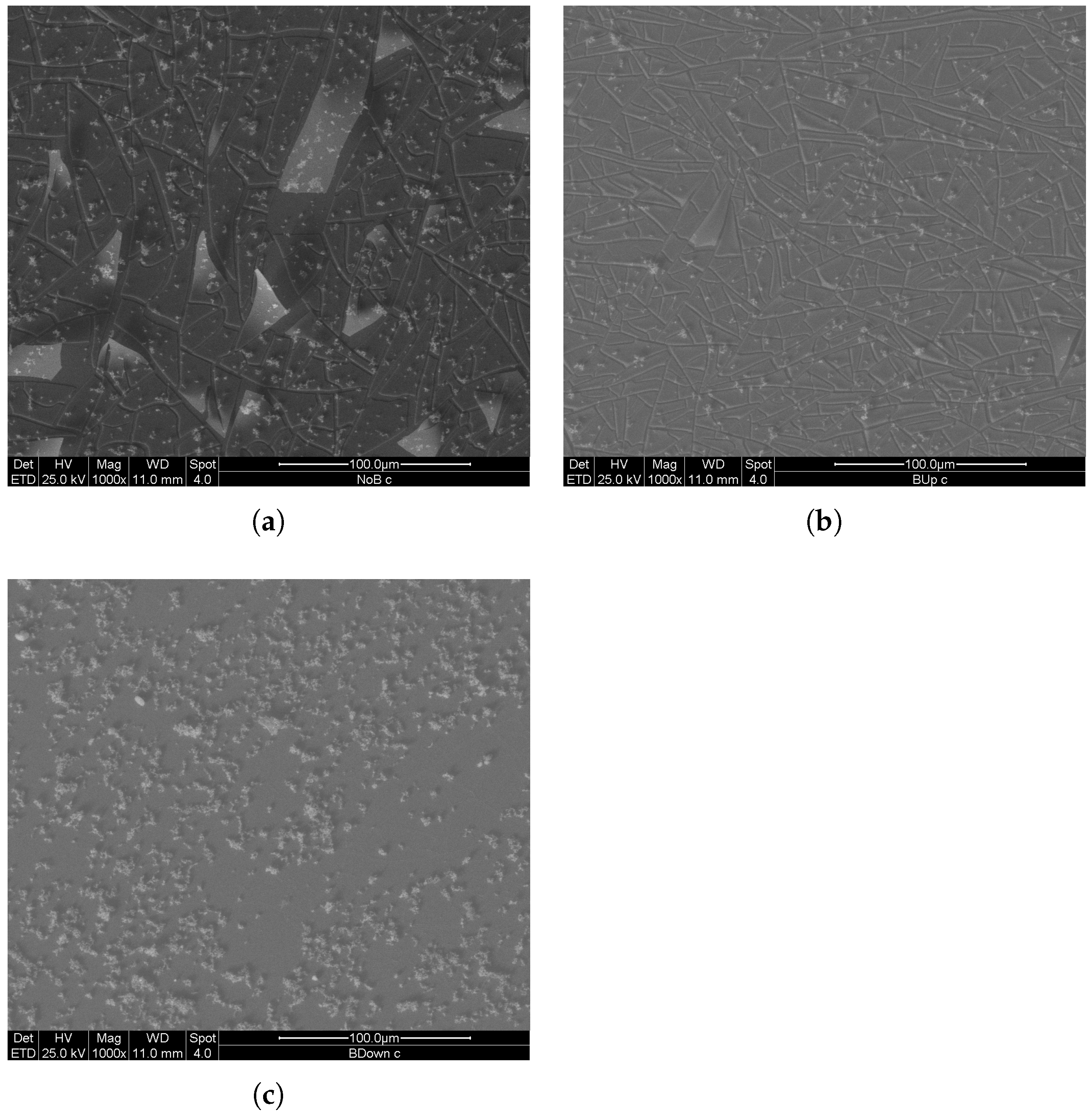
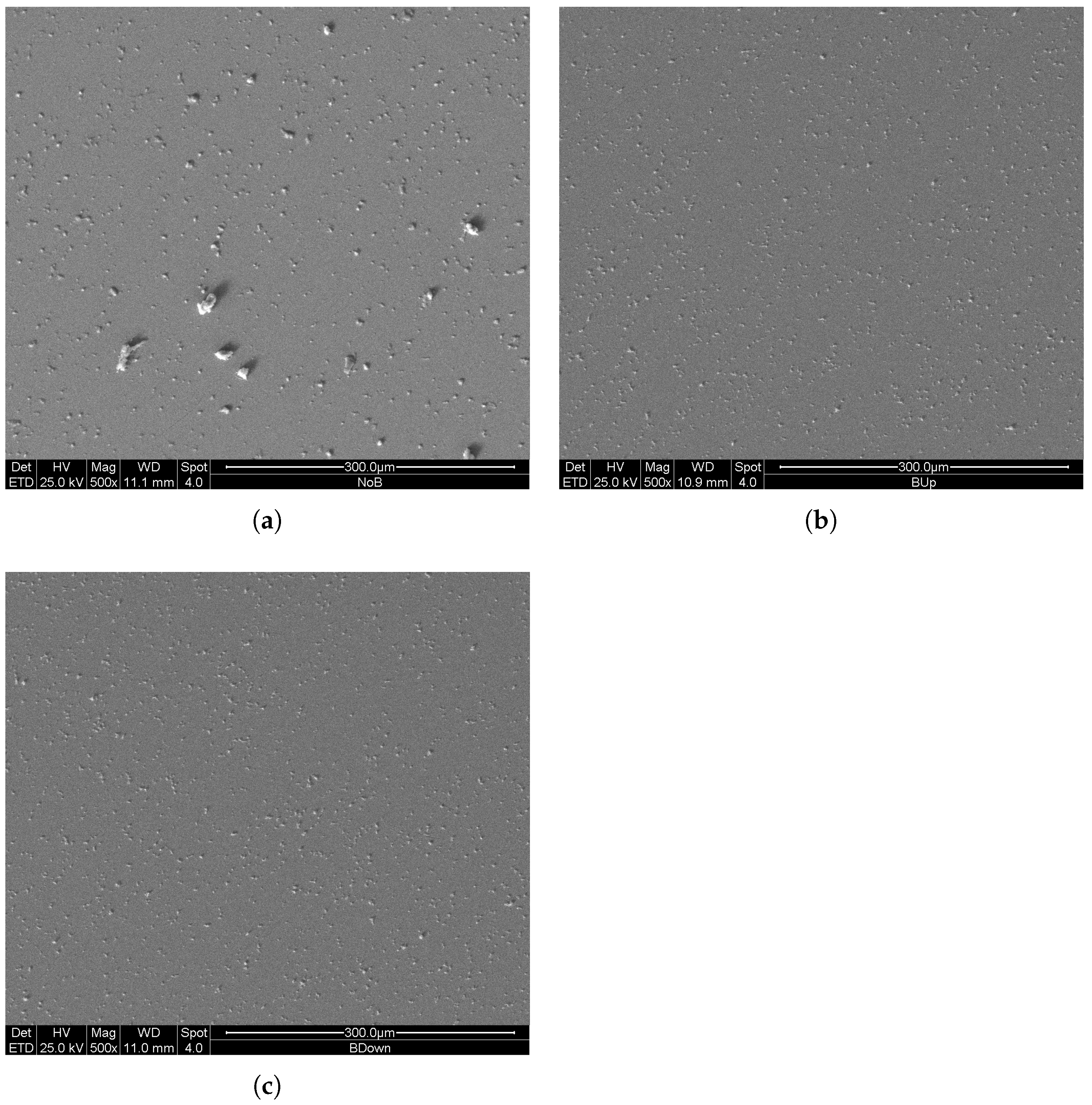
Finally, micrographs and optical image analysis confirm the hypothesis that the presence of the magnetic field introduces a convection and different hydrogen reduction kinetic and bubbling, which directly influences the nucleation and growth of the electrodeposited material. The number of nuclei counted in BDown and BUp is higher and bigger in dimensions, with a more uniform distribution over the whole surface. This is the effect expected for the setup used for the electrodeposition: while the magnetic field decreases the diffusion layer thickness, thanks to a local convection effect [
18], the limiting current of the reduction reaction is increased, the nucleation rate consequently increases. Also due to the forced convection,
bubbles tend to detach from the surface before coalescing with other bubbles that are forming nearby, favoring nucleation on new free sites and lowering the actual current density, thus leading the pre-existing deposit to grow in size and to create clusters that occupy a greater surface area. SEM analysis, performed on two new sets of samples, further confirms our previous hypothesis, showing an increase of uniformity and compactness of BUp and BDown samples and adding the possibility to analyze the first instants of electrodeposition, further underlining the difference between electrodes obtained with and without a magnetic field exposition, showing that nucleation sites increase greatly for BUp and BDown.
5. Conclusions
In this work, we have described the preparation and characterization of
on
Au/Si electrodes, in the view of their possible use in supercapacitor devices. The
plating and capacitive performance has been studied and compared between three different deposition conditions: in the absence of the magnetic field and in the presence of a upwards or downwards axial magnetic field, the latter is applied by placing a permanent magnet underneath the working electrode surface. GCD, EIS, and CV electrochemical techniques are used to characterize the physical and chemical properties of our samples. On the one hand, the experimental results show that the obtained
electrodes show capacitance values which are consistent with results already reported in the literature for pseudocapacitive systems [
10].
The highest capacitive performance is recorded for the sample obtained without the presence of the magnetic field during the electrodeposition process. Other samples, obtained with the presence of a magnetic field during the electrodeposition process, showed lower capacitance values.
This result can be rationalized in combination with the study of the deposition curves, the optical microscope and scanning electron microscope outcome, all of these results strongly suggest the beneficial influence of the magnetic field on the electrochemical efficiency of the deposition. In fact, the MHD effects lead to a more dense, packed, and ordered
microstructure, which is a less synergic environment for pseudocapacitive charge–discharge mechanisms. The use of the
on Au/Si electrodes here obtained, in combination with surface functionalization using organic polymers and a spin-dependent electrochemistry effect, paves the way to new developments in the field of magneto-electrochemistry systems [
19,
20,
21].