Polarized Neutron Diffraction: An Excellent Tool to Evidence the Magnetic Anisotropy—Structural Relationships in Molecules
Abstract
:1. Introduction
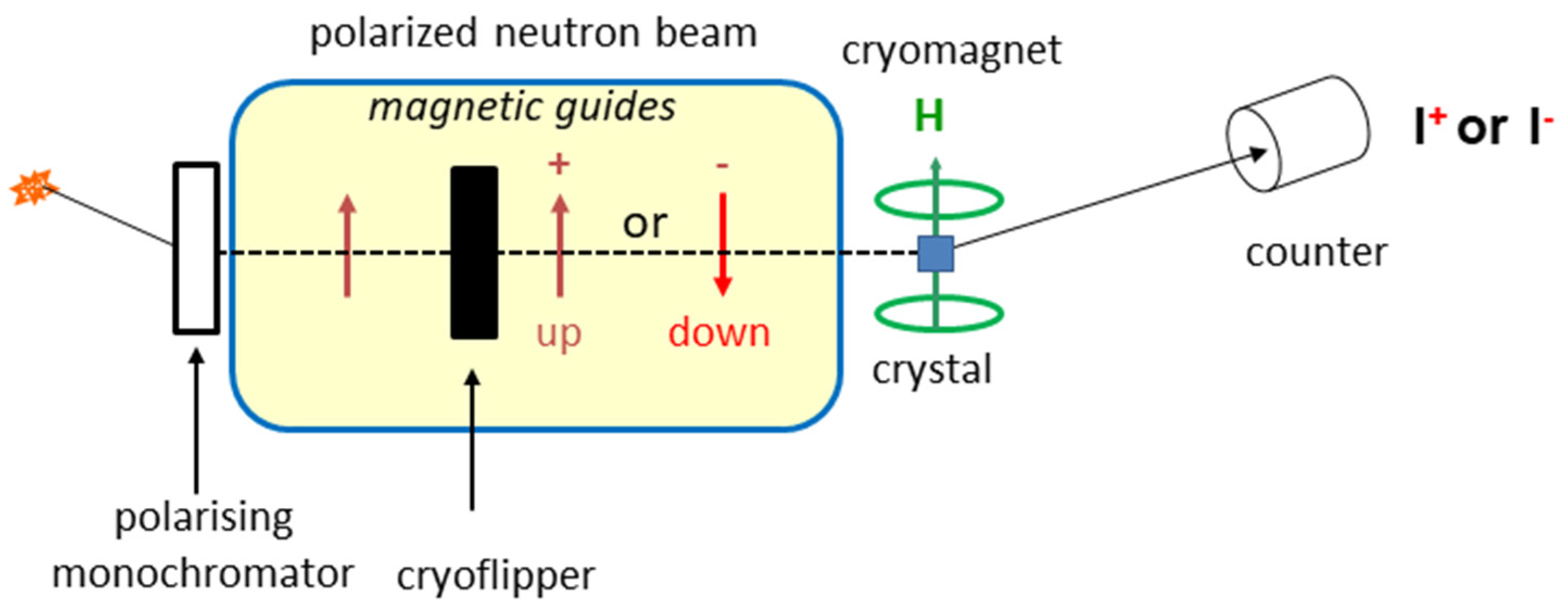
2. Basics of Polarized Neutron Diffraction
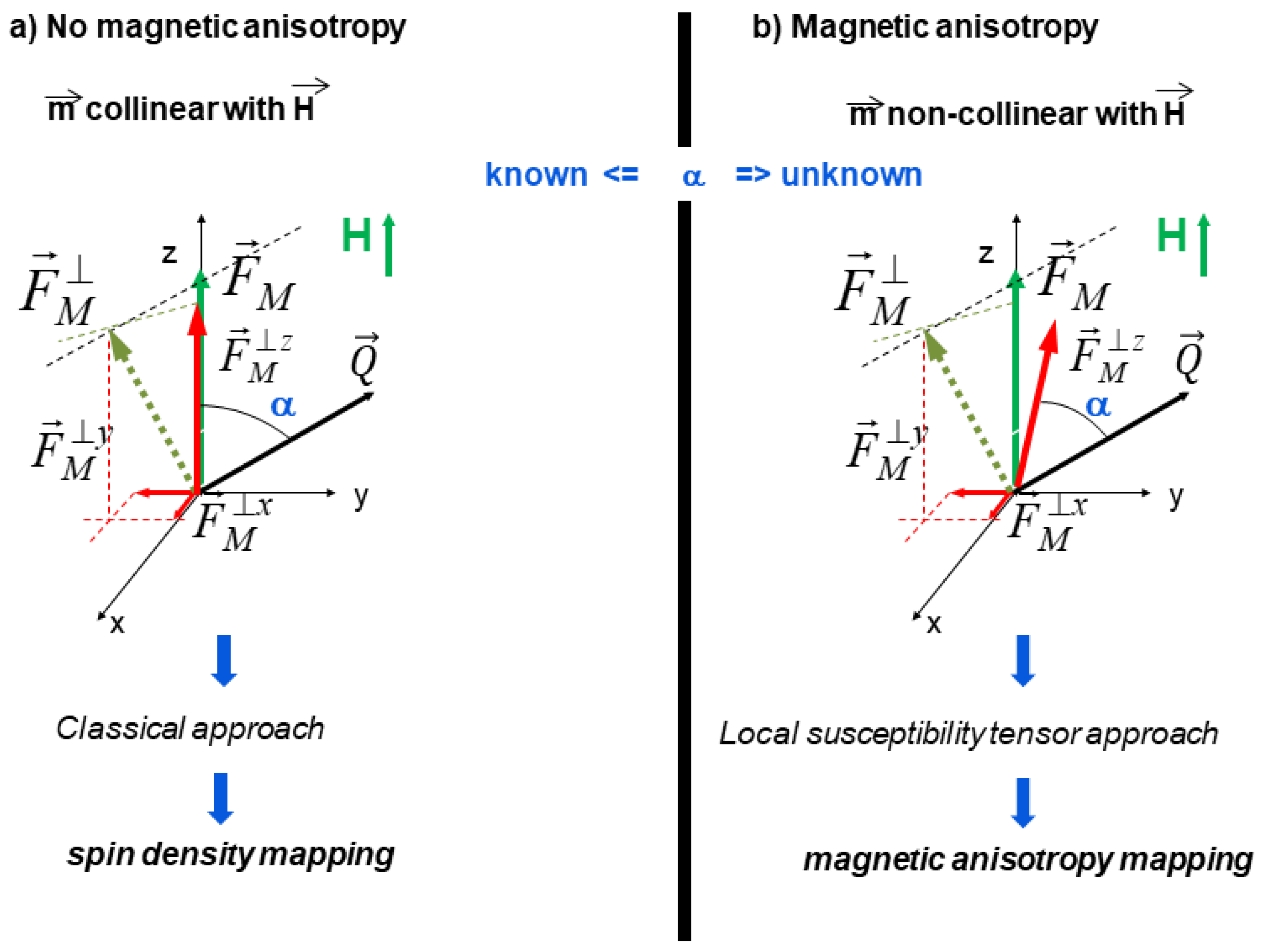
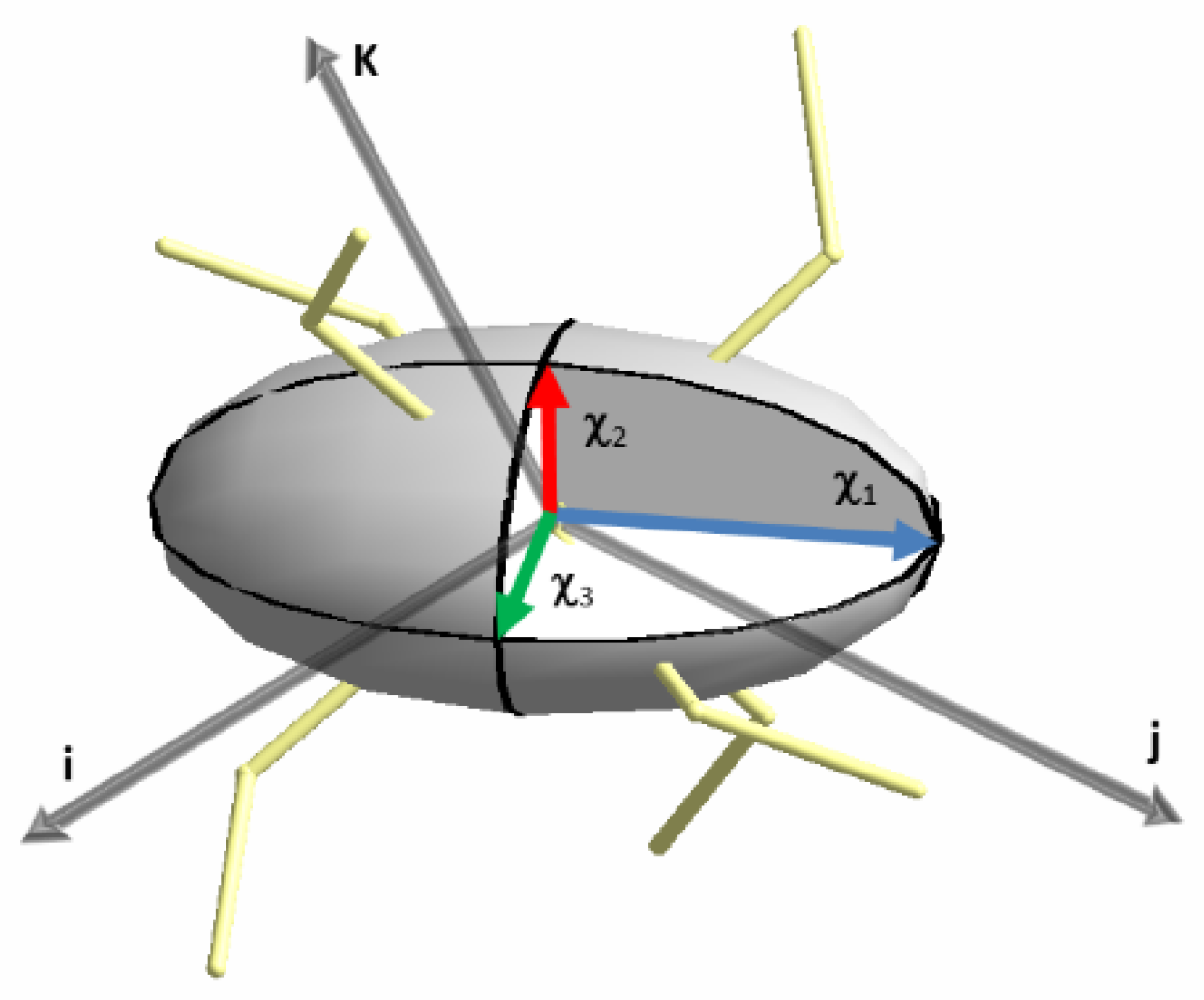
3. PND Mapping of the Magnetic Anisotropy in Coordination Compounds
3.1. Mononuclear Coordination Compounds
3.1.1. Transition Metal Compounds
3.1.2. Lanthanide Metal Compounds
3.2. Polynuclear Coordination Compounds
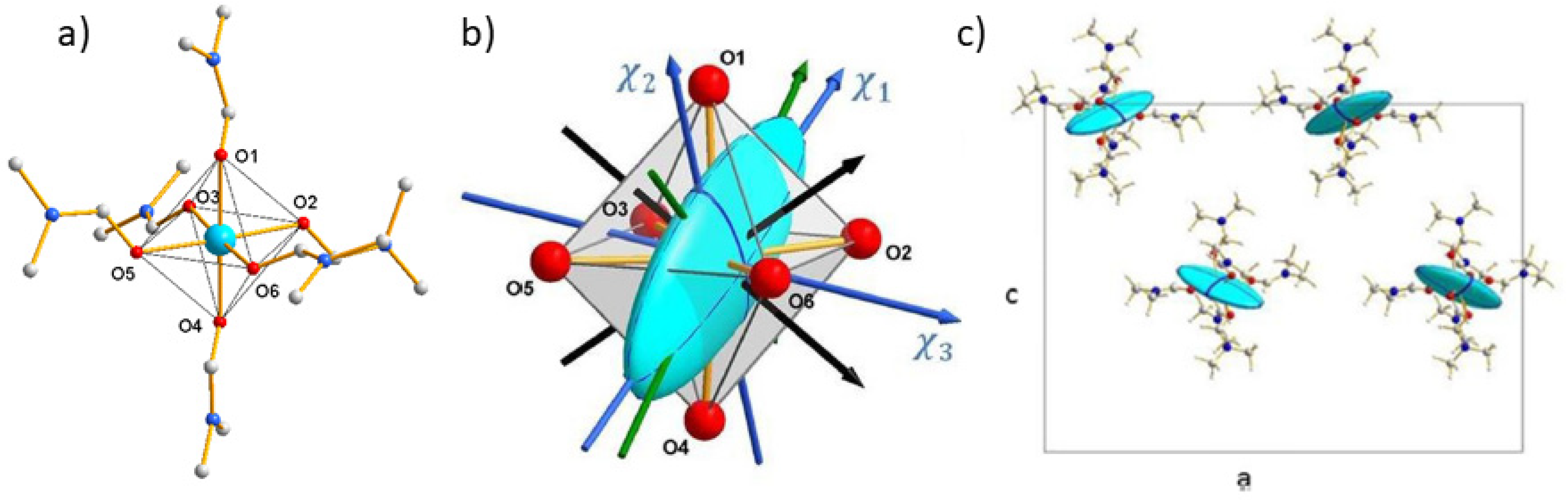
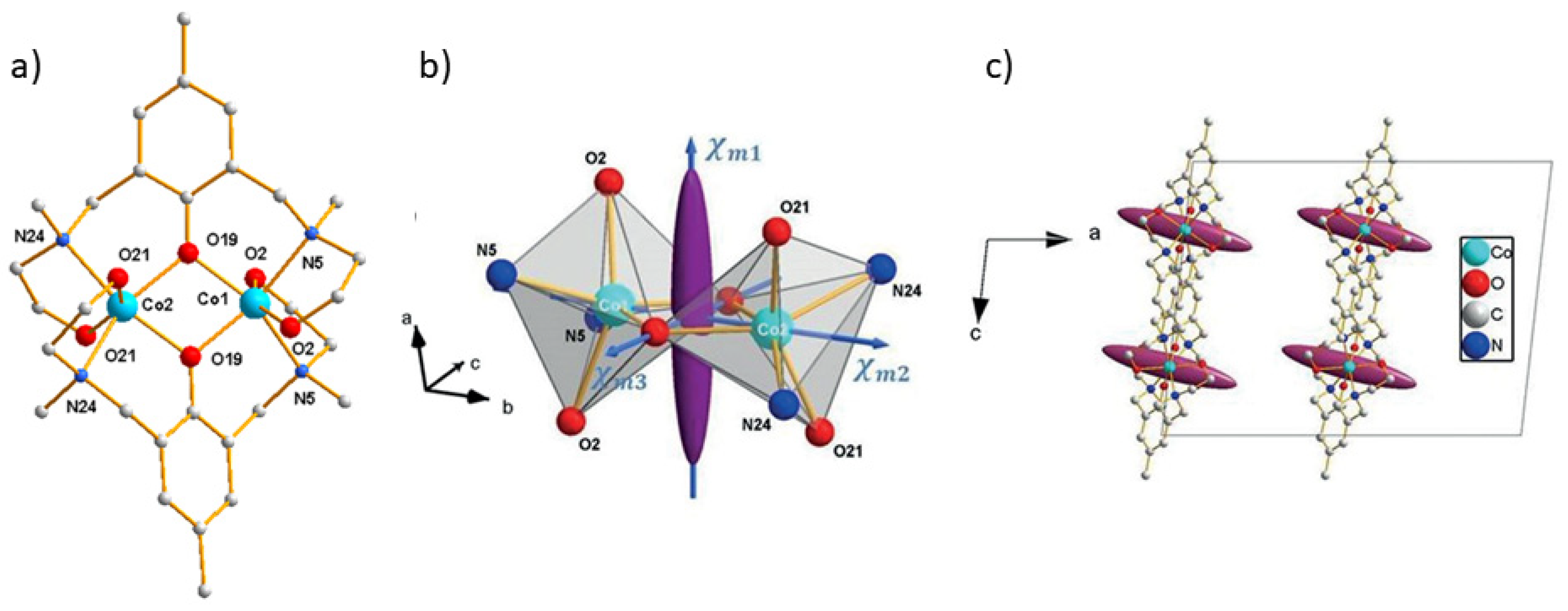
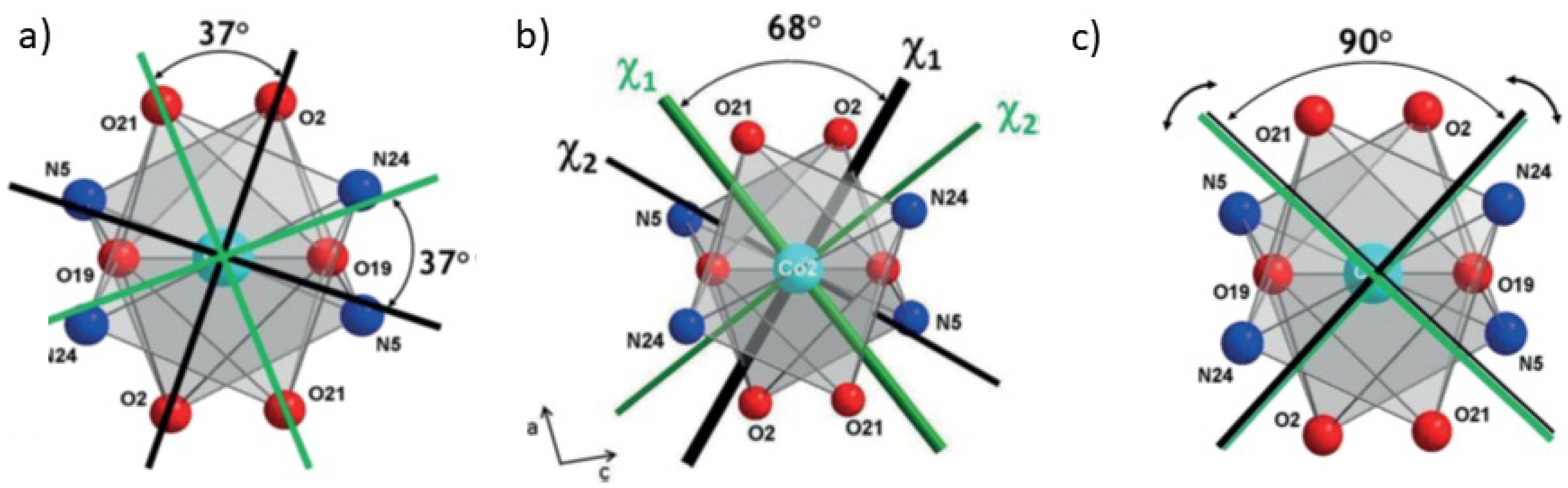
3.2.1. Dinuclear Cobalt(II) Complex [Co2(sym-hmp)2](BPh4)2
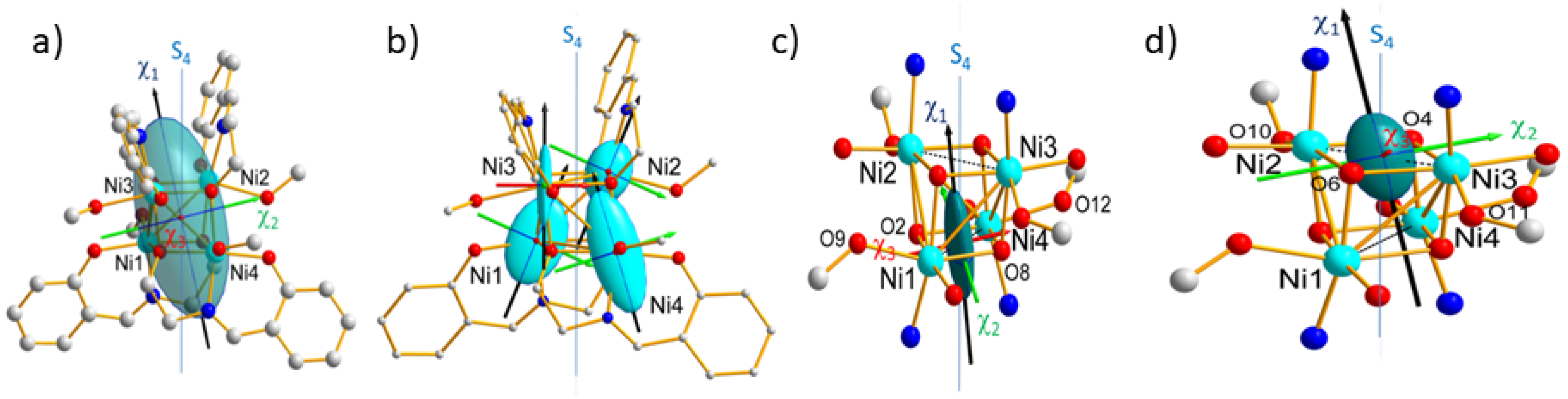
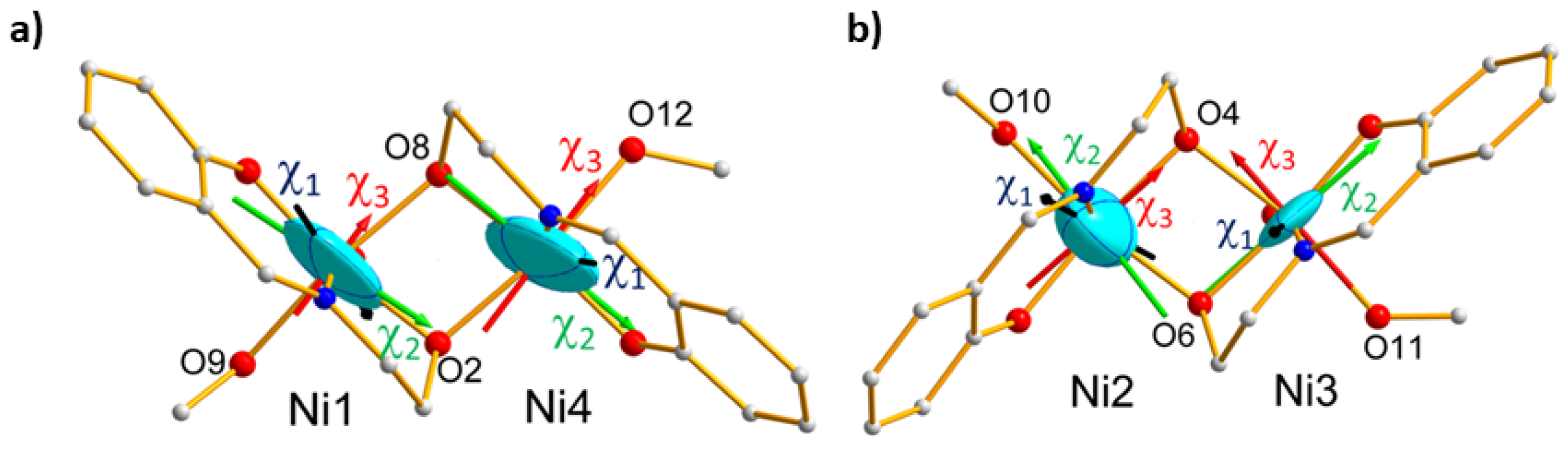
3.2.2. Tetranuclear Nickel(II) Complex [Ni4(L)4(MeOH)4]
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
References
- Squires, G.L. Introduction to the Theory of Thermal Neutron Scattering; Cambridge University Press: Cambridge, UK, 2012. [Google Scholar]
- Xue, Z.L.; Ramirez-Cuesta, A.J.; Brown, C.M.; Calder, S.; Cao, H.B.; Chakoumakos, B.C.; Daemen, L.L.; Huq, A.; Kolesnikov, A.I.; Mamontov, E.; et al. Neutron Instruments for Research in Coordination Chemistry. Eur. J. Inorg. Chem. 2019, 1065–1089. [Google Scholar] [CrossRef]
- Gatteschi, D. Physical Techniques for the Investigation of Molecular Magnetic Clusters. J. Phys. Chem. B 2000, 104, 9780–9787. [Google Scholar] [CrossRef]
- Ressouche, E.; Schweizer, J. Ab Initio Calculations versus Polarized Neutron Diffraction for the Spin Density of Free Radicals. Mon. Chem. 2003, 134, 235–253. [Google Scholar] [CrossRef]
- Basler, R.; Sieber, A.; Chaboussant, G.; Gudel, H.U.; Chakov, N.E.; Soler, M.; Christou, G.; Desmedt, A.; Lechner, R. Inelastic Neutron Scattering Study of Electron Reduction in Mn-12 Derivatives. Inorg. Chem. 2005, 44, 649–653. [Google Scholar] [CrossRef]
- Prsa, K.; Nehrkorn, J.; Corbey, J.F.; Evans, W.J.; Demir, S.; Long, J.R.; Guidi, T.; Waldmann, O. Perspectives on Neutron Scattering in Lanthanide-Based Single-Molecule Magnets and a Case Study of the Tb-2(Mu-N-2) System. Magnetochemistry 2016, 2, 45. [Google Scholar] [CrossRef] [Green Version]
- Etcheverry-Berrios, A.; Parsons, S.; Kamenev, K.V.; Probert, M.R.; Moggach, S.A.; Murrie, M.; Brechin, E.K. Putting the Squeeze on Molecule-Based Magnets: Exploiting Pressure to Develop Magneto-Structural Correlations in Paramagnetic Coordination Compounds. Magnetochemistry 2020, 6, 32. [Google Scholar] [CrossRef]
- Sieber, A.; Boskovic, C.; Bircher, R.; Waldmann, O.; Ochsenbein, S.T.; Chaboussant, G.; Güdel, H.U.; Kirchner, N.; van Slageren, J.; Wernsdorfer, W.; et al. Synthesis and Spectroscopic Characterization of a New Family of Ni4 Spin Clusters. Inorg. Chem. 2005, 44, 4315–4325. [Google Scholar] [CrossRef]
- Ballou, R.; Ouladdiaf, B. Representation Analysis of Magnetic Structures. In Neutron Scattering from Magnetic Materials; Chatterji, T., Ed.; Elsevier Science: Amsterdam, The Netherlands, 2006; pp. 93–151. [Google Scholar]
- Schweizer, J. Spin densities in magnetic molecular compounds. Phys. B Condens. Matter 1997, 234–236, 772–779. [Google Scholar] [CrossRef]
- Schweizer, J. Polarized Neutrons and Polarization Analysis. Neutron Scattering from Magnetic Materials; Chatterji, T., Ed.; Elsevier Science: Amsterdam, The Netherlands, 2006; pp. 153–213. [Google Scholar]
- Gillon, B. The Classical Flipping Ratio Technique Applied to Non Classical Magnetic Materials: Molecule-Based and Photoswitchable Magnetic Compounds. J. Phys. 2007, 13, 1–30. [Google Scholar]
- Ressouche, E.; Boucherle, J.X.; Gillon, B.; Rey, P.; Schweizer, J. Spin Density Maps in Nitroxide-Copper(II) Complexes. A Polarized Neutron Diffraction Determination. J. Am. Chem. Soc. 1993, 115, 3610–3617. [Google Scholar] [CrossRef]
- Zheludev, A.; Grand, A.; Ressouche, E.; Schweizer, J.; Morin, B.G.; Epstein, A.J.; Dixon, D.A.; Miller, J.S. Experimental Determination of the Spin Density in the Tetracyanoethenide Free Radical, [TCNE].bul. -, by Single-Crystal Polarized Neutron Diffraction. A View of a .pi.* Orbital. J. Am. Chem. Soc. 1994, 116, 7243–7249. [Google Scholar] [CrossRef]
- Baron, V.; Gillon, B.; Plantevin, O.; Cousson, A.; Mathoniere, C.; Kahn, O.; Grand, A.; Ohrstrom, L.; Delley, B. Spin-Density Maps for an Oxamido-Bridged Mn(II) Cu(II) Binuclear Compound. Polarized Neutron Diffraction and Theoretical Studies. J. Am. Chem. Soc. 1996, 118, 11822–11830. [Google Scholar] [CrossRef]
- Gillon, B.; Mathoniere, C.; Ruiz, E.; Alvarez, S.; Cousson, A.; Rajendiran, T.M.; Kahn, O. Spin Densities in a Ferromagnetic Bimetallic Chain Compound: Polarized Neutron Diffraction and Dft Calculations. J. Am. Chem. Soc. 2002, 124, 14433–14441. [Google Scholar] [CrossRef] [PubMed]
- Goujon, A.; Gillon, B.; Gukasov, A.; Jeftic, J.; Nau, Q.; Codjovi, E.; Varret, F. Photoinduced Molecular Switching Studied by Polarized Neutron Diffraction. Phys. Rev. B 2003, 67, 220401(R). [Google Scholar] [CrossRef]
- Aronica, C.; Jeanneau, E.; el Moll, H.; Luneau, D.; Gillon, B.; Goujon, A.; Cousson, A.; Carvajal, M.A.; Robert, V. Ferromagnetic Interaction in an Asymmetric End-to-End Azido Double-Bridged Copper(II) Dinuclear Complex: A Combined Structure, Magnetic, Polarized Neutron Diffraction and Theoretical Study. Chem. Eur. J. 2007, 13, 3666–3674. [Google Scholar] [CrossRef] [PubMed]
- Aronica, C.; Chumakov, Y.; Jeanneau, E.; Luneau, D.; Neugebauer, P.; Barra, A.L.; Gillon, B.; Goujon, A.; Cousson, A.; Tercero, J.; et al. Structure, Magnetic Properties, Polarized Neutron Diffraction, and Theoretical Study of a Copper(II) Cubane. Chem. Eur. J. 2008, 14, 9540–9548. [Google Scholar] [CrossRef]
- Kahn, O. Chemistry and Physics of Supramolecular Magnetic Materials. Acc. Chem. Res. 2000, 33, 647–657. [Google Scholar] [CrossRef]
- Schweizer, J.; Golhen, S.; Lelievre-Berna, E.; Ouahab, L.; Pontillon, Y.; Ressouche, E. Magnetic Interactions and Spin Densities in Molecular Compounds: An Example. Phys. B Condens. Matter 2001, 297, 213–220. [Google Scholar] [CrossRef]
- Claiser, N.; Souhassou, M.; Lecomte, C.; Pontillon, Y.; Romero, F.; Ziessel, R. Understanding Magnetic Interaction Pathways: An Experimental Determination of Electron Density in an Alkyne-Substituted Nitronyl Nitroxide Radical. J. Phys. Chem. B 2002, 106, 12896–12907. [Google Scholar] [CrossRef]
- Borta, A.; Gillon, B.; Gukasov, A.; Cousson, A.; Luneau, D.; Jeanneau, E.; Ciumacov, I.; Sakiyama, H.; Tone, K.; Mikuriya, M. Local Magnetic Moments in a Dinuclear Co2+ Complex as Seen by Polarized Neutron Diffraction: Beyond the Effective Spin-1/2 Model. Phys. Rev. B 2011, 83, 184429. [Google Scholar] [CrossRef]
- Ridier, K.; Gillon, B.; Gukasov, A.; Chaboussant, G.; Cousson, A.; Luneau, D.; Borta, A.; Jacquot, J.F.; Checa, R.; Chiba, Y.; et al. Polarized Neutron Diffraction as a Tool for Mapping Molecular Magnetic Anisotropy: Local Susceptibility Tensors in Co-Ii Complexes. Chem. Eur. J. 2016, 22, 724–735. [Google Scholar] [CrossRef] [PubMed]
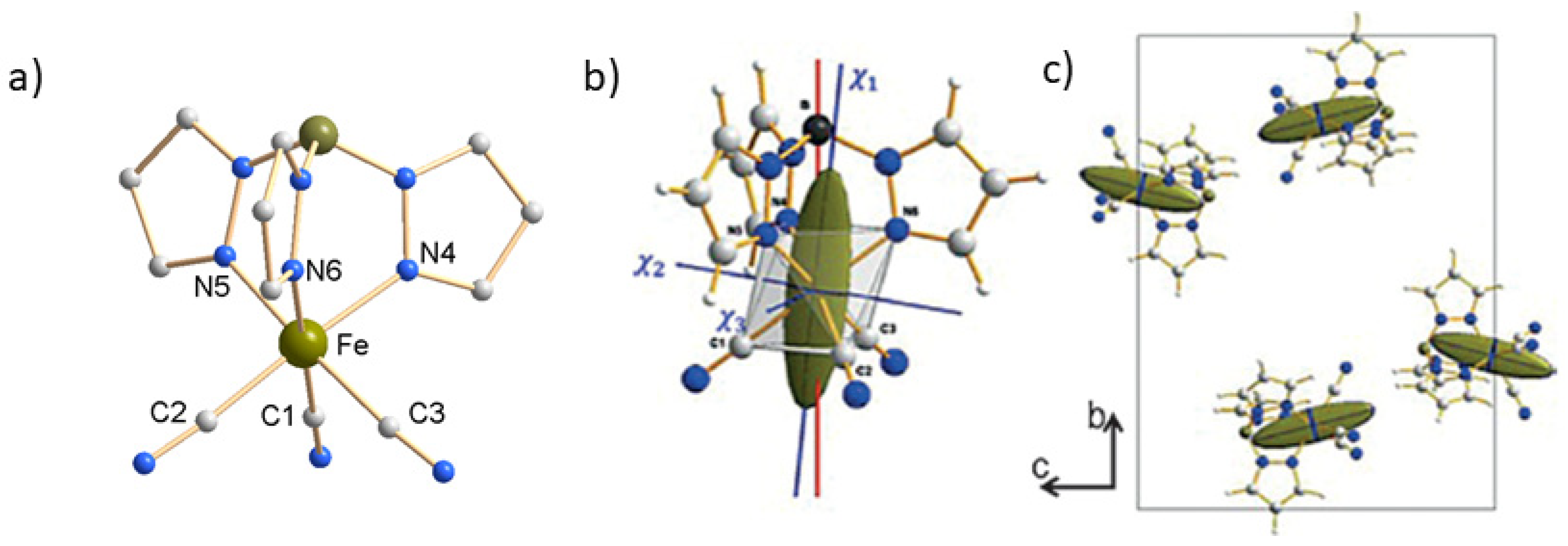
- Ridier, K.; Mondal, A.; Boilleau, C.; Cador, O.; Gillon, B.; Chaboussant, G.; le Guennic, B.; Costuas, K.; Lescouezec, R. Polarized Neutron Diffraction to Probe Local Magnetic Anisotropy of a Low-Spin Fe(III) Complex. Angew. Chem. Int. Ed. 2016, 55, 3963–3967. [Google Scholar] [CrossRef] [PubMed]
- De, S.; Flambard, A.; Garnier, D.; Herson, P.; Khler, F.H.; Mondal, A.; Costuas, K.; Gillon, B.; Lescouzec, R.; Guennic, B.; et al. Probing the Local Magnetic Structure of the Fe- III (Tp)(CN) 3- Building Block Via Solid-State Nmr Spectroscopy, Polarized Neutron Diffraction, and First-Principle Calculations. Chem. Eur. J. 2019, 25, 12120–12136. [Google Scholar] [CrossRef] [PubMed] [Green Version]
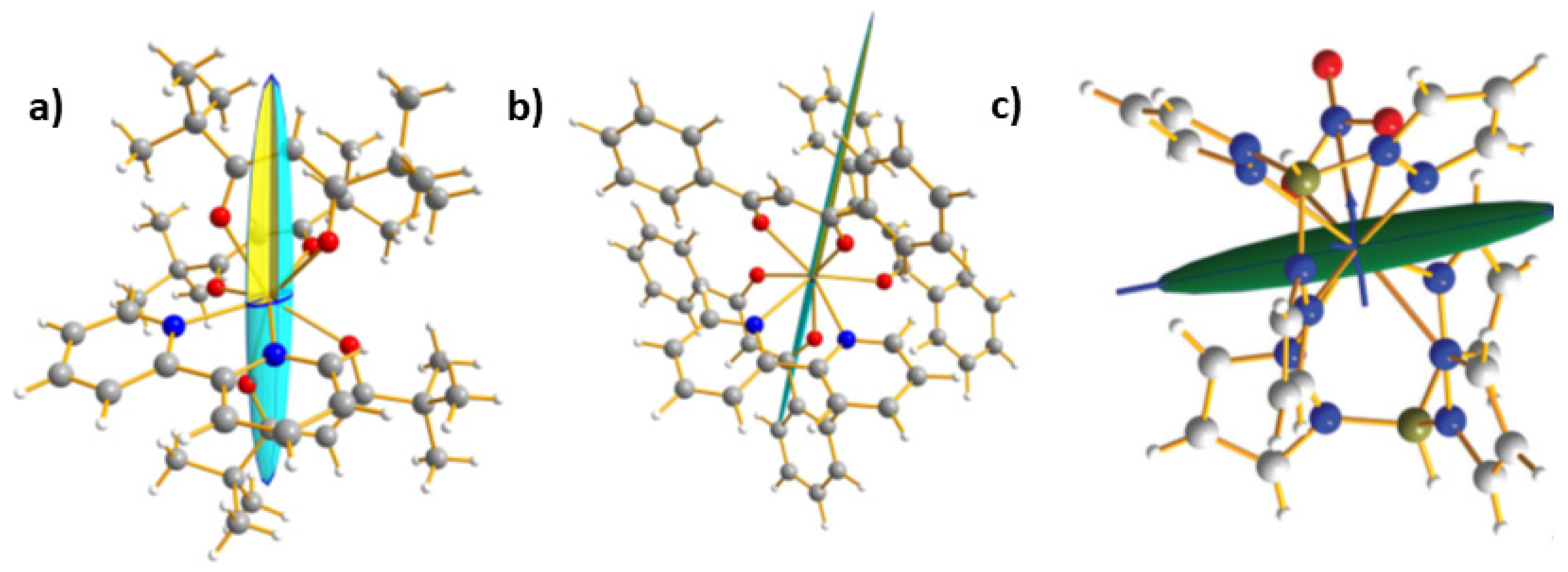
- Iasco, O.; Chumakov, Y.; Guegan, F.; Gillon, B.; Lenertz, M.; Bataille, A.; Jacquot, J.F.; Luneau, D. Mapping the Magnetic Anisotropy inside a Ni4 Cubane Spin Cluster Using Polarized Neutron Diffraction. Magnetochemistry 2017, 3, 25. [Google Scholar] [CrossRef] [Green Version]
- Klahn, E.A.; Gao, C.; Gillon, B.; Gukasov, A.; Fabreges, X.; Piltz, R.O.; Jiang, S.D.; Overgaard, J. Mapping the Magnetic Anisotropy at the Atomic Scale in Dysprosium Single-Molecule Magnets. Chem. Eur. J. 2018, 24, 16576–16581. [Google Scholar] [CrossRef] [PubMed]
- Guegan, F.; Jung, J.; le Guennic, B.; Riobe, F.; Maury, O.; Gillon, B.; Jacquot, J.F.; Guyot, Y.; Morell, C.; Luneau, D. Evidencing under-Barrier Phenomena in a Yb(III) Smm: A Joint Luminescence/Neutron Diffraction/Squid Study. Inorg. Chem. Front. 2019, 6, 3152–3157. [Google Scholar] [CrossRef]
- Gukasov, A.; Brown, P.J. Determination of Atomic Site Susceptibility Tensors from Polarized Neutron Diffraction Data. Phys. Condens. Mat. 2002, 14, 8831–8839. [Google Scholar] [CrossRef]
- Nye, J.F. Physical Properties of Crystals: Their Representation by Tensors and Matrices; Clarendon Press: London, UK, 1985. [Google Scholar]
- Matthewman, J.C.; Thompson, P.; Brown, P.J. The Cambridge Crystallography Subroutine Library. J. Appl. Crystallogr. 1982, 15, 167–173. [Google Scholar] [CrossRef]
- Kahn, O. Molecular Magnetism; VCH: New York, NY, USA, 1993. [Google Scholar]
- Gatteschi, D.; Barra, A.L.; Caneschi, A.; Cornia, A.; Sessoli, R.; Sorace, L. Epr of Molecular Nanomagnets. Coord. Chem. Rev. 2006, 250, 1514–1529. [Google Scholar] [CrossRef]
- Castelli, L.; Fittipaldi, M.; Powell, A.K.; Gatteschi, D.; Sorace, L. Single Crystal Epr Study at 95 Ghz of a Large Fe Based Molecular Nanomagnet: Toward the Structuring of Magnetic Nanoparticle Properties. Dalton Trans. 2011, 40, 8145–8155. [Google Scholar] [CrossRef] [PubMed]
- Palacios, M.A.; Nehrkorn, J.; Suturina, E.A.; Ruiz, E.; Gomez-Coca, S.; Holldack, K.; Schnegg, A.; Krzystek, J.; Moreno, J.M.; Colacio, E. Analysis of Magnetic Anisotropy and the Role of Magnetic Dilution in Triggering Single-Molecule Magnet (Smm) Behavior in a Family of (Co VIII)-YII Dinuclear Complexes with Easy-Plane Anisotropy. Chem. Eur. J. 2017, 23, 11649–11661. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zhai, Y.Q.; Ge, N.; Li, Z.H.; Chen, W.P.; Han, T.; Ouyang, Z.W.; Wang, Z.X.; Zheng, Y.Z. Magnetic Anisotropy: Structural Correlation of a Series of Chromium(II)-Amidinate Complexes. Inorg. Chem. 2021, 60, 1344–1351. [Google Scholar] [CrossRef]
- Perfetti, M.; Sorensen, M.A.; Hansen, U.B.; Bamberger, H.; Lenz, S.; Hallmen, P.P.; Fennell, T.; Simeoni, G.G.; Arauzo, A.; Bartolome, J.; et al. Magnetic Anisotropy Switch: Easy Axis to Easy Plane Conversion and Vice Versa. Adv. Funct. Mater. 2018, 28, 1801846. [Google Scholar] [CrossRef]
- Cornia, A.; Affronte, M.; Jansen, A.G.M.; Gatteschi, D.; Caneschi, A.; Sessoli, R. Magnetic Anisotropy of Mn-12-Acetate Nanomagnets from High-Field Torque Magnetometry. Chem. Phys. Lett. 2000, 322, 477–482. [Google Scholar] [CrossRef]
- Cucinotta, G.; Perfetti, M.; Luzon, J.; Etienne, M.; Car, P.-E.; Caneschi, A.; Calvez, G.; Bernot, K.; Sessoli, R. Magnetic Anisotropy in a Dysprosium/Dota Single-Molecule Magnet: Beyond Simple Magneto-Structural Correlations. Angew. Chem. Int. Ed. 2012, 51, 1606–1610. [Google Scholar] [CrossRef]
- Briganti, M.; Lucaccini, E.; Chelazzi, L.; Ciattini, S.; Sorace, L.; Sessoli, R.; Totti, F.; Perfetti, M. Magnetic Anisotropy Trends Along a Full 4f-Series: The F(N)+7 Effect. J. Am. Chem. Soc. 2021, 143, 8108–8115. [Google Scholar] [CrossRef]
- Abe, K.; Chiba, Y.; Yoshioka, D.; Yamaguchi, R.; Mikuriya, M.; Sakiyama, H. X-ray Structure Analysis Online. Jpn. Soc. Anal. Chem. 2012, 28, 65–66. [Google Scholar]
- Lescouëzec, R.; Vaissermann, J.; Lloret, F.; Julve, M.; Verdaguer, M. Ferromagnetic Coupling between Low- and High-Spin Iron(Iii) Ions in the Tetranuclear Complex Fac-{[Feiii{Hb(Pz)3}(Cn)2(Μ-Cn)]3feiii(H2o)3}·6h2o ([Hb(Pz)3]- = Hydrotris(1-Pyrazolyl)Borate). Inorg. Chem. 2002, 41, 5943–5945. [Google Scholar] [CrossRef]
- Qian, K.; Baldovi, J.J.; Jiang, S.D.; Gaita-Arino, A.; Zhang, Y.Q.; Overgaard, J.; Wang, B.W.; Coronado, E.; Gao, S. Does the Thermal Evolution of Molecular Structures Critically Affect the Magnetic Anisotropy? Chem. Sci. 2015, 6, 4587–4593. [Google Scholar] [CrossRef] [Green Version]
- Chilton, N.F.; Collison, D.; McInnes, E.J.; Winpenny, R.E.; Soncini, A. An Electrostatic Model for the Determination of Magnetic Anisotropy in Dysprosium Complexes. Nat. Commun. 2013, 4, 2551. [Google Scholar] [CrossRef] [PubMed]
- Tone, K.; Sakiyama, H.; Mikuriya, M.; Yamasaki, M.; Nishida, Y. Magnetic behavior of dinuclear cobalt (II) complexes assumed to be caused by a paramagnetic impurity can be explained by tilts of local distortion axes. Inorg. Chem. Commun. 2007, 10, 944–947. [Google Scholar] [CrossRef]
- Ruiz, E.; Rodríguez-Fortea, A.; Alemany, P.; Alvarez, S. Density Functional Study of the Exchange Coupling in Distorted Cubane Complexes Containing the Cu4o4 Core. Polyhedron 2001, 20, 1323–1327. [Google Scholar] [CrossRef]
- Nihei, M.; Hoshino, N.; Ito, T.; Oshio, H. Structures and Magnetic Properties of Metal Cubes. Polyhedron 2003, 22, 2359–2362. [Google Scholar] [CrossRef]
- Kibalin, I.A.; Gukasov, A. Local Magnetic Anisotropy by Polarized Neutron Powder Diffraction: Application of Magnetically Induced Preferred Crystallite Orientation. Phys. Rev. Res. 2019, 1, 033100. [Google Scholar] [CrossRef] [Green Version]
| Co(1) | Co(2) | ||
|---|---|---|---|
| (a, c) plane | χ1 () | 0.69(9) | 0.68(8) |
| χ2 () | −0.24(9) | −0.17(8) | |
| b axis | χ3 () | 0.03(4) | 0.06(8) |
| χ11 | χ22 | χ33 | χ23 | χ31 | χ12 | ||
|---|---|---|---|---|---|---|---|
| Cluster 1 | [Ni4] | 1.88(24) | 2.32(12) | 1.04(12) | 0.0 | 0.0 | −0.72(28) |
| Ions 2 | Ni1 | 0.63(18) | 0.68(12) | 0.16(9) | 0.0 | 0.0 | 0.0 |
| Ni2 | 0.42(20) | 0.48(8) | 0.33(6) | 0.0 | 0.0 | 0.0 | |
| Ni3 | 0.23(18) | 0.58(9) | 0.32(7) | 0.0 | 0.0 | −0.18(12) | |
| Ni4 | 0.74(21) | 0.85(11) | 0.20(9) | 0.0 | 0.0 | −0.33(13) | |
| Pairs 3 | Ni1-Ni4 | 1.40(24) | 1.50(14) | 0.36(10) | 0.0 | 0.0 | −0.26(16) |
| Ni2-Ni3 | 0.60(16) | 1.02(10) | 0.66(8) | 0.0 | 0.0 | 0.0 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Luneau, D.; Gillon, B. Polarized Neutron Diffraction: An Excellent Tool to Evidence the Magnetic Anisotropy—Structural Relationships in Molecules. Magnetochemistry 2021, 7, 158. https://doi.org/10.3390/magnetochemistry7120158
Luneau D, Gillon B. Polarized Neutron Diffraction: An Excellent Tool to Evidence the Magnetic Anisotropy—Structural Relationships in Molecules. Magnetochemistry. 2021; 7(12):158. https://doi.org/10.3390/magnetochemistry7120158
Chicago/Turabian StyleLuneau, Dominique, and Béatrice Gillon. 2021. "Polarized Neutron Diffraction: An Excellent Tool to Evidence the Magnetic Anisotropy—Structural Relationships in Molecules" Magnetochemistry 7, no. 12: 158. https://doi.org/10.3390/magnetochemistry7120158
APA StyleLuneau, D., & Gillon, B. (2021). Polarized Neutron Diffraction: An Excellent Tool to Evidence the Magnetic Anisotropy—Structural Relationships in Molecules. Magnetochemistry, 7(12), 158. https://doi.org/10.3390/magnetochemistry7120158







