Resonant Soft X-ray Reflectivity in the Study of Magnetic Properties of Low-Dimensional Systems
Abstract
1. Introduction
2. Methods
2.1. Experimental Setup
2.2. Origin of the Magnetic Dichorism Effect
3. Applications
3.1. First Studies
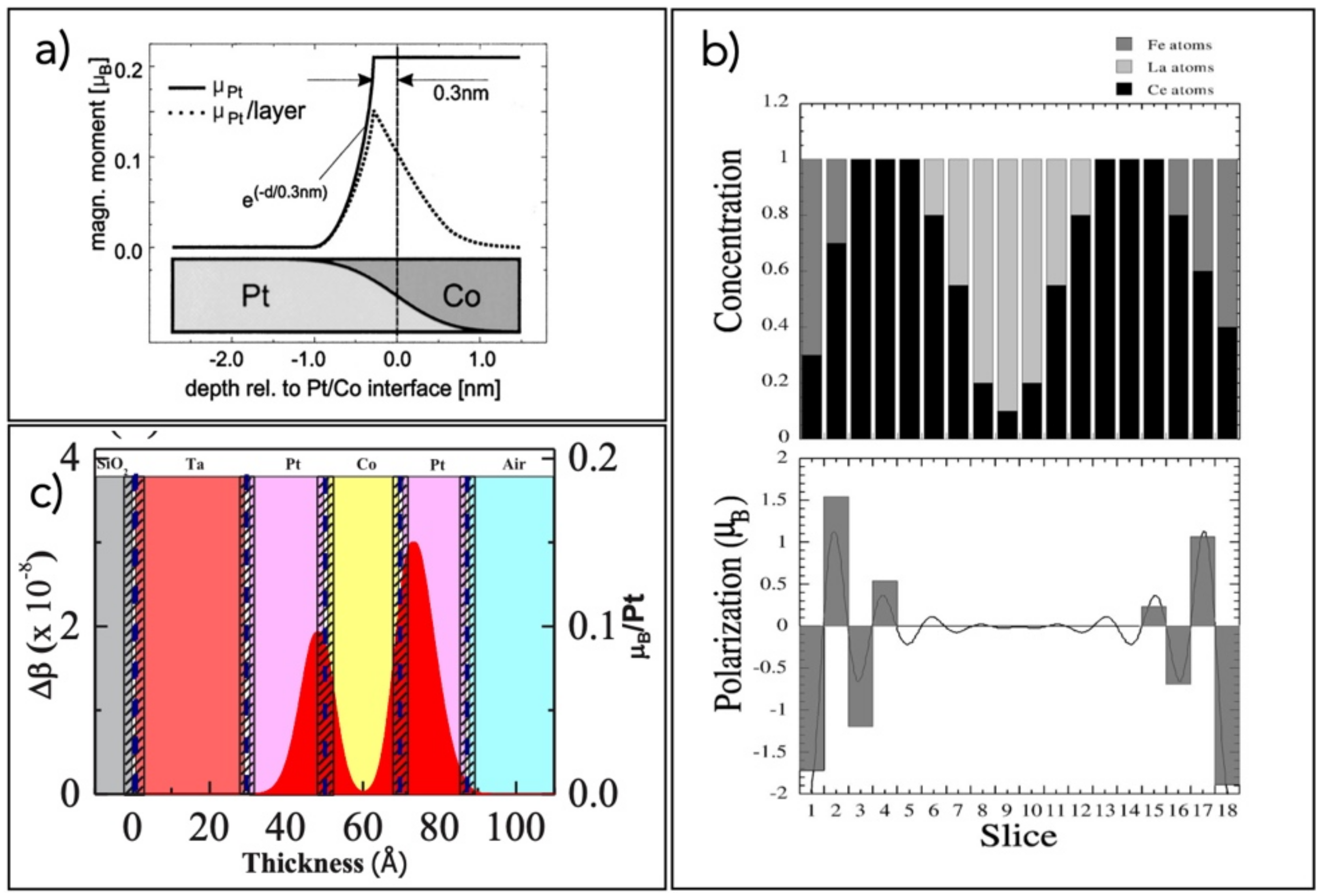
3.2. Magnetic Proximity Effects
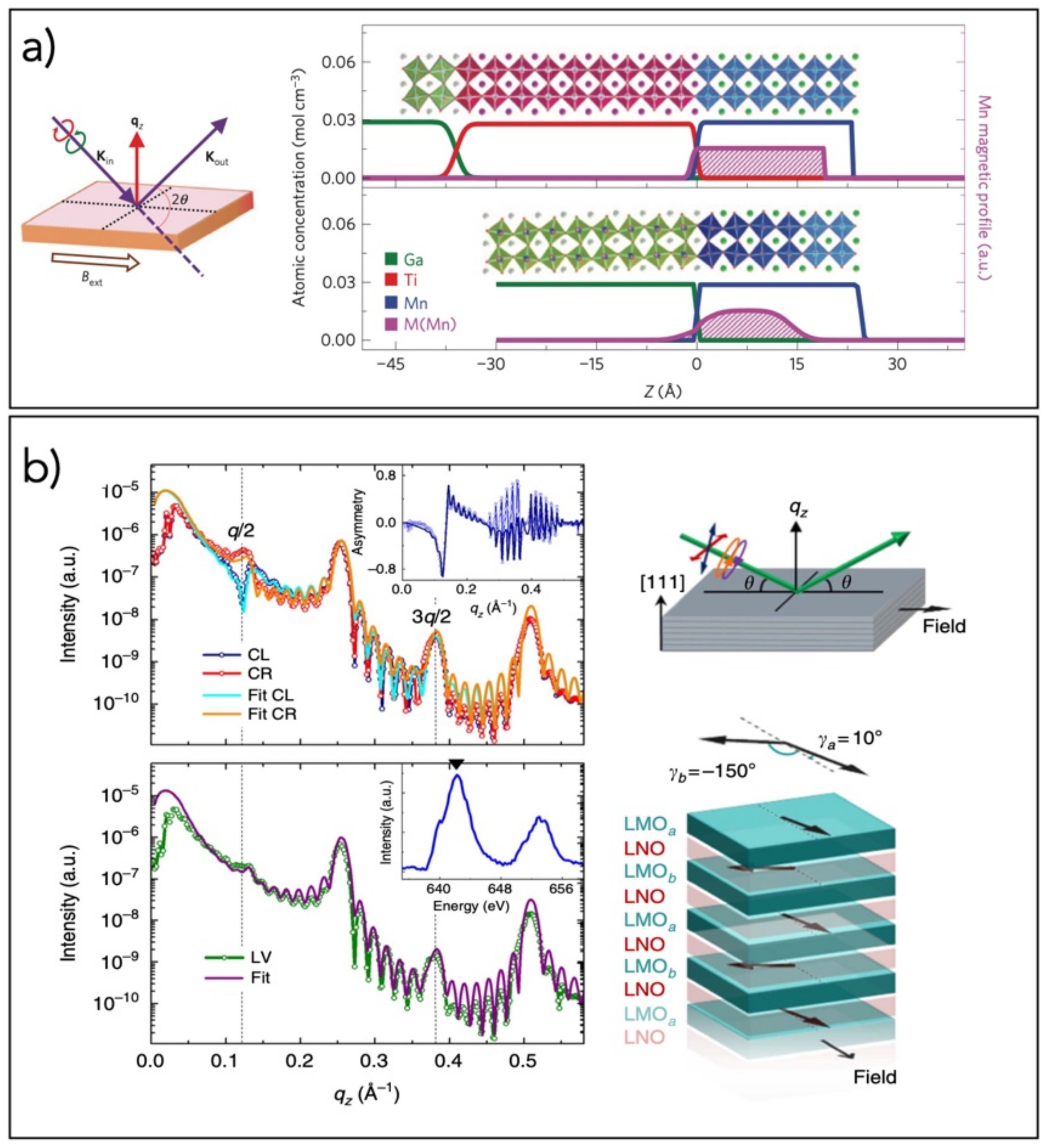
3.3. Transition Metal Oxides
3.4. Exchange-Bias Systems
3.5. Metallic Thin Films and Multilayers
3.6. Other Cases
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
References
- Als-Nielsen, J. Elements of Modern X-ray Physics; Wiley: New York, NY, USA, 2001. [Google Scholar]
- Atwood, D. Soft X-rays and Extreme Ultraviolet Radiation; Cambridge University Press: Cambridge, UK, 1999. [Google Scholar]
- Macke, S.; Radi, A.; Hamann-Borrero, J.E.; Verna, A.; Bluschke, M.; Brück, S.; Goering, E.; Sutarto, R.; He, F.; Cristiani, G.; et al. Element specific monolayer depth profiling. Adv. Mater. 2014, 26, 6554–6559. [Google Scholar] [CrossRef]
- Zwiebler, M.; Hamann-Borrero, J.E.; Vafaee, M.; Komissinskiy, P.; Macke, S.; Sutarto, R.; He, F.; Büchner, B.; Sawatzky, G.A.; Alff, L.; et al. Electronic depth profiles with atomic layer resolution from resonant soft X-ray reflectivity. New J. Phys. 2015, 17, 083046. [Google Scholar] [CrossRef]
- Ade, H. Characterization of organic thin films with resonant soft X-ray scattering and reflectivity near the carbon and fluorine absorption edges. Eur. Phys. J. Spec. Top. 2012, 208, 305–318. [Google Scholar] [CrossRef][Green Version]
- Mezger, M.; Ocko, B.M.; Reichert, H.; Deutsch, M. Surface layering and melting in an ionic liquid studied by resonant soft X-ray reflectivity. Proc. Natl. Acad. Sci. USA 2013, 110, 3733–3737. [Google Scholar] [CrossRef] [PubMed]
- Pasquali, L.; Mukherjee, S.; Terzi, F.; Giglia, A.; Mahne, N.; Koshmak, K.; Esaulov, V.; Toccafondi, C.; Canepa, M.; Nannarone, S. Structural and electronic properties of anisotropic ultrathin organic films from dichroic resonant soft X-ray reflectivity. Phys. Rev. B—Condens. Matter Mater. Phys. 2014, 89, 045401. [Google Scholar] [CrossRef]
- Stohr, J.; Siegmann, H.C. Magnetism: From Fundamentals to Nanoscale Dynamics; Springer: Berlin/Heidelberg, Germany, 2006; Volume 152. [Google Scholar]
- Stohr, J. X-ray Magnetic Circular Dichroism: Basic Concepts and Theory for 3D Transition Metal Atoms. In New Directions in Research with Third-Generation Soft X-ray Synchrotron Radiation Sources; Schlachter, A.S., Wuilleumier, F.J., Eds.; Springer: Dordrecht, The Netherlands, 1994; pp. 221–250. [Google Scholar]
- Mariot, J.-M.; Brouder, C. Spectroscopy and Magnetism: An Introduction. In Magnetism and Synchrotron Radiation; Beaurepaire, E., Scheurer, F., Krill, G., Kappler, J.-P., Eds.; Springer: Berlin/Heidelberg, Germany, 2002; pp. 24–59. [Google Scholar]
- Paroli, P. An introduction to magneto-optics. In Magnetic Properties of Matter; Borsa, F., Tognetti, V., Eds.; World Scientific: Singapore, 1988; pp. 335–368. [Google Scholar]
- Landau, L.D.; Lifshitz, E.M. Electrodynamics of Continous Media; Pergamon Press: Oxford, UK, 1984. [Google Scholar]
- Freiser, M.J. A Survey of Magnetooptic Effects. IEEE Trans. Magn. 1968, 4, 152–161. [Google Scholar] [CrossRef]
- Capelli, R.; Mahne, N.; Koshmak, K.; Giglia, A.; Doyle, B.P.; Mukherjee, S.; Nannarone, S.; Pasquali, L. Quantitative resonant soft X-ray reflectivity of ultrathin anisotropic organic layers: Simulation and experiment of PTCDA on Au. J. Chem. Phys. 2016, 145, 024201. [Google Scholar] [CrossRef]
- Parratt, L.G. Surface studies of solids by total reflection of X-rays. Phys. Rev. 1954, 95, 359–369. [Google Scholar] [CrossRef]
- Macke, S.; Goering, E. Magnetic reflectometry of heterostructures. J. Phys. Condens. Matter 2014, 26, 363201. [Google Scholar] [CrossRef]
- Yeh, P. Optical Waves in Layered Media; Wiley Series in Pure and Applied Optics; Wiley: Hoboken, NJ, USA, 2005. [Google Scholar]
- Zak, J.; Moog, E.R.; Liu, C.; Bader, S.D. Magneto-optics of multilayers with arbitrary magnetization directions. Phys. Rev. B—Condens. Matter Mater. Phys. 1991, 43, 6423–6429. [Google Scholar] [CrossRef]
- Yeh, P. Optics of anisotropic layered media: A new 4 × 4 matrix algebra. Surf. Sci. 1980, 96, 41–53. [Google Scholar] [CrossRef]
- Yariv, A.; Yeh, P. Optical Waves in Crystals: Propagation and Control of Laser Radiation; Wiley Series in Pure and Applied Optics; Wiley: New York, NY, USA, 1984. [Google Scholar]
- Zak, J.; Moog, E.R.; Liu, C.; Bader, S.D. Universal approach to magneto-optics. J. Magn. Magn. Mater. 1990, 89, 107–123. [Google Scholar] [CrossRef]
- Yeh, P. Electromagnetic propagation in birefringent layered media. J. Opt. Soc. Am. 1979, 69, 742–756. [Google Scholar] [CrossRef]
- Berreman, D.W. Optics in Stratified and Anisotropic Media: 4×4-Matrix Formulation. J. Opt. Soc. Am. 1972, 62, 502. [Google Scholar] [CrossRef]
- Bertrand, P.; Hermann, C.; Lampel, G.; Peretti, J.; Safarov, V.I. General analytical treatment of optics in layered structures: Application to magneto-optics. Phys. Rev. B—Condens. Matter Mater. Phys. 2001, 64, 235421. [Google Scholar] [CrossRef]
- Pasquali, L.; Mahne, N.; Giglia, A.; Verna, A.; Sponza, L.; Capelli, R.; Bonfatti, M.; Mezzadri, F.; Galligani, E.; Nannarone, S. Analysis of Resonant Soft X-ray Reflectivity of Anisotropic Layered Materials. Surfaces 2021, 4, 18–30. [Google Scholar] [CrossRef]
- Smith, D.Y. Superconvergence and sum rules for the optical constants: Natural and magneto-optical activity. Phys. Rev. B 1976, 13, 5303–5315. [Google Scholar] [CrossRef]
- Nannarone, S.; Borgatti, F.; Deluisa, A.; Doyle, B.P.; Gazzadi, G.C.; Giglia, A.; Finetti, P.; Mahne, N.; Pasquali, L.; Pedio, M.; et al. The BEAR beamline at elettra. AIP Conf. Proc. 2004, 705, 450–453. [Google Scholar]
- BEAR beamline. 2021. Available online: www.elettra.trieste.it/elettra-beamlines/bear.html (accessed on 3 October 2021).
- Pasquali, L.; De Luisa, A.; Nannarone, S. The UHV experimental chamber for optical measurements (reflectivity and absorption) and angle resolved photoemission of the BEAR beamline at ELETTRA. AIP Conf. Proc. 2004, 705, 1142–1145. [Google Scholar]
- Haverkort, M.W.; Hollmann, N.; Krug, I.P.; Tanaka, A. Symmetry analysis of magneto-optical effects: The case of X-ray diffraction and X-ray absorption at the transition metal L2,3 edge. Phys. Rev. B—Condens. Matter Mater. Phys. 2010, 82, 094403. [Google Scholar] [CrossRef]
- Smith, D.Y. Dispersion relations and sum rules for magnetoreflectivity. J. Opt. Soc. Am. 1976, 66, 547–554. [Google Scholar] [CrossRef]
- Smith, D.Y. Comments on the dispersion relations for the complex refractive index of circularly and elliptically polarized light*. J. Opt. Soc. Am. 1976, 66, 454–460. [Google Scholar] [CrossRef]
- Kao, C.; Hastings, J.B.; Johnson, E.D.; Siddons, D.P.; Smith, G.C.; Prinz, G.A. Magnetic-resonance exchange scattering at the iron LII and LIII edges. Phys. Rev. Lett. 1990, 65, 373–376. [Google Scholar] [CrossRef]
- Kao, C.C.; Chen, C.T.; Johnson, E.D.; Hastings, J.B.; Lin, H.J.; Ho, G.H.; Meigs, G.; Brot, J.M.; Hulbert, S.L.; Idzerda, Y.U.; et al. Dichroic interference effects in circularly polarized soft-X-ray resonant magnetic scattering. Phys. Rev. B 1994, 50, 9599–9602. [Google Scholar] [CrossRef]
- Kortright, J.B.; Kim, S.K. Resonant magneto-optical properties of Fe near its 2p levels: Measurement and applications. Phys. Rev. B—Condens. Matter Mater. Phys. 2000, 62, 12216–12228. [Google Scholar] [CrossRef]
- Mertins, H.C.; Abramsohn, D.; Gaupp, A.; Schäfers, F.; Gudat, W.; Zaharko, O.; Grimmer, H.; Oppeneer, P.M. Resonant magnetic reflection coefficients at the Fe (formula presented) edge obtained with linearly and circularly polarized soft x rays. Phys. Rev. B—Condens. Matter Mater. Phys. 2002, 66, 1–8. [Google Scholar] [CrossRef]
- Sacchi, M.; Hague, C.F.; Pasquali, L.; Mirone, A.; Mariot, J.M.; Isberg, P.; Gullikson, E.M.; Underwood, J.H. Optical constants of ferromagnetic iron via 2p resonant magnetic scattering. Phys. Rev. Lett. 1998, 81, 1521–1524. [Google Scholar] [CrossRef]
- Sacchi, M.; Mirone, A. Resonant reflectivity from a Ni(110) crystal: Magnetic effects at the Ni 2p edges using linearly and circularly polarized photons. Phys. Rev. B—Condens. Matter Mater. Phys. 1998, 57, 8408–8415. [Google Scholar] [CrossRef]
- Tonnerre, J.M.; Jaouen, N.; Bontempi, E.; Carbone, D.; Babonneau, D.; De Santis, M.; Tolentino, N.; Grenier, S.; Garaudee, S.; Staub, U. Soft X-ray resonant magnetic reflectivity studies for in-and out-of-plane magnetization profile in ultra thin films. J. Phys. Conf. Ser. 2010, 211, 012015. [Google Scholar] [CrossRef]
- Abes, M.; Atkinson, D.; Tanner, B.K.; Charlton, T.R.; Langridge, S.; Hase, T.P.A.; Ali, M.; Marrows, C.H.; Hickey, B.J.; Neudert, A.; et al. Spin polarization and exchange coupling of Cu and Mn atoms in paramagnetic CuMn diluted alloys induced by a Co layer. Phys. Rev. B—Condens. Matter Mater. Phys. 2010, 82, 184412. [Google Scholar] [CrossRef]
- Awaji, N.; Noma, K.; Nomura, K.; Doi, S.; Hirono, T.; Kimura, H.; Nakamura, T. Soft X-ray resonant magnetic reflectivity study on induced magnetism in [Fe70Co30/Pd]nsuper-lattice films. J. Phys. Conf. Ser. 2007, 83, 012034. [Google Scholar] [CrossRef]
- Moriya, T. Anisotropic Superexchange Interaction and Weak Ferromagnetism. Phys. Rev. 1960, 120, 91–98. [Google Scholar] [CrossRef]
- Dzyaloshinsky, I. A thermodynamic theory of “weak” ferromagnetism of antiferromagnetics. J. Phys. Chem. Solids 1958, 4, 241–255. [Google Scholar] [CrossRef]
- Belmeguenai, M.; Roussigné, Y.; Bouloussa, H.; Chérif, S.M.; Stashkevich, A.; Nasui, M.; Gabor, M.S.; Mora-Hernández, A.; Nicholson, B.; Inyang, O.O.; et al. Thickness Dependence of the Dzyaloshinskii-Moriya Interaction in Co2FeAl Ultrathin Films: Effects of Annealing Temperature and Heavy-Metal Material. Phys. Rev. Appl. 2018, 9, 044044. [Google Scholar] [CrossRef]
- Geissler, J.; Goering, E.; Justen, M.; Weigand, F.; Schütz, G.; Langer, J.; Schmitz, D.; Maletta, H.; Mattheis, R. Pt magnetization profile in a Pt/Co bilayer studied by resonant magnetic X-ray reflectometry. Phys. Rev. B—Condens. Matter Mater. Phys. 2002, 65, 1–4. [Google Scholar] [CrossRef]
- Graulich, D.; Krieft, J.; Moskaltsova, A.; Demir, J.; Peters, T.; Pohlmann, T.; Bertram, F.; Wollschläger, J.; Jose, J.R.; Francoual, S.; et al. Quantitative comparison of the magnetic proximity effect in Pt detected by XRMR and XMCD. Appl. Phys. Lett. 2021, 118, 012407. [Google Scholar] [CrossRef]
- Hosoito, N.; Ohkochi, T.; Kodama, K.; Suzuki, M. Charge and induced magnetic structures of Au layers in Fe/Au bilayer and Fe/Au/Fe trilayer films by resonant X-ray magnetic reflectivity at the Au L 3 absorption edge. J. Phys. Soc. Japan 2014, 83. [Google Scholar] [CrossRef]
- Jaouen, N.; Tonnerre, J.M.; Raoux, D.; Bontempi, E.; Ortega, L.; Müenzenberg, M.; Felsch, W.; Rogalev, A.; Dürr, H.A.; Dudzik, E.; et al. Ce 5d magnetic profile in Fe/Ce multilayers for the (formula presented) and (formula presented) -like Ce phases by X-ray resonant magnetic scattering. Phys. Rev. B—Condens. Matter Mater. Phys. 2002, 66, 1–14. [Google Scholar] [CrossRef]
- Kim, D.O.; Song, K.M.; Choi, Y.; Min, B.C.; Kim, J.S.; Choi, J.W.; Lee, D.R. Asymmetric magnetic proximity effect in a Pd/Co/Pd trilayer system. Sci. Rep. 2016, 6, 1–8. [Google Scholar] [CrossRef]
- Klewe, C.; Kuschel, T.; Schmalhorst, J.M.; Bertram, F.; Kuschel, O.; Wollschläger, J.; Strempfer, J.; Meinert, M.; Reiss, G. Static magnetic proximity effect in Pt/ Ni1-x Fex bilayers investigated by X-ray resonant magnetic reflectivity. Phys. Rev. B 2016, 93, 214440. [Google Scholar] [CrossRef]
- Macke, S. ReMagX. 2018. Available online: https://www.remagx.org/wiki/doku.php (accessed on 3 October 2021).
- Krieft, J.; Graulich, D.; Moskaltsova, A.; Bouchenoire, L.; Francoual, S.; Kuschel, T. Advanced data analysis procedure for hard X-ray resonant magnetic reflectivity discussed for Pt thin film samples of various complexity. J. Phys. D Appl. Phys. 2020, 53, 375004. [Google Scholar] [CrossRef]
- Kuschel, T.; Klewe, C.; Schmalhorst, J.M.; Bertram, F.; Kuschel, O.; Schemme, T.; Wollschläger, J.; Francoual, S.; Strempfer, J.; Gupta, A.; et al. Static Magnetic Proximity Effect in Pt/NiFe2O4 and Pt/Fe Bilayers Investigated by X-ray Resonant Magnetic Reflectivity. Phys. Rev. Lett. 2015, 115, 097401. [Google Scholar] [CrossRef] [PubMed]
- Moskaltsova, A.; Krieft, J.; Graulich, D.; Matalla-Wagner, T.; Kuschel, T. Impact of the magnetic proximity effect in Pt on the total magnetic moment of Pt/Co/Ta trilayers studied by X-ray resonant magnetic reflectivity. AIP Adv. 2020, 10, 015154. [Google Scholar] [CrossRef]
- Mukhopadhyay, A.; Koyiloth Vayalil, S.; Graulich, D.; Ahamed, I.; Francoual, S.; Kashyap, A.; Kuschel, T.; Anil Kumar, P.S. Asymmetric modification of the magnetic proximity effect in Pt/Co/Pt trilayers by the insertion of a Ta buffer layer. Phys. Rev. B 2020, 102, 144435. [Google Scholar] [CrossRef]
- Rowan-Robinson, R.M.; Stashkevich, A.A.; Roussigné, Y.; Belmeguenai, M.; Chérif, S.M.; Thiaville, A.; Hase, T.P.A.; Hindmarch, A.T.; Atkinson, D. The interfacial nature of proximity-induced magnetism and the Dzyaloshinskii-Moriya interaction at the Pt/Co interface. Sci. Rep. 2017, 7, 1–11. [Google Scholar] [CrossRef]
- Rowan-Robinson, R.M.; Hindmarch, A.T.; Atkinson, D. Efficient current-induced magnetization reversal by spin-orbit torque in Pt/Co/Pt. J. Appl. Phys. 2018, 124, 183901. [Google Scholar] [CrossRef]
- Sève, L.; Jaouen, N.; Tonnerre, J.M.; Raoux, D.; Bartolomé, F.; Arend, M.; Felsch, W.; Rogalev, A.; Goulon, J.; Gautier, C.; et al. Profile of the induced 5d magnetic moments in Ce/Fe and La/Fe multilayers probed by X-ray magnetic-resonant scattering. Phys. Rev. B—Condens. Matter Mater. Phys. 1999, 60, 9662–9674. [Google Scholar] [CrossRef]
- Szuszkiewicz, W.; Ott, F.; Kisielewski, J.; Sveklo, I.; Dynowska, E.; Minikayev, R.; Kurant, Z.; Kuna, R.; Jakubowski, M.; Wawro, A.; et al. Polarized neutron reflectivity and X-ray scattering measurements as tools to study properties of Pt/Co/Pt ultrathin layers irradiated by femtosecond laser pulses. Phase Transit. 2016, 89, 328–340. [Google Scholar] [CrossRef]
- Huijben, M.; Koster, G.; Liao, Z.L.; Rijnders, G. Interface-engineered oxygen octahedral coupling in manganite heterostructures. Appl. Phys. Rev. 2017, 4, 041103. [Google Scholar] [CrossRef]
- Liao, Z.; Huijben, M.; Zhong, Z.; Gauquelin, N.; Macke, S.; Green, R.J.; Van Aert, S.; Verbeeck, J.; Van Tendeloo, G.; Held, K.; et al. Controlled lateral anisotropy in correlated manganite heterostructures by interface-engineered oxygen octahedral coupling. Nat. Mater. 2016, 15, 425–431. [Google Scholar] [CrossRef] [PubMed]
- Fabbris, G.; Jaouen, N.; Meyers, D.; Feng, J.; Hoffman, J.D.; Sutarto, R.; Chiuzbǎian, S.G.; Bhattacharya, A.; Dean, M.P.M. Emergent c-axis magnetic helix in manganite-nickelate superlattices. Phys. Rev. B. 2018, 98, 180401. [Google Scholar] [CrossRef]
- Gibert, M.; Viret, M.; Torres-Pardo, A.; Piamonteze, C.; Zubko, P.; Jaouen, N.; Tonnerre, J.M.; Mougin, A.; Fowlie, J.; Catalano, S.; et al. Interfacial Control of Magnetic Properties at LaMnO3/LaNiO3 Interfaces. Nano Lett. 2015, 15, 7355–7361. [Google Scholar] [CrossRef] [PubMed]
- Gibert, M.; Viret, M.; Zubko, P.; Jaouen, N.; Tonnerre, J.M.; Torres-Pardo, A.; Catalano, S.; Gloter, A.; Stéphan, O.; Triscone, J.M. Interlayer coupling through a dimensionality-induced magnetic state. Nat. Commun. 2016, 7, 1–7. [Google Scholar] [CrossRef][Green Version]
- Hühn, S.; Jungbauer, M.; Michelmann, M.; Massel, F.; Koeth, F.; Ballani, C.; Moshnyaga, V. Modeling of colossal magnetoresistance in La0.67Ca0.33MnO3/Pr0.67Ca0.33MnO3 superlattices: Comparison with individual (La1−yPry)0.67Ca0.33MnO3 films. J. Appl. Phys. 2013, 113, 17–701. [Google Scholar] [CrossRef]
- Freeland, J.W.; Gray, K.E.; Ozyuzer, L.; Berghuis, P.; Badica, E.; Kavich, J.; Zheng, H.; Mitchell, J.F. Full bulk spin polarization and intrinsic tunnel barriers at the surface of layered manganites. Nat. Mater. 2005, 4, 62–67. [Google Scholar] [CrossRef][Green Version]
- Verna, A.; Davidson, B.A.; Szeto, Y.; Petrov, A.Y.; Mirone, A.; Giglia, A.; Mahne, N.; Nannarone, S. Measuring magnetic profiles at manganite surfaces with monolayer resolution. J. Magn. Magn. Mater. 2010, 322, 1212–1216. [Google Scholar] [CrossRef][Green Version]
- Liao, Z.; Gauquelin, N.; Green, R.J.; Macke, S.; Gonnissen, J.; Thomas, S.; Zhong, Z.; Li, L.; Si, L.; Van Aert, S.; et al. Thickness Dependent Properties in Oxide Heterostructures Driven by Structurally Induced Metal-Oxygen Hybridization Variations. Adv. Funct. Mater. 2017, 27, 1606717. [Google Scholar] [CrossRef]
- Bertinshaw, J.; Brück, S.; Lott, D.; Fritzsche, H.; Khaydukov, Y.; Soltwedel, O.; Keller, T.; Goering, E.; Audehm, P.; Cortie, D.L.; et al. Element-specific depth profile of magnetism and stoichiometry at the La0.67Sr0.33MnO3/BiFeO3 interface. Phys. Rev. B—Condens. Matter Mater. Phys. 2014, 90, 041113. [Google Scholar] [CrossRef]
- Brück, S.; Treiber, S.; MacKe, S.; Audehm, P.; Christiani, G.; Soltan, S.; Habermeier, H.U.; Goering, E.; Albrecht, J. The temperature-dependent magnetization profile across an epitaxial bilayer of ferromagnetic La2/3Ca1/3MnO3 and superconducting YBa2Cu3O7-δ. New J. Phys. 2011, 13, 033023. [Google Scholar] [CrossRef]
- Satapathy, D.K.; Uribe-Laverde, M.A.; Marozau, I.; Malik, V.K.; Das, S.; Wagner, T.; Marcelot, C.; Stahn, J.; Brück, S.; Rühm, A.; et al. Magnetic proximity effect in YBa 2Cu3O7/La2/3Ca1/3MnO3 and YBa2Cu3O7/LaMnO3+δ superlattices. Phys. Rev. Lett. 2012, 108, 197201. [Google Scholar] [CrossRef]
- Freeland, J.W.; Chakhalian, J.; Boris, A.V.; Tonnerre, J.M.; Kavich, J.J.; Yordanov, P.; Grenier, S.; Zschack, P.; Karapetrova, E.; Popovich, P.; et al. Charge transport and magnetization profile at the interface between the correlated metal CaRuO3 and the antiferromagnetic insulator CaMnO3. Phys. Rev. B—Condens. Matter Mater. Phys. 2010, 81, 094414. [Google Scholar] [CrossRef]
- Brück, S.; Paul, M.; Tian, H.; Müller, A.; Kufer, D.; Praetorius, C.; Fauth, K.; Audehm, P.; Goering, E.; Verbeeck, J.; et al. Magnetic and electronic properties of the interface between half metallic Fe3O4 and semiconducting ZnO. Appl. Phys. Lett. 2012, 100, 081603. [Google Scholar] [CrossRef]
- Zafar, K.; Audehm, P.; Schütz, G.; Goering, E.; Pathak, M.; Chetry, K.B.; Leclair, P.R.; Gupta, A. Cr magnetization reversal at the CrO2/RuO2 interface: Origin of the reduced GMR effect. Phys. Rev. B—Condens. Matter Mater. Phys. 2011, 84, 134412. [Google Scholar] [CrossRef]
- Verna, A.; Davidson, B.A.; Mirone, A.; Nannarone, S. The influence of surface roughness in X-ray resonant magnetic reflectivity experiments. Eur. Phys. J. Spec. Top. 2012, 208, 165–175. [Google Scholar] [CrossRef][Green Version]
- Nogués, J.; Schuller, I.K. Exchange bias. J. Magn. Magn. Mater. 1999, 192, 203–232. [Google Scholar] [CrossRef]
- Roy, S.; Fitzsimmons, M.R.; Park, S.; Dorn, M.; Petracic, O.; Roshchin, I.V.; Li, Z.P.; Batlle, X.; Morales, R.; Misra, A.; et al. Depth profile of uncompensated spins in an exchange bias system. Phys. Rev. Lett. 2005, 95, 047201. [Google Scholar] [CrossRef]
- Roy, S.; Sanchez-Hanke, C.; Park, S.; Fitzsimmons, M.R.; Tang, Y.J.; Hong, J.I.; Smith, D.J.; Taylor, B.J.; Liu, X.; Maple, M.B.; et al. Evidence of modified ferromagnetism at a buried Permalloy/CoO interface at room temperature. Phys. Rev. B—Condens. Matter Mater. Phys. 2007, 75, 014442. [Google Scholar] [CrossRef]
- Blackburn, E.; Sanchez-Hanke, C.; Roy, S.; Smith, D.J.; Hong, J.I.; Chan, K.T.; Berkowitz, A.E.; Sinha, S.K. Pinned Co moments in a polycrystalline permalloy/CoO exchange-biased bilayer. Phys. Rev. B—Condens. Matter Mater. Phys. 2008, 78, 180408. [Google Scholar] [CrossRef]
- Lee, J.S.; Kao, C.C.; Jang, H.; Ko, K.T.; Park, J.H.; Rhie, K.; Kim, J.Y. Uncompensated spins in trilayer CoFe/IrMn/NiFe exchange bias: Soft X-ray resonant magnetic scattering study. J. Phys. Condens. Matter 2011, 23, 256001. [Google Scholar] [CrossRef]
- Brück, S.; MacKe, S.; Goering, E.; Ji, X.; Zhan, Q.; Krishnan, K.M. Coupling of Fe and uncompensated Mn moments in exchange-biased Fe/MnPd. Phys. Rev. B—Condens. Matter Mater. Phys. 2010, 81, 134414. [Google Scholar] [CrossRef]
- Brück, S.; Schütz, G.; Goering, E.; Ji, X.; Krishnan, K.M. Uncompensated moments in the MnPd/Fe Exchange Bias System. Phys. Rev. Lett. 2008, 101, 126402. [Google Scholar] [CrossRef] [PubMed]
- Radu, F.; Nefedov, A.; Grabis, J.; Nowak, G.; Bergmann, A.; Zabel, H. Soft X-ray resonant magnetic scattering studies on Fe/CoO exchange bias system. J. Magn. Magn. Mater. 2006, 300, 206–210. [Google Scholar] [CrossRef][Green Version]
- Gruyters, M.; Schmitz, D. Microscopic nature of ferro- and antiferromagnetic interface coupling of uncompensated magnetic moments in exchange bias systems. Phys. Rev. Lett. 2008, 100, 077205. [Google Scholar] [CrossRef]
- Mishra, S.K.; Radu, F.; Valencia, S.; Schmitz, D.; Schierle, E.; Dürr, H.A.; Eberhardt, W. Dual behavior of antiferromagnetic uncompensated spins in NiFe/IrMn exchange biased bilayers. Phys. Rev. B—Condens. Matter Mater. Phys. 2010, 81, 212404. [Google Scholar] [CrossRef]
- Mishra, S.K.; Radu, F.; Dürr, H.A.; Eberhardt, W. Training-induced positive exchange bias in NiFe/IrMn bilayers. Phys. Rev. Lett. 2009, 102, 177208. [Google Scholar] [CrossRef] [PubMed]
- Zaharko, O.; Oppeneer, P.M.; Grimmer, H.; Horisberger, M.; Mertins, H.C.; Abramsohn, D.; Schäfers, F.; Bill, A.; Braun, H.B. Exchange coupling in Fe/NiO/Co film studied by soft X-ray resonant magnetic reflectivity. Phys. Rev. B—Condens. Matter Mater. Phys. 2002, 66, 1–10. [Google Scholar] [CrossRef]
- Jungbauer, M.; Hühn, S.; Michelmann, M.; Goering, E.; Moshnyaga, V. Exchange bias in La0.7Sr0.3MnO3/SrMnO3/La0.7Sr0.3MnO3 trilayers. J. Appl. Phys. 2013, 113, 17–709. [Google Scholar] [CrossRef]
- Hase, T.P.A.; Fulthorpe, B.D.; Wilkins, S.B.; Tanner, B.K.; Marrows, C.H.; Mickey, B.J. Weak magnetic moment on IrMn exchange bias pinning layers. Appl. Phys. Lett. 2001, 79, 985–987. [Google Scholar] [CrossRef][Green Version]
- Mohanty, J.; Persson, A.; Arvanitis, D.; Temst, K.; Van Haesendonck, C. Direct observation of frozen moments in the NiFe/FeMn exchange bias system. New J. Phys. 2013, 15, 033016. [Google Scholar] [CrossRef]
- Audehm, P.; Schmidt, M.; Bruck, S.; Tietze, T.; Grafe, J.; MacKe, S.; Schutz, G.; Goering, E. Pinned orbital moments—A new contribution to magnetic anisotropy. Sci. Rep. 2016, 6, 1–8. [Google Scholar] [CrossRef] [PubMed]
- Doi, S.; Nomura, K.; Awaji, N.; Hosoito, N.; Yamagishi, R.; Suzuki, M. Magnetization profile of Ir in a MnIr/CoFe exchange bias system evaluated by hard X-ray resonant magnetic reflectivity. J. Appl. Phys. 2009, 106, 123919. [Google Scholar] [CrossRef]
- Violbarbosa, C.E.; Meyerheim, H.L.; Jal, E.; Tonnerre, J.M.; Przybylski, M.; Sandratskii, L.M.; Yildiz, F.; Staub, U.; Kirschner, J. Inhomogeneous temperature dependence of the magnetization in fcc-Fe on Cu(001). Phys. Rev. B—Condens. Matter Mater. Phys. 2012, 85, 184414. [Google Scholar] [CrossRef]
- Meyerheim, H.L.; Tonnerre, J.M.; Sandratskii, L.; Tolentino, H.C.N.; Przybylski, M.; Gabi, Y.; Yildiz, F.; Fu, X.L.; Bontempi, E.; Grenier, S.; et al. New model for magnetism in ultrathin fcc Fe on Cu(001). Phys. Rev. Lett. 2009, 103, 267202. [Google Scholar] [CrossRef]
- Brown, S.D.; Bouchenoire, L.; Thompson, P.; Springell, R.; Mirone, A.; Stirling, W.G.; Beesley, A.; Thomas, M.F.; Ward, R.C.C.; Wells, M.R.; et al. Profile of the U 5f magnetization in U/Fe multilayers. Phys. Rev. B—Condens. Matter Mater. Phys. 2008, 77, 014427. [Google Scholar] [CrossRef]
- Valvidares, S.M.; Quirós, C.; Mirone, A.; Tonnerre, J.M.; Stanescu, S.; Bencok, P.; Souche, Y.; Zárate, L.; Martín, J.I.; Vélez, M.; et al. Resolving antiferromagnetic states in magnetically coupled amorphous Co-Si-Si multilayers by soft X-ray resonant magnetic scattering. Phys. Rev. B—Condens. Matter Mater. Phys. 2008, 78, 064406. [Google Scholar] [CrossRef]
- Zaharko, O.; Mertins, H.C.; Grimmer, H.; Schäfers, F. Soft X-ray resonant magnetic reflectivity from Fe/C multilayers. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2001, 467–468, 1419–1422. [Google Scholar] [CrossRef]
- Meltchakov, E.; Mertins, H.C.; Scheer, M.; Di Fonzo, S.; Jark, W.; Schäfers, F. Soft X-ray resonant magnetic reflectivity of Gd/Fe multilayers. J. Magn. Magn. Mater. 2002, 240, 550–552. [Google Scholar] [CrossRef]
- Choi, Y.; Haskel, D.; Camley, R.E.; Lee, D.R.; Lang, J.C.; Srajer, G.; Jiang, J.S.; Bader, S.D. Temperature evolution of the Gd magnetization profile in strongly coupled Gd/Fe multilayers. Phys. Rev. B—Condens. Matter Mater. Phys. 2004, 70, 134420. [Google Scholar] [CrossRef]
- Haskel, D.; Srajer, G.; Lang, J.C.; Pollmann, J.; Nelson, C.S.; Jiang, J.S.; Bader, S.D. Enhanced interfacial magnetic coupling of Gd /Fe multilayers. Phys. Rev. Lett. 2001, 87, 207201. [Google Scholar] [CrossRef] [PubMed]
- Jonnard, P.; Le Guen, K.; André, J.M.; Delaunay, R.; Mahne, N.; Giglia, A.; Nannarone, S.; Verna, A.; Wang, Z.S.; Zhu, J.T.; et al. Determination of the magnetization profile of Co/Mg periodic multilayers by magneto-optic Kerr effect and X-ray magnetic resonant reflectivity. J. Phys. Conf. Ser. 2013, 417, 12025. [Google Scholar] [CrossRef]
- Sacchi, M.; Mirone, A.; Hague, C.F.; Hague, C.F.; Castrucci, P.; Gunnella, R.; De Crescenzi, M. Resonant magnetic scattering from fcc Cu/Fe/Cu/Si(111) heterostructures. Phys. Rev. B—Condens. Matter Mater. Phys. 2001, 64, 124031–124034. [Google Scholar] [CrossRef]
- Carlomagno, I.; Verna, A.; Forrest, T.; Meneghini, C. Structural Profile of a MgO/Co/MgO Trilayer Using Soft X-ray Resonant Magnetic Reflectivity. Springer Proc. Phys. 2021, 220, 155–167. [Google Scholar] [CrossRef]
- Lee, J.S.; Vescovo, E.; Arena, D.A.; Kao, C.C.; Beaujour, J.M.; Kent, A.D.; Jang, H.; Park, J.H.; Kim, J.Y. Longitudinal and transverse magnetization components in thin films: A resonant magnetic reflectivity investigation using circularly polarized soft X-rays. Appl. Phys. Lett. 2010, 96, 42507. [Google Scholar] [CrossRef]
- Tonnerre, J.M.; Przybylski, M.; Ragheb, M.; Yildiz, F.; Tolentino, H.C.N.; Ortega, L.; Kirschner, J. Direct in-depth determination of a complex magnetic configuration in an exchange-coupled bilayer with perpendicular and in-plane anisotropy. Phys. Rev. B—Condens. Matter Mater. Phys. 2011, 84, 100407. [Google Scholar] [CrossRef]
- Przybylski, M.; Tonnerre, J.M.; Yildiz, F.; Tolentino, H.C.N.; Kirschner, J. Non-collinear magnetic profile in (Rh/Fe1−xCox)2/Rh(001) bilayer probed by polarized soft X-ray resonant magnetic reflectivity. J. Appl. Phys. 2012, 111, 07C103. [Google Scholar] [CrossRef]
- Tonnerre, J.M.; De Santis, M.; Grenier, S.; Tolentino, H.C.N.; Langlais, V.; Bontempi, E.; García-Fernández, M.; Staub, U. Depth magnetization profile of a perpendicular exchange coupled system by soft-X-ray resonant magnetic reflectivity. Phys. Rev. Lett. 2008, 100, 157202. [Google Scholar] [CrossRef]
- Kortright, J.B.; Kim, S.K.; Denbeaux, G.P.; Zeltzer, G.; Takano, K.; Fullerton, E.E. Soft-X-ray small-angle scattering as a sensitive probe of magnetic and charge heterogeneity. Phys. Rev. B—Condens. Matter Mater. Phys. 2001, 64, 092401. [Google Scholar] [CrossRef]
- Jal, E.; Dąbrowski, M.; Tonnerre, J.M.; Przybylski, M.; Grenier, S.; Jaouen, N.; Kirschner, J. Magnetization profile across Au-covered bcc Fe films grown on a vicinal surface of Ag(001) as seen by X-ray resonant magnetic reflectivity. Phys. Rev. B—Condens. Matter Mater. Phys. 2013, 87, 224418. [Google Scholar] [CrossRef]
- Verna, A.; Bergenti, I.; Pasquali, L.; Giglia, A.; Albonetti, C.; Dediu, V.; Borgatti, F. Magnetic Depth Profiling of the Co/C60 Interface through Soft X-ray Resonant Magnetic Reflectivity. IEEE Trans. Magn. 2020, 56, 1–6. [Google Scholar] [CrossRef]
- Sperl, M.; MacCherozzi, F.; Borgatti, F.; Verna, A.; Rossi, G.; Soda, M.; Schuh, D.; Bayreuther, G.; Wegscheider, W.; Cezar, J.C.; et al. Identifying the character of ferromagnetic Mn in epitaxial Fe/(Ga,Mn)As heterostructures. Phys. Rev. B—Condens. Matter Mater. Phys. 2010, 81, 035211. [Google Scholar] [CrossRef]
- Golias, E.; Kumberg, I.; Gelen, I.; Thakur, S.; Gördes, J.; Hosseinifar, R.; Guillet, Q.; Dewhurst, J.K.; Sharma, S.; Schuler-Langeheine, C.; et al. Ultrafast Optically Induced Ferromagnetic State in an Elemental Antiferromagnet. Phys. Rev. Lett. 2021, 126, 107202. [Google Scholar] [CrossRef] [PubMed]
- Yamamoto, K.; El Moussaoui, S.; Hirata, Y.; Yamamoto, S.; Kubota, Y.; Owada, S.; Yabashi, M.; Seki, T.; Takanashi, K.; Matsuda, I.; et al. Element-selectively tracking ultrafast demagnetization process in Co/Pt multilayer thin films by the resonant magneto-optical Kerr effect. Appl. Phys. Lett. 2020, 116, 172406. [Google Scholar] [CrossRef]
- Gutt, C.; Sant, T.; Ksenzov, D.; Capotondi, F.; Pedersoli, E.; Raimondi, L.; Nikolov, I.P.; Kiskinova, M.; Jaiswal, S.; Jakob, G.; et al. Probing ultrafast changes of spin and charge density profiles with resonant XUV magnetic reflectivity at the free-electron laser FERMI. Struct. Dyn. 2017, 4, 55101. [Google Scholar] [CrossRef] [PubMed]
- Tsuyama, T.; Chakraverty, S.; Macke, S.; Pontius, N.; Schüßler-Langeheine, C.; Hwang, H.Y.; Tokura, Y.; Wadati, H. Photoinduced Demagnetization and Insulator-to-Metal Transition in Ferromagnetic Insulating BaFeO3 Thin Films. Phys. Rev. Lett. 2016, 116, 256402. [Google Scholar] [CrossRef] [PubMed]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Verna, A.; Capelli, R.; Pasquali, L. Resonant Soft X-ray Reflectivity in the Study of Magnetic Properties of Low-Dimensional Systems. Magnetochemistry 2021, 7, 136. https://doi.org/10.3390/magnetochemistry7100136
Verna A, Capelli R, Pasquali L. Resonant Soft X-ray Reflectivity in the Study of Magnetic Properties of Low-Dimensional Systems. Magnetochemistry. 2021; 7(10):136. https://doi.org/10.3390/magnetochemistry7100136
Chicago/Turabian StyleVerna, Adriano, Raffaella Capelli, and Luca Pasquali. 2021. "Resonant Soft X-ray Reflectivity in the Study of Magnetic Properties of Low-Dimensional Systems" Magnetochemistry 7, no. 10: 136. https://doi.org/10.3390/magnetochemistry7100136
APA StyleVerna, A., Capelli, R., & Pasquali, L. (2021). Resonant Soft X-ray Reflectivity in the Study of Magnetic Properties of Low-Dimensional Systems. Magnetochemistry, 7(10), 136. https://doi.org/10.3390/magnetochemistry7100136






