Organic Spintronics: A Theoretical Investigation of a Graphene-Porphyrin Based Nanodevice
Abstract
1. Introduction
2. Computational Details
3. FeP Molecular Junction between Graphene Electrodes
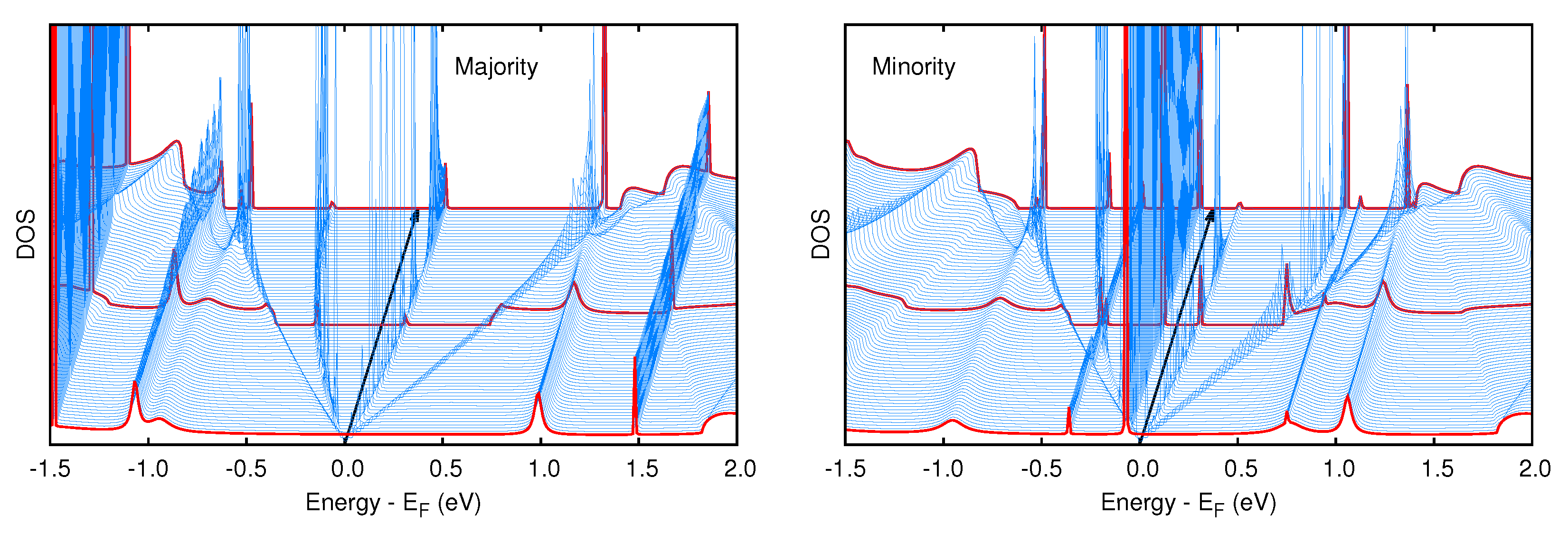
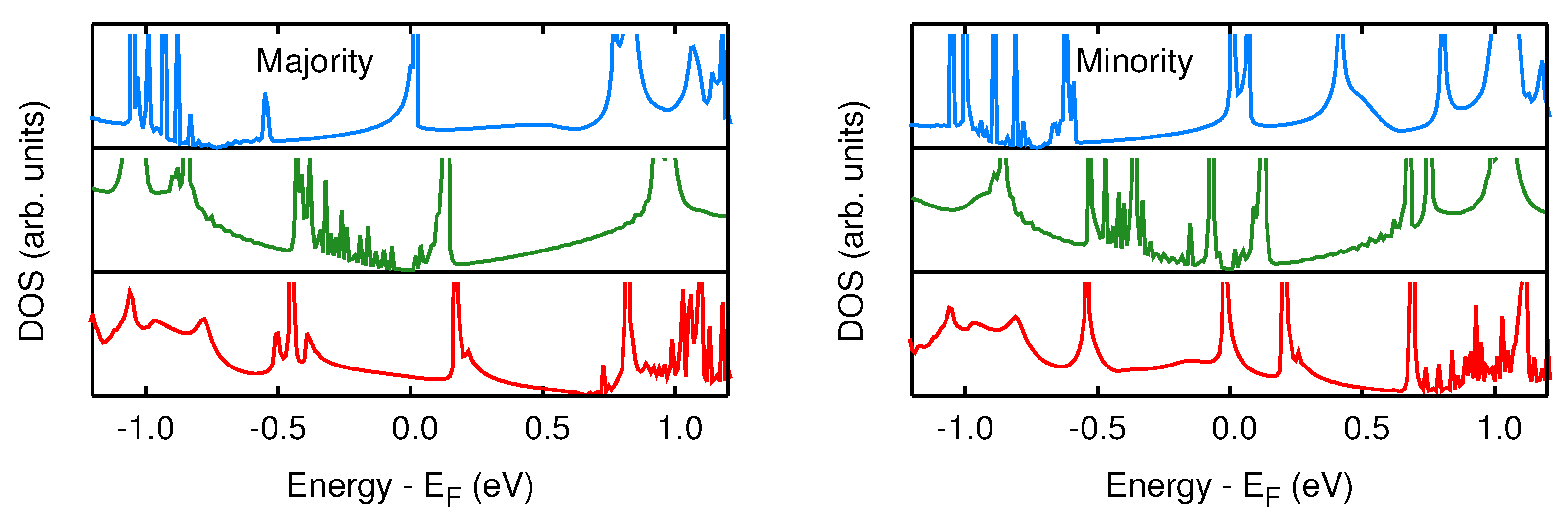
3.1. Spectral and Electronic Properties
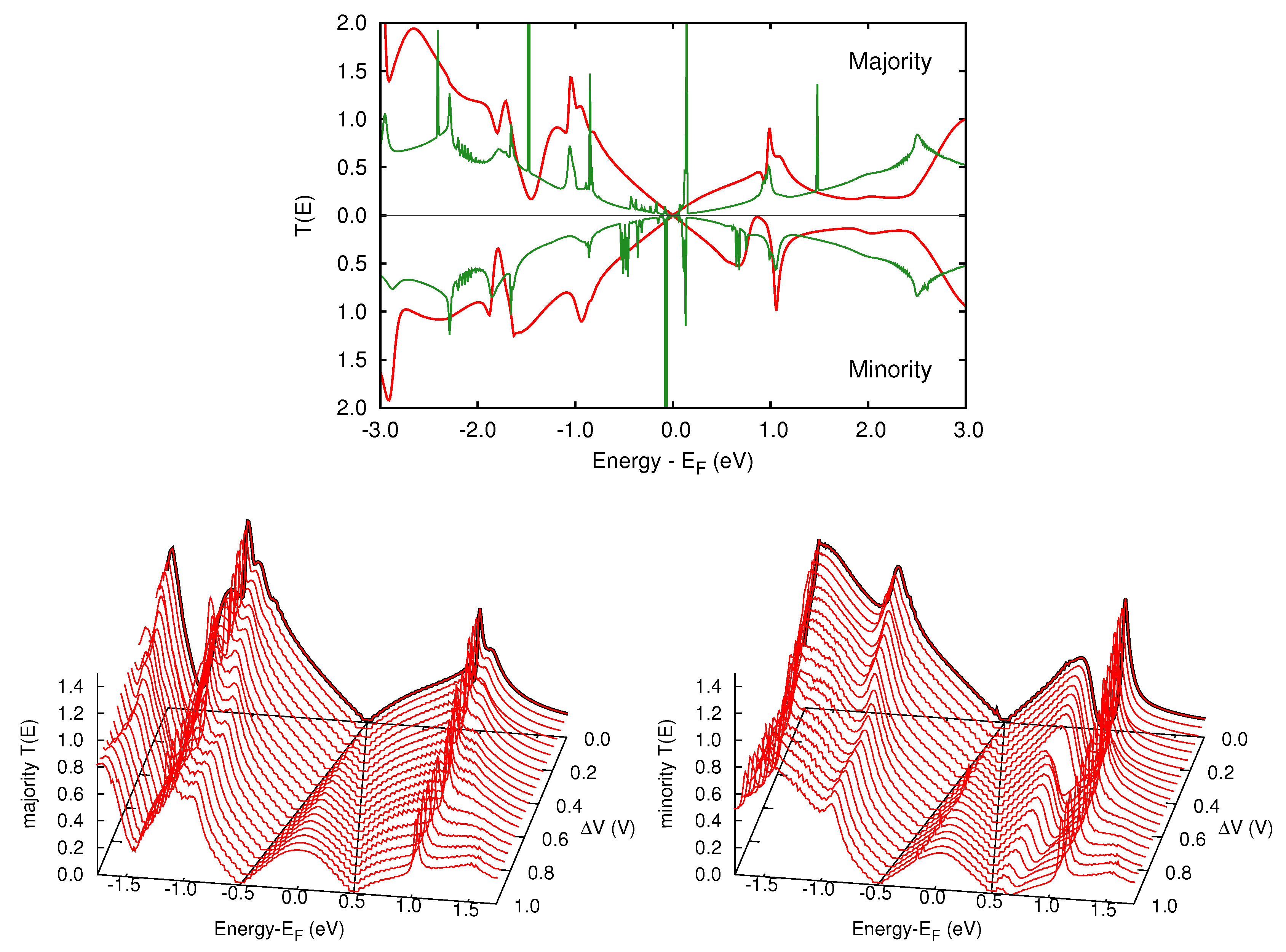
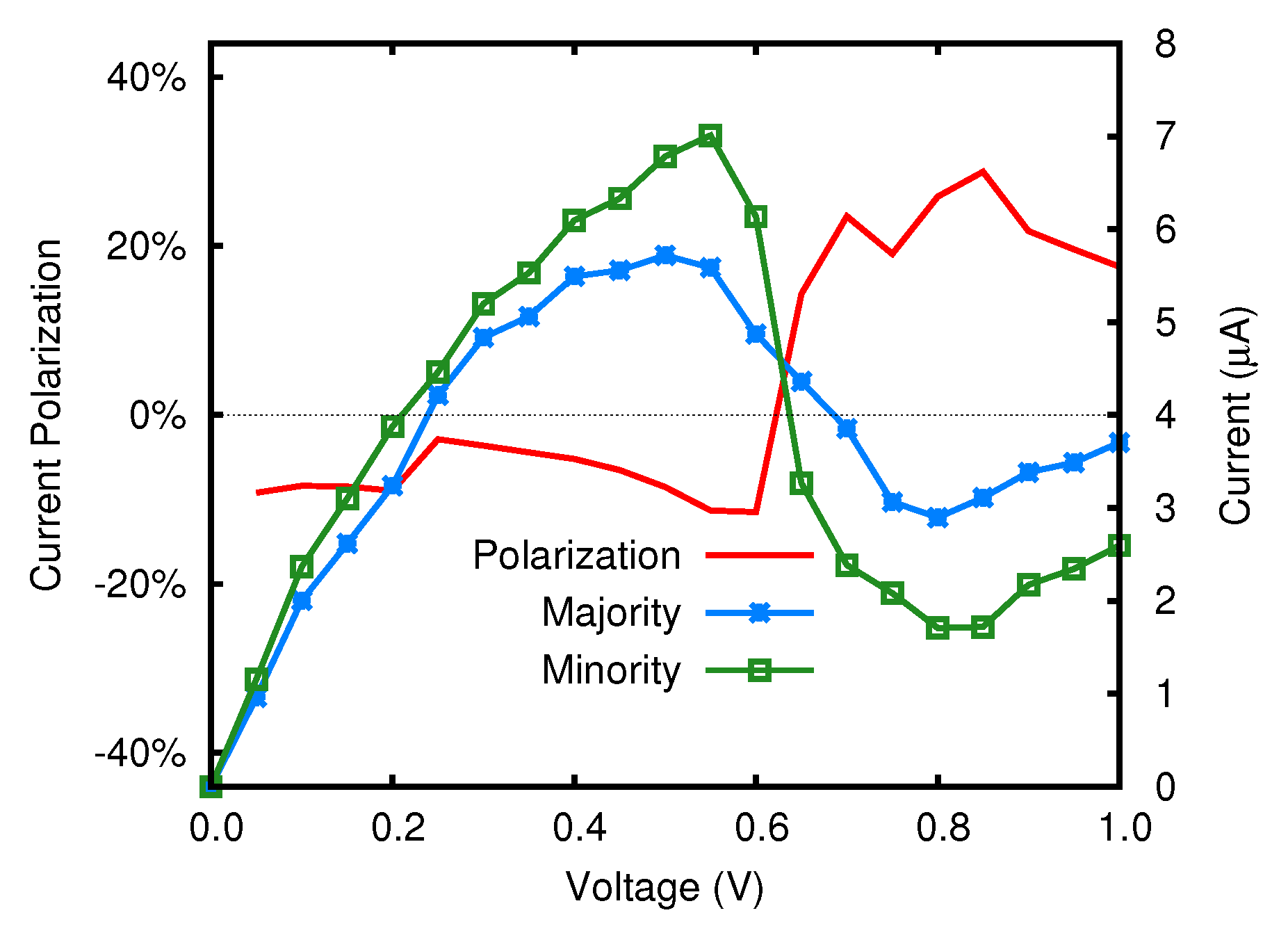
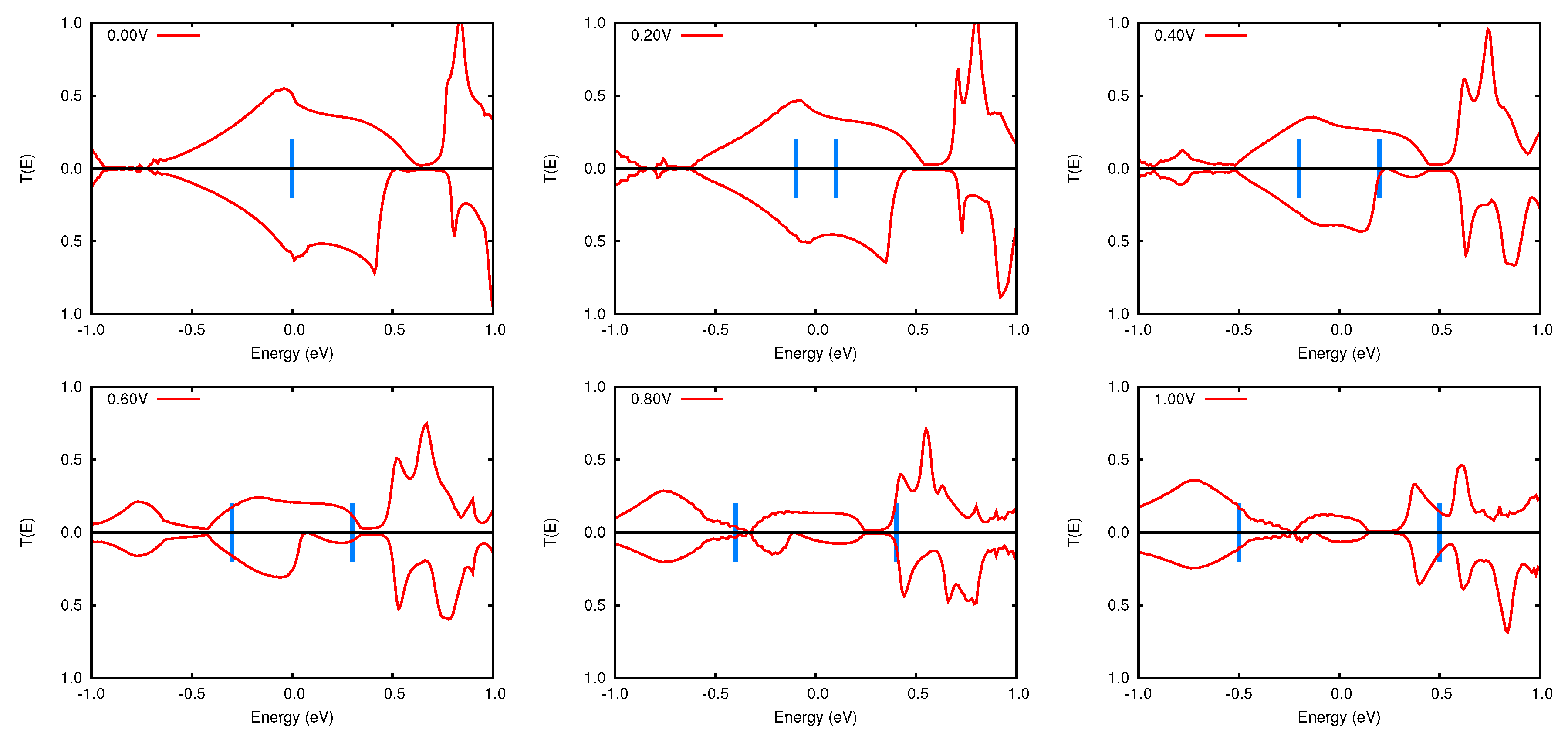
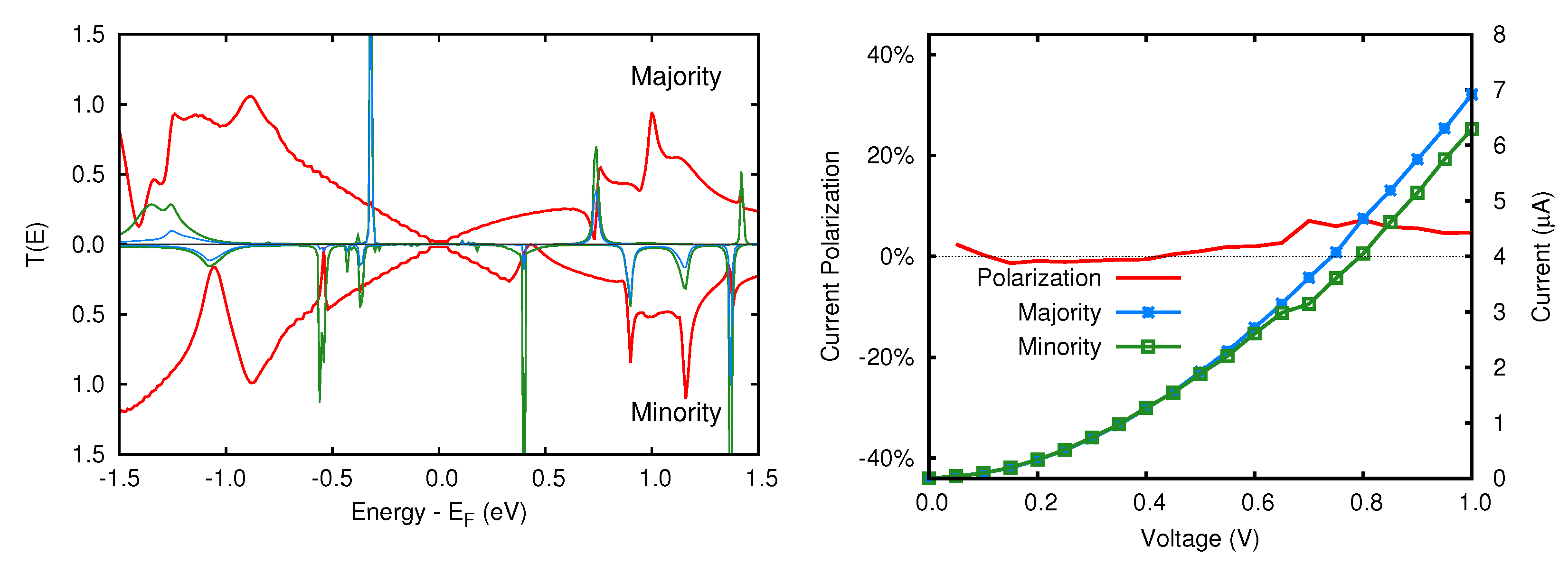
3.2. Transmission Properties
4. Effects of Doped Electrodes
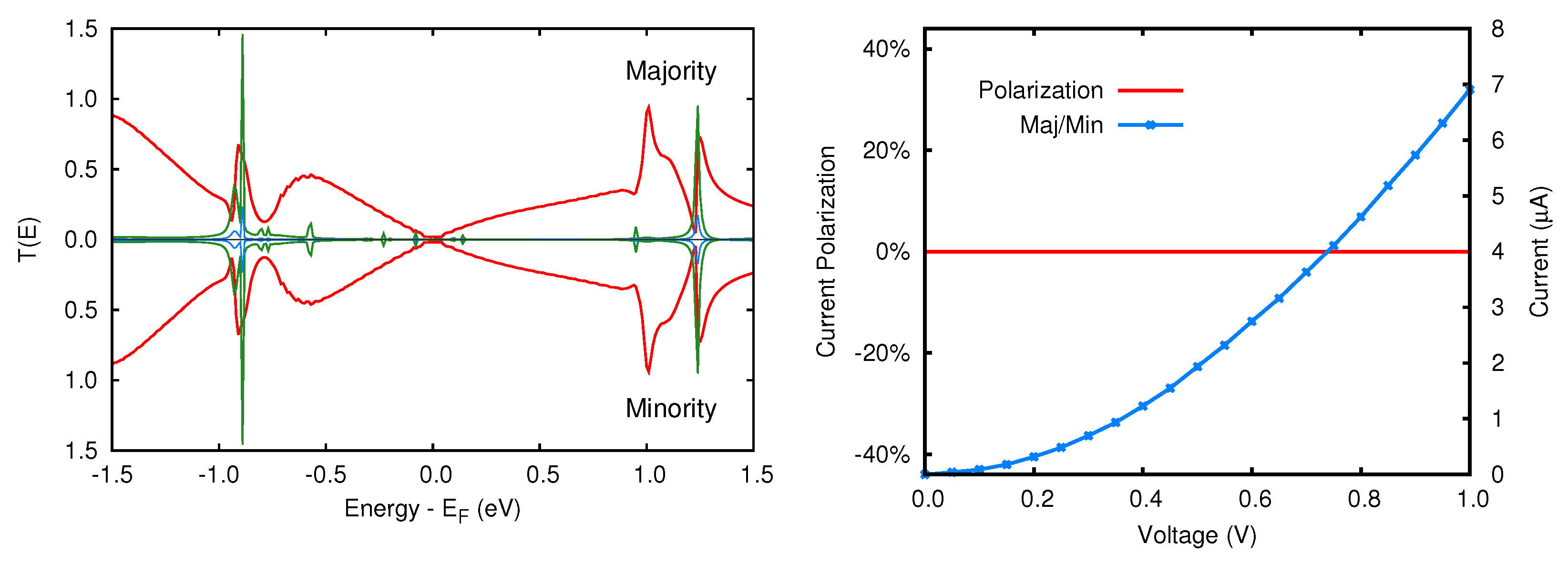
4.1. N-Doped System
4.2. B-Doped System
4.3. BN-Doped System
5. Gas Molecules Adsorption
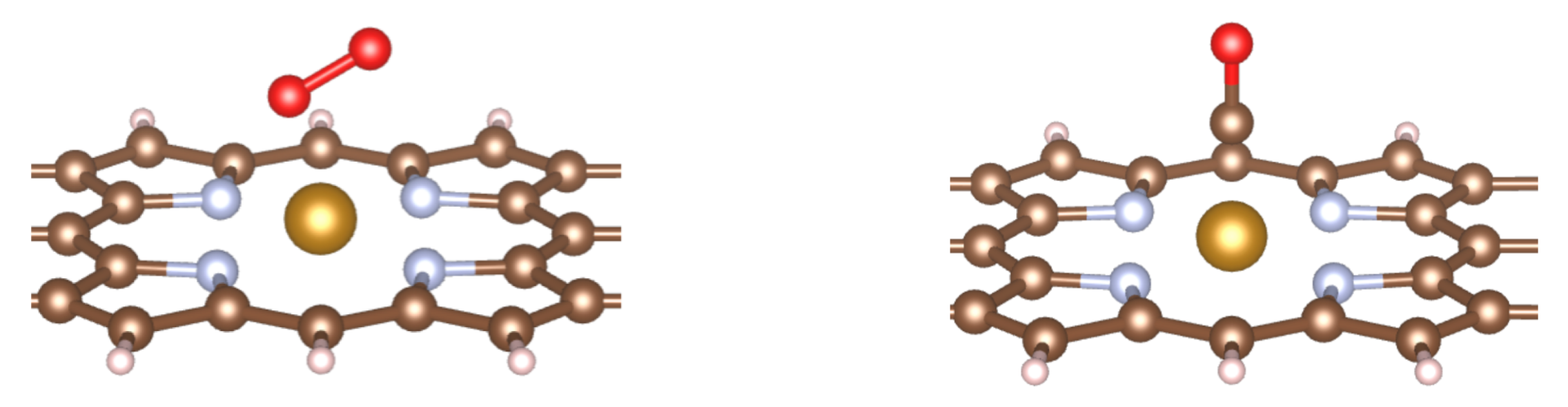
5.1. Geometric Structures
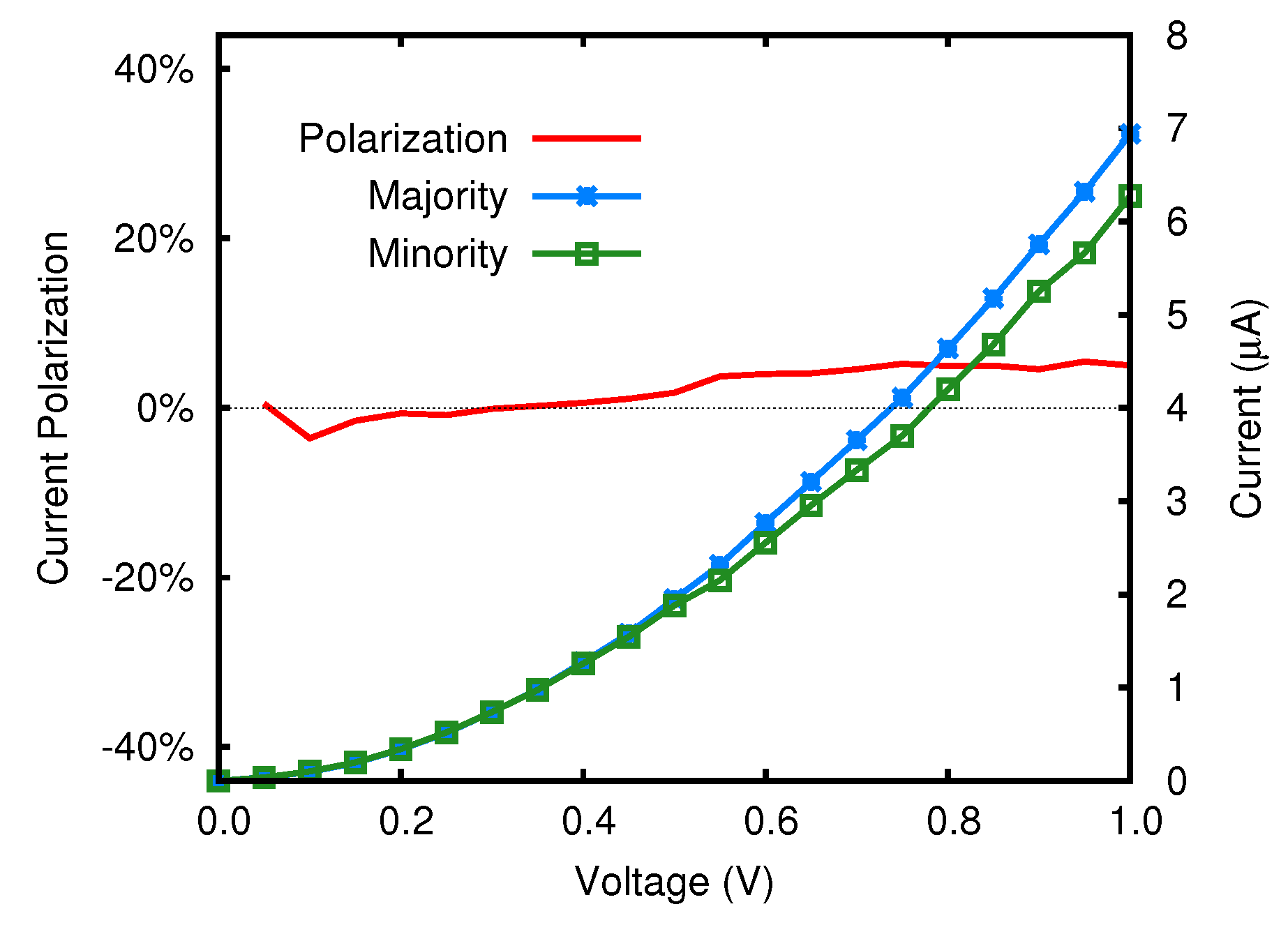
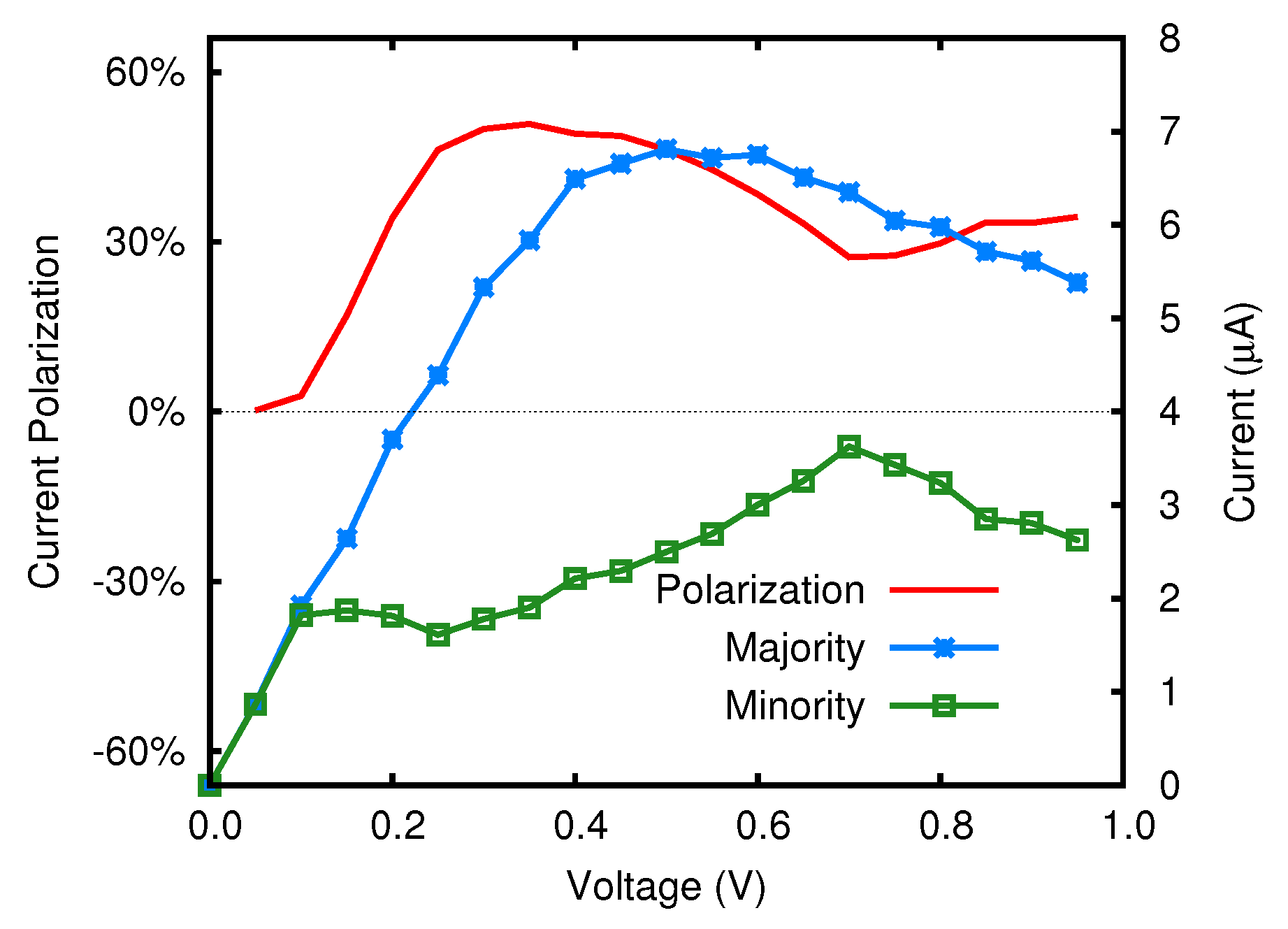
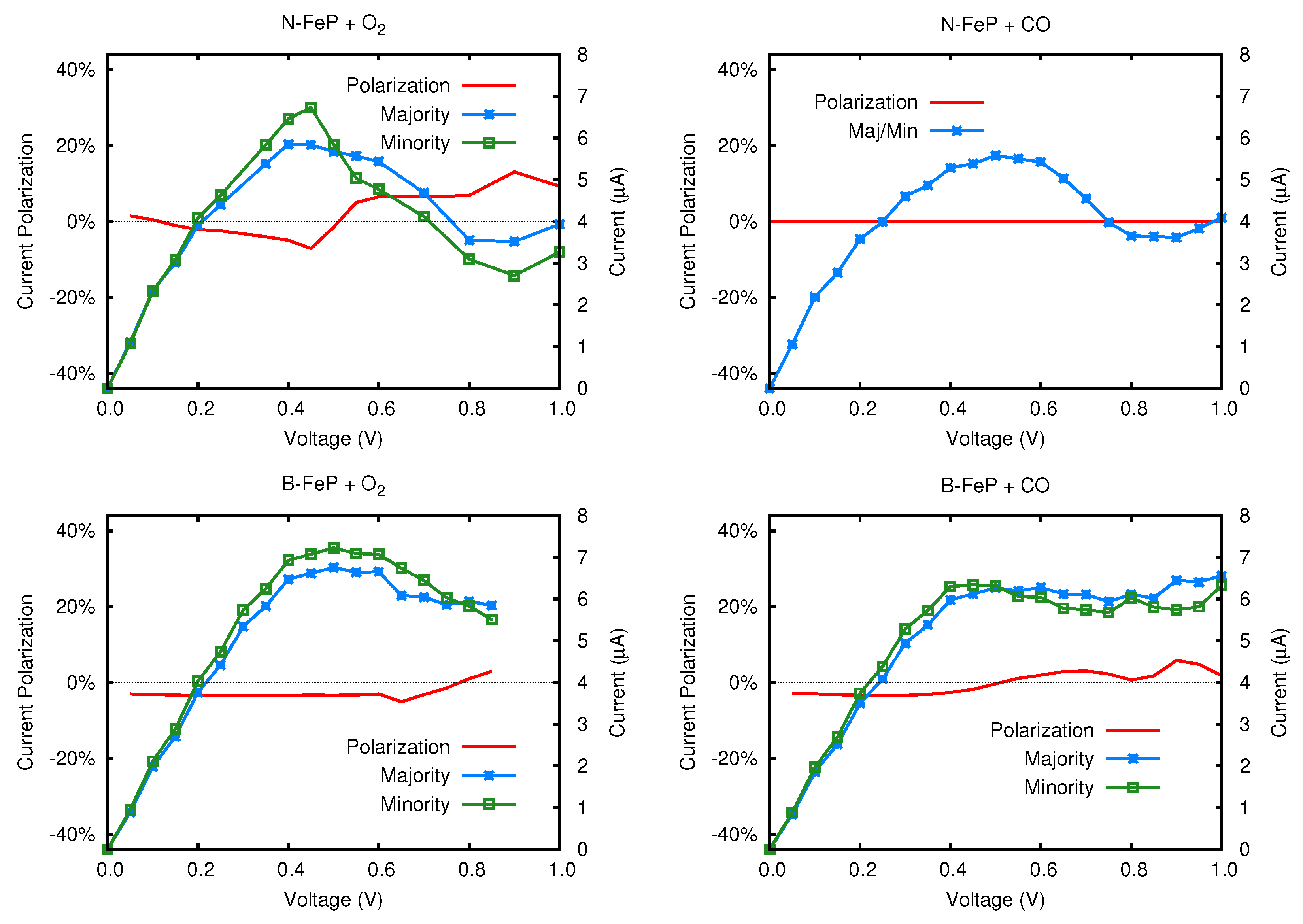
5.2. Transmission Function and Electronic Current
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References and Note
- Aviram, A.; Ratner, M.A. Molecular rectifiers. Chem. Phys. Lett. 1974, 29, 277. [Google Scholar] [CrossRef]
- Tao, N.J. Probing Potential-Tuned Resonant Tunneling through Redox Molecules with Scanning Tunneling Microscopy. Phys. Rev. Lett. 1996, 76, 4066. [Google Scholar] [CrossRef] [PubMed]
- Green, J.E.; Choi, J.W.; Boukai, A.; Bunimovich, Y.; Johnston-Halperin, E.; DeIonno, E.; Luo, Y.; Sheriff, B.A.; Xu, K.; Shin, Y.S.; et al. A 160-kilobit molecular electronic memory patterned at 1011 bits per square centimetre. Nature 2007, 445, 414. [Google Scholar] [CrossRef] [PubMed]
- Benesch, C.; Rode, M.F.; Čížek, M.; Härtle, R.; Rubio-Pons, O.; Thoss, M.; Sobolewski, A.L. Switching the Conductance of a Single Molecule by Photoinduced Hydrogen Transfer. J. Phys. Chem. C 2009, 113, 10315. [Google Scholar] [CrossRef]
- Li, Y.; Yang, C.; Guo, X. Single-Molecule Electrical Detection: A Promising Route toward the Fundamental Limits of Chemistry and Life Science. Acc. Chem. Res. 2020, 53, 159. [Google Scholar] [CrossRef] [PubMed]
- Rocha, A.R.; Garcia-Suarez, V.M.; Bailey, S.W.; Lambert, C.J.; Ferrer, J.; Sanvito, S. Towards molecular spintronics. Nat. Mater. 2005, 5, 335. [Google Scholar] [CrossRef]
- Liu, X.; Wang, T.; Niu, L.; Wang, Y.; Zhang, Q. Magnetic proximity, magnetoresistance and spin filtering effect in a binuclear ferric phthalocyanine from first principles. J. Phys. D Appl. Phys. 2020, 53, 035305. [Google Scholar] [CrossRef]
- Sanvito, S. Spintronics goes plastic. Nat. Mater. 2007, 6, 803. [Google Scholar] [CrossRef]
- Szulczewski, G.; Sanvito, S.; Coey, M. A spin of their own. Nat. Mater. 2009, 8, 693. [Google Scholar] [CrossRef]
- Sanvito, S. Molecular spintronics. Chem. Soc. Rev. 2011, 40, 3336. [Google Scholar] [CrossRef]
- Lee, S.U.; Belosludov, R.V.; Mizuseki, H.; Kawazoe, Y. The Role of Aromaticity and the π-Conjugated Framework in Multiporphyrinic Systems as Single-Molecule Switches. Small 2008, 7, 962. [Google Scholar] [CrossRef] [PubMed]
- Wang, N.; Liu, H.; Zhao, J.; Cui, Y.; Xu, Z.; Ye, Y.; Kiguchi, M.; Murakoshi, K. Theoretical investigation on the electron transport path through the Porphyrin molecules and chemisorption of CO. J. Phys. Chem. C 2009, 113, 7416. [Google Scholar] [CrossRef]
- Li, Y.W.; Yao, J.H.; Liu, C.J.; Yang, J.W.; Yang, C.L. Theoretical investigation of the O2 adsorption effect in the electron transport of single Fe-porphyrin molecule. Phys. Lett. A 2009, 373, 3974. [Google Scholar] [CrossRef]
- Kondo, H.; Nara, J.; Ohno, T. Possibility of gas sensor using electronic transport properties of iron-porphyrin molecular junction system. J. Phys. Chem. C 2011, 115, 6886. [Google Scholar] [CrossRef]
- Toyoda, K. Theoretical investigation of chemical spin doping into single porphyrin junctions toward ultrahigh-sensitive nitric oxide sensor. Jpn. J. Appl. Phys. 2012, 51, 045202. [Google Scholar] [CrossRef]
- Huang, J.; Wang, W.; Yang, S.; Su, H.; Li, Q.; Yang, J. A theoretical study of spin-polarized transport properties of planar four-coordinate Fe complexes. Chem. Phys. Lett. 2012, 539, 102. [Google Scholar] [CrossRef]
- Huang, J.; Xu, K.; Lei, S.; Su, H.; Yang, S.; Li, Q.; Yang, J. Iron-phthalocyanine molecular junction with high spin filter efficiency and negative differential resistance. J. Chem. Phys. 2012, 136, 064707. [Google Scholar] [CrossRef]
- Huang, J.; Wang, W.; Yang, S.; Li, Q.; Yang, J. Efficient spin filter based on FeN4 complexes between carbon nanotube electrodes. Nanotechnology 2012, 23, 255202. [Google Scholar] [CrossRef]
- Lörtscher, E. Wiring molecules into circuits. Nat. Nanotech. 2013, 8, 381. [Google Scholar] [CrossRef]
- Bruot, C.; Hihath, J.; Tao, N. Mechanically controlled molecular orbital alignment in single molecule junctions. Nat. Nanotech. 2011, 7, 35. [Google Scholar] [CrossRef]
- Liang, W.; Shores, M.O.; Bockrath, M.; Long, J.R.; Park, H. Kondo resonance in a single-molecule transistor. Nature 2002, 417, 725. [Google Scholar] [CrossRef] [PubMed]
- Guédon, C.M.; Valkenier, H.; Markussen, T.; Thygesen, K.S.; Hummelen, J.C.; van der Molen, S.J. Observation of quantum interference in molecular charge transport. Nat. Nanotech. 2012, 7, 305. [Google Scholar] [CrossRef] [PubMed]
- Metzger, R.M.; Chen, B.; Höpfner, U.; Lakshmikantham, M.V.; Vuillaume, D.; Kawai, T.; Wu, X.; Tachibana, H.; Hughes, T.V.; Sakurai, H.; et al. Unimolecular Electrical Rectification in Hexadecylquinolinium Tricyanoquinodimethanide. J. Am. Chem. Soc. 1997, 119, 10455. [Google Scholar] [CrossRef]
- Kubatkin, S.; Danilov, A.; Hjort, M.; Cornil, J.; Brédas, J.L.; Stuhr-Hansen, N.; Hedegard, P.; Bjornholm, T. Single-electron transistor of a single organic molecule with access to several redox states. Nature 2003, 425, 698. [Google Scholar] [CrossRef] [PubMed]
- Quek, S.Y.; Kamenetska, M.; Steigerwald, M.L.; Choi, H.J.; Louie, S.G.; Hybertsen, M.S.; Neaton, J.B.; Venkataraman, L. Mechanically-Controlled Binary Conductance Switching of a Single-Molecule Junction. Nat. Nanotech. 2009, 4, 230. [Google Scholar] [CrossRef] [PubMed]
- Thiele, S.; Balestro, F.; Ballou, R.; Klyatskaya, S.; Ruben, M.; Wernsdorfer, W. Electrically driven nuclear spin resonance in single-molecule magnets. Science 2014, 344, 1135. [Google Scholar] [CrossRef]
- Nazin, G.V.; Qiu, X.H.; Ho, W. Visualization and Spectroscopy of a Metal-Molecule-Metal Bridge. Science 2003, 302, 77. [Google Scholar] [CrossRef]
- He, Y.; Garnica, M.; Bischoff, F.; Ducke, J.; Bocquet, M.L.; Batzill, M.; Auwärter, W.; Barth, J.V. Fusing tetrapyrroles to graphene edges by surface-assisted covalent coupling. Nat. Chem. 2017, 9, 33. [Google Scholar] [CrossRef]
- Zeng, J.; Chen, K.Q. A nearly perfect spin filter and a spin logic gate based on a porphyrin/graphene hybrid material. Phys. Chem. Chem. Phys. 2018, 20, 3997. [Google Scholar] [CrossRef]
- Dayen, J.F.; Ray, S.J.; Karis, O.; Vera-Marun, I.J.; Kamalakar, M.V. Two-dimensional van der Waals spinterfaces and magnetic-interfaces. App. Phys. Rev. 2020, 7, 011303. [Google Scholar] [CrossRef]
- Han, W.; Kawakami, R.K.; Gmitra, M.; Fabian, J. Graphene spintronics. Nat. Nanotech. 2014, 9, 794. [Google Scholar] [CrossRef] [PubMed]
- Huang, J.; Wan, Q. Gas Sensors Based on Semiconducting Metal Oxide One-Dimensional Nanostructures. Sensors 2009, 9, 9903. [Google Scholar] [CrossRef] [PubMed]
- Kim, H.W.; Kwon, Y.J.; Mirzaei, A.; Kang, S.Y.; Choi, M.S.; Bang, J.H.; Kim, S.S. Synthesis of zinc oxide semiconductors-graphene nanocomposites by microwave irradiation for application to gas sensors. Sensor Actuators B Chem. 2017, 249, 590–601. [Google Scholar] [CrossRef]
- Chen, H.; Bo, R.; Shrestha, A.; Xin, B.; Nasiri, N.; Zhou, J.; Di Bernardo, I.; Dodd, A.; Saunders, M.; Lipton-Duffin, J.; et al. NiO–ZnO Nanoheterojunction Networks for Room-Temperature Volatile Organic Compounds Sensing. Adv. Opt. Mater. 2018, 6, 1800677. [Google Scholar] [CrossRef]
- Yang, J.; Liu, J.; Xu, Y.; Li, X.; Wu, J.; Han, Y.; Wang, Z.; Zhang, X. Enhanced selective acetone-sensing performance of hierarchical hollow SnO2/a-Fe2O3 microcubes. J. Mater. Chem. C 2019, 7, 11984. [Google Scholar] [CrossRef]
- Hittini, W.; Abu-Hani, A.F.; Reddy, N.; Mahmoud, S.T. Cellulose-copper oxide hybrid nanocomposites membranes for H2S gas detection at low temperatures. Sci. Rep. 2020, 10, 2940. [Google Scholar] [CrossRef]
- Park, J.; Pasupathy, A.N.; Goldsmith, J.I.; Chang, C.; Yaish, Y.; Petta, J.R.; Rinkoski, M.; Sethna, J.P.; Abruña, H.D.; McEuen, P.L.; et al. Coulomb blockade and the Kondo effect in single-atom transistors. Nature 2002, 417, 722. [Google Scholar] [CrossRef]
- Chen, Y.; Prociuk, A.; Perrine, T.; Dunietz, B.D. Spin-dependent electronic transport through a porphyrin ring ligating an FeII atom: An ab initio study. Phys. Rev. B 2006, 74, 245320. [Google Scholar] [CrossRef]
- Okuno, Y.; Yokoyama, S. Theoretical study of molecular rectification in porphyrin dimer. Thin Solid Films 2008, 516, 2630. [Google Scholar] [CrossRef]
- Salazar-Salinas, K.; Jauregui, L.A.; Kubli-Garfias, C.; Seminario, J.M. Molecular biosensor based on a coordinated iron complex. J. Chem. Phys. 2009, 130, 105101. [Google Scholar] [CrossRef] [PubMed]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized gradient approximation made simple. Phys. Rev. Lett. 1996, 77, 3865. [Google Scholar] [CrossRef] [PubMed]
- Troullier, N.; Martins, J.L. Efficient pseudopotentials for plane-wave calculations. Phys. Rev. B 1991, 43, 1993. [Google Scholar] [CrossRef] [PubMed]
- Soler, J.M.; Artacho, E.; Gale, J.D.; García, A.; Junquera, J.; Ordejón, P.; Sánchez-Portal, D. The SIESTA method for ab initio order-N materials simulations. J. Phys. Condens. Matter 2002, 14, 2745. [Google Scholar] [CrossRef]
- Landauer, R. Spatial Variation of Currents and Fields Due to Localized Scatterers in Metallic Conduction. IBM J. Res. Dev. 1957, 1, 233. [Google Scholar] [CrossRef]
- Landauer, R. Electrical resistance of disordered one-dimensional lattices. Phil. Mag. 1970, 21, 863. [Google Scholar] [CrossRef]
- Boumrar, H.; Hamidi, M.; Zenia, H.; Lounis, S. Equivalence of wave function matching and Green’s functions methods for quantum transport: Generalized Fisher-Lee relation. J. Phys. Cond. Matter 2020. [Google Scholar] [CrossRef]
- Keldysh, L.V. Diagram technique for nonequilibrium processes. Sov. Phys. JETP 1965, 20, 1018. [Google Scholar]
- Caroli, C.; Combescot, R.; Nozieres, P.P.; Saint-James, D. Direct calculation of the tunneling current. J. Phys. C Solid State Phys. 1971, 4, 916. [Google Scholar] [CrossRef]
- Brandbyge, M.; Mozos, J.L.; Ordejón, P.; Taylor, J.; Stokbro, K. Density-Functional Method for Nonequilibrium Electron Transport. Phys. Rev. B 2002, 65, 165401. [Google Scholar] [CrossRef]
- Papior, N.; Lorente, N.; Frederiksen, T.; García, A.; Brandbyge, M. Improvements on non-equilibrium and transport Green function techniques: The next-generation transiesta. Comput. Phys. Commun. 2017, 212, 8. [Google Scholar] [CrossRef]
- Fisher, D.S.; Lee, P.A. Relation between conductivity and transmission matrix. Phys. Rev. B 1981, 23, 6851. [Google Scholar] [CrossRef]
- Sanvito, S.; Lambert, C.J.; Jefferson, J.H.; Bratkovsky, A.M. General Green’s-function formalism for transport calculations with spd Hamiltonians and giant magnetoresistance in Co- and Ni-based magnetic multilayers. Phys. Rev. B 1999, 59, 11936. [Google Scholar] [CrossRef]
- Levy Yeyati, A.; Buttiker, M. Scattering phases in quantum dots: An analysis based on lattice models. Phys. Rev. B 2000, 62, 7307. [Google Scholar] [CrossRef]
- Paulsson, M. Non Equilibrium Green’s Functions for Dummies: Introduction to the One Particle NEGF equations. arXiv 2008, arXiv:cond-mat/0210519. [Google Scholar]
- For the calculation of the non-equilibrium Green’s function we used an integration path in the complex-energy plane constituted by 38 points for the Gaussian quadrature, 32 energy point near the real axis (ℑm(E) = 10−5 eV) in the bias windows and 16 poles on the imaginary axis of the energy. To work-out the values of the electron current we used a finer k-point mesh equal to 50 points in the 1D Brillouin zone.
- Guo, X.; Small, J.P.; Klare, J.E.; Wang, Y.; Purewal, M.S.; Tam, I.M.; Hong, B.H.; Caldwell, R.; Huang, L.; O’Brien, S.; et al. Covalently Bridging Gaps in Single-Walled Carbon Nanotubes with Conducting Molecules. Science 2006, 311, 356. [Google Scholar] [CrossRef] [PubMed]
- Marquardt, C.W.; Grunder, S.; Baszczyk, A.; Dehm, S.; Hennrich, F.; von Löhneysen, H.; Mayor, M.; Krupke, R. Electroluminescence from a single nanotube-molecule-nanotube junction. Nat. Nanotech. 2010, 5, 863. [Google Scholar] [CrossRef]
- Prins, F.; Barreiro, A.; Ruitenberg, J.W.; Seldenthuis, J.S.; Aliaga-Alcalde, N.; Vandersypen, L.M.K.; van der Zant, H.S.J. Room-Temperature Gating of Molecular Junctions Using Few-Layer Graphene Nanogap Electrodes. Nano Lett. 2011, 11, 4607. [Google Scholar] [CrossRef]
- Lee, D.H.; Lee, W.J.; Lee, W.J.; Kim, S.O.; Kim, Y.H. Theory, synthesis and oxygen reduction catalysis of Fe-porphyrin-like carbon nanotube. Phys. Rev. Lett. 2011, 106, 175502. [Google Scholar] [CrossRef]
- Liao, M.S.; Watts, J.D.; Huang, M.J. FeII in Different Macrocycles: Electronic Structures and Properties. J. Phys. Chem. A 2005, 109, 7988. [Google Scholar] [CrossRef]
- Wassmann, T.; Seitsonen, A.P.; Saitta, A.M.; Lazzeri, M.; Mauri, F. Structure, Stability, Edge States, and Aromaticity of Graphene Ribbons. Phys. Rev. Lett. 2008, 101, 096402. [Google Scholar] [CrossRef]
- Ijäs, M.; Ervasti, M.; Uppstu, A.; Liljeroth, P.; van der Lit, J.; Swart, I.; Harju, A. Electronic states in finite graphene nanoribbons: Effect of charging and defects. Phys. Rev. B 2013, 88, 075429. [Google Scholar] [CrossRef]
- Yamanaka, A.; Okada, S. Energetics and electronic structure of graphene nanoribbons under a lateral electric field. Carbon 2016, 96, 351. [Google Scholar] [CrossRef]
- del Castillo, E.; Cargnoni, F.; Achilli, S.; Tantardini, G.; Trioni, M.I. Spin asymmetric band gap opening in graphene by Fe adsorption. Surf. Sci. 2015, 634, 62–67. [Google Scholar] [CrossRef]
- del Castillo, E.; Cargnoni, F.; Soave, R.; Trioni, M.I. Spin-polarized charge transfer induced by transition metal adsorption on graphene. Phys. Scripta 2016, 91, 1–7. [Google Scholar] [CrossRef]
- del Castillo, E.; Achilli, S.; Cargnoni, F.; Ceresoli, D.; Soave, R.; Trioni, M.I. Spin-filtering in graphene junctions with Ti and Co adsorbates. Chem. Phys. 2016, 478, 91–96. [Google Scholar] [CrossRef]
- Panchakarla, L.S.; Subrahmanyam, K.S.; Saha, S.K.; Govindaraj, A.; Krishnamurthy, H.R.; Waghmare, U.V.; Rao, C.N.R. Synthesis, Structure, and Properties of Boron- and Nitrogen-Doped Graphene. Adv. Mater. 2009, 21, 4726. [Google Scholar] [CrossRef]
- Lherbier, A.; Blase, X.; Niquet, Y.M.; Triozon, F.; Roche, S. Charge Transport in Chemically Doped 2D Graphene. Phys. Rev. Lett. 2008, 101, 036808. [Google Scholar] [CrossRef]
- Lin, Y.C.; Lin, C.Y.; Chiu, P.W. Controllable graphene N-doping with ammonia plasma. Appl. Phys. Lett. 2010, 96, 133110. [Google Scholar] [CrossRef]
- Rovira, C.; Ballone, P.; Parrinello, M. A density functional study of iron-porphyrin complexes. Chem. Phys. Lett. 1997, 271, 247. [Google Scholar] [CrossRef]
- Momenteau, M.; Reed, C.A. Synthetic Heme Dioxygen Complexes. Chem. Rev. 1994, 94, 659. [Google Scholar] [CrossRef]
- Fano, U. Effects of Configuration Interaction on Intensities and Phase Shifts. Phys. Rev. 1961, 124, 1866–1878. [Google Scholar] [CrossRef]
- Nöckel, J.U.; Stone, A.D. Resonance line shapes in quasi-one-dimensional scattering. Phys. Rev. B 1994, 50, 17415–17432. [Google Scholar] [CrossRef] [PubMed]
- Datta, S. Electronic Transport in Mesoscopic Systems; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]




© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
del Castillo, E.; Cargnoni, F.; Soave, R.; Trioni, M.I. Organic Spintronics: A Theoretical Investigation of a Graphene-Porphyrin Based Nanodevice. Magnetochemistry 2020, 6, 27. https://doi.org/10.3390/magnetochemistry6020027
del Castillo E, Cargnoni F, Soave R, Trioni MI. Organic Spintronics: A Theoretical Investigation of a Graphene-Porphyrin Based Nanodevice. Magnetochemistry. 2020; 6(2):27. https://doi.org/10.3390/magnetochemistry6020027
Chicago/Turabian Styledel Castillo, Elisabetta, Fausto Cargnoni, Raffaella Soave, and Mario Italo Trioni. 2020. "Organic Spintronics: A Theoretical Investigation of a Graphene-Porphyrin Based Nanodevice" Magnetochemistry 6, no. 2: 27. https://doi.org/10.3390/magnetochemistry6020027
APA Styledel Castillo, E., Cargnoni, F., Soave, R., & Trioni, M. I. (2020). Organic Spintronics: A Theoretical Investigation of a Graphene-Porphyrin Based Nanodevice. Magnetochemistry, 6(2), 27. https://doi.org/10.3390/magnetochemistry6020027




