Structural Descriptors of Benzenoid Hydrocarbons: A Mismatch between the Estimates and Parity Effects in Helicenes
Abstract
:1. Introduction
2. Computational Details
2.1. Information Entropy Calculations
2.2. Calculations of Topologial Descriptors
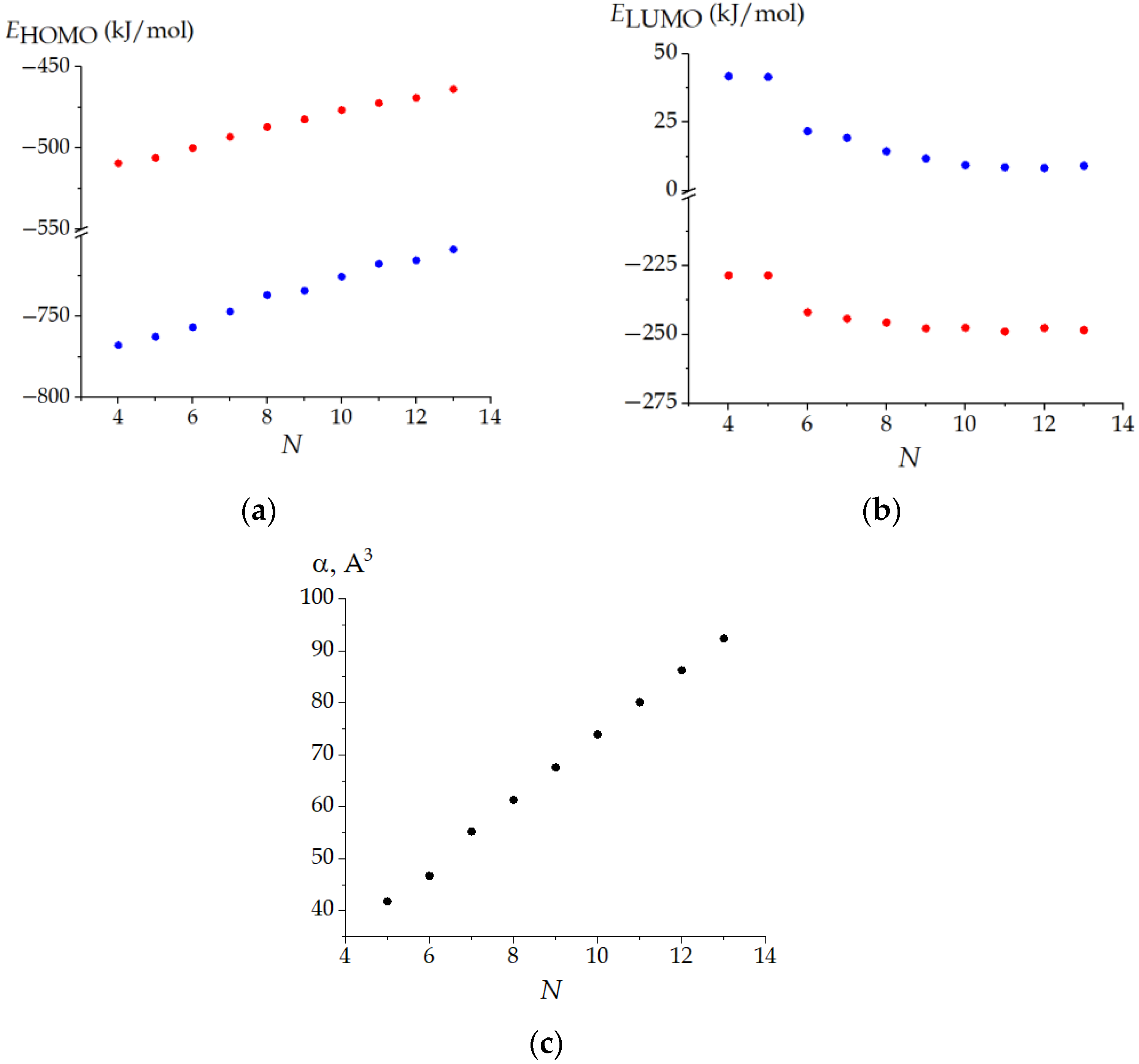
2.3. Auxiliary Quantum Chemical Calculations
3. Results
3.1. Symmetry Trends in the Series of Regular Benzenoid PAHs
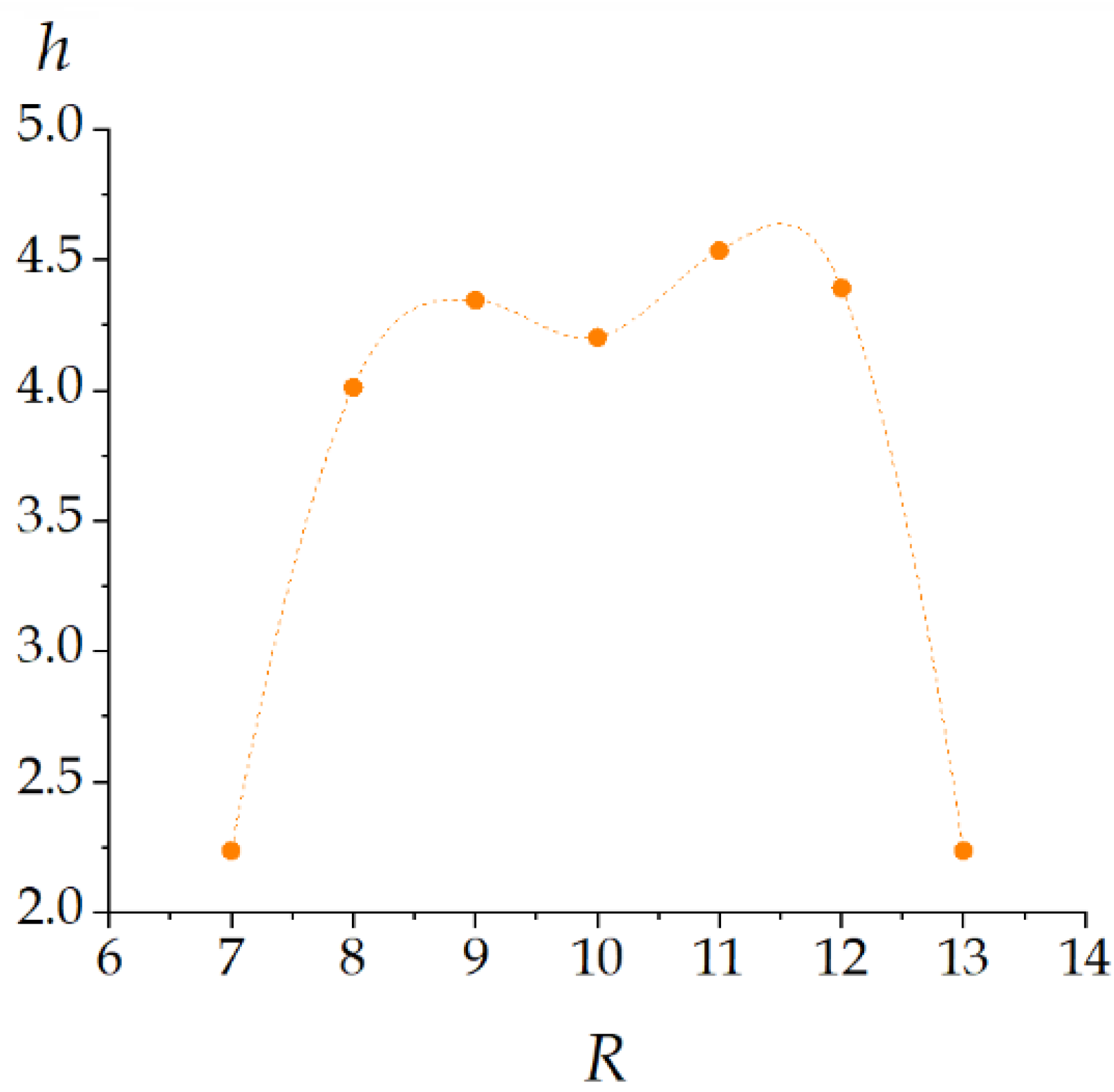
3.2. Information Entropy in the Series of Regular Benzenoid PAHs
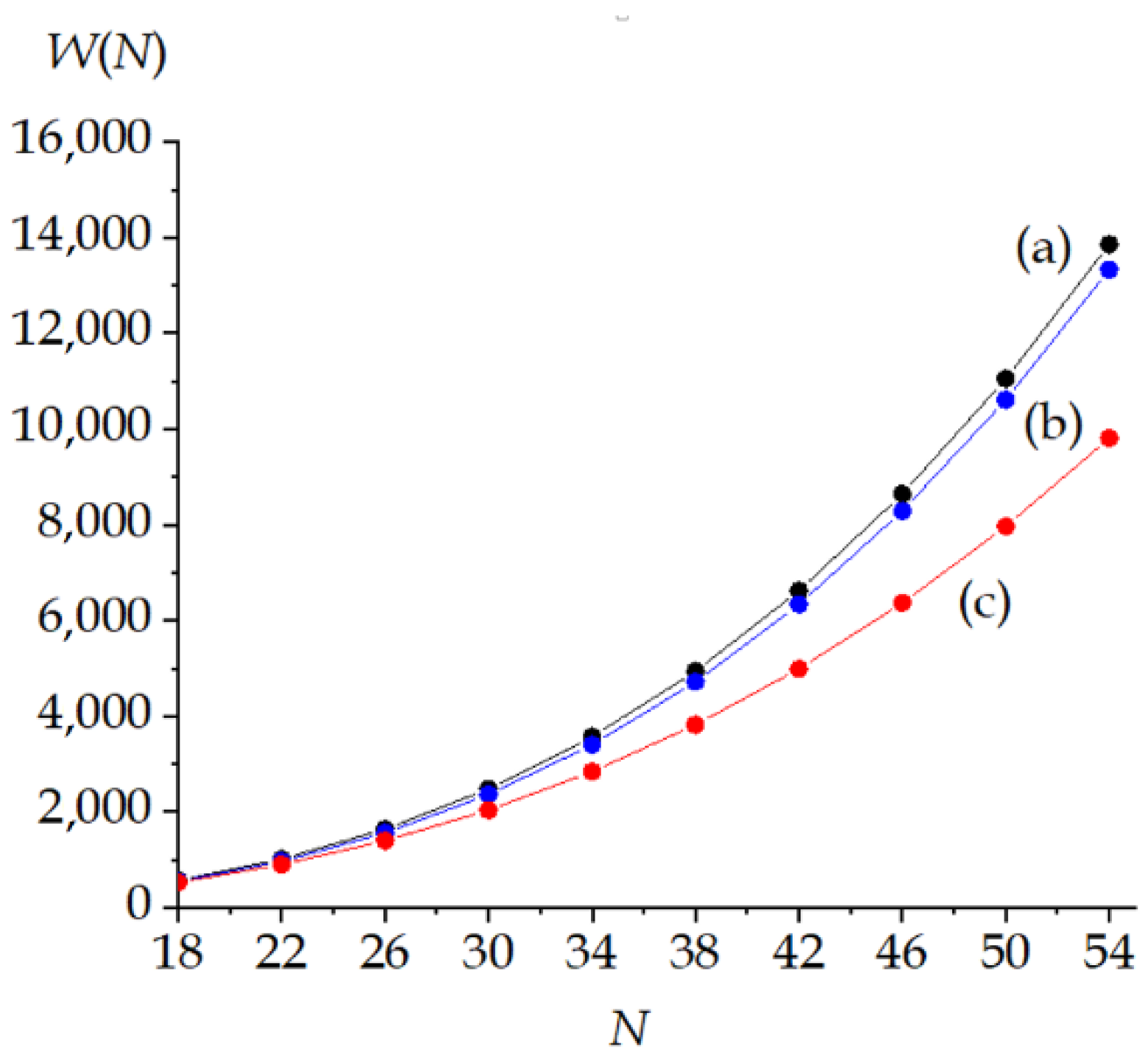
3.3. Topological Indices in the Series of Regular Benzenoid PAHs
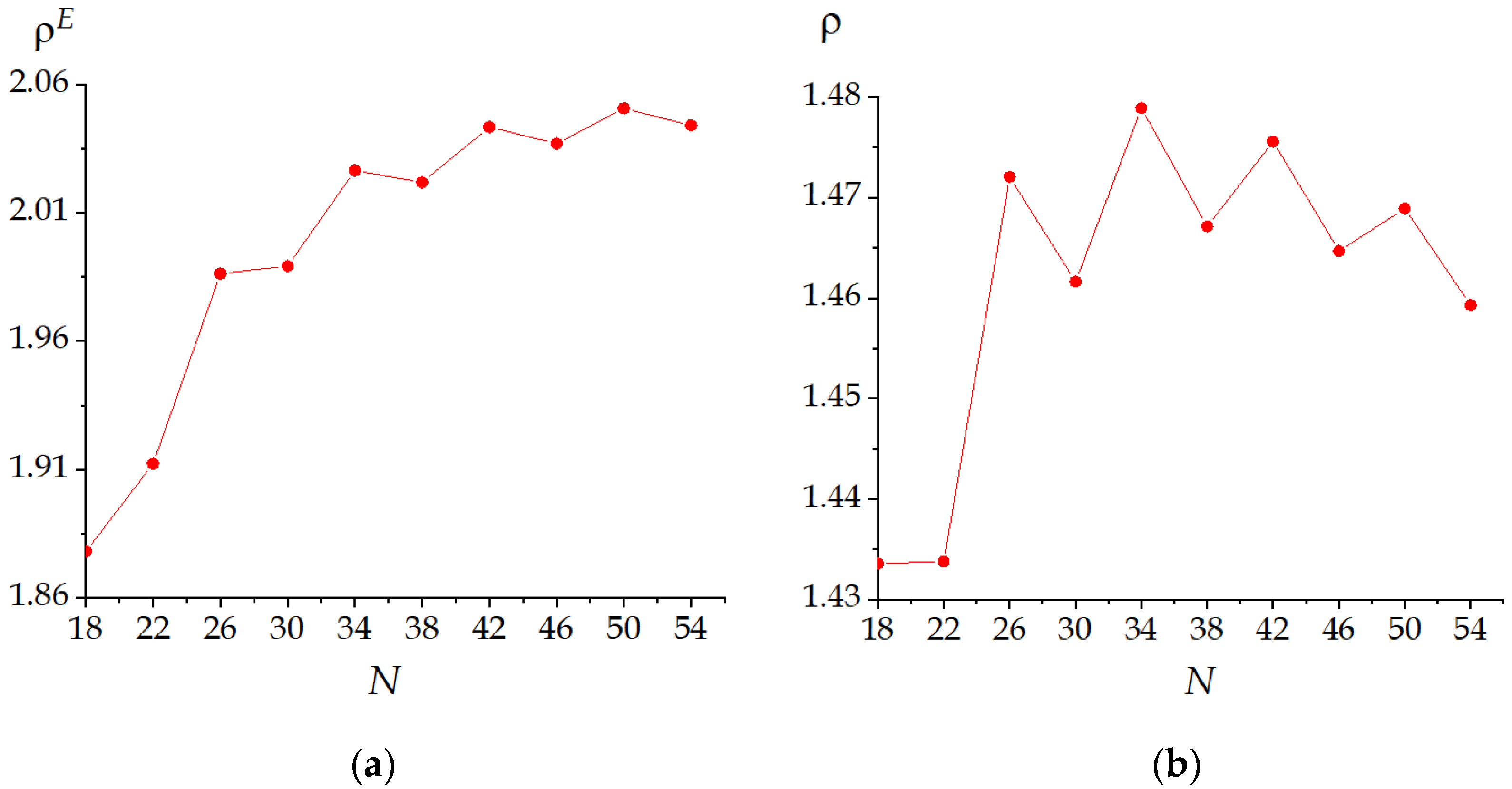
3.3.1. Topological Stability in Terms of Molecular Compactness
3.3.2. Topological Stability of Helicenes in Terms of Molecular Roundness
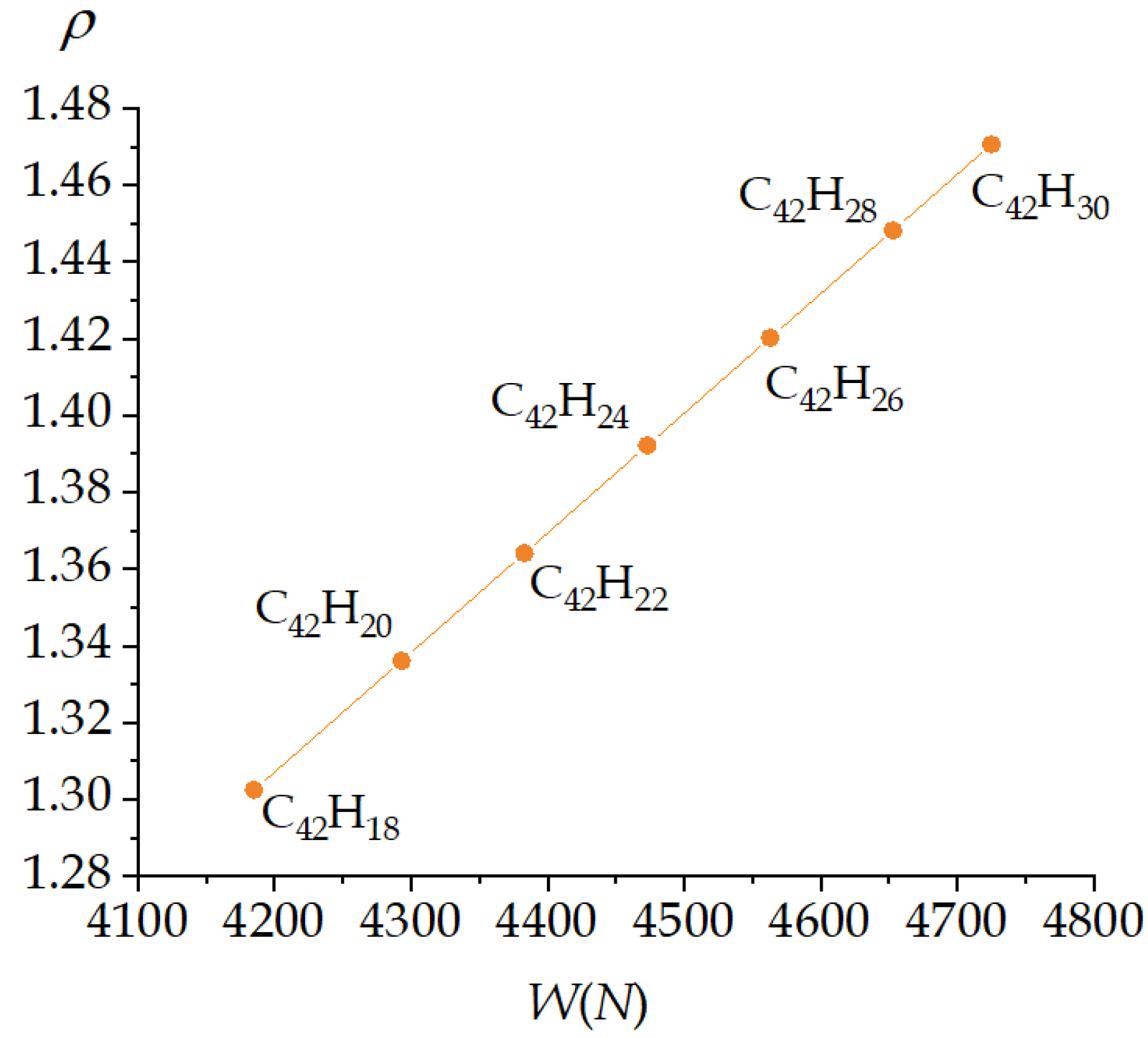
3.4. Toward the Use of Strucutral Descriptors for Chemical Reaction Series of PAHs
4. Discussion
4.1. Relevance of Parity Effects to Molecular Properties
4.2. Information Entropy and Reaction Path
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Marr, L.C.; Kirchstetter, T.W.; Harley, R.A.; Miguel, A.H.; Hering, S.V.; Hammond, S.K. Characterization of polycyclic aromatic hydrocarbons in motor vehicle fuels and exhaust emissions. Environ. Sci. Technol. 1999, 33, 3091–3099. [Google Scholar] [CrossRef]
- De Souza, C.V.; Correa, S.M. Polycyclic aromatic hydrocarbons in diesel emission, diesel fuel and lubricant oil. Fuel 2016, 185, 925–931. [Google Scholar] [CrossRef]
- Dolomatov, M.Y.; Burangulov, D.Z.; Dolomatova, M.M.; Osipenko, D.F.; Zaporin, V.P.; Tukhbatullina, A.A.; Akhmetov, A.F.; Sabirov, D.S. Low-sulphur vacuum gasoil of western siberia oil: The impact of its structural and chemical features on the properties of the produced needle coke. C—J. Carbon Res. 2022, 8, 19. [Google Scholar] [CrossRef]
- Achtena, C.; Hofmann, T. Native polycyclic aromatic hydrocarbons (PAH) in coals—A hardly recognized source of environmental contamination. Sci. Total Environ. 2009, 407, 2461–2473. [Google Scholar] [CrossRef]
- Wang, R.; Liu, G.; Zhang, J.; Chou, C.-L.; Liu, J. Abundances of polycyclic aromatic hydrocarbons (PAHs) in 14 Chinese and American coals and their relation to coal rank and weathering. Energy Fuels 2010, 24, 6061–6066. [Google Scholar] [CrossRef]
- Cataldo, F.; García-Hernández, D.A.; Manchado, A. Far- and mid-infrared spectroscopy of complex organic matter of astrochemical interest: Coal, heavy petroleum fractions and asphaltenes. MNRAS Mon. Not. R. Astron. Soc. 2013, 429, 3025–3039. [Google Scholar] [CrossRef]
- Mastral, A.M.; Callen, M.S. A review on polycyclic aromatic hydrocarbon (PAH) emissions from energy generation. Environ. Sci. Technol. 2000, 34, 3051–3057. [Google Scholar] [CrossRef]
- Du, W.; Wang, J.; Zhuo, S.; Zhong, Q.; Wang, W.; Chen, Y.; Wang, Z.; Mao, K.; Shen, G.; Tao, S. Emissions of particulate PAHs from solid fuel combustion in indoor cookstoves. Sci. Total Environ. 2021, 771, 145411. [Google Scholar] [CrossRef]
- Snow, T.; Le Page, V.; Keheyan, Y.; Bierbaum, V.M. The interstellar chemistry of PAH cations. Nature 1998, 391, 259–260. [Google Scholar] [CrossRef]
- Ehrenfreund, P.; Ruiterkamp, R.; Peeters, Z.; Foing, B.; Salama, F.; Martins, Z. The ORGANICS experiment on BIOPAN V: UV and space exposure of aromatic compounds. Planet. Space Sci. 2007, 55, 383–400. [Google Scholar] [CrossRef]
- Bauschlicher, C.W., Jr.; Peeters, E.; Allamandola, L.J. The Infrared Spectra of Very Large, Compact, Highly Symmetric, Polycyclic Aromatic Hydrocarbons (PAHs). ApJ Astrophys. J. Lett. 2008, 678, 316–327. [Google Scholar] [CrossRef]
- Ai-Haimoud, Y.; Perez, L.M.; Maddalena, R.J.; Roshi, D.A. Search for polycyclic aromatic hydrocarbons in the Perseus molecular cloud with the Green Bank Telescope. MNRAS Mon. Not. R. Astron. Soc. 2015, 447, 315–324. [Google Scholar] [CrossRef]
- Slayden, S.W.; Liebman, J.F. The energetics of aromatic hydrocarbons: An experimental thermochemical perspective. Chem. Rev. 2001, 101, 1541–1566. [Google Scholar] [CrossRef] [PubMed]
- Anthony, J.E. The larger acenes: Versatile organic semiconductors. Angew. Chem. Int. Ed. 2008, 47, 452–483. [Google Scholar] [CrossRef] [PubMed]
- Malloci, G.; Cappellini, G.; Mulas, G.; Mattoni, A. Electronic and optical properties of families of polycyclic aromatic hydrocarbons: A systematic (time-dependent) density functional theory study. Chem. Phys. 2011, 384, 19–27. [Google Scholar] [CrossRef]
- Portella, G.; Poater, J.; Bofill, J.M.; Alemany, P.; Sola, M. Local aromaticity of [n]acenes, [n]phenacenes, and [n]helicenes (n = 1−9). J. Org. Chem. 2005, 70, 2509–2521. [Google Scholar] [CrossRef]
- Zhang, X.; Xin, J.; Ding, F. The edges of graphene. Nanoscale 2013, 5, 2556–2569. [Google Scholar] [CrossRef]
- Pauncz, R.; Berencz, F. The diamagnetic anisotropy of four-ring condensed aromatic hydrocarbons. Acta Phys. Hung. 1952, 2, 183–193. [Google Scholar] [CrossRef]
- Jones, L.; Lin, L. An in silico study on the isomers of pentacene: The case for air-stable and alternative C22H14 acenes for organic electronics. J. Phys. Chem. A 2017, 121, 2804–2813. [Google Scholar] [CrossRef]
- Sabirov, D.S. A correlation between the mean polarizability of the “kinked” polycyclic aromatic hydrocarbons and the number of H…H bond critical points predicted by Atoms-in-Molecules theory. Comput. Theor. Chem. 2014, 1030, 81–86. [Google Scholar] [CrossRef]
- Sabirov, D.S.; Garipova, R.R.; Cataldo, F. Polarizability of isomeric and related interstellar compounds in the aspect of their abundance. Mol. Astrophys. 2018, 12, 10–19. [Google Scholar] [CrossRef]
- Sabirov, D.S.; Tukhbatullina, A.A.; Shepelevich, I.S. Polarzability in astrochemical studies of complex carbon-based compounds. ACS Earth Space Chem. 2022, 6, 1–17. [Google Scholar] [CrossRef]
- Pino-Rios, R.; Báez-Grez, R.; Solà, M. Acenes and phenacenes in their lowest-lying triplet states. Does kinked remain more stable than straight? Phys. Chem. Chem. Phys. 2021, 23, 13574–13582. [Google Scholar] [CrossRef] [PubMed]
- Poater, J.; Visser, R.; Sola, M.; Bickelhaupt, F.M. Polycyclic benzenoids: Why kinked is more stable than straight. J. Org. Chem. 2007, 72, 1134–1142. [Google Scholar] [CrossRef] [PubMed]
- Pankratyev, E.Y.; Tukhbatullina, A.A.; Sabirov, D.S. Dipole polarizability, structure, and stability of [2+2]-linked fullerene nanostructures (C60) n (n ≤ 7). Physica E 2017, 86, 237–242. [Google Scholar] [CrossRef]
- Sabirov, D.S.; Terentyev, A.O.; Bulgakov, R.G. Polarizability of fullerene [2+2]-dimers: A DFT study. Phys. Chem. Chem. Phys. 2014, 16, 14594–14600. [Google Scholar] [CrossRef]
- Tukhbatullina, A.A.; Shepelevich, I.S.; Sabirov, D.S. Exaltation of polarizability as a common property of fullerene dimers with diverse intercage bridges. Fuller. Nanotub. Carbon Nanostruct. 2018, 26, 661–666. [Google Scholar] [CrossRef]
- Sabirov, D.S.; Garipova, R.R. The increase in the fullerene cage volume upon its chemical functionalization. Fuller. Nanotub. Carbon Nanostruct. 2019, 27, 702–709. [Google Scholar] [CrossRef]
- Zakirova, A.D.; Sabirov, D.S. Volume of the fullerene cages of endofullerenes and hydrogenated endofullerenes with encapsulated atoms of noble gases and nonadditivity of their polarizability. Russ. J. Phys. Chem. 2020, 94, 963–971. [Google Scholar] [CrossRef]
- Sabirov, D.S.; Ori, O.; László, I. Isomers of the C84 fullerene: A theoretical consideration within energetic, structural, and topological approaches. Fuller. Nanotub. Carbon Nanostruct. 2018, 26, 100–110. [Google Scholar] [CrossRef]
- Sabirov, D.S.; Ori, O.; Tukhbatullina, A.A.; Shepelevich, I.S. Covalently bonded fullerene nano-aggregates (C60)n: Digitalizing their energy-topology-symmetry. Symmetry 2021, 13, 1899. [Google Scholar] [CrossRef]
- Sabirov, D.S.; Ori, O. Skeletal rearrangements of the C240 Fullerene: Efficient topological descriptors for monitoring Stone–Wales transformations. Mathematics 2020, 8, 968. [Google Scholar] [CrossRef]
- Sabirov, D.S.; Ōsawa, E. Information entropy of fullerenes. J. Chem. Inf. Model. 2015, 55, 1574–1576. [Google Scholar] [CrossRef] [PubMed]
- Sabirov, D.S.; Terentyev, A.O.; Sokolov, V.I. Activation energies and information entropies of helium penetration through fullerene walls. Insights into the formation of endofullerenes nX@C60/70 (n = 1 and 2) from the information entropy approach. RSC Adv. 2016, 6, 72230–72237. [Google Scholar] [CrossRef]
- Sabirov, D.S. Information entropy of interstellar and circumstellar carbon-containing molecules: Molecular size against structural complexity. Comput. Theor. Chem. 2016, 1097, 83–91. [Google Scholar] [CrossRef]
- Sabirov, D.S.; Garipova, R.R.; Kinzyabaeva, Z.S. Fullerene–1,4-dioxane adducts: A DFT study of the structural features and molecular properties. Fuller. Nanotub. Carbon Nanostruct. 2020, 28, 154–159. [Google Scholar] [CrossRef]
- Sabirov, D.; Koledina, K. Classification of isentropic molecules in terms of Shannon entropy. EPJ Web Conf. 2020, 244, 01016. [Google Scholar] [CrossRef]
- Bertz, S.H. Complexity of synthetic reactions. The use of complexity indices to evaluate reactions, transforms and disconnections. New J. Chem. 2003, 27, 860–869. [Google Scholar] [CrossRef]
- Böttcher, T. An additive definition of molecular complexity. J. Chem. Inf. Model. 2016, 56, 462–470. [Google Scholar] [CrossRef]
- Bonchev, D. Kolmogorov’s information, Shannon’s entropy, and topological complexity of molecules. Bulgar. Chem. Commun. 1995, 28, 567–582. Available online: https://www.researchgate.net/publication/255992446 (accessed on 21 August 2022).
- Barigye, S.J.; Marrero-Ponce, Y.; Pérez-Giménez, F.; Bonchev, D. Trends in information theory-based chemical structure codification. Mol. Divers. 2014, 18, 673–686. [Google Scholar] [CrossRef]
- Basak, S.C.; Gute, B.D.; Grunwald, G.D. Use of topostructural, topochemical, and geometric parameters in the prediction of vapor pressure: A hierarchical QSAR approach. J. Chem. Inf. Comput. Sci. 1997, 37, 651–655. [Google Scholar] [CrossRef]
- Sabirov, D. Information entropy changes in chemical reactions. Comput. Theor. Chem. 2018, 1123, 169–179. [Google Scholar] [CrossRef]
- Sabirov, D.S. Information entropy of mixing molecules and its application to molecular ensembles and chemical reactions. Comput. Theor. Chem. 2020, 1187, 112933. [Google Scholar] [CrossRef]
- Wiener, H. Structural determination of paraffin boiling points. J. Am. Chem. Soc. 1947, 69, 17–20. [Google Scholar] [CrossRef] [PubMed]
- Smolenskii, E.A. The Wiener distance matrix for acyclic compounds and polymers. J. Chem. Inf. Comput. Sci. 2004, 44, 522–528. [Google Scholar] [CrossRef]
- Nikolić, S.; Trinajstić, N.; Mihalić, Z. The Wiener index: Development and applications. Croat. Chem. Acta 1995, 68, 105–129. [Google Scholar]
- Vukicevic, D.; Cataldo, F.; Ori, O.; Graovac, A. Topological efficiency of C66 fullerene. Chem. Phys. Lett. 2011, 501, 442–445. [Google Scholar] [CrossRef]
- Iranmanesh, A.; Ashrafi, A.R.; Graovac, A.; Cataldo, F.; Ori, O. Wiener index role in topological modeling of hexagonal systems-from fullerenes to graphene. In Distance in Molecular Graphs-Applications; Gutman, I., Furtula, B., Eds.; University Kragujevac: Kragujevac, Serbia, 2012; pp. 135–155. [Google Scholar]
- Ori, O.; Cataldo, F.; Graovac, A. Topological ranking of C28 fullerenes reactivity. Fuller. Nanotub. Carbon Nanostruct. 2009, 17, 308–323. [Google Scholar] [CrossRef]
- Koorepazan-Moftakhar, F.; Ashrafi, A.R.; Ori, O.; Putz, M.V. Sphericality of some classes of fullerenes measured by topology. In Fullerenes: Chemistry, Natural Sources and Technological Applications; Ellis, S.B., Ed.; Nova Science Publishers, Inc.: New York, NY, USA, 2014; pp. 285–304. [Google Scholar]
- Putz, M.V.; Ori, O.; Cataldo, F.; Putz, A.M. Parabolic reactivity “coloring” molecular topology: Application to carcinogenic PAHs. Curr. Org. Chem. 2013, 17, 2816–2830. [Google Scholar] [CrossRef]
- Cataldo, F.; Ori, O.; Iglesias-Groth, S. Topological lattice descriptors of graphene sheets with fullerene-like nanostructures. Mol. Simul. 2010, 36, 341–353. [Google Scholar] [CrossRef]
- Bonchev, D.; Mekenyan, O. A topological approach to the calculation of the π–electron energy and energy gap of infinite conjugated polymers. Z. Nat. A 1980, 35, 739–747. [Google Scholar] [CrossRef]
- Ori, O.; Cataldo, F.; Vukicevic, D.; Graovac, A. Wiener way to dimensionality. Iran. J. Math. Chem. 2010, 1, 5–15. [Google Scholar] [CrossRef]
- Ori, O.; Cataldo, F.; Putz, M.V.; Kaatz, F.; Bultheel, A. Cooperative topological accumulation of vacancies in honeycomb lattices. Fuller. Nanotub. Carbon Nanostruct. 2016, 24, 353–362. [Google Scholar] [CrossRef]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized gradient approximation made simple. Phys. Rev. Lett. 1996, 77, 3865–3868. [Google Scholar] [CrossRef]
- Laikov, D.N.; Ustynyuk, Y.A. PRIRODA-04: A quantum-chemical program suite. New possibilities in the study of molecular systems with the application of parallel computing. Russ. Chem. Bull. 2005, 54, 820–826. [Google Scholar] [CrossRef]
- Cárdenas, C.; Ayers, P.; De Proft, F.; Tozer, D.J.; Geerlings, P. Should negative electron affinities be used for evaluating the chemical hardness? Phys. Chem. Chem. Phys. 2011, 13, 2285–2293. [Google Scholar] [CrossRef]
- Wannere, C.S.; Sattelmeyer, K.W.; Schaefer, H.F., III; von Ragué Schleyer, P. Aromaticity: The alternating C–C bond length structures of [14]-, [18]-, and [22]annulene. Angew. Chem. Int. Ed. 2004, 43, 4200–4206. [Google Scholar] [CrossRef]
- Szczepanik, D.W.; Solà, M.; Andrzejak, M.; Pawełek, B.; Dominikowska, J.; Kukułka, M.; Dyduch, K.; Krygowski, T.M.; Szatylowicz, H. The role of the long-range exchange corrections in the description of electron delocalization in aromatic species. J. Comput. Chem. 2017, 38, 1640–1654. [Google Scholar] [CrossRef]
- Torrent-Sucarrat, M.; Navarro, S.; Cossío, F.P.; Anglada, J.M.; Luis, J.M. Relevance of the DFT method to study expanded porphyrins with different topologies. J. Comput. Chem. 2017, 38, 2819–2828. [Google Scholar] [CrossRef]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Fox, D.J. Gaussian 09; Gaussian Inc.: Wallingford, CT, USA, 2009. [Google Scholar]
- Tampieri, F.; Barbon, A.; Tommasini, M. Analysis of the Jahn–Teller effect in coronene and corannulene ions and its effect in EPR spectroscopy. Chem. Phys. Impact 2021, 2, 100012. [Google Scholar] [CrossRef]
- Bultheel, A.; Ori, O. Topological modeling of 1-pentagon carbon nanocones–topological efficiency and magic sizes. Fuller. Nanotub. Carbon Nanostruct. 2018, 26, 291–302. [Google Scholar] [CrossRef]
- Rempala, P.; Kroulik, J.; King, B.T. A Slippery Slope: Mechanistic analysis of the intramolecular Scholl reaction of hexaphenylbenzene. J. Am. Chem. Soc. 2004, 126, 15002–15003. [Google Scholar] [CrossRef] [PubMed]
- Lukmanov, T.I.; Shepelevich, I.S.; Sabirov, D.S. Polarizability of polycyclic aromatic hydrocarbon compounds from the intermediate stages of the oxidative condensation of hexaphenylbenzene into hexa-peri-hexabenzocoronene. Vestn. Bashkir. Univ. 2022, 27, 98–101. [Google Scholar] [CrossRef]
- Sabirov, D.; Tukhbatullina, A.; Shepelevich, I. Information entropy of regular dendrimer aggregates and irregular intermediate structures. Liquids 2021, 1, 2. [Google Scholar] [CrossRef]
- Balasubramanian, K. Symmetry and combinatorial concepts for cyclopolyarenes, nanotubes and 2D-sheets: Enumerations, isomers, structures spectra & properties. Symmetry 2021, 14, 34. [Google Scholar] [CrossRef]
- Sabirov, D.S.; Shepelevich, I.S. Information entropy in chemistry: An overview. Entropy 2021, 23, 1240. [Google Scholar] [CrossRef]

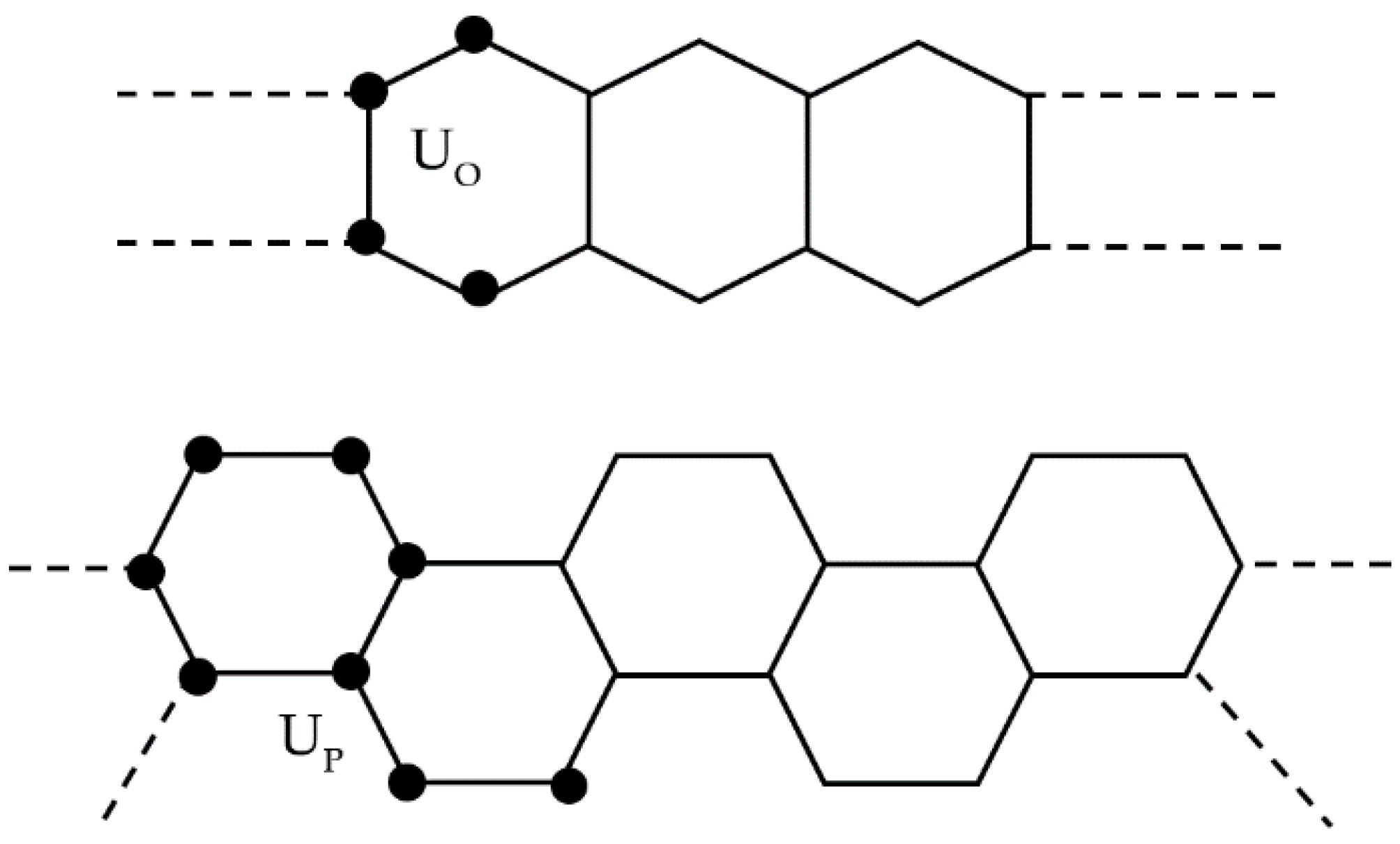
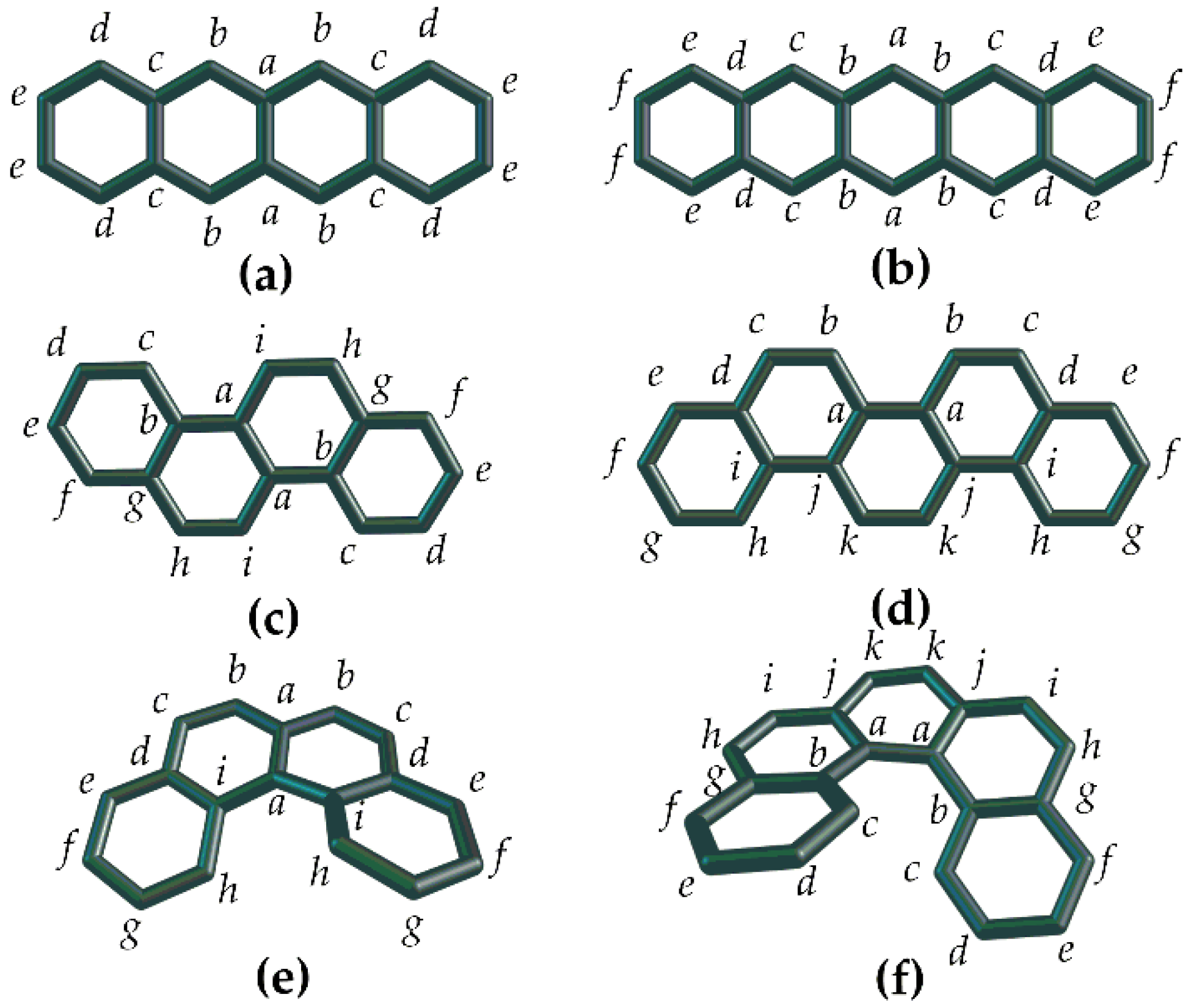
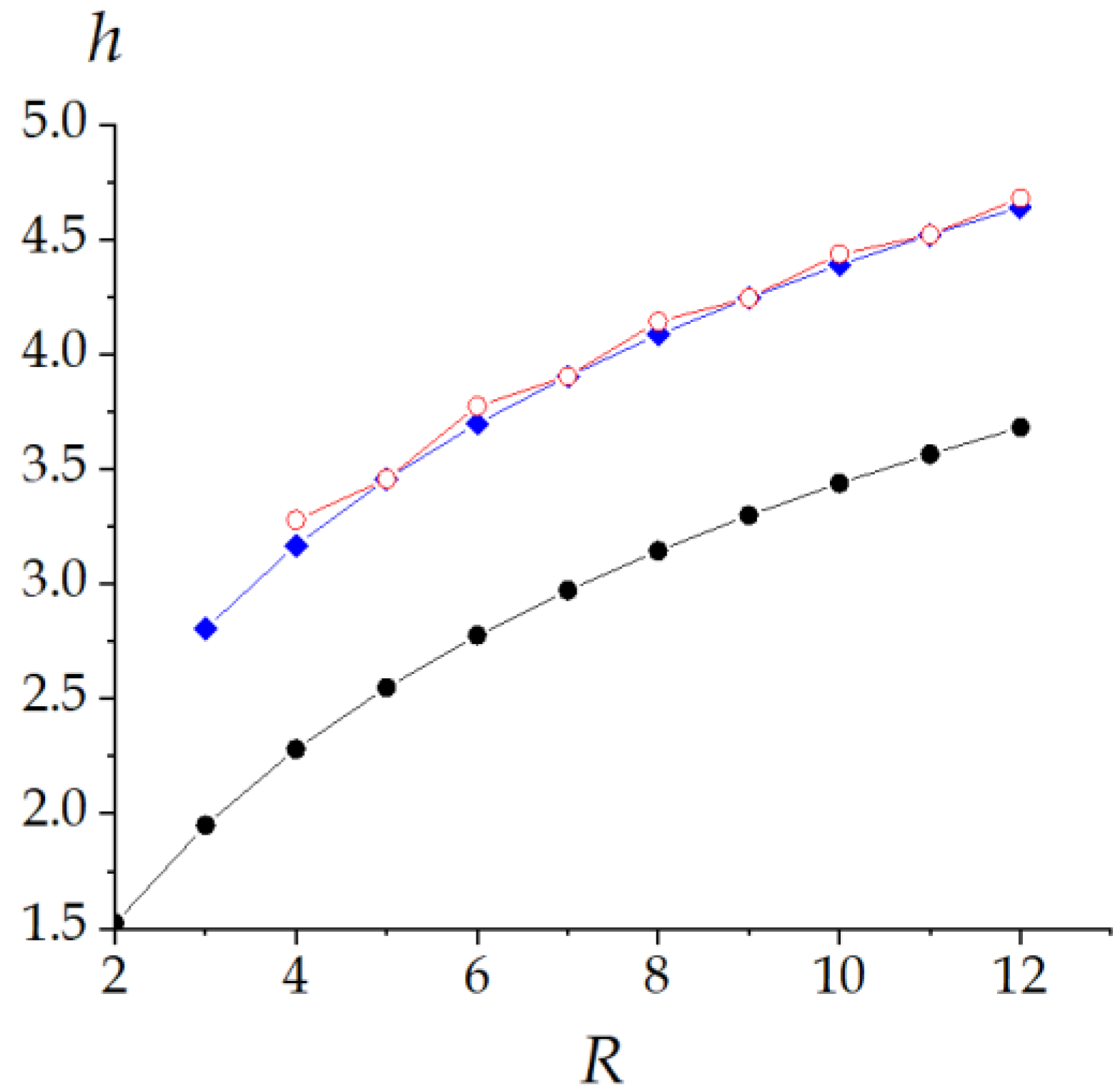
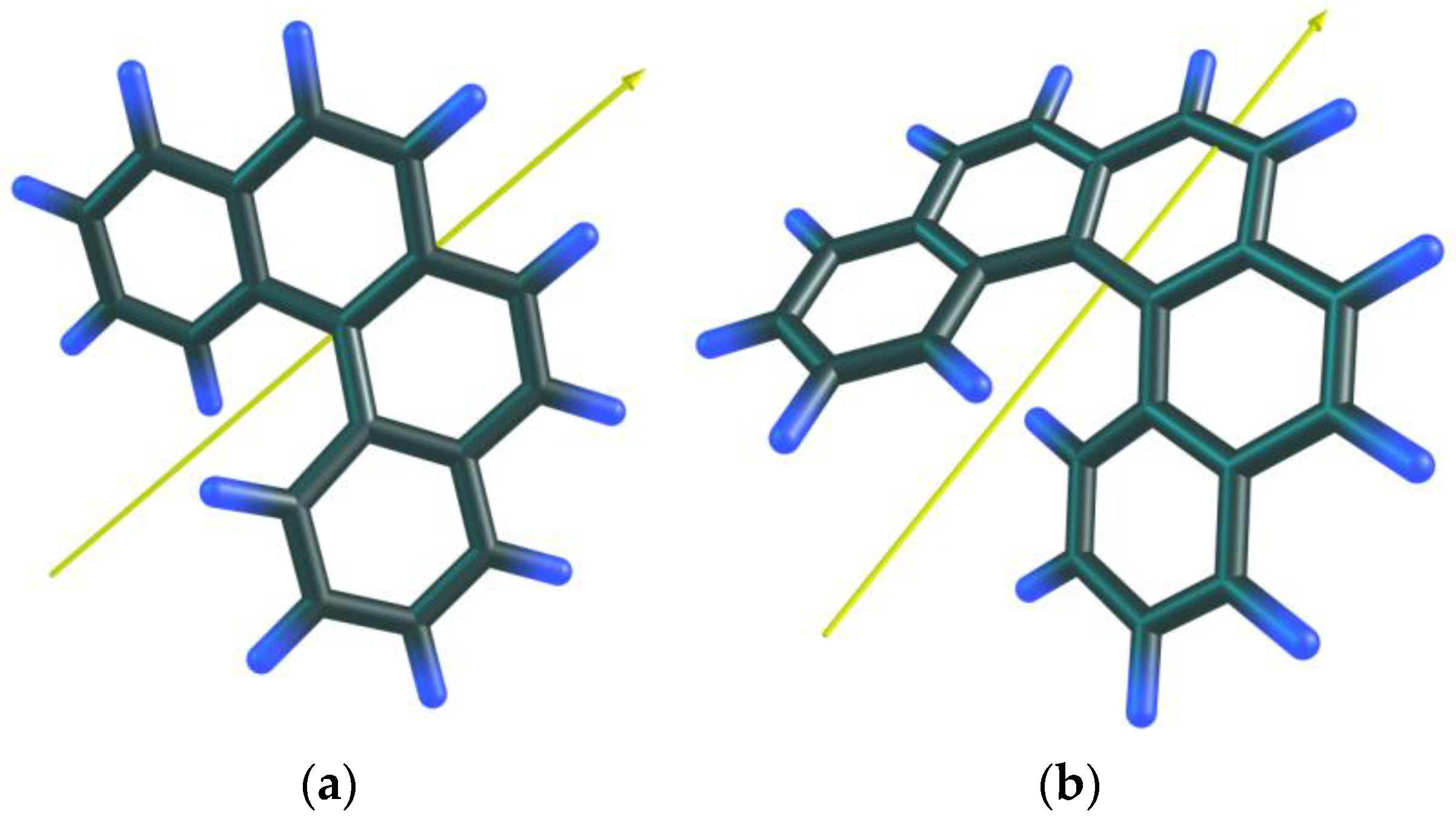

| Parameter | Oligoacenes | Phenacenes and Odd Helicenes | Even Helicenes |
|---|---|---|---|
| Partition | |||
| Expression h = f(N) | |||
| Expression h = f(R) |
| Index | Oligoacenes | Phenacenes | Helicenes |
|---|---|---|---|
| W(N) | |||
| , k = −28 for odd members; k = −12 for even members | |||
| for large N | for large N | for large N | |
| for large N | for large N | for large N |
| Molecule | Parition of the Carbon Skeleton | Number of Benzene Rings (R) | Information Entropy (h) |
|---|---|---|---|
| C42H30 | 3 × 6 + 2 × 12 | 7 | 2.236 |
| C42H28 | 13 × 2 + 4 × 4 | 8 | 4.011 |
| C42H26 | 6 × 1 + 14 × 2+ 2 × 4 | 9 | 4.345 |
| C42H24 | 2 × 4 + 17 × 2 | 10 | 4.202 |
| C42H22 | 6 × 1 + 18 × 2 | 11 | 4.535 |
| C42H20 | 21 × 2 | 12 | 4.392 |
| C42H18 | 3 × 6 + 2 × 12 | 13 | 2.236 |
| Molecule | Wiener Index (W) | Topological Efficiency (ρ) | Topological Roundness (ρE) |
|---|---|---|---|
| C42H30 | 4725 | 1.4706 | 1.9020 |
| C42H28 | 4653 | 1.4482 | 1.9020 |
| C42H26 | 4563 | 1.4202 | 1.9020 |
| C42H24 | 4473 | 1.3922 | 1.9020 |
| C42H22 | 4383 | 1.3641 | 1.9020 |
| C42H20 | 4293 | 1.3361 | 1.7451 |
| C42H18 | 4185 | 1.3025 | 1.5882 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sabirov, D.S.; Ori, O.; Tukhbatullina, A.A.; Shepelevich, I.S. Structural Descriptors of Benzenoid Hydrocarbons: A Mismatch between the Estimates and Parity Effects in Helicenes. C 2022, 8, 42. https://doi.org/10.3390/c8030042
Sabirov DS, Ori O, Tukhbatullina AA, Shepelevich IS. Structural Descriptors of Benzenoid Hydrocarbons: A Mismatch between the Estimates and Parity Effects in Helicenes. C. 2022; 8(3):42. https://doi.org/10.3390/c8030042
Chicago/Turabian StyleSabirov, Denis Sh., Ottorino Ori, Alina A. Tukhbatullina, and Igor S. Shepelevich. 2022. "Structural Descriptors of Benzenoid Hydrocarbons: A Mismatch between the Estimates and Parity Effects in Helicenes" C 8, no. 3: 42. https://doi.org/10.3390/c8030042
APA StyleSabirov, D. S., Ori, O., Tukhbatullina, A. A., & Shepelevich, I. S. (2022). Structural Descriptors of Benzenoid Hydrocarbons: A Mismatch between the Estimates and Parity Effects in Helicenes. C, 8(3), 42. https://doi.org/10.3390/c8030042









